Mathematical and Physical Characteristics of the Phase Spectrum of Earthquake Ground Motions
Abstract
1. Introduction
2. Mathematical Characteristics of the Phase Angles and Phase Differences
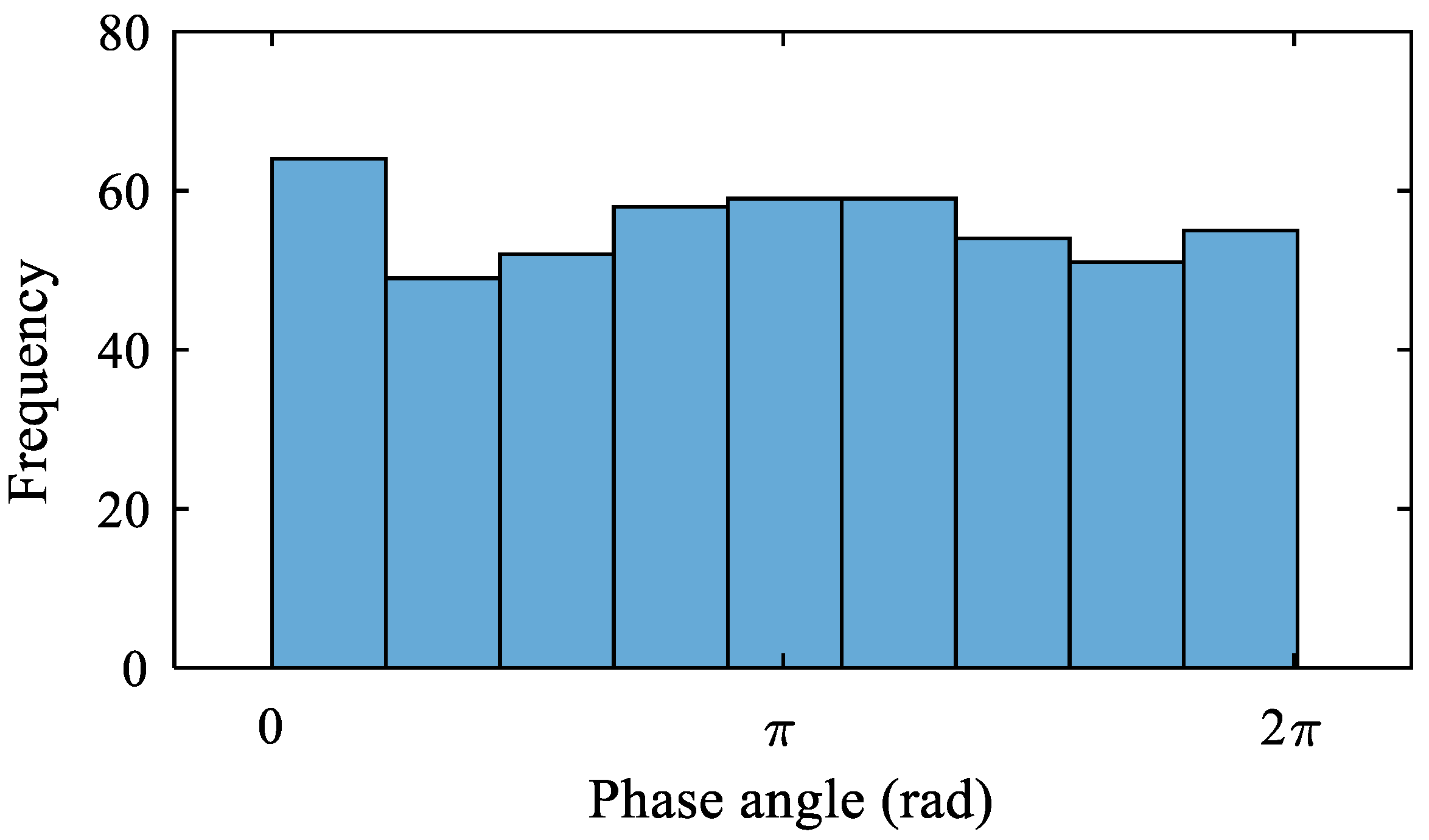
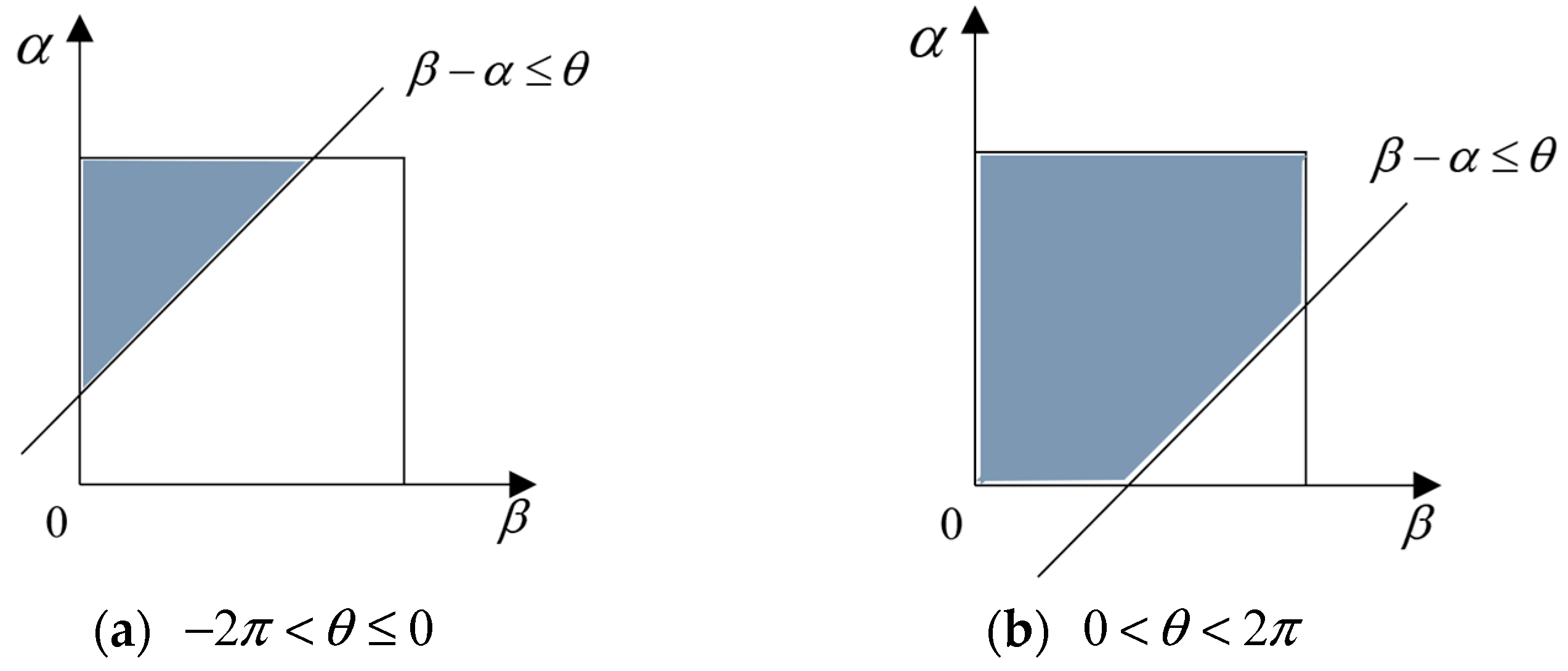
2.1. Probability Distribution of the Phase Angles
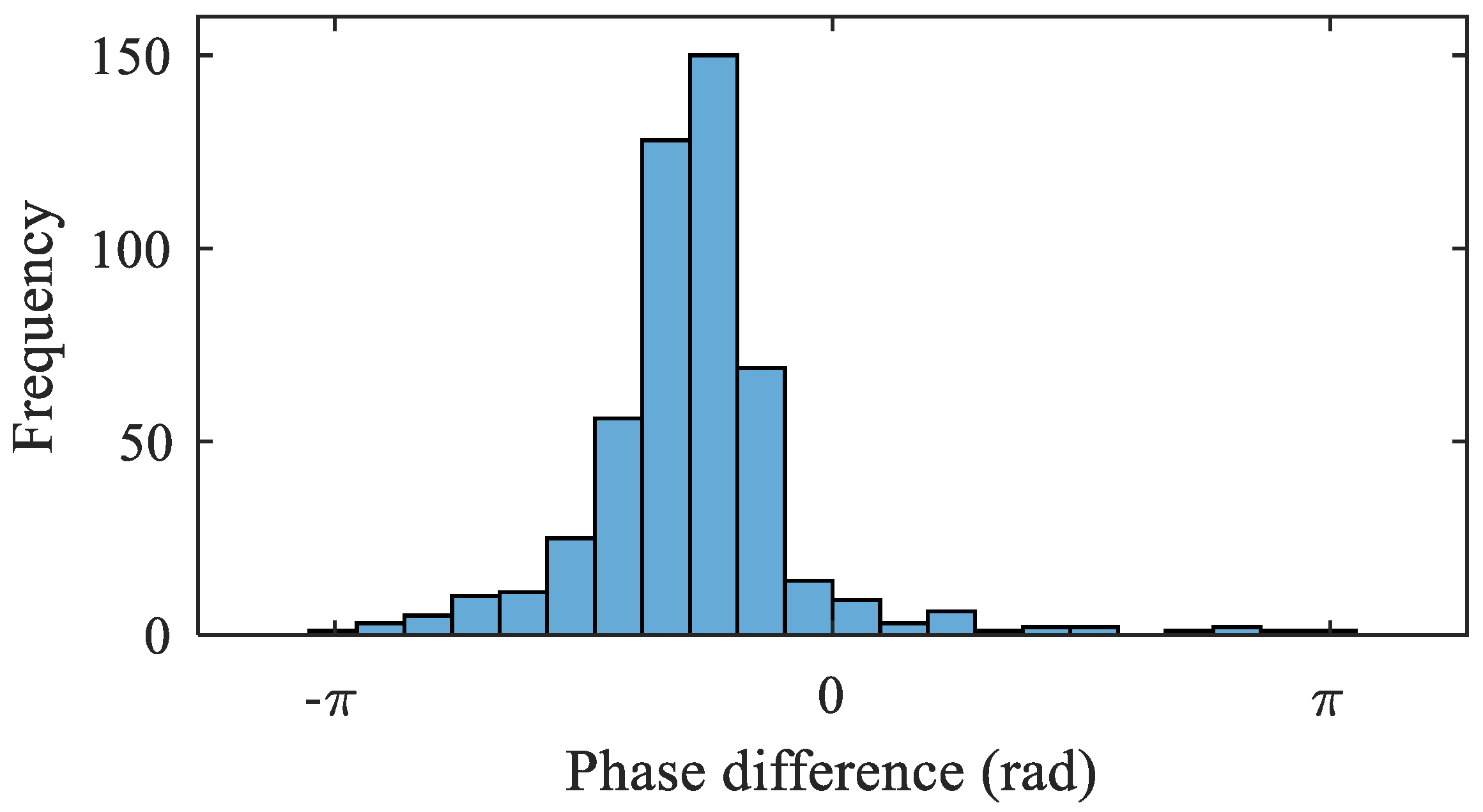
2.2. Probability Distribution of the Phase Differences
3. Physical Characteristics of the Phase Spectrum
3.1. Circular Frequency-Dependent Phase Derivative
- (1)
- The amplitude is a constant in , giving:
- (2)
- The phase angle is approximated using Taylor’s expansion in the neighborhood of , in which only the first two terms are maintained, giving:
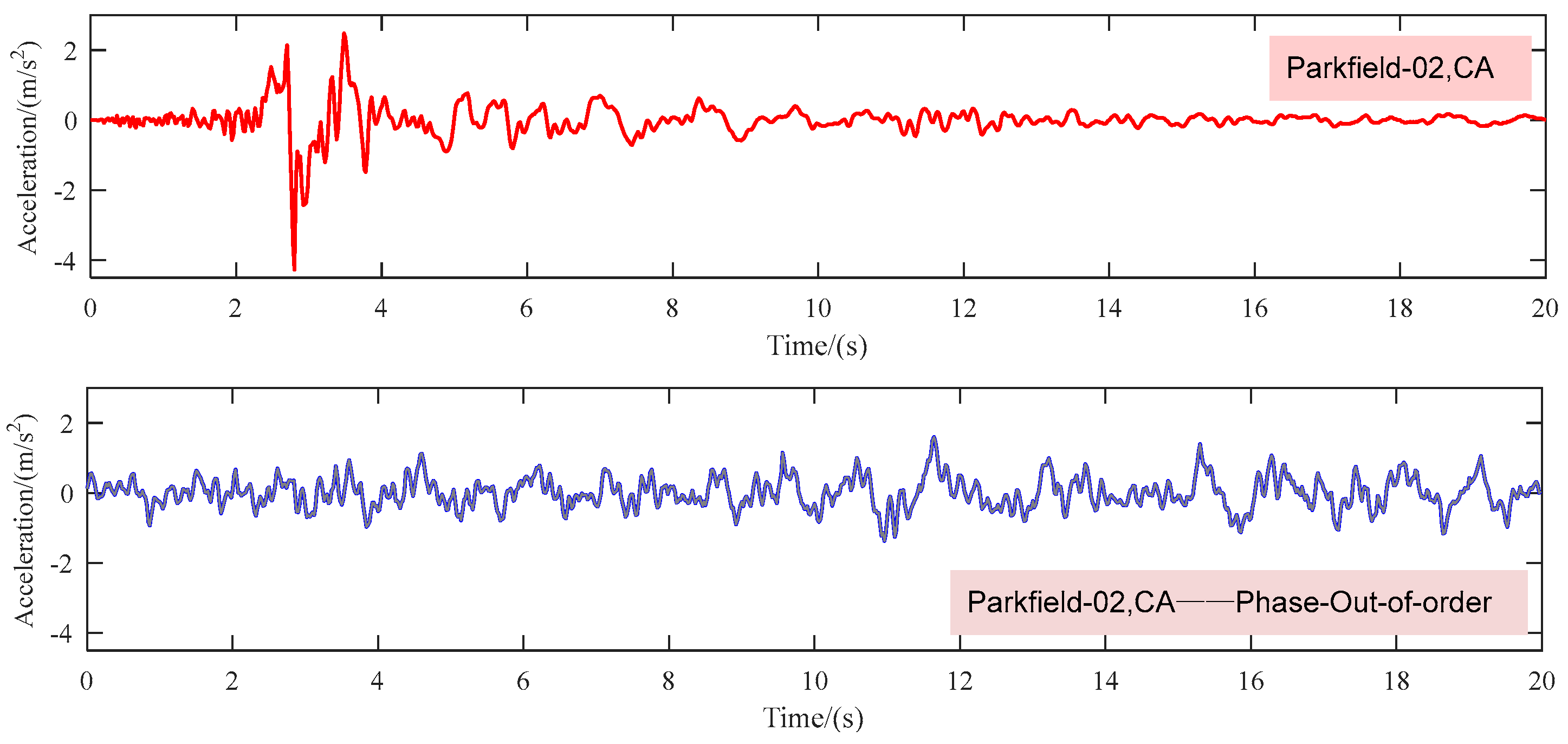
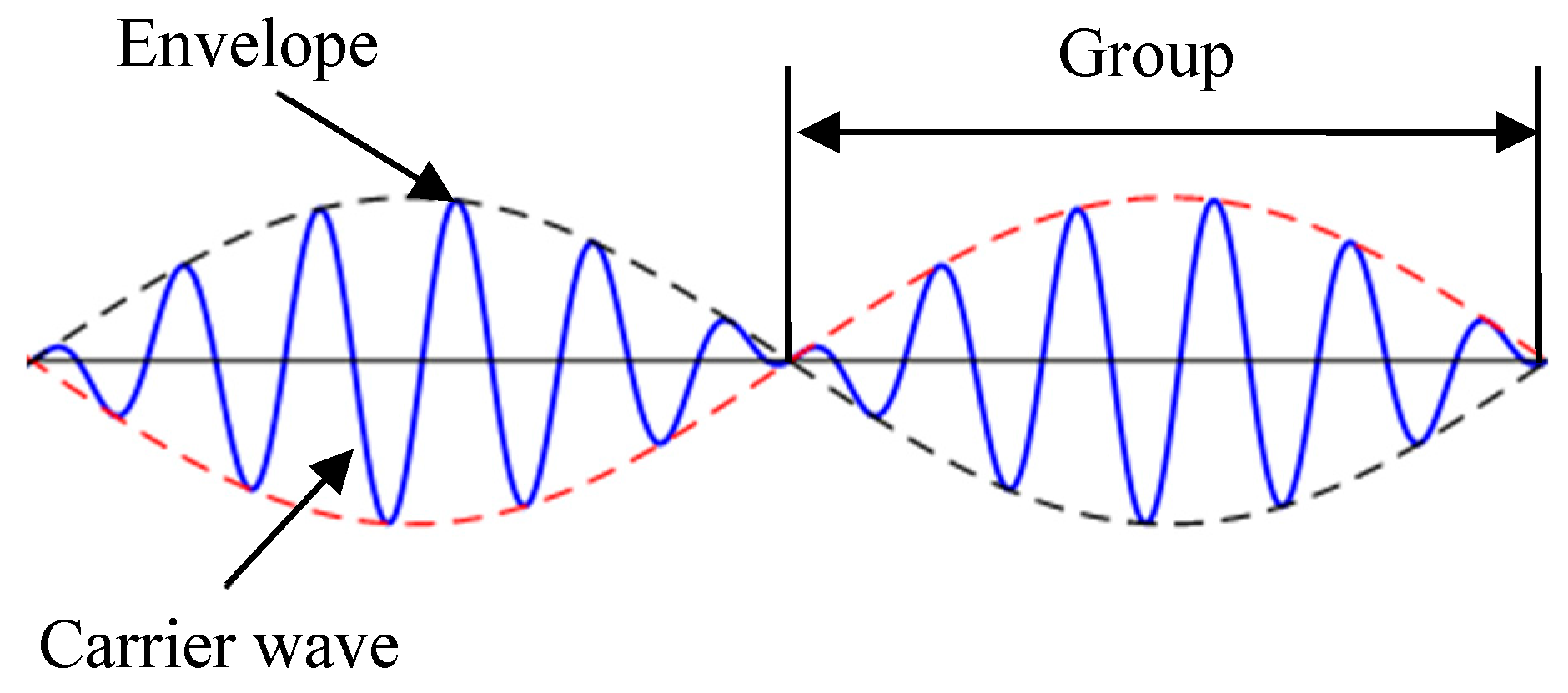
3.2. Relation of the Envelope Delay and Fourier Amplitudes
4. Influence of the Source, Propagation Path, and Site on the Phase Spectrum
4.1. Data
4.2. Influence of the Source
4.3. Influence of the Propagation Path
4.4. Influence of the Site
5. Conclusions and Discussion
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sertel, E. Identification of Earthquake Induced Damage Areas Using Fourier Transform and SPOT HRVIR Pan Images. Sensors 2009, 9, 1471–1484. [Google Scholar] [CrossRef] [PubMed]
- Madariaga, R.; Ruiz, S.; Rivera, E.; Leyton, F.; Baez, J.C. Near-field spectra of large earthquake. Pure Appl. Geophys. 2019, 176, 983–1001. [Google Scholar] [CrossRef]
- Cho, I.H. Sharpen data-driven prediction rules of individual large earthquakes with aid of Fourier and Gauss. Sci. Rep. 2023, 13, 16009. [Google Scholar] [CrossRef] [PubMed]
- Boore, D.M. Simulation of ground motion using the stochastic method. Pure Appl. Geophys. 2003, 160, 635–676. [Google Scholar] [CrossRef]
- Rezaeian, S.; Der Kiureghian, A. A stochastic ground motion model with separable temporal and spectral nonstationarities. Earthq. Eng. Struct. Dyn. 2008, 37, 1565–1584. [Google Scholar] [CrossRef]
- Wang, D.; Li, J. Physical random function model of ground motions for engineering purposes. Sci. China Technol. Sci. 2011, 54, 175–182. [Google Scholar] [CrossRef]
- Kanai, K. Semi-empirical formula for the earthquake characteristics of the ground. Bull. Earthq. Res. Inst. Univ. Tokyo Jpn. 1957, 35, 309–325. [Google Scholar]
- Tajimi, H. A statistical method of determining the maximum response of a building structure during an earthquake. In Proceedings of the 2th World Conference on Earthquake Engineering, Tokyo, Japan, 11–18 July 1960. [Google Scholar]
- Clough, R.W.; Penzien, J. Dynamics of Structures; McGraw-Hill Book Co.: New York, NY, USA, 1975. [Google Scholar]
- Oppenheim, A.V.; Lim, J.S. The importance of phase in signals. Proc. IEEE 1981, 69, 529–541. [Google Scholar] [CrossRef]
- Yegnanarayana, B.; Saikia, D.; Krishnan, T. Significance of group delay functions in signal reconstruction from spectral magnitude or phase. IEEE Trans. Acoust. Speech Signal Process. 1984, 32, 610–623. [Google Scholar] [CrossRef][Green Version]
- Shi, G.; Shanechi, M.M.; Aarabi, P. On the importance of phase in human speech recognition. IEEE Trans. Audio Speech Lang. Process. 2006, 14, 1867–1874. [Google Scholar]
- Skarbnik, N.; Zeevi, Y.Y.; Sagiv, C. The Importance of Phase in Image Processing; Faculty of Electrical Engineering, Technion-Israel Institute of Technology: Haifa, Israel, 2009. [Google Scholar]
- Kakarala, R. A signal processing approach to Fourier analysis of ranking data: The importance of phase. IEEE Trans. Signal Process. 2011, 59, 1518–1527. [Google Scholar] [CrossRef]
- Bakulin, A.; Silvestrov, I.; Neklyudov, D. Importance of phase guides from beamformed data for processing multi-channel data in highly scattering media. J. Acoust. Soc. Am. 2020, 147, EL447–EL452. [Google Scholar] [CrossRef] [PubMed]
- Shinozuka, M. Simulation of multivariate and multidimensional random processes. J. Acoust. Soc. Am. 1971, 49, 357–368. [Google Scholar] [CrossRef]
- Shinozuka, M.; Deodatis, G. Simulation of stochastic processes by spectral representation. Appl. Mech. Rev. 1991, 44, 191–204. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, W.; Peng, Y. Random function based spectral representation of stationary and non-stationary stochastic processes. Probabilistic Eng. Mech. 2016, 45, 115–126. [Google Scholar] [CrossRef]
- Sarkar, K.; Gupta, V.K.; George, R.C. Wavelet-based generation of spatially correlated accelerograms. Soil Dyn. Earthq. Eng. 2016, 87, 116–124. [Google Scholar] [CrossRef]
- Chen, J.; Kong, F.; Peng, Y. A stochastic harmonic function representation for non-stationary stochastic processes. Mech. Syst. Signal Process. 2017, 96, 31–44. [Google Scholar] [CrossRef]
- Ohsaki, Y. On the significance of phase content in earthquake ground motions. Earthq. Eng. Struct. Dyn. 1979, 7, 427–439. [Google Scholar] [CrossRef]
- Nigam, N.C. Phase properties of a class of random processes. Earthq. Eng. Struct. Dyn. 1982, 10, 711–717. [Google Scholar] [CrossRef]
- Sawada, T. Application of phase differences to the analysis of nonstationarity of earthquake ground motion. In Proceedings of the 8th World Conference on Earthquake Engineering, Prentice Hall, New York, NY, USA, 21–28 July 1984. [Google Scholar]
- Jin, X.; Liao, Z. Relation between envelope function of strong ground motions and frequency number distribution function of phase difference spectrum. Earthq. Eng. Eng. Vib. 1990, 10, 20–26. (In Chinese) [Google Scholar]
- Thráinsson, H.; Kiremidjian, A.S. Simulation of digital earthquake accelerograms using the inverse discrete Fourier transform. Earthq. Eng. Struct. Dyn. 2002, 31, 2023–2048. [Google Scholar] [CrossRef]
- Montaldo, V.; Kiremidjian, A.S.; Thráinsson, H.; Zonno, G. Simulation of the Fourier phase spectrum for the generation of synthetic accelerograms. J. Earthq. Eng. 2003, 7, 427–445. [Google Scholar] [CrossRef]
- Nagao, K.; Kanda, J. Study of a Ground-Motion Simulation Method using a Causality Relationship. J. Earthq. Eng. 2014, 18, 891–907. [Google Scholar] [CrossRef]
- Han, X.; Wang, Z.; Peng, L.; Su, J.; Wang, L. Numerical Simulation of Seismic Waves with Peak Arrival Time and Amplitude-Frequency Correlation. KSCE J. Civ. Eng. 2019, 23, 4389–4406. [Google Scholar] [CrossRef]
- Zhu, Y.; Feng, Q. Distribution characteristic of phase difference spectrum and artificial accelerogram. Earthq. Eng. Eng. Vib. 1992, 12, 37–44. [Google Scholar]
- Zhang, C.; Sato, T.; Lu, L. A phase model of earthquake motions based on stochastic differential equation. KSCE J. Civ. Eng. 2011, 15, 161–166. [Google Scholar] [CrossRef]
- Sato, T. Fractal characteristics of phase spectrum of earthquake motion. J. Earthq. Tsunami 2013, 7, 1350010. [Google Scholar] [CrossRef]
- Baglio, M.G. Stochastic Ground Motion Method Combining a Fourier Amplitude Spectrum Model from a Response Spectrum with Application of Phase Derivatives Distribution Prediction. Ph.D. Thesis, Politecnico di Torino, Turin, Italy, 2017. [Google Scholar]
- Ding, Y.; Peng, Y.; Li, J. A stochastic semi-physical model of seismic ground motions in time domain. J. Earthq. Tsunami 2018, 12, 1850006. [Google Scholar] [CrossRef]
- Lavrentiadis, G.; Abrahamson, N. Generation of surface-slip profiles in the wavenumber domain. Bull. Seism.-Log. Soc. Am. 2019, 109, 888–907. [Google Scholar] [CrossRef]
- Han, X.; Liu, Y.; Wang, L. The normal distribution fitting method for frequency distribution characteristics of peak arrival time of earthquake. Adv. Compos. Lett. 2020, 29, 2633366X20921411. [Google Scholar] [CrossRef]
- Wang, H.; Wang, F.; Yang, H.; Feng, Y.; Bayless, J.; Abrahamson, N.A.; Jeremić, B. Time domain intrusive probabilistic earthquake risk analysis of nonlinear shear frame structure. Soil Dyn. Earthq. Eng. 2020, 136, 106201. [Google Scholar] [CrossRef]
- Boore, D.M. Phase derivatives and simulation of strong ground motions. Bull. Seismol. Soc. Am. 2003, 93, 1132–1143. [Google Scholar] [CrossRef]
- Ding, Y.Q.; Peng, Y.B.; Li, J. Physically based phase spectrum and simulation of strong earthquake ground motions. In Proceedings of the 16th World Conference on Earthquake Engineering, Santiago, Chile, 9–13 January 2017. [Google Scholar]
- Li, L.; Silva-Castro, J. Synthesis of single-hole signatures by group delay for ground vibration control in rock blasting. J. Vib. Control 2020, 26, 1273–1284. [Google Scholar] [CrossRef]
- Dai, M.; Li, Y.; Dong, Y. Incorporation of envelope delays and amplifications into simulation of far-field long-period ground motions. Soil Dyn. Earthq. Eng. 2020, 136, 106192. [Google Scholar] [CrossRef]
- Kumari, N.; Gupta, I.D.; Sharma, M.L. Synthesizing nonstationary earthquake ground motion via empirically simulated equivalent group velocity dispersion curves for Western Himalayan region. Bull. Seismol. Soc. Am. 2018, 108, 3469–3487. [Google Scholar] [CrossRef]
- Liao, Z. Introduction to Wave Motion Theories in Engineering, 2nd ed.; Science Press: Beijing, China, 2002. [Google Scholar]
- El-Nabulsi, R.A.; Anukool, W. Fractal dimension modeling of seismology and earthquakes dynamics. Acta Mech. 2022, 233, 2107–2122. [Google Scholar] [CrossRef]
- Perez, J.S.; Llamas, D.C.; Buhay, D.J.; Constantino, R.C.; Legaspi, C.J.; Lagunsad, K.D.; Grutas, R.N.; Quimson, M.M. Impacts of a Moderate-Sized Earthquake: The 2023 Magnitude (Mw) 4.7 Leyte, Leyte Earthquake, Philippines. Geosciences 2024, 14, 61. [Google Scholar] [CrossRef]









| No. | Earthquake | Date | Magnitude | Station Name/Code | Azimuth |
|---|---|---|---|---|---|
| 1 | Chi-Chi | 20 September 1999 | 7.62 | HWA041 | EW |
| 2 | Big Bear-01 | 28 June 1992 | 6.46 | LA-1955 1/2 Purdue Ave. Bsmt | 235° |
| 3 | Wenchuan | 12 May 2008 | 8.0 | 051AXT | NS |
| No. | Earthquake | Magnitude | Date | Epicenter | Station | Azimuth | Epicentral Distance (km) | Site Condition | vs30 (m/s) | |||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Latitude | Longitude | No. | Latitude | Longitude | ||||||||
| 1 | Chi-Chi | 7.62 | 20 September 1999 | 23.86 | 120.80 | ILA067 | 24.44 | 121.37 | EW | 86.38 | Soil | 553.4 |
| 2 | Chi-Chi | 7.62 | 20 September 1999 | 23.86 | 120.80 | TAP081 | 25.02 | 121.98 | EW | 175.3 | Soil | 553.4 |
| 3 | Chi-Chi (aftershock) | 6.2 | 20 September 1999 | 23.81 | 120.85 | ILA067 | 24.44 | 121.37 | EW | 87.94 | Soil | 553.4 |
| 4 | Wenchuan | 8.0 | 12 May 2008 | 31.00 | 103.40 | 51BXZ | 30.50 | 102.90 | EW | -- | Rock | -- |
| 5 | Wenchuan | 8.0 | 12 May 2008 | 31.00 | 103.40 | 51BXY | 30.50 | 102.90 | EW | -- | Soil | -- |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, Y.; Xu, Y.; Miao, H. Mathematical and Physical Characteristics of the Phase Spectrum of Earthquake Ground Motions. Buildings 2024, 14, 1250. https://doi.org/10.3390/buildings14051250
Ding Y, Xu Y, Miao H. Mathematical and Physical Characteristics of the Phase Spectrum of Earthquake Ground Motions. Buildings. 2024; 14(5):1250. https://doi.org/10.3390/buildings14051250
Chicago/Turabian StyleDing, Yanqiong, Yazhou Xu, and Huiquan Miao. 2024. "Mathematical and Physical Characteristics of the Phase Spectrum of Earthquake Ground Motions" Buildings 14, no. 5: 1250. https://doi.org/10.3390/buildings14051250
APA StyleDing, Y., Xu, Y., & Miao, H. (2024). Mathematical and Physical Characteristics of the Phase Spectrum of Earthquake Ground Motions. Buildings, 14(5), 1250. https://doi.org/10.3390/buildings14051250






