Influence and Mechanism of the Excavation Width on Excavation Deformations in Shanghai Soft Clay
Abstract
1. Introduction
2. Numerical Model
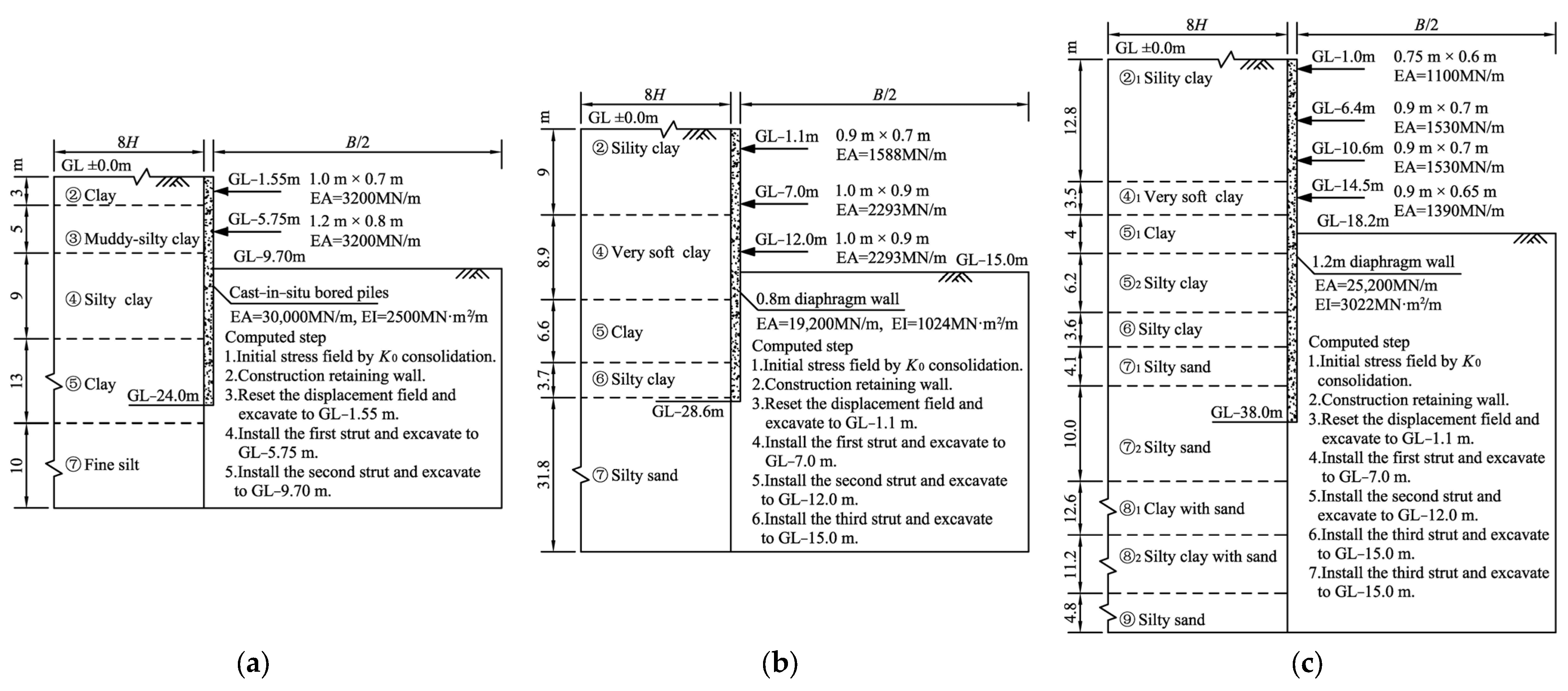
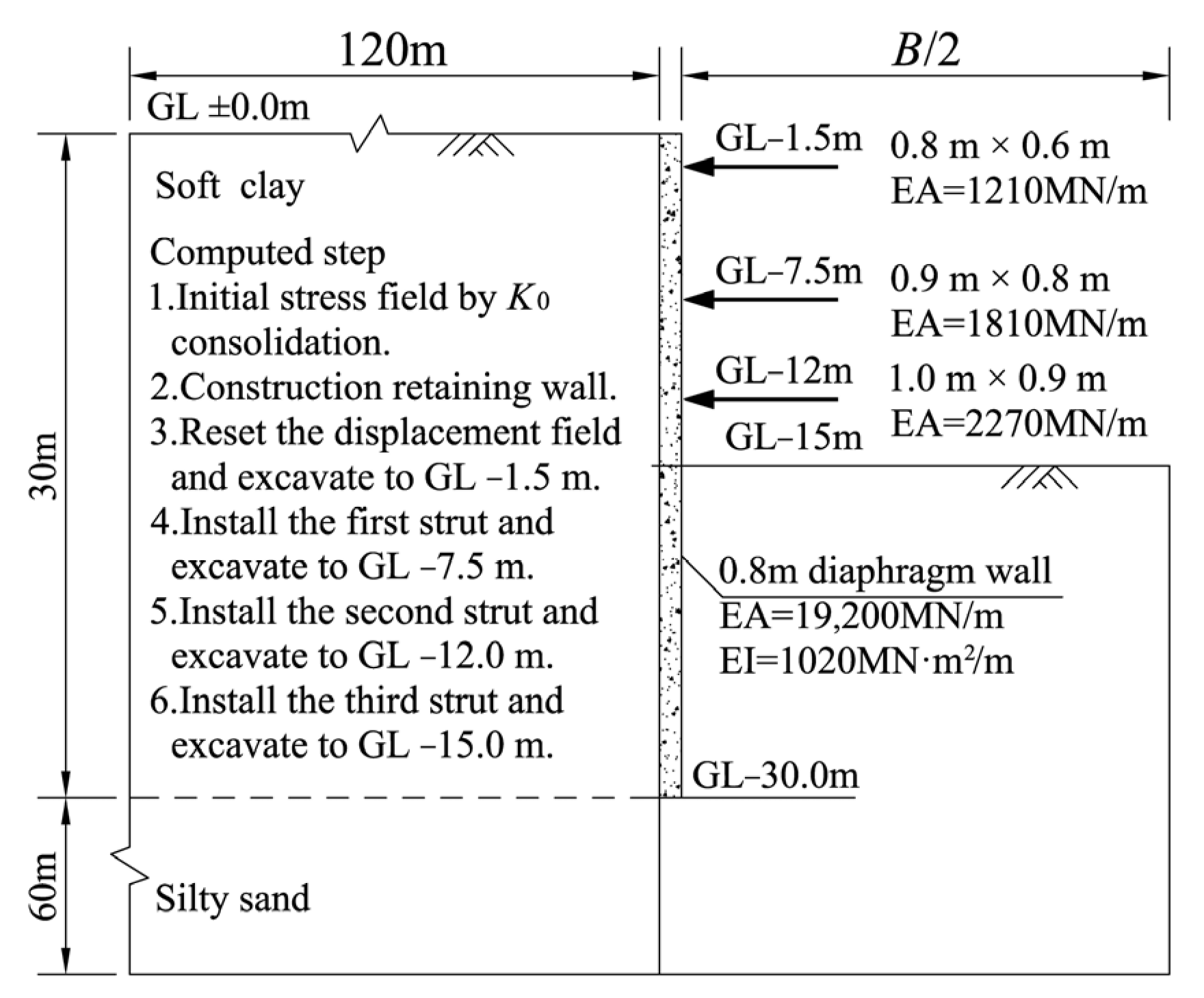
2.1. Project Overview
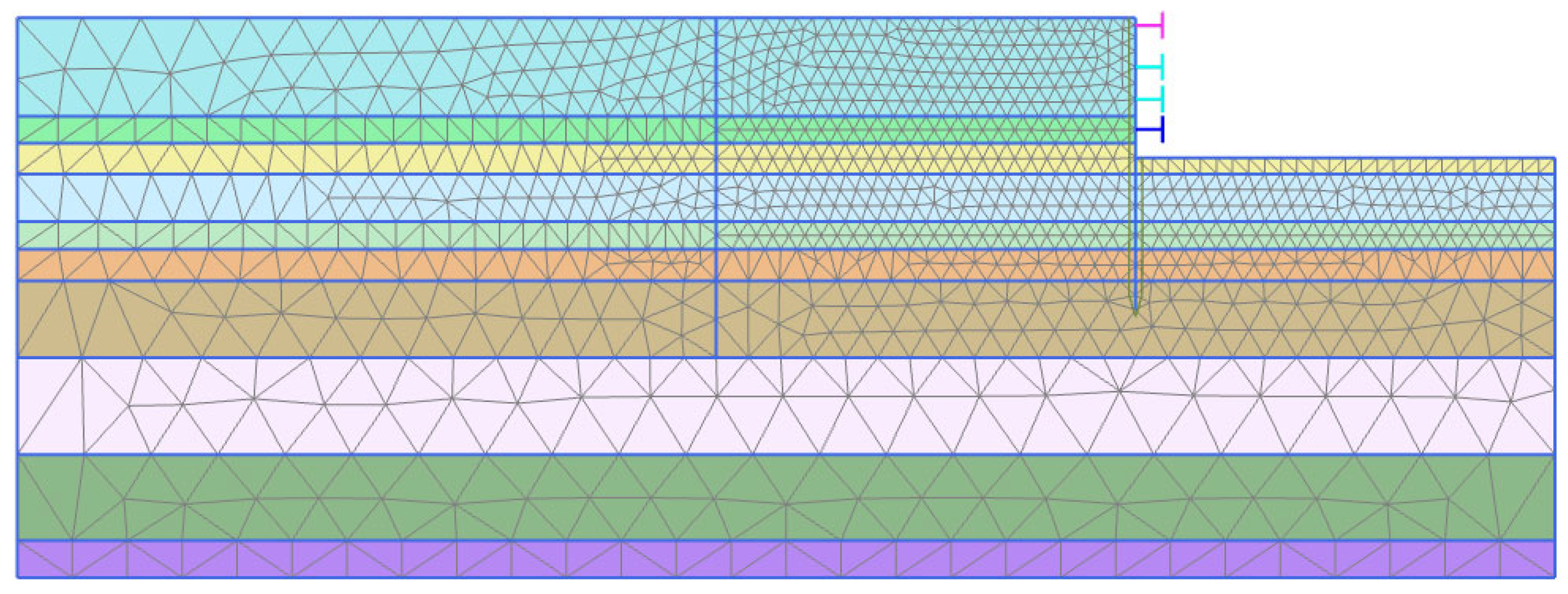
2.2. Numerical Analysis Models
2.3. Constitutive Models and Input Parameters
2.4. Validation of the Model Parameters
3. Influence of Excavation Width on Deformations
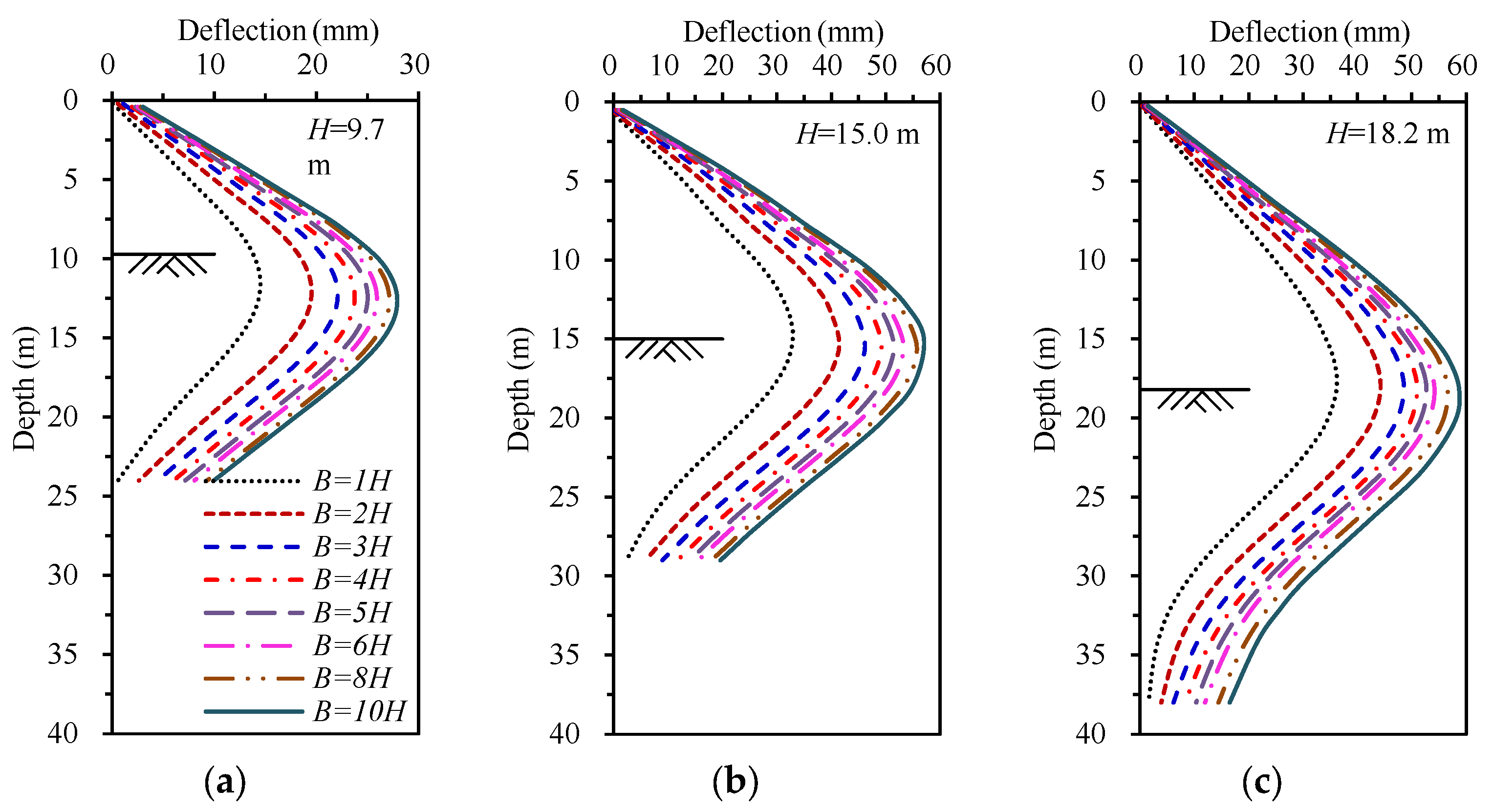
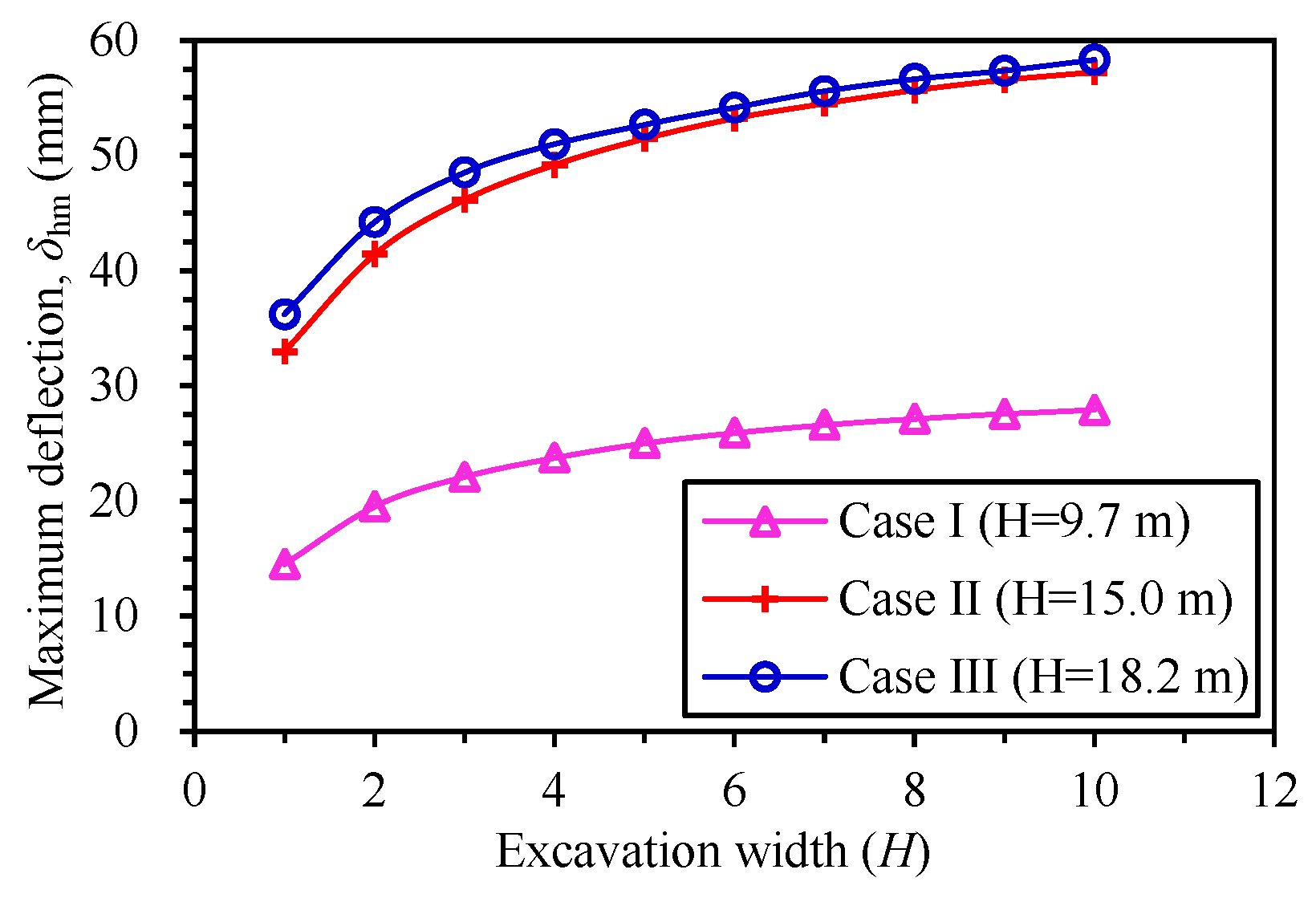
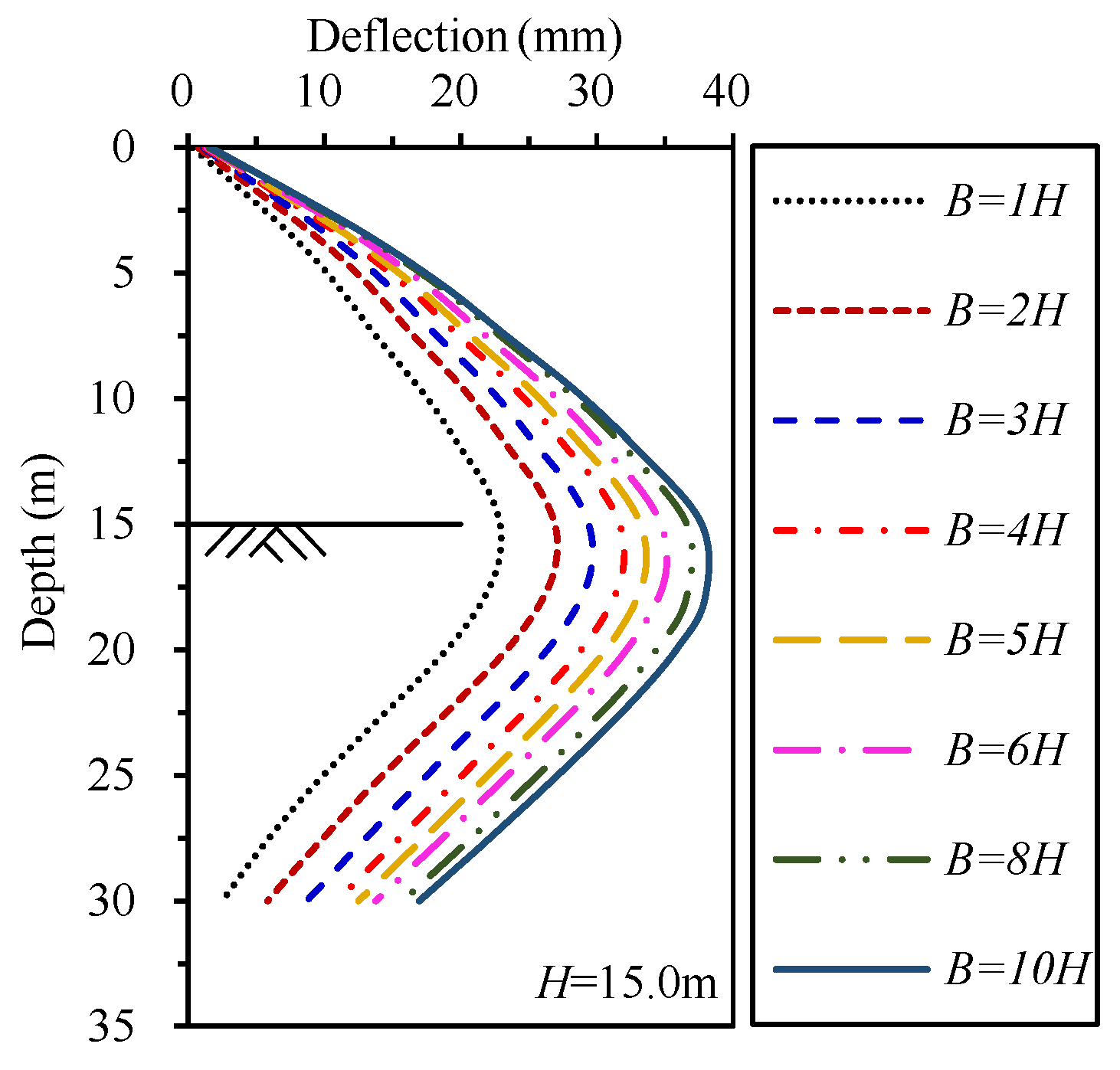
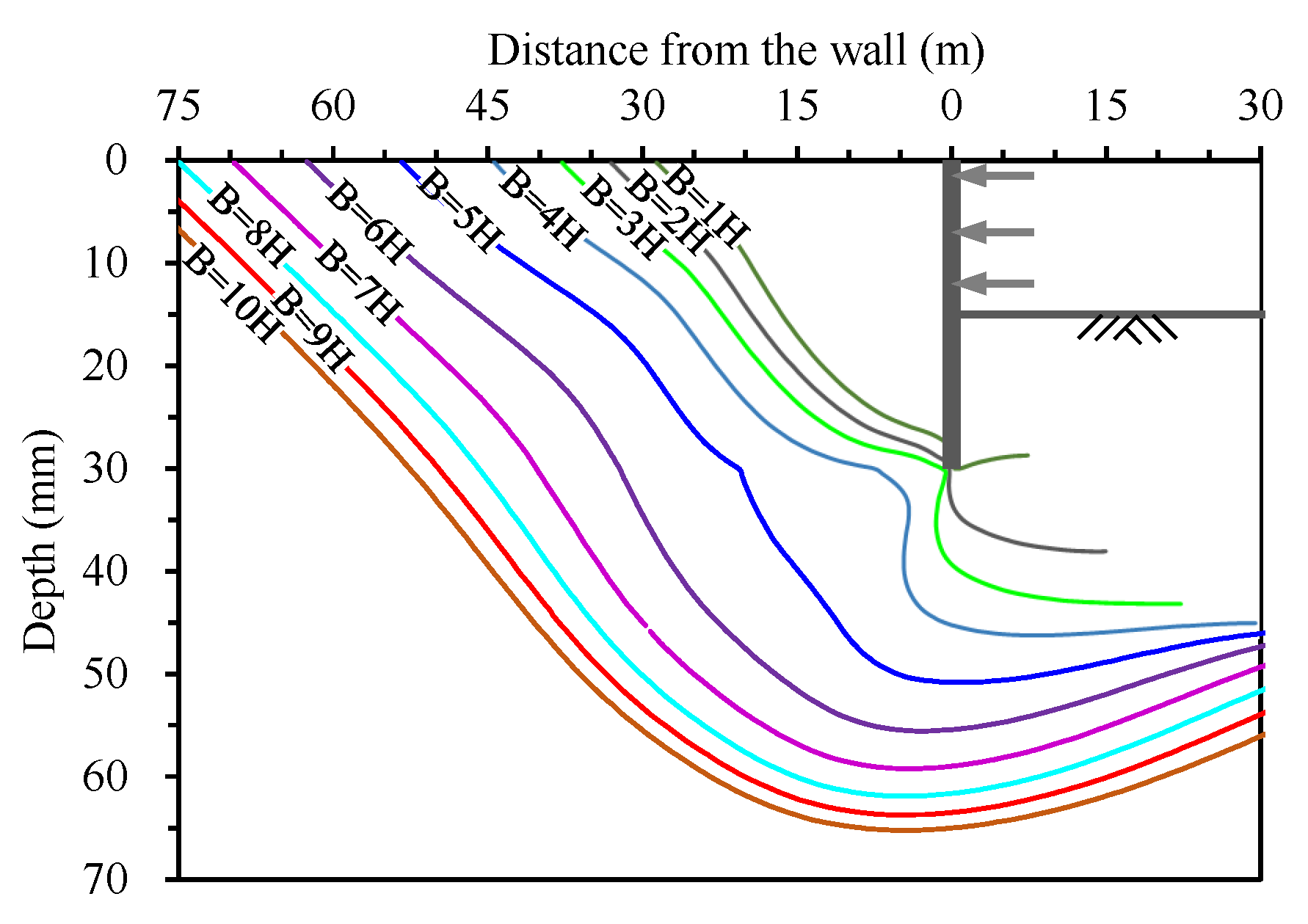
3.1. Deflections of Retaining Wall
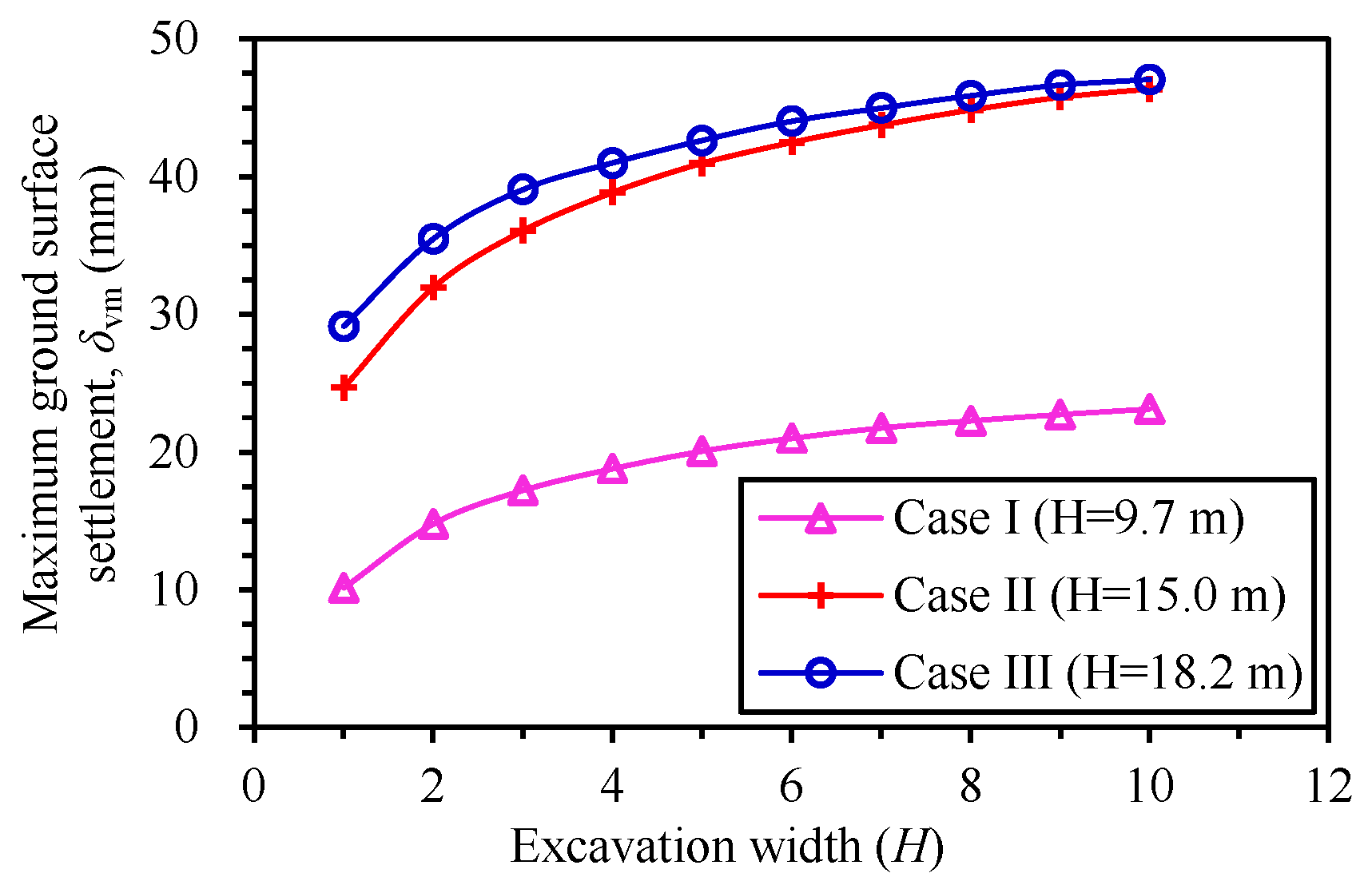
3.2. Ground Surface Settlements
4. Influence Mechanism of Excavation Width on Deformation
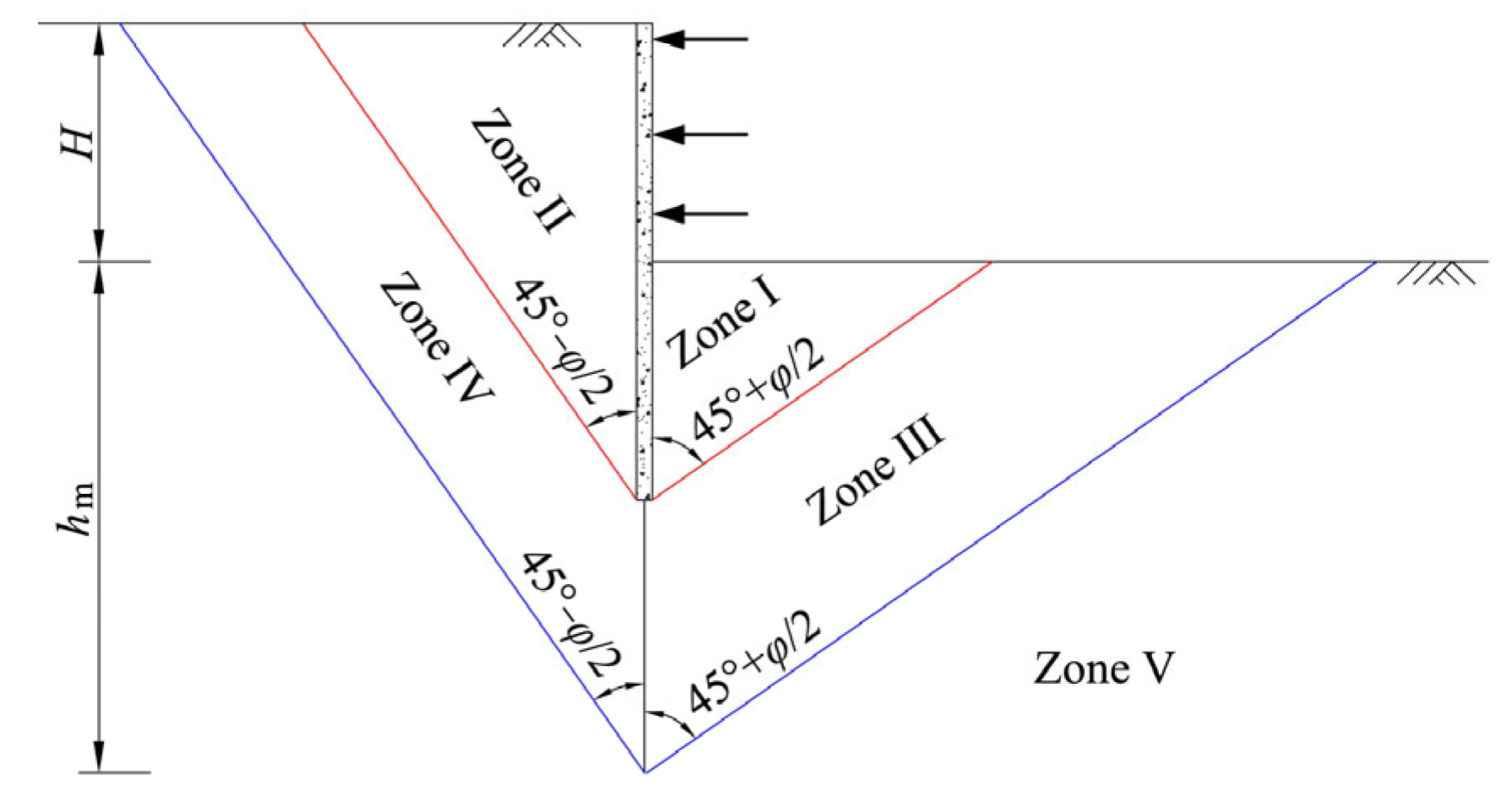
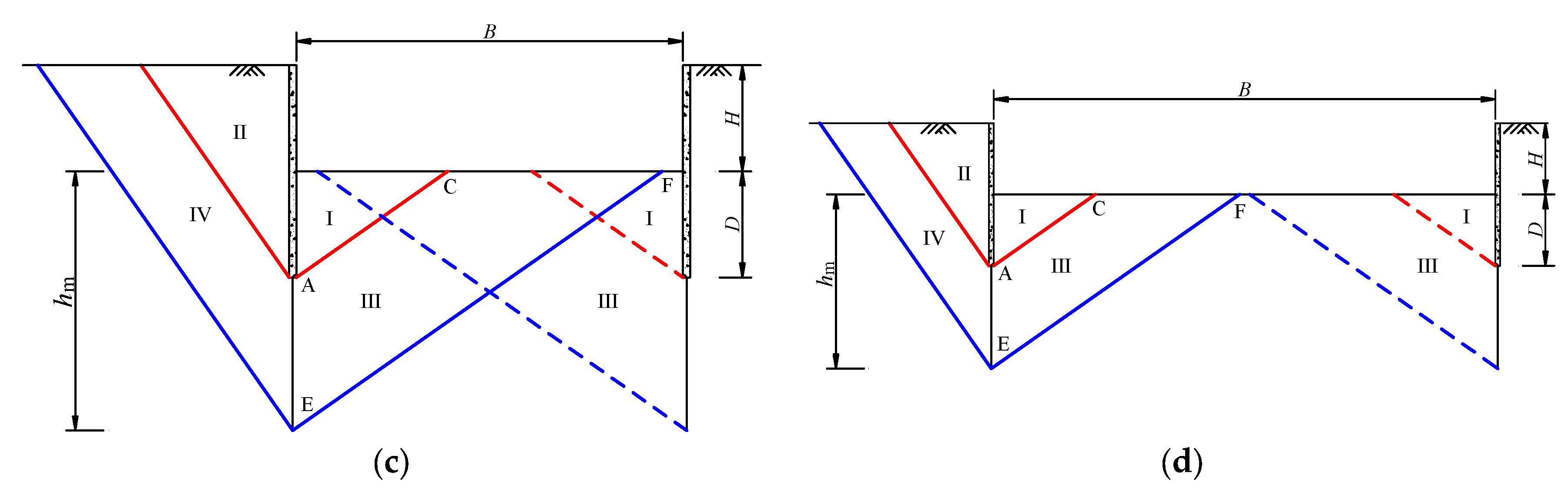
4.1. Analysis Models
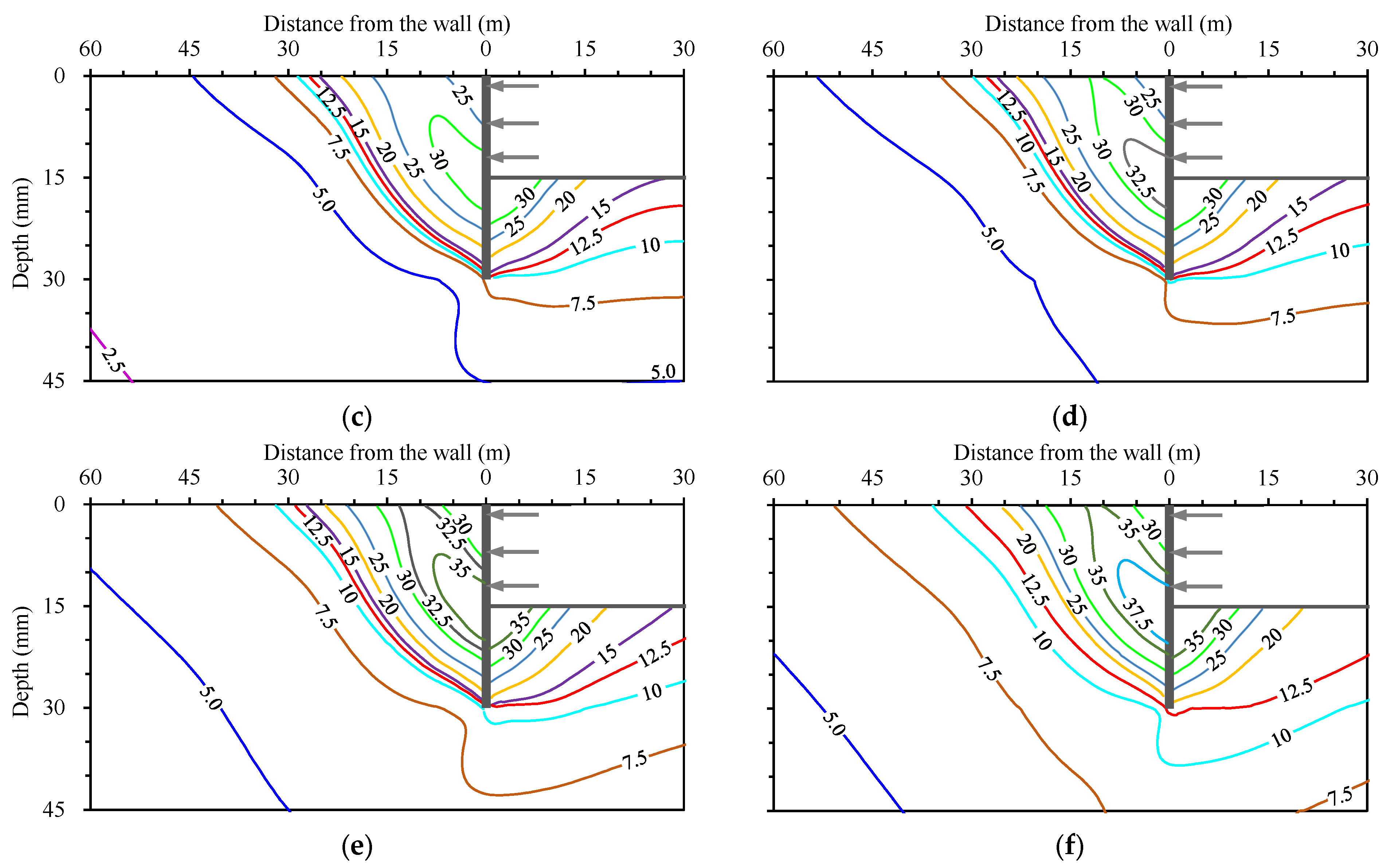
4.2. Development of the Total Ground Displacements
4.3. Basal Heaves
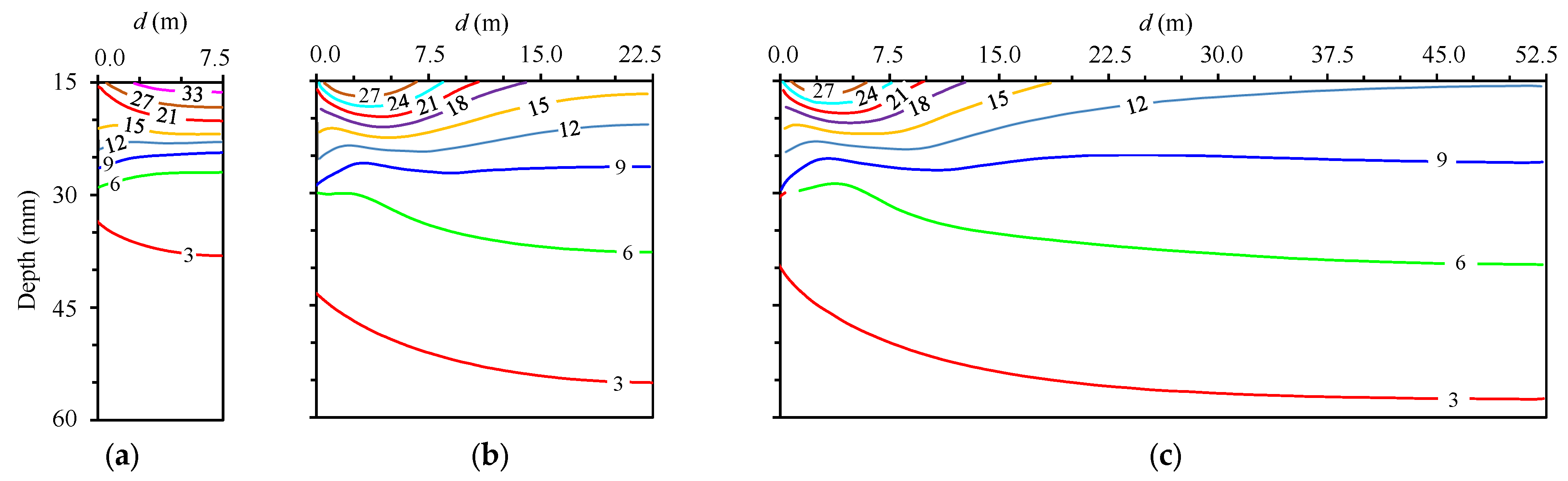
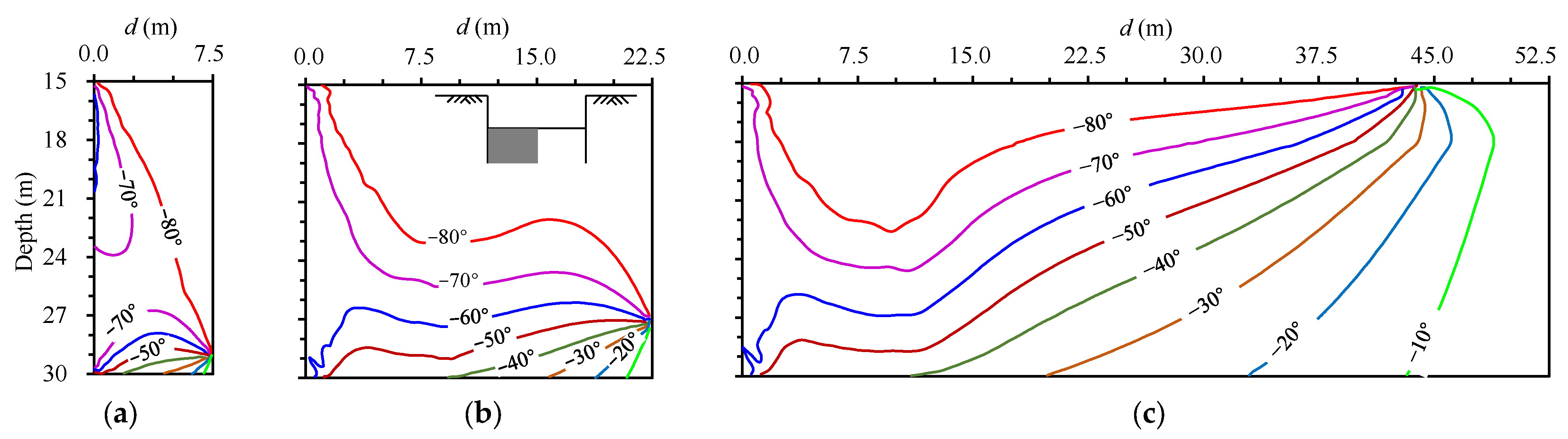
4.4. Overlap Degree of the Passive Zone Soil under Excavation Surface
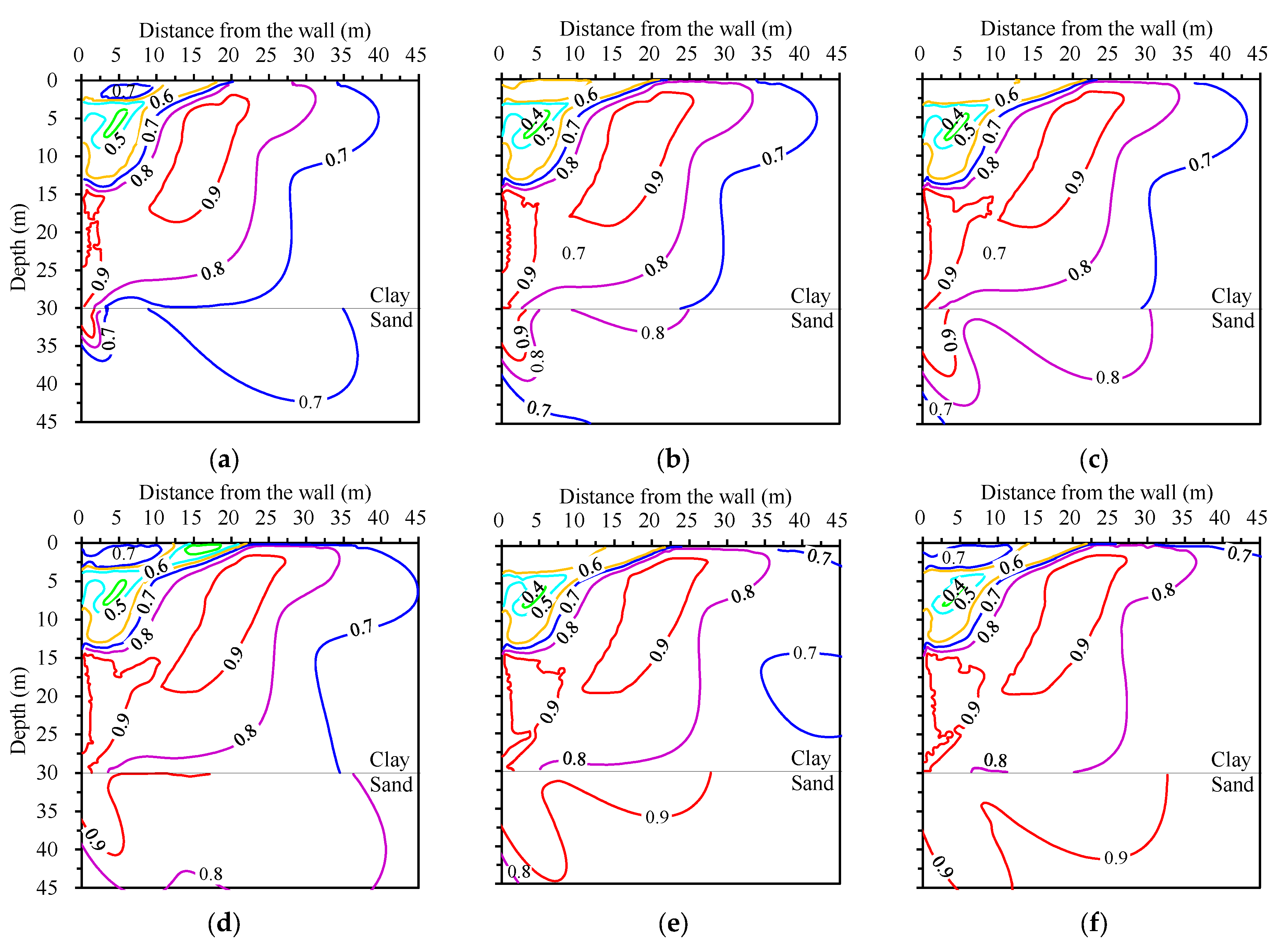
4.5. Stress State of the Basal Soils
4.6. Relative Shear Stress
5. Conclusions
- (1)
- The variation in the excavation width significantly impacts excavation deformations. The horizontal displacements of the retaining walls, ground surface settlements and maximum unloading influence depth increase with the increasing excavation width, but the increasing rate gradually reduces. When B ≤ 4H, the excavation width significantly affects the excavation deformations. When B > 4H, the impact of the excavation width on the deformations gradually decreases. Smaller excavation widths correlate with greater influences on deformations.
- (2)
- The overlap of the passive zone beneath the excavation’s bottom gradually decreases as the excavation width increases. The larger the overlapped area of the passive zone, the stronger the restraint effect is on the retaining wall and the smaller the deformations induced by excavation are.
- (3)
- When the excavation width is small, the major principal stress direction of basal soils tends to be closer to horizontal, and the soil states are closer to the passive state. Hence, the constraint ability of the passive zone on excavation deformations strengthens. With increasing excavation width, the depth and direction rotation of the major principal stress gradually decrease. Consequently, the constraint ability of the passive zone on excavation deformations is further reduced.
- (4)
- As the excavation width increases, the relative shear stress of the soils near the excavation gradually stabilizes, and the relative shear stress of the distant and deep soils gradually expands. Therefore, the deformations attributed to excavation slowly increase, especially after an excavation width larger than 7H.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| H | The final excavation depth. |
| B | Excavation width. |
| h | Layer thickness. |
| γ | Unit weight. |
| Es1–2 | Compressive modulus of 100~200 kPa corresponding to consolidation test. |
| e | Void ratio. |
| c′ | Effective cohesion. |
| φ′ | Effective internal friction angle (φ′). |
| Secant stiffness in standard drained triaxial test. | |
| Tangent stiffness for primary oedometer loading. | |
| Unloading/reloading stiffness for drained triaxial test. | |
| m | Power for stress level dependency of stiffness. |
| ψ | Dilatancy angle. |
| γ0.7 | Threshold shear strain. |
| Shear modulus specified at the reference mean stress. | |
| K0 | Coefficient of lateral earth pressure at rest. |
| Rf | Failure ratio. |
| pref | Reference stress for stiffness. |
| vu | Poisson’s ratio for unloading-reloading. |
| δhm | The maximum wall deflection. |
| δvm | The maximum ground surface settlements. |
| α | The direction rotation angle of the major principal stress. |
| τrel | The variations in soil relative shear stress. |
| τmob | The maximum value of shear stress at the current stress state (half the maximum principal stress difference). |
| τmax | The maximum value of shear stress for the case where Mohr’s circle is expanded to touch the Coulomb failure envelope while keeping the center of Mohr’s circle constant. |
References
- Wang, J.H.; Xu, Z.H.; Wang, W.D. Wall and ground movements due to deep excavations in Shanghai soft soils. J. Geotech. Geoenviron. Eng. 2010, 136, 985–994. [Google Scholar] [CrossRef]
- Bolton, M.D.; Lam, S.Y.; Vardanega, P.J.; Ng, C.W.W.; Ma, X.F. Ground movements due to deep excavations in Shanghai: Design charts. Front. Struct. Civ. Eng. 2014, 8, 201–236. [Google Scholar] [CrossRef]
- Tan, Y.; Fan, D.; Lu, Y. Statistical analyses on a database of deep excavations in Shanghai soft clays in China from 1995–2018. Pr. Period. Struct. Des. Constr. 2022, 27, 04021067. [Google Scholar] [CrossRef]
- Mana, A.I.; Clough, G.W. Prediction of movements for braced cuts in clay. J. Geotech. Geoenviron. Eng. 1981, 107, 759–777. [Google Scholar] [CrossRef]
- Jia, J.; Xie, X.L. Unloading deformation mechanism of deep-large excavation in Shanghai clay area. J. Shanghai Jiaotong Univ. 2009, 43, 1005–1010. (In Chinese) [Google Scholar]
- Tan, Y.; Wang, D. Characteristics of a large-scale deep foundation pit excavated by the central-island technique in Shanghai soft clay. I: Bottom-up construction of the central cylindrical shaft. J. Geotech. Geoenviron. Eng. 2013, 139, 1875–1893. [Google Scholar] [CrossRef]
- Tan, Y.; Wang, D. Characteristics of a large-scale deep foundation pit excavated by central-island technique in shanghai soft clay. II: Top-down construction of the peripheral rectangular pit. J. Geotech. Geoenviron. Eng. 2013, 139, 1894–1910. [Google Scholar] [CrossRef]
- Xiao, H.; Zhou, S.; Sun, Y. Wall deflection and ground surface settlement due to excavation width and foundation pit classification. Ksce J. Civ. Eng. 2019, 23, 1537–1547. [Google Scholar] [CrossRef]
- Wang, H.X. Influence of excavation width on enclosure-structure stability of foundation pits. China Civ. Eng. J. 2011, 44, 120–126. (In Chinese) [Google Scholar]
- Cheng, K.; Xu, R.; Ying, H.; Gan, X.; Zhang, L.; Liu, S. Observed performance of a 30.2 m deep-large basement excavation in Hangzhou soft clay. Tunn. Undergr. Space Technol. 2021, 111, 103872. [Google Scholar] [CrossRef]
- Jia, J.; Zhai, J.Q.; Li, M.G.; Zhang, L.L.; Xie, X.L. Performance of large-diameter circular diaphragm walls in a deep excavation: Case study of Shanghai Tower. J. Aerosp. Eng. 2019, 32, 04019078. [Google Scholar] [CrossRef]
- Liu, G.; Guo, J.; Li, M.; Qin, T.; Huang, P. Measured behaviors of an oversized irregular basement excavation and its surrounding responses in thick soft clay. Arab. J. Geosci. 2020, 13, 3. [Google Scholar] [CrossRef]
- Liu, Y.; Xiang, B.; Fu, M. Observed performance of a large-scale deep triangular excavation in Shanghai soft clays. Geotech. Geol. Eng. 2019, 37, 2791–2809. [Google Scholar] [CrossRef]
- Tan, Y.; Lu, Y.; Wang, D. Deep excavation of the Gate of the Orient in Suzhou stiff clay: Composite earth retaining systems and dewatering plans. J. Geotech. Geoenviron. Eng. 2018, 144, 05017009. [Google Scholar] [CrossRef]
- Xu, G.; Zhang, J.; Liu, H.; Ren, C. Shanghai center project excavation induced ground surface movements and deformations. Front. Struct. Civ. Eng. 2018, 12, 26–43. [Google Scholar] [CrossRef]
- Ni, X.; Lu, J.; Wang, Y.; Shi, J.; Chen, W.; Tang, L. Field investigation of the influence of basement excavation and dewatering on ground and structure responses. Tunn. Undergr. Space Technol. 2021, 117, 104121. [Google Scholar] [CrossRef]
- Tan, Y.; Li, M. Measured performance of a 26 m deep top-down excavation in downtown Shanghai. Can. Geotech. J. 2011, 48, 704–719. [Google Scholar] [CrossRef]
- Tan, Y.; Wang, D.; Lu, Y.; Fang, T. Excavation of Middle Huai-Hai Road station of Shanghai metro line 13: Challenges, risks, countermeasures and performance assessment. Pract. Pr. Period. Struct. Des. Constr. 2017, 22, 05017003. [Google Scholar] [CrossRef]
- Tan, Y.; Wei, B.; Diao, Y.; Zhou, X. Spatial corner effects of long and narrow multipropped deep excavations in Shanghai soft clay. J. Perform. Constr. Facil. 2014, 28, 04014015. [Google Scholar] [CrossRef]
- Wang, Z.W.; Ng, C.W.W.; Liu, G.B. Characteristics of wall deflections and ground surface settlements in Shanghai. Can. Geotech. J. 2005, 42, 1243–1254. [Google Scholar] [CrossRef]
- Maleki, M.; Khezri, A.; Nosrati, M.; Majdeddin, M.M.H.S. Seismic amplification factor and dynamic response of soil-nailed walls. Model. Earth Syst. Environ. 2023, 9, 1181–1198. [Google Scholar] [CrossRef]
- Maleki, M.; Majdeddin, M.M.H.S. Assessment of the Pseudo-static seismic behavior in the soil nail walls using numerical analysis. Innov. Infrastruct. So. 2022, 7, 1–18. [Google Scholar] [CrossRef]
- Shi, J.; Wei, J.; Ng, C.W.W.; Lu, H.; Ma, S.; Shi, C.; Li, P. Effects of construction sequence of double basement excavations on an existing floating pile. Tunn. Undergr. Space Technol. 2022, 119, 104230. [Google Scholar] [CrossRef]
- Soomro, M.A.; Mangnejo, D.A.; Bhanbhro, R.; Memon, N.A.; Memon, M.A. 3D finite element analysis of pile responses to adjacent excavation in soft clay: Effects of different excavation depths systems relative to a floating pile. Tunn. Undergr. Space Technol. 2019, 86, 138–155. [Google Scholar] [CrossRef]
- Tan, Y.; Jiang, W.Z.; Rui, H.S.; Lu, Y.; Wang, D.L. Forensic geotechnical analyses on the 2009 building-overturning accident in Shanghai, China: Beyond common recognitions. J. Geotech. Geoenviron. Eng. 2020, 146, 05020005. [Google Scholar] [CrossRef]
- Zhang, R.; Goh, A.T.C.; Li, Y.; Hong, L.; Zhang, W. Assessment of apparent earth pressure for braced excavations in anisotropic clay. Acta Geotech. 2021, 16, 1615–1626. [Google Scholar] [CrossRef]
- Zhang, D.M.; Xie, X.C.; Li, Z.L.; Zhang, J. Simplified analysis method for predicting the influence of deep excavation on existing tunnels. Comput. Geotech. 2020, 121, 103477. [Google Scholar] [CrossRef]
- Gu, X.Q.; Wu, R.T.; Liang, F.Y.; Gao, G.Y. On HSS model parameters for Shanghai soils with engineering verification. Rock. Soil. Mech. 2021, 42, 833–845. (In Chinese) [Google Scholar]
- Lim, A.; Ou, C.Y.; Hsieh, P.G. A novel strut-free retaining wall system for deep excavation in soft clay: Numerical study. Acta Geotech. 2020, 15, 1557–1576. [Google Scholar] [CrossRef]
- Benz, T. Small-Strain Stiffness of Soils and Its Numerical Consequences. Ph.D. Thesis, Northwestern University, Evanston, IL, USA, 2007. [Google Scholar]
- Wang, W.D.; Wang, H.R.; Xu, Z.H. Study of parameters of HS-Small model used in numerical analysis of excavations in Shanghai area. Rock. Soil. Mech. 2013, 83, 413–419. (In Chinese) [Google Scholar]
- ECADI; SCG. Technical Code for Excavation Engineering; DG/TJ 08-61-2018; Tongji University Press: Shanghai, China, 2018. (In Chinese) [Google Scholar]
- Brinkgreve, R.B.J.; Kumarswamy, S.; Swolfs, W.M. PLAXIS 3D Tutorial Manual; Plaxis B.V.: Delft, The Netherlands, 2015. [Google Scholar]
- Harahap, S.E.; Ou, C.Y. Finite element analysis of time-dependent behavior in deep excavations. Comput. Geotech. 2020, 119, 103300. [Google Scholar] [CrossRef]
- Liu, S.; Li, H.; Tong, L. Simulation of pile cap contribution to the lateral pile performance due to adjacent excavation. Acta Geotech. 2021, 16, 1895–1907. [Google Scholar] [CrossRef]
- Zhang, W.; Li, Y.; Goh, A.T.C.; Zhang, R. Numerical study of the performance of jet grout piles for braced excavations in soft clay. Comput. Geotech 2020, 124, 103631. [Google Scholar] [CrossRef]





| Case | Layer | h (m) | γ (kN/m3) | Es1–2 (MPa) | e | c′ (kPa) | φ′ (°) | vur | (MPa) | (MPa) | (MPa) | m | ψ (°) | γ0.7 (10−4) | (MPa) | K0 | Rf | pref (kPa) | Rint |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Case I [27] | ② | 3 | 19.0 | — | — | 8.0 | 27.0 | 0.2 | 4.20 | 3.50 | 24.50 | 0.8 | 0 | 2.0 | 98.00 | 0.54 | 0.9 | 100 | 0.65 |
| ③ | 5 | 18.1 | — | — | 2.0 | 30.0 | 0.2 | 3.40 | 2.80 | 19.60 | 0.8 | 0 | 2.0 | 78.40 | 0.50 | 0.6 | 100 | 0.65 | |
| ④ | 9 | 17.8 | — | — | 3.0 | 28.0 | 0.2 | 2.20 | 1.80 | 12.90 | 0.8 | 0 | 2.0 | 51.70 | 0.53 | 0.6 | 100 | 0.65 | |
| ⑤ | 13 | 18.7 | — | — | 1.0 | 38.0 | 0.2 | 7.00 | 5.80 | 40.60 | 0.8 | 0 | 2.0 | 163.00 | 0.39 | 0.9 | 100 | 0.65 | |
| ⑦ | 10 | 17.8 | — | — | 1.0 | 38.0 | 0.2 | 8.50 | 8.50 | 33.90 | 0.5 | 8 | 2.0 | 169.00 | 0.38 | 0.9 | 100 | 0.70 | |
| Case II [28] | ② | 9.0 | 18.8 | — | 0.889 | 4.0 | 32.8 | 0.2 | 4.54 | 4.02 | 27.88 | 0.65 | 0 | 3.2 | 102.50 | 0.41 | 0.95 | 100 | 0.65 |
| ④ | 8.9 | 17.1 | — | 1.343 | 9.8 | 25.2 | 0.2 | 2.40 | 2.23 | 16.02 | 0.65 | 0 | 3.2 | 47.90 | 0.52 | 0.64 | 100 | 0.65 | |
| ⑤ | 6.6 | 18.5 | — | 0.949 | 5.0 | 31.4 | 0.2 | 4.20 | 3.74 | 26.02 | 0.65 | 0 | 3.2 | 90.60 | 0.43 | 0.95 | 100 | 0.65 | |
| ⑥ | 3.7 | 20 | — | 0.657 | 20.0 | 35.0 | 0.2 | 6.12 | 5.33 | 36.58 | 0.65 | 0 | 3.2 | 159.90 | 0.38 | 0.95 | 100 | 0.65 | |
| ⑦ | 31.9 | 18.9 | — | 0.820 | 2.6 | 31.0 | 0.2 | 10.59 | 8.41 | 50.83 | 0.70 | 1 | 3.9 | 131.80 | 0.48 | 0.95 | 100 | 0.70 | |
| Case III | ②1 | 12.8 | 18.5 | 8.02 | 0.875 | 5.0 | 26.0 | 0.2 | 6.35 | 5.29 | 31.76 | 0.8 | 0 | 2.0 | 95.28 | 0.56 | 0.9 | 100 | 0.65 |
| ④ | 3.5 | 16.8 | 2.14 | 1.397 | 4.0 | 23.0 | 0.2 | 2.31 | 1.93 | 15.41 | 0.8 | 0 | 2.0 | 46.22 | 0.61 | 0.6 | 100 | 0.65 | |
| ⑤1 | 4.0 | 17.9 | 3.62 | 1.068 | 3.0 | 27.0 | 0.2 | 3.91 | 3.26 | 19.55 | 0.8 | 0 | 2.0 | 58.64 | 0.54 | 0.9 | 100 | 0.65 | |
| ⑤2 | 6.2 | 18.2 | 4.48 | 0.970 | 5.0 | 30.0 | 0.2 | 4.84 | 4.03 | 24.19 | 0.8 | 0 | 3.2 | 96.77 | 0.51 | 0.9 | 100 | 0.65 | |
| ⑥ | 3.6 | 19.6 | 6.26 | 0.691 | 14.0 | 34.0 | 0.2 | 6.76 | 5.63 | 33.80 | 0.8 | 0 | 3.2 | 135.22 | 0.45 | 0.9 | 100 | 0.65 | |
| ⑦1 | 4.1 | 18.7 | 10.98 | 0.826 | 4.0 | 30.0 | 0.2 | 10.98 | 10.98 | 43.92 | 0.5 | 0 | 3.9 | 219.60 | 0.50 | 0.9 | 100 | 0.70 | |
| ⑦2 | 10.0 | 18.9 | 12.37 | 0.769 | 2.0 | 31.0 | 0.2 | 12.37 | 12.37 | 49.48 | 0.5 | 1 | 3.9 | 247.40 | 0.48 | 0.9 | 100 | 0.70 | |
| ⑧1 | 12.6 | 18.1 | 5.15 | 1.013 | 5.0 | 30.0 | 0.2 | 5.56 | 4.64 | 27.81 | 0.5 | 0 | 2.0 | 111.24 | 0.49 | 0.9 | 100 | 0.65 | |
| ⑧2 | 11.2 | 18.4 | 5.44 | 0.921 | 4.0 | 32.0 | 0.2 | 5.88 | 4.90 | 29.38 | 0.5 | 0 | 2.0 | 117.50 | 0.47 | 0.9 | 100 | 0.65 | |
| ⑨ | 17.4 | 19.2 | 10.06 | 0.721 | 2.0 | 32.0 | 0.2 | 10.06 | 10.06 | 40.24 | 0.5 | 2 | 3.9 | 201.2 | 0.47 | 0.9 | 100 | 0.70 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, P.; Dang, K.; Shi, H.; Yang, K.; Wu, J. Influence and Mechanism of the Excavation Width on Excavation Deformations in Shanghai Soft Clay. Buildings 2024, 14, 1450. https://doi.org/10.3390/buildings14051450
Huang P, Dang K, Shi H, Yang K, Wu J. Influence and Mechanism of the Excavation Width on Excavation Deformations in Shanghai Soft Clay. Buildings. 2024; 14(5):1450. https://doi.org/10.3390/buildings14051450
Chicago/Turabian StyleHuang, Pei, Kexin Dang, Haili Shi, Kun Yang, and Jiacheng Wu. 2024. "Influence and Mechanism of the Excavation Width on Excavation Deformations in Shanghai Soft Clay" Buildings 14, no. 5: 1450. https://doi.org/10.3390/buildings14051450
APA StyleHuang, P., Dang, K., Shi, H., Yang, K., & Wu, J. (2024). Influence and Mechanism of the Excavation Width on Excavation Deformations in Shanghai Soft Clay. Buildings, 14(5), 1450. https://doi.org/10.3390/buildings14051450






