A Numerical Study of the Lateral Load-Sharing Mechanism of the Pile Cap in a 3 × 3 Pile Group
Abstract
:1. Introduction
2. Field Test and Numerical Model
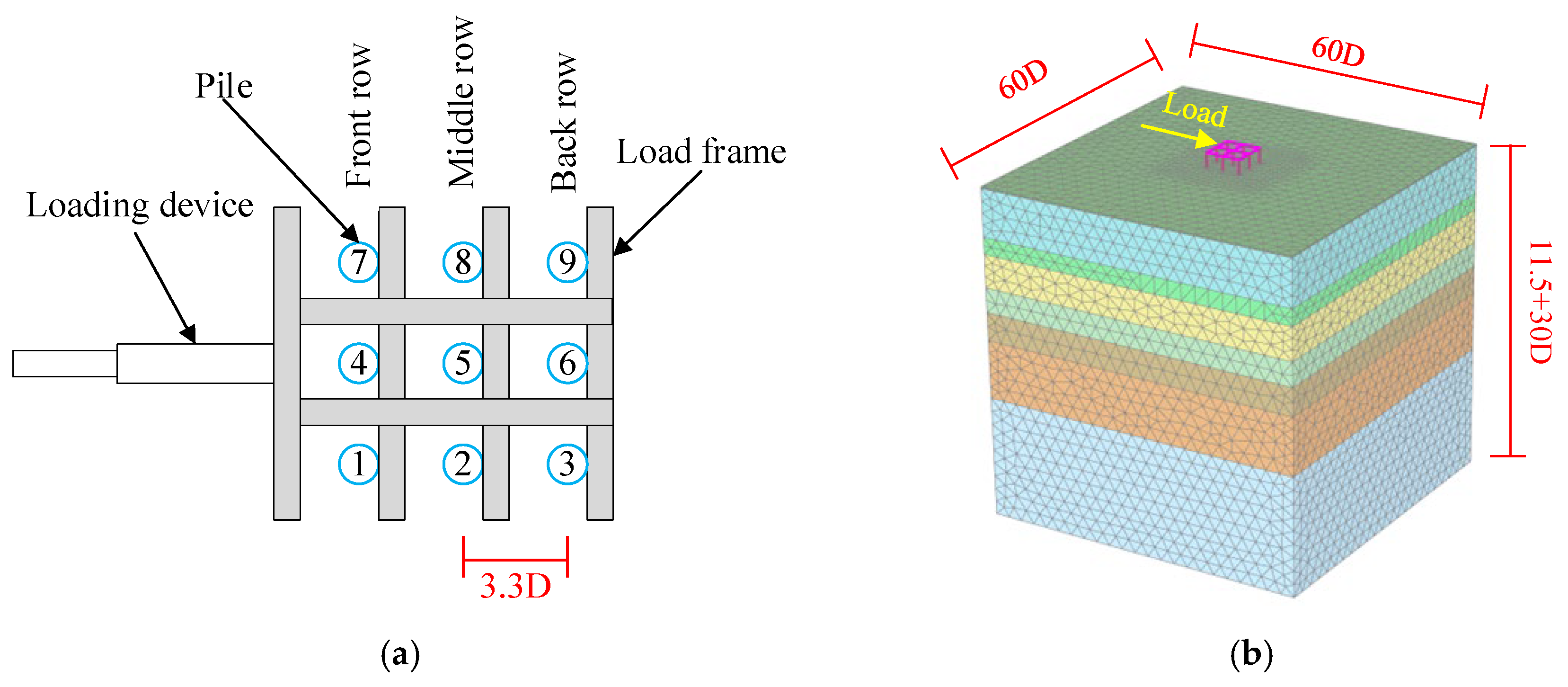
2.1. Introduction to the Field Test
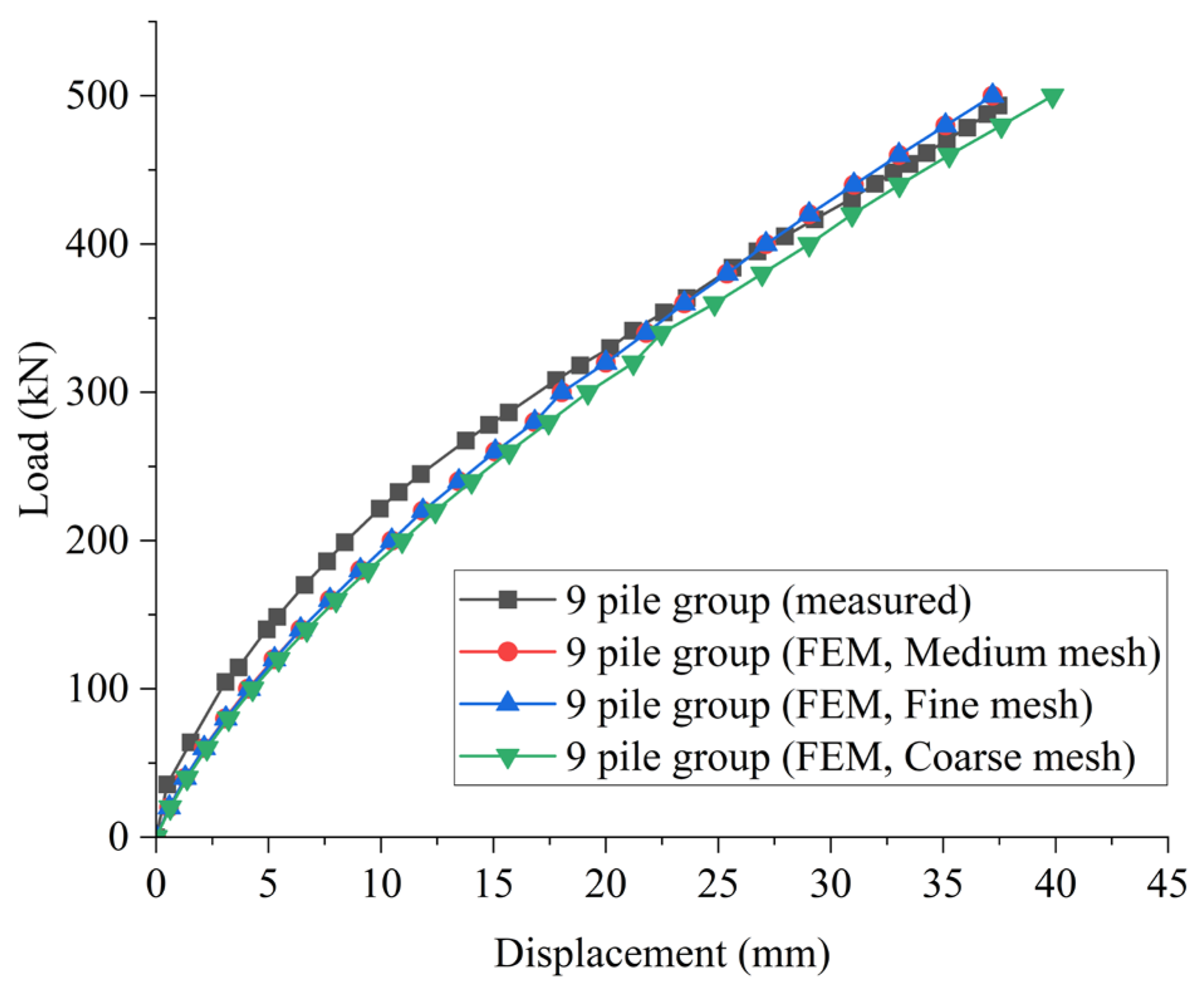
2.2. Reliability Verification of the Numerical Model
3. Horizontal Load Response Analysis of the Pile Group with a Pile Cap
3.1. Establishment of the Finite Element Model
3.2. The Impact of the Pile Cap on the Horizontal Load Bending Characteristics of the Pile Group
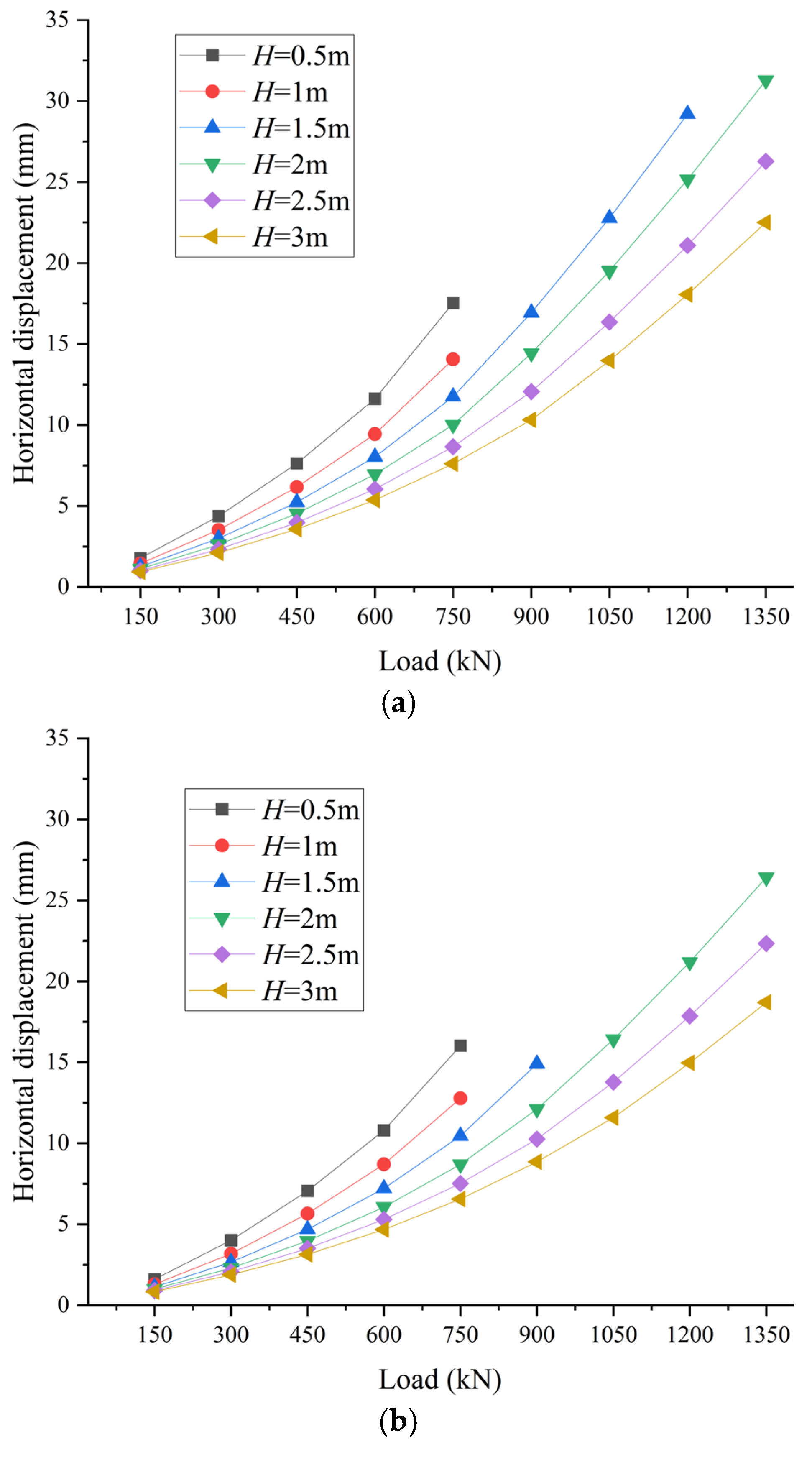
3.2.1. The Load–Displacement Curve
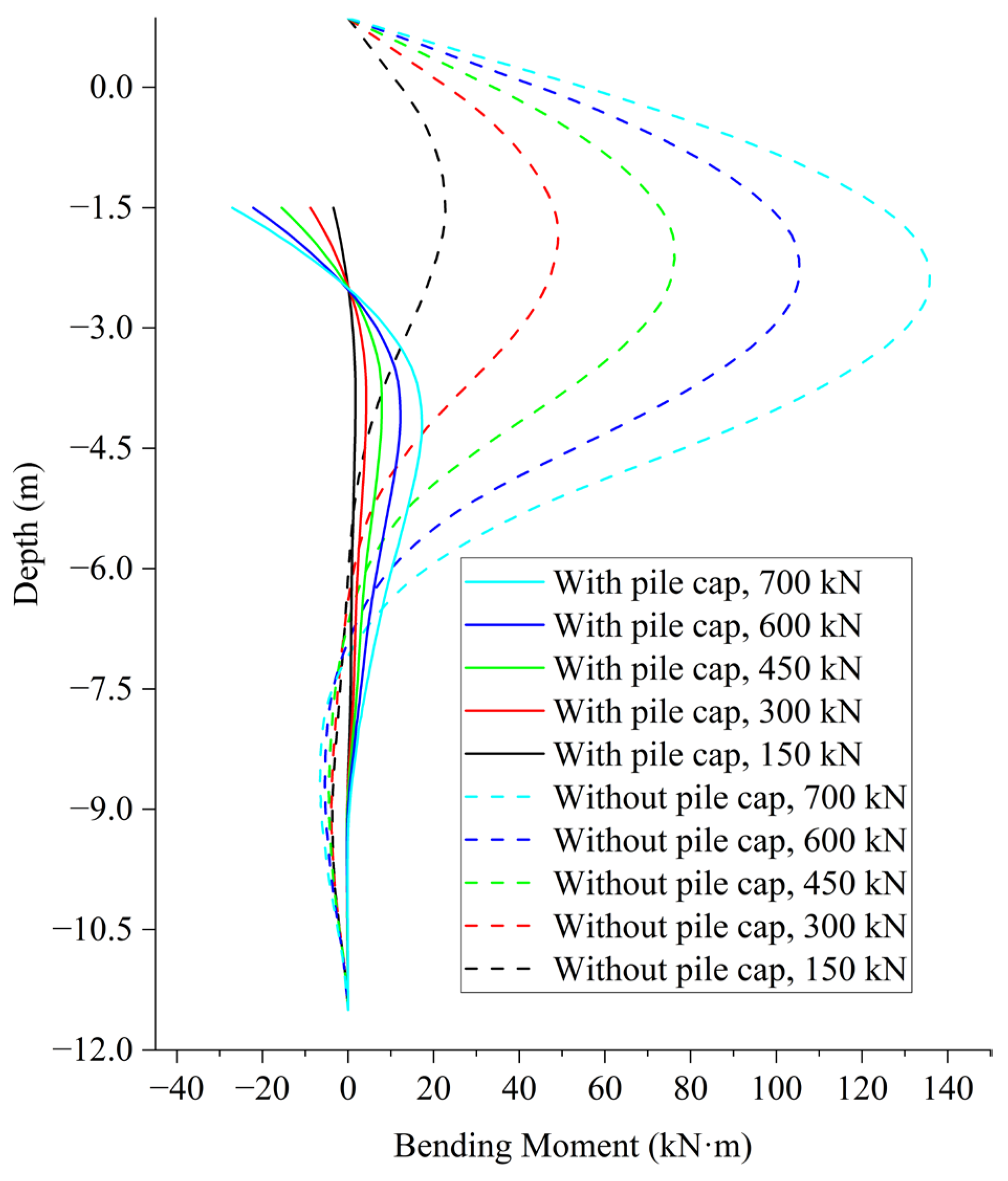
3.2.2. The Impact of the Pile Cap on the Bending Moment of Piles in the Pile Group
3.2.3. Horizontal Displacement of the Pile Cap
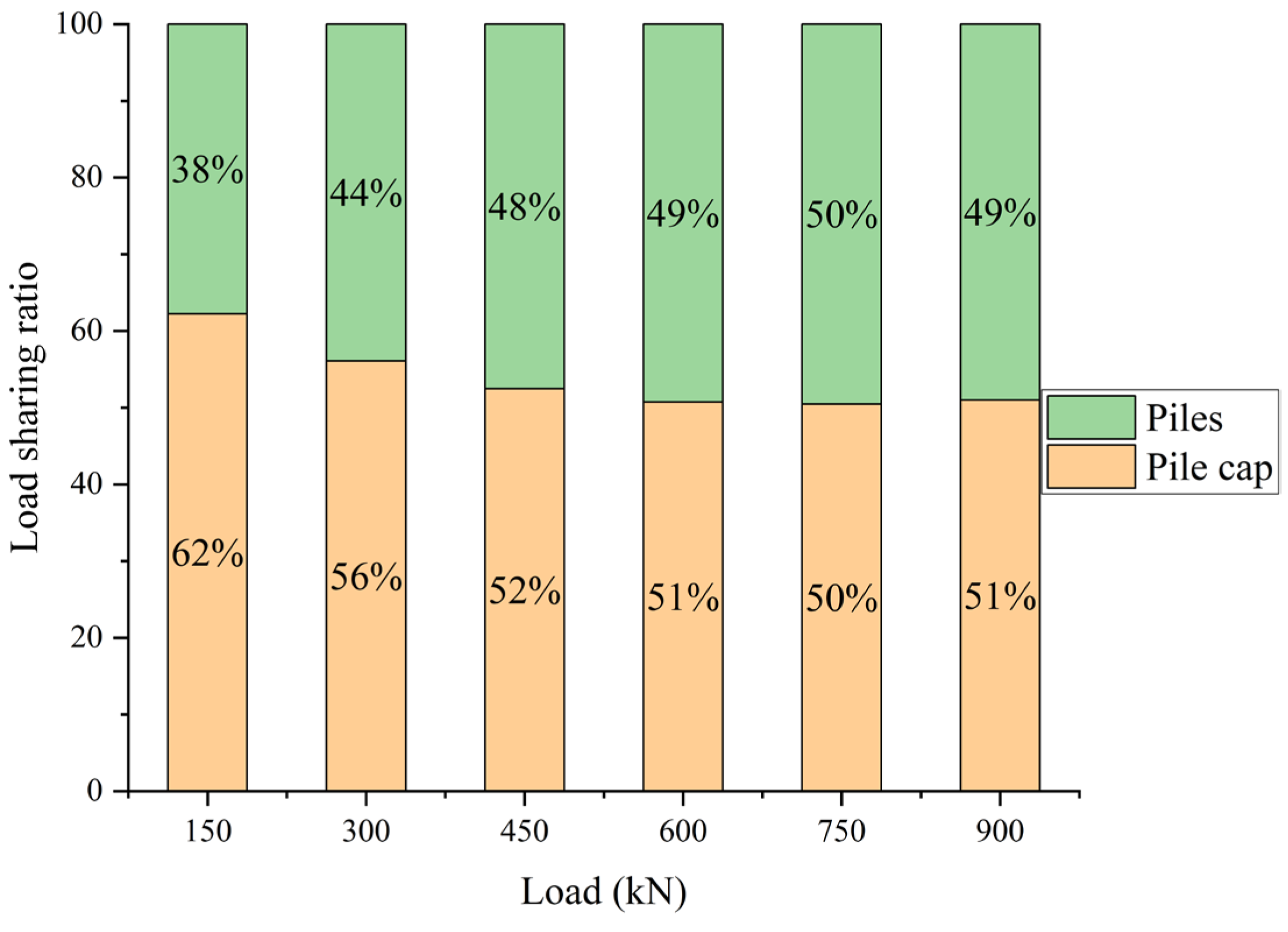
3.2.4. Load Sharing on the Pile Cap
4. Parametric Studies
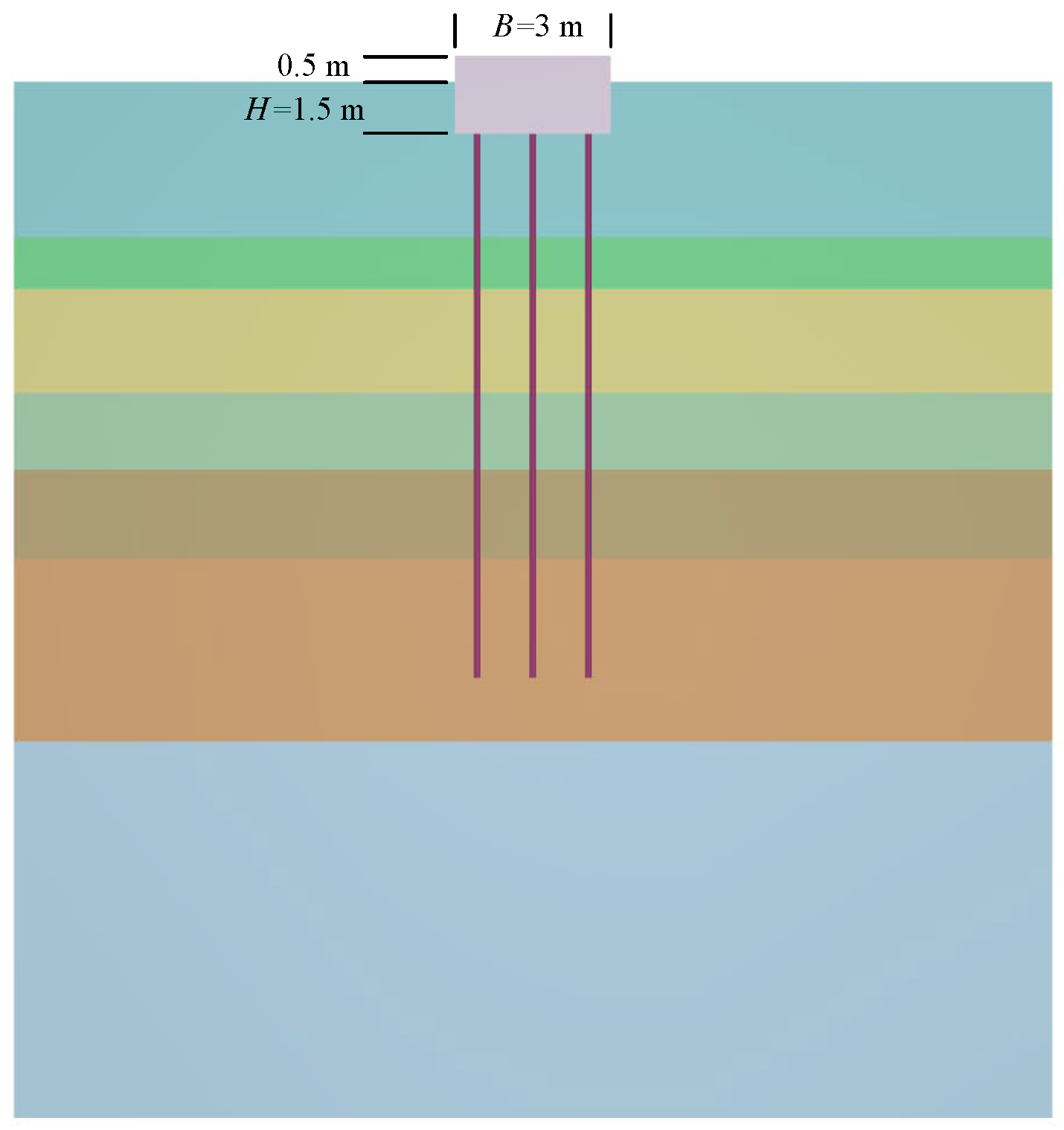
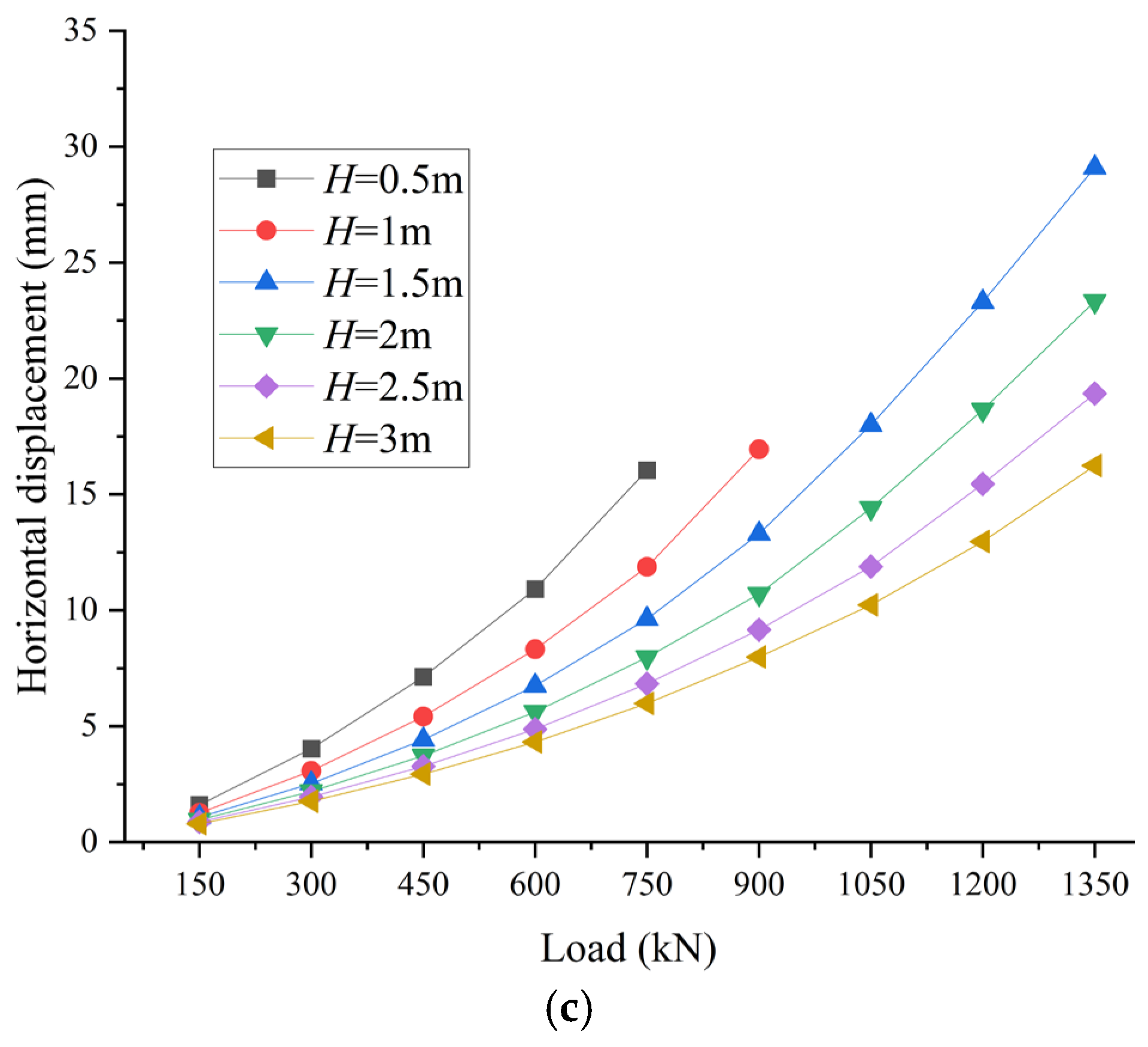
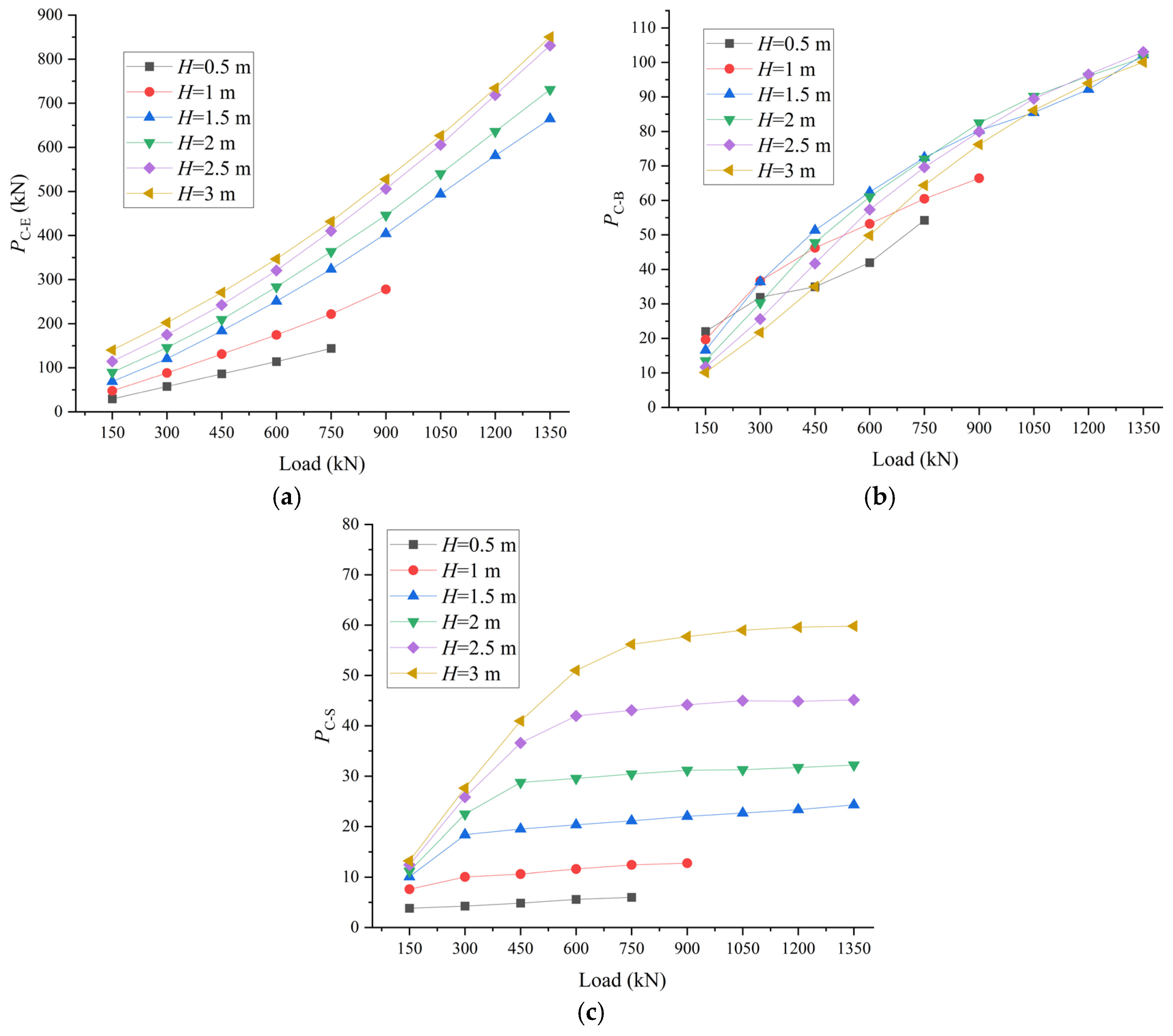
4.1. The Embedment Depth of the Cap
4.1.1. The Load–Displacement Curve
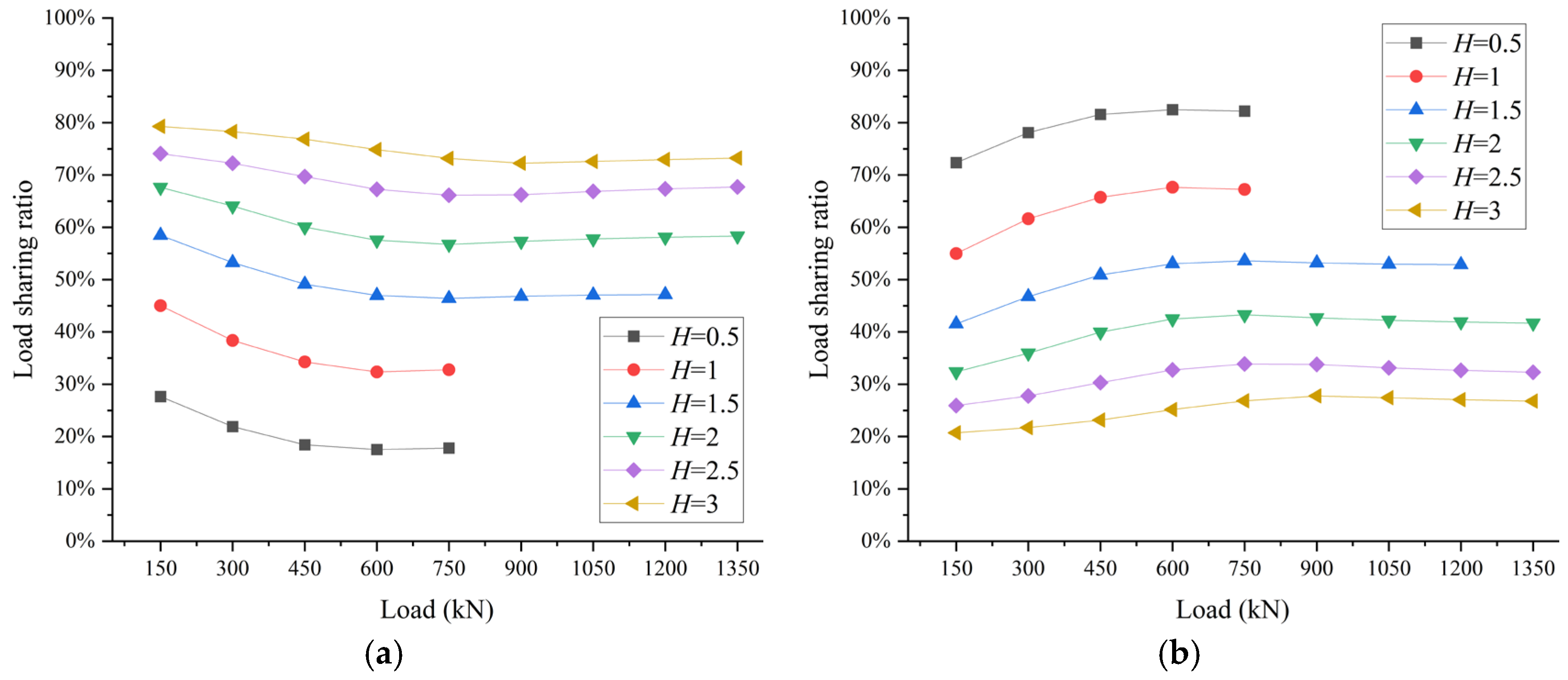
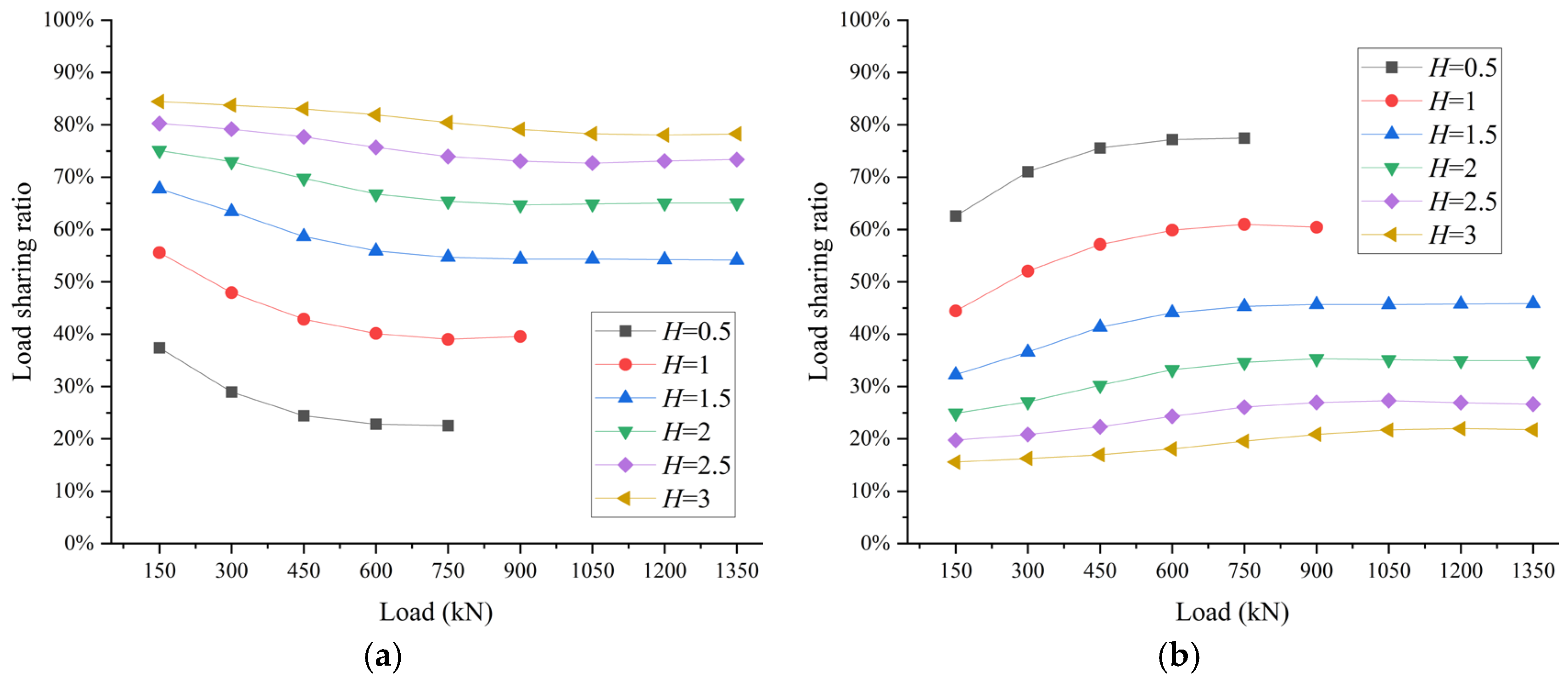
4.1.2. Load Sharing on the Pile Cap and Piles
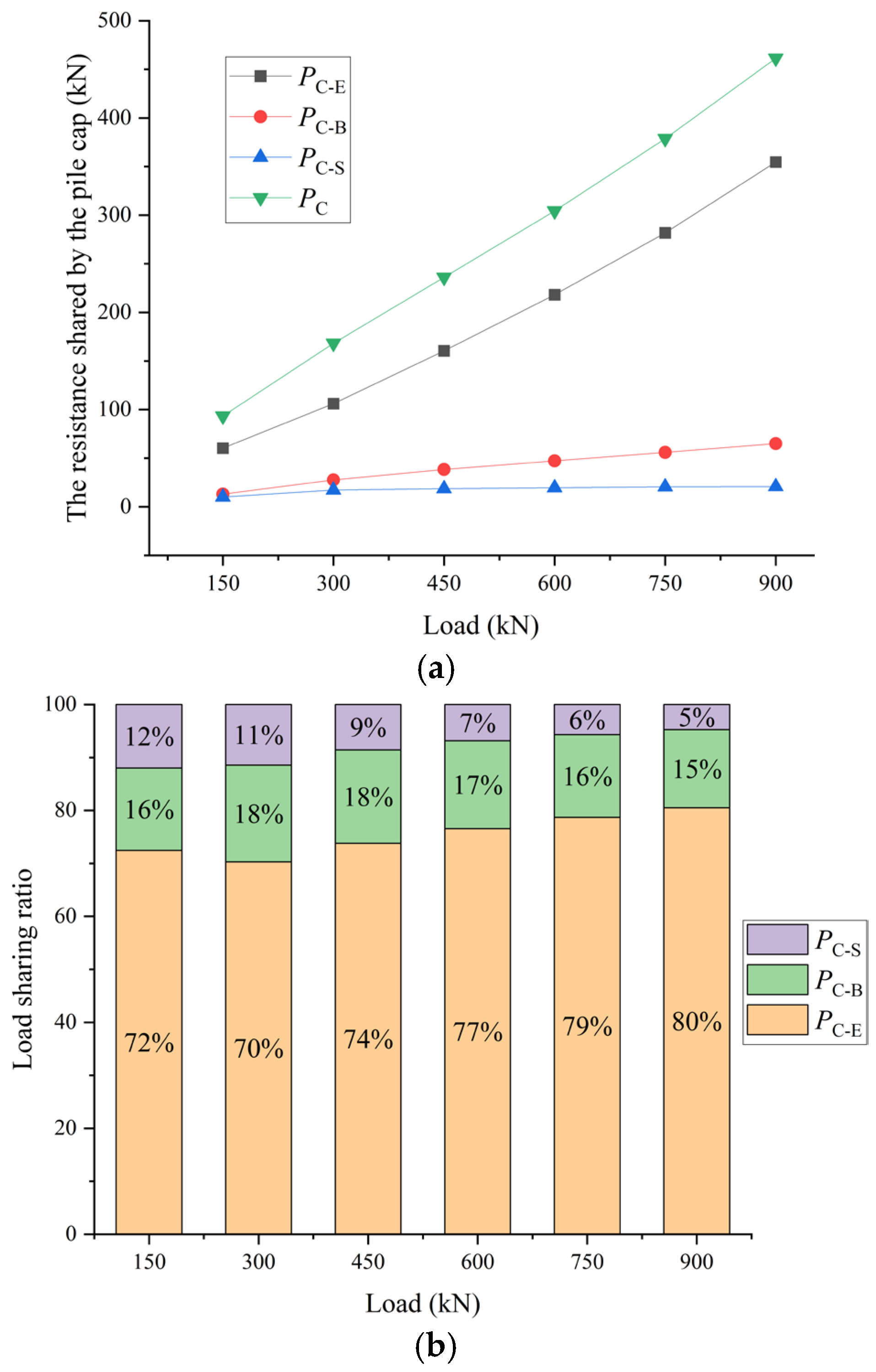
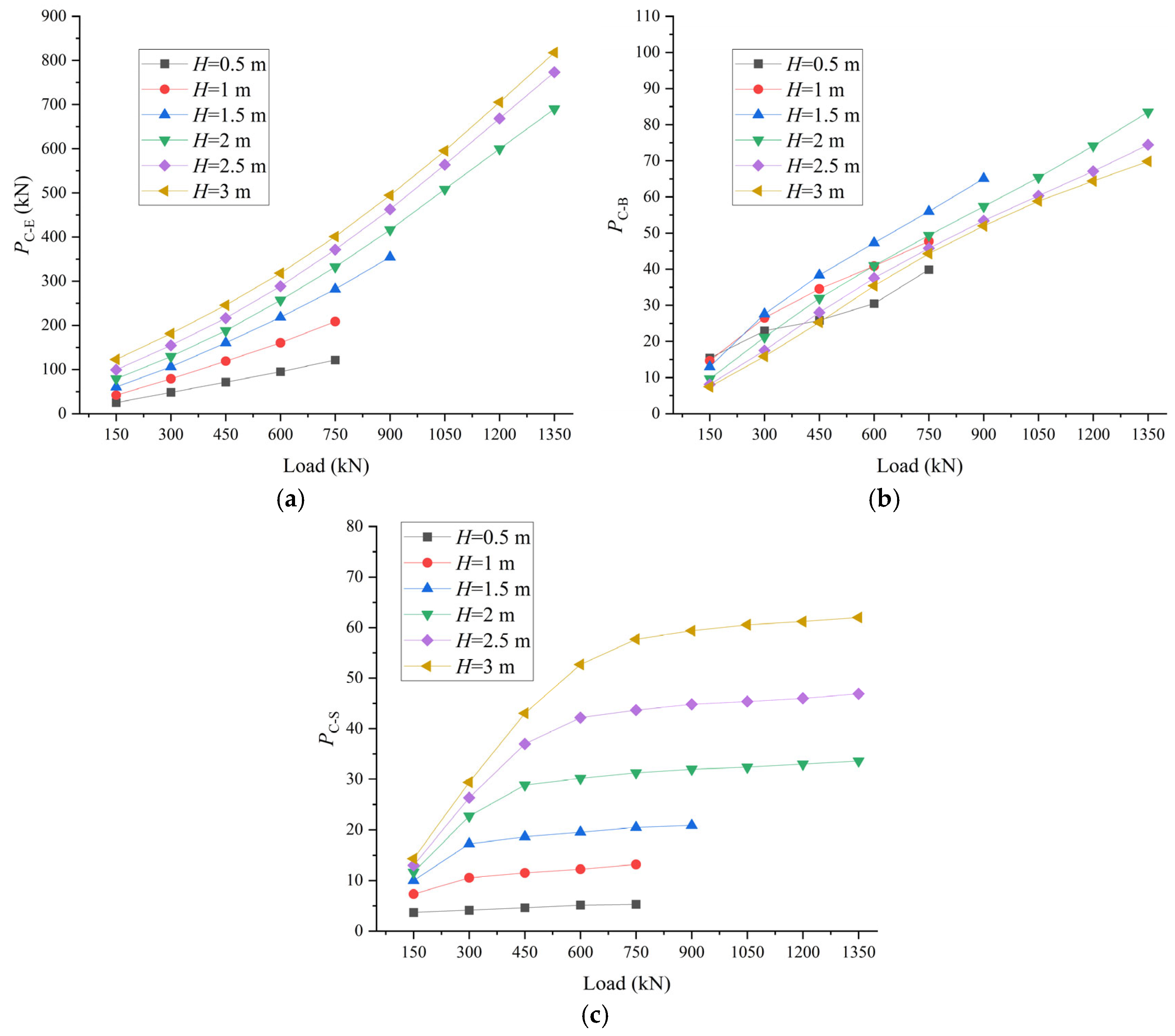
4.1.3. Load Sharing Among Different Parts of the Pile Cap
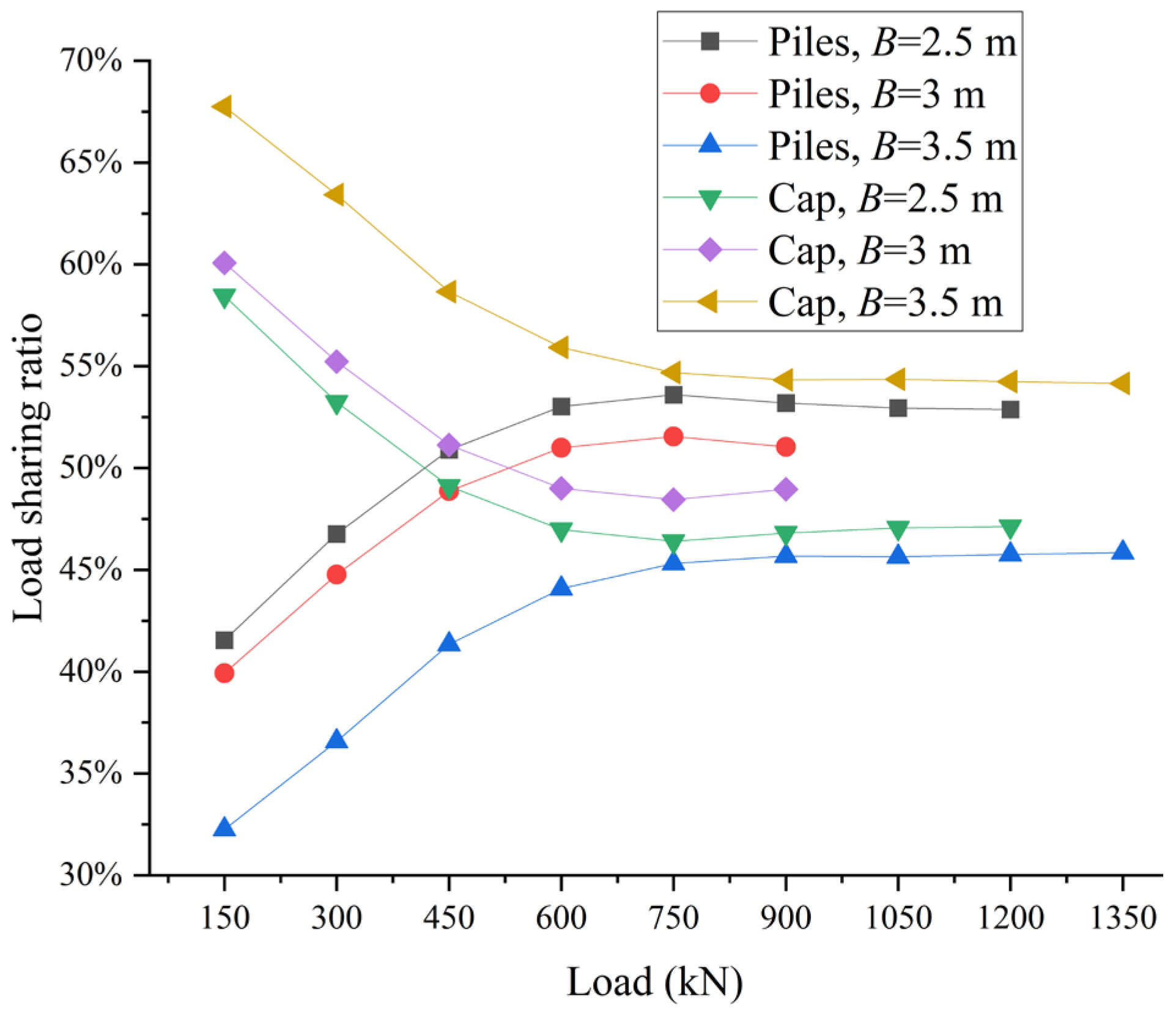
4.2. The Width of the Cap
4.2.1. The Load–Displacement Curve
4.2.2. Load Sharing on the Pile Cap and Piles
4.2.3. Load Sharing Among Different Parts of the Pile Cap
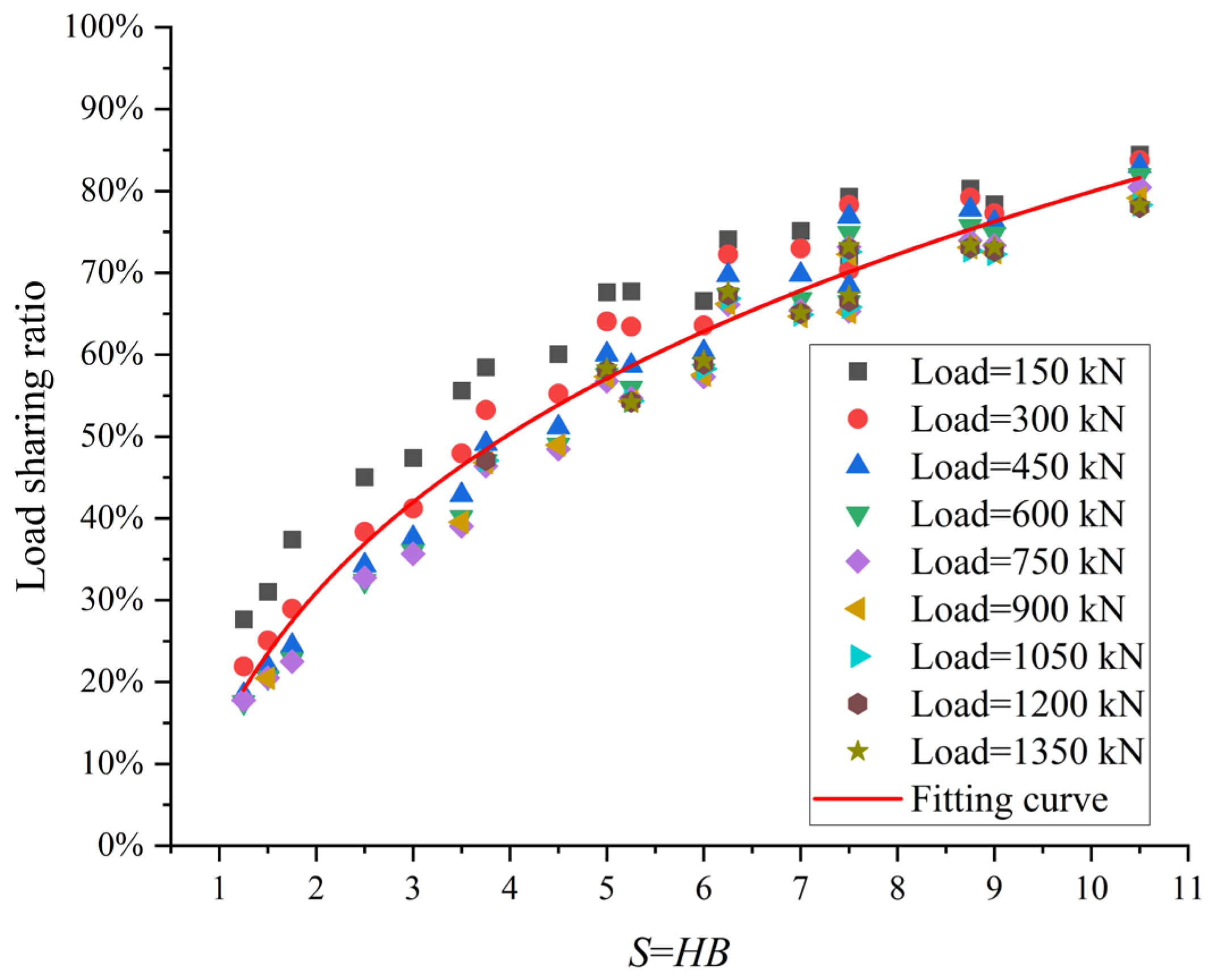
4.3. The Relationship Between the Load-Sharing Ratio and HB
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ates, B.; Şadoğlu, E. Experimental investigation of optimum piles spacing for piled raft foundation in sandy soils. Tek. Dergi 2021, 32, 10477–10494. [Google Scholar] [CrossRef]
- Basha, A.; Elmorsy, S.; Mansour, W.; Ramadan, B. Effect of the pile cap length and the soil relative density on the pile cap–pile–dense soil interaction: Experimental investigation. Case Stud. Constr. Mater. 2024, 20, e03169. [Google Scholar] [CrossRef]
- Mostafa, Y.E.; Naggar, M.H.E. Dynamic analysis of laterally loaded pile groups in sand and clay. Can. Geotech. J. 2002, 39, 1358–1383. [Google Scholar] [CrossRef]
- Munawir, A.; Harimurti; Sumarsono, Q.A.R.P. Lateral Load Capacity and p-Multiplier of Group Piles with Asymmetrical Pile Cap under Seismic Load. Appl. Sci. 2022, 12, 8142. [Google Scholar] [CrossRef]
- Chen, Z.; Deng, H.; Dai, G.; Zhu, M.; Gong, W.; Azadi, M.R. Study on the influence of unsymmetrical surcharge on adjacent pile foundations in a coastal soft soil area. Soil Dyn. Earthq. Eng. 2025, 194, 109365. [Google Scholar] [CrossRef]
- Unsever, Y.S.; Matsumoto, T.; Özkan, M.Y. Numerical analyses of load tests on model foundations in dry sand. Comput. Geotech. 2015, 63, 255–266. [Google Scholar] [CrossRef]
- Deb, P.; Pal, S.K. Numerical analysis of piled raft foundation under combined vertical and lateral loading. Ocean Eng. 2019, 190, 106431. [Google Scholar] [CrossRef]
- Ateş, B.; Şadoğlu, E. Experimental investigation for group efficiency of driven piles embedded in cohesionless soil. KSCE J. Civ. Eng. 2023, 27, 5123–5134. [Google Scholar] [CrossRef]
- Bandyopadhyay, P.; Teng, F. Analysis of pile-soil-excavation interaction and load transfer mechanism in multi-layered soil for an in-service pile group. Comput. Geotech. 2024, 171, 106378. [Google Scholar] [CrossRef]
- Wu, T.; Gao, Y.; Huang, C.; Zhou, Y.; Li, J. Mechanical Behavior of Single and Group Piles with a Low Cap Adjacent to Shield Tunneling in Composite Ground: Insights from Centrifugal Model Testing. Geotech. Geol. Eng. 2024, 42, 7451–7480. [Google Scholar] [CrossRef]
- Ateş, B.; Şadoğlu, E. Experimental investigation of pile addition and length on bearing capacity and settlement of rafts on loose sandy soil. Afyon Kocatepe Üniv. Fen Ve Mühendis. Bilim. Derg. 2021, 21, 399–407. [Google Scholar]
- Jeong, S.-S.; Won, J.-O.; Kim, Y.-H. Effect of pile cap flexibility on the response of pile group supported column. J. Korean Geotech. Soc. 2007, 23, 39–49. [Google Scholar] [CrossRef]
- Ashour, M.; Abbas, A.I.; Boskovic, S. Pile Cap Interaction with Bridge Pile Foundations under Lateral Loads. J. Bridge Eng. 2019, 24, 04019053. [Google Scholar] [CrossRef]
- Varghese, R.; Boominathan, A.; Banerjee, S. Stiffness and load sharing characteristics of piled raft foundations subjected to dynamic loads. Soil Dyn. Earthq. Eng. 2020, 133, 106117. [Google Scholar] [CrossRef]
- Zhong, C.; Chen, Z.; Zhou, J. Numerical Investigations of Pile Group Foundations under Different Pile Length Conditions. Appl. Sci. 2024, 14, 1908. [Google Scholar] [CrossRef]
- Qiu, H.; Wang, H.; Ayasrah, M.M.; Zhou, Z.; Li, B. Study on Horizontal Bearing Capacity of Pile Group Foundation Composed of Inclined and Straight Piles. Buildings 2023, 13, 690. [Google Scholar] [CrossRef]
- Jamil, I.; Ahmad, I.; Rehman, A.U.; Siddiqi, M.I.; Ahmed, A.; Khan, A.M. Piles’ load distribution in pile raft and pile group under lateral loading. Mar. Georesour. Geotechnol. 2024, 42, 1034–1049. [Google Scholar] [CrossRef]
- Won, J.; Ahn, S.Y.; Jeong, S.; Lee, J.; Jang, S.Y. Nonlinear three-dimensional analysis of pile group supported columns considering pile cap flexibility. Comput. Geotech. 2006, 33, 355–370. [Google Scholar] [CrossRef]
- Bessimbayev, Y.; Niyetbay, S.; Zhambakina, Z.; Enayat, T. Exploring the Influence of Pile Cap Thickness on the Effectiveness of Pile Cap Foundations through Numerical Analysis. Archit. Civ. Eng. 2025, 2, 22–27. [Google Scholar] [CrossRef]
- Pilling, P.A. The Response of a Group of Flexible Piles and the Associated Pile Cap to Lateral Loading as Characterized by the Strain Wedge Model. Ph.D. Thesis, University of Nevada, Reno, NV, USA, 1997. [Google Scholar]
- Nguyen, N.V.; Vinh, L.B.; Vo, T.-T. Load-sharing mechanism of piled-raft foundation: A numerical study. Eur. J. Environ. Civ. Eng. 2022, 26, 7916–7931. [Google Scholar] [CrossRef]
- Siddiqi, M.I.; Jamil, I.; Hussain, M.A. Lateral Load Analysis of Piled Raft Foundation: A Review. Tech. J. 2024, 3, 584–590. [Google Scholar]
- Swasdi, S.; Chub-Uppakarn, T.; Chompoorat, T.; Sae-Long, W. Numerical study on the influence of embedment footing and vertical load on lateral load sharing in piled raft foundations. Geomech. Eng. 2024, 36, 545–561. [Google Scholar] [CrossRef]
- Espinoza, J.P.; Tamayo, J.P. Numerical simulation and parametric study of pile groups under lateral loads. J. Braz. Soc. Mech. Sci. Eng. 2023, 45, 364. [Google Scholar] [CrossRef]
- McVay, M.C.; Zhang, L.; Han, S.; Lai, P. Experimental and numerical study of laterally loaded pile groups with pile caps at variable elevations. Transp. Res. Rec. 2000, 1736, 12–18. [Google Scholar] [CrossRef]
- Mokwa, R.L.; Duncan, J.M. Experimental evaluation of lateral-load resistance of pile caps. J. Geotech. Geoenviron. Eng. 2001, 127, 185–192. [Google Scholar] [CrossRef]
- Jamil, I.; Ahmad, I.; Ullah, W. Contribution of raft to resist lateral loads in a piled raft foundation-Experimental findings. Earthq. Struct. 2021, 21, 275–286. [Google Scholar] [CrossRef]
- Malviya, D.K.; Samanta, M. Lateral load sharing and response of piled raft foundation in cohesionless medium: An experimental approach. Geomech. Eng. 2024, 38, 139–155. [Google Scholar] [CrossRef]
- Rollins, K.M.; Sparks, A. Lateral Resistance of Full-Scale Pile Cap with Gravel Backfill. J. Geotech. Geoenviron. Eng. 2002, 128, 711–723. [Google Scholar] [CrossRef]
- Rollins, K.M.; Lane, J.D.; Gerber, T.M. Measured and computed lateral response of a pile group in sand. J. Geotech. Geoenviron. Eng. 2005, 131, 103–114. [Google Scholar] [CrossRef]
- Dong, J.; Chen, F.; Zhou, M.; Zhou, X. Numerical analysis of the boundary effect in model tests for single pile under lateral load. Bull. Eng. Geol. Environ. 2018, 77, 1057–1068. [Google Scholar] [CrossRef]
- Jones, K.; Sun, M.; Lin, C. Numerical analysis of group effects of a large pile group under lateral loading. Comput. Geotech. 2022, 144, 104660. [Google Scholar] [CrossRef]
- Alzabeebee, S. A comparative study of the effect of the soil constitutive model on the seismic response of buried concrete pipes. J. Pipeline Sci. Eng. 2022, 2, 87–96. [Google Scholar] [CrossRef]
- Bowles, J.E.; Guo, Y. Foundation Analysis and Design; McGraw-Hill: New York, NY, USA, 1996; Volume 5. [Google Scholar]
- Ateş, B.; Şadoğlu, E. Experimental and Numerical Investigation of Single Pile Subjected to Vertical Load in Sand. In Proceedings of the 3rd International Conference on Advanced Engineering Technologies, Bayburt, Turkey, 19–21 September 2019. [Google Scholar]
- Liu, B.; Wang, X.; Liu, C.; Kong, J. Effect of Relative Stiffness of Pile and Soil on Pile Group Effect. J. Mar. Sci. Eng. 2023, 11, 192. [Google Scholar] [CrossRef]
- Tschuchnigg, F.; Schweiger, H.F. The embedded pile concept—Verification of an efficient tool for modelling complex deep foundations. Comput. Geotech. 2015, 63, 244–254. [Google Scholar] [CrossRef]
- Ghiasi, V.; Eskandari, S. Comparing a single pile’s axial bearing capacity using numerical modeling and analytical techniques. Results Eng. 2023, 17, 100893. [Google Scholar] [CrossRef]
- Bahri, M.A.; Arabani, M. Effect of geometric characteristics of helical piles on the lateral displacement in sandy slopes by loading surcharge. Results Eng. 2025, 25, 103896. [Google Scholar] [CrossRef]


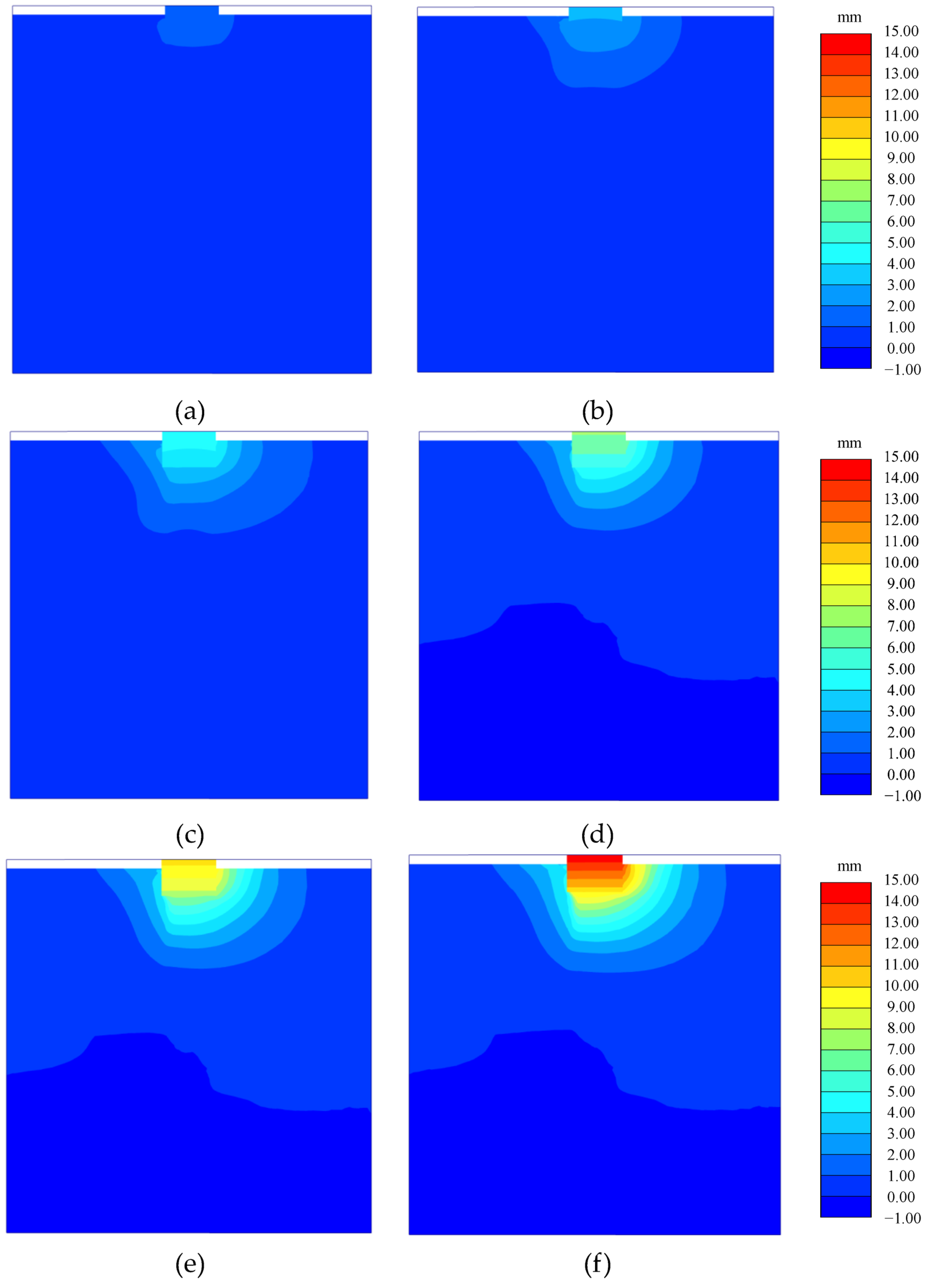





| Depth Below the Ground | Type | Unit (kN/m3) | Cohesion (kPa) | Friction Angle (°) | SPT N | CPT qc (MPa) | |
|---|---|---|---|---|---|---|---|
| Top/(m) | Bottom/(m) | ||||||
| 0.00 | 0.10 | Sand | 19.5 | 0 | 39 | 10 | |
| 0.10 | 2.97 | Sand | 11.1 | 0 | 39 | 10 | |
| 2.97 | 3.99 | Sand | 11.1 | 0 | 37 | 10 | |
| 3.99 | 6.00 | Sand | 11.1 | 0 | 36 | 7 | |
| 6.00 | 7.49 | Sand | 11.1 | 0 | 35 | 7 | |
| 7.49 | 9.20 | Soft Clay | 9.5 | 19.2 | 0 | 2 | |
| 9.20 | 12.73 | Sand | 11.1 | 0 | 32 | 3.5 | |
| 12.73 | 14.51 | Soft Clay | 9.5 | 19.2 | 0 | 2 | |
| Type | (kN/m3) | (MPa) | (MPa) | (MPa) | (MPa) | m | (°) | |
|---|---|---|---|---|---|---|---|---|
| Sand | 19.5 | 35 | 35 | 105 | 0.2 | 0.5 | 100 | 9 |
| Sand | 11.1 | 35 | 35 | 105 | 0.2 | 0.5 | 100 | 9 |
| Sand | 11.1 | 35 | 35 | 105 | 0.2 | 0.5 | 100 | 7 |
| Sand | 11.1 | 30 | 30 | 90 | 0.2 | 0.5 | 100 | 6 |
| Sand | 11.1 | 30 | 30 | 90 | 0.2 | 0.5 | 100 | 5 |
| Soft Clay | 9.5 | 16 | 16 | 80 | 0.2 | 0.8 | 100 | 0 |
| Sand | 11.1 | 19 | 19 | 57 | 0.2 | 0.5 | 100 | 2 |
| Soft Clay | 9.5 | 16 | 16 | 80 | 0.2 | 0.8 | 100 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ren, Y.; Chen, Z.; Zhu, W. A Numerical Study of the Lateral Load-Sharing Mechanism of the Pile Cap in a 3 × 3 Pile Group. Buildings 2025, 15, 1431. https://doi.org/10.3390/buildings15091431
Ren Y, Chen Z, Zhu W. A Numerical Study of the Lateral Load-Sharing Mechanism of the Pile Cap in a 3 × 3 Pile Group. Buildings. 2025; 15(9):1431. https://doi.org/10.3390/buildings15091431
Chicago/Turabian StyleRen, Yuanyuan, Zhiwei Chen, and Wenbo Zhu. 2025. "A Numerical Study of the Lateral Load-Sharing Mechanism of the Pile Cap in a 3 × 3 Pile Group" Buildings 15, no. 9: 1431. https://doi.org/10.3390/buildings15091431
APA StyleRen, Y., Chen, Z., & Zhu, W. (2025). A Numerical Study of the Lateral Load-Sharing Mechanism of the Pile Cap in a 3 × 3 Pile Group. Buildings, 15(9), 1431. https://doi.org/10.3390/buildings15091431






