Robust Stabilization of Underactuated TORA System Based on Disturbance Observer and Fixed-Time Sliding Mode Control Method
Abstract
:1. Introduction
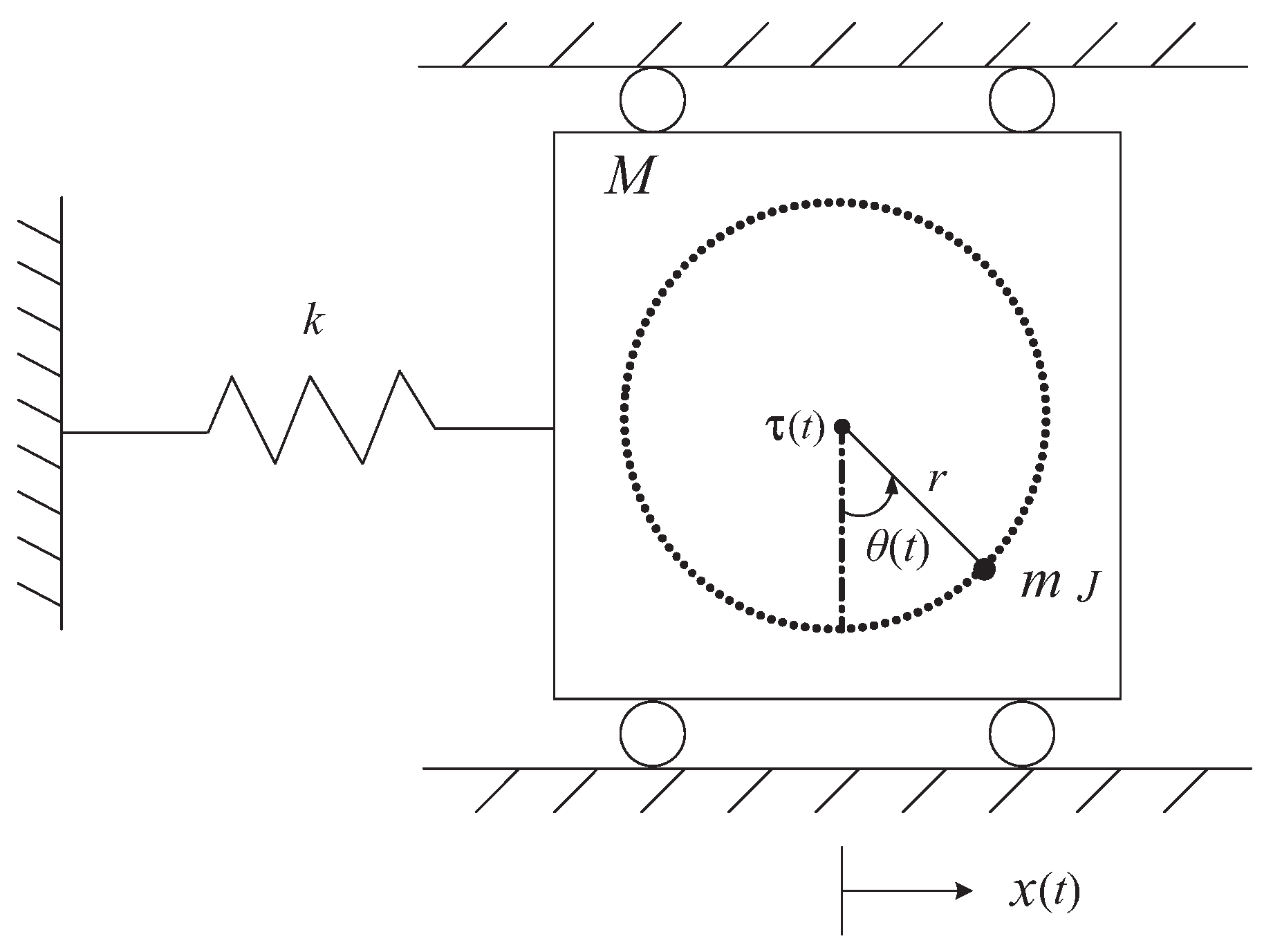
2. Dynamic Motion Equations of TORA
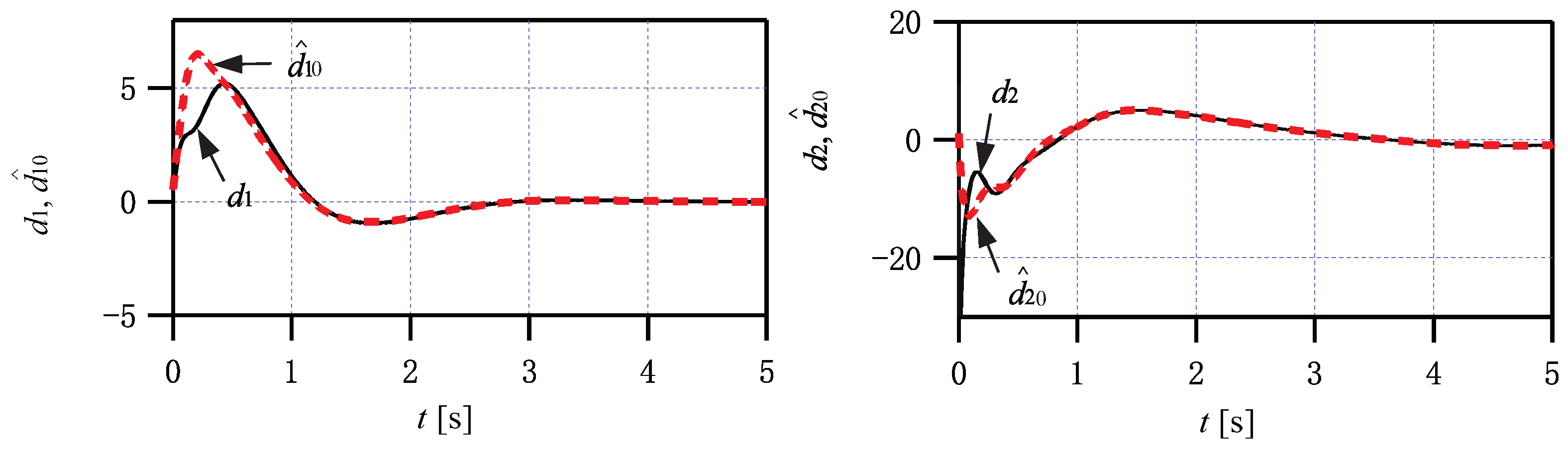
3. Design of a Disturbance Observer
4. Design of Robust Stabilization Controller
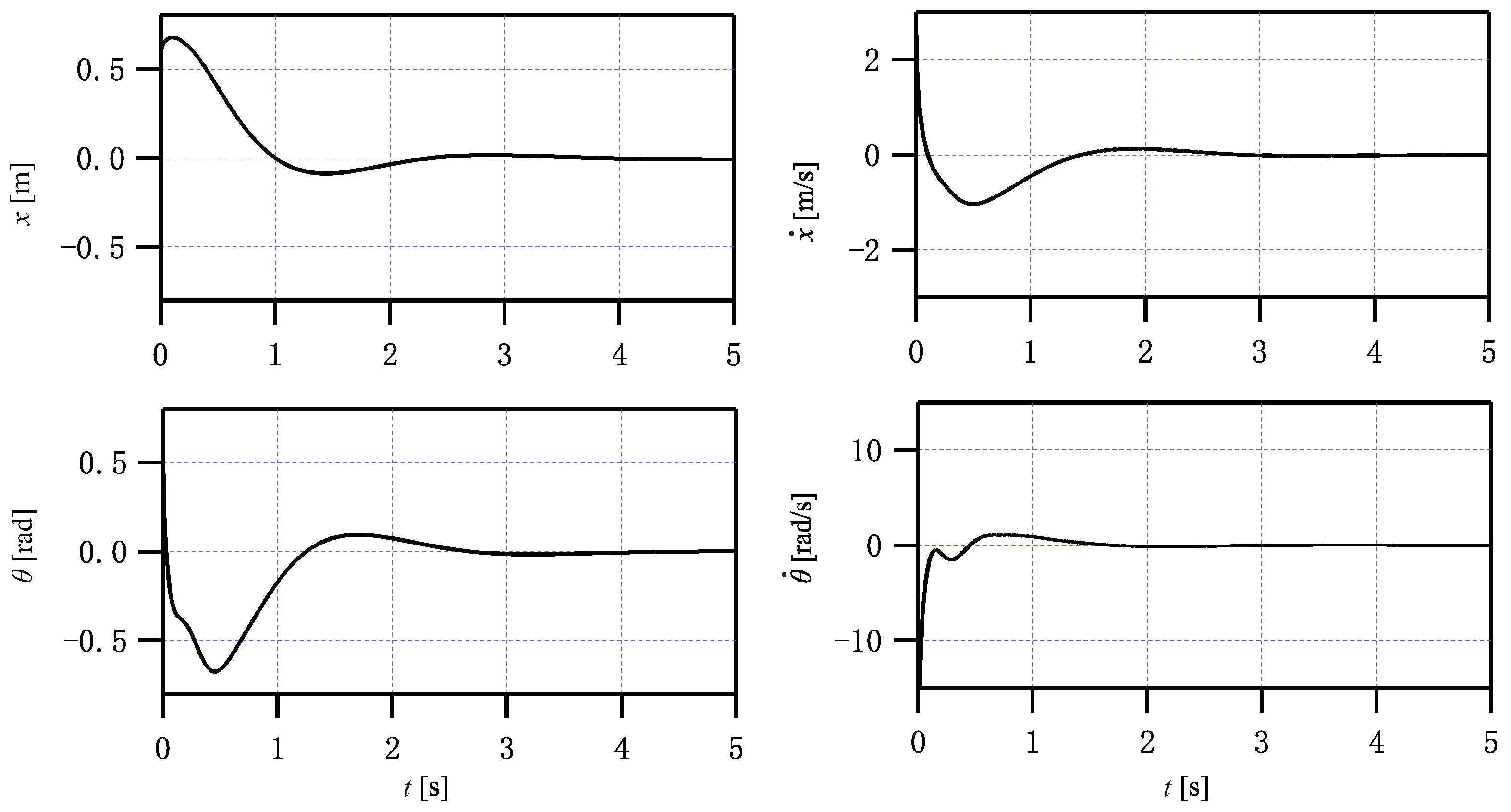
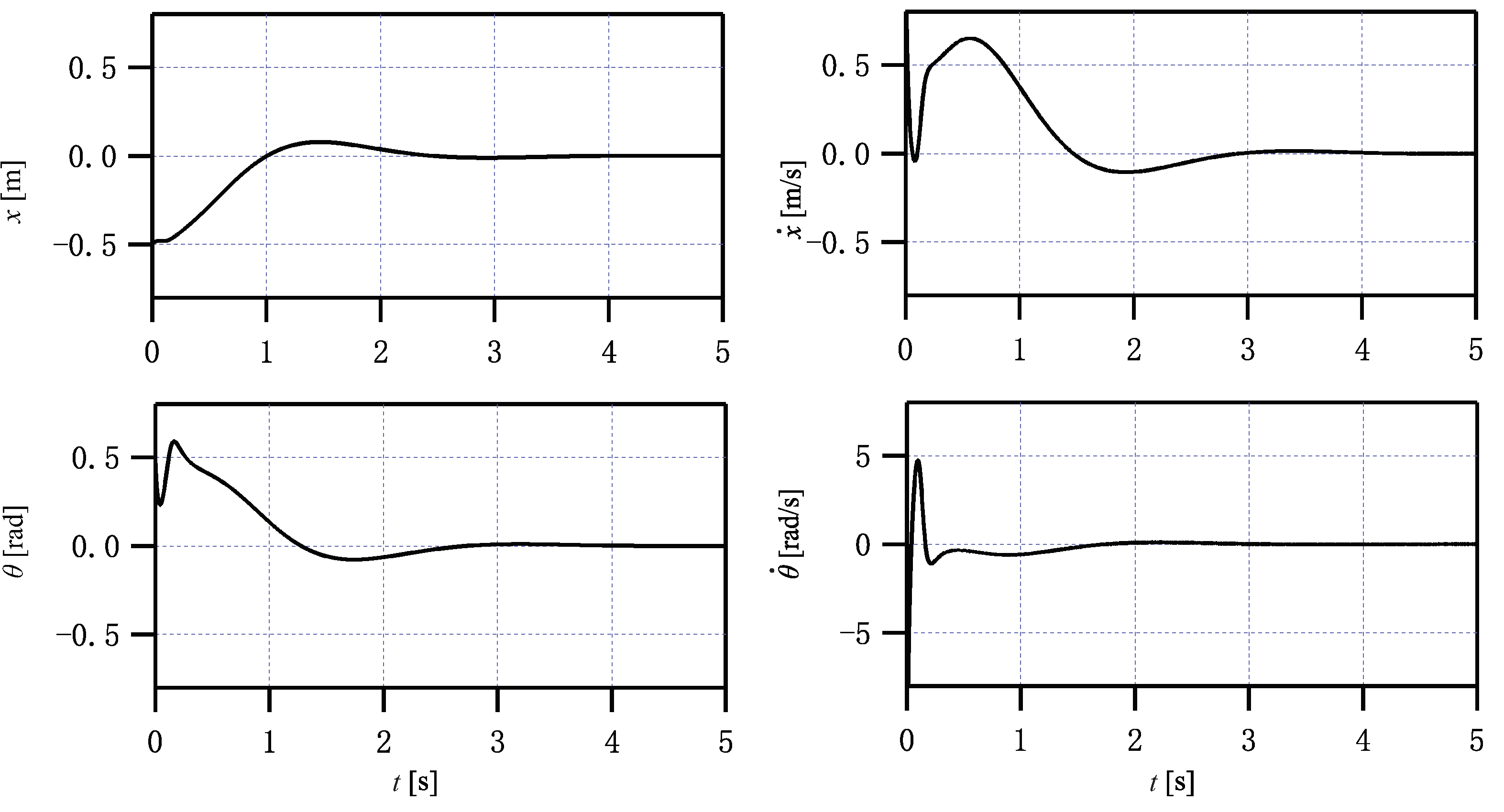
5. Numerical Examples
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rand, R.H.; Kinsey, R.J.; Mingori, D.L. Dynamics of spinup through resonance. Int. J. Nonlin. Mech. 1992, 27, 489–502. [Google Scholar] [CrossRef]
- Avis, J.M.; Nersesov, S.G.; Nathan, R.; Ashrafiuon, H.; Muske, K.R. A comparison study of nonlinear control techniques for the rtac system. Nolinear Anal. -Real. 2010, 11, 2647–2658. [Google Scholar] [CrossRef]
- Sa’ Id, W.; Al-Samarraie, S.; Mshari, M. Simple flatness condition for 2-DOF underactuated mechanical systems with application to controller design. Math. Model. Eng. Probl. 2020, 7, 119–126. [Google Scholar] [CrossRef]
- Zhao, G.; Li, H.; Song, Z. Adaptive dynamic fuzzy neural network-based decoupled sliding-mode controller with hybrid sliding surfaces. Int. J. Autom. Control 2013, 7, 183–201. [Google Scholar] [CrossRef]
- Tadmor, G. Dissipative design, lossless dynamics, and the nonlinear TORA benchmark example. IEEE Trans. Control. Syst. Technol. 2001, 9, 391–398. [Google Scholar] [CrossRef]
- Jankovic, M.; Fontaine, D.; Kokotovic, P.V. TORA example: Cascade and passivity-based control designs. IEEE Trans. Control. Syst. Technol. 1996, 4, 292–297. [Google Scholar] [CrossRef]
- Liu, Y.; Yu, H. A survey of underactuated mechanical systems. IET Control. Theory Appl. 2013, 7, 921–935. [Google Scholar] [CrossRef]
- Gao, B.; Xu, J.; Zhao, J.; Huang, X. Stabilizing control of an underactuated 2-dimensional TORA with only rotor angle measurement. Asian J. Control. 2013, 15, 1477–1488. [Google Scholar] [CrossRef]
- Gutiérrez-Oribio, D.; Mercado-Uribe, J.A.; Moreno, J.A.; Fridman, L. Robust global stabilization of a class of underactuated mechanical systems of two degrees of freedom. Int. J. Robust. Nonlin. 2021, 31, 3908–3928. [Google Scholar] [CrossRef]
- Sun, N.; Wu, Y.; Fang, Y.; Chen, H.; Lu, B. Nonlinear continuous global stabilization control for underactuated RTAC systems: Design, analysis, and experimentation. IEEE-Asme T. Mech. 2016, 22, 1104–1115. [Google Scholar] [CrossRef]
- Feng, Q.; Zhang, A.; Li, N. Design of stabilizing controllers for underactuated TORA system. In Proceedings of the 2021 China Automation Congress (CAC), Beijing, China, 22–24 October 2021; pp. 3323–3327. [Google Scholar]
- Liu, D.; Guo, W. Nonlinear backstepping design for the underactuated TORA system. J. Vibroeng. 2014, 16, 552–559. [Google Scholar]
- Alleyne, A. Physical insights on passivity-based TORA control designs. IEEE Trans. Control. Syst. Technol. 1998, 6, 436–439. [Google Scholar] [CrossRef]
- Xu, R.; Özgüner, Ü. Sliding mode control of a class of underactuated systems. Automatica 2008, 44, 233–241. [Google Scholar] [CrossRef]
- Wu, T.; Gui, W.; Hu, D.; Du, C. Adaptive fuzzy sliding mode control for translational oscillator with rotating actuator: A fuzzy model. IEEE Access 2018, 6, 55861–55869. [Google Scholar] [CrossRef]
- Gao, B.; Ye, F. Fuzzy Lyapunov synthesis control of an underactuated 2D TORA system. J. Intell. Fuzzy Syst. 2015, 28, 581–589. [Google Scholar] [CrossRef]
- She, J.; Zhang, A.; Lai, X.; Wu, M. Global stabilization of 2—DOF underactuated mechanical systems an equivalent-input-disturbance approach. Nonlinear Dynam. 2012, 69, 495–509. [Google Scholar] [CrossRef]
- Sun, N.; Wu, Y.; Fang, Y.; Chen, H. Nonlinear stabilization control of multipleRTAC systems subject to amplitude-restricted actuating torques using only angular position feedback. IEEE Trans. Ind. Electron. 2017, 64, 3084–3094. [Google Scholar] [CrossRef]
- Wu, Y.; Sun, N.; Fang, Y.; Liang, D. An increased nonlinear coupling motion controller for underactuated multi—TORA systems: Theoretical design and hardware experimentation. IEEE Trans. Syst. Man Cybern. Part B 2017, 49, 1186–1193. [Google Scholar] [CrossRef]
- Tavakoli, M.; Taghirad, H.D.; Abrishamchian, M. Identification and robust H∞control of the rotational/translational actuator system. Int. J. Control Autom. 2005, 3, 387–396. [Google Scholar]
- Wu, X.; Zhao, Y.; Xu, K. Nonlinear disturbance observer based sliding mode control for a benchmark system with uncertain disturbances. ISA Trans. 2020, 110, 63–70. [Google Scholar] [CrossRef]
- Huang, J.; Ri, S.; Fukuda, T.; Wang, Y. A disturbance observer based sliding mode control for a class of underactuated robotic system with mismatched uncertainties. IEEE Trans. Autom. Control 2019, 64, 2480–2487. [Google Scholar] [CrossRef]
- Li, S.; Sun, H.; Yang, J.; Yu, X. Continuous finite-time output regulation for disturbed systems under mismatching condition. IEEE Trans. Autom. Control 2015, 60, 277–282. [Google Scholar] [CrossRef]
- Parsegov, S.E.; Polyakov, A.E.; Shcherbakov, P.S. Fixed-time consensus algorithm for multi-agent systems with integrator dynamics. IFAC J. Syst. Control 2013, 46, 110–115. [Google Scholar] [CrossRef] [Green Version]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Feng, Q.; Zhang, A.; Zhang, X.; Pang, G.; Liu, Z. Robust Stabilization of Underactuated TORA System Based on Disturbance Observer and Fixed-Time Sliding Mode Control Method. Actuators 2022, 11, 271. https://doi.org/10.3390/act11100271
Feng Q, Zhang A, Zhang X, Pang G, Liu Z. Robust Stabilization of Underactuated TORA System Based on Disturbance Observer and Fixed-Time Sliding Mode Control Method. Actuators. 2022; 11(10):271. https://doi.org/10.3390/act11100271
Chicago/Turabian StyleFeng, Qixuan, Ancai Zhang, Xinghui Zhang, Guocheng Pang, and Zhi Liu. 2022. "Robust Stabilization of Underactuated TORA System Based on Disturbance Observer and Fixed-Time Sliding Mode Control Method" Actuators 11, no. 10: 271. https://doi.org/10.3390/act11100271
APA StyleFeng, Q., Zhang, A., Zhang, X., Pang, G., & Liu, Z. (2022). Robust Stabilization of Underactuated TORA System Based on Disturbance Observer and Fixed-Time Sliding Mode Control Method. Actuators, 11(10), 271. https://doi.org/10.3390/act11100271