Development of Air Bearing Stage Using Flexure for Yaw Motion Compensation
Abstract
:1. Introduction
2. Design of Air Bearing Stage
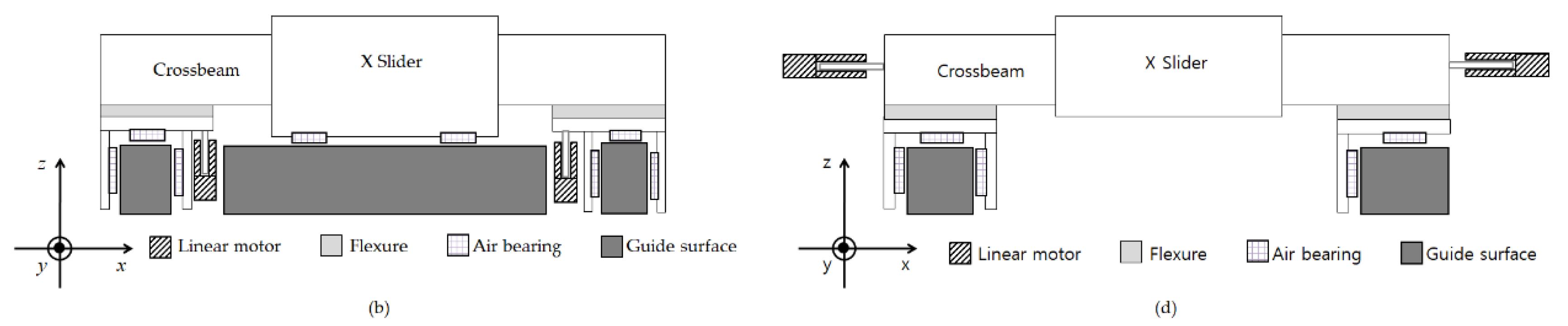
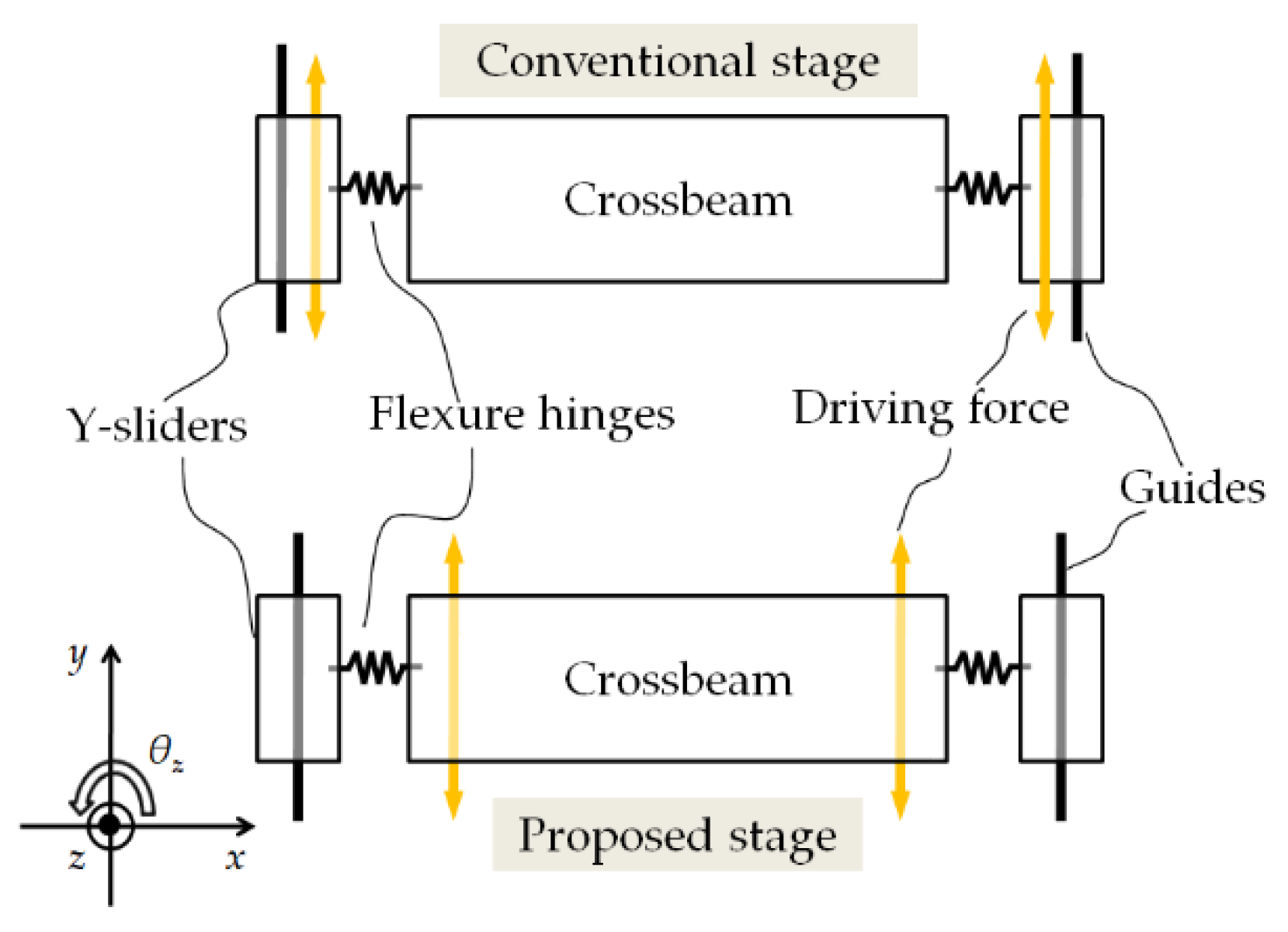
2.1. Stacked Gantry Structure
2.2. Air Bearings and Flexure
2.3. Linear Motor and Flexure
3. Design of Flexure
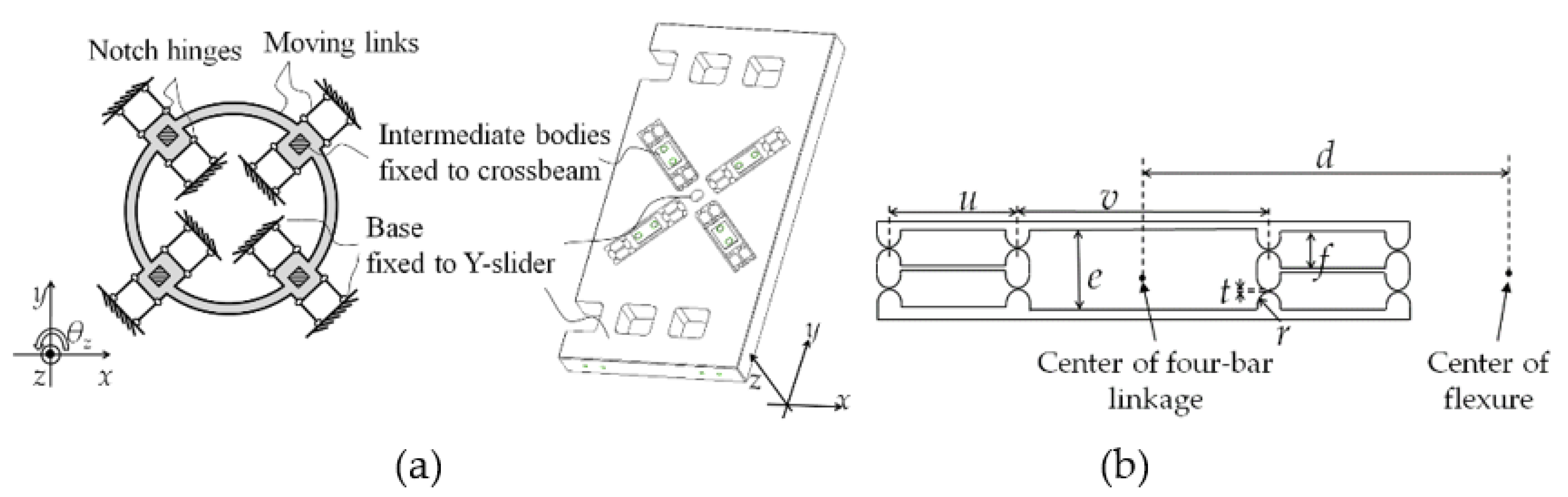
3.1. Conceptual Design
3.2. Mathematical Modeling
3.3. Optimization
4. Implementation of Stage
4.1. Manufacturing
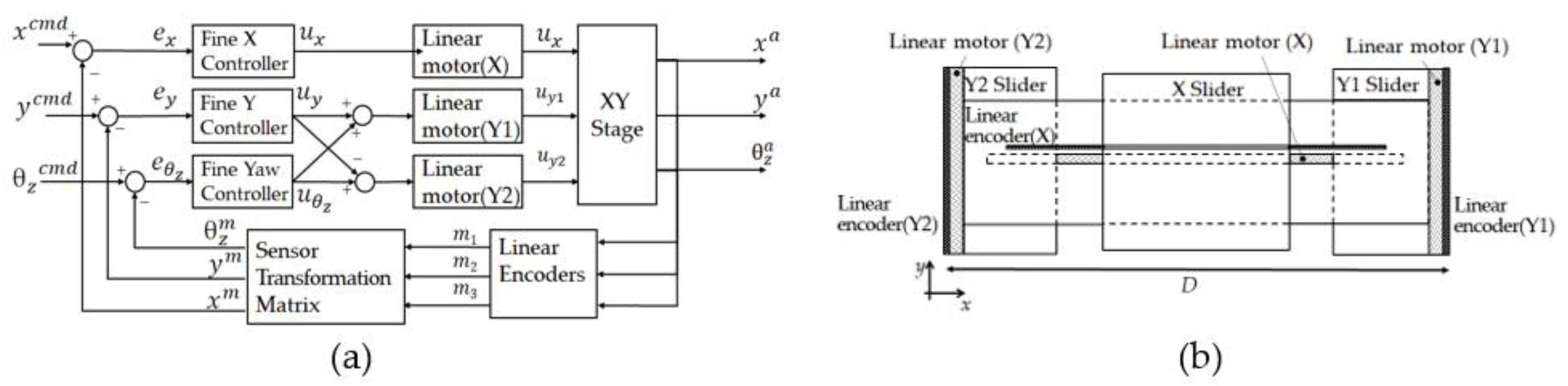
4.2. Experimental Setup
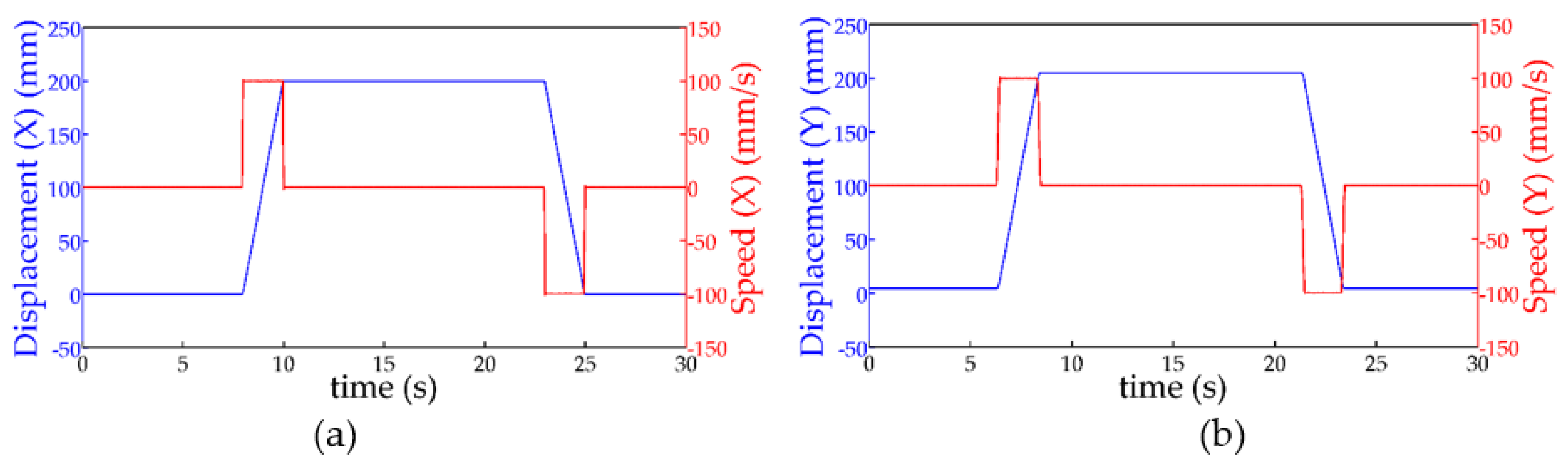
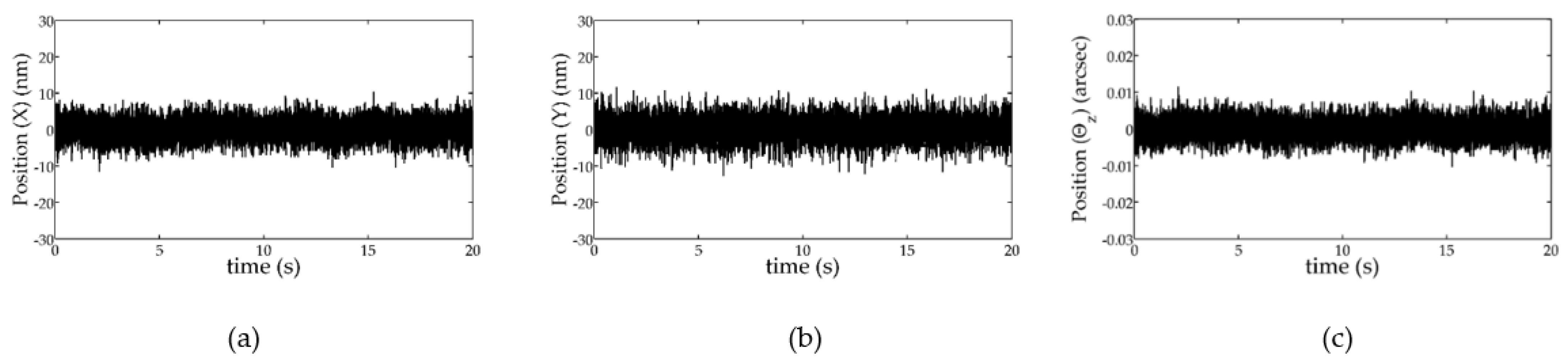
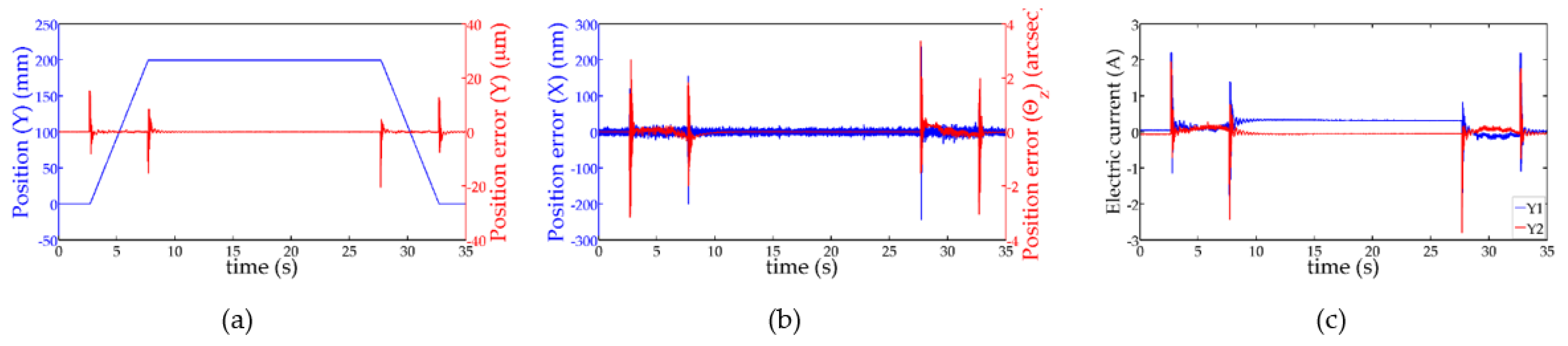
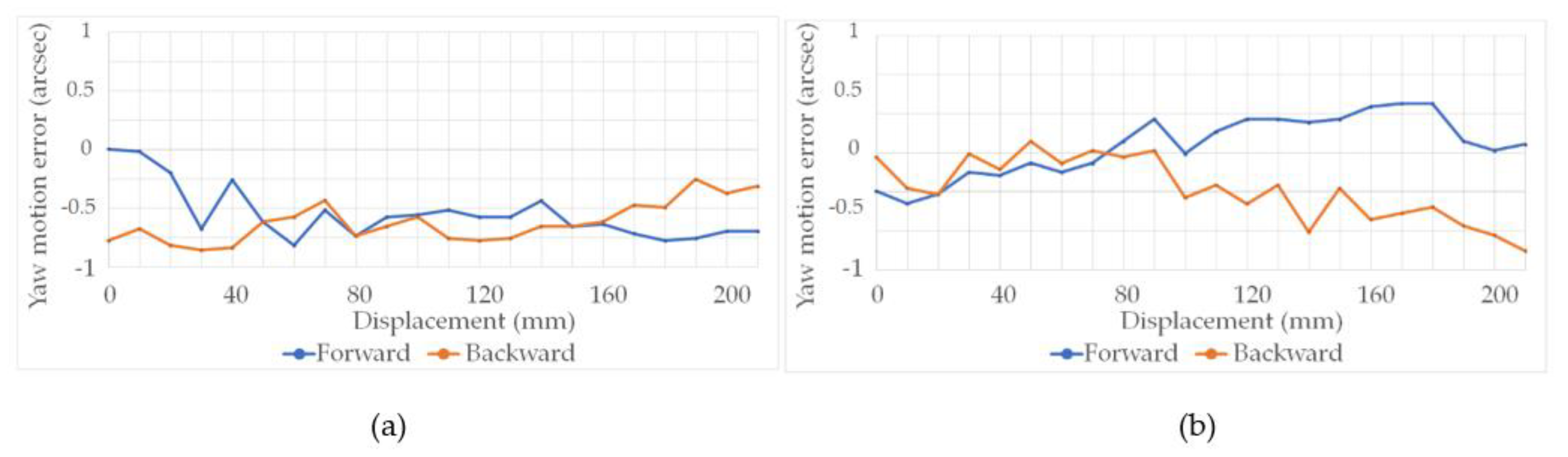
4.3. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Li, Y.T.; Ding, H. Design analysis and experimental study of aerostatic linear guideways used in a high acceleration and high precision xy stage. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2007, 221, 1–9. [Google Scholar] [CrossRef]
- Bin, Z.; Zhaoyao, S.; Jiachun, L.; Hua, Z. Design for H type co-planar precision stage based on closed air bearing guideway with vacuum attraction force. In Proceedings of the Seventh International Symposium on Precision Engineering Measurements and Instrumentation, Yunnan, China, 7–11 August 2011; p. 83211B. [Google Scholar]
- Shinno, H.; Hashizume, H.; Yoshioka, H.; Komatsu, K.; Shinshi, T.; Sato, K. X-Y-Θ Nano-Positioning Table System for a Mother Machine. CIRP Ann.-Manuf. Technol. 2004, 53, 337–340. [Google Scholar] [CrossRef]
- Choi, Y.M.; Kim, J.J.; Kim, J.; Gweon, D.-G. Design and control of a nanoprecision XYΘ scanner. Rev. Sci. Instrum. 2008, 79, 045109. [Google Scholar] [CrossRef] [PubMed]
- Kim, K.; Choi, Y.-M.; Gweon, D.-G.; Lee, M.G. A novel laser micro/nano-machining system for FPD process. J. Mater. Process. Technol. 2008, 201, 497–501. [Google Scholar] [CrossRef]
- Lee, C.-W.; Kim, S.-W. An ultraprecision stage for alignment of wafers in advanced microlithography. Precis. Eng. 1997, 21, 113–122. [Google Scholar] [CrossRef]
- Kumar, R.; Khatait, J.P. Design of XY Air Bearing Stage for Ultra-Precision. Ph.D. Thesis, Indian Institute of Technology Delhi, Delhi, India, 2018. [Google Scholar]
- Zhang, F.; Liu, P. Accuracy improvement of the H-drive air-levitating wafer inspection stage based on error analysis and compensation. Meas. Sci. Technol. 2018, 29, 045013. [Google Scholar] [CrossRef]
- Kim, K.; Choi, Y.-M.; Nam, B.-U.; Lee, M.G. Dual servo stage without mechanical coupling for process of manufacture and inspection of flat panel displays via modular design approach. Int. J. Precis. Eng. Manuf. 2012, 13, 407–412. [Google Scholar] [CrossRef]
- Kim, K.-H.; Choi, Y.-M.; Gweon, D.-G.; Hong, D.-P.; Kim, K.-S.; Lee, S.-W.; Lee, M.-G. Design of decoupled dual servo stage with voice coil motor and linear motor for XY long stroke ultra-precision scanning system. In Proceedings of the ICMIT, Chongqing, China, 20–23 September 2005; p. 60401C. [Google Scholar]
- Choi, Y.-M.; Gweon, D.-G. A high-precision dual-servo stage using halbach linear active magnetic bearings. IEEE/ASME Trans. Mechatron. 2011, 16, 925–931. [Google Scholar] [CrossRef]
- Giam, T.S.; Tan, K.K.; Huang, S. Precision coordinated control of multi-axis gantry stages. ISA Trans. 2007, 46, 399–409. [Google Scholar] [CrossRef] [PubMed]
- Ma, J.; Chen, S.-L.; Kamaldin, N.; Teo, C.S.; Tay, A.; Mamun, A.A.; Tan, K.K. A novel constrained H2 optimization algorithm for mechatronics design in flexure-linked biaxial gantry. ISA Trans. 2017, 71, 467–479. [Google Scholar] [CrossRef] [PubMed]
- Ma, J.; Teo, C.S.; Tay, A.; Mamun, A.A.; Tan, K.K. Integrated Mechatronic Design in the Flexure-Linked Dual-Drive Gantry by Constrained Linear–Quadratic Optimization. IEEE Trans. Ind. Electron. 2018, 65, 2408–2418. [Google Scholar] [CrossRef]
- Kim, K.; Ahn, D.; Gweon, D. Optimal design of a 1-rotational DOF flexure joint for a 3-DOF H-type stage. Mechatronics 2012, 22, 24–32. [Google Scholar] [CrossRef]
- Newway Air Bearings. Air Bearing Application and Design Guide, Revision E; Newway Air Bearings: Aston, PA, USA, 2006; Available online: https://www.newwayairbearings.com/technology/technical-resources/tech-links/handbooks (accessed on 1 May 2020).
- Kondo, T.; Fujisawa, S.; Kitade, N. XY Table using a Linear Electromagnetic Actuator. U.S. Patent 5,760,500, 2 June 1998. [Google Scholar]
- Novak, W.T. Dual Guide Beam Stage Mechanism with Yaw Control. U.S. Patent 5,760,564, 2 June 1998. [Google Scholar]
- Tan, K.K.; Lim, S.Y.; Huang, S.; Dou, H.F.; Giam, T.S. Coordinated motion control of moving gantry stages for precision applications based on an observer-augmented composite controller. IEEE Trans. Control Syst. Technol. 2004, 12, 984–991. [Google Scholar] [CrossRef]
- Lee, M.E. Window Frame-Guided Stage Mechanism. U.S. Patent 5,874,820, 23 February 1999. [Google Scholar]
- Sakino, S.; Osanai, E. Motion Guiding Device. U.S. Patent 5,228,358, 20 July 1993. [Google Scholar]
- Teo, C.S.; Tan, K.K.; Lim, S.Y.; Huang, S.; Tay, E.B. Dynamic modeling and adaptive control of a H-type gantry stage. Mechatronics 2007, 17, 361–367. [Google Scholar] [CrossRef]
- Koseki, Y.; Tanikawa, T.; Koyachi, N.; Arai, T. Kinematic analysis of a translational 3-dof micro-parallel mechanism using the matrix method. Adv. Robot. 2002, 16, 251–264. [Google Scholar] [CrossRef]
- Ryu, J.W.; Gweon, D.-G.; Moon, K.S. Optimal design of a flexure hinge based XYφ wafer stage. Precis. Eng. 1997, 21, 18–28. [Google Scholar] [CrossRef]
- Kang, S.; Lee, M.G.; Choi, Y.-M. Six degrees-of-freedom direct-driven nanopositioning stage using crab-elg flexures. IEEE/ASME Trans. Mechatron. 2020, 25, 513–525. [Google Scholar] [CrossRef]
- Zheng, L.; Chen, W.; Huo, D.; Lyu, X. Design, analysis, and control of a two-Dimensional vibration device for vibration-assisted micromilling. IEEE/ASME Trans. Mechatron. 2020, 25, 1510–1518. [Google Scholar] [CrossRef]
- Mao, W.-L.; Hung, C.-W.; Suprapto. The application of proportional–integral–derivative–type fuzzy controller for synchronized XY motion gantry stage system. Adv. Mech. Eng. 2018, 10, 1–15. [Google Scholar] [CrossRef]
- Kamaldin, N.; Chen, S.; Teo, C.S.; Lin, W.; Tan, K.K. A novel adaptive jerk control with application to large workspace tracking on a flexure-linked dual-drive gantry. IEEE Trans. Ind. Electron. 2019, 66, 5353–5363. [Google Scholar] [CrossRef]




| Unit | Specification | |
|---|---|---|
| Motion range | mm | 200 |
| In-position stability | μm | ±0.05 |
| Yaw motion error | arcsec | ±1.0 |
| Maximum speed | mm/s | 100 |
| Maximum acceleration | m/s2 | 2 |
| Settling time 2 μm move, 1% (20 nm) | msec | 500 |
| Notation | Compliance |
|---|---|
| c1 | |
| c2 | |
| c3 | |
| c4 | |
| c5 | |
| c6 | |
| c7 | |
| c8 |
| Parameters | Design Results (mm) |
|---|---|
| u | 19.0 |
| v | 48.8 |
| d | 63.4 |
| e | 23.0 |
| f | 10.5 |
| r | 3.2 |
| t | 0.6 |
| Stiffness | Modeling | FEM | Error (%) |
|---|---|---|---|
| X (MN/m) | 976 | 996 | 2.01 |
| Y (MN/m) | 976 | 949 | 2.85 |
| Z (MN/m) | 492 | 523 | 5.93 |
| Θx (kNm/rad) | 1360 | 1453 | 6.4 |
| Θy (kNm/rad) | 1360 | 1406 | 3.27 |
| Θz (kNm/rad) | 86.3 | 83.1 | 3.85 |
| Newly Developed Stage | Conventional Stage [20] | |
|---|---|---|
| Settling time (2 µm step, 1%) | 48 ms | 73 ms |
| Speed ripple (100 mm/s) | 0.127 mm/s | 0.3 mm/s |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, H.-J.; Ahn, D. Development of Air Bearing Stage Using Flexure for Yaw Motion Compensation. Actuators 2022, 11, 100. https://doi.org/10.3390/act11040100
Lee H-J, Ahn D. Development of Air Bearing Stage Using Flexure for Yaw Motion Compensation. Actuators. 2022; 11(4):100. https://doi.org/10.3390/act11040100
Chicago/Turabian StyleLee, Hak-Jun, and Dahoon Ahn. 2022. "Development of Air Bearing Stage Using Flexure for Yaw Motion Compensation" Actuators 11, no. 4: 100. https://doi.org/10.3390/act11040100
APA StyleLee, H.-J., & Ahn, D. (2022). Development of Air Bearing Stage Using Flexure for Yaw Motion Compensation. Actuators, 11(4), 100. https://doi.org/10.3390/act11040100






