Experimental Study on Magnetic Resonant Coupling AC Magnetic Suspension Considering Electrical Power Transmission
Abstract
:1. Introduction
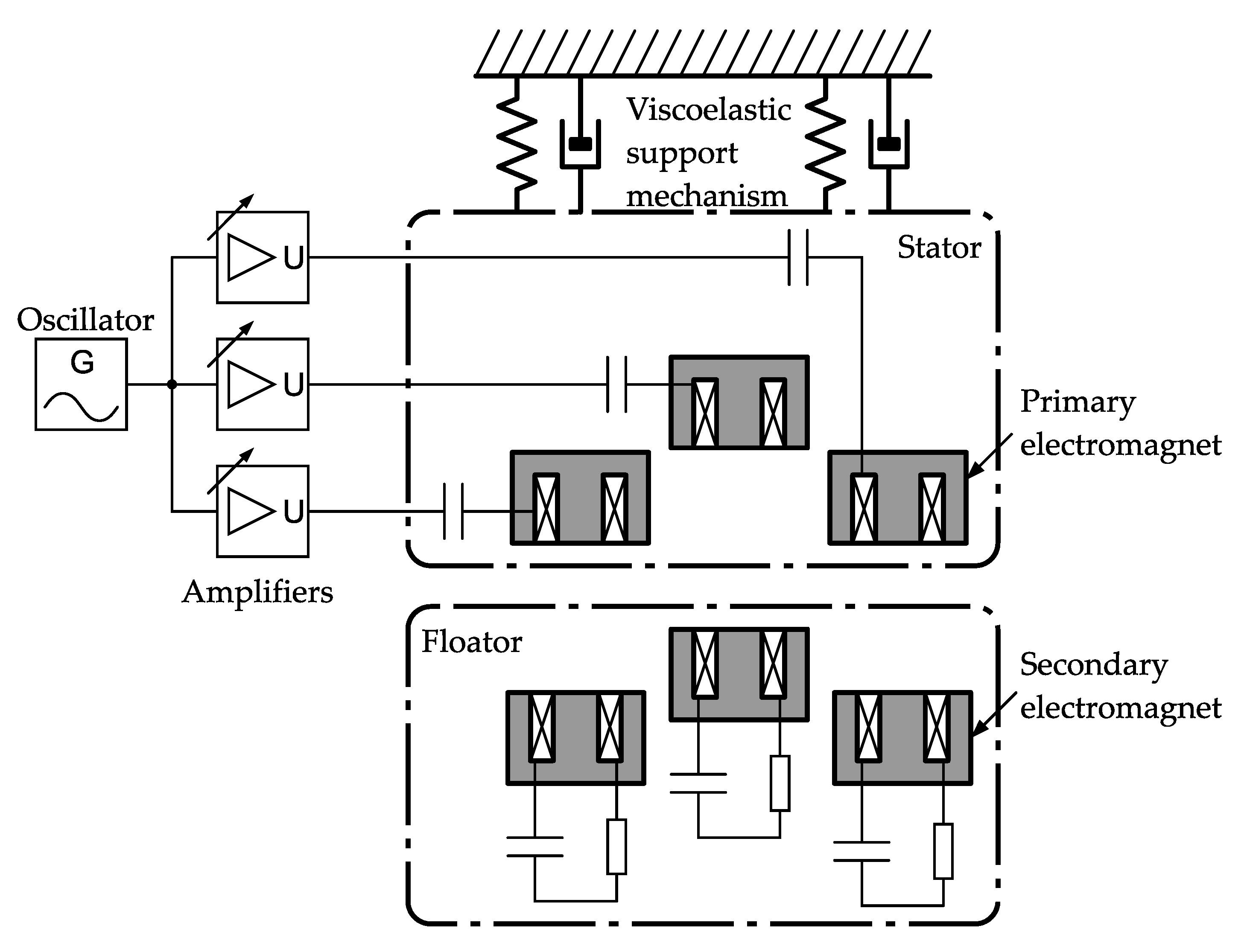
2. Principle
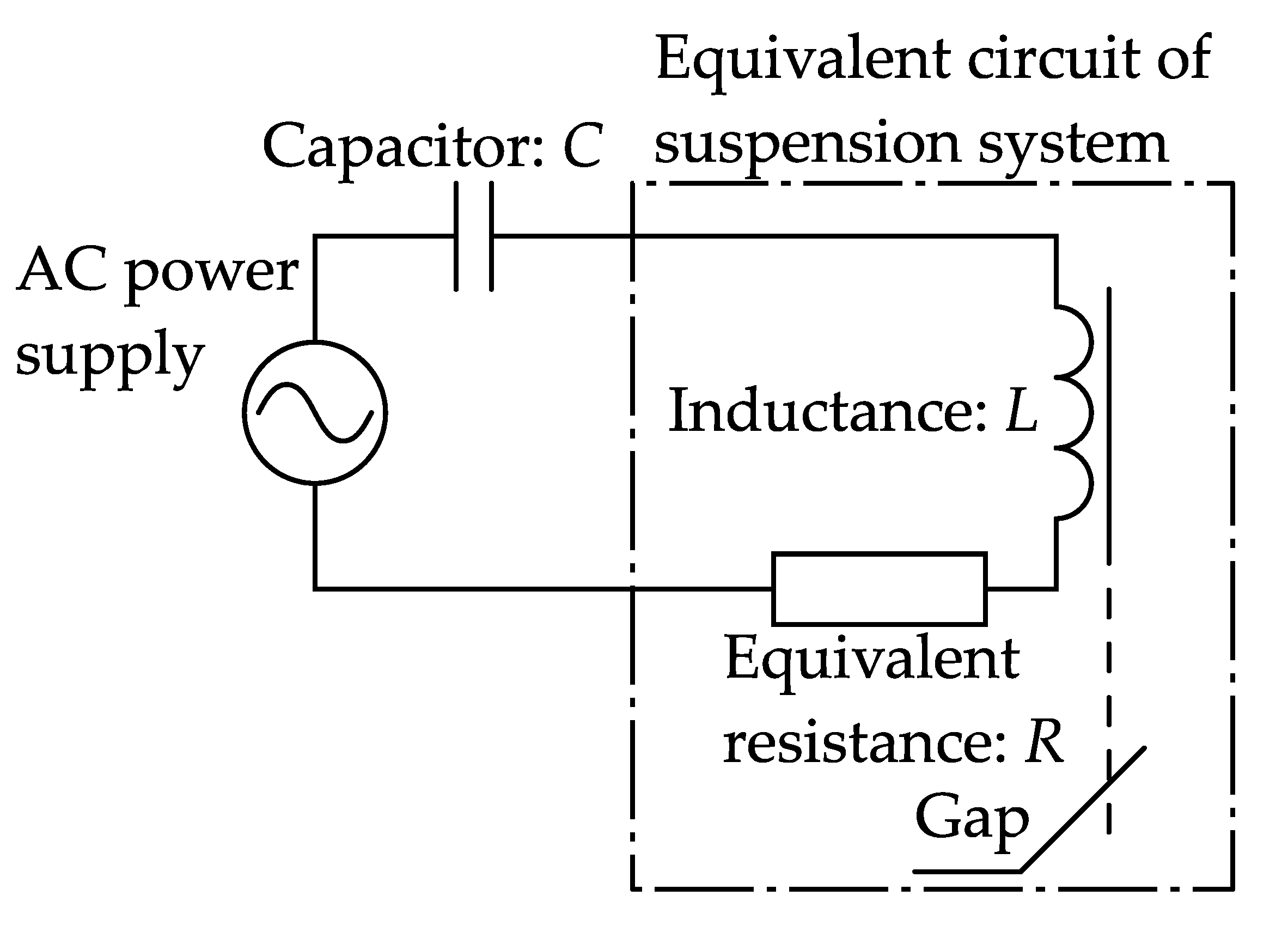
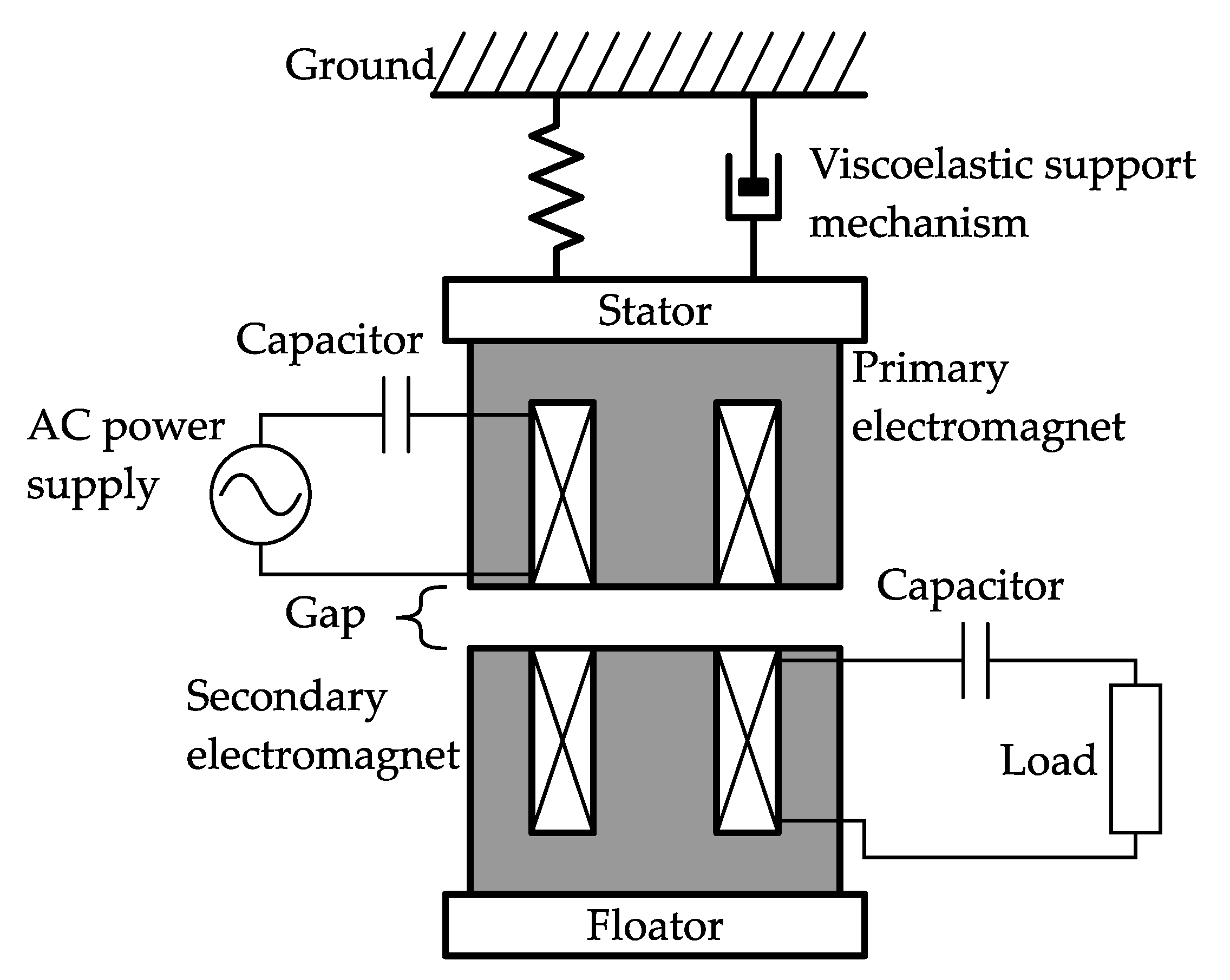
2.1. AC Magnetic Suspension System
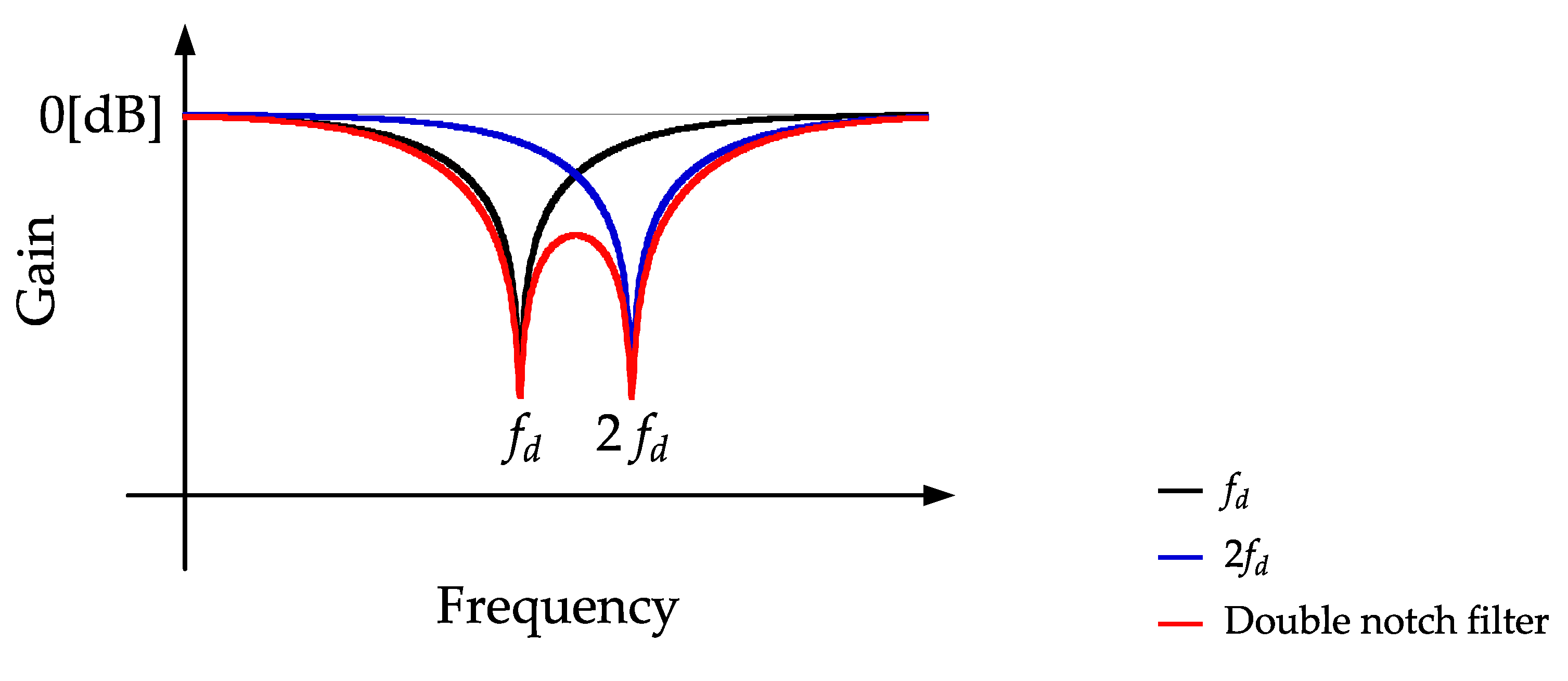
2.2. Magnetic Resonant Coupling Electric Power Transmission
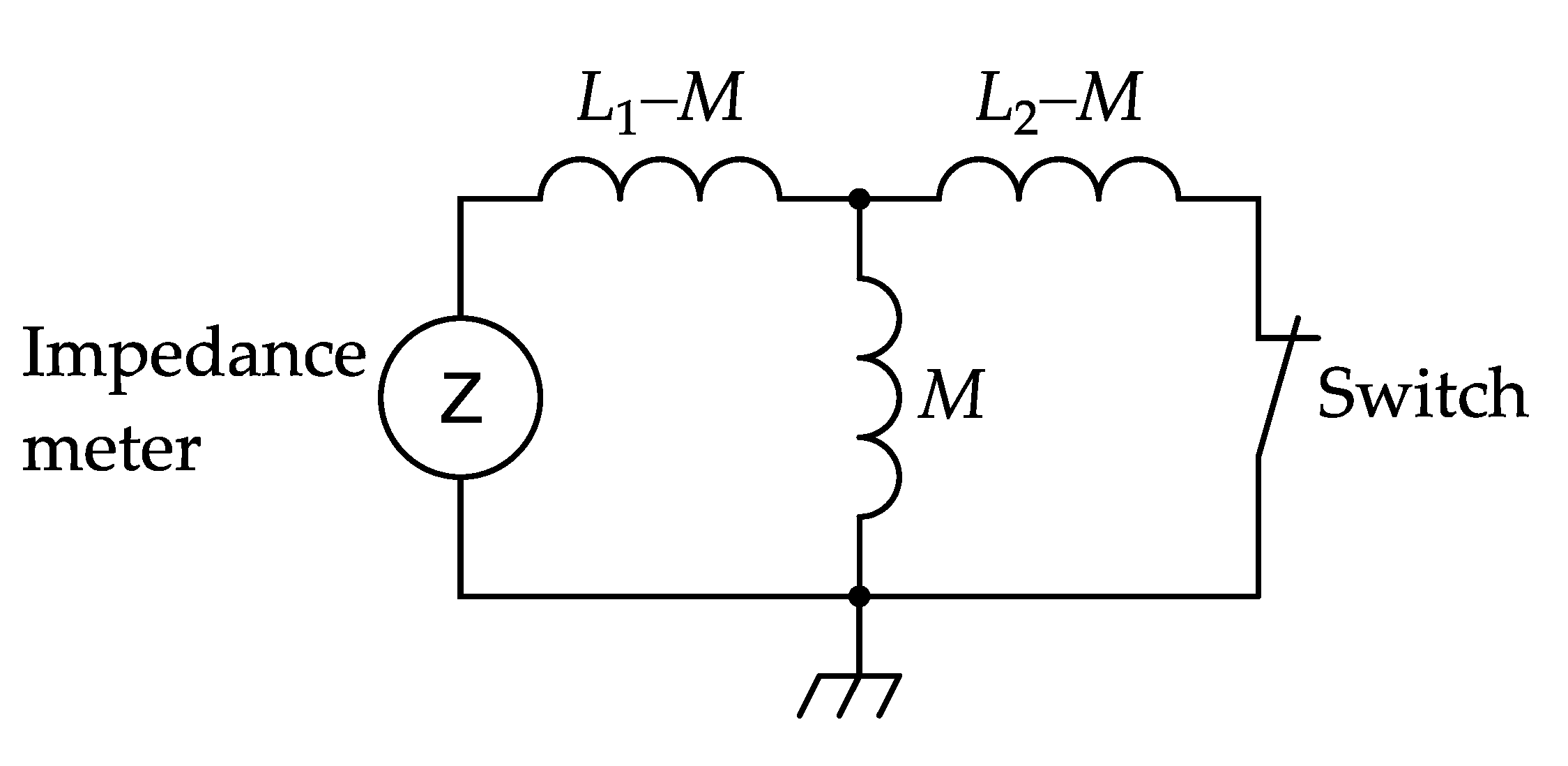
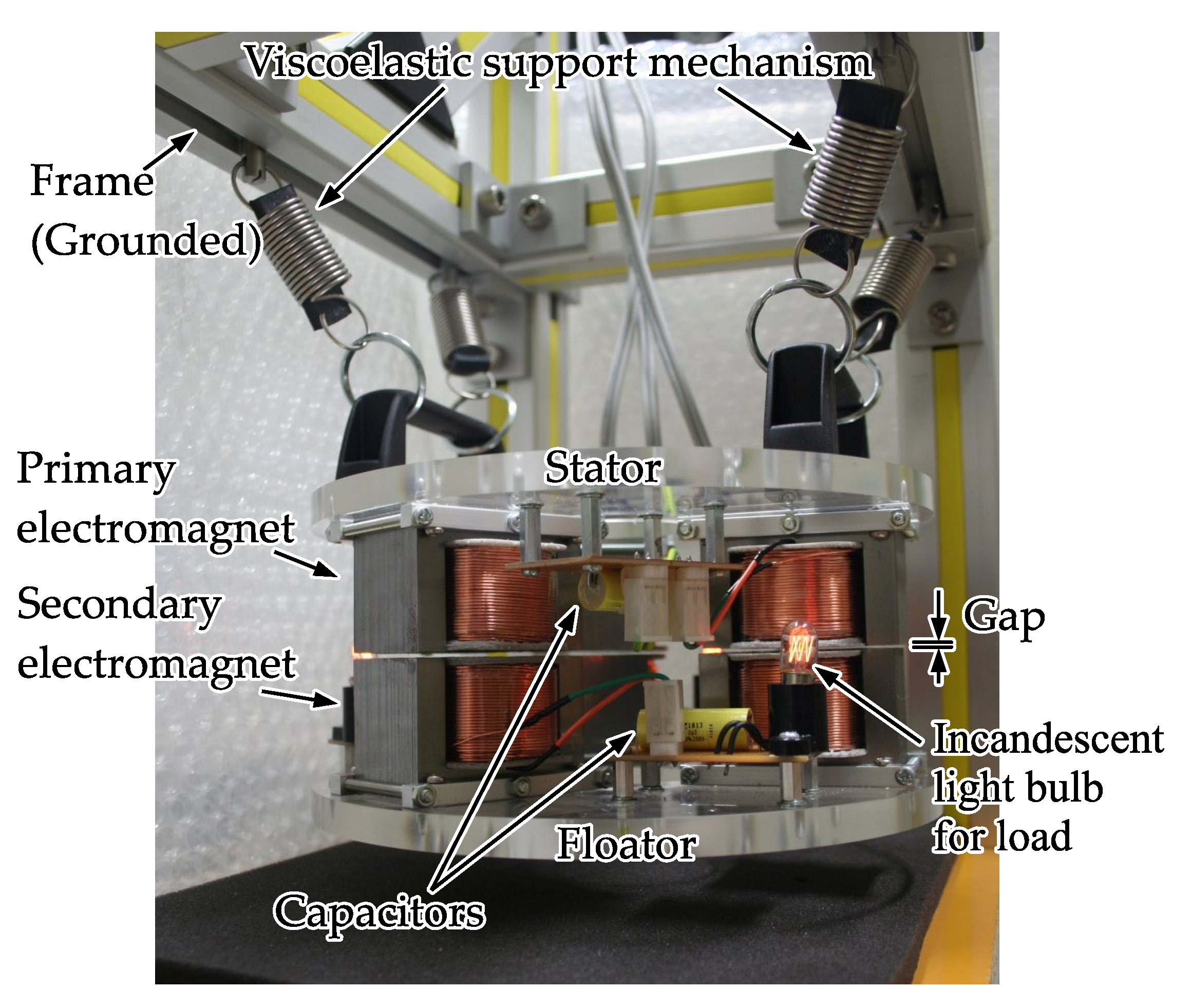
3. Experimental Setup
4. Experimental Results
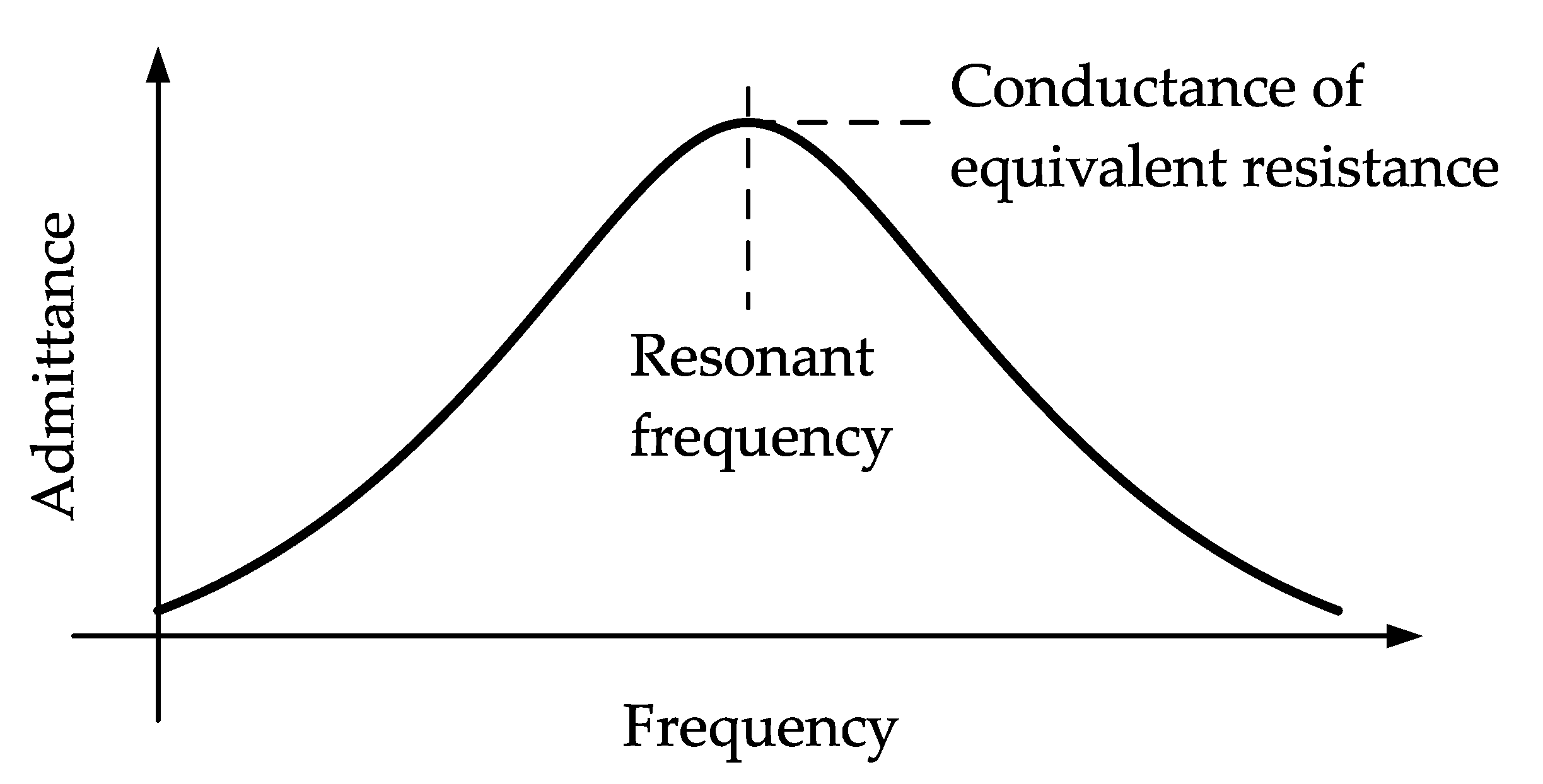
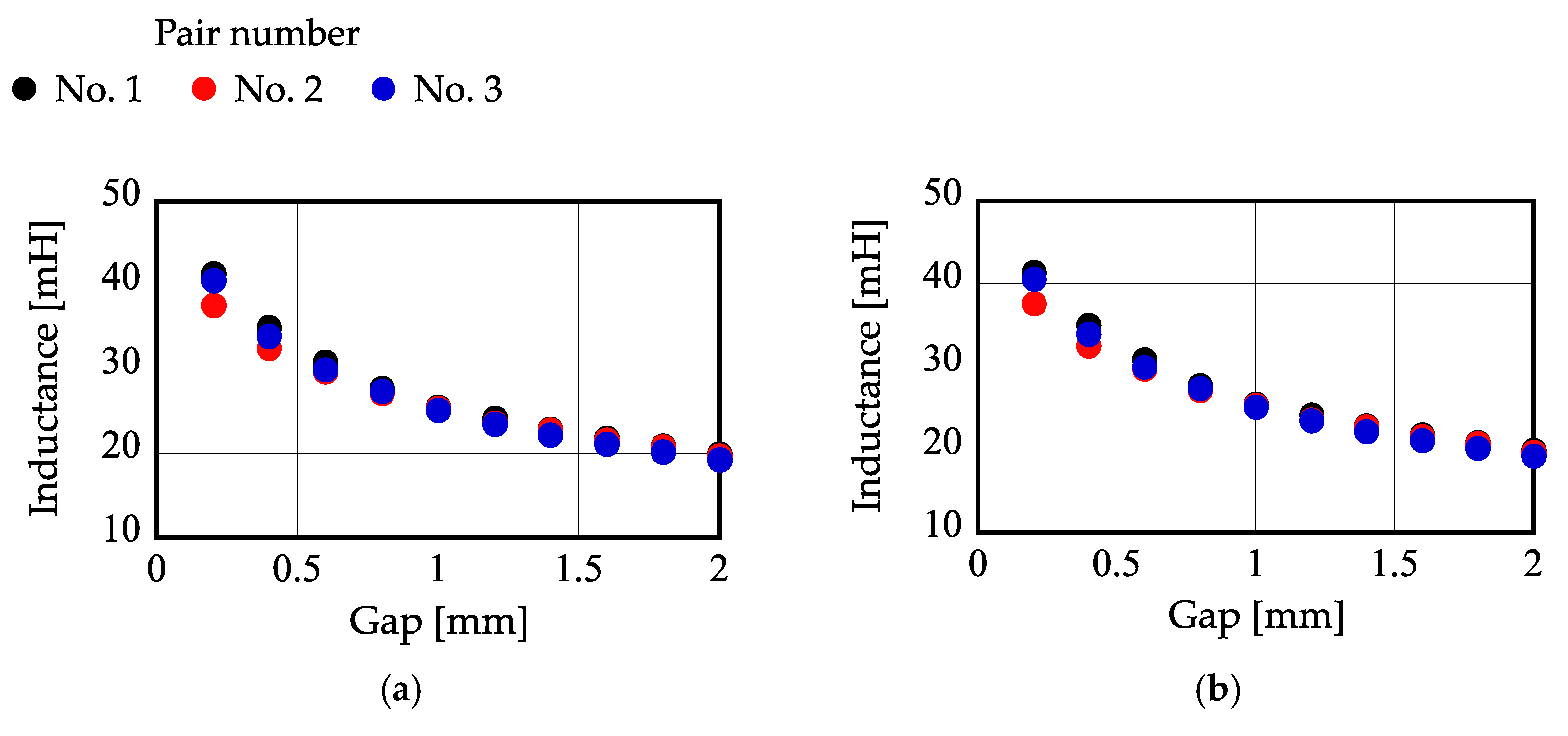
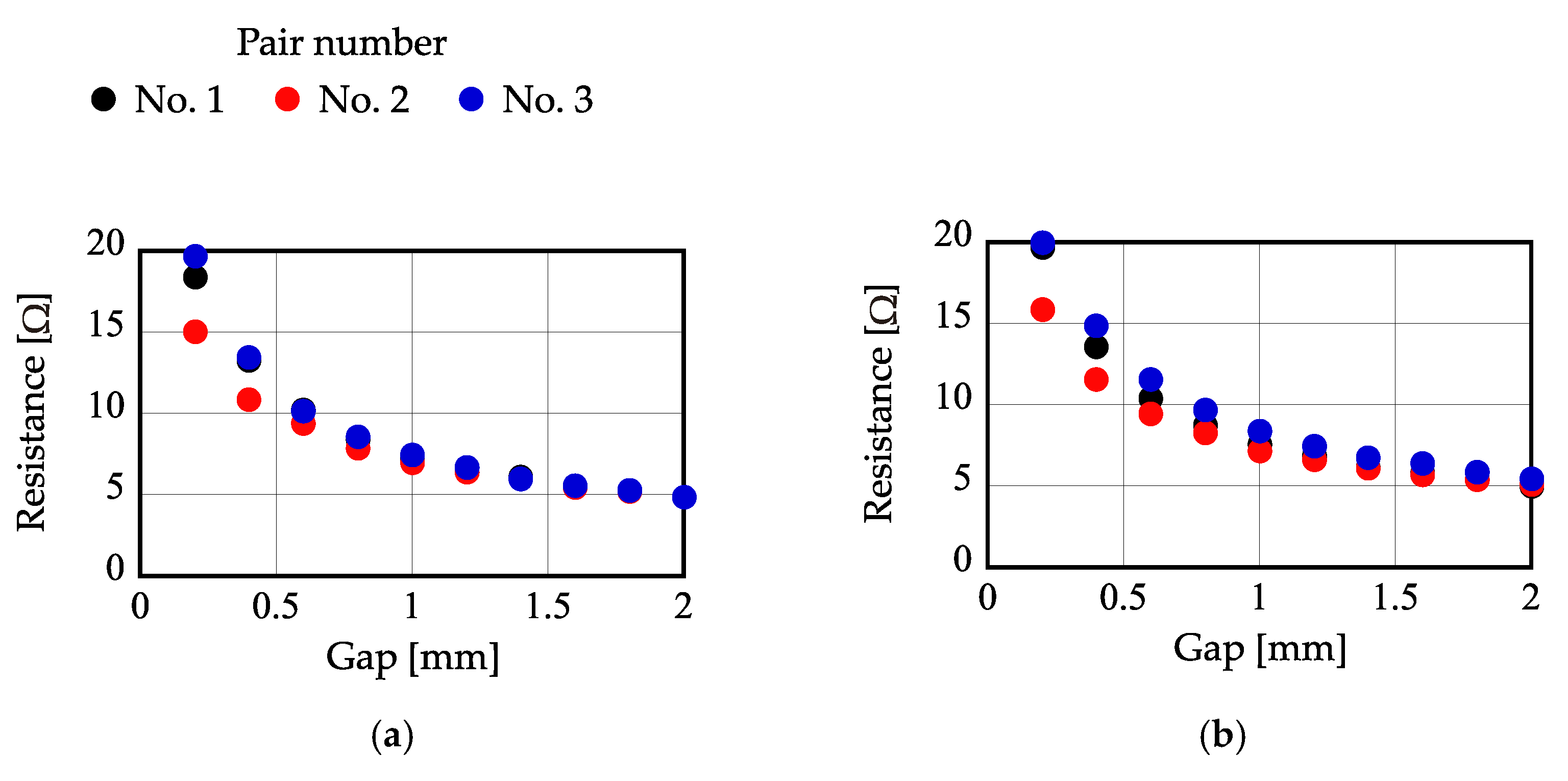
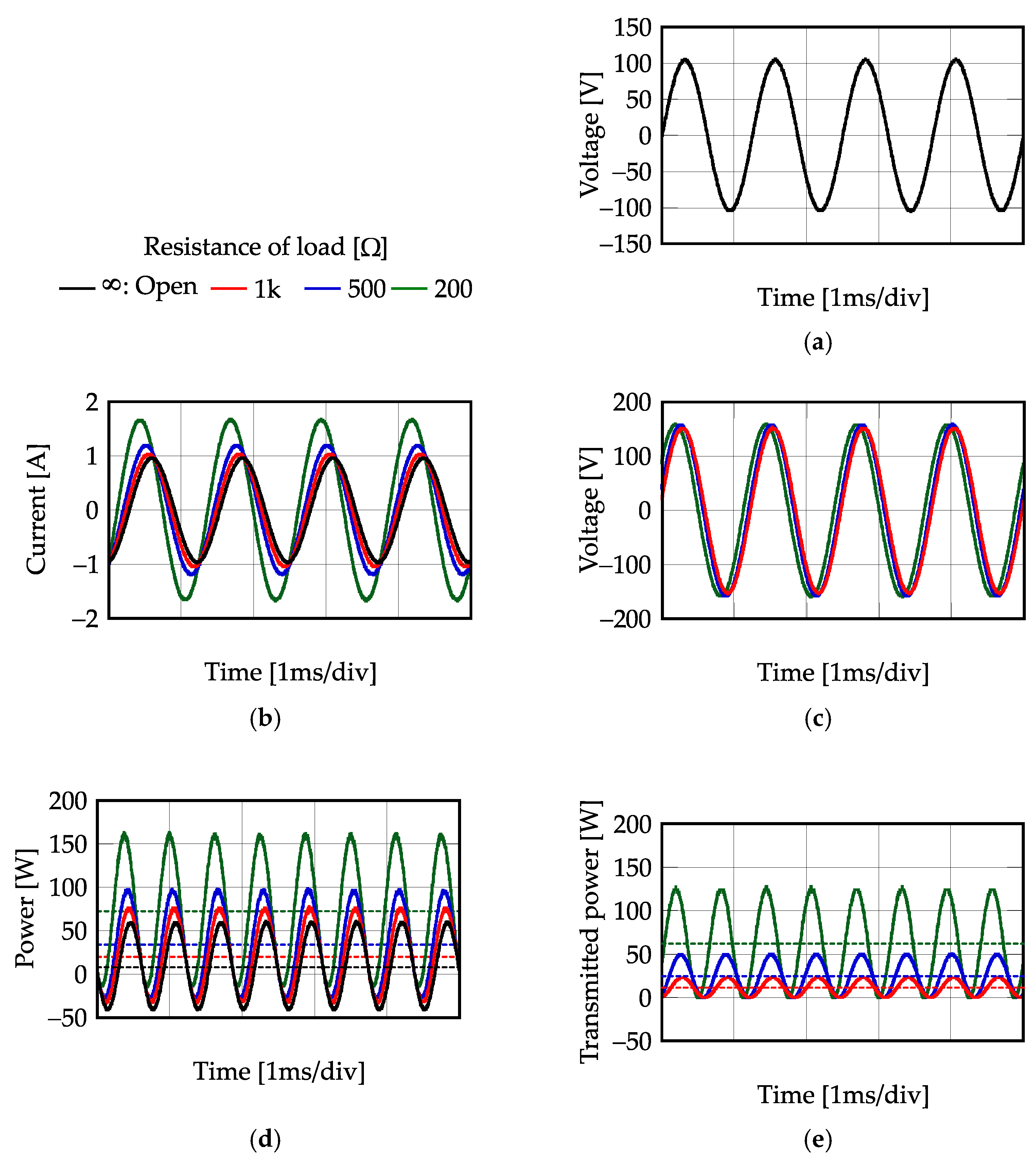
4.1. Electric Characteristics
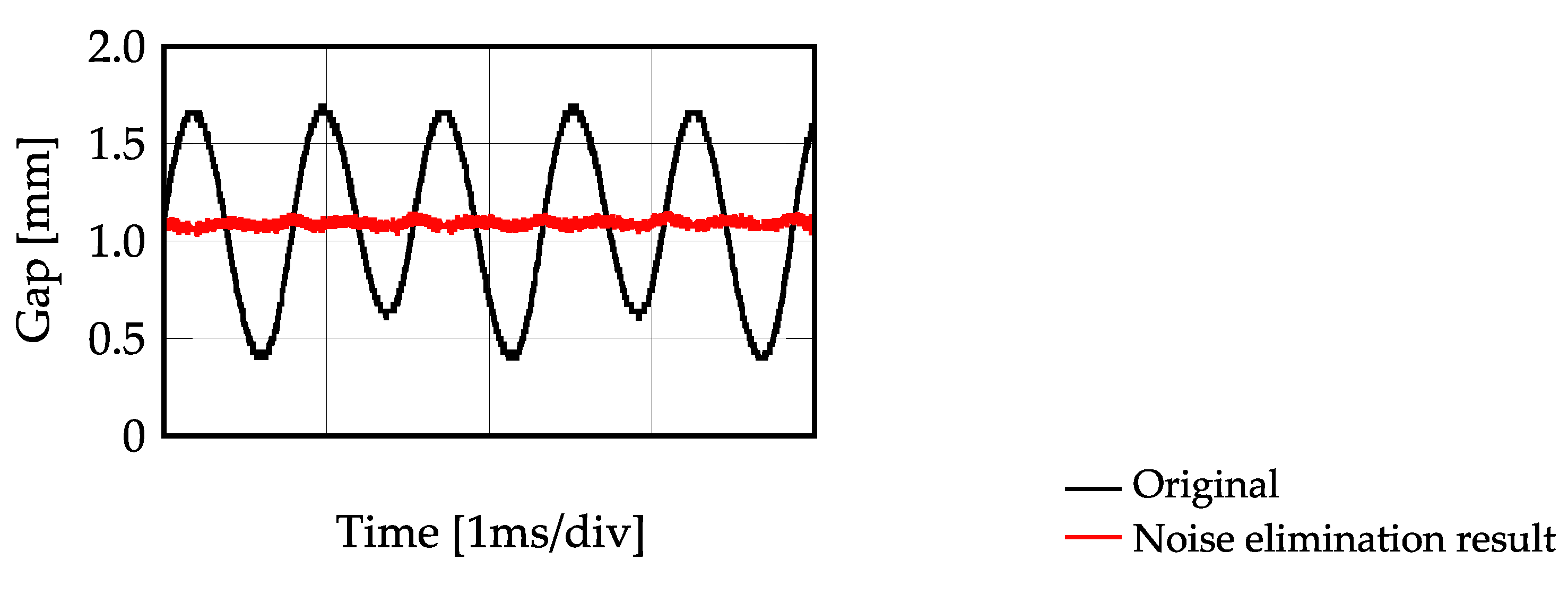
4.2. Levitation Characteristics
5. Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Jayawant, B. Electromagnetic suspension and levitation. IEE Proc. A Phys. Sci. Meas. Instrum. Manag. Educ. Rev. 1982, 129, 549–581. [Google Scholar] [CrossRef] [Green Version]
- Bleuler, H. A Survey of Magnetic Levitation and Magnetic Bearing Types. JSME Int. J. Ser. 3 Vib. Control Eng. Eng. Ind. 1992, 35, 335–342. [Google Scholar] [CrossRef] [Green Version]
- Jayawant, B.V.; Sinha, P.K.; Wheeler, A.R.; Whorlow, R.J.; Willsher, J. Development of 1-ton magnetically suspended vehicle using controlled d.c. electromagnets. Proc. Inst. Electr. Eng. 1976, 123, 941–948. [Google Scholar] [CrossRef]
- Ohura, Y.; Ueda, K.; Sugita, S. Performance of touchdown bearings for turbo molecular pumps. In Proceedings of the 8th International Symptom on Magnetic Bearings, Mito, Japan, 26–28 August 2002; pp. 515–520. [Google Scholar]
- Masuzawa, T.; Onuma, H.; Okada, Y. An ultradurable and compact rotary blood pump with a magnetically suspended impeller in the radial direction. Artif. Organs. 2001, 25, 395–399. [Google Scholar] [CrossRef] [PubMed]
- Morishita, M.; Ito, H. The Self-gap-Detecting Electromagnetic Suspension System with Robustness Against Variation of Coil Resistance. IEEJ Trans. Ind. App. 2006, 126, 1280–1285. [Google Scholar] [CrossRef] [Green Version]
- Maruyama, Y.; Mizuno, T.; Takasaki, M.; Ishino, Y.; Ishigami, T.; Kameno, H. An Application of Active Magnetic Bearing to Gyroscopic and Inertial Sensors. J. Syst. Des. Dyn. 2008, 2, 155–164. [Google Scholar] [CrossRef] [Green Version]
- Ahrens, M.; Kucera, L.; Larsonneur, R. Performance of a magnetically suspended flywheel energy storage device. IEEE Trans. Control Syst. Technol. 1996, 4, 494–502. [Google Scholar] [CrossRef]
- Cao, G.Z.; Li, W.B.; Huang, S.D.; He, J.B. Modeling, Analysis, and Implementation of a Novel Wireless-Moving-Levitating System with Multiple Magnetic Fields. Electr. Power Compon. Syst. 2019, 47, 2565. [Google Scholar] [CrossRef]
- Lu, Z.Y.; Li, D.S.; Ye, L.Z.; Wang, W.; Guo, Q.H. The Study of PDF Control Technology for a Levitated Globe with Hybrid-excited Magnets. In Proceedings of the Electromagnetics Research Symposium, Hangzhou, China, 24–28 March 2008. [Google Scholar]
- Kaplan, B.-Z.; Regev, D. Dynamic stabilization of tuned-circuit levitators. IEEE Trans. Magn. 1976, 12, 556–559. [Google Scholar] [CrossRef]
- Hagihara, S. Performance and stability of a magnetic suspension device using a tuned LCR circuit. Proc. Inst. Electr. Eng. 1978, 125, 153–156. [Google Scholar] [CrossRef]
- Jin, J.; Higuchi, T. Dynamics and Stability of Magnetic Suspension Systems Using Tuned LC Circuit. JSME Int. J. Ser. C Dyn. Control Robot. Des. Manuf. 1994, 37, 494–498. [Google Scholar] [CrossRef]
- Ishino, Y.; Takasaki, M.; Mizuno, T. Fabrication of non-contact carrier system using solar magnetic suspension. J. Syst. Des. Dynamics 2015, 2, 15-00143. [Google Scholar] [CrossRef] [Green Version]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljacic, M. Wireless power transfer via strongly coupled magnetic resonances. Sci. Express 2007, 317, 83–86. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mizuno, T.; Takahashi, K.; Ishino, Y.; Takasaki, M. Novel AC magnetic suspension using magnetic resonant coupling. Mech. Eng. J. 2016, 3, 15-00687. [Google Scholar] [CrossRef] [Green Version]
- Rahman, A.; Mizuno, T.; Takasaki, M.; Ishino, Y. An Equivalent Circuit Analysis and Suspension Characteristics of AC Magnetic Suspension Using Magnetic Resonant Coupling. Actuators 2020, 9, 52. [Google Scholar] [CrossRef]
- Yamamoto, T.; Nara, K.; Kaneko, Y. An Evaluation of Wireless Power Transfer System with Plural Repeater Coils for Moving Objects. In Proceedings of the IECON 2018—44th Annual Conference of the IEEE Industrial Electronics Society, Washington, DC, USA, 21–23 October 2018. [Google Scholar] [CrossRef]
- Johnson, R.L.; Rea, J.B. Importance of Extending Nyquist Servomechanism Analysis to Include Transient Response. J. Aeronaut. Sci. 1951, 18, 43–49. [Google Scholar] [CrossRef]







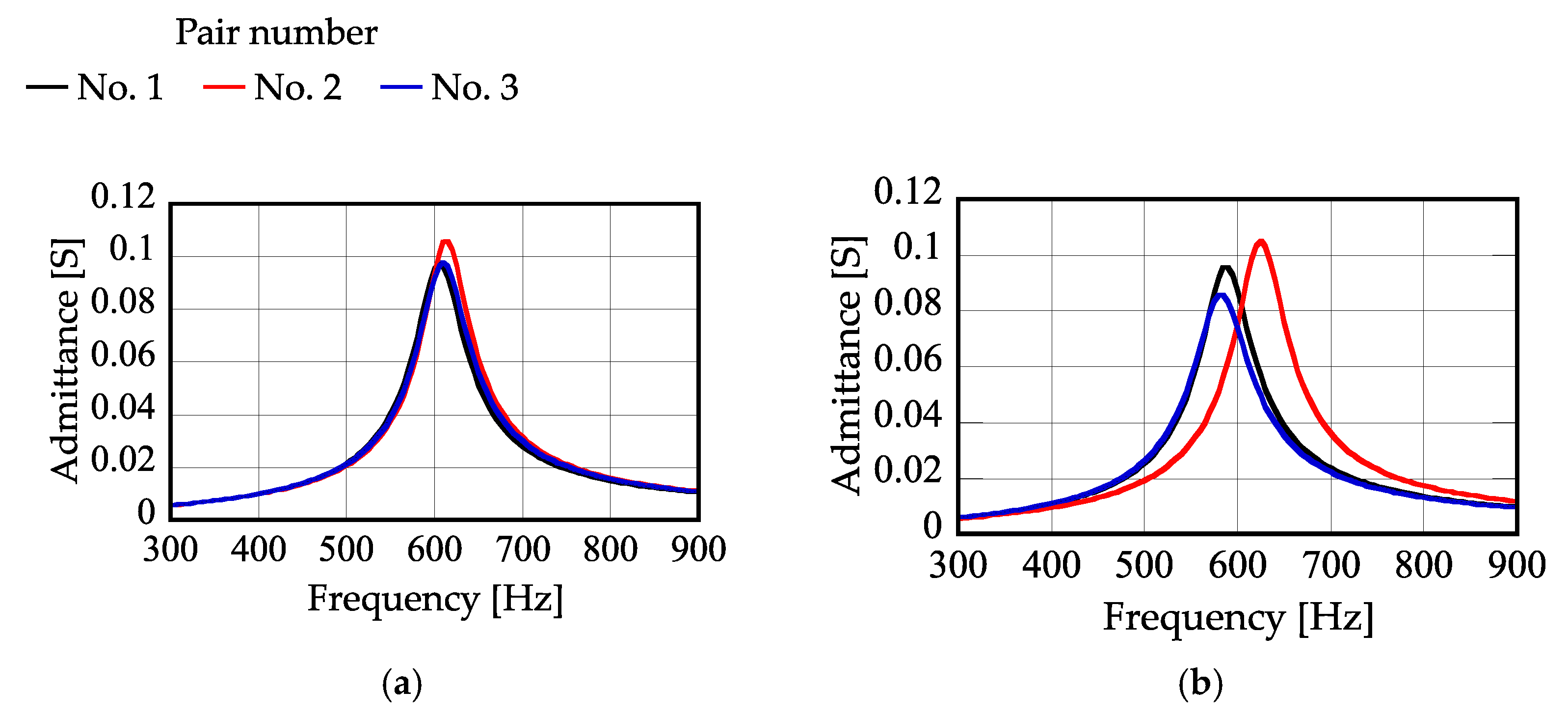


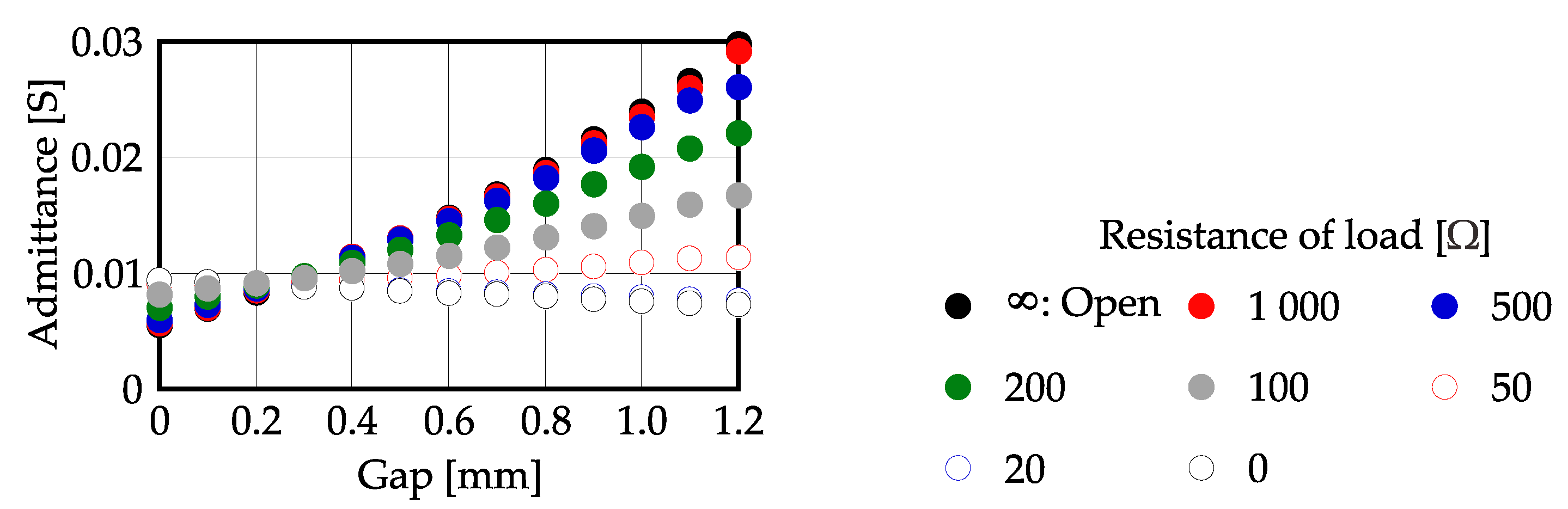



| Number of Pair | For Primary Electromagnet (μF) | For Secondary Electromagnet (μF) |
|---|---|---|
| 1 | 2.23 | 2.32 |
| 2 | 2.26 | 2.23 |
| 3 | 2.27 | 2.31 |
| Load Resistance (Ω) | Active Input Power (W) | Transmitted Power (W) |
|---|---|---|
| Infinite | 8.7 | |
| 1000 | 21.1 | 11.6 |
| 500 | 34.4 | 25.0 |
| 200 | 73.1 | 62.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ishino, Y.; Mizuno, T.; Takasaki, M. Experimental Study on Magnetic Resonant Coupling AC Magnetic Suspension Considering Electrical Power Transmission. Actuators 2022, 11, 208. https://doi.org/10.3390/act11080208
Ishino Y, Mizuno T, Takasaki M. Experimental Study on Magnetic Resonant Coupling AC Magnetic Suspension Considering Electrical Power Transmission. Actuators. 2022; 11(8):208. https://doi.org/10.3390/act11080208
Chicago/Turabian StyleIshino, Yuji, Takeshi Mizuno, and Masaya Takasaki. 2022. "Experimental Study on Magnetic Resonant Coupling AC Magnetic Suspension Considering Electrical Power Transmission" Actuators 11, no. 8: 208. https://doi.org/10.3390/act11080208
APA StyleIshino, Y., Mizuno, T., & Takasaki, M. (2022). Experimental Study on Magnetic Resonant Coupling AC Magnetic Suspension Considering Electrical Power Transmission. Actuators, 11(8), 208. https://doi.org/10.3390/act11080208






