A Novel Hierarchical Recursive Nonsingular Terminal Sliding Mode Control for Inverted Pendulum
Abstract
:1. Introduction
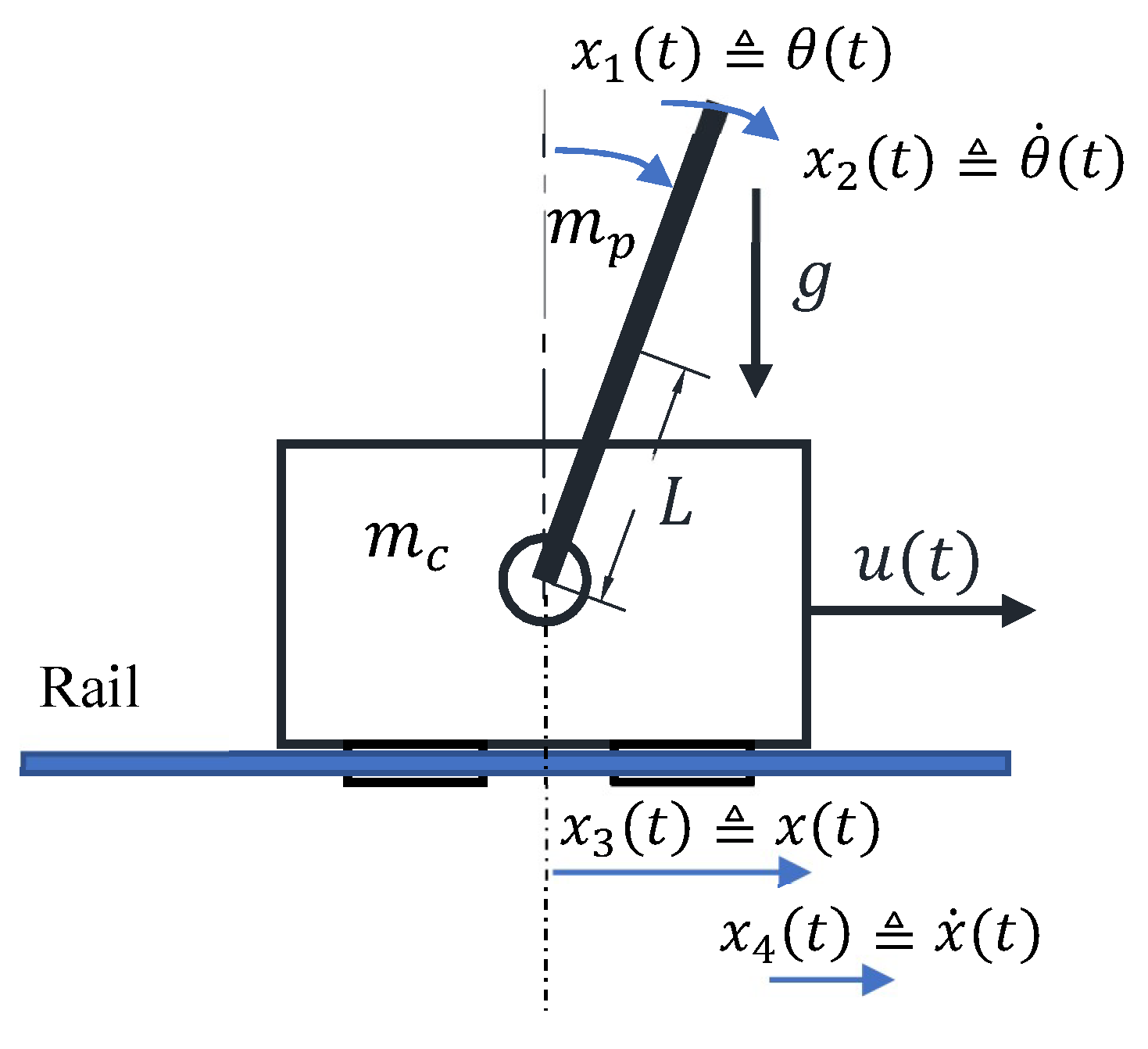
2. Problem Formulation
3. Control Strategy and Stability Analysis
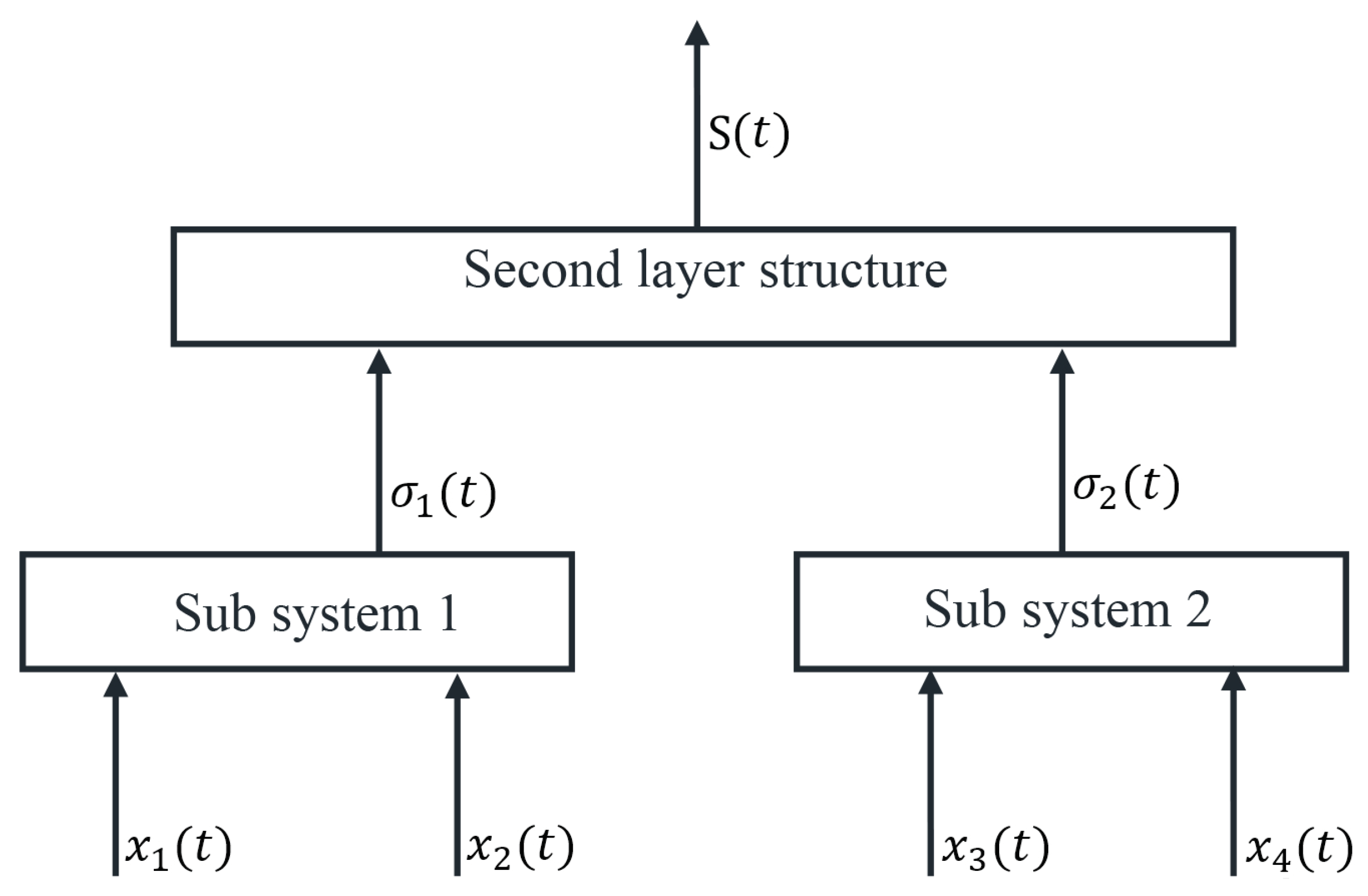
3.1. Design Hierarchical Recursive Nonsingular Terminal Sliding Mode Control
3.2. Design of Extended State Observer
3.3. Parameters Selection
3.4. Detailed Step for Designing Proposed Controller
4. Simulation Verification
4.1. Simulation Condition
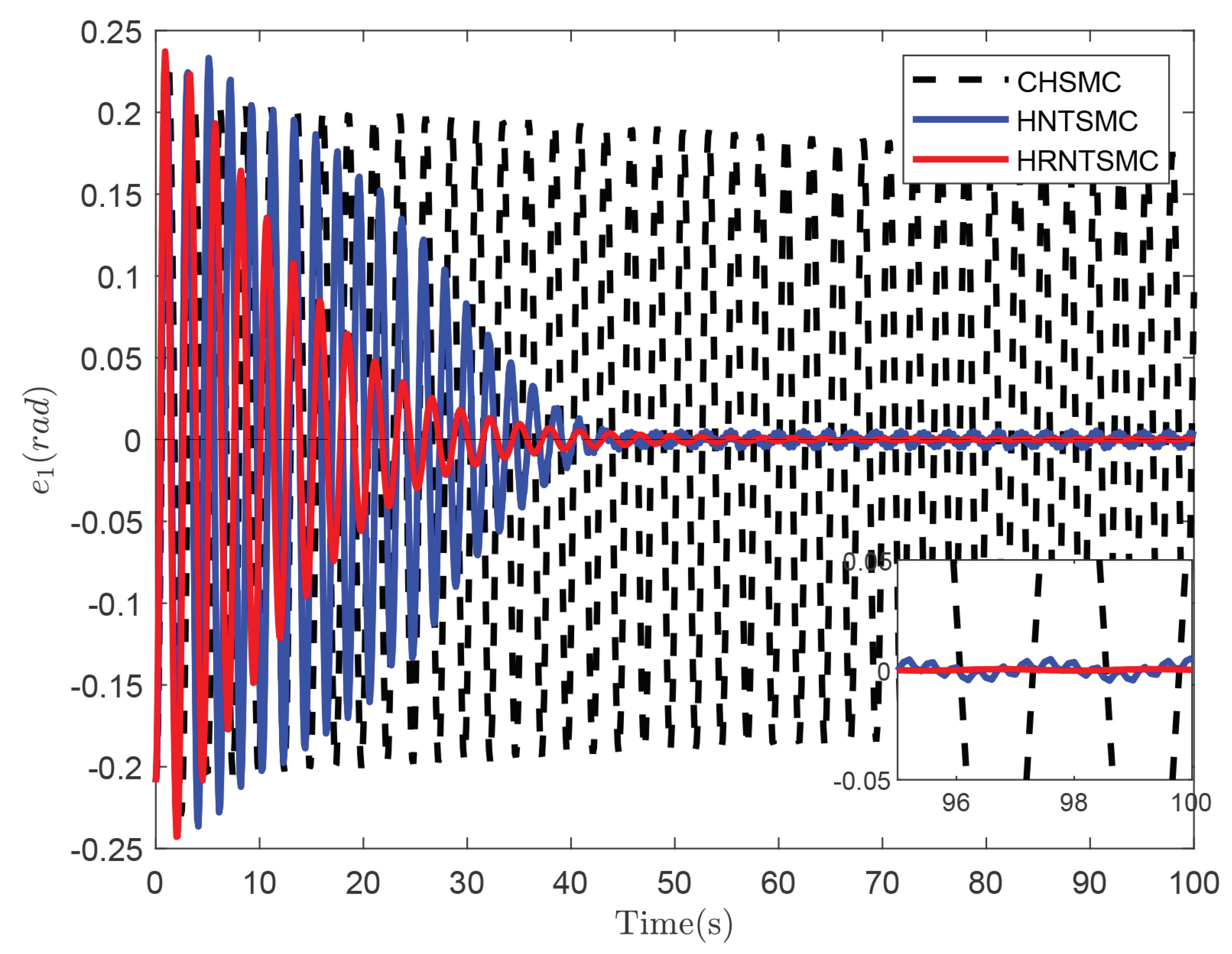
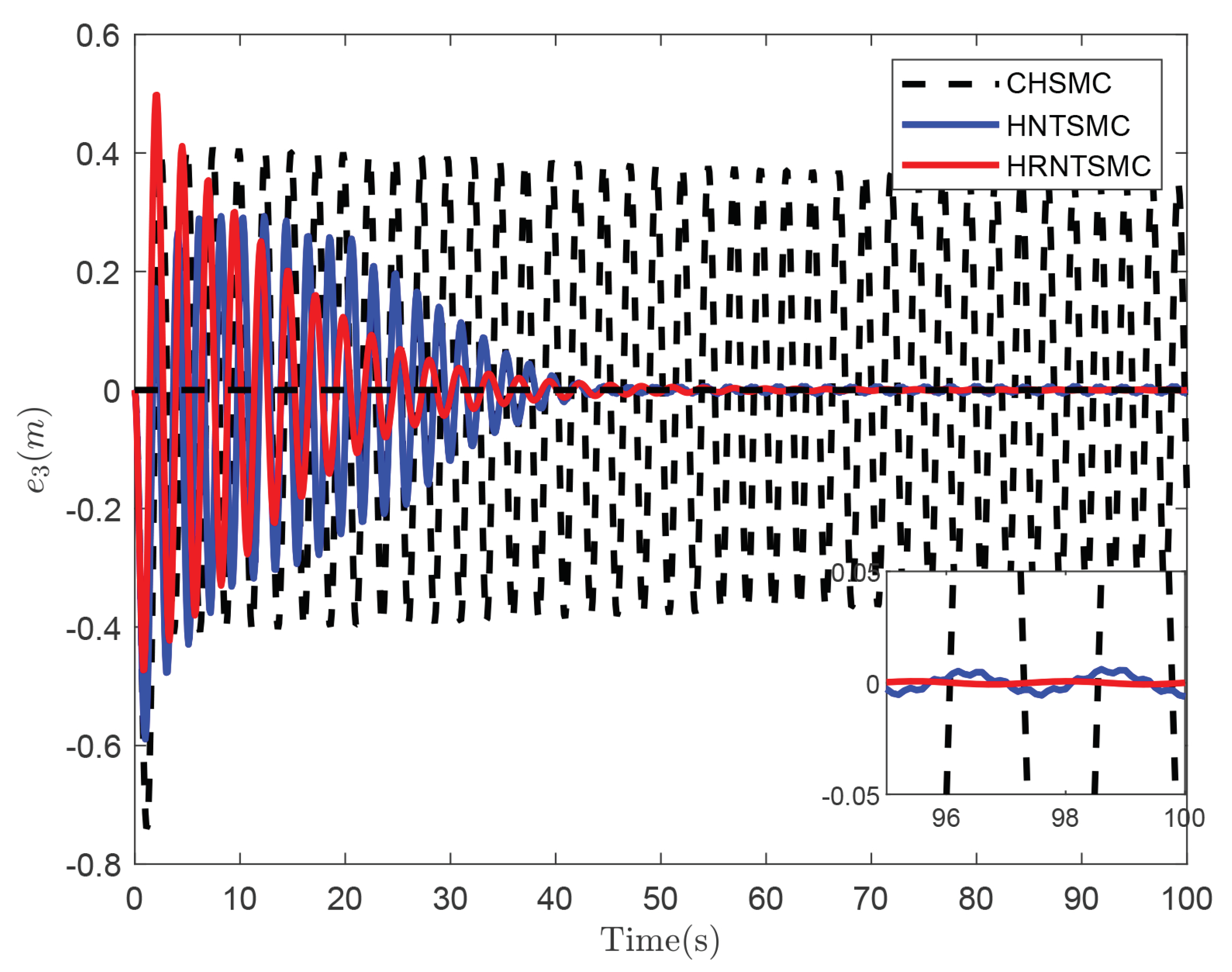
4.2. Simulation Results
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Stability of the LESO
References
- Irfan, S.; Mehmood, A.; Razzaq, M.T.; Iqbal, J. Advanced sliding mode control techniques for inverted pendulum: Modelling and simulation. Eng. Sci. Technol. Int. J. 2018, 21, 753–759. [Google Scholar] [CrossRef]
- Kim, S.; Kwon, S. Nonlinear optimal control design for underactuated two-wheeled inverted pendulum mobile platform. IEEE/ASME Trans. Mechatronics 2017, 22, 2803–2808. [Google Scholar] [CrossRef]
- Sentis, L. Compliant control of whole-body multi-contact behaviors in humanoid robots. In Motion Planning for Humanoid Robots; Springer: Berlin/Heidelberg, Germany, 2010; pp. 29–66. [Google Scholar]
- Wang, W.; Yi, J.; Zhao, D.; Liu, D. Design of a stable sliding-mode controller for a class of second-order underactuated systems. IEE Proc. -Control Theory Appl. 2004, 151, 683–690. [Google Scholar] [CrossRef]
- Horibe, T.; Sakamoto, N. Optimal swing up and stabilization control for inverted pendulum via stable manifold method. IEEE Trans. Control. Syst. Technol. 2017, 26, 708–715. [Google Scholar] [CrossRef]
- Ismail, N. Fuzzy Logic Controller Design for Inverted Pendulum System. Ph.D. Thesis, Universiti Tun Hussein Malaysia, Johor, Malaysia, 2013. [Google Scholar]
- Bennett, S. Development of the PID controller. IEEE Control Syst. Mag. 1993, 13, 58–62. [Google Scholar]
- Ghosh, A.; Krishnan, T.; Subudhi, B. Robust proportional–integral–derivative compensation of an inverted cart–pendulum system: An experimental study. IET Control Theory Appl. 2012, 6, 1145–1152. [Google Scholar] [CrossRef]
- Batista, J.G.; Souza, D.A.; dos Reis, L.L.; Filgueiras, L.V.; Ramos, K.M.; Junior, A.B.; Correia, W.B. Performance comparison between the PID and LQR controllers applied to a robotic manipulator joint. In Proceedings of the IECON 2019-45th Annual Conference of the IEEE Industrial Electronics Society, Lisbon, Portugal, 14–17 October 2019; Volume 1, pp. 479–484. [Google Scholar]
- Wang, H.; Dong, H.; He, L.; Shi, Y.; Zhang, Y. Design and simulation of LQR controller with the linear inverted pendulum. In Proceedings of the 2010 International Conference on Electrical and Control Engineering, Wuhan, China, 25–27 June 2010; pp. 699–702. [Google Scholar]
- Camacho, O.; Smith, C.A. Sliding mode control: An approach to regulate nonlinear chemical processes. ISA Trans. 2000, 39, 205–218. [Google Scholar] [CrossRef]
- Huang, C.H.; Wang, W.J.; Chiu, C.H. Design and implementation of fuzzy control on a two-wheel inverted pendulum. IEEE Trans. Ind. Electron. 2010, 58, 2988–3001. [Google Scholar] [CrossRef]
- Yang, C.; Li, Z.; Cui, R.; Xu, B. Neural network-based motion control of an underactuated wheeled inverted pendulum model. IEEE Trans. Neural Netw. Learn. Syst. 2014, 25, 2004–2016. [Google Scholar] [CrossRef]
- Shtessel, Y.; Edwards, C.; Fridman, L.; Levant, A. Sliding Mode Control and Observation; Springer: Berlin/Heidelberg, Germany, 2014; Volume 10. [Google Scholar]
- Krafes, S.; Chalh, Z.; Saka, A. Linear, nonlinear and intelligent controllers for the inverted pendulum problem. In Proceedings of the 2016 International Conference on Electrical and Information Technologies (ICEIT), Tangiers, Morocco, 4–7 May 2016; pp. 136–141. [Google Scholar]
- Utkin, V.I. Sliding mode control in discrete-time and difference systems. In Variable Structure and Lyapunov Control; Springer: Berlin/Heidelberg, Germany, 2005; pp. 87–107. [Google Scholar]
- Utkin, V.I. Sliding Modes in Control and Optimization; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
- Basin, M.V.; Yu, P.; Shtessel, Y.B. Hypersonic missile adaptive sliding mode control using finite-and fixed-time observers. IEEE Trans. Ind. Electron. 2017, 65, 930–941. [Google Scholar] [CrossRef]
- Wang, G.; Xu, Q. Design and precision position/force control of a piezo-driven microinjection system. IEEE/ASME Trans. Mechatronics 2017, 22, 1744–1754. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, Y.; Xu, Q. Design and control of a novel compliant constant-force gripper based on buckled fixed-guided beams. IEEE/ASME Trans. Mechatronics 2016, 22, 476–486. [Google Scholar] [CrossRef]
- Zhihong, M.; Paplinski, A.P.; Wu, H.R. A robust MIMO terminal sliding mode control scheme for rigid robotic manipulators. IEEE Trans. Autom. control 1994, 39, 2464–2469. [Google Scholar] [CrossRef]
- Yu, X.; Zhihong, M. Fast terminal sliding-mode control design for nonlinear dynamical systems. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2002, 49, 261–264. [Google Scholar]
- Feng, Y.; Yu, X.; Man, Z. Non-singular terminal sliding mode control of rigid manipulators. Automatica 2002, 38, 2159–2167. [Google Scholar] [CrossRef]
- Shao, K.; Zheng, J.; Huang, K.; Wang, H.; Man, Z.; Fu, M. Finite-time control of a linear motor positioner using adaptive recursive terminal sliding mode. IEEE Trans. Ind. Electron. 2019, 67, 6659–6668. [Google Scholar] [CrossRef]
- Utkin, V.; Lee, H. Chattering problem in sliding mode control systems. In Proceedings of the International Workshop on Variable Structure Systems, 2006. VSS’06, Alghero, Sardinia, 5–7 June 2006; pp. 346–350. [Google Scholar]
- Asad, M.; Bhatti, A.I.; Iqbal, S. A novel reaching law for smooth sliding mode control using inverse hyperbolic function. In Proceedings of the 2012 International Conference on Emerging Technologies, Islamabad, Pakistan, 8–9 October 2012; pp. 1–6. [Google Scholar]
- Le, H.D.; Nestorović, T. Adaptive Proportional Integral Derivative Nonsingular Dual Terminal Sliding Mode Control for Robotic Manipulators. Dynamics 2023, 3, 656–677. [Google Scholar] [CrossRef]
- Kim, K.S.; Rew, K.H.; Kim, S. Disturbance observer for estimating higher order disturbances in time series expansion. IEEE Trans. Autom. control 2010, 55, 1905–1911. [Google Scholar]
- Zhang, J.; Liu, X.; Xia, Y.; Zuo, Z.; Wang, Y. Disturbance observer-based integral sliding-mode control for systems with mismatched disturbances. IEEE Trans. Ind. Electron. 2016, 63, 7040–7048. [Google Scholar] [CrossRef]
- Mobayen, S.; Tchier, F. Nonsingular fast terminal sliding-mode stabilizer for a class of uncertain nonlinear systems based on disturbance observer. Sci. Iran. 2017, 24, 1410–1418. [Google Scholar] [CrossRef]
- Chen, W.H.; Yang, J.; Guo, L.; Li, S. Disturbance-observer-based control and related methods—An overview. IEEE Trans. Ind. Electron. 2015, 63, 1083–1095. [Google Scholar] [CrossRef]
- Guo, B.Z.; Zhao, Z.L. Extended state observer for nonlinear systems with uncertainty. IFAC Proc. Vol. 2011, 44, 1855–1860. [Google Scholar] [CrossRef]
- Liu, Y.C.; Laghrouche, S.; Depernet, D.; N’Diaye, A.; Djerdir, A.; Cirrincione, M. Super-twisting sliding-mode observer-based model reference adaptive speed control for PMSM drives. J. Frankl. Inst. 2023, 360, 985–1004. [Google Scholar] [CrossRef]
- Zhang, J.; Gao, W.; Guo, Q. Extended State Observer-Based Sliding Mode Control Design of Two-DOF Lower Limb Exoskeleton. Actuators 2023, 12, 402. [Google Scholar] [CrossRef]
- Chen, H.; Sun, N. Nonlinear control of underactuated systems subject to both actuated and unactuated state constraints with experimental verification. IEEE Trans. Ind. Electron. 2019, 67, 7702–7714. [Google Scholar] [CrossRef]
- Utkin, V.; Guldner, J.; Shi, J. Sliding Mode Control in Electro-Mechanical Systems; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Idrees, M.; Muhammad, S.; Ullah, S. Robust hierarchical sliding mode control with state-dependent switching gain for stabilization of rotary inverted pendulum. Kybernetika 2019, 55, 455–471. [Google Scholar] [CrossRef]
- Zhao, H.; Zong, G.; Zhao, X.; Wang, H.; Xu, N.; Zhao, N. Hierarchical Sliding-Mode Surface-Based Adaptive Critic Tracking Control for Nonlinear Multiplayer Zero-Sum Games Via Generalized Fuzzy Hyperbolic Models. IEEE Trans. Fuzzy Syst. 2023, 31, 4010–4023. [Google Scholar] [CrossRef]
- Hwang, C.L.; Chiang, C.C.; Yeh, Y.W. Adaptive fuzzy hierarchical sliding-mode control for the trajectory tracking of uncertain underactuated nonlinear dynamic systems. IEEE Trans. Fuzzy Syst. 2013, 22, 286–299. [Google Scholar] [CrossRef]
- Hwang, C.L.; Wu, H.M. Trajectory tracking of a mobile robot with frictions and uncertainties using hierarchical sliding-mode under-actuated control. IET Control Theory Appl. 2013, 7, 952–965. [Google Scholar] [CrossRef]
- Nafa, F.; Labiod, S.; Chekireb, H. A structured sliding mode controller for a class of underactuated mechanical systems. In Proceedings of the International Workshop on Systems, Signal Processing and their Applications, WOSSPA, Tipaza, Algeria, 9–11 May 2011; pp. 243–246. [Google Scholar]
- Yue, S.; Niu, B.; Wang, H.; Zhang, L.; Ahmad, A.M. Hierarchical sliding mode-based adaptive fuzzy control for uncertain switched under-actuated nonlinear systems with input saturation and dead-zone. Robot. Intell. Autom. 2023, 43, 523–536. [Google Scholar] [CrossRef]
- Min-xiu, Y.; Yuan-wei, J. Terminal sliding mode decomposed control for a class of nonlinear systems. In Proceedings of the 2008 Chinese Control and Decision Conference, Yantai, China, 2–4 July 2008; pp. 4988–4991. [Google Scholar]
- Bayramoglu, H.; Komurcugil, H. Nonsingular decoupled terminal sliding-mode control for a class of fourth-order nonlinear systems. Commun. Nonlinear Sci. Numer. Simul. 2013, 18, 2527–2539. [Google Scholar] [CrossRef]
- Edwards, C.; Shtessel, Y.B. Adaptive continuous higher order sliding mode control. Automatica 2016, 65, 183–190. [Google Scholar] [CrossRef]
- Wang, H.; Li, Z.; Jin, X.; Huang, Y.; Kong, H.; Yu, M.; Ping, Z.; Sun, Z. Adaptive integral terminal sliding mode control for automobile electronic throttle via an uncertainty observer and experimental validation. IEEE Trans. Veh. Technol. 2018, 67, 8129–8143. [Google Scholar] [CrossRef]
- Liu, W.; Chen, S.; Huang, H. Actor-Critic learning hierarchical sliding mode control for a class of underactuated systems. In Proceedings of the 2019 Chinese Automation Congress (CAC), Hangzhou, China, 22–24 November 2019; pp. 1–6. [Google Scholar]
- Li, S.; Yang, J.; Chen, W.H.; Chen, X. Generalized extended state observer based control for systems with mismatched uncertainties. IEEE Trans. Ind. Electron. 2011, 59, 4792–4802. [Google Scholar] [CrossRef]
- Czyżniewski, M.; Łangowski, R.; Klassa, D.; Matwiszyn, M. A case study of robust sliding mode control applied to inverted pendulum on a cart. In Proceedings of the 2021 25th International Conference on Methods and Models in Automation and Robotics (MMAR), Miedzyzdroje, Poland, 23–26 August 2021; pp. 156–161. [Google Scholar]
- Haimo, V.T. Finite time controllers. SIAM J. Control Optim. 1986, 24, 760–770. [Google Scholar] [CrossRef]
- Qian, D.; Yi, J.; Zhao, D.; Hao, Y. Hierarchical sliding mode control for series double inverted pendulums system. In Proceedings of the 2006 IEEE/RSJ International Conference on Intelligent Robots and Systems, Beijing, China, 9–15 October 2006; pp. 4977–4982. [Google Scholar]
- Chiu, C.S. Derivative and integral terminal sliding mode control for a class of MIMO nonlinear systems. Automatica 2012, 48, 316–326. [Google Scholar] [CrossRef]
- Bartoszewicz, A. A new reaching law for sliding mode control of continuous time systems with constraints. Trans. Inst. Meas. Control 2015, 37, 515–521. [Google Scholar] [CrossRef]
- Yue, M.; Liu, B. Adaptive control of an underactuated spherical robot with a dynamic stable equilibrium point using hierarchical sliding mode approach. Int. J. Adapt. Control Signal Process. 2014, 28, 523–535. [Google Scholar] [CrossRef]
- Qian, D.; Yi, J.; Zhao, D. Multiple layers sliding mode control for a class of under-actuated systems. In Proceedings of the Multiconference on Computational Engineering in Systems Applications, Beijing, China, 4–6 October 2006; Volume 1, pp. 530–535. [Google Scholar]
- Wang, H.; Shi, L.; Man, Z.; Zheng, J.; Li, S.; Yu, M.; Jiang, C.; Kong, H.; Cao, Z. Continuous fast nonsingular terminal sliding mode control of automotive electronic throttle systems using finite-time exact observer. IEEE Trans. Ind. Electron. 2018, 65, 7160–7172. [Google Scholar] [CrossRef]
- Gao, Z. Scaling and bandwidth-parameterization based controller tuning. In Proceedings of the ACC, Denver, CO, USA, 4–6 June 2003; pp. 4989–4996. [Google Scholar]
- Sussmann, H.; Kokotovic, P. The peaking phenomenon and the global stabilization of nonlinear systems. IEEE Trans. Autom. Control 1991, 36, 424–440. [Google Scholar] [CrossRef]
- Huang, Y.; Han, J. Analysis and design for the second order nonlinear continuous extended states observer. Chin. Sci. Bull. 2000, 45, 1938–1944. [Google Scholar] [CrossRef]
- Wang, J.; Wu, Y.; Dong, X. Recursive terminal sliding mode control for hypersonic flight vehicle with sliding mode disturbance observer. Nonlinear Dyn. 2015, 81, 1489–1510. [Google Scholar] [CrossRef]
- Shi, S.L.; Li, J.X.; Fang, Y.M. Extended-state-observer-based chattering free sliding mode control for nonlinear systems with mismatched disturbance. IEEE Access 2018, 6, 22952–22957. [Google Scholar] [CrossRef]
- González, I.; Salazar, S.; Lozano, R. Chattering-free sliding mode altitude control for a quad-rotor aircraft: Real-time application. J. Intell. Robot. Syst. 2014, 73, 137–155. [Google Scholar] [CrossRef]
- Liu, Q.; Lv, Z.; Wu, Y. Design of sliding mode controller based on high-gain observer of inverted pendulum on a cart. In Proceedings of the 2019 Chinese Control Conference (CCC), Guangzhou, China, 27–30 July 2019; pp. 846–851. [Google Scholar]
- Cui, M.; Liu, W.; Liu, H.; Jiang, H.; Wang, Z. Extended state observer-based adaptive sliding mode control of differential-driving mobile robot with uncertainties. Nonlinear Dyn. 2016, 83, 667–683. [Google Scholar] [CrossRef]
- Muhammad, S.; Idrees, M. Comparative study of hierarchical sliding mode control and decoupled sliding mode control. In Proceedings of the 2017 12th IEEE Conference on Industrial Electronics and Applications (ICIEA), Siem Reap, Cambodia, 18–20 June 2017; pp. 818–824. [Google Scholar]
- Guo, B.; Bacha, S.; Alamir, M.; Hably, A.; Boudinet, C. Generalized integrator-extended state observer with applications to grid-connected converters in the presence of disturbances. IEEE Trans. Control Syst. Technol. 2020, 29, 744–755. [Google Scholar] [CrossRef]
- Mahmoodabadi, M.J.; Khoobroo Haghbayan, H. An optimal adaptive hybrid controller for a fourth-order under-actuated nonlinear inverted pendulum system. Trans. Inst. Meas. Control 2020, 42, 285–294. [Google Scholar] [CrossRef]
- Haghighi, D.A.; Mobayen, S. Design of an adaptive super-twisting decoupled terminal sliding mode control scheme for a class of fourth-order systems. ISA Trans. 2018, 75, 216–225. [Google Scholar] [CrossRef] [PubMed]
- Boukattaya, M.; Gassara, H.; Damak, T. A global time-varying sliding-mode control for the tracking problem of uncertain dynamical systems. ISA Trans. 2020, 97, 155–170. [Google Scholar] [CrossRef] [PubMed]
- Mei, K.; Ding, S. Second-order sliding mode controller design subject to an upper-triangular structure. IEEE Trans. Syst. Man Cybern. Syst. 2018, 51, 497–507. [Google Scholar] [CrossRef]
- Qiao, L.; Zhang, W. Trajectory tracking control of AUVs via adaptive fast nonsingular integral terminal sliding mode control. IEEE Trans. Ind. Inform. 2019, 16, 1248–1258. [Google Scholar] [CrossRef]
- Yao, Q. Adaptive finite-time sliding mode control design for finite-time fault-tolerant trajectory tracking of marine vehicles with input saturation. J. Frankl. Inst. 2020, 357, 13593–13619. [Google Scholar] [CrossRef]
- Sai, H.; Xu, Z.; He, S.; Zhang, E.; Zhu, L. Adaptive nonsingular fixed-time sliding mode control for uncertain robotic manipulators under actuator saturation. ISA Trans. 2022, 123, 46–60. [Google Scholar] [CrossRef] [PubMed]
- Li, H.; Yang, S.; Kong, L.; Wen, T. High-precision angular speed tracking control of gimbal system with harmonic reducer. IEEE Trans. Ind. Electron. 2021, 69, 8168–8177. [Google Scholar] [CrossRef]
- Lau, J.Y.; Liang, W.; Tan, K.K. Motion control for piezoelectric-actuator-based surgical device using neural network and extended state observer. IEEE Trans. Ind. Electron. 2019, 67, 402–412. [Google Scholar] [CrossRef]




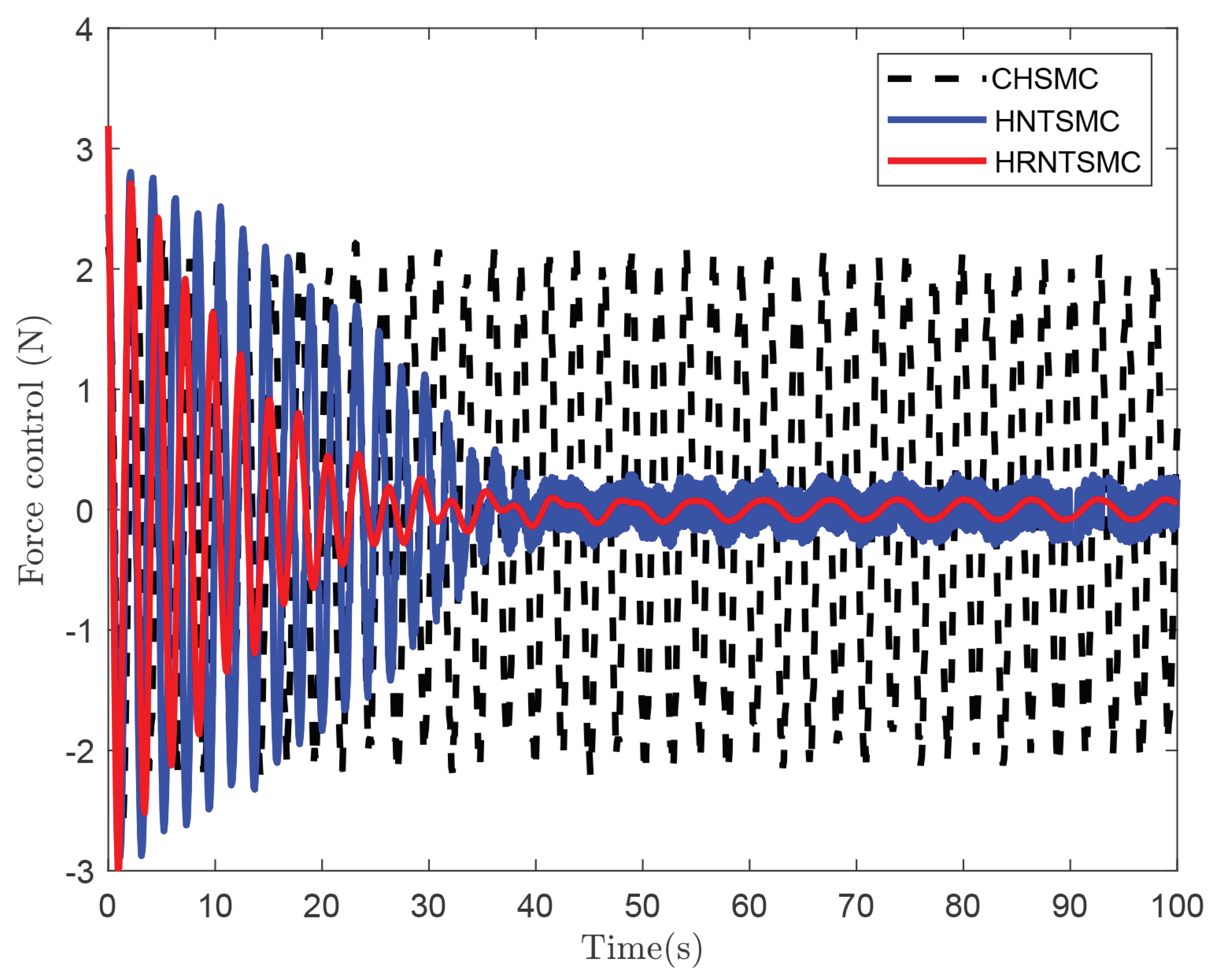

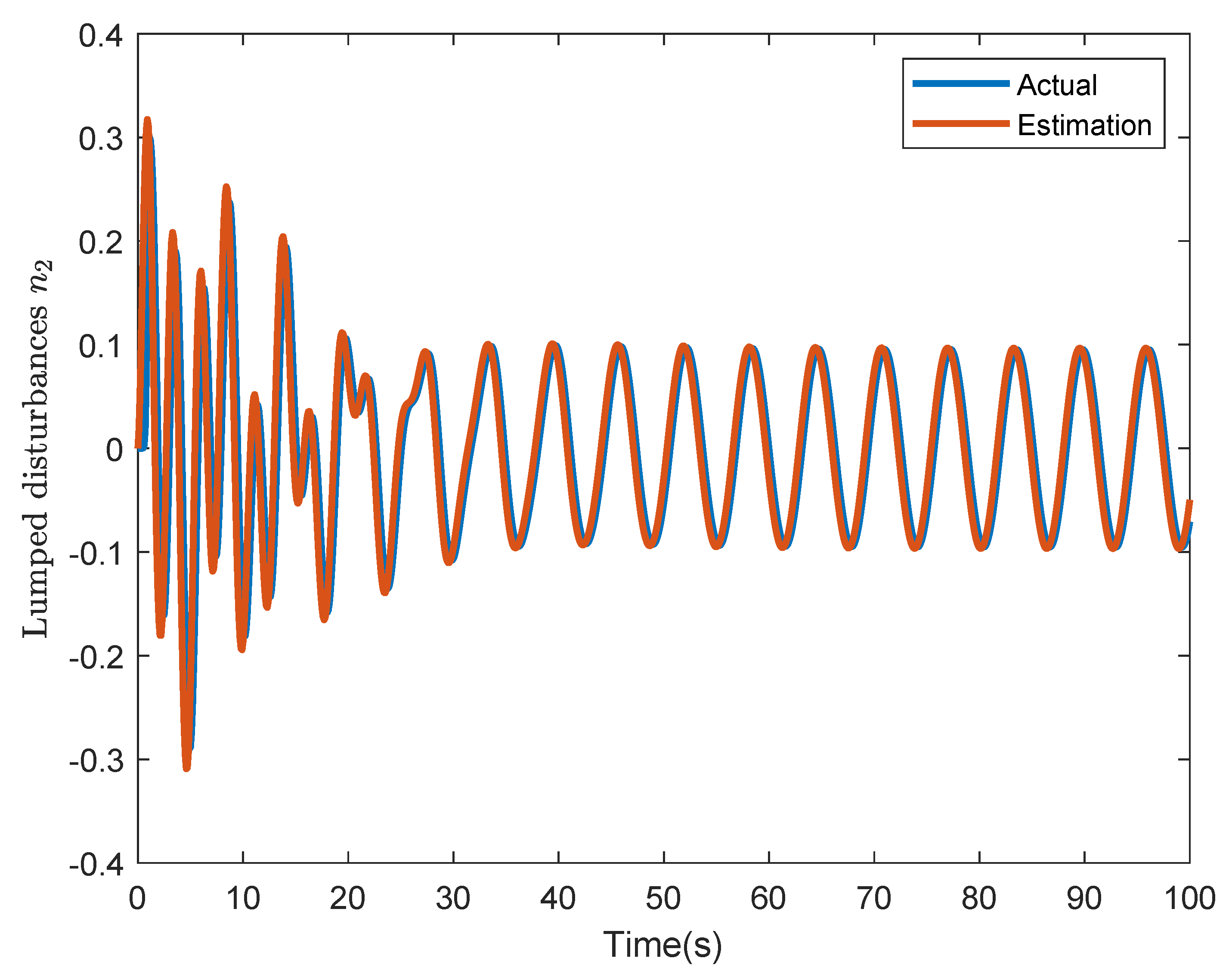

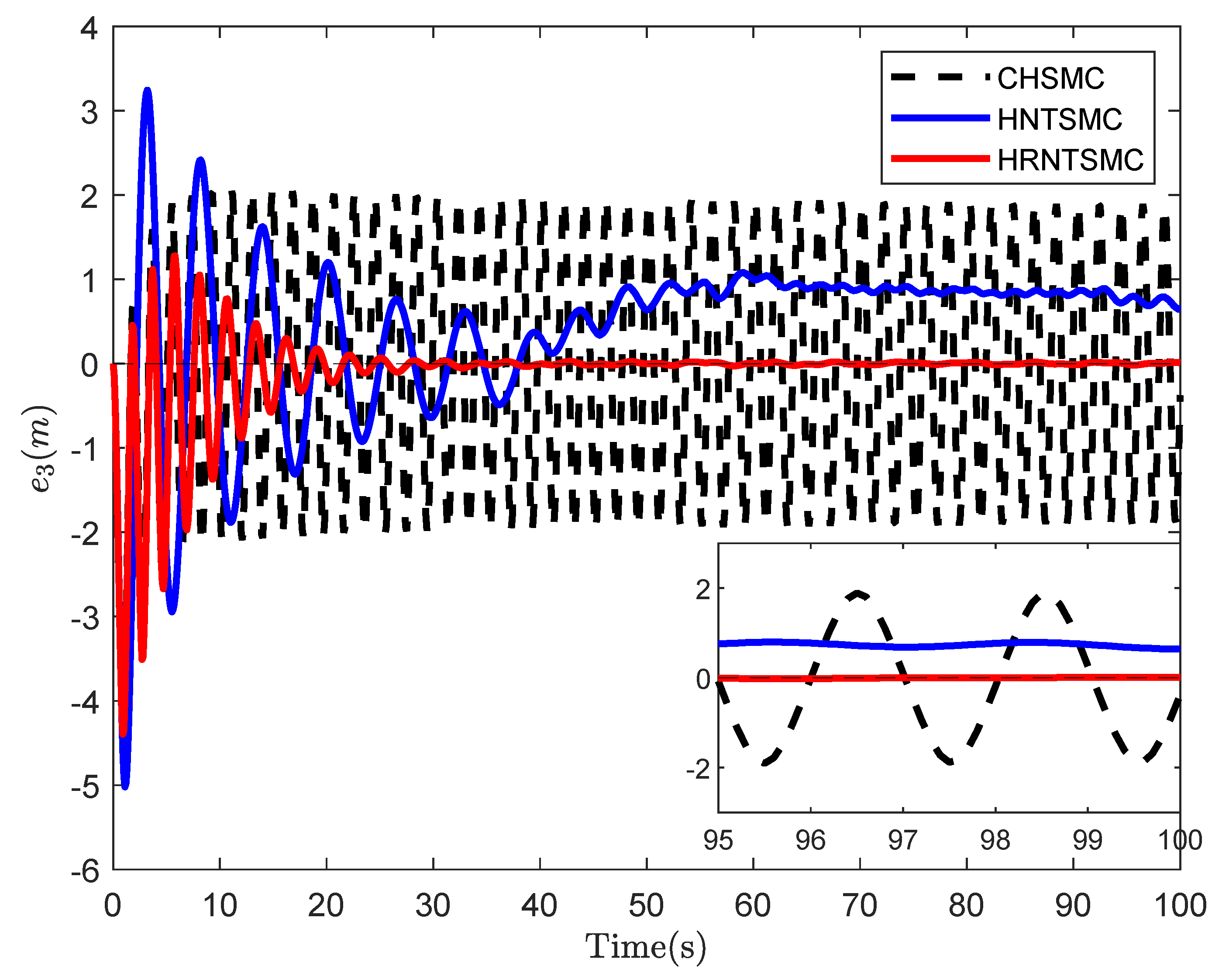

| Controller | Tuning Parameters |
|---|---|
| CHSMC | |
| HNTSMC | |
| HRNTSMC |
| Controller | Tuning Parameters |
|---|---|
| CHSMC | |
| HNTSMC | |
| HRNTSMC |
| IAE | IAE | ECI | AECI | |
|---|---|---|---|---|
| CHSMC | 0.1214 | 0.2445 | 1.1972 | 0.3294 |
| HNTSMC | 0.0381 | 0.0601 | 0.5212 | 0.2814 |
| HRNTSMC | 0.0217 | 0.0448 | 0.2156 | 0.0605 |
| IAE | IAE | ECI | AECI | |
|---|---|---|---|---|
| CHSMC | 0.1303 | 0.2629 | 1.2877 | 0.3322 |
| HNTSMC | 0.0411 | 0.0651 | 0.5471 | 0.3117 |
| HRNTSMC | 0.0263 | 0.0555 | 0.2672 | 0.0629 |
| IAE | IAE | ECI | AECI | |
|---|---|---|---|---|
| CHSMC | 0.6058 | 1.2271 | 9.2318 | 4.0046 |
| HNTSMC | 0.0807 | 0.8674 | 0.9029 | 0.6959 |
| HRNTSMC | 0.0746 | 0.1816 | 1.0154 | 0.4238 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Le, H.D.; Nestorović, T. A Novel Hierarchical Recursive Nonsingular Terminal Sliding Mode Control for Inverted Pendulum. Actuators 2023, 12, 462. https://doi.org/10.3390/act12120462
Le HD, Nestorović T. A Novel Hierarchical Recursive Nonsingular Terminal Sliding Mode Control for Inverted Pendulum. Actuators. 2023; 12(12):462. https://doi.org/10.3390/act12120462
Chicago/Turabian StyleLe, Hiep Dai, and Tamara Nestorović. 2023. "A Novel Hierarchical Recursive Nonsingular Terminal Sliding Mode Control for Inverted Pendulum" Actuators 12, no. 12: 462. https://doi.org/10.3390/act12120462
APA StyleLe, H. D., & Nestorović, T. (2023). A Novel Hierarchical Recursive Nonsingular Terminal Sliding Mode Control for Inverted Pendulum. Actuators, 12(12), 462. https://doi.org/10.3390/act12120462








