Design and Assembly of a Thin-Plate Mechatronic Atomizer by 3D Printing
Abstract
:1. Introduction
2. Design and 3D Printing
2.1. Design of a Microfluidic Structure
2.2. Design of a Microfluidic Structure
3. Experimental
3.1. Fused Deposition of Filament Material
3.2. Assembly of a Microfluidic Mechatronic Device
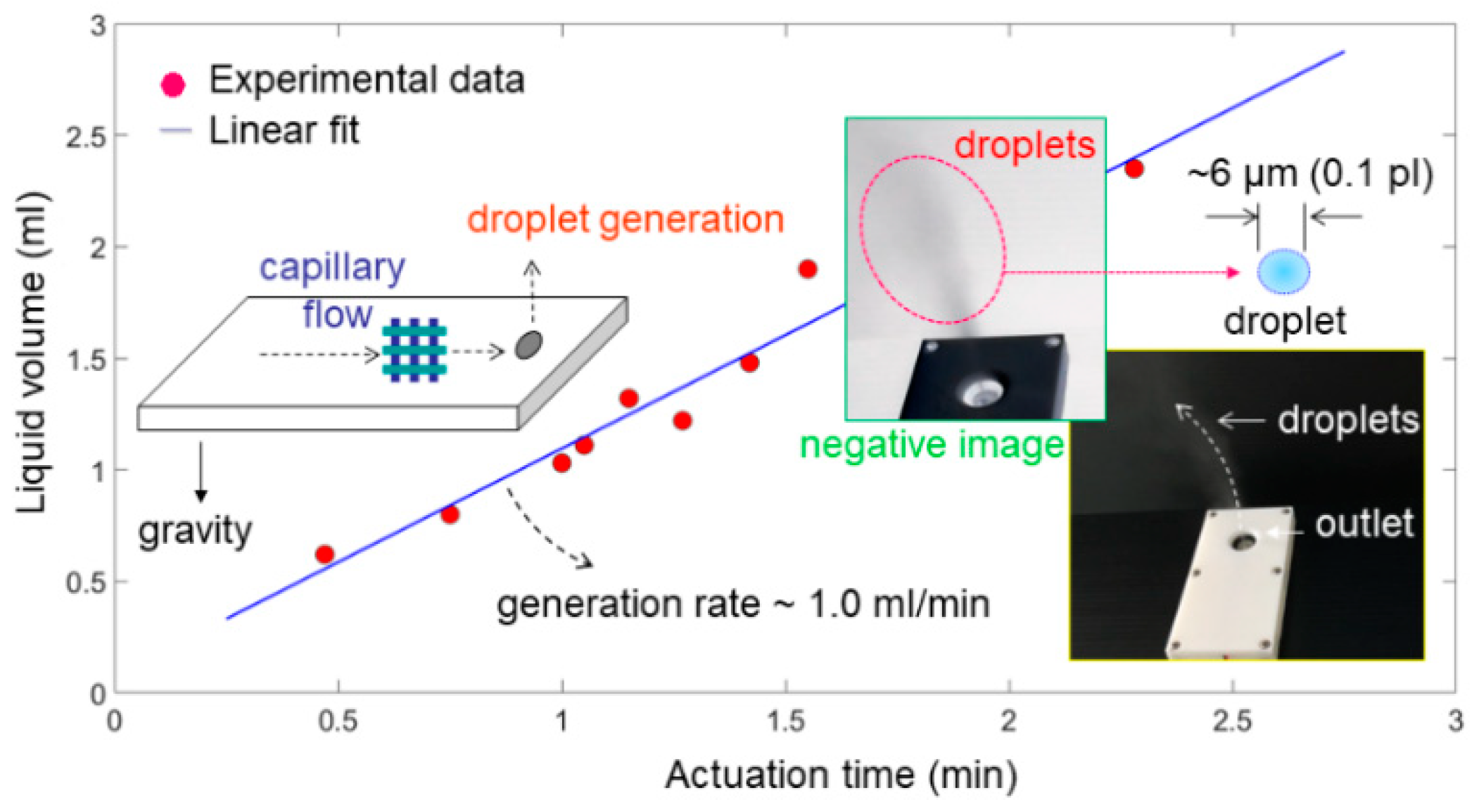
3.3. Tests for Generation Rate of Droplets
4. Discussion and Analysis
4.1. Reduction in Surface Roughness
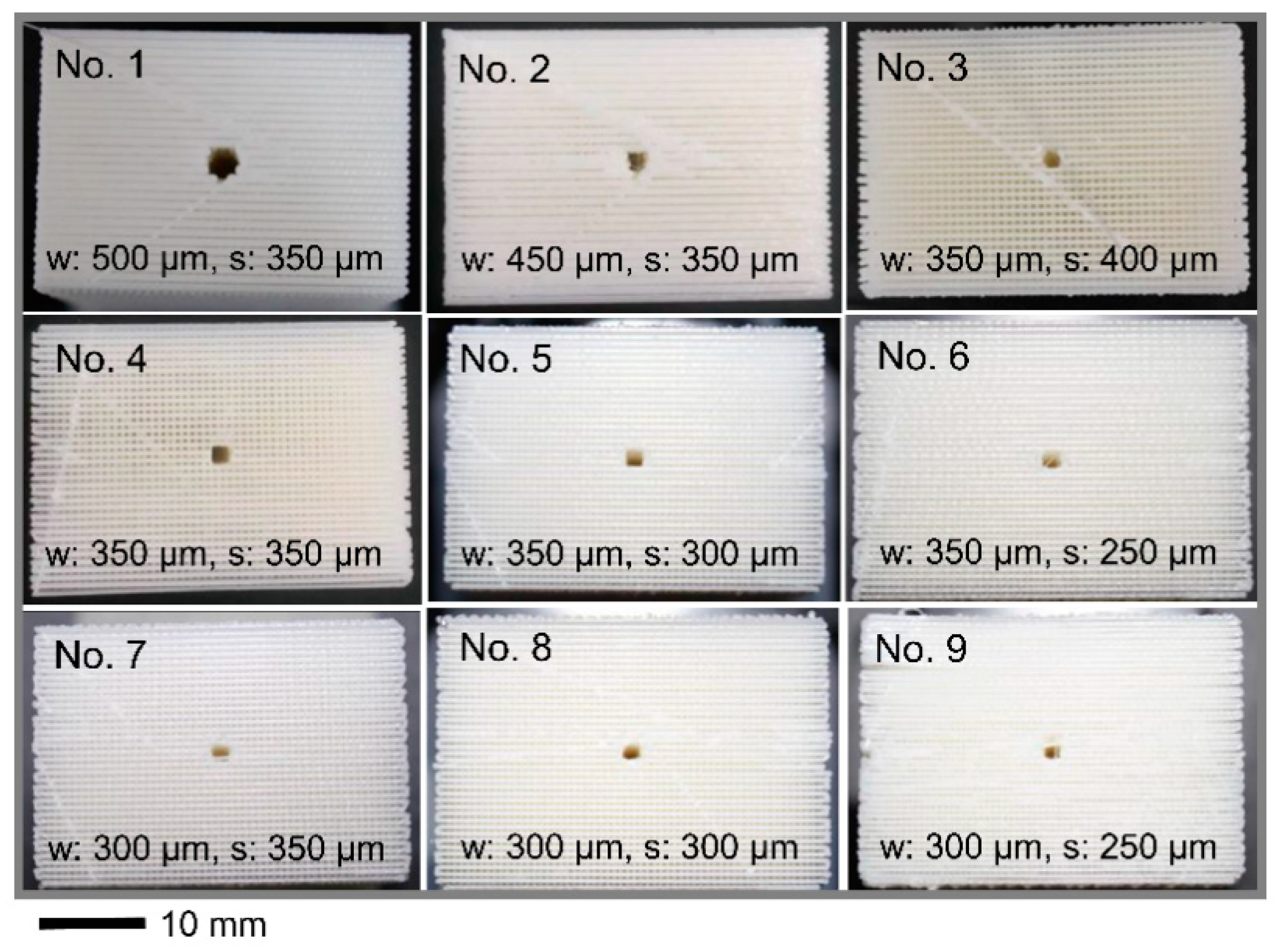
4.2. Characteristics of the Grid Structures
4.3. Capillary Liquid Flow within a Grid Structure
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Verpoorte, E.; De Rooij, N.F. Microfluidics meets MEMS. Proc. IEEE 2003, 91, 930–953. [Google Scholar] [CrossRef] [Green Version]
- Yoon, Y.K.; Park, J.H.; Allen, M.G. Multidirectional UV lithography for complex 3-D MEMS structures. J. Microelectromech. Syst. 2006, 15, 1121–1130. [Google Scholar] [CrossRef]
- Zhang, J.; Tan, K.L.; Hong, G.D.; Yang, L.J.; Gong, H.Q. Polymerization optimization of SU-8 photoresist and its applications in microfluidic systems and MEMS. J. Micromech. Microeng. 2001, 11, 20–26. [Google Scholar] [CrossRef]
- Tsai, N.C.; Sue, C.Y. Review of MEMS-based drug delivery and dosing systems. Sens. Actuators A-Phys. 2007, 134, 555–564. [Google Scholar] [CrossRef]
- Liu, C. Recent development in polymer MEMS. Adv. Mater. 2007, 19, 3783–3790. [Google Scholar] [CrossRef]
- Fachin, F.; Chen, G.D.; Toner, M.; Wardle, B.L. Integration of bulk nanoporous elements in microfluidic devices with applications to biomedical diagnostics. J. Microelectromech. Syst. 2011, 20, 1428–1438. [Google Scholar] [CrossRef]
- Lee, K.B.; Lin, L. Surface micromachined glass and polysilicon microchannels using MUMPs for BioMEMS applications. Sens. Actuators A-Phys. 2004, 111, 44–50. [Google Scholar] [CrossRef]
- Lifton, V.A.; Simon, S.; Holmqvist, J.; Ebefors, T.; Jansson, D.; Svedin, K. Design and fabrication of addressable microfluidic energy storage MEMS device. J. Microelectromech. Syst. 2012, 21, 1392–1400. [Google Scholar] [CrossRef]
- Chen, C.T.; Tsai, T.W. Multilayer polymer light guides integrated with refractive microlenses and liquid by micromolding and inkjet printing. Sens. Actuators A-Phys. 2016, 111, 252–260. [Google Scholar] [CrossRef]
- Guo, N.; Leu, M.C. Additive manufacturing: Technology, applications and research needs. Front. Mech. Eng. 2013, 8, 215–243. [Google Scholar] [CrossRef]
- Li, F.; Macdonald, N.P.; Guijt, R.M.; Breadmore, M.C. Microfluidic chips made by fused deposition modeling 3D printing. Anal. Chem. 2017, 89, 12805–12811. [Google Scholar] [CrossRef] [PubMed]
- Xing, J.F.; Zheng, M.L.; Duan, X.M. Two-photon polymerization microfabrication of hydrogels: An advanced 3D printing technology for tissue engineering and drug delivery. Chem. Soc. Rev. 2015, 44, 5031–5039. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lee, J.Y.; Tan, W.S.; An, J.; Chua, C.K.; Tang, C.Y.; Fane, A.G.; Chong, T.H. The potential to enhance membrane module design with 3D printing technology. J. Membr. Sci. 2016, 499, 480–490. [Google Scholar] [CrossRef]
- Lifton, V.A.; Lifton, G.; Simon, S. Options for additive rapid prototyping methods (3D printing) in MEMS technology. Rapid Prototyp. J. 2014, 20, 403–412. [Google Scholar] [CrossRef]
- Parekh, D.; Ladd, C.; Panich, L.; Moussa, K.; Dickey, M. 3D printing of liquid metals as fugitive inks for fabrication of 3D microfluidic channels. Lab Chip 2016, 16, 1812–1820. [Google Scholar] [CrossRef]
- Ozbolat, V.; Dey, M.; Ayan, B.; Ozbolat, I.T. Extrusion-based printing of sacrificial Carbopol ink for fabrication of microfluidic devices. Biofabrication 2019, 11, 034101. [Google Scholar] [CrossRef]
- Helmer, D.; Voigt, A.; Wagner, S.; Keller, N.; Sachsenheimer, K.; Kotz, F.; Nargang, T.M.; Rapp, B.E. Suspended liquid subtractive lithography: One-step generation of 3D channel geometries in viscous curable polymer matrices. Sci. Rep. 2017, 7, 7387. [Google Scholar] [CrossRef]
- Gong, H.; Bickham, B.P.; Woolley, A.T.; Nordin, G.P. Custom 3D printer and resin for 18 μm × 20 μm microfluidic flow channels. Lab Chip 2017, 17, 2899–2909. [Google Scholar] [CrossRef]
- Cooley, P.; Wallace, D.; Antohe, B. Applications of ink-jet printing technology to bioMEMS and microfluidic systems. Proc. SPIE 2001, 4560, 177–188. [Google Scholar]
- Hwang, Y.; Paydar, O.H.; Candler, R.N. 3D printed molds for non-planar PDMS microfluidic channels. Sens. Actuators A-Phys. 2015, 226, 137–142. [Google Scholar] [CrossRef]
- Serien, D.; Morimoto, Y.; Takeuchi, S. Photo-induced fabrication technology for 3D microdevices. In Advanced Mechatronics and MEMS Devices II; Zhang, D., Wei, B., Eds.; Springer: Cham, Switzerland, 2017; pp. 469–494. [Google Scholar]
- Kumar, S.; Bhushan, P.; Pandey, M.; Bhattacharya, S. Additive manufacturing as an emerging technology for fabrication of micoelectromechanical systems (MEMS). J. Micromanuf. 2019, 2, 175–197. [Google Scholar] [CrossRef]
- Snelling, D.; Li, Q.; Meisel, N.; Willaims, C.B.; Batra, R.C.; Druschitz, A.P. Lightweight metal cellular structures fabricated via 3D printing of sand cast molds. Adv. Eng. Mater. 2015, 17, 923–932. [Google Scholar] [CrossRef]
- Chen, C.T.; Hsu, S.F. AM of three-dimensional spongy microstrucures for a piezoelectric sprayer. Micro Nano Lett. 2018, 13, 1662–1666. [Google Scholar] [CrossRef]
- Kotz, F.; Mader, M.; Dellen, N.; Risch, P.; Kick, A.; Helmer, D.; Rapp, B.E. Fused deposition modeling of microfluidic chips in polymethylmethacrylate. Micromachines 2020, 11, 873. [Google Scholar] [CrossRef] [PubMed]
- Mostafaei, A.; Elliott, A.M.; Barnes, J.E.; Li, F.; Tan, F.; Cramer, C.L.; Mandwana, P.; Chmielus, M. Binder jet 3D printing—Process parameters, materials, properties, and challenges. Prog. Mater. Sci. 2020. [Google Scholar] [CrossRef]
- Wu, D.; Zhao, Z.; Zhang, Q.; Qi, H.J.; Fang, D. Mechanics of shape distortion of DLP 3D printed structures during UV post-curing. Soft Matter 2019, 15, 6151–6159. [Google Scholar] [CrossRef]
- Al-Ketan, O.; Pelanconi, M.; Ortona, A.; Abu Al-Rub, R.K. Additive manufacturing of architected catalytic ceramic substrates based on triple periodic minimal surfaces. J. Am. Ceram. Soc. 2019, 102, 6176–6193. [Google Scholar] [CrossRef]
- Chen, C.T.; Huang, C.C. Microelectroforming and evaluation of honeycomb-groove nozzle plates of piezoelectric actuators for microspray generation. J. Micro/Nanolithogr. MEMS MOEMS 2016, 15, 035002. [Google Scholar] [CrossRef]
- Zhang, Y.; Ge, S.; Yu, J. Chemical and biochemical analysis on lab-on-a-chip devices fabricated using three-dimensional printing. Trends Anal. Chem. 2016, 85, 166–190. [Google Scholar] [CrossRef]
- Khalid, N.; Kobayashi, I.; Nakajima, M. Recent lab-on-chip developments for novel drug discovery. WIREs Syst. Biol. Med. 2017, 9, e1381. [Google Scholar]
- Metcalf, A.R.; Narayan, S.; Dutcher, C.S. A review of microfluidic concepts and applications for atmospheric aerosol science. Aerosol Sci. Tech. 2018, 52, 310–329. [Google Scholar] [CrossRef]
- Wallin, M.; Tagami, T.; Chen, L.; Yang, M.; Chan, H.K. Pulmonary drug delivery to older people. Adv. Drug Deliv. Rev. 2018, 135, 50–61. [Google Scholar] [CrossRef]
- Yan, X.; Yu, M.; Ramakrishna, S.; Russell, S.J.; Long, Y.Z. Advances in portable electrospinning devices for in situ delivery of personalized would care. Nanoscale 2019, 11, 19166–19178. [Google Scholar] [CrossRef]
- Farzal, Z.; Basu, S.; Burke, A.; Bs, O.O.F.; Lopez, E.M.; Bennett, W.D.; Ebert, C.S.; Zanation, A.M.; Senior, B.A.; Kimbell, J.S. Comparative study of simulated nebulized and spray particle deposition in chronic rhinosinusitis patients. Int. Forum Allergy Rhinol. 2019, 9, 746–758. [Google Scholar] [CrossRef] [Green Version]
- Navascues, G. Liquid surfaces: Theory of surface tension. Rep. Prog. Phys. 1979, 42, 1131–1186. [Google Scholar] [CrossRef]
- Avinc, O.; Khoddami, A. Overview of poly(lactic acid) (PLA) fibre. Fibre Chem. 2009, 41, 391–401. [Google Scholar] [CrossRef]
- Layer One Company (ATOM). Available online: https://atom3dp.com/en/ (accessed on 1 October 2020).
- Chen, H.; Cheng, W.; Peng, Y.; Zhang, W.; Jiang, L. Experimental study on optimal spray parameters of piezoelectric atomizer based spray cooling. Int. J. Heat Mass Transf. 2016, 103, 57–65. [Google Scholar] [CrossRef] [Green Version]
- Baran, E.H.; Erbil, H.Y. Surface modification of 3D printed PLA objects by fused deposition modeling: A review. Colloids Interfaces 2019, 3, 43. [Google Scholar] [CrossRef] [Green Version]
- Waldbaur, A.; Kittelmann, J.; Padtke, C.P.; Hubbuch, J.; Rapp, B.E. Microfluidics on liquid handling stations (μF-on-LHS): An industry compatible chip interface between microfluidics and automated liquid handling stations. Lab Chip 2013, 13, 2337–2343. [Google Scholar] [CrossRef]
- Li, M.; Lai, C.; Zheng, Q.; Han, B.; Wu, H.; Fan, H. Design and mechanical properties of hierarchical isogrid structures validated by 3D printing technique. Mater. Des. 2019, 168, 107664. [Google Scholar] [CrossRef]
- Ahmed, K.; Shiblee, N.I.; Khosla, A.; Nagahara, L.; Thundat, T.; Furukawa, H. Review- recent progress in 4D printing of gel materials. J. Electrochem. Soc. 2020, 167, 037563. [Google Scholar] [CrossRef]
- Maurel, A.; Grugeon, S.; Fleutot, B.; Courty, M.; Prashantha, K.; Tortajada, H.; Armand, M.; Panier, S.; Dupont, L. Three-dimensional printing of a LiFePO4/graphite battery cell via fused deposition modeling. Sci. Rep. 2019, 9, 18031. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, Y. Post-printing surface modification and functionalization of 3D-printed biomedical device. Int. J. Bioprinting 2017, 3, 93–99. [Google Scholar] [CrossRef] [Green Version]
- Olanrewaju, A.; Beaugrand, M.; Yafla, M.; Juncker, D. Capillary microfluidics in microchannels: From microfluidic networks to capillaric circuits. Lab Chip 2018, 18, 2323–2347. [Google Scholar] [CrossRef] [Green Version]







| Sample Number (No.) | Estimated Line Width, w (μm) | Estimated Spacing, s (μm) | Estimated Solid Volume, VMA (mL) | Estimated Void Volume, VVD (mL) | Porosity of Total Volume 1 (%) | Theoretical Laplace Pressure, Δp (Pa) | Theoretical Capillary Height (cm) | Theoretica lLiquid Volume 2 (mL) | Estimated Liquid Volume (mL) | Error in Liquid Volume 3 (%) |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 656 | 195 | 37.40 | 11.60 | 24 | 307 | 3.1 | 6.17 | 6.53 | 6 |
| 2 | 621 | 185 | 38.33 | 10.67 | 22 | 324 | 3.3 | 5.98 | 7.36 | 23 |
| 3 | 321 | 463 | 20.30 | 28.70 | 59 | 129 | 1.3 | 6.42 | 8.14 | 27 |
| 4 | 351 | 361 | 22.06 | 26.94 | 55 | 166 | 1.7 | 7.72 | 7.63 | 1 |
| 5 | 363 | 314 | 23.51 | 25.49 | 52 | 191 | 1.9 | 8.41 | 9.63 | 15 |
| 6 | 317 | 284 | 25.44 | 23.56 | 48 | 211 | 2.1 | 8.59 | 9.88 | 15 |
| 7 | 308 | 332 | 22.10 | 26.90 | 55 | 181 | 1.8 | 8.40 | 8.73 | 4 |
| 8 | 334 | 274 | 24.15 | 24.85 | 51 | 218 | 2.2 | 9.38 | 9.45 | 1 |
| 9 | 301 | 277 | 25.06 | 23.94 | 49 | 216 | 2.2 | 8.96 | 9.89 | 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, C.-T.; Hsu, H.-F. Design and Assembly of a Thin-Plate Mechatronic Atomizer by 3D Printing. Actuators 2020, 9, 110. https://doi.org/10.3390/act9040110
Chen C-T, Hsu H-F. Design and Assembly of a Thin-Plate Mechatronic Atomizer by 3D Printing. Actuators. 2020; 9(4):110. https://doi.org/10.3390/act9040110
Chicago/Turabian StyleChen, Chin-Tai, and Hsin-Fang Hsu. 2020. "Design and Assembly of a Thin-Plate Mechatronic Atomizer by 3D Printing" Actuators 9, no. 4: 110. https://doi.org/10.3390/act9040110
APA StyleChen, C.-T., & Hsu, H.-F. (2020). Design and Assembly of a Thin-Plate Mechatronic Atomizer by 3D Printing. Actuators, 9(4), 110. https://doi.org/10.3390/act9040110





