Evaluation of Thermal Indices as the Indicators of Heat Stress in Dairy Cows in a Temperate Climate
Abstract
:Simple Summary
Abstract
1. Introduction
2. Materials and Methods
2.1. Cows, Housing, and Management
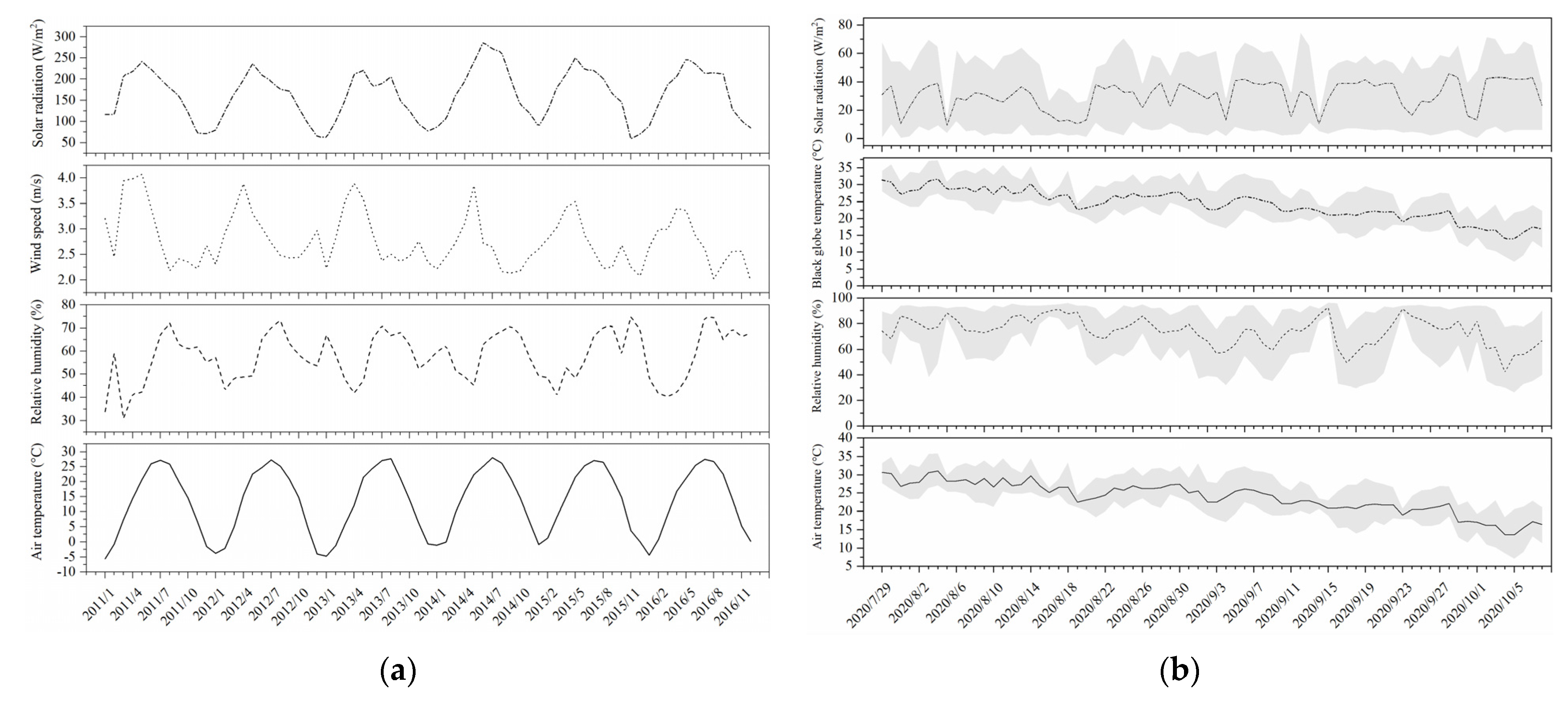
2.2. Environmental Parameters and Thermal Indices of Heat Stress
2.3. Animal-Based Indicators of Heat Stress
2.4. Statistical Analysis
2.4.1. Equivalent Air Temperature Change
2.4.2. Analysis of Variance
2.4.3. Correlation Analysis
3. Results
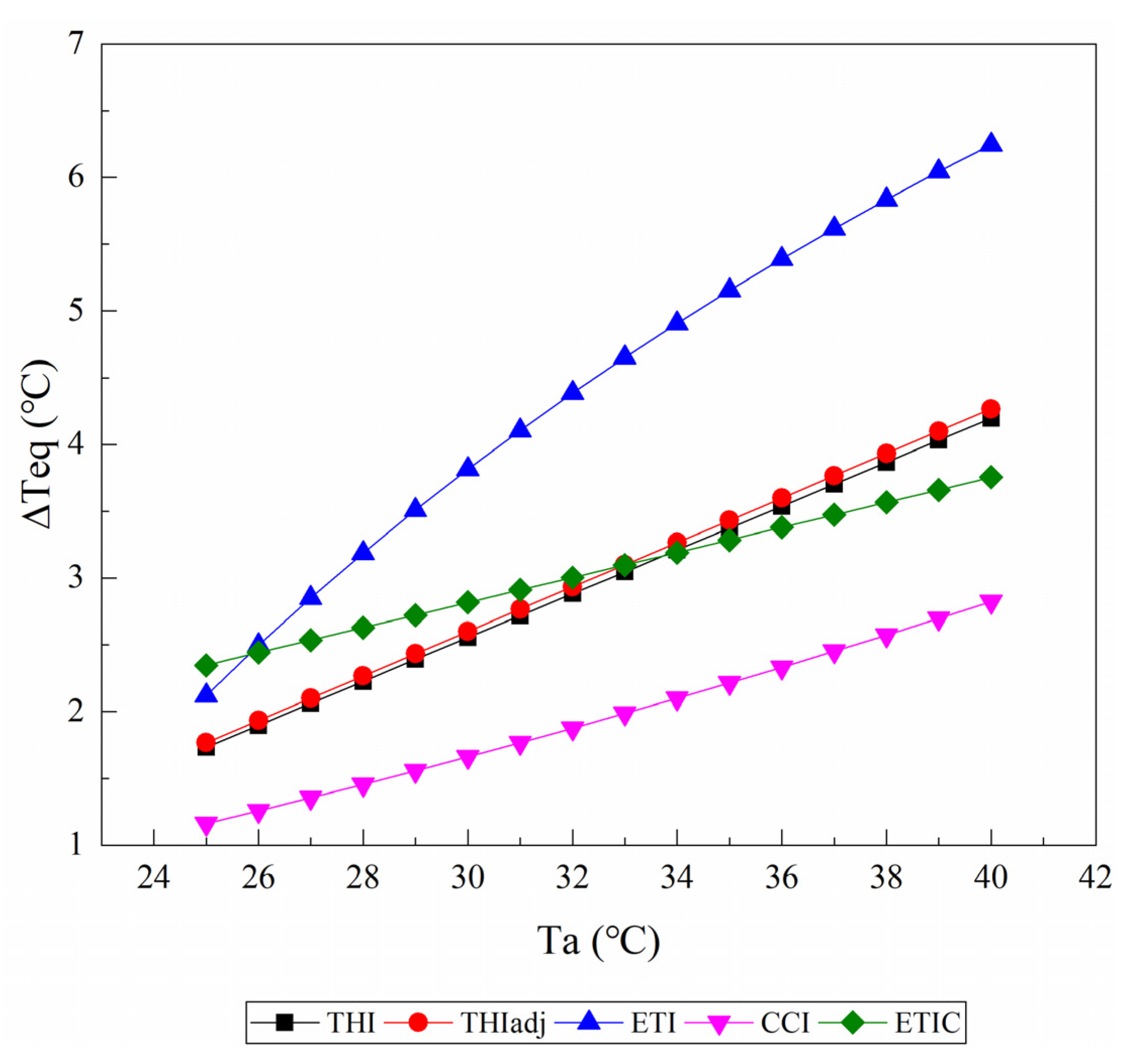
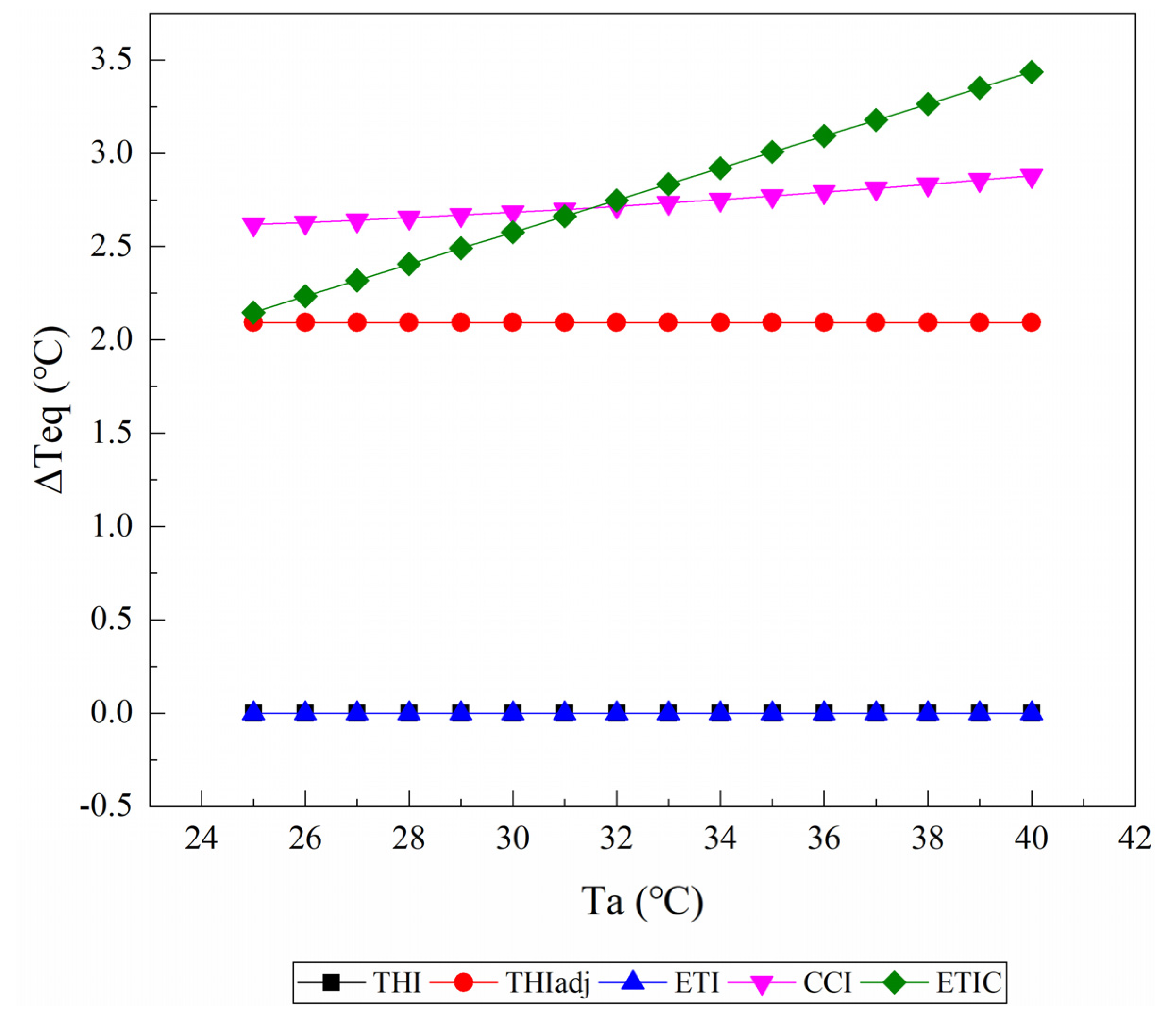
3.1. Equivalent Ambient Temperature Change
3.1.1. Relative Humidity
3.1.2. Wind Speed
3.1.3. Solar Radiation
3.2. Effect of the TI Values on the Animal-Based Indicators
3.2.1. Rectal Temperature
3.2.2. Respiration Rate
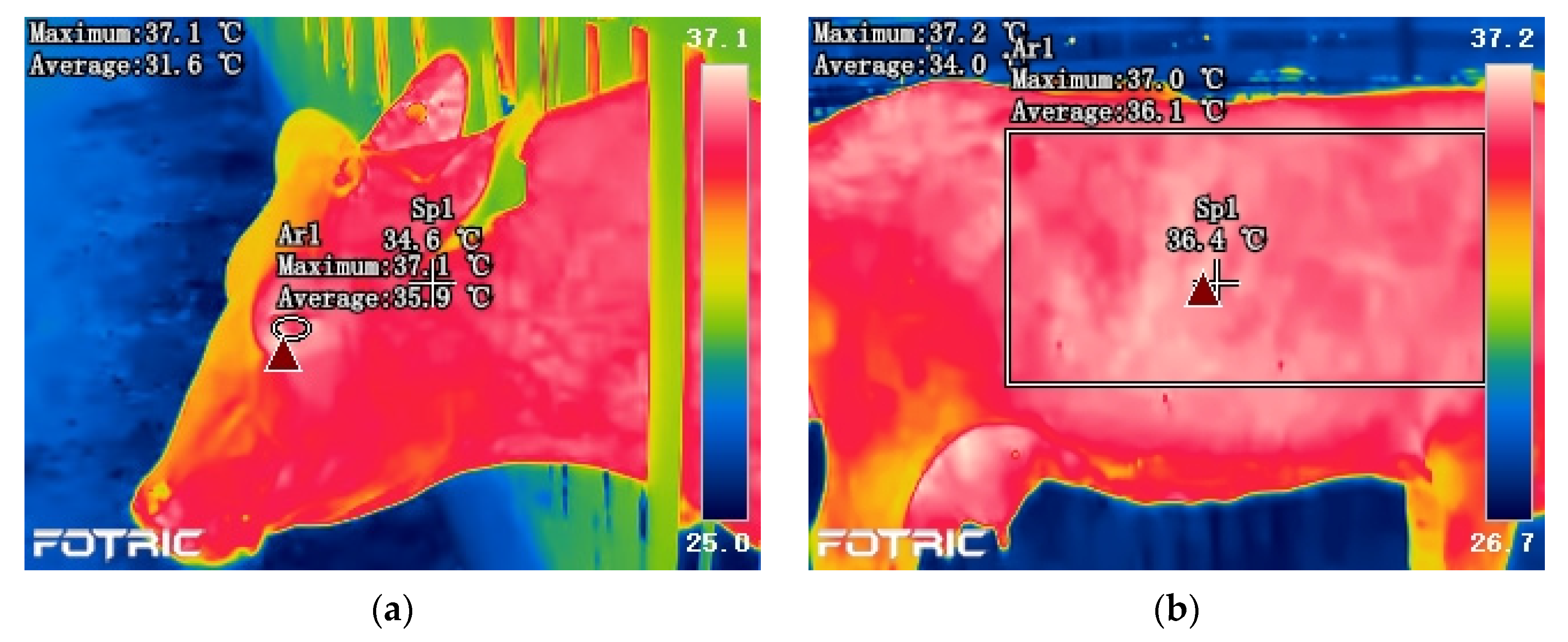
3.2.3. Skin Temperature
3.2.4. Eye Temperature
3.3. Correlations between Indices and Animal-Based Indicators
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Hoffmann, G.; Herbut, P.; Pinto, S.; Heinicke, J.; Kuhla, B.; Amon, T. Animal-related, non-invasive indicators for determining heat stress in dairy cows. Biosyst. Eng. 2020, 199, 83–96. [Google Scholar] [CrossRef]
- Polsky, L.; von Keyserlingk, M.A. Invited review: Effects of heat stress on dairy cattle welfare. J. Dairy. Sci. 2017, 100, 8645–8657. [Google Scholar] [CrossRef] [Green Version]
- West, J.W. Effects of heat-stress on production in dairy cattle. J. Dairy. Sci. 2003, 86, 2131–2144. [Google Scholar] [CrossRef]
- Wang, T.; Zhong, R.; Zhou, D. Temporal–spatial distribution of risky sites for feeding cattle in China based on temperature/humidity index. Agriculture 2020, 10, 571. [Google Scholar] [CrossRef]
- Yan, G.; Liu, K.; Hao, Z.; Shi, Z.; Li, H. The effects of cow-related factors on rectal temperature, respiration rate, and temperature-humidity index thresholds for lactating cows exposed to heat stress. J. Therm. Biol. 2021, 100, 103041. [Google Scholar] [CrossRef]
- Becker, C.A.; Collier, R.J.; Stone, A.E. Invited review: Physiological and behavioral effects of heat stress in dairy cows. J. Dairy. Sci. 2020, 103, 6751–6770. [Google Scholar] [CrossRef]
- Berman, A.; Folman, Y.; Kaim, M.; Mamen, M.; Herz, Z.; Wolfenson, D.; Arieli, A.; Graber, Y. Upper critical temperatures and forced ventilation effects for high-yielding dairy cows in a subtropical climate. J. Dairy. Sci. 1985, 68, 1488–1495. [Google Scholar] [CrossRef]
- Thom, E.C. The discomfort index. Weatherwise 1959, 12, 57–61. [Google Scholar] [CrossRef]
- Hahn, G.L.; Gaughan, J.B.; Mader, T.L.; Eigenberg, R.A. Chapter 5: Thermal indices and their applications for livestock environments. In Livestock Energetics and Thermal Environmental Management; DeShazer, J.A., Ed.; American Society of Agricultural and Biological Engineers: St. Joseph, MI, USA, 2009; pp. 113–130. [Google Scholar]
- Bohmanova, J.; Misztal, I.; Cole, J.B. Temperature-humidity indices as indicators of milk production losses due to heat stress. J. Dairy. Sci. 2007, 90, 1947–1956. [Google Scholar] [CrossRef] [PubMed]
- Dikmen, S.; Hansen, P.J. Is the temperature-humidity index the best indicator of heat stress in lactating dairy cows in a subtropical environment? J. Dairy. Sci. 2009, 92, 109–116. [Google Scholar] [CrossRef] [Green Version]
- Heinicke, J.; Hoffmann, G.; Ammon, C.; Amon, B.; Amon, T. Effects of the daily heat load duration exceeding determined heat load thresholds on activity traits of lactating dairy cows. J. Therm. Biol. 2018, 77, 67–74. [Google Scholar] [CrossRef]
- Pinto, S.; Hoffmann, G.; Ammon, C.; Amon, T. Critical THI thresholds based on the physiological parameters of lactating dairy cows. J. Therm. Biol. 2020, 88, 102523. [Google Scholar] [CrossRef]
- Buffington, D.E.; Collazo-Arocho, A.; Canton, G.H.; Pitt, D.; Thatcher, W.W.; Collier, R.J. Black globe-humidity index (BGHI) as comfort equation for dairy cows. Trans. ASABE 1981, 24, 711–714. [Google Scholar] [CrossRef]
- Mader, T.L.; Davis, M.S.; Brown-Brandl, T. Environmental factors influencing heat stress in feedlot cattle. J. Anim. Sci. 2006, 84, 712–719. [Google Scholar] [CrossRef] [Green Version]
- Baeta, F.C.; Meador, N.F.; Shanklin, M.D.; Johnson, H.D. Equivalent temperature index at temperatures above the thermoneutral for lactating dairy cows. In Proceedings of the International Winter Meeting of American Society of Agricultural Engineers (ASAE), Chicago, IL, USA. Available online: https://agris.fao.org/agris-search/search.do?recordID=US8853966 (accessed on 15 February 2021).
- Mader, T.L.; Johnson, L.J.; Gaughan, J.B. A comprehensive index for assessing environmental stress in animals. J. Anim. Sci. 2010, 88, 2153–2165. [Google Scholar] [CrossRef] [Green Version]
- Wang, X.; Gao, H.; Gebremedhin, K.G.; Bjerg, B.S.; van Os, J.; Tucker, C.B.; Zhang, G. A predictive model of equivalent temperature index for dairy cattle (ETIC). J. Therm. Biol. 2018, 76, 165–170. [Google Scholar] [CrossRef] [PubMed]
- Ji, B.; Banhazi, T.; Perano, K.; Ghahramani, A.; Bowtell, L.; Wang, C.; Li, B. A review of measuring, assessing and mitigating heat stress in dairy cattle. Biosyst. Eng. 2020, 199, 4–26. [Google Scholar] [CrossRef]
- Romanovsky, A.A. Skin temperature: Its role in thermoregulation. Acta. Physiol. 2014, 210, 498–507. [Google Scholar] [CrossRef]
- Godyń, D.; Herbut, P.; Angrecka, S. Measurements of peripheral and deep body temperature in cattle—A review. J. Therm. Biol. 2019, 79, 42–49. [Google Scholar] [CrossRef] [PubMed]
- McManus, C.; Tanure, C.B.; Peripolli, V.; Seixas, L.; Fischer, V.; Gabbi, A.M.; Menegassi, S.R.O.; Stumpf, M.T.; Kolling, G.J.; Dias, E.; et al. Infrared thermography in animal production: An overview. Comput. Electron. Agric. 2016, 123, 10–16. [Google Scholar] [CrossRef]
- Hoffmann, G.; Schmidt, M.; Ammon, C.; Rose-Meierhöfer, S.; Burfeind, O.; Heuwieser, W.; Berg, W. Monitoring the body temperature of cows and calves using video recordings from an infrared thermography camera. Vet. Res. Commun. 2013, 37, 91–99. [Google Scholar] [CrossRef] [PubMed]
- Armstrong, D.V. Heat Stress Interaction with Shade and Cooling. J. Dairy. Sci. 1994, 77, 2044–2050. [Google Scholar] [CrossRef]
- Da Silva, R.G.; Morais, D.A.E.F.; Guilhermino, M.M. Evaluation of thermal stress indexes for dairy cows in tropical regions. Rev. Bras. de Zootec. 2007, 36, 1192–1198. [Google Scholar] [CrossRef] [Green Version]
- Macmillan, K.; Colazo, M.G.; Cook, N.J. Evaluation of infrared thermography compared to rectal temperature to identify illness in early postpartum dairy cows. Res. Vet. Sci. 2019, 125, 315–322. [Google Scholar] [CrossRef] [PubMed]
- Montanholi, Y.R.; Lim, M.; Macdonald, A.; Smith, B.A.; Goldhawk, C.; Schwartzkopf-Genswein, K.; Miller, S.P. Technological, environmental and biological factors: Referent variance values for infrared imaging of the bovine. J. Anim. Sci. Biotechno. 2015, 6, 1–16. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Bjerg, B.S.; Choi, C.Y.; Zong, C.; Zhang, G. A review and quantitative assessment of cattle-related thermal indices. J. Therm. Biol. 2018, 77, 24–37. [Google Scholar] [CrossRef]
- Diedenhofen, B.; Musch, J. cocor: A comprehensive solution for the statistical comparison of correlations. PLoS ONE 2015, 10, e0121945. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bjerg, B.; Wang, X.; Zhang, G. The effect of air velocity on heat stress at increased air temperature. In Proceedings of the CIGR-AgEng Conference, Aarhus, Denmark, 26–29 June 2016. [Google Scholar]
- Collier, R.J.; Gebremedhin, K.G. Thermal biology of domestic animals. Annu. Rev. Anim. Biosci. 2015, 3, 513–532. [Google Scholar] [CrossRef] [PubMed]
- Stewart, M.; Webster, J.R.; Schaefer, A.L.; Cook, N.J.; Scott, S.L. Infrared thermography as a non-invasive tool to study animal welfare. Anim. Welf. 2005, 14, 319–325. [Google Scholar]
- Yan, G.; Li, H.; Zhao, W.; Shi, Z. Evaluation of thermal indices based on their relationships with some physiological responses of housed lactating cows under heat stress. Int. J. Biometeorol. 2020, 64, 2077–2091. [Google Scholar] [CrossRef]
- Da Silva, R.G.; Maia, A.S.C.; de Macedo Costa, L.L. Index of thermal stress for cows (ITSC) under high solar radiation in tropical environments. Int. J. Biometeorol. 2015, 59, 551–559. [Google Scholar] [CrossRef] [PubMed]
- Li, S.; Gebremedhin, K.G.; Lee, C.; Collier, R. Evaluation of thermal stress indices for cattle. In Proceedings of the ASABE Annual Meeting, Reno, NV, USA, 21–24 June 2009; American Society of Agricultural and Biological Engineers: St. Joseph, MI, USA, 2009; p. 1. [Google Scholar] [CrossRef]
- Fournel, S.; Rousseau, A.N.; Laberge, B. Rethinking environment control strategy of confined animal housing systems through precision livestock farming. Biosyst. Eng. 2017, 155, 96–123. [Google Scholar] [CrossRef]
- Yan, G.; Li, H.; Shi, Z.; Wang, C. Research status and existing problems in establishing cow heat stress indices. Trans. Chin. Soc. Agric. Eng. 2019, 35, 226–233. [Google Scholar]
- Ji, B.; Banhazi, T.; Ghahramani, A.; Bowtell, L.; Wang, C.; Li, B. Modelling of heat stress in a robotic dairy farm. Part 1: Thermal comfort indices as the indicators of production loss. Biosyst. Eng. 2020, 199, 27–42. [Google Scholar] [CrossRef]





| Item | Number | Minimum | Maximum | Mean | Standard Deviation |
|---|---|---|---|---|---|
| Air temperature (°C) | 3005 | 13.55 | 36.00 | 26.33 | 4.72 |
| Relative humidity (%) | 3005 | 27.57 | 91.70 | 69.75 | 15.89 |
| Black globe temperature (°C) | 3005 | 13.55 | 36.70 | 26.93 | 4.86 |
| Wind speed (m/s) | 3005 | 0 | 4.50 | 2.50 | 0.90 |
| Solar radiation (W/m2) | 3005 | 0 | 64.30 | 24.68 | 13.42 |
| Temperature–humidity index (THI1) 1 | 3005 | 56.76 | 87.48 | 75.68 | 6.87 |
| Temperature–humidity index (THI2) 1 | 3005 | 57.08 | 86.31 | 75.35 | 6.35 |
| Temperature–humidity index (THI3) 1 | 3005 | 57.02 | 86.44 | 74.99 | 6.28 |
| Black globe humidity index (BGHI) | 3005 | 57.02 | 87.70 | 75.59 | 6.38 |
| Ajusted THI (THIadj) | 3005 | 59.30 | 89.23 | 75.34 | 6.47 |
| Equivalent tempeprature index (ETI) | 3005 | 14.18 | 41.64 | 28.93 | 6.12 |
| Comprehensive climate index (CCI) | 3005 | 13.91 | 40.13 | 27.16 | 5.62 |
| Equivalent temperature index for dairy cattle (ETIC) | 3005 | 8.40 | 29.68 | 20.63 | 4.76 |
| Item | Number | Minimum | Maximum | Mean | Standard Deviation |
|---|---|---|---|---|---|
| Rectal temperature (°C) | 3005 | 37.87 | 41.4 | 38.92 | 0.47 |
| Respiration rate (bpm) | 3005 | 19 | 114 | 62.77 | 19.34 |
| Skin temperature (°C) | 2996 | 30.5 | 40.7 | 35.23 | 1.67 |
| Eye temperature (°C) | 2924 | 33.4 | 39.6 | 36.41 | 0.96 |
| Variable | Statistic | THI1 | THI2 | THI3 | BGHI | THIadj | ETI | CCI | ETIC | RT | RR | ST | ET |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| THI1 | r | 1 | 0.998 | 0.996 | 0.995 | 0.966 | 0.987 | 0.947 | 0.984 | 0.643 | 0.843 | 0.793 | 0.572 |
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | ||
| THI2 | r | 1 | 0.999 | 0.996 | 0.962 | 0.980 | 0.943 | 0.985 | 0.640 | 0.844 | 0.792 | 0.574 | |
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | |||
| THI3 | r | 1 | 0.999 | 0.961 | 0.974 | 0.946 | 0.984 | 0.645 | 0.847 | 0.801 | 0.586 | ||
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | ||||
| BGHI | r | 1 | 0.961 | 0.970 | 0.948 | 0.982 | 0.649 | 0.846 | 0.809 | 0.598 | |||
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | |||||
| THIadj | r | 1 | 0.980 | 0.994 | 0.990 | 0.667 | 0.837 | 0.827 | 0.586 | ||||
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | ||||||
| ETI | r | 1 | 0.958 | 0.984 | 0.640 | 0.828 | 0.782 | 0.546 | |||||
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | |||||||
| CCI | r | 1 | 0.980 | 0.676 | 0.833 | 0.845 | 0.617 | ||||||
| p | <0.01 | <0.01 | <0.01 | <0.01 | <0.01 | ||||||||
| ETIC | r | 1 | 0.662 | 0.850 | 0.820 | 0.592 | |||||||
| p | <0.01 | <0.01 | <0.01 | <0.01 | |||||||||
| RT | r | 1 | 0.741 | 0.681 | 0.574 | ||||||||
| p | <0.01 | <0.01 | <0.01 | ||||||||||
| RR | r | 1 | 0.775 | 0.598 | |||||||||
| p | <0.01 | <0.01 | |||||||||||
| ST | r | 1 | 0.715 | ||||||||||
| p | <0.01 | ||||||||||||
| ET | r | 1 |
| Variable | RT | RR | ST | ET | ||||
|---|---|---|---|---|---|---|---|---|
| Hotelling’s t (df = 3002) | p | Hotelling’s t (df = 3002) | p | Hotelling’s t (df = 2993) | p | Hotelling’s t (df = 2921) | p | |
| r.THI1 vs. r.THI2 | 3.3956 | 0.0007 | −1.6156 | 0.1063 | 1.4200 | 0.1557 | −2.0880 | 0.0369 |
| r.THI1 vs. r.THI3 | −1.6033 | 0.109 | −4.6097 | <0.0001 | −8.2067 | <0.0001 | −10.5800 | <0.0001 |
| r.THI1 vs. r.BGHI | −4.3239 | <0.0001 | −3.0837 | 0.0021 | −15.2100 | <0.0001 | −18.3000 | <0.0001 |
| r.THI1 vs. r.THIadj | −6.6583 | <0.0001 | 2.3754 | 0.0176 | −12.6980 | <0.0001 | −3.5820 | 0.0003 |
| r.THI1 vs. r.ETI | 1.3323 | 0.1829 | 9.4858 | <0.0001 | 6.1263 | <0.0001 | 10.7310 | <0.0001 |
| r.THI1 vs. r.CCI | −7.5368 | <0.0001 | 3.1935 | 0.0014 | −16.3540 | <0.0001 | −9.504 | <0.0001 |
| r.THI1 vs. r.ETIC | −7.7799 | <0.0001 | −4.0801 | <0.0001 | −14.5620 | <0.0001 | −7.5180 | <0.0001 |
| r.THI2 vs. r.THI3 | −8.0820 | <0.0001 | −6.9426 | <0.0001 | −19.3190 | <0.0001 | −18.8600 | <0.0001 |
| r.THI2 vs. r.BGHI | −7.2790 | <0.0001 | −2.2988 | 0.0216 | −18.3300 | <0.0001 | −18.9800 | <0.0001 |
| r.THI2 vs. r.THIadj | −7.2026 | <0.0001 | 2.6328 | 0.0085 | −12.1970 | <0.0001 | −2.9060 | 0.0037 |
| r.THI2 vs. r.ETI | 0.0000 | 1 | 8.1727 | <0.0001 | 4.4856 | <0.0001 | 9.2882 | <0.0001 |
| r.THI2 vs. r.CCI | −7.9281 | <0.0001 | 3.4025 | 0.0007 | −16.0650 | <0.0001 | −8.7500 | <0.0001 |
| r.THI2 vs. r.ETIC | −9.3260 | <0.0001 | −3.6130 | 0.0003 | −15.6510 | <0.0001 | −6.9840 | <0.0001 |
| r.THI3 vs. r.BGHI | −6.4730 | <0.0001 | 2.3047 | 0.0212 | −17.3100 | <0.0001 | −19.0900 | <0.0001 |
| r.THI3 vs. r.THIadj | −5.7939 | <0.0001 | 3.7366 | 0.0002 | −9.0665 | <0.0001 | 0.0000 | 1 |
| r.THI3 vs. r.ETI | 1.5757 | 0.1152 | 8.5904 | <0.0001 | 7.6148 | <0.0001 | 11.8080 | <0.0001 |
| r.THI3 vs. r.CCI | −7.0155 | <0.0001 | 4.4672 | <0.0001 | −13.6980 | <0.0001 | −6.4790 | <0.0001 |
| r.THI3 vs. r.ETIC | −6.9551 | <0.0001 | −1.7555 | 0.0793 | −10.1690 | <0.0001 | −2.2500 | 0.0245 |
| r.BGHI vs. r.THIadj | −4.7432 | <0.0001 | 3.3562 | 0.0008 | −6.2982 | <0.0001 | 2.9011 | 0.0037 |
| r.BGHI vs. r.ETI | 2.6504 | 0.0081 | 7.5636 | <0.0001 | 10.2609 | <0.0001 | 14.5390 | <0.0001 |
| r.BGHI vs. r.CCI | −6.2288 | <0.0001 | 4.2133 | <0.0001 | −11.4330 | <0.0001 | −4.0520 | 0.0001 |
| r.BGHI vs. r.ETIC | −5.0088 | <0.0001 | −2.2070 | 0.0274 | −5.5448 | <0.0001 | 2.1334 | 0.033 |
| r.THIadj vs. r.ETI | 9.9701 | <0.0001 | 4.5172 | <0.0001 | 22.6397 | <0.0001 | 13.5500 | <0.0001 |
| r.THIadj vs. r.CCI | −6.1202 | <0.0001 | 3.6567 | 0.0003 | −17.2370 | <0.0001 | −20.4900 | <0.0001 |
| r.THIadj vs. r.ETIC | 2.6003 | 0.0094 | −9.5786 | <0.0001 | 4.8173 | <0.0001 | −2.84500 | 0.0045 |
| r.ETI vs. r.CCI | −9.2415 | <0.0001 | −1.7398 | 0.082 | −22.6040 | <0.0001 | −17.1700 | <0.0001 |
| r.ETI vs. r.ETIC | −9.0234 | <0.0001 | −12.8430 | <0.0001 | −20.9370 | <0.0001 | −17.8300 | <0.0001 |
| r.CCI vs. r.ETIC | 5.2047 | <0.0001 | −8.8408 | <0.0001 | 12.8251 | <0.0001 | 8.6128 | <0.0001 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yan, G.; Li, H.; Shi, Z. Evaluation of Thermal Indices as the Indicators of Heat Stress in Dairy Cows in a Temperate Climate. Animals 2021, 11, 2459. https://doi.org/10.3390/ani11082459
Yan G, Li H, Shi Z. Evaluation of Thermal Indices as the Indicators of Heat Stress in Dairy Cows in a Temperate Climate. Animals. 2021; 11(8):2459. https://doi.org/10.3390/ani11082459
Chicago/Turabian StyleYan, Geqi, Hao Li, and Zhengxiang Shi. 2021. "Evaluation of Thermal Indices as the Indicators of Heat Stress in Dairy Cows in a Temperate Climate" Animals 11, no. 8: 2459. https://doi.org/10.3390/ani11082459
APA StyleYan, G., Li, H., & Shi, Z. (2021). Evaluation of Thermal Indices as the Indicators of Heat Stress in Dairy Cows in a Temperate Climate. Animals, 11(8), 2459. https://doi.org/10.3390/ani11082459







