Growth Modeling of the Giant Electric Ray Narcine entemedor in the Southern Gulf of California: Analyzing the Uncertainty of Three Data Sets
Abstract
:Simple Summary
Abstract
1. Introduction
2. Materials and Methods
2.1. Collection of Samples
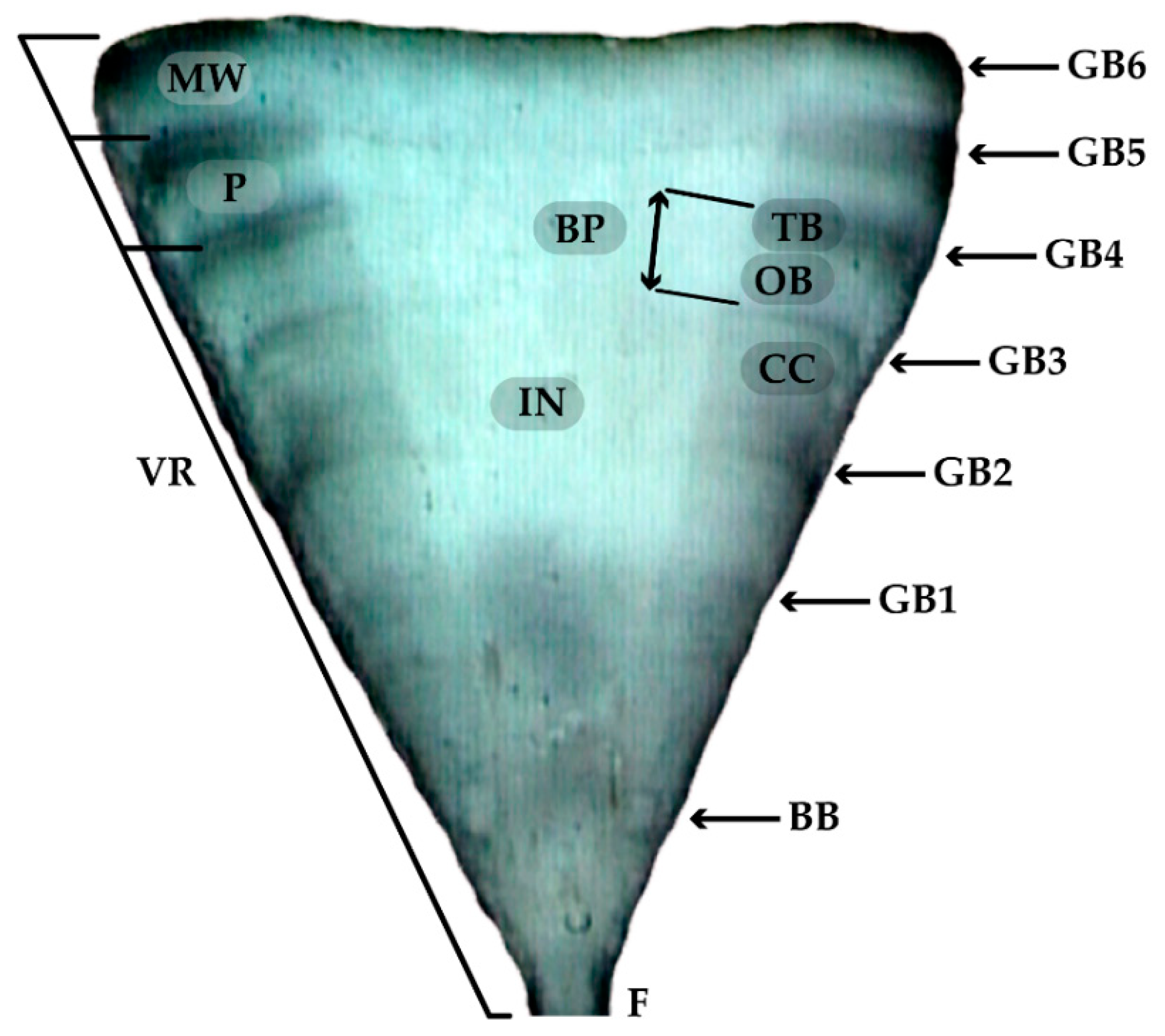
2.2. Vertebral Preparation
2.3. Reading of Growth Bands
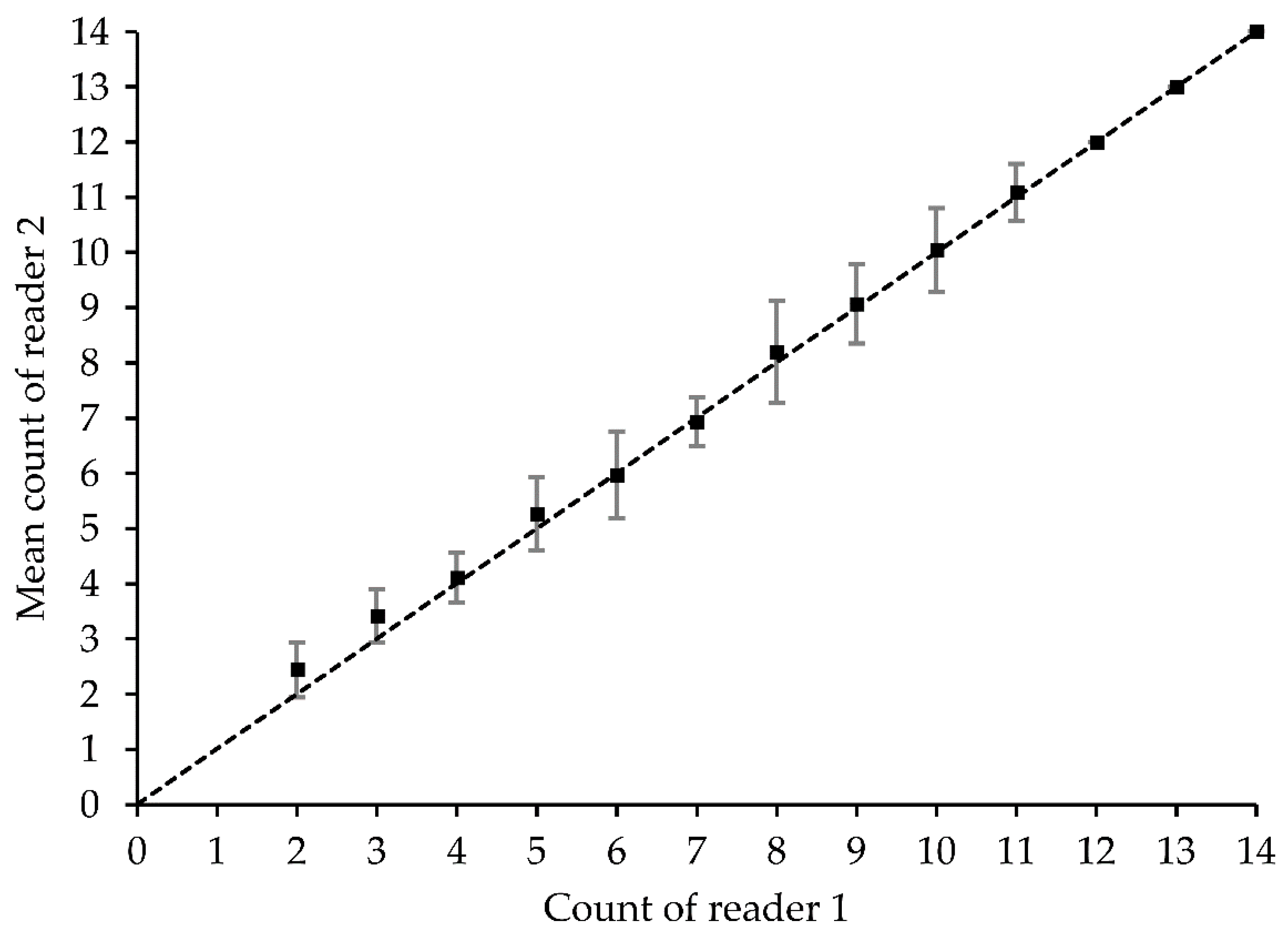
2.4. Precision and Accuracy
2.5. Periodicity of Band Formation
2.6. Age Adjustment
2.7. Back-Calculation
2.8. Growth Estimation
2.9. Confidence Intervals
2.10. Model Selection
3. Results
3.1. Collection of Samples
3.2. Precision and Accuracy
3.3. Periodicity of Band Formation
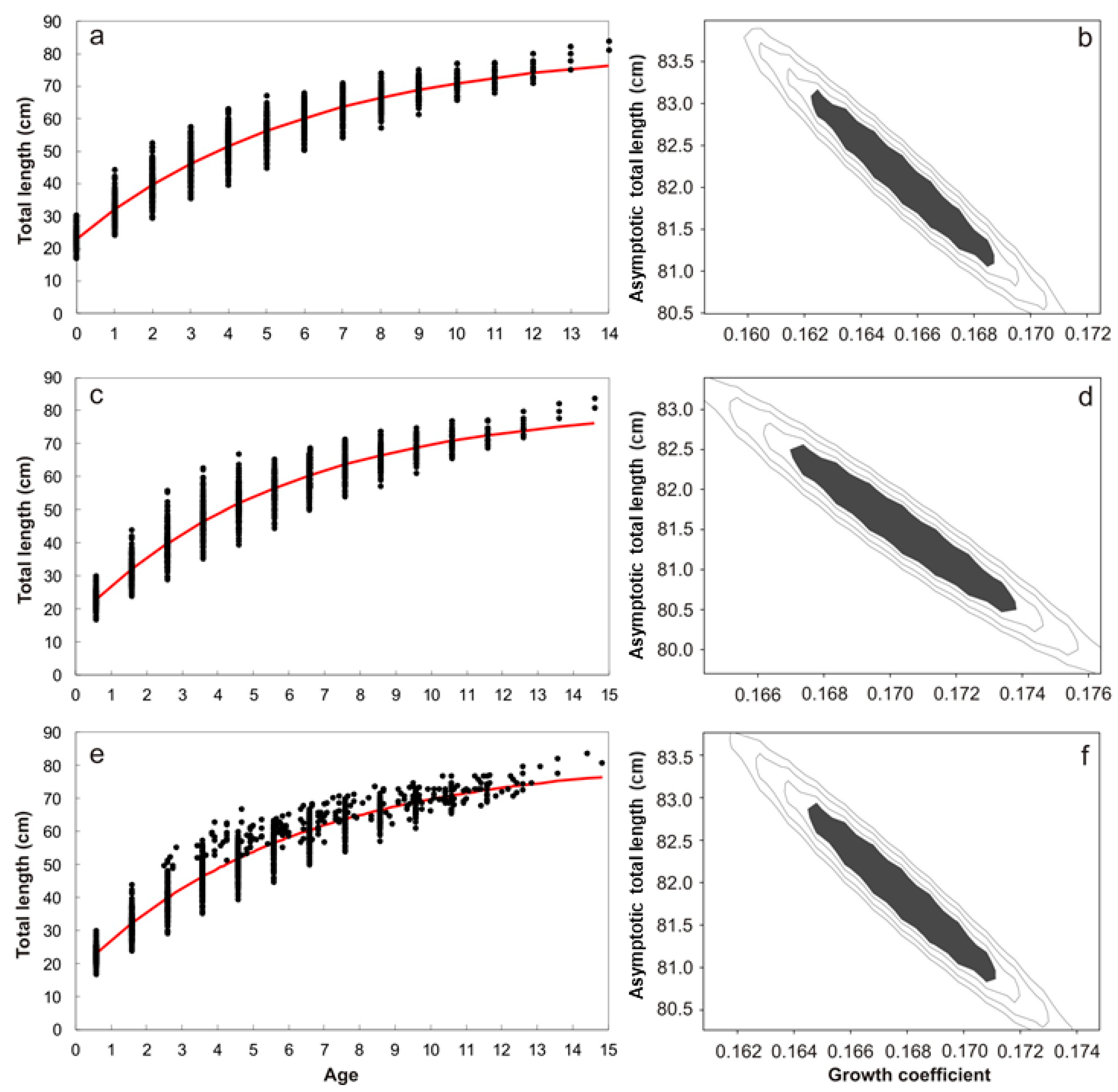
3.4. Growth Estimation and Model Selection
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Holden, M.J. Elasmobranchs. In Fish Population Dynamics; Gulland, J.A., Ed.; John Wiley & Sons: London, UK, 1977; pp. 187–215. [Google Scholar]
- Stevens, J.D. Variable Resilience to Fishing Pressure in Two Sharks: The Significance of Different Ecological and Life History Parameters. In Life in the Slow Lane: Ecology and Conservation of Long-Lived Marine Animals; Musick, J.A., Ed.; American Fisheries Society symposium: Bethesda, MD, USA, 1999; pp. 11–15. Available online: http://hdl.handle.net/102.100.100/214311?index=1 (accessed on 4 November 2021).
- Cortés, E.; Arocha, F.; Beerkircher, L.; Carvalho, F.; Domingo, A.; Heupel, M.; Holtzhausen, H.; Santos, M.N.; Ribera, M.; Simpfendorfer, C. Ecological Risk Assessment of Pelagic Sharks Caught in Atlantic Pelagic Longline Fisheries. Aquat. Living Resour. 2010, 23, 25–34. [Google Scholar] [CrossRef]
- Coelho, R.; Alpizar-Jara, R.; Erzini, K. Demography of a Deep-Sea Lantern Shark (Etmopterus spinax) Caught in Trawl Fisheries of the Northeastern Atlantic: Application of Leslie Matrices with Incorporated Uncertainties. Deep Sea Res. Part II Top. Stud. Oceanogr. 2015, 115, 64–72. [Google Scholar] [CrossRef] [Green Version]
- Walker, P. Sensitive Skates or Resilient Rays? Spatial and Temporal Shifts in Ray Species Composition in the Central and North-Western North Sea between 1930 and the Present Day. ICES J. Mar. Sci. 1998, 55, 392–402. [Google Scholar] [CrossRef]
- Simpfendorfer, C.A. Mortality Estimates and Demographic Analysis for the Australian Sharpnose Shark, Rhizoprionodon Taylori, from Northern Australia. Fish. Bull. 1999, 97, 978–986. [Google Scholar] [CrossRef]
- Mejía-Falla, P.A.; Cortés, E.; Navia, A.F.; Zapata, F.A. Age and Growth of the Round Stingray Urotrygon rogersi, a Particularly Fast-Growing and Short-Lived Elasmobranch. PLoS ONE 2014, 9, e96077. [Google Scholar] [CrossRef] [PubMed]
- Cailliet, G.; Mollet, H.; Pittenger, G.; Bedford, D.; Natanson, L. Growth and Demography of the Pacific Angle Shark (Squatina californica), Based upon Tag Returns off California. Mar. Freshw. Res. 1992, 43, 1313–1330. [Google Scholar] [CrossRef]
- Mollet, H.F.; Cailliet, G.M. Comparative Population Demography of Elasmobranchs Using Life History Tables, Leslie Matrices and Stage-Based Matrix Models. Mar. Freshw. Res. 2002, 53, 503–515. [Google Scholar] [CrossRef]
- Cortés, E. Chondrichthyan Demographic Modelling: An Essay on Its Use, Abuse and Future. Mar. Freshw. Res. 2007, 58, 4–6. [Google Scholar] [CrossRef]
- Cortés, E.; Brooks, E.N.; Gedamke, T. Population dynamics, demography, and stock assessment. In Biology of Sharks and Their Relatives; Carrier, J.C., Musick, J.A., Heithaus, M.R., Eds.; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2012; pp. 453–485. ISBN 084931514X. [Google Scholar]
- Pollerspöck, J.; Straube, N. Bibliography database of living/fossil sharks, rays and chimaeras (Chondrichthyes: Elasmobranchii, Holocephali) Papers of the Year 2018. Available online: https://shark-references.com/ (accessed on 9 December 2021).
- Cailliet, G.M.; Goldman, K.J. Age determination and validation in chondrichthyan fishes. In Biology of Sharks and Their Relatives; Carrier, J., Musick, J.A., Heithaus, M.R., Eds.; CRC Press: Boca Raton, FL, USA, 2004; pp. 399–439. ISBN 9780203491317. [Google Scholar]
- Goldman, K.; Cailliet, G.; Andrews, A.; Natanson, L. Assessing the age and growth of chondrichthyan fishes. In Biology of Sharks and Their Relatives; Carrier, J.C., Musick, J.A., Heithaus, M.R., Eds.; CRC Press: Boca Raton, FL, USA, 2012; pp. 423–451. [Google Scholar]
- Cortés, E. Incorporating Uncertainty into Demographic Modeling: Application to Shark Populations and Their Conservation. Conserv. Biol. 2002, 16, 1048–1062. [Google Scholar] [CrossRef]
- Dulvy, N.K.; Reynolds, J.D. Predicting Extinction Vulnerability in Skates. Conserv. Biol. 2002, 16, 440–450. [Google Scholar] [CrossRef] [Green Version]
- Dulvy, N.K.; Reynolds, J.D. Life history, population dynamics and extinction risks in chondrichthyans. In Biology of Sharks and Their Relatives II: Biodiversity, Adaptive Physiology, and Conservation; Carrier, J., Musick, J.A., Heithaus, M.R., Eds.; CRC Press, Taylor & Francis Group: Boca Raton, FL, USA, 2010; pp. 639–679. [Google Scholar]
- Cortés, E. Perspectives on the Intrinsic Rate of Population Growth. Methods Ecol. Evol. 2016, 7, 1136–1145. [Google Scholar] [CrossRef]
- Bizzarro, J.J.; Smith, W.D.; Hueter, R.E.; Tyminski, J.; Márquez-farias, J.F.; Castillo-Géniz, J.L.; Cailliet, G.M.; Villavicencio Garayzar, C.J. El Estado Actual de Los Tiburones y Rayas Sujetos a Explotación Comercial En El Golfo de California: Una Investigación Aplicada al Mejoramiento de Su Manejo Pesquero y Conservación; Moss Landing Marine Laboratories Tech. Pub. 2009–02; 2007; Available online: https://aquadocs.org/bitstream/handle/1834/20226/MLML_Tech_Pub_09_02.pdf?sequence=1&isAllowed=y (accessed on 9 December 2021).
- Bizzarro, J.J.; Smith, W.D.; Márquez-Farías, J.F.; Tyminski, J.; Hueter, R.E. Temporal Variation in the Artisanal Elasmobranch Fishery of Sonora, Mexico. Fish. Res. 2009, 97, 103–117. [Google Scholar] [CrossRef]
- Smith, W.D.; Bizzarro, J.J.J.; Cailliet, G.M. The Artisanal Elasmobranch Fishery on the East Coast of Baja California, Mexico: Characteristics and Management Considerations. Ciencias Marinas. 2009, 35, 209–236. [Google Scholar] [CrossRef] [Green Version]
- Ramírez-Amaro, S.R.; Cartamil, D.; Galvan-Magaña, F.; Gonzalez-Barba, G.; Graham, J.B.; Carrera-Fernandez, M.; Escobar-Sanchez, O.; Sosa-Nishizaki, O.; Rochin-Alamillo, A. The artisanal elasmobranch fishery of the Pacific coast of Baja California Sur, Mexico, management implications. Sci. Mar. 2013, 77, 473–487. [Google Scholar] [CrossRef] [Green Version]
- Cartamil, D.; Santana-Morales, O.; Escobedo-Olvera, M.; Kacev, D.; Castillo-Geniz, L.; Graham, J.B.; Rubin, R.D.; Sosa-Nishizaki, O. The Artisanal Elasmobranch Fishery of the Pacific Coast of Baja California, Mexico. Fish. Res. 2011, 108, 393–403. [Google Scholar] [CrossRef]
- Villavicencio-Garayzar, C.J. Taxonomia, Abundancia Estacional, Edad y Crecimiento y Biologia Reproductiva de Narcine entemedor Jordan y Starks (Chondrichthyes; Narcinidae), En Bahia Almejas, B.C.S, Mexico. Ph.D. Dissertation, Universidad Autónoma de Nuevo León, Nuevo Leon 64460, Mexico, 2000; 138p. [Google Scholar]
- Márquez-Farías, J.F. The Artisanal Ray Fishery in the Gulf of California: Development, Fisheries Research and Management Issues. The IUCN/SSC Shark Specialist Group. Shark News 2002, 14, 12–13. [Google Scholar]
- González-González, L.; Mejía-Falla, P.A.; Navia, A.F.; de la Cruz-Agüero, G.; Ehemann, N.R.; Peterson, M.S.; Cruz-Escalona, V.H. The Espiritu Santo Island as a Critical Area for Conserving Batoid Assemblage Species within the Gulf of California. Environ. Biol. Fishes 2021, 104, 1359–1379. [Google Scholar] [CrossRef]
- Valadez-González, C. Distribución, Abundancia y Alimentación de Las Rayas Bentónicas de La Costa de Jalisco y Colima, México. Ph.D. Dissertation, Centro Interdisciplinario de Ciencias Marinas, La Paz, Mexico, 2007; p. 119. [Google Scholar]
- Burgos-Vázquez, M.I.; Mejía-Falla, P.A.; Cruz-Escalona, V.H.; Brown-Peterson, N.J. Reproductive Strategy of the Giant Electric Ray in the Southern Gulf of California. Mar. Coast. Fish. 2017, 9, 577–596. [Google Scholar] [CrossRef] [Green Version]
- Goldman, K.K. Age and growth of elasmobranch fishes. In Management Techniques for Elasmobranch Fisheries, FAO Fisheries Tech. Pap., 474; Musick, J.A., Bonfil, R., Eds.; Food and Agriculture Organization of the United Nations: Rome, Italy, 2005; pp. 76–102. ISBN 92-5-105403-7. [Google Scholar]
- Zar, J. Biostatistical Analysis; Pearson Prentice-Hall: Hoboken, NJ, USA, 2010; ISBN 9788578110796. [Google Scholar]
- Campana, S.E. Accuracy, Precision and Quality Control in Age Determination, Including a Review of the Use and Abuse of Age Validation Methods. J. Fish Biol. 2001, 59, 197–242. [Google Scholar] [CrossRef]
- Cailliet, G.M. Elasmobranch age determination and verification: An updated review. In Elasmobranchs as Living Resources: Advances in the Biology, Ecology, Systematics and the Status of the Fisheries; NOAA Technical Report NMFS; Pratt, H.L., Gruber, S.H., Taniuchi, T., Eds.; National Marine Fisheries Service, NOAA: Washington, DC, USA, 1990; pp. 157–165. [Google Scholar]
- Beamish, R.J.; Fournier, D.A. A Method for Comparing the Precision of a Set of Age Determinations. Can. J. Fish. Aquat. Sci. 1981, 38, 982–983. [Google Scholar] [CrossRef]
- Chang, W.Y.B. A Statistical Method for Evaluating the Reproducibility of Age Determination. Can. J. Fish. Aquat. Sci. 1982, 39, 1208–1210. [Google Scholar] [CrossRef]
- Campana, S.E.; Annand, M.C.; McMillan, J.I. Graphical and Statistical Methods for Determining the Consistency of Age Determinations. Trans. Am. Fish. Soc. 1995, 124, 131–138. [Google Scholar] [CrossRef]
- Bowker, A.H. A Test for Symmetry in Contingency Tables. J. Am. Stat. Assoc. 1948, 43, 572–574. [Google Scholar] [CrossRef]
- Kusher, D.I.; Smith, S.E.; Cailliet, G.M. Validated Age and Growth of the Leopard Shark, Triakis semifasciata, with Comments on Reproduction. Environ. Biol. Fishes 1992, 35, 187–203. [Google Scholar] [CrossRef]
- Branstetter, S. Age, Growth and Reproductive Biology of the Silky Shark, Carcharhinus falciformis, and the Scalloped Hammerhead, Sphyrna lewini, from the Northwestern Gulf of Mexico. Environ. Biol. Fishes 1987, 19, 161–173. [Google Scholar] [CrossRef]
- Metochis, C.P.; Carmona-Antoñanzas, G.; Kousteni, V.; Damalas, D.; Megalofonou, P. Population Structure and Aspects of the Reproductive Biology of the Blackmouth Catshark, Galeus Melastomus Rafinesque, 1810 (Chondrichthyes: Scyliorhinidae) Caught Accidentally off the Greek Coasts. J. Mar. Biol. Assoc. UK 2018, 98, 909–925. [Google Scholar] [CrossRef]
- Francis, R.I.C.C. Back-Calculation of Fish Length: A Critical Review. J. Fish Biol. 1990, 36, 883–902. [Google Scholar] [CrossRef]
- Hile, R. Age and Growth of the Rock Bass, Ambloplites rupestris (Rafinesque), in Nebish Lake, Wisconsin. Trans. Wis. Acad. Sci. Arts Lett. 1941, 33, 189–337. [Google Scholar]
- Burnham, K.P.; Anderson, D.R. Model Selection and Multimodel Inference: A Practical Information Theoretic Approach, 2nd ed.; Springer: New York, NY, USA, 2002; ISBN 0387953647. [Google Scholar]
- von Bertalanffy, L. A Quantitative Theory of Organic Growth (Inquiries on Growth Laws II). Hum. Biol. 1938, 10, 181–213. [Google Scholar]
- Fabens, A.J. Properties and Fitting of the Von Bertalanffy Growth Curve. Growth 1965, 29, 265–289. [Google Scholar]
- Ricker, W.E. Computation and Interpretation of Biological Statistics of Fish Populations. Bull. Fish. Res. Board Can. 1975, 191, 1–382. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Q. Comparison of Akaike Information Criterion (AIC) and Bayesian Information Criterion (BIC) in Selection of Stock-Recruitment Relationships. Fish. Res. 2006, 77, 220–225. [Google Scholar] [CrossRef]
- García-Borbón, J.A.; Morales-Bojórquez, E.; Aguirre-Villaseñor, H. Long-Term Changes in the Fraction of Mature Brown Shrimp Farfantepenaeus californiensis (Holmes, 1900) Females and Their Impact on Length at First Maturity. J. Shellfish. Res. 2018, 37, 1103–1111. [Google Scholar] [CrossRef]
- Hilborn, R.; Mangel, M. The Ecological Detective. Confronting Models with Data, 1st ed.; Princeton University Press: Princeton, NJ, USA, 1997. [Google Scholar]
- Haddon, M. Modelling and Quantitative Methods in Fisheries; Statistics/Biology; Chapman and Hall: Boca Raton, FL, USA, 2001; ISBN 9781584881773. [Google Scholar]
- Cerdenares-Ladrón de Guevara, G.; Morales-Bojórquez, E.; Rodriguez-Sánchez, R. Age and Growth of the Sailfish Istiophorus Platypterus (Istiophoridae) in the Gulf of Tehuantepec, Mexico. Mar. Biol. Res. 2011, 7, 488–499. [Google Scholar] [CrossRef]
- Luquin-Covarrubias, M.A.; Morales-Bojórquez, E.; González-Peláez, S.S.; Hidalgo-De-La-Toba, J.Á.; Lluch-Cota, D.B. Modeling of Growth Depensation of Geoduck Clam Panopea Globosa Based on a Multimodel Inference Approach. J. Shellfish. Res. 2016, 35, 379–387. [Google Scholar] [CrossRef]
- Rolim, F.; Caltabellotta, F.; Rotundo, M.; Vaske-Júnior, T. Sexual Dimorphism Based on Body Proportions and Ontogenetic Changes in the Brazilian Electric Ray Narcine Brasiliensis (von Olfers, 1831) (Chondrichthyes: Narcinidae). Afr. J. Mar. Sci. 2015, 37, 167–176. [Google Scholar] [CrossRef] [Green Version]
- Neer, J.A.; Cailliet, G.M. Aspects of the Life History of the Pacific Electric Ray, Torpedo californica (Ayres). Copeia 2001, 2001, 842–847. [Google Scholar] [CrossRef]
- Omer, V.D.; Basusta, N. Age and Growth Characteristics of Marbled Electric Ray Torpedo marmorata (Risso, 1810) Inhabiting Iskenderun Bay, North-Eastern Mediterranean Sea. Turk. J. Fish. Aquat. Sci. 2013, 13, 541–549. [Google Scholar] [CrossRef]
- Kaya, G.; Basusta, N. A Study on Age and Growth of Juvenile and Semi Adult Torpedo nobiliana Bonaparte, 1835 Inhabiting Iskenderun Bay, Northeastern Mediterranean Sea. Acta Biol. Turc. 2016, 29, 143–149. [Google Scholar]
- Hamlett, W.C. Reproductive Biology and Phylogeny of Chondrichthyes: Sharks, Batoids and Chimaeras. In Reproductive Biology and Phylogeny; Hamlett, W.C., Ed.; Science Publishers, Inc.: Enfield, NH, USA, 2005; ISBN 9781578082711. [Google Scholar]
- Wearmouth, V.J.; Sims, D.W. Sexual Segregation in Marine Fish, Reptiles, Birds and Mammals Behaviour Patterns, Mechanisms and Conservation Implications. Adv. Mar. Biol. 2008, 54, 107–170. [Google Scholar] [CrossRef] [PubMed]
- Cailliet, G.; Martin, D.; Kusher, P.; Wolf, B.; Welde, A. Techniques for Enhancing Vertebral Bands in Age Estimation of California Elasmobranchs. In Proceedings of an International Workshop on Age Determination of Oceanic Pelagic Fishes: Tunas, Billfishes, and Sharks, NOAA Tech. Rep. NMFS 8; Prince, E.D., Pulos, L.M., Eds.; National Marine Fisheries Service, NOAA: Miami, FL, USA, 1983; pp. 157–165. [Google Scholar]
- Licandeo, R.; Cerna, F.; Céspedes, R. Age, Growth, and Reproduction of the Roughskin Skate, Dipturus trachyderma, from the Southeastern Pacific. ICES J. Mar. Sci. 2007, 64, 141–148. [Google Scholar] [CrossRef]
- Matta, M.E.; Gunderson, D.R. Age, Growth, Maturity, and Mortality of the Alaska Skate, Bathyraja parmifera, in the Eastern Bering Sea. Environ. Biol. Fishes 2007, 80, 309–323. [Google Scholar] [CrossRef]
- Smart, J.J.; Chin, A.; Tobin, A.J.; Simpfendorfer, C.A. Multimodel Approaches in Shark and Ray Growth Studies: Strengths, Weaknesses and the Future. Fish Fish. 2016, 17, 955–971. [Google Scholar] [CrossRef]
- Keil, G.; Cummings, E.; de Magalhães, J.P. Being Cool: How Body Temperature Influences Ageing and Longevity. Biogerontology 2015, 16, 383–397. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cailliet, G.; Tanaka, S. Recommendations for research needed to better understand the age and growth of elasmobranchs. In Elasmobranchs as Living Resources: Advances in the Biology, Ecology, Systematics, and the Status of the Fisheries; Pratt, W.S., Jr., Gruber, S.H., Taniuchi, T., Eds.; National Marine Fisheries Service, NOAA: Washington, DC, USA, 1990; pp. 505–507. [Google Scholar]

 ) determined from thin sections and monthly variation of mean MIR. Sample size is indicated on top of the graph; (▪) mean monthly MIR; bars show the standard error for monthly values.
) determined from thin sections and monthly variation of mean MIR. Sample size is indicated on top of the graph; (▪) mean monthly MIR; bars show the standard error for monthly values.
 ) determined from thin sections and monthly variation of mean MIR. Sample size is indicated on top of the graph; (▪) mean monthly MIR; bars show the standard error for monthly values.
) determined from thin sections and monthly variation of mean MIR. Sample size is indicated on top of the graph; (▪) mean monthly MIR; bars show the standard error for monthly values.
| Month | January | February | March | April | May | June | July | August | September | October | November | December |
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Sample size | 18 | 33 | 7 | 3 | 29 | 10 | 42 | 37 | 20 | 14 | 10 | 22 |
| Total Length (cm) | Age Group (Down-Rounded) | Total | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | ||
| 42 | 1 | 1 | |||||||||||||
| 44 | 2 | 2 | |||||||||||||
| 46 | 0 | ||||||||||||||
| 48 | 2 | 1 | 1 | 4 | |||||||||||
| 50 | 1 | 3 | 1 | 5 | |||||||||||
| 52 | 5 | 5 | 10 | ||||||||||||
| 54 | 1 | 4 | 5 | 1 | 11 | ||||||||||
| 56 | 1 | 1 | 10 | 3 | 15 | ||||||||||
| 58 | 4 | 6 | 3 | 3 | 1 | 17 | |||||||||
| 60 | 3 | 7 | 9 | 3 | 22 | ||||||||||
| 62 | 1 | 3 | 12 | 6 | 22 | ||||||||||
| 64 | 1 | 3 | 7 | 2 | 2 | 1 | 16 | ||||||||
| 66 | 3 | 5 | 7 | 3 | 1 | 19 | |||||||||
| 68 | 1 | 3 | 5 | 1 | 3 | 1 | 14 | ||||||||
| 70 | 3 | 5 | 2 | 5 | 6 | 1 | 22 | ||||||||
| 72 | 4 | 1 | 6 | 8 | 2 | 1 | 22 | ||||||||
| 74 | 3 | 4 | 8 | 5 | 3 | 23 | |||||||||
| 76 | 2 | 2 | 1 | 2 | 2 | 9 | |||||||||
| 78 | 2 | 4 | 2 | 8 | |||||||||||
| 80 | 0 | ||||||||||||||
| 82 | 1 | 1 | 2 | ||||||||||||
| 84 | 1 | 1 | |||||||||||||
| Total | 2 | 12 | 18 | 40 | 38 | 26 | 24 | 13 | 21 | 26 | 14 | 8 | 1 | 2 | 245 |
| Total Length (cm) | Age Group (Down-Rounded) | |||||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 11 | 12 | 13 | 14 | Total | |
| 16 | 1 | 1 | ||||||||||||||
| 18 | 3 | 3 | ||||||||||||||
| 20 | 10 | 10 | ||||||||||||||
| 22 | 59 | 59 | ||||||||||||||
| 24 | 78 | 78 | ||||||||||||||
| 26 | 67 | 4 | 71 | |||||||||||||
| 28 | 14 | 19 | 33 | |||||||||||||
| 30 | 9 | 37 | 2 | 48 | ||||||||||||
| 32 | 5 | 48 | 3 | 56 | ||||||||||||
| 34 | 63 | 10 | 73 | |||||||||||||
| 36 | 31 | 25 | 2 | 58 | ||||||||||||
| 38 | 27 | 34 | 5 | 66 | ||||||||||||
| 40 | 6 | 48 | 7 | 1 | 62 | |||||||||||
| 42 | 7 | 49 | 16 | 2 | 74 | |||||||||||
| 44 | 2 | 25 | 31 | 5 | 63 | |||||||||||
| 46 | 1 | 23 | 41 | 8 | 2 | 75 | ||||||||||
| 48 | 2 | 16 | 44 | 19 | 4 | 85 | ||||||||||
| 50 | 3 | 38 | 32 | 7 | 80 | |||||||||||
| 52 | 8 | 22 | 45 | 11 | 4 | 90 | ||||||||||
| 54 | 1 | 13 | 33 | 24 | 8 | 79 | ||||||||||
| 56 | 1 | 9 | 40 | 31 | 8 | 3 | 92 | |||||||||
| 58 | 6 | 21 | 32 | 25 | 7 | 1 | 92 | |||||||||
| 60 | 3 | 13 | 34 | 19 | 12 | 2 | 83 | |||||||||
| 62 | 1 | 4 | 23 | 26 | 19 | 6 | 1 | 80 | ||||||||
| 64 | 1 | 3 | 14 | 22 | 21 | 18 | 1 | 80 | ||||||||
| 66 | 3 | 16 | 23 | 15 | 12 | 1 | 70 | |||||||||
| 68 | 1 | 7 | 12 | 21 | 15 | 5 | 61 | |||||||||
| 70 | 3 | 11 | 14 | 17 | 14 | 3 | 62 | |||||||||
| 72 | 5 | 5 | 16 | 12 | 5 | 1 | 44 | |||||||||
| 74 | 3 | 8 | 12 | 7 | 4 | 34 | ||||||||||
| 76 | 2 | 2 | 4 | 4 | 3 | 15 | ||||||||||
| 78 | 2 | 5 | 3 | 1 | 11 | |||||||||||
| 80 | 0 | |||||||||||||||
| 82 | 1 | 1 | 1 | 3 | ||||||||||||
| 84 | 1 | 1 | 2 | |||||||||||||
| Total | 245 | 247 | 248 | 239 | 227 | 185 | 138 | 113 | 87 | 73 | 50 | 24 | 12 | 3 | 2 | 1893 |
| Dataset | Model | L∞ | k | t0 | L0 | −LL | AIC | Δi | Wi |
|---|---|---|---|---|---|---|---|---|---|
| Unadjusted | VBG-3 | 82.09 (80.59–83.69) | 0.165 (0.170–0.173) | −1.988 (−2.008–−1.968) | 23.01 | 3507.23 | −7008.45 | 0.00 | 1.00 |
| VBG-2 | 69.35 (67.25–71.65) | 0.314 (0.294–0.334) | - | 14.50 | 2158.51 | −4313.03 | 2695.43 | 0.00 | |
| GG-3 | 73.49 (72.89–74.14) | 0.301 (0.292–0.310) | 0.46 (0.437–0.477) | 23.32 | 3486.66 | −6967.31 | 41.14 | 0.00 | |
| LG-3 | 69.79 (69.44–70.14) | 0.444 (0.435–0.453) | 1.500 (1.475–1.525) | 23.69 | 3439.89 | −6873.77 | 134.68 | 0.00 | |
| Adjusted-RC | VBG-3 | 81.50 (80.0–83.10) | 0.170 (0.165–0.175) | −1.363 (−1.383–−1.343) | 16.89 | 3413.10 | −6820.20 | 0.00 | 1.00 |
| VBG-2 | 75.52 (74.02–77.12) | 0.215 (0.206–0.224) | - | 14.50 | 3352.04 | −6700.08 | 120.11 | 0.00 | |
| GG-3 | 73.16 (72.51–73.81) | 0.308 (0.299–0.317) | 1.020 (0.999–1.039) | 18.60 | 3395.95 | −6785.90 | 34.30 | 0.00 | |
| LG-3 | 69.53 (69.39–70.19) | 0.454 (0.434–0.455) | 2.040 (2.017–2.067) | 19.71 | 3354.06 | −6702.12 | 118.08 | 0.00 | |
| Adjusted-DC | VBG-3 | 81.87 (80.37–83.47) | 0.168 (0.163–0.173) | −1.384 (−1.404–−1.364) | 16.96 | 3454.00 | −6902.00 | 0.00 | 1.00 |
| VBG-2 | 75.59 (74.09–77.19) | 0.213 (0.204–0.222) | - | 14.50 | 3386.07 | −6768.15 | 133.85 | 0.00 | |
| GG-3 | 73.36 (72.71–74.01) | 0.305 (0.296–0.314) | 1.030 (1.011–1.051) | 18.65 | 3436.19 | −6866.39 | 35.61 | 0.00 | |
| LG-3 | 69.70 (69.35–70.05) | 0.449 (0.439–0.460) | 2.063 (2.040–2.090) | 19.77 | 3392.80 | −6779.60 | 122.40 | 0.00 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mora-Zamacona, P.; Melo-Barrera, F.N.; Cruz-Escalona, V.H.; Navia, A.F.; Morales-Bojórquez, E.; Pérez-Palafox, X.A.; Mejía-Falla, P.A. Growth Modeling of the Giant Electric Ray Narcine entemedor in the Southern Gulf of California: Analyzing the Uncertainty of Three Data Sets. Animals 2022, 12, 19. https://doi.org/10.3390/ani12010019
Mora-Zamacona P, Melo-Barrera FN, Cruz-Escalona VH, Navia AF, Morales-Bojórquez E, Pérez-Palafox XA, Mejía-Falla PA. Growth Modeling of the Giant Electric Ray Narcine entemedor in the Southern Gulf of California: Analyzing the Uncertainty of Three Data Sets. Animals. 2022; 12(1):19. https://doi.org/10.3390/ani12010019
Chicago/Turabian StyleMora-Zamacona, Pablo, Felipe N. Melo-Barrera, Víctor H. Cruz-Escalona, Andrés F. Navia, Enrique Morales-Bojórquez, Xchel A. Pérez-Palafox, and Paola A. Mejía-Falla. 2022. "Growth Modeling of the Giant Electric Ray Narcine entemedor in the Southern Gulf of California: Analyzing the Uncertainty of Three Data Sets" Animals 12, no. 1: 19. https://doi.org/10.3390/ani12010019






