Energy-Based Pore Pressure Generation Models in Silty Sands under Earthquake Loading

Abstract

During an earthquake, excess pore water pressure generation in saturated silty sands causes a reduction in shear strength and even liquefaction of the soil. A comprehensive experimental program consisting of undrained cyclic simple-shear tests was undertaken to explore the key factors affecting the energy-based excess pore water pressure generation models for non-plastic silty sands. The examined influencing factors were non-plastic fines content (less than and greater than the threshold value ≅ 25%), packing density, vertical effective stress, applied cyclic stress ratio, and soil fabric. The relationship between excess pore water pressure ratio and dissipated energy per unit volume was found to be mainly dependent on the relative density and fines content of soil, whereas the cyclic stress ratio, initial vertical effective stress, and soil fabric (i.e. the reconstitution method) appeared to have a minor impact. A revision of the original energy-based model developed for clean sand by Berrill and Davis was proposed to improve prediction accuracy in terms of residual excess pore water pressures versus normalised cumulative dissipated energy. Nonlinear multivariable regression analyses were performed to develop correlations for the calibration parameters of the revised model. Lastly, these correlations were validated through additional cyclic simple-shear tests performed on different silty sands recovered at a site where liquefaction occurred after the 2012 Emilia Romagna (Italy) earthquake.

1. Introduction

The generation of excess pore water pressure (PWP) in saturated clean sands and silty sands during earthquake loading reduces soil strength and stiffness and, in some cases, can induce liquefaction phenomena [1,2,3,4]. Therefore, an accurate prediction of PWP buildup induced by seismic events is a fundamental requirement for assessing the seismic safety and resilience of structures and infrastructures in saturated soils. In addition, liquefaction hazard is expected to be more severe, especially in low-lying coastal areas due to the increase in sea-level associated with climate change effects [5,6,7,8].

Contradictory results regarding the effect of fines content on the undrained cyclic strength of silty sands can be found in the literature. In particular, some studies [9,10,11] showed that the liquefaction resistance (CRR) of silty sands increased with f_c, whereas other researchers [12,13,14] highlighted that the addition of f_c to clean sand caused a decrease in CRR up to a threshold fines content (f_thre), beyond which CRR increased with f_c. Threshold fines content (f_thre) represents the limiting value of f_c separating “sand-dominated” to “fines-dominated” types of behaviour of sand–silt mixtures [15,16,17,18]. These controversial findings suggest that the influence of fines is still a challenging topic and more studies are needed. The different experimental evidences can be explained to some extent by considering the different state variables used in the analysis of test results and the complex types of intergrain contacts, which depend on the fines content, the plasticity of fines, the grading features of host sand and fines, and particle shape [19,20,21,22].

Different methods have been employed in the literature to predict excess PWP induced by seismic events in clean sands and silty sands, namely, stress-based [23,24,25], strain-based [26,27,28], energy-based [29,30,31], and plasticity-theory-based approaches [32,33,34]. Several researchers have developed sophisticated plasticity-theory-based models wherein excess PWP assessment is founded on constitutive models. However, these models often require many parameters that are difficult to be obtained [35,36]. For this reason, stress-based, strain-based, and energy-based models usually implemented with loosely coupled approaches in 1D non-linear analysis codes are the most used in designing [37].

Stress-based models correlate the excess pore water pressure ratio R_u (i.e., the ratio of excess pore water pressure Δu to initial vertical effective stress σ′_v0) with the normalised number of applied cycles N/N_f, where N represents the number of applied uniform loading cycles and N_f denotes the number of uniform cycles at the point of liquefaction. Conversely, strain-based models relate R_u to the maximum cyclic shear strain γ_max recorded in a specific applied uniform loading cycle. The proposed stress- or strain-based models have been calibrated using laboratory undrained cyclic triaxial [38,39,40], simple shear [3,25,41], and torsional tests [24,42]. In these tests, a sinusoidal shear stress or shear strain loading history is applied, and the increase in excess PWP with the number of loading cycles is measured. Consequently, implementing stress-based and strain-based methods for earthquake site response analyses requires that seismic history is converted to an equivalent motion characterised by constant-amplitude uniform cycles. Such conversion depends on the technique used to choose and count the applied stress cycles and the adopted conversion curve. Thus, the necessary procedures are frequently complex and unreliable [43,44,45]. For this reason, the adopted methodology generally consists of relating earthquake magnitude to the equivalent number of loading cycles [22,46,47,48] even if these correlations often result in inaccurate estimates of the number of equivalent loading cycles [44]. On the other hand, the energy-based models overcome this drawback, relating R_u to the cumulative energy dissipated per unit volume of soil (W) without the need to convert ground motion into uniform cycles. In fact, W is obtained directly from the knowledge of the cyclic stress–strain behaviour of soil. Considering that both PWP buildup and energy dissipated during cyclic loading are linked to the breakdown of the soil skeleton, many studies have been carried out to correlate R_u with cumulative dissipated energy. Nemat-Nasser and Shokooh [49] first proposed an energy-based method for predicting excess PWP generation in the 1970s; afterwards, many efforts were made to develop energy-based models for sands based on extensive laboratory investigations considering different influencing factors, such as relative density, fines content, applied cyclic stress ratio, overburden stress, and initial static shear stress [29,30,40,42,50,51,52,53,54,55,56]. These studies demonstrated that a unique correlation between R_u and W does exist, regardless of the apparatus adopted to carry out laboratory tests (cyclic triaxial, cyclic simple shear, cyclic torsional) and the applied loading history (uniform or random cyclic loading).

In particular, Berrill and Davis [29] proposed the following simple empirical formulation:

$$R_u=\alpha ·W_n^{\beta }$$

(1)

where W_n is the cumulative dissipated energy per unit volume of the soil normalised to the initial vertical effective stress, while α and β are empirical parameters to be calibrated through cyclic laboratory tests.

Berrill and Davis [29] showed that R_u-W_n curves obtained from cyclic triaxial tests were less sensitive to the change in β value with respect to α. The latter parameter strongly regulates the shape of the curve.

It is worth noting that the model of Berrill and Davis’s [29] does not provide a limiting value of R_u at liquefaction, as expected in granular soils, and consequently R_u increases continuously with the increase in W_n. This aspect requires particular attention, especially in silty sands, and the present study aims to overcome this drawback. Indeed, when liquefaction is reached according to a selected criterion, excess pore water pressure reaches a constant value, and the cumulative dissipated energy corresponding to this condition attains a limiting value denoted as W_f.

Another well-known energy-based PWP model proposed in the literature is the GMP model [30], expressed through the following equation:

$$R_u=\sqrt{W_n/PEC}\le 1,$$

(2)

where the pseudo energy capacity PEC (dimensionless) is the calibration parameter of the model, and it can be determined experimentally by following the procedures suggested by Green et al. [30] and Green [57]. The GMP model represents a special case of the more general model of Berrill and Davis [29]; in fact, the GMP model was obtained by setting the empirical constants α and β equal to 1/PEC^0.5 and 0.5, respectively.

Actually, the energy-based models proposed by Berrill and Davis [29] and Green et al. [30] are implemented in the 1D non-linear analysis code DEEPSOIL [58]. Nevertheless, although the equations of these models depend on one or two parameters [PEC in Equation (2) and α and β in Equation (1)], the calibration of these parameters from laboratory tests is not simple, and, for this reason, the applications of these models are rather limited. Consequently, correlations for assessing these calibration parameters based on the main factors affecting the R_u-W_n relationship appear helpful for practical purposes.

Polito et al. [40], starting from an extensive database of cyclic triaxial tests, correlated PEC with the relative density (D_R) of sands with a fines content (f_c) less and higher than 35%, as follows:

$$\ln \left(PEC\right)=\left\{\begin{array}{ll}\exp \left(0.0139·D_R\right)-1.021& \mathrm{if}{f}_c<35\%\\ -0.597·f_c^{0.312}+\exp \left(0.0139·D_R\right)-1.021& \mathrm{if}{f}_c\ge 35\%\end{array}\right.$$

(3)

where D_R and f_c are expressed as percentages, and PEC values result in kPa.

Mele et al. [59], using a dataset composed of 43 undrained cyclic triaxial tests and 3 undrained cyclic simple-shear tests carried out on six sands, provided a calibration procedure for the parameters of the Berrill and Davis [29] model. In particular, the authors noted that the two parameters (α and β) are linked, and correlations between in situ CPT or SPT test results and the model parameters were proposed. However, few studies based on cyclic triaxial tests have systematically investigated the effect of fines on energy-based PWP approach and cumulative dissipated energy at liquefaction of silty sands. The main findings gathered from these studies are as follows: (a) the addition of f_c (up to 20%) to silica sand reduced the growth in residual excess PWP in the R_u-W plane [56], whereas for carbonate coral sands, an opposite trend was observed for f_c < 30% [55]; (b) W_f in silica sands first increased with fines content up to a limiting value of f_c and decreased afterwards [56,60,61]. Conversely, for carbonate sands, Qin et al. [55] evidenced a different behaviour, where W_f decreased with fines content up to f_c = 30%.

A comprehensive cyclic simple-shear test program was used in the present work to analyse the fundamental factors affecting the development of excess PWP through an energy-based approach for Ticino sand mixed with fines contents ranging from 0% to 40%. The well-known model proposed by Berrill and Davis [29] was properly modified to better capture the experimental data, and specific correlations for the model parameters as a function of packing density and fines content, were provided. The capability of the proposed equations was verified with additional experimental results performed on different silty sands. The topic of the present study falls within the framework of the Tech4You Project “Technologies for Climate Change Adaptation and Quality of Life Improvement”, which is funded by the National Recovery and Resilience Plan (PNRR) and supported by the European Union – NextGenerationEU.

2. Test Materials and Procedure

2.1. Test Materials

The materials tested in this study comprised clean sands and sand–silt mixtures. Ticino sand (TS) is a uniform coarse-to-medium natural sand recovered from the Ticino River in Italy, whereas Emilia Romagna sand (ES) is a poorly graded, medium-to-fine sand. Mixtures of Ticino sand with non-plastic fines were tested, namely, 90% sand/10% fines (TS10), 80% sand/20% fines (TS20), 70% sand/30% fines (TS30), and 60% sand/40% fines (TS40). Since the f_thre value for the Ticino–silt mixture was found to be 24.5% [22], the present study takes into account both cases of f_c < f_thre and f_c > f_thre. Emilia Romagna’s silty sand (ES40) consists of 40% low-plasticity fines (with a plasticity index less than 8). Both ES and ES40 were recovered from a site where liquefaction phenomena occurred in Italy after the 2012 earthquake.

Grain size distribution curves for all the tested materials are shown in Figure 1, while the material properties are listed in

Table 1. Material properties.

Material	GS	D10 (mm)	D50 (mm)	CU	emax	emin
TS	2.68	0.417	0.564	1.5	0.93	0.58
TS10	2.67	0.059	0.547	10.2	0.81	0.47
Fines	2.72	0.004	0.024	8.5	-	-
TS20	2.68	0.023	0.518	24.7	0.79	0.37
TS30	2.69	0.013	0.483	41.6	0.80	0.37
TS40	2.70	0.010	0.440	50.5	0.95	0.48
ES	2.70	0.120	0.320	2.8	0.93	0.54
ES40	2.77	0.005	0.096	24.0	-	-

Note: G_S = specific gravity, D₁₀ = grain diameter at 10% passing, D₅₀ = mean grain size, C_U = uniformity coefficient, and e_max, e_min = maximum and minimum void ratios.

. On the basis of the grain size distribution curves, the tested materials can be considered susceptible to liquefaction in accordance with Italian Building Code [62].

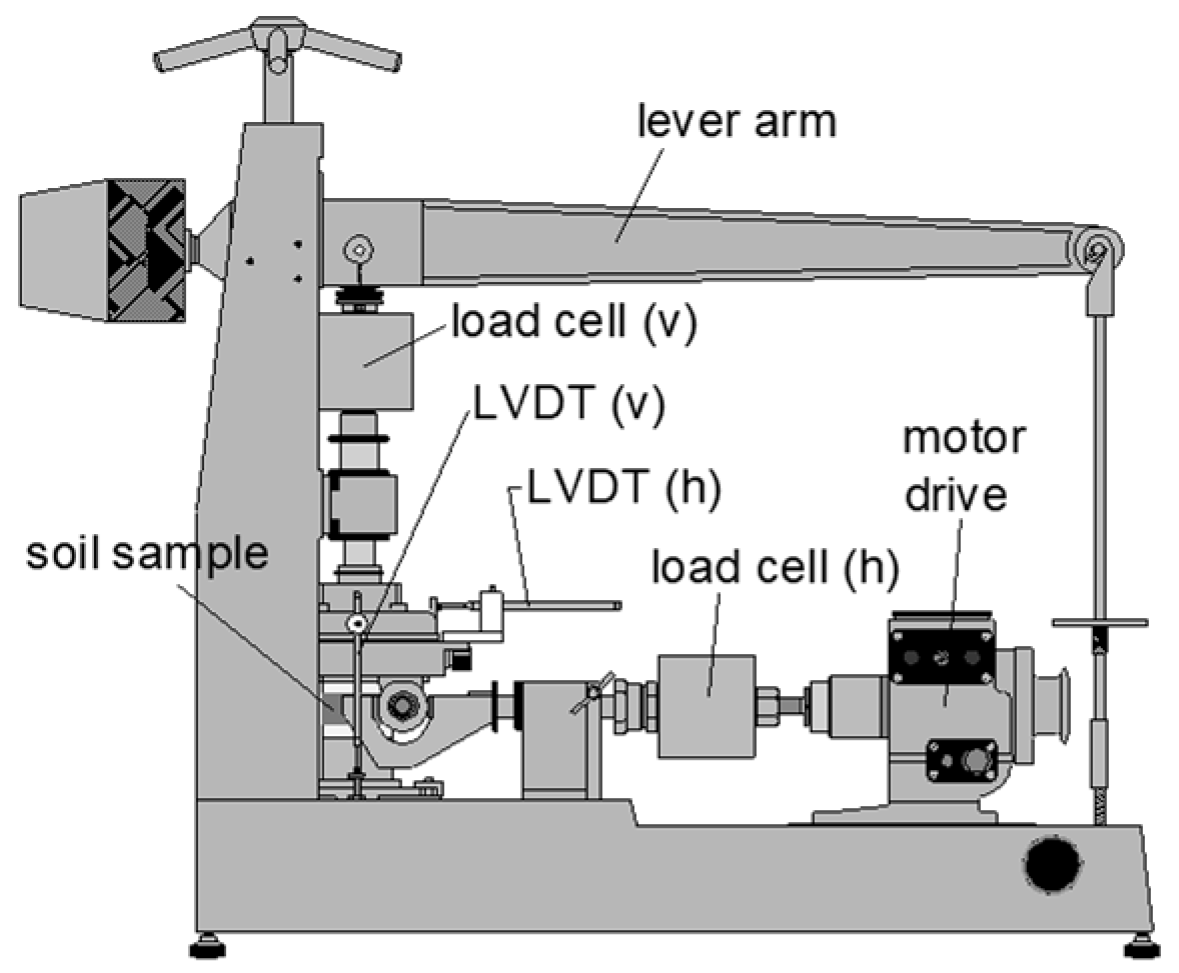
2.2. Test Procedure

The NGI-type simple-shear (SS) device adopted to perform undrained cyclic tests on clean sands and sand–silt mixtures is shown in Figure 2. The specimen diameter was 80 mm, and it was laterally confined by a wire-reinforced rubber membrane to prevent lateral deformation of the sample. A detailed description of the SS device was reported by Porcino et al. [63].

Except for high-quality undisturbed samples of ES40 taken from an Emilia Romagna site (Italy) [3,64], the specimens were prepared using three different reconstitution methods, namely, moist tamping (MT), water sedimentation (WS), and air pluviation (AP), to represent different soil fabrics, as shown in Figure 3. Ticino sand–silt mixtures were reconstituted only using the moist-tamping method to avoid the segregation phenomenon between the host sand and silt observed for specimens formed by water sedimentation and air pluviation in previous studies [65,66,67]. Considering that some void ratios may not be reachable for certain mixtures, a relative density parameter was used in the present study for comparison purposes, following the example from previous studies [10,11,12,56]. In particular, cyclic simple-shear tests were performed considering a wide range of relative density of tested soils from 26% to 94%.

The air pluviation method consisted of depositing the sand through a funnel that was traversed laterally to maintain an approximately levelled surface of the soil. After filling the cavity, the excess material over the final grade was siphoned off by applying a slight vacuum. Loose samples were prepared using the above-described procedure while to obtain high-density specimens, when required, the mould was tapped laterally using a small rubber hammer, maintaining at the same time a small seating load on the top of the specimen.

When preparing SS specimens using the water sedimentation method, the sand was spooned gently layer by layer into the water until the height was just above the top of the mould. Relative density values in the range of D_R = 37.5 ± 2.5% were initially achieved by following this procedure, regardless of the drop height. Similar to the case for the air-pluviated specimens, higher densities were obtained by tapping the mould laterally while a small seating load was maintained on the sample cap and the drainage lines were kept open.

Finally, the moist-tamping method consisted of preparing a prefixed weight of wet soil (sand or silty sand) in two layers, each of them measuring 10 mm high. First, dry material was prepared at the selected weight ratio to achieve the desired relative density; afterwards, an amount of de-aired water was added to the mixture, which was placed in the reinforced membrane. Each layer was compacted to a reference height. Due to the limited height of the specimen (only 20 mm), the effects of inhomogeneity along its height can be neglected.

In the cyclic simple-shear test, a liquefaction criterion based on the attainment of a 3.75% single-amplitude shear strain (γ_SA) was adopted, as specified in previous studies [69,70,71]. The test specimens were first consolidated until reaching the desired effective vertical stress σ′_v0, ranging from 50 kPa to 130 kPa, and then a cyclic shear stress (τ_cyc) was applied under stress-controlled conditions. Cyclic stress ratios (CSR = τ_cyc/σ′_v0) ranging from 0.08 to 0.26 were applied to the specimens at a loading frequency of 0.1 Hz. CSRs were applied to the specimens so that liquefaction conditions were achieved in a given number of loading cycles representative of a typical earthquake magnitude. All cyclic tests were performed in constant volume conditions, closely representing undrained conditions in the NGI simple-shear apparatus [72,73]. Under constant-volume simple-shear conditions, the measured change in vertical stress is assumed to be equal to the excess pore water pressure that would develop in a truly undrained test. Constant-volume tests were performed using an automatic control system that suppresses the vertical movement of a specimen by applying changes in vertical load. According to ASTM D8296 [74], constant volume conditions are considered satisfied when a limiting vertical strain of a sample of less than 0.05% is measured during tests. The experimental program comprised 68 cyclic simple-shear tests, as reported in

Table 2. Constant-volume cyclic simple-shear test program.

Material	RC	fc (%)	e0	DR (%)	σ′v0 (kPa)	CSR	No. Tests	Behaviour
TS	MT	0	0.78	43	100	0.12–0.16	3	CM
TS	MT	0	0.74	54	100	0.12–0.18	4	CM
TS	MT	0	0.68	71	100	0.16–0.23	4	CM
TS	MT	0	0.63	86	100	0.20–0.25	3	CM
TS	MT	0	0.60	94	100	0.23–0.26	2	CM
TS	WS	0	0.79	42	100	0.10–0.15	2	CM
TS	AP	0	0.78	43	100	0.10–0.16	5	CM
ES	WS	0	0.69	60 *	100	0.13–0.23	4	CM
TS10	MT	10	0.60	62	100	0.14–0.20	3	CM
TS10	MT	10	0.60	62	50	0.16–0.18	2	CM
TS10	MT	10	0.68	38	100	0.10–0.16	3	CM
TS10	MT	10	0.68	38	50	0.14–0.15	2	CM
TS10	MT	10	0.55	76	100	0.18–0.20	2	CM
TS20	MT	20	0.51	67	100	0.14–0.19	4	CM
TS20	MT	20	0.55	57	100	0.14–0.16	2	CM
TS20	MT	20	0.58	50	100	0.12–0.16	3	CM
TS20	MT	20	0.58	50	50	0.14–0.15	2	CM
TS20	MT	20	0.68	26	100	0.08–0.10	2	FF
TS30	MT	30	0.49	72	100	0.20–0.22	2	CM
TS30	MT	30	0.55	58	100	0.16–0.20	2	CM
TS30	MT	30	0.58	51	100	0.12–0.18	3	CM
TS30	MT	30	0.68	28	100	0.12–0.14	2	FF
TS30	MT	30	0.68	28	50	0.14–0.18	2	CM
TS40	MT	30	0.68	57	100	0.12	1	CM
ES40	UND	40	0.69 ± 0.15	32 *	130	0.17–0.26	4	CM

Note: RC = reconstitution method, MT = moist tamping, WS = water sedimentation, AP = air pluviation, UND = undisturbed, e₀ = global void ratio, D_R = relative density, σ′_v0 = initial vertical effective stress, CSR = cyclic stress ratio, CM = cyclic mobility, and FF = flow failure. * inferred from in situ tests based on Reyna and Chameau [75] correlation.

.

3. Analysis of Test Results

3.1. Undrained Cyclic Simple-Shear Responses of Silty Sand Specimens

Figure 4 illustrates for two silty sand specimens (TS and TS20) the undrained cyclic simple-shear response in terms of shear stress (τ)–shear strain (γ) curves (Figure 4a,c) and the development of shear strain (Figure 4b,d). Figure 4 shows the two typical behaviours observed in all the CSS tests (Table 2), namely, cyclic mobility (Figure 4a,b) and flow failure response (Figure 4c,d).

The cyclic mobility type of the response reveals that the shear strains remained initially negligible for up to 43 cycles; after a few cycles, the sample failed according to the selected failure criterion (γ = 3.75% in single-amplitude). The stress–strain loops exhibited a typical S-shaped trend and developed on both the positive and negative sides of strains, as shown in Figure 4a. On the other hand, when the silty sand exhibited a flow failure type of response (Figure 4c,d), a rapid and uncontrollable development of shear strains was observed when the flow was triggered (N_f = 19).

It is worth noting that a flow failure type of behaviour occurred only in a few CSS tests for mixtures with high fines content (TS20, TS30) with an initial state parameter ranging from 0.066 to 0.188 [76].

Figure 5a,b report the development of the excess pore water pressure ratio (R_u) with the number of cycles (N) for the same specimens shown in Figure 4. The residual pore water pressure ratio R_u,res, i.e. the excess pore water pressure ratio measured at the end of each applied cycle when τ_cyc = 0 kPa, influences directly soil stiffness and strength [40]. Such values are clearly represented in Figure 5a,b by empty red circles. As expected, the R_u,res continuously increased with an increasing N in both cyclic mobility (Figure 5a,c) and flow failure modes (Figure 5b,d). It is important to observe that R_u,res at the onset of liquefaction could not reach unity, as will be discussed in subsequent sections.

Figure 5c,d present the cumulative dissipated energy along with N for the two specimens described in Figure 4, corresponding to the cyclic mobility and flow-failure patterns, respectively. The dissipated energy per unit volume in each loading cycle (W_i) was calculated by referring to the hysteresis loops (for example, Figure 4a,c), as follows:

$$W_i=\int \tau ·d\gamma$$

(4)

where τ is the applied shear stress and γ is the corresponding shear strain. Then, the cumulative dissipated energy per unit volume (W) was derived as the sum of the dissipated energies of all loading cycles up to N cycles:

$$W=\sum _{i=1}^N{W}_i$$

(5)

Cumulative dissipated energy increased continuously with N cycles, as shown in Figure 5c,d. Notably, just before reaching the liquefaction condition (denoted by the filled red circles), W increases abruptly, especially for specimens exhibiting a flow failure response (Figure 5d). These results are consistent with those reported by other authors, such as Pan and Yang [77] and Zhou et al. [56]. The value of W in correspondence with the triggering of liquefaction (W_f.) was defined as the “capacity energy” of the soil.

Considering that both residual excess pore water pressure and dissipated energy per unit volume increased with N, R_u and W can be easily related to one another, as demonstrated in previous research [29,30,51,54,55,56,78].

3.2. Key Factors Influencing Cyclic Excess Pore Water Pressure in Silty Sands Based on an Energy Approach

In order to calibrate the proposed energy-based model for silty sands based on the experimental data obtained from undrained cyclic simple-shear tests, the dominant factors influencing R_u vs. W relationships were analysed and discussed. Key factors such as relative density, vertical effective stress, applied cyclic stress ratio, fines content, and soil fabric were explored.

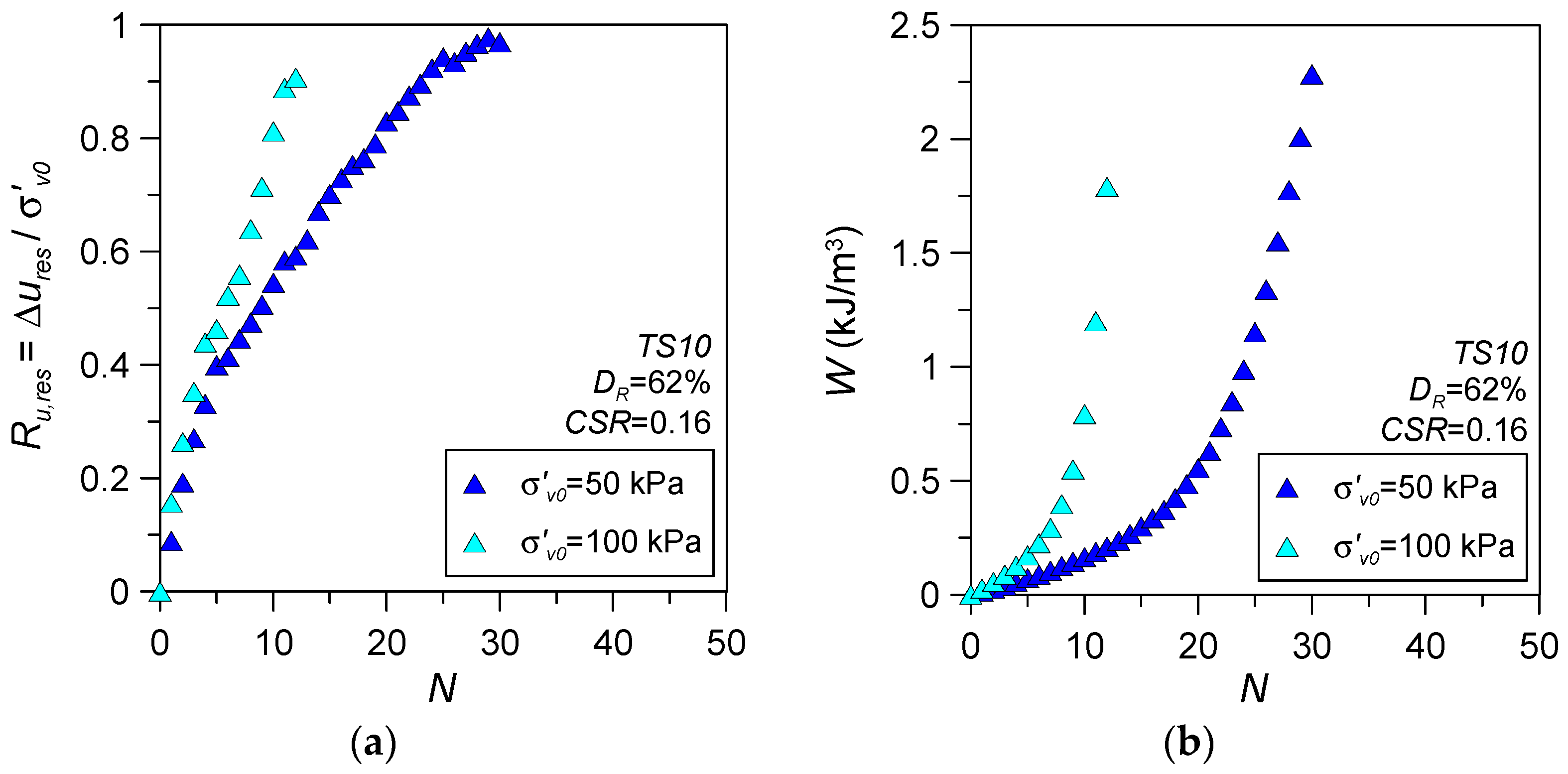
3.2.1. Influence of Applied Cyclic Stress Ratio

Figure 6 presents the residual excess pore water pressure ratio (R_u) versus cumulative dissipated energy (W) for clean Ticino sand and the Ticino sand mixture (f_c = 20%) tested in the same initial state but subjected to different cyclic stress amplitudes (CSRs).

There is an apparent non-linear increasing trend between R_u and W, regardless of CSR. It can be observed that CSR does not have a significant effect on pore water pressure generation for both tested materials. This finding also applies to the other mixtures investigated in the present study and it is in accordance with previous research [42,56,79].

The relative independence of R_u-W relationships from the cyclic stress ratio and load shape (i.e. irregular, sinusoidal, triangular) represents one of the main advantages of the models based on energy principles [52,80].

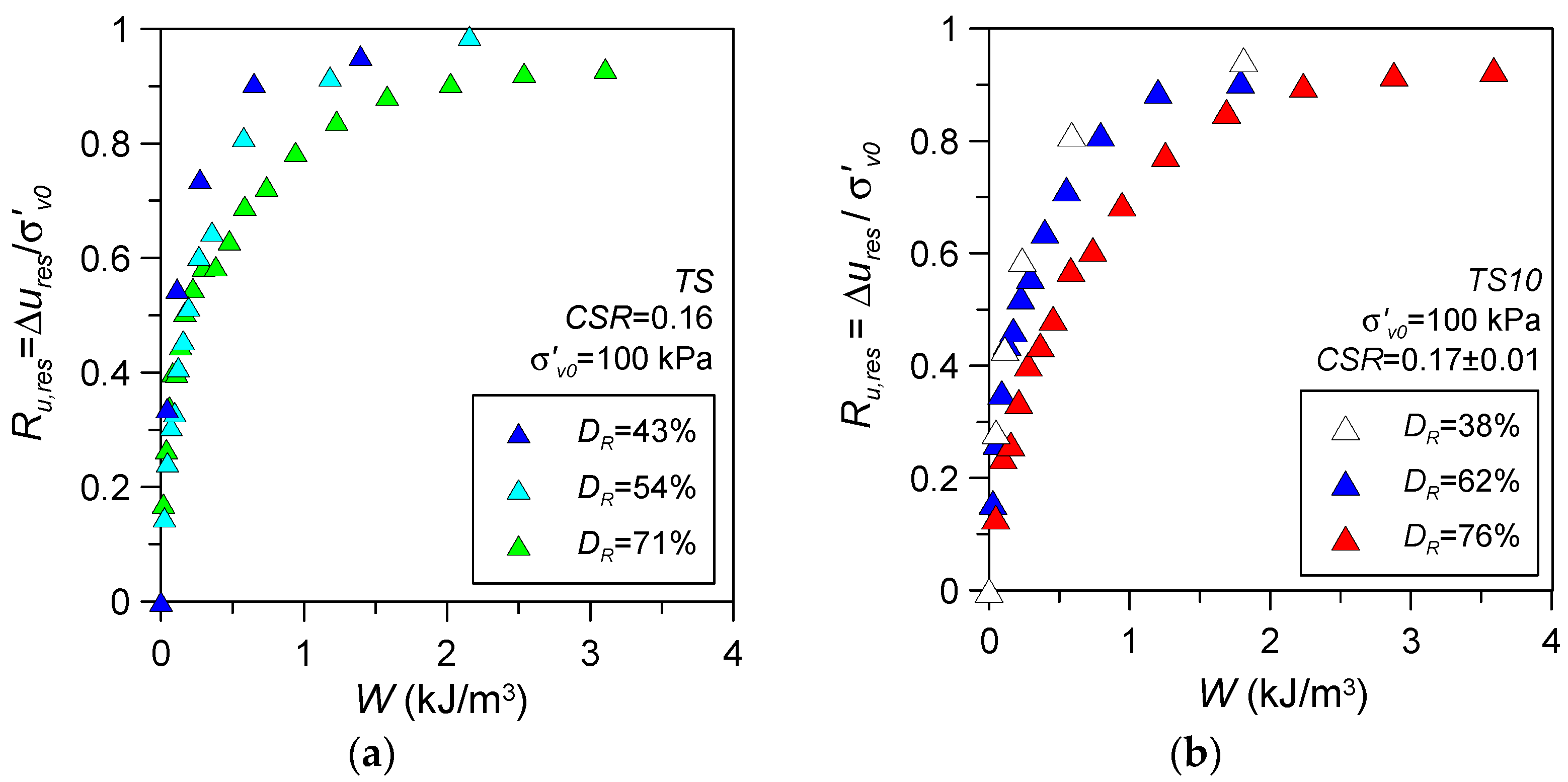
3.2.2. Influence of Fines Content and Packing Density

Figure 7 plots the relationship between R_u and W of TS–fines mixtures characterised by different finescontents equal to 0%, 10%, 20%, 30%, and 40%. The sand–fines mixtures in Figure 7 were reconstituted at a relative density of 58 ± 4% and consolidated at an initial vertical stress of 100 kPa.

Figure 7 shows that f_c has a remarkable effect on the variation in R_u with respect to W, which means that the addition of f_c entails a strengthening effect with a more gradual generation of excess pore water pressure and higher values of the energy required for liquefaction, W_f. These findings are consistent with those reported by other authors [10,11,56,61] and are valid when relative density is selected as a control variable to describe the undrained response of silty sands. Different results can be expected when other control variables, such as the global void ratio, skeleton void ratio, or equivalent granular void ratio, are employed in place of D_R [14,81,82]. Another interesting finding is that residual excess pore water pressure at liquefaction decreases with an increase in the amount of non-plastic fines, a finding that is in agreement with previous works [3,83,84].

Figure 8 explores the effect of packing density on the generation of excess pore pressure versus cumulative dissipated energy for two specimens (TS and TS10) consolidated under an initial vertical effective stress of 100 kPa and cyclically sheared at a similar applied stress ratio (CSR = 0.17 ± 0.01).

Figure 8a,b show that when relative density increased excess pore pressure accumulated more slowly, and higher values of capacity energy W_f were measured. Similar experimental evidence was also observed in undrained cyclic triaxial tests performed on Toyoura sand by Yang and Pan [85] (among other authors). An explanation for this behaviour is that when relative density increases, the compressibility of a material decreases, meaning that silty sand behaviour is more dilatant (Chien et al. [86] and Yang and Pan [85], among others). It is also interesting to note that for both specimens the limiting value of R_u (R_u,lim) in correspondence with γ_SA = 3.75% decreases with an increasing relative density [87].

This comparative analysis shows that D_R and f_c play significant roles in the development of excess PWP in saturated silty sands when interpreted by using an energy-based approach.

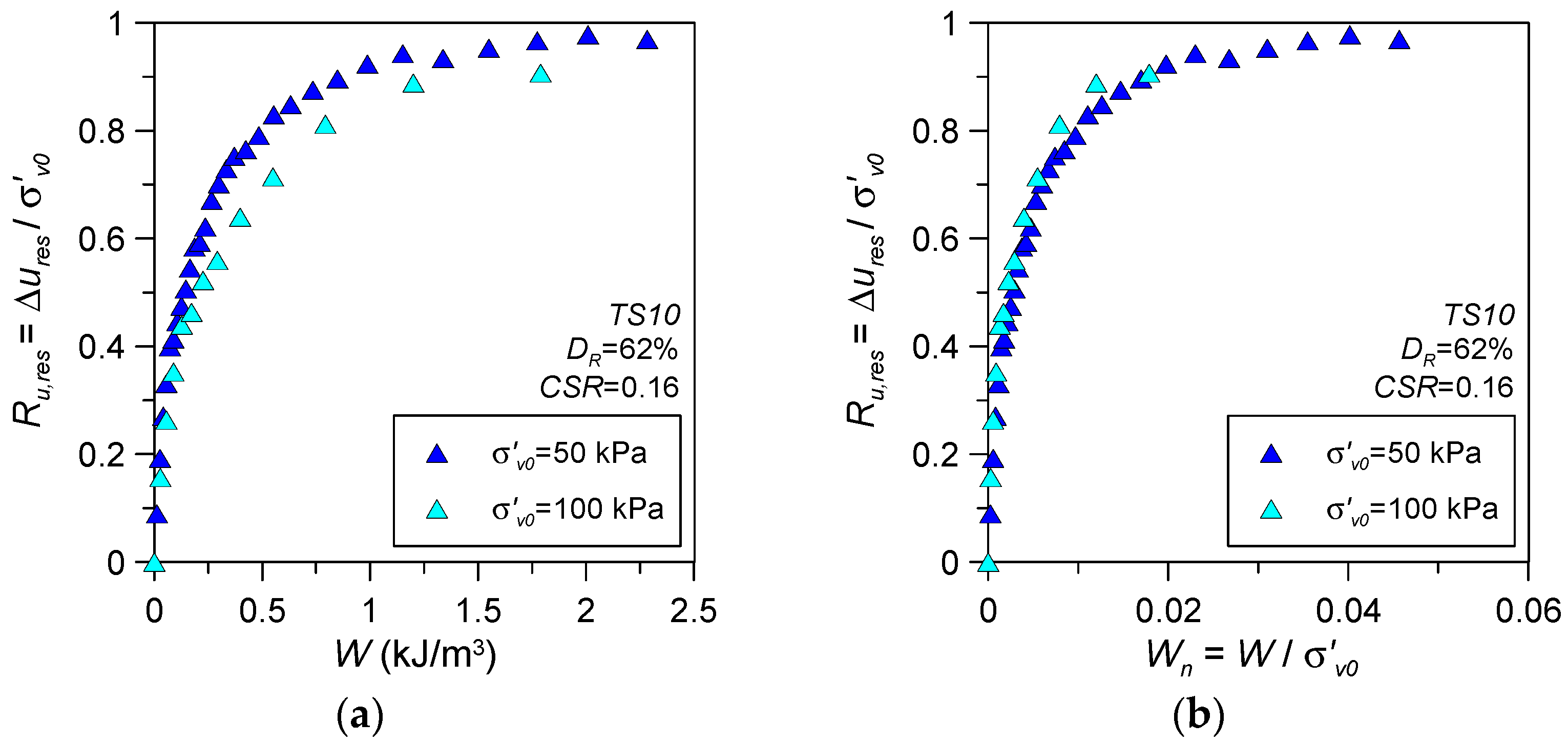
3.2.3. Influence of Vertical Effective Stress

Figure 9 shows the influence of initial vertical effective stress on undrained cyclic response of TS10 in terms of (a) R_u,res versus N and (b) cumulative dissipated energy measured at the end of each cycle.

As expected, for TS10 consolidated under σ′_v0 = 100 kPa, the development of R_u,res (Figure 9a) is faster than the corresponding specimen consolidated under low values of σ′_v0 (50 kPa) so that a lower number of cycles is needed for liquefaction. Similarly, for the specimen tested at σ′_v0 = 100 kPa, the W versus N curve (Figure 9b) grows more rapidly with lower values of cumulative dissipated energy at liquefaction compared to the corresponding specimen consolidated under σ′_v0 = 50 kPa. This experimental evidence can be explained by the fact that higher material compressibility is expected for higher confining stresses, as demonstrated by different studies in the literature [50,60].

Figure 10a shows the influence of initial vertical effective stress on the undrained cyclic response of TS10 in terms of R_u,res versus W curves. When R_u,res was linked to W, distinct curves were obtained for different σ′_v0 values. Consequently, to unify the effects of overburden stress on the relationship between R_u,res and W, a stress-normalised W was introduced, as follows:

$$W_n=\frac{W}{{\sigma \prime }_{v0}}$$

(6)

When normalisation was applied, a unique R_u,res-W_n relationship for different σ′_v0 values was obtained, as shown in Figure 10b. The observation that the influence of σ′_v0 is negligible in the R_u-W_n relationship is consistent with the results of the study conducted by Konstadinou and Georgiannou [42] (among others). This experimental evidence stresses the importance of normalising cumulative dissipated energy to initial vertical effective stress to unify R_u,res-W_n trends, regardless of the initial vertical effective stress value.

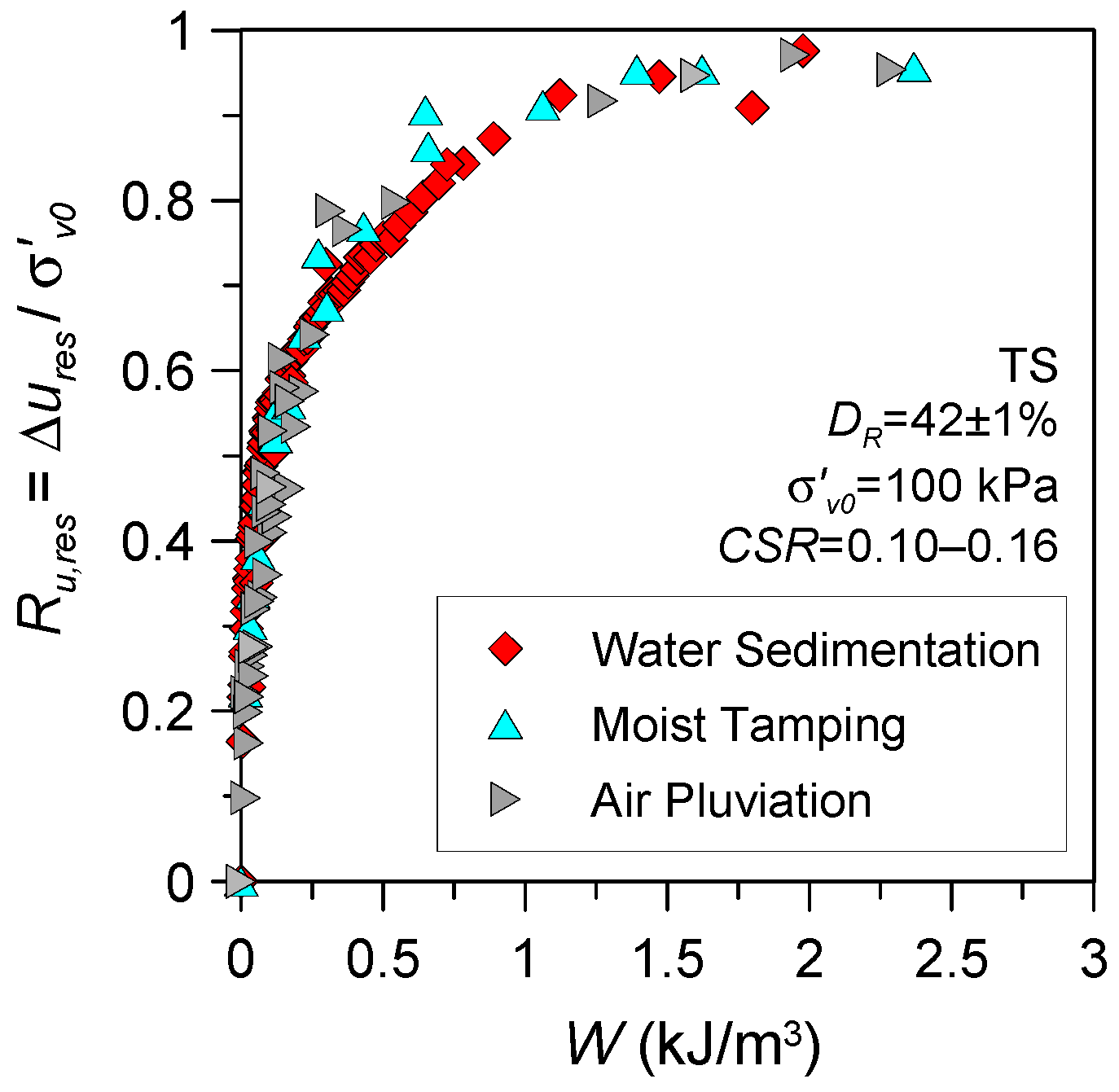
3.2.4. Influence of Initial Soil Fabric/Reconstitution Method

The initial soil fabric of natural soils is relevant in geotechnical engineering analyses. Soil fabric is reproduced in laboratory using reconstitution methods that should reproduce the arrangement of grains as much as possible [65,68,88,89]. In this context, test results gathered from clean Ticino sand were analysed to verify the dependence of the correlation between R_u and W for the initial fabric induced by the sand reconstitution method. Previous research did not thoroughly investigate this aspect, which needs further verification [35,41].

Figure 11 shows the residual excess pore water pressure ratio versus cumulative dissipated energy for Ticino clean sand specimens prepared in the same initial state (a combination of packing density and initial vertical effective stress) but reconstituted using three different reconstitution methods, namely moist tamping, air pluviation, and water sedimentation. Interestingly, different soil fabrics induced by different reconstitution methods do not influence significantly the trend of R_u vs. W. A similar conclusion was made in previous research considering a pore-water-pressure-strain-based approach, while for stress-based PWP models this finding does not appear applicable [41].

3.3. Modelling of Excess Pore Water Pressure Based on Energy Approach for Silty Sand and Validation

The analysis of key factors influencing the prediction of cyclic excess PWP in silty sands provided in Section 3.2 highlighted that (i) a non-linear increasing trend between R_u,res and W can be observed, and it is dependent on f_c, D_R, and σ′_v0, while CSR and soil fabric appeared to have a negligible effect; (ii) the normalisation of W to σ′_v0 allows the incorporation of the effect of overburden stress; and (iii) a limiting excess PWP value less than unity was measured, especially in dense silty sands with high fines content.

The pore water pressure model proposed by Berrill and Davis [29] for clean sands was revised considering the experimental evidence emerging from the present study to predict R_u in silty sands in a satisfactory way. In addition, this section aims to provide a simple calibration procedure for the energy-based pore pressure model to make its use simpler and easier for practitioners.

To account for the fact that the value of R_u at liquefaction (R_u,lim) for clean sands and sands containing non-plastic fines can be less than unity, Equation (1) was properly modified, as follows:

$$R_u=\alpha ·W_n^{\beta }\le R_{u,lim}$$

(7)

In the present study, the calibration parameters α, β, and R_u,lim of the revised model [Equation (7)], corresponding to the best fit of all the experimental data of moist-tamped silty sand specimens, were determined. Figure 12 shows the trend of the calculated empirical parameters as a function of (i) fines content (at the same relative density) and (ii) relative density (at the same fines content).

The best-fitting correlations (i.e., those with the highest coefficient of determination R²) were obtained using Curve Expert Professional v. 1.0.2 software, which provides a large number of functional equations. The authors properly selected simpler functional forms over complex equations. It is interesting to note that all the model parameters for Ticino–silty sands decrease with an increasing D_R and f_c.

Nonlinear multivariable regression analyses were performed to derive the calibration parameters α, β, and R_u,lim as a function of f_c and D_R. The proposed equations characterised by the highest coefficient of determination R² are the following:

$$\alpha =\alpha \left(D_R,f_c\right)=\left(\frac{1}{0.0281+0.000041·f_c}\right)+42.338·{D_R}^{-0.0736}-63.447 (R^2=0.72)$$

(8)

$$\beta =\beta \left(\alpha \right)=0.0975+0.1741·\mathrm{l}\mathrm{n}\left(\alpha \right) (R^2=0.87)$$

(9)

$$R_{u,lim}=R_{u,lim}\left(D_R,f_c\right)=0.995-0.0019·f_c-0.00105·D_R (R^2=0.64)$$

(10)

The performance of the revised model with respect to the original one proposed for clean sands by Berrill and Davis [29] was verified and illustrated in Figure 13 in the R_u,res-W_n plane. It is notable that when the revised model was applied to the CSS test data for Ticino silty sand, all data points were well located (R² > 0.91) within a narrow zone bounded by an upper and lower bound defined by the following range of parameters: α = 5.29–2.15, β = 0.25–0.38, and R_u,lim = 0.97–0.91 (Figure 13a).

Conversely, the original model proposed by Berrill and Davis [29] (Figure 13b) is able to predict the response of Ticino clean sand but does not capture adequately the trend of the experimental data for silty sand, especially in the last parts of the tests, thus resulting in lower R² values (>0.78). The knowledge of model parameters gathered from Equations (8)–(10) can be useful for the non-linear seismic ground response analysis of a site carried out using DEEPSOIL [58].

To validate the proposed correlations for predicting the parameters of the revised model, additional CSS tests performed on reconstituted clean sands and undisturbed silty sands recovered from the Emilia Romagna region (Italy) were analysed. CSS tests were carried out on Emilia clean sand prepared at D_R = 60% and consolidated under σ′_v0 = 100 kPa, while Emilia silty sand (D_R = 32%, f_c = 40%) was consolidated under a value of σ′_v0 = 130 kPa. For these materials and test conditions, Equations (8)–(10) provide values of the model parameters α, β, and R_u,lim reported in Figure 14. The good performance regarding the model predictions (Figure 14a,b) against the experimental trend highlights that the proposed relationships can be considered reasonable not only for reconstituted silty sands but also for undisturbed silty sands.

Finally, the results obtained from the current analysis show the significance of the R_u,lim parameter introduced in the revised energy-based model of silty sands. It allows one to adjust R_u,res-W_n trends to accurately model the observed overall response of sands with fines (f_c ≠ 0%). In fact, in these cases, R_u,res increases with W_n up to a limiting PWP value (R_u,lim = 0.88–0.97), which remains approximately constant while increasing further the cumulative dissipated energy up to W_f. The energy required for liquefaction was found to increase with an increasing fines content.

4. Conclusions

This study investigated the excess pore water pressure generation of low-plasticity silty sands using data from 68 undrained cyclic simple-shear tests covering a wide range of fines contents, packing densities, effective vertical stresses, sample preparation methods, and cyclic stress ratios. The test results were interpreted using an energy-based approach to highlight the factors influencing the relationship between excess pore water pressure and cumulative dissipated energy. The relative density of soil was assumed as a reference density index of soil for comparison and analysis. The main conclusions of this study are as follows:

• Two types of undrained cyclic response were observed in clean sand and silty sand, namely cyclic mobility and flow failure. In both types of behaviour, the normalised cumulative dissipated energy and the excess pore water pressure ratio increased with increasing the applied number of cyclic loading, confirming that R_u and W are strongly linked. Notably, just before liquefaction conditions are reached (γ_SA = 3.75%) W increases abruptly, especially for specimens exhibiting a flow failure response.
• A nonlinear increasing trend between the residual excess pore water pressure ratio R_u,res and normalised dissipated energy W_n was observed for silty sands. The R_u,res-W_n relationship was found to be dependent on the fines content of the mixture as well as relative density, whereas the applied cyclic stress ratio CSR, initial vertical effective stress σ′_v0, and the sample reconstitution method/soil fabric appeared to have a minor impact.
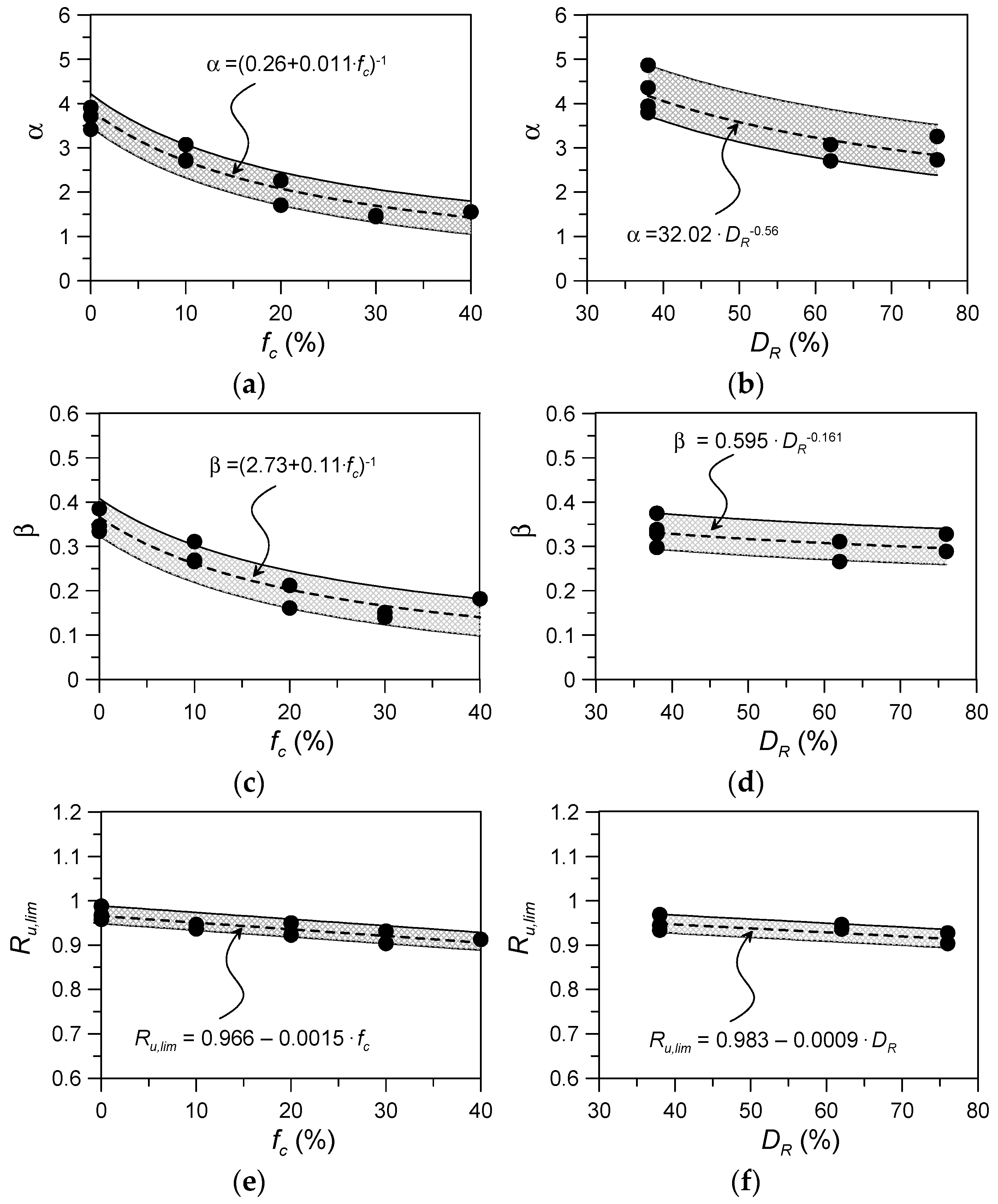
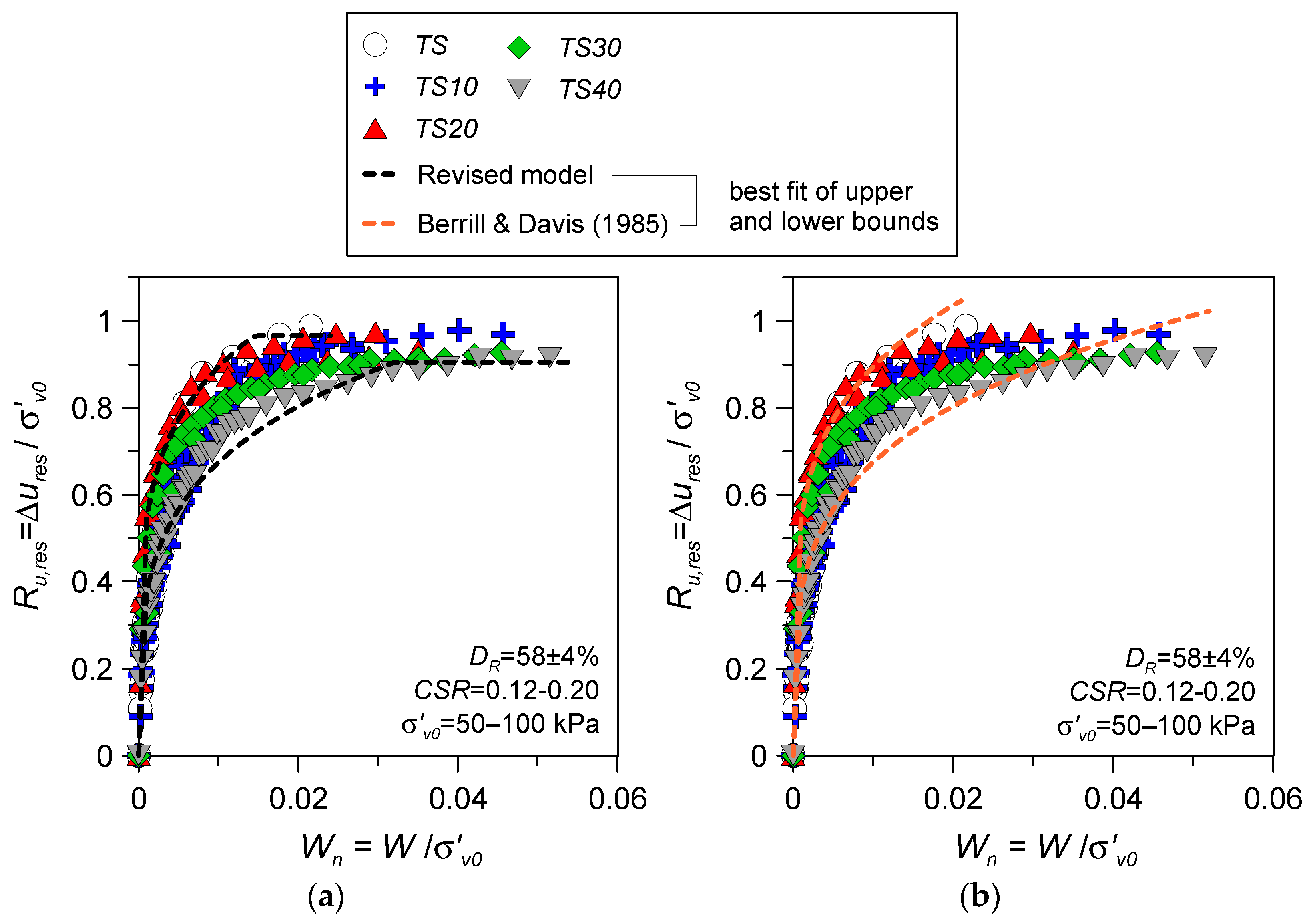
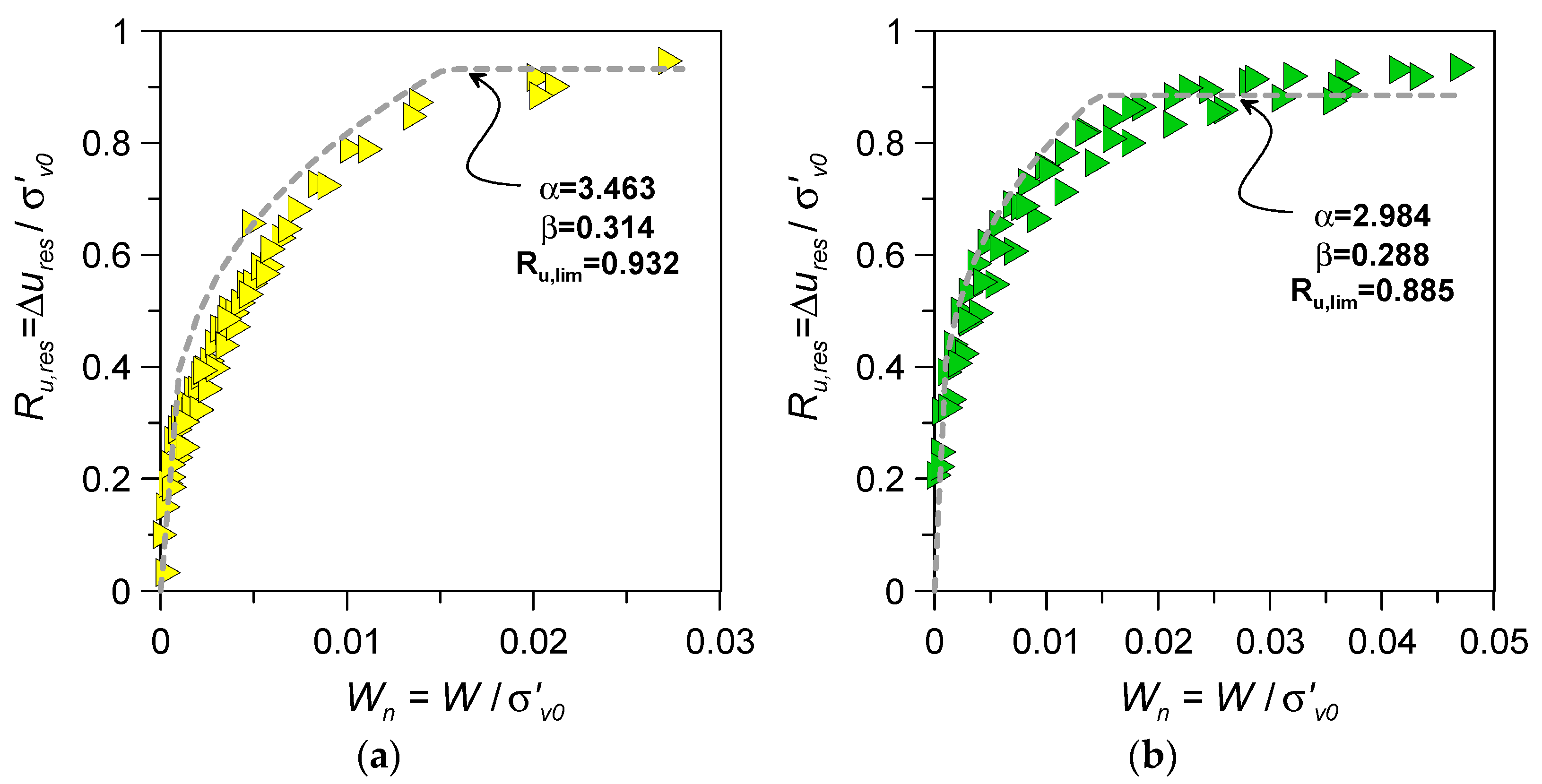
• To determine the response of silty sands in a more accurate way, the original energy-based PWP model of Berrill and Davis [29] for clean sands under cyclic loading was properly revised, introducing an additional calibration parameter, namely the limiting value of excess pore water pressure at liquefaction (R_u,lim). It was found that W_f increased with an increasing fines content when tested under the same initial test conditions (D_R, σ′_v0).
• Specific correlations for the calibration parameters α, β, and R_u,lim of the modified model were developed and proposed for soils with f_c ≤ 40%, D_R = 26–94%, and CSR = 0.08–0.26. The trends in all the calibration parameters evidenced a decrease with an increasing packing density and fines content of silty sands.
• The validation of the proposed correlations for the model parameters based on the results of CSS tests performed on additional materials, i.e., Emilia Romagna clean sand and silty sand, indicated that such correlations provided accurate predictions of the experimental PWP response, even for undisturbed natural sand–silt mixtures.

The results gathered in the present study can be helpful for practitioners to accurately predict excess pore water pressures in silty sands when analysing nonlinear seismic site response through numerical analysis. The findings gathered in this study are based on specific materials, sample preparation methods (air pluviation, moist tamping, water sedimentation), testing apparatus (constant volume CSS), and initial states (D_R, σ′_v0). Additional studies considering other silty sands and testing apparatus (i.e. cyclic triaxial, cyclic torsional) are required to reach more definitive conclusions and perform comparisons.