Ants Can Anticipate the Following Quantity in an Arithmetic Sequence
Abstract
:1. Introduction
2. Material and Methods
2.1. Collection and Maintenance of Ants
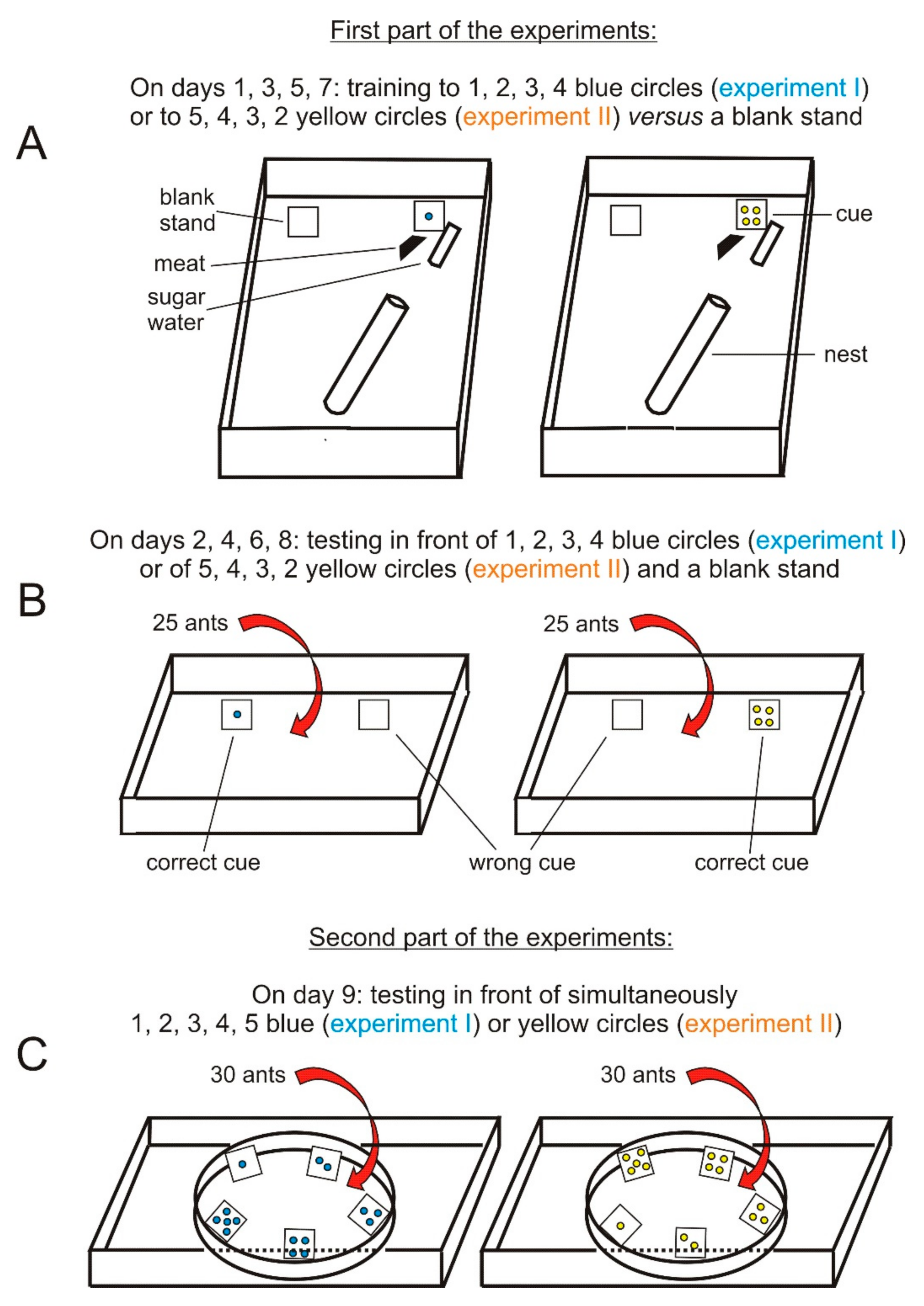
2.2. Experimental Planning
2.3. Experimental Materials
2.4. Experimental Methods, Assessments, Statistics
3. Results
3.1. Anticipation of the Following Number in an Increasing Arithmetic Sequence
- First part of the experiment
- Second part of the experiment
3.2. Anticipation of the Following Number in a Decreasing Arithmetic Sequence
- First part of the experiment
- Second part of the experiment
4. Discussion
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Raby, C.R.; Clayton, N.S. Prospective cognition in animals. Behav. Process. 2009, 80, 314–324. [Google Scholar] [CrossRef] [PubMed]
- Raby, C.R.; Alexis, D.M.; Dickinson, A.; Clayton, N.S. Planning for the future by western scrub-jays. Nature 2007, 445, 919–921. [Google Scholar] [CrossRef] [PubMed]
- Correia, S.P.C.; Dickinson, A.; Clayton, N.S. Western scrub-jays anticipate future needs independently of their current motivational state. Curr. Biol. 2007, 17, 856–861. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shettleworth, S.J. Planning for breakfast. Nature 2007, 114, 825–926. [Google Scholar] [CrossRef]
- Clayton, N.S.; Dickinson, A. Episodic-like memory during cache recovery by scrub jays. Nature 1998, 395, 272–274. [Google Scholar] [CrossRef]
- Fortin, N.J.; Agster, K.L.; Eichenbaum, H.B. Critical role of the hippocampus in memory for sequences of events. Nat. Neurosci. 2002, 5, 458–462. [Google Scholar] [CrossRef] [Green Version]
- Hassabis, D.; Kumaran, D.; Vann, S.D.; Maguire, E.A. Patients with hippocampal amnesia cannot imagine new experiences. Proc. Natl. Acad. Sci. USA 2007, 104, 1726–1731. [Google Scholar] [CrossRef] [Green Version]
- Hassabis, D.; Kumaran, D.; Maguire, E.A. Using imagination to understand the neural basis of episodic memory. J. Neurosci. 2007, 27, 14365–14374. [Google Scholar] [CrossRef]
- Suddendorf, T. Episodic memory versus episodic foresight: Similarities and differences. WIREs Cogn. Sci. 2010, 1, 99–107. [Google Scholar] [CrossRef]
- Schacter, D.L.; Benoit, R.G.; Szpunar, K.K. Episodic future thinking: Mechanisms and functions. Curr. Opin. Behav. Sci. 2017, 17, 41–50. [Google Scholar] [CrossRef]
- Naqshbandi, M.; Roberts, W.A. Anticipation of future events in squirrel monkeys (Saimiri sciureus) and rats (Rattus norvegicus): Tests of the Bischof-Kohler hypothesis. J. Comp. Psychol. 2006, 120, 345–357. [Google Scholar] [CrossRef] [PubMed]
- Mulcahy, N.J.; Call, J. Apes save tools for future use. Science 2006, 312, 1038–1040. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Osvath, M.; Osvath, H. Chimpanzee (Pan troglodytes) and orangutan (Pongo abelii) forethought: Self-control and pre-experience in the face of future tool use. Anim. Cogn. 2008, 11, 661–674. [Google Scholar] [CrossRef] [PubMed]
- Enquist, M.; Lind, J.; Ghirlanda, S. The power of associative learning and the ontogeny of optimal behaviour. R. Soc. Open Sci. 2016, 3, 160734. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lind, J. What can associative learning do for planning? R. Soc. Open Sci. 2018, 5, 180778. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cammaerts, M.-C.; Cammaerts, R. Ants’ numerosity ability defined in nine studies. J. Biol. Life Sci. 2020, 11, 121–142. [Google Scholar] [CrossRef]
- Cammaerts, M.-C.; Cammaerts, R. Summary of seven more studies on numerosity abilities in an ant, four of them relating to human competence. J. Biol. Life Sci. 2020, 11, 296–326. [Google Scholar] [CrossRef]
- Brannon, E.M.; Terrace, H.S. Representation of the numerosities 1–9 by rhesus macaques (Macaca mulatta). J. Exp. Psych. Anim. Behav. Process. 2000, 26, 31–49. [Google Scholar] [CrossRef]
- Biro, D.; Matsuzawa, T. Use of numerical symbols by the chimpanzee (Pan troglodytes): Cardinals, ordinals, and the introduction of zero. Anim. Cogn. 2001, 4, 193–199. [Google Scholar] [CrossRef]
- Hodges, C.M. Optimal foraging in bumblebees: Hunting by expectation. Anim. Behav. 1981, 29, 1166–1171. [Google Scholar] [CrossRef]
- Bortot, M.; Agrillo, C.; Avarguès-Weber, A.; Bisazza, A.; Miletto Petrazzini, M.E.; Giurfa, M. Honeybees use absolute rather than relative numerosity in number discrimination. Biol. Lett. 2019, 15, 2019138. [Google Scholar] [CrossRef] [PubMed]
- Howard, S.R.; Avarguès-Weber, A.; Garcia, J.E.; Greentree, A.D.; Dyer, A.G. Numerical cognition in honeybees enables addition and subtraction. Sci. Adv. 2019, 5, eaav0961. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Howard, S.R.; Avarguès-Weber, A.; Garcia, J.E.; Greentree, A.D.; Dyer, A.G. Achieving arithmetic learning in honeybees and examining how individuals learn. Com. Int. Biol. 2019, 12, 166–170. [Google Scholar] [CrossRef]
- Howard, S.R.; Avarguès-Weber, A.; Garcia, J.E.; Greentree, A.D.; Dyer, A.G. Numerical ordering of zero in honey bees. Science 2018, 360, 1124–1126. Available online: http://science.sciencemag.org/ (accessed on 5 January 2019). [CrossRef] [PubMed] [Green Version]
- Howard, S.R.; Avarguès-Weber, A.; Garcia, J.E.; Greentree, A.D.; Dyer, A.G. Symbolic representation of numerosity by honeybees (Apis mellifera): Matching characters to small quantities. Proc. R. Soc. B 2019, 286, 20190238. [Google Scholar] [CrossRef] [Green Version]
- Cammaerts, M.-C.; Cammaerts, R. Spatial expectation of food location in an ant on basis of previous food locations (Hymenoptera, Formicidae). J. Ethol. 2016, 35, 9. [Google Scholar] [CrossRef]
- Cammaerts, M.-C.; Cammaerts, R. Ants can expect the time of an event on basis of previous experiences. ISRN Entomol. 2016, 9. [Google Scholar] [CrossRef]
- Cammaerts, M.-C.; Cammaerts, R. Are ants (Hymenoptera, Formicidae) capable of self-recognition? J. Sci. 2015, 5, 521–532. Available online: www.journalofscience.net>showpdf (accessed on 28 December 2020).
- Cammaerts, M.-C. Ants’ Ability in Solving Simple Problems. Int. J. Biol. 2017, 9, 26–37. Available online: http://www.ccsenet.org/journal/index.php/ijb/issue/view/1810 (accessed on 28 December 2020). [CrossRef] [Green Version]
- Cammaerts, M.-C. In a novel situation, ants can learn to react as never before—A preliminary study. J. Behav. 2017, 2, 1–8. Available online: www.jscimedcentral.com>Articles (accessed on 28 December 2020).
- Cammaerts, M.-C.; Cammaerts, R. Learning a behavioral sequence: An accessible challenge for Myrmica sabuleti workers? Int. J. Biol. 2018, 10, 1–14. [Google Scholar] [CrossRef] [Green Version]
- Cammaerts, M.-C.; Cammaerts, R. Ants can acquire some serial recognition. Int. J. Biol. 2018, 10, 23–32. [Google Scholar] [CrossRef] [Green Version]
- Cammaerts, M.-C.; Cammaerts, R. Can ants apply what they acquired through operant conditioning? Int. J. Biol. 2018, 10, 16–22. [Google Scholar] [CrossRef] [Green Version]
- Cammaerts, M.-C.; Cammaerts, R. Ants are at the first stage of the notion of zero. Int. J. Biol. 2019, 11, 54–65. [Google Scholar] [CrossRef]
- Cammaerts, M.-C.; Cammaerts, R. Ants correctly locate the zero in a continuous series of numbers. Int. J. Biol. 2019, 11, 16–25. [Google Scholar] [CrossRef] [Green Version]
- Cammaerts, M.-C. Colour vision in the ant Myrmica sabuleti Meinert, 1861 (Hymenoptera: Formicidae). Myrmecol. News 2007, 10, 41–50. Available online: Myrmecologicalnews.org>cms>m (accessed on 28 December 2020).
- Siegel, S.; Castellan, N.J. Non-Parametric Statistics for the Behavioural Sciences; McGraw-Hill Book Company: Singapore, 1988; p. 396. [Google Scholar]
- Cammaerts, M.-C.; Rachidi, Z.; Cammaerts, D. Collective operant conditioning and circadian rhythms in the ant Myrmica sabuleti (Hymenoptera, Formicidae). Bull. Soc. R. Belg. Ent. 2011, 147, 142–154. Available online: www.srbe-kbve.be (accessed on 28 December 2020).
- Cammaerts, M.-C.; Cammaerts, R. Ethological and physiological effects of Sativex, a cannabis-based medicine, examined on ants as models. Acta Sci. Pharmac. Sci. 2020, 4, 63–84. [Google Scholar] [CrossRef]
- Cammaerts, M.-C.; Cammaerts, R. Ants can associate a symbol with a number of elements through conditioning. Int. J. Biol. 2020, 12, 1–13. [Google Scholar] [CrossRef]
- Cammaerts, M.-C. Estimation of elapsed time by ants. Bull Soc. R. Ent. Belg. 2010, 146, 189–195. Available online: www.srbe-kbve.be (accessed on 28 December 2020).
- Cammaerts, M.-C.; Rachidi, Z. Olfactory conditioning and use of visual and odorous elements for movement in the ant Myrmica sabuleti (Hymenoptera, Formicidae). Myrmecol. News 2009, 12, 117–127. Available online: Myrmecologicalnews.org>cms>m (accessed on 28 December 2020).
- Clayton, N.S.; Bussey, T.J.; Dickinson, A. Can animals recall the past and plan for the future? Neuroscience 2003, 4, 685–691. [Google Scholar] [CrossRef] [PubMed]
- Osvath, M.; Martin-Ordas, G. The future of future-oriented cognition in non-humans: Theory and the empirical case of the great apes. Phil. Trans. R. Soc. B 2014, 369, 20130486. [Google Scholar] [CrossRef] [Green Version]
- Dufour, V.; Pelé, M.; Sterck, E.H.M.; Thierry, B. Chimpanzee (Pan troglodytes) anticipation of food return: Coping with waiting time in an exchange task. J. Comp. Psychol. 2007, 121, 145–155. [Google Scholar] [CrossRef] [Green Version]
- Osvath, M.; Persson, T. Great apes can defer exchange: A replication with different results suggesting future oriented behavior. Front. Psychol. 2013, 4, 698. [Google Scholar] [CrossRef] [Green Version]
- Bourjade, M.; Call, J.; Pelé, M.; Maumy, M.; Dufour, V. Bonobos and orangutans, but not chimpanzees, flexibly plan for the future in a token-exchange task. Anim. Cogn. 2014, 17, 1329–1340. [Google Scholar] [CrossRef] [Green Version]
- Osvath, M.; Karvonen, E. Spontaneous innovation for future deception in a male chimpanzee. PLoS ONE 2012, 7, e36782. [Google Scholar] [CrossRef] [Green Version]
- Van Schaik, C.P.; Damerius, L.; Isler, K. Wild orangutan males plan and communicate their travel direction one day in advance. PLoS ONE 2013, 8, e74896. [Google Scholar] [CrossRef]
- Kabadayi, C.; Osvath, M. Ravens parallel great apes in flexible planning for tool use and bartering. Science 2017, 357, 202–204. [Google Scholar] [CrossRef] [Green Version]
- Hoerl, C.; McCormack, T. Thinking in and about time: A dual systems perspective on temporal cognition. Behav. Brain Sci. 2019, 42, 1–69. [Google Scholar] [CrossRef] [Green Version]
- Shanks, D.R. Learning: From association to cognition. Annu. Rev. Psychol. 2010, 61, 273–301. [Google Scholar] [CrossRef] [PubMed]
- Vasas, V.; Chittka, L. Insect-inspired sequential inspection strategy enables an artificial network of four neurons to estimate numerosity. iScience 2019, 11, 85–92. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mizunami, M.; Yamagata, N.; Nishino, H. Alarm pheromone processing in the ant brain: An evolutionary perspective. Front. Behav. Neurosci. 2010. [Google Scholar] [CrossRef] [Green Version]
- Kamhi, J.F.; Traniello, J.F.A. Biogenic amines and collective organization in a super organism: Neuromodulation of social behavior in ants. Brain Behav. Evol. 2013, 82, 220–236. [Google Scholar] [CrossRef]
- Cammaerts, M.-C.; Cammaerts, R. Expectative behavior can be acquired by ants in the course of their life. Trends Entomol. 2015, 11, 73–83. [Google Scholar] [CrossRef]
| First Part of the Experiment. | ||||||||
|---|---|---|---|---|---|---|---|---|
| Training: Mean n° of Ants Near the Two Cues | Training: Number of Circles Borne by the Cues | Tests Made at Day | Tests: n° of Ants Sighted Near Each Cue, for Colony A; Colony B | Tests: % of Correct Responses | Tests: n°s of Ants of the Two Colonies Sighted Near Each Cue and Chronologically Ordered by Four | Wilcoxon Test N T P | ||
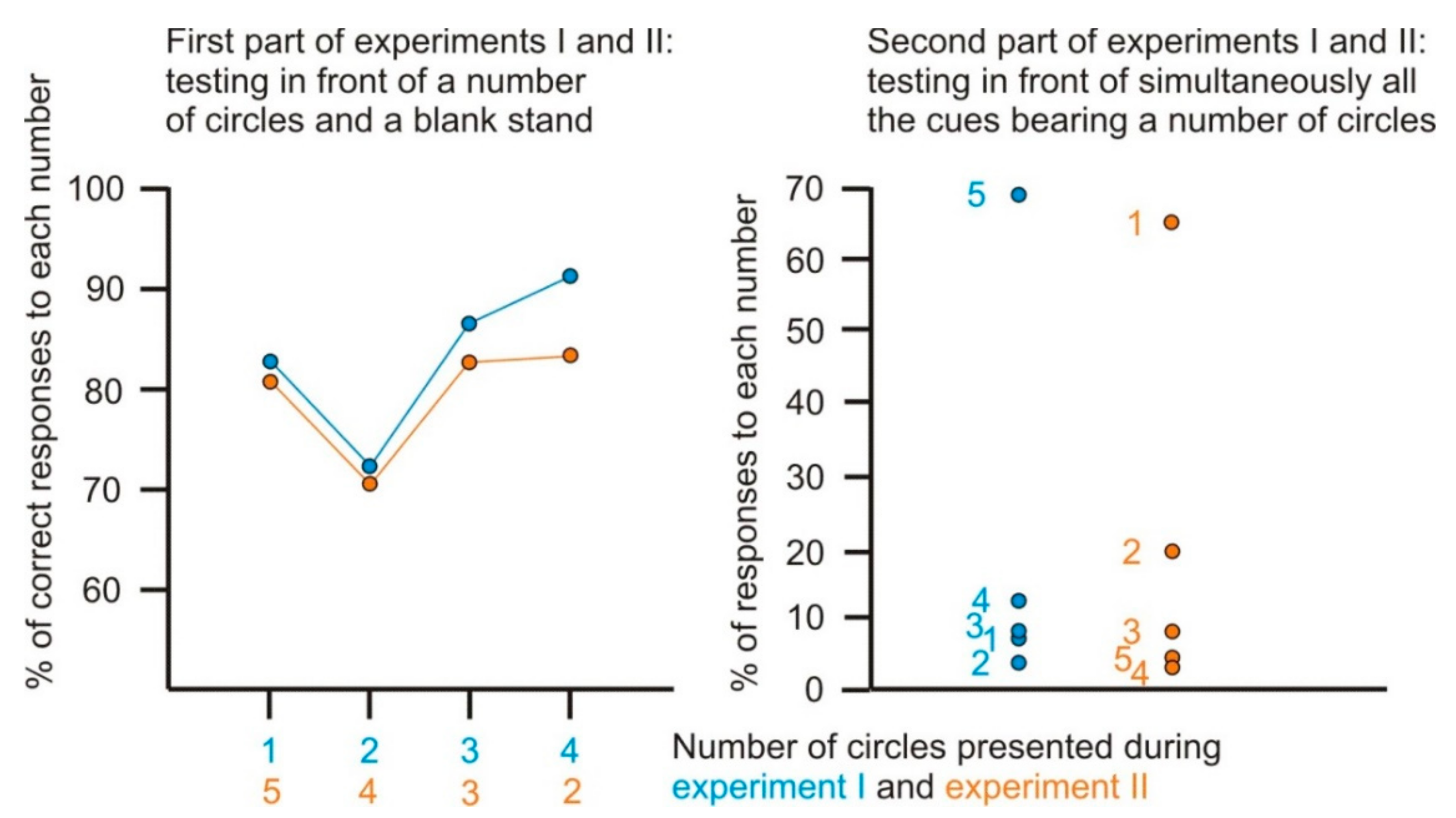
| 4.3 | 1 vs. 0, at days 1, 2 | 2 | 64 vs. 7; 32 vs. 13 | 82.75 | 15,14,24,21,22 vs. 4,3,7,0,6 | 5 15 0.031 | ||
| 5.4 | 2 vs. 0, at days 3, 4 | 4 | 40 vs. 13; 51 vs. 22 | 72.22 | 17,16,15,21,22 vs. 9,7,6,5,8 | 5 15 0.031 | ||
| 6.0 | 3 vs. 0, at days 5, 6 | 6 | 38 vs. 8; 45 vs. 5 | 86.46 | 14,21,20,17,11 vs. 2,3,6,2,0 | 5 15 0.031 | ||
| 6.0 | 4 vs. 0, at days 7, 8 | 8 | 33 vs. 5; 51 vs. 3 | 91.30 | 13,16,19,18,18 vs. 1,0,3,3,1 | 5 15 0.031 | ||
| Second Part of the Experiment. | ||||||||
| At day 9, Presentation of 1, 2, 3, 4, and 5 Circles at the Same Time: | n° of Ants Sighted in Front of Each Number: | |||||||
| Colony A: | 5, | 1, | 4, | 11, 44 | ||||
| Colony B: | 3, | 3, | 5, | 3, 33 | ||||
| Total: | 8, | 4, | 9, | 14 77 | ||||
| χ2 = 119,22, df = 4, p < 0.001 | ||||||||
| First Part of the Experiment | ||||||||
|---|---|---|---|---|---|---|---|---|
| Training: Mean n° of Ants Near the Two Cues | Training: Number of Circles Borne by the Cues | Tests made at day | Tests: n° of Ants Sighted Near Each Cue, for Colony A; Colony B | Tests: % of Correct Responses | Tests: n°s of Ants of the Two Colonies Sighted Near Each Cue and Chronologically Ordered by Four | Wilcoxon test N T P | ||
| 5.5 | 5 vs. 0, at days 1, 2 | 2 | 68 vs. 15; 57 vs. 15 | 80.65 | 22,27,27,19,30 vs. 2,7,6,7,8 | 5 15 0.031 | ||
| 6.1 | 4 vs. 0, at days 3, 4 | 4 | 38 vs. 8; 55 vs. 31 | 70.45 | 12,20,23,22,16 vs. 5,7,11,8,8 | 5 15 0.031 | ||
| 6.1 | 3 vs. 0, at days 5, 6 | 6 | 43 vs. 12; 38 vs. 5 | 82.65 | 16,18,17,17,13 vs. 3,5,7,1,1 | 5 15 0.031 | ||
| 6.5 | 2 vs. 0, at days 7, 8 | 8 | 43 vs. 17; 61 vs. 4 | 83.20 | 21,20,21,23,19 vs. 1,4,4,8,4 | 5 15 0.031 | ||
| Second Part of the Experiment. | ||||||||
| At day 9, Presentation of 1, 2, 3, 4, and 5 Circles at the Same Time: | n° of Ants Sighted in Front of Each Number: | |||||||
| Colony A: | 43, | 17, | 4, | 3 5 | ||||
| Colony B: | 46, | 9 | 7 | 1 1 | ||||
| Total: | 89, | 26 | 11 | 4 6 | ||||
| χ2 = 186.41, df = 4, p < 0.001 | ||||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cammaerts, M.-C.; Cammaerts, R. Ants Can Anticipate the Following Quantity in an Arithmetic Sequence. Behav. Sci. 2021, 11, 18. https://doi.org/10.3390/bs11020018
Cammaerts M-C, Cammaerts R. Ants Can Anticipate the Following Quantity in an Arithmetic Sequence. Behavioral Sciences. 2021; 11(2):18. https://doi.org/10.3390/bs11020018
Chicago/Turabian StyleCammaerts, Marie-Claire, and Roger Cammaerts. 2021. "Ants Can Anticipate the Following Quantity in an Arithmetic Sequence" Behavioral Sciences 11, no. 2: 18. https://doi.org/10.3390/bs11020018
APA StyleCammaerts, M.-C., & Cammaerts, R. (2021). Ants Can Anticipate the Following Quantity in an Arithmetic Sequence. Behavioral Sciences, 11(2), 18. https://doi.org/10.3390/bs11020018






