Remodeling of Biomembranes and Vesicles by Adhesion of Condensate Droplets
Abstract
1. Introduction
2. Condensate Droplets Adhering to Giant Vesicles
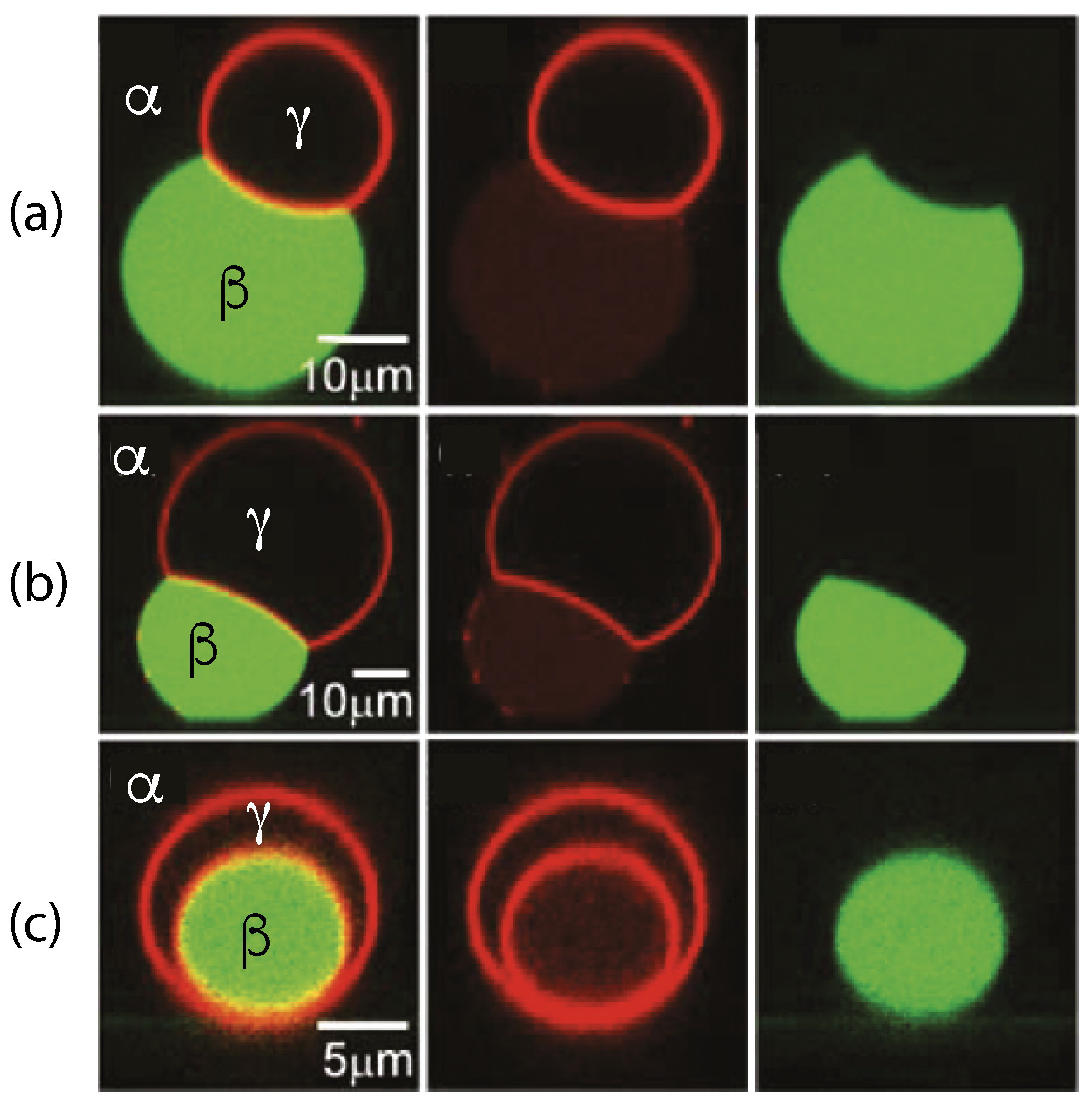
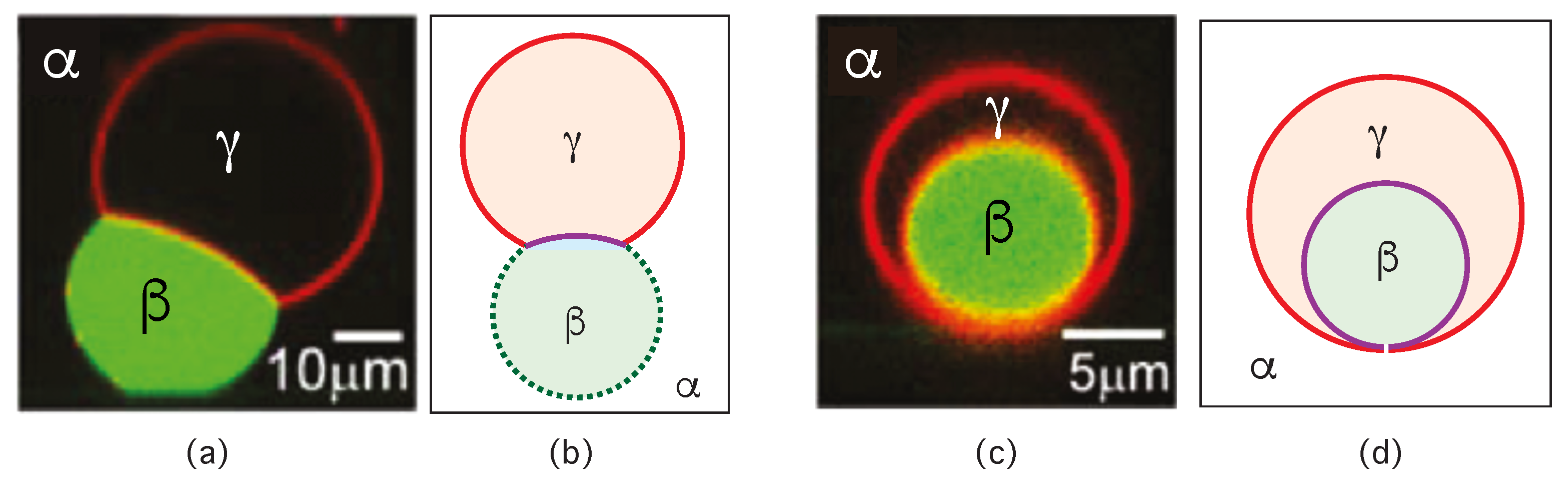
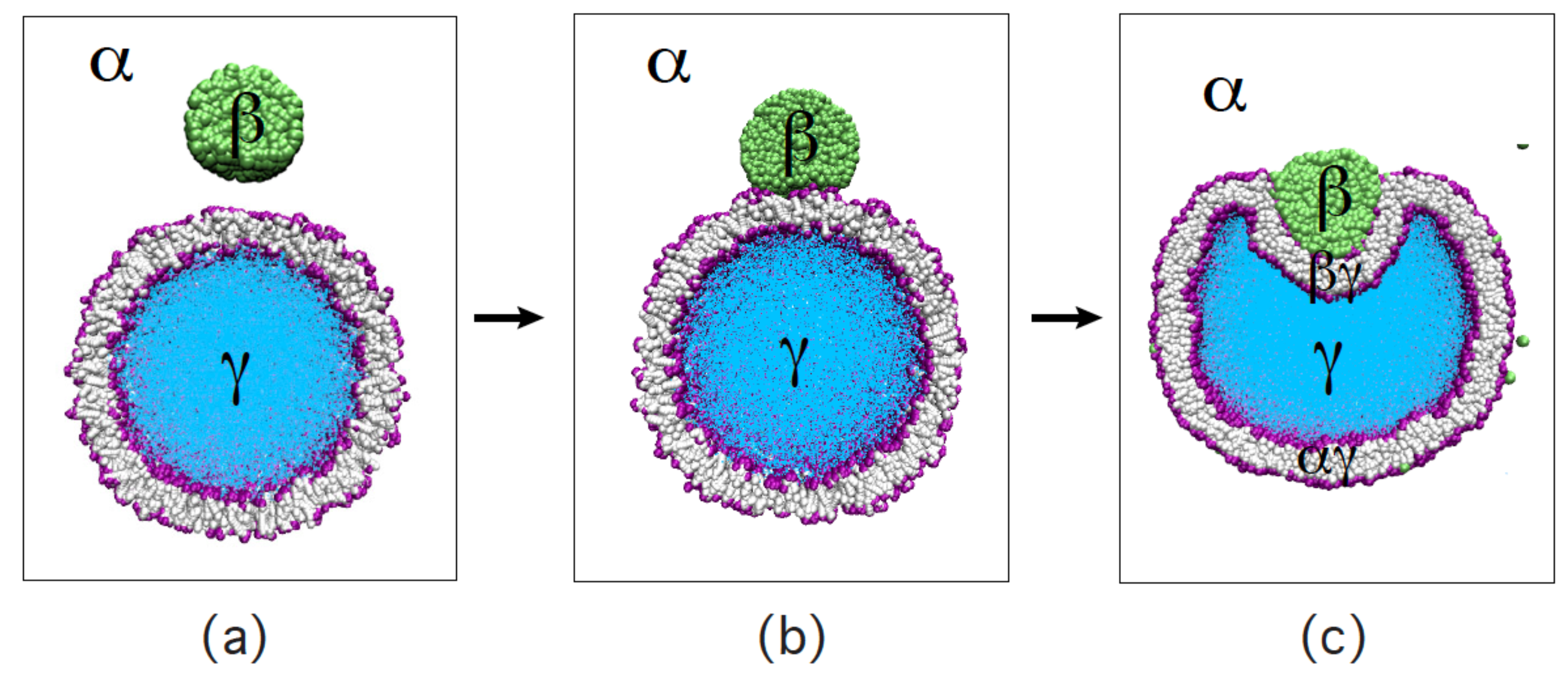
2.1. Different Adhesion and Wetting Morphologies
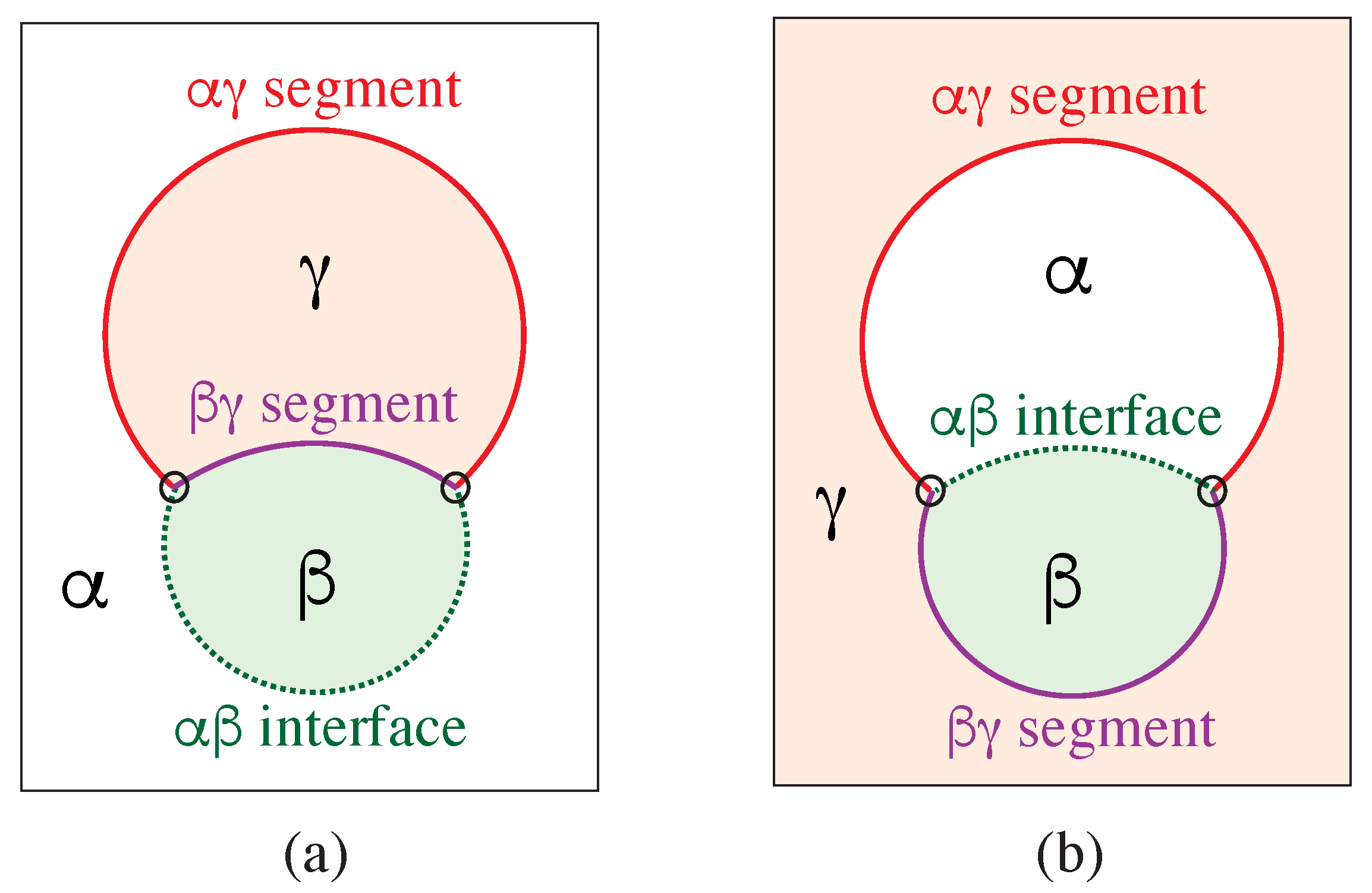
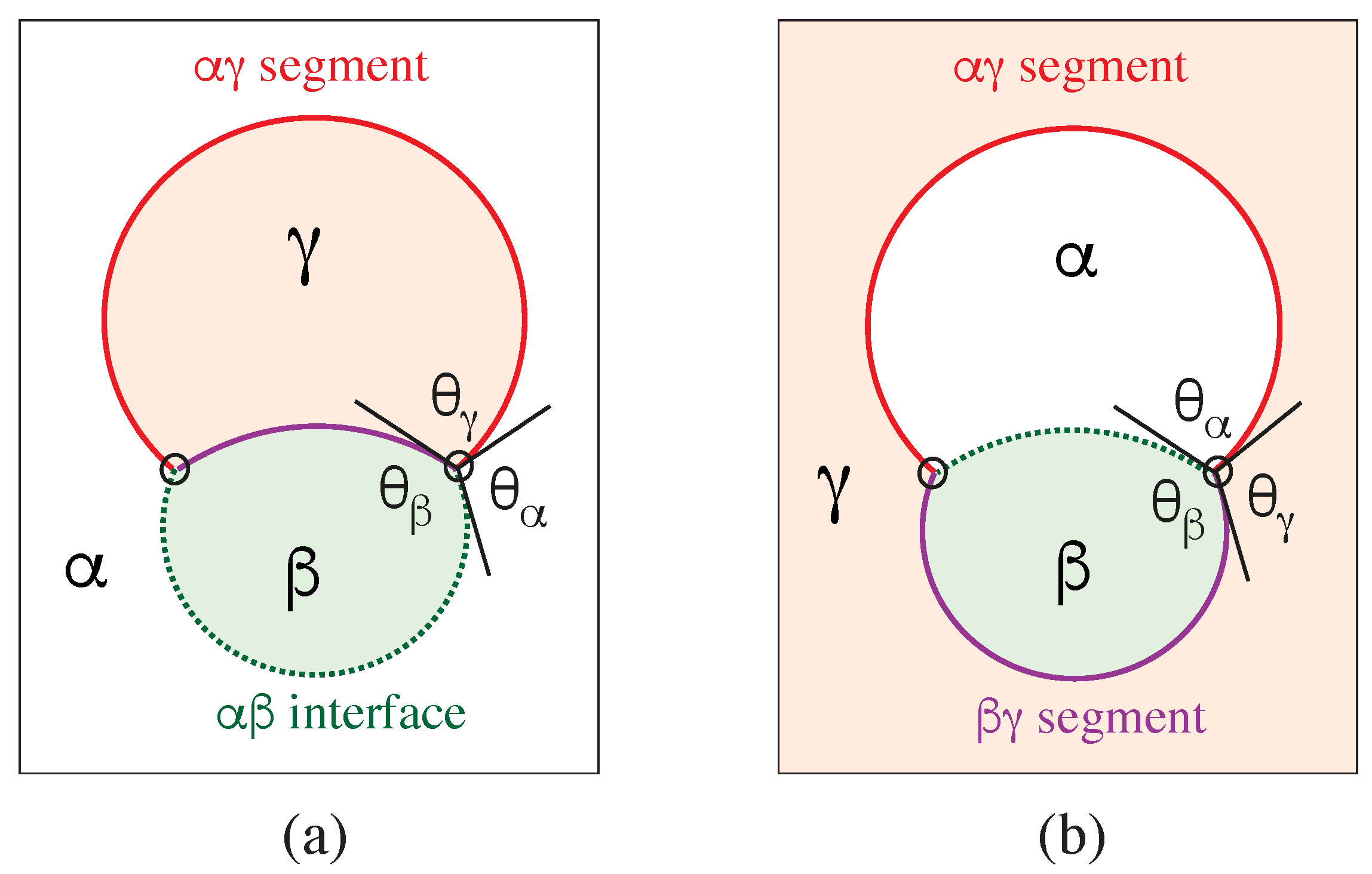
2.2. Basic Geometric Features of the Adhesion Morphologies
3. Phase Diagrams of Some Condensate-Forming Systems
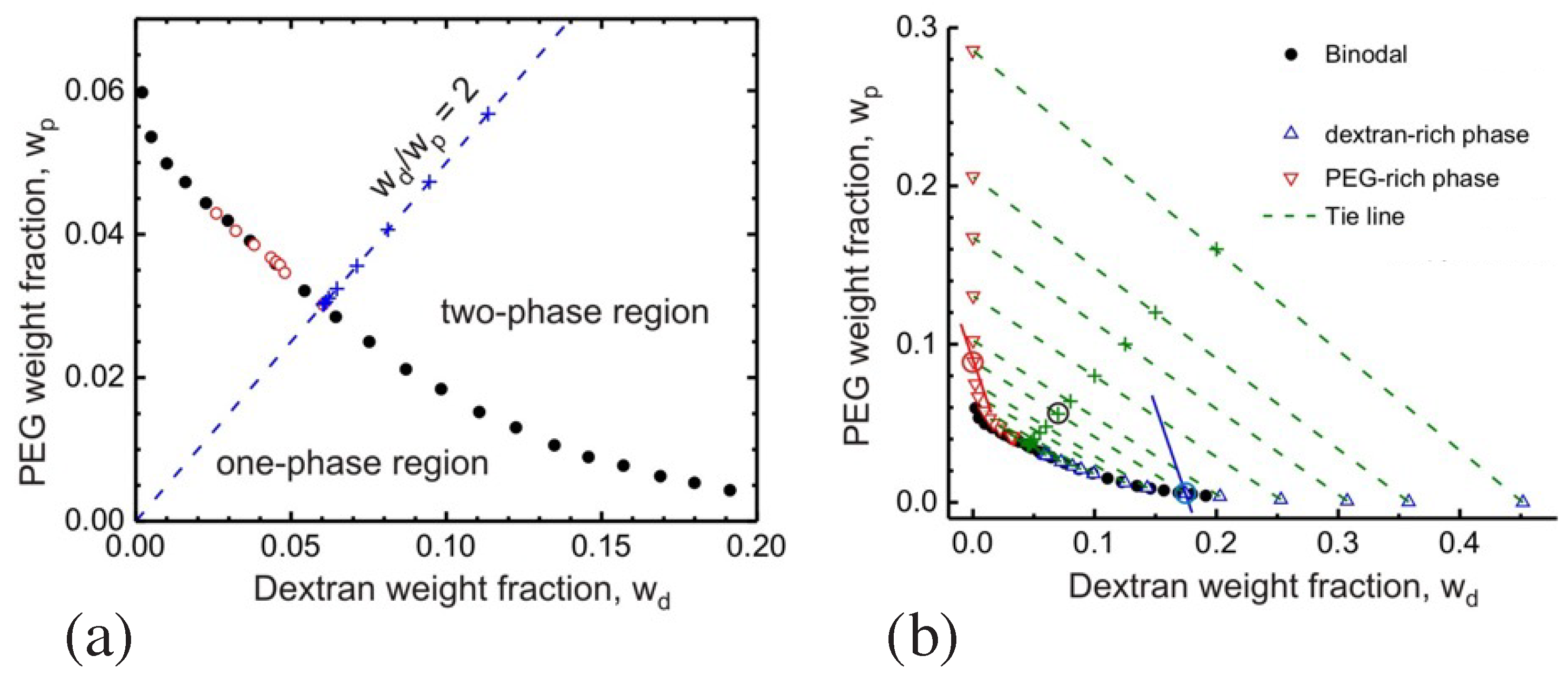
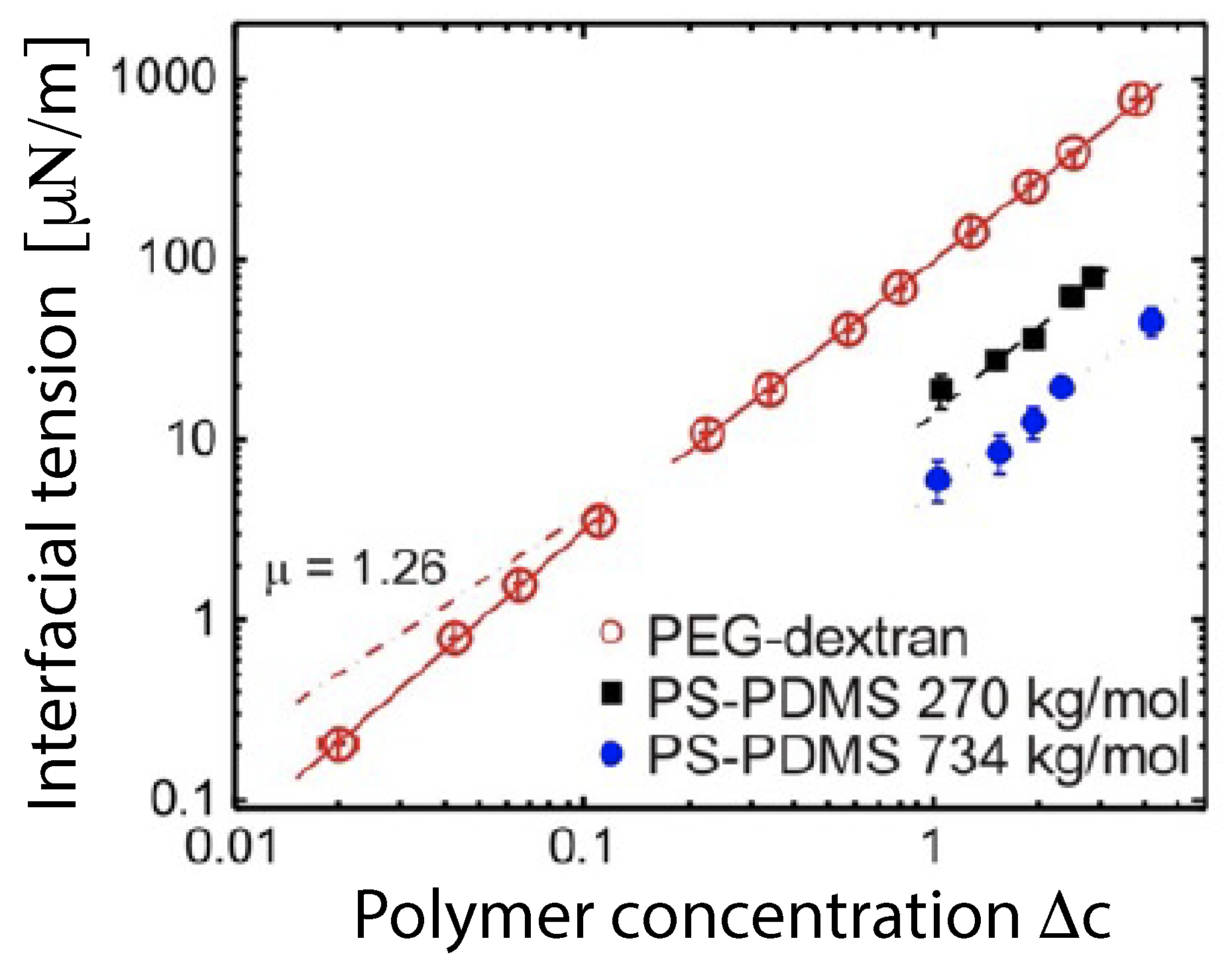
3.1. Aqueous Two-Phase Systems
3.2. Binary Liquid Mixture in Silico
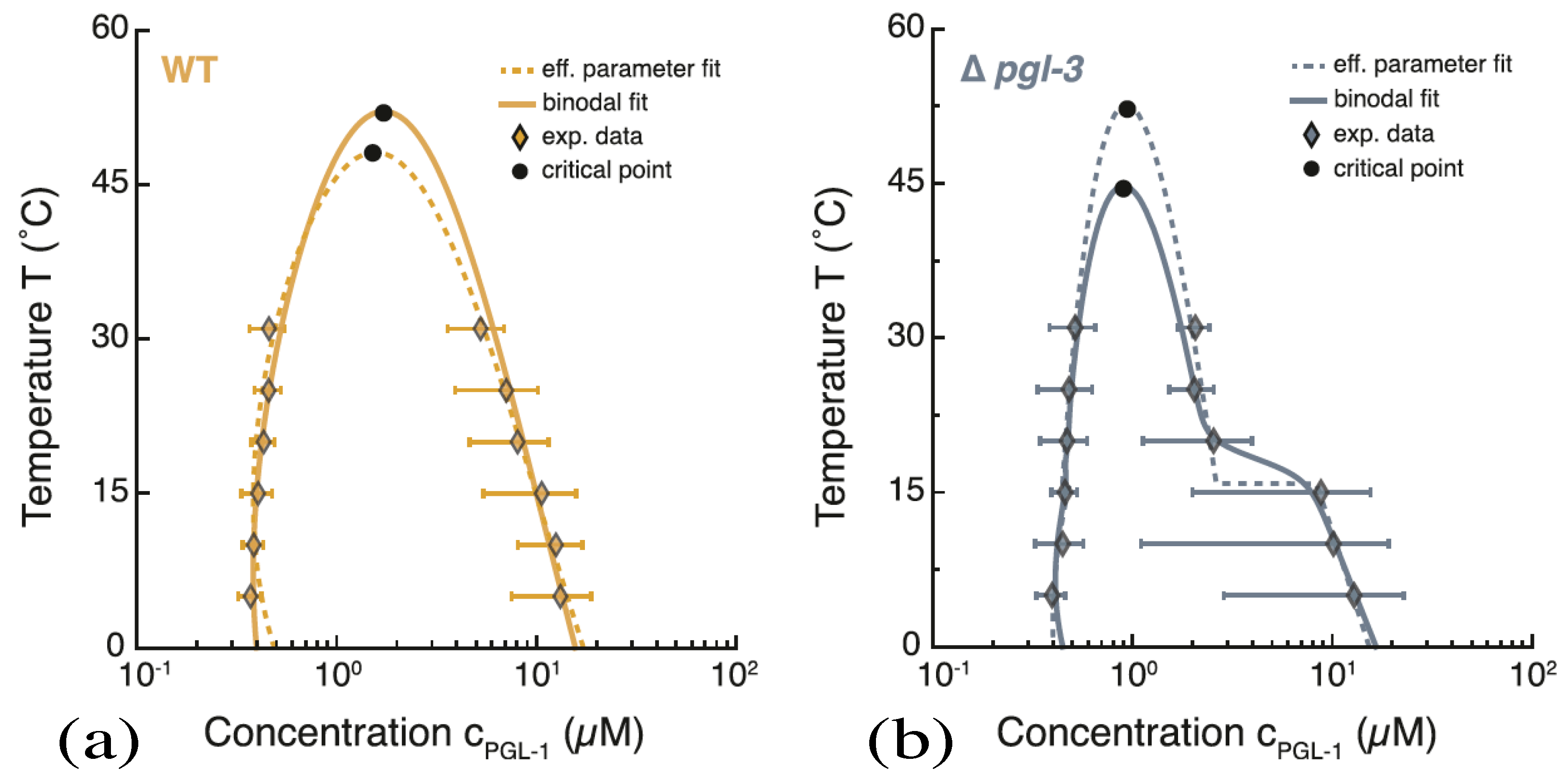
3.3. Phase Behavior of Protein Condensates
4. Contact Angles of Adhesion Morphologies
4.1. Apparent Versus Intrinsic Contact Angles
4.2. Different Wetting Regimes from Apparent Contact Angles
5. Balance of Surface Tensions along Contact Line
5.1. From Apparent Contact Angles to Surface Tensions
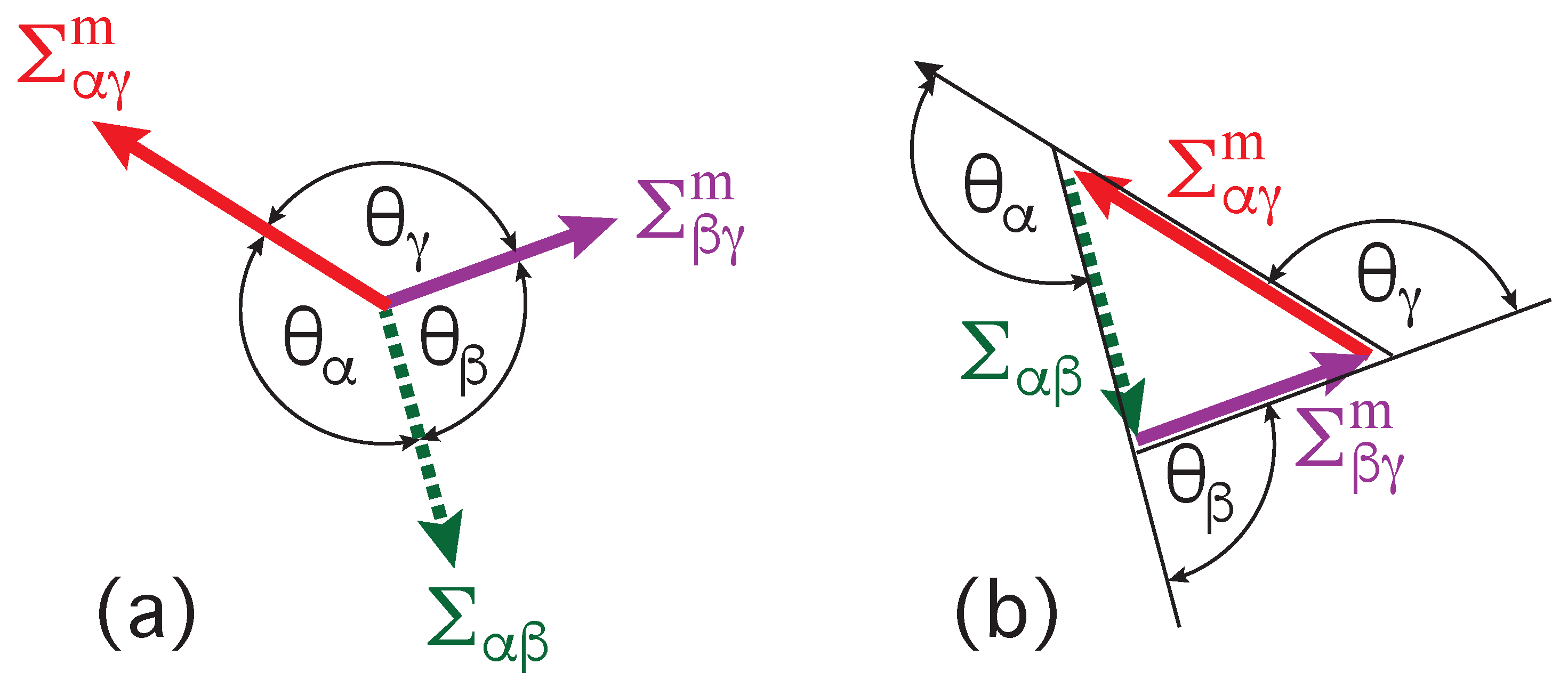
5.2. Triangle Formed by Three Surface Tensions
5.3. General Consequences of the Tension Triangle
5.4. Limit of Small Contact Angle
6. Balanced Surface Tensions and Affinity Contrast
6.1. Force Balance Regime for Surface Tensions
6.2. Affinity Contrast between Coexisting Liquid Phases
6.3. Relation between Affinity Contrast and Apparent Contact Angles
7. Transitions between Different Wetting Morphologies
7.1. Different Morphological Pathways
7.2. Complete-to-Partial Wetting Transitions
7.3. Vesicle-Droplet Systems with Two Wetting Transitions
8. Theory of Curvature Elasticity and Vesicle-Droplet Adhesion
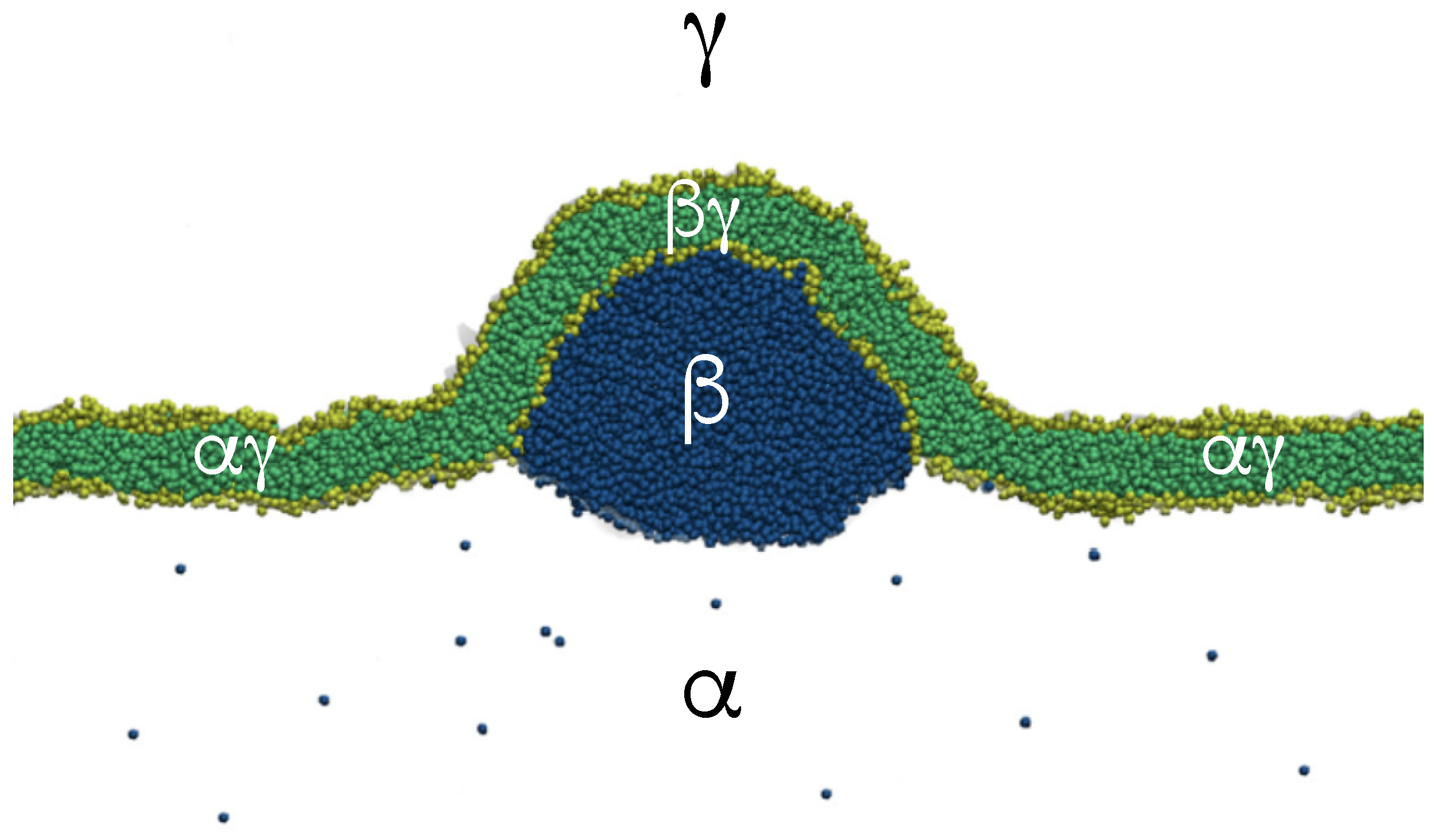
8.1. Fine Structure of Apparent Membrane Kinks
8.2. Curvature and Curvature Elasticity of Membranes
8.3. Shapes of Giant Vesicles in the Absence of Condensate Droplets
8.4. Shape Functional of Vesicle-Droplet System
8.5. Decomposition of Membrane Segment Tensions
8.6. Transfer of Membrane Area between Membrane Segments
9. Intrinsic Contact Angles at Smoothly Curved Membranes
9.1. “No Kink” Requirement and Smoothly Curved Membranes
9.2. Affinity Contrast from Intrinsic Contact Angles
9.3. “No Kink” Requirement and Continuity of Mean Curvature
9.4. Relation between Apparent and Intrinsic Contact Angles
9.5. Force Balance Perpendicular to the Membrane
9.6. Threshold of Droplet Size for the Onset of Adhesion
10. Spontaneous Curvature and Formation of Membrane Nanotubes
10.1. Transbilayer Asymmetry and Spontaneous Curvature
10.2. Different Patterns of Membrane Nanotubes
10.3. Spontaneous Tubulation without Liquid-Liquid Phase Separation
11. Engulfment of Condensate Droplets by Vesicle Membranes
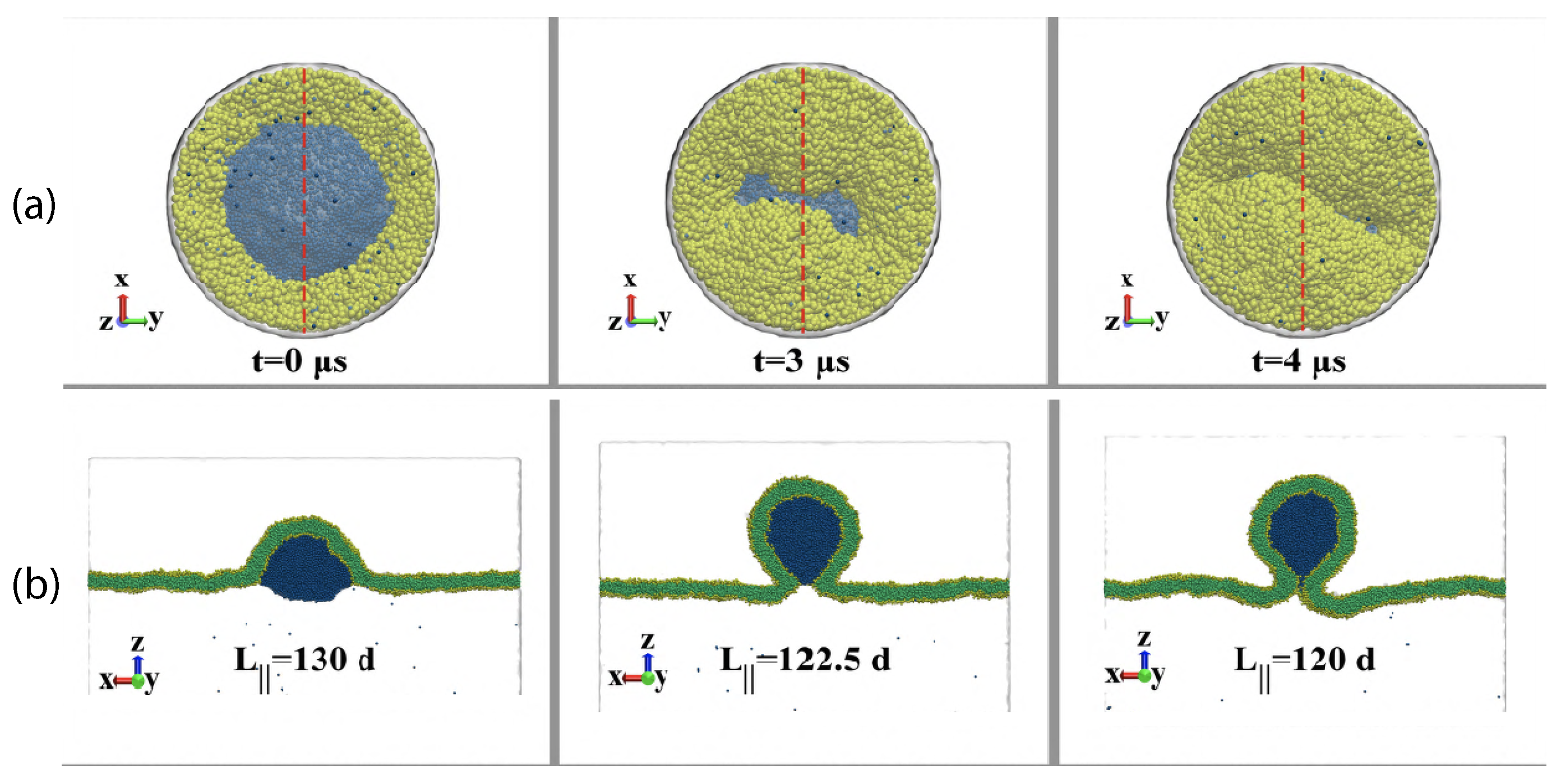
11.1. Partial and Complete Engulfment by Giant Vesicles
11.2. Partial Engulfment by Nanovesicles
11.3. Stalled Engulfment for Sufficiently Large Droplets
12. Line Tension of Contact Line
12.1. Positive and Negative Line Tensions
12.2. Interfacial Tension Versus Line Tension
12.3. Force Balance between Surface Tensions and Line Tension
13. Different Shapes of Closed Membrane Necks
13.1. Tight-Lipped Membrane Necks for Planar Bilayers
13.2. Stress Asymmetry between Two Bilayer Leaflets
13.3. Tight-Lipped Membrane Necks for Small Stress Asymmetries
13.4. Axisymmetric Necks and Endocytosis for Large Stress Asymmetries
14. Summary and Outlook
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CE | Complete engulfment |
| CE | Complete engulfment of an droplet |
| CE | Complete engulfment of a droplet |
| CW | Complete wetting |
| CW | Complete wetting by the phase |
| CW | Complete wetting by the phase |
| DOPC | Dioleoylphosphatidylcholine, a phospholipid |
| DPPC | Dipalmitoylphosphatidylcholine, a phospholipid |
| GFP | Green fluorescent protein |
| GM1 | Monosialotetrahexosylganglioside, a glycolipid |
| GUV | Giant unilamellar vesicle |
| Ld | Liquid disordered lipid phase |
| Lo | Liquid ordered lipid phase |
| PEG | Polyethylene glycol |
| POPC | Palmitoyloleoylphosphatidylcholine, a phospholipid |
| PW | Partial wetting |
| S beads | Solute beads |
| STED | Stimulated Emission Depletion |
| W beads | water beads |
Glossary of Mathematical Symbols
| A | Surface area of membrane |
| Aαβ | Surface area of αβ interface |
| Aαγ | Surface area of αγ membrane segment |
| Aβγ | Surface area of βγ membrane segment |
| A0 | Surface area of tensionless membrane |
| α, β | Two coexisting liquid phases α and β as in Figure 1 |
| αβ | Label for interface between α and β phase |
| αγ | Label for membrane segment between α and γ phase |
| βγ | Label for membrane segment between β and γ phase |
| C1 | First principal curvature of membrane surface |
| C2 | Second principal curvature of membrane surface |
| χ | Euler characteristic of membrane surface |
| d | Diameter of beads in coarse-grained molecular dynamics simulations |
| ΔΣ | Stress asymmetry between two bilayer leaflets, defined by Equation (64) |
| Eαβ | Interfacial free energy of αβ interface, Eαβ = Σαβ Aαβ |
| Ead | Adhesion free energy of vesicle-droplet system as in Equation (35) |
| Ebe | Elastic bending energy of vesicle membrane as in Equation (29) |
| Eco | Free energy of contact line, Eco = λLco, with positive or negative line tension λ |
| Ecu | Elastic curvature energy of vesicle membrane as in Equation (27) |
| EG | Gaussian curvature energy of vesicle membrane as in Equation (28) |
| ηi | Internal angles of tension triangle, ηi = π − θi, see Figure 10b |
| Fex | Shape functional for exterior phase separation as in Equation (38) and Figure 1a |
| Fin | Shape functional for interior phase separation as in Equation (38) and Figure 1b |
| Pressure-dependent term of Fex as defined by Equation (36) | |
| Pressure-dependent term of Fin as defined by Equation (37) | |
| Fve | Shape functional of vesicle without droplet as given by Equation (31) |
| G | Gaussian curvature of membrane surface, G = C1 C2 as in Equation (26) |
| Topological genus of membrane surface | |
| γ | Third liquid phase that plays the role of a spectator phase, see Figure 1 |
| kB | Boltzmann constant |
| KA | Area compressibility modulus of membrane as in Equation (33) |
| κ | Bending rigidity of membrane, which provides the basic energy scale |
| κG | Gaussian curvature modulus of membrane |
| Lco | Length of contact line between droplet and membrane |
| λ | Line tension of contact line between membrane and condensate droplet |
| m | Spontaneous (or preferred) curvature of membrane |
| M | Mean curvature of membrane surface, M = (C1 + C2) as in Equation (25) |
| Pα | Pressure within liquid phase α |
| Pβ | Pressure within liquid phase β |
| Pγ | Pressure within liquid phase γ |
| Pex | Pressure in the exterior solution of freely suspended vesicle |
| Pex | Pressure in the interior solution of freely suspended vesicle |
| ΦS | Mole fraction of solute molecules as in Figure 6 |
| φ | Tilt angle of normal vector for axisymmetric vesicle shape |
| φco | Tilt angle of normal vector at contact line |
| Rco | Radius of circular contact line |
| Rdr | Radius of spherical condensate droplet |
| Threshold value for droplet radius as given by Equation (53) | |
| s | Arc length of vesicle contour for axisymmetric vesicle shape |
| sco | Value of arc length s at the contact line |
| Σ | Lateral stress within membrane, equal to mechanical bilayer tension |
| Σαβ | Interfacial tension of interface between coexisting phases α and β |
| Mechanical tension of αγ membrane segment | |
| Mechanical tension of βγ membrane segment | |
| Σbil | Mechanical tension of bilayer membrane |
| Σil | Mechanical tension of inner leaflet |
| Σol | Mechanical tension of outer leaflet |
| T | Temperature |
| θα | Apparent contact angle between αβ interface and αγ membrane segment |
| θβ | Apparent contact angle between αβ interface and βγ membrane segment |
| θβ | Apparent contact angle between αγ and βγ membrane segments |
| θi | External angles of tension triangle as in Figure 10b |
| Intrinsic contact angle between αβ interface and αγ membrane segment | |
| Intrinsic contact angle between αβ interface and βγ membrane segment | |
| v | Volume-to-area ratio, defined by Equation (48) |
| V | Volume of vesicle |
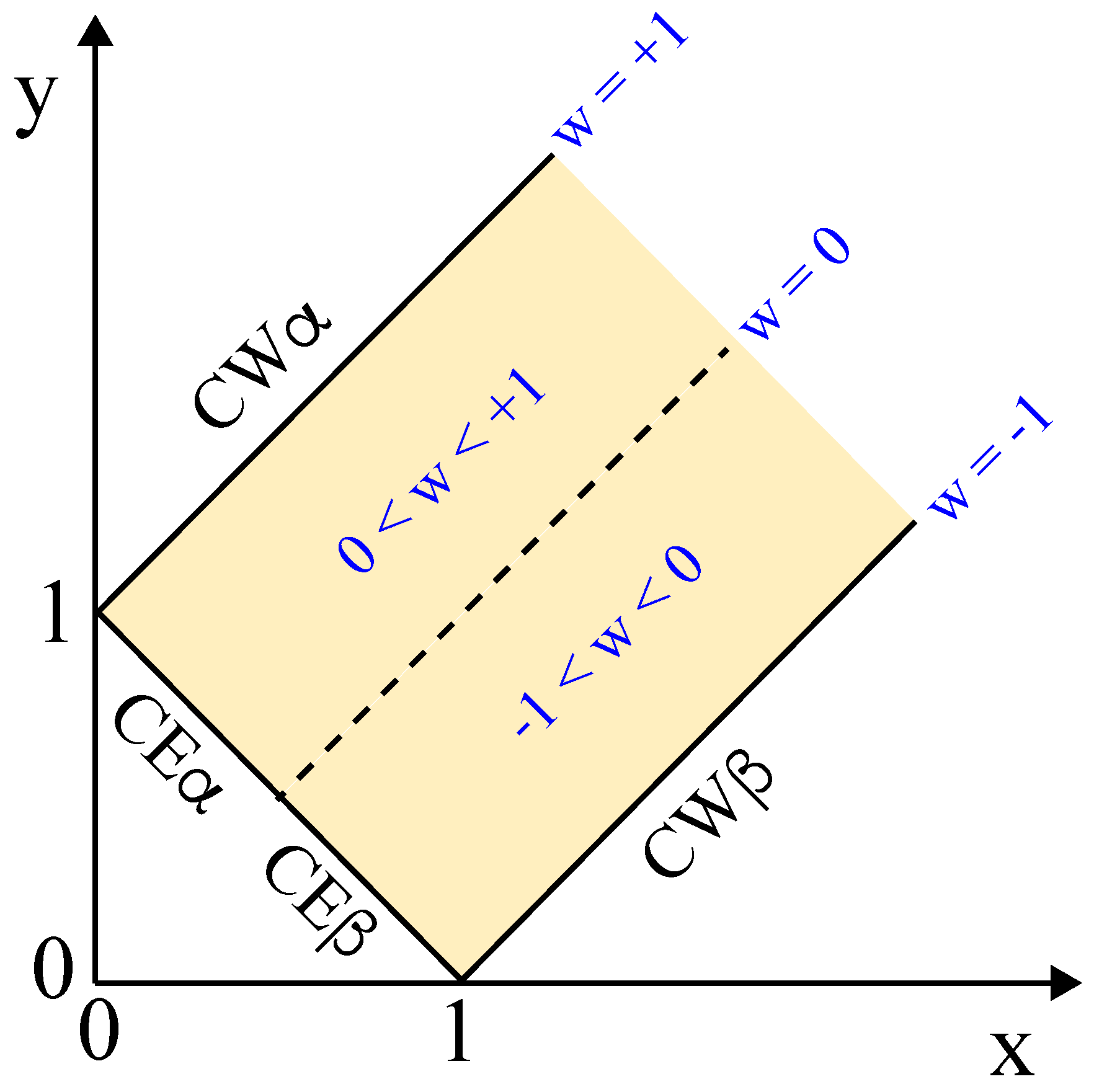
| w | Rescaled affinity contrast, w = W/Σαβ, as in Equation (18) and in Figure 11 and Figure 12 |
| W | Affinity contrast between two coexisting phases α and β, defined by Equation (15) |
| wp | Weight fraction of PEG as in Figure 4 and Figure 13 |
| wd | Weight fraction of dextran as in Figure 4 and Figure 13 |
| x | Rescaled tension of αγ membrane segment, x = as in Figure 11 and Figure 12 |
| y | Rescaled tension of βγ membrane segment, y = as in Figure 11 and Figure 12 |
| ζ | Solubility of solute molecules in water as in Figure 6 |
References
- Lipowsky, R. Remodeling of Membrane Shape and Topology by Curvature Elasticity and Membrane Tension. Adv. Biol. 2022, 6, 2101020. [Google Scholar] [CrossRef]
- Bassereau, P.; Sens, P. (Eds.) Physics of Biological Membranes; Springer Nature: Cham, Switzerland, 2018. [Google Scholar]
- Dimova, R.; Marques, C. (Eds.) The Giant Vesicle Book; Taylor & Francis: Boca Raton, FL, USA, 2020. [Google Scholar]
- Young, T. An Essay on the Cohesion of Fluids. Philos. Trans. R. Soc. Lond. 1805, 95, 65–87. [Google Scholar]
- Kusumaatmaja, H.; Li, Y.; Dimova, R.; Lipowsky, R. Intrinsic contact angle of aqueous phases at membranes and vesicles. Phys. Rev. Lett. 2009, 103, 238103. [Google Scholar] [CrossRef]
- Li, Y.; Lipowsky, R.; Dimova, R. Transition from complete to partial wetting within membrane compartments. J. Am. Chem. Soc. 2008, 130, 12252–12253. [Google Scholar] [CrossRef]
- Li, Y.; Lipowsky, R.; Dimova, R. Membrane nanotubes induced by aqueous phase separation and stabilized by spontaneous curvature. Proc. Natl. Acad. Sci. USA 2011, 108, 4731–4736. [Google Scholar] [CrossRef] [PubMed]
- Lipowsky, R. Spontaneous tubulation of membranes and vesicles reveals membrane tension generated by spontaneous curvature. Faraday Discuss. 2013, 161, 305–331. [Google Scholar] [CrossRef] [PubMed]
- Liu, Y.; Agudo-Canalejo, J.; Grafmüller, A.; Dimova, R.; Lipowsky, R. Patterns of flexible nanotubes formed by liquid-ordered and liquid-disordered membranes. ACS Nano 2016, 10, 463–474. [Google Scholar] [CrossRef]
- Andes-Koback, M.; Keating, C.D. Complete budding and asymmetric division of primitive model cells to produce daughter vesicles with different interior and membrane compositions. J. Am. Chem. Soc. 2011, 133, 9545–9555. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Kusumaatmaja, H.; Lipowsky, R.; Dimova, R. Wetting-induced budding of vesicles in contact with several aqueous phases. J. Phys. Chem. B 2012, 116, 1819–1823. [Google Scholar] [CrossRef] [PubMed]
- Albertsson, P.A. Partition of Cell Particles and Macromolecules: Separation and Purification of Biomolecules, Cell Organelles Membranes, and Cells in Aqueous Polymer Two-Phase Systems and Their Use in Biochemical Analysis and Biotechnology, 3rd ed.; Wiley: New York, NY, USA, 1986. [Google Scholar]
- Esquena, J. Water-in-water (W/W) emulsions. Curr. Opin. Colloid Interface Sci. 2016, 25, 109–119. [Google Scholar] [CrossRef]
- Helfrich, M.; Mangeney-Slavin, L.; Long, M.; Djoko, K.; Keating, C. Aqueous Phase Separation in Giant Vesicles. J. Am. Chem. Soc. 2002, 124, 13374–13375. [Google Scholar] [CrossRef] [PubMed]
- Scholten, E.; Tuinier, R.; Tromp, R.H.; Lekkerkerker, H.N.W. Interfacial Tension of a Decomposed Biopolymer Mixture. Langmuir 2002, 18, 2234–2238. [Google Scholar] [CrossRef]
- Liu, Y.; Lipowsky, R.; Dimova, R. Concentration dependence of the interfacial tension for aqueous two-phase polymer solutions of dextran and polyethylene glycol. Langmuir 2012, 28, 3831–3839. [Google Scholar] [CrossRef] [PubMed]
- Atefi, E.; Adinin Mann, J., Jr.; Tavana, H. Ultralow Interfacial Tensions of Aqueous Two-Phase Systems Measured Using Drop Shape. Langmuir 2014, 30, 9691–9699. [Google Scholar] [CrossRef]
- de Freitas, R.A.; Nicolai, T.; Chassenieux, C.; Benyahia, L. Stabilization of Water-in-Water Emulsions by Polysaccharide-Coated Protein Particles. Langmuir 2016, 32, 1227–1232. [Google Scholar] [CrossRef]
- Crowe, C.D.; Keating, C.D. Liquid–liquid phase separation in artificial cells. Interface Focus 2018, 8, 20180032. [Google Scholar] [CrossRef]
- de Jong, H.G.B.; Kruyt, H.R. Coacervation (Partial miscibility in colloid systems) (Preliminary Communication). Proc. K. Ned. Akad. Wet 1929, 32, 849–856. [Google Scholar]
- Sing, C.E. Development of the modern theory of polymeric complex coacervation. Adv. Colloid Interface Sci. 2017, 239, 2–16. [Google Scholar] [CrossRef]
- Li, L.; Srivastava, S.; Andreev, M.; Marciel, A.B.; de Pablo, J.J.; Tirrell, M.V. Phase Behavior and Salt Partitioning in Polyelectrolyte Complex Coacervates. Macromolecules 2018, 51, 2988–2995. [Google Scholar] [CrossRef]
- Love, C.; Steinkühler, J.; Gonzales, D.T.; Yandrapalli, N.; Robinson, T.; Dimova, R.; Tang, T.Y.D. Reversible pH-Responsive Coacervate Formation in Lipid Vesicles Activates Dormant Enzymatic Reactions. Angew. Chem. 2020, 132, 6006–6013. [Google Scholar] [CrossRef]
- Last, M.G.F.; Deshpande, S.; Dekker, C. pH-Controlled Coacervate-Membrane Interactions within Liposomes. ACS Nano 2020, 14, 4487–4498. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Y.; Chen, Y.; Yang, X.; He, X.; Li, M.; Liu, S.; Wang, K.; Liu, J.; Mann, S. Giant Coacervate Vesicles as an Integrated Approach to Cytomimetic Modeling. J. Am. Chem. Soc. 2021, 143, 2866–2874. [Google Scholar] [CrossRef] [PubMed]
- Cakmak, F.P.; Marianelli, A.M.; Keating, C.D. Phospholipid Membrane Formation Templated by Coacervate Droplets. Langmuir 2021, 37, 10366–10375. [Google Scholar] [CrossRef] [PubMed]
- Lu, T.; Liese, S.; Schoenmakers, L.; Weber, C.A.; Suzuki, H.; Huck, W.T.S.; Spruijt, E. Endocytosis of Coacervates into Liposomes. J. Am. Chem. Soc. 2022, 144, 13451–13455. [Google Scholar] [CrossRef]
- Lipowsky, R. Response of Membranes and Vesicles to Capillary Forces Arising from Aqueous Two-Phase Systems and Water-in-Water Emulsions. J. Phys. Chem. B 2018, 122, 3572–3586. [Google Scholar] [CrossRef] [PubMed]
- Satarifard, V.; Grafmüller, A.; Lipowsky, R. Nanodroplets at Membranes Create Tight-Lipped Membrane Necks via Negative Line Tension. ACS Nano 2018, 12, 12424–12435. [Google Scholar] [CrossRef]
- Ghosh, R.; Satarifard, V.; Lipowsky, R. Different pathways for engulfment and endocytosis of droplets by nanovesicles. Nat. Commun. 2023, in press. [Google Scholar] [CrossRef]
- Brangwynne, C.P.; Eckmann, C.R.; Courson, D.S.; Rybarska, A.; Hoege, C.; Gharakhani, J.; Jülicher, F.; Hyman, A.A. Germline P Granules Are Liquid Droplets That Localize by Controlled Dissolution/Condensation. Science 2009, 324, 1729–1732. [Google Scholar] [CrossRef]
- Fritsch, A.W.; Diaz-Delgadillob, A.F.; Adame-Arana, O.; Hoege, C.; Mittasch, M.; Kreysing, M.; Leaver, M.; Hyman, A.A.; Jülicher, F.; Weber, C.A. Local thermodynamics govern formation and dissolution of Caenorhabditis elegans P granule condensates. Proc. Natl. Acad. Sci. USA 2021, 118, e2102772118. [Google Scholar] [CrossRef]
- Berry, J.; Weber, S.C.; Vaidya, N.; Haatajaa, M.; Brangwynne, C.P. RNA transcription modulates phase transition-driven nuclear body assembly. Proc. Natl. Acad. Sci. USA 2015, 112, E5237–E5245. [Google Scholar] [CrossRef]
- Jain, S.; Wheeler, J.R.; Walters, R.W.; Agrawal, A.; Barsic, A.; Parker, R. ATPase-Modulated Stress Granules Contain a Diverse Proteome and Substructure. Cell 2016, 164, 487–498. [Google Scholar] [CrossRef]
- Banani, S.F.; Lee, H.O.; Hyman, A.A.; Rosen, M.K. Biomolecular condensates: Organizers of cellular biochemistry. Nat. Rev. Mol. Cell Biol. 2017, 18, 285–295. [Google Scholar] [CrossRef] [PubMed]
- Li, P.; Banjade, S.; Cheng, H.C.; Kim, S.; Chen, B.; Guo, L.; Llaguno, M.; Hollingsworth, J.V.; King, D.S.; Banani, S.F.; et al. Phase transitions in the assembly of multivalent signalling proteins. Nature 2012, 483, 336–341. [Google Scholar] [CrossRef]
- Patel, A.; Lee, H.O.; Jawerth, L.; Maharana, S.; Jahnel, M.; Hein, M.Y.; Stoynov, S.; Mahamid, J.; Saha, S.; Franzmann, T.M.; et al. A Liquid-to-Solid Phase Transition of the ALS Protein FUS Accelerated by Disease Mutation. Cell 2015, 162, 1066–1077. [Google Scholar] [CrossRef] [PubMed]
- Lin, Y.; Protter, D.S.W.; Rosen, M.K.; Parker, R. Formation and Maturation of Phase-Separated Liquid Droplets by RNA-Binding Proteins. Mol. Cell 2015, 60, 208–219. [Google Scholar] [CrossRef]
- Molliex, A.; Temirov, J.; Lee, J.; Kim, H.J.; Mittag, T.; Taylor, J.P. Phase Separation by Low Complexity Domains Promotes Stress Granule Assembly and Drives Pathological Fibrillization. Cell 2015, 163, 123–133. [Google Scholar] [CrossRef]
- Han, T.W.; Kato, M.; Xie, S.; Wu, L.C.; Mirzaei, H.; Pei, J.; Chen, M.; Xie, Y.; Allen, J.; Xiao, G.; et al. Cell-free Formation of RNA Granules: Bound RNAs Identify Features and Components of Cellular Assemblies. Cell 2012, 149, 768–779. [Google Scholar] [CrossRef] [PubMed]
- Guo, L.; Shorter, J. It’s Raining Liquids: RNA Tunes Viscoelasticity and Dynamics of Membraneless Organelles. Mol. Cell 2015, 60, 189–192. [Google Scholar] [CrossRef] [PubMed]
- Milovanovic, D.; Wu, Y.; Bian, X.; Camilli, P.D. A liquid phase of synapsin and lipid vesicles. Science 2018, 361, 604–607. [Google Scholar] [CrossRef]
- Ma, W.; Mayr, C. A Membraneless Organelle Associated with the Endoplasmic Reticulum Enables 3’UTR-Mediated Protein-Protein Interactions. Cell 2018, 175, 1492–1506. [Google Scholar] [CrossRef]
- Bergeron-Sandoval, L.P.; Kumar, S.; Heris, H.K.; Chang, C.; Cornell, C.E.; Keller, S.L.; François, P.; Hendricks, A.; Ehrlicher, A.J.; Pappu, R.V.; et al. Proteins with prion-like domains form viscoelastic condensates that enable membrane remodeling and endocytosis. Proc. Natl. Acad. Sci. USA 2021, 118, e2113789118. [Google Scholar] [CrossRef]
- Day, K.J.; Kago, G.; Wang, L.; Richter, J.B.; Hayden, C.C.; Lafer, E.M.; Stachowiak, J.C. Liquid-like protein interactions catalyse assembly of endocytic vesicles. Nat. Cell Biol. 2021, 23, 366–376. [Google Scholar] [CrossRef] [PubMed]
- Witkowska, A.; Haucke, V. Liquid-like protein assemblies initiate endocytosis. Nat. Cell Biol. 2021, 23, 301–302. [Google Scholar] [CrossRef]
- Snead, W.T.; Jalihal, A.P.; Gerbich, T.M.; Seim, I.; Hu, Z.; Gladfelter, A.S. Membrane surfaces regulate assembly of ribonucleoprotein condensates. Nat. Cell Biol. 2022, 24, 461–470. [Google Scholar] [CrossRef] [PubMed]
- Lipowsky, R. Multispherical shapes of vesicles highlight the curvature elasticity of biomembranes. Adv. Colloid Interface Sci. 2022, 301, 102613. [Google Scholar] [CrossRef]
- Widom, B. Surface Tension and Molecular Correlations Near the Critical Point. J. Chem. Phys. 1965, 43, 3892–3897. [Google Scholar] [CrossRef]
- Ghosh, R.; Satarifard, V.; Grafmüller, A.; Lipowsky, R. Budding and Fission of Nanovesicles induced by membrane adsorption of small solutes. ACS Nano 2021, 15, 7237–7248. [Google Scholar] [CrossRef] [PubMed]
- Chen, N.; Zhao, Z.; Wang, Y.; Dimova, R. Resolving the Mechanisms of Soy Glycinin Self-Coacervation and Hollow-Condensate Formation. ACS Macro Lett. 2020, 9, 1844–1852. [Google Scholar] [CrossRef]
- Zhao, Z.; Roy, D.; Steinkühler, J.; Robinson, T.; Lipowsky, R.; Dimova, R. Super-resolution imaging of highly curved membrane structures in giant vesicles encapsulating molecular condensates. Adv. Mater. 2022, 34, 2106633. [Google Scholar] [CrossRef]
- Lipowsky, R. Remodeling of Membrane Compartments: Some Consequences of Membrane Fluidity. Biol. Chem. 2014, 395, 253–274. [Google Scholar] [CrossRef]
- Rowlinson, J.; Widom, B. Molecular Theory of Capillarity; Oxford University Press: New York, NY, USA, 1989. [Google Scholar]
- Veatch, S.; Keller, S. Separation of liquid phases in giant vesicles of ternary mixtures of phospholipids and cholesterol. Biophys. J. 2003, 85, 3074–3083. [Google Scholar] [CrossRef]
- Tian, A.; Capraro, B.R.; Esposito, C.; Baumgart, T. Bending Stiffness Depends on Curvature of Ternary Lipid Mixture Tubular Membranes. Biophys. J. 2009, 97, 1636–1646. [Google Scholar] [CrossRef] [PubMed]
- Heinrich, M.; Tian, A.; Esposito, C.; Baumgart, T. Dynamic sorting of lipids and proteins in membrane tubes with a moving phase boundary. Proc. Natl. Acad. Sci. USA 2010, 107, 7208–7213. [Google Scholar] [CrossRef]
- Zhao, X.; Bartolucci, G.; Honigmann, A.; Jülicher, F.; .Weber, C.A. Thermodynamics of wetting, prewetting and surface phase transitions with surface binding. New J. Phys. 2021, 23, 123003. [Google Scholar] [CrossRef]
- Lipowsky, R. Critical surface phenomena at first order bulk transitions. Phys. Rev. Lett. 1982, 49, 1575–1578. [Google Scholar] [CrossRef]
- Lipowsky, R.; Speth, W. Semi-infinite systems with first order bulk transitions. Phys. Rev. B 1983, 28, 3983–3993. [Google Scholar] [CrossRef]
- Mangiarotti, A.; Chen, N.; Zhao, Z.; Lipowsky, R.; Dimova, R. Wetting and complex remodeling of membranes by biomolecular condensates. 2023; under review. [Google Scholar]
- Cahn, J.W. Critical point wetting. J. Chem. Phys. 1977, 66, 3667–3672. [Google Scholar] [CrossRef]
- Lipowsky, R. Upper critical dimension for wetting in systems with long–range forces. Phys. Rev. Lett. 1984, 52, 1429–1432. [Google Scholar] [CrossRef]
- Gau, H.; Herminghaus, S.; Lenz, P.; Lipowsky, R. Liquid Morphologies on Structured Surfaces: From Microchannels to Microchips. Science 1999, 283, 46–49. [Google Scholar] [CrossRef]
- Bonn, D.; Eggers, J.; Indekeu, J.; Meunier, J.; Rolley, E. Wetting and Spreading. Rev. Mod. Phys. 2009, 81, 739–805. [Google Scholar] [CrossRef]
- Goetz, R.; Gompper, G.; Lipowsky, R. Mobilitiy and elasticity of self-assembled membranes. Phys. Rev. Lett. 1999, 82, 221–224. [Google Scholar] [CrossRef]
- do Carmo, M. Differential Geometry of Curves and Surfaces; Prentice-Hall: Englewood Cliffs, NJ, USA, 1976. [Google Scholar]
- Lipowsky, R. Understanding giant vesicles: A theoretical perspective. In The Giant Vesicle Book; Dimova, R., Marques, C., Eds.; Taylor & Francis: Boca Raton, FL, USA, 2020; Chapter 5; pp. 73–168. [Google Scholar]
- Helfrich, W. Elastic Properties of Lipid Bilayers: Theory and Possible Experiments. Z. Naturforsch. 1973, 28, 693–703. [Google Scholar] [CrossRef]
- Seifert, U.; Berndl, K.; Lipowsky, R. Shape transformations of vesicles: Phase diagram for spontaneous curvature and bilayer coupling model. Phys. Rev. A 1991, 44, 1182–1202. [Google Scholar] [CrossRef]
- Deuling, H.; Helfrich, W. The Curvature Elasticity of Fluid Membranes: A Catalogue of Vesicle Shapes. J. Phys. 1976, 37, 1335–1345. [Google Scholar] [CrossRef]
- Bhatia, T.; Christ, S.; Steinkühler, J.; Dimova, R.; Lipowsky, R. Simple sugars shape giant vesicles into multispheres with many membrane necks. Soft Matter 2020, 16, 1246–1258. [Google Scholar] [CrossRef] [PubMed]
- Steinkühler, J.; Knorr, R.L.; Zhao, Z.; Bhatia, T.; Bartelt, S.; Wegner, S.; Dimova, R.; Lipowsky, R. Controlled division of cell-sized vesicles by low densities of membrane-bound proteins. Nat. Commun. 2020, 11, 905. [Google Scholar] [CrossRef]
- Seifert, U.; Miao, L.; Döbereiner, H.G.; Wortis, M. Budding transition for bilayer fluid vesicles. In The Structure and Conformation of Amphiphilic Membranes; Lipowsky, R., Richter, D., Kremer, K., Eds.; Springer Proceedings in Physics; Springer: Berlin/Heidelberg, Germany, 1992; pp. 93–96. [Google Scholar]
- Döbereiner, H.G.; Evans, E.; Kraus, M.; Seifert, U.; Wortis, M. Mapping vesicle shapes into the phase diagram: A comparison of experiment and theory. Phys. Rev. E 1997, 55, 4458–4474. [Google Scholar] [CrossRef]
- Svetina, S.; Žekš, B. Shape Behavior of Lipid Vesicles as the Basis of Some Cellular Processes. Anat. Rec. 2002, 268, 215–225. [Google Scholar] [CrossRef] [PubMed]
- Lipowsky, R. Coupling of bending and stretching deformations in vesicle membranes. Adv. Colloid Interface Sci. 2014, 208, 14–24. [Google Scholar] [CrossRef]
- Jülicher, F.; Lipowsky, R. Shape transformations of inhomogeneous vesicles with intramembrane domains. Phys. Rev. E 1996, 53, 2670–2683. [Google Scholar] [CrossRef]
- Seifert, U.; Lipowsky, R. Adhesion of vesicles. Phys. Rev. A 1990, 42, 4768–4771. [Google Scholar] [CrossRef] [PubMed]
- Agudo-Canalejo, J.; Lipowsky, R. Critical Particle Sizes for the Engulfment of Nanoparticles by Membranes and Vesicles with Bilayer Asymmetry. ACS Nano 2015, 9, 3704–3720. [Google Scholar] [CrossRef]
- Lipowsky, R.; Dimova, R. Introduction to remodeling of biomembranes. Soft Matter 2021, 17, 214–221. [Google Scholar] [CrossRef] [PubMed]
- Lipowsky, R.; Döbereiner, H.G. Vesicles in contact with nanoparticles and colloids. Europhys. Lett. 1998, 43, 219–225. [Google Scholar] [CrossRef]
- Różycki, B.; Lipowsky, R. Spontaneous curvature of bilayer membranes from molecular simulations: Asymmetric lipid densities and asymmetric adsorption. J. Chem. Phys. 2015, 142, 054101. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Z.; Satarifard, V.; Lipowsky, R.; Dimova, R. Membrane nanotubes transform into double-membrane sheets at condensate droplet interfaces. 2023; in preparation. [Google Scholar]
- Bhatia, T.; Agudo-Canalejo, J.; Dimova, R.; Lipowsky, R. Membrane Nanotubes Increase the Robustness of Giant Vesicles. ACS Nano 2018, 12, 4478–4485. [Google Scholar] [CrossRef]
- Aureli, M.; Mauri, L.; Ciampa, M.G.; Prinetti, A.; Toffano, G.; Secchieri, C.; Sonnino, S. GM1 Ganglioside: Past Studies and Future Potential. Mol. Neurobiol. 2016, 53, 1824–1842. [Google Scholar] [CrossRef]
- Schengrund, C.L. Gangliosides: Glycosphingolipids essential for normal neural development and function. Trends Biochemical. Sci. 2015, 40, 397–406. [Google Scholar] [CrossRef]
- Ewers, H.; Römer, W.; Smith, A.E.; Bacia, K.; Dmitrieff, S.; Chai, W.; Mancini, R.; Kartenbeck, J.; Chambon, V.; Berland, L.; et al. GM1 structure determines SV40-induced membrane invagination and infection. Nature Cell Biol. 2010, 12, 11–18. [Google Scholar] [CrossRef] [PubMed]
- Kusumaatmaja, H.; Lipowsky, R. Droplet-induced budding transitions of membranes. Soft Matter 2011, 7, 6914–6919. [Google Scholar] [CrossRef]
- Osserman, R. The Isoperimetric Inequality. Bull. Am. Math. Soc. 1978, 84, 1182–1238. [Google Scholar] [CrossRef]
- Hildebrandt, S.; Tromba, A. Mathematics and Optimal Form; American Scientific Books, Inc.: New York, NY, USA, 1985. [Google Scholar]
- Gibbs, J.W. The Scientific Papers of J. Willard Gibbs; Longman, Green, and Co.: London, UK, 1906; Volume I. [Google Scholar]
- Berg, J.K.; Weber, C.M.; Riegler, H. Impact of Negative Line Tension on the Shape of Nanometer-Size Sessile Droplets. Phys. Rev. Lett. 2010, 105, 076103. [Google Scholar] [CrossRef]
- Matsubara, H.; Ushijima, B.; Law, B.M.; Takiue, T.; Aratono, M. Line Tension of Alkane Lenses on Aqueous Surfactant Solutions at Phase Transitions of Coexisting Interfaces. Adv. Colloid Interface Sci. 2014, 206, 186–194. [Google Scholar] [CrossRef] [PubMed]
- Weijs, J.H.; Marchand, A.; Andreotti, B.; Lohse, D.; Snoeijer, J.H. Origin of Line Tension for a Lennard-Jones Nanodroplet. Phys. Fluids 2011, 23, 022001. [Google Scholar] [CrossRef]
- Geminard, J.C.; Zywocinski, A.; Caillier, F.; Oswald, P. Observation of Negative Line Tensions from Plateau Border Regions in Dry Foam Films. Philos. Mag. Lett. 2004, 84, 199–204. [Google Scholar] [CrossRef]
- Satarifard, V.; Lipowsky, R. Mutual remodeling of interacting nanodroplets and vesicles. Commun. Phys. 2023, 6, 6. [Google Scholar] [CrossRef]
- Brakke, K. The Surface Evolver. Exp. Math. 1992, 1, 141–165. [Google Scholar] [CrossRef]
- Sreekumari, A.; Lipowsky, R. Lipids with Bulky Head Groups Generate Large Membrane Curvatures by Small Compositional Asymmetries. J. Chem. Phys. 2018, 149, 084901. [Google Scholar] [CrossRef]
- Ghosh, R.; Satarifard, V.; Grafmüller, A.; Lipowsky, R. Spherical Nanovesicles Transform into a Multitude of Nonspherical Shapes. Nano Lett. 2019, 19, 7703–7711. [Google Scholar] [CrossRef] [PubMed]
- Lipowsky, R. Understanding and controlling the morphological complexity of biomembranes. In Multiresponsive Behavior of Biomembranes and Giant Vesicles; Advances in Biomembranes and Lipid Self-Assembly; Academic Press: Cambridge, MA, USA, 2019; Volume 30, Chapter 3; pp. 105–155. [Google Scholar]
- Dimova, R.; Lipowsky, R. Giant Vesicles Exposed to Aqueous Two-Phase Systems: Membrane Wetting, Budding Processes, and Spontaneous Tubulation. Adv. Mater. Interfaces 2017, 4, 1600451. [Google Scholar] [CrossRef]
- Helfrich, W.; Harbich, W. Equilibrium Configurations of Fluid Membranes. In Physics of Amphiphilic Layers; Meunier, J., Langevin, D., Boccara, N., Eds.; Springer Proceedings in Physics; Springer: Berlin/Heidelberg, Germany, 1987; Volume 21, pp. 58–63. [Google Scholar]
- Derzhanski, A.; Petrov, A.G.; Mitov, M.D. Molecular Asymmetry and Saddle-Splay Elasticity in Lipid Bilayers. Ann. Phys. 1978, 3, 297. [Google Scholar] [CrossRef]
- Lorenzen, S.; Servuss, R.M.; Helfrich, W. Elastic Torques about Membrane Edges: A Study of Pierced Egg Lecithin Vesicles. Biophys. J. 1986, 50, 565–572. [Google Scholar] [CrossRef] [PubMed]
- Hu, M.; Briguglio, J.J.; Deserno, M. Determining the Gaussian Curvature Modulus of Lipid Membranes in Simulations. Biophys. J. 2012, 102, 1403–1410. [Google Scholar] [CrossRef] [PubMed]
- Shillcock, J.; Lipowsky, R. Tension-induced fusion of bilayer membranes and vesicles. Nat. Mater. 2005, 4, 225–228. [Google Scholar] [CrossRef]
- Grafmüller, A.; Shillcock, J.; Lipowsky, R. Pathway of Membrane Fusion with Two Tension-Dependent Energy Barriers. Phys. Rev. Lett. 2007, 98, 218101. [Google Scholar] [CrossRef]



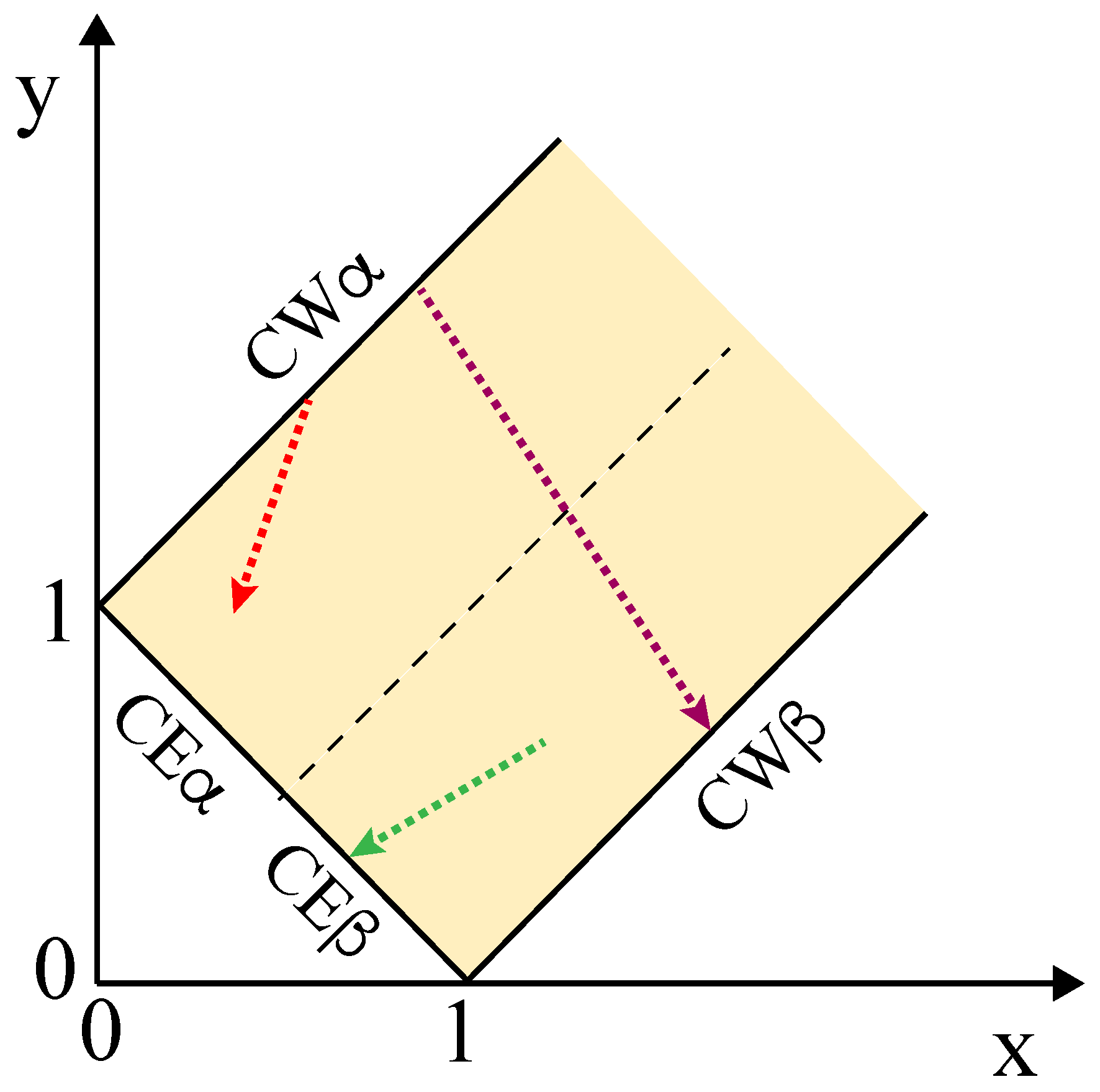
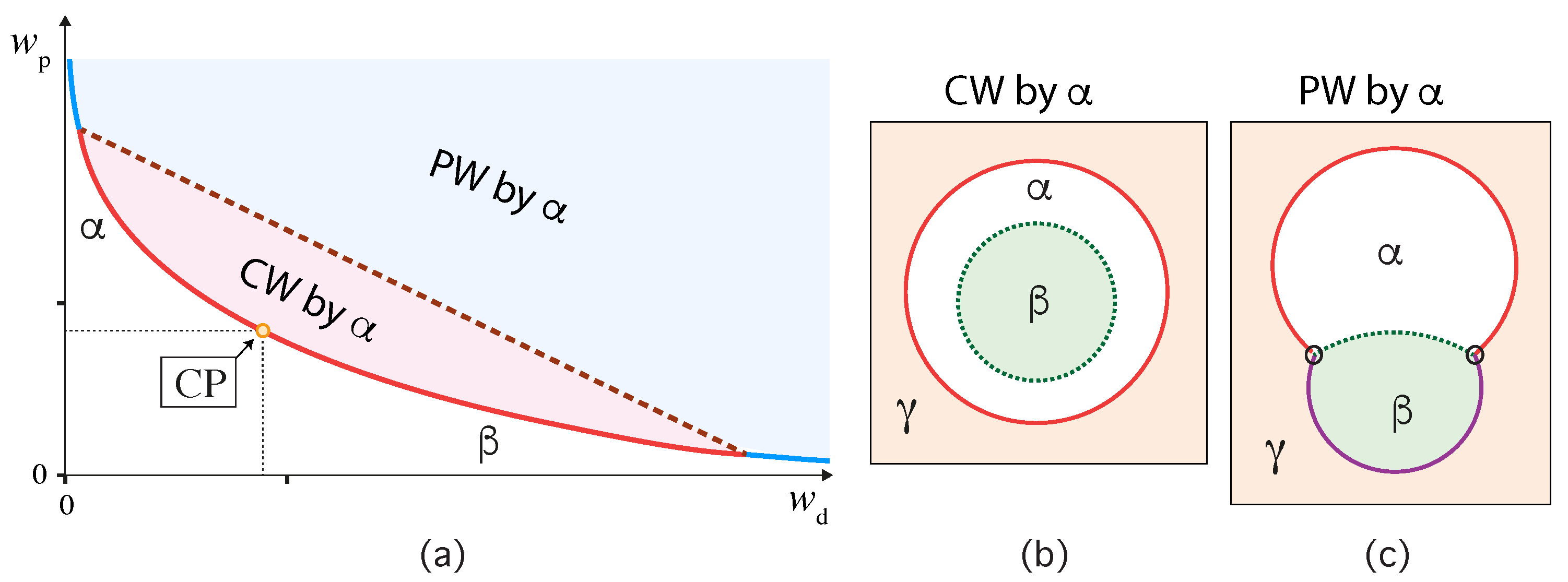


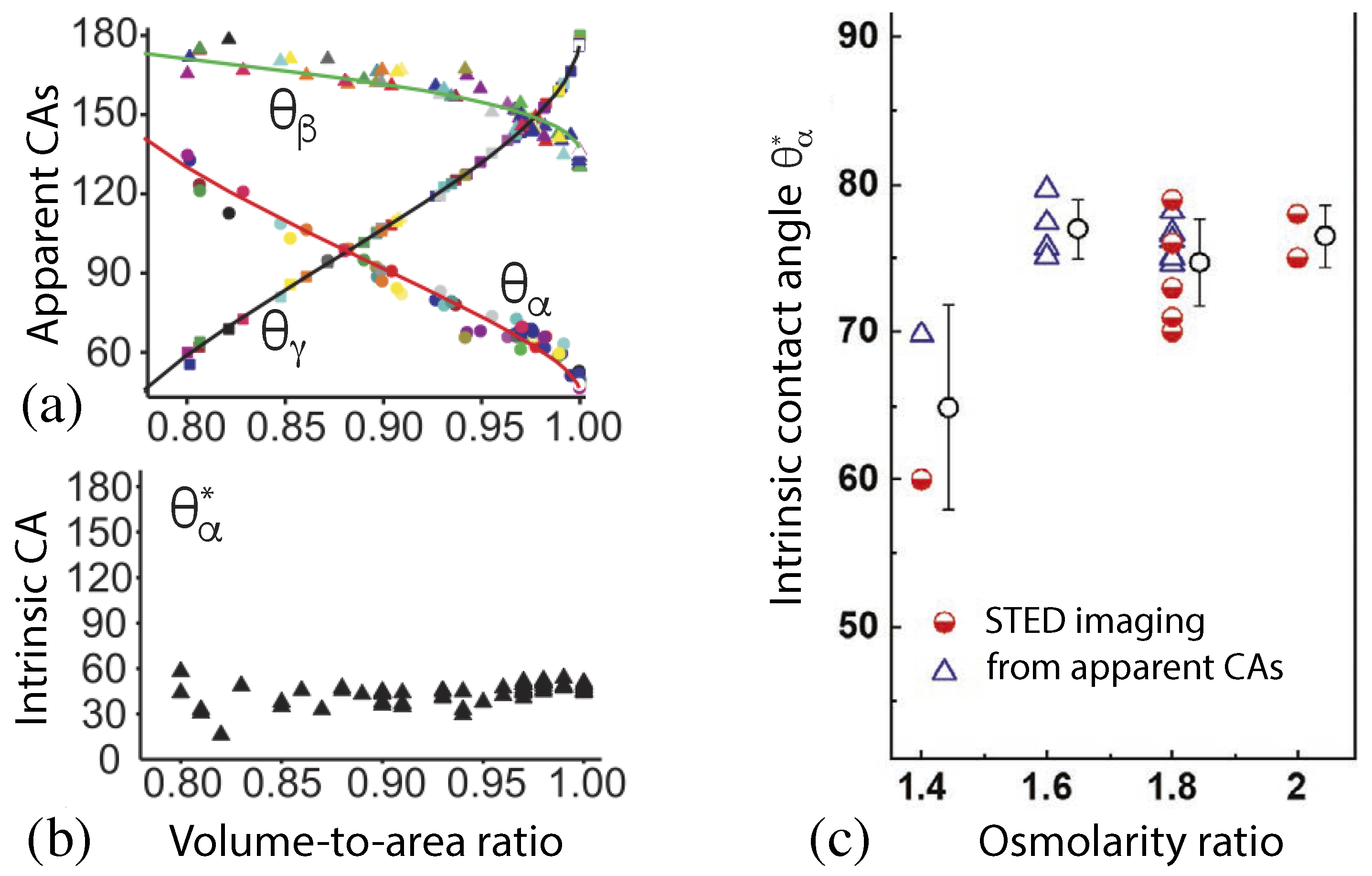

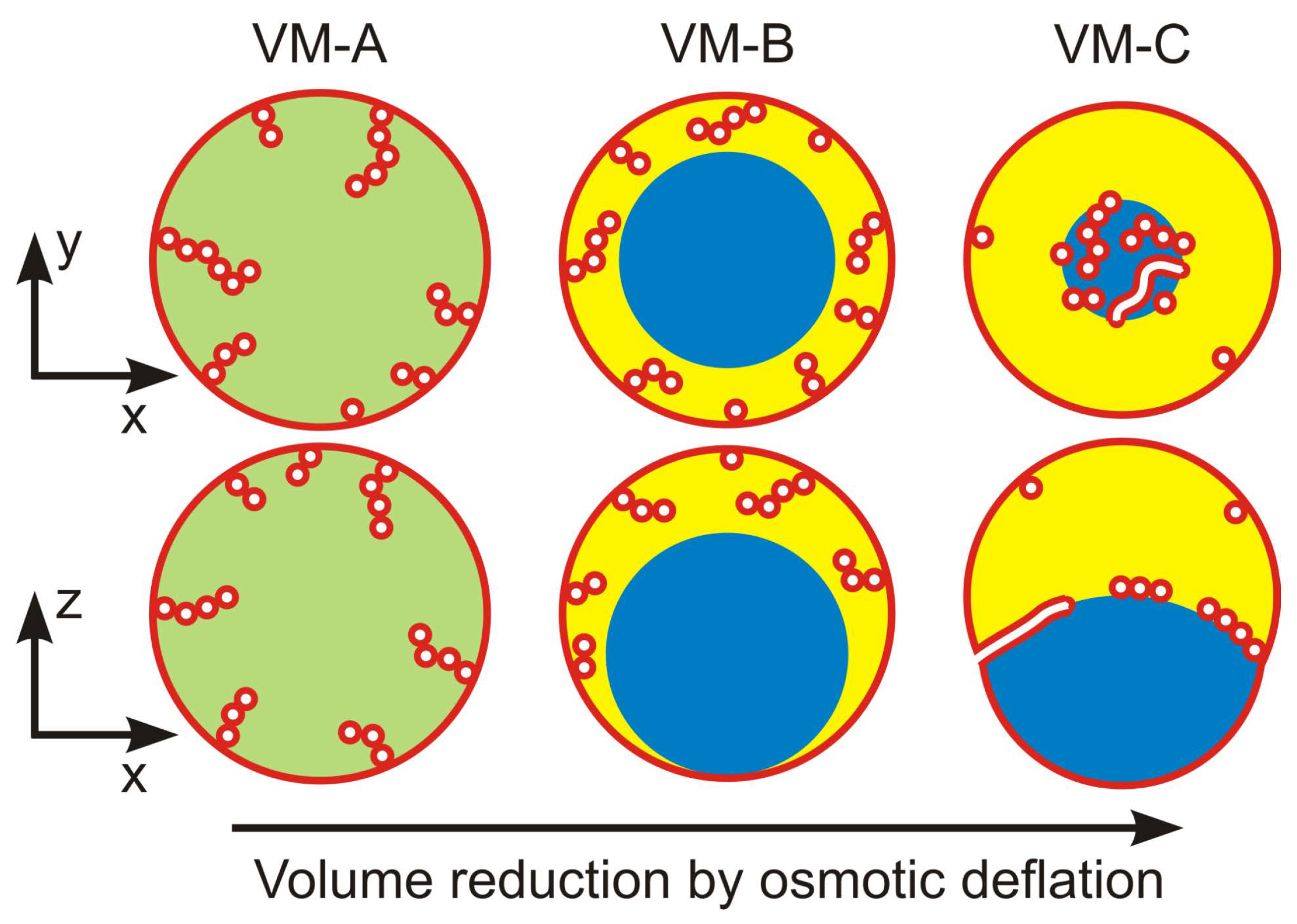
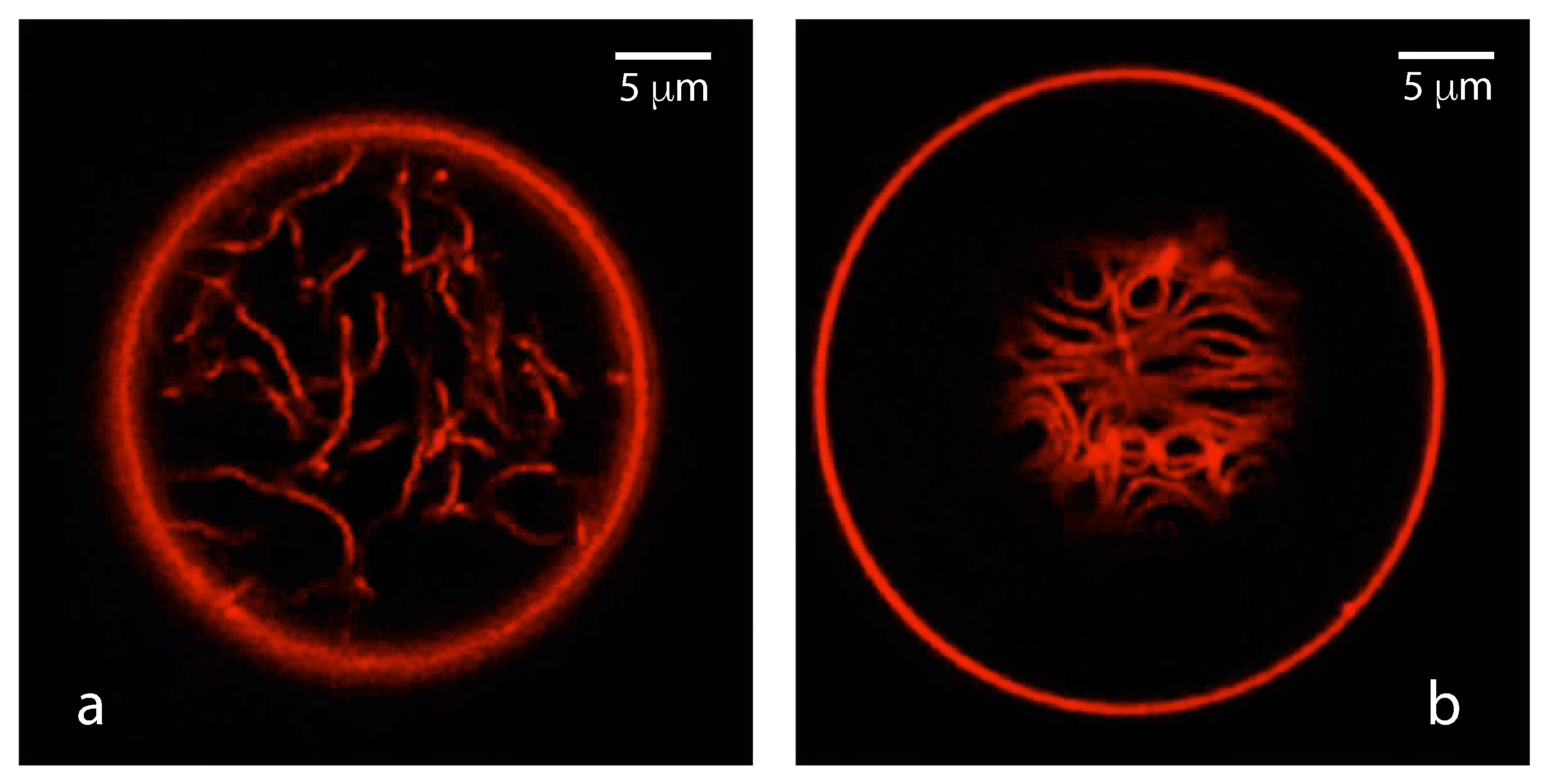
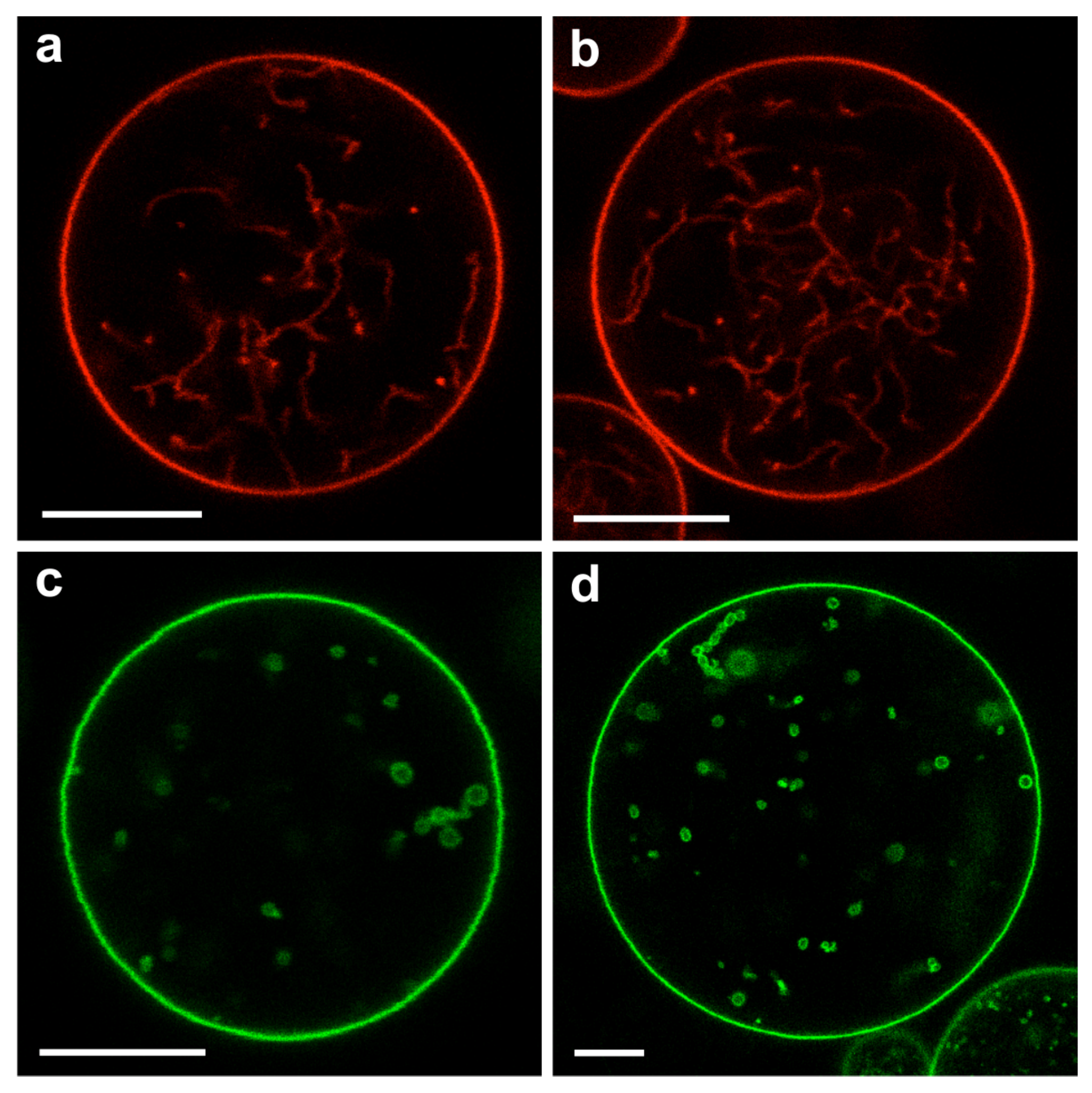
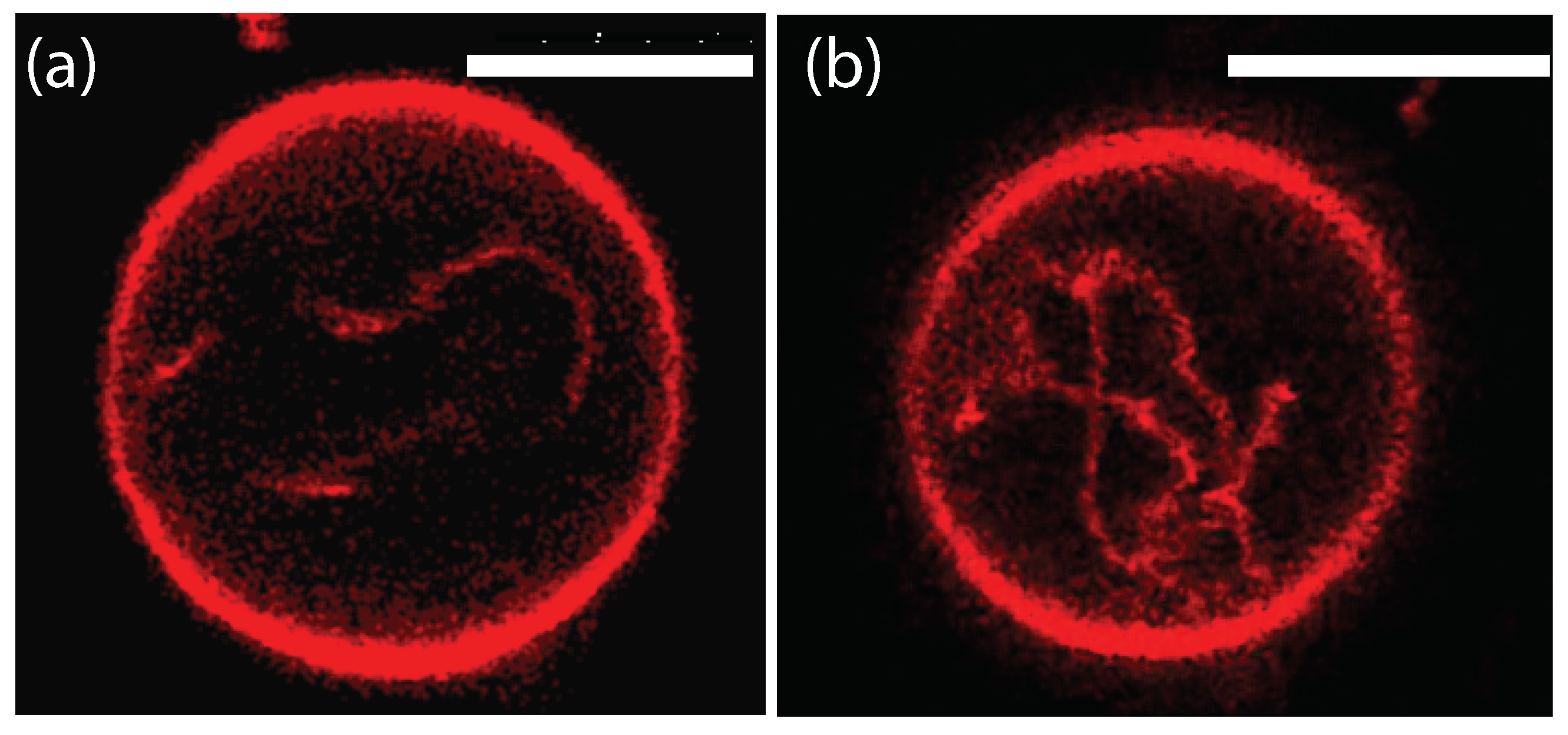


| Phase at Membrane | Phase at Membrane | Contact Angles |
|---|---|---|
| complete wetting by | complete dewetting from | and |
| partial wetting by | partial dewetting from | |
| balanced adhesion | ||
| partial dewetting from | partial wetting by | |
| complete dewetting from | complete wetting by | and |
| Phase at Membrane | Phase at Membrane | Surface Tensions |
|---|---|---|
| complete wetting by | complete dewetting from | |
| partial wetting by | partial dewetting from | |
| balanced adhesion | ||
| partial dewetting from | partial wetting by | |
| complete dewetting from | complete wetting by | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lipowsky, R. Remodeling of Biomembranes and Vesicles by Adhesion of Condensate Droplets. Membranes 2023, 13, 223. https://doi.org/10.3390/membranes13020223
Lipowsky R. Remodeling of Biomembranes and Vesicles by Adhesion of Condensate Droplets. Membranes. 2023; 13(2):223. https://doi.org/10.3390/membranes13020223
Chicago/Turabian StyleLipowsky, Reinhard. 2023. "Remodeling of Biomembranes and Vesicles by Adhesion of Condensate Droplets" Membranes 13, no. 2: 223. https://doi.org/10.3390/membranes13020223
APA StyleLipowsky, R. (2023). Remodeling of Biomembranes and Vesicles by Adhesion of Condensate Droplets. Membranes, 13(2), 223. https://doi.org/10.3390/membranes13020223






