Virtual Surgical Planning: Modeling from the Present to the Future
Abstract
:1. Introduction
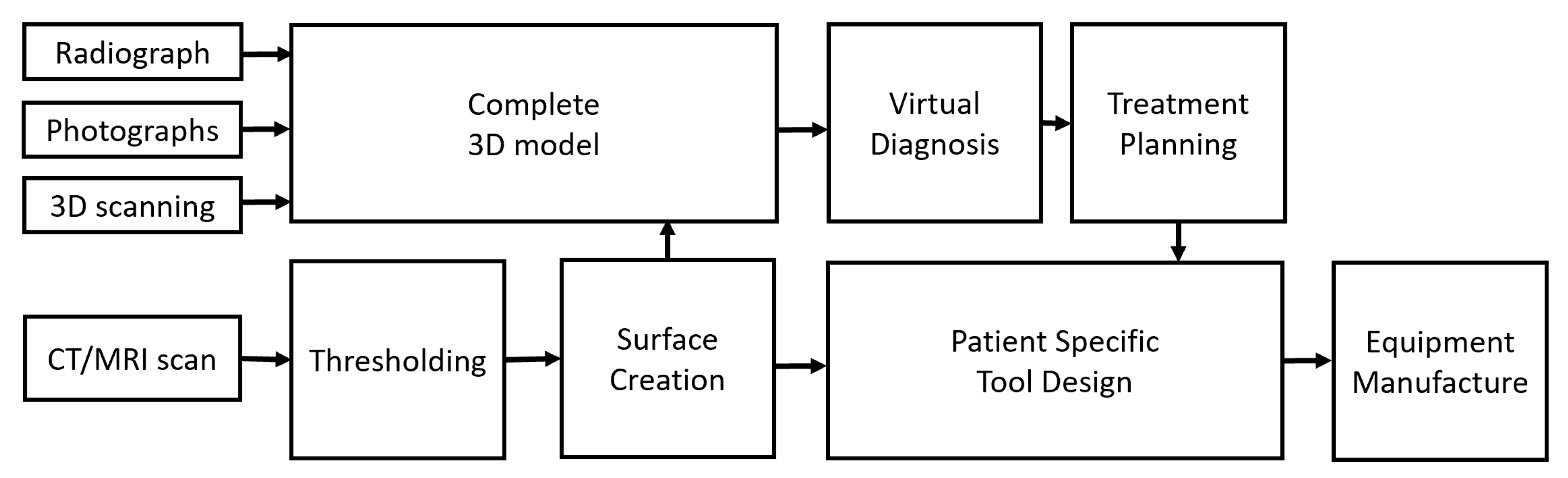
2. Virtual Surgery Planning Procedure
2.1. Data Acquisition
- Radiographs: Traditional 2D radiographs are an old but common method of visualizing the bony tissues to enable their comparison with adjacent soft tissues. Radiographs provide the hard and soft tissue shape and size information in 2D, which can be used in conjunction with 2D photographs to visualize the planned surgical outcome. However, providing only 2D information about shape and size is a significant limitation since VSP requires specific movements, and alignment from planar information is a difficult task that requires skill and experience. Moreover, cephalometric 2D data do not exist in 3D space and are considered by some to be inappropriate for clinical diagnosis and treatment planning [1,2].
- Photographs: 2D photography is the easiest, least invasive and a low-cost source of collecting facial information. For cranio-maxillofacial surgery in the past, the following parameters were suggested when collecting data using a series of photographs:
- The photographs should be in color.
- The photographs should include lateral profiles from both sides, 45° photographs from both sides, and frontal photographs.
- The photographs should be taken in the natural head position.
- 3.
- 3D scanning: 3D scanners can provide high resolution 3D data, including texture and color information, if required. Two types of 3D scanners can be used, i.e., facial scanners and intra-oral scanners. A disadvantage of using 3D scanners is that black-colored surfaces, such as hair, are not easily scanned and are often neglected [4]. Therefore, the 3D information can remain incomplete in certain situations.
- 4.
- CT/CBCT/MRI scanning: Tomographic data from traditional CT scans or cone-beam (CB) CT scans or MRI scans are now the preferred methods for clinical imaging. These techniques provide a series of planar images that can be easily concatenated to create a 3D object from digital data. One limitation of CT and CBCT imaging is the exposure to ionizing radiation that the patient must go through for the clinician to obtain relevant information. In this regard, CBCT scans are preferred over traditional CT scans due to their lower levels of radiation exposure [5,6]. However, although CBCT scanning is preferable, it can be less precise compared to traditional CT scanning on account of image clarity. In contrast, MRI scans do not deploy ionizing radiation. In some instances, particularly those where soft tissues are the primary concern [7], MRI scanning might be preferred; however, in some cases, it is difficult for a patient to hold steady for the duration of the scan. Nevertheless, some recommendations for CT/CBCT/MRI scanning for VSP are as follows:
- Subject position: The patient should be standing or sitting in the natural head position, with the facial expression in repose. Currently, there are no guidelines on the stage of respiration [8], but chin rests and mouthpieces that affect the upper airway are best avoided for the sake of consistency.
- Setup: The field of view should extend at least 10 mm beyond the outermost tissues in order to avoid distortions of any significant structures. The soft tissues especially should not be altered due to equipment fixtures and/or attachments, such as head stabilizers.
- Resolution: If patient-specific tooling is required, a maximum resolution of 0.3 mm in all axes is required; otherwise, a maximum resolution of 0.5 mm can be used.
2.2. Segmentation and Visualization of the Virtual Model
2.3. Alignment and Integration
2.4. Virtual Surgery Planning
- 1.
- Virtual diagnosis: The first and most important step is proper diagnosis. Various analytical tools are often available for the measurement of 3D data. These features can be used to quantify the defect, deficiency or dysmorphology, which might not be possible using traditional surgical planning methods. Note that Euclidean measurements integrate size information, which may mask subtle shape changes associated with clinical behavior.
- 2.
- Treatment planning: Once the differential diagnoses have been excluded and a working diagnosis has been achieved and quantified, optimum plan parameters can be calculated. Craniofacial surgical plan parameters generally include, but are not limited to, an osteotomy location and angle. A virtual osteotomy can thus be simulated, and the final alignment accuracy can be checked. While this approach is summarized in Figure 3, the virtual osteotomy in this example is crucial yet sensitive to the expertise of the technician, making it the weakest point of the VSP as it lacks automation and relies on human decision making. Here, predictive modeling comes into play. According to the spatial matrix hypothesis [12], historically speaking, there is a tacit assertion that the craniofacial complex consists of a series of structural components of genetically predetermined form. Generally, surgically induced changes of these biologically active structures are simply perceived as differential movements. These concepts do not permit the dynamic, biologic behavior of the craniofacial structures to be taken into account, which constantly regress to homeostasis and perhaps lead to relapse in some instances. Conversely, using a cohort of cases that have had the same surgical intervention, it should be possible to compute the mean, underlying 3D transformation for a sample of cases, using techniques derived from mathematical modeling, including geometric morphometrics [13]. If this transformation can then be applied to a naïve subject, a predictive model can be achieved, assuming the new subject behaves in the same way that the sample did on average. This novel data-driven predictive modeling is unlike the animations that are used for arbitrary ‘morphing’ in some orthodontic software. Therefore, the use of mathematical modeling on 3D digital data provides a promising avenue of research in terms of VSP.
- 3.
- Patient specific tool design: Using this approach, patient specific parameters, such as bone thickness, nerve location etc., can be visualized (Figure 4a). If required, patient specific tools and surgery guides can be designed using surgery plan parameters, such as osteotomy location and screw placement.
2.5. Manufacturing
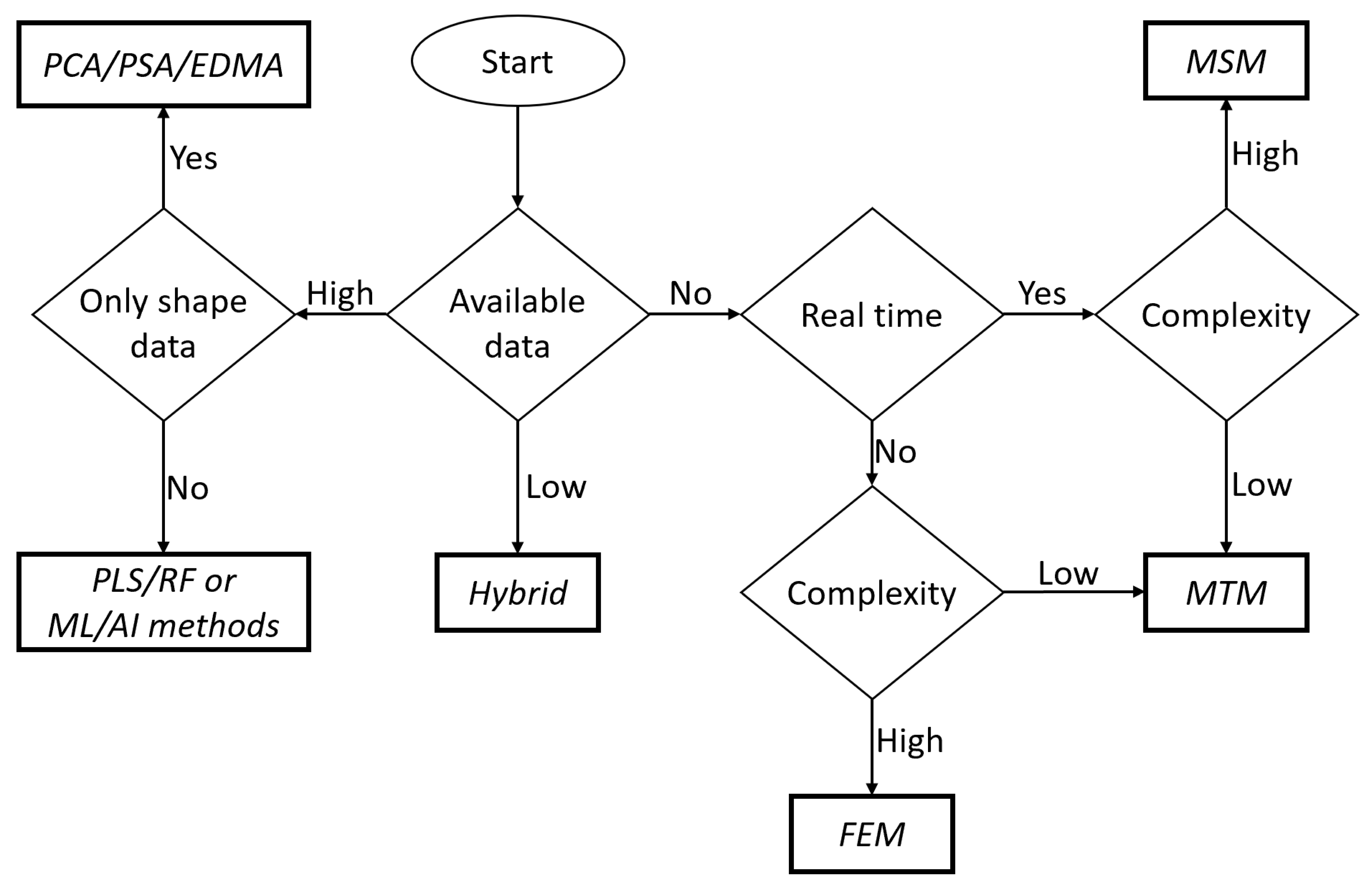
3. Prediction Based Virtual Surgery Planning
- Model-based prediction methods;
- Data-driven predictive modeling;
- Hybrid prediction methods.
3.1. Model-Based Prediction
- A large database is not required to develop an accurate model. Therefore, the methods are directly applicable for procedures in which data are not easily available.
- Properties quantified and/or measured in ideal conditions (such as tissue density, elasticity, stiffness, etc.) can be directly used.
- Both population and individual parameters are taken into account for prediction. The underlying mathematical model considers population-specific parameters, and the discretized anatomy considers the individual parameters.
- Solving non-linear models is time-consuming.
- Developing and using model-based methods is difficult.
- The method cannot be used in conditions where the parameters of the underlying mathematical model are unknown.
- Changes unaccounted for in the mathematical model cannot be predicted.
- Finite element method (FEM): FEM is an old but reliable method. This technique finds extensive use in bioengineering since FEM can be used to solve any set of partial differential equations on the nodes of the discretized model. For observing the change in shape, structural equilibrium equations are used (Equation (1)). Here, σ and τ are the normal and shear stresses in an element under consideration, is the force, and , , are the directions in Cartesian coordinate systems.Tissues can be modeled considering linearity and elasticity models [14]. Therefore, for realizing the relation between stress and strain, Hook’s law is used, and both linear and non-linear behavior can easily be modeled using FEM. Since FEM can simulate details of the non-linear behavior of tissue structures, it has been used for prediction of lip [15,16] and facial soft tissue changes [17].
- Probabilistic FEM [18,19]: Often, the exact material properties are not known. However, the material properties vary within a known range. In such a situation, the use of probabilistic FEM is preferred. In this method, the equations used for simple FEM are solved over a defined interval instead of a defined constant value. This approach is often regarded as a subclass of FEM.
- Mass spring model (MSM): This model consists of discrete mass nodes distributed throughout an object and interconnected via a network of springs and dampers. Therefore, the solutions of MSM are considered linear in nature. MSM is suitable for modeling objects with complex material properties, such as non-linearity and viscoelasticity and has been utilized extensity in the literature [20,21,22,23], but the challenge with MSM is always the correct approximation of spring constants.
- Mass tensor model (MTM) [24,25]: This model tries to combine the advantages of FEA and MSM and provides quasi-linear solutions. For example, it can be used to simulate soft tissue displacement after bone deformation. It uses tetrahedral or triangular elements to approximate the shape, making it fast, but also placing an upper limit on the complexity that MTM can handle [26]. Table 1 compares the advantages and limitations of commonly used model-based prediction methods.
3.2. Data-Based Prediction
- Applicable in most cases and do not require a specific mathematical model for defined conditions.
- Easier to learn, develop and implement as compared to model-based methods.
- Can provide complex results relatively rapidly.
- When using machine-learning algorithms, the accuracy improves with every prediction.
- They require a large database, which might not be readily available.
- The precision of even highly accurate models is limited by their training database. Thus, a patient-type or procedure-type that is not extensively referenced in the training database cannot be assured to produce an accurate prediction.
- Principal components analysis (PCA): PCA finds its origins as a model reduction technique. PCA provides new components that are a linear combination of the original components of data. The new components are formed to capture most of the variation in the data, using the least number of components. PCA is, therefore, a useful technique for data analysis and outlier detection. Using this approach, the underlying behavior of the data can be identified and described in statistical shape space as a series of eigenvalues. Thereafter, eigenvectors can be applied to a new dataset to visualize possible outcomes. Thus, PCA is particularly useful as a data pre-processing technique in predictive modeling and is the most commonly used data-based method [27].
- Euclidian distance matrix analysis (EDMA): Since some are of the opinion that principal components analysis (PCA) methods, based on superimposition, can lead to an incorrect estimation of form and variance–covariance structures, EDMA has been advocated as an alternative [28]. Euclidean distance is one of the most used metrics, and EDMA is a space ratio method, providing a coordinate-free approach to the analysis of form using landmark data, which may provide another approach to data-driven predictive modeling.
- Principal Shape Analysis (PSA): A common limitation in PCA is the difficulty in relating the components modes with the intuitive shape descriptions used by clinical practitioners. Aguirre et al. [29] proposed the use of PSA to counter this problem. PSA models covariance between variables rather than the total covariance in the data. Hence, PSA provides better interpretation by proving the components that can account for the variance in the entire dataset.
3.3. Hybrid Prediction
- Model + Model: Two or more models can be used to make predictions. For example, combination of a biochemical model for wound healing with a biomechanical tissue deformation model was used for surgical prediction by Vavourakis et al. [33].
- Data + Data: Two data-driven models can also be used for improving the overall accuracy of the prediction. For example, different regions of the same anatomy can be analyzed separately to create separate prediction models. The results from all the models can be combined to have an overall prediction. Bayesian-based, patient-specific growth models for anatomical changes associated with fast changing anatomies have shown promising results.
- Model + Data: This is probably the most powerful hybrid model with many possible applications. Model-based methods and data-based methods can be combined to perform the following tasks:
- 1.
- 2.
- 3.
- Data-driven techniques can be used to create population-specific parameters required by model-based methods [37].
- 4.
- A data-driven method can be used to predict the boundary conditions required for model-based method. The model-based method can then make accurate predictions [38].
- 5.
- Both data-based methods and model-based methods can make individual predictions, which can be fused to provide a highly accurate, final prediction.
4. Selection of Prediction Method
5. Prediction Analysis
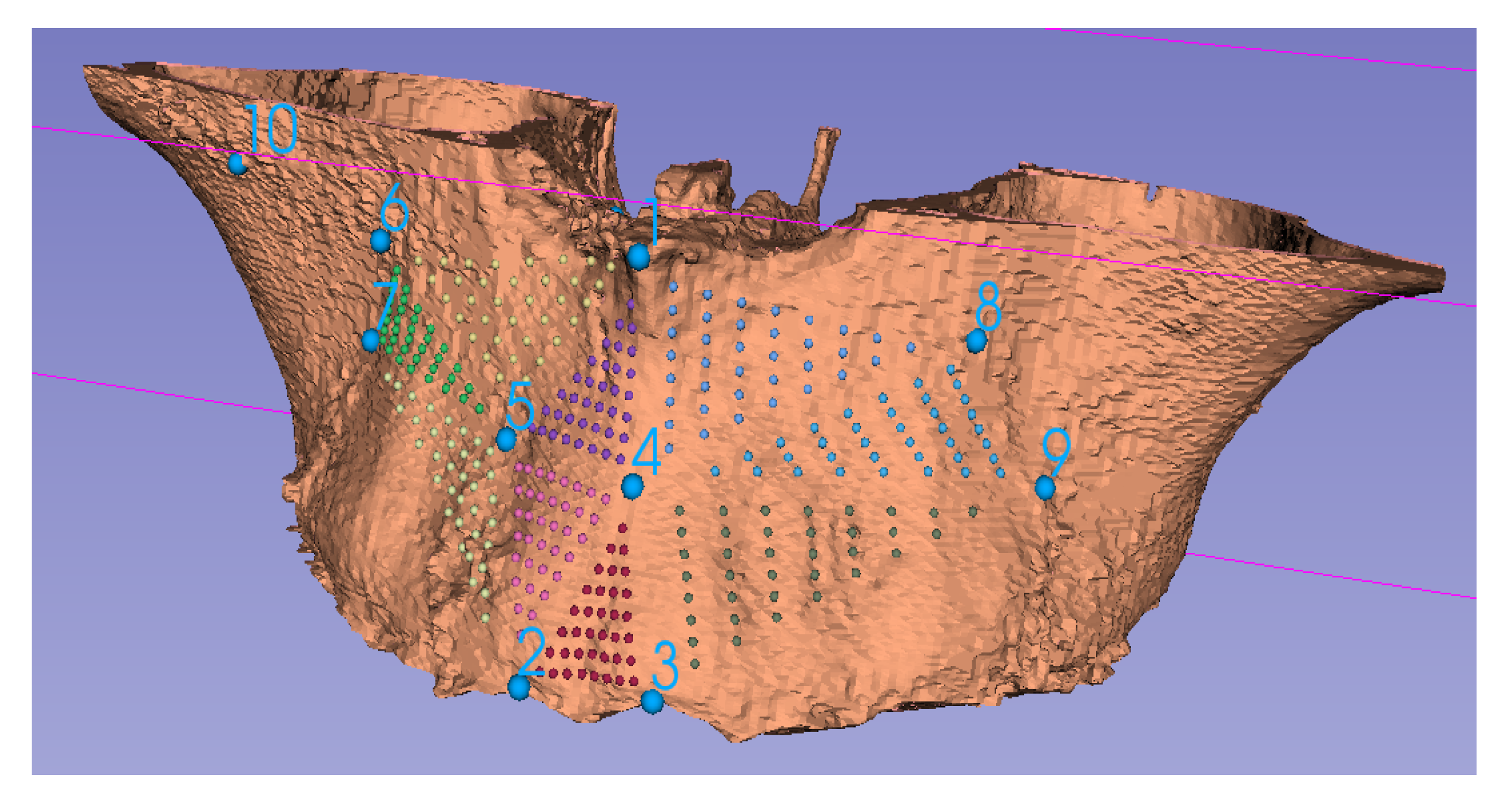
5.1. Landmark Selection
- Anatomical landmarks: These are called homologous landmarks, which are agreed by experts to represent various anatomical features.
- Mathematical landmarks: These are the points on the surface located according to a specific geometric property, such as maxima or minima of high curvature.
- Pseudo-landmarks: These are also called semi-landmarks and are points that are constructed using other types of landmarks and mathematical logic.
5.2. Automated Landmark Detection
- a.
- Knowledge based [42]: These methods use mathematical definitions such as curvature, maxima, minima, etc. to locate the landmark on an anatomical contour of the 3D object or surface.
- b.
- Atlas based [43]: In these methods, before automatic placement begins, a reference atlas is created using a manual landmarking process. The reference atlas is assumed to be accurate and inclusive. For automatic placement, the subject under consideration is matched with the reference elements in the atlas. The closest matching reference is used to transfer the landmarks from the selected reference to the subject.
- c.
- Learning based: These methods rely on learning algorithms and available datasets to automatically locate landmarks. The learning methods employed can either be statistical or based entirely on machine learning.
5.3. Alignment
- For analysis of morphology of the same patient, usually the non-morphing configurations are used as the reference. Homologous landmarks are placed on locations that do not change due to the treatment. The pre-treatment and post-treatment objects are then translated and rotated iteratively for optimal alignment. Note that scaling is not performed in this technique unless natural growth needs to be accommodated.
- When analyzing and comparing configurations from different patients or if an unaffected reference is are not available, generalized Procrustes analysis (GPA) is used to align homologous landmarks as close as possible. The Procrustes mean is then calculated. The configurations are then aligned to the mean using thin plate spline (TPS), explicitly minimizing the bending energy [39].
5.4. Region of Interest (ROI)
- Total anatomy: The entire geometry for which point correspondence is available can be considered. The advantage of such ROIs is that it is easier to implement. The disadvantage is that the change due to treatment is localized, therefore, tracking the actual change becomes difficult as the numeric values are dominated by unchanged parts of the anatomy. When the entire anatomy is analyzed, it is also important to adjust for outliers. Two methods have been employed in the literature for handling outliers. First, the absolute distance between the points from the two objects is ordered in decreasing magnitude. Instead of using all the points, only a certain lower percentile is used, traditionally the 90th percentile. An alternate method is to use a fixed tolerance where points that lie outside the tolerance are neglected from analysis; traditionally, a 2 mm tolerance is accepted.
- Local area: In this case, instead of using the entire geometry, only a local area is selected. The advantage is that changes are easier to track. The disadvantage is that an additional step (manual, automatic or semi-automatic) is often required to segment the local ROI. For analysis of a local ROI, semi-landmarks can preferably be used.
- Local curve: Sometimes instead of using a local ROI, a local curve is considered. This can be useful in certain applications such as prediction of the facial midline, shape of the spine, etc. For generation of curves, mathematical landmarks are used.
5.5. Correspondence
5.6. Difference Quantification
6. Discussion
Author Contributions
Funding
Conflicts of Interest
References
- Moyers, R.E.; Bookstein, F.L. The inappropriateness of conventional cephalometrics. Am. J. Orthod. 1979, 75, 599–617. [Google Scholar] [CrossRef]
- Bookstein, F.L. Reconsidering “The inappropriateness of conventional cephalometrics”. Am. J. Orthod. Dentofac. Orthop. 2016, 149, 784–797. [Google Scholar] [CrossRef] [Green Version]
- Singh, G.D. 3-D Photography Combined with Conventional and 3-D Analysis. J. Oral Maxillofac. Surg. 2005, 63, 7. [Google Scholar] [CrossRef]
- Singh, G.D.; Levy-Bercowski, D.; Yáñez, M.A.; Santiago, P.E. Three-dimensional facial morphology following surgical repair of unilateral cleft lip and palate in patients after nasoalveolar molding. Orthod. Craniofacial Res. 2007, 10, 161–166. [Google Scholar] [CrossRef]
- Ludlow, J.B.; E Davies-Ludlow, L.; Brooks, S.L. Dosimetry of two extraoral direct digital imaging devices: NewTom cone beam CT and Orthophos Plus DS panoramic unit. Dentomaxillofac. Radiol. 2003, 32, 229–234. [Google Scholar] [CrossRef]
- Ludlow, J.B. A manufacturer’s role in reducing the dose of cone beam computed tomography examinations: Effect of beam filtration. Dentomaxillofac. Radiol. 2011, 40, 115–122. [Google Scholar] [CrossRef] [Green Version]
- Kamburoğlu, K. Use of dentomaxillofacial cone beam computed tomography in dentistry. World J. Radiol. 2015, 7, 128–130. [Google Scholar] [CrossRef] [PubMed]
- Singh, G.D. Mechanisms of upper airway remodeling following surgical interventions remain elusive. Sleep 2021, 44, zsab201. [Google Scholar] [CrossRef] [PubMed]
- Celenk, M.; Farrel, M.; Eren, H.; Kumar, K.; Singh, G.; Lazanoff, S. Upper airway detection in cone beam images. In Proceedings of the 3rd International Conference on Bioinformatics and Biomedical Engineering, Beijing, China, 11–13 June 2009. [Google Scholar]
- Singh, G.D.; McNamara, J.A.J.; Lozanoff, S. Morphometry of the cranial base in subjects with Class III malocclusion. J. Dent. Res. 1997, 76, 694–703. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gilani, S.Z.; Mian, A.; Shafait, F.; Reid, I. Dense 3D face correspondence. IEEE Trans. Pattern Anal. Mach. Intell. 2017, 40, 1584–1598. [Google Scholar] [CrossRef] [Green Version]
- Singh, G.D. Spatial matrix hypothesis. Br. Dent. J. 2007, 202, 238–239. [Google Scholar] [CrossRef] [Green Version]
- Singh, G.D. Digital diagnostics: Three-dimensional modelling. Br. J. Oral Maxillofac. Surg. 2008, 46, 22–26. [Google Scholar] [CrossRef]
- Freutel, M.; Schmidt, H.; Dürselen, L.; Ignatius, A.; Galbusera, F. Finite element modeling of soft tissues: Material models, tissue interaction and challenges. Clin. Biomech. 2014, 29, 363–372. [Google Scholar] [CrossRef] [PubMed]
- Kim, D.; Kuang, T.; Rodrigues, Y.L.; Gateno, J.; Shen, S.G.F.; Wang, X.; Deng, H.; Yuan, P.; Alfi, D.M.; Liebschner, M.A.K.; et al. A New Approach of Predicting Facial Changes Following Orthognathic Surgery Using Realistic Lip Sliding Effect. In Medical Image Computing and Computer Assisted Intervention—MICCAI 2019; Lecture Notes in Computer Science; Springer: Cham, Switzerland, 2019; pp. 336–344. [Google Scholar]
- Kim, D.; Kuang, T.; Rodrigues, Y.L.; Gateno, J.; Shen, S.G.; Wang, X.; Stein, K.; Deng, H.H.; Liebschner, M.A.; Xia, J.J. A novel incremental simulation of facial changes following orthognathic surgery using FEM with realistic lip sliding effect. Med. Image Anal. 2021, 72, 102095. [Google Scholar] [CrossRef] [PubMed]
- Kim, D.; Ho, D.C.; Mai, H.; Zhang, X.; Shen, S.G.; Shen, S.; Yuan, P.; Liu, S.; Zhang, G.; Zhou, X.; et al. A clinically validated prediction method for facial soft-tissue changes following double-jaw surgery. Med. Phys. 2017, 44, 4252–4261. [Google Scholar] [CrossRef] [PubMed]
- Knoops, P.G.M.; Borghi, A.; Ruggiero, F.; Badiali, G.; Bianchi, A.; Marchetti, C.; Rodriguez-Florez, N.; Breakey, R.W.F.; Jeelani, O.; Dunaway, D.J.; et al. A novel soft tissue prediction methodology for orthognathic surgery based on probabilistic finite element modelling. PLoS ONE 2018, 13, e0197209. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, W.; Zhou, C.; Guo, R.; Cha, T.; Li, G. Prediction of biomechanical responses of human lumbar discs—A stochastic finite element model analysis. Methods Biomech. Biomed. Eng. 2021, 24, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Mollemans, W.; Schutyser, F.; Cleynenbreugel, J.V.; Suetens, P. Tetrahedral Mass Spring Model for Fast Soft Tissue Deformation. In International Symposium on Surgery Simulation and Soft Tissue Modeling IS4TM 2003; Lecture Notes in Computer Science; Springer: Berlin/Heidelberg, Germany, 2003; Volume 2673. [Google Scholar] [CrossRef]
- Mollemans, W.; Schutyser, F.; Van Cleynenbreugel, J.; Suetens, P. Fast Soft Tissue Deformation with Tetrahedral Mass Spring Model for Maxillofacial Surgery Planning Systems. In Medical Image Computing and Computer-Assisted Intervention—MICCAI 2004; Lecture Notes in Computer Science; Springer: Berlin/Heidelberg, Germany, 2004; pp. 371–379. [Google Scholar]
- Patete, P.; Iacono, M.I.; Spadea, M.F.; Trecate, G.; Vergnaghi, D.; Mainardi, L.T.; Baroni, G. A multi-tissue mass-spring model for computer assisted breast surgery. Med. Eng. Phys. 2013, 35, 47–53. [Google Scholar] [CrossRef]
- Hammer, P.E.; del Nido, P.J.; Howe, R.D. Anisotropic Mass-Spring Method Accurately Simulates Mitral Valve Closure from Image-Based Models. In Functional Imaging and Modeling of the Heart; Lecture Notes in Computer Science; Springer: Berlin/Heidelberg, Germany, 2011; pp. 233–240. [Google Scholar]
- Xu, S.; Liu, X.P.; Zhang, H.; Hu, L. A Nonlinear Viscoelastic Tensor-Mass Visual Model for Surgery Simulation. IEEE Trans. Instrum. Meas. 2010, 60, 14–20. [Google Scholar] [CrossRef]
- Guo, S.; Cai, X.; Gao, B.; Yang, Q.; Zhao, Y.; Xiao, N. Tensor-mass Model based real-time simulation of vessel deformation and force feedback for the interventional surgery training system. In Proceedings of the IEEE International Conference on Mechatronics and Automation (ICMA), Takamatsu, Japan, 6–9 August 2017. [Google Scholar]
- Chen, Z.; Guo, S.; Zhou, W.; Meng, F. Performance Evaluation of the Vascular Model Based on the Nonlinear Viscoelastic Tensor-Mass Method. In Proceedings of the IEEE International Conference on Mechatronics and Automation, Takamatsu, Japan, 8–11 August 2021. [Google Scholar]
- Cerrolaza, J.J.; Summers, R.M.; Linguraru, M.G. Soft Multi-organ Shape Models via Generalized PCA: A General Framework. In Medical Image Computing and Computer-Assisted Intervention—MICCAI 2016; Lecture Notes in Computer Science; Ourselin, S., Joskowicz, L., Sabuncu, M., Unal, G., Wells, W., Eds.; Springer: Cham, Switzerland, 2016; Volume 9902, pp. 219–228. [Google Scholar]
- Lele, S. Euclidean distance matrix analysis (EDMA): Estimation of mean form and mean form difference. Math. Geol. 1993, 25, 573–602. [Google Scholar] [CrossRef]
- Aguirre, M.R.; Linguraru, M.G.; Marias, K.; Ayache, N.; Nolte, L.-P.; Ballester, M.Á.G. Statistical shape analysis via principal factor analysis. In Proceedings of the 4th IEEE international symposium on biomedical imaging: From nano to macro, Arlington, VA, USA, 12–15 April 2007. [Google Scholar]
- Knoops, P.G.M.; Papaioannou, A.; Borghi, A.; Breakey, R.W.F.; Wilson, A.T.; Jeelani, O.; Zafeiriou, S.; Steinbacher, D.; Padwa, B.L.; Dunaway, D.J.; et al. A machine learning framework for automated diagnosis and computer-assisted planning in plastic and reconstructive surgery. Sci. Rep. 2019, 9, 13597. [Google Scholar] [CrossRef]
- Zolfagharnasab, H.; Bessa, S.; Oliveira, S.P.; Faria, P.; Teixeira, J.F.; Cardoso, J.S.; Oliveira, H.P. A regression model for predicting shape deformation after breast conserving surgery. Sensors 2018, 18, 167. [Google Scholar] [CrossRef] [Green Version]
- Liao, L.; Kottig, F. Review of hybrid prognostics approaches for remaining useful life prediction of engineered systems, and an application to battery life prediction. IEEE Trans. Reliab. 2014, 63, 191–207. [Google Scholar] [CrossRef]
- Vavourakis, V.; Eiben, B.; Hipwell, J.H.; Williams, N.R.; Keshtgar, M.; Hawkes, D.J. Multiscale mechano-biological finite element modelling of oncoplastic breast surgery—Numerical study towards surgical planning and cosmetic outcome prediction. PLoS ONE 2016, 11, e0159766. [Google Scholar]
- Grassi, L.; Schileo, E.; Boichon, C.; Viceconti, M.; Taddei, F. Comprehensive evaluation of PCA-based finite element modelling of the human femur. Med. Eng. Phys. 2014, 36, 1246–1252. [Google Scholar] [CrossRef] [PubMed]
- Taghizadeh, E.; Kistler, M.; Büchler, P.; Reyes, M. Fast Prediction of Femoral Biomechanics Using Supervised Machine Learning and Statistical Shape Modeling. In Computational Biomechanics for Medicine; Springer: Cham, Switzerland, 2016; pp. 107–116. [Google Scholar]
- Jiang, Z.; Do, H.N.; Choi, J.; Lee, W.; Baek, S. A deep learning approach to predict abdominal aortic aneurysm expansion using longitudinal data. Front. Phys. 2020, 7, 235. [Google Scholar] [CrossRef] [Green Version]
- Scarton, A.; Sawacha, Z.; Cobelli, C.; Li, X. Towards the generation of a parametric foot model using principal component analysis: A pilot study. Med. Eng. Phys. 2016, 38, 547–559. [Google Scholar] [CrossRef] [PubMed]
- Ou, H.; Su, J.; Lan, S.; Wang, L.; Xu, X.; Johnson, S. Development of a simplified, reproducible, parametric 3D model of the talus. Med. Eng. Phys. 2019, 71, 3–9. [Google Scholar] [CrossRef] [PubMed]
- Bookstein, F.L. Landmark methods for forms without landmarks: Morphometrics of group differences in outline shape. Med. Image Anal. 1997, 1, 225–243. [Google Scholar] [CrossRef]
- Dot, G.; Rafflenbeul, F.; Arbotto, M.; Gajny, L.; Rouch, P.; Schouman, T. Accuracy and reliability of automatic three-dimensional cephalometric landmarking. Int. J. Oral Maxillofac. Surg. 2020, 49, 1367–1378. [Google Scholar] [CrossRef]
- Hassan, B.; Nijkamp, P.; Verheij, H.; Tairie, J.; Vink, C.; van der Stelt, P.; Van Beek, H. Precision of identifying cephalometric landmarks with cone beam computed tomography in vivo. Eur. J. Orthod. 2013, 35, 38–44. [Google Scholar] [CrossRef]
- Neelapu, B.C.; Kharbanda, O.P.; Sardana, V.; Gupta, A.; Vasamsetti, S.; Balachandran, R.; Sardana, H.K. Automatic localization of three-dimensional cephalometric landmarks on CBCT images by extracting symmetry features of the skull. Dentomaxillofac. Radiol. 2018, 47, 20170054. [Google Scholar] [CrossRef]
- Li, M.; Cole, J.; Manyama, M.; Larson, J.R.; Liberton, D.; Riccardi, S.L.; Ferrara, T.M.; Santorico, S.A.; Bannister, J.J.; Forkert, N.D.; et al. Rapid automated landmarking for morphometric analysis of three-dimensional facial scans. J. Anat. 2017, 230, 607–618. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, X.; Chen, C.; Boone, S.; Joshi, V.; Welbeck, A.; Liang, G.; Chang, G.; Saha, P.K. MRI-based active shape model of the human proximal femur using fiducial and secondary landmarks and its validation. In Proceedings of the Medical Imaging 2018: Biomedical Applications in Molecular, Structural, and Functional Imaging, Houston, TX, USA, 12 March 2018. [Google Scholar]
- Montúfar, J.; Romero, M.; Scougall-Vilchis, R.J. Hybrid approach for automatic cephalometric landmark annotation on cone-beam computed tomography volumes. Am. J. Orthod. Dentofac. Orthop. 2018, 154, 140–150. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lindner, C.; Cootes, T.F. Fully automatic cephalometric evaluation using random forest regression-voting. In Proceedings of the IEEE International Symposium on Biomedical Imaging (ISBI) 2015–Grand Challenges in Dental X-ray Image Analysis–Automated Detection and Analysis for Diagnosis in Cephalometric X-ray Image, New York, NY, USA, 16–19 April 2015. [Google Scholar]
- Yeh, Y.-C.; Weng, C.-H.; Huang, Y.-J.; Fu, C.-J.; Tsai, T.-T.; Yeh, C.-Y. Deep learning approach for automatic landmark detection and alignment analysis in whole-spine lateral radiographs. Sci. Rep. 2021, 11, 7618. [Google Scholar] [CrossRef] [PubMed]
- Marlière, D.-A.-A.; Demétrio, M.-S.; Schmitt, A.-R.-M.; Lovisi, C.-B.; Asprino, L.; Chaves-Netto, H.-D.-d.M. Accuracy between virtual surgical planning and actual outcomes in orthognathic surgery by iterative closest point algorithm and color maps: A retrospective cohort study. Med. Oral Patología Oral y Cirugía Bucal Ed. Inglesa 2019, 24, 1. [Google Scholar] [CrossRef]
- Gracia-Abuter, B.; Noguera-Pantoja, A.; Führer-Valdivia, A.; Solé-Ventura, P.; Haidar, Z.S. 3-H in 3-D: Envisaging Beyond the Current Hype, the Hope and Hurdles of Three-Dimensional “Virtual Planning” in Orthognathic Surgery. Int. J. Morphol. 2018, 36, 14–21. [Google Scholar] [CrossRef]
- Borba, A.M.; Haupt, D.; Romualdo, L.T.D.A.; da Silva, A.L.F.; Homem, M.D.G.N.; Miloro, M. How many oral and maxillofacial surgeons does it take to perform virtual orthognathic surgical planning? J. Oral Maxillofac. Surg. 2016, 74, 1807–1826. [Google Scholar] [CrossRef] [Green Version]
- Quereshy, F.A.; Levintov, N.; Nguyen, J.L.; DeLeonibus, M.A.; Demko, C.; Bazina, M.; Valiathan, M.; Baur, D.A. The Role of Virtual Surgical Planning in Increasing the Predictability in Orthognathic Surgery. J. Dent. Maxillofac. Surg. 2019, 73, 136–141. [Google Scholar] [CrossRef]
- Kwon, T.-G.; Miloro, M.; Han, M.D. How Accurate Is 3-Dimensional Computer-Assisted Planning for Segmental Maxillary Surgery? J. Oral Maxillofac. Surg. 2020, 78, 1597–1608. [Google Scholar] [CrossRef]
- Qadry, Y.; Hassan, S.A.; Mobarak, F.A.; Abdelrahman, M.A. Surgery-first Approach Using Virtual Setup for the Treatment of Dentoskeletal Deformities. Al-Azhar Dent. J. Girls 2021, 8, 99–104. [Google Scholar] [CrossRef]
- Tecuta-Busoi, A.-I.; Matei, M.; Florescu, L.M.; Gheonea, I.A. Developmental Abnormalities of the Skull Base in Patients with Turner Syndrome. Curr. Health Sci. J. 2020, 46, 329. [Google Scholar] [PubMed]
- Park, S.-Y.; Hwang, D.-S.; Song, J.-M.; Kim, U.-K. Comparison of time and cost between conventional surgical planning and virtual surgical planning in orthognathic surgery in Korea. Maxillofac. Plast. Reconstr. Surg. 2021, 43, 1–7. [Google Scholar] [CrossRef] [PubMed]
- Paunović, N.; Bao, Y.; Coulter, F.B.; Masania, K.; Geks, A.K.; Klein, K.; Rafsanjani, A.; Cadalbert, J.; Kronen, P.W.; Kleger, N.; et al. Digital light 3D printing of customized bioresorbable airway stents with elastomeric properties. Sci. Adv. 2021, 7, eabe9499. [Google Scholar] [CrossRef]





| Technique | Advantages | Limitations |
|---|---|---|
| Model based techniques |
|
|
| Mass spring model |
|
|
| Mass tensor model |
|
|
| Finite element model |
|
|
| Method | Advantages | Limitations |
|---|---|---|
| Data based methods |
|
|
| Statistical methods |
|
|
| ML/AI methods |
|
|
| Method | Advantages | Limitations |
|---|---|---|
| Knowledge-based |
|
|
| Atlas-based |
|
|
| Learning-based |
|
|
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Singh, G.D.; Singh, M. Virtual Surgical Planning: Modeling from the Present to the Future. J. Clin. Med. 2021, 10, 5655. https://doi.org/10.3390/jcm10235655
Singh GD, Singh M. Virtual Surgical Planning: Modeling from the Present to the Future. Journal of Clinical Medicine. 2021; 10(23):5655. https://doi.org/10.3390/jcm10235655
Chicago/Turabian StyleSingh, G. Dave, and Manarshhjot Singh. 2021. "Virtual Surgical Planning: Modeling from the Present to the Future" Journal of Clinical Medicine 10, no. 23: 5655. https://doi.org/10.3390/jcm10235655
APA StyleSingh, G. D., & Singh, M. (2021). Virtual Surgical Planning: Modeling from the Present to the Future. Journal of Clinical Medicine, 10(23), 5655. https://doi.org/10.3390/jcm10235655






