Design and Experiment of Spiral Discharge Anti-Blocking and Row-Sorting Device of Wheat No-Till Planter
Abstract
:1. Introduction
2. Materials and Methods
2.1. Machine Structure and Working Principle of the SDARD
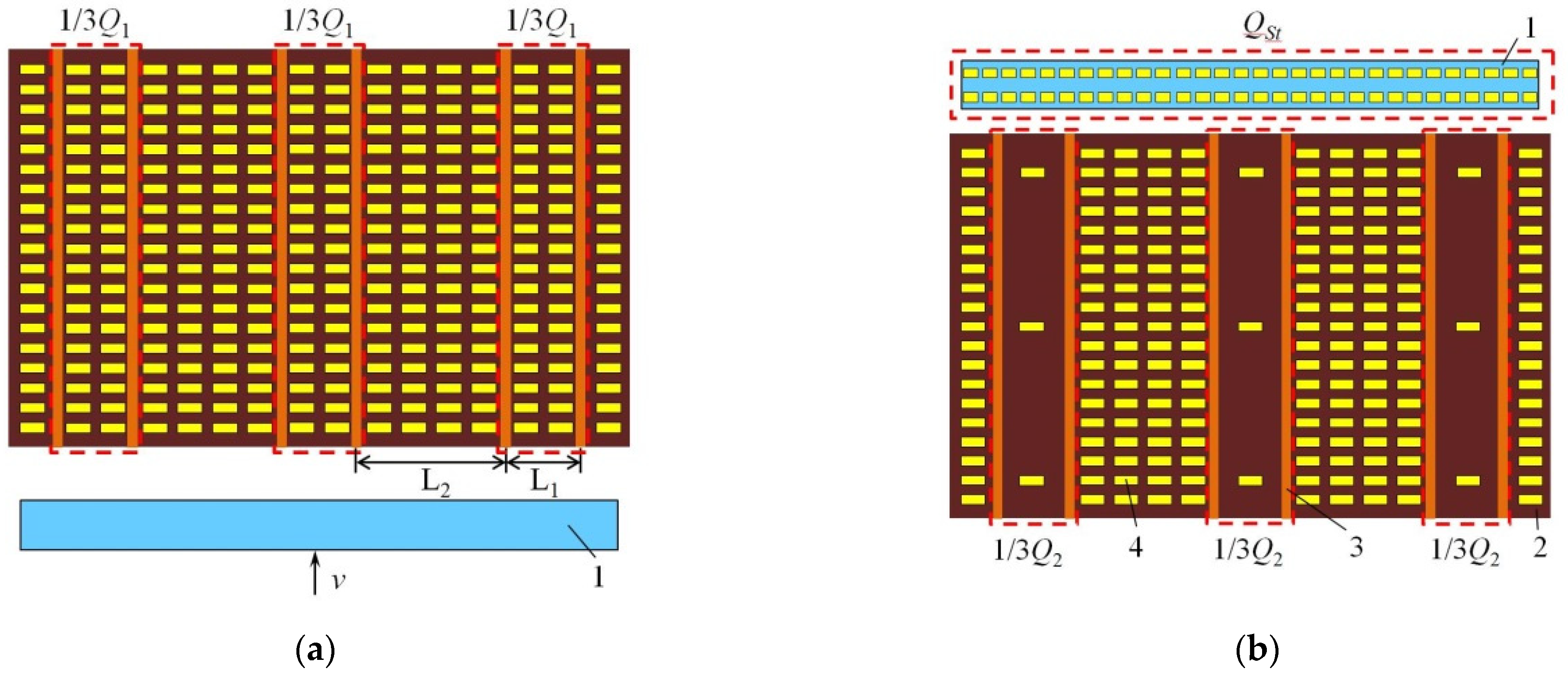
2.1.1. Structure of the SDARD
2.1.2. Working Principle
2.2. Parameter Design of the SDM
2.2.1. The SFA Qs
2.2.2. Outer Diameter of the Spiral Blade D
2.2.3. Pitch S
2.2.4. The HHHG h
2.2.5. The RVSS n
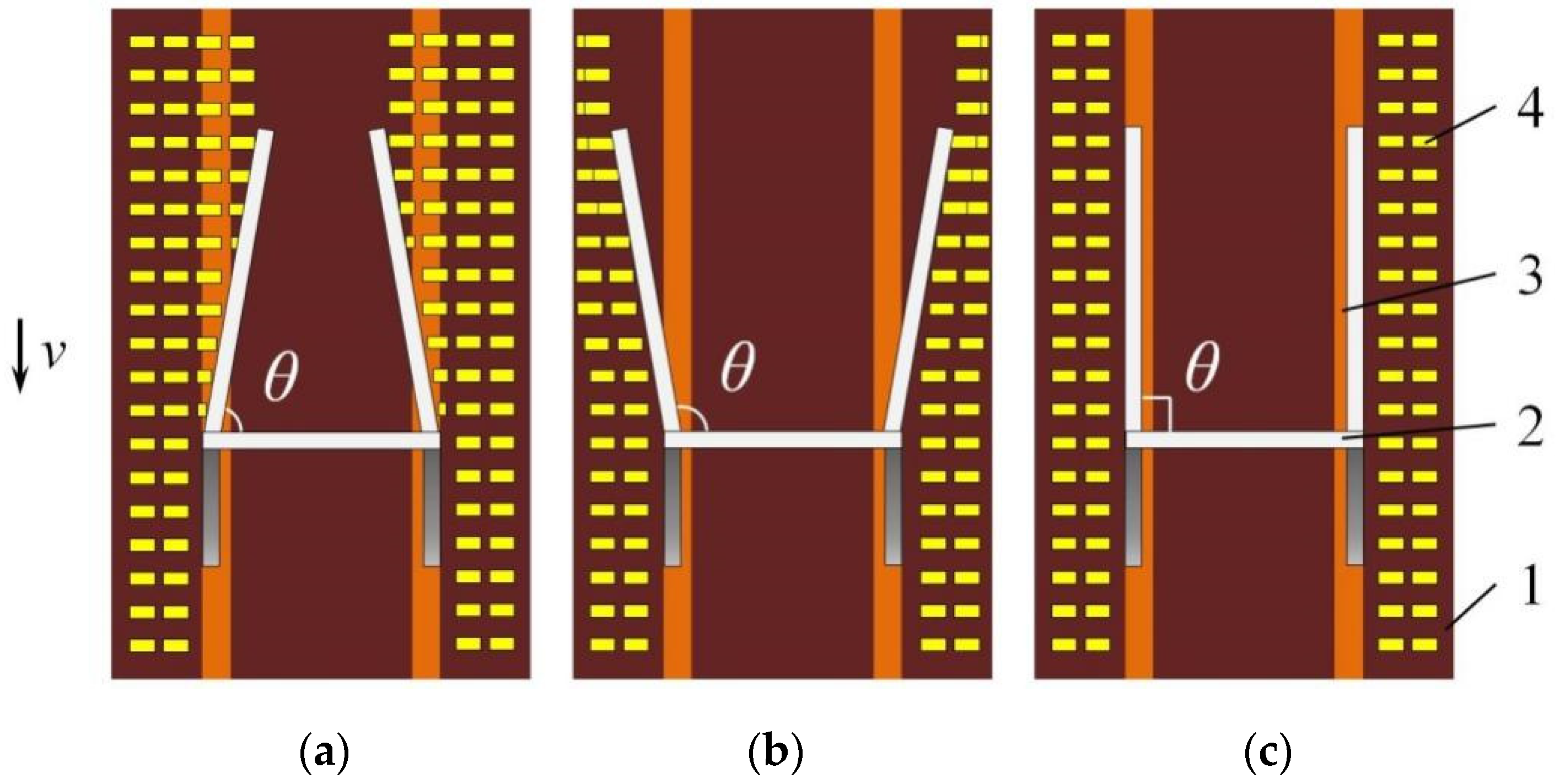
2.3. Parameter Design of the RSM
2.3.1. The Width of the RSM bG
2.3.2. The Height of the RSM hG
2.3.3. The Length of the Side Baffle lG
2.3.4. The Included Angle between the Seed Strip Baffle and the Side Baffle θ
2.4. Rational and Reasonable Parameters of the SDARD by EDEM Optimization Method
2.4.1. Establishment of the Discrete Element Simulation Model
2.4.2. Simulation Process
2.4.3. Simulation Experimental Design
3. Results and Discussion
3.1. Regression Model Construction
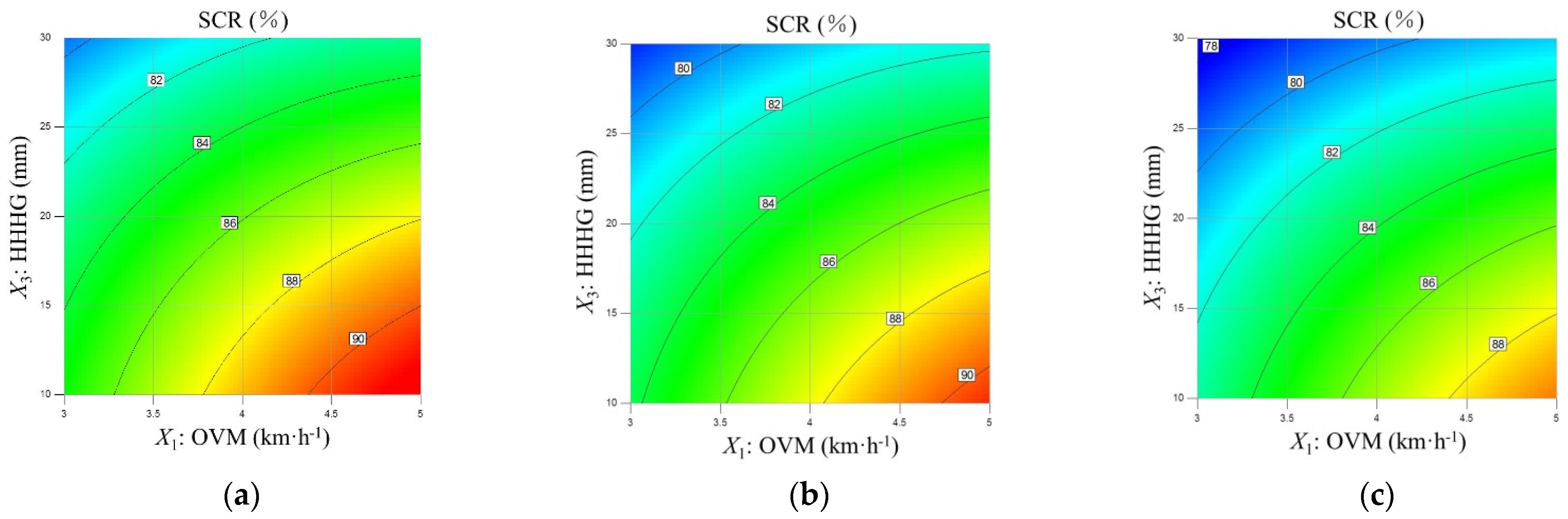
3.2. Analysis of the Influence of Particular Factor Numerical Change on Indicator
3.3. Basic Theoretical Analysis of the Influence of Interaction Factors on Indicator
3.4. Discussion
4. Optimization and Verification
4.1. Parameter Optimization
4.2. Field Test Verification
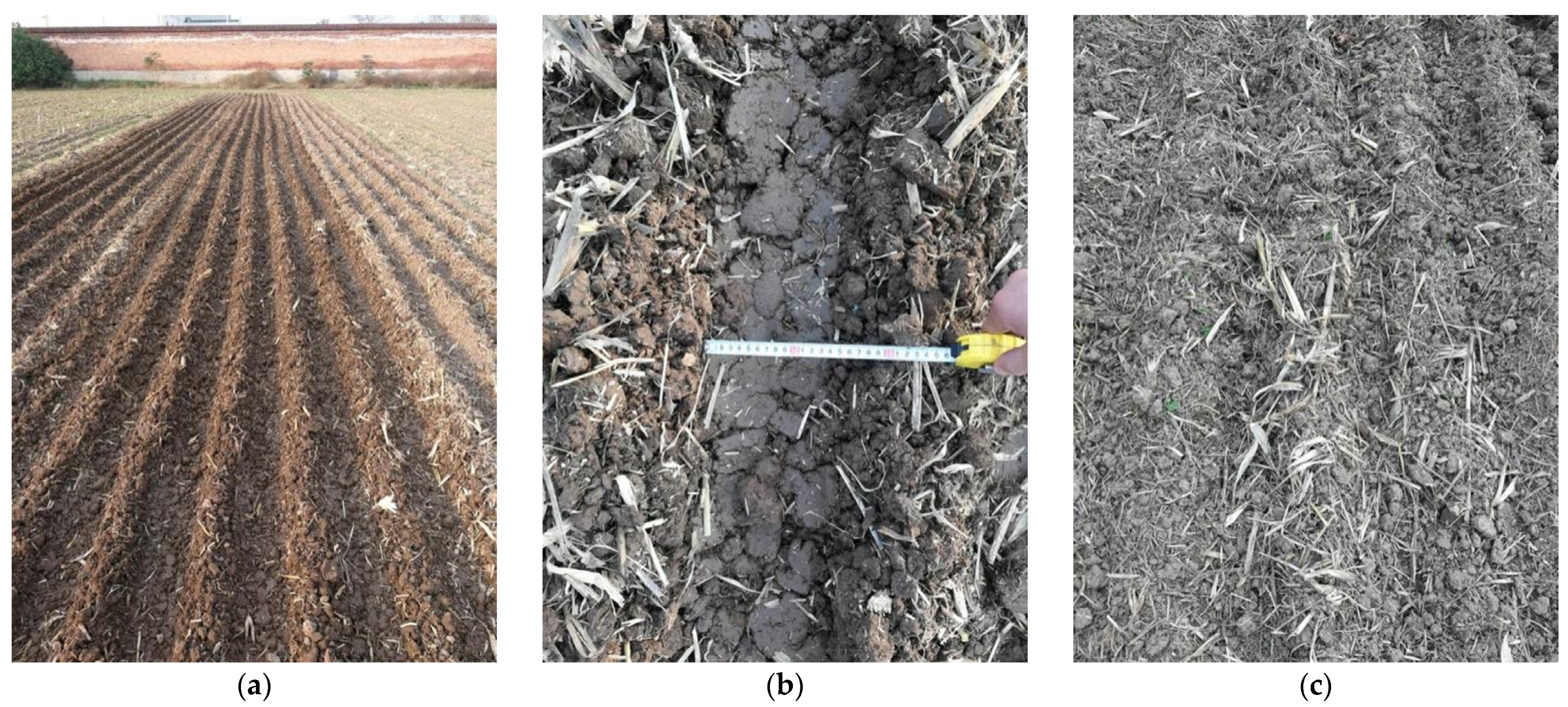
4.2.1. Field Test Conditions
4.2.2. Test Method and Result Analysis
The Passing Capacity of No-Till Planter
The SCR of the Sowing Strip
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| SDARD | Spiral discharge anti-blocking and row-sorting device |
| NAKG | No-till anti-blocking knife group |
| SDM | Spiral discharging mechanism |
| RSM | Row-sorting of straw mechanism |
| OVM/v | Operating velocity of machines |
| RVSS/n | Rotary velocity of the spiral shaft |
| HHHG/h | Height of the holding hopper from the ground |
| SCR/λ | Straw cleaning rate |
| SFA/Qs | Straw feeding amounts |
| SMQ/CS | Straw mulching quantity |
| Q1 | Mass of straw on the sowing strip before an operation |
| Q2 | Residual mass of straw on the sowing strip after an operation |
| QSt | Total mass of straw fed into the hopper after a certain period of operation |
| L1 | Total cleaning width of sowing strip |
| L2 | Total width of non-sowing area |
| D | Outer diameter of the spiral blade |
| S | Pitch |
| z | Number of corresponding sowing strips |
| K | Material characteristic coefficient |
| ψ | Filling factor |
| ρ | Material density |
| C | Inclination coefficient |
| K1 | Pitch coefficient |
| bG | Width of the RSM |
| hG | Height of the RSM |
| lG | Length of the side baffle |
| θ | Included angle between the strip baffle and the side baffle |
| m1 | Mass of straw before the operation |
| m2 | Mass of straw after the operation |
References
- He, J.; Li, H.; Chen, H.; Lu, C.; Wang, Q. Research progress of conservation tillage technology and machine. Trans. Chin. Soc. Agric. Mach. 2018, 49, 1–19. [Google Scholar] [CrossRef]
- Cao, X.; Wang, Q.; Li, H.; He, J.; Lu, C. Design and experiment of active rotating collective straw-cleaner. Trans. Chin. Soc. Agric. Eng. 2021, 37, 26–34. [Google Scholar] [CrossRef]
- Cao, X.; Wang, Q.; Li, H.; He, J.; Lu, C. Combined row cleaners research with side cutter and stubble clean disk of corn no-till seeder. Trans. Chin. Soc. Agric. Mach. 2021, 52, 9. [Google Scholar] [CrossRef]
- Wang, Q.; Cao, X.; Wang, C.; Li, H.; He, J.; Lu, C. Research progress of no/minimum tillage corn seeding technology and machine in northeast black soil regions of China. Trans. Chin. Soc. Agric. Mach. 2021, 52, 1–15. [Google Scholar]
- Jia, H.; Zhao, J.; Jiang, X.; Jiang, T.; Wang, Y.; Guo, H. Design and experiment of anti-blocking mechanism for inter-row no-tillage seeder. Trans. Chin. Soc. Agric. Eng. 2013, 29, 16–25. [Google Scholar] [CrossRef]
- Yao, Z.; Gao, H.; Wang, X.; Li, H.; Li, W. Design and experiment on 2BMX-5 no-till wheat-maize seeder. Trans. Chin. Soc. Agric. Mach. 2008, 39, 64–68. [Google Scholar]
- Fallahi, S.; Raoufat, M.H. Row-crop planter attachments in a conservation tillage system: A comparative study. Soil Tillage Res. 2008, 98, 27–34. [Google Scholar] [CrossRef]
- He, J.; Li, H.W.; Wang, Q.J.; Gao, H.W.; Li, W.Y.; Zhang, X.M.; McGiffen, M. The adoption of conservation tillage in China. Ann. N. Y. Acad. Sci. 2010, 1195, E96–E106. [Google Scholar] [CrossRef]
- He, J.; Zhang, Z.; Li, H.; Wang, Q. Development of small/medium size no-till and minimum-till seeders in Asia: A review. Int. J. Agric. Biol. Eng. 2014, 7, 1–12. [Google Scholar] [CrossRef]
- Siemens, M.C.; Wilkins, D.E.; Correa, R.F. Development and evaluation of a residue management wheel for hoe-type no-till drills. Trans. ASAE 2003, 47, 397–404. [Google Scholar] [CrossRef]
- Tourn, M.; Soza, E.; Botta, G.; Mete, A. Direct corn seedling. Effects of residue clearance on implant efficiency. Span. J. Agric. Res. 2003, 1, 99–103. [Google Scholar] [CrossRef] [Green Version]
- Morris, N.L.; Miller, P.C.H.; Orson, J.H.; Froud-Williams, R.J. The adoption of non-inversion tillage systems in the United Kingdom and the agronomic impact on soil, crops and the environment—A review. Soil Tillage Res. 2010, 108, 1–15. [Google Scholar] [CrossRef]
- Celik, A.; Altikat, S.; Way, T.R. Strip tillage width effects on sunflower seed emergence and yield. Soil Tillage Res. 2013, 131, 20–27. [Google Scholar] [CrossRef]
- Li, H.; Liu, H.; Zhou, J.; Wei, G.; Shi, S.; Zhang, X.; Zhang, R.; Zhu, H.; He, T. Development and first results of a no-till pneumatic seeder for maize precise sowing in Huang-Huai-Hai plain of China. Agriculture 2021, 11, 1023. [Google Scholar] [CrossRef]
- Wang, C.; Li, H.; He, J.; Wang, Q.; Lu, C.; Yang, H. Optimization design of a pneumatic wheat-shooting device based on numerical simulation and field test in rice–wheat rotation areas. Agriculture 2022, 12, 56. [Google Scholar] [CrossRef]
- Zhao, H. Study on Driven Seedbed-Cleaning and Anti-Blocking Device of Residue Inter-Row Side-Throwing for Minimum till Wheat Seeding; China Agricultural University: Beijing, China, 2019. [Google Scholar]
- Zheng, Z. Study on Corn Straw Pickup-Chopping and Ditch-Burying Returning Field Machine; China Agricultural University: Beijing, China, 2017. [Google Scholar]
- Wang, Q.; He, J.; Li, H.; Lu, C.; Rasaily, R.G.; Su, Y. Design and experiment on furrowing and anti-blocking unit for no-till planter. Trans. Chin. Soc. Agric. Eng. 2012, 28, 27–31. [Google Scholar] [CrossRef]
- Lin, J.; Li, B.; Li, H. Design and experiment of Archimedes spiral type stubble breaking ditching device and stubble breaking anti blocking device. Trans. Chin. Soc. Agric. Eng. 2015, 31, 10–19. [Google Scholar] [CrossRef]
- Lin, J.; Li, B.; Li, B.; Niu, J.; Qian, W. Parameter optimization and experiment on archimedes spiral type of gap cutting disc. Trans. Chin. Soc. Agric. Mach. 2014, 45, 118–124. [Google Scholar] [CrossRef]
- Lu, C. Study on Anti-Blocking Technology and Device of Rotary Cutting with Slide Plate Pressing Straw for No-Till Planter; China Agricultural University: Beijing, China, 2014. [Google Scholar]
- Lu, C.; Zhao, C.; Meng, Z.; Wang, X.; Wu, G.; Gao, N. Straw friction characteristic based on rotary cutting anti-blocking device with slide plate pressing straw. Trans. Chin. Soc. Agric. Eng. 2016, 32, 83–89. [Google Scholar] [CrossRef]
- Lu, C.; Li, H.; He, J.; Zhu, H.; Xu, D. Floated support anti-blocking device of wheat no-till seeder. Trans. Chin. Soc. Agric. Eng. 2013, 44, 52–55, 153. [Google Scholar] [CrossRef]
- Lu, C.; He, J.; Li, H.; Wang, Q.; Zhang, X.; Liu, J. Finite element analysis and experiment on anti-blocking device based on support cutting. Trans. Chin. Soc. Agric. Mach. 2013, 44, 61–66. [Google Scholar] [CrossRef]
- Yuan, P.; Li, H.; Jiang, G.; He, J.; Lu, C.; Huang, S. Design and experiment of straw cleaning device for wide narrow maize no-tillage sowing strip in drip irrigation area. Trans. Chin. Soc. Agric. Mach. 2021, 52, 43–52. [Google Scholar] [CrossRef]
- Yu, C.; Wang, Q.; Li, H.; He, J.; Lu, C. Design and experiment of spiral-split sowing strip cleaning device. Trans. Chin. Soc. Agric. Eng. 2020, 51, 212–219. [Google Scholar]
- Zhou, H. The Application of Quadrat Method in Parameters of Forest Spatial Structure Investigation; Chinese Academy of Forestry: Beijing, China, 2009. [Google Scholar] [CrossRef]
- Darnell, W.H. Solid Conveying in Extruders. SPE J. 1956, 12, 20–29. [Google Scholar]
- Yuan, Q.; Xu, L.; Niu, C.; Ma, S.; Yan, C.; Zhao, S.; Liu, F.; Wang, K. Development of soil-fertilizer mixing layered backfiller for organic fertilizer deep applicator in orchard. Trans. Chin. Soc. Agric. Eng. 2021, 37, 11–19. [Google Scholar] [CrossRef]
- “Transportation Machinery Design and Selection Manual” Committee. Transport Machinery Design and Selection Manual, the Next Volume; Chemical Industry Press: Beijing, China, 1999. [Google Scholar]
- Wang, C.; Zhao, F.; Wang, X. Experiment and optimization of screw conveyor parameters for rubbing and breaking corn straw. J. China Agric. Univ. 2019, 24, 115–122. [Google Scholar] [CrossRef]
- Wang, C.; Qi, S.; Yan, J.; Wang, J. Test and analysis of performance of screw conveyor for rubbing and breaking corn straw. Trans. Chin. Soc. Agric. Eng. 2015, 31, 51–59. [Google Scholar] [CrossRef]
- Study on the Mechanism of Screw Conveying Theory for Rubbing and Breaking Corn Straw; Inner Mongolia Agricultural University: Hohhot, China, 2016.
- Wang, X.; Hu, H.; Wang, Q.; Li, H.; He, J.; Chen, W. Calibration method of soil contact characteristic parameters based on DEM theory. Trans. Chin. Soc. Agric. Eng. 2017, 48, 78–85. [Google Scholar] [CrossRef]
- Fang, H.; Ji, C.; Ahmed, A.T.; Zhang, Q.; Guo, J. Simulation analysis of straw movement in straw soil rotary blade system. Trans. Chin. Soc. Agric. Mach. 2016, 47, 60–67. [Google Scholar] [CrossRef]
- Ni, H.; Lu, F.; Luo, X.; Tian, H.; Wang, J.; Guan, Y.; Chen, S.; Luo, X.; Zeng, E. Assessment of sampling designs to measure riverine fluxes from the pearl river delta, china to the south china sea. Environ. Monit. Assess. 2008, 143, 291–301. [Google Scholar] [CrossRef]






| Parameters | Value | Parameters | Value |
|---|---|---|---|
| Coefficient of restitution among soil | 0.20 | Coefficient of rolling friction between soil and steel | 0.04 |
| Coefficient of static friction among soil | 0.40 | Coefficient of restitution among straw | 0.40 |
| Coefficient of rolling friction among soil | 0.31 | Coefficient of static friction among straw | 0.30 |
| Coefficient of restitution between soil and straw | 0.30 | Coefficient of rolling friction among straw | 0.01 |
| Coefficient of static friction between soil and straw | 0.30 | Coefficient of static friction between straw and steel | 0.30 |
| Coefficient of rolling friction between soil and straw | 0.01 | Coefficient of rolling friction between straw and steel | 0.30 |
| Coefficient of restitution between soil and steel | 0.30 | Coefficient of restitution between straw and steel | 0.01 |
| Coefficient of static friction between soil and steel | 0.50 |
| Code | Factors | ||
|---|---|---|---|
| The OVM X1/(km∙h−1) | The RVSS X2/(r∙min−1) | The HHHG X3/mm | |
| −1 | 3 | 80 | 10 |
| 0 | 4 | 120 | 20 |
| 1 | 5 | 160 | 30 |
| Test No. | Factors | ||||
|---|---|---|---|---|---|
| The OVM X1 | The RVSS X2 | The HHHG X3 | SCR y/% | Calculated Values y | |
| 1 | −1 | −1 | 0 | 83.25 | 86.43 |
| 2 | 1 | −1 | 0 | 87.28 | 91.53 |
| 3 | −1 | 1 | 0 | 80.75 | 84.33 |
| 4 | 1 | 1 | 0 | 85.85 | 89.43 |
| 5 | −1 | 0 | −1 | 83.65 | 87.32 |
| 6 | 1 | 0 | −1 | 91.15 | 94.28 |
| 7 | −1 | 0 | 1 | 78.15 | 82.14 |
| 8 | 1 | 0 | 1 | 81.92 | 85.38 |
| 9 | 0 | −1 | −1 | 88.59 | 92.40 |
| 10 | 0 | 1 | −1 | 86.48 | 90.30 |
| 11 | 0 | −1 | 1 | 82.01 | 85.36 |
| 12 | 0 | 1 | 1 | 79.65 | 83.26 |
| 13 | 0 | 0 | 0 | 84.86 | 88.48 |
| 14 | 0 | 0 | 0 | 84.05 | 88.48 |
| 15 | 0 | 0 | 0 | 86.03 | 88.48 |
| 16 | 0 | 0 | 0 | 84.66 | 88.48 |
| 17 | 0 | 0 | 0 | 84.97 | 88.48 |
| Variation Source | Sum of Squares | Degree Freedom | Mean Square | F Values | p-Values |
|---|---|---|---|---|---|
| Model | 166.57 | 6 | 27.76 | 86.21 | <0.0001 *** |
| X1 | 52.02 | 1 | 52.02 | 161.54 | <0.0001 *** |
| X2 | 8.82 | 1 | 8.82 | 27.39 | 0.0004 *** |
| X3 | 98.98 | 1 | 98.98 | 307.37 | <0.0001 *** |
| X1X3 | 3.48 | 1 | 3.48 | 10.80 | 0.0082 *** |
| X12 | 1.29 | 1 | 1.29 | 4.00 | 0.0733 * |
| X32 | 1.80 | 1 | 1.80 | 5.58 | 0.0397 ** |
| Residual | 3.22 | 10 | 0.32 | ||
| Lack of fit | 1.16 | 6 | 0.19 | 0.37 | 0.8642 |
| Pure error | 2.06 | 4 | 0.52 | ||
| Total sum | 169.79 | 16 |
| Items | Parameters | Values |
|---|---|---|
| The straw of the field | Average length/mm | 138 |
| Length range/mm | 65–220 | |
| Average diameter/mm | 4.32 | |
| Diameter range/mm | 1–12 | |
| Covering thickness/mm | 47 | |
| SMQ/(kg·m−2) | 1.63 | |
| Moisture content/% | 68.06 | |
| 0–100 mm soil layer | Firmness/kPa | 532 |
| Moisture content/% | 21.46 | |
| Bulk density/(g·cm−3) | 1.49 | |
| Temperature/℃ | 16.8 |
| Parameters | The SCR of Seed Strip Installed with SDARD/% | The SCR of Seed Strip Installed without SDARD/% |
|---|---|---|
| Average | 84.49 | 73.16 |
| Variation | 6.73 | 9.48 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Lu, C.; Li, H.; He, J.; Wang, Q.; Huang, S.; Gao, Z.; Yuan, P.; Wei, X.; Zhan, H. Design and Experiment of Spiral Discharge Anti-Blocking and Row-Sorting Device of Wheat No-Till Planter. Agriculture 2022, 12, 468. https://doi.org/10.3390/agriculture12040468
Li Y, Lu C, Li H, He J, Wang Q, Huang S, Gao Z, Yuan P, Wei X, Zhan H. Design and Experiment of Spiral Discharge Anti-Blocking and Row-Sorting Device of Wheat No-Till Planter. Agriculture. 2022; 12(4):468. https://doi.org/10.3390/agriculture12040468
Chicago/Turabian StyleLi, Yunxiang, Caiyun Lu, Hongwen Li, Jin He, Qingjie Wang, Shenghai Huang, Zhen Gao, Panpan Yuan, Xuyang Wei, and Huimin Zhan. 2022. "Design and Experiment of Spiral Discharge Anti-Blocking and Row-Sorting Device of Wheat No-Till Planter" Agriculture 12, no. 4: 468. https://doi.org/10.3390/agriculture12040468
APA StyleLi, Y., Lu, C., Li, H., He, J., Wang, Q., Huang, S., Gao, Z., Yuan, P., Wei, X., & Zhan, H. (2022). Design and Experiment of Spiral Discharge Anti-Blocking and Row-Sorting Device of Wheat No-Till Planter. Agriculture, 12(4), 468. https://doi.org/10.3390/agriculture12040468









