Ramie Field Distribution Model and Miss Cutting Rate Prediction Based on the Statistical Analysis
Abstract
:1. Introduction
2. Materials and Methods
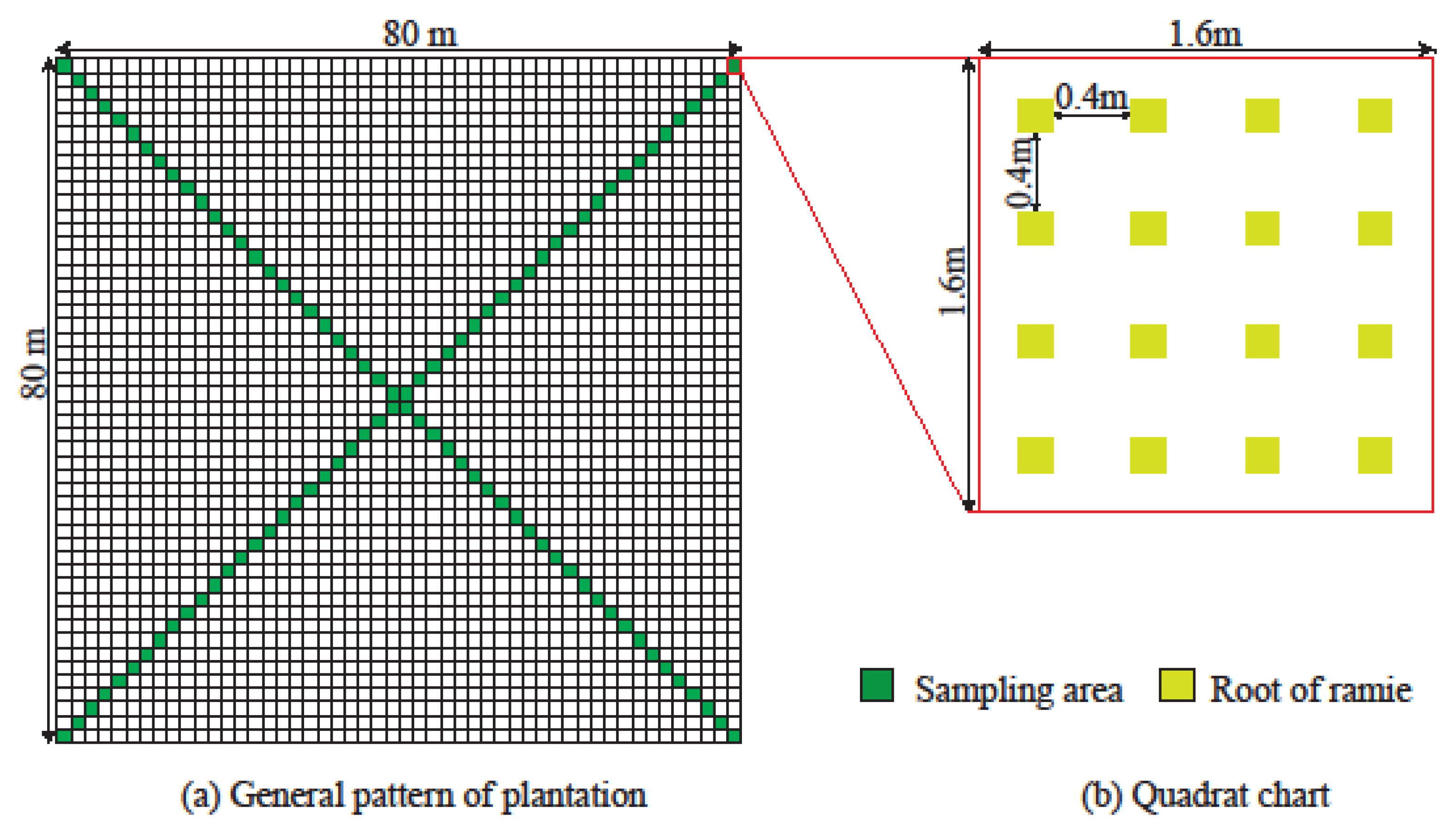
2.1. Statistical Analysis of Ramie Field Plant Distribution
2.2. Ramie Field Harvest Test
2.2.1. Overview of Ramie Harvester
2.2.2. Field Test Method
3. Results
3.1. Distribution Pattern of Ramie Plant Number
3.2. Normality Test
3.2.1. Normality Test: Graphic Qualitative Judgment
3.2.2. Normality Test: Shapiro–Wilk Test
3.2.3. Normality Test: Skewness and Kurtosis Quantitative Judgment Method
3.3. Normal Distribution Parameters
3.4. Feeding Quantity Model of Ramie Harvester
3.4.1. Normality Test
3.4.2. Normal Distribution Parameters and Probability of Ramie Plant Range
3.5. Kinematic Analysis of Reciprocating Cutter
3.5.1. Cutting Knife Stroke
3.5.2. Displacement of Cutting Knife
3.5.3. Absolute Motion Trail of Cutting Knife
3.6. Analysis on the Results of Ramie Field Harvesting Experiment
4. Discussion
5. Conclusions
- (1)
- The number of ramie stalks per unit area (1.6 m × 1.6 m) X obeys the normal distribution N (72.5, 4.456), when the ramie plant number range is in (67, 78), the probability is 99.1%. At this probability level, according to the ramie harvester forward speed, the feed quantity of the ramie harvester in different forward speeds (0.8–1 m/s) was determined.
- (2)
- The absolute motion trail of the ramie harvester cutting knife is analyzed and the motion equation is obtained. The calculation equation of the area of the missing cutting area is obtained by integral operation, as shown in Formula (15), and then the prediction model of the mis-cutting rate is obtained, as shown in Formulas (16) and (17).
- (3)
- The results of the ramie field harvest were all within the predicted range, showing that the prediction model of the feed quantity and mis-cutting rate was effective. These methods can provide references to the control and optimization of ramie harvester parameters, improve the efficiency of harvester power utilization, and reduce harvesting losses.
- (4)
- In this study, only the spatial distribution model of ramie stems under common ramie cropping patterns was investigated. However, other cropping patterns and mechanized harvesting studies of bush crops can also refer to the research ideas in this paper.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Numbering of Land Parcel | First Crop Ramie | Second Crop Ramie | Third Crop Ramie |
|---|---|---|---|
| 1 | 72 | 74 | 73 |
| 2 | 70 | 67 | 74 |
| 3 | 72 | 72 | 76 |
| 4 | 69 | 72 | 66 |
| 5 | 73 | 71 | 72 |
| 6 | 70 | 73 | 71 |
| 7 | 77 | 71 | 70 |
| 8 | 72 | 75 | 74 |
| 9 | 71 | 75 | 75 |
| 10 | 73 | 75 | 69 |
| 11 | 72 | 71 | 70 |
| 12 | 70 | 71 | 72 |
| 13 | 73 | 72 | 73 |
| 14 | 69 | 72 | 74 |
| 15 | 75 | 76 | 71 |
| 16 | 70 | 73 | 73 |
| 17 | 70 | 73 | 74 |
| 18 | 74 | 75 | 74 |
| 19 | 77 | 75 | 67 |
| 20 | 70 | 74 | 71 |
| 21 | 74 | 74 | 75 |
| 22 | 71 | 73 | 73 |
| 23 | 74 | 75 | 71 |
| 24 | 73 | 70 | 76 |
| 25 | 74 | 72 | 71 |
| 26 | 74 | 71 | 72 |
| 27 | 73 | 68 | 71 |
| 28 | 68 | 74 | 69 |
| 29 | 74 | 73 | 71 |
| 30 | 72 | 71 | 74 |
| 31 | 72 | 76 | 75 |
| 32 | 73 | 70 | 74 |
| 33 | 72 | 73 | 72 |
| 34 | 73 | 71 | 76 |
| 35 | 71 | 70 | 72 |
| 36 | 73 | 75 | 73 |
| 37 | 74 | 72 | 72 |
| 38 | 72 | 71 | 78 |
| 39 | 72 | 74 | 74 |
| 40 | 74 | 74 | 74 |
| 41 | 71 | 75 | 70 |
| 42 | 71 | 72 | 75 |
| 43 | 72 | 72 | 73 |
| 44 | 72 | 72 | 68 |
| 45 | 67 | 75 | 72 |
| 46 | 76 | 71 | 74 |
| 47 | 71 | 76 | 71 |
| 48 | 73 | 76 | 77 |
| 49 | 71 | 74 | 74 |
| 50 | 71 | 69 | 71 |
| 51 | 73 | 72 | 75 |
| 52 | 75 | 74 | 71 |
| 53 | 72 | 72 | 74 |
| 54 | 70 | 73 | 72 |
| 55 | 72 | 72 | 73 |
| 56 | 73 | 75 | 73 |
| 57 | 72 | 70 | 75 |
| 58 | 76 | 73 | 73 |
| 59 | 71 | 74 | 73 |
| 60 | 73 | 72 | 72 |
| 61 | 76 | 72 | 75 |
| 62 | 73 | 73 | 74 |
| 63 | 71 | 71 | 72 |
| 64 | 68 | 71 | 70 |
| 65 | 74 | 73 | 71 |
| 66 | 73 | 74 | 69 |
| 67 | 70 | 71 | 68 |
| 68 | 73 | 77 | 69 |
| 69 | 72 | 75 | 70 |
| 70 | 74 | 75 | 72 |
| 71 | 73 | 72 | 75 |
| 72 | 74 | 73 | 70 |
| 73 | 72 | 75 | 73 |
| 74 | 70 | 71 | 69 |
| 75 | 69 | 74 | 72 |
| 76 | 70 | 76 | 75 |
| 77 | 73 | 73 | 73 |
| 78 | 73 | 69 | 72 |
| 79 | 74 | 75 | 75 |
| 80 | 76 | 71 | 69 |
| 81 | 71 | 72 | 68 |
| 82 | 75 | 70 | 72 |
| 83 | 72 | 72 | 72 |
| 84 | 70 | 77 | 71 |
| 85 | 74 | 71 | 72 |
| 86 | 72 | 76 | 73 |
| 87 | 74 | 69 | 74 |
| 88 | 74 | 73 | 73 |
| 89 | 70 | 75 | 73 |
| 90 | 70 | 73 | 70 |
| 91 | 74 | 73 | 72 |
| 92 | 74 | 72 | 71 |
| 93 | 75 | 72 | 76 |
| 94 | 69 | 74 | 71 |
| 95 | 72 | 69 | 71 |
| 96 | 70 | 74 | 74 |
| 97 | 73 | 72 | 75 |
| 98 | 73 | 72 | 74 |
| 99 | 76 | 70 | 74 |
| 100 | 74 | 74 | 75 |
Appendix B

References
- Djafar, Z.; Renreng, I.; Jannah, M. Tensile and Bending Strength Analysis of Ramie Fiber and Woven Ramie Reinforced Epoxy Composite. J. Nat. Fibers 2021, 18, 2315–2326. [Google Scholar] [CrossRef]
- Yu, M.; Zhang, H.; Liu, Z.; Ge, Z.; Kong, F.; Shao, H.; Hu, X. Effects of Fiber Dimension and Its Distribution on the Properties of Lyocell and Ramie Fibers Reinforced Polylactide Composites. Fibers Polym. 2019, 20, 1726–1732. [Google Scholar] [CrossRef]
- Dilfi, A.K.F.; Che, Z.-J.; Xian, G.-J. Grafting of nano-silica onto ramie fiber for enhanced mechanical and interfacial properties of ramie/epoxy composite. J. Zhejiang Univ.-Sci. A 2019, 20, 660–674. [Google Scholar] [CrossRef]
- Xu, Y.; Tang, Q.; Dai, Z.G.; Yang, Z.M.; Cheng, C.H.; Deng, C.H.; Liu, C.; Chen, J.H.; Su, J.G. Yield components of forage ramie (Boehmeria nivea L.) and their effects on yield. Genet. Resour. Crop Evol. 2019, 66, 1601–1613. [Google Scholar] [CrossRef]
- Zhang, H.; He, Y.; Yu, L.H.; Tang, S.X.; Tan, Z.L.; Zhou, C.S.; Dong, L.; Wang, H.R. The potential of ramie as forage for ruminants: Impacts on growth, digestion, ruminal fermentation, carcass characteristics and meat quality of goats. Anim. Sci. J. 2019, 90, 481–492. [Google Scholar] [CrossRef] [PubMed]
- Lu, J.; Long, C.; Zhao, J.; Ma, L.; Lu, H.; Liu, J.; He, H. Design and experiment of transverse-feeding ramie decorticator. Trans. Chin. Soc. Agric. Eng. 2013, 29, 16–21. [Google Scholar]
- Tan, X.; Guo, K.; Zhang, D.; Su, N.; Man, D.; Yuan, X. Design of the lifting ramie decorticator. J. For. Eng. 2019, 4, 106–111. [Google Scholar]
- Xiang, W.; Ma, L.; Liu, J.; Xiao, L.; Long, C.; Wen, Q.; Lyu, J. Research Progress on Technology and Equipment of Ramie Fibre Stripping and Processing in China. J. Agric. Sci. Technol. 2019, 21, 59–69. [Google Scholar]
- Pari, L.; Baraniecki, P.; Kaniewski, R.; Scarfone, A. Harvesting strategies of bast fiber crops in Europe and in China. Ind. Crops Prod. 2015, 68, 90–96. [Google Scholar] [CrossRef]
- Huang, J.C.; Tian, K.P.; Shen, C.; Bin; Liu, H.L.; Chen, Q.M.; Li, X.W.; Ji, A.M. Design and parameters optimization for cutting-conveying mechanism of ramie combine harvester. Int. J. Agric. Biol. Eng. 2020, 13, 94–103. [Google Scholar] [CrossRef]
- Chen, Z.; Niu, R.; Wang, J.; Zhu, H.; Yu, B. Navigation Line Extraction Method for Ramie Combine Harvester Based on U-Net. In Proceedings of the 6th Asia-Pacific Conference on Intelligent Robot Systems (ACIRS), Tokyo, Japan, 16–18 July 2021; pp. 94–100. [Google Scholar]
- Shen, C.; Li, X.; Tian, K.; Zhang, B.; Huang, J.; Chen, Q. Experimental analysis on mechanical model of ramie stalk. Trans. Chin. Soc. Agric. Eng. 2015, 31, 26–33. [Google Scholar]







| Parameter Items | Engine Parameter | Swath | Productivity | Stubble Height |
|---|---|---|---|---|
| Numerical value | 25.7 kW | 1600 mm | 0.1–0.2 hm2/h | ≤10 cm |
| Distribution Pattern | Progressive Significance (Bilateral) | ||
|---|---|---|---|
| First Crop Ramie | Second Crop Ramie | Third Crop Ramie | |
| Normal distribution | 0.125 | 0.105 | 0.194 |
| Uniform distribution | 0.016 | 0.003 | 0.000 |
| Poisson distribution | 0.000 | 0.000 | 0.000 |
| Exponential distribution | 0.000 | 0.000 | 0.000 |
| Ramie Season | Statistic | Df | Sig. |
|---|---|---|---|
| First crop ramie | 0.973 | 100 | 0.036 |
| Second crop ramie | 0.971 | 100 | 0.028 |
| Third crop ramie | 0.977 | 100 | 0.072 |
| Parameters | First Crop Ramie | Second Crop Ramie | Third Crop Ramie | |
|---|---|---|---|---|
| Skewness | statistics | −0.075 | −0.184 | −0.302 |
| standard error | 0.241 | 0.241 | 0.241 | |
| Z-score | −0.311 | −0.763 | −1.253 | |
| Kurtosis | statistics | −0.128 | −0.249 | 0.023 |
| standard error | 0.478 | 0.478 | 0.478 | |
| Z-score | −0.268 | −0.521 | 0.048 | |
| Parameters | First Crop Ramie | Second Crop Ramie | Third Crop Ramie |
|---|---|---|---|
| Mean value | 72.34 | 72.74 | 72.42 |
| standard deviation | 2.026 | 2.043 | 2.257 |
| - | Skewness | Kurtosis | ||||
|---|---|---|---|---|---|---|
| Statistics | Standard Error | Z-Score | Statistics | Standard Error | Z-Score | |
| Ramie | −0.203 | 0.141 | −1.440 | −0.106 | 0.281 | −0.377 |
| - | Mean Value | Standard Deviation |
|---|---|---|
| Ramie | 72.5 | 2.111 |
| Ramie Plant Range | (72, 73) | (71, 74) | (70, 75) | (69, 76) | (68, 77) | (67, 78) |
|---|---|---|---|---|---|---|
| Probability/% | 18.96 | 52.22 | 76.2 | 90.3 | 96.68 | 99.1 |
| Forward Speed vm (m/s) | Cutting Speed Ratio K | Experimental Value | Calculated Value | ||
|---|---|---|---|---|---|
| Feed Quantity (Plant/s) | Miss Cutting Rate/% | Feed Quantity (Plant/s) | Miss Cutting Rate/% | ||
| 0.8 | 1.75 | 36.7 ± 1.059 | 2.183 ± 0.1172 | (33.5,39) | (1.9,2.4) |
| 0.85 | 1.65 | 38.8 ± 1.135 | 2.481 ± 0.0999 | (35.6,41.4) | (2.1,2.7) |
| 0.9 | 1.56 | 41.3 ± 1.252 | 2.748 ± 0.0629 | (37.7,43.8) | (2.2,2.9) |
| 0.95 | 1.47 | 40.7 ± 1.059 | 3.049 ± 0.0950 | (39.8,46.3) | (2.4,3.3) |
| 1 | 1.4 | 45.7 ± 1.160 | 3.318 ± 0.1311 | (41.9,48.7) | (2.7,3.8) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, B.; Liu, H.; Huang, J.; Tian, K.; Shen, C.; Li, X.; Wang, X. Ramie Field Distribution Model and Miss Cutting Rate Prediction Based on the Statistical Analysis. Agriculture 2022, 12, 651. https://doi.org/10.3390/agriculture12050651
Zhang B, Liu H, Huang J, Tian K, Shen C, Li X, Wang X. Ramie Field Distribution Model and Miss Cutting Rate Prediction Based on the Statistical Analysis. Agriculture. 2022; 12(5):651. https://doi.org/10.3390/agriculture12050651
Chicago/Turabian StyleZhang, Bin, Haolu Liu, Jicheng Huang, Kunpeng Tian, Cheng Shen, Xianwang Li, and Xingsong Wang. 2022. "Ramie Field Distribution Model and Miss Cutting Rate Prediction Based on the Statistical Analysis" Agriculture 12, no. 5: 651. https://doi.org/10.3390/agriculture12050651
APA StyleZhang, B., Liu, H., Huang, J., Tian, K., Shen, C., Li, X., & Wang, X. (2022). Ramie Field Distribution Model and Miss Cutting Rate Prediction Based on the Statistical Analysis. Agriculture, 12(5), 651. https://doi.org/10.3390/agriculture12050651







