Study on the Dynamic Cutting Mechanism of Green Pepper (Zanthoxylum armatum) Branches under Optimal Tool Parameters
Abstract
:1. Introduction
2. Materials and Methods
2.1. Determination of Basic Properties of Green Pepper Branches
2.2. Establishment and Validation of the Finite Element Model
2.2.1. Establishment of the Assembly Model
2.2.2. Material Model
2.2.3. Boundary Condition Setting
2.2.4. Mesh Generation
2.2.5. Job Submission and Data Post-Processing
2.3. Model Comparison Test
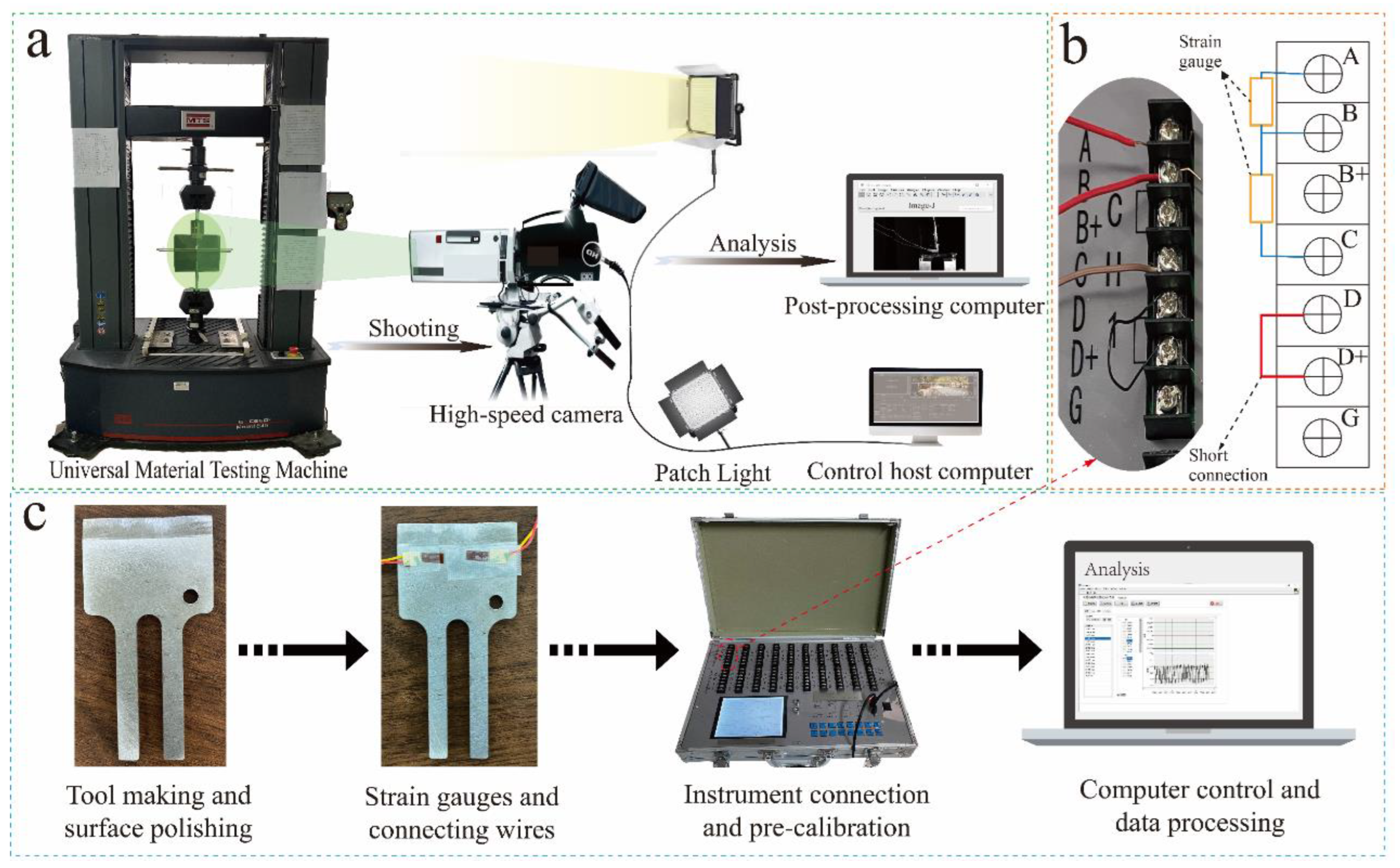
2.3.1. Tension Machine Test Design
2.3.2. Finite Element Model Validation Comparison
2.4. Determination of the Friction Coefficient between the Tool and the Green Pepper Branches
2.5. Tool Force Analysis
2.6. Cutting Tool Orthogonal Test
2.7. Induction Comparison Experiment
3. Analysis and Discussion
3.1. Results and Analysis of Multi-Factor Simulation Optimization Test
3.2. Kinematic and Impact Mechanics Analysis of Cutting
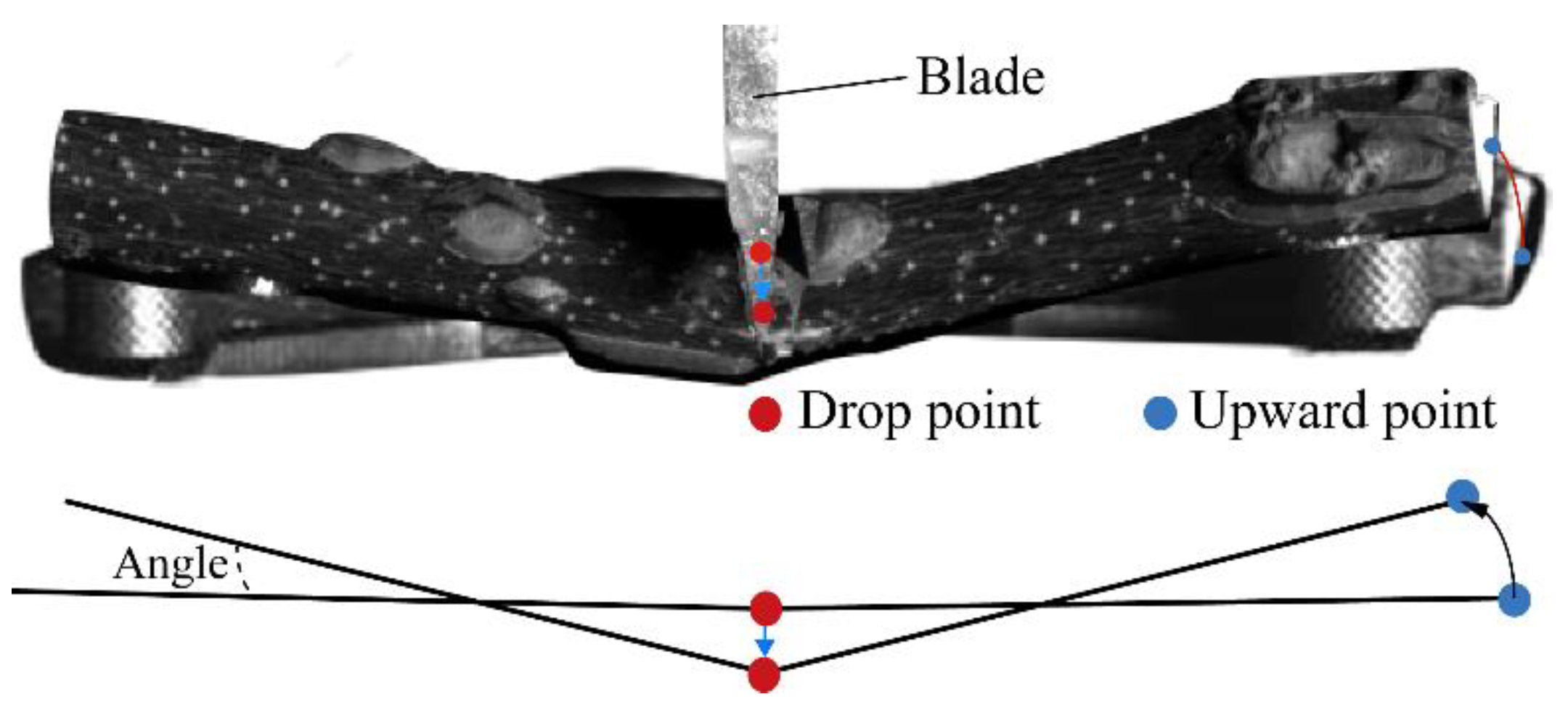
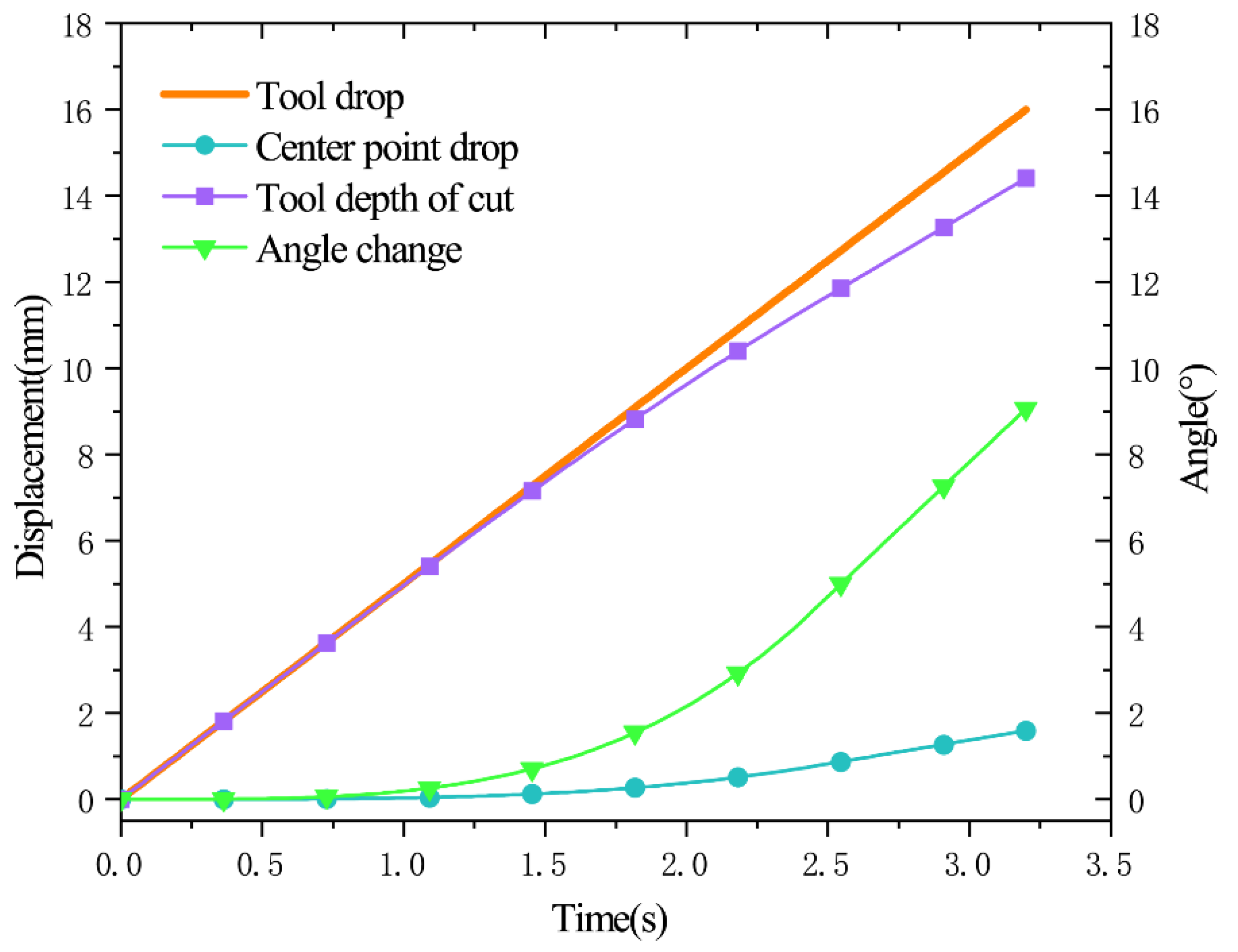
3.2.1. Kinematic Analysis of Green Pepper Branches
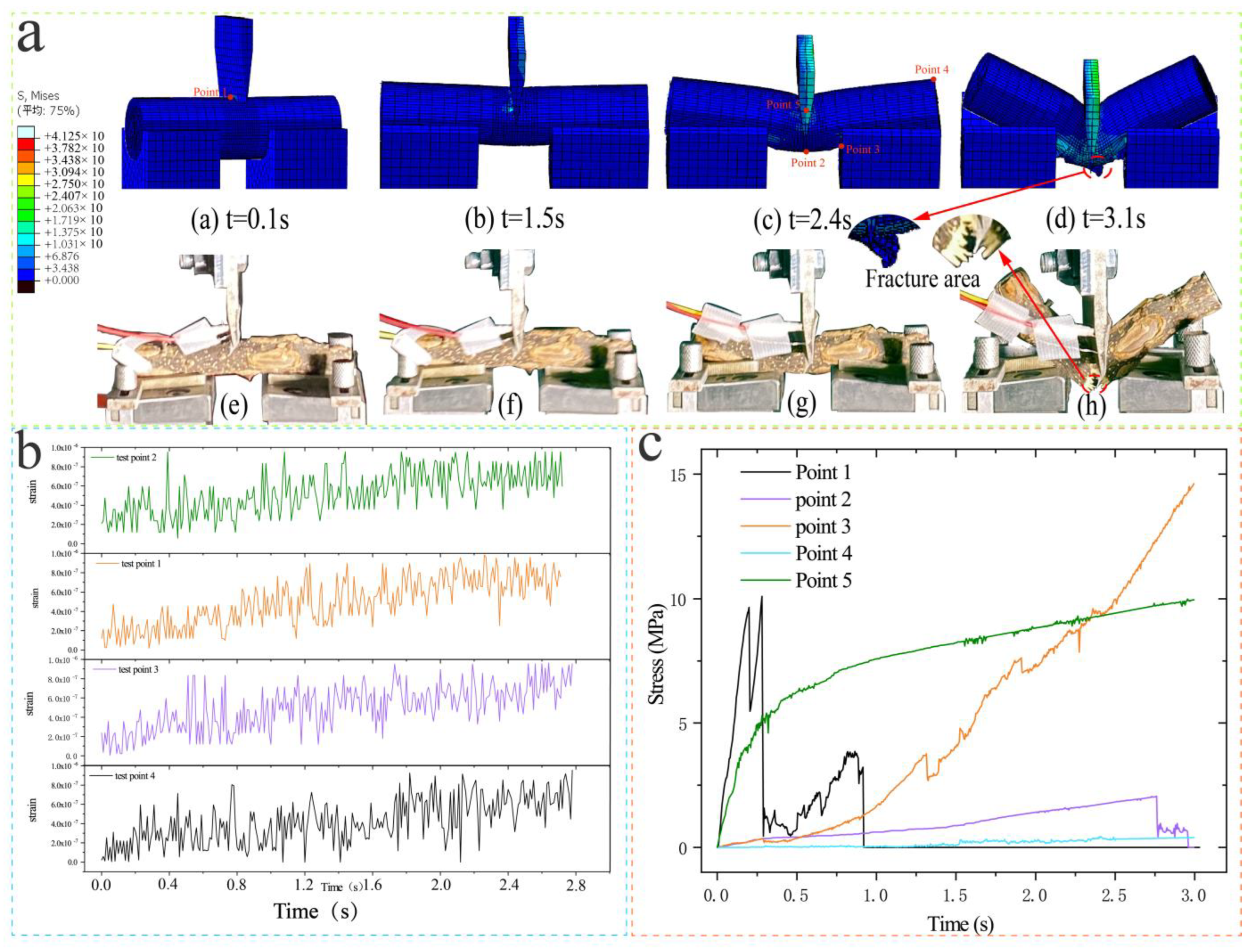
3.2.2. Green Pepper Branch Cutting Process and Stress Analysis
4. Conclusions
- (1)
- The finite element model is accurate, and the prediction results are verified by comparison with the experimental results. The comparison shows that the prediction results are in good agreement with the measured results, and the error is less than 5%, and the minimum Pearson correlation coefficient on the trend is 0.98342.
- (2)
- Within the selected parameters, the optimal structure and operating parameters of the shearing device were determined as follows: an edge angle of 16°, a cutting angle of 73.23°, and a cutting rate of 5.01 mm·s−1. At this time, the theoretical maximum cutting force and cutting completion are 803.35 N and 98.58% respectively. In addition, the thickness of the tool used in this study was 4 mm, the material was 45 Cr, and the cutting span was 20 mm. These parameters will provide a direct design basis for the design of the harvesting device.
- (3)
- According to the ANOVA results, the R2( r-squared value) value of the RSM quadratic regression model is greater than 98% and the significance is greater than 99%, which indicates that the model prediction is reliable.
- (4)
- For the sliding friction factor of green pepper branches needed for the study, relevant experiments were designed to determine it. The transverse friction factor of 0.676 ± 0.06 and the longitudinal friction factor of 0.725 ± 0.07 were obtained, and the parameters were determined to be reliable in orthogonal tests, which can save time for the related study.
- (5)
- The force of the tool after cutting into the green pepper branch was analyzed, and a reaction force model was established in the ideal state, and the model was verified by the test. The quantitative theoretical analysis of the model and the test were compared with each other, which can provide a reference for the relevant test.
- (6)
- A model for monitoring the cutting process of green pepper was established, and the stress, changing morphology, strain, and reference point displacement were linked together with time as a carrier to reveal the mechanism of span cutting of green pepper branches across the whole process. Through cross-referencing comparison, the damage limit of the cutting stress of green pepper branch at 16° and 5.01 mm·s−1 is 10.07 ± 0.02 MPa, the bending limit stress is 2.14 ± 0.01 MPa, and the limit stresses on the tool and the support member during the whole cutting process are 9.93 ± 0.02 MPa and 14.5 ± 0.02 MPa, respectively. The maximum strain on the tool is 9.57 × 10−7. The above findings have a very positive effect on the research of the devices for green pepper harvesting and branch handlings, such as the selection of materials, the determination of cutting structure, and the optimization of parameters.
- (7)
- The proposed approach consists of parameter optimization, practical manipulation and modeling, which ensures better machinability and applicability.
Author Contributions
Funding
Conflicts of Interest
References
- Wang, M.; Tong, S.; Ma, T.; Xi, Z.; Liu, J. Chromosome-level Genome Assembly of Sichuan Pepper Provides Insights into Apomixis, Drought Tolerance, and Alkaloid Biosynthesis. Mol. Ecol. Resour. 2021, 21, 2533–2545. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Wang, J.; Zhu, L.; Li, T.; Jiang, W.; Zhou, J.; Peng, W.; Wu, C. Zanthoxylum bungeanum Maxim. (Rutaceae): A Systematic Review of Its Traditional Uses, Botany, Phytochemistry, Pharmacology, Pharmacokinetics, and Toxicology. Int. J. Mol. Sci. 2017, 18, 2172. [Google Scholar] [CrossRef] [PubMed]
- Mukhtar, H.M.; Kalsi, V. A Review on Medicinal Properties of Zanthoxylum armatum DC. Res. J. Pharm. Technol. 2018, 11, 2131. [Google Scholar] [CrossRef]
- Supabphol, R.; Tangjitjareonkun, J. Chemical Constituents and Biological Activities of Zanthoxylum Limonella (Rutaceae): A Review. Trop. J. Pharm. Res. 2015, 13, 2119. [Google Scholar] [CrossRef] [Green Version]
- Cao, M.; Zhang, S.; Li, M.; Liu, Y.; Dong, P.; Li, S.; Kuang, M.; Li, R.; Zhou, Y. Discovery of Four Novel Viruses Associated with Flower Yellowing Disease of Green Sichuan Pepper (Zanthoxylum armatum) by Virome Analysis. Viruses 2019, 11, 696. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xiong, Y.; Huang, G.; Yao, Z.; Zhao, C.; Zhu, X.; Wu, Q.; Zhou, X.; Li, J. Screening Effective Antifungal Substances from the Bark and Leaves of Zanthoxylum Avicennae by the Bioactivity-Guided Isolation Method. Molecules 2019, 24, 4207. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cho, J.-Y.; Hwang, T.-L.; Chang, T.-H.; Lim, Y.-P.; Sung, P.-J.; Lee, T.-H.; Chen, J.-J. New Coumarins and Anti-Inflammatory Constituents from Zanthoxylum Avicennae. Food Chem. 2012, 135, 17–23. [Google Scholar] [CrossRef]
- Feng, S.; Liu, Z.; Chen, L.; Hou, N.; Yang, T.; Wei, A. Phylogenetic Relationships among Cultivated Zanthoxylum Species in China Based on CpDNA Markers. Tree Genet. Genomes 2016, 12, 45. [Google Scholar] [CrossRef]
- Guo, J.; Tian, C. Analysis of the Current Situation and Prospects of Pepper Development and Utilization. Food Res. Dev. 2008, 167–170. [Google Scholar] [CrossRef]
- Zhang, H. Reflections on the Current Status of Pepper Harvester Research. Agric. Mech. Res. 2009, 31, 250–252. [Google Scholar]
- Zhang, Y. Gansu Pepper Harvesting Machinery Status and Countermeasures. Agric. Mach. 2011, 108–109. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, S. Design of Pepper Harvester. Mod. Agric. 2021, 8, 71–72. Available online: https://kns.cnki.net/KCMS/detail/detail.aspx?dbcode=CJFD&dbname=CJFDLAST2021&filename=XDHY202108039&v= (accessed on 11 April 2022).
- Li, X.; Du, Y.; Liu, L.; Mao, E.; Yang, F.; Wu, J.; Wang, L. Research on the Constitutive Model of Low-Damage Corn Threshing Based on DEM. Comput. Electron. Agric. 2022, 194, 106722. [Google Scholar] [CrossRef]
- Qin, K.; Xie, N.; Tang, Y.; Wong, L.; Zhang, J. Perceived Parental Attitude toward Sex Education as Predictor of Sex Knowledge Acquisition: The Mediating Role of Global Self Esteem. Curr. Psychol. 2019, 38, 84–91. [Google Scholar] [CrossRef]
- Qing, Y.; Li, Y.; Yang, Y.; Xu, L.; Ma, Z. Development and Experiments on Reel with Improved Tine Trajectory for Harvesting Oilseed Rape. Biosyst. Eng. 2021, 206, 19–31. [Google Scholar] [CrossRef]
- Salur, E.; Aslan, A.; Kuntoglu, M.; Gunes, A.; Sahin, O.S. Experimental Study and Analysis of Machinability Characteristics of Metal Matrix Composites during Drilling. Compos. Part B Eng. 2019, 166, 401–413. [Google Scholar] [CrossRef]
- Khuri, A.I.; Mukhopadhyay, S. Response Surface Methodology. Wiley Interdiscip. Rev. Comput. Stat. 2010, 2, 128–149. [Google Scholar] [CrossRef]
- Majdi, H.; Esfahani, J.A.; Mohebbi, M. Optimization of Convective Drying by Response Surface Methodology. Comput. Electron. Agric. 2019, 156, 574–584. [Google Scholar] [CrossRef]
- Karunanithy, C.; Muthukumarappan, K. Optimization of Switchgrass and Extruder Parameters for Enzymatic Hydrolysis Using Response Surface Methodology. Ind. Crops Prod. 2011, 33, 188–199. [Google Scholar] [CrossRef]
- Yang, W.; Zhao, W.; Liu, Y.; Chen, Y.; Yang, J. Simulation of Forces Acting on the Cutter Blade Surfaces and Root System of Sugarcane Using FEM and SPH Coupled Method. Comput. Electron. Agric. 2021, 180, 105893. [Google Scholar] [CrossRef]
- Aslan, A. Optimization and Analysis of Process Parameters for Flank Wear, Cutting Forces and Vibration in Turning of AISI 5140: A Comprehensive Study. Measurement 2020, 163, 107959. [Google Scholar] [CrossRef]
- Chattopadhyay, P.S.; Pandey, K.P. Mechanical Properties of Sorghum Stalk in Relation to Quasi-Static Deformation. J. Agric. Eng. Res. 1999, 73, 199–206. [Google Scholar] [CrossRef]
- Kuntoğlu, M.; Aslan, A.; Pimenov, D.Y.; Giasin, K.; Mikolajczyk, T.; Sharma, S. Modeling of Cutting Parameters and Tool Geometry for Multi-Criteria Optimization of Surface Roughness and Vibration via Response Surface Methodology in Turning of AISI 5140 Steel. Materials 2020, 13, 4242. [Google Scholar] [CrossRef] [PubMed]
- Kuntoğlu, M.; Aslan, A.; Pimenov, D.Y.; Usca, Ü.A.; Salur, E.; Gupta, M.K.; Mikolajczyk, T.; Giasin, K.; Kapłonek, W.; Sharma, S. A Review of Indirect Tool Condition Monitoring Systems and Decision-Making Methods in Turning: Critical Analysis and Trends. Sensors 2020, 21, 108. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Li, P.; Yang, H.; Liu, J. Internal Mechanical Damage Prediction in Tomato Compression Using Multiscale Finite Element Models. J. Food Eng. 2013, 116, 639–647. [Google Scholar] [CrossRef]
- Stopa, R.; Komarnicki, P.; Kuta, Ł.; Szyjewicz, D.; Słupska, M. Modeling with the Finite Element Method the Influence of Shaped Elements of Loading Components on the Surface Pressure Distribution of Carrot Roots. Comput. Electron. Agric. 2019, 167, 105046. [Google Scholar] [CrossRef]
- Yousefi, S.; Farsi, H.; Kheiralipour, K. Drop Test of Pear Fruit: Experimental Measurement and Finite Element Modelling. Biosyst. Eng. 2016, 147, 17–25. [Google Scholar] [CrossRef]
- Zhang, G.; Zhang, Z.; Xiao, M.; Bartos, P.; Bohata, A. Soil-Cutting Simulation and Parameter Optimization of Rotary Blade’s Three-Axis Resistances by Response Surface Method. Comput. Electron. Agric. 2019, 164, 104902. [Google Scholar] [CrossRef]
- Qiu, M.; Meng, Y.; Li, Y.; Shen, X. Sugarcane Stem Cut Quality Investigated by Finite Element Simulation and Experiment. Biosyst. Eng. 2021, 206, 135–149. [Google Scholar] [CrossRef]
- Umbrello, D.; Filice, L.; Rizzuti, S.; Micari, F.; Settineri, L. On the Effectiveness of Finite Element Simulation of Orthogonal Cutting with Particular Reference to Temperature Prediction. J. Mater. Process. Technol. 2007, 189, 284–291. [Google Scholar] [CrossRef]
- Wang, S.; Hu, Z.; Yao, L.; Peng, B.; Wang, B.; Wang, Y. Simulation and Parameter Optimisation of Pickup Device for Full-Feed Peanut Combine Harvester. Comput. Electron. Agric. 2022, 192, 106602. [Google Scholar] [CrossRef]
- Xie, L.; Wang, J.; Cheng, S.; Zeng, B.; Yang, Z. Optimisation and Finite Element Simulation of the Chopping Process for Chopper Sugarcane Harvesting. Biosyst. Eng. 2018, 175, 16–26. [Google Scholar] [CrossRef]
- Zhang, J.; Xie, C.; Cao, L.; Zhou, H.; Li, C.; Wang, L. Determination of Physical and Interaction Parameters of Green Pepper (Zanthoxylum armatum): Investigation of the Mechanism of Significant Factors against the Repose Angle. LWT 2022, 162, 113409. [Google Scholar] [CrossRef]
- Desai, C.S.; Gallagher, R.H. (Eds.) Mechanics of Engineering Materials; Wiley Series in Numerical Methods in Engineering; Wiley: Chichester, UK; New York, NY, USA, 1984. [Google Scholar]
- Zhuang, Z. ABAQUS-Based Finite Element Analysis and Applications; Tsinghua University Press: Beijing, China, 2009. [Google Scholar]
- Adajar, J.B.; Alfaro, M.; Chen, Y.; Zeng, Z. Calibration of Discrete Element Parameters of Crop Residues and Their Interfaces with Soil. Comput. Electron. Agric. 2021, 188, 106349. [Google Scholar] [CrossRef]
- Xu, X.; Yan, J.; Wei, H.; Bao, G.; Ji, L.; Xie, H. Determination of Static Friction Coefficient of Peanut Pods at Different Moisture Contents. J. Chin. Agric. Mech. 2022, 43, 93–97. [Google Scholar] [CrossRef]
- Agricultural Machinery Design Manual. The Next Volume. Agricultural Machinery Design Manual; China Agricultural Science and Technology Press: Beijing, China, 2007. [Google Scholar]
- Pang, S. About Slip-Cut Theory and the Choice of Slip-Cut Angle. J. Huazhong Agric. Coll. 1982, 64–69. [Google Scholar] [CrossRef]
- Hu, K. Analysis of Slip-Cutting Angle of Working Parts of Agricultural Machinery. J. Southwest Agric. Coll. 1984, 62–66. [Google Scholar]
- Yang, W.; Yang, J.; Liu, Z.; Liang, Z.; Mo, J. Dynamic Simulation Experiment on Effects of Sugarcane Cutting beneath Surface Soil. Trans. Chin. Soc. Agric. Eng. 2011, 27, 150–156. [Google Scholar]


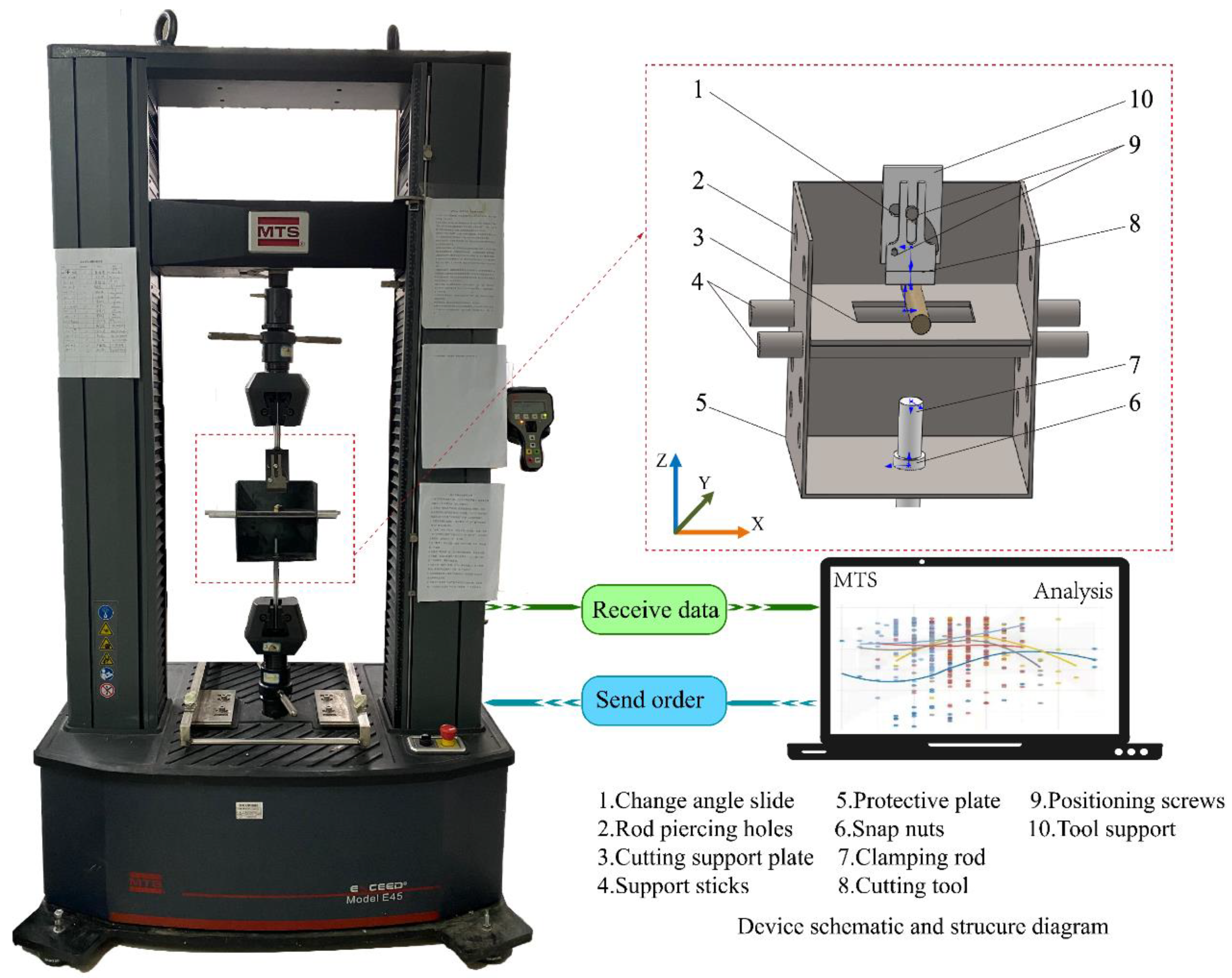


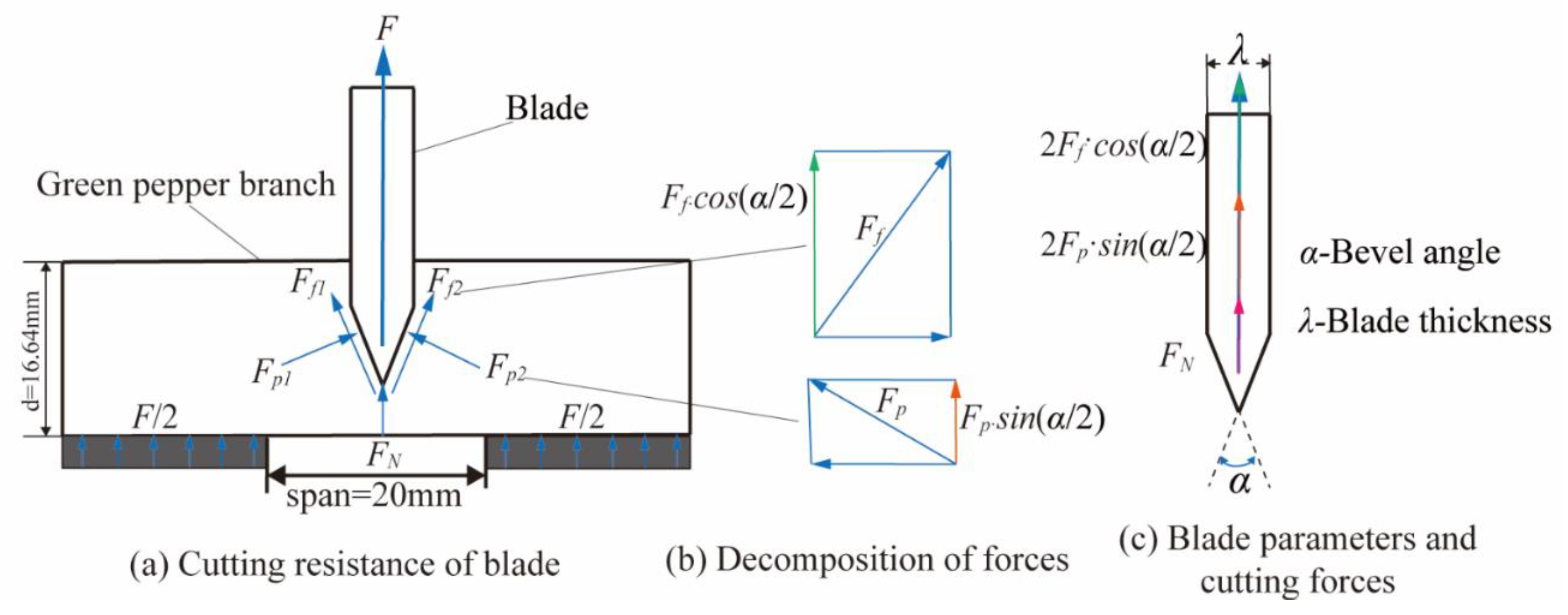
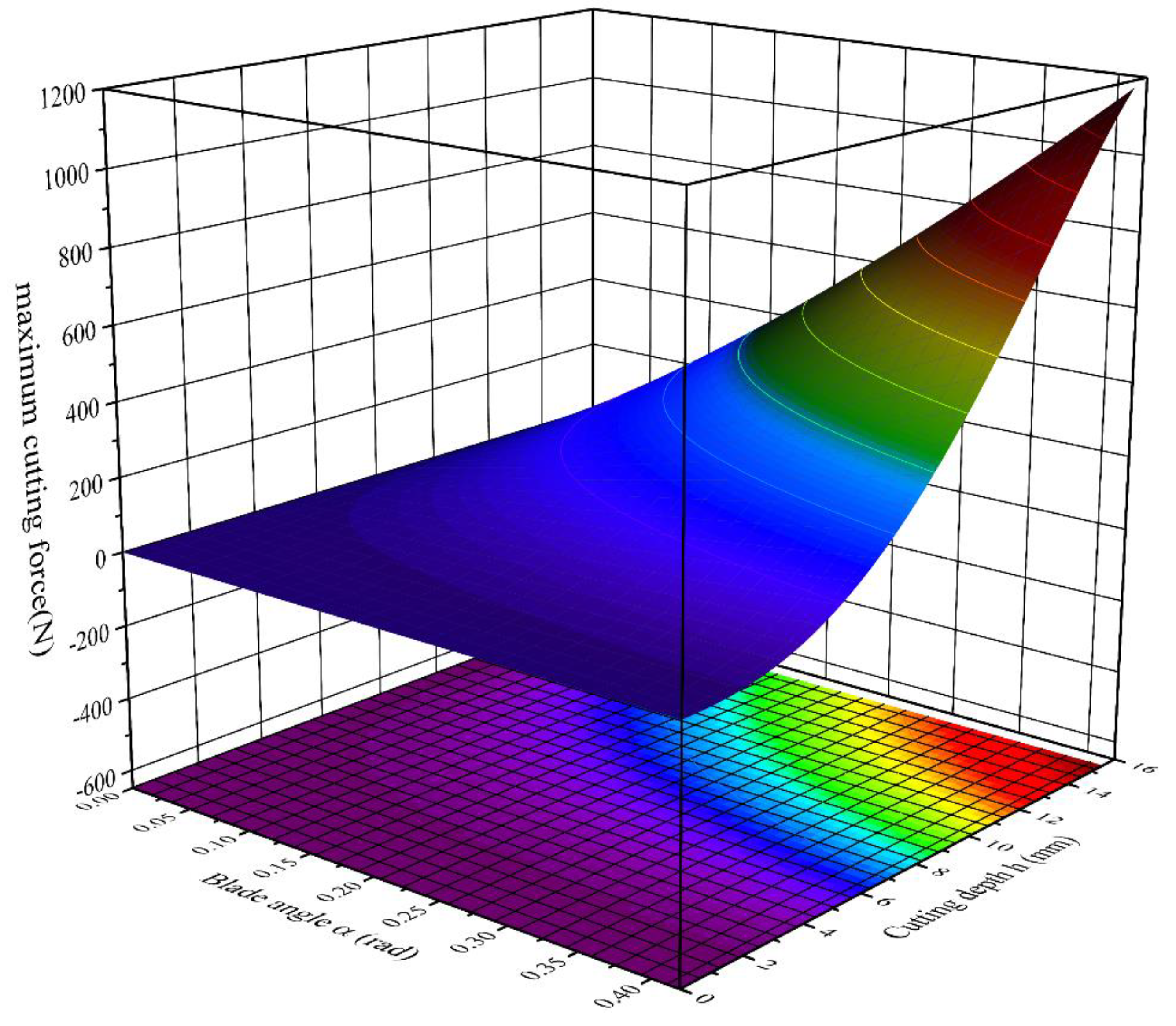
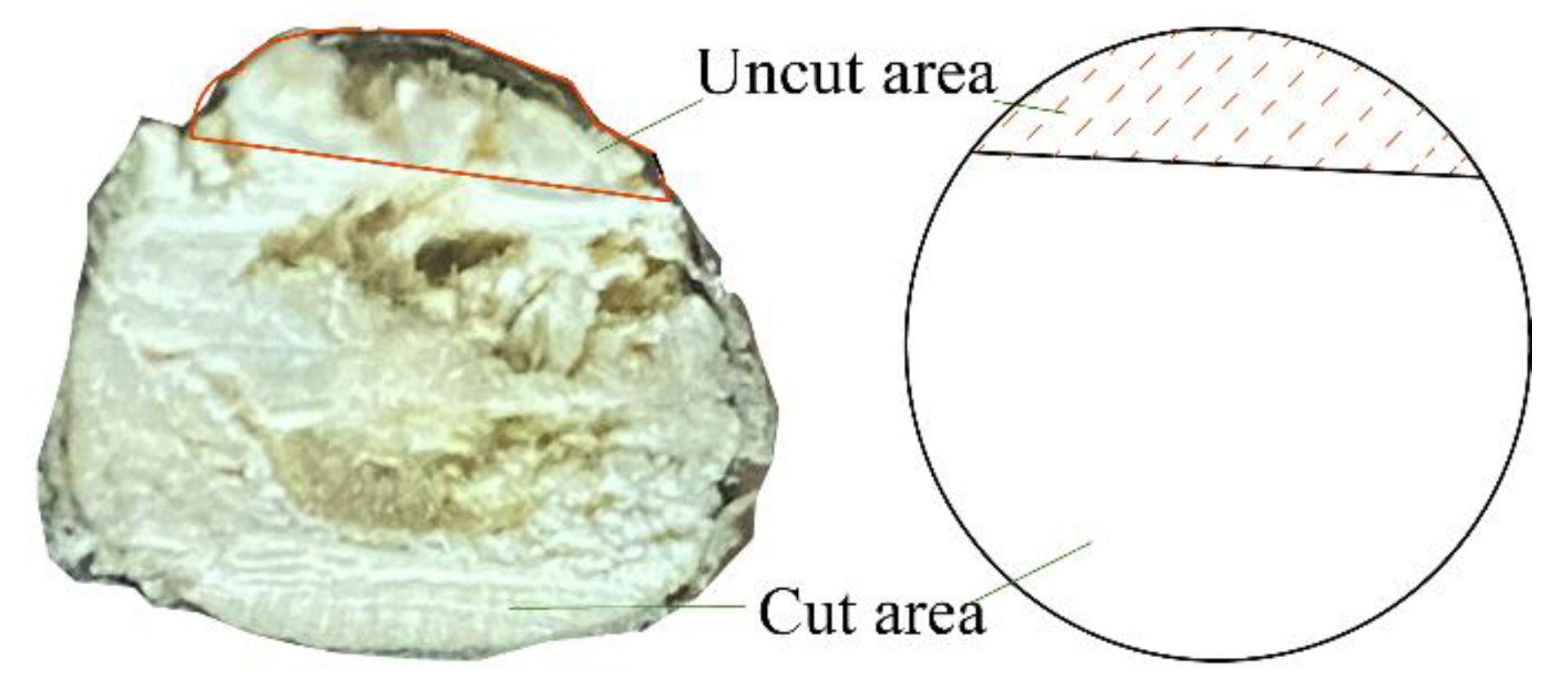

| Parameter | Symbol | Value |
|---|---|---|
| Longitudinal elastic modulus (MPa) | 288.67 | |
| Tangential(radial) elastic molus (MPa) | , | 26.14 |
| Longitudinal shear modulus (MPa) | 107.552 | |
| Tangential(radial) shear modulus (MPa) | , | 9.834 |
| Longitudinal Poisson’s ratio | 0.342 | |
| Tangential(radial) Poisson’s ratio | 0.329 | |
| Density (g·cm−3) | 0.88 | |
| Longitudinal tensile limit (MPa) | XT | 14.131 |
| Longitudinal compression limit (MPa) | XC | 12.91 |
| Tangential (radial) tensile limit (MPa) | YT, ZT | 8.57 |
| Tangential (radial) Compression limit (MPa) | YC, ZC | 11.835 |
| Moisture content (%) | W | 68.636 |
| Parameter | Symbol | Value |
|---|---|---|
| Elastic modulus (MPa) | 2.1 × 105 | |
| Density (g·cm−3) | 7.85 | |
| Tensile strength (MPa) | ≥1030 | |
| Yield strength (MPa) | ≥835 | |
| Poisson’ sratio | 0.31 |
| Edge Angle | 16 | 20 | 24 |
|---|---|---|---|
| Simulation data (N) | 881.93 | 1017.85 | 1256.39 |
| Test data (N) | 841.42 | 969.15 | 1207.95 |
| Error rate (%) | 4.59 | 4.78 | 3.86 |
| Symbol | Parameters | −1 | 0 | 1 | ∆j |
|---|---|---|---|---|---|
| X1 | Cutting Angle/° | 60 | 75 | 90 | 15 |
| X2 | Load rate | 5 | 10 | 15 | 5 |
| X3 | Edge angle/° | 16 | 20 | 24 | 6 |
| Factors | Responses | ||||
|---|---|---|---|---|---|
| Test Group | X1 (°) | X2 (°) | X3 (mm·s−1) | Y1 (N) | Y2 (%) |
| 1 | 24 (1) | 60 (−1) | 10 (0) | 1148.71 | 83.1 |
| 2 | 16 (−1) | 75 (0) | 5 (−1) | 801.12 | 98.3 |
| 3 | 20 (0) | 75 (0) | 10 (0) | 909.62 | 87.2 |
| 4 | 24 (1) | 75 (0) | 5 (−1) | 1157.71 | 87.3 |
| 5 | 20 (0) | 75 (0) | 10 (0) | 920.32 | 86.1 |
| 6 | 20 (0) | 90 (1) | 15 (1) | 984.49 | 75.8 |
| 7 | 20 (0) | 90 (1) | 5 (−1) | 1017.48 | 91.5 |
| 8 | 16 (−1) | 75 (0) | 15 (1) | 765.0 | 83.5 |
| 9 | 20 (0) | 75 (0) | 10 (0) | 921.36 | 86.9 |
| 10 | 20 (0) | 60 (−1) | 5 (−1) | 981.69 | 96.5 |
| 11 | 16 (−1) | 90 (1) | 10 (0) | 869.78 | 88.5 |
| 12 | 24 (1) | 75 (0) | 15 (1) | 1129.62 | 70.2 |
| 13 | 20 (0) | 75 (0) | 10 (0) | 946.93 | 85.8 |
| 14 | 24 (1) | 90 (1) | 10 (0) | 1236.30 | 79.6 |
| 15 | 20 (0) | 60 (−1) | 15 (1) | 949.63 | 80.5 |
| 16 | 20 (0) | 75 (0) | 10 (0) | 899.32 | 84.2 |
| 17 | 16 (−1) | 60 (−1) | 10 (0) | 820.59 | 97.1 |
| Source | Sum of Squares | Degree of Freedom | Mean Square | F Value | p-Value | |
|---|---|---|---|---|---|---|
| Y1 | ||||||
| Model | 281,352.5 | 9 | 31261.39 | 115.5878 | <0.0001 ** | significant |
| X1-Edge angle | 250,402 | 1 | 250,402 | 925.8517 | <0.0001 ** | |
| X2-Cutting angle | 5378.401 | 1 | 5378.401 | 19.88643 | 0.0029 * | |
| X3-Tool feed speed | 2072.392 | 1 | 2072.392 | 7.662591 | 0.0278 * | |
| X1X2 | 368.64 | 1 | 368.64 | 1.363032 | 0.2812 | |
| X1X3 | 14.17523 | 1 | 14.17523 | 0.052412 | 0.8255 | |
| X2X3 | 0.216225 | 1 | 0.216225 | 0.000799 | 0.9782 | |
| X12 | 6652.861 | 1 | 6652.861 | 24.5987 | 0.0016 * | |
| X22 | 14,948.89 | 1 | 14,948.89 | 55.27293 | 0.0001 ** | |
| X32 | 75.24594 | 1 | 75.24594 | 0.278219 | 0.6142 | |
| Residual | 1893.191 | 7 | 270.4558 | |||
| Lack of Fit | 631.8272 | 3 | 210.6091 | 0.667877 | 0.6145 | not significant |
| Pure Error | 1261.363 | 4 | 315.3409 | |||
| Cor Total | 283,245.7 | 16 | ||||
| R2 = 98.4%; C.V. = 1.7 | ||||||
| Y2 | ||||||
| Model | 861.53 | 9 | 95.73 | 103.06 | <0.0001 ** | significant |
| X1-Edge angle | 278.48 | 1 | 278.48 | 299.81 | <0.0001 ** | |
| X2-Cutting angle | 59.40 | 1 | 59.40 | 63.95 | <0.0001 ** | |
| X3-Tool feed speed | 505.62 | 1 | 505.62 | 544.35 | <0.0001 ** | |
| X1X2 | 6.50 | 1 | 6.50 | 7.00 | 0.0331 * | |
| X1X3 | 1.32 | 1 | 1.32 | 1.42 | 0.2716 | |
| X2X3 | 0.023 | 1 | 0.023 | 0.024 | 0.8807 | |
| X12 | 0.049 | 1 | 0.049 | 0.052 | 0.8255 | |
| X22 | 5.50 | 1 | 5.50 | 5.92 | 0.0453 * | |
| X32 | 5.16 | 1 | 5.16 | 5.56 | 0.0505 * | |
| Residual | 6.50 | 7 | 0.93 | |||
| Lack of Fit | 0.97 | 3 | 0.32 | 0.23 | 0.8688 | not significant |
| Pure Error | 5.53 | 4 | 1.38 | |||
| Cor Total | 868.03 | 16 | ||||
| R2 = 98.3%; C.V. = 1.12 | ||||||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Y.; Li, B.; Jiang, Y.; Xu, C.; Zhou, B.; Niu, Q.; Li, C. Study on the Dynamic Cutting Mechanism of Green Pepper (Zanthoxylum armatum) Branches under Optimal Tool Parameters. Agriculture 2022, 12, 1165. https://doi.org/10.3390/agriculture12081165
Li Y, Li B, Jiang Y, Xu C, Zhou B, Niu Q, Li C. Study on the Dynamic Cutting Mechanism of Green Pepper (Zanthoxylum armatum) Branches under Optimal Tool Parameters. Agriculture. 2022; 12(8):1165. https://doi.org/10.3390/agriculture12081165
Chicago/Turabian StyleLi, Yexin, Binjie Li, Yiyao Jiang, Chengrui Xu, Baidong Zhou, Qi Niu, and Chengsong Li. 2022. "Study on the Dynamic Cutting Mechanism of Green Pepper (Zanthoxylum armatum) Branches under Optimal Tool Parameters" Agriculture 12, no. 8: 1165. https://doi.org/10.3390/agriculture12081165
APA StyleLi, Y., Li, B., Jiang, Y., Xu, C., Zhou, B., Niu, Q., & Li, C. (2022). Study on the Dynamic Cutting Mechanism of Green Pepper (Zanthoxylum armatum) Branches under Optimal Tool Parameters. Agriculture, 12(8), 1165. https://doi.org/10.3390/agriculture12081165






