Research on the Contact Pressure Calculation Method for the Misaligned Elastomeric Journal Bearing
Abstract
:1. Introduction
2. Adopted Approaches
2.1. Model of the Misaligned Bearing
2.2. The ICs Method
2.3. The Determination of the Cylindrical Influence Coefficient Factors
2.4. The Geometric Boundary Condition
2.5. Numerical Calculation Process of the C-ICs Method
2.6. The Finite Element Calculation
3. Results and Discussion
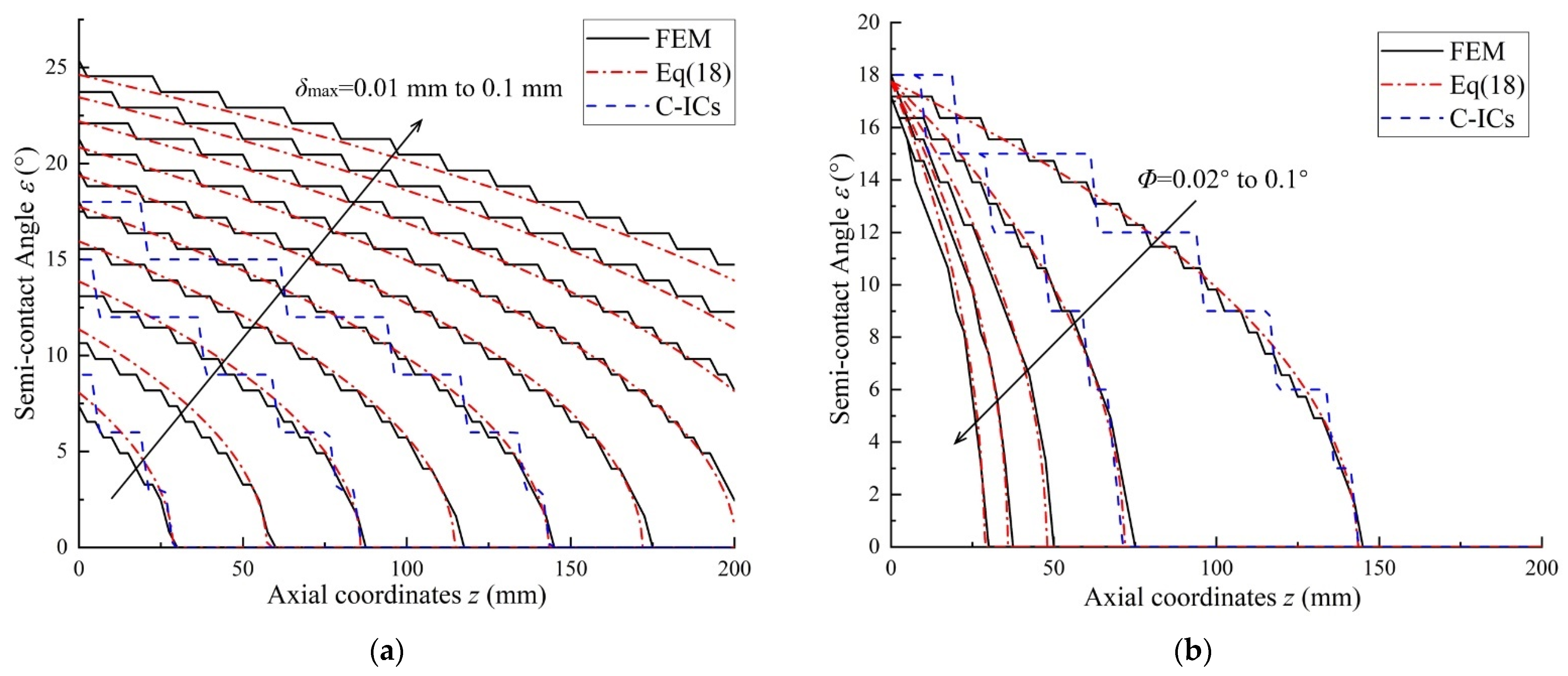
3.1. Comparison of Different Influence Coefficient Factors
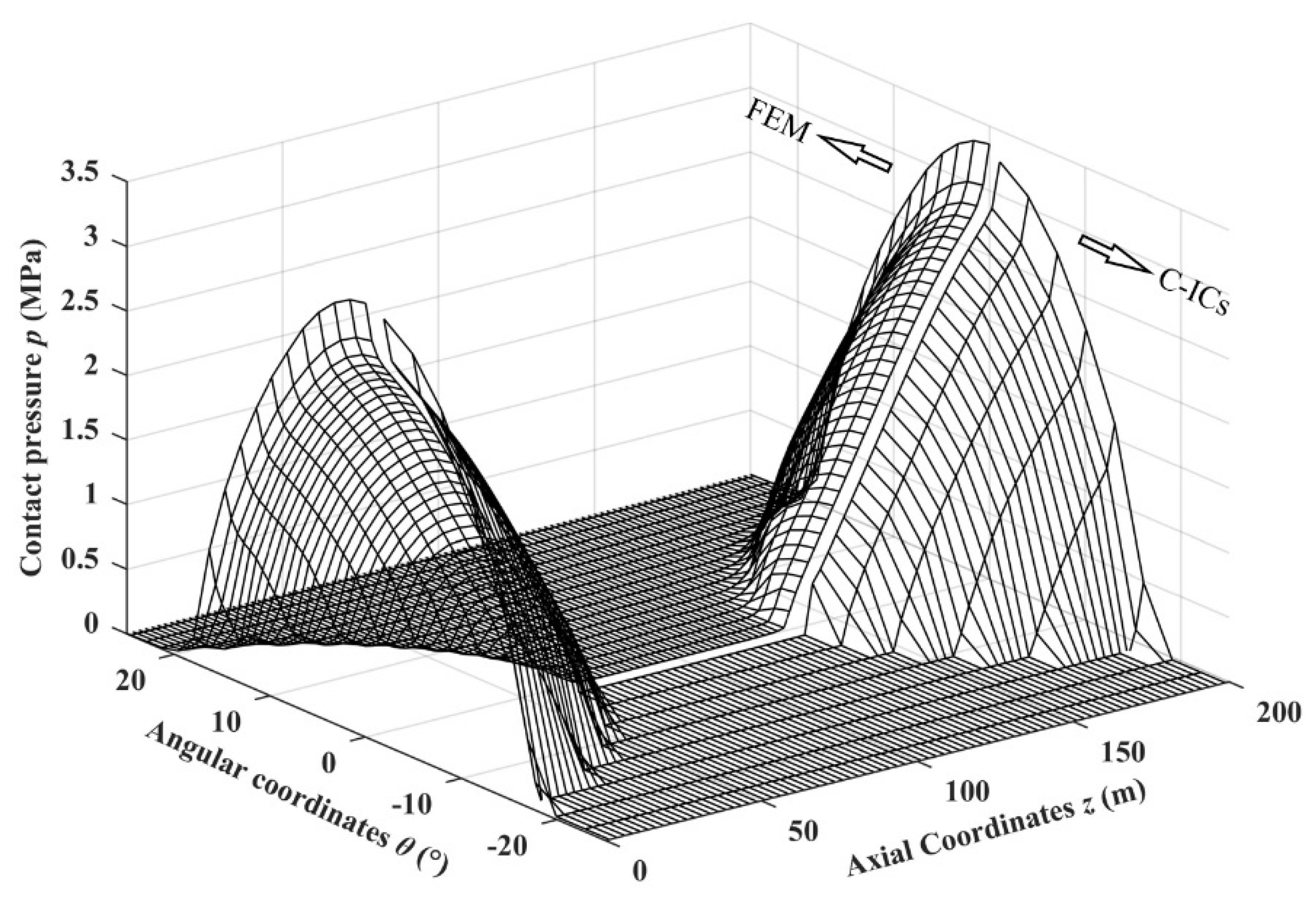
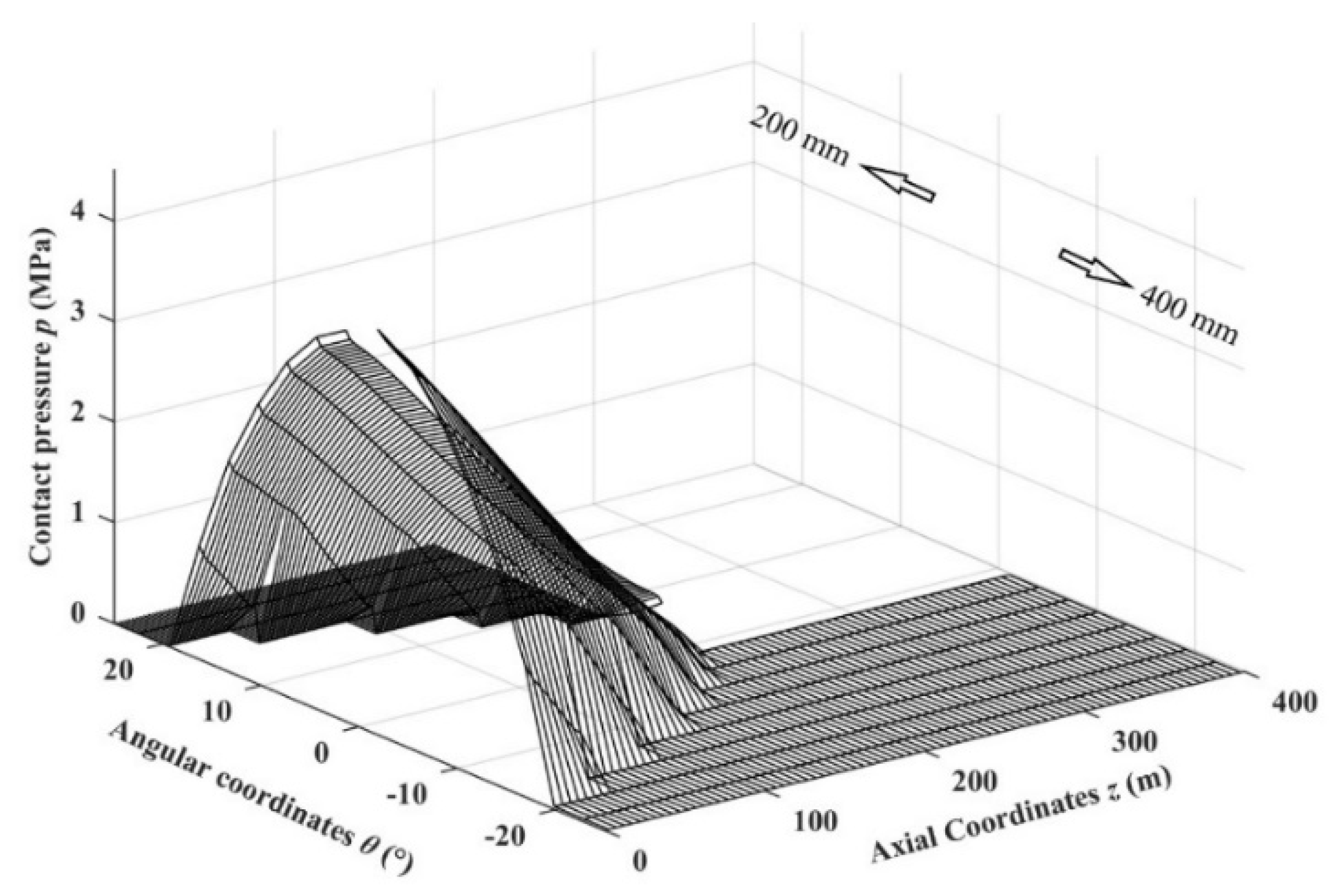
3.2. Contact Calculation of Misaligned Bearing
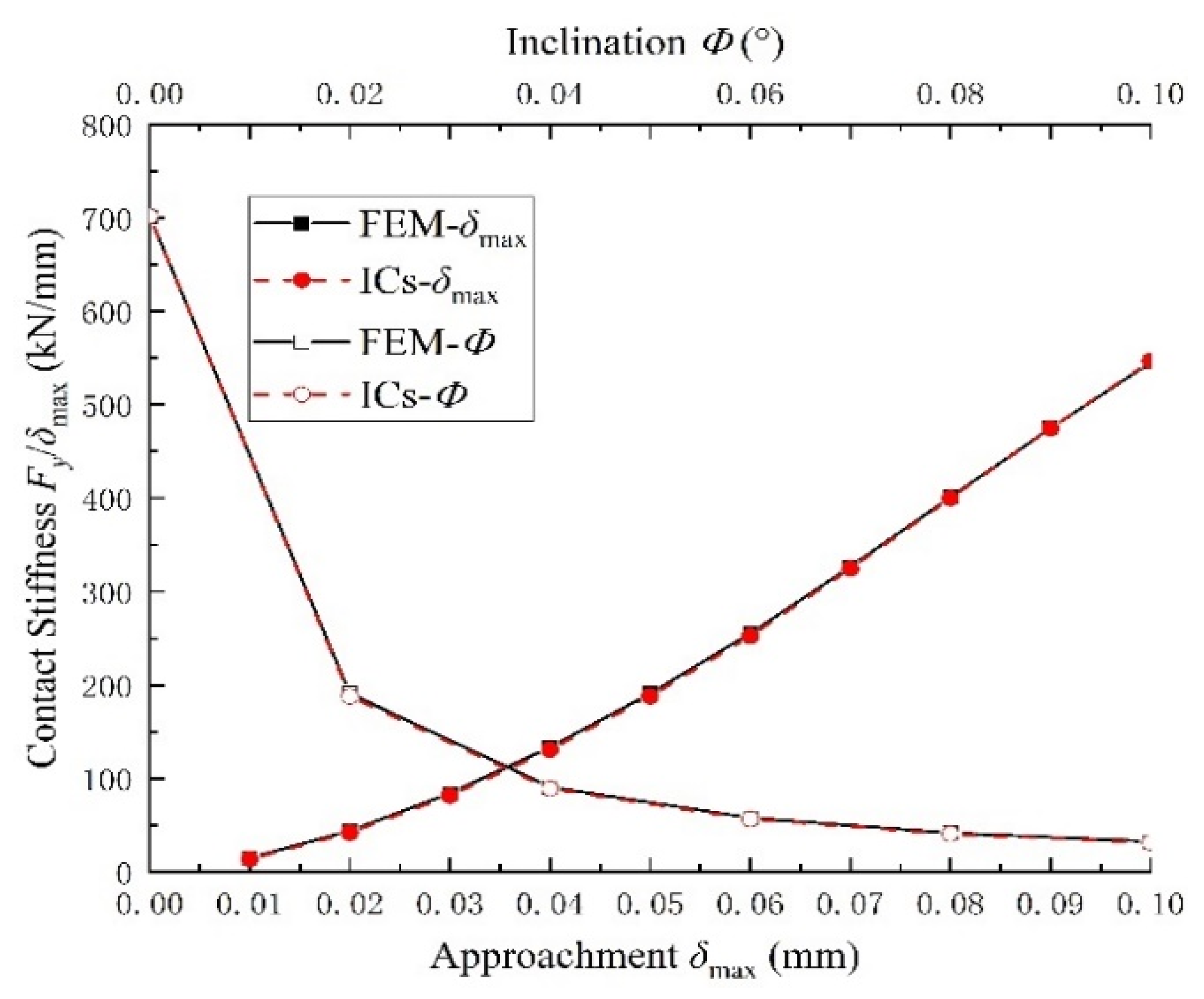
3.3. Influence of Misalignment on Journal Bearing Capacity and Contact Stiffness
4. Conclusions and Future works
- (1)
- The calculation of the cylindrical shell subjected to uniform internal pressure with different coefficient influence factors (ICs factor) obtained significantly different results, proving that the contact body’s geometric shapes and boundary conditions affect the ICs factor. The ICs factor shown in this article is suitable for cylindrical surface contact analysis;
- (2)
- The proposed method has similar accuracy in the contact pressure calculation of a misaligned elastomeric journal bearing with the FEM. Nevertheless, the time consumption is only half of the FEM and can be further reduced to 1/30th by storing the ICs factor in advance;
- (3)
- The bearing misalignment influences the load-carrying capacity and the contact stiffness. The key to improving the load-carrying capacity is to increase the effective contact area. Additionally, the contact stiffness has the same changing trend as the contact area.
- (1)
- The theoretical solution form of the cylindrical shell subjected to a concentrated force contains the first and second types of deformed Bessel functions, which are prone to data overflow during numerical processing and reduce accuracy. Finding other theoretical solutions with compact forms can further improve the method;
- (2)
- The non-alignment conditions of the bearings in this paper are assumed according to the rules. The work would be more meaningful if the actual propulsion shafting data could be obtained and input.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Nomenclature
| r | radial coordinate |
| r1 | the outer radius of the shaft |
| r2 | the inner radius of the bearing |
| z | axial coordinate |
| θ | circumferential coordinate |
| Φ | the inclination angle between shaft and bearing |
| δ | the approachment of the shaft presses into bearing |
| δmax | the maximum approachment δ |
| L | the length of bearing |
| α | load area dimension of circumstantial direction |
| b | load area dimension of axial direction |
| N | axial segments of the contact area |
| M | circumferential segments of the contact area |
| p | radial pressure |
| [p] | matrix form of radial pressure |
| p(z, θ) | radial pressure at (z, θ) location |
| pij | radial pressure of (i, j) grid |
| the influence coefficient in continuous field, discrete field | |
| q | loading function of concentrated force |
| m | circumferential wave numbers of concentrated load |
| β | axial wave numbers of concentrated load |
| ν | Poisson’s ratio |
| σr | direct stresses in r direction |
| τrθ τrz | radial shearing stresses normal to θ, z axes |
| uz, uθ, ur | axial, circumferential and radial displacement |
| [ur] | matrix form of radial displacement |
| [C] | matrix form of the ICs factors |
| Cm1–Cm6 | constant coefficients of elastic solution of a cylindrical tube |
| r/r0 | dimensionless radius |
| E | elastic modulus |
| G | shear modulus |
| Im(x), Km(x) | the first, and second types of m-order deformed Bessel functions at |
| Aσrm1–Aurm6 | multiplier of the constant coefficient Cm1–Cm6 in Equations (5)–(10) |
| ε | semi-contact angle |
References
- Choi, S.; Lee, J.; Park, J. Application of deep reinforcement learning to predict shaft deformation considering hull deformation of medium-sized oil/chemical tanker. J. Mar. Sci. Eng. 2021, 9, 767. [Google Scholar] [CrossRef]
- Hirani, H.; Verma, M. Tribological study of elastomeric bearings for marine propeller shaft system. Tribol. Int. 2009, 42, 378–390. [Google Scholar] [CrossRef]
- Lee, J.; Jeong, B.; An, T.H. Investigation on effective support point of single stern tube bearing for marine propulsion shaft alignment. Mar. Struct. 2019, 64, 1–17. [Google Scholar] [CrossRef] [Green Version]
- DNV GL. Incomplete propeller immersion risk of propeller shaft bearing damage. Tech. Regul. News 2017, 1, 1–3. [Google Scholar]
- ABS. Guidance Notes on ‘Propulsion Shafting Alignment’. Available online: https://ww2.eagle.org/content/dam/eagle/rules-and-guides/current/design_and_analysis/128_propulsionshaftalignment/shaft-alignment-gn-sept-19.pdf (accessed on 1 July 2021).
- CCS. Part 3 Main and Auxiliary Machinery, Chapter 12 shaft vibration and alignment. In Rules for the Construction of Domestic Sailing Sea Vessels; CCS: Beijing, China, 2018. [Google Scholar]
- Lloyd’s Register. Rules and Regulations for the Classification of Ships. Available online: https://www.lr.org/en/rules-and-regulations-for-the-classification-of-ships/ (accessed on 1 July 2021).
- Johnson, K.L. Contact Mechanics; Press Syndicate of the University of Cambridge: New York, NY, USA, 1985; pp. 114–118. [Google Scholar]
- Ciavarella, M.; Decuzzi, P. The state of stress induced by the plane frictionless cylindrical contact. I. The case of elastic similarity. Int. J. Solids Struct. 2001, 38, 4507–4523. [Google Scholar] [CrossRef]
- Ciavarella, M.; Decuzzi, P. The state of stress induced by the plane frictionless cylindrical contact. II. The general case. Int. J. Solids Struct. 2001, 38, 4525–4533. [Google Scholar] [CrossRef] [Green Version]
- Pereira, C.; Ramalho, A.; Ambrosio, J. Applicability domain of internal cylindrical contact force models. Mech. Mach. Theory 2014, 78, 141–157. [Google Scholar] [CrossRef]
- Steven, G.P. A non-axisymmetric cylindrical contact problem. Int. J. Eng. Sci. 1977, 15, 95–103. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, K.; Yang, L. The compliance contact model of cylindrical joints with clearances. Acta Mech. Sin. 2005, 21, 451–458. [Google Scholar] [CrossRef]
- Liu, C.; Zhang, K.; Yang, L. The FEM analysis and approximate model for cylindrical joints with clearances. Mech. Mach. Theory 2007, 42, 183–197. [Google Scholar] [CrossRef]
- Lai, G.; Liu, J.; Liu, S.; Zeng, F.; Zhou, R.; Lei, J. Comprehensive optimization for the alignment quality and whirling vibration damping of a motor drive shafting. Ocean Eng. 2018, 157, 26–34. [Google Scholar] [CrossRef]
- Hartnett, M.J. The analysis of contact stresses in rolling element bearings. J. Lubr. Tech. 1979, 101, 105–109. [Google Scholar] [CrossRef]
- Lv, F.; Ya, N.; Rao, Z. Analysis of equivalent supporting point location and carrying capacity of misaligned journal bearing. Tribol. Int. 2017, 116, 26–38. [Google Scholar] [CrossRef]
- Gong, J.; Jin, Y.; Liu, Z.; Jiang, H.; Xiao, M. Study on influencing factors of lubrication performance of water-lubricated micro-groove bearing. Tribol. Int. 2019, 129, 390–397. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Goodier, J.N. Theory of Elasticity, 3rd ed.; McGraw-Hill: New York, NY, USA, 1970; pp. 366–368. [Google Scholar]
- Yao, J.C. Long cylindrical tube subjected to two diametrically opposite loads. Aeronaut. Q. 1969, 20, 365–381. [Google Scholar] [CrossRef]
- Shang, X.; Qiu, F.; Zhao, H.; Li, W. Ansys Advanced Structural Finite Element Analysis Methods and Sample Applications, 1st ed.; China WaterPower Press: Beijing, China, 2006; pp. 180–224. [Google Scholar]
- Madenci, E.; Guven, I. The Finite Element Method and Applications in Engineering Using ANSYS, 2nd ed.; Springer: Boston, MA, USA, 2015; pp. 579–594. Available online: https://link.springer.com/book/10.1007/978-1-4899-7550-8 (accessed on 1 July 2021).
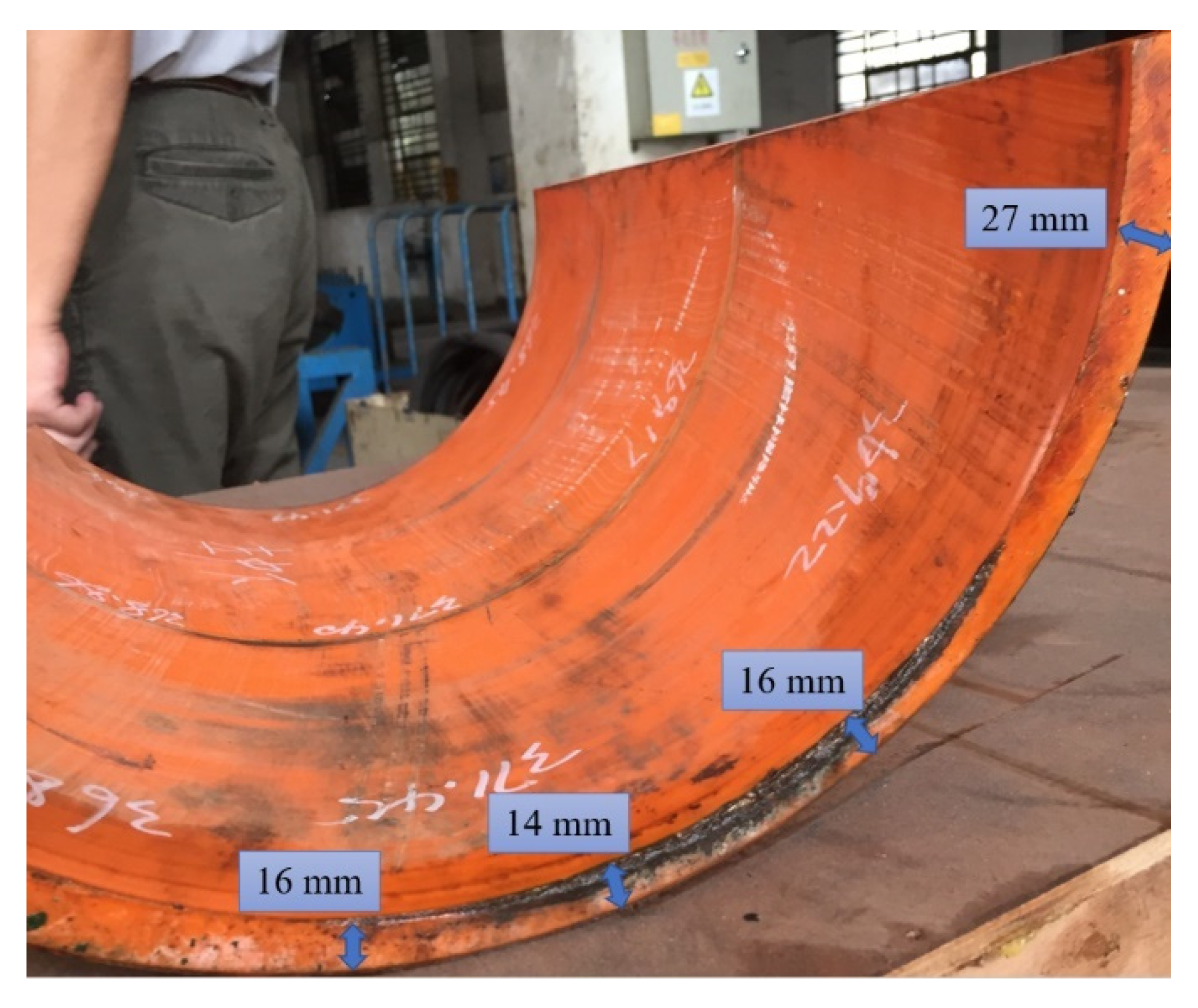


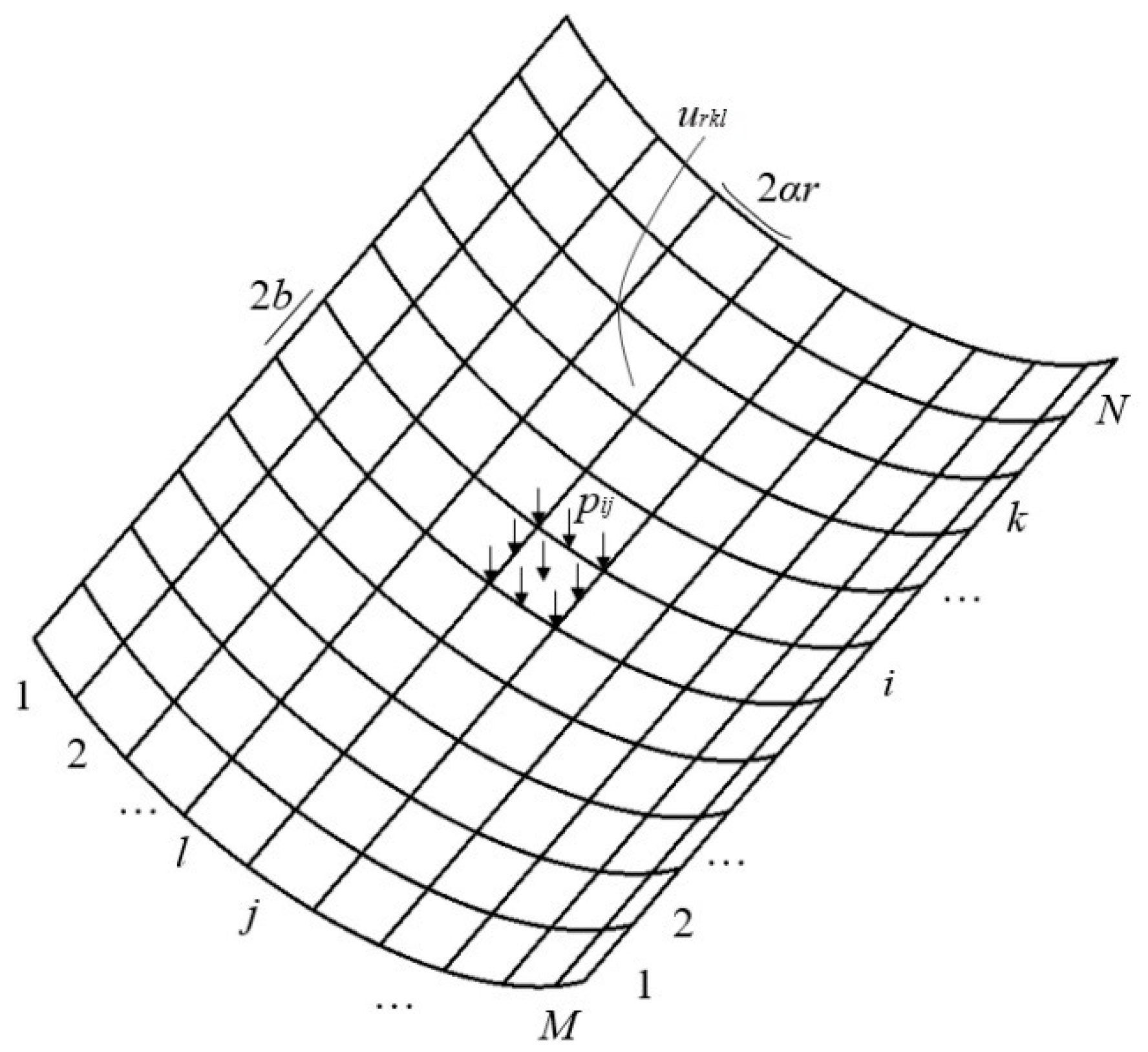
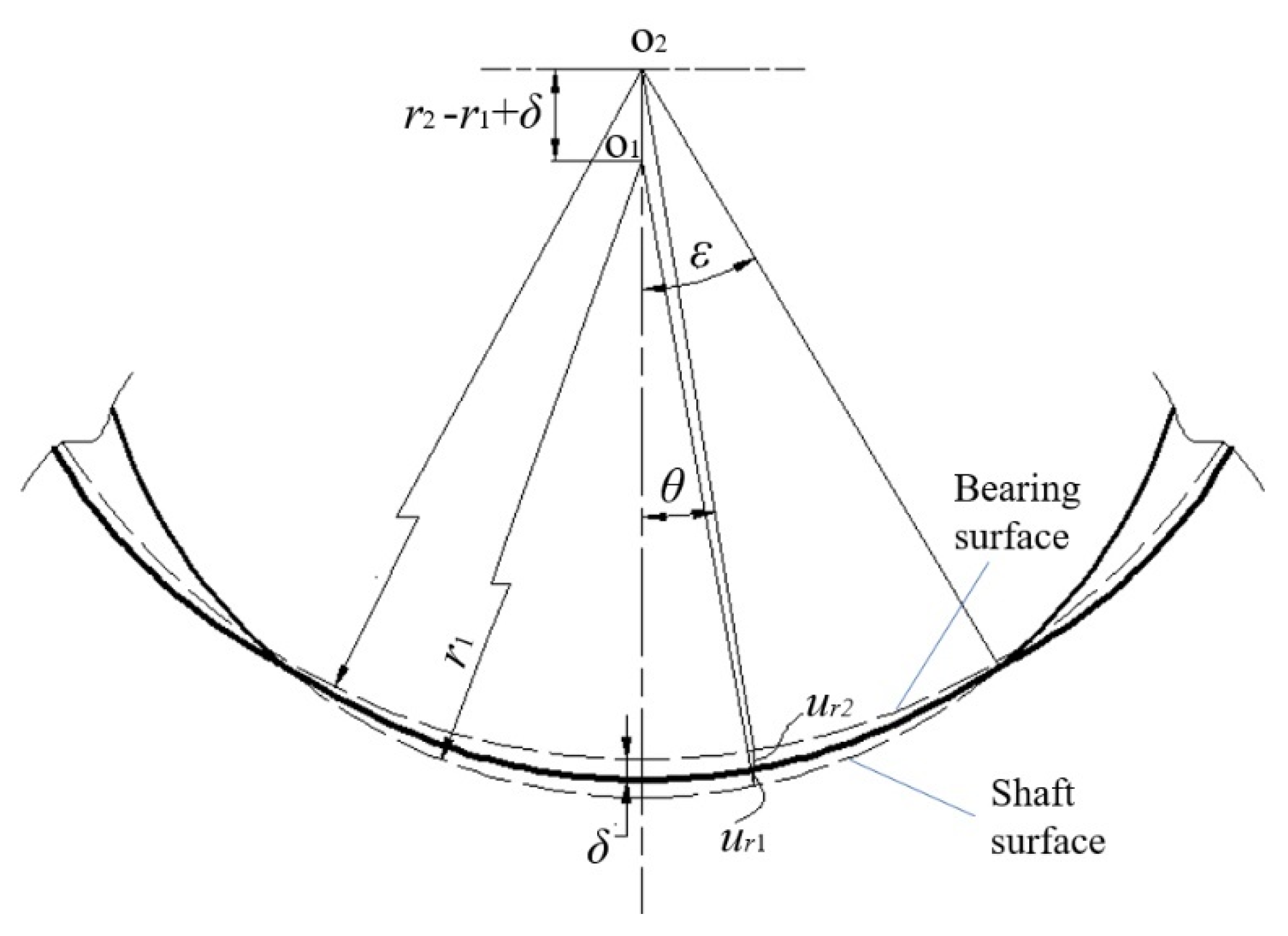







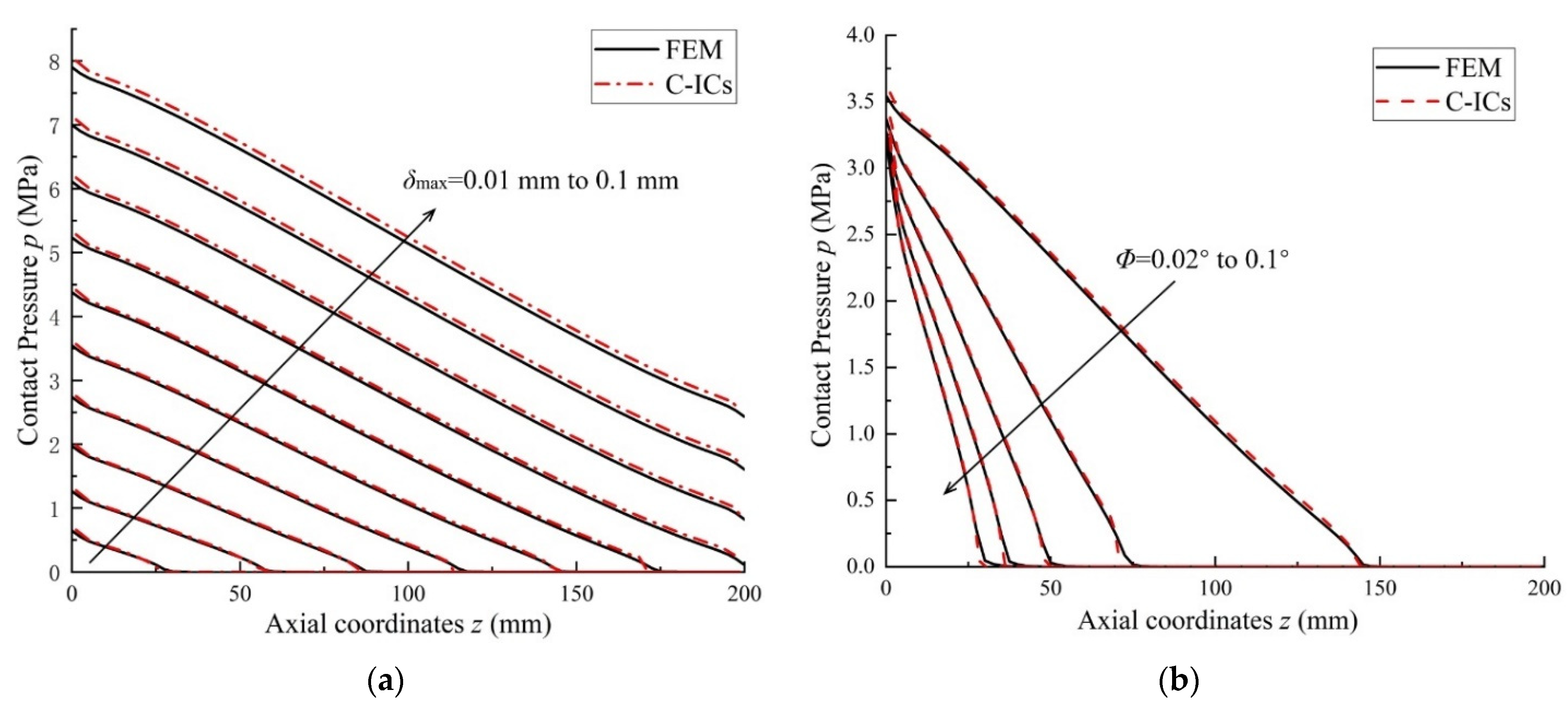
| Parameter | Shaft | Bearing |
|---|---|---|
| Out radius (mm) | 100 | 121 |
| Inner radius (mm) | 60 | 101 |
| Material | Steel | Thordon |
| Elastic modulus (MPa) | 210,000 | 605 |
| Poisson’s ratio (-) | 0.3 | 0.45 |
| Length (mm) | >200 1 | 200 |
| HS-ICs | C-ICs | Theoretical Solution (TS) | |
|---|---|---|---|
| rout = 121 mm | 0.91 mm | 0.90 × 10−2 mm | 0.92 × 10−2 mm |
| rout = 111 mm | 0.91 mm | 0.44 × 10−2 mm | 0.45 × 10−2 mm |
| rout = 131 mm | 0.91 mm | 1.39 × 10−2 mm | 1.41 × 10−2 mm |
| rout = 1001 mm | 0.91 mm | 2.16 × 10−2 mm | 2.17 × 10−2 mm |
| FEM | C-ICs | |
|---|---|---|
| Time consumption * | 17 min | 9 min |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, W.; Zhao, Y.; Yuan, H.; Liu, J.; Wang, X. Research on the Contact Pressure Calculation Method for the Misaligned Elastomeric Journal Bearing. J. Mar. Sci. Eng. 2022, 10, 141. https://doi.org/10.3390/jmse10020141
Zhou W, Zhao Y, Yuan H, Liu J, Wang X. Research on the Contact Pressure Calculation Method for the Misaligned Elastomeric Journal Bearing. Journal of Marine Science and Engineering. 2022; 10(2):141. https://doi.org/10.3390/jmse10020141
Chicago/Turabian StyleZhou, Weixin, Yao Zhao, Hua Yuan, Jingxi Liu, and Xiaoqiang Wang. 2022. "Research on the Contact Pressure Calculation Method for the Misaligned Elastomeric Journal Bearing" Journal of Marine Science and Engineering 10, no. 2: 141. https://doi.org/10.3390/jmse10020141





