Optimal Smoothing of the Wind–Wave Spectrum Based on the Confidence-Interval Trade-Off
Abstract
:1. Introduction
2. Observation Information and Water Surface Elevation Data
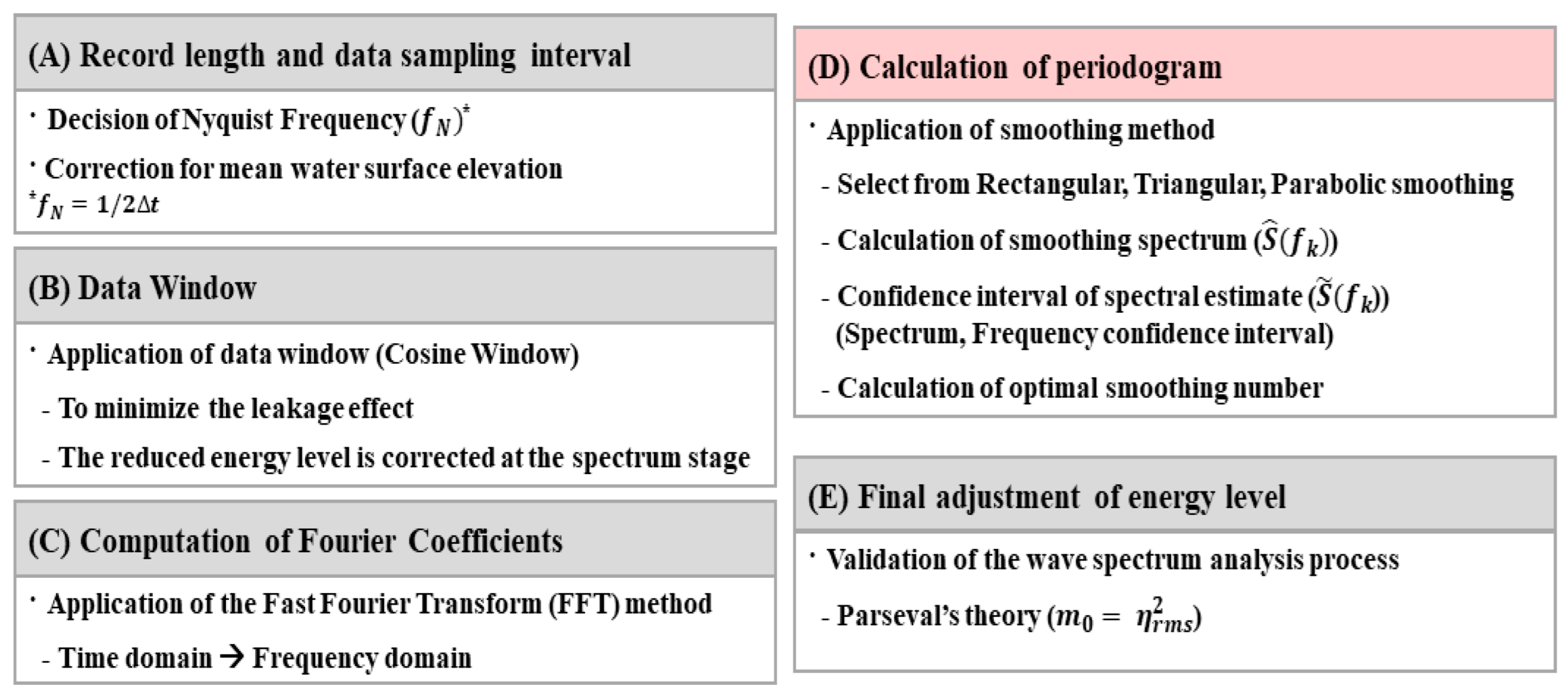
3. Method for Analysis
3.1. Methodology of Smoothing
3.2. Result Validation Method Using Parseval’s Theory
4. Results and Discussion
4.1. Validation of Wave Parameter Result
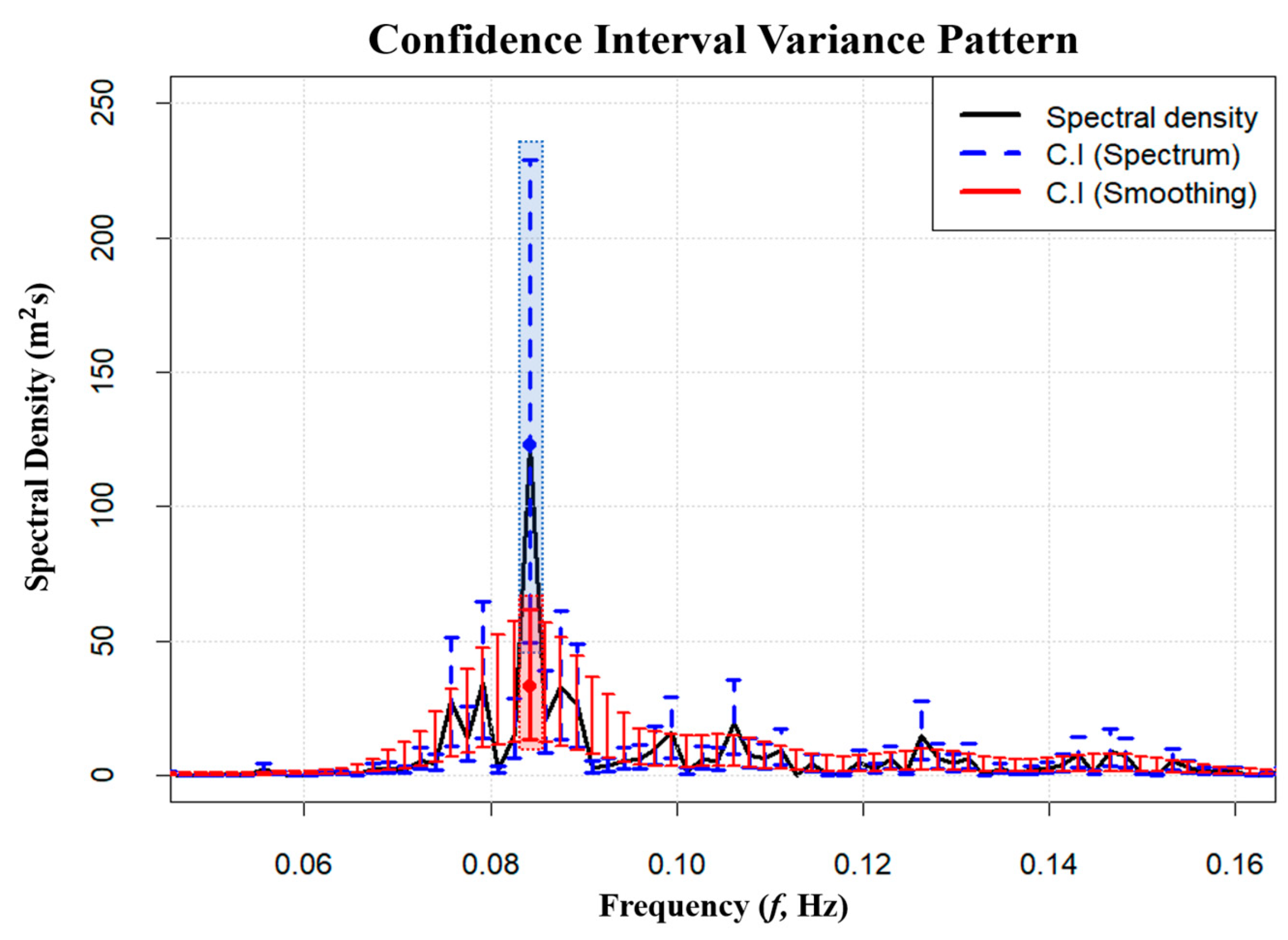
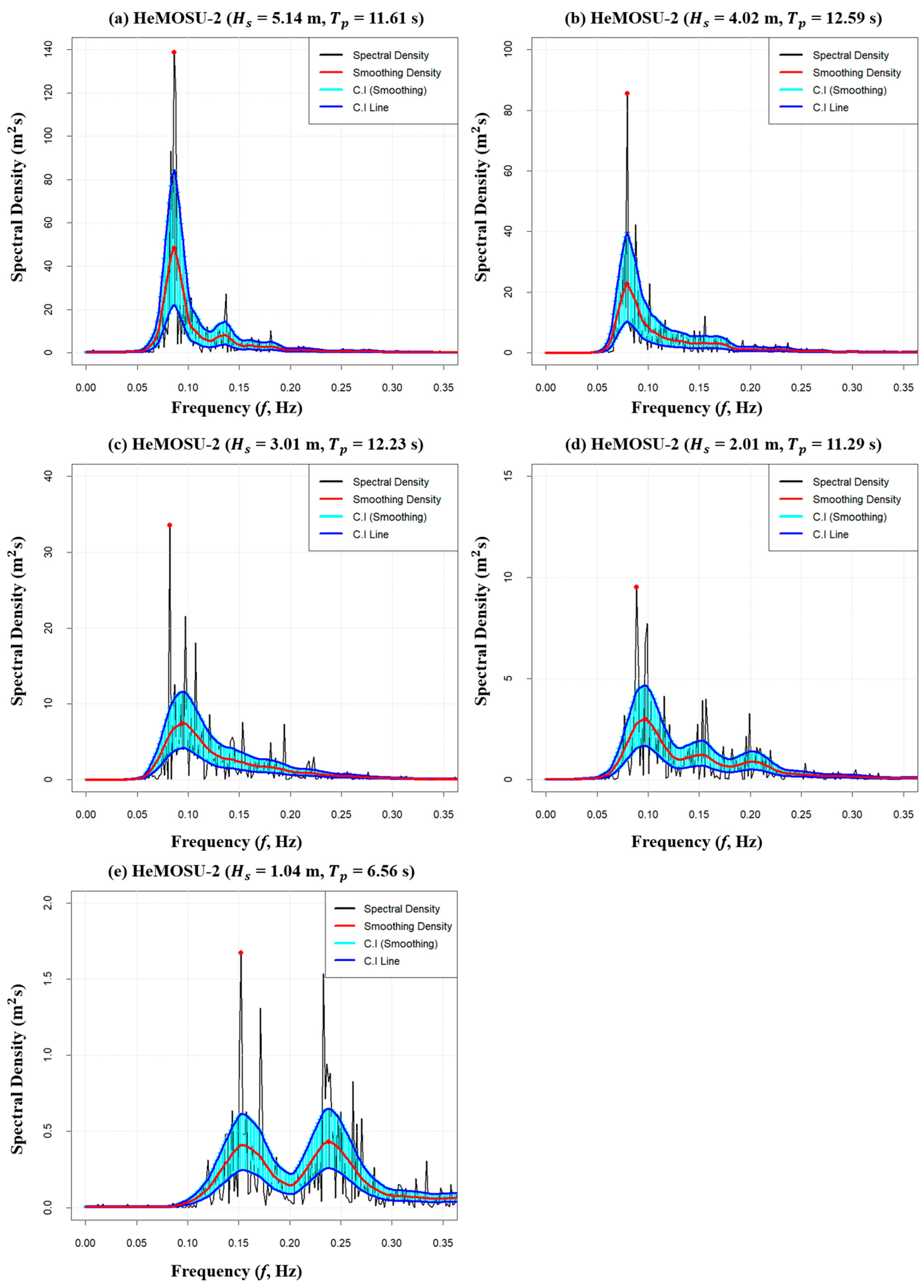
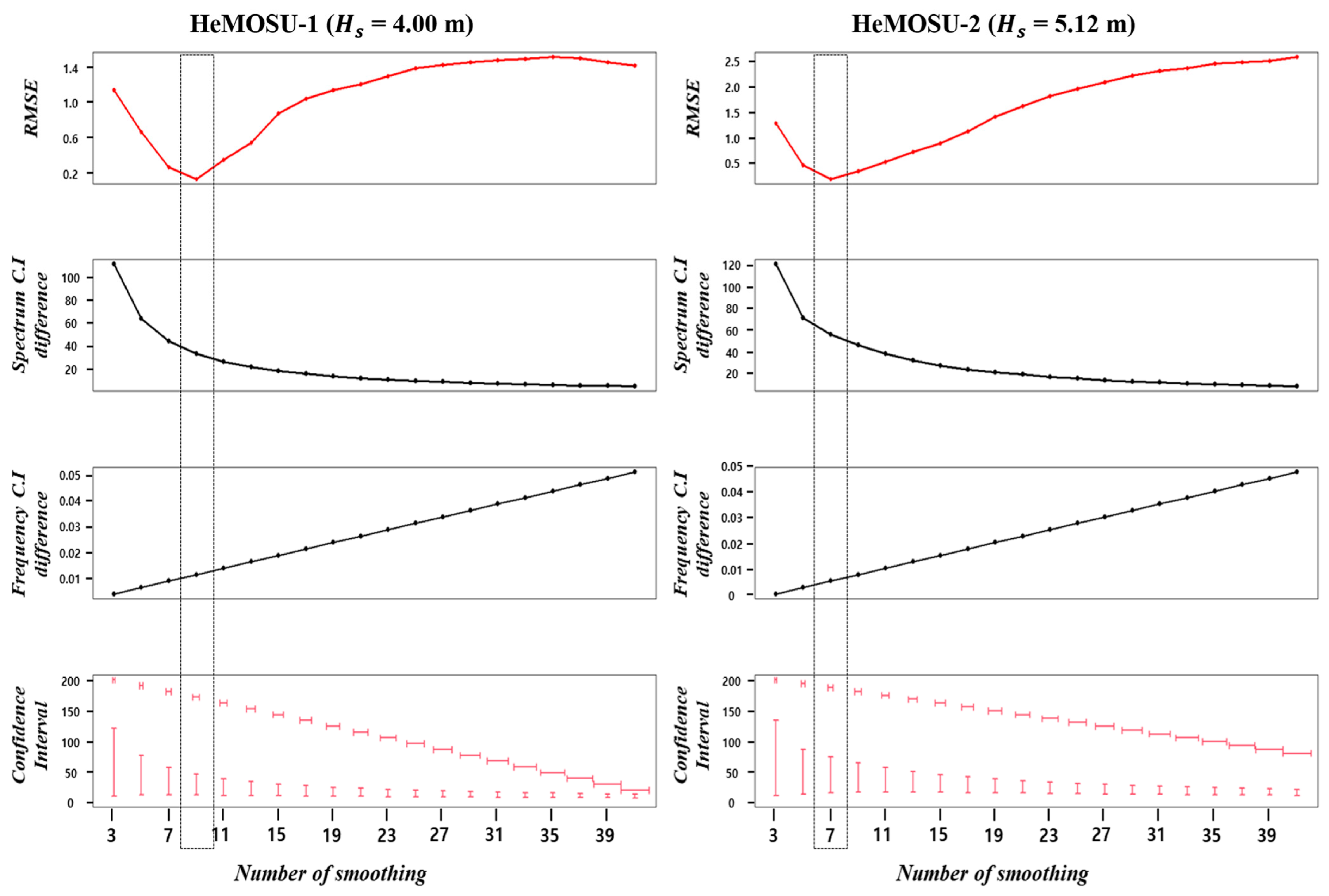
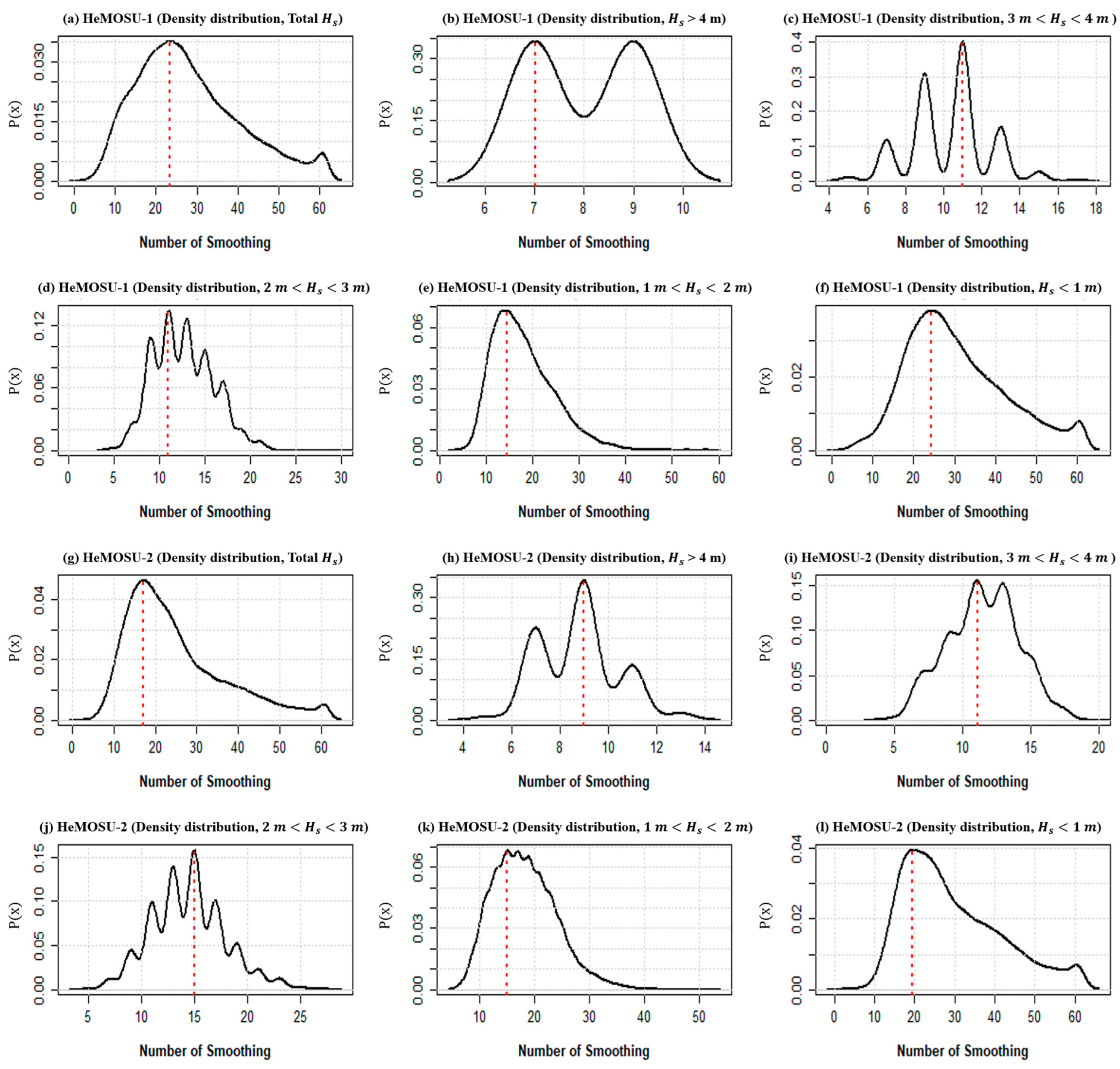
4.2. Smoothing Effect
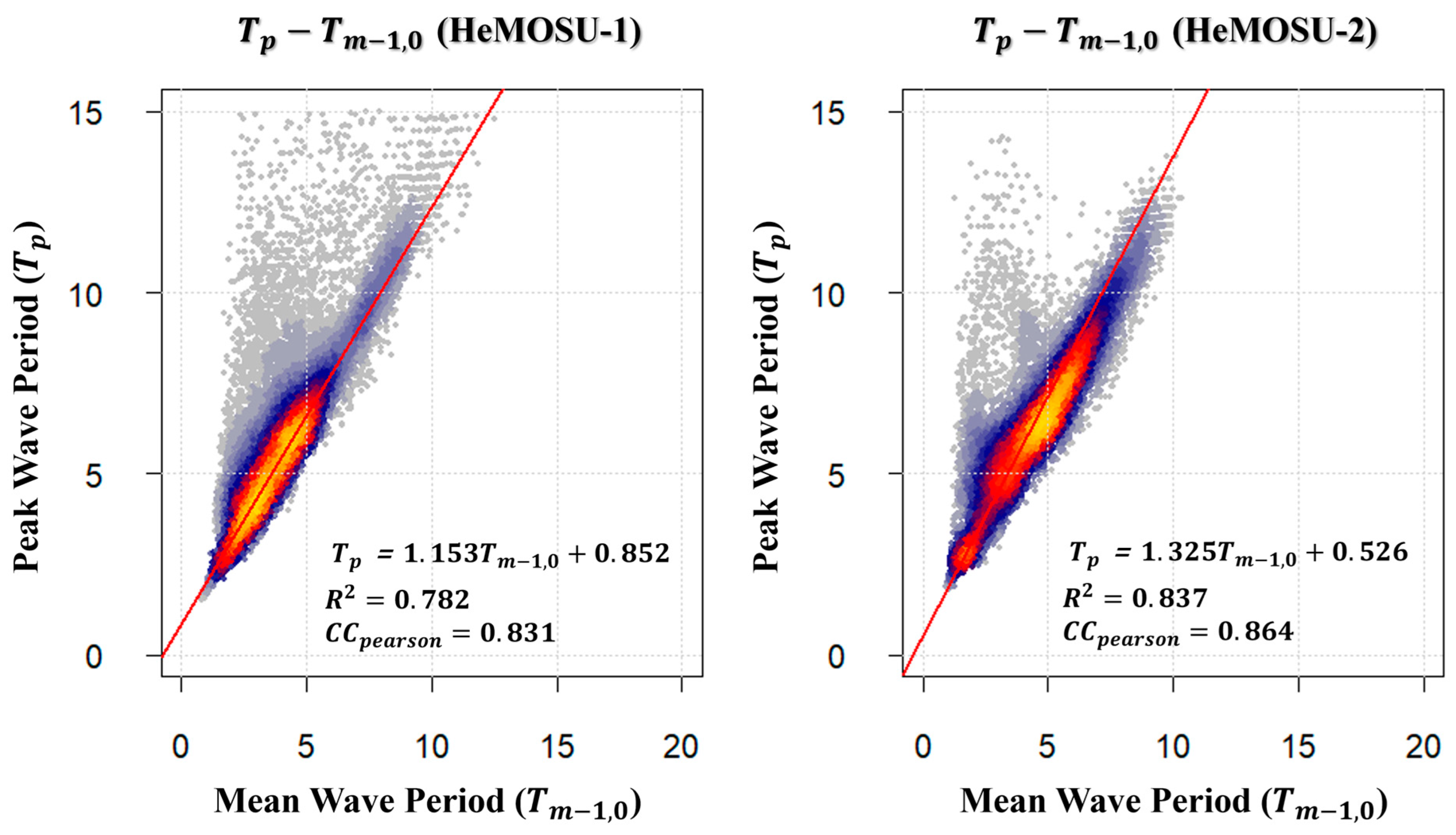
4.3. Estimation of Representative Wave Period
5. Conclusions
- To ascertain the variation pattern and suitability of the calculated peak wave period, a correlation analysis was conducted on the peak wave period derived from observation equipment and the peak period analyzed with the applied smoothing technique in this study. The computed CC (Pearson, Kendall, Spearman method) for HeMOSU-1 and HeMOSU-2 locations was more than 0.8, respectively. While the majority of peak wave periods clustered around 3–5 s, any variations in peak wave periods observed in other intervals were attributed to the impact of the applied smoothing method.
- In typical wave parameter estimation via wave spectrum analysis, verification of the analysis approach is commonly executed using Parseval’s theory, which equates the variance of waveforms to the total energy of observed data. Correlation analysis employing the two calculated variables () yielded highly robust CC of 0.9994 and 0.9991, demonstrating their statistical equivalence. This confirms the integrity of the wave spectrum analysis undertaken in this study.
- The estimated energy density of wave spectra exhibits notably broad confidence intervals. To address the uncertainty challenges within the confidence interval during peak wave period estimation via wave spectrum analysis, smoothing was implemented employing various statistical techniques. Through estimation involving the 95% chi-square distribution, post-smoothing confidence intervals exhibited an average reduction of about 27%. Additionally, investigating peak wave period variation fluctuations concerning the range of significant wave heights revealed minimal variation in the high significant wave height range, characterized by concentrated spectral energy density. In contrast, a variation of approximately 1–2 s in peak wave period was evident, especially in lower significant wave height ranges. This phenomenon is attributed to complexities such as bimodal spectrum shape, where the spectral energy density exhibits an unstable pattern with diminishing significant wave height. Moreover, a refined smoothing number was calculated to enhance precision in smoothing relative to the significant wave height range. In other words, the analysis results show a negative correlation between the number of smoothed waves and the significant wave height: : −0.66, : −0.71 (the number of smoothed waves decreases as the significant wave height increases). This means that there are limits to applying the smoothing technique due to the unstable characteristic of the spectral energy density as the significant wave height decreases.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| HeMOSU | Herald of Meteorological and Oceanographic Special Research Units |
| SWAP | Standard Wave Analysis Package |
| CC | Pearson’s Correlation Coefficients |
| WSE | Water Surface Elevation |
| FFT | Fast Fourier Transform |
| MSE | Mean Square Error |
| RMSE | Root Mean Square Error |
| CI | Confidence Interval |
| LPI | Local Plug-In |
| DPI | Direct Plug-In |
| Nomenclature | |
| Fourier Coefficients | |
| the Zeroth Moment of the Estimated Wave Spectrum | |
| Second Moment in Wave Spectrum | |
| First-Inverse Moment in Wave Spectrum | |
| Water Surface Elevation | |
| Total Energy of the Surface Elevation | |
| Significant Wave Height (using Wave Spectrum Analysis Method) | |
| Peak Wave Period (using Wave Spectrum Analysis Method) | |
| Mean Period (, using Wave Train Analysis Method) | |
| Mean Period (using Wave Spectrum Analysis Method) | |
| Frequency | |
| Frequency Interval | |
| Nyquist Frequency | |
| Frequency Resolution | |
| Confidence Interval of Spectrum | |
| Actual Spectrum | |
| Triangular Smoothing Spectrum | |
| LPI Smoothing Spectrum | |
| Spectral Confidence Interval with 95% Chi-square Distribution | |
| Number of spectrum data | |
| Smoothing Function | |
| Rectangular Smoothing | |
| Triangular Smoothing | |
| Parabolic Smoothing | |
| Spectral Density | |
| Correction Factor | |
References
- Cho, H.-Y.; Kweon, H.-M.; Jeong, W.-M. A study on the optimal equation of the continuous wave spectrum. Int. J. Nav. Arch. 2015, 7, 1056–1063. [Google Scholar] [CrossRef]
- Horikawa, K. Coastal Engineering, an Introduction to Ocean Engineering; University of Tokyo Press: Tokyo, Japan, 1978. [Google Scholar]
- Mizuguchi, M. Individual wave analysis of irregular wave deformation in the nearshore zone. In Coastal Engineering 1982; American Society of Civil Engineers: Reston, VA, USA, 1982; pp. 485–504. [Google Scholar]
- Goda, Y. Random Seas and Design of Maritime Structures; World Scientific Publishing Company: Singapore, 2010; Volume 33. [Google Scholar]
- Stansell, P.; Wolfram, J.; Linfoot, B. Effect of sampling rate on wave height statistics. Ocean Eng. 2002, 29, 1023–1047. [Google Scholar] [CrossRef]
- Mazeika, L.; Draudviliene, L. Analysis of the zero-crossing technique in relation to measurements of phase velocities of the Lamb waves. Ultragarsas/Ultrasound 2010, 65, 7–12. [Google Scholar]
- Nishi, R.; Sato, M.; Kraus, N.C. Development of Wave Train Analysis Package: WAVETRAP; The Research Report of the Faculty of Engineering, Kagoshima University; Kagoshima University: Kagoshima, Japan, 1997; Volume 39, pp. 179–198. [Google Scholar]
- Choi, H.; Jeong, S.T.; Cho, H.Y.; Ko, D.H.; Kang, K.S. Development of wave by wave analysis program using MATLAB. J. Korean Soc. Coast. Ocean Eng. 2017, 29, 239–246. (In Korean) [Google Scholar] [CrossRef]
- Zheng, G.; Cong, P.; Pei, Y. On the improvements to the wave statistics of narrowbanded waves when applied to broadbanded waves. J. Geophys. Res. Atmos. 2006, 111. [Google Scholar] [CrossRef]
- Thompson, E.F. Energy Spectra in Shallow US Coastal Waters. 1980. Available online: https://hdl.handle.net/11681/22206 (accessed on 15 September 2023).
- Pierson, W.J.; Marks, W. The power spectrum analysis of ocean-wave records. Trans. Am. Geophys. Union 1952, 33, 834–844. [Google Scholar]
- Casas Prat, M. Overview of Ocean Wave Statistics. 2008. Available online: https://upcommons.upc.edu/handle/2099.1/6034 (accessed on 15 September 2023).
- Liu, P.C. Is the wind wave frequency spectrum outdated. Ocean Eng. 2000, 27, 577–588. [Google Scholar] [CrossRef]
- Liu, P.C.; Babanin, A.V. Using wavelet spectrum analysis to resolve breaking events in the wind wave time series. In Annales Geophysicae; Copernicus GmbH: Göttingen, Germany, 2004; Volume 22, pp. 3335–3345. [Google Scholar]
- Aranuvachapun, S. Parameters of Jonswap spectral model for surface gravity waves—II. Predictability from real data. Ocean Eng. 1987, 14, 101–115. [Google Scholar] [CrossRef]
- Soares, C.G.; Carvalho, A.N. Probability distributions of wave heights and periods in combined sea-states measured off the Spanish coast. Ocean Eng. 2012, 52, 13–21. [Google Scholar] [CrossRef]
- Rodriguez, G.R.; Soares, C.G. The bivariate distribution of wave height and periods in mixed sea states. J. Offshore Mech. Arct. Eng. 1999, 121, 102–108. [Google Scholar] [CrossRef]
- IAHR Working Group on Wave Generation and Analysis. List of sea-state parameters. J. Waterw. Port Coast. Ocean Eng. 1989, 115, 793–808. [Google Scholar] [CrossRef]
- Allsop, N.W.H.; Bruce, T.; De Rouck, J.; Kortenhaus, A.; Pullen, T.; Schüttrumpf, H.; Troch, P.; Zanuttigh, B. EurOtop II Manual on Wave Overtopping of Sea Defences and Related Structures: An Overtopping Manual Largely Based on European Research, but for Worldwide Application, 2nd ed.; HR Wallingford: Wallingford, UK, 2016; 252p. [Google Scholar]
- Construction Industry Research, Information Association; Civieltechnisch Centrum Uitvoering Research en Regelgeving (Netherlands); Centre Detudes Maritimes et Fluviales. The Rock Manual: The Use of Rock in Hydraulic Engineering. 2007, Volume 683. Available online: https://www.cob.nl/wp-content/uploads/2018/01/K100-02.pdf (accessed on 15 September 2023).
- Kim, J.Y.; Kim, M.S. A Comparison of Offshore Met-mast and Lidar Wind Measurements at Various Heights. J. Korean Soc. Coast. Ocean Eng. 2017, 29, 12–19. (In Korean) [Google Scholar] [CrossRef]
- Hoekstra, G.W.; Boere, L.; van der Vlugt, A.J.M.; van Rijn, T. Mathematical Description of the Standard Wave Analysis Package; The Oceanographic Company of the Netherlands bv: Castricum, The Netherlands, 1994. [Google Scholar]
- Brigham, E.O. The Fast Fourier Transform and Its Applications; Prentice-Hall, Inc.: Upper Saddle River, NJ, USA, 1988. [Google Scholar]
- Pearson, K. Notes on regression and inheritance in the case of two parents. Proc. R. Soc. Lond. 1895, 58, 240–242. [Google Scholar]
- Thomson, R.E.; Emery, W.J. Data Analysis Methods in Physical Oceanography; Elsevier: Amsterdam, The Netherlands, 2014. [Google Scholar]
- Wand, M.P.; Jones, M.C. Kernel Smoothing; Chapman and Hall: London, UK, 1994. [Google Scholar]
- Lee, U.-J.; Ko, D.-H.; Cho, H.-Y.; Oh, N.-S. Correlation Analysis between Wave Parameters using Wave Data Observed in HeMOSU-1&2. J. Korean Soc. Coast. Ocean Eng. 2021, 33, 139–147. (In Korean) [Google Scholar]
- Ang, A.H.-S.; Tang, W.H. Probability Concepts in Engineering: Emphasis on Applications to Civil and Environmental Engineering, 2nd ed; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2007. [Google Scholar]
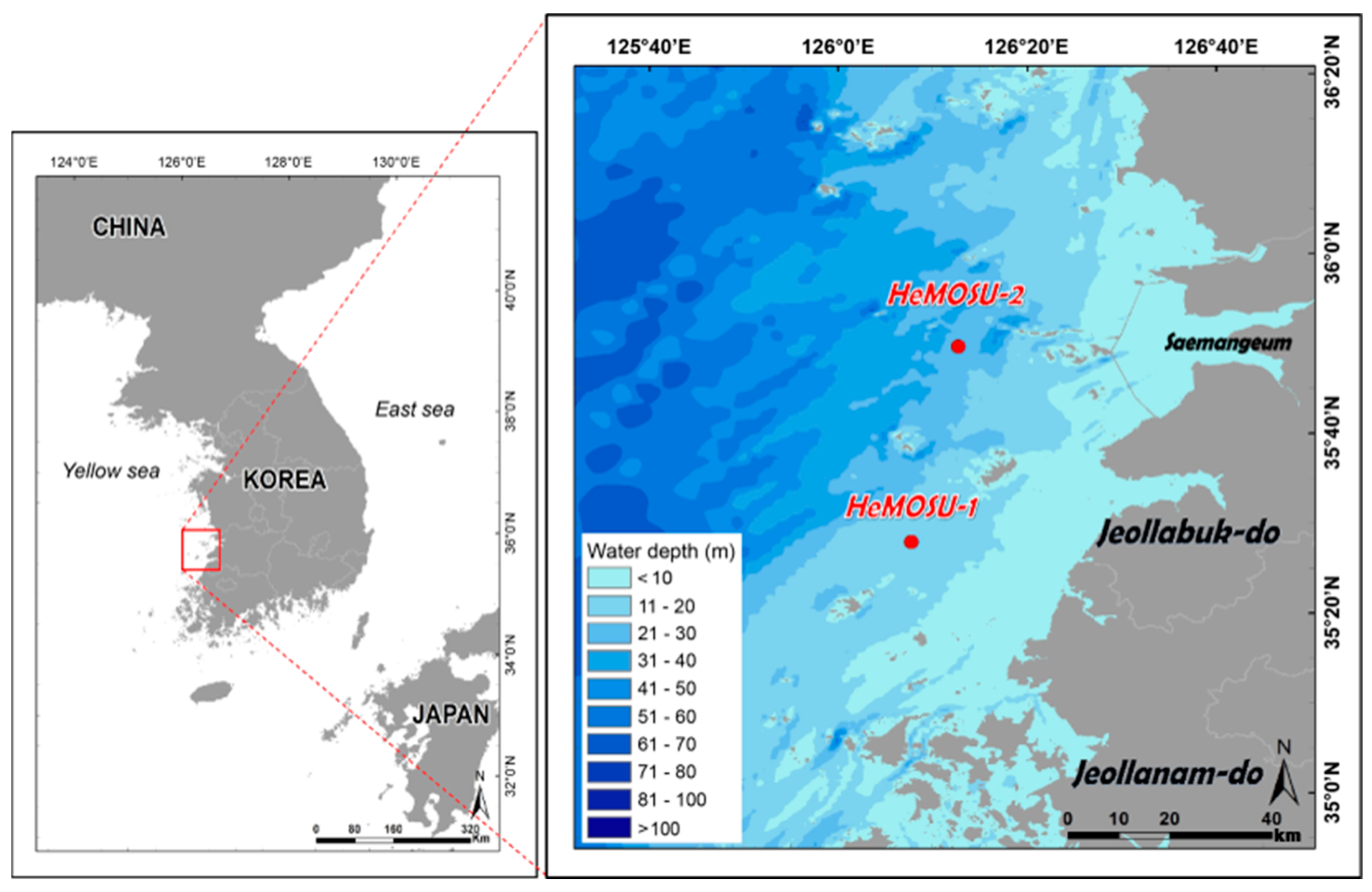





| Platform Name | ||
|---|---|---|
| HeMOSU-1 | HeMOSU-2 | |
| Classification |
|
|
| Characteristic |
|
|
| Longitude and latitude | 126°07′45″ E, 35°27′55″ N | 126°12′45″ E, 35°49′40″ N |
| Model | Waveguide Radar System | |
|---|---|---|
| Manufacturer | RADAC (NL) | |
| Sampling rate | 5 Hz | |
| Wave heights | 0–60 m | |
| Wave periods | 1–100 s | |
| Accuracy water level | 1.0 cm | |
| Electrical | Radar frequency | 9.9–10.2 GHz |
| Modulation | Triangular FM | |
| Power requirements | 24–64 VDC, 6 W/radar | |
| Mechanical | Dimensions | 26/21 cm (diameter/height) |
| Weight | Approximately 9 kg | |
| Environmental conditions | Temperature | −40 °C to 65 °C |
| Humidity | 0–100% | |
| Accuracy | Water surface elevation | |
| Wave height | ||
| Wave period | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, U.-J.; Ko, D.-H.; Cho, H.-Y. Optimal Smoothing of the Wind–Wave Spectrum Based on the Confidence-Interval Trade-Off. J. Mar. Sci. Eng. 2023, 11, 2062. https://doi.org/10.3390/jmse11112062
Lee U-J, Ko D-H, Cho H-Y. Optimal Smoothing of the Wind–Wave Spectrum Based on the Confidence-Interval Trade-Off. Journal of Marine Science and Engineering. 2023; 11(11):2062. https://doi.org/10.3390/jmse11112062
Chicago/Turabian StyleLee, Uk-Jae, Dong-Hui Ko, and Hong-Yeon Cho. 2023. "Optimal Smoothing of the Wind–Wave Spectrum Based on the Confidence-Interval Trade-Off" Journal of Marine Science and Engineering 11, no. 11: 2062. https://doi.org/10.3390/jmse11112062
APA StyleLee, U.-J., Ko, D.-H., & Cho, H.-Y. (2023). Optimal Smoothing of the Wind–Wave Spectrum Based on the Confidence-Interval Trade-Off. Journal of Marine Science and Engineering, 11(11), 2062. https://doi.org/10.3390/jmse11112062






