Numerical Analysis of Mechanical Behaviors of Composite Tensile Armored Flexible Risers in Deep-Sea Oil and Gas

Abstract

As oil and natural gas production continue to go deeper into the ocean, the flexible riser, as a connection to the surface of the marine oil and gas channel, will confront greater problems in its practical application. Composite materials are being considered to replace steel in the unbonded flexible pipe in order to successfully meet the lightweight and high-strength criteria of ultra-deep-water oil and gas production. The carbon-fiber-reinforced material substitutes the steel of the tensile armor layer with a greater strength-to-weight ratio. However, its performance in deep-water environments is less researched. To investigate the mechanical response of a carbon fiber composite flexible riser in the deep sea, this study establishes the ABAQUS quasi-static analysis model to predict the performance of the pipe. Considering the special constitutive relations of composite materials, the tensile stiffness of steel pipe and carbon fiber-reinforced composite flexible pipe are predicted. The results show that the replacement of steel strips with carbon fiber can provide 85.06% tensile stiffness while reducing the weight by 77.7%. Moreover, carbon-fiber-reinforced strips have a lower radial modulus, which may not be sufficient to cause buckling under axial compression, so the instability of the carbon fiber composite armor layer under axial compression is further studied in this paper; furthermore, the characteristics of axial stiffness are analyzed, and the effects of the friction coefficient and hydrostatic pressure are discussed.

1. Introduction

The offshore flexible riser is a pipeline that connects the subsea oil and gas wellhead to the gathering station (ship) and is crucial to the development of offshore oil and gas resources [1,2,3]. It has gradually replaced traditional steel pipe due to its high corrosion resistance, good terrain adaptability, long continuous length, ease of installation, and other advantages [4]. The unbonded flexible pipe is a composite structure formed by a metal winding structure and polymer extrusion; they work together but are independent of each other. Flexible pipes have been frequently utilized in offshore oil and gas production throughout the past few decades. During installation and operation, risers may be subjected to axial compression and bending loads, and complex constructions composed of numerous layers are extremely deformable when subjected to axisymmetric loads. The failure modes of tensile armor wire include fracture, radial, and lateral buckling. The unbonded flexible pipes typically consist of a carcass layer, a pressure armor layer, tensile armor layers, inner and outer sheaths, anti-wear layers, and an anti-buckling layer. During installation and operation, the riser may be subjected to axial compression and bending loads. Fracture, radial buckling, and lateral buckling are the mechanisms of failure for tensile armor layers. Theoretical analysis, numerical simulation, and experimental verification are the three main methods in engineering applications of flexible risers. The theoretical analysis is to obtain the mechanical property parameters of the structure by solving Equations [5,6,7,8]. Typical unbonded flexible (Figure 1) pipes have complex structural layers, making it difficult to perform a structural mechanical study of the entire pipe. Therefore, a large number of scholars use numerical simulation to analyze flexible risers [9,10,11,12,13,14,15,16,17,18,19]. Because the working environment of the flexible riser is located in the deep sea, it is difficult to conduct field experimental research, so there are only a few experimental studies [20,21,22,23].

In recent years, the growth of the deep-sea oil and gas industry has highlighted the importance of considering the weight of flexible pipes in their design. The weight of the pipe has a significant impact on the size of the platform and the magnitude of the axial tensile load. To address this challenge, researchers and industry practitioners have been exploring the potential of new materials, such as fiber-reinforced composites. These materials offer a higher strength-to-mass ratio than traditional metals, meaning that less material is required to achieve the same strength. Additionally, fiber-reinforced composites exhibit a lower modulus in the non-fiber direction, resulting in greater flexibility and enhanced resistance to corrosion and fatigue compared to metals. As such, fiber-reinforced composites hold great promise for improving the performance and efficiency of flexible pipe systems in deep-sea applications [24]. The application of carbon fiber-reinforced plastic composites in offshore platforms is growing rapidly, especially in flexible risers and pressure vessels [25,26].

The application of composite materials in flexible risers began in the eighties of the last century. In the 1980s, the French Institute for Petroleum Research (IFP) and the French Institute for Aerospace Research (Aerospatiale) made the initial effort to design and assess composite production risers [27]. Belye et al. [28] conducted a comparative study on steel and composite drilling risers and developed a design method. Lotveit and Ward [29] first proposed the use of aramid fibers as tensile sheath lines in unbonded flexible risers but did not consider the poor corrosion resistance of aramid materials. In 1993, Coflexip Stena Offshore manufactured the first flexible pipe with a stretched composite sheath line using a fiberglass-reinforced epoxy composite. In the mid-1990s, under the supervision of the National Institute of Standards and Technology Advanced Technology program, a joint industrial project was started to develop a composite flexible riser [30,31,32] and a composite drilling riser [33,34,35]. Kalman et al. [36] proposed a lightweight design that uses composite tape instead of steel tensile armored wire. In 2001, composite risers were first used on the Heidrun TLP platform in the Norwegian Sea and their successful deployment accelerated the commercial pace of fiber-reinforced materials for deep-sea applications. The 2002 OTC conference went into the deep ocean and presented many ideas, conceptual designs, and analyses for composite applications in the ocean, indicating that composites will gradually replace traditional steel in deep-sea applications in the future. Ochoa and Salama [37,38] proposed several difficulties in the use of composite materials to replace metal materials, two of which are the in-depth excavation of the damage mechanism of composite materials and the effective application of nondestructive monitoring technology (NDE) on composite materials. In 2003, the Norwegian Register of Shipping gave a design specification for a composite riser [39], and Ochoa gave a design guideline for a composite rigid riser in a report for the US Federal Minerals Management Service (MMS) [40]. Ramirez et al. conducted a collapse pressure test on the composite flexible riser for offshore drilling; it was found that the carbon fiber tube can be stratified under pressure but there is only one experimental sample [41]. Almeida et al. [42,43] evaluated the deterioration and failure of carbon fiber composite armored pipes and studied the failure of composite pipes under static pressure through numerical and experimental analysis. The damage degradation of the composite riser was evaluated by a genetic algorithm for the first time. Toh et al. [44] analyzed the composite flexible pipe and believed that the weight of the flexible composite pipe could be reduced and the bearing pressure of the offshore platform could be reduced through appropriate design on the premise of maintaining its mechanical properties. The results show that the composite riser has a higher safety margin than the traditional steel pipe under the same sea conditions. Amaechi et al. [25] used the ANSYS Composite PrepPost module to study the influence of composite and put forward the optimization design method. Zhang et al. [45] proposed a cylindrical composite tensile armor layer. The optimum fiber helix angle was obtained by analysis and calculation.

Although several theoretical and experimental studies have been conducted on the application of new composite materials, previous research has primarily focused on the mechanical response of fiber-reinforced risers under axial tensile loads. Most of these studies have only considered the composite cylinder, with little attention paid to the fine tensile armor structure. The link between a riser’s global response and its local behavior has not been fully explored and there is almost no research on the buckling performance of composite risers.

Since fiber-reinforced materials are prone to buckling failure due to their small radial stiffness resulting from their orthogonal anisotropic material properties, studying the compression response of composite risers is of great significance. Fiber-reinforced materials are prone to buckling failure because they have small radial stiffness due to their orthogonal anisotropic material properties, so it is of great significance to study the compression response of composite risers. Firstly, this paper summarizes the vertical axial theoretical analysis method and uses ABAQUS software to establish an unbonded flexible riser. Because the experiment of the riser is affected by the actual length and production environment, it is difficult to experiment, and the accuracy of the model is verified by the experimental data. Then, the tensile stiffness and ultimate tensile strength of the carbon fiber riser were analyzed based on ABAQUS. Finally, the mechanical response of composite flexible risers under compression load was considered, and the buckling resistance of the risers under different external pressures was studied.

2. Theoretical Formulations

Non-bonded flexible risers are constructed with multiple layers that are allowed to slip relative to one another. Because each layer has unique geometric forms and material properties, they play different roles in the stress process, resulting in a complex analysis process. Currently, there are two main methods for analyzing the section characteristics of flexible risers: analytical and numerical methods. These methods can be used to study both axisymmetric and bending responses. The mechanical properties analysis of these two parts are closely related; for example, the interlayer contact pressure generated by the axisymmetric response can have a significant impact on the bending response. Previous investigations have shown that the axisymmetric response of flexible risers is linearly variable, whereas the bending response exhibits clear hysteresis behavior.

This chapter is based on the analysis of some basic assumptions: (1) The flexible riser has large axial and radial stiffness and small deformation. (2) Assume that the friction between each layer is zero, and the layers can slide freely without friction energy consumption. (3) Ignore residual stresses inherited from the manufacturing process. (4) The axial force and torque are uniformly distributed on each layer and the pressure distribution inside and outside the pipeline does not change as the pipe length increases.

According to the different characteristics of the pipeline, the pipeline is divided into three types: (1) Isotropic cylindrical shell—inner sheath, outer sheath, and anti-wear layer. (2) Small-angle spiral winding layer—tensile armor layer. (3) Large-angle spiral layer—carcass layer and pressure armor layer.

2.1. Theoretical Calculation of Cylindrical Shells

In the case of flexible risers, the outer layer, inner layer, and anti-wear layer, which primarily consist of polymer materials, serve the functions of preventing leakage, reducing friction, and providing protection but contribute very little to bearing external loads. For the purposes of this study, these layers are uniformly classified as polymer layers. Polymer materials can be considered isotropic and linearly elastic within a small deformation range. Moreover, due to the relatively low elastic modulus of polymer materials, the thick-walled cylinder theory is utilized to accurately simulate the radial displacement of these layers. The definition of coordinates and symbols for the bounded flexible pipe as shown in Figure 2.

The stiffness of the inner sheath, the anti-wear layer, and the outer layer can be calculated by the analysis of the isotropic cylinder. This section gives an expression for the isotropic layer. Assuming that the strain is the same along the length of the pipe, the relationship between strain and displacement can be written as:

$${\mathsf{\epsilon }}_1=\frac{{\mathsf{\Delta }\mathrm{u}}_{\mathrm{z}}}{\mathrm{L}}+\mathrm{y}\frac{{\mathsf{\Delta }\mathsf{\psi }}_{\mathrm{x}}}{\mathrm{L}}-\mathrm{x}\frac{{\mathsf{\Delta }\mathsf{\psi }}_{\mathrm{y}}}{\mathrm{L}}$$

(1)

$${\mathsf{\epsilon }}_2=\frac{{\mathrm{u}}_{\mathrm{r}}}{\mathrm{L}}$$

(2)

$${\mathsf{\gamma }}_{12}=\mathrm{R}\frac{\mathsf{\Delta }\mathsf{\varphi }}{\mathrm{L}}$$

(3)

where R is the radius of the middle plane and L is the length of the pipe. The strain energy can be derived by:

$$\mathrm{U}=\frac{1}{2}{{\displaystyle \int }}_{\mathrm{V}}\left({\mathsf{\sigma }}_1{\mathsf{\epsilon }}_1+{\mathsf{\sigma }}_2{\mathsf{\epsilon }}_2+{\mathsf{\sigma }}_3{\mathsf{\epsilon }}_3+{\mathsf{\tau }}_{23}{\mathsf{\gamma }}_{23}+{\mathsf{\tau }}_{31}{\mathsf{\gamma }}_{31}+{\mathsf{\tau }}_{12}{\mathsf{\gamma }}_{12}\right)\mathrm{dV}$$

(4)

The work performed by the external load on the specified displacement increment can be expressed as:

$$\mathrm{W}={\mathrm{N}\mathsf{\Delta }\mathrm{u}}_{\mathrm{z}}+\mathrm{T}\mathsf{\Delta }\mathsf{\varphi }+{\mathrm{M}}_{\mathrm{x}}{\mathsf{\Delta }\mathsf{\psi }}_{\mathrm{x}}+{\mathrm{M}}_{\mathrm{y}}{\mathsf{\Delta }\mathsf{\psi }}_{\mathrm{y}}+\mathsf{\Delta }\mathrm{p}\left(2\frac{{\mathrm{u}}_{\mathrm{r}}}{\mathrm{R}}+\frac{{\mathsf{\Delta }\mathrm{u}}_{\mathrm{z}}}{\mathrm{L}}\right){\mathsf{\pi }\mathrm{R}}^2\mathrm{L}$$

(5)

where $\mathrm{N}, \mathrm{T}, {\mathrm{M}}_{\mathrm{x}}$, and ${\mathrm{M}}_{\mathrm{y}}$ are the axial force, axial torque, and bending moment for two radial directions, respectively. $\mathsf{\Delta }\mathrm{p}$ radial pressure difference in the tube layer, $\mathsf{\Delta }\mathrm{p}={\mathrm{p}}^{\mathrm{in}}-{\mathrm{p}}^{\mathrm{out} }$.

2.2. Theoretical Calculation of Large-Angle Spiral Layer

According to the generalized Hooke’s law, the stress–strain relation of the thin-walled cylinder in the orthogonal coordinate system can be expressed as:

$$\left[\begin{array}{c}{\mathsf{\sigma }}_1\\ {\mathsf{\sigma }}_2\\ {\mathsf{\sigma }}_{12}\end{array}\right]=\left[\begin{array}{ccc}\frac{{\mathrm{E}}_1}{1-{\mathrm{v}}_{12}{\mathrm{v}}_{21}}& \frac{{\mathrm{E}}_2{\mathrm{v}}_{12}}{1-{\mathrm{v}}_{12}{\mathrm{v}}_{21}}& 0\\ \frac{{\mathrm{E}}_1{\mathrm{v}}_{21}}{1-{\mathrm{v}}_{12}{\mathrm{v}}_{21}}& \frac{{\mathrm{E}}_2}{1-{\mathrm{v}}_{12}{\mathrm{v}}_{21}}& 0\\ 0& 0& {\mathrm{G}}_{12}\end{array}\right]\left[\begin{array}{c}{\mathsf{\epsilon }}_1\\ {\mathsf{\epsilon }}_2\\ {\mathsf{\epsilon }}_{12}\end{array}\right]$$

(6)

According to the plane stress assumption, ${\mathsf{\tau }}_{12}$, ${\mathsf{\tau }}_{13}$, ${\mathsf{\tau }}_{23}=$ 0, so the strain energy can be expressed as:

$$\mathrm{U}=\frac{1}{2}{{\displaystyle \int }}_{\mathrm{V}}\left({\mathsf{\sigma }}_1{\mathsf{\epsilon }}_1+{\mathsf{\sigma }}_2{\mathsf{\epsilon }}_2+{\mathsf{\sigma }}_{12}{\mathsf{\epsilon }}_{12}\right)\mathrm{dV}$$

(7)

where ${\mathsf{\sigma }}_1$ is axial stress, ${\mathsf{\sigma }}_2$ is annular stress, and ${\mathsf{\sigma }}_{12}$ is shear stress.

2.3. Theoretical Calculation of Tensile Armor Layer

It is assumed that each spiral armor flat steel is linearly elastic and only its axial stress is considered, other stresses are ignored, so the elastic energy of this layer is the sum of all the elastic energy of each spiral armor flat.

$$\mathrm{U}=\frac{1}{2}{{\displaystyle \sum }}_{\mathrm{i}=1}^{\mathrm{n}}{\mathrm{EA}}^{\mathrm{i}}{\int }_{\mathrm{L}}{\left({\mathsf{\epsilon }}^{\mathrm{i}}\right)}^2\frac{\mathrm{dz}}{\mathsf{\alpha }}$$

(8)

Here, n is the number of flat steels in the helical armor layer, Aⁱ is the cross-sectional area of its i^th stripe, and α is the angle of its spiral winding.

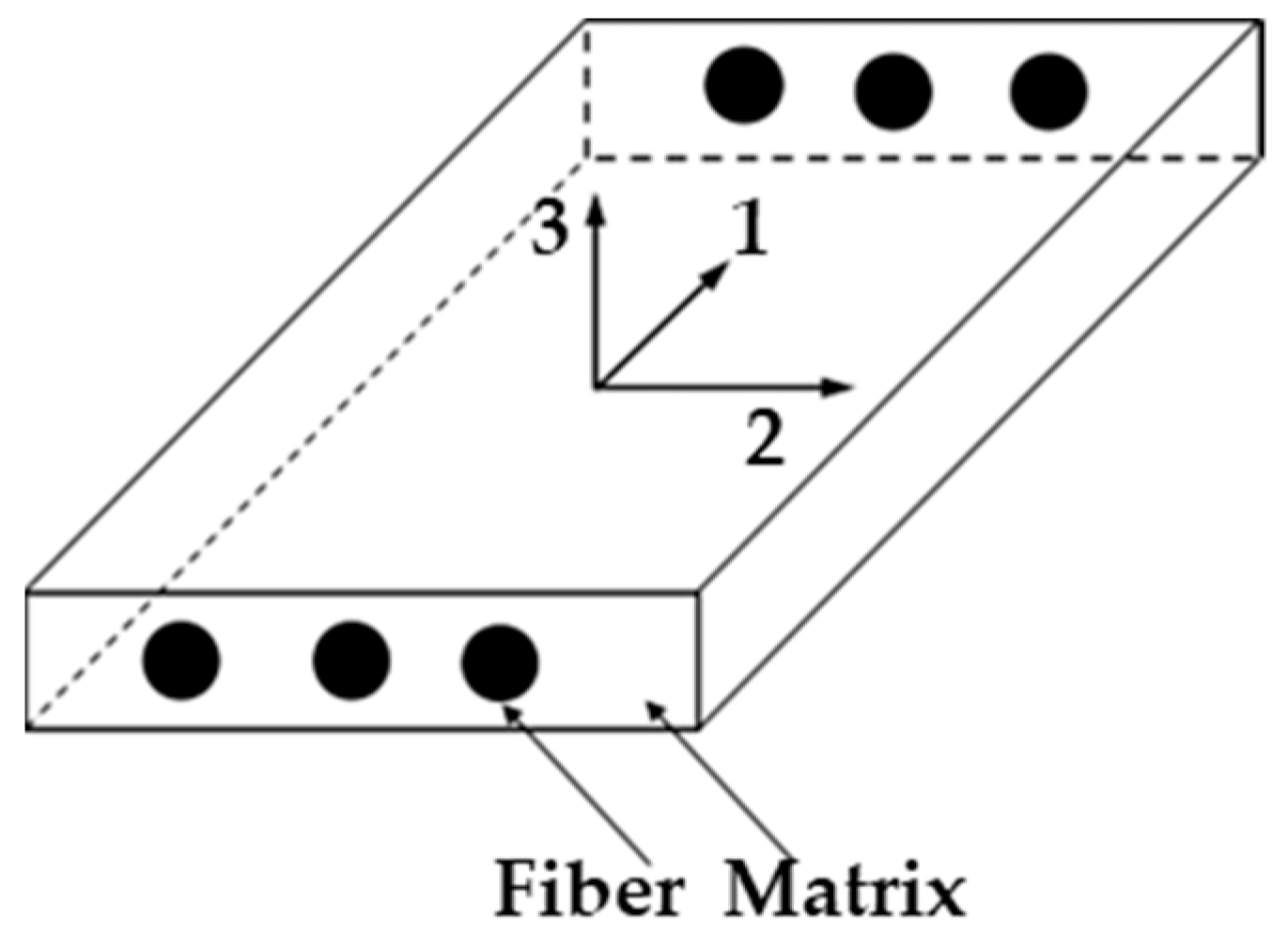
2.4. Elastic Constants of Composite Material

At the macroscopic mechanical scale, composite monolayers can be regarded as an orthotropic material. The constitutive relation is expressed in the local coordinate system: where the strength and stiffness of the fiber direction are stronger, called longitudinal, and represented by “1” or “R”; the direction perpendicular to the fiber is called transverse and represented by “2” or “T”; and the thickness direction is represented by “3” or “Z”. The same coordinate system is needed to establish the mechanical equilibrium equation for the whole structure. The corresponding coordinate directions of the composite strips are shown in Figure 3.

The micromechanics method was used to figure out the properties of composite tensile armor strips. The subscripts F and M denote the fiber and matrix. Based on the prediction formula of the transverse modulus of composite material proposed by Halpin and Tsai [46], the elastic modulus of composite strips in the local coordinate system can be written as follows:

$$\{\begin{array}{c}\begin{array}{l}{\mathrm{E}}_1={\mathrm{E}}_{\mathrm{f}}{\mathrm{V}}_{\mathrm{f}}+{\mathrm{E}}_{\mathrm{m}}\left(1-{\mathrm{V}}_{\mathrm{f}}\right)\hfill \\ {\mathrm{E}}_2={\mathrm{E}}_3={\mathrm{E}}_{\mathrm{m}}\frac{1+2{\mathsf{\beta }\mathrm{V}}_{\mathrm{f}}}{1-{\mathsf{\beta }\mathrm{V}}_{\mathrm{f}}},\mathsf{\beta }=\frac{{\mathrm{E}}_{\mathrm{f}}-{\mathrm{E}}_{\mathrm{m}}}{{\mathrm{E}}_{\mathrm{f}}+2{\mathrm{E}}_{\mathrm{m}}}\hfill \end{array}\end{array}$$

(9)

$$\{\begin{array}{c}{\mathrm{G}}_{12}={\mathrm{G}}_{13}=\frac{{\mathrm{G}}_{\mathrm{f}}{\mathrm{G}}_{\mathrm{m}}\frac{{\mathrm{V}}_{\mathrm{f}}}{{\mathrm{V}}_1}}{{\mathrm{G}}_{\mathrm{m}}{\mathrm{V}}_1+{\mathrm{G}}_{\mathrm{f}}{\mathrm{V}}_2}+{\mathrm{G}}_{\mathrm{m}}\left(1-\frac{{\mathrm{V}}_{\mathrm{f}}}{{\mathrm{V}}_1}\right)\\ {\mathrm{G}}_{23}=\frac{{\mathrm{G}}_{\mathrm{f}}{\mathrm{G}}_{\mathrm{m}}{\mathrm{V}}_1}{{\mathrm{G}}_{\mathrm{m}}\frac{{\mathrm{V}}_{\mathrm{f}}}{{\mathrm{V}}_1}+{\mathrm{G}}_{\mathrm{f}}\left(1-\frac{{\mathrm{V}}_{\mathrm{f}}}{{\mathrm{V}}_1}\right)}+{\mathrm{G}}_{\mathrm{m}}{\mathrm{V}}_2\end{array}$$

(10)

$$\{\begin{array}{c}{\mathsf{\mu }}_{12}={\mathsf{\mu }}_{13}={\mathrm{V}}_{\mathrm{f}}{\mathsf{\mu }}_{\mathrm{f}}+\left(1-{\mathrm{V}}_{\mathrm{f}}\right){\mathsf{\mu }}_{\mathrm{m}}\\ {\mathsf{\mu }}_{23}={\mathsf{\mu }}_{\mathrm{f}}{\mathrm{V}}_{\mathrm{f}}+{\mathsf{\mu }}_{\mathrm{m}}\left(1-{\mathrm{V}}_{\mathrm{f}}\right)\frac{{\mathrm{E}}_1\left(1+{\mathsf{\mu }}_{\mathrm{m}}\right)-{\mathsf{\mu }}_{12}{\mathrm{E}}_{\mathrm{m}}}{{\mathrm{E}}_1\left(1-{\mathsf{\mu }}_{\mathrm{m}}^2\right)+{\mathsf{\mu }}_{\mathrm{m}}{\mathsf{\mu }}_{12}{\mathrm{E}}_{\mathrm{m}}}\end{array}$$

(11)

where E₁, E₂, E₃ are the elastic modulus in all three directions; G₁₂, G₁₃, G₂₃ are the shear modulus in all three directions; and ${\mathsf{\mu }}_{12},{ \mathsf{\mu }}_{13},{ \mathsf{\mu }}_{23}$ are Poisson’s ratio in all three directions. The subscripts f and m denote the fiber and matrix. The generalized Hooke’s law which is the stress–strain relation can be written in the local coordinate system.

2.5. Overall Stiffness of a Typical Unbonded Flexible Riser

According to the principle of virtual work, the relationship between work performed by external forces and strain energy can be obtained, as shown in Equation (6).

$$\mathrm{dU}=\mathrm{dW}$$

(12)

The equilibrium equation of the whole flexible pipe can be obtained by synthesizing the balance equation of each layer. To be consistent, the same flexible pipe length, axial displacement, axial torsion, and bending were used for each layer when deriving the equilibrium equations for each layer. Moreover, to consider the separation between layers, we allow different radial displacements for each layer. By superimposing the balance equations obtained in the previous sections, we can obtain the global stiffness matrix of the eight-layer flexible riser.

$$\left[\mathrm{K}\right][\Delta ]=[\mathrm{F}]$$

(13)

where $\left[\mathrm{K}\right]$ is the superimposed overall stiffness matrix; $[\Delta ]$ is the displacement matrix; and $[\mathrm{F}]$ is the load matrix. To solve Equation (12), using the interlayer pressures ${\mathsf{\Delta }\mathrm{p}}_1$, ${\mathsf{\Delta }\mathrm{p}}_2$,……${\mathsf{\Delta }\mathrm{p}}_8$, the interlayer pressure of this equation in the flexible tube can be solved by an iterative method, and the external pressure directly acts on the outermost layer of the flexible tube, namely the outer sheath. The internal pressure acts on the inner sheath.

2.6. Compatibility Equation

The radial displacement and deformation of the flexible pipe layer are shown in Figure 4. Assuming that there is no gap between the pipe layers, the pipe should meet the continuum equation of radial displacement.

Where ${\mathsf{\Delta }\mathrm{r}}_{ }^{\mathrm{out} }$ and ${\mathsf{\Delta }\mathrm{r}}_{ }^{\mathrm{in} }$ are the changes in ${\mathrm{R}}_{\mathrm{in}}$ and ${\Delta }_{\mathrm{t}}$ thickness of each layer defined in Figure 4, respectively. Due to the large rigidity of the spiral armor layer (5, 7), pressure armor layer (3), and carcass layer (1), each layer is assumed to have a constant thickness and move along radial stiffness. We can obtain:

$${\mathsf{\Delta }\mathrm{r}}_{\mathrm{i}}^{\mathrm{out} }={\mathsf{\Delta }\mathrm{r}}_{\mathrm{i}}^{\mathrm{in}},{ \mathsf{\Delta }\mathrm{t}}_{\mathrm{i}}=0, \mathrm{i}=1, 3, 5, 7$$

(14)

The other layers (2, 4, 6, 8) can be represented as:

$${\mathsf{\Delta }\mathrm{t}}_{\mathrm{i}}={\mathsf{\Delta }\mathrm{p}}_{\mathrm{i}}\frac{\left(2-{\mathsf{\nu }}_{\mathrm{i}}\right){\mathrm{R}}_{\mathrm{i}}^2}{2{\mathrm{E}}_{\mathrm{i}}{\mathrm{t}}_{\mathrm{i}}}-\frac{{\mathsf{\nu }}_{\mathrm{i}}{\mathrm{N}}_{\mathrm{i}}{\mathrm{R}}_{\mathrm{i}}}{{\mathrm{A}}_{\mathrm{i}}{\mathrm{E}}_{\mathrm{i}}}, \mathrm{i}=2, 4, 6, 8$$

(15)

The equilibrium of pressure in the flexible riser can be described by the compatibility equation, which states that the sum of the contact pressures between the layers is equal to the pressure difference between the outer layers of the riser:

$${\displaystyle \sum }_{\mathrm{i}=1}^8{\mathsf{\Delta }\mathrm{p}}_{\mathrm{i}}={\mathrm{P}}_{\mathrm{in} }-{\mathrm{P}}_{\mathrm{out} }$$

(16)

The pressure difference equation of spiral armor flat steel layer deduced by Lanteigne is:

$${\mathsf{\Delta }\mathrm{p}}_{\mathrm{i}}=\mathrm{nA}\mathsf{\epsilon }\frac{{\sin }^2\mathsf{\alpha }}{2{\mathsf{\pi }\mathrm{R}}^2\cos \mathsf{\alpha }}, \left(\mathrm{i}=5, 7\right)$$

(17)

Considering the relationship between the radius and displacement of each layer of flexible pipe, the following equation can be derived:

$${\mathrm{R}}_{\mathrm{i}}+{\mathrm{u}}_{\mathrm{ri}}=\frac{{\mathrm{R}}_{\mathrm{i}}^{\mathrm{in} }+{\mathsf{\Delta }\mathrm{r}}_{\mathrm{i}}^{\mathrm{in} }+{\mathrm{R}}_{\mathrm{i}}^{\mathrm{out} }+{\mathsf{\Delta }\mathrm{r}}_{\mathrm{i}}^{\mathrm{out} }}{2}\mathrm{i}=1,2,\dots ,8$$

(18)

Substituting the deformation coordination relations derived above into the equation, seven deformation coordination equations can be obtained:

$$\begin{array}{c}2{\mathrm{R}}_{\mathrm{i}}+2{\mathrm{u}}_{\mathrm{ri}}={\mathrm{R}}_{\mathrm{i}}^{\mathrm{in}}+2\Delta {\mathrm{r}}_{\mathrm{i}}^{\mathrm{in}}+{\mathrm{R}}_{\mathrm{i}}^{\mathrm{out} }+\Delta {\mathrm{p}}_{\mathrm{i}}\frac{\left(2-{\mathsf{\nu }}_{\mathrm{i}}\right){\mathrm{R}}_{\mathrm{i}}^2}{2{\mathrm{E}}_{\mathrm{i}}{\mathrm{t}}_{\mathrm{i}}}-\frac{{\mathsf{\nu }}_{\mathrm{i}}{\mathrm{N}}_{\mathrm{i}}{\mathrm{R}}_{\mathrm{i}}}{{\mathrm{A}}_{\mathrm{i}}{\mathrm{E}}_{\mathrm{i}}},\mathrm{i}=2,4,6,8\\ 2{\mathrm{R}}_{\mathrm{i}}+2{\mathrm{u}}_{\mathrm{ri}}={\mathrm{R}}_{\mathrm{i}}^{\mathrm{in}}+2\Delta {\mathrm{r}}_{\mathrm{i}-1}^{\mathrm{in}}+{\mathrm{R}}_{\mathrm{i}}^{\mathrm{out} }+2\Delta {\mathrm{p}}_{\mathrm{i}-1}\frac{\left(2-{\mathsf{\nu }}_{\mathrm{i}-1}\right){\mathrm{R}}_{\mathrm{i}-1}^2}{2{\mathrm{E}}_{\mathrm{i}-1}{\mathrm{t}}_{\mathrm{i}-1}}-\frac{{\mathsf{\nu }}_{\mathrm{i}-1}{\mathrm{N}}_{\mathrm{i}-1}{\mathrm{R}}_{\mathrm{i}-1}}{{\mathrm{A}}_{\mathrm{i}-1}{\mathrm{E}}_{\mathrm{i}-1}},\mathrm{i}=3,5,7\end{array}$$

(19)

3. Finite Element Model

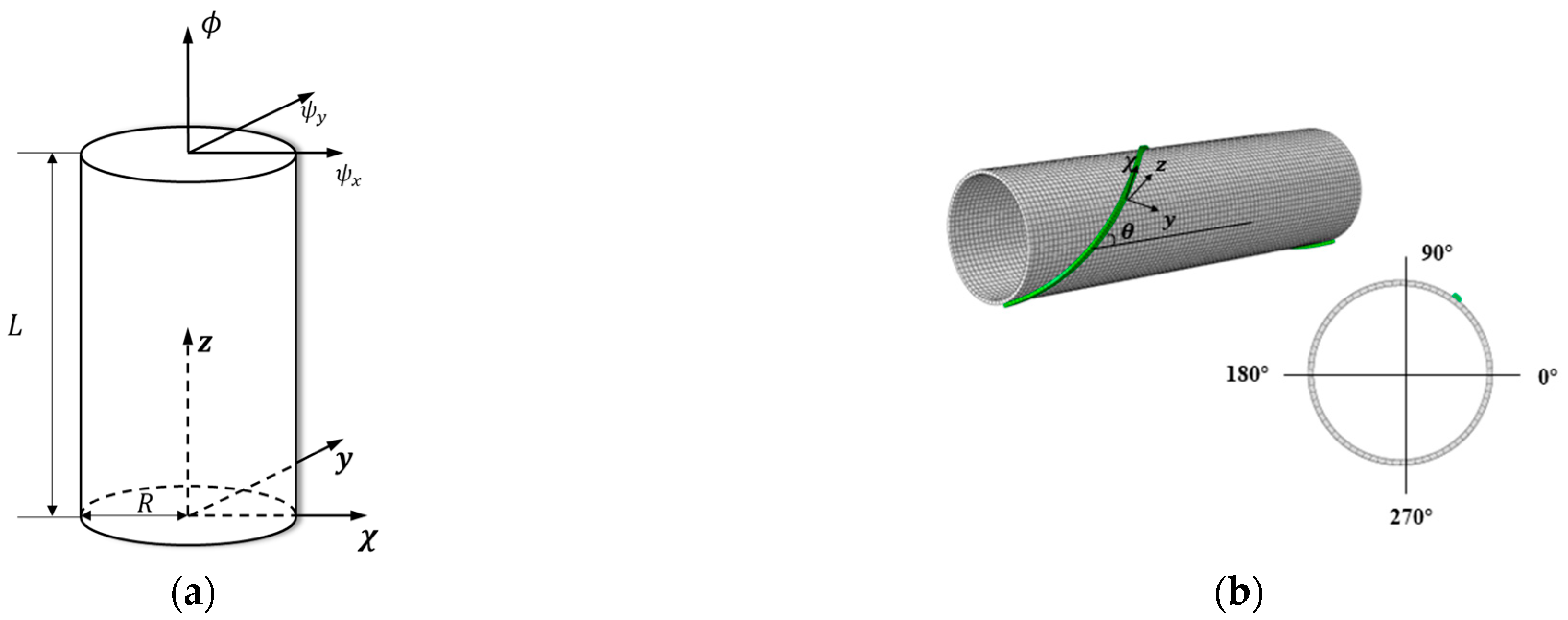
3.1. Geometric and Material Parameters

A flexible riser has a complex structure and high cost, and its performance test usually depends on special experimental equipment. It is not practical to use the composite riser since it is situated in a deep-sea environment that is unsuitable for effective experimentation. Therefore, this paper adopts a numerical simulation research method to simulate the composite riser and solve the buckling critical of CFRP risers under the action of axial compression load. In this paper, a 3D finite element model of the flexible riser is established by using ABAQUS, and the length of the pipe is 1000 mm. The geometric and material parameters of each layer are given in

Table 1. Parameters of the unbonded flexible riser with a composite reinforced layer.

Layer	Internal Radius (mm)	External Radius (mm)	External Radius (mm)	Thickness (mm)	Laying Angle	Number	Material
Carcass	31.6	35.1	35.1	3.5	87.5°	-	AISI304
Inner sheath	35.1	40.05	40.05	5	-	-	Nylon 12
Pressure armor	40.05	46.25	46.25	6.2	85.5°	-	F115
Anti-wear tap	46.25	47.75	47.75	1.5	-	-	Nylon 11
Inner tensile armor	47.75	50.75	50.75	3	35°	40	F141/T300
Anti-wear tap	50.75	52.25	52.25	1.5	-	-	Nylon 11
Outer tensile armor	52.25	55.25	55.25	4	-35°	44	F141/T300
Outer Sheath	55.25	55.75	55.75	0.5	-	-	Polymer

. Some geometric characteristics of each layer refer to Witz’s research data [13].

To better simulate the mechanical response of the actual riser under axial compression load during installation and operation, the anti-wear layer is added to the riser studied in this paper to more accurately simulate the actual contact between layers. The internal carcass layer and pressure armor layer are simplified as orthotropic cylindrical shells, the anti-wear layer, inner sheath, and outer sheath are modeled as an isotropic cylinder, and the tensile armor layer is modeled as spiral strip winding. For an accurate simulation of the inter-layer contact stress, the previous research is optimized, and the models of all layers are established as C3D8I elements. The key to simplifying the carcass layer into an orthotropic cylindrical shell is the accurate definition of orthotropic material parameters, here we have to solve for nine parameters: E₁, E₂, and E₃ are the elastic modulus of the three axes; G₁₂, G₂₃, and G₁₃ are the shear modulus; ${\mathrm{v}}_{12}$, ${\mathrm{v}}_{13}$, and ${\mathrm{v}}_{23}$ are Poisson’s ratio in three directions. The above parameters in ABAQUS correspond to the engineering constants, which correspond to the defined material properties.

The FE model of the flexible pipe adopts carbon-fiber-reinforced strips as orthogonal anisotropic materials defined by engineering constants. The material parameters of the remaining layers are shown in

Table 2. Material properties of traditional unbonded flexible risers.

Materials	E (MPa)	υ	Density (kg∙m−3)
AISI304	199	0.3	7930
Nylon 12	0.284	0.3	1080
F115	0.301	0.3	1040
Nylon 11	207	0.3	7850
F141	211	0.3	7870
Polymer	0.6	0.3	800

. The material performance is characterized by nine parameters, including the elastic modulus in three directions, shear modulus in three directions, and Poisson’s ratio in three directions, as shown in

Table 3. Material properties of T300/5208 graphite-epoxy.

E1	E2	E3	${\mathit{v}}_{12}$	${\mathit{v}}_{13}$	${\mathit{v}}_{23}$	G12	G13	G23
181,000 MPa	10,300 MPa	10,300 MPa	0.28	0.3	0.28	7170 MPa	7170 MPa	3780 MPa

. The parameters about T300/5208 graphite-epoxy come from Tawfik’s [47] research data. All layers, except for the carcass layer and the pressure armor layer, are isotropic cylindrical shells.

In addition, the ABAQUS includes two solvers: implicit solver ABAQUS/Standard and explicit solver ABAQUS/Explicit. The standard implicit solver can be easily applied to solve general problems. However, when solving nonlinear problems with a large number of contact behaviors, the iterative analysis will incur a huge computational cost and the analysis results may not converge. The explicit solver solves nonlinear problems without iteration and the result converges more easily.

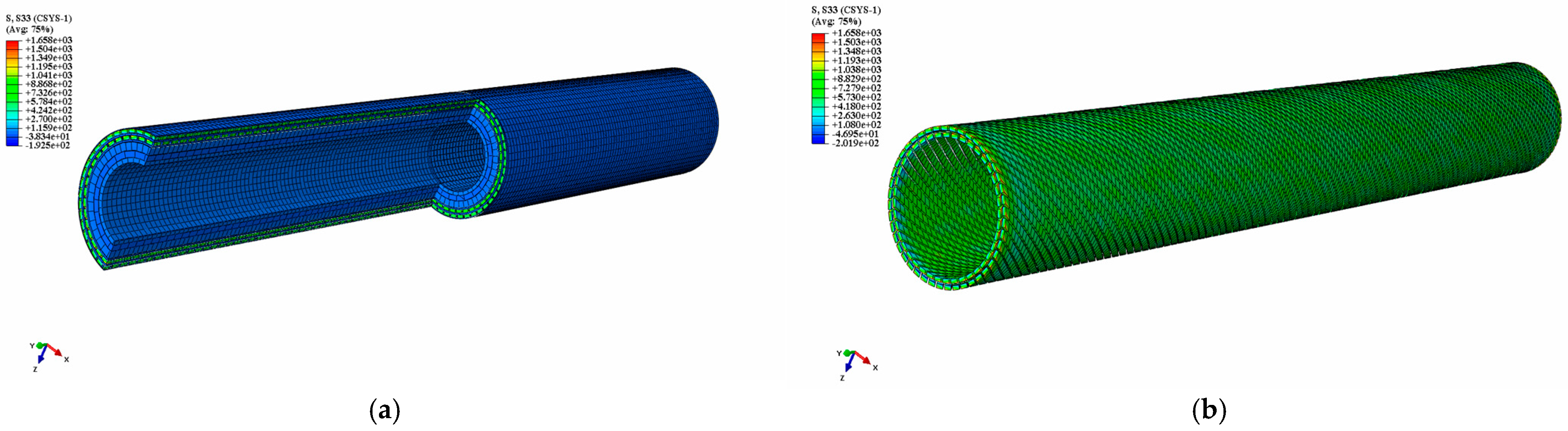
3.2. Boundary Conditions and Settings

In the finite element model, the normal direction of the interlayer contact type of the riser is selected as hard contact, and the axis is selected as a penalty function. The friction coefficient is selected as 0.1 according to Sævik and Berge [22]. ABAQUS/Explicit is used to avoid nonconvergent results at the center of the cross-section at both ends, adopt kinematic coupling at the end of the layer, and apply all loads and boundary conditions to the reference point, as shown in Figure 5. To reduce the instability in the numerical simulation, smooth step loading is used to avoid the fluctuation of the results caused by the discontinuity of loading. Moreover, to ensure the effectiveness of the simulation results, the ratio of kinetic energy (ALLKE) to internal energy (ALLIE) is controlled below 5%.

4. Case Study

4.1. Axial Tensile Response

In previous research, numerous studies have examined the tensile response of traditional steel flexible risers. To verify the proposed FE model, we referred to the experimental data of Witz [13] on a 1000 mm flexible riser in the initial stage of the study. Witz’s study is a seminal work on the experimental investigation of flexible hoses. It provides detailed information on the dimensions and material parameters of each layer of the one-inch flexible riser and the test results for its axial symmetric response and bending response.

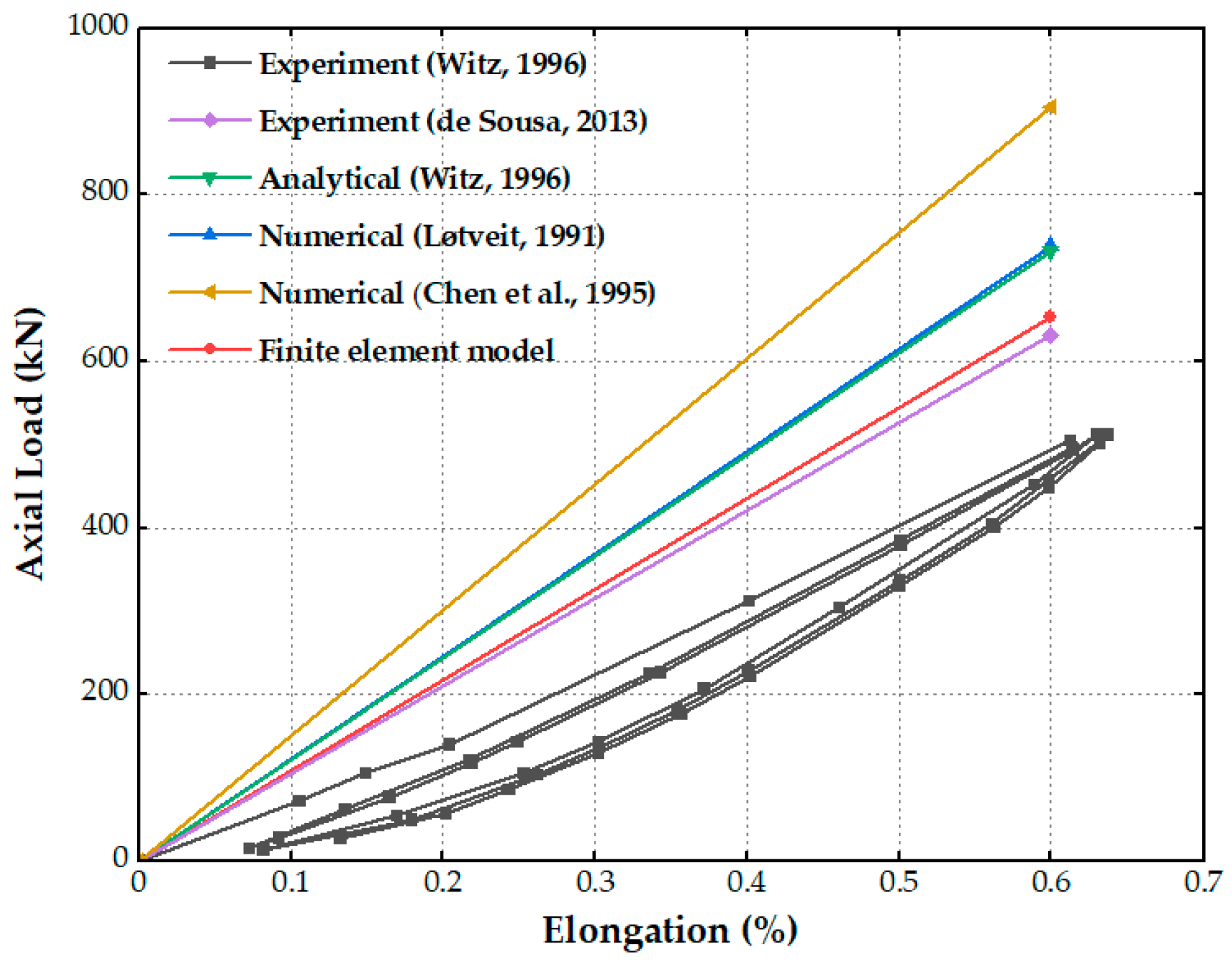
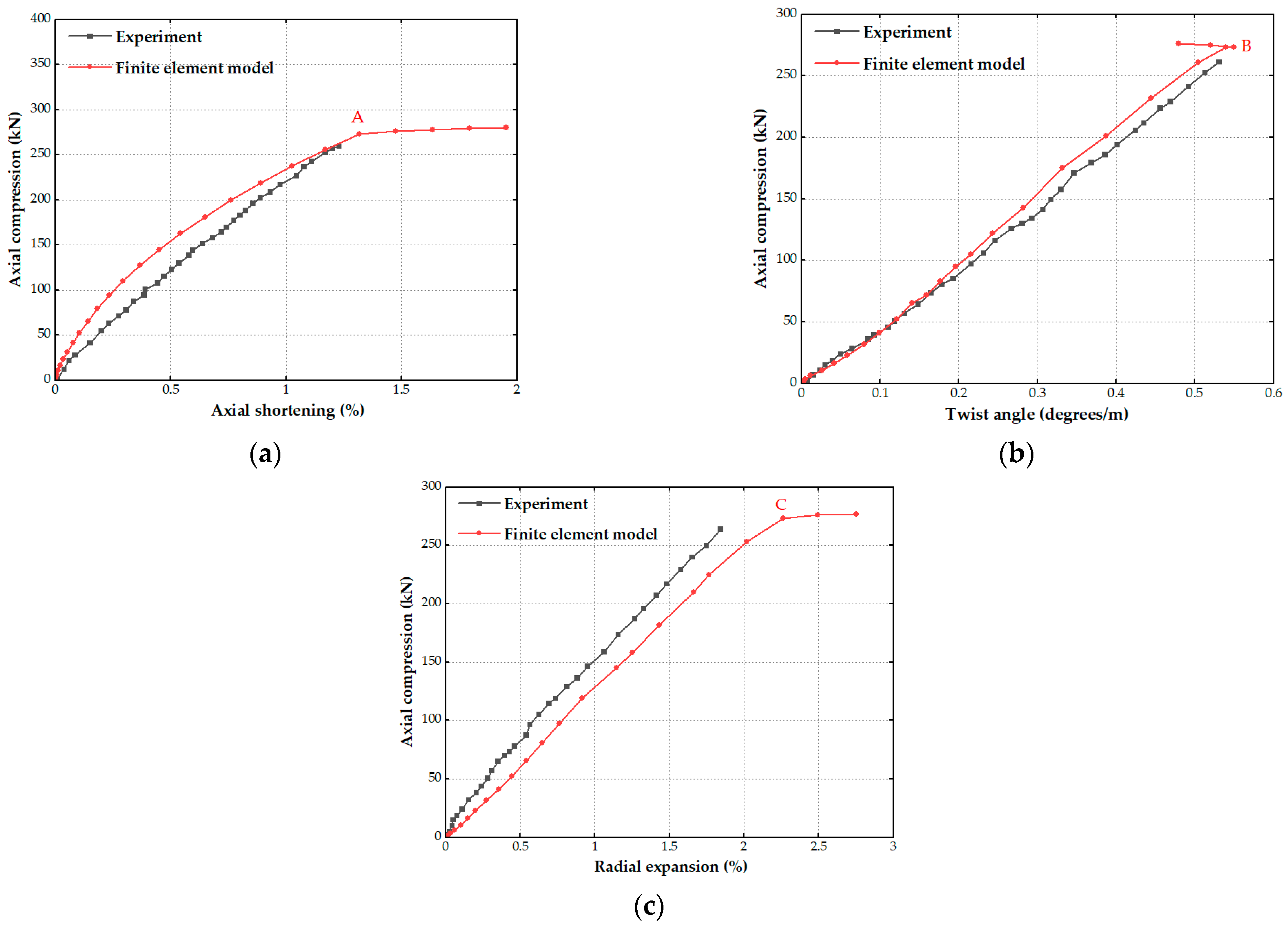
4.1.1. Model Verification

Table 4. Axial tensile stiffness obtained by different scholars.

Scholar	$\mathbf{F}/\mathsf{\epsilon }$ (MN)	References
Witz	91.19	Experiment (Witz, 1996) [38]
de Sousa	105.1	Experiment (de Sousa, 2012) [37]
Witz	123.33	Analytical (Witz, 1996) [38]
Seaflex	122	Numerical (Løtveit, 1991) [39]
Wellstream	151	Numerical (Chen et al., 1995) [40]
	108.64	Finite element model (This article)

shows some representative results. In this paper, the 3D finite element model is established by ABAQUS, and the numerical simulation results are compared with these results and experimental data to verify the accuracy of the numerical model. The tensile stiffness mentioned in the paper refers to the equivalent tensile stiffness, which can be expressed as: ${\mathrm{K}}_{\mathrm{tensile}}=\mathrm{F}/\mathsf{\epsilon }$.

Under the condition that the end is allowed to twist, the tensile strain curve of the 2.5-inch flexible riser measured experimentally is shown in Figure 6, including three loading periods in total. It can be seen that the curve presents weak nonlinearity with certain hysteresis behavior, and the axial stiffness of the hose (i.e., the slope of the curve) is relatively small when the strain is low, and larger when the strain is higher. The test curve of the subsequent loading cycle is different from that of the first cycle, which is common in the test of the flexible riser and is mainly attributed to the winding process of making the spiral strip.

Figure 6 shows that both theoretical analysis and numerical simulation results exhibit a linear trend. However, the axial stiffness values obtained from these methods are generally higher than those obtained from experimental measurements. Numerical simulations often result in stiffness values that are greater than those obtained in experiments. There are several reasons for this. Firstly, numerical simulations typically involve simplifications and assumptions to make the calculations computationally feasible. These simplifications may not fully capture the complexities and non-linearities of the physical system, leading to an overestimation of stiffness. Secondly, the boundary conditions used in numerical simulations may not perfectly match the experimental conditions, leading to differences in stiffness. Thirdly, the material properties used in numerical simulations may not accurately reflect the properties of the actual materials, leading to differences in stiffness between the simulated and actual systems. Finally, there may be errors or uncertainties in the experimental measurements of stiffness that contribute to the difference between the numerical and experimental stiffness values. The measured quantity in the experiment is relatively small compared to the length of the pipe, and a minor deviation may significantly affect the final measurement outcome.

Figure 7 illustrates the axial stress distribution of the entire pipe and the tensile armor layers in different flexible pipe models under tensile conditions. The results show that the two tensile armor layers primarily bear the tensile load of the pipeline, while the other layers contribute very little to bearing the load. Additionally, a comparison of the stress distribution in the two tensile armor layers reveals that the axial stress in the inner layer is slightly higher than that in the outer layer. This is primarily due to the fact that the number of armored steel wires in the inner layer is less than that in the outer layer, resulting in higher axial stress in the inner armored layer.

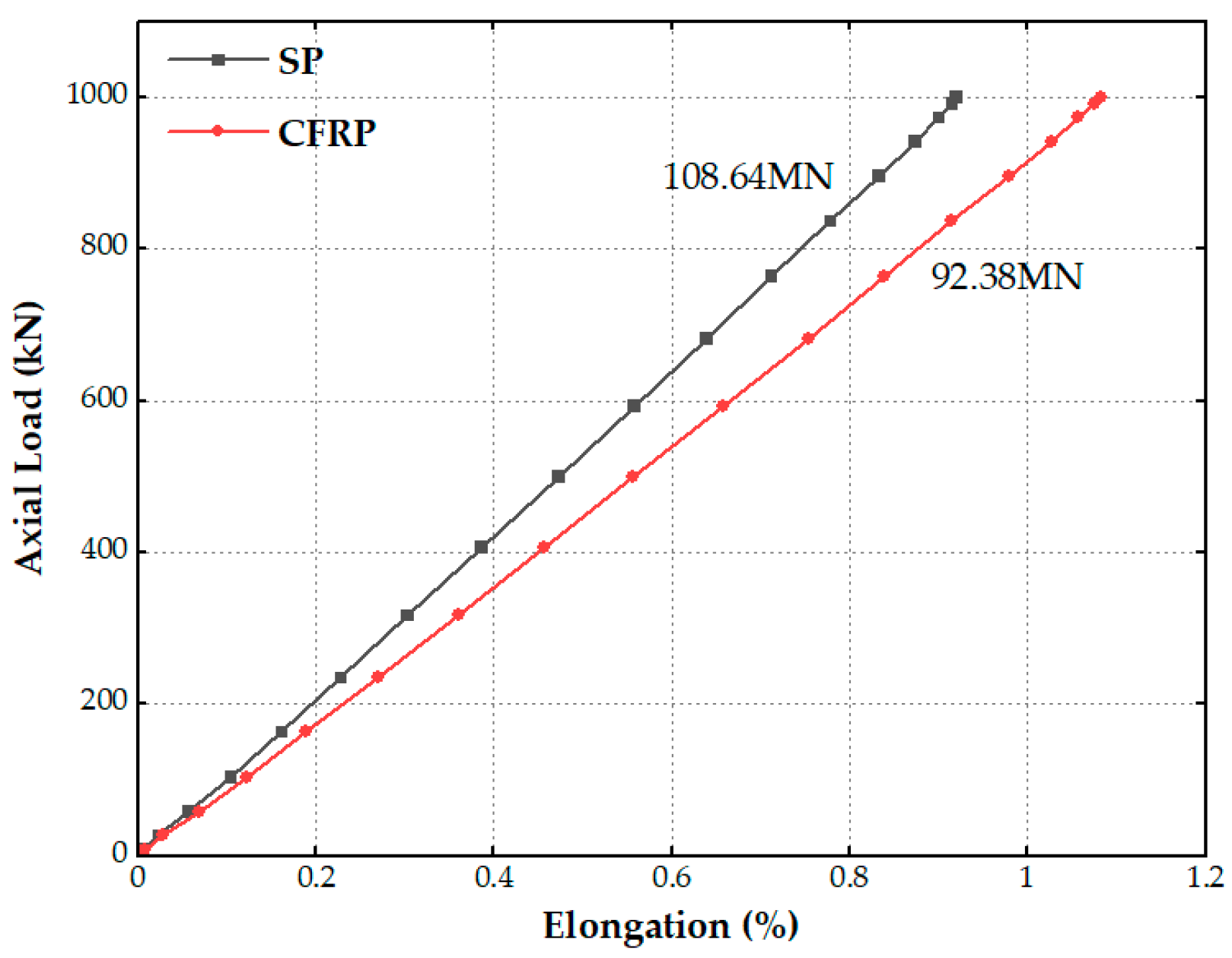
4.1.2. Carbon Fiber Composite Materials Armored Flexible Pipe

The mechanical properties of composite materials are primarily determined by the fibers used. For comparison with steel armored flexible pipes, the high-modulus carbon fiber composite armored strip was selected. Carbon-fiber-reinforced composite materials are only 20% as dense as conventional steel, making them a highly effective weight-saving alternative with a reduction of up to 80%. To predict the axial stiffness of the carbon-fiber-reinforced material armored flexible pipe (CFRP), the numerical model validated in Section 4.1.1 is used in this section. The results of the numerical simulations are compared with those of the traditional steel armored flexible pipe (SP).

As can be seen from Figure 8, the tensile stiffness of the CFRP riser is very close to that of the steel riser, and the axial stiffness only decreases by 14.94% when the weight loss is close to 80%. Marine flexible risers need strong tensile performance. With the increase in the depth of ocean exploration, the vertical load increases due to the self-weight of the riser, which becomes the main limiting factor. The application of carbon fiber in the deep sea can greatly reduce the axial tensile load of the riser without reducing the axial height of the riser, which increases its application prospects in the deep sea.

4.1.3. Ultimate Tensile Strength

Axial ultimate tensile load is also an important index to evaluate the performance of flexibility vertically. When studying the axial stiffness of the flexible riser, the excessive strain of the flexible riser will cause a self-locking phenomenon of the carcass layer, leading to a significant increase in axial stiffness. The results show that the interlocking of carcasses occurs at about 4% longitudinal elongation [48].

Figure 9 shows the failure behavior of two risers. The numerical simulation results show that at point P1 of SP, the inner steel tensile armor wire enters the plastic deformation stage from the elastic stage, and the axial stiffness of the riser decreases. At point P2, the outer tensile armor wires also enter the plastic stage. The steel armored flexible riser had reached its tensile strength limit at the F1 spot at which point the standpipe axial strain was 1.72% and the riser failed. Compared to SP, the failure of the CFRP showed a completely linear behavior, which was attributed to the high brittleness of fiber reinforcement material, leading to no plastic behavior during deformation. When the axial strain of the riser reached 2.157%, the CFRP flexible riser failed. The CFRP has slightly less axial tensile stiffness than the SP at the same section size. The ultimate tensile strength of CFRP during simulations was 2083 kN, which is 1.29 times the ultimate tensile load of SP (1620 kN). Therefore, CFRP has a better tensile capacity and can work in a higher tension load environment.

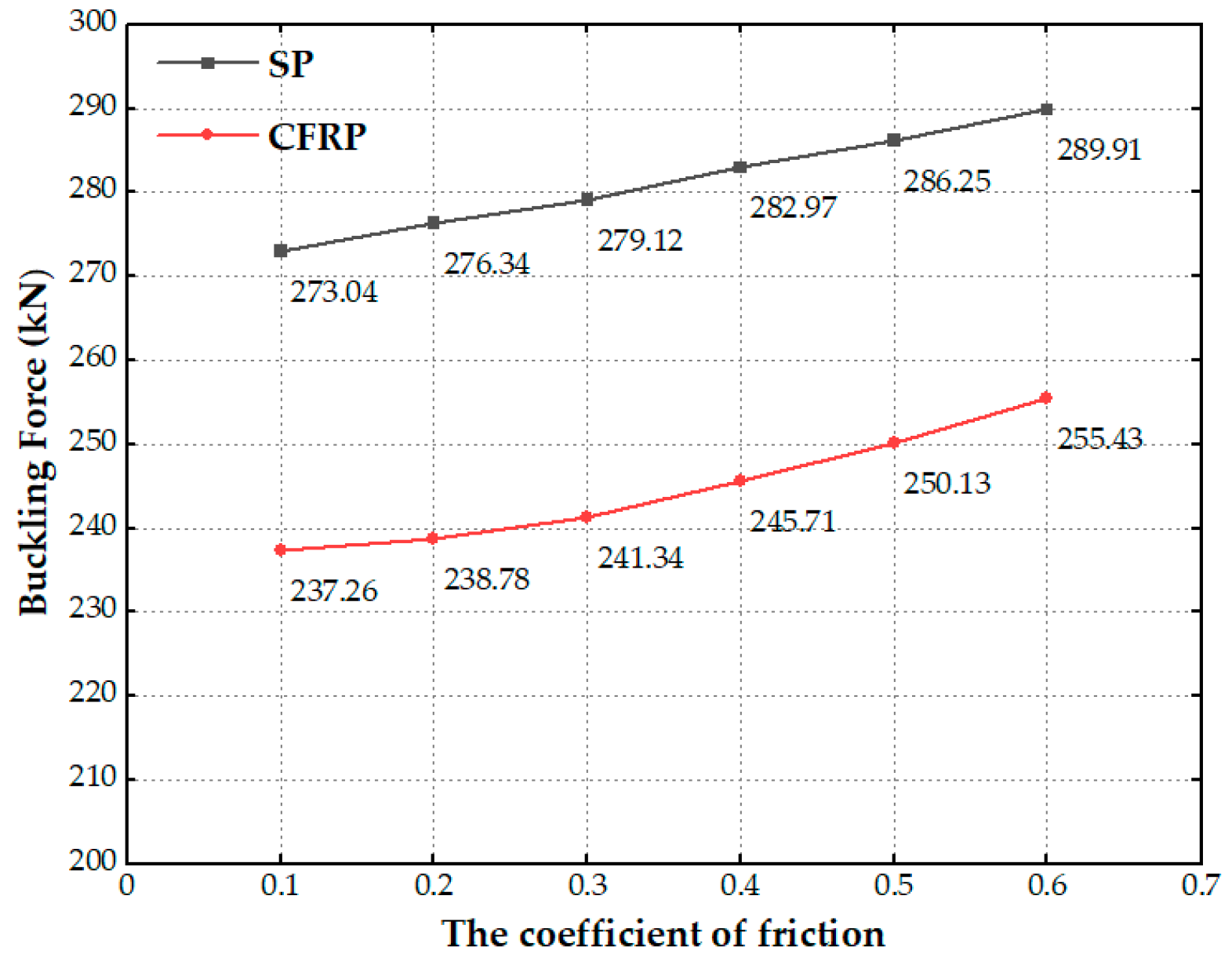
4.1.4. Effect of Coefficient Friction

As can be seen from Figure 10, the friction coefficient between layers does not affect the axial stiffness of the riser basically when the axial tensile response is studied. This is because the axial stiffness of the riser is mainly provided by the tensile armor layer, and the other layers rarely bear the axial tensile load. Therefore, the influence of the friction coefficient can be neglected in the simulation of axial tensile response.

4.2. Axial Compression Response

For deep-water flexible riser systems, the riser is bearing axial load, bending, and pressure during installation and operation, which may lead to local buckling. As this is a complex mechanical response with inter-layer contact between layers of the flexible riser, previous studies adjust for the buckling of steel tensile armor layers. With the gradual application of carbon-fiber-reinforced materials in the deep sea, using CFRP instead of steel tensile belts has practical applications. It is necessary to study the buckling of carbon fiber tensile armor layers. This part aims to use the finite element simulation software ABAQUS to analyze the flexion resistance of the carbon fiber tensile armor layer, and evaluate the flexion resistance of CFRP by comparing them with SP.

To confirm the accuracy and dependability of the theoretical model described in this work, a typical 4-inch unbonded flexible pipe is used as an illustration. First, de Sousa’s experimental data [18] were used to calibrate the FE modeling processes. The geometrical and material parameters are shown in

Table 5. Characteristics of the analyzed 4-inch (101.6 mm) flexible pipe.

Layer	Material Type	Properties	Element Type
Carcass	Stainless steel	t = 4.0 mm; E = 193 GPa; v = 0.3	C3D8I
Inner sheath	Polyamide 11	t = 5.0 mm; E = 345 MPa; v = 0.3	C3D8I
Pressure armor	Carbon steel	t = 6.2 mm; E= 205 GPa; v = 0.3	C3D8I
Tensile armor 1	CFRP(T300)	t = 2 mm; Lay angle = 35°	C3D8I
Tensile armor 2	CFRP(T300)	t = 2 mm; Lay angle = −35°	C3D8I
High strength tape	Aramid fiber	t = 1.2 mm; v = 0.3	C3D8I
Outer sheath	Polyamide 11	t = 2 mm; E = 345 MPa; v = 0.3	C3D8I

, and carbon fiber still uses the Table 3 model, T300 carbon fiber. ABAQUS/Explicit is used to avoid nonconvergent results. Create two reference points at the center of the cross-section at both ends, adopt kinematic coupling at the end of the layer, and apply all loads and boundary conditions to the reference point, as shown in Figure 11. There is no unified specification for selecting the contact friction coefficient of flexible pipe, and we set it as 0.1. For normal contact behavior, we choose the default ‘hard contact’ in the software.

Radial buckling under axial compression load is actually a local instability effect. It is manifested in the sudden decrease in axial stiffness, sudden change in the torsion angle of the stable armored wire, and sudden increase in radial displacement. A comparison of the axial compression-shortening curves from the FEA and the test is shown in Figure 12a. A good correlation is achieved. Initially, for very low loads, flexible pipes have similar stiffness as when they are subjected to axial tension, so the deformation rate produced by the load is very small. As the load increases, the stiffness decreases when the interlayer gap begins to form. When the axial expression load of the FEA is up to 273.04 kN, radial buckling of the tensile armor layer occurs, which corresponds to point A in Figure 12a.

In addition, when the steel tension armor wire is unstable, its torsion direction will change. In this paper, the expression load applied end node is extracted to establish the relationship between the axial compression load and torsion angle per unit length. At point B in Figure 12b, the tensile armor layers buckled, and the corresponding compression load was 273.04 kN. Radial buckling is a local effect, and the reference point at the end selected may have some difference from the actual local measurement date. The axial compression-radial expansion curves from FEA are also consistent with the experimental results, as shown in Figure 12c.

Compared with the experimental data, the finite element model in this paper has the same trend with only a small difference. The error of radial buckling load between FEA and experimental data is only 3.82%, as shown in

Table 6. Difference between finite element model and experiment.

	Buckling Force (kN)	Axial Strain (%)	Twist Angle (deg/m)
Experiment (de Sousa)	263	1.21	0.53
Finite element model	273.04	1.31	0.55
Difference	3.82%	8.26%	3.77%

. The comparison shows that this model is effective for the prediction of riser buckling. Figure 12 and Table 6 demonstrate that the finite element model aligns well with the experimental test in terms of axial force and displacement response. The model is still considered to be consistent with the experiment. The discrepancies observed between the two can be attributed to variations in the material properties, interlayer friction, and contact interactions of the carcass and pressure armor layers. Furthermore, data uncertainty may also arise from the limited information regarding the test procedures and measurements provided by de Sousa, as well as some simplifications made during the numerical simulation process. Nevertheless, the numerical model’s accuracy is still deemed satisfactory for the purposes of this study.

4.2.1. Carbon Fiber Composite Materials Armored Flexible Pipe

Figure 13a presents the axial shortening of different material armored risers under axial compression force. At the beginning of load application, the steel armored pipe (SP) has greater axial stiffness compared to carbon fiber composite materials armored pipe (CFRP) because steel has a larger modulus. As the load gradually increases, the transverse movement of steel tensile armored strips occurs because the interlayer friction is insufficient to support the load. Carbon fiber materials armored strips have a low lateral modulus, and the armor wires move laterally when the load is applied, so the stiffness of CFRP is linear, showing a different trend compared with SP. SP and CFRP begin to buckle radially at points A1 and A2. The axial strain increases rapidly after buckling, and the buckling loads are 273.04 kN and 237.26 kN, respectively. Compared with CFRP, SP has better buckling resistance.

Figure 13b presents the change in twist angle per unit length at the end of the pipe under compressive load. The twist angle of SP changes linearly before radial buckling, while the twist angle of CFRP changes nonlinearly due to the anisotropic material parameters. Radial buckling of SP and CFRP occurs at points B1 and B2, respectively. As identified in Figure 13b, at the early stage of loading, SP and CFRP have similar radial displacements. With the increase in load, the radial stiffness of SP is higher than that of CFRP, and the increase rate in the radial displacement of SP is smaller than that in CFRP. CFRP is subjected to radial buckling failure under smaller compression loads, which correspond to points C1 and C2 in Figure 13c.

4.2.2. Effect of Hydrostatic Pressure

Flexible risers operate in deep water, where the outer side of the pipe is subjected to the hydrostatic pressure of seawater. The effect of hydrostatic pressure on the outer sheath prevents the radial movement of the flexible riser and increases the contact pressure between layers to increase the friction between layers. In this section, the flexible riser is subjected to a combined load of external pressure and axial compression to evaluate the anti-buckling ability of the riser under external hydrostatic pressure.

Figure 14 presents the axial compression response under different external hydrostatic pressures. The hydrostatic force is applied to the outer sheath, which restrains the radial deformation of the flexible risers, CFRP and SP. Therefore, the axial stiffness of CFRP and SP also increases with the increase in external pressure. When the external pressure increases from 0 MPa to 2 MPa, the axial stiffness of the pipeline increases significantly at the initial stage of load loading, and CFRP and SP have a larger buckling load at 2 MPa. However, as the external pressure increases further, the stiffness of the flexible riser changes very little, with only a small increase. With the change in external pressure, CFRP and SP have the same trend. CFRP has a small axial stiffness, and its stiffness increases more obviously under hydrostatic pressure.

4.2.3. Effect of Coefficient Friction

In the previous study, it was found that the interlayer friction coefficient had no effect on the axial tensile stiffness of the flexible riser. However, in this section, we investigate the effect of the interlayer friction coefficient on the compression response of the riser. As shown in Figure 15, as the interlayer friction coefficient increases, the transverse deformation of the steel tensile armor wires is inhibited, and the occurrence of radial buckling nodes is delayed, which results in an increase in the critical buckling load of the riser. The critical buckling loads of both the CFRP and SP show a linear increase.

5. Conclusions

This study aims to investigate the mechanical behavior of unbonded flexible risers with composite armor layers under axial tension and compression. To simulate the instability of unbonded flexible pipe armor wires under axial compression, a non-linear finite element model is presented in this work. Furthermore, the effect of external hydrostatic pressure on the buckling mechanism of the flexible riser in a deep-water environment is also explored. The benefits of replacing the steel tensile armor in unbonded flexible pipes with carbon fiber composite materials are discussed. The following conclusions are finally obtained in this paper:

(1)

The model proposed in this article demonstrates its effectiveness in predicting the tensile response of the riser. When utilizing carbon fiber composite material to replace steel in the tensile armor of the flexible riser, the tensile stiffness of the pipe experiences a decrease of only 15% while maintaining the same section size. Moreover, the ultimate tensile stiffness of the composite flexible pipe is significantly increased. The substitution of carbon fiber material results in a 77.7% reduction in the weight of the tensile armor layer;

(2)

The axial tensile rigidity of the flexible riser remains unaffected by the coefficient of friction between layers. However, the coefficient of friction significantly impacts the compression response of the pipe. An increase in the friction coefficient delays the lateral movement of the tensile armor wires, leading to a gradual increase in the critical buckling load of the flexible riser;

(3)

The finite element analysis (FEA) conducted in this study revealed that the failure of the flexible pipe is caused by excessive radial expansion of the tensile armor wires. This type of failure is identified by the rupture of both the high-strength tape and outer sheath layers of the pipe. The consequence is an abrupt reduction in axial stiffness and a rapid increase in radial expansion;

(4)

The radial expansion of the tensile armor is constrained as the hydrostatic pressure increases. Due to the lower radial stiffness of the carbon fiber tensile armor layer, the axial stiffness of the composite flexible riser increases more significantly under external pressure. The critical load of the carbon fiber armor layer can reach up to 86.8% of that of the steel armor layer.

The utilization of composite materials in deep-sea flexible risers has revealed promising prospects for composite flexible risers. The further objectives of this study involve conducting experimental studies on composite flexible risers and considering the progressive damage characteristics of composite materials.