Modes of Weather System-Induced Flows through an Arctic Lagoon
Abstract
:1. Introduction
1.1. Climate Change and Challenges in Studying Arctic Coastal Water
1.2. Estuarine Gravitational, Tidally Induced and Weather-Induced Circulation
1.3. Wind-Driven Circulations in Coastal Embayment
1.4. This Study
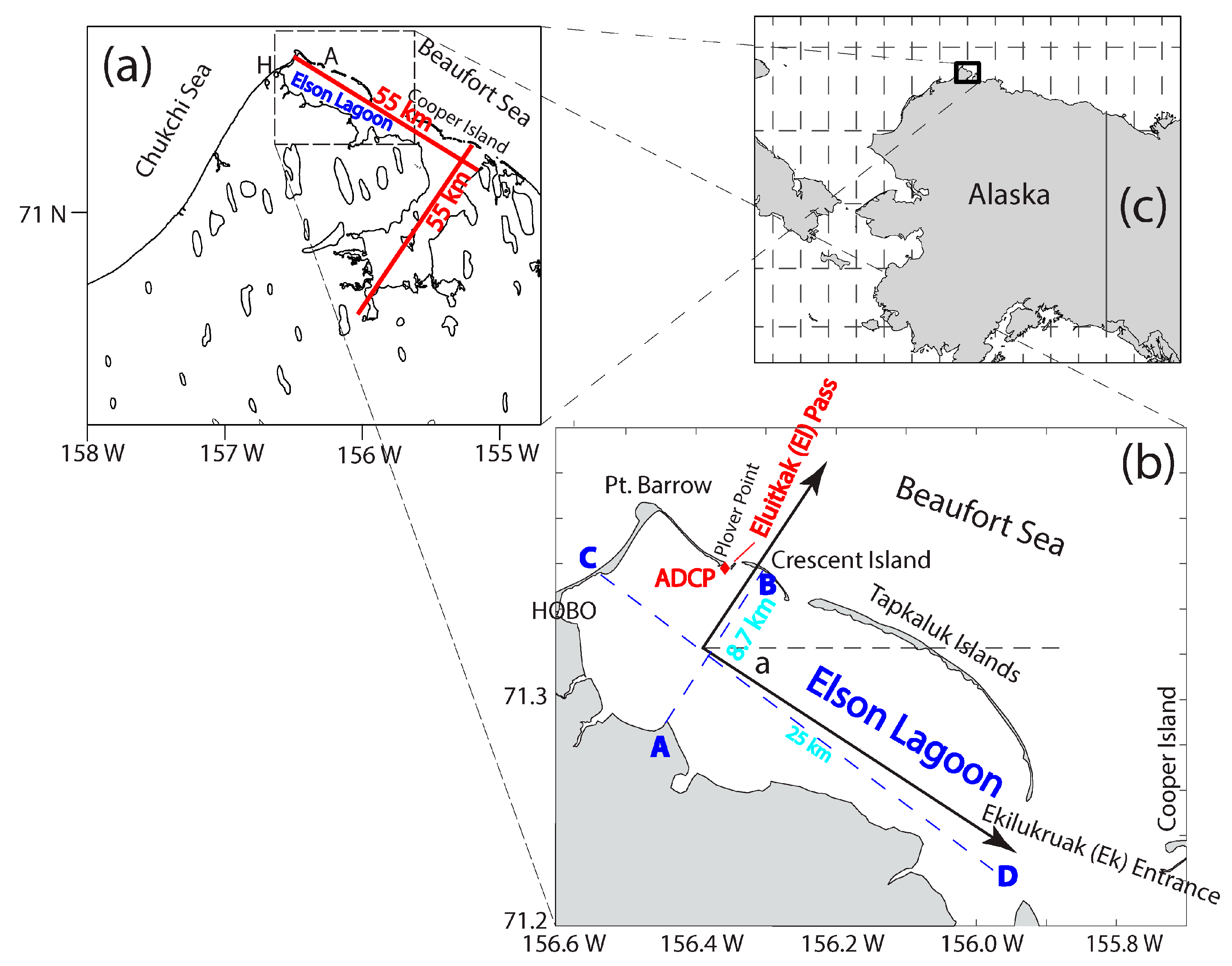
2. Study Site
3. Methods
3.1. Observations
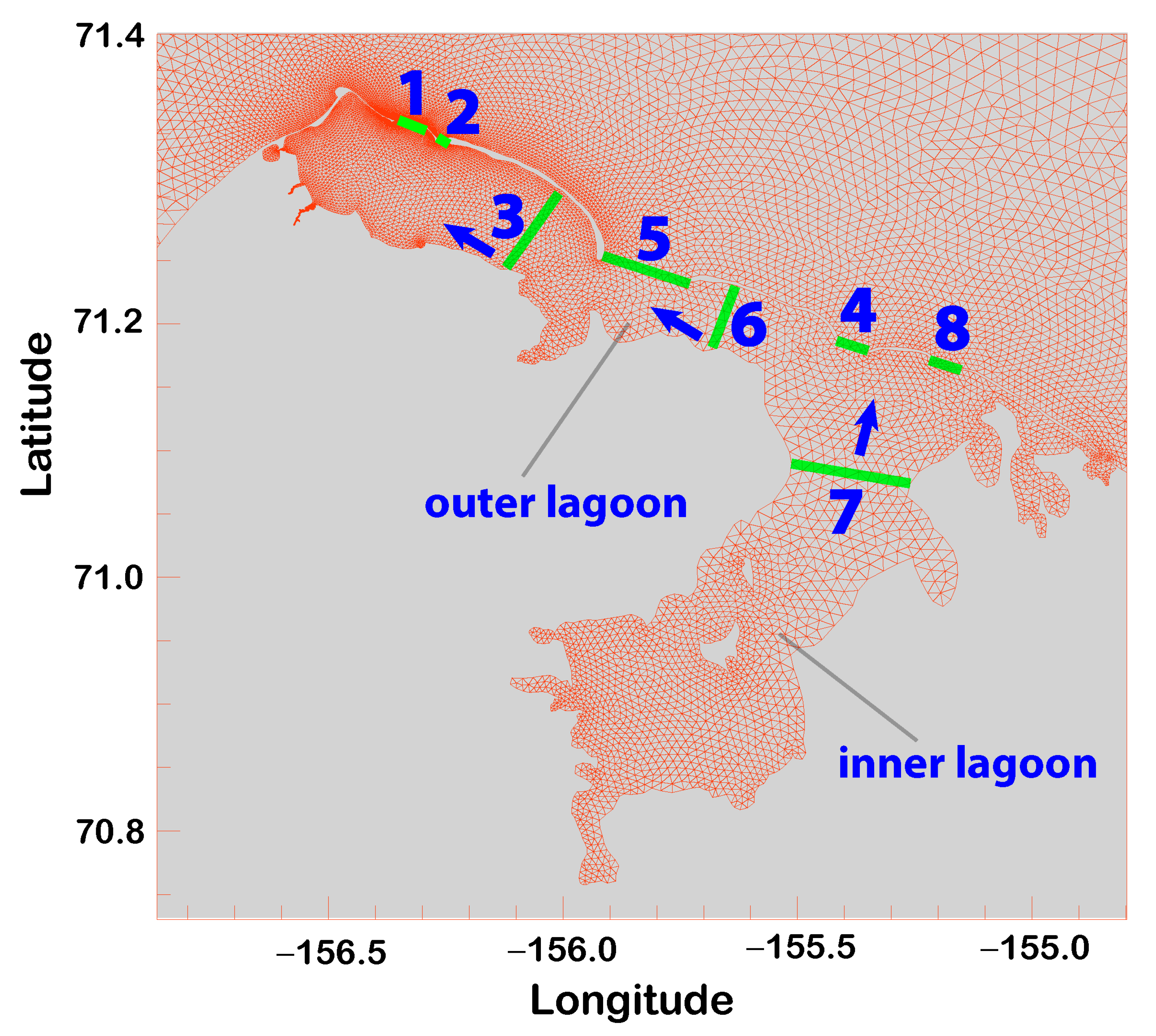
3.2. FVCOM Simulation
3.3. The fr-EOF Analysis
4. Results
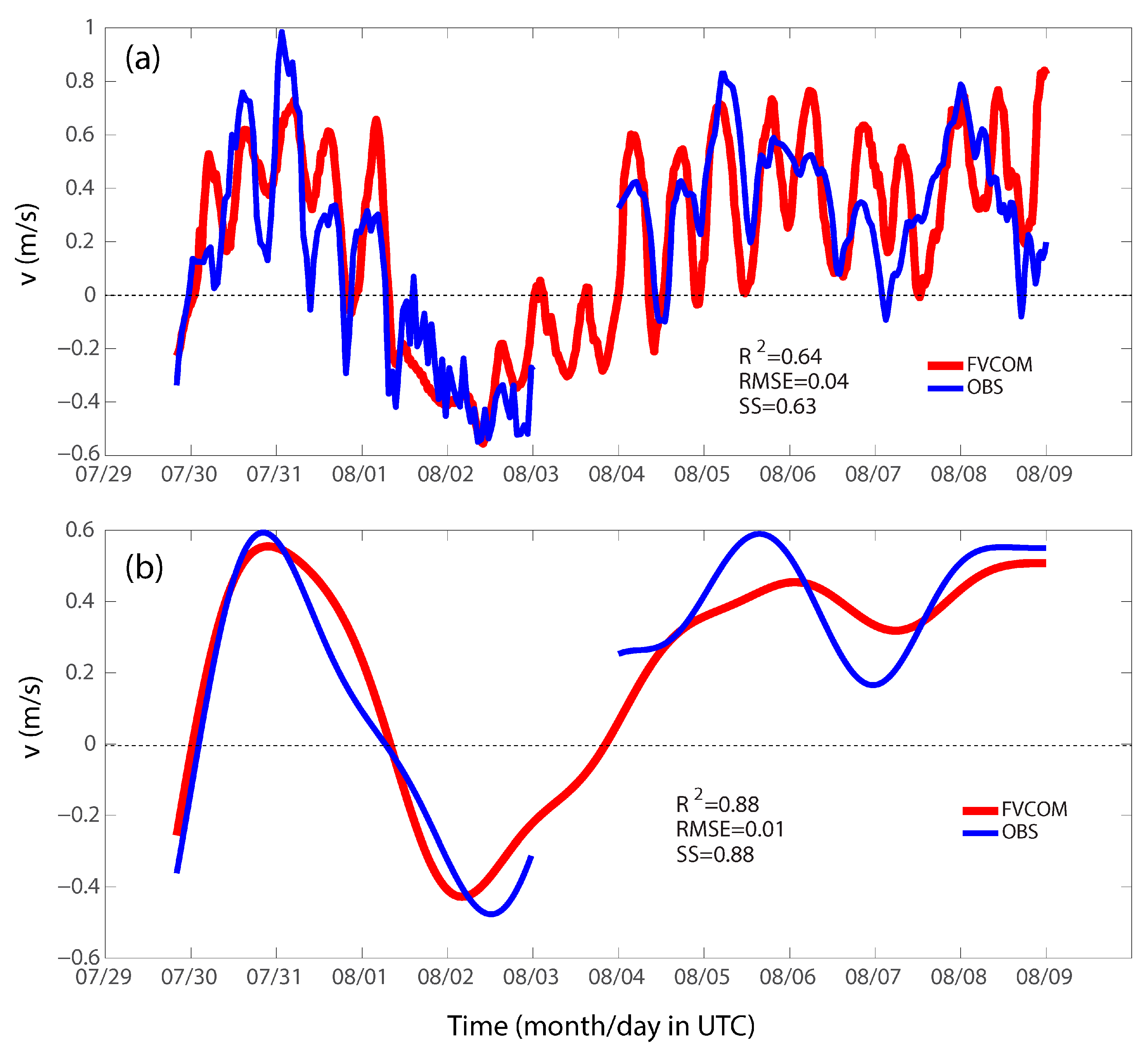
4.1. FVCOM Model-Data Comparison
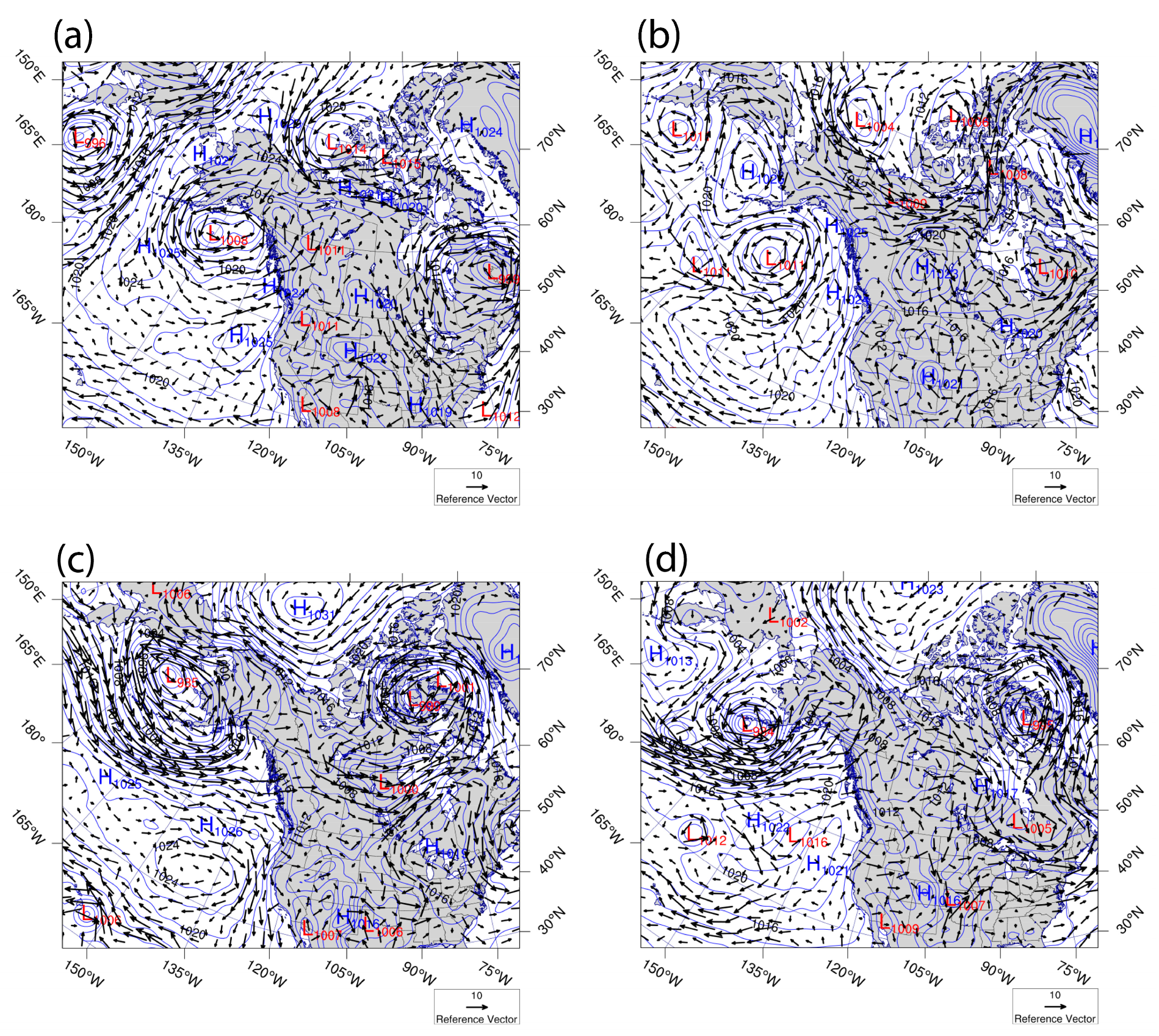
4.2. Weather Conditions and Inlet Flows
4.3. Transport across Eight Transects
4.4. Modes from fr-EOF Analysis
4.5. Conceptual Model for Counter-Wind Flows
5. Discussion
5.1. Significance of Study and Major New Findings
5.2. Meteorological Tides
5.3. Limitations and Future Studies
6. Concluding Remarks
- (1)
- Studies on the exchange flows at the multiple inlets of arctic lagoons must consider the wind variations as part of the arctic weather systems. Despite the complications of weather systems, the dominant Beaufort High (BH) is more common than the transient arctic cyclones. The BH provides a dominant easterly wind at the study site which pushes water into the lagoon (water setup) causing an outward flow at Eluitkak Pass, producing a counter-wind flow. The migrating arctic cyclone on the other hand, usually brings in a westerly wind over the lagoon and pushes the water out (water set-down) of the lagoon through the eastern inlets. This causes an inward flow at Eluitkak Pass, and also a counter-wind flow;
- (2)
- Three major exchange flow modes are identified by this study. The first two modes (accounting for a total of ~96% of the variability) are all counter-wind flows, consistent with the weather system-induced wind-driven flows as concluded in (1);
- (3)
- In addition to the water setup and set down by the direct wind forcing from the weather systems, the wind stress and water depth work together to generate a velocity shear and vorticity tendency. This vorticity effect produces the same type of exchange flow as the water setup and set-down mechanisms in this arctic lagoon. Together they produce an overwhelming counter-wind flow in Eluitkak Pass. In other lagoon systems, however, these two mechanisms may compete with each other, depending on the geometry, such as the orientations of the lagoon and inlets, and the wind directions.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alizadeh, O.; Lin, Z. Rapid Arctic warming and its link to the waviness and strength of the westerly jet stream over West Asia. Glob. Planet. Chang. 2021, 199, 103447. [Google Scholar] [CrossRef]
- You, Q.; Cai, Z.; Pepin, N.; Chen, D.; Ahrens, B.; Jiang, Z.; Wu, F.; Kang, S.; Zhang, R.; Wu, T.; et al. Warming amplification over the Arctic Pole and Third Pole: Trends, mechanisms and consequences. Earth-Sci. Rev. 2021, 217, 103625. [Google Scholar] [CrossRef]
- Kumar, A.; Yadav, J.; Mohan, R. Global warming leading to alarming recession of the Arctic sea-ice cover: Insights from remote sensing observations and model reanalysis. Heliyon 2020, 6, e04355. [Google Scholar] [CrossRef] [PubMed]
- Peterson, B.; Holmes, R.; McClelland, J.; Vörösmarty, C.; Lammers, R.; Shiklomanov, A.; Shiklomanov, I.; Rahmstorf, S. Increasing River Discharge to the Arctic Ocean. Science 2002, 298, 2171–2173. [Google Scholar] [CrossRef] [PubMed]
- Zheng, L.; Overeem, I.; Wang, K.; Clow, G.D. Changing Arctic River dynamics cause localized permafrost thaw. J. Geophys. Res. Earth Surf. 2019, 124, 2324–2344. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, C.; Wang, D.; Cole, R.C.B.S.; Gao; Lin, H. Changing Sources and pathways of freshwater accumulation in the Beaufort Sea of the Arctic Ocean. J. Geophys. Res.-Oceans 2023. in revision. [Google Scholar]
- Proshutinsky, A.; Bourke, R.H.; McLaughlin, F.A. The role of the Beaufort Gyre in Arctic climate variability: Seasonal to decadal climate scales. Geophys. Res. Lett. 2002, 29, 15-1–15-4. [Google Scholar] [CrossRef]
- Proshutinsky, A.; Krishfield, R.; Timmermans, M.L.; Toole, J.M.; Carmack, E.; McLaughlin, F.; Williams, W.J.; Zimmermann, S.; Itoh, M.; Shimada, K. The Beaufort Gyre Fresh Water Reservoir: State and variability from observations. J. Geophys. Res. Oceans 2009, 114, C00A10. [Google Scholar] [CrossRef]
- Rabe, B.; Karcher, M.; Kauker, F.; Schauer, U.; Toole, J.M.; Krishfield, R.A.; Pisarev, S.; Kikuchi, T.; Su, J. Arctic Ocean basin liquid freshwater storage trend 1992–2012. Geophys. Res. Lett. 2014, 41, 961–968. [Google Scholar] [CrossRef]
- Wang, Q.; Wekerle, C.; Danilov, S.; Koldunov, N.; Sidorenko, D.; Sein, D.; Rabe, B.; Jung, T. Arctic Sea Ice Decline Significantly Contributed to the Unprecedented Liquid Freshwater Accumulation in the Beaufort Gyre of the Arctic Ocean. Geophys. Res. Lett. 2018, 45, 4956–4964. [Google Scholar] [CrossRef]
- Chen, C.; Qi, J.; Li, C.; Beardsley, R.C.; Lin, H.; Walker, R.; Gates, K. Complexity of the flooding/drying process in an estuarine tidal-creek salt-marsh system: An application of FVCOM. J. Geophys. Res. 2008, 113, C07052. [Google Scholar] [CrossRef]
- Pritchard, D.W. The dynamic structure of a coastal plain estuary. J. Mar. Res. 1956, 15, 33–42. [Google Scholar]
- Hansen, D.V.; Rattray, M., Jr. Gravitational circulation in straits and estuaries. J. Mar. Res. 1965, 23, 104–122. [Google Scholar] [CrossRef]
- Burchard, H.; Hetland, R.D. Quantifying the Contributions of Tidal Straining and Gravitational Circulation to Residual Circulation in Periodically Stratified Tidal Estuaries. J. Phys. Oceanogr. 2010, 40, 1243–1262. [Google Scholar] [CrossRef]
- Cheng, P.; Valle-Levinson, A.; de Swart, H.E. A numerical study of residual circulation induced by asymmetric tidal mixing in tidally dominated estuaries. J. Geophys. Res. 2011, 116, C01017. [Google Scholar] [CrossRef]
- Cheng, P.; Mao, J.; Yu, F.; Chen, N.; Wang, A.; Xu, F. A numerical study of residual flow induced by eddy viscosity-shear covariance in a tidally energetic estuary. Estuar. Coast. Shelf Sci. 2019, 230, 106446. [Google Scholar] [CrossRef]
- Burchard, H.; Schuttelaars, H.M. Analysis of Tidal Straining as Driver for Estuarine Circulation in Well-Mixed Estuaries. J. Phys. Oceanogr. 2012, 42, 261–271. [Google Scholar] [CrossRef]
- Dijkstra, Y.M.; Schuttelaars, H.M.; Burchard, H. Generation of exchange flows in estuaries by tidal and gravitational eddy viscosity-shear covariance (ESCO). J. Geophys. Res. Ocean. 2017, 122, 4217–4237. [Google Scholar] [CrossRef]
- Ianniello, J.P. Tidally induced residual currents in estuaries of constant breadth and depth. J. Mar. Res. 1977, 35, 755–785. [Google Scholar]
- Barlow, J. Effect of wind on salinity distribution in an estuary. J. Mar. Res. 1956, 15, 193–203. [Google Scholar]
- Xue, P.; Chen, C.; Ding, P.; Beardsley, R.C.; Lin, H.; Ge JY Kong, Y. Saltwater intrusion into the Changjiang River: A model-guided mechanism study. J. Geophys. Res. 2009, 114, C02006. [Google Scholar] [CrossRef]
- Scully, M.E.; Friedrichs, C.; Brubaker, J. Control of Estuarine Stratification and Mixing by Wind-induced Straining of the Estuarine Density Field. Estuaries 2005, 28, 321–326. [Google Scholar] [CrossRef]
- Li, C.; Walker, N.; Hou, A.; Georgiou, I.; Roberts, H.; Laws, E.; McCorquodale, J.A.; Weeks, E.; Li, X.; Crochet, J. Circular Plumes in Lake Pontchartrain Estuary under Wind Straining. Estuar. Coast. Shelf Sci. 2008, 80, 161–172. [Google Scholar] [CrossRef]
- Burchard, H. Combined effects of wind, tide, and horizontal density gradients on stratification in estuaries and coastal seas. J. Phys. Oceanogr. 2009, 39, 2117–2136. [Google Scholar] [CrossRef]
- Juarez, B.; Valle-Levinson, A.; Chant, R.; Li, M. Observations of the lateral structure of wind-driven flow in a coastal plain estuary. Estuar. Coast. Shelf Sci. 2019, 217, 262–270. [Google Scholar] [CrossRef]
- Zhao, L.; Chen, C.; Vallino, J.; Hopkinson, C.; Beardsley, R.C.; Lin, H.; Lerczak, J. Wetland-estuarine-shelf interactions in the Plum Island Sound and Merrimack River in the Massachusetts coast. J. Geophys. Res. Ocean. 2010, 115, C10039. [Google Scholar] [CrossRef]
- Li, C. Subtidal Water Flux through a Multi-inlet System: Observations Before and During a Cold Front Event and Numerical Experiments. J. Geophys. Res.-Ocean. 2013, 118, 1877–1892. [Google Scholar] [CrossRef]
- Welander, P. Wind-driven circulation in one-and two-layer oceans of variable depth. Tellus 1968, 20, 1–16. [Google Scholar] [CrossRef]
- Csanady, G.T. Wind-driven summer circulation in the Great Lakes. J. Geophys. Res. 1968, 73, 2579–2589. [Google Scholar] [CrossRef]
- Garvine, R.W. A simple model of estuarine subtidal fluctuations forced by local and remote wind stress. J. Geophys. Res. 1985, 90, 11945–11948. [Google Scholar] [CrossRef]
- Engelund, F. Steady wind set-up in prismatic lakes. In Environmental Hydraulics: Stratified Flows; Pedersen, F.B., Ed.; Lecture Notes on Coastal and Estuarine Studies; Springer: New York, NY, USA, (originally published in 1973); 1986; Volume 18, pp. 205–212. [Google Scholar]
- Elliott, A.J. Observations of the meteorologically induced circulation in the Potomac Estuary. Estuar. Coast. Mar. Sci. 1978, 6, 285–299. [Google Scholar] [CrossRef]
- Gibbs, M.; Abell, J.; Hamilton, D. Wind forced circulation and sediment disturbance in a temperate lake. N. Z. J. Mar. Freshw. Res. 2016, 50, 209–227. [Google Scholar] [CrossRef]
- Ge, J.; Chen, C.; ZWang, B.; Ke, K.; Yi, J.; Ding, P. Dynamic Response of the Fluid Mud to a Tropical Storm. J. Geophys. Res.-Ocean. 2020, 125, e2019JC015419. [Google Scholar] [CrossRef]
- Fischer, H.B. Mass transport mechanisms in partially stratified estuaries. J. Fluid Mech. 1972, 53, 671–687. [Google Scholar] [CrossRef]
- Duran-Matute, M.; Gerkema, T.; Sassi, M.G. Quantifying the residual volume transport through a multiple-inlet system in response to wind forcing: The case of the western Dutch Wadden Sea. J. Geophys. Res. Ocean. 2016, 121, 8888–8903. [Google Scholar] [CrossRef]
- Li, C.; Huang, W.; Milan, B. Atmospheric Cold Front–Induced Exchange Flows through a Microtidal Multi-Inlet Bay: Analysis Using Multiple Horizontal ADCPs and FVCOM Simulations. J. Atmos. Ocean. Technol. 2019, 36, 443–472. [Google Scholar] [CrossRef]
- Wong, K.-C. On the nature of transverse variability in a coastal plain estuary. J. Geophys. Res. 1994, 99, 14209–14222. [Google Scholar] [CrossRef]
- Chaney, P.L.; Stone, G.W. Soundside erosion of a nourished beach and implications for winter cold front forcing: West Ship Island. Mississippi. Oceanogr. Lit. Rev. 1996, 43, 844. [Google Scholar]
- Weaver, R.J.; Taeb, P.; Lazarus, S.; Splitt, M.; Holman, B.P.; Colvin, J. Sensitivity of modeled estuarine circulation to spatial and temporal resolution of input meteorological forcing of a cold frontal passage. Estuar. Coast. Shelf Sci. 2016, 183, 28–40. [Google Scholar] [CrossRef]
- Appendini, C.M.; Hernández-Lasheras, J.; Meza-Padilla, R.J.; Kurczyn, J.A. Effect of climate change on wind waves generated by anticyclonic cold front intrusions in the Gulf of Mexico. Clim. Dyn. 2018, 51, 3747–3763. [Google Scholar] [CrossRef]
- Fritz, H.; Coauthors, M. Hurricane Katrina storm surge distribution and field observations on the Mississippi Barrier Islands. Estuar. Coast. Shelf Sci. 2007, 74, 12–20. [Google Scholar] [CrossRef]
- Fearnley, S.M.; Miner, M.D.; Kulp, M.; Bohling, C.; Penland, S. Hurricane impact and recovery shoreline change analysis of the Chandeleur Islands, Louisiana, USA: 1855 to 2005. Geo-Mar. Lett. 2009, 29, 455–466. [Google Scholar] [CrossRef]
- Ebersole, B.A.; Westerink, J.J.; Bunya, S.; Dietrich, J.C.; Cialone, M.A. Development of storm surge which led to flooding in St. Bernard polder during Hurricane Katrina. Ocean Eng. 2010, 37, 91–103. [Google Scholar] [CrossRef]
- Li, C.; Boswell, K.M.; Chaichitehrani, N.; Huang, W.; Wu, R. Weather induced subtidal flows through multiple inlets of an arctic microtidal lagoon. Acta Oceanol. Sin. 2019, 38, 1–16. [Google Scholar] [CrossRef]
- Chen, C.; Liu, H.; Beardsley, R.C. An Unstructured Grid, Finite-Volume, Three-Dimensional, Primitive Equations Ocean Model: Application to Coastal Ocean and Estuaries. J. Atmos. Ocean. Technol. 2003, 20, 159–186. [Google Scholar] [CrossRef]
- Chen, C.; Huang, H.; Beardsley, R.C.; Liu, H.; Xu, Q.; Cowles, G. A finite-volume numerical approach for coastal ocean circulation studies: Comparisons with finite difference models. J. Geophys. Res. 2007, 112, C03018. [Google Scholar] [CrossRef]
- Aoki, K.; Isobe, A. Application of Finite Volume Coastal Ocean Model to hindcasting the wind-induced sea-level variation in Fukuoka Bay. J. Phys. Oceanogr. 2007, 63, 333–339. [Google Scholar] [CrossRef]
- Weisberg, R.H.; Zheng, L.Y. Hurricane storm surge simulation for Tampa Bay. Estuaries Coasts 2006, 29, 899–913. [Google Scholar] [CrossRef]
- Weisberg, R.H.; Zheng, L.Y. A simulation of the Hurricane Charley storm surge and its breach of North Captiva Island. Florida. Sci. 2006, 69, 152–165. [Google Scholar]
- Chen, C.; Gao, G.; Qi, J.; Proshutinsky, A.; Beardsley, R.C.; Kowalik, Z.; Lin, H.; Cowles, G. A new high-resolution unstructured-grid finite-volume Arctic Ocean model (AO-FVCOM): An application for tidal studies. J. Geophys. Res.-Ocean. 2009, 114, C0807. [Google Scholar] [CrossRef]
- Chen, C.; Gao, G.; Zhang, Y.; Beardsley, R.C.; Lai, Z.; Qi, J.; Lin, H. Circulation in the Arctic Ocean: Results from a high-resolution coupled ice-sea nested Global-FVCOM and Arctic-FVCOM system. Prog. Oceanogr. 2016, 141, 60–80. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, C.; Beardsley, R.C.; Gao, G.; Lai, Z.; Curry, B.; Lee, C.M.; Lin, H.; Qi, J.; Xu, Q. Studies of the Canadian Arctic Archipelago water transport and its relationship to basin-local forcings: Results from AO-FVCOM. J. Geophys. Res.-Ocean. 2016, 121, 4392–4415. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, C.; Beardsley, R.C.; Gao, G.; Qi, J.; Lin, H. Seasonal and interannual variability of the Arctic sea ice: A comparison between AO-FVCOM and observations. J. Geophys. Res.-Ocean. 2016, 121, 8320–8350. [Google Scholar] [CrossRef]
- Zhang, Y.; Chen, C.; Beardsley, R.C.; Perrie, W.; Gao, G.; Zhang, Y.; Qi, J.; Lin, H. Applications of an unstructured grid surface wave model (FVCOM-SWAVE) to the Arctic Ocean: The interaction between ocean waves and sea ice. Ocean Model. 2020, 145, 101532. [Google Scholar] [CrossRef]
- Mellor, G.L.; Yamada, T. Development of a turbulence closure model for geophysical fluid problem. Rev. Geophys. Space Phys. 1982, 20, 851–875. [Google Scholar] [CrossRef]
- Galperin, B.; Kantha, L.H.; Hassid, S.; Rosati, A. A quasi-equilibrium turbulent energy model for geophysical flows. J. Atmos. Sci. 1988, 45, 55–62. [Google Scholar] [CrossRef]
- Large, W.; Pond, S. Open ocean momentum flux measurements in moderate to strong winds. J. Phys. Oceanogr. 1981, 11, 324–336. [Google Scholar] [CrossRef]
- Emery, W.J.; Thomson, R.E. Data Analysis Methods in Physical Oceanography, 2nd ed.; Elsevier: New York, NY, USA, 2004; p. 638. [Google Scholar]
- Murphy, A.H. Skill scores based on the mean square error and their relationships to the correlation coefficient. Mon. Weather Rev. 1988, 116, 2417–2424. [Google Scholar] [CrossRef]
- Allen, J.I.; Somerfield, P.J.; Gilbert, F.J. Quantifying uncertainty in high-resolution coupled hydrodynamic-ecosystem models. J. Mar. Syst. 2007, 64, 3–14. [Google Scholar] [CrossRef]
- Ralston, D.K.; Geyer, W.R.; Lerczak, J.A. Structure, variability, and salt flux in a strongly forced salt wedge estuary. J. Geophys. Res. 2010, 115, C06005. [Google Scholar] [CrossRef]

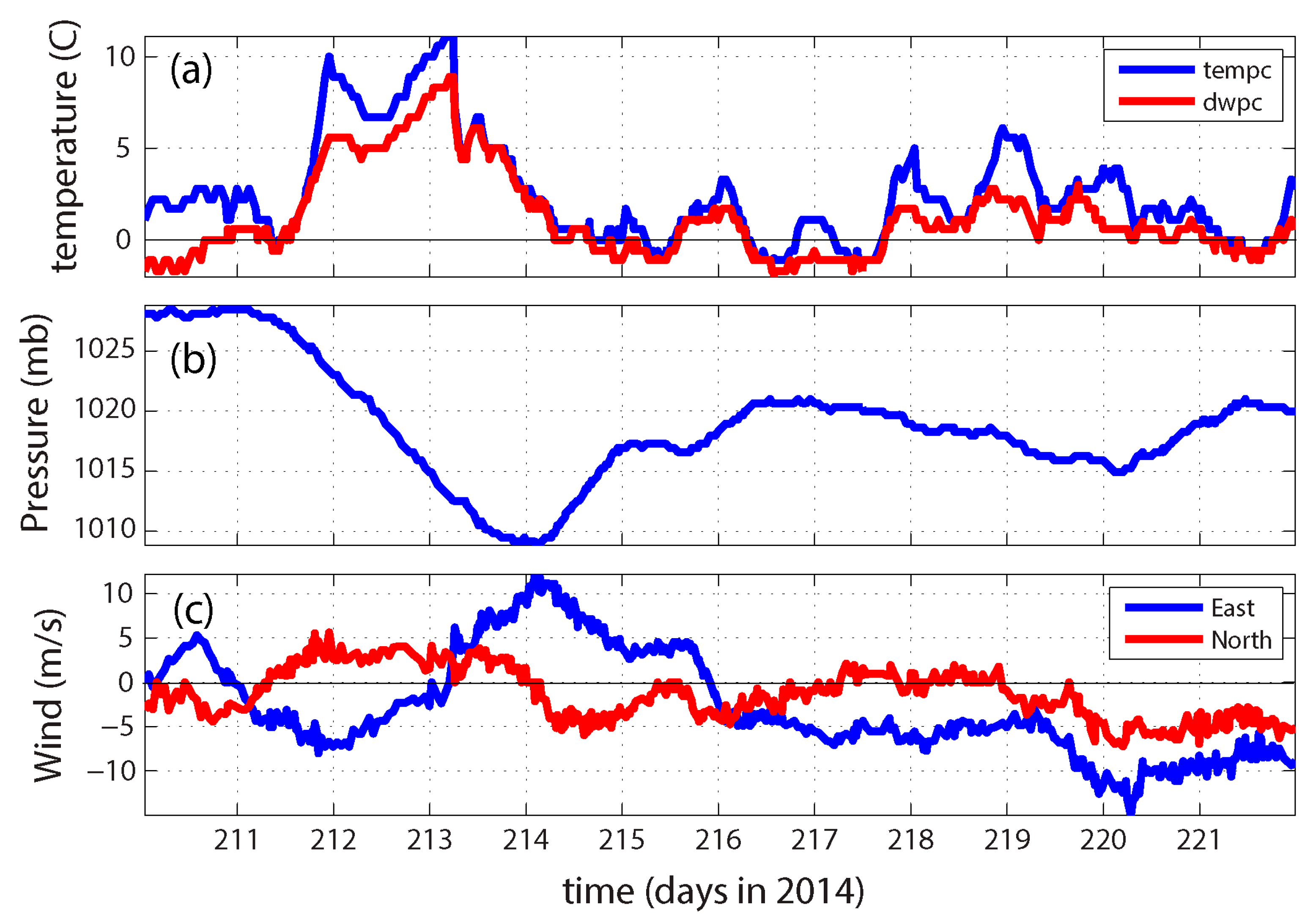
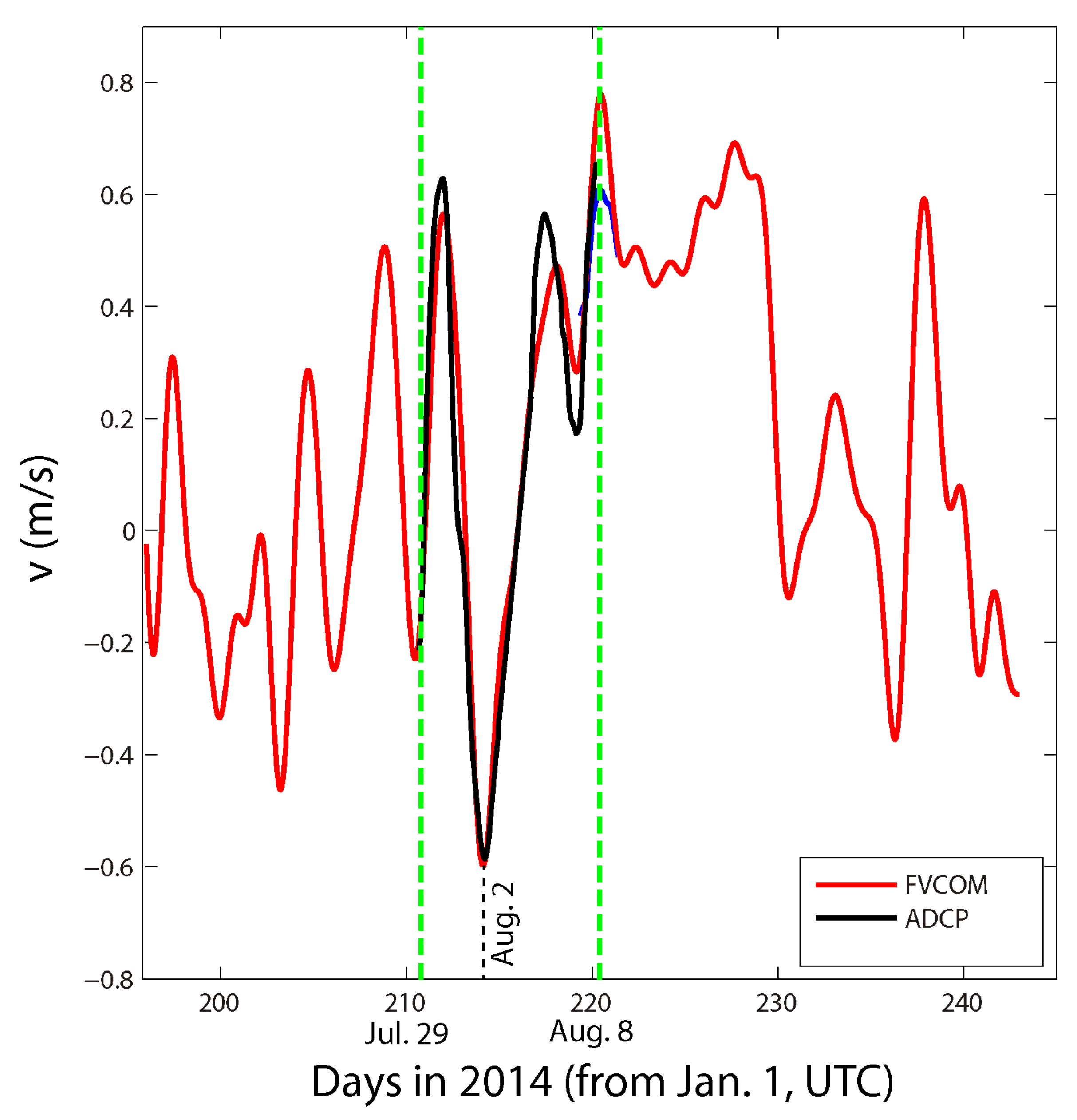
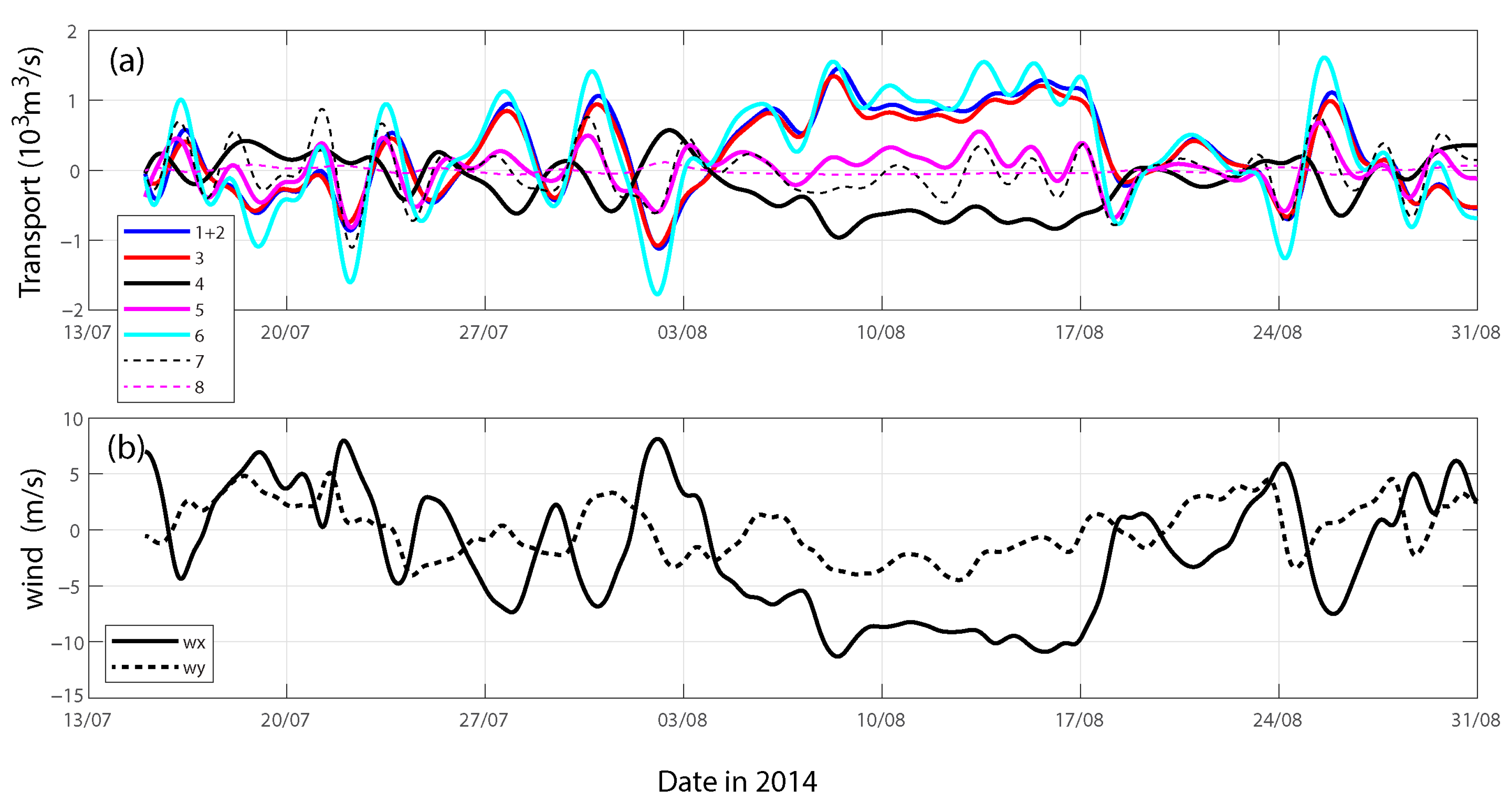
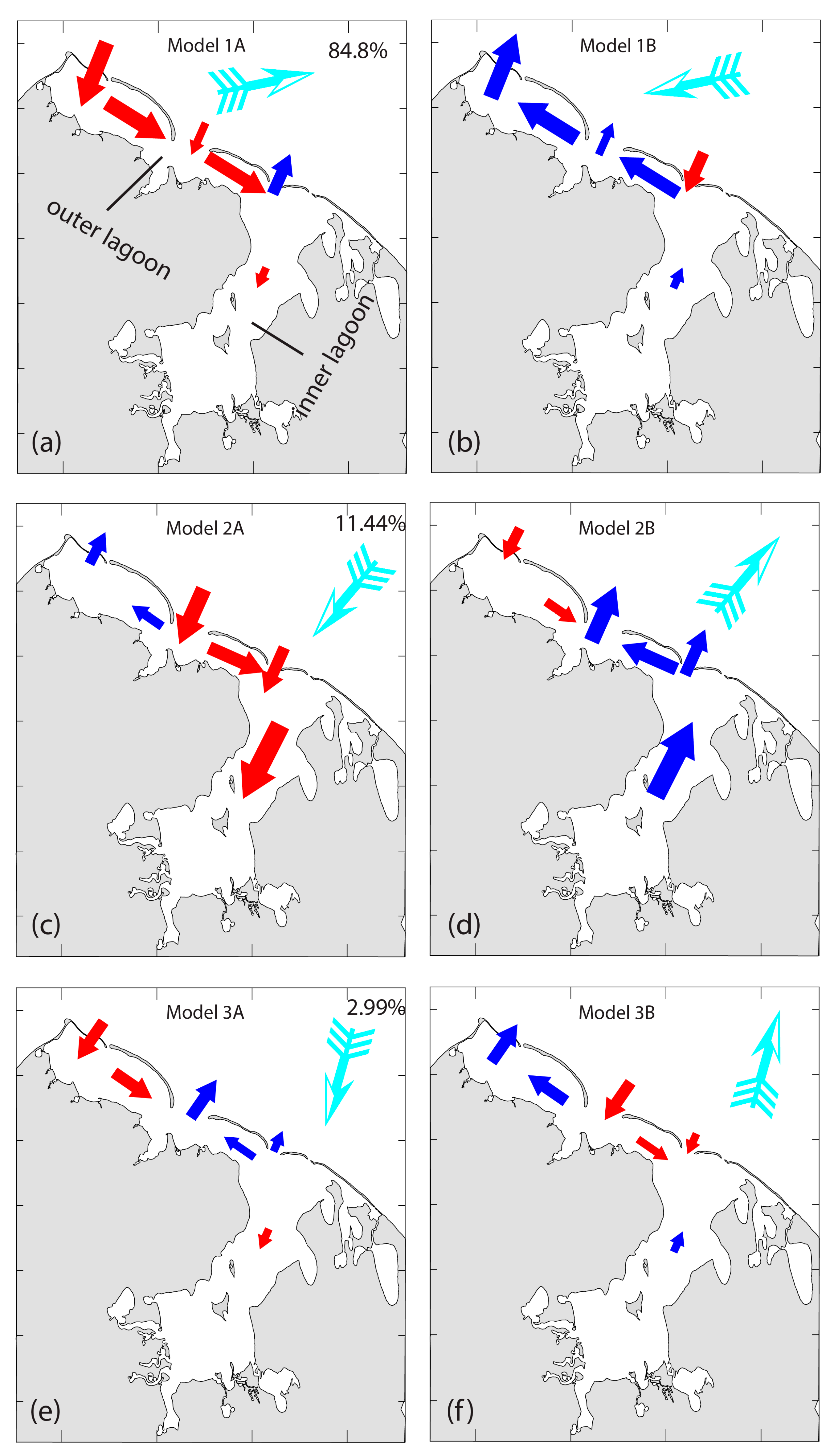


| Deploy # | Time Valid Data Starts | Deployed at Longitude Latitude | Time Valid Data Ends | Recovered at Longitude Latitude | Data File Name | Location of Deployment |
|---|---|---|---|---|---|---|
| 1 | 29 July 2014 | 156.3561° W 71.3593° N | 8/3, 01:29 | - | Elson2014-1 | Eluitkak Pass |
| 2 | 4 August 2014 | 156.3561° W 71.3593° N | 8/18, 21:01 | 156.3960° W 71.3749° N | Elson2014-2 Elson2014-3 | Eluitkak Pass |
| Correlation Coefficient | T2 | T3 | T4 | T5 | T6 | T7 | T8 | wx | wy | wxr | wyr |
|---|---|---|---|---|---|---|---|---|---|---|---|
| T1 | 0.973 | 0.996 | −0.918 | 0.592 | 0.943 | 0.181 | −0.910 | −0.974 | −0.276 | −0.977 | −0.792 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Huang, W.; Chen, C.; Boswell, K.M.; Wu, R. Modes of Weather System-Induced Flows through an Arctic Lagoon. J. Mar. Sci. Eng. 2024, 12, 767. https://doi.org/10.3390/jmse12050767
Li C, Huang W, Chen C, Boswell KM, Wu R. Modes of Weather System-Induced Flows through an Arctic Lagoon. Journal of Marine Science and Engineering. 2024; 12(5):767. https://doi.org/10.3390/jmse12050767
Chicago/Turabian StyleLi, Chunyan, Wei Huang, Changsheng Chen, Kevin M. Boswell, and Renhao Wu. 2024. "Modes of Weather System-Induced Flows through an Arctic Lagoon" Journal of Marine Science and Engineering 12, no. 5: 767. https://doi.org/10.3390/jmse12050767
APA StyleLi, C., Huang, W., Chen, C., Boswell, K. M., & Wu, R. (2024). Modes of Weather System-Induced Flows through an Arctic Lagoon. Journal of Marine Science and Engineering, 12(5), 767. https://doi.org/10.3390/jmse12050767









