A Methodology to Evaluate the Real-Time Stability of Submarine Slopes under Rapid Sedimentation
Abstract
:1. Introduction
2. Study Area
3. Methodology
3.1. Governing Equations in the Sedimentation Process
3.2. Implementation, Validation, and Calibration of the Sedimentation Process
3.3. The Modified Cam-Clay (MCC) Model
3.4. Stability Analysis Methods for Sedimenting Slopes
4. Sedimentary Recurrence and Stability Analysis
4.1. Modelling and Parameterization
4.2. Calibration of the Layer Height LH
4.3. Result and Analysis
5. Parametric Analysis on the Stability of Rapidly Sedimenting Slopes
5.1. Sedimentation Rate, r
5.2. Loading Pattern of Seismic Events
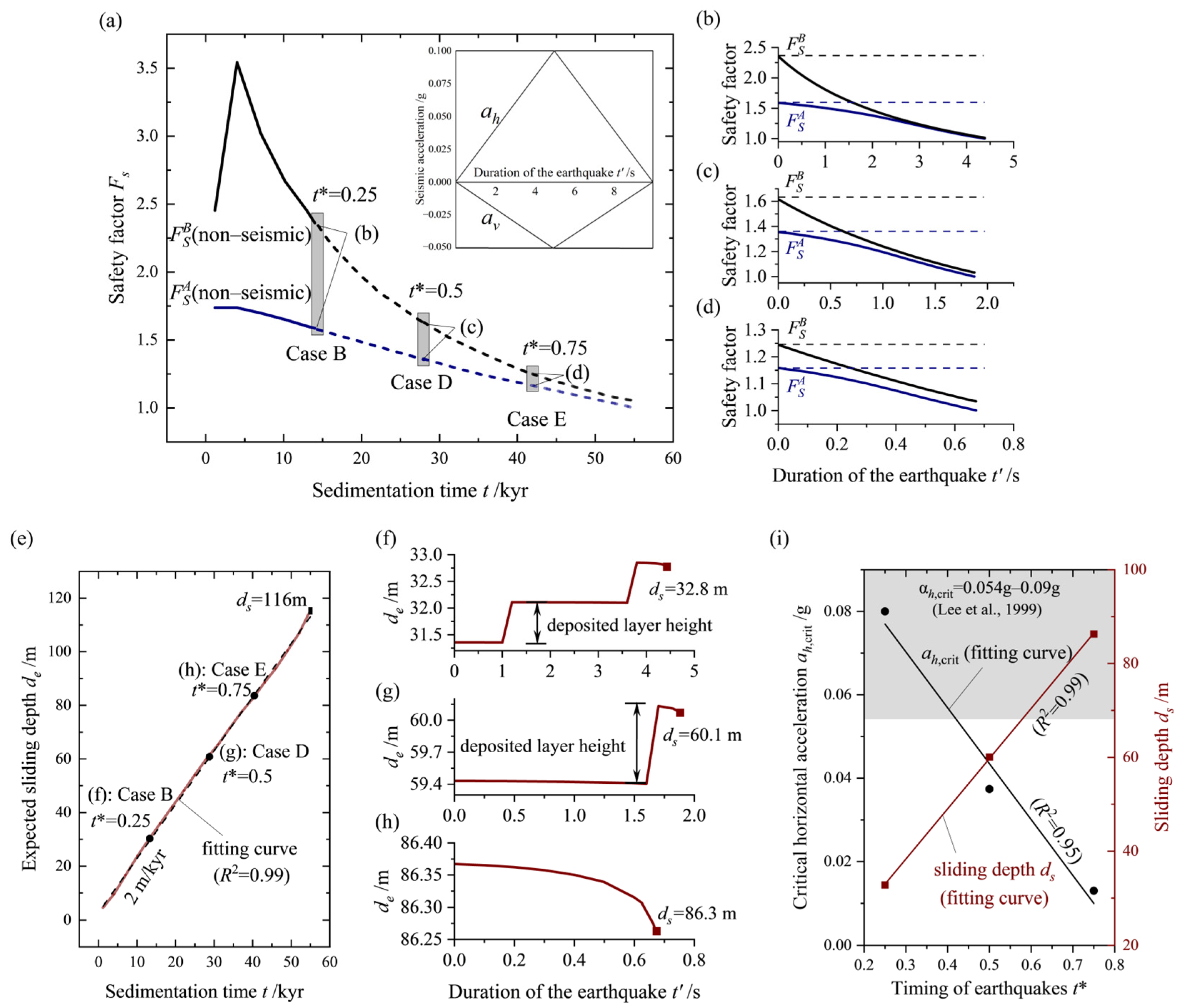
5.3. Occurrence Timing of Seismic Events, t*
6. Conclusions
- To evaluate the reliability of the FE model-change approach, a crucial dimensionless number, NL, is proposed as a calibration metric, and the method is deemed accurate when NL > 100. The calibrated numerical approach effectively replicates the sedimenting process of submarine slopes and accurately captures the accumulation of excess pore pressure, the reduction in strength, and the instability of the submarine slope.
- is proposed as a conservative metric for assessing the stability of submarine slopes during rapid sedimentation, and its decreasing rate, m, is introduced to predict the slope stability within a specific period. The distribution of strength transitions from a linear pattern to a weakened state, which is considered a key factor triggering slope instability.
- Through a variable parameter analysis of sedimentation rates, it is concluded that an increase in the sedimentation rate makes shallow landslides more prone to occurrence. A further analysis of the sediment strength and sliding surface depth yields a method for calculating the critical sedimentation rate.
- In assessing the stability of rapidly sedimenting slopes under seismic action, the most critical condition compared to other load combinations is determined. To quantify the individual impacts of seismic events and rapid sedimentation on slope instability, a relative rate of decrease in the safety factor, m*, is proposed.
- During the sedimenting process, earthquake events prematurely interrupt the downward movement trend of the sliding surface, leading to a shallower sliding surface. Additionally, a method for calculating the critical horizontal seismic acceleration of the slope is proposed. Furthermore, the relevant results help to identify potential historical seismic fault events.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Guo, X.; Fan, N.; Zheng, D.; Fu, C.; Wu, H.; Zhang, Y.; Song, X.; Nian, T. Predicting impact forces on pipelines from deep-sea fluidized slides: A comprehensive review of key factors. Int. J. Min. Sci. Technol. 2024, 34, 211–225. [Google Scholar] [CrossRef]
- Guo, X.; Liu, X.; Li, M.; Lu, Y. Lateral force on buried pipelines caused by seabed slides using a CFD method with a shear interface weakening model. Ocean Eng. 2023, 280, 114663. [Google Scholar] [CrossRef]
- Talling, P.J.; Clare, M.L.; Urlaub, M.; Pope, E.; Hunt, J.E.; Watt, S.F. Large submarine landslides on continental slopes: Geohazards, methane release, and climate change. Oceanography 2014, 27, 32–45. [Google Scholar] [CrossRef]
- Hampton, M.A.; Lee, H.J.; Locat, J. Submarine landslides. Rev. Geophys. 1996, 34, 33–59. [Google Scholar] [CrossRef]
- Zhu, Z.; Wang, D.; Zhang, W. Catastrophic submarine landslides with non-shallow shear band propagation. Comput. Geotech. 2023, 163, 105751. [Google Scholar] [CrossRef]
- Zhang, W.; Klein, B.; Randolph, M.F.; Puzrin, A.M. Upslope failure mechanisms and criteria in submarine landslides: Shear band propagation, slab failure and retrogression. J. Geophys. Res. Solid Earth 2021, 126, e2021JB022041. [Google Scholar] [CrossRef]
- Puzrin, A.M.; Germanovich, L.N.; Friedli, B. Shear band propagation analysis of submarine slope stability. Géotechnique 2016, 66, 188–201. [Google Scholar] [CrossRef]
- Yenes, M.; Monterrubio, S.; Nespereira, J.; Casas, D. Apparent overconsolidation and its implications for submarine landslides. Eng. Geol. 2020, 264, 105375. [Google Scholar] [CrossRef]
- Kaminski, P.; Sager, T.; Grabe, J.; Urlaub, M. A new methodology to assess the potential of conjectural trigger mechanisms of submarine landslides exemplified by marine gas occurrence on the Balearic Promontory. Eng. Geol. 2021, 295, 106446. [Google Scholar] [CrossRef]
- Nian, T.; Shen, Y.; Zheng, D.; Lei, D. Research advances on the chain disasters of submarine landslides. J. Eng. Geol. 2021, 29, 1657–1675. (In Chinese) [Google Scholar] [CrossRef]
- Guo, X.; Liu, X.; Zheng, T.; Zhang, H.; Lu, Y.; Li, T. A mass transfer-based LES modelling methodology for analyzing the movement of submarine sediment flows with extensive shear behavior. Coast. Eng. 2024, 191, 104531. [Google Scholar] [CrossRef]
- Guo, X.; Fan, N.; Liu, Y.; Liu, X.; Wang, Z.; Xie, X.; Jia, Y. Deep seabed mining: Frontiers in engineering geology and environment. Int. J. Coal Sci. Technol. 2023, 10, 23. [Google Scholar] [CrossRef]
- Zheng, D.F.; Nian, T.K.; Liu, B.; Liu, M.; Yin, P.; Huo, Y.D. Investigation of the stability of submarine sensitive clay slopes under wave-induced pressure. Mar. Georesour. Geotechnol. 2019, 37, 116–127. [Google Scholar] [CrossRef]
- Shan, Z.; Wu, H.; Ni, W.; Sun, M.; Wang, K.; Zhao, L.; Lou, Y.; Liu, A.; Xie, W.; Zheng, X.; et al. Recent technological and methodological advances for the investigation of submarine landslides. J. Mar. Sci. Eng. 2022, 10, 1728. [Google Scholar] [CrossRef]
- Fan, N.; Jiang, J.; Nian, T.; Dong, Y.; Guo, L.; Fu, C.; Tian, Z.; Guo, X. Impact action of submarine slides on pipelines: A review of the state-of-the-art since 2008. Ocean Eng. 2023, 286, 115532. [Google Scholar] [CrossRef]
- Bea, R.G. How sea floor slides affect offshore structures. Oil Gas J. 1971, 69, 88–92. [Google Scholar]
- Sterling, G.H.; Strohbeck, E.E. The Failure of the South Pass 70 “B” Platform Hurricane Camille. In Proceedings of the Offshore Technology Conference, Houston, TX, USA, 28 April 1973. [Google Scholar] [CrossRef]
- Hsu, S.K.; Kuo, J.; Chung-Liang, L.; Ching-Hui, T.; Doo, W.B.; Ku, C.Y.; Sibuet, J.C. Turbidity currents, submarine landslides and the 2006 Pingtung earthquake off SW Taiwan. Terr. Atmos. Ocean. Sci. 2008, 19, 7. [Google Scholar] [CrossRef]
- Nian, T.K.; Gu, Z.; Guo, X.; Zhang, H.; Jia, Y. Effect of temperature rise on the mechanical behaviour of deep-sea clay surrounding oil and gas pipelines. Ocean Eng. 2024, 302, 117533. [Google Scholar] [CrossRef]
- Wang, Z.; Zheng, D.; Guo, X.; Gu, Z.; Shen, Y.; Nian, T. Investigation of offshore landslides impact on bucket foundations using a coupled SPH–FEM method. Geoenviron. Disasters 2024, 11, 2. [Google Scholar] [CrossRef]
- Pszonka, J.; Wendorff, M.; Godlewski, P. Sensitivity of marginal basins in recording global icehouse and regional tectonic controls on sedimentation. Example of the Cergowa Basin, (Oligocene) Outer Carpathians. Sediment. Geol. 2023, 444, 106326. [Google Scholar] [CrossRef]
- Moernaut, J.; De Batist, M. Frontal emplacement and mobility of sublacustrine landslides: Results from morphometric and seismostratigraphic analysis. Mar. Geol. 2011, 285, 29–45. [Google Scholar] [CrossRef]
- Horozal, S.; Bahk, J.J.; Cukur, D.; Urgeles, R.; Buchs, D.M.; Lee, S.H.; Um, I.; Kim, S.P. Factors for pre-conditioning and post-failure behaviour of submarine landslides in the margins of Ulleung Basin, East Sea (Japan Sea). Mar. Geol. 2023, 455, 106956. [Google Scholar] [CrossRef]
- Hance, J.J. Submarine Slope Stability. Master’s Thesis, The University of Texas at Austin, Austin, TX, USA, 2003. [Google Scholar]
- Pszonka, J.; Godlewski, P.; Fheed, A.; Dwornik, M.; Schulz, B.; Wendorff, M. Identification and quantification of intergranular volume using SEM automated mineralogy. Mar. Petrol. Geol. 2024, 162, 106708. [Google Scholar] [CrossRef]
- Pszonka, J.; Schulz, B.; Sala, D. Application of mineral liberation analysis (MLA) for investigations of grain size distribution in submarine density flow deposits. Mar. Petrol. Geol. 2021, 129, 105109. [Google Scholar] [CrossRef]
- Rodrigues, S.; Hernández-Molina, F.J.; Fonnesu, M.; Miramontes, E.; Rebesco, M.; Campbell, D.C. A new classification system for mixed (turbidite-contourite) depositional systems: Examples, conceptual models and diagnostic criteria for modern and ancient records. Earth-Sci. Rev. 2022, 230, 104030. [Google Scholar] [CrossRef]
- Couvin, B.; Georgiopoulou, A.; Amy, L.A. A guide to recognising slow-moving subaqueous landslides in seismic and bathymetry datasets. Earth-Sci. Rev. 2024, 252, 104749. [Google Scholar] [CrossRef]
- Reynolds, T. Grain size from source to sink–modern and ancient fining rates. Earth-Sci. Rev. 2024, 250, 104699. [Google Scholar] [CrossRef]
- Bennett, R.H.; Fischer, K.M.; Lavoie, D.L.; Bryant, W.R.; Rezak, R. Porometry and fabric of marine clay and carbonate sediments: Determinants of permeability. Mar. Geol. 1989, 89, 127–152. [Google Scholar] [CrossRef]
- Dimitrova, R.S.; Yanful, E.K. Factors affecting the shear strength of mine tailings/clay mixtures with varying clay content and clay mineralogy. Eng. Geol. 2012, 125, 11–25. [Google Scholar] [CrossRef]
- Gatter, R.; Clare, M.A.; Kuhlmann, J.; Huhn, K. Characterisation of weak layers, physical controls on their global distribution and their role in submarine landslide formation. Earth-Sci. Rev. 2021, 223, 103845. [Google Scholar] [CrossRef]
- Li, Z.; Yang, Y.; Zhu, C. Contribution of rapid sedimentation to submarine landslides and its influencing factors. Chin. J. Rock Mech. Eng. 2023, 42, 1225–1236. (In Chinese) [Google Scholar] [CrossRef]
- Lee, H.J.; Edwards, B.D. Regional method to assess offshore slope stability. J. Geotech. Eng. 1986, 112, 489–509. [Google Scholar] [CrossRef]
- Greene, H.G.; Murai, L.Y.; Watts, P.; Maher, N.A.; Fisher, M.A.; Paull, C.E.; Eichhubl, P. Submarine landslides in the Santa Barbara Channel as potential tsunami sources. Nat. Hazards Earth Syst. Sci. 2006, 6, 63–88. [Google Scholar] [CrossRef]
- D’Acremont, E.; Lafuerza, S.; Rabaute, A.; Lafosse, M.; Jollivet Castelot, M.; Gorini, C.; Alonso, B.; Ercilla, G.; Vazquez, J.T.; Vandorpe, C.; et al. Distribution and origin of submarine landslides in the active margin of the southern Alboran Sea (Western Mediterranean Sea). Mar. Geol. 2022, 445, 106739. [Google Scholar] [CrossRef]
- Paola, C.; Straub, K.; Mohrig, D.; Reinhardt, L. The “unreasonable effectiveness” of stratigraphic and geomorphic experiments. Earth-Sci. Rev. 2009, 97, 1–43. [Google Scholar] [CrossRef]
- Vanneste, M.; Forsberg, C.F.; Glimsdal, S.; Harbitz, C.B.; Issler, D.; Kvalstad, T.J.; Løvholt, F.; Nadim, F. Submarine landslides and their consequences: What do we know, what can we do? In Landslide Science and Practice, 1st ed.; Margottini, C., Canuti, P., Sassa, K., Eds.; Springer: Berlin, Germany, 2013; pp. 5–17. [Google Scholar]
- Gibson, R.E. The progress of consolidation in a clay layer increasing in thickness with time. Geotechnique 1958, 8, 171–182. [Google Scholar] [CrossRef]
- Stigall, J.; Dugan, B. Overpressure and earthquake initiated slope failure in the Ursa region, northern Gulf of Mexico. J. Geophys. Res. Solid Earth 2010, 115, B04101. [Google Scholar] [CrossRef]
- Hustoft, S.; Dugan, B.; Mienert, J. Effects of rapid sedimentation on developing the Nyegga pockmark field: Constraints from hydrological modeling and 3-D seismic data, offshore mid-Norway. Geochem. Geophys. Geosyst. 2009, 10, Q06012. [Google Scholar] [CrossRef]
- Sawyer, D.E.; Reece, R.S.; Gulick, S.P.; Lenz, B.L. Submarine landslide and tsunami hazards offshore southern Alaska: Seismic strengthening versus rapid sedimentation. Geophys. Res. Lett. 2017, 44, 8435–8442. [Google Scholar] [CrossRef]
- Bijesh, C.M.; Twinkle, D.; Susanth, S.; Kurian, P.J. Large-scale submarine landslide in the Cochin offshore region, southwestern continental margin of India: A preliminary geophysical understanding. Landslides 2023, 20, 177–187. [Google Scholar] [CrossRef]
- Gales, J.A.; McKay, R.M.; De Santis, L.; Rebesco, M.; Laberg, J.S.; Shevenell, A.E.; Ferrante, G.M. Climate-controlled submarine landslides on the Antarctic continental margin. Nat. Commun. 2023, 14, 2714. [Google Scholar] [CrossRef]
- Towhata, I.; KIM, S.R. Undrained strength of underconsolidated clays and its application to stability analysis of submarine slopes under rapid sedimentation. Soils Found. 1990, 30, 100–114. [Google Scholar] [CrossRef]
- Urlaub, M.; Talling, P.J.; Zervos, A.; Masson, D. What causes large submarine landslides on low gradient (<2°) continental slopes with slow (~0.15 m/kyr) sediment accumulation? J. Geophys. Res. Solid Earth 2015, 120, 6722–6739. [Google Scholar] [CrossRef]
- Stoecklin, A.; Friedli, B.; Puzrin, A.M. Sedimentation as a control for large submarine landslides: Mechanical modeling and analysis of the Santa Barbara Basin. J. Geophys. Res. Solid Earth 2017, 122, 8645–8663. [Google Scholar] [CrossRef]
- Bellwald, B.; Urlaub, M.; Hjelstuen, B.O.; Sejrup, H.P.; Sørensen, M.B.; Forsberg, C.F.; Vanneste, M. NE Atlantic continental slope stability from a numerical modeling perspective. Quat. Sci. Rev. 2019, 203, 248–265. [Google Scholar] [CrossRef]
- Thornton, S.E. Basin model for hemipelagic sedimentation in a tectonically active continental margin: Santa Barbara Basin, California Continental Borderland. Geol. Soc. Lond. Spec. Publ. 1984, 15, 377–394. [Google Scholar] [CrossRef]
- Blum, J.A.; Chadwell, C.D.; Driscoll, N.; Zumberge, M.A. Assessing slope stability in the Santa Barbara Basin, California, using seafloor geodesy and CHIRP seismic data. Geophys. Res. Lett. 2010, 37, L13308. [Google Scholar] [CrossRef]
- Fisher, M.A.; Normark, W.R.; Greene, H.G.; Lee, H.J.; Sliter, R.W. Geology and tsunamigenic potential of submarine landslides in Santa Barbara Channel, Southern California. Mar. Geol. 2005, 224, 1–22. [Google Scholar] [CrossRef]
- Napier, T.J.; Hendy, I.L.; Fahnestock, M.F.; Bryce, J.G. Provenance of detrital sediments in Santa Barbara Basin, California, USA: Changes in source contributions between the Last Glacial Maximum and Holocene. Geol. Soc. Am. Bull. 2019, 132, 65–84. [Google Scholar] [CrossRef]
- Kluesner, J.W.; Brothers, D.S.; Wright, A.L.; Johnson, S.Y. Structural Controls on Slope Failure within the Western Santa Barbara Channel Based on 2-D and 3-D Seismic Imaging. Geochem. Geophys. Geosyst. 2020, 21, e2020GC009055. [Google Scholar] [CrossRef]
- Warrick, J.A.; Farnsworth, K.L. Sources of sediment to the coastal waters of the Southern California Bight. In Earth Science in the Urban Ocean—The Southern California Continental Borderland; Lee, H.J., Normark, W.R., Eds.; Special Paper 454; Geological Society of America: Boulder, CO, USA, 2009; pp. 39–52. [Google Scholar]
- Robert, C. Late Quaternary variability of precipitation in Southern California and climatic implications: Clay mineral evidence from the Santa Barbara Basin, ODP Site 893. Quat. Sci. Rev. 2004, 23, 1029–1040. [Google Scholar] [CrossRef]
- Berger, W.H.; Lange, C.B.; Weinheimer, A. Silica depletion of the thermocline in the eastern North Pacific during glacial conditions: Clues from Ocean Drilling Program Site 893, Santa Barbara basin, California. Geology 1997, 25, 619–622. [Google Scholar] [CrossRef]
- Multichannel Minisparker Seismic-Reflection Data of USGS Field Activity 2016-666-FA Collected in the Santa Barbara Basin in September and October of 2016. Available online: https://www.sciencebase.gov/catalog/item/5ebedb6c82ce476925e5e065 (accessed on 1 September 2023).
- Sussman, T.; Bathe, K.J. A finite element formulation for nonlinear incompressible elastic and inelastic analysis. Comput. Struct. 1987, 26, 357–409. [Google Scholar] [CrossRef]
- Hamann, T.; Qiu, G.; Grabe, J. Application of a Coupled Eulerian–Lagrangian approach on pile installation problems under partially drained conditions. Comput. Geotech. 2015, 63, 279–290. [Google Scholar] [CrossRef]
- Swartzendruber, D. Modification of Darcy’s law for the flow of water in soils. Soil Sci. 1962, 93, 22–29. [Google Scholar] [CrossRef]
- Staubach, P.; Machaček, J.; Skowronek, J.; Wichtmann, T. Vibratory pile driving in water-saturated sand: Back-analysis of model tests using a hydro-mechanically coupled CEL method. Soils Found. 2021, 61, 144–159. [Google Scholar] [CrossRef]
- Terzaghi, K.; Peck, R.B.; Mesri, G. Soil Mechanics in Engineering Practice; Wiley: New York, NY, USA, 1996. [Google Scholar]
- Simulia, D.S.; Fallis, A.G. ABAQUS documentation. Mendeley 2013, 53, 1689–1699. [Google Scholar]
- Roscoe, K.H.; Burland, J.B. On the generalized stress-strain behavior of “wet” clay. J. Terramech. 1970, 7, 107–108. [Google Scholar] [CrossRef]
- Morgenstern, N.U.; Price, V.E. The analysis of the stability of general slip surfaces. Géotechnique 1965, 15, 79–93. [Google Scholar] [CrossRef]
- Puzrin, A.M.; Germanovich, L.N. The growth of shear bands in the catastrophic failure of soils. Proc. R. Soc. A. Math. Phys. Eng. Sci. 2005, 461, 1199–1228. [Google Scholar] [CrossRef]
- Zhang, W.; Wang, D.; Randolph, M.F.; Puzrin, A.M. From progressive to catastrophic failure in submarine landslides with curvilinear slope geometries. Géotechnique 2017, 67, 1104–1119. [Google Scholar] [CrossRef]
- Schwalbach, J.R.; Gorsline, D.S. Holocene sediment budgets for the basins of the California continental borderland. J. Sediment. Res. 1985, 55, 829–842. [Google Scholar] [CrossRef]
- Burland, J.B. On the compressibility and shear strength of natural clays. Géotechnique 1990, 40, 329–378. [Google Scholar] [CrossRef]
- Stein, R. Clay and bulk mineralogy of late Quaternary sediments at Site 893, Santa Barbara Basin. In Proceedings-Ocean Drilling Program Scientific Results; National Science Foundation: Alexandria, VA, USA, 1992; pp. 89–102. [Google Scholar]
- Edwards, B.D.; Lee, H.J.; Field, M.E. Mudflow generated by retrogressive slope failure, Santa Barbara Basin, California continental borderland. J. Sediment. Res. 1995, 65, 57–68. [Google Scholar] [CrossRef]
- Masson, D.G.; Harbitz, C.B.; Wynn, R.B.; Pedersen, G.; Løvholt, F. Submarine landslides: Processes, triggers and hazard prediction. Philos. Trans. R. Soc. A. 2006, 364, 2009–2039. [Google Scholar] [CrossRef]
- Strozyk, F.; Strasser, M.; Förster, A.; Kopf, A.; Huhn, K. Slope failure repetition in active margin environments: Constraints from submarine landslides in the Hellenic fore arc, eastern Mediterranean. J. Geophys. Res. Solid Earth 2010, 115, B08103. [Google Scholar] [CrossRef]
- Nian, T.; Wang, G.; Zheng, D.; Wang, D. Seismic Stability of Steep Slope Groups in Typical Canyons of Northern South China Sea. J. Jilin Univ. (Earth Sci. Ed.) 2023, 53, 1785–1798. (In Chinese) [Google Scholar] [CrossRef]
- Stoecklin, A.; Trapper, P.; Puzrin, A.M. Controlling factors for post-failure evolution of subaqueous landslides. Géotechnique 2021, 71, 879–892. [Google Scholar] [CrossRef]
- Su, C.; Wang, P.; Zhao, M.; Zhang, G.; Bao, X. Dynamic interaction analysis of structure-water-soil-rock systems under obliquely incident seismic waves for layered soils. Ocean Eng. 2022, 244, 110256. [Google Scholar] [CrossRef]
- Lee, H.; Locat, J.; Dartnell, P.; Israel, K.; Wong, F. Regional variability of slope stability: Application to the Eel margin, California. Mar. Geol. 1999, 154, 305–321. [Google Scholar] [CrossRef]




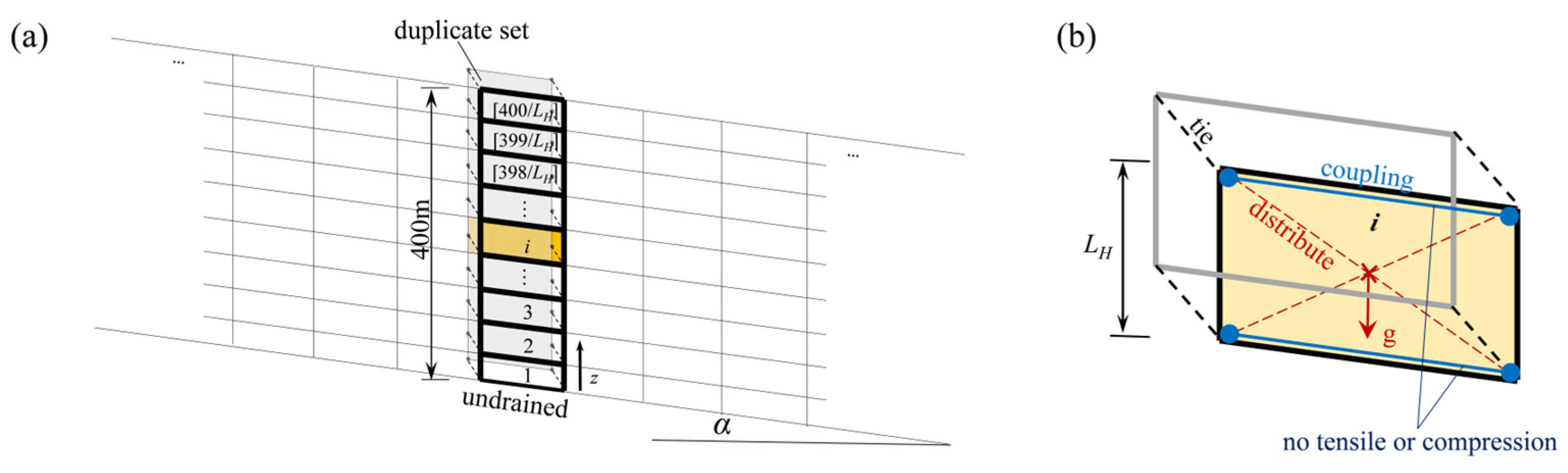
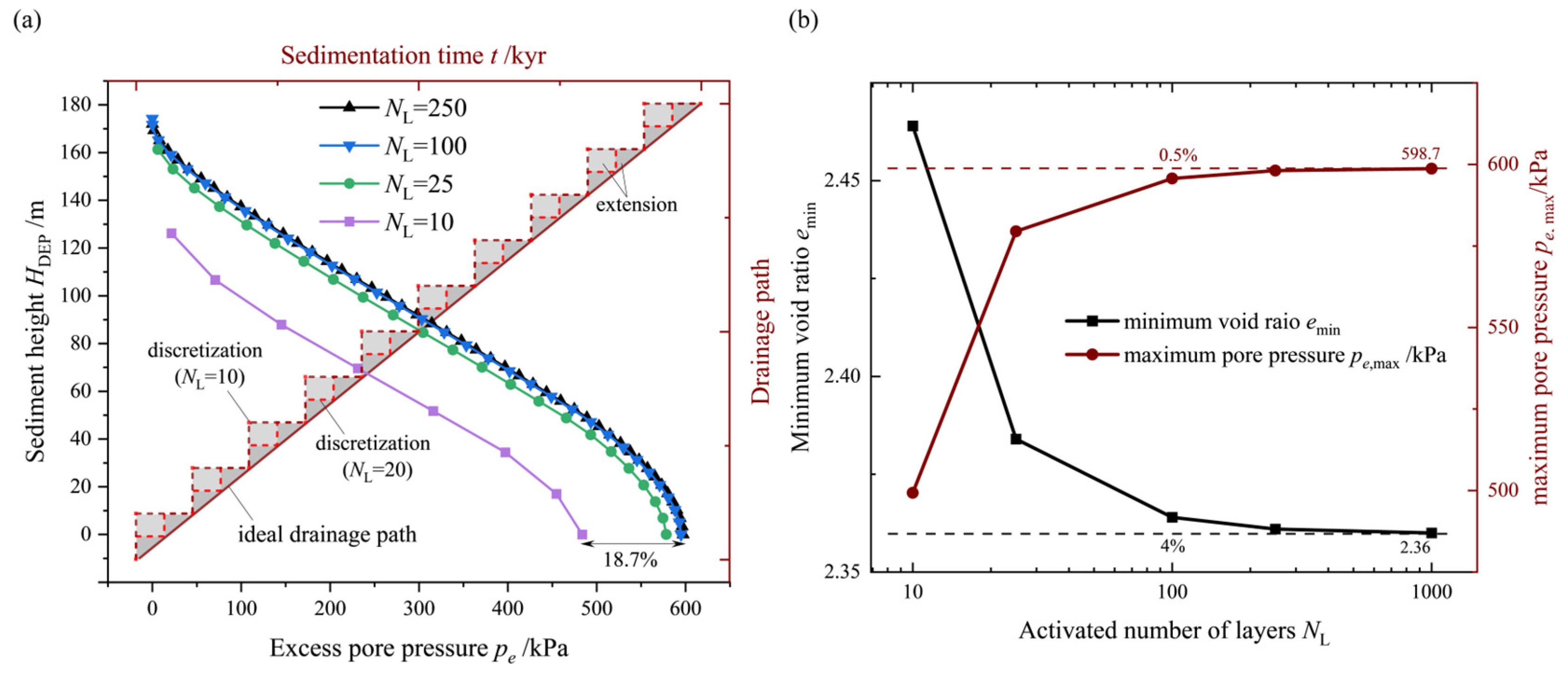

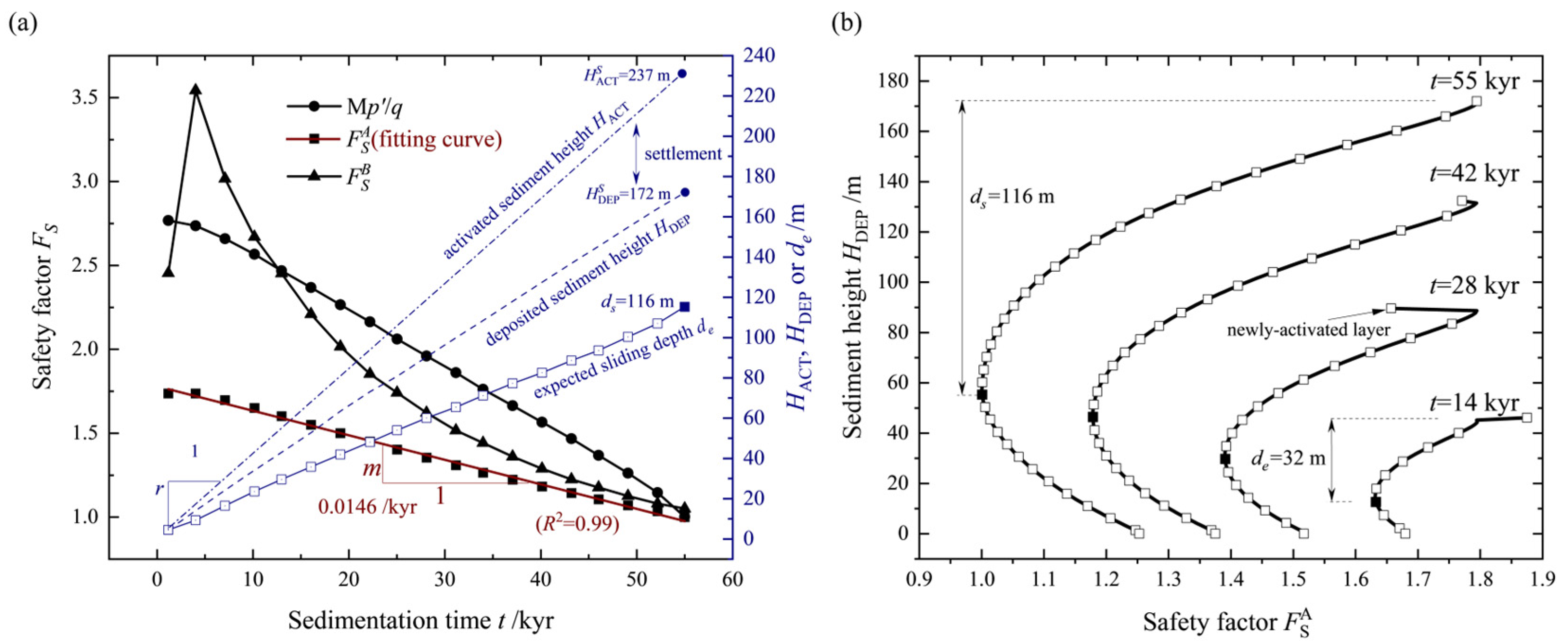
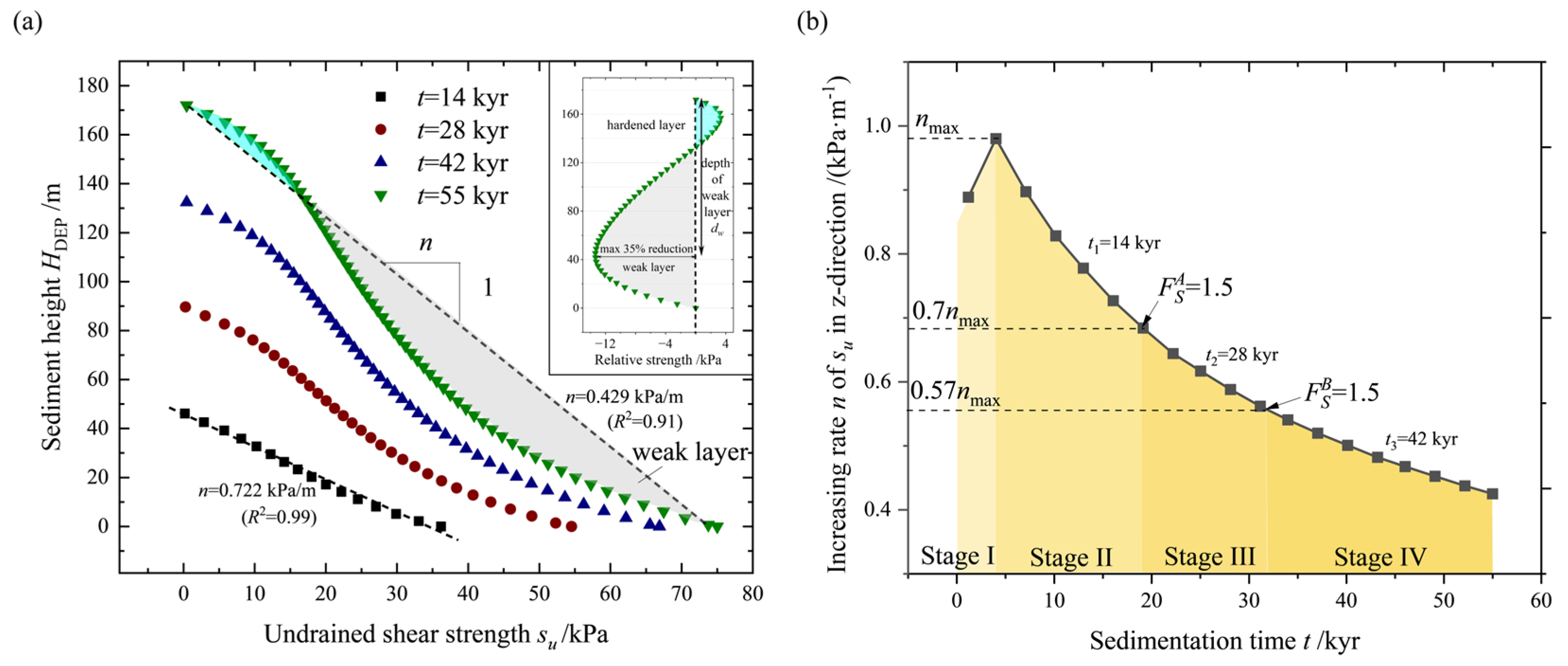


| Parameter | Value (Unit) |
|---|---|
| Sedimentation rate, r | 1.0 m/kyr |
| Compression modulus of the sediment, Es | 10 MPa |
| Poisson’s ratio, υ | 0 |
| Bulk modulus of duplicate grid copies, E′ | 10 Pa |
| Hydraulic conductivity of the sediment, k | 0.05 m/kyr |
| Effective bulk density of the sediment, γ′ | 5 kN/m3 |
| Bulk density of water, γw | 10 kN/m3 |
| Layer height, LH | 10 m |
| 200 m |
| Parameter | Value (Unit) |
|---|---|
| Slope inclination, α | 3.9° |
| Initial void ratio, e0 | 3.8 |
| Internal friction angle, φ′ | 30° |
| Initial hydraulic conductivity, k0 | 2 × 10−9 m/s |
| Compression index, cc | 0.6 |
| Swell index, cs | 0.15 |
| Average sedimentation rate, r | 4.24 m/kyr |
| Density of the soil grains, ρs | 2.7 g/cm3 |
| Initial stress-required height, h0 | 0.3 m |
| Plastic index, IP | 35 |
| Hydraulic conductivity, k |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Z.; Zheng, D.; Gu, Z.; Guo, X.; Nian, T. A Methodology to Evaluate the Real-Time Stability of Submarine Slopes under Rapid Sedimentation. J. Mar. Sci. Eng. 2024, 12, 823. https://doi.org/10.3390/jmse12050823
Wang Z, Zheng D, Gu Z, Guo X, Nian T. A Methodology to Evaluate the Real-Time Stability of Submarine Slopes under Rapid Sedimentation. Journal of Marine Science and Engineering. 2024; 12(5):823. https://doi.org/10.3390/jmse12050823
Chicago/Turabian StyleWang, Zehao, Defeng Zheng, Zhongde Gu, Xingsen Guo, and Tingkai Nian. 2024. "A Methodology to Evaluate the Real-Time Stability of Submarine Slopes under Rapid Sedimentation" Journal of Marine Science and Engineering 12, no. 5: 823. https://doi.org/10.3390/jmse12050823
APA StyleWang, Z., Zheng, D., Gu, Z., Guo, X., & Nian, T. (2024). A Methodology to Evaluate the Real-Time Stability of Submarine Slopes under Rapid Sedimentation. Journal of Marine Science and Engineering, 12(5), 823. https://doi.org/10.3390/jmse12050823







