Practical Formula for Predicting the Residual Deflection Evolution of Steel Plates Subjected to Repeated Impacts
Abstract
:1. Introduction
2. Methodology
2.1. Selection of Stiffened Plates and Striking Object
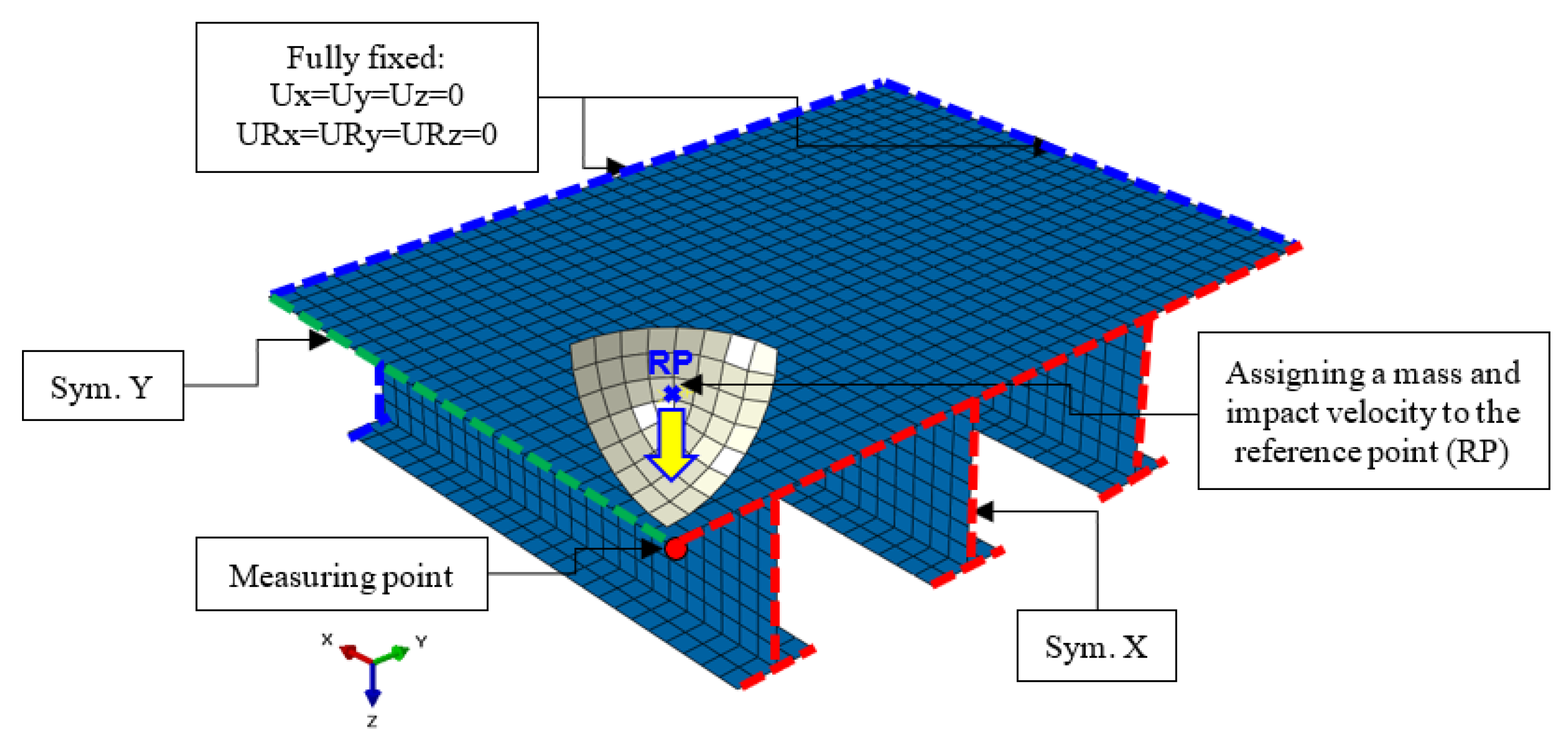
2.2. Finite Element Modeling
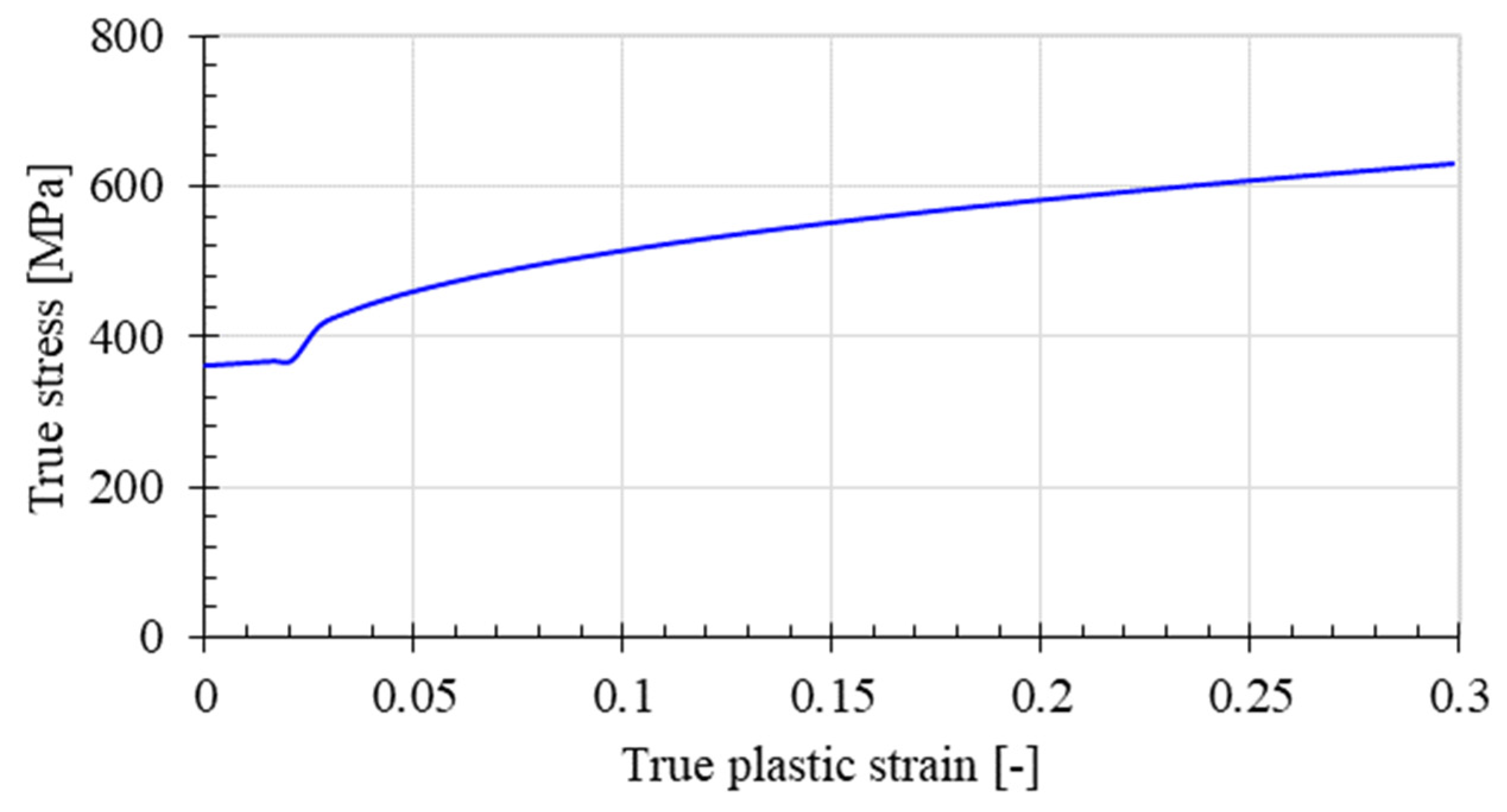
2.3. Definition of Material Properties
2.4. Definition of Repeated Impact Scenario
3. Repeated Mass Impact Tests on Structural Components for Validation
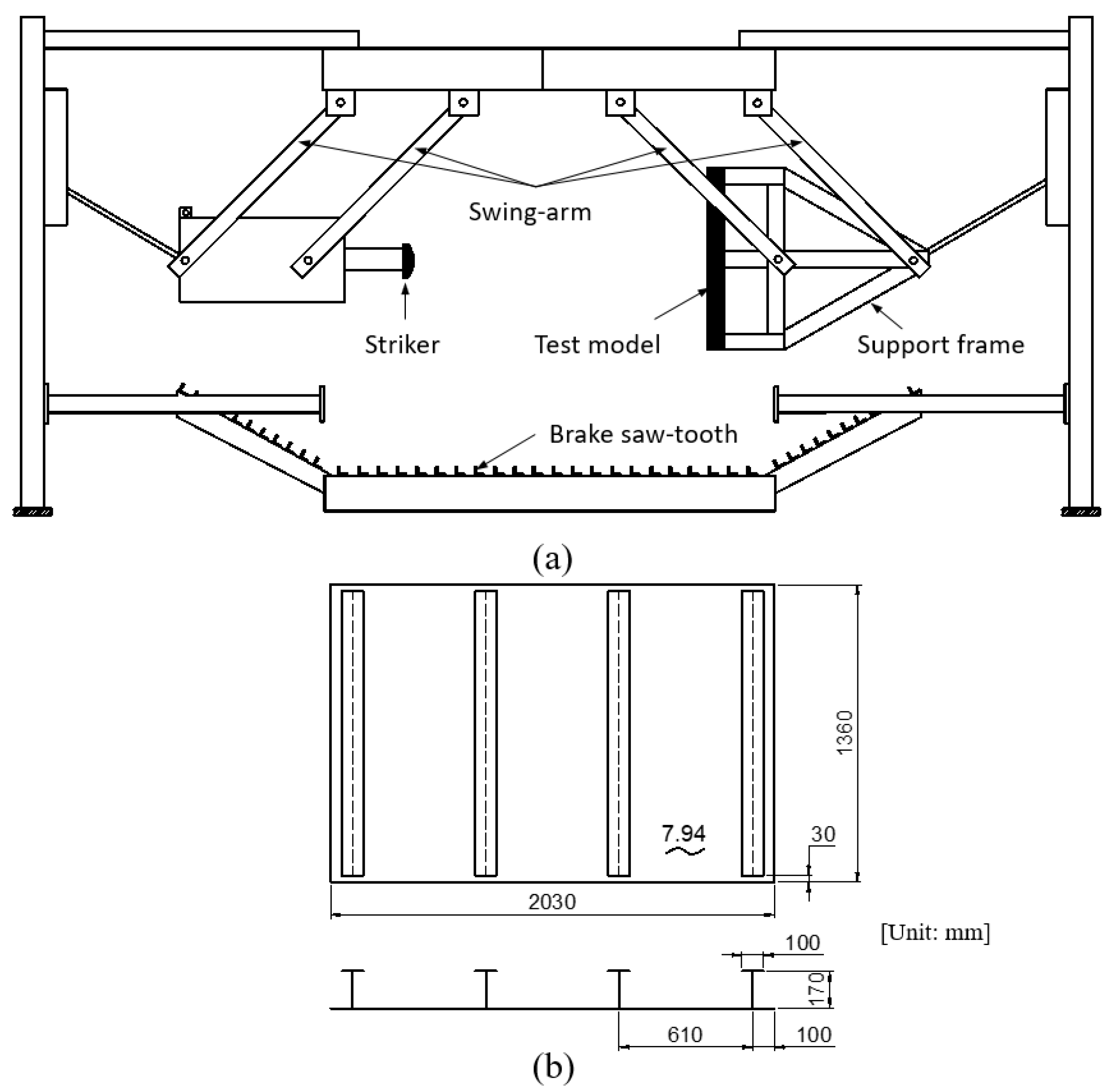
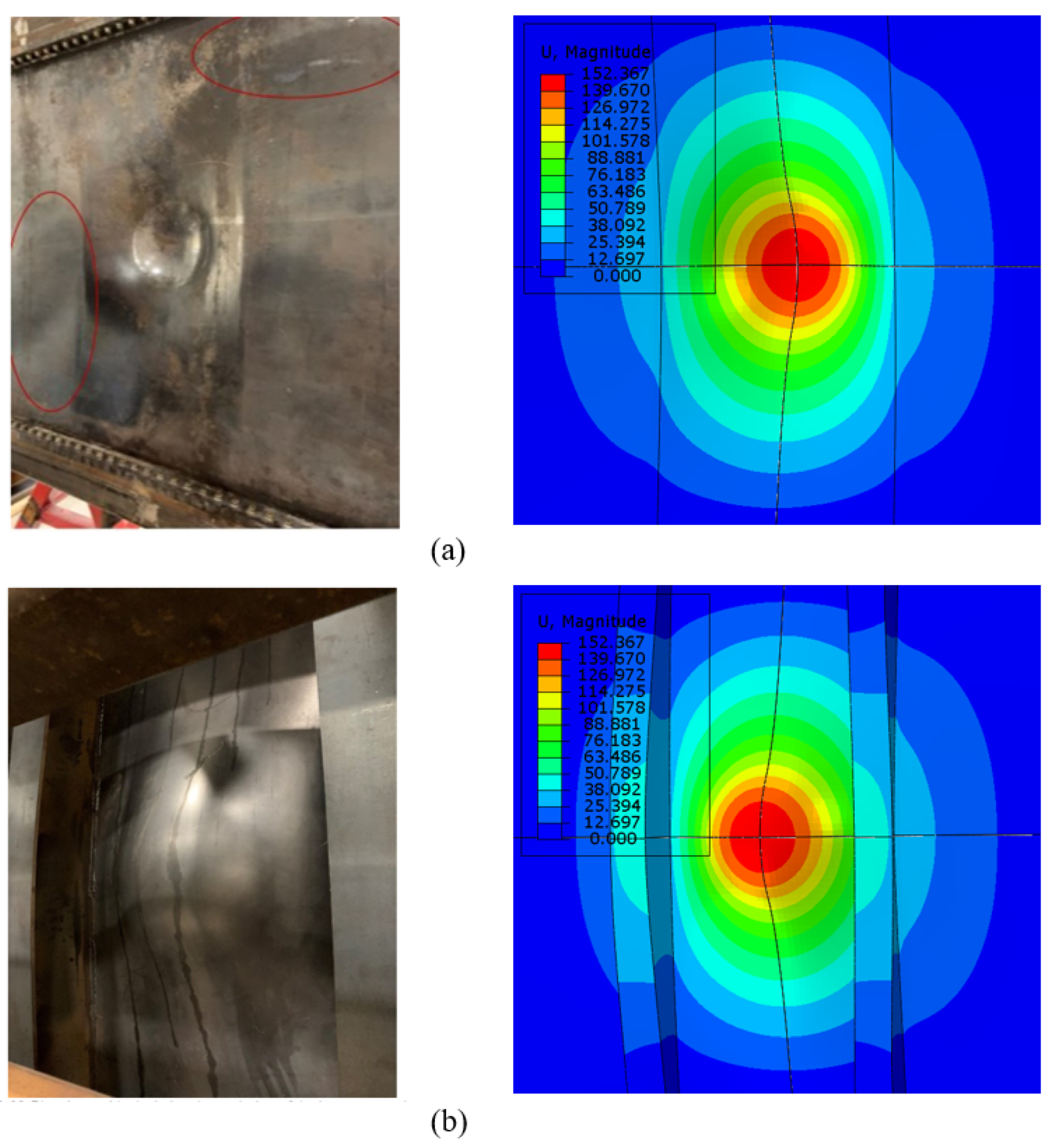
3.1. Brief Description of Repeated Mass Impact Tests
3.2. Numerical Simulations
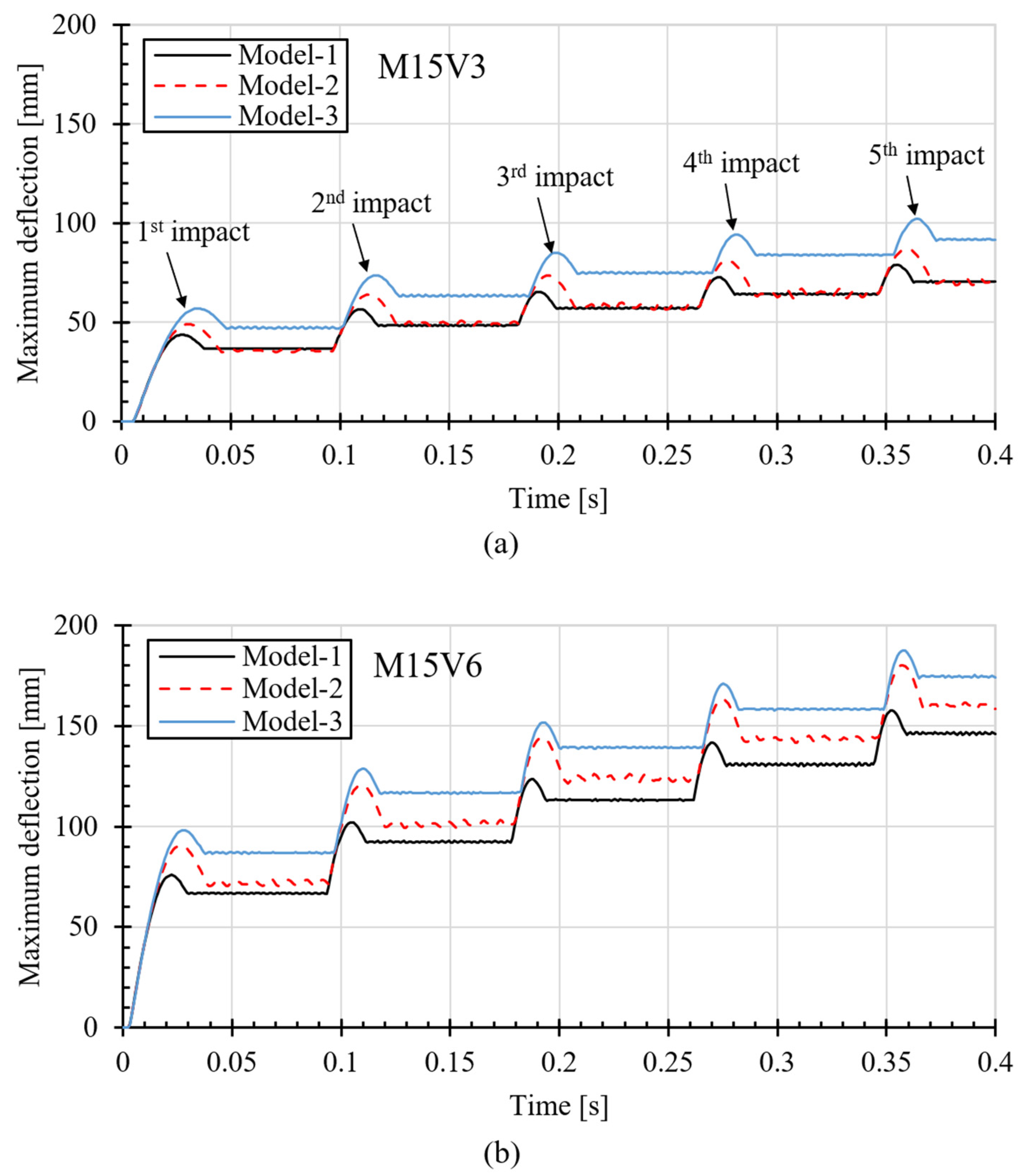
4. Calculation Results and Discussion
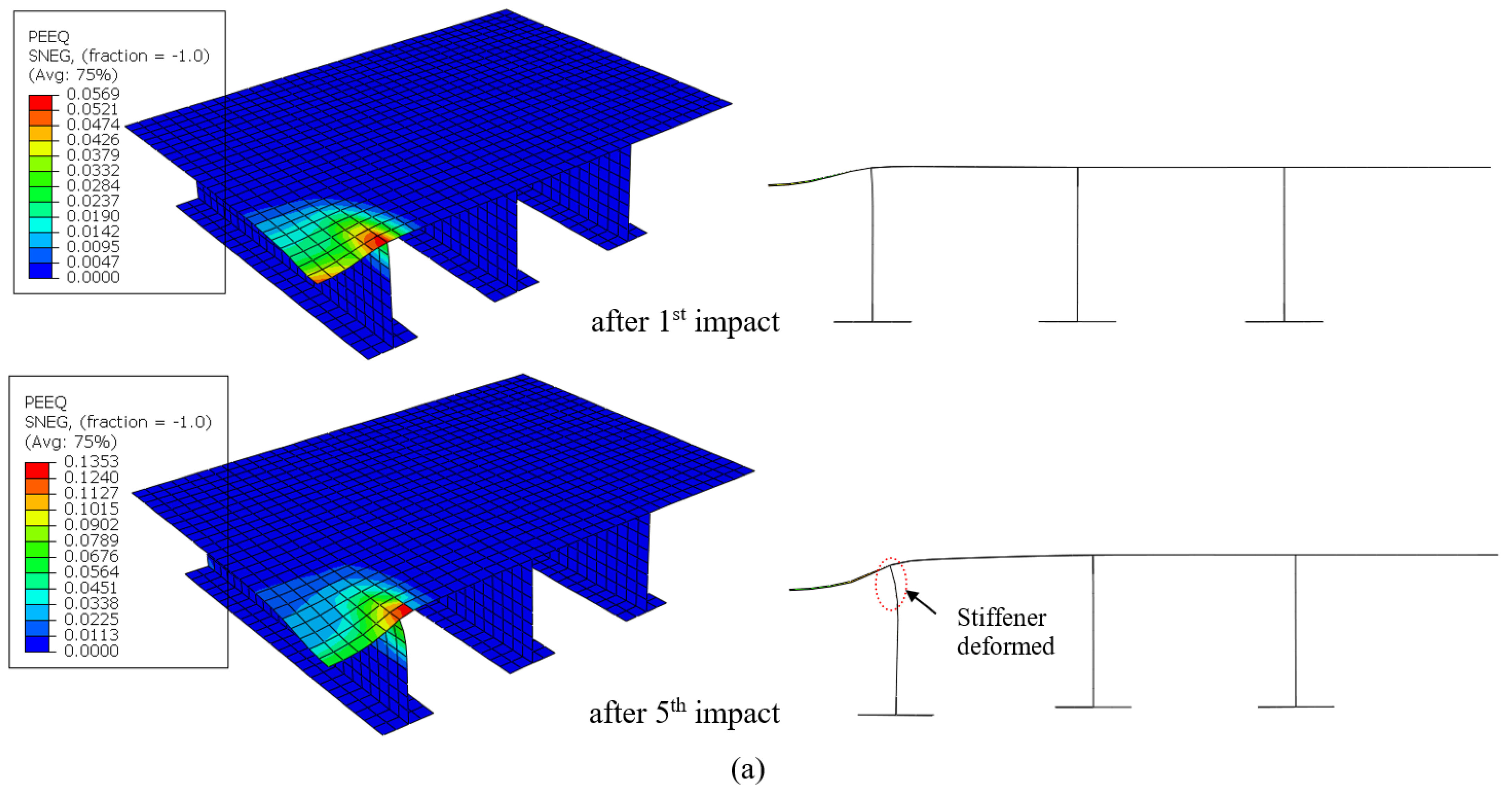
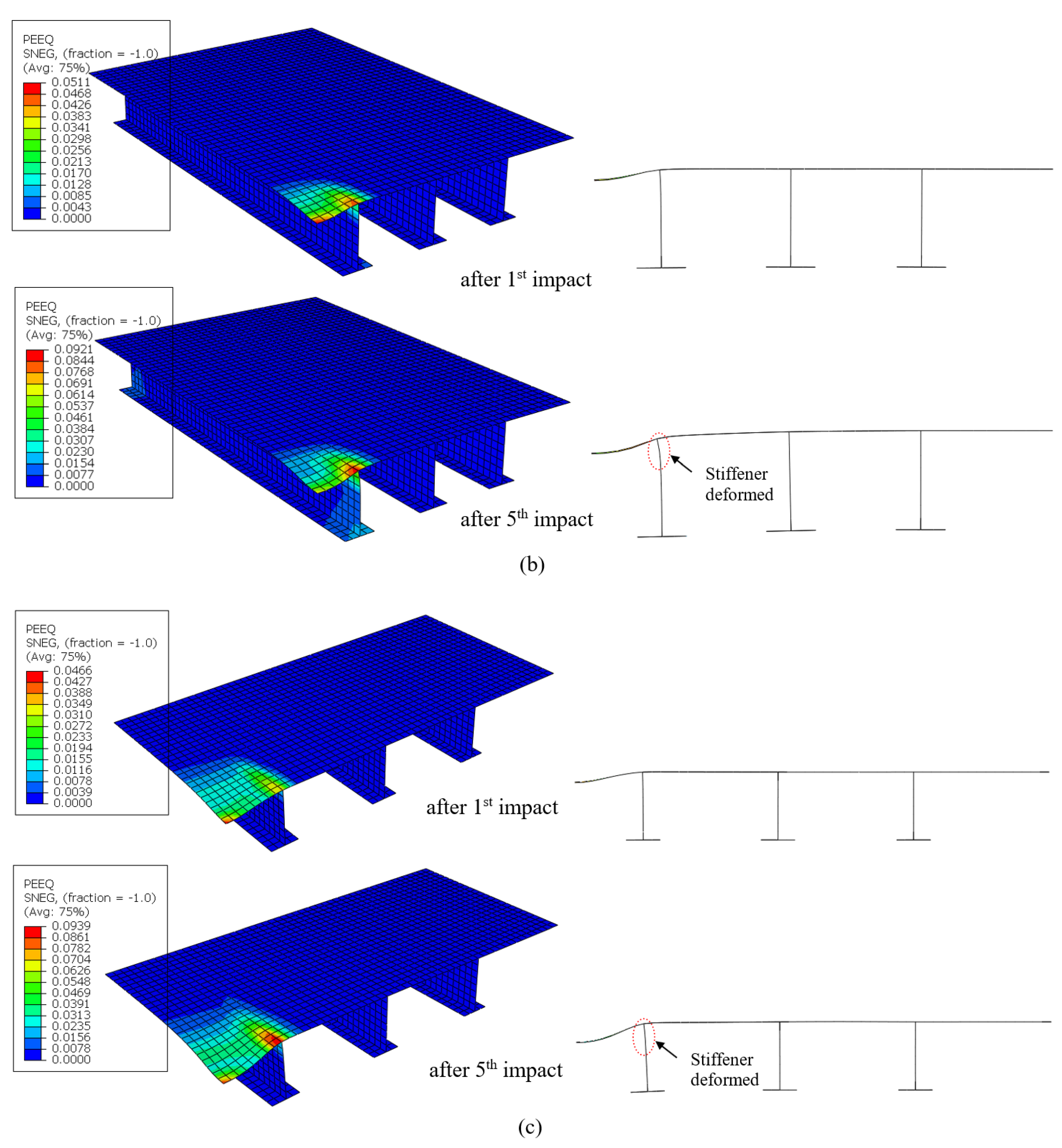
4.1. Plastic Strain Distribution and Damage Extent
4.2. Impact Force and Striker Velocity Time History
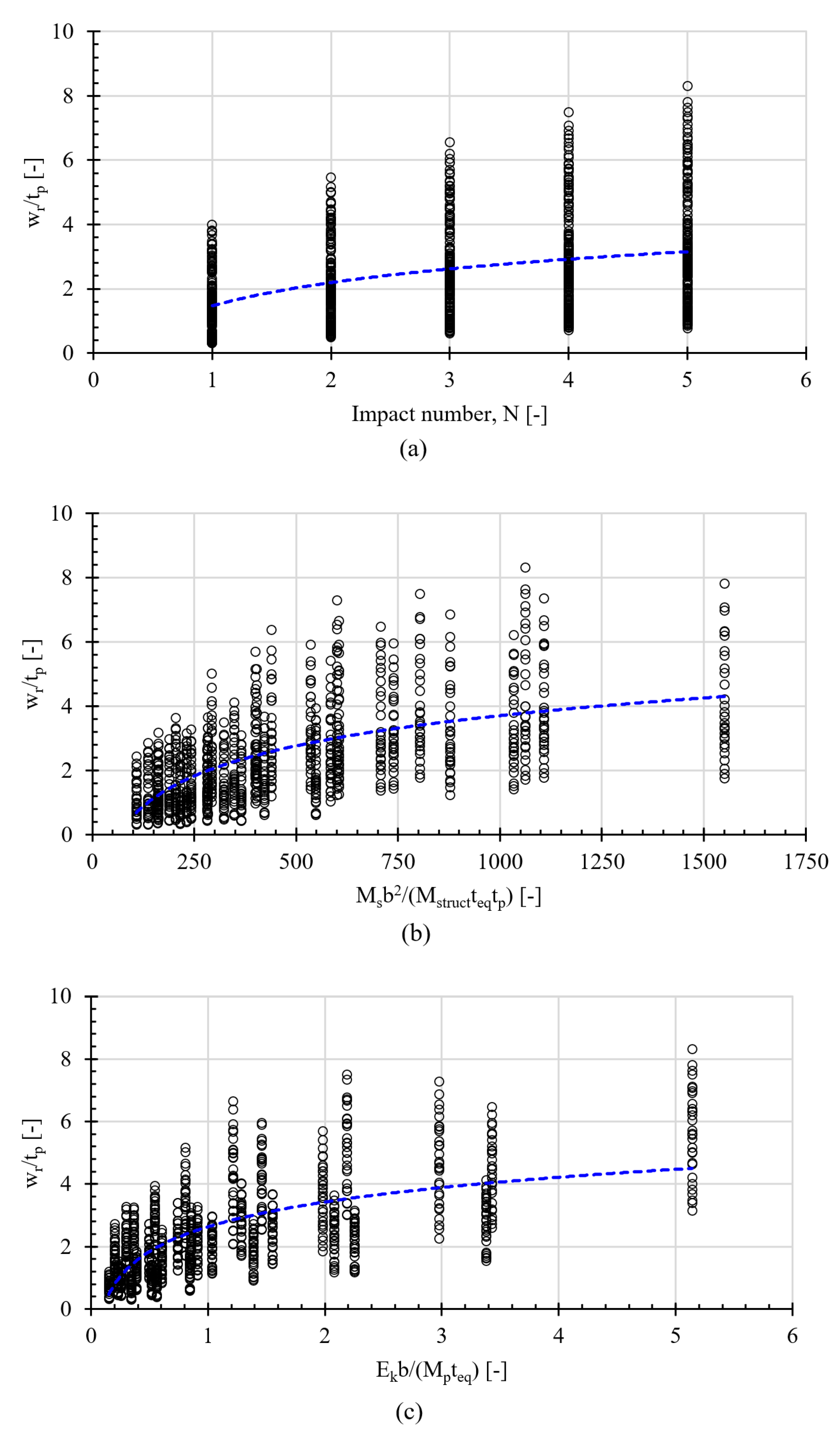
5. Derivation of Empirical Formulation for Prediction of Residual Deflection Evolution
5.1. General Procedure
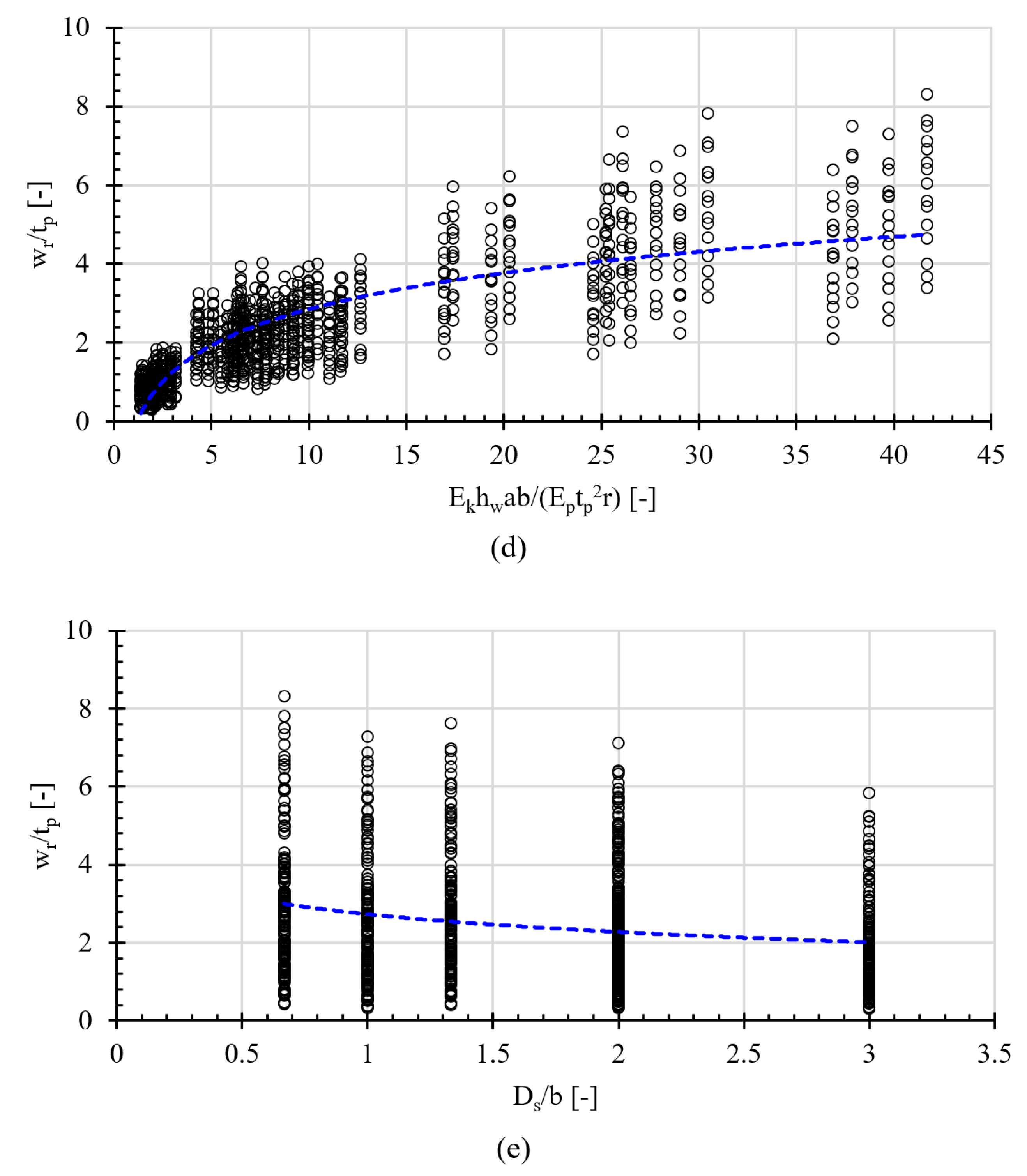
5.2. Derivation of Empirical Formulation
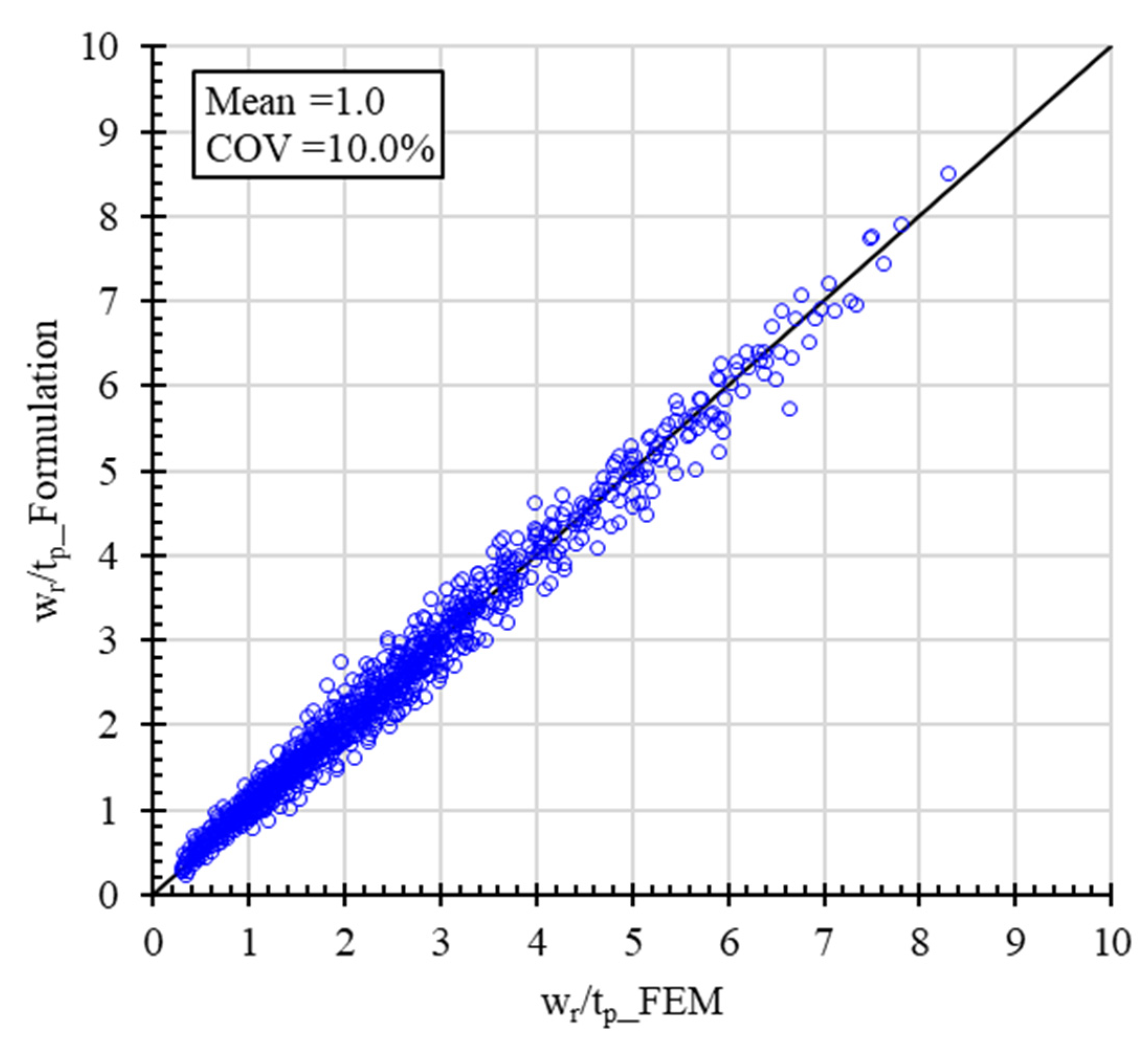
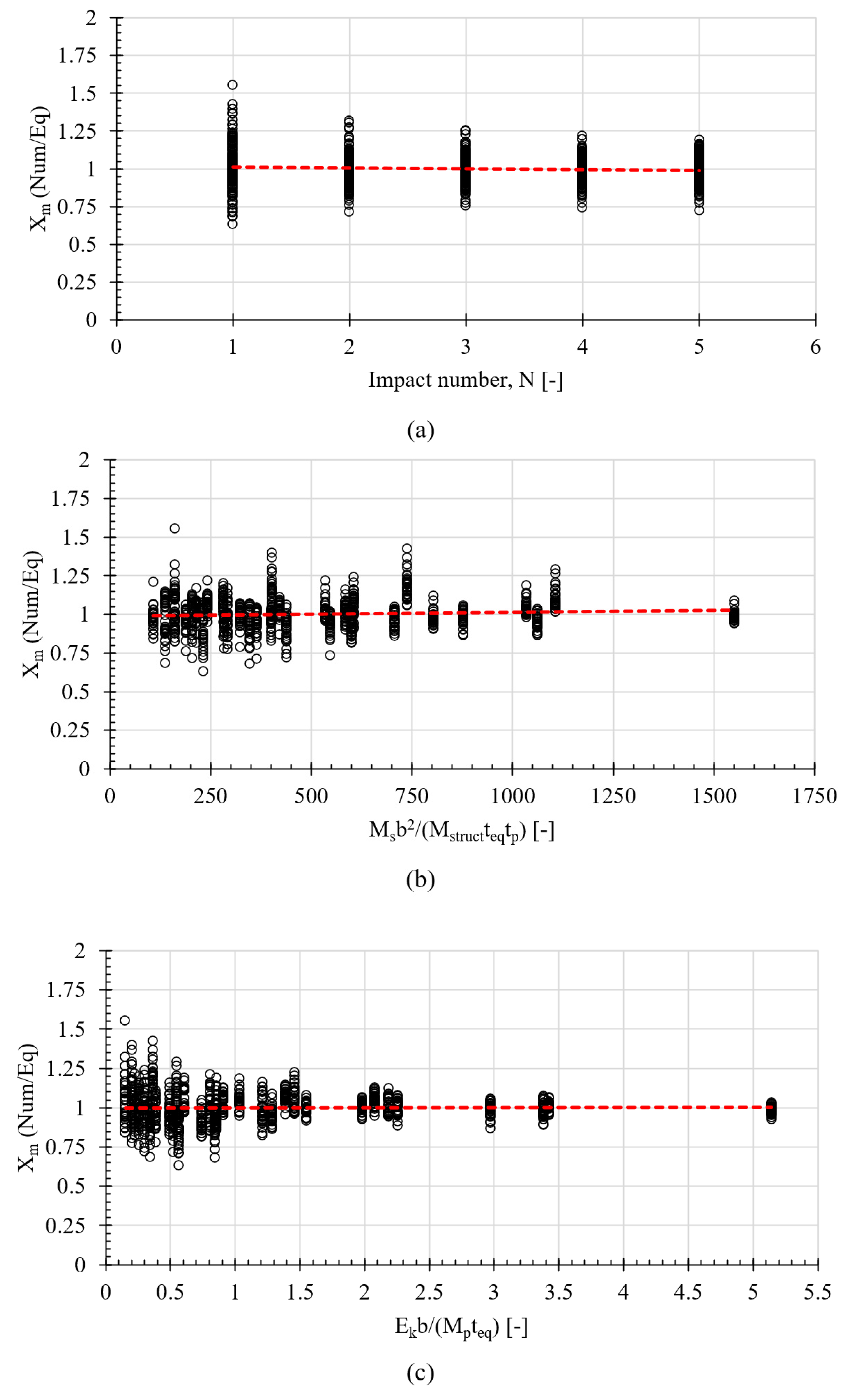
5.3. Verification of Empirical Formulation
5.4. Accuracy of Proposed Formulation
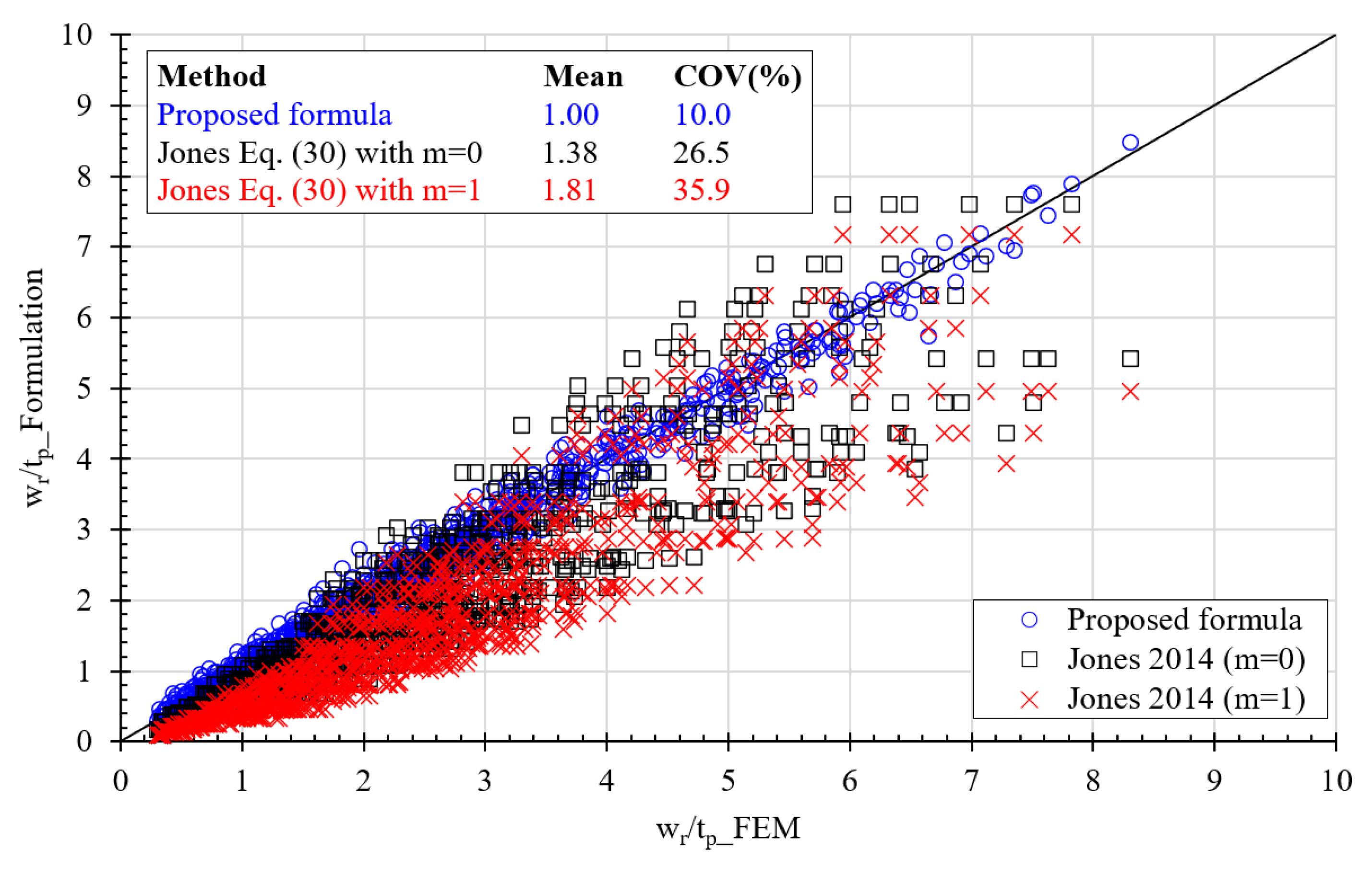
5.4.1. Comparison with Existing Formulations
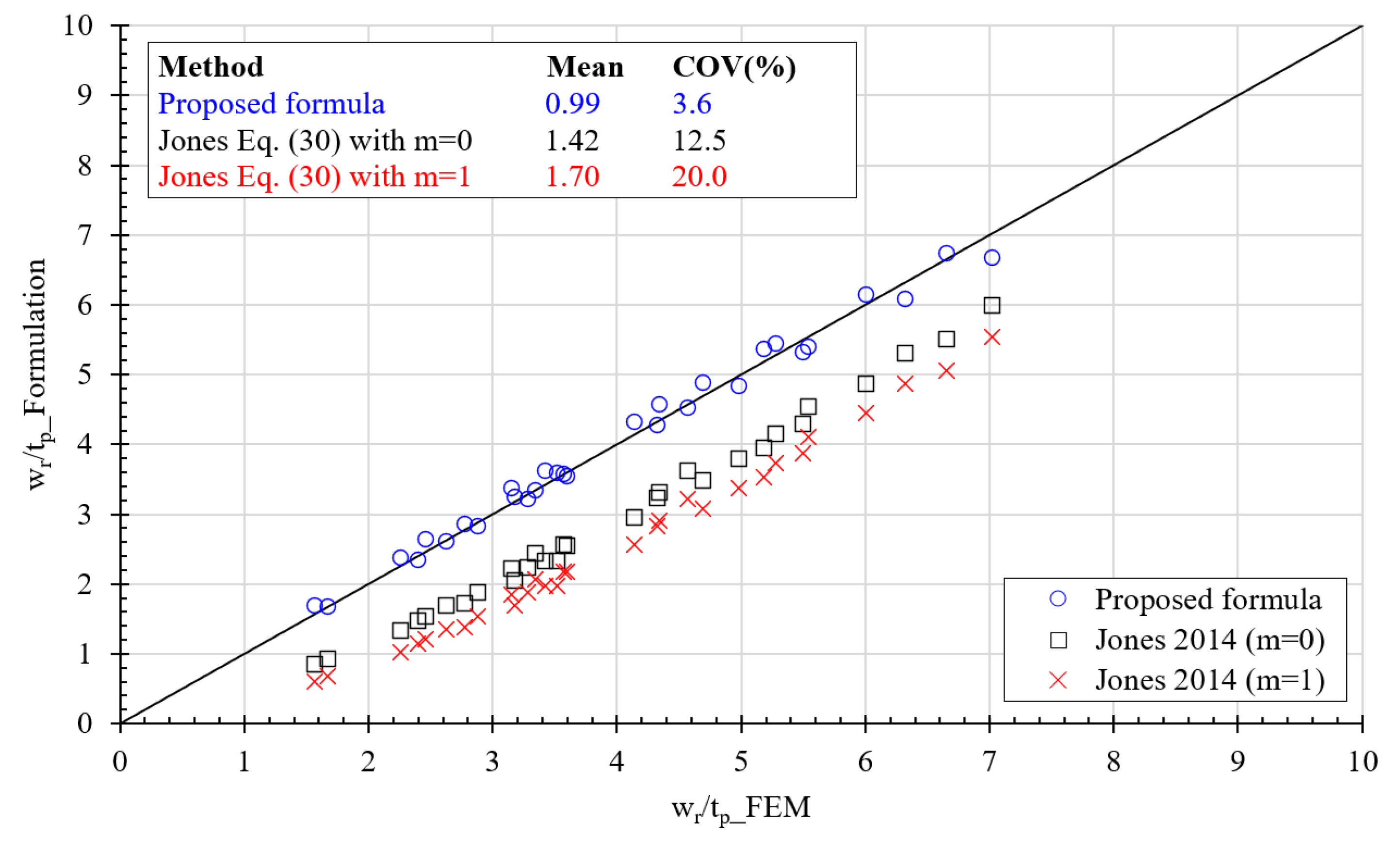
5.4.2. Comparison with Numerical Simulations
6. Conclusions
- The numerical simulation results showed that the deformation after each impact mainly occurred at the impact location, and the stiffeners near the impact location progressively deformed when the impact number rose. The residual deflection of the stiffened steel plates due to the first impact was the most significant and after consecutive impacts, the deflection tended to approach a certain value regardless of the plate scantlings, impact energy, or stiffener shapes. The peak impact force and the rebound velocity of the striker increased, whereas the impact duration reduced with the number of impacts.
- The accuracy and reliability of the newly proposed formula were verified using relevant existing formulations and numerical simulations. The proposed formula is expected to reliably estimate the residual deflection of steel-stiffened plates due to repeated impacts induced by contact with floating objects or ice floes. The formula can be conveniently used in the early marine structure design stage without the need for any numerical simulations or analytical works.
- Furthermore, the proposed numerical simulation techniques, validated with existing test data, are capable of estimating the deflection accumulation of stiffened plates under repeated mass impacts with reasonable accuracy and reliability. Hence, the techniques may be confidently applied for further research on relevant structures in damage-collision assessments.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zhu, L.; Faulkner, D. Damage estimate for plating of ships and platforms under repeated impacts. Mar. Struct. 1996, 9, 697–720. [Google Scholar] [CrossRef]
- Huang, Z.; Chen, Q.; Zhang, W. Pseudo-shakedown in the collision mechanics of ships. Int. J. Impact Eng. 2000, 24, 19–31. [Google Scholar] [CrossRef]
- Chae, G.I. Nonlinear Structural Behavior Due to Repeated Interaction between Ice Breaking Commercial Ships and Sea Ice. Master’s Thesis, University of Ulsan, Ulsan, Republic of Korea, 2008. [Google Scholar]
- Jones, N. Pseudo-shakedown phenomenon for the mass impact loading of plating. Int. J. Impact Eng. 2014, 65, 33–39. [Google Scholar] [CrossRef]
- Cho, S.-R.; Truong, D.D.; Shin, H.K. Repeated lateral impacts on steel beams at room and sub-zero temperatures. Int. J. Impact Eng. 2014, 72, 75–84. [Google Scholar] [CrossRef]
- Truong, D.D.; Shin, H.K.; Cho, S.-R. Repeated lateral impacts on steel grillage structures at room and sub-zero temperatures. Int. J. Impact Eng. 2018, 113, 40–53. [Google Scholar] [CrossRef]
- Truong, D.D.; Jung, H.-J.; Shin, H.K.; Cho, S.-R. Response of low-temperature steel beams subjected to single and repeated lateral impacts. Int. J. Nav. Arch. Ocean Eng. 2018, 10, 670–682. [Google Scholar] [CrossRef]
- Zhu, L.; Shi, S.; Jones, N. Dynamic response of stiffened plates under repeated impacts. Int. J. Impact Eng. 2018, 117, 113–122. [Google Scholar] [CrossRef]
- Zhu, L.; Guo, K.L.; Li, Y.G.; Yu, T.X.; Zhou, Q.W. Experimental study on the dynamic behavior of aluminum foam sandwich plates under single and repeated impacts at low temperature. Int. J. Impact Eng. 2018, 114, 123–132. [Google Scholar] [CrossRef]
- Guo, K.L.; Zhu, L.; Li, Y.G.; Yu, T.X.; Shenoi, A.; Zhou, Q.W. Experimental investigation on the dynamic behavior of aluminum foam sandwich plate under repeated impacts. Compos. Struct. 2018, 200, 298–305. [Google Scholar] [CrossRef]
- Guo, K.; Zhu, L.; Li, Y.; Yu, T. Numerical study on mechanical behavior of foam core sandwich plates under repeated impact loadings. Compos. Struct. 2019, 224, 111030. [Google Scholar] [CrossRef]
- Duan, F.; Liu, J.; Wang, G.; Yu, Z.L. Dynamic behavior of aluminum alloy plates with surface cracks subjected to repeated impacts. Ships Offshore Struct. 2019, 14, 478–491. [Google Scholar] [CrossRef]
- Zeng, Y.; Chen, H.; Yu, R.; Shen, Z.; Yu, Z.; Liu, J. Experimental research on dynamic behavior of circular mild steel plates with surface cracks subjected to repeated impacts in low temperature. Shock Vib. 2020, 2020, 3713709. [Google Scholar] [CrossRef]
- Robbins, M.L. Characterization of Post-Yield Behavior of a Warship Grillage Subject to Repeated Impacts. Master’s Thesis, Memorial University of Newfoundland, St. John’s, NL, Canada, 2020. [Google Scholar]
- Zhang, Y.; Li, Y.; Guo, K.; Zhu, L. Dynamic mechanical behaviour and energy absorption of aluminium honeycomb sandwich panels under repeated impact loads. Ocean Eng. 2021, 219, 108344. [Google Scholar] [CrossRef]
- He, X.; Guedes Soares, C. Experimental study on the dynamic behaviour of beams under repeated impacts. Int. J. Impact Eng. 2021, 147, 103724. [Google Scholar] [CrossRef]
- He, X.; Soares, C.G. Numerical study on the pseudo-shakedown of beams under repeated impacts. Ocean Eng. 2021, 242, 110137. [Google Scholar] [CrossRef]
- He, X.; Soares, C.G. Pseudo-shakedown of rectangular plates under repeated impacts. Mar. Struct. 2022, 85, 103258. [Google Scholar] [CrossRef]
- Cai, W.; Zhu, L.; Qian, X. Dynamic responses of steel plates under repeated ice impacts. Int. J. Impact Eng. 2021, 162, 104129. [Google Scholar] [CrossRef]
- He, X.; Garbatov, Y.; Soares, C.G. Analysis of pseudo-shakedown of rectangular plates under repeated impacts. Ocean Eng. 2022, 265, 112609. [Google Scholar] [CrossRef]
- Zhu, L.; Wang, X.; Guo, K.; Cai, W.; Jones, N.; Dai, S. Effects of indenter geometry on pseudo-shakedown of steel plates under repeated mass impacts. Int. J. Impact Eng. 2023, 185, 104865. [Google Scholar] [CrossRef]
- He, X.; Soares, C.G. Experimental and numerical study on the dynamic response of rectangular plates under repeated impacts. Mar. Struct. 2024, 96, 103606. [Google Scholar] [CrossRef]
- Xu, J.; Guo, Y.; He, Z.; Li, Z.; Liu, G.; He, Y. Simplified analytical method for predicting the large deformation of stiffened plates with initial cracks under repeated impacts. Ocean Eng. 2024, 304, 117886. [Google Scholar] [CrossRef]
- Jones, N. Slamming damage. J. Ship Res. 1973, 17, 80–86. [Google Scholar] [CrossRef]
- Truong, D.D.; Huynh, V.V.; Jang, B.-S.; Quach, H.N.; Dang, X.-P.; Duong, H.D.; Cho, S.-R. Empirical formulations for prediction of permanent set evolution of steel plates due to repeated impulsive pressure loadings induced by slamming. Ocean Eng. 2023, 268, 113430. [Google Scholar] [CrossRef]
- Daley, C.; Kim, H.W. Ice collision forces considering structural deformation. In Proceedings of the ASME 2010 29th International Conference on Ocean, Offshore and Arctic Engineering (OMAE2010), Shanghai, China, 6–11 June 2010. [Google Scholar]
- Zhang, M.; Liu, J.X. Experimental and numerical analysis of tanker double-hull structures punched by a wedge indenter. In Proceedings of the 6th International Conference on Marine Structures (MARSTRUCT 2017), Lisbon, Portugal, 8–10 May 2017. [Google Scholar]
- Villavicencio, R.; Soares, C.G. Numerical modelling of the boundary conditions on beams stuck transversely by a mass. Int. J. Impact Eng. 2011, 38, 384–396. [Google Scholar] [CrossRef]
- Villavicencio, R.; Liu, B.; Soares, C.G. Experimental and numerical analysis of a tanker side panel laterally punched by a knife edge indenter. Mar. Struct. 2014, 37, 173–202. [Google Scholar] [CrossRef]
- Storheim, M.; Amdahl, J. On the sensitivity to work hardening and strain-rate effects in nonlinear FEM analysis of ship collisions. Ships Offshore Struct. 2015, 12, 100–115. [Google Scholar] [CrossRef]
- Cho, S.-R.; Choi, S.I.; Son, S.K. Dynamic material properties of marine steels under impact loadings. In Proceedings of the 2015 World Congress on Advances in Structural Engineering and Mechanics, ASEM15, Incheon, Republic of Korea, 25–29 August 2015. [Google Scholar]
- Cowper, G.; Symonds, P. Strain Hardening and Strain Rate Effects in the Loading of Cantilever Beams; Report No. 28 1957; Brown University: Providence, RI, USA.
- Liu, B.; Villavicencio, R.; Guedes Soares, C. Simplified analytical method to evaluate tanker side panels during minor collision incidents. Int. J. Impact Eng. 2015, 78, 20–33. [Google Scholar] [CrossRef]
- Cerik, B.C. Large inelastic deformation of aluminium alloy plates in high-speed vessels subjected to slamming. J. Mar. Sci. Technol. 2017, 22, 301–312. [Google Scholar] [CrossRef]





| No. | Stiffened Plate | Striker | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Plate | Stiffener | |||||||||||||
| B [mm] | a [mm] | b [mm] | tp [mm] | α | β | hw [mm] | tw [mm] | bf [mm] | tf [mm] | λ | Ds [m] | Ms [t] | V0 [m/s] | |
| 1 | 2800 | 2000 | 400 | 25 | 5 | 2.607 | 300 | 20 | 150 | 25 | 0.198 | 0.4, 0.8, 1.2 | 10, 15 | 3, 6 |
| 2 | 2800 | 4000 | 400 | 25 | 10 | 0.669 | 300 | 20 | 150 | 25 | 0.396 | |||
| 3 | 4200 | 2000 | 600 | 25 | 6.667 | 1.003 | 300 | 20 | 150 | 25 | 0.208 | |||
| 4 | 4200 | 4000 | 600 | 25 | 6.667 | 1.003 | 300 | 20 | 150 | 25 | 0.416 | |||
| 5 | 2800 | 2000 | 400 | 40 | 5 | 0.418 | 300 | 20 | 150 | 25 | 0.205 | |||
| 6 | 2800 | 4000 | 400 | 40 | 10 | 0.418 | 300 | 20 | 150 | 25 | 0.409 | |||
| 7 | 4200 | 2000 | 600 | 40 | 3.333 | 0.627 | 300 | 20 | 150 | 25 | 0.222 | |||
| 8 | 4200 | 4000 | 600 | 40 | 6.667 | 0.627 | 300 | 20 | 150 | 25 | 0.444 | |||
| 9 | 2800 | 2000 | 400 | 25 | 5 | 0.669 | 500 | 20 | 150 | 25 | 0.127 | |||
| 10 | 2800 | 4000 | 400 | 25 | 10 | 0.669 | 500 | 20 | 150 | 25 | 0.254 | |||
| 11 | 4200 | 2000 | 600 | 25 | 3.333 | 1.003 | 500 | 20 | 150 | 25 | 0.130 | |||
| 12 | 4200 | 4000 | 600 | 25 | 6.667 | 1.003 | 500 | 20 | 150 | 25 | 0.261 | |||
| 13 | 2800 | 2000 | 400 | 40 | 5 | 0.418 | 500 | 20 | 150 | 25 | 0.129 | |||
| 14 | 2800 | 4000 | 400 | 40 | 10 | 0.418 | 500 | 20 | 150 | 25 | 0.258 | |||
| 15 | 4200 | 2000 | 600 | 40 | 3.333 | 0.627 | 500 | 20 | 150 | 25 | 0.137 | |||
| 16 | 4200 | 4000 | 600 | 40 | 6.667 | 0.627 | 500 | 20 | 150 | 25 | 0.274 | |||
| Yield Strength, σY [MPa] | Hardening Start Strain, εHS | Ultimate Stress, σT [MPa] | Ultimate Strain, εT | Young’s Modulus, E [MPa] | Density, ρ [kg/m3] | Poisson Ratio, ν | Cowper–Symonds Material Coefficient | |
|---|---|---|---|---|---|---|---|---|
| D [/s] | q | |||||||
| 360 | 0.0229 | 476 | 0.2094 | 206,000 | 7850 | 0.3 | 3200 | 5 |
| Yield Strength, σY [MPa] | Ultimate Stress, σT [MPa] | Failure Strain, εf | Young’s Modulus, E [MPa] | Density, ρ [kg/m3] | Poisson Ratio, ν | Cowper–Symonds Material Coefficient | |
|---|---|---|---|---|---|---|---|
| D [/s] | q | ||||||
| 414 | 483.7 | 0.323 | 206,000 | 7850 * | 0.3 * | 40.4 * | 5 * |
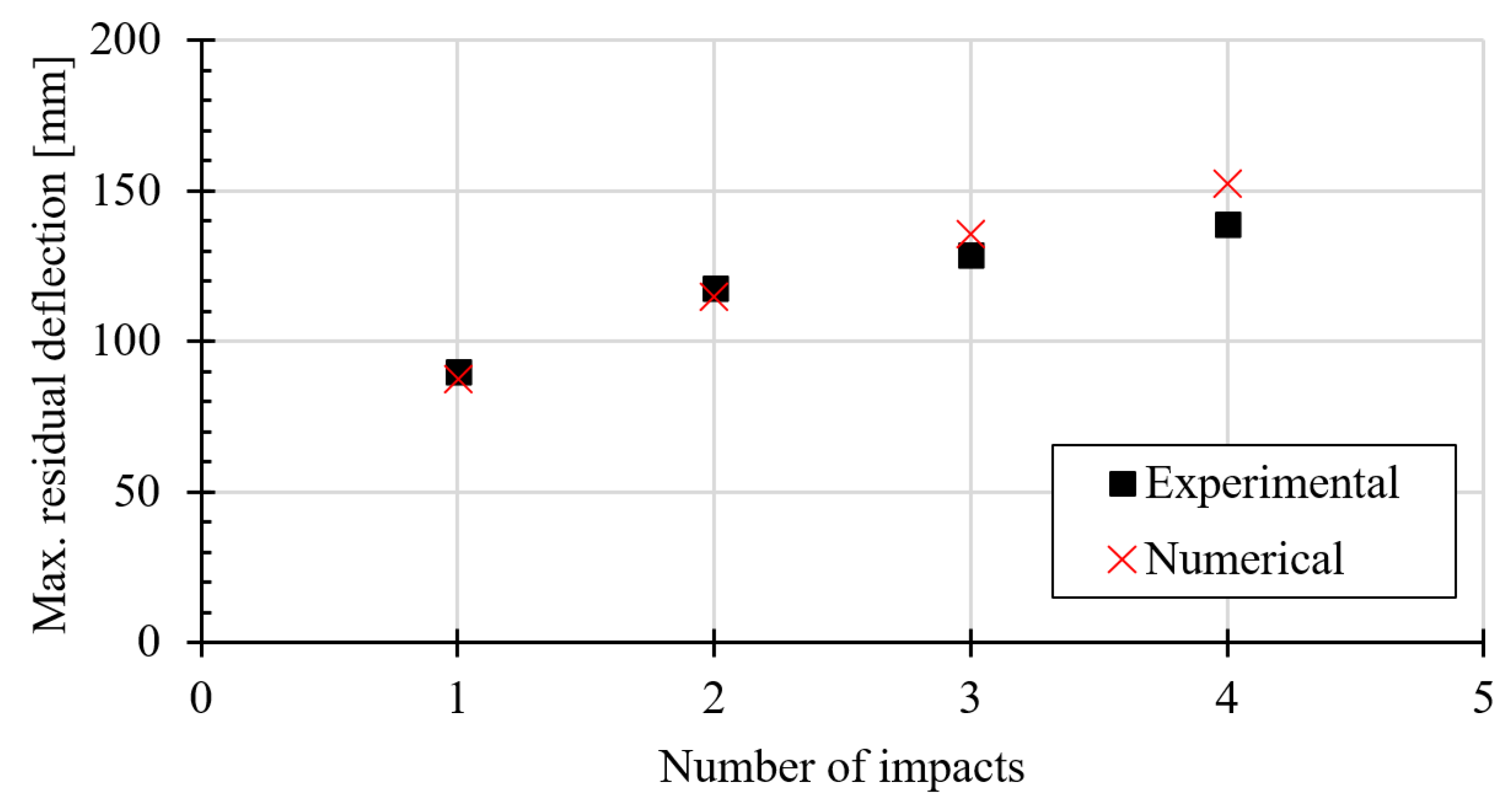
| Impact | Mass [ton] | Dual-Pendulum Closing Velocity [m/s] | Equivalent Impact Velocity (1 Moving Carriage) [m/s] | Maximum Residual Deflection [mm] | |
|---|---|---|---|---|---|
| Experimental | Numerical | ||||
| 1 | 4.711 | 3.744 | 5.295 | 90.10 | 87.49 |
| 2 | 4.711 | 3.744 | 5.096 * | 117.70 | 115.00 |
| 3 | 4.711 | 3.744 | 5.034 * | 128.60 | 135.40 |
| 4 | 4.711 | 3.744 | 5.010 * | 138.72 | 152.30 |
| No. | Stiffened Plate | Striker | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Plate | Stiffener | ||||||||||||||
| B [mm] | a [mm] | b [mm] | tp [mm] | α | β | Type | hw [mm] | tw [mm] | bf [mm] | tf [mm] | λ | Ds [m] | Ms [t] | V0 [m/s] | |
| 1 | 3500 | 3000 | 500 | 25 | 6 | 0.836 | T | 300 | 20 | 150 | 25 | 0.304 | 0.8 | 20 | 5 |
| 2 | 3850 | 3500 | 550 | 30 | 6.364 | 0.766 | T | 350 | 20 | 150 | 25 | 0.318 | |||
| 3 | 4200 | 4000 | 600 | 40 | 6.667 | 0.627 | T | 400 | 20 | 150 | 25 | 0.339 | |||
| 1 | 3500 | 3000 | 500 | 25 | 6 | 0.836 | T | 300 | 20 | 150 | 25 | 0.304 | 0.8 | 12 | 7 |
| 2 | 3850 | 3500 | 550 | 30 | 6.364 | 0.766 | T | 350 | 20 | 150 | 25 | 0.318 | |||
| 3 | 4200 | 4000 | 600 | 40 | 6.667 | 0.627 | T | 400 | 20 | 150 | 25 | 0.339 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huynh, V.-V.; Cho, S.-R.; Dang, X.-P.; Truong, D.D. Practical Formula for Predicting the Residual Deflection Evolution of Steel Plates Subjected to Repeated Impacts. J. Mar. Sci. Eng. 2024, 12, 956. https://doi.org/10.3390/jmse12060956
Huynh V-V, Cho S-R, Dang X-P, Truong DD. Practical Formula for Predicting the Residual Deflection Evolution of Steel Plates Subjected to Repeated Impacts. Journal of Marine Science and Engineering. 2024; 12(6):956. https://doi.org/10.3390/jmse12060956
Chicago/Turabian StyleHuynh, Van-Vu, Sang-Rai Cho, Xuan-Phuong Dang, and Dac Dung Truong. 2024. "Practical Formula for Predicting the Residual Deflection Evolution of Steel Plates Subjected to Repeated Impacts" Journal of Marine Science and Engineering 12, no. 6: 956. https://doi.org/10.3390/jmse12060956
APA StyleHuynh, V.-V., Cho, S.-R., Dang, X.-P., & Truong, D. D. (2024). Practical Formula for Predicting the Residual Deflection Evolution of Steel Plates Subjected to Repeated Impacts. Journal of Marine Science and Engineering, 12(6), 956. https://doi.org/10.3390/jmse12060956






