Sub-Nappe Air Cavity Pressure and Cavity Water Depth during Caisson Breakwater Overtopping by a Tsunami
Abstract
1. Introduction
2. Materials and Methods
2.1. Flume and Vertical Caisson Breakwater Model
2.2. Measurement Approaches for Air Cavity Pressure and Cavity Water Depth
2.3. Ventilated vs. Non-Ventilated: Air Cavity Pressure and Cavity Water Depth
2.4. Estimating Air Flow Rate into the Cavity: A Bernoulli Equation Approach
2.5. Effects of Downstream Flow Regime on Air Cavity Pressure and Cavity Water Depth
2.6. Dimensionless Analysis for Air Cavity Pressure and Cavity Water Depth
3. Results
3.1. Comparison of Ventilated and Non-Ventilated Cases for Air Cavity Pressure and Cavity Water Depth
3.2. Dimensionless Equation for Air Cavity Pressure with Subcritical Flow Downstream
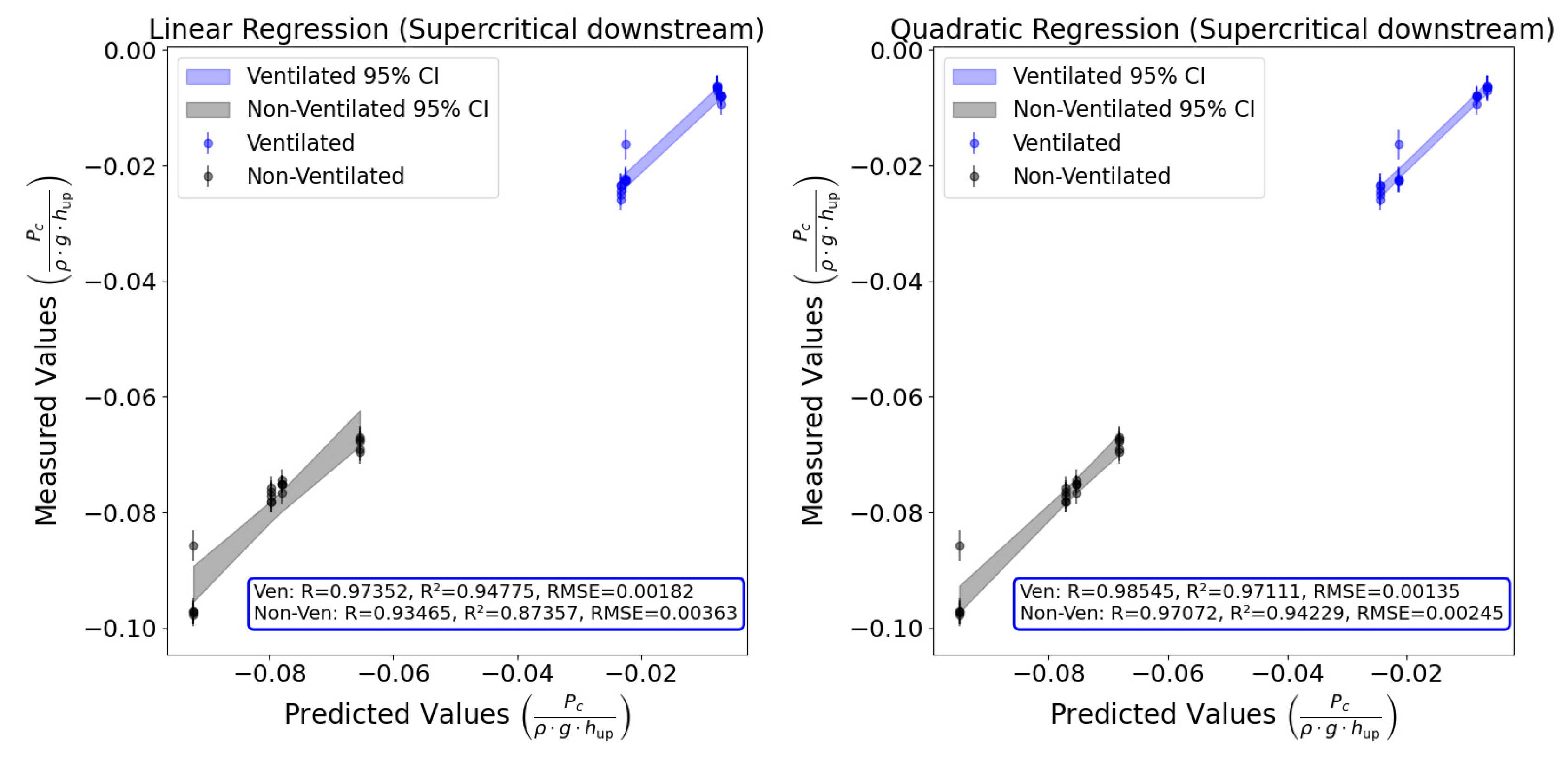
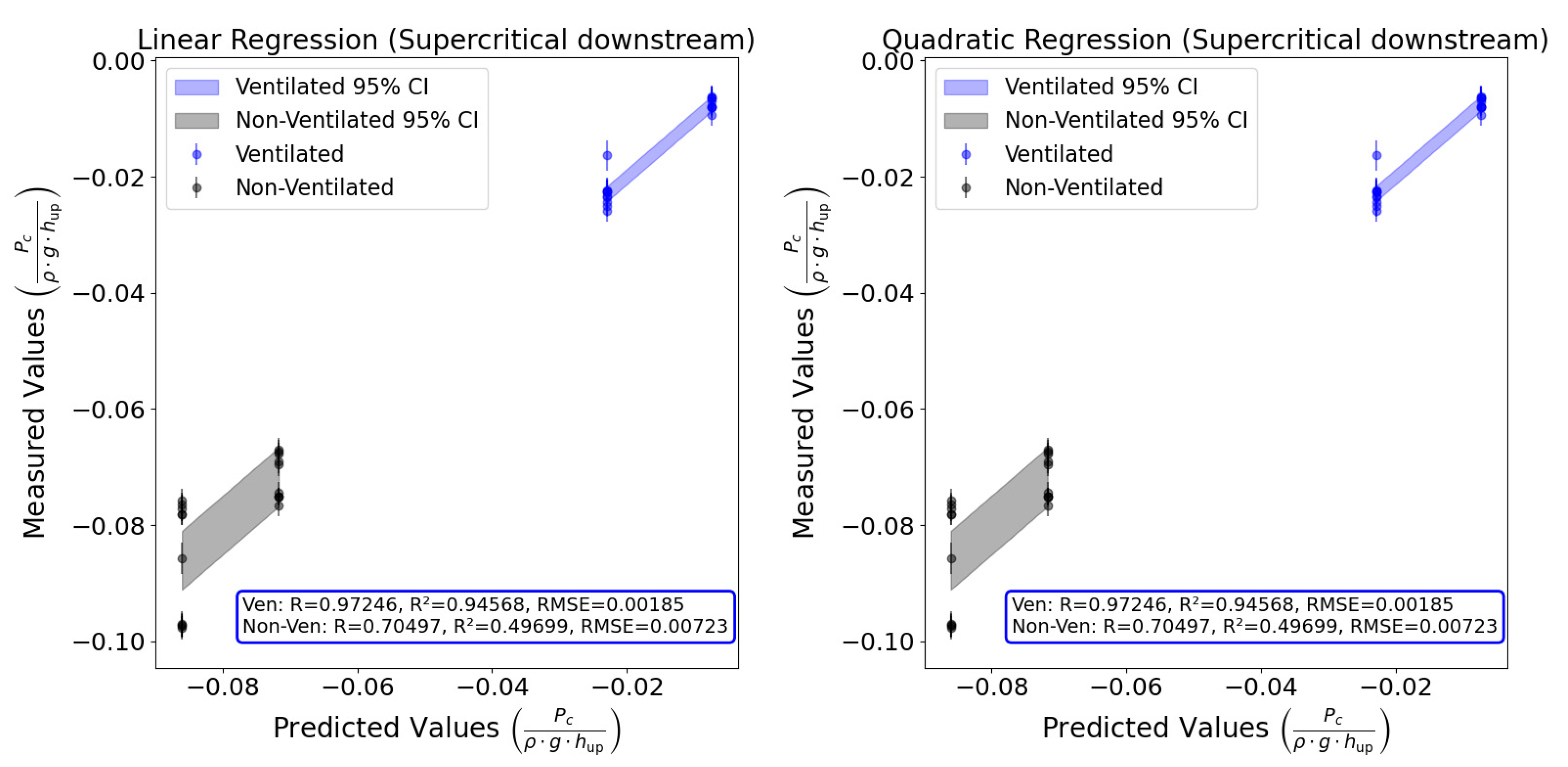
3.3. Dimensionless Equation for Air Cavity Pressure with Supercritical Flow Downstream
3.4. Dimensionless Equation for Cavity Water Depth with Subcritical Flow Downstream
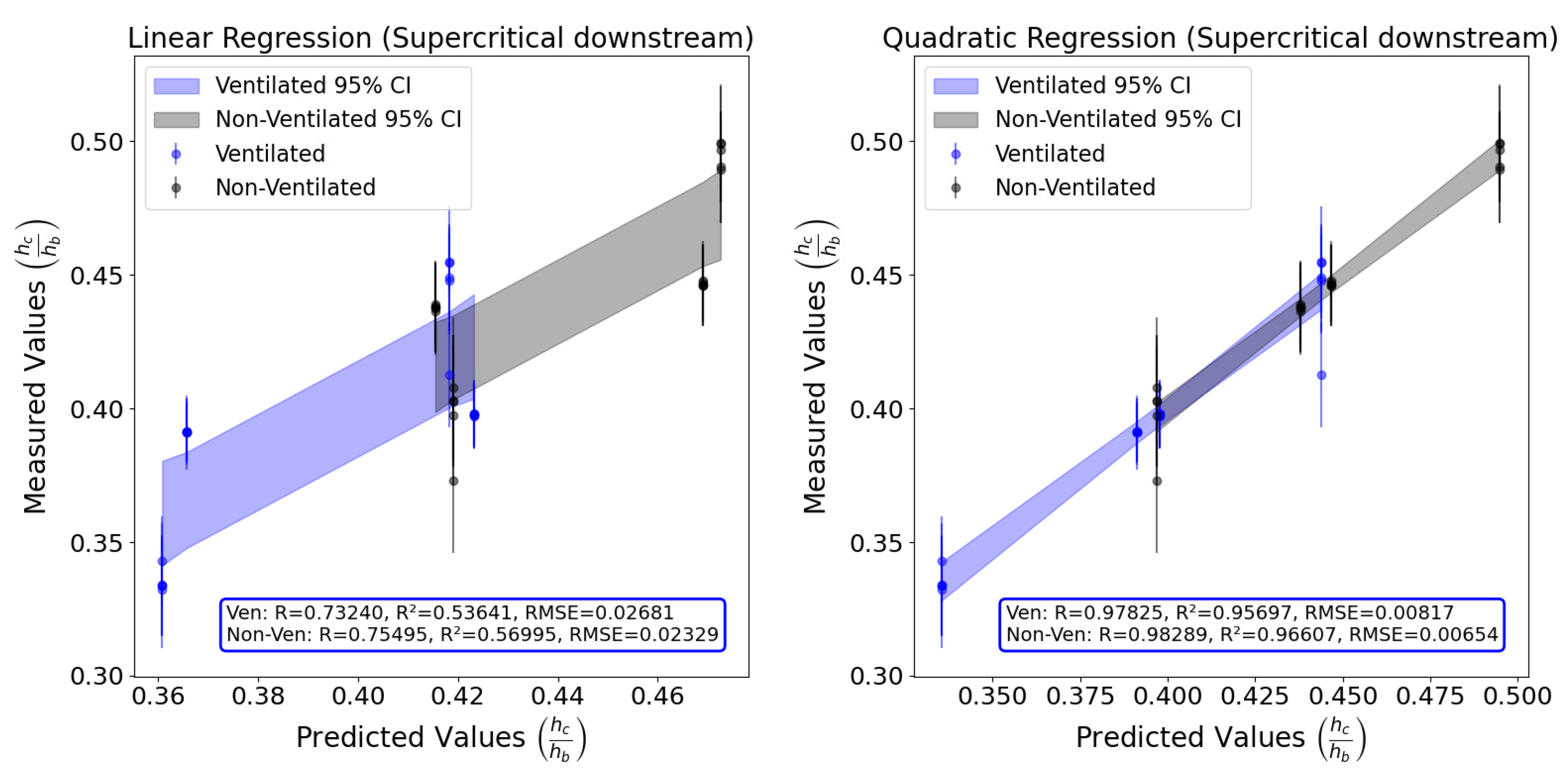
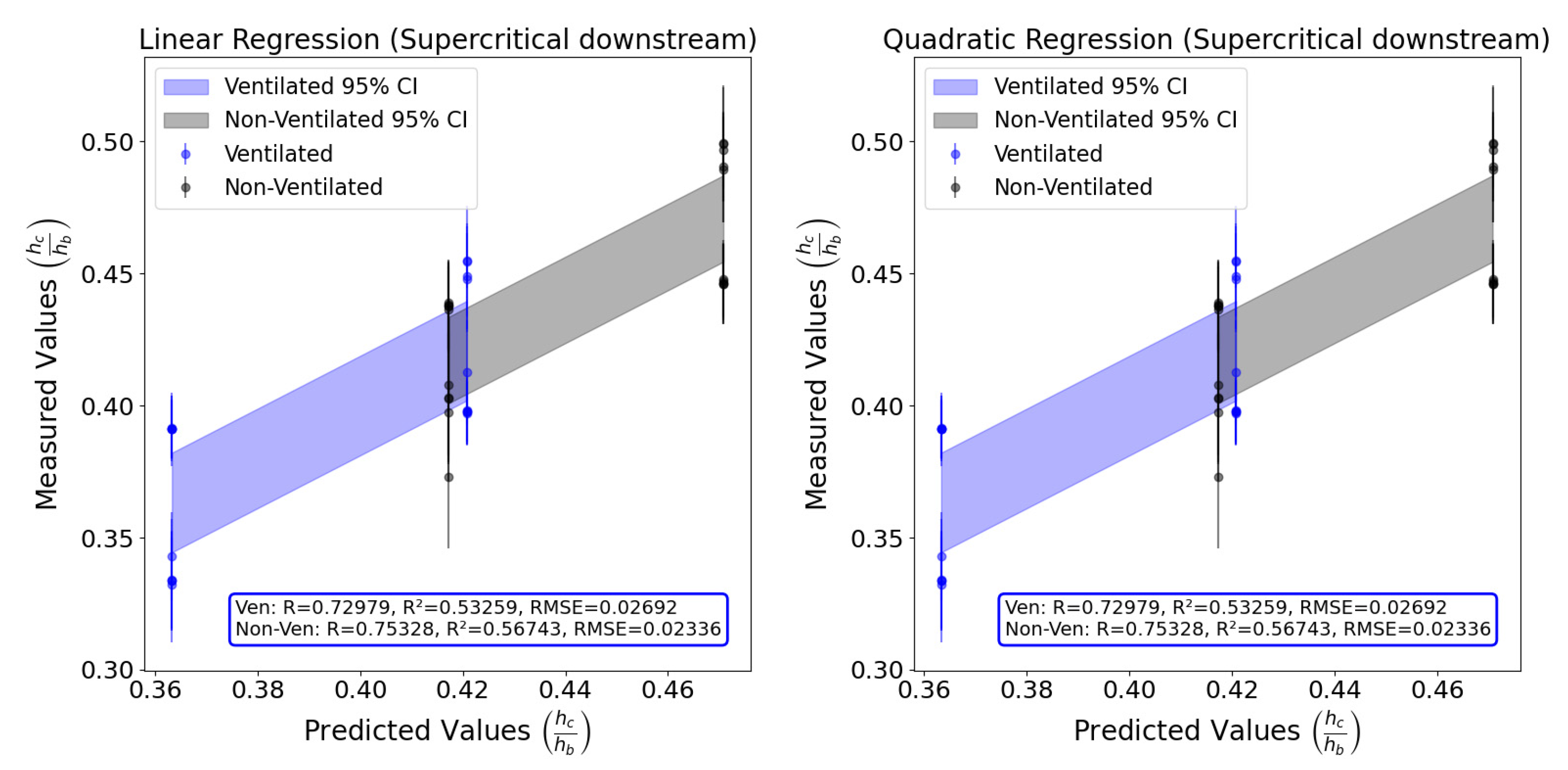
3.5. Dimensionless Equation for Cavity Water Depth with Supercritical Flow Downstream
4. Discussion
4.1. Limited Data for Supercritical Downstream Case
4.2. Steady-State Assumption
4.3. Scale Effect
5. Conclusions
- (1)
- Key Findings
- (a)
- This study is the first to express air cavity pressure and cavity water depth as functions of structure height, overflow depth, and outlet height, rather than the traditionally used unit flow discharge, making it more applicable to real-world design and construction.
- (b)
- The comparative analysis of ventilated and non-ventilated cases reveals a significant influence of cavity ventilation on air cavity pressure and cavity water depth.
- (c)
- Specifically, the non-ventilated case demonstrates lower air cavity pressure compared to the ventilated case, corroborating findings from previous research.
- (d)
- The non-ventilated case typically features deeper cavity water, aligning with historical data.
- (2)
- Derived Equations
- (a)
- Dimensionless equations for both air cavity pressure and cavity water depth across subcritical and supercritical downstream flow conditions illuminate the relationships between various hydraulic parameters-such as structure height, overflow depth, and flume outlet height-and the dependent variables.
- (b)
- The analysis showcases strong correlations with high R-squared values, underscoring the precision and robustness of the equations.
- (c)
- For air cavity pressure, the quadratic dimensionless equation was identified as the best model across different downstream flow regimes.
- (d)
- For cavity water depth, the linear equation was most suitable for subcritical conditions, while the quadratic equation provided the best fit in supercritical conditions.
- (3)
- Practical Applications
- (a)
- This study helps accurately estimate the pressure acting on structures during extreme cases, such as tsunami-induced overtopping of breakwaters. By integrating the pressure profile, it is possible to estimate the forces acting on the structure, enabling safer design.
- (b)
- Given that the experiments were conducted with appropriate Froude, Weber, and Reynolds numbers, the developed nondimensional regressions can be effectively utilized by engineers to estimate air cavity pressure and cavity water depth at prototype scale for civil engineering applications such as dam spillways, floodwalls, and caissons.
- (c)
- This will allow determination of the full horizontal force on a structure, from which design to resist sliding, overturning, and foundation punching can be robustly calculated.
- (d)
- The findings are applicable not only in coastal engineering but also in any scenario where overtopping causes a structure to function as a weir.
- (4)
- Limitations and Future Work
- (a)
- The scarcity of data points for supercritical downstream conditions limits the robustness of the derived equations. Future research should expand the dataset through additional experiments or numerical simulations.
- (b)
- The study’s reliance on time-averaged values under steady-state conditions may not fully capture dynamic behaviors. Future research should measure instantaneous values to understand real-time interactions.
- (c)
- Extrapolating laboratory data to prototype-scale applications requires considering scale-dependent effects. Future research should investigate the influence of scale on cavity water depth and pressure to ensure applicability across different scales.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Independent Variables | Average Cavity Pressure, [Pa] | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ventilated Case | Non-Ventilated Case | |||||||||||||
| [m] | [m] | [m] | 1 | 1 | ||||||||||
| 0.055 | 0.300 | 0.100 | 0.01 | −0.03 | 0.00 | 0.00 | −0.02 | −0.01 | −29.58 | −29.74 | −29.44 | −27.21 | −28.43 | −28.88 |
| 0.061 | 0.300 | 0.100 | −0.04 | −0.01 | −0.05 | −0.05 | −0.04 | −0.04 | −33.48 | −32.87 | −33.91 | −35.82 | −34.05 | −34.02 |
| 0.065 | 0.300 | 0.100 | −0.05 | −0.05 | −0.04 | −0.04 | −0.04 | −0.04 | −37.99 | −38.02 | −38.55 | −37.36 | −38.69 | −38.12 |
| 0.083 | 0.300 | 0.100 | −6.53 | −6.44 | −6.52 | −6.47 | −7.57 | −6.70 | −61.10 | −61.15 | −61.15 | −60.61 | −62.36 | −61.27 |
| 0.101 | 0.300 | 0.100 | −22.18 | −22.44 | −22.35 | −22.28 | −16.17 | −21.08 | −96.04 | −96.79 | −96.44 | −96.32 | −84.88 | −94.09 |
| 0.055 | 0.300 | 0.125 | 0.04 | −0.04 | −0.02 | −0.04 | −0.04 | −0.02 | −34.51 | −34.47 | −34.93 | −32.90 | −34.56 | −34.27 |
| 0.061 | 0.300 | 0.125 | −0.07 | −0.08 | −0.06 | −0.03 | −0.03 | −0.05 | −38.99 | −38.97 | −37.87 | −37.60 | −38.41 | −38.37 |
| 0.065 | 0.300 | 0.125 | −0.10 | −0.07 | −0.08 | −0.08 | −0.02 | −0.07 | −39.59 | −39.55 | −42.45 | −40.97 | −42.46 | −41.00 |
| 0.083 | 0.300 | 0.125 | −5.33 | −5.65 | −5.20 | −5.32 | −5.08 | −5.31 | −54.51 | −55.13 | −54.79 | −56.16 | −56.61 | −55.44 |
| 0.101 | 0.300 | 0.125 | −24.13 | −23.38 | −23.16 | −24.80 | −25.57 | −24.21 | −76.33 | −75.80 | −75.12 | −77.41 | −77.37 | −76.40 |
| 0.055 | 0.300 | 0.150 | −0.03 | 0.00 | −0.03 | −0.01 | −0.02 | −0.02 | −60.67 | −64.37 | −65.35 | −64.21 | −64.42 | −63.81 |
| 0.061 | 0.300 | 0.150 | 4.54 | 0.13 | −0.03 | −0.01 | −0.01 | 0.92 | −66.72 | −63.24 | −60.26 | −68.14 | −65.54 | −64.78 |
| 0.065 | 0.300 | 0.150 | −0.04 | −0.05 | −0.07 | −0.04 | −0.05 | −0.05 | −71.90 | −70.49 | −72.38 | −72.74 | −73.93 | −72.29 |
| 0.083 | 0.300 | 0.150 | −3.31 | −3.42 | −3.60 | −3.73 | −3.69 | −3.55 | −92.88 | −96.06 | −93.80 | −96.40 | −99.37 | −95.70 |
| 0.101 | 0.300 | 0.150 | −18.58 | −18.34 | −18.40 | −19.18 | −18.56 | −18.61 | −113.1 | −119.3 | −112.7 | −116.3 | −110.4 | −114.4 |
| Independent Variables | Standard Deviation of Cavity Pressure, [Pa] | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ventilated Case | Non-Ventilated Case | |||||||||||||
| [m] | [m] | [m] | ||||||||||||
| 0.055 | 0.300 | 0.100 | 0.22 | 0.23 | 0.20 | 0.22 | 0.22 | 0.22 | 3.10 | 3.33 | 2.91 | 2.70 | 2.85 | 2.99 |
| 0.061 | 0.300 | 0.100 | 0.20 | 0.22 | 0.22 | 0.22 | 0.23 | 0.22 | 3.69 | 3.87 | 4.29 | 3.53 | 3.70 | 3.82 |
| 0.065 | 0.300 | 0.100 | 0.23 | 0.20 | 0.23 | 0.22 | 0.22 | 0.22 | 7.18 | 6.40 | 6.06 | 5.35 | 5.48 | 6.13 |
| 0.083 | 0.300 | 0.100 | 1.18 | 1.24 | 1.15 | 1.51 | 1.56 | 1.34 | 7.46 | 7.11 | 7.89 | 6.90 | 6.96 | 7.27 |
| 0.101 | 0.300 | 0.100 | 2.13 | 2.01 | 1.98 | 2.08 | 2.62 | 2.18 | 11.31 | 11.04 | 11.96 | 9.94 | 9.05 | 10.71 |
| 0.055 | 0.300 | 0.125 | 0.24 | 0.24 | 0.24 | 0.23 | 0.24 | 0.24 | 5.05 | 4.51 | 3.27 | 3.85 | 4.16 | 4.21 |
| 0.061 | 0.300 | 0.125 | 0.23 | 0.23 | 0.23 | 0.23 | 0.24 | 0.23 | 5.26 | 5.34 | 5.42 | 5.34 | 4.83 | 5.24 |
| 0.065 | 0.300 | 0.125 | 0.23 | 0.23 | 0.23 | 0.23 | 0.22 | 0.23 | 5.08 | 5.17 | 5.78 | 5.97 | 5.15 | 5.44 |
| 0.083 | 0.300 | 0.125 | 1.66 | 1.53 | 1.55 | 1.70 | 1.61 | 1.61 | 6.06 | 5.53 | 5.57 | 6.47 | 5.67 | 5.87 |
| 0.101 | 0.300 | 0.125 | 2.30 | 2.00 | 2.05 | 1.88 | 1.93 | 2.04 | 10.09 | 8.22 | 7.68 | 8.54 | 8.90 | 8.72 |
| 0.055 | 0.300 | 0.150 | 0.23 | 0.23 | 0.23 | 0.22 | 0.22 | 0.22 | 5.67 | 5.72 | 5.39 | 6.04 | 4.53 | 5.49 |
| 0.061 | 0.300 | 0.150 | 1.20 | 0.22 | 0.23 | 0.23 | 0.24 | 0.57 | 6.51 | 6.09 | 7.75 | 6.01 | 7.52 | 6.82 |
| 0.065 | 0.300 | 0.150 | 0.22 | 0.24 | 0.23 | 0.21 | 0.23 | 0.22 | 8.59 | 7.49 | 9.02 | 8.39 | 7.97 | 8.31 |
| 0.083 | 0.300 | 0.150 | 1.57 | 1.48 | 1.51 | 1.57 | 1.62 | 1.55 | 15.83 | 17.35 | 16.84 | 13.94 | 15.63 | 15.96 |
| 0.101 | 0.300 | 0.150 | 2.21 | 2.49 | 2.39 | 2.37 | 2.34 | 2.36 | 19.57 | 19.34 | 17.64 | 18.77 | 20.41 | 19.17 |
Appendix B
| Independent Variables | Average Cavity Water Depth, [m] | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ventilated Case | Non-Ventilated Case | |||||||||||||
| [m] | [m] | [m] | ||||||||||||
| 0.055 | 0.300 | 0.100 | 0.0857 | 0.0850 | 0.0848 | 0.0851 | 0.0845 | 0.0850 | 0.0985 | 0.0982 | 0.0979 | 0.0980 | 0.0978 | 0.0981 |
| 0.061 | 0.300 | 0.100 | 0.0936 | 0.0939 | 0.0936 | 0.0933 | 0.0922 | 0.0933 | 0.1036 | 0.1034 | 0.1028 | 0.1017 | 0.1013 | 0.1026 |
| 0.065 | 0.300 | 0.100 | 0.0953 | 0.0929 | 0.0901 | 0.0893 | 0.0915 | 0.0918 | 0.1036 | 0.1029 | 0.1003 | 0.0995 | 0.1008 | 0.1014 |
| 0.083 | 0.300 | 0.100 | 0.1001 | 0.1002 | 0.1001 | 0.0997 | 0.1029 | 0.1006 | 0.1119 | 0.1209 | 0.1223 | 0.1208 | 0.1192 | 0.1190 |
| 0.101 | 0.300 | 0.100 | 0.1364 | 0.1365 | 0.1238 | 0.1344 | 0.1347 | 0.1332 | 0.1468 | 0.1490 | 0.1471 | 0.1497 | 0.1498 | 0.1485 |
| 0.055 | 0.300 | 0.125 | 0.1194 | 0.1187 | 0.1197 | 0.1189 | 0.1195 | 0.1192 | 0.1344 | 0.1334 | 0.1347 | 0.1340 | 0.1339 | 0.1341 |
| 0.061 | 0.300 | 0.125 | 0.1170 | 0.1166 | 0.1182 | 0.1172 | 0.1175 | 0.1173 | 0.1329 | 0.1327 | 0.1335 | 0.1325 | 0.1326 | 0.1328 |
| 0.065 | 0.300 | 0.125 | 0.1167 | 0.1177 | 0.1183 | 0.1181 | 0.1200 | 0.1182 | 0.1340 | 0.1324 | 0.1334 | 0.1329 | 0.1337 | 0.1333 |
| 0.083 | 0.300 | 0.125 | 0.1174 | 0.1173 | 0.1175 | 0.1173 | 0.1174 | 0.1174 | 0.1317 | 0.1309 | 0.1315 | 0.1314 | 0.1313 | 0.1314 |
| 0.101 | 0.300 | 0.125 | 0.1193 | 0.1194 | 0.1192 | 0.1191 | 0.1195 | 0.1193 | 0.1344 | 0.1338 | 0.1338 | 0.1341 | 0.1339 | 0.1340 |
| 0.055 | 0.300 | 0.150 | 0.1545 | 0.1505 | 0.1518 | 0.1549 | 0.1574 | 0.1538 | 0.1709 | 0.1674 | 0.1701 | 0.1737 | 0.1699 | 0.1704 |
| 0.061 | 0.300 | 0.150 | 0.1578 | 0.1584 | 0.1578 | 0.1610 | 0.1591 | 0.1588 | 0.1718 | 0.1690 | 0.1660 | 0.1648 | 0.1682 | 0.1680 |
| 0.065 | 0.300 | 0.150 | 0.1527 | 0.1554 | 0.1558 | 0.1565 | 0.1588 | 0.1558 | 0.1696 | 0.1697 | 0.1692 | 0.1713 | 0.1712 | 0.1702 |
| 0.083 | 0.300 | 0.150 | 0.1521 | 0.1540 | 0.1549 | 0.1562 | 0.1540 | 0.1542 | 0.1698 | 0.1699 | 0.1701 | 0.1720 | 0.1713 | 0.1706 |
| 0.101 | 0.300 | 0.150 | 0.1547 | 0.1563 | 0.1545 | 0.1537 | 0.1538 | 0.1546 | 0.1680 | 0.1682 | 0.1673 | 0.1701 | 0.1692 | 0.1686 |
| Independent Variables | Standard Deviation of Cavity Water Depth, [m] | |||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ventilated Case | Non-Ventilated Case | |||||||||||||
| [m] | [m] | [m] | ||||||||||||
| 0.055 | 0.300 | 0.100 | 0.0036 | 0.0035 | 0.0035 | 0.0033 | 0.0035 | 0.0035 | 0.0021 | 0.0022 | 0.0021 | 0.0020 | 0.0019 | 0.0021 |
| 0.061 | 0.300 | 0.100 | 0.0040 | 0.0043 | 0.0042 | 0.0057 | 0.0058 | 0.0049 | 0.0035 | 0.0034 | 0.0034 | 0.0076 | 0.0074 | 0.0054 |
| 0.065 | 0.300 | 0.100 | 0.0064 | 0.0067 | 0.0044 | 0.0042 | 0.0073 | 0.0059 | 0.0068 | 0.0069 | 0.0055 | 0.0051 | 0.0061 | 0.0061 |
| 0.083 | 0.300 | 0.100 | 0.0070 | 0.0055 | 0.0057 | 0.0053 | 0.0050 | 0.0057 | 0.0081 | 0.0073 | 0.0079 | 0.0075 | 0.0078 | 0.0077 |
| 0.101 | 0.300 | 0.100 | 0.0063 | 0.0031 | 0.0059 | 0.0060 | 0.0060 | 0.0056 | 0.0060 | 0.0043 | 0.0063 | 0.0064 | 0.0066 | 0.0060 |
| 0.055 | 0.300 | 0.125 | 0.0033 | 0.0024 | 0.0028 | 0.0026 | 0.0029 | 0.0028 | 0.0043 | 0.0039 | 0.0045 | 0.0042 | 0.0041 | 0.0042 |
| 0.061 | 0.300 | 0.125 | 0.0034 | 0.0033 | 0.0033 | 0.0033 | 0.0035 | 0.0034 | 0.0044 | 0.0045 | 0.0041 | 0.0042 | 0.0040 | 0.0042 |
| 0.065 | 0.300 | 0.125 | 0.0028 | 0.0043 | 0.0051 | 0.0048 | 0.0044 | 0.0044 | 0.0042 | 0.0044 | 0.0050 | 0.0049 | 0.0043 | 0.0046 |
| 0.083 | 0.300 | 0.125 | 0.0033 | 0.0042 | 0.0036 | 0.0033 | 0.0037 | 0.0036 | 0.0047 | 0.0049 | 0.0050 | 0.0052 | 0.0049 | 0.0049 |
| 0.101 | 0.300 | 0.125 | 0.0036 | 0.0038 | 0.0036 | 0.0036 | 0.0036 | 0.0036 | 0.0044 | 0.0046 | 0.0045 | 0.0044 | 0.0045 | 0.0045 |
| 0.055 | 0.300 | 0.150 | 0.0066 | 0.0059 | 0.0063 | 0.0080 | 0.0061 | 0.0066 | 0.0057 | 0.0057 | 0.0053 | 0.0057 | 0.0040 | 0.0053 |
| 0.061 | 0.300 | 0.150 | 0.0029 | 0.0024 | 0.0040 | 0.0018 | 0.0041 | 0.0032 | 0.0019 | 0.0025 | 0.0027 | 0.0022 | 0.0034 | 0.0026 |
| 0.065 | 0.300 | 0.150 | 0.0046 | 0.0040 | 0.0046 | 0.0029 | 0.0024 | 0.0038 | 0.0024 | 0.0024 | 0.0024 | 0.0020 | 0.0021 | 0.0023 |
| 0.083 | 0.300 | 0.150 | 0.0042 | 0.0044 | 0.0045 | 0.0021 | 0.0024 | 0.0037 | 0.0025 | 0.0028 | 0.0026 | 0.0023 | 0.0021 | 0.0025 |
| 0.101 | 0.300 | 0.150 | 0.0040 | 0.0047 | 0.0037 | 0.0016 | 0.0022 | 0.0034 | 0.0028 | 0.0028 | 0.0033 | 0.0033 | 0.0034 | 0.0031 |
References
- Seed, R.B.; Bea, R.G.; Athanasopoulos–Zekkos, A.; Boutwell, G.P.; Bray, J.D.; Cheung, C.; Cobos–Roa, D.; Ehrensing, L.; Harder, L.F.; Pestana, J.M.; et al. New Orleans and Hurricane Katrina. II: The Central Region and the Lower Ninth Ward. J. Geotech. Geoenvironmental Eng. 2008, 134, 718–739. [Google Scholar] [CrossRef]
- Satake, K.; Aung, T.T.; Sawai, Y.; Okamura, Y.; Win, K.S.; Swe, W.; Swe, C.; Swe, T.L.; Tun, S.T.; Soe, M.M.; et al. Tsunami heights and damage along the Myanmar coast from the December 2004 Sumatra–Andaman earthquake. Earth Planets Space 2006, 58, 243–252. [Google Scholar] [CrossRef][Green Version]
- Mori, N.; Cox, D.T.; Yasuda, T.; Mase, H. Overview of the 2011 Tohoku Earthquake Tsunami Damage and Its Relation to Coastal Protection along the Sanriku Coast. Earthq. Spectra 2013, 29, 127–143. [Google Scholar] [CrossRef]
- Mikami, T.; Shibayama, T.; Takagi, H.; Matsumaru, R.; Esteban, M.; Thao, N.D.; Leon, M.D.; Valenzuela, V.P.; Oyama, T.; Nakamura, R.; et al. Storm Surge Heights and Damage Caused by the 2013 Typhoon Haiyan Along the Leyte Gulf Coast. Coast. Eng. J. 2016, 58, 1640005. [Google Scholar] [CrossRef]
- Arikawa, T.; Sato, M.; Shimosako, K.; Hasegawa, I.; Yeom, G.-S.; Tomita, T. Failure mechanism of Kamaishi breakwaters due to the Great East Japan earthquake tsunami. Coast. Eng. 2012, 3, 1–13. [Google Scholar]
- Bricker, J.D.; Takagi, H.; Mitsui, J. Turbulence model effects on VOF analysis of breakwater overtopping during the 2011 Great East Japan Tsunami. In Proceedings of the 2013 IAHR World Congress, Chengdu, China, 8–13 September 2013; Tsinghua University Press: Beijing, China, 2013. [Google Scholar]
- Jayaratne, R.; Abimola, A.; Mikami, T.; Matsuba, S.; Esteban, M.; Shibayama, T. Predictive model for scour depth of coastal structure failures due to tsunamis. Coast. Eng. Proc. 2014, 1, 56. [Google Scholar] [CrossRef]
- Chock, G.; Robertson, I.; Kriebel, D.; Francis, M.; Nistor, I. Chapter 13: Scour Effects. In Tohoku, Japan, Earthquake and Tsunami of 2011; American Society of Civil Engineers: Reston, VA, USA, 2013; pp. 250–283. [Google Scholar]
- Gao, J.; Ma, X.; Zang, J.; Dong, G.; Ma, X.; Zhu, Y.; Zhou, L. Numerical investigation of harbor oscillations induced by focused transient wave groups. Coast. Eng. 2020, 158, 103670. [Google Scholar] [CrossRef]
- Gao, J.; Shi, H.; Zang, J.; Liu, Y. Mechanism analysis on the mitigation of harbor resonance by periodic undulating topography. Ocean Eng. 2023, 281, 114923. [Google Scholar] [CrossRef]
- Gao, J.; Hou, L.; Liu, Y.; Shi, H. Influences of bragg reflection on harbor resonance triggered by irregular wave groups. Ocean Eng. 2024, 305, 117941. [Google Scholar] [CrossRef]
- Chanson, H. Chapter 16: Ventilated Cavity Flow. In Air Bubble Entrainment in Free–Surface Turbulent Shear Flows; Elsevier: Amsterdam, The Netherlands, 1996; pp. 189–199. [Google Scholar]
- Disanayaka Mudiyanselage, S. Effect of Nappe Non–aeration on Caisson Sliding Force during Tsunami Breakwater Overtopping. Master’s Thesis, Delft University of Technology, TU Delft Repositories, Delft, The Netherlands, 2017. [Google Scholar]
- Patil, A.; Mudiyanselage, S.D.; Bricker, J.D.; Uijttewaal, W.; Keetels, G. Effect of Overflow Nappe Non--Aeration on Tsunami Breakwater Failure. Coast. Eng. Proc. 2018, 1, 18. [Google Scholar] [CrossRef]
- Kim, T.; Malherbe, J.; Shimpalee, S.; Bricker, J. SUB–Nappe Air Cavity Pressure during Overflow of a Vertical Structure. Coast. Eng. Proc. 2023, 37, 4. [Google Scholar] [CrossRef]
- Zou, P.; Kim, T.; Bricker, J.D.; Uijttewaal, W.S.J. Assessment of interfacial turbulence treatment models for free surface flows. J. Hydraul. Res. 2023, 61, 651–667. [Google Scholar] [CrossRef]
- Goda, Y. Chapter 4: Design of Vertical Breakwaters. In Random Seas and Design of Maritime Structures; World Scientific Publishing Company: Singapore, 2010; Volume 33, pp. 161–200. [Google Scholar]
- Tanimoto, K.; Takahashi, S. Design and construction of caisson breakwaters—the Japanese experience. Coast. Eng. 1994, 22, 57–77. [Google Scholar] [CrossRef]
- Yoshida, K.; Maeno, S.; Iiboshi, T.; Araki, D. Estimation of hydrodynamic forces acting on concrete blocks of toe protection works for coastal dikes by tsunami overflows. Appl. Ocean Res. 2018, 80, 181–196. [Google Scholar] [CrossRef]
- Hess, F.; Boes, R.M.; Evers, F.M. Forces on a Vertical Dam due to Solitary Impulse Wave Run–Up and Overtopping. J. Hydraul. Eng. 2023, 149, 04023020. [Google Scholar] [CrossRef]
- Chapter 6: TSUNAMI LOADS AND EFFECTS. In ASCE/SCI 7–22 Minimum Design Loads and Associated Criteria for Buildings and Other Structures; American Society of Civil Engineers: Reston, VA, USA, 2021; pp. 25–54.
- Esteban, M.; Roubos, J.J.; Iimura, K.; Salet, J.T.; Hofland, B.; Bricker, J.; Ishii, H.; Hamano, G.; Takabatake, T.; Shibayama, T. Effect of bed roughness on tsunami bore propagation and overtopping. Coast. Eng. 2020, 157, 103539. [Google Scholar] [CrossRef]
- Bricker, J.D.; Nakayama, A. Contribution of Trapped Air, Deck Superelevation, and Nearby Structures to Bridge Deck Failure during a Tsunami. J. Hydraul. Eng. 2014, 140, 05014002. [Google Scholar] [CrossRef]
- Motley, M.R.; Wong, H.K.; Qin, X.; Winter, A.O.; Eberhard, M.O. Tsunami–Induced Forces on Skewed Bridges. J. Waterw. Port Coast. Ocean Eng. 2016, 142, 04015025. [Google Scholar] [CrossRef]
- Michel, J.M. Some Features of Water Flows with Ventilated Cavities. J. Fluids Eng. 1984, 106, 319–326. [Google Scholar] [CrossRef]
- Laali, A.R.; Michel, J.M. Air Entrainment in Ventilated Cavities: Case of the Fully Developed “Half–Cavity”. J. Fluids Eng. 1984, 106, 327–335. [Google Scholar] [CrossRef]
- Anderson, A.; Tullis, B.P. Finite Crest Length Weir Nappe Oscillation. J. Hydraul. Eng. 2018, 144, 04018020. [Google Scholar] [CrossRef]
- Lodomez, M.; Pirotton, M.; Dewals, B.; Archambeau, P.; Erpicum, S. Nappe Oscillations on Free–Overfall Structures: Experimental Analysis. J. Hydraul. Eng. 2018, 144, 04018001. [Google Scholar] [CrossRef]
- Lodomez, M.; Tullis, B.P.; Dewals, B.; Archambeau, P.; Pirotton, M.; Erpicum, S. Nappe Oscillations on Free–Overfall Structures: Size Scale Effects. J. Hydraul. Eng. 2019, 145, 04019022. [Google Scholar] [CrossRef]
- Moore, W.L. Energy Loss at the Base of A Free Overfall. Trans. Am. Soc. Civ. Eng. 1943, 108, 1343–1360. [Google Scholar] [CrossRef]
- Rand, W.H. Flow Geometry at Straight Drop Spillways. In Proceedings of the American Society of Civil Engineers; American Society of Civil Engineers: Reston, VA, USA, 1955; pp. 1–13. [Google Scholar]
- Rajaratnam, N.; Chamani, M.R. Energy loss at drops. J. Hydraul. Res. 1995, 33, 373–384. [Google Scholar] [CrossRef]
- Toombes, L.; Wagner, C.; Chanson, H. Flow patterns in nappe flow regime down low gradient stepped chutes. J. Hydraul. Res. 2008, 46, 4–14. [Google Scholar] [CrossRef]
- Castillo, L.G.; Carrillo, J.M. Analysis of the scale ratio in nappe flow case by means of CFD numerical simulation. In Proceedings of the 2013 IAHR World Congress, Chengdu, China, 8–13 September 2013; Tsinghua University Press: Beijing, China, 2013; pp. 8–13. [Google Scholar]
- Canny, J. A Computational Approach to Edge Detection. IEEE Trans. Pattern Anal. Mach. Intell. 1986, PAMI–8, 679–698. [Google Scholar] [CrossRef]
- Darcy, H. Les Fontaines Publiques de la Ville de Dijon: Exposition et Application des Principes à Suivre et des Formules à Employer Dans Les Questions de Distribution d’eau; Victor Dalmont: Paris, France, 1856; Volume 1. [Google Scholar]
- Weisbach, J. Lehrbuch der Ingenieur–und Maschinen–Mechanik; F. Vieweg und Sohn: Braunschweig, Germany, 1850; Volume 1. [Google Scholar]
- Roberson, J.A.; Cassidy, J.J.; Chaudhry, M.H. Hydraulic Engineering; John Wiley & Sons: Hoboken, NJ, USA, 1998. [Google Scholar]
- Mäkiharju, S.A.; Elbing, B.R.; Wiggins, A.; Schinasi, S.; Vanden–Broeck, J.-M.; Perlin, M.; Dowling, D.R.; Ceccio, S.L. On the scaling of air entrainment from a ventilated partial cavity. J. Fluid Mech. 2013, 732, 47–76. [Google Scholar] [CrossRef]
- Rouse, H. Discharge characteristics of the free overfall: Use of crest section as a control provides easy means of measuring discharge. Civ. Eng. 1936, 6, 257–260. [Google Scholar]
- Jain, A.K.; Garde, R.J.; Raju, K.G.R. Vortex Formation at Vertical Pipe Intakes. J. Hydraul. Div. 1978, 104, 1429–1445. [Google Scholar] [CrossRef]
- Felder, S.; Chanson, H. Scale effects in microscopic air–water flow properties in high–velocity free–surface flows. Exp. Therm. Fluid Sci. 2017, 83, 19–36. [Google Scholar] [CrossRef]
- Felder, S.; Geuzaine, M.; Dewals, B.; Erpicum, S. Nappe flows on a stepped chute with prototype–scale steps height: Observations of flow patterns, air–water flow properties, energy dissipation and dissolved oxygen. J. Hydro Environ. Res. 2019, 27, 1–19. [Google Scholar] [CrossRef]
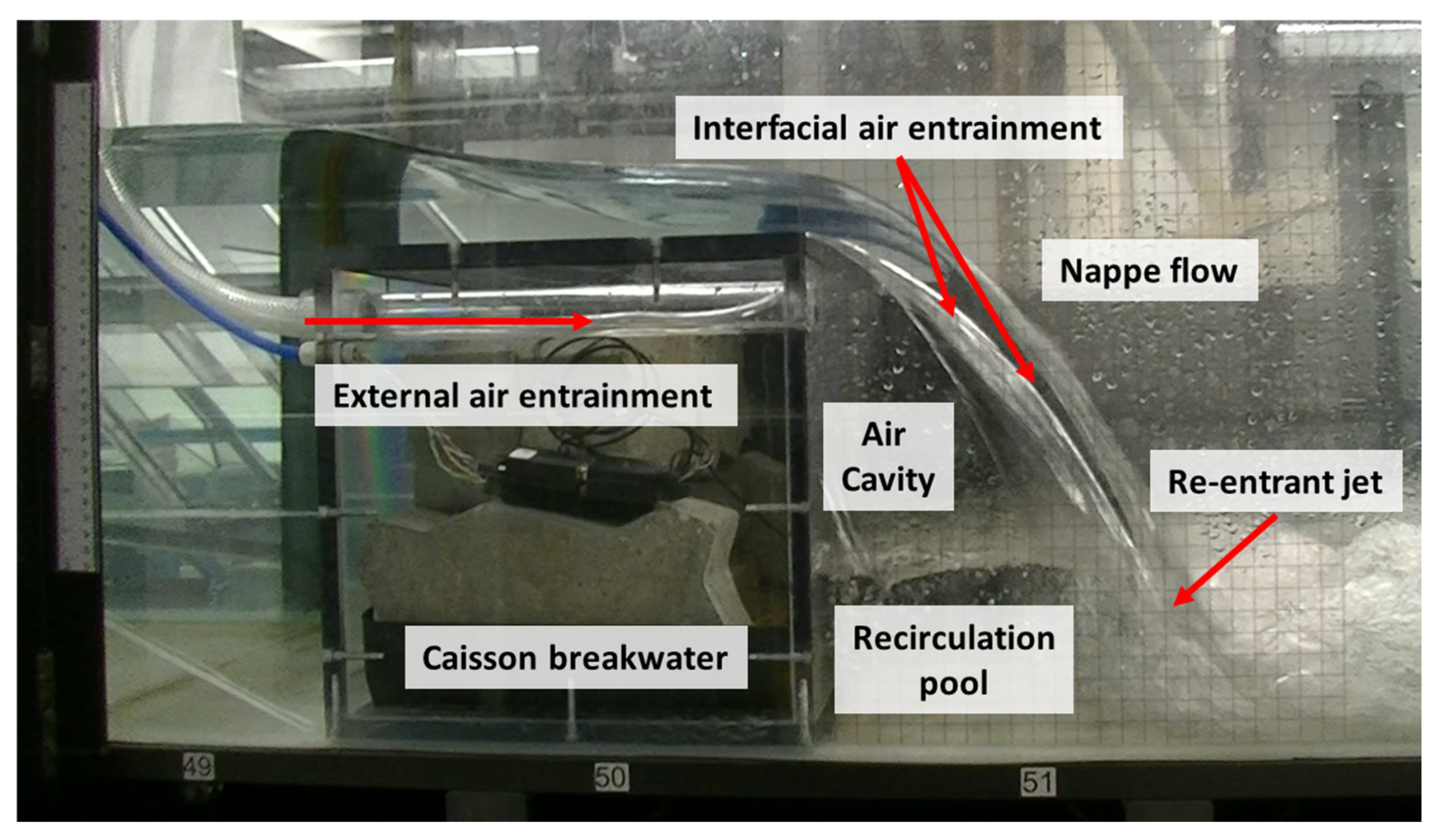
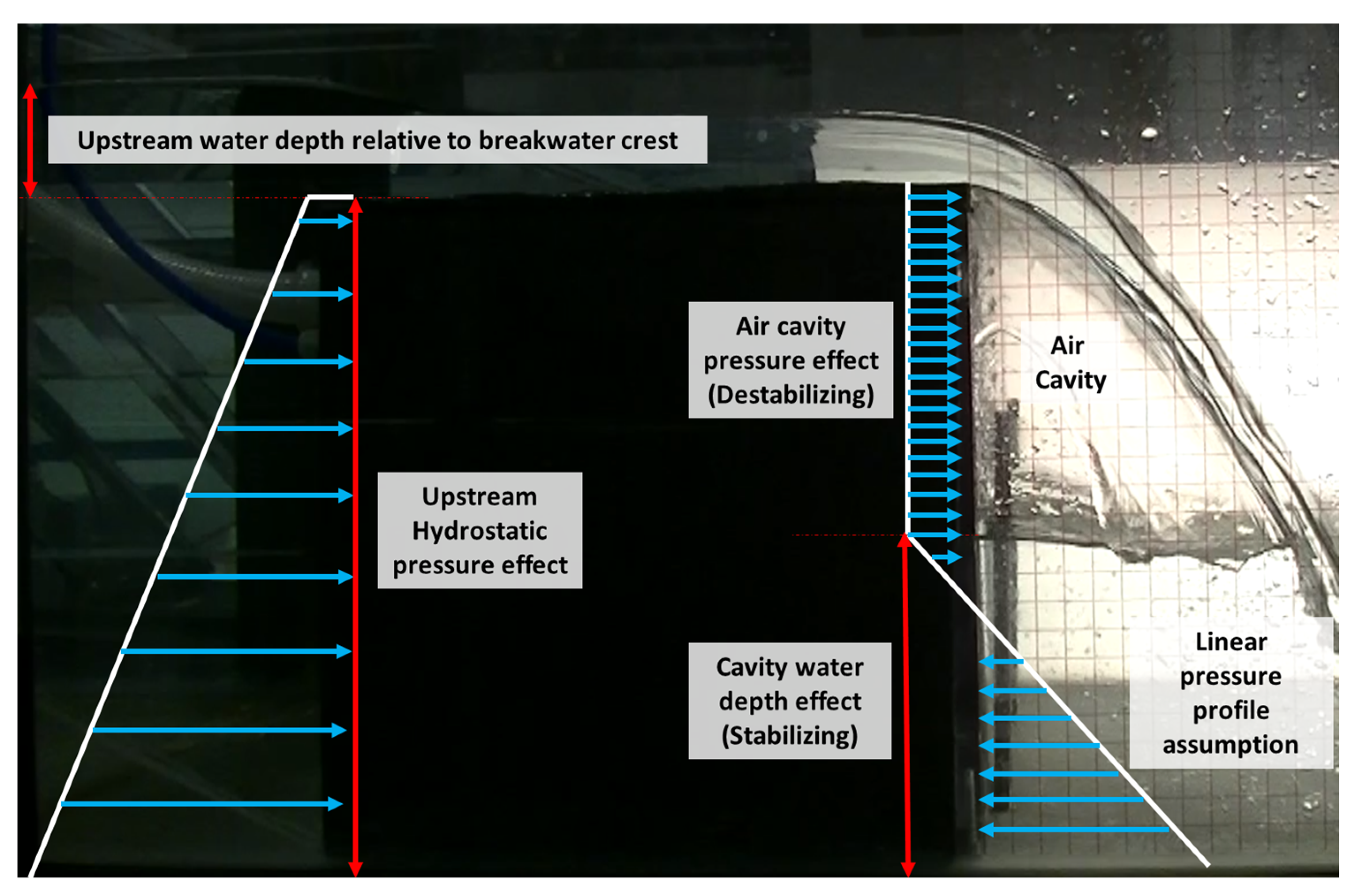

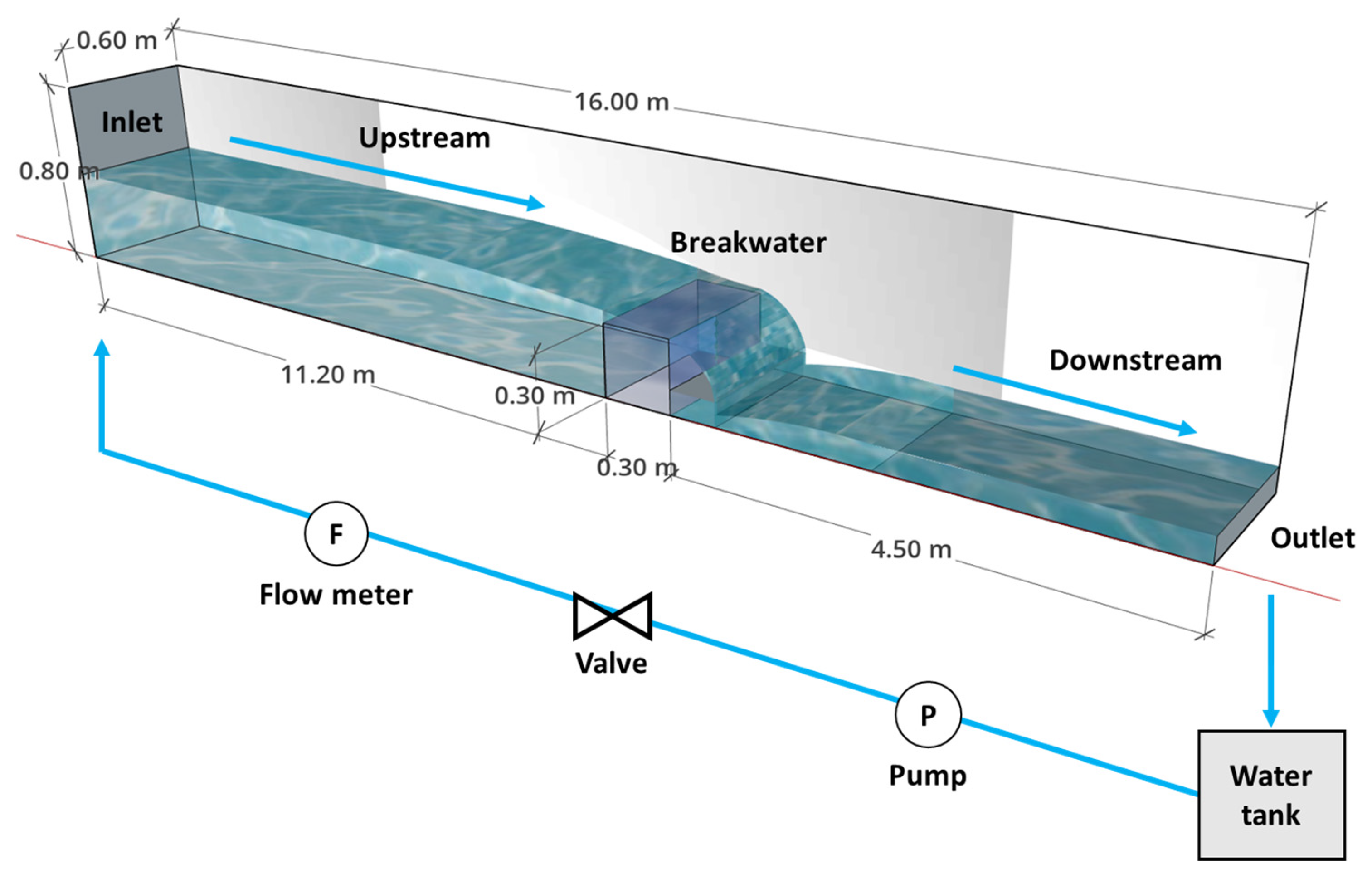


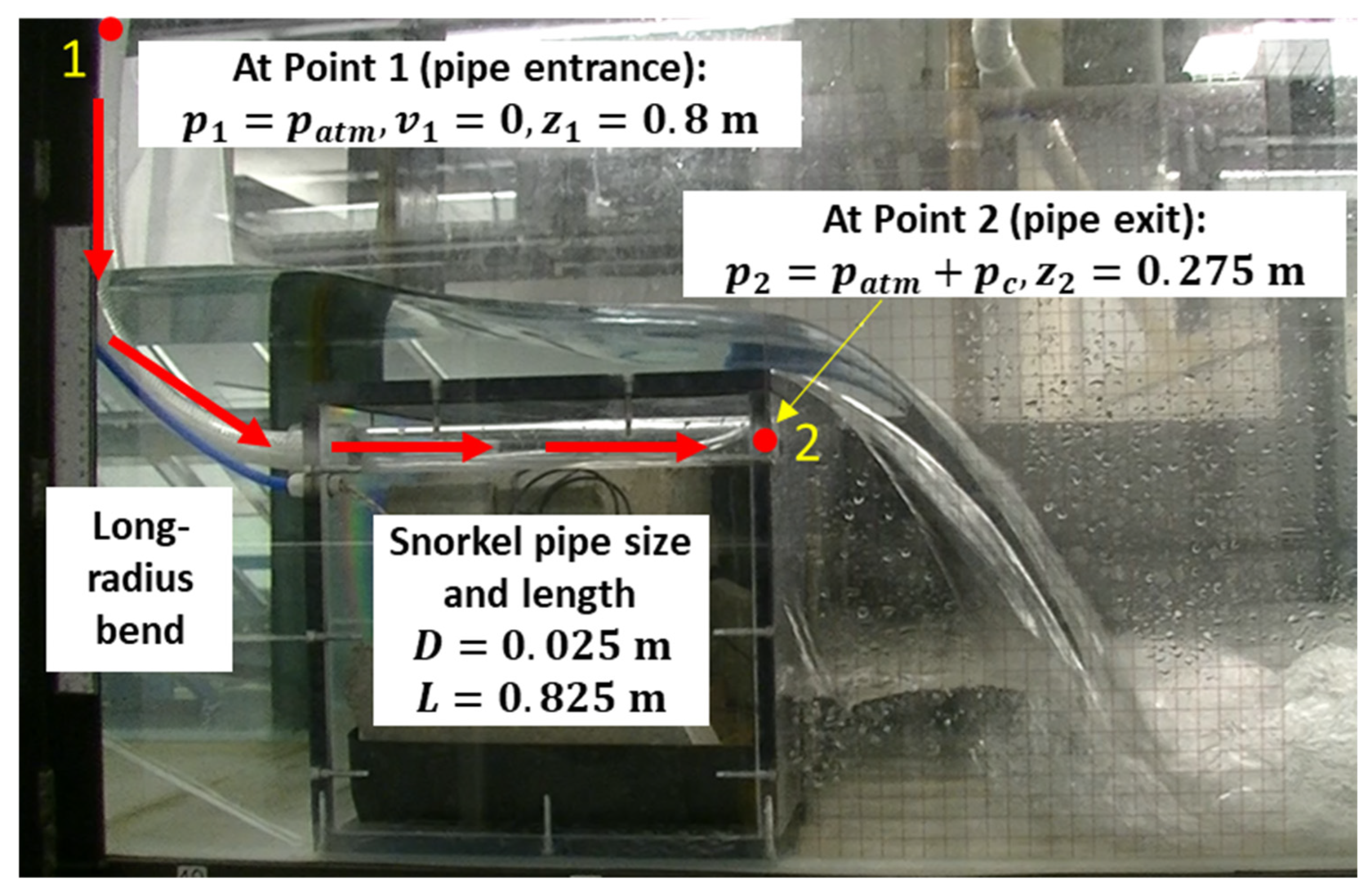



| Variables | Values | Unit | |
|---|---|---|---|
| Independent variables | Upstream depth relative to crest | 0.055, 0.061, 0.065, 0.083, and 0.101 (5 cases) | [m] |
| Caisson breakwater height | 0.300 (1 case) | [m] | |
| Downstream flume outlet height | 0.100, 0.125, and 0.150 (3 cases) | [m] | |
| Cavity ventilation condition | Ventilated or Non-ventilated (2 cases) | [] | |
| Dependent variables | Air cavity pressure | Measured | [Pa] |
| Cavity water depth | Measured | [m] | |
| Dependent Variable | Downstream Flow Regime | Dimensionless Equation | ||||
|---|---|---|---|---|---|---|
| Equation Form | ||||||
| Air cavity pressure | Subcritical | (4) | ||||
| Supercritical | (4) | |||||
| (5) | ||||||
| Cavity water depth | Subcritical | (4) | ||||
| Supercritical | (4) | |||||
| (5) | ||||||
| Independent Variables | Ventilated Case | Non-Ventilated Case | Downstream Regime | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
[m] | [m] | [m] | [Pa] | [m] | [m] | [lpm] | [Pa] | [m] | [m] | ||||
| 0.055 | 0.300 | 0.100 | −0.01 | 0.086 | 0.070 | 0.329 | 2656 | 46.3 | −28.88 | 0.095 | 0.070 | 0.329 | Subcritical |
| 0.061 | 0.300 | 0.100 | −0.04 | 0.088 | 0.070 | 0.410 | 2663 | 46.4 | −34.02 | 0.093 | 0.067 | 0.410 | Subcritical |
| 0.065 | 0.300 | 0.100 | −0.04 | 0.088 | 0.064 | 0.488 | 2664 | 46.4 | −38.12 | 0.093 | 0.064 | 0.488 | Subcritical |
| 0.083 | 0.300 | 0.100 | −6.70 | 0.093 | 0.013 | 7.876 | 3761 | 65.5 | −61.27 | 0.108 | 0.013 | 7.876 | Supercritical |
| 0.101 | 0.300 | 0.100 | −21.08 | 0.108 | 0.013 | 10.572 | 5419 | 94.5 | −94.09 | 0.121 | 0.013 | 10.572 | Supercritical |
| 0.055 | 0.300 | 0.125 | −0.02 | 0.112 | 0.102 | 0.188 | 2659 | 46.4 | −34.27 | 0.122 | 0.102 | 0.188 | Subcritical |
| 0.061 | 0.300 | 0.125 | −0.05 | 0.110 | 0.100 | 0.240 | 2665 | 46.5 | −38.37 | 0.122 | 0.095 | 0.240 | Subcritical |
| 0.065 | 0.300 | 0.125 | −0.07 | 0.110 | 0.089 | 0.295 | 2669 | 46.5 | −41.00 | 0.123 | 0.089 | 0.295 | Subcritical |
| 0.083 | 0.300 | 0.125 | −5.31 | 0.105 | 0.013 | 7.876 | 3560 | 62.1 | −55.44 | 0.114 | 0.013 | 7.876 | Supercritical |
| 0.101 | 0.300 | 0.125 | −24.21 | 0.110 | 0.013 | 10.572 | 5716 | 99.7 | −76.40 | 0.121 | 0.013 | 10.572 | Supercritical |
| 0.055 | 0.300 | 0.150 | −0.02 | 0.141 | 0.133 | 0.125 | 2658 | 46.3 | −63.81 | 0.159 | 0.140 | 0.125 | Subcritical |
| 0.061 | 0.300 | 0.150 | 0.92 | 0.137 | 0.120 | 0.170 | 2464 | 43.0 | −64.78 | 0.152 | 0.121 | 0.170 | Subcritical |
| 0.065 | 0.300 | 0.150 | −0.05 | 0.138 | 0.114 | 0.202 | 2665 | 46.5 | −72.29 | 0.156 | 0.114 | 0.202 | Subcritical |
| 0.083 | 0.300 | 0.150 | −3.55 | 0.137 | 0.102 | 0.348 | 3287 | 57.3 | −95.70 | 0.159 | 0.102 | 0.348 | Subcritical |
| 0.101 | 0.300 | 0.150 | −18.61 | 0.133 | 0.089 | 0.572 | 5172 | 90.2 | −114.4 | 0.152 | 0.089 | 0.572 | Subcritical |
| Dimensionless Parameters | Dimensionless Equation (Subcritical Downstream) | ||||||
|---|---|---|---|---|---|---|---|
| Goodness of fit | Coefficients | Ventilated/ non-ventilated | Coefficients | Ventilated/ non-ventilated | |||
| Indicator | Ventilated/ non-ventilated | −0.095/−0.137 | 1.343/−0.699 | ||||
| −0.611/2.440 | 3.026/−2.400 | ||||||
| R | 0.984/0.995 | 0.324/−1.043 | −0.266/0.397 | ||||
| R-squared | 0.969/0.989 | 0.542/0.150 | −1.051/2.523 | ||||
| RMSE | 0.001/0.003 | −2.660/−3.669 | −1.011/−0.697 | ||||
| Dimensionless Parameters | Dimensionless Equation (Supercritical Downstream) | ||||||
|---|---|---|---|---|---|---|---|
| Goodness of fit | Coefficients | Ventilated/ non-ventilated | Coefficients | Ventilated/ non-ventilated | |||
| Indicator | Ventilated/ non-ventilated | −0.010/−0.045 | 0.000/0.000 | ||||
| 0.000/0.000 | 0.012/−0.018 | ||||||
| R | 0.985/0.971 | 0.000/0.000 | 0.000/−0.001 | ||||
| R-squared | 0.971/0.942 | 0.122/−0.184 | −0.022/0.048 | ||||
| RMSE | 0.001/0.002 | 0.000/0.000 | 0.091/−0.138 | ||||
| Dimensionless Parameters | Dimensionless Equation without (Supercritical Downstream) | ||||||
|---|---|---|---|---|---|---|---|
| Goodness of fit | Coefficients | Ventilated/ non-ventilated | Coefficients | Ventilated/ non-ventilated | |||
| Indicator | Ventilated/ non-ventilated | −0.010/−0.045 | 0.000/−0.010 | ||||
| 0.000/0.000 | −0.022/−0.048 | ||||||
| R | 0.985/0.971 | 0.122/−0.185 | 0.092/−0.139 | ||||
| R-squared | 0.971/0.942 | ||||||
| RMSE | 0.001/0.002 | ||||||
| Dimensionless Parameters | Dimensionless Equation (Subcritical Downstream) | ||||||
|---|---|---|---|---|---|---|---|
| Goodness of fit | Coefficients | Ventilated/non-ventilated | |||||
| Indicator | Ventilated/ non-ventilated | −0.221/−0.153 | |||||
| −0.781/−0.257 | |||||||
| R | 0.984/0.995 | 0.265/0.087 | |||||
| R-squared | 0.969/0.989 | 1.708/1.510 | |||||
| RMSE | 0.001/0.003 | ||||||
| Dimensionless Parameters | Dimensionless Equation (Supercritical Downstream) | ||||||
|---|---|---|---|---|---|---|---|
| Goodness of fit | Coefficients | Ventilated/-ventilated | Coefficients | Ventilated/-ventilated | |||
| Indicator | Ventilated/-ventilated | −0.254/−0.098 | 0.000/0.000 | ||||
| 0.000/0.000 | 0.264/0.225 | ||||||
| R | 0.978/0.983 | 0.001/0.000 | 0.010/0.009 | ||||
| R-squared | 0.957/0.966 | 2.704/2.307 | −0.460/−0.403 | ||||
| RMSE | 0.008/0.007 | 0.000/0.000 | 2.028/1.730 | ||||
| Dimensionless Parameters | Dimensionless Equation without (Supercritical Downstream) | ||||||
|---|---|---|---|---|---|---|---|
| Goodness of fit | Coefficients | Ventilated/ non-ventilated | Coefficients | Ventilated/ non-ventilated | |||
| Indicator | Ventilated/ non-ventilated | −0.252/−0.097 | 0.010/0.009 | ||||
| 0.001/0.000 | −0.455/−0.398 | ||||||
| R | 0.978/0.983 | 2.721/2.321 | 2.041/1.741 | ||||
| R-squared | 0.957/0.966 | ||||||
| RMSE | 0.008/0.007 | ||||||
| Independent Variable | Dimensionless Numbers | Air Cavity Pressure [Pa] | Cavity Water Depth [m] | ||||||
|---|---|---|---|---|---|---|---|---|---|
| [m] | [m] | [m] | Ventilated | Non- Ventilated | Ventilated | Non- Ventilated | |||
| 0.055 | 0.300 | 0.100 | 1.654 | 209 | 19,042 | −0.01 | −28.88 | 0.086 | 0.095 |
| 0.061 | 0.300 | 0.100 | 1.654 | 257 | 22,241 | −0.04 | −34.02 | 0.088 | 0.093 |
| 0.065 | 0.300 | 0.100 | 1.654 | 292 | 24,464 | −0.04 | −38.12 | 0.088 | 0.093 |
| 0.083 | 0.300 | 0.100 | 1.654 | 476 | 35,300 | −6.70 | −61.27 | 0.093 | 0.108 |
| 0.101 | 0.300 | 0.100 | 1.654 | 705 | 47,385 | −21.08 | −94.09 | 0.108 | 0.121 |
| 0.055 | 0.300 | 0.125 | 1.654 | 209 | 19,042 | −0.02 | −34.27 | 0.112 | 0.122 |
| 0.061 | 0.300 | 0.125 | 1.654 | 257 | 22,241 | −0.05 | −38.37 | 0.110 | 0.122 |
| 0.065 | 0.300 | 0.125 | 1.654 | 292 | 24,464 | −0.07 | −41.00 | 0.110 | 0.123 |
| 0.083 | 0.300 | 0.125 | 1.654 | 476 | 35300 | −5.31 | −55.44 | 0.105 | 0.114 |
| 0.101 | 0.300 | 0.125 | 1.654 | 705 | 47,385 | −24.21 | −76.40 | 0.110 | 0.121 |
| 0.055 | 0.300 | 0.150 | 1.654 | 209 | 19,042 | −0.02 | −63.81 | 0.141 | 0.159 |
| 0.061 | 0.300 | 0.150 | 1.654 | 257 | 22,241 | 0.92 | −64.78 | 0.137 | 0.152 |
| 0.065 | 0.300 | 0.150 | 1.654 | 292 | 24,464 | −0.05 | −72.29 | 0.138 | 0.156 |
| 0.083 | 0.300 | 0.150 | 1.654 | 476 | 35,300 | −3.55 | −95.70 | 0.137 | 0.159 |
| 0.101 | 0.300 | 0.150 | 1.654 | 705 | 47385 | −18.61 | −114.35 | 0.133 | 0.152 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, T.; Malherbe, J.N.; Shimpalee, S.; Bricker, J.D. Sub-Nappe Air Cavity Pressure and Cavity Water Depth during Caisson Breakwater Overtopping by a Tsunami. J. Mar. Sci. Eng. 2024, 12, 1135. https://doi.org/10.3390/jmse12071135
Kim T, Malherbe JN, Shimpalee S, Bricker JD. Sub-Nappe Air Cavity Pressure and Cavity Water Depth during Caisson Breakwater Overtopping by a Tsunami. Journal of Marine Science and Engineering. 2024; 12(7):1135. https://doi.org/10.3390/jmse12071135
Chicago/Turabian StyleKim, Taeksang, Julien Noé Malherbe, Sirawit Shimpalee, and Jeremy David Bricker. 2024. "Sub-Nappe Air Cavity Pressure and Cavity Water Depth during Caisson Breakwater Overtopping by a Tsunami" Journal of Marine Science and Engineering 12, no. 7: 1135. https://doi.org/10.3390/jmse12071135
APA StyleKim, T., Malherbe, J. N., Shimpalee, S., & Bricker, J. D. (2024). Sub-Nappe Air Cavity Pressure and Cavity Water Depth during Caisson Breakwater Overtopping by a Tsunami. Journal of Marine Science and Engineering, 12(7), 1135. https://doi.org/10.3390/jmse12071135







