The Effect of Corner Structure on the Optimisation of Fishable Flow Field in Aquaculture Tanks
Abstract
1. Introduction
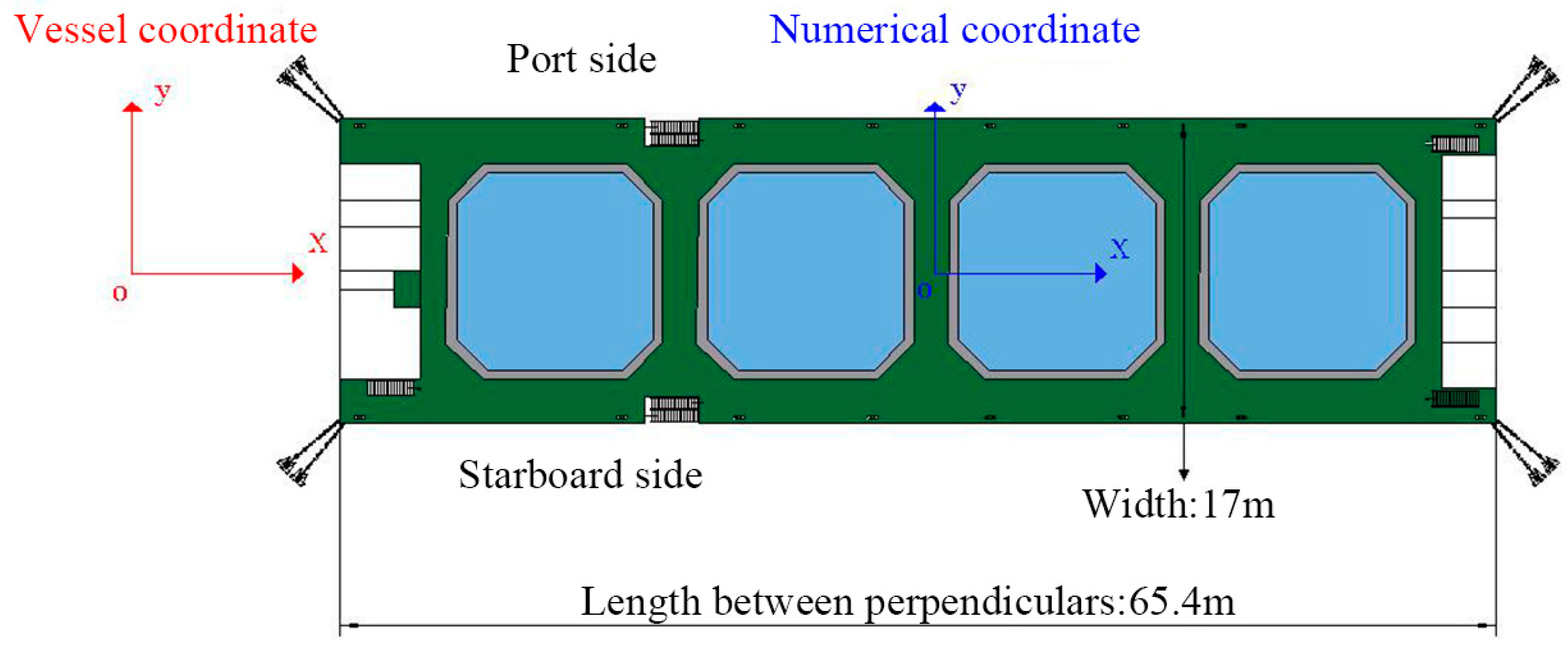
2. Research Object and Model Construction
2.1. Study Objects and Numerical Model Setup
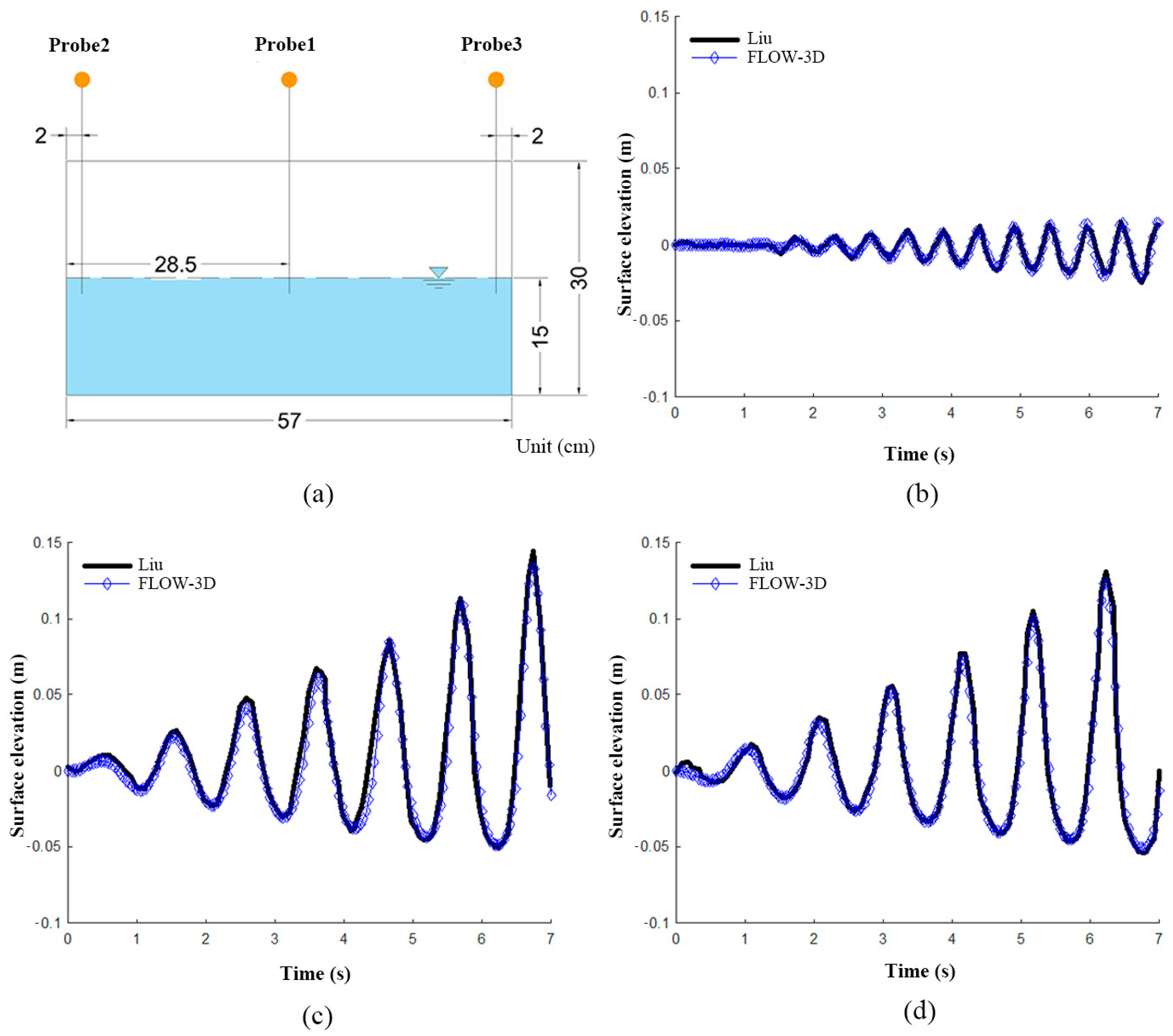
2.2. Model Construction
2.3. Experimental Group
2.4. Data Processing
2.4.1. Drag Coefficient Ct
2.4.2. Energy Use Efficiency
2.4.3. Percentage of Areas with Suitable Flow Rates
3. Results and Discussion
3.1. Effect of Corner Ratio on the Percentage of Areas with Fishable Flow Rates
3.2. Energy Transfer Impact
3.3. Local Vectors at Corners
3.4. Impact of Effluent Efficiency
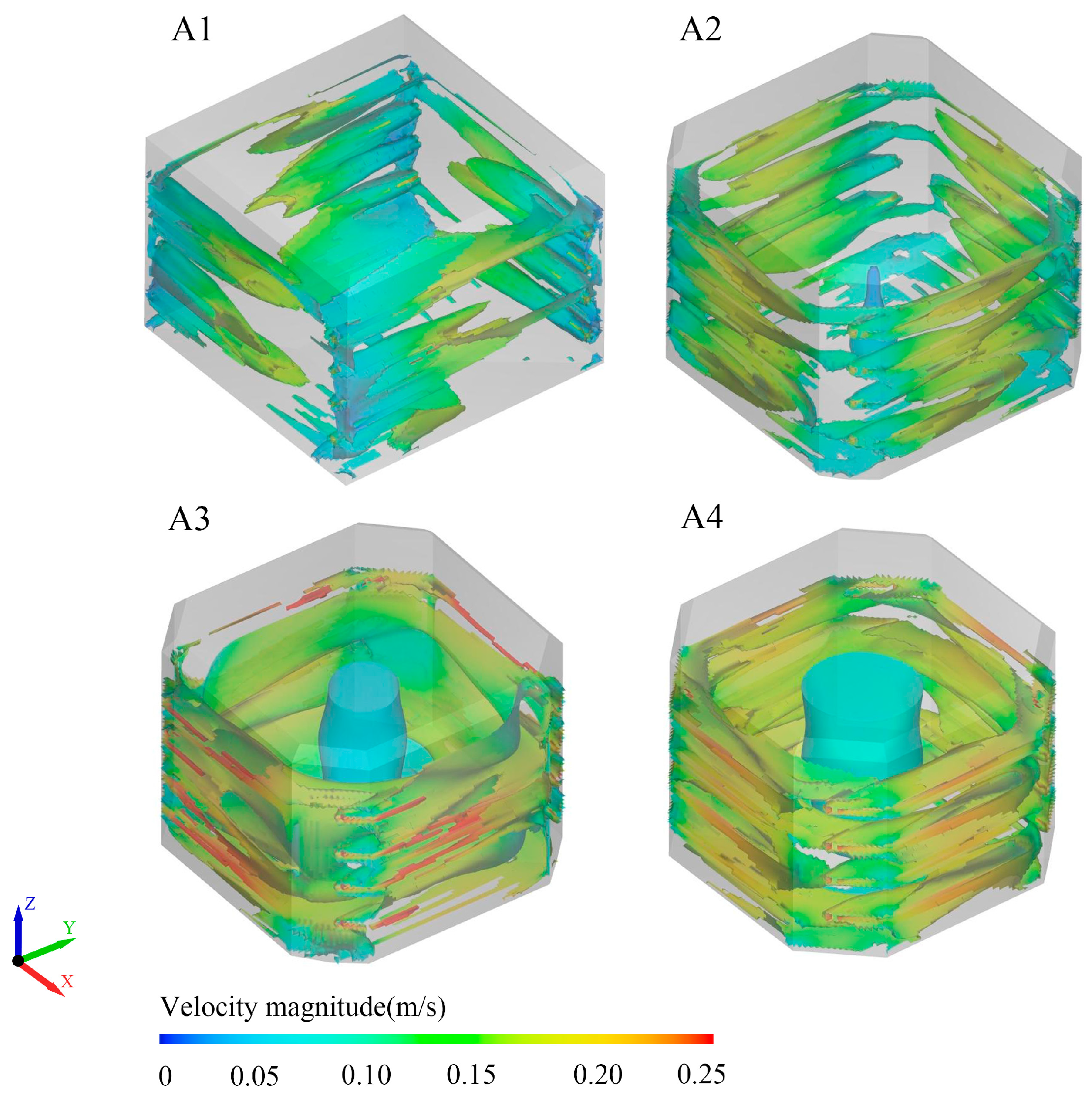
3.5. Vortex Structural Characteristics
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- FAO. The State of World Fisheries and Aquaculture 2024; Blue Transformation in Action; FAO: Rome, Italy, 2024.
- Zhang, Y.; Ni, Q.; Liu, H.; Zhang, C. Status quo of industrialized aquaculture of Atlantic salmon in Norway and its implications for China. Trans. Chin. Soc. Agric. Eng. 2020, 36, 310–315. [Google Scholar]
- Xu, H.; Chen, Z.; Cai, J.; Huang, Y.; Liu, H. Research on the development of deep sea aquaculture engineering equipment in China. Fish. Mod. 2016, 43, 1–6. [Google Scholar]
- Guo, Y.C.; Mohapatra, S.C.; Guedes, S.C. Review of developments in porous membranes and net-type structures for breakwaters and fish cages. Ocean Eng. 2020, 200, 107027. [Google Scholar] [CrossRef]
- Xu, Z.; Qin, H. Fluid-structure interactions of cage based aquaculture: From structures to organisms. Ocean Eng. 2020, 217, 107961. [Google Scholar] [CrossRef]
- Huang, X.; Pang, G.; Yuan, T.; Hu, Y.; Wang, S.; Guo, G.; Tao, Q.Y. Review of engineering and equipment technologies for deep-sea cage aquaculture in China. Prog. Fish. Sci. 2022, 43, 121–131. [Google Scholar]
- Xu, H.; Jiang, T. Development strategy of offshore aquaculture engineering in China. Fish. Mod. 2012, 39, 1–7. [Google Scholar]
- Cai, J.; Zhang, Y.; Li, J. General technology research of 100 thousand ton deep sea aquaculture platform. Ship Eng. 2017, 39, 198–203. [Google Scholar]
- Cui, M.; Jin, J.; Huang, W. Discussion about system construction and general technology of aquaculture platform. Fish. Mod. 2019, 46, 61–66. [Google Scholar]
- Cui, M.; Wang, Q.; Zhang, B. Numerical Analysis on Water Flow Field Characteristics of Aquaculture Vessel under Pitch Motion. Ship Eng. 2020, 10, 56–60. [Google Scholar]
- Tao, Y.; Zhu, R.; Gu, J.; Li, Z.; Zhang, Z.; Xu, X. Experimental and numerical investigation of the hydrodynamic response of an aquaculture vessel. Ocean Eng. 2023, 279, 114505. [Google Scholar] [CrossRef]
- Wang, W.; Li, M.; Fan, G.; Zhang, K.; Huang, Y. Engineering. Analysis of Fluid Field in Fish Tank of Breeding Vessel with Perforated Broadsides under Wave Conditions. J. Mar. Sci. Eng. 2024, 12, 367. [Google Scholar] [CrossRef]
- Wang, Y.; Li, Z.; Guo, X.; Cui, M. Numerical investigation on the flow field in prototype aquaculture tanks. Fish. Mod. 2022, 49, 24–34. [Google Scholar]
- Burrows, R.E.; Chenoweth, H.H. The Rectangular Circulating Rearing Pond. Progress. Fish-Cult. 1970, 32, 67–80. [Google Scholar] [CrossRef]
- Zhang, J.; Wang, M.H.; Jia, G.C. Effect of Structures on Hydrodynamic Characteristics of Recirculating Aquaculture Pond. Trans. Chin. Soc. Agric. Mach. 2022, 53, 311–320. [Google Scholar]
- Summerfelt, S.T.; Davidson, J.W.; Waldrop, T.B.; Tsukuda, S.M.; Bebak-Williams, J. A partial-reuse system for coldwater aquaculture. Aquac. Eng. 2004, 31, 157–181. [Google Scholar] [CrossRef]
- Oca, J.; Masaló, I.; Reig, L. Comparative analysis of flow patterns in aquaculture rectangular tanks with different water inlet characteristics. Aquac. Eng. 2004, 31, 221–236. [Google Scholar] [CrossRef]
- Oca, J.; Masaló, I. Design criteria for rotating flow cells in rectangular aquaculture tanks. Aquac. Eng. 2007, 36, 36–44. [Google Scholar] [CrossRef]
- Lee, J.-V.; Loo, L.; Chuah, Y.; Tang, P.; Tan, Y.; Wong, C. The design of a culture tank in an automated recirculating aquaculture system. Int. J. Eng. Appl. Sci. 2013, 2, 67–77. [Google Scholar]
- Plew, D.R.; Klebert, P.; Rosten, T.W.; Aspaas, S.; Birkevold, J. Changes to flow and turbulence caused by different concentrations of fish in a circular tank. J. Hydraul. Res. 2015, 53, 364–383. [Google Scholar] [CrossRef]
- Liu, Y.; Liu, B.L.; Lei, J.L.; Guan, C.T.; Huang, B. Numerical simulation of the hydrodynamics within octagonal tanks in recirculating aquaculture systems. Chin. J. Oceanol. Limnol. 2017, 35, 912–920. [Google Scholar] [CrossRef]
- Guo, X.; Li, Z.; Cui, M.; Wang, B. Numerical investigation on flow characteristics of water in the fish tank on a force-rolling aquaculture platform. Ocean Eng. 2020, 217, 107936. [Google Scholar] [CrossRef]
- Li, Z.; Guo, X.; Cui, M. Numerical investigation of flow characteristics in a rearing tank aboard an aquaculture vessel. Aquac. Eng. 2022, 98, 102272. [Google Scholar] [CrossRef]
- Cui, M.; Li, Z.; Zhang, C.; Guo, X. Statistical investigation into the flow field of closed aquaculture tanks aboard a platform under periodic oscillation. Ocean Eng. 2022, 248, 110677. [Google Scholar] [CrossRef]
- Xiong, Z.X.; He, M.X.; Zhu, W.Y.; Sun, Y.; Hou, X.R. Analysis of Flow Field Characteristics of Aquaculture Cabin of Aquaculture Ship. J. Mar. Sci. Eng. 2023, 11, 390. [Google Scholar] [CrossRef]
- Xue, B.R.; Zhao, Y.P.; Bi, C.W.; Cheng, Y.; Ren, X.Z.; Liu, Y. Investigation of flow field and pollutant particle distribution in the aquaculture tank for fish farming based on computational fluid dynamics. Comput. Electron. Agric. 2022, 200, 107243. [Google Scholar] [CrossRef]
- Pope, S.B. Turbulent flows. J. Meas. Sci. Technol. 2001, 12, 2020–2021. [Google Scholar] [CrossRef]
- Liu, D.; Lin, P. Three-dimensional liquid sloshing in a tank with baffles. Ocean Eng. 2009, 36, 202–212. [Google Scholar] [CrossRef]
- Hirt, C.; Sicilian, J. A porosity technique for the definition of obstacles in rectangular cell meshes. In Proceedings of the International Conference on Numerical Ship Hydrodynamics, 4th, Washington, DC, USA, 24–27 September 1985. [Google Scholar]
- Xue, B.-r.; Yu, L.-p.; Zhang, Q.; Ren, X.-z.; Bi, C.-w. A numerical study of the effect of relative inflow distance on hydrodynamic characteristics in the single-drain rectangular aquaculture tank with arc angles. J. Fish. China 2021, 45, 444–452. [Google Scholar]
- Masalo, I.; Oca, J. Influence of fish swimming on the flow pattern of circular tanks. Aquac. Eng. 2016, 74, 84–95. [Google Scholar] [CrossRef]
- Gorle, J.M.R.; Terjesen, B.F.; Summerfelt, S.T. Hydrodynamics of Atlantic salmon culture tank: Effect of inlet nozzle angle on the velocity field. Comput. Electron. Agric. 2019, 158, 79–91. [Google Scholar] [CrossRef]
- Wang, J.; Zhang, J.; Zhang, X.; Li, H.-X.; Hu, Y.; Ma, Z. Flow velocity on growth and behavior in black rockfish (Sebastes schlegelii). Acta Hydrobiol. Sin. 2023, 47, 973–981. [Google Scholar]
- Timmerhaus, G.; Lazado, C.C.; Cabillon, N.A.R.; Reiten, B.K.M.; Johansen, L.-H. The optimum velocity for Atlantic salmon post-smolts in RAS is a compromise between muscle growth and fish welfare. Aquaculture 2021, 532, 736076. [Google Scholar] [CrossRef]
- Moghadam, M.M.; Islami, H.R.; Ezam, M.; Mousavi, S.A. Optimizing flow uniformity and velocity fields in Aquaculture Tanks by modifying water inlets and nozzles arrangement: A Computational Fluid Dynamics Study. Aquac. Eng. 2024, 106, 102431. [Google Scholar] [CrossRef]
- Letelier-Gordo, C.O.; Aalto, S.L.; Suurnäkki, S.; Pedersen, P.B. Increased sulfate availability in saline water promotes hydrogen sulfide production in fish organic waste. Aquac. Eng. 2020, 89, 102062. [Google Scholar] [CrossRef]
- Gorle, J.M.R.; Terjesen, B.F.; Summerfelt, S.T. Influence of inlet and outlet placement on the hydrodynamics of culture tanks for Atlantic salmon. Int. J. Mech. Sci. 2020, 188, 105944. [Google Scholar] [CrossRef]
- Lingfei, Z.; Boru, X.; Yunpeng, Z. The influence of The bend ratios on The hydrodynamic characteristics in The tank of aquaculture vessel. Fish. Mod. 2024, 51, 22–31. [Google Scholar]
- Schlatter, P.; Orlu, R. Quantifying the interaction between large and small scales in wall-bounded turbulent flows: A note of caution. Phys. Fluids 2010, 22, 051704. [Google Scholar] [CrossRef]
- Lupandin, A.I. Effect of flow turbulence on swimming speed of fish. Biol. Bull. 2005, 32, 461–466. [Google Scholar] [CrossRef]
- Hockley, F.A.; Wilson, C.; Brew, A.; Cable, J. Fish responses to flow velocity and turbulence in relation to size, sex and parasite load. J. R. Soc. Interface 2014, 11, 20130814. [Google Scholar] [CrossRef]

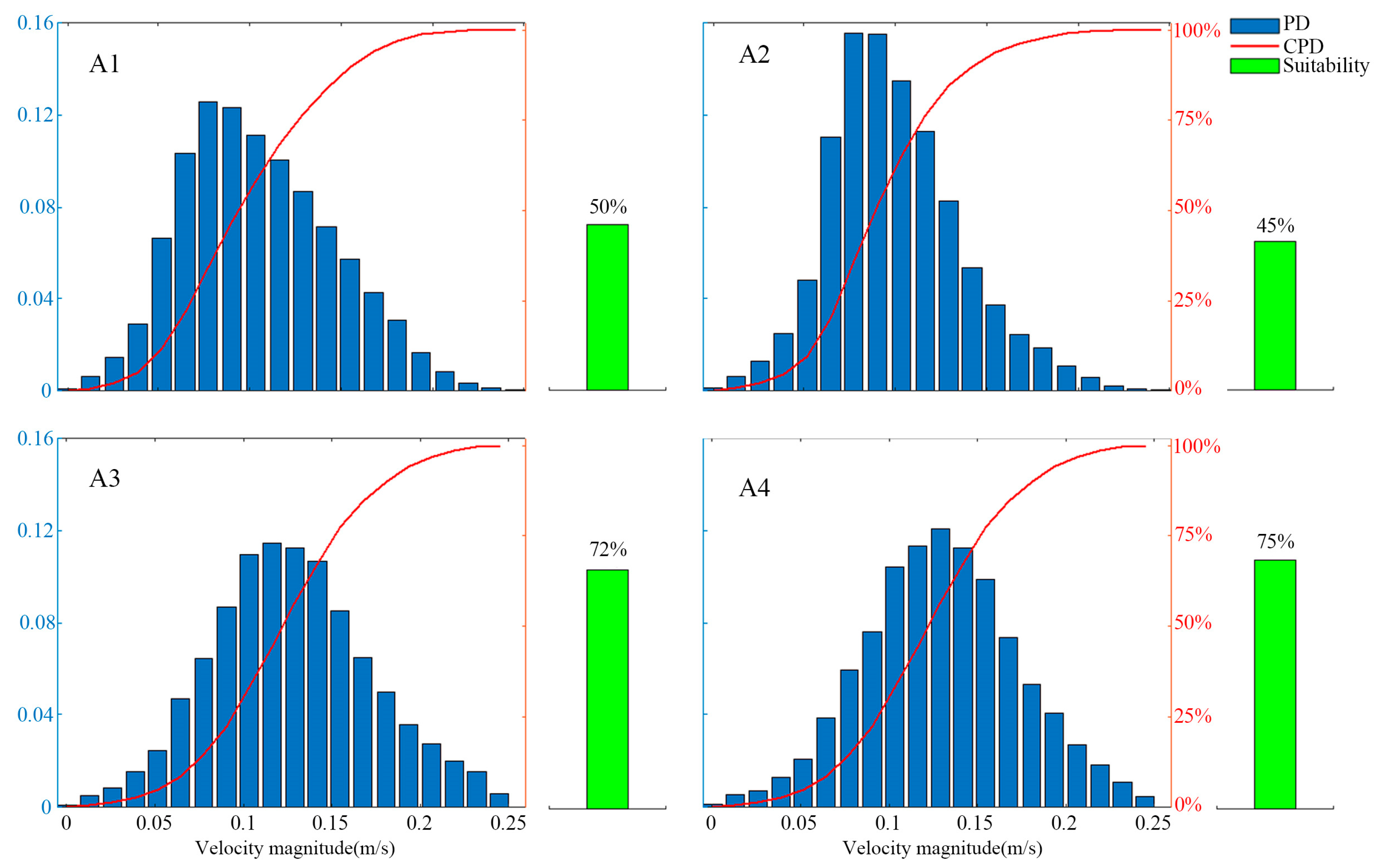
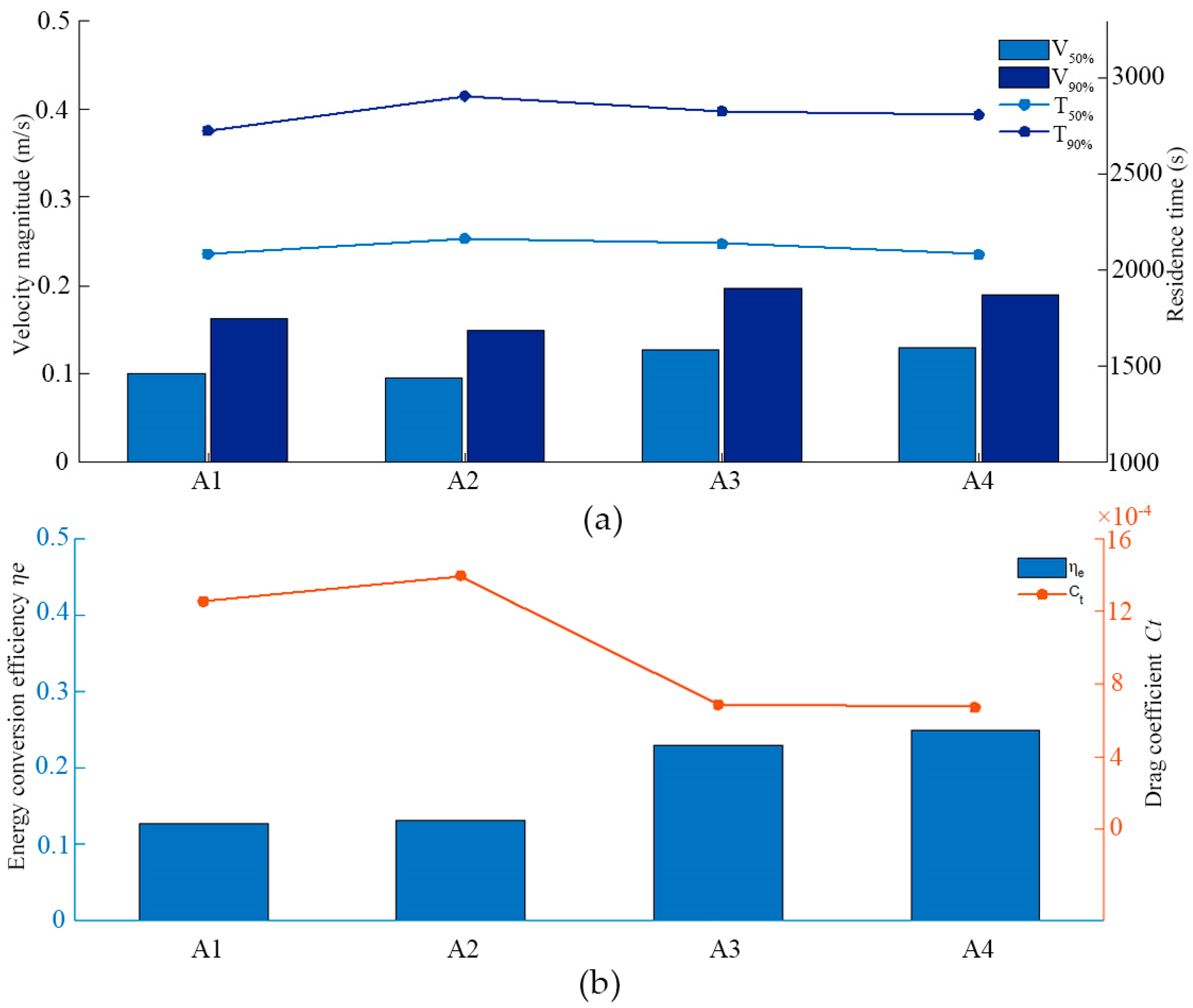

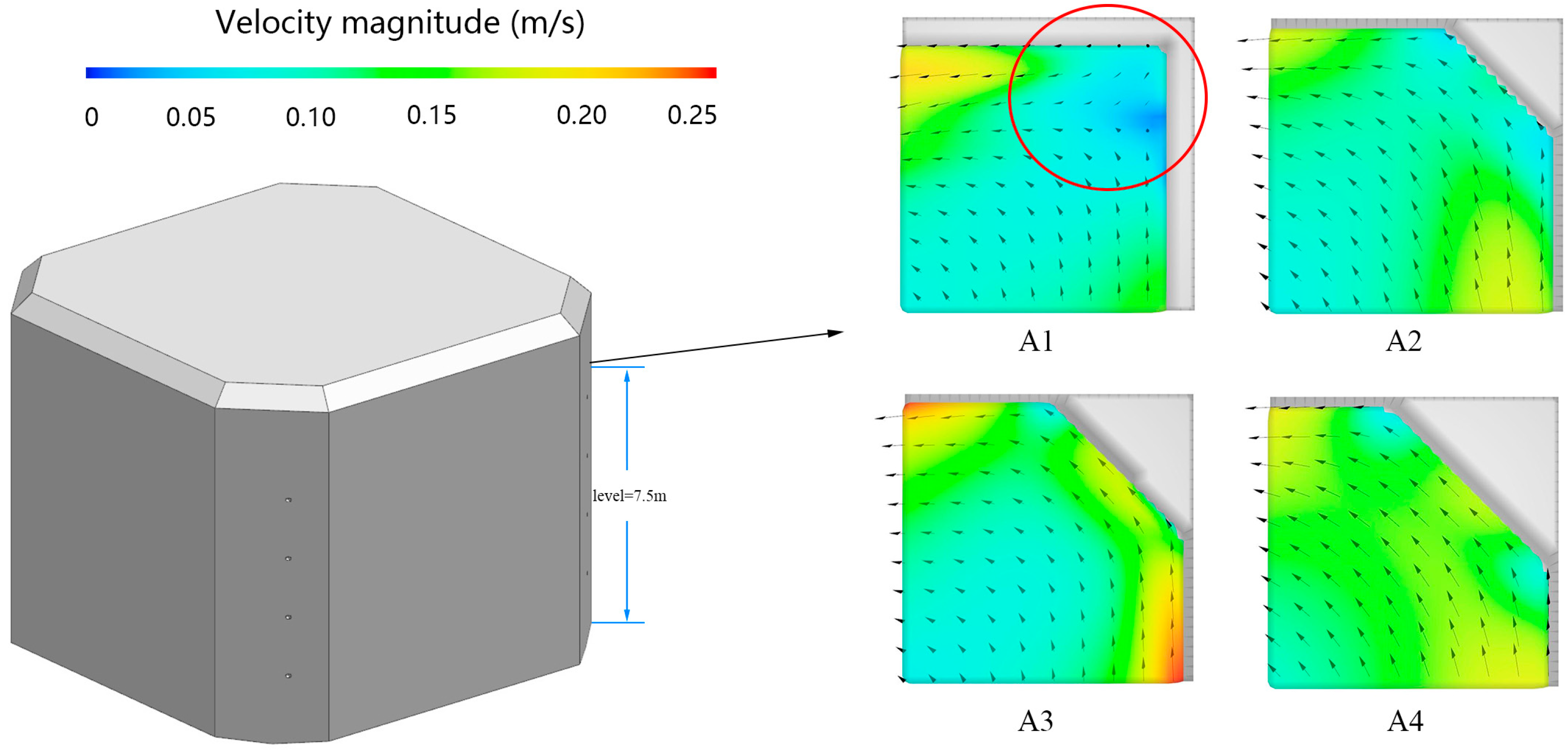

| Corner Ratio | Corner Distance (m) | Water Inflow (m3/s) | Water Outflow (m3/s) | |
|---|---|---|---|---|
| A1 | 0:1 | 0 | 43.43 | 694.81 |
| A2 | 0.26:1 | 1.56 | 42.00 | 672.04 |
| A3 | 0.33:1 | 2.0 | 41.06 | 656.88 |
| A4 | 0.40:1 | 2.4 | 40.02 | 640.34 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, F.; Cui, M.; Liu, H.; Zhang, C. The Effect of Corner Structure on the Optimisation of Fishable Flow Field in Aquaculture Tanks. J. Mar. Sci. Eng. 2024, 12, 1185. https://doi.org/10.3390/jmse12071185
Zhang F, Cui M, Liu H, Zhang C. The Effect of Corner Structure on the Optimisation of Fishable Flow Field in Aquaculture Tanks. Journal of Marine Science and Engineering. 2024; 12(7):1185. https://doi.org/10.3390/jmse12071185
Chicago/Turabian StyleZhang, Fan, Mingchao Cui, Huang Liu, and Chen Zhang. 2024. "The Effect of Corner Structure on the Optimisation of Fishable Flow Field in Aquaculture Tanks" Journal of Marine Science and Engineering 12, no. 7: 1185. https://doi.org/10.3390/jmse12071185
APA StyleZhang, F., Cui, M., Liu, H., & Zhang, C. (2024). The Effect of Corner Structure on the Optimisation of Fishable Flow Field in Aquaculture Tanks. Journal of Marine Science and Engineering, 12(7), 1185. https://doi.org/10.3390/jmse12071185






