Kinematic Stability Analysis of Anchor Cable Structures in Submerged Floating Tunnel under Combined Parametric–Vortex Excitation
Abstract
:1. Introduction
2. Mathematical Modeling and Validation
2.1. Mathematical Modeling
2.2. Numerical Model Validation
3. Effect of Float-to-Weight Ratio and Flow Angle on the Kinematic Response of Anchor Structures
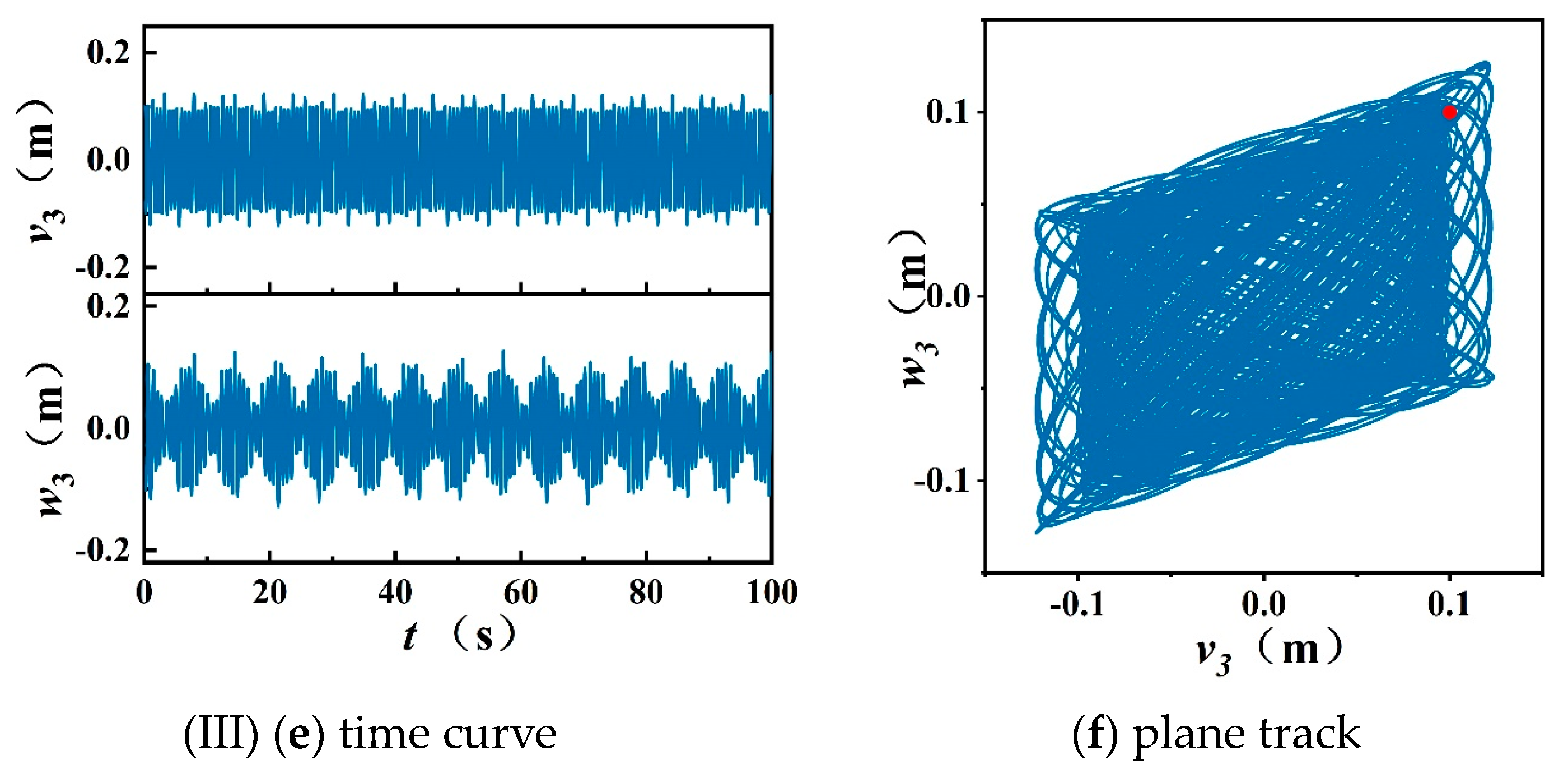
3.1. Effect of Float-to-Weight Ratio on the Kinematic Response of Anchor Cable
3.2. Effect of Flow Angle on the Kinematic Response of an Anchor Cable Structure
4. Instability Analysis of Analytical Model of Three-Way Coupled Motion of Anchor Cable
4.1. Instability Analysis of an Analytical Model of Three-Way Coupled Motion of an Anchor Cable
4.2. Instability Analysis
5. Conclusions
- (1)
- The float-to-weight ratio is a crucial parameter influencing the primary tension of the anchor cable system, whereby a heightened ratio corresponds to an increased tension level. As the initial tension escalates, the displacement response amplitude across various orientations diminishes. Notably, a ratio below 1.4 intensifies the impact on anchor cable displacement, while ratios surpassing 1.4 alleviate such effects;
- (2)
- The alteration in the flow angle has a direct impact on the intensity of the downstream and transverse references. As the flow angle changes and results in a decrease in the strength of the downstream reference, there is a corresponding increase in the strength of the transverse reference. Consequently, the transverse displacement response of the anchor cable structure aligns with the direction of the transverse reference, leading to a proportional increase;
- (3)
- The depiction of the instability range derived from Hill’s equation serves as a tool for forecasting the instability of the anchor cable structure when subjected to parametric excitation. When the relevant parameter falls within the instability zone, the structure is deemed unstable; conversely, it is stable. Parametric resonance is incited when the input frequency of the parametric excitation aligns with approximately one or two times the intrinsic frequency of any order of the anchor structure. This resonance leads to a substantial escalation in the displacement of the corresponding structure modes, resulting in instability. Notably, when the parametric excitation frequency is double the intrinsic frequency, the resonance span broadens significantly.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xiang, Y.; Yang, Y. Challenge in design and construction of submerged floating tunnel and state-of-art. Procedia Eng. 2016, 166, 53–60. [Google Scholar] [CrossRef]
- Deng, S.; Ren, H.; Xu, Y.; Fu, S.; Moan, T.; Gao, Z. Experimental study of vortex-induced vibration of a twin-tube submerged floating tunnel segment model. J. Fluids Struct. 2020, 94, 102908. [Google Scholar] [CrossRef]
- Ding, H.; Cheng, L.; Li, K. Progress and prospect of research on structural dynamic response of submerged floating tunnels. Tunn. Constr. 2019, 39, 901–912. (In Chinese) [Google Scholar]
- Huang, L.-N.; Li, X.; Wu, S. Research progress on key issues of submerged floating tunnel. China Harb. Constr. 2017, 37, 7–10, 70. [Google Scholar]
- Seo, S.-I.; Mun, H.-S.; Lee, J.-H.; Kim, J.-H. Simplified analysis for estimation of the behavior of a submerged floating tunnel in waves and experimental verification. Mar. Struct. 2015, 44, 142–158. [Google Scholar] [CrossRef]
- Chen, X.; Chen, Q.; Chen, Z.; Cai, S.; Zhuo, X.; Lv, J. Numerical modeling of the interaction between submerged floating tunnel and surface waves. Ocean Eng. 2021, 220, 108494. [Google Scholar] [CrossRef]
- Luo, W.; Huang, B.; Tang, Y.; Ding, H.; Li, K.; Cheng, L.; Ren, Q. Numerical Simulation of Dynamic Response of Submerged Floating Tunnel under Regular Wave Conditions. Shock Vib. 2022, 2022, 4940091. [Google Scholar] [CrossRef]
- Wang, F.; Li, K.; Huang, B.; Cheng, L.; Ding, H. Experimental Investigation of the Dynamic Behavior of Submerged Floating Tunnels under Regular Wave Conditions. J. Mar. Sci. Eng. 2022, 10, 1623. [Google Scholar] [CrossRef]
- Li, Q.; Jiang, S.; Chen, X. Experiment on Pressure Characteristics of Submerged Floating Tunnel with Different Section Types under Wave Condition. Pol. Marit. Res. 2018, 25, 54–60. [Google Scholar] [CrossRef]
- Paik, I.Y.; Oh, C.K.; Kwon, J.S.; Chang, S.P. Analysis of wave force induced dynamic response of submerged floating tunnel. KSCE J. Civ. Eng. 2004, 8, 543–550. [Google Scholar] [CrossRef]
- Zou, P.X.; Bricker, J.D.; Uijttewaal, W.S.J. Impacts of extreme events on hydrodynamic characteristics of a submerged floating tunnel. Ocean Eng. 2020, 218, 108221. [Google Scholar] [CrossRef]
- Yuan, X.; Liu, J.; Zhou, J.; Guo, A. Effect of cable layout on hydrodynamic response of submerged floating tunnel under wave action. Ocean Eng. 2023, 280, 114257. [Google Scholar] [CrossRef]
- Cifuentes, C.; Seungjun, K.; Kim, M.H.; Park, W.S. Numerical simulation of the coupled dynamic response of a submerged floating tunnel with mooring lines in regular waves. Ocean Syst. Eng. 2015, 5, 109–123. [Google Scholar] [CrossRef]
- Brancaleoni, F.; Castellani, A.; D’Asdia, P. The response of submerged tunnels to their environment. Eng. Struct. 1989, 11, 47–56. [Google Scholar] [CrossRef]
- Zou, P.X.; Ruiter, N.; Bricker, J.D.; Uijttewaal, W.S.J. Effects of roughness on hydrodynamic characteristics of a submerged floating tunnel subject to steady currents. Mar. Struct. 2023, 89, 103405. [Google Scholar] [CrossRef]
- Xu, W.; Ma, Y.; Ji, C.; Sun, C. Laboratory measurements of vortex_induced vibrations of a yawed flexible cylinder at different yaw angles. Ocean Eng. 2018, 154, 27–42. [Google Scholar] [CrossRef]
- Xu, G.; Chen, X.; Xue, S.; Townsend, J.F.; Chen, X.; Tang, M. Numerical assessment of non-uniform terrain and inhomogeneous wave–current loading effects on the dynamic response of a submerged floating tunnel. Ocean Eng. 2023, 288, 115942. [Google Scholar] [CrossRef]
- Xu, L.; Fei, G.; Youshi, H. Feasibility study on buoyancy-weight ratios of a submergedd floating tunnel prototype subjected to hydrodynamic loads. J. Mech. (Engl. Ed.) 2015, 5, 750–761. [Google Scholar]
- Wang, G.; Zhang, N.; Huang, G. Coupled effect of multi-factor on the vibration of submergedd floating tunnel under impact load. Ocean Eng. 2022, 262, 112181. [Google Scholar] [CrossRef]
- Su, Z.B.; Sun, S.N. Seismic Response of Submergedd Floating Tunnel Tether. China Ocean Eng. (Engl. Ed.) 2013, 27, 43–50. [Google Scholar] [CrossRef]
- Han, S.M.; Benaroya, H. Nonlinear coupled transverse and axial vibration of a compliant structure 2: Orced vibration. J. Sound Vib. 2000, 237, 874–899. [Google Scholar]
- Kims, H.; Yamato, H. An Experimental Study of the Longitudinal Motion Control of a Fully Submerged Hydrofoil Model in Following Seas. Ocean Eng. 2004, 31, 523–537. [Google Scholar]
- Bai, J.; Kim, Y. Control of the Vertical Motion of a Hydrofoil Vessel. Ships Offshore Struct. 2010, 5, 189–198. [Google Scholar] [CrossRef]
- Mai, J.; Luo, Z.; Guan, B. Vortex agitation response of tension legs in submerged floating tunnel under flow. J. Southwest Jiaotong Univ. 2004, 39, 600–604. [Google Scholar]
- Wang, G. Numerical Analysis and Experimental Study on the Structural Response of Submerged Floating Tunnel under Wave Current. Ph.D. Thesis, Southwest Jiaotong University, Chengdu, China, 2008. [Google Scholar]
- Liu, Y.; Chen, L. Nonlinear Vibration. Master’s Thesis, Higher Education Press, Beijing, China, 2001. [Google Scholar]
- Tang, Y.-G. Advanced Structural Dynamics. Master’s Thesis, Tianjin University Press, Tianjin, China, 2002. (In Chinese). [Google Scholar]
- Yang, S.; Yan, C.; Wu, Z. Dynamic response analysis of anchor cables in submerged floating tunnel under random seismic effects. J. Disaster Prev. Mitig. Eng. 2021, 41, 304–310. (In Chinese) [Google Scholar]

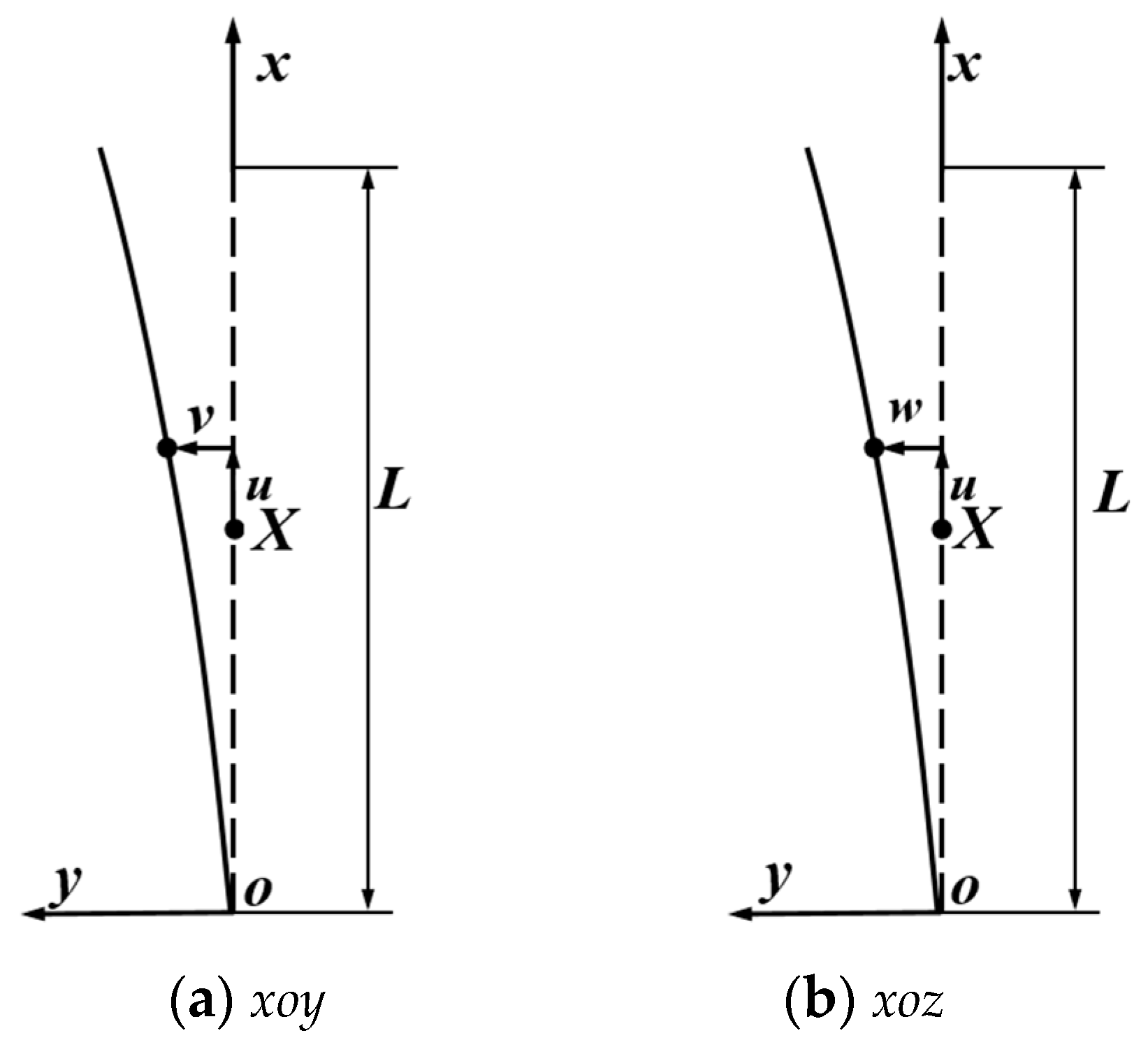




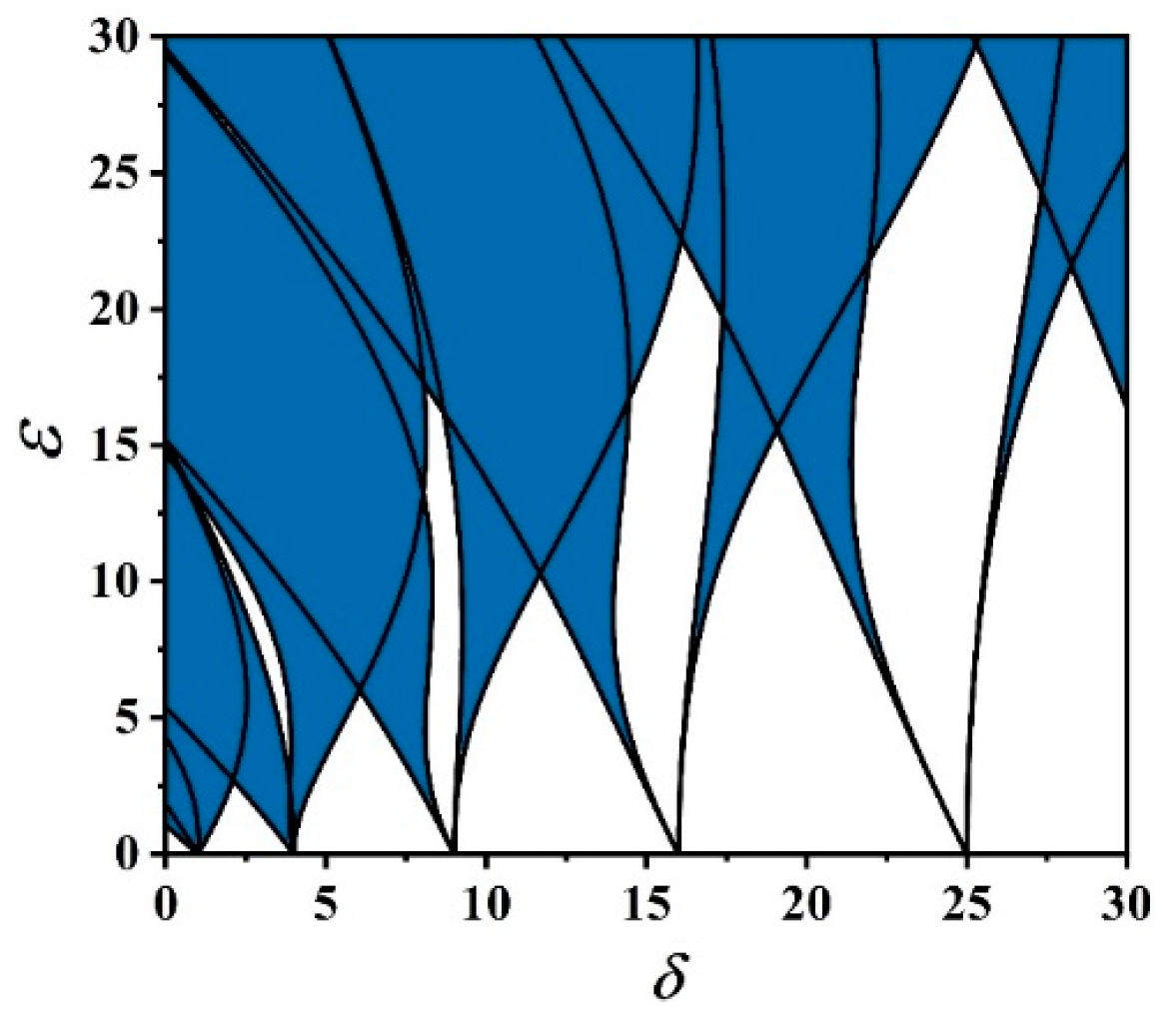
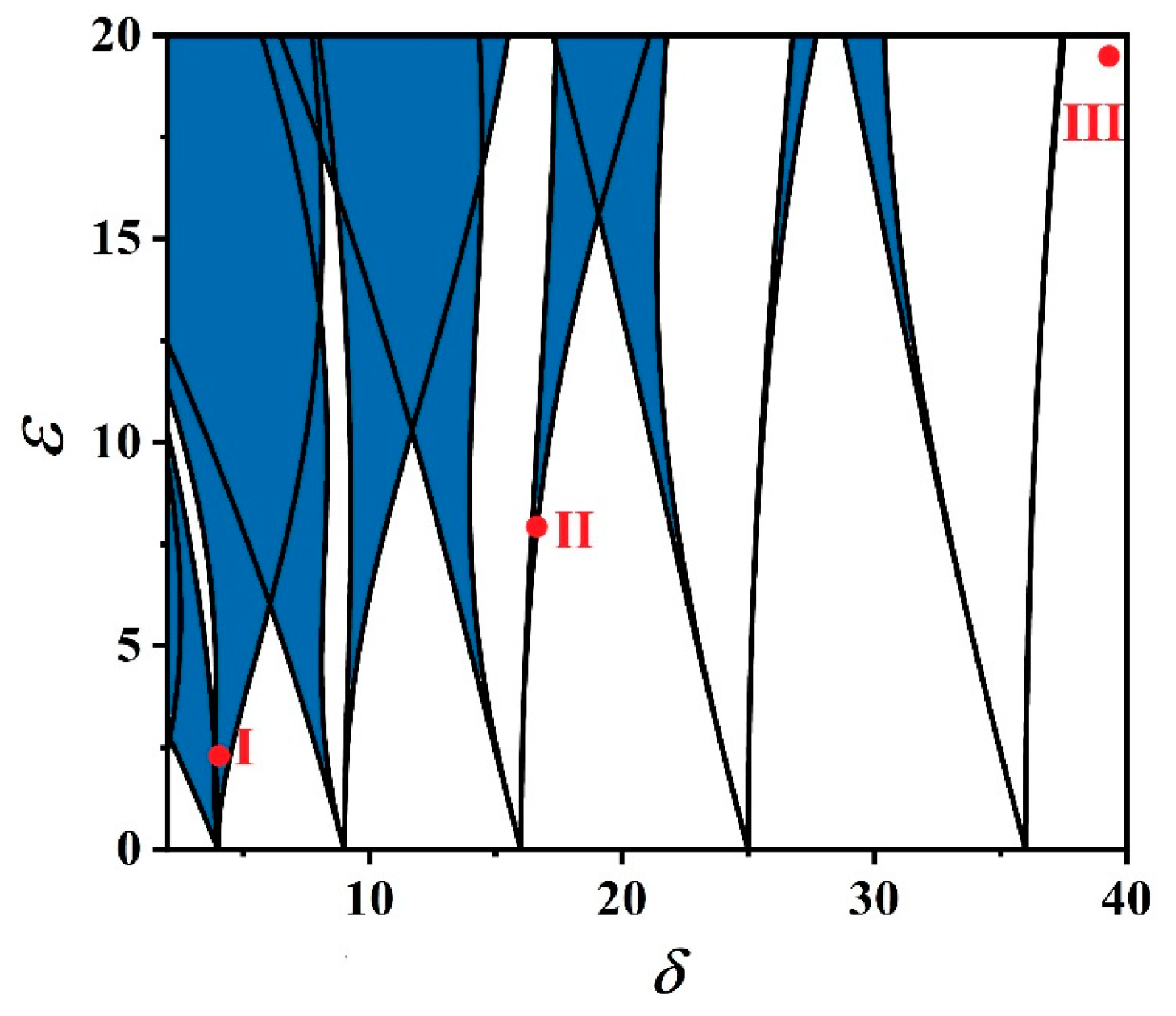
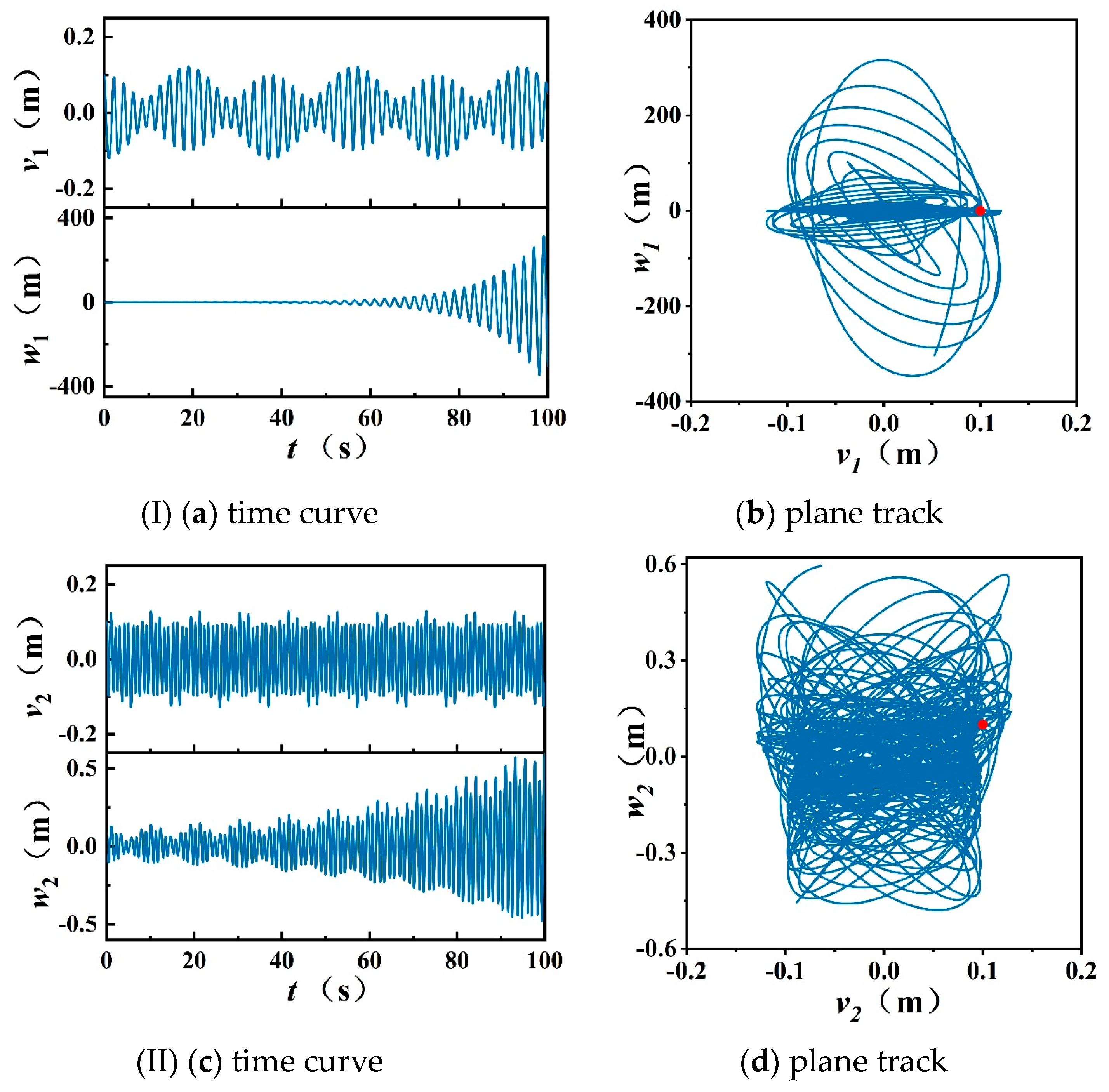
| BWR | Initial Tension T (×107 N) | BWR | Initial Tension T (×107 N) |
|---|---|---|---|
| 1.1 | 0.86 | 1.6 | 3.55 |
| 1.2 | 1.58 | 1.7 | 3.89 |
| 1.3 | 2.18 | 1.8 | 4.21 |
| 1.4 | 2.70 | 1.9 | 4.48 |
| 1.5 | 3.16 | 2.0 | 4.73 |
| Working Condition | 15° | 45° | 90° | 120° | 180° | |
|---|---|---|---|---|---|---|
| = 1 | 1.80 | 3.41 | 4.32 | 3.94 | 1.33 | |
| = 1 | 5.33 | 4.34 | 1.83 | 3.68 | 5.47 | |
| = 1 | 0.70 | 1.65 | 2.34 | 1.99 | 0.30 | |
| = 2 | 2.59 | 1.84 | 0.48 | 1.34 | 2.52 | |
| = 2 | 2.48 | 4.17 | 5.11 | 4.87 | 2.23 | |
| = 1 | 7.03 | 5.88 | 3.21 | 5.70 | 7.15 | |
| = 2 | 0.46 | 1.31 | 1.91 | 1.60 | 0.04 | |
| = 2 | 1.58 | 1.10 | 0.30 | 0.76 | 1.55 | |
| Parameter | Symbol/Unit | Numerical Value |
|---|---|---|
| Anchor length | L/m | 140 |
| Diameter | D/m | 0.5 |
| Density | ρ/(kg·m−3) | 7850 |
| Seawater density | ρwater/(kg·m−3) | 1025 |
| Modulus of elasticity | E/Pa | 2.1 × 1011 |
| Gravity acceleration | g/(N·kg−1) | 9.8 |
| Working Condition | (rad/s) | (, ) | ||
|---|---|---|---|---|
| I | II | III | ||
| F1 | 1.4 | (16.11, 8.68) | (66.74, 34.73) | (158.78, 78.15) |
| F2 | 2.8 | (4.03, 2.17) | (16.68, 8.68) | (39.69, 19.53) |
| F3 | 4.4 | (1.63, 0.87) | (6.75, 3.51) | (16.07, 7.91) |
| F4 | 5.4 | (1.08, 0.58) | (4.49, 2.33) | (10.67, 5.25) |
| F5 | 5.9 | (0.90, 0.48) | (3.75, 1.95) | (8.94, 4.40) |
| F6 | 6.6 | (0.73, 0.39) | (3.01, 1.96) | (7.15, 3.52) |
| F7 | 8.4 | (0.45, 0.24) | (1.85, 0.96) | (4.41, 2.17) |
| F8 | 10.2 | (0.30, 0.16) | (1.26, 0.65) | (2.99, 1.47) |
| F9 | 13.8 | (0.17, 0.09) | (0.69, 0.36) | (1.63, 0.80) |
| F10 | 15.6 | (0.13, 0.07) | (0.54, 0.28) | (1.28, 0.63) |
| F11 | 16.2 | (0.12, 0.06) | (0.50, 0.26) | (1.19, 0.58) |
| F12 | 21.3 | (0.07, 0.04) | (0.29, 0.15) | (0.69, 0.34) |
| Working Condition | I | II | III | |||
|---|---|---|---|---|---|---|
| Stability | Convergence | Stability | Convergence | Stability | Convergence | |
| F1 | ○ | ✔ | ○ | ✔ | ○ | ✔ |
| F2 | ● | ✘ | ● | ✘ | ○ | ✔ |
| F3 | ○ | ✔ | ○ | ✔ | ○ | ✔ |
| F4 | ● | ✘ | ○ | ✔ | ○ | ✔ |
| F5 | ● | ✘ | ● | ✘ | ○ | ✔ |
| F6 | ● | ✘ | ● | ✘ | ○ | ✔ |
| F7 | ○ | ✔ | ○ | ✔ | ● | ✘ |
| F8 | ○ | ✔ | ● | ✘ | ○ | ✔ |
| F9 | ○ | ✔ | ● | ✘ | ○ | ✔ |
| F10 | ○ | ✔ | ○ | ✔ | ● | ✘ |
| F11 | ○ | ✔ | ○ | ✔ | ● | ✘ |
| F12 | ○ | ✔ | ○ | ✔ | ● | ✘ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, J.; Sang, S.; Du, Y.; Gan, C.; Zhang, A.; Liu, F. Kinematic Stability Analysis of Anchor Cable Structures in Submerged Floating Tunnel under Combined Parametric–Vortex Excitation. J. Mar. Sci. Eng. 2024, 12, 1478. https://doi.org/10.3390/jmse12091478
Xiong J, Sang S, Du Y, Gan C, Zhang A, Liu F. Kinematic Stability Analysis of Anchor Cable Structures in Submerged Floating Tunnel under Combined Parametric–Vortex Excitation. Journal of Marine Science and Engineering. 2024; 12(9):1478. https://doi.org/10.3390/jmse12091478
Chicago/Turabian StyleXiong, Jiaming, Song Sang, Youwei Du, Chaojie Gan, Ao Zhang, and Fugang Liu. 2024. "Kinematic Stability Analysis of Anchor Cable Structures in Submerged Floating Tunnel under Combined Parametric–Vortex Excitation" Journal of Marine Science and Engineering 12, no. 9: 1478. https://doi.org/10.3390/jmse12091478





