Study on the Correlation between Soil Consolidation and Pile Set-Up Considering Pile Installation Effect
Abstract
:1. Introduction
2. Numerical Model
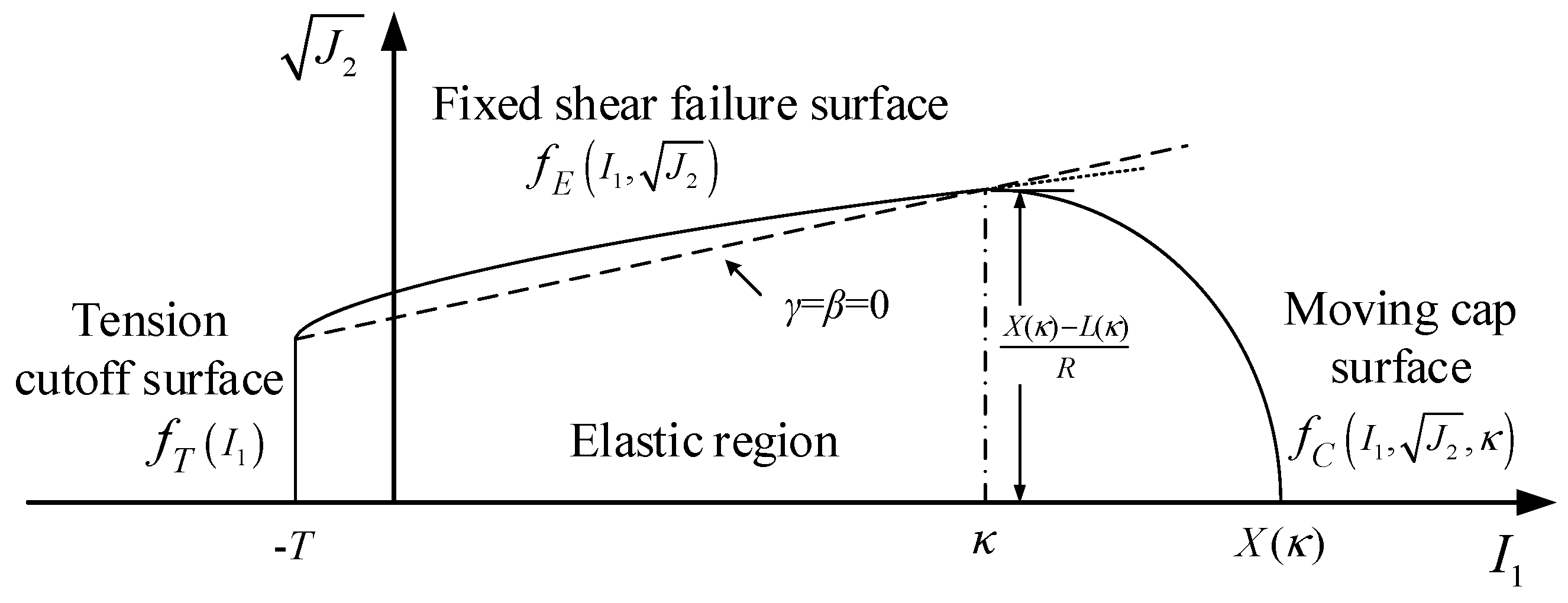
2.1. Soil Model Description
- J2 is the second invariant of the deviatoric stress tensor,
- I1 is the first stress invariant,
- α, γ, β and θ are the material parameters of the failure surface [38], which can be defined by and ,
- c, φ are the frictional and cohesive strengths of the material,
- R is the curvature of the hardening cap, which can be defined by ,
- v is the Poisson’s ratio,
- X(κ) is the intersection of the cap surface with the I1 axis,
- L(κ) is the value of I1 at the location of the start of the cap,
- κ is the I1 coordinate of the intersection of the cap surface and the failure surface,
- T is the maximum allowable hydrostatic tension.
- W is related to the porosity n and the degree of saturation Sr, [38], which represents the void fraction of the uncompressed sample,
- D governs the slope of the initial loading curve in hydrostatic compression, and
- X0 is thought of as the preconsolidation hydrostatic pressure, which is the product of OCR and the overburden pressure σz (i.e., X0 = OCR·σz) [38].
- Dijkl is elastic stiffness matrix,
- K is the bulk modulus of soil,
- G is the shear modulus of soil, and
- δij is the Kronecker operator, δij = 1 when i = j; δij = 0 when i ≠ j.
2.2. Pore Water Pressure Effect Description
- B, C, H, Q, and S are the stiffness, damping, permeability coefficient, coupling, and flow compressibility matrices respectively, and the superimposed dot represents the time derivative of variables, and
- FP and FU are the vectors of fluid supply and external nodal forces, respectively.
- ks is the permeability coefficient of soil,
- pw is the pressure head,
- vf is the fluid velocity vector, and
- zco is z-coordinate.
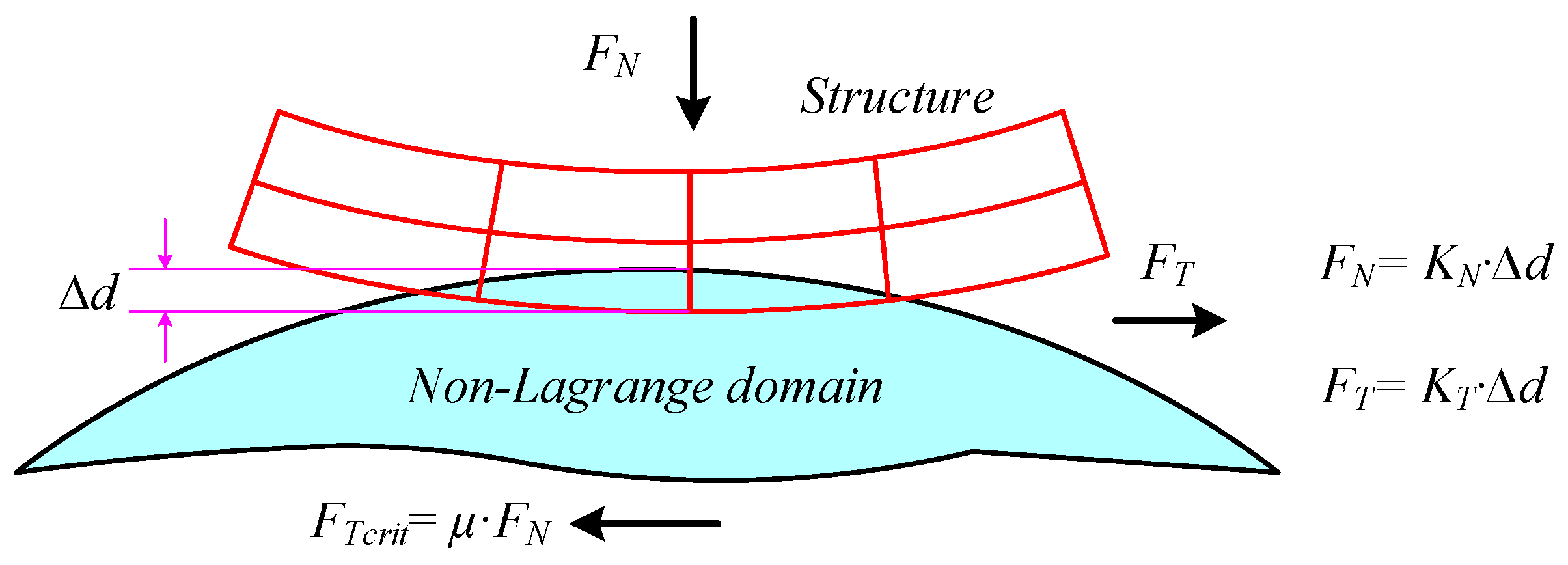
2.3. Interface Modeling in Pile Jacking Simulation
- Eu, E′ are the elastic moduli in undrained and drained conditions, respectively,
- Gu, G′ are the shear moduli in undrained and drained conditions, respectively,
- v′, vu are the Poisson’s ratios in drained and undrained conditions, respectively, and vu is assumed as 0.49 to avoid numerical troubles.
- μ is the friction coefficient of the pile–soil interface,
- ∆d is the penetration displacement of the structure into the non-Lagrange domain,
- FT is the tangential force,
- FN is the normal coupling nodal force,
- FTcrit is the maximum tangential force.
2.4. Establishment and Parameters of Modeling Pile Jacking
2.5. Comparison with Field Data
3. Numerical Simulation Results
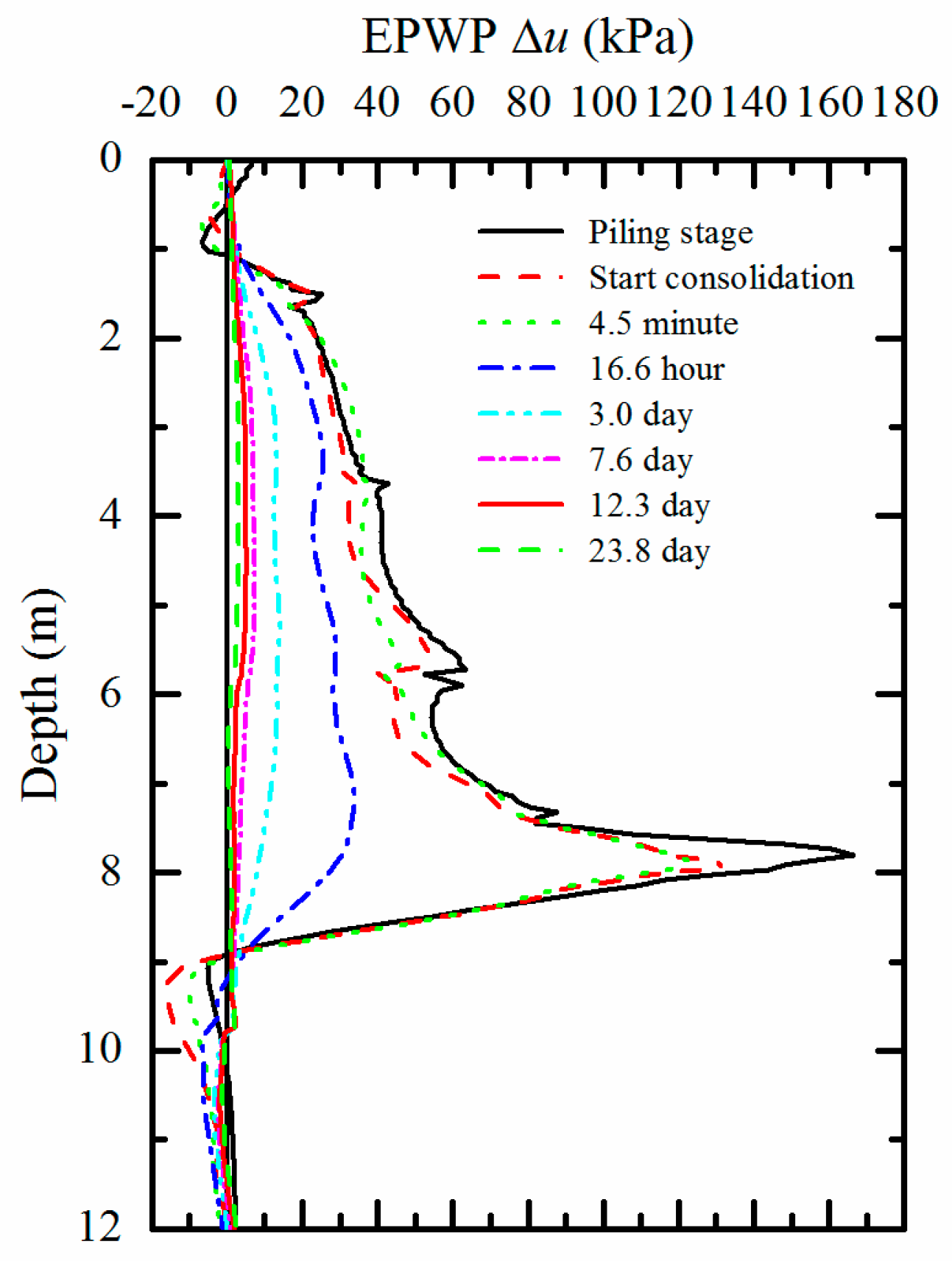
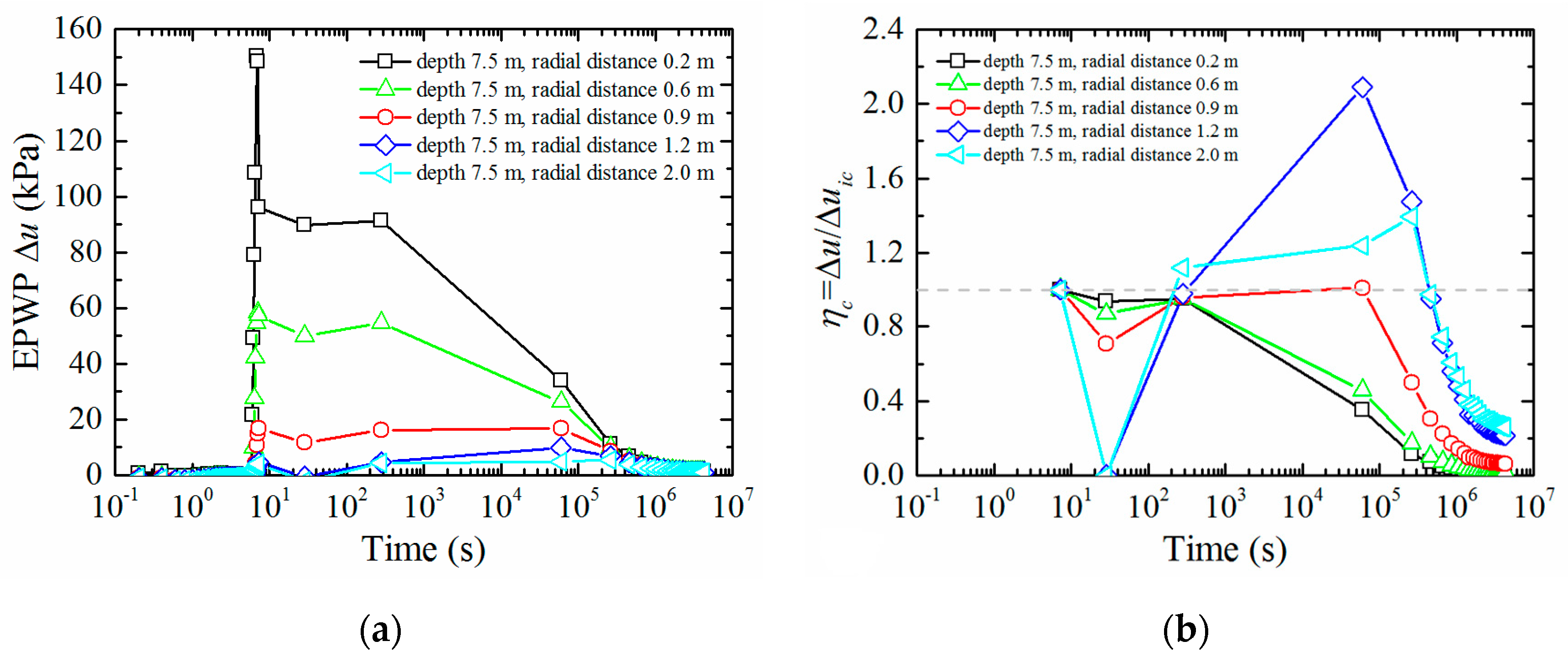
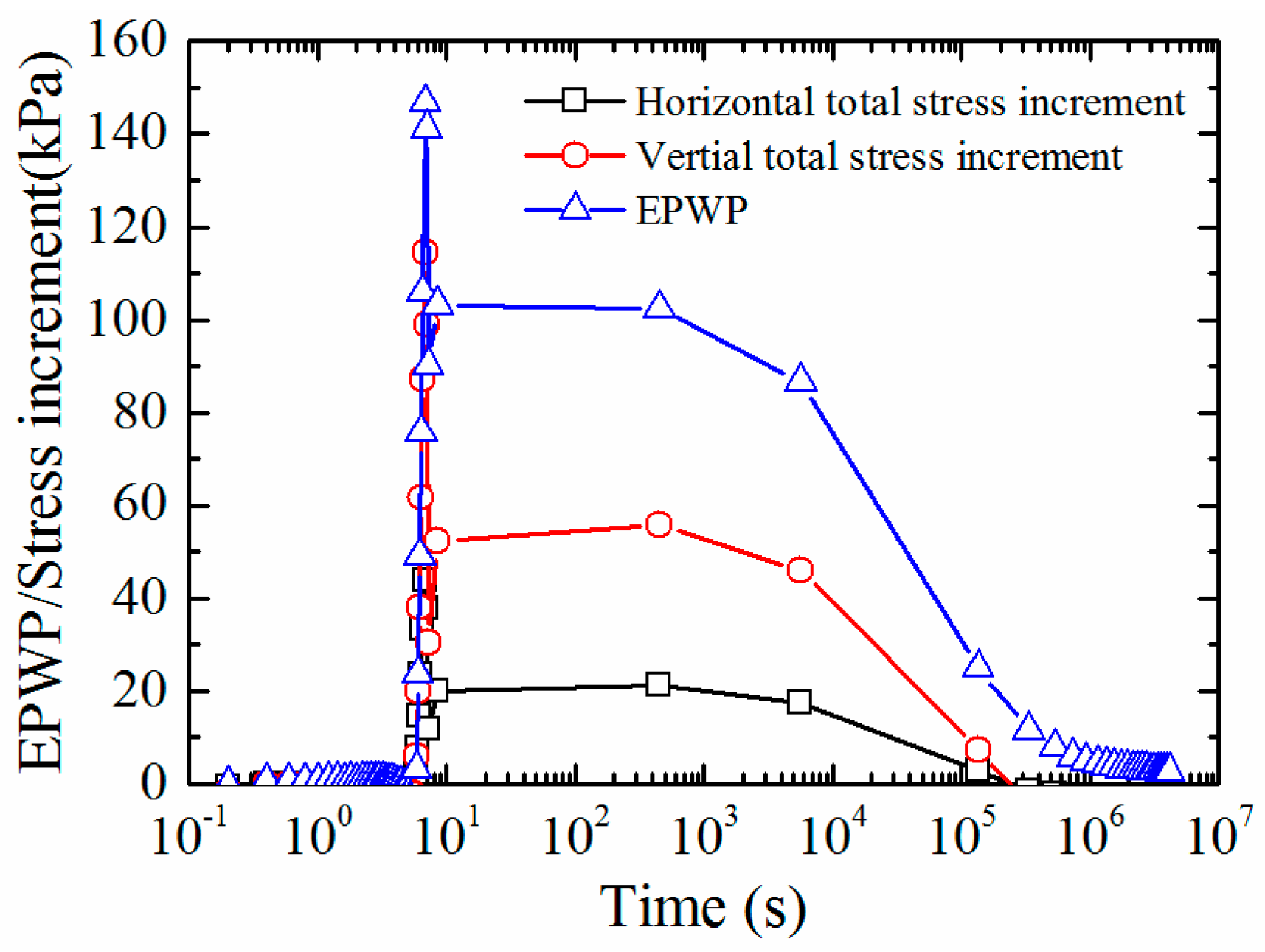
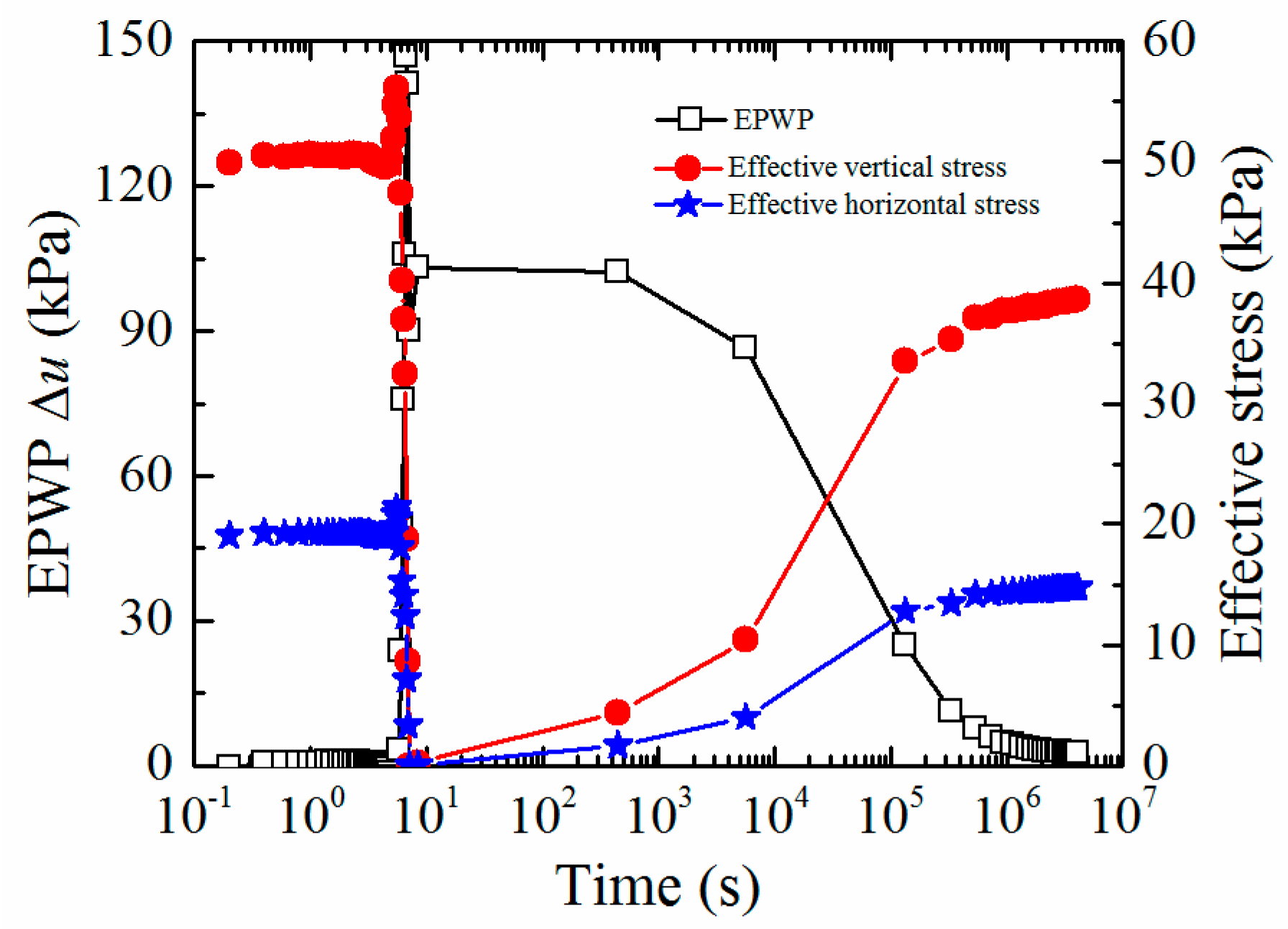
3.1. Mechanism Analysis of Consolidation Effect of Soil around Pile
3.2. Parametric Studies of Consolidation Effect
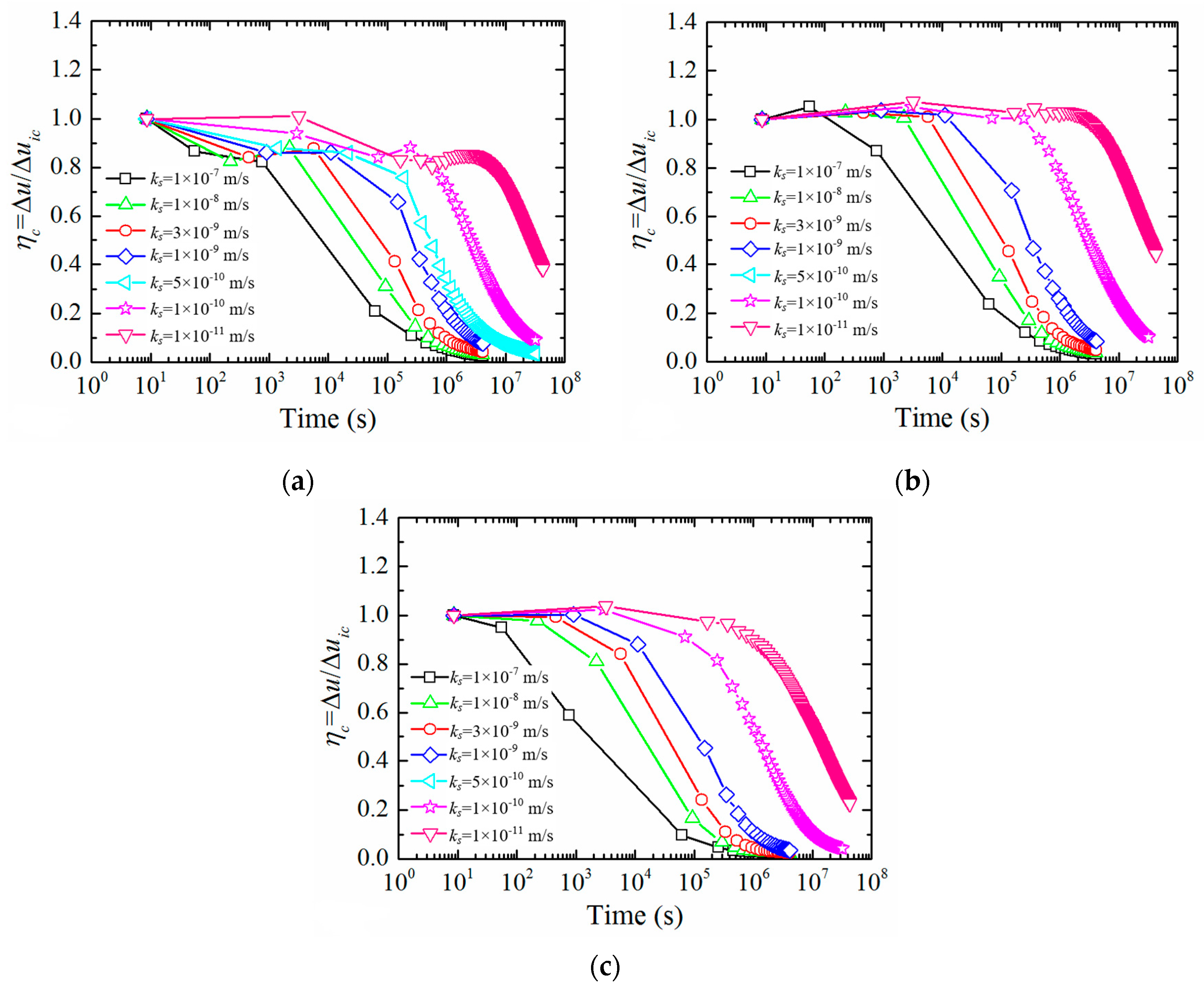
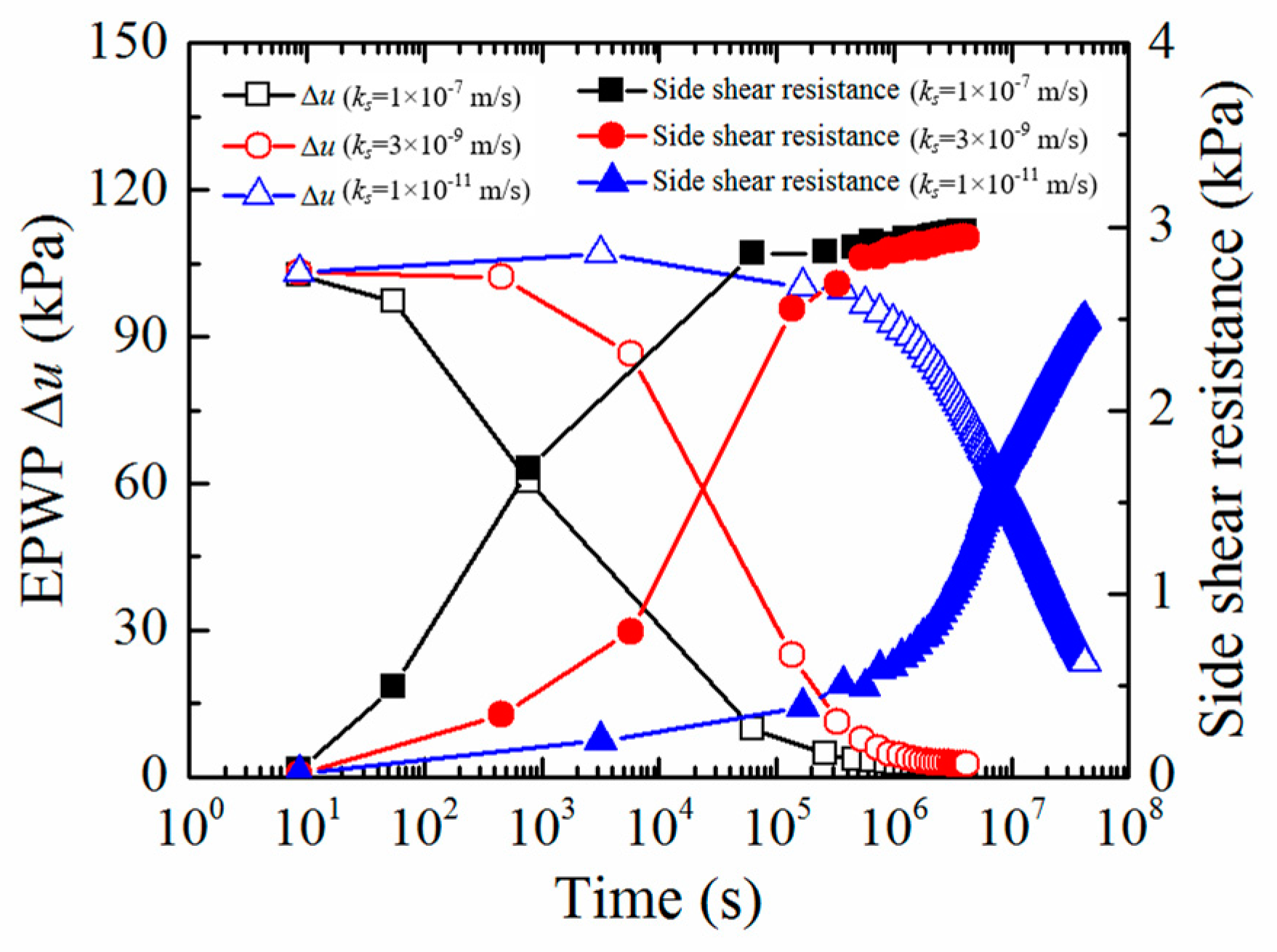
3.2.1. Effect of Soil Permeability Coefficient ks
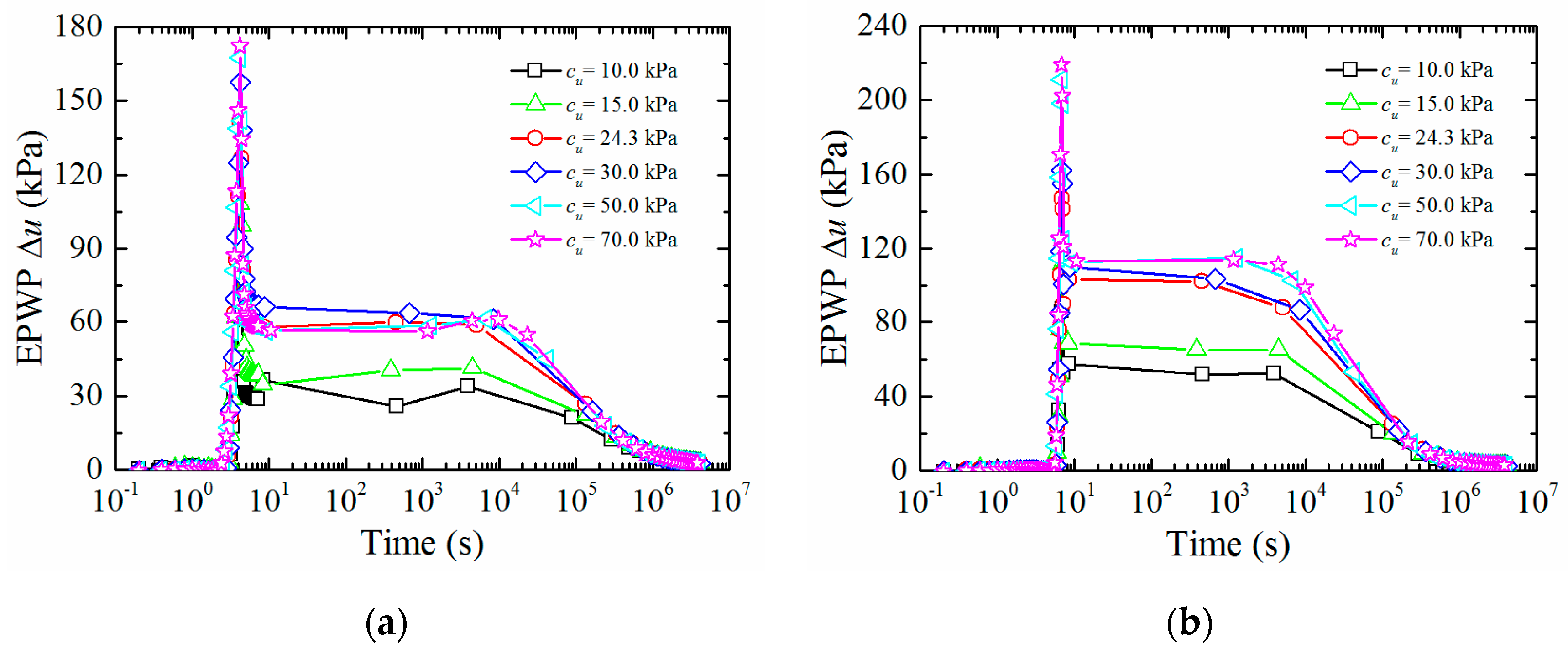
3.2.2. Effect of Soil Undrained Shear Strength cu
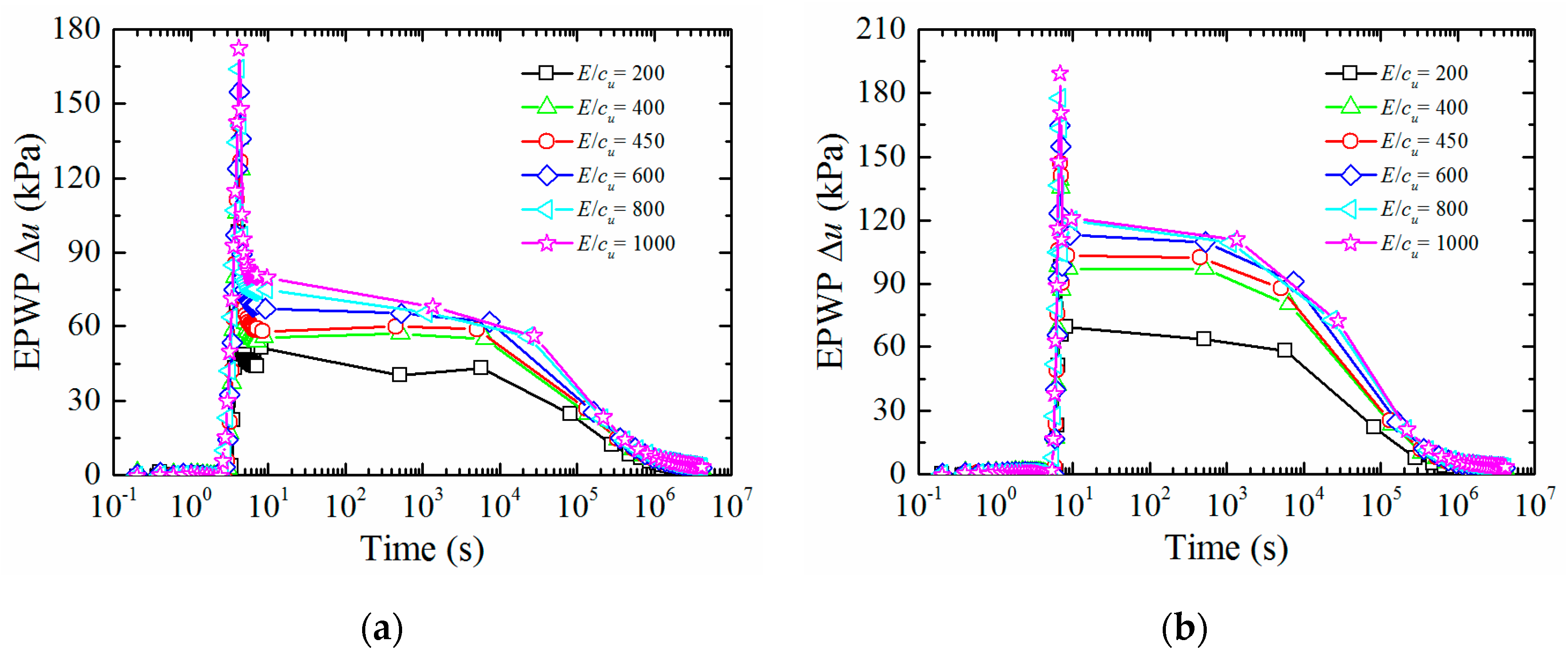
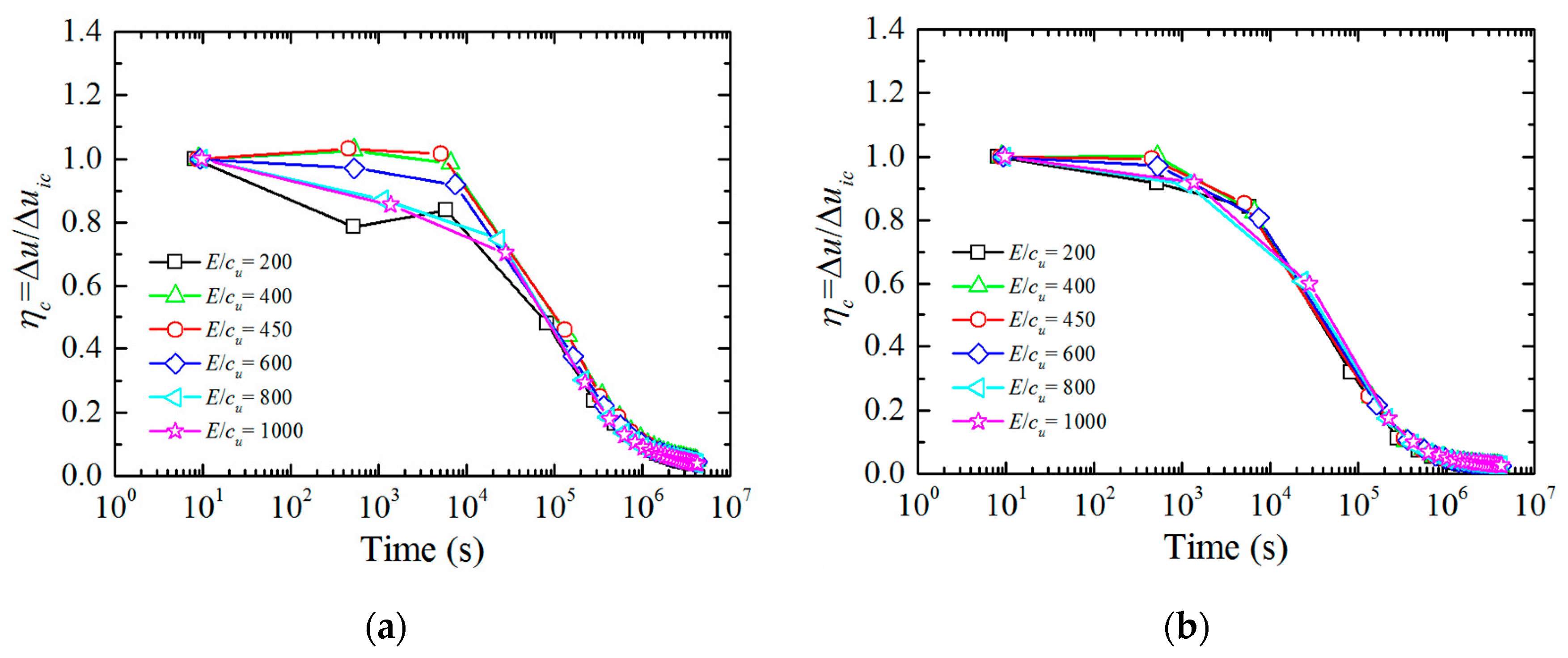
3.2.3. Effect of Soil Elastic Modulus E
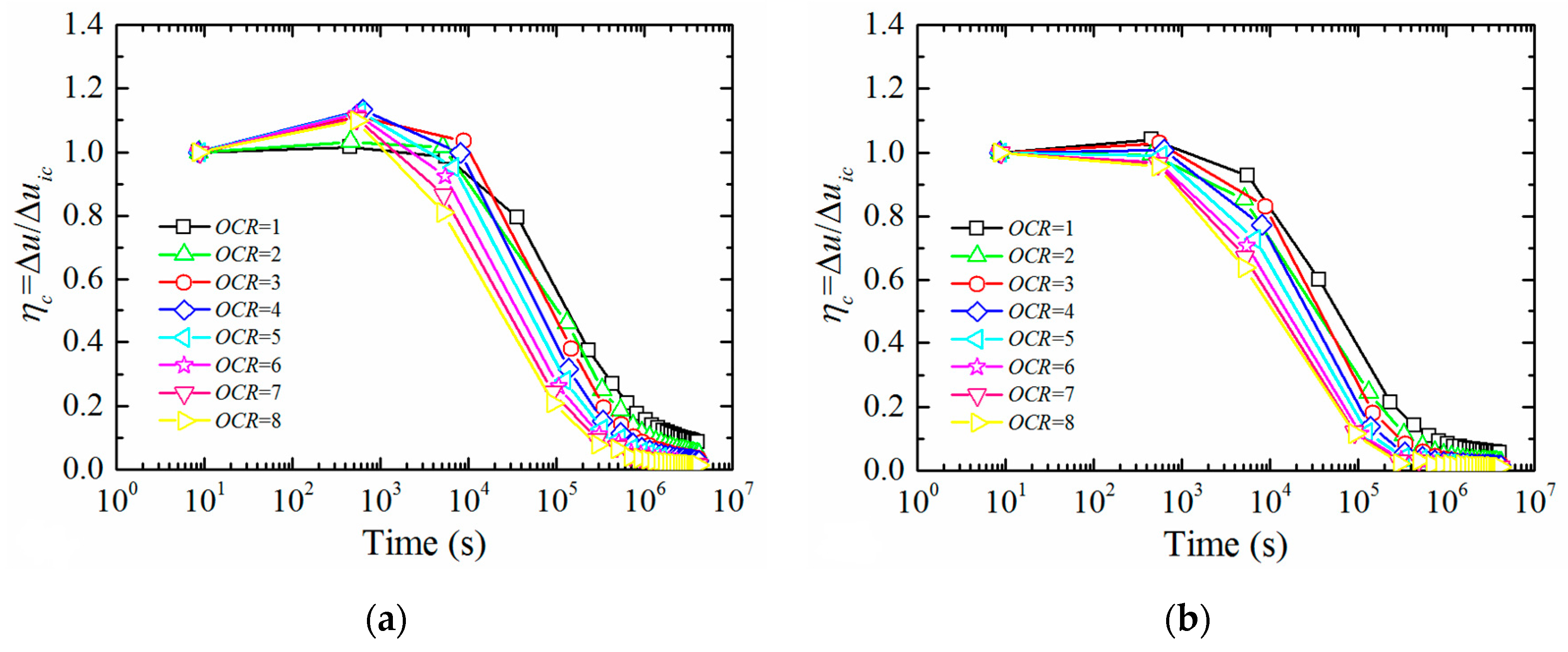
3.2.4. Effect of Soil Overconsolidation Ratio OCR
3.3. Time-Dependent Analysis of Pile Set-Up Based on Consolidation Effect
- τf is the local unit shaft resistance,
- δ is the friction angle of the pile–soil interface,
- , are the vertical and horizontal effective stress acting on the pile, respectively,
- βf is expressed by .
4. Conclusions
- (1)
- Good agreements between the calculated and measured time histories of EPWP are obtained. Besides, the continuity of soil stress at the interface of the mixed grid has been observed. Thus, the feasibility and reliability of the hybrid Lagrangian–ALE approach to establish finite element model to simulate the installation process of jacked pile and subsequent consolidation phase after installation in saturated fine-grained soil are verified.
- (2)
- When the entire process of pile jacking is conducted in saturated fine-grained soil, of which the normalized permeability Kn is less than 10 × 10−6, ks and OCR affect the rate and duration of EPWP dissipation; OCR also affects the value of EPWP at the end of pile jacking; however, cu and E only affect the value of EPWP at the end of pile jacking. A suggestion is that the static load test should be carried out after a period of time to avoid low effective stress after pile installation in soil with low ks and OCR.
- (3)
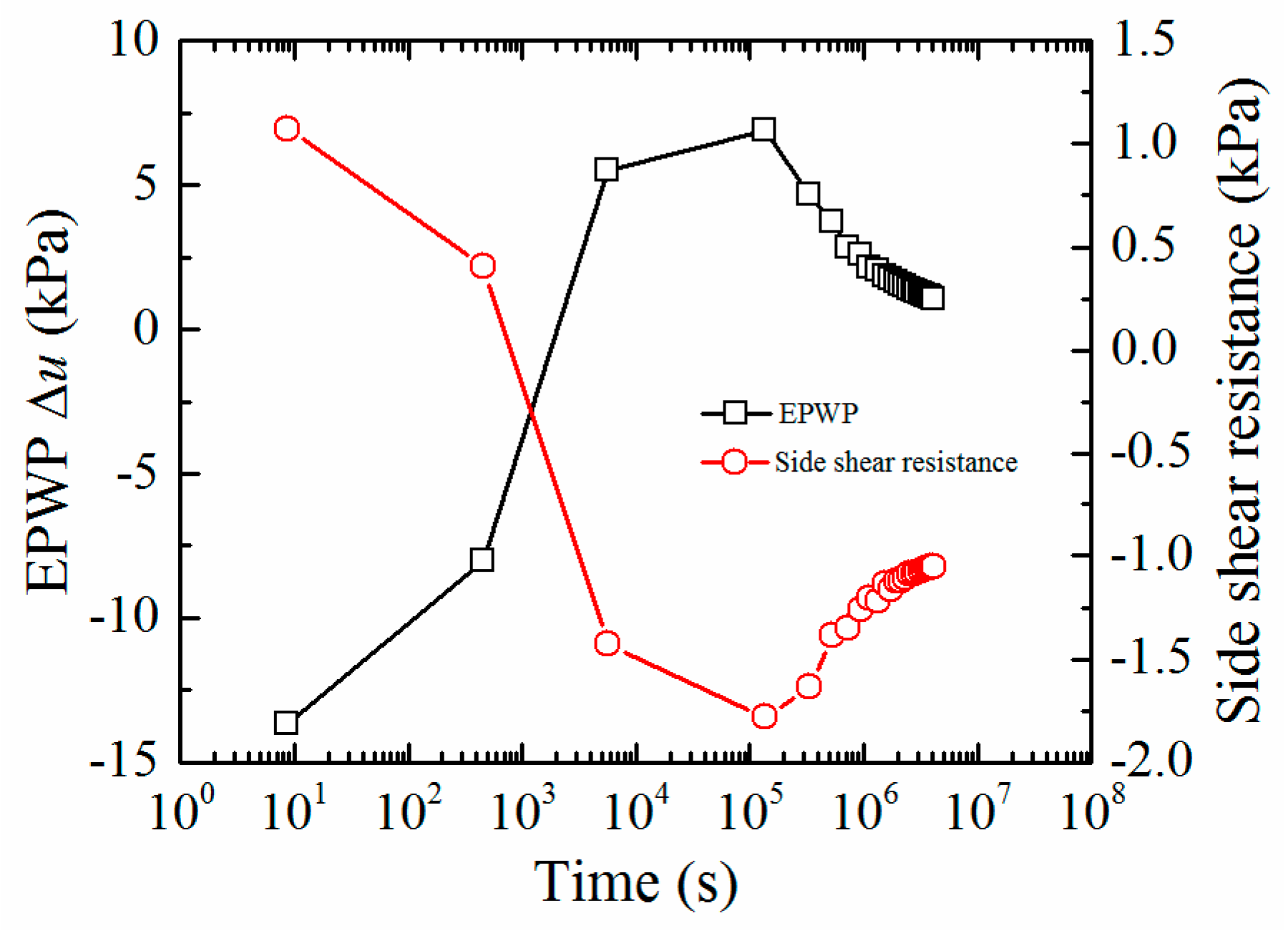
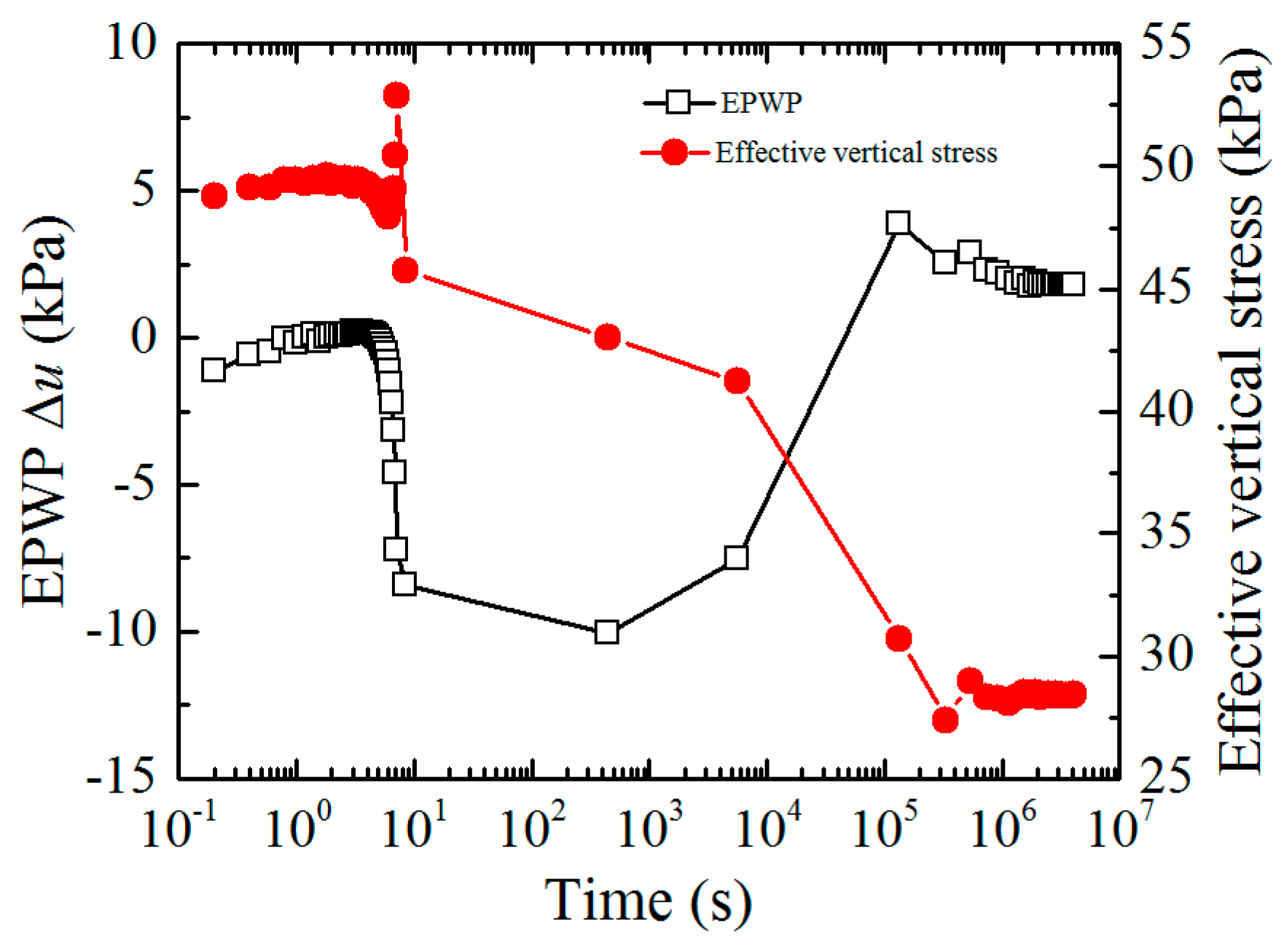
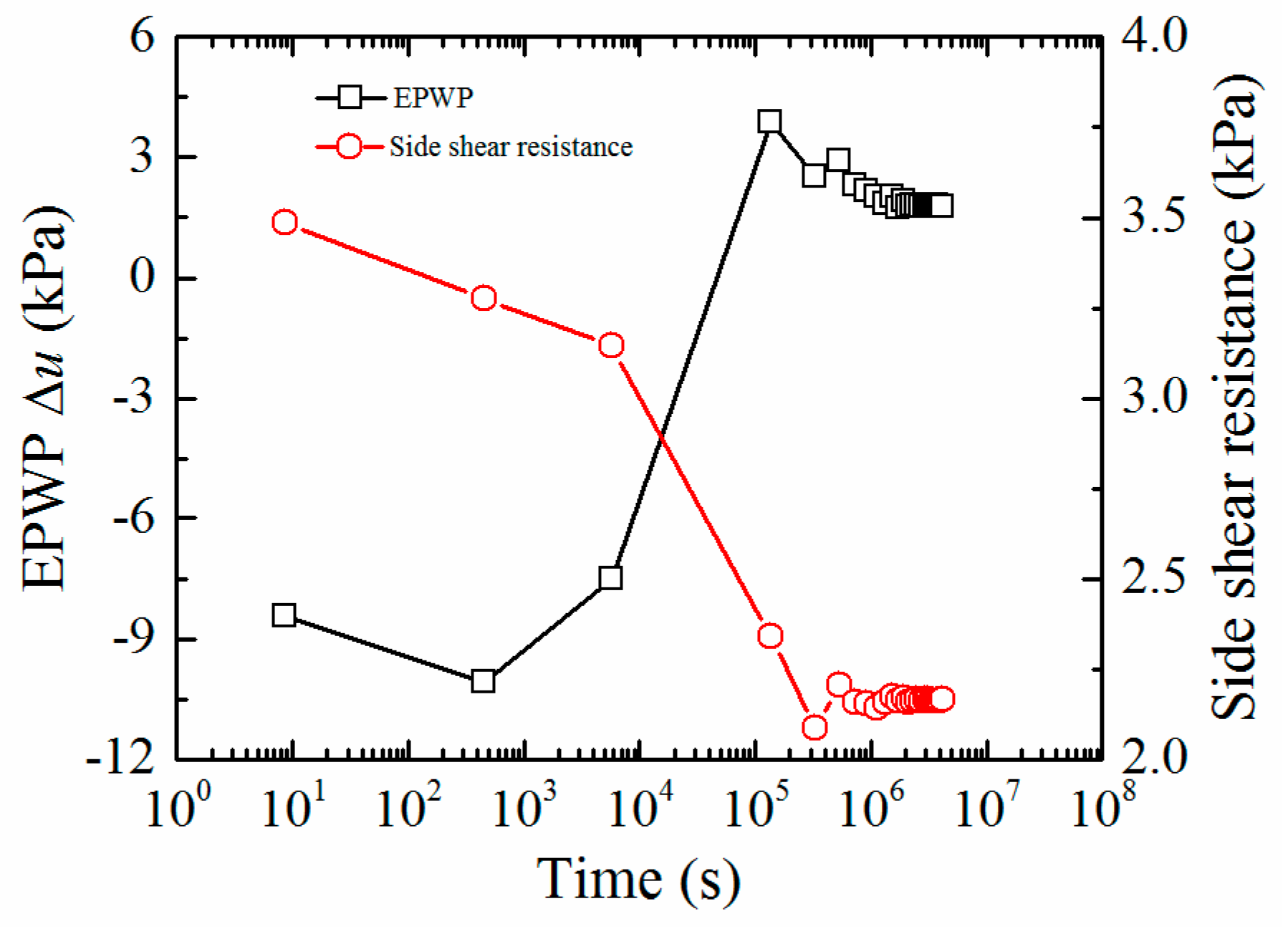
- During pile installation, the negative EPWP near ground surface around pile and at a certain depth below the pile tip would increase the effective stress and hence the penetration resistance; at the early stage of consolidation, the negative EPWP increases gradually to positive value, which could cause the decrease of bearing capacity of pile and even lead to the static load test pile sank suddenly; when the consolidation time reaches a certain value, the EPWP reaches a maximum, then the dissipation of the positive EPWP would increase the bearing capacity of pile to a certain extent.
- (4)
- During pile installation, the total stress increment is generally less than EPWP, thus temporarily reducing the effective stress and hence the penetration resistance; after pile installation, the side shear resistance of the pile increases gradually with the dissipation of positive EPWP, and the adhesion due to the aging effect should also be considered.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Notations
| I1 | the first stress invariant |
| J2 | the second invariant of the deviatoric stress tensor |
| α, γ, β, θ | the material parameters of the failure surface |
| c, φ | the frictional and cohesive strengths of the material |
| R | the curvature of the hardening cap |
| v | the Poisson’s ratio |
| X(κ) | the intersection of the cap surface with the I1 axis |
| L(κ) | the value of I1 at the location of the start of the cap |
| κ | the I1 coordinate of the intersection of the cap surface and the failure surface |
| the plastic volume change | |
| T | the maximum allowable hydrostatic tension |
| W | is related to the porosity n and the degree of saturation Sr |
| D | governs the slope of the initial loading curve in hydrostatic compression |
| X0 | is thought of as the preconsolidation hydrostatic pressure |
| σz | the overburden pressure |
| B, C, H | the stiffness, damping and permeability coefficient matrices, respectively |
| Q, S | the coupling and flow compressibility matrices, respectively |
| FP, FU | the vectors of fluid supply and external nodal forces, respectively |
| ks | the permeability coefficient of soil |
| pw | the pressure head |
| vf | the fluid velocity vector |
| zco | z-coordinate |
| zp | the final jacking depth |
| Eu, E′ | the elastic moduli in undrained and drained conditions, respectively |
| Gu, G′ | the shear moduli in undrained and drained conditions, respectively |
| v′, vu | the Poisson’s ratios in drained and undrained conditions, respectively |
| Δd | the penetration displacement of the structure into the Non-Lagrange domain |
| KN, KT | the normal and tangential contact stiffness, respectively |
| FT | the tangential force |
| FN | the normal coupling nodal force |
| FTcrit | the maximum tangential force |
| μ | the friction coefficient of the pile–soil interface |
| Rp | pile radius |
| Ir | the rigidity index |
| E | the Young’s modulus |
| cu | the undrained shear strength of soil |
| OCR | soil overconsolidation ratio |
| ω | the water content |
| ρ | the natural density of soil |
| K0 | the coefficient of earth pressure at rest |
| Ip | the plastic index |
| IL | the liquidity index |
| vc | the average pile installation rate |
| Kn | the normalized permeability |
| Δu | the value of EPWP |
| Δuic | the EPWP at the initial consolidation phase |
| ηc | the normalized EPWP |
| τf | the local unit shaft resistance |
| δ | the friction angle of the pile–soil interface |
| , | the vertical and horizontal effective stress acting on the pile, respectively |
| βf | is expressed by βf = K0tanδ |
References
- Su, J.B.; Cai, G.J.; Li, J.F.; Zhu, R.H.; Qin, W.G.; Zhai, Q. Safety assessment of buried pipeline during pile driving vibration in offshore engineering. Mar. Georesources Geotechnol. 2016, 34, 689–702. [Google Scholar] [CrossRef]
- Chen, Q.M.; Haque, M.N.; Abu-farsakh, M.; Fernandez, B.A. 2014. Field investigation of pile setup in mixed soil. Geotech. Test. J. 2014, 37, 1–14. [Google Scholar] [CrossRef]
- Komurka, V.E.; Wagner, A.B.; Edil, T.B. A review of pile set-up. In Proceedings of the 51st Annual Geotechnical Engineering Conference, St. Paul, MI, USA, 1 February 2003; pp. 105–130. [Google Scholar]
- Konkol, J.; Bałachowski, L. Large deformation finite element analysis of undrained pile installation. Studia Geotech. Et Mech. 2016, 38, 45–54. [Google Scholar] [CrossRef] [Green Version]
- Clark, J.I.; Meyerhof, G.G. The behavior of piles driven in clay. I. an investigation of soil stress and pore water pressure as related to soil properties. Can. Geotech. J. 1972, 9, 351–373. [Google Scholar] [CrossRef]
- Roy, M.; Tremblay, M.; Tavenas, F.; Rochelle, P. La. Development of pore pressures in quasi-static penetration tests in sensitive clay. Can. Geotech. J. 1982, 19, 124–138. [Google Scholar] [CrossRef]
- Liu, J.W.; Zhang, Z.M.; Yu, F.; Xie, Z.Z. Case history of installing instrumented jacked open-ended piles. J. Geotech. Geoenvironmental Eng. 2012, 138, 810–820. [Google Scholar] [CrossRef]
- Hwang, J.H.; Liang, N.; Chen, C.H. Ground response during pile driving. J. Geotech. Geoenvironmental Eng. 2001, 127, 939–949. [Google Scholar] [CrossRef]
- Hu, X.Q.; Jiao, Z.B.; Li, Y.H. Distribution and dissipation laws of excess static pore water pressures induced by pile driving in saturated soft clay with driven plastic drainage plate. Rock Soil Mech. 2011, 32, 3733–3737. (In Chinese) [Google Scholar]
- Dou, J.Z.; Chen, J.J.; Liao, C.C. Method for estimating initial excess pore water pressure during pile jacking into saturated fine-grained soil. Comput. Geotech. 2019, 116, 103203. [Google Scholar] [CrossRef]
- Ni, P.P.; Mangalathu, S.; Mei, G.X.; Zhao, Y.L. Permeable piles: An alternative to improve the performance of driven piles. Comput. Geotech. 2017, 84, 78–87. [Google Scholar] [CrossRef]
- Sheng, D.; Eigenbrod, K.D.; Wriggers, P. Finite element analysis of pile installation using large-slip frictional contact. Comput. Geotech. 2005, 32, 17–26. [Google Scholar] [CrossRef]
- Mabsout, M.E.; Tassoulas, J.L. A finite element model for the simulation of pile driving. Int. J. Numer. Methods Eng 1994, 37, 257–278. [Google Scholar] [CrossRef]
- Mabsout, M.E.; Sadek, S. A study of the effect of driving on pre-bored piles. Int. J. Numer. Anal. Methods Geomech. 2003, 27, 133–146. [Google Scholar] [CrossRef]
- Randolph, M.F.; Carter, J.P.; Wroth, C.P. Driven piles in clay-the effects of installation and subsequent consolidation. Géotechnique 1979, 29, 361–393. [Google Scholar] [CrossRef] [Green Version]
- Carter, J.P.; Randolph, M.F.; Wroth, C.P. Stress and pore pressure changes in clay during and after the expansion of a cylindrical cavity. Int. J. Numer. Anal. Methods Geomech. 1979, 3, 305–322. [Google Scholar] [CrossRef]
- Chandra, S.; Md Hossain, I. Prediction and observation of pore pressure due to pile driving. In Proceedings of the 3th International Conference on Case Histories in Geotechnical Engineering, St. Louis, MI, USA, 2 June 1993; pp. 279–284. [Google Scholar]
- Abu-Farsakh, M.; Tumay, M.; Voyiadjis, G. Numerical parametric study of piezocone penetration test in clays. Int. J. Geomech. 2003, 3, 170–181. [Google Scholar] [CrossRef]
- Abu-Farsakh, M.; Rosti, F.; Souri, A. Evaluating pile installation and subsequent thixotropic and consolidation effects on setup by numerical simulation for full-scale pile load tests. Can. Geotech. J. 2015, 52, 1734–1746. [Google Scholar] [CrossRef]
- Rosti, F.; Abu-Farsakh, M.; Jung, J. Development of analytical models to estimate pile setup in cohesive soils based on FE numerical analyses. Geotech. Geol. Eng. 2016, 34, 1119–1134. [Google Scholar] [CrossRef]
- Elias, M.B. Numerical Simulation of Pile Installation and Setup. Ph.D. dissertation, The University of Wisconsin, Milwaukee, WI, USA, 2008. [Google Scholar]
- Qiu, G.; Henke, S.; Grabe, J. Application of a Coupled Eulerian-Lagrangian approach on geomechanical problems involving large deformations. Comput. Geotech. 2011, 38, 30–39. [Google Scholar] [CrossRef]
- Pucker, T.; Grabe, J. Numerical simulation of the installation process of full displacement piles. Comput. Geotech. 2012, 45, 93–106. [Google Scholar] [CrossRef]
- Zhang, C.; Nguyen, G.D.; Einav, I. The end-bearing capacity of piles penetrating into crushable soils. Géotechnique 2013, 63, 341–354. [Google Scholar] [CrossRef] [Green Version]
- Xu, B.Z.; Li, S.; Xia, L.X.; Dai, X. Numerical simulation analysis of driving methods affecting soil around pipe pile. Hydro-Sci. Eng. 2015, 2, 38–43. (In Chinese) [Google Scholar]
- Sabetamal, H.; Nazem, M.; Carter, J.P.; Sloan, S.W. Large deformation dynamic analysis of saturated porous media with applications to penetration problems. Comput. Geotech. 2014, 55, 117–131. [Google Scholar] [CrossRef]
- Sabetamal, H.; Carter, J.P.; Sloan, S.W. Pore pressure response to dynamically installed penetrometers. Int. J. Geomech. 2018, 18, 04018061. [Google Scholar] [CrossRef]
- Hamann, T.; Qiu, G.; Grabe, J. Application of a coupled Eulerian-Lagrangian approach on pile installation problems under partially drained conditions. Comput. Geotech. 2015, 63, 279–290. [Google Scholar] [CrossRef]
- Qiu, G.; Grabe, J. Explicit modeling of cone and strip footing penetration under drained and undrained conditions using a visco-hypoplastic model. Geotechnik 2011, 34, 205–217. [Google Scholar] [CrossRef]
- Wang, D.; Bienen, B.; Nazem, M.; Tian, Y.H.; Zheng, J.B.; Pucker, T.; Randolph, M.F. Large deformation finite element analyses in geotechnical engineering. Comput. Geotech. 2015, 65, 104–114. [Google Scholar] [CrossRef]
- Schwer, L.E. Preliminary assessment of non-Lagrangian methods for penetration simulation. In Proceedings of the 8th International LS-DYNA Users Conference, Dearborn, MI, USA, 2–4 May 2004. [Google Scholar]
- Dou, J.Z.; Chen, J.J.; Zhang, Z.J. Influence of large-diameter pipe pile driving on surrounding marine soils and adjacent submarine pipelines. Mar. Georesources Geotechnol. 2019, 39, 1–19. [Google Scholar] [CrossRef]
- Roy, M.; Blanchet, R.; Tavenas, F.; Rochelle, P. La. Behaviour of a sensitive clay during pile driving. Can. Geotech. J. 1981, 18, 67–85. [Google Scholar] [CrossRef]
- Drucker, D.C. On uniqueness in the theory of plasticity. Q. Appl. Math. 1956, 14, 35–42. [Google Scholar] [CrossRef] [Green Version]
- Dou, J.Z.; Chen, J.J.; Wang, W. Method for estimating the degree of improvement in soil between adjacent tamping locations under dynamic compaction. Int. J. Geomech. 2019, 19, 04019134. [Google Scholar] [CrossRef]
- Tong, X.L.; Tuan, C.Y. Viscoplastic cap model for soils under high strain rate loading. J. Geotech. Geoenvironmental Eng. 2007, 133, 206–214. [Google Scholar] [CrossRef]
- Sandler, I.S.; Rubin, D. An algorithm and a modular subroutine for the CAP model. Int. J. Numer. Anal. Methods Geomech. 1979, 3, 173–186. [Google Scholar] [CrossRef]
- Chen, W.F.; Baladi, G.Y. Soil Plasticity: Theory and Implementation; Elsevier: New York, NY, USA, 1985. [Google Scholar]
- Gu, Q.; Lee, F.H. Ground response to dynamic compaction of dry sand. Geotechnique 2002, 52, 481–493. [Google Scholar] [CrossRef]
- Biot, M.A. Theory of propagation of elastic waves in a fluid-saturated porous solid. I. Low Freq. Range. II. High. Freq. Range. J. Acoust. Soc. Am. 1956, 28, 168–191. [Google Scholar]
- Zienkiewicz, O.C.; Chang, C.T.; Bettess, P. Drained, undrained, consolidating and dynamic behaviour assumptions in soils. Géotechnique 1980, 30, 385–395. [Google Scholar] [CrossRef]
- Zienkiewicz, O.C.; Shiomi, T. Dynamic behaviour of saturated porous media: The generalized Biot formulation and its numerical solution. Int. J. Numer. Anal. Methods Geomech. 1984, 8, 71–96. [Google Scholar] [CrossRef]
- Hallquist, J.O. LS-DYNA Theoretical Manual Version 971; Livermore Software Technology Corporation: Livermore, CA, USA, 2006. [Google Scholar]
- Massarsch, K.R.; Broms, B.B. Fracturing of soil caused by pile driving in clay. In Proceedings of the 9th International Conference on Soil Mechanics and Foundation Engineering, Tokyo, Japan, 10–15 July 1977; pp. 197–200. [Google Scholar]
- Wang, Y.X.; Sun, J. Influence of pile driving on properties of soils around pile and pore water pressure. Chin. J. Rock Mech. Eng. 2004, 23, 153–158. (In Chinese) [Google Scholar]
- Gibson, R.E.; Anderson, W.F. In situ measurement of soil properties with the pressuremeter. Civ. Eng. Public Work. Rev. 1961, 56, 615–618. [Google Scholar]
- Massarsch, K.R. Soil Movements Caused by Pile Driving in Clay. Ph.D. Thesis, Royal Institute of Technology, Brinellvägen, Stockholm, 1976. [Google Scholar]
- Hajduk, E.L. Full Scale Field Testing Examination of Pile Capacity Gain with Time. Ph.D. Thesis, University of Massachusetts, Lowell, MA, USA, 2006. [Google Scholar]

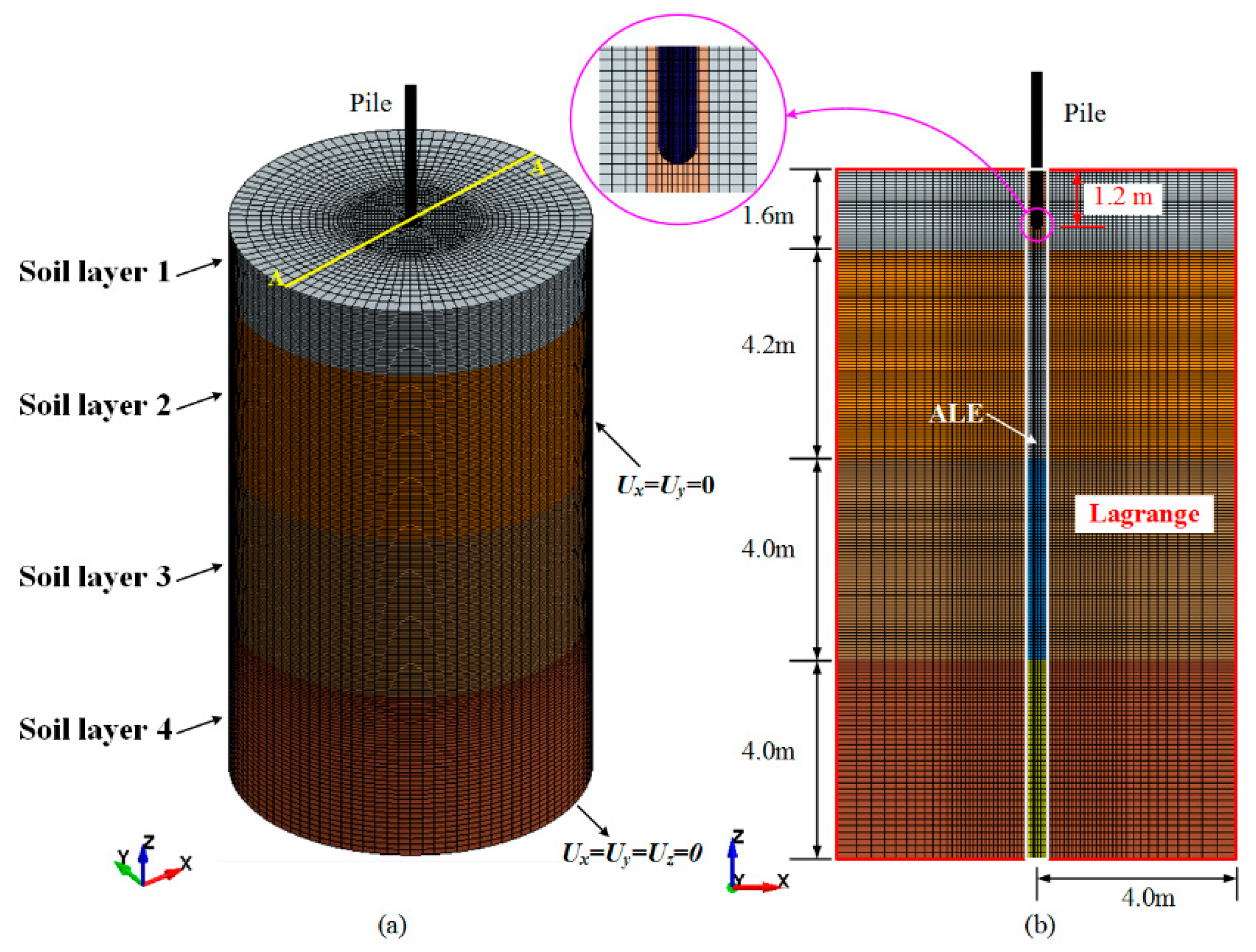







| Soil Name | Thickness (m) | ρ (kg/m3) | ν | K0 | cu (kPa) | ω (%) | Ip | IL |
|---|---|---|---|---|---|---|---|---|
| Clay crust | 1.6 | 1751 | 0.305 | 0.438 | 19.9 | 42.7 | 24.3 | 0.88 |
| Silty clay | 4.2 | 1625 | 0.288 | 0.405 | 12.5 | 58.6 | 17.0 | 2.42 |
| Clayey silt | 4.0 | 1720 | 0.276 | 0.382 | 24.3 | 40.7 | 11.7 | 2.16 |
| Clayey silt with sand | 4.0 | 1920 | 0.266 | 0.362 | 30.0 | 27.3 | 7.0 | 2.48 |
| Sand | >13.7 |
| Soil Layer | K (kPa) | G (kPa) | α (kPa) | γ | β | θ | W | R | D (kPa−1) | T (kPa) |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 6659 | 2985 | 23.8 | 0 | 0 | 0.238 | 0.5 | 4.48 | 0.0003 | 0 |
| 2 | 3797 | 1875 | 14.6 | 0 | 0 | 0.275 | 0.5 | 4.13 | 0.0003 | 0 |
| 3 | 6921 | 3645 | 23.8 | 0 | 0 | 0.238 | 0.5 | 4.48 | 0.0003 | 0 |
| 4 | 8115 | 4500 | 14.6 | 0 | 0 | 0.275 | 0.5 | 4.13 | 0.0003 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dou, J.; Chen, J.; Liao, C.; Sun, M.; Han, L. Study on the Correlation between Soil Consolidation and Pile Set-Up Considering Pile Installation Effect. J. Mar. Sci. Eng. 2021, 9, 705. https://doi.org/10.3390/jmse9070705
Dou J, Chen J, Liao C, Sun M, Han L. Study on the Correlation between Soil Consolidation and Pile Set-Up Considering Pile Installation Effect. Journal of Marine Science and Engineering. 2021; 9(7):705. https://doi.org/10.3390/jmse9070705
Chicago/Turabian StyleDou, Jinzhong, Jinjian Chen, Chencong Liao, Min Sun, and Lei Han. 2021. "Study on the Correlation between Soil Consolidation and Pile Set-Up Considering Pile Installation Effect" Journal of Marine Science and Engineering 9, no. 7: 705. https://doi.org/10.3390/jmse9070705
APA StyleDou, J., Chen, J., Liao, C., Sun, M., & Han, L. (2021). Study on the Correlation between Soil Consolidation and Pile Set-Up Considering Pile Installation Effect. Journal of Marine Science and Engineering, 9(7), 705. https://doi.org/10.3390/jmse9070705





