Mathematical Model and Numerical Method of Calculating the Dynamics of High-Temperature Drying of Milled Peat for the Production of Fuel Briquettes
Abstract
1. Introduction
- Darcy’s law for calculating the filtration velocities of the phases;
- the equation of state for calculating the pressures of the gas phase components;
- the expression for the capillary pressure of the liquid;
- the formula for the contact surface area of the liquid and gas phases in the pores of the body;
- the thermal-concentration deformation equation;
- the formulas for the intensity of the phase transitions on the outer and inner surfaces of peat particles and the diffusion coefficients of the liquid and gas phases.
2. Materials and Methods
2.1. Mathematical Model
2.2. Single-Valued Condition
2.3. Numerical Method of Solution
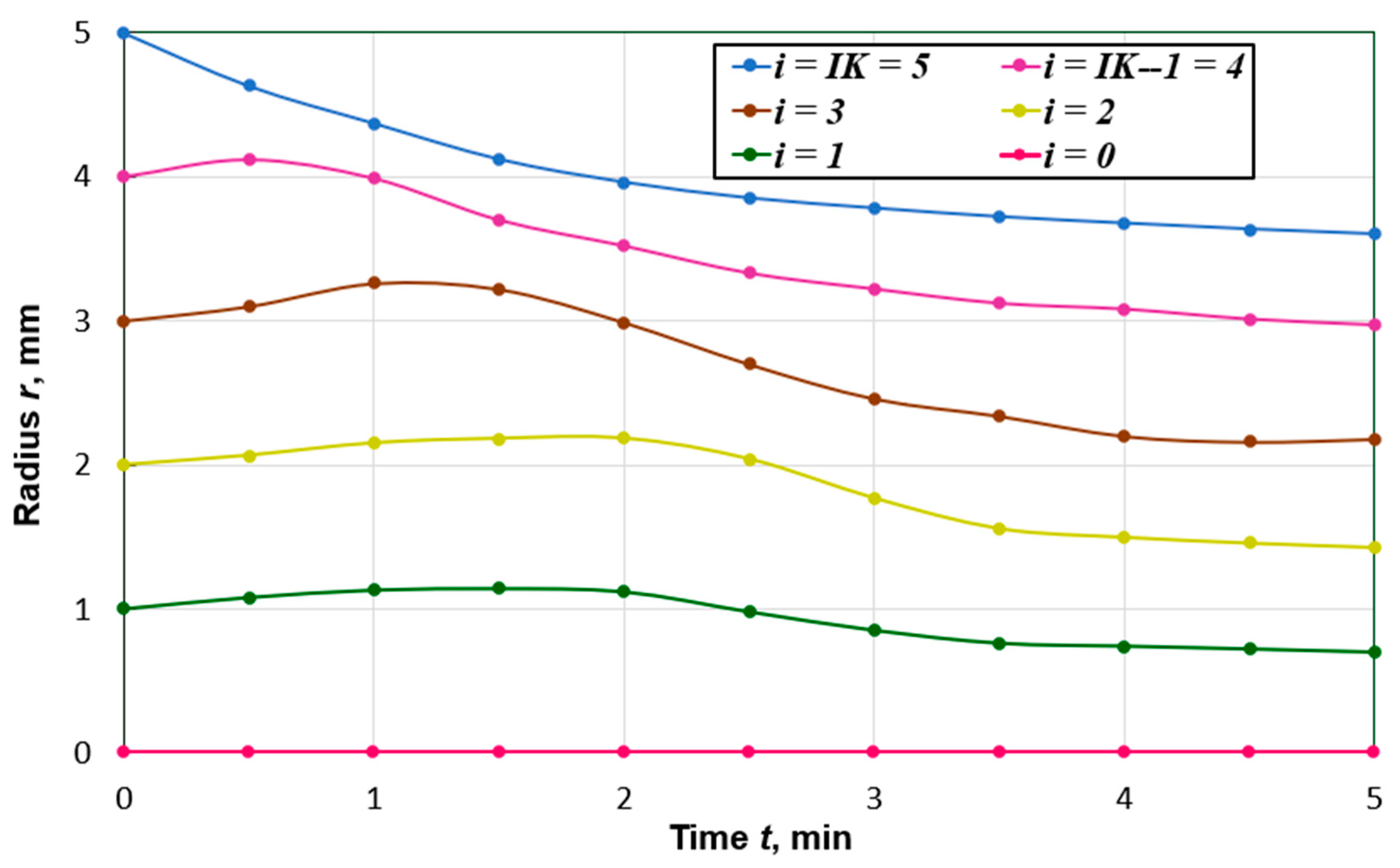
2.4. Approbation
3. Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Stolbikova, G.E.; Kuporova, A.V. Milled peat drying in case of different size of feed and conditions. Gorn. Inf. Anal. Byulleten 2018, 5, 65–73. Available online: https://cyberleninka.ru/article/n/osobennosti-sushki-frezernogo-torfa-razlichnoy-udelnoy-zagruzki-i-rezhimov-sushki/viewer (accessed on 12 November 2022). (In Russian). [CrossRef]
- Kindzera, D.P.; Khanyk, Y.a.M.; Atamanyuk, V.M.; Duleba, V.P. Kinetic of filtration drying of peat. NU Lviv. Polytech. Chem. Technol. Subst. Appl. 2002, 447, 179–182. Available online: https://ena.lpnu.ua:8443/server/api/core/bitstreams/10659275-4d3c-4960-baf9-d8ae59a11013/content (accessed on 13 November 2022).
- Smolyaninov, S.I.; Lozbin, V.I.; Ikryn, V.M.; Bblichmaer, Y.A. Features of thermal decomposition of peat according to derivatographic data. Bull. Tomsk. Polytech. Univ. Geo Assets Eng. 1976, 274, 56–60. Available online: https://cyberleninka.ru/article/n/osobennosti-termicheskogo-razlozheniya-torfa-po-derivatograficheskim-dannym (accessed on 1 December 2022).
- Mykhailyk, V.A.; Snezhkin, Y.u.F.; Oranska, O.I.; Korinchevska, T.V.; Korinchuk, D.M. Study of the thermal properties of solid residues of milled peat after the humus substances extraction. Ind. Heat Eng. 2015, 37, 54–64. (In Ukrainian) [Google Scholar] [CrossRef]
- Korinchuk, D. Non-isothermal analysis of components of composite fuels based on peat and biomass. Energy Autom. 2018, 1, 56–71. Available online: http://nbuv.gov.ua/UJRN/eia_2018_1_8 (accessed on 1 December 2022). (In Russian). [CrossRef]
- Leroy-Cancellieri, V.; Cancellieri, D.; Leoni, E.; Filkov, A.I.; Simeoni, A. A global mechanism for the thermal degradation of peat. In Proceedings of the 4th Fire Behavior and Fuels Conference, St. Petersburg, Russia, 1–4 July 2013; International Association of Wildland Fire: Missoula, MT, USA, 2013. [Google Scholar]
- Chen, W.-H.; Kuo, P.-C. Isothermal torrefaction kinetics of hemicellulose, cellulose, lignin and xylan using thermogravimetric analysis. Energy 2011, 36, 6451–6460. [Google Scholar] [CrossRef]
- Nikitenko, N.I.; Snezhkin, Y.F.; Sorokovaya, N.N. Mathematical simulation of heat and mass transfer, phase conversions, and shrinkage for optimization of the process of drying of thermolabile materials. J. Eng. Phys. Thermophys. 2005, 78, 75–89. [Google Scholar] [CrossRef]
- Fil’kov, A.I.; Gladkii, D.A. Mathematical modeling of low-temperature drying of a peat layer. Tomsk. State Univ. J. 2012, 3, 93–106. (In Russian) [Google Scholar]
- Luikov, A.V. Drying Theory; Energy: Moscow, Russia, 1968; p. 472. Available online: https://www.twirpx.com/file/1241798/ (accessed on 10 December 2022). (In Russian)
- Wang, Z.; Wang, Q.; Lai, J.; Liu, D.; Hu, A.; Xu, L.; Chen, Y. Numerical Simulation of Heat and Mass Transfer in Sludge Low-Temperature Drying Process. Entropy 2022, 24, 1682. [Google Scholar] [CrossRef]
- Nikitenko, N.I. Problems of the radiation theory of heat and mass transfer in solid and liquid media. J. Eng. Phys. Thermophys. 2000, 73, 840–848. [Google Scholar] [CrossRef]
- Aleksanyan IYu Titova, L.M.; Nugmanov, A.K. Simulation of the process of drying a dispersed material in a fluidized bed. Tech. Technol. Food Prod. 2014, 3, 96–102. Available online: https://cyberleninka.ru/article/n/modelirovanie-protsessa-sushki-dispersnogo-materiala-v-kipyaschem-sloe/viewer (accessed on 15 November 2022). (In Russian).
- Strumillo, C.; Grinchik, N.N.; Kuts, P.S.; Akulich, P.V.; Zbicinski, I. Numerical modeling of nonisothermal moisture transfer in biological colloidal porous materials. J. Eng. Phys. Thermophys. 1994, 66, 181–190. [Google Scholar] [CrossRef]
- Huzova, I.O.; Atamanyuk, V.M. Dynamics of drying processes of plant raw material in the period of decreasing speed. J. Chem. Technol. 2022, 30, 419–430. Available online: http://chemistry.dnu.dp.ua/article/view/259694 (accessed on 9 December 2022).
- Luikov, A.V. Heat and Mass Transfer in Capillary Porous Bodies; Pergamon Press: Oxford, UK, 1966; pp. 233–303. [Google Scholar] [CrossRef]
- Akulich, P.V. Thermohydrodynamic Processes in Drying Technique; ITMO: Minsk, Belarus, 2002; p. 268. [Google Scholar]
- Kotov, B.I.; Bandura, V.N.; Kalinichenko, R.A. Mathematical modeling and identification of heat and mass transfer in plant dispersed material during drying and heating by an ultra-high frequency electromagnetic field. Energy Autom. 2018, 6, 35–50. Available online: http://nbuv.gov.ua/UJRN/eia_2018_6_6 (accessed on 3 December 2022).
- Akulich, P.V.; Slizhuk, D.S. Heat and Mass Transfer in a Dense Layer during Dehydration of Colloidal and Sorption Capillary-Porous Materials under Conditions of Unsteady Radiation-Convective Energy Supply. Theor. Found. Chem. Eng. 2022, 56, 152–161. [Google Scholar] [CrossRef]
- Rudobashta, S.P.; Kartashov, E.M.; Zueva, G.A. Mathematical modeling of convective drying of materials with taking into account their shrinking. Inzh. Fiz. Zh. 2020, 93, 1394–1401. [Google Scholar] [CrossRef]
- Rudobashta, S.P.; Dmitriev, V.M. Investigation of the Diffusion Properties of Plant Capillary-Porous Colloidal Materials with Regard to Their Shrinkage. J. Eng. Phys. Thermophys. 2022, 95, 1357–1365. [Google Scholar] [CrossRef]
- Narang, H.; Wu, F.; Mohammed, A.R. An Efficient Acceleration of Solving Heat and Mass Transfer Equations with the First Kind Boundary Conditions in Capillary Porous Radially Composite Cylinder Using Programmable Graphics Hardware. J. Comput. Commun. 2019, 7, 267–281. [Google Scholar] [CrossRef]
- Nikitenko, N.I.; Snezhkin, Y.u.F.; Sorokovaya, N.N.; Kolchik Yu, N. Molecular Radiation Theory and Methods for Calculating Heat and Mass Transfer; Naukova Dumka: Kyiv, Ukraine, 2014; p. 744. Available online: http://ittf.kiev.ua/wp-content/uploads/2016/12/nikitenko.pdf (accessed on 3 December 2022). (In Russian)
- Sneszkin, Y.F.; Korinchuk, D.N. Modeling of high-temperature drying of peat and biomass in biofuel production technologies. Sci. Work. 2017, 81, 125–130. Available online: https://sciworks.ontu.edu.ua/en/site/archives/81-1?page=2 (accessed on 15 January 2023). (In Russian).
- Leibenzon, L.S. Variational Methods for Solving Problems in the Theory of Elasticity; Gostekhizdat: Moscow, Russia, 1943; p. 286. (In Russian) [Google Scholar]
- Nikitenko, N.I. Investigation of dynamics of evaporation of condensed bodies on the basis of the law of spectral-radiation intensity of particles. Inzh. Fiz. Zh. 2002, 75, 128–134. [Google Scholar] [CrossRef]
- Sorokovaya, N.N.; Snezhkin, Y.F.; Shapar, R.A.; Sorokovoi, R.Y. Mathematical Simulation and Optimization of the Continuous Drying of Thermolabile Materials. J. Eng. Phys. Thermophys. 2019, 92, 1180–1190. [Google Scholar] [CrossRef]
- Sorokova, N.; Didur, V.; Variny, M. Mathematical Modeling of Heat and Mass Transfer during Moisture–Heat Treatment of Castor Beans to Improve the Quality of Vegetable Oil. Agriculture 2022, 12, 1356. [Google Scholar] [CrossRef]
- Broido, A. A Simple, sensitive graphical method of treating thermogravimetric analysis data. J. Polym. Sci. Part B Polym. Phys. 1969, 7, 1761–1773. [Google Scholar] [CrossRef]
- Oostindie, K. A Simulation Model for the Calculation of Water Balance, Cracking and Surface Subsidence of Clay Soils; Rep. 47; Winand Staring Centre for Integrated Land, Soil and Water Research: Wageningen, The Netherlands, 1992; p. 65. Available online: https://books.google.com.ua/books/about/FLOCR.html?id=D39jHAAACAAJ&redir_esc=y (accessed on 12 January 2023).
- Garnier, P.; Perrier, E.; Angulo, A.J.; Baveye, P. Numerical model of 3-dimensional anisotropic deformation and water flow in welling soil. Soil Sci. 1997, 162, 410–420. [Google Scholar] [CrossRef]
- Rudobashta, S.P. Mass Transfer in Systems with a Solid Phase; Chemistry: Moscow, Russia, 1980; p. 248. Available online: https://www.libex.ru/detail/book796756.html (accessed on 11 January 2023). (In Russian)
- Keltsev, N.V. Fundamentals of Sorption Technology, 2nd ed.; Chemistry: Moscow, Russia, 1984; p. 590. (In Russian) [Google Scholar]
- Nikitenko, N.I. A method for calculating the temperature field by the data of measurement of the deformation of a body. Inzh. Fiz. Zh. 1980, 39, 281–285. [Google Scholar]
- Nikitenko, N.I. Radiation heat conduction mikromechanism. In Proceedings of the First International Conference on Transport Phenomena in Processing, Lancaster, PA, USA, 22–26 March 1992; pp. 1580–1588. [Google Scholar]
- Kutateladze, S.S. Fundamentals of the Theory of Heat Transfer; Atomizdat: Moscow, Russia, 1979; p. 416. (In Russian) [Google Scholar]
- Voznyuk, S.T.; Moshinsky, V.S.; Klymenko, M.O.; Lyko, D.V.; Gneushev, V.O.; Lagodnyuk, O.A.; Voznyuk, N.M.; Kucherova, A.V. Peat Land Resource of the North-Western Region of Ukraine; NUWEE: Rivne, Ukraine, 2017; p. 117. Available online: http://ep3.nuwm.edu.ua/id/eprint/7506 (accessed on 11 October 2022). (In Ukrainian)





| Name | Meaning |
|---|---|
| Constants for coefficients of diffusion, | γDfl = 0.9 × 10−8 m2/s; γDv = 0.134 × 10−4 m2/s; |
| Coefficient of evaporation from surface, | γc = 0.2578 × 10−4 kg/(m2∙s); |
| Total permeability of the medium, | K0 = 1 × 10−5; |
| Relative permeability of the fluid, | Kfl = 0.2 × 10−14; |
| Relative permeability of the gas, | Kg = 1.1 × 10−8; |
| Characteristic parameter of pore size dispersion | r* = 1 × 10−8 m. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sorokova, N.; Variny, M.; Pysmennyy, Y.; Kol’chik, Y. Mathematical Model and Numerical Method of Calculating the Dynamics of High-Temperature Drying of Milled Peat for the Production of Fuel Briquettes. Computation 2023, 11, 53. https://doi.org/10.3390/computation11030053
Sorokova N, Variny M, Pysmennyy Y, Kol’chik Y. Mathematical Model and Numerical Method of Calculating the Dynamics of High-Temperature Drying of Milled Peat for the Production of Fuel Briquettes. Computation. 2023; 11(3):53. https://doi.org/10.3390/computation11030053
Chicago/Turabian StyleSorokova, Natalia, Miroslav Variny, Yevhen Pysmennyy, and Yuliia Kol’chik. 2023. "Mathematical Model and Numerical Method of Calculating the Dynamics of High-Temperature Drying of Milled Peat for the Production of Fuel Briquettes" Computation 11, no. 3: 53. https://doi.org/10.3390/computation11030053
APA StyleSorokova, N., Variny, M., Pysmennyy, Y., & Kol’chik, Y. (2023). Mathematical Model and Numerical Method of Calculating the Dynamics of High-Temperature Drying of Milled Peat for the Production of Fuel Briquettes. Computation, 11(3), 53. https://doi.org/10.3390/computation11030053












