Spatial Reasoning and Its Contribution to Mathematical Performance Across Different Content Domains: Evidence from Chinese Students
Abstract
:1. Introduction
1.1. Spatial Reasoning Constructs
1.1.1. Mental Rotation
1.1.2. Spatial Orientation
1.1.3. Spatial Visualization
1.2. Links Between Spatial Reasoning and Mathematical Performance
1.3. Present Study
- To what extent does spatial reasoning continue to predict mathematical performance among Chinese students who have had relatively limited exposure to space-related curriculum content?
- How do different constructs of spatial reasoning (mental rotation, spatial visualization, and spatial orientation) specifically impact mathematical performance in various content domains (number, geometric shapes and measures, and data display) among Chinese elementary school students?
2. Materials and Methods
2.1. Design
2.2. Procedure and Participants
2.3. Materials
2.3.1. Spatial Reasoning Test
2.3.2. Mathematics Test
2.4. Data Analysis
3. Results
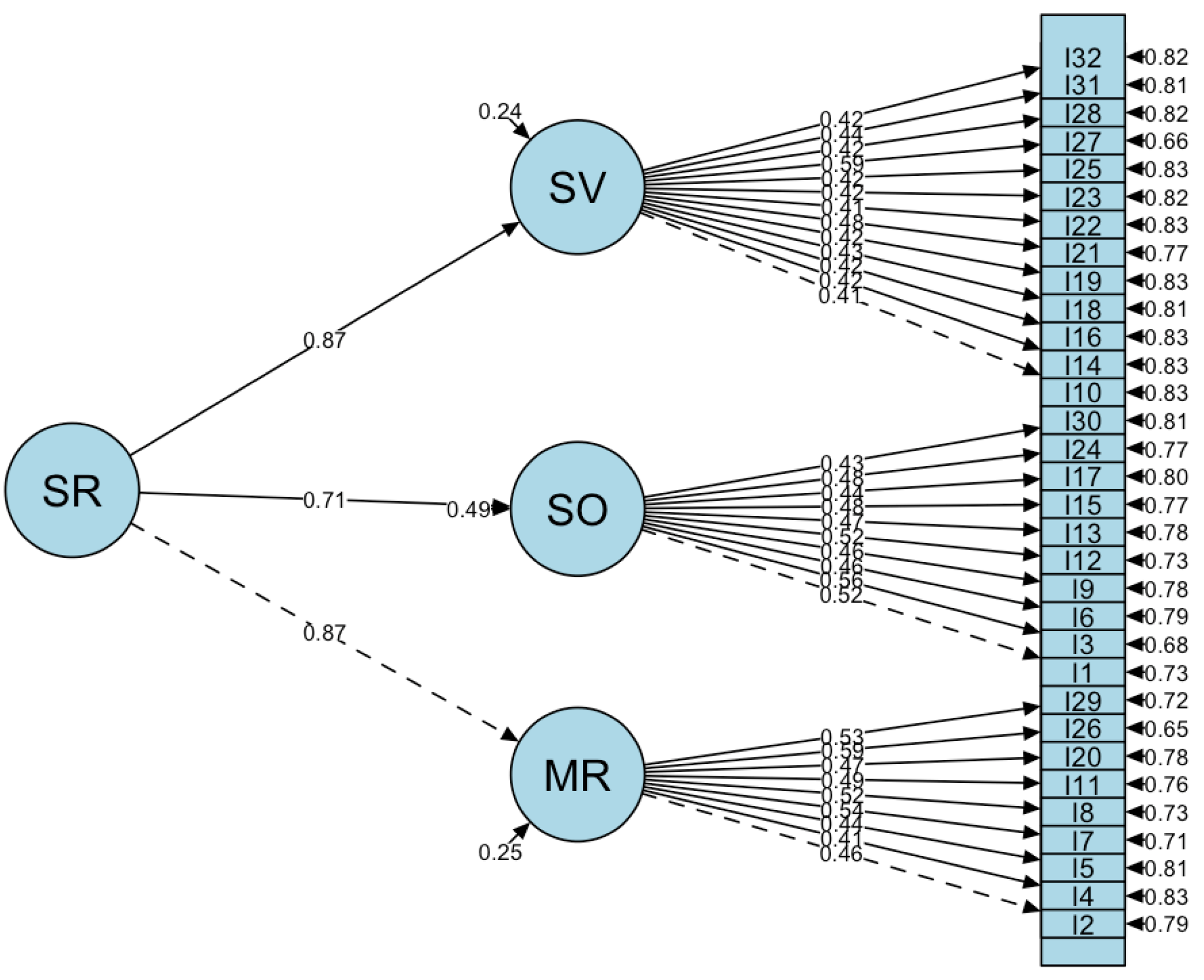
3.1. Phase I: Instrument Analysis Results
3.1.1. Reliability and Construct Validity
3.1.2. Item Difficulty and Discrimination
3.1.3. Item Analysis Based on Rasch Model
3.2. Phase II: Main Study Results
3.2.1. Descriptive Statistics
3.2.2. Correlations Between Spatial and Math Variables
3.2.3. Regression Analysis
Predictors of Overall Mathematical Performance
Predictors of Performance in Number
Predictors of Performance in Geometric Shapes and Measures
Predictors of Performance in Data Display
4. Discussion
4.1. Summary of Findings
4.2. Educational Implications
4.3. Limitations and Future Directions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Battista, Michael. T. 2007. The development of geometric and spatial thinking. In Second Handbook of Research on Mathematics Teaching and Learning. Edited by Frank K. Lester, Jr. Charlotte: Information Age Publishing, pp. 843–908. [Google Scholar]
- Boone, William J., John R. Staver, and Melissa S. Yale. 2014. Rasch Analysis in the Human Sciences. New York: Springer. [Google Scholar]
- Borges-Rey, Eddy. L. 2017. Data literacy and citizenship: Understanding ‘big Data’ to boost teaching and learning in science and mathematics. In Handbook of Research on Driving STEM Learning with Educational Technologies. Hershey: IGI Global, pp. 65–79. [Google Scholar]
- Bruce, Catherine D., Brent Davis, Nathalie Sinclair, Lynn McGarvey, David Hallowell, Michelle Drefs, Krista Francis, Zachary Hawes, Joan Moss, Joanne Mulligan, and et al. 2017. Under-standing gaps in research networks: Using spatial reasoning as a window into the importance of networked educational research. Educational Studies in Mathematics 95: 143–61. [Google Scholar] [CrossRef]
- Butterworth, Brian, Robert Reeve, and Fiona Reynolds. 2011. Using mental representations of space when words are unavailable: Studies of enumeration and arithmetic in indigenous Australia. Journal of Cross-Cultural Psychology 42: 630–38. [Google Scholar] [CrossRef]
- Casey, Beth M., Elizabeth Pezaris, Bonnie Fineman, Amanda Pollock, Lindsay Demers, and Eric Dearing. 2015. A longitudinal analysis of early spatial skills compared to arithmetic and verbal skills as predictors of fifth-grade girls’ math reasoning. Learning and Individual Differences 40: 90–100. [Google Scholar] [CrossRef]
- Cheng, Yi-Ling, and Kelly S. Mix. 2014. Spatial training improves children’s mathematics ability. Journal of Cognition and Development 15: 2–11. [Google Scholar] [CrossRef]
- Cipora, Krzysztof, Katarzyna Patro, and Hans-Christoph Nuerk. 2015. Are Spatial-Numerical Associations a Cornerstone for Arithmetic Learning? The Lack of Genuine Correlations suggests: No. Mind, Brain, and Education 9: 190–207. [Google Scholar] [CrossRef]
- Clements, Douglas H., and Julie Sarama. 2014. Learning and Teaching Early Math: The Learning Trajectories Approach. New York: Routledge, pp. 107–11. First published 2009. [Google Scholar] [CrossRef]
- Clements, Douglas H., and Michael T. Battista. 1992. Geometry and spatial reasoning. In Handbook of Research on Mathematics Teaching and Learning. Edited by Douglas A. Grouws. New York: Macmillan, pp. 420–64. [Google Scholar]
- Congdon, Eliza L., Marina Vasilyeva, Kelly S. Mix, and Susan C. Levine. 2018b. From intuitive spatial measurement to understanding of units. In Visualizing Mathematics: The Role of Spatial Reasoning in Mathematical Thought. Edited by Kelly S. Mix and Michael T. Battista. Berlin: Springer, pp. 25–46. [Google Scholar]
- Congdon, Eliza L., Mee-Kyoung Kwon, and Susan C. Levine. 2018a. Learning to measure through action and gesture: Children’s prior knowledge matters. Cognition 180: 182–90. [Google Scholar] [CrossRef]
- Cornu, Véronique, Christine Schiltz, Tahereh Pazouki, and Romain Martin. 2017. Training early visuo-spatial abilities: A controlled classroom-based intervention study. Applied Developmental Science 23: 1–21. [Google Scholar] [CrossRef]
- Danan, Yehudit, and Sarit Ashkenazi. 2022. The influence of sex on the relations among spatial ability, math anxiety, and math performance. Trends in Neuroscience and Education 29: 100196. [Google Scholar] [CrossRef]
- Davis, Brent, and The Spatial Reasoning Study Group, eds. 2015. Spatial Reasoning in the Early Years: Principles, Assertions, and Speculations. New York: Routledge. [Google Scholar]
- Dziuban, Charles D., and Edwin C. Shirkey. 1974. When is a correlation matrix appropriate for factor analysis? Some decision rules. Psychological Bulletin 81: 358. [Google Scholar]
- Frick, Andrea. 2019. Spatial transformation abilities and their relation to later mathematics performance. Psychological Research 83: 1465–84. [Google Scholar] [CrossRef]
- Gilligan, Katie A., Alex Hodgkiss, Michael SC Thomas, and Emily K. Farran. 2019. The developmental relations between spatial cognition and mathematics in primary school children. Developmental Science 22: e12786. [Google Scholar] [CrossRef] [PubMed]
- Gilligan, Katie A., Eirini Flouri, and Emily K. Farran. 2017. The contribution of spatial ability to mathematics achievement in middle childhood. Journal of Experimental Child Psychology 163: 107–25. [Google Scholar] [CrossRef] [PubMed]
- Gunderson, Elizabeth A., Gerardo Ramirez, Sian L. Beilock, and Susan C. Levine. 2012. The relation between spatial skill and early number knowledge: The role of the linear number line. Developmental Psychology 48: 1229–41. [Google Scholar] [CrossRef]
- Harris, Danielle, Tom Lowrie, Tracy Logan, and Mary Hegarty. 2021. Spatial reasoning, mathematics, and gender: Do spatial constructs differ in their contribution to performance? British Journal of Educational Psychology 91: 409–41. [Google Scholar] [CrossRef]
- Hawes, Zachary, and Daniel Ansari. 2020. What explains the relationship between spatial and mathematical skills? A review of evidence from brain and behavior. Psychonomic Bulletin & Review 27: 465–82. [Google Scholar] [CrossRef]
- Hawes, Zachary, Joan Moss, Beverly Caswell, and Daniel Poliszczuk. 2015. Effects of mental rotation training on children’s spatial and mathematics performance: A randomized controlled study. Trends in Neuroscience and Education 4: 60–68. [Google Scholar] [CrossRef]
- Hawes, Zachary, Joan Moss, Beverly Caswell, Jisoo Seo, and Daniel Ansari. 2019. Relations between numerical, spatial, and executive function skills and mathematics achievement: A latent-variable approach. Cognitive Psychology 109: 68–90. [Google Scholar] [CrossRef]
- Hawes, Zachary, Joan Moss, Beverly Caswell, Sarah Naqvi, and Sharla MacKinnon. 2017. Enhancing children’s spatial and numerical skills through a dynamic spatial approach to early geometry instruction: Effects of a 32-week intervention. Cognition and Instruction 35: 236–64. [Google Scholar] [CrossRef]
- Hegarty, Mary. 2018. Ability and sex differences in spatial thinking: What does the mental rotation test really measure? Psychonomic Bulletin & Review 25: 1212–19. [Google Scholar] [CrossRef]
- Hegarty, Mary, and David Waller. 2005. Individual Differences in Spatial Abilities. In The Cambridge Handbook of Visuospatial Thinking. Edited by Priti Shah and Akira Miyake. Cambridge: Cambridge University Press, pp. 121–69. [Google Scholar] [CrossRef]
- Hegarty, Mary, and Maria Kozhevnikov. 1999. Types of visual–spatial representations and mathematical problem solving. Journal of Educational Psychology 91: 684–89. [Google Scholar] [CrossRef]
- Hu, Tao, Jing Yang, Rongxiu Wu, and Xiaopeng Wu. 2021. An international comparative study of students’ scientific explanation based on cognitive diagnostic assessment. Frontiers in Psychology 12: 795497. [Google Scholar] [CrossRef] [PubMed]
- Hubbard, Edward M., Manuela Piazza, Philippe Pinel, and Stanislas Dehaene. 2005. Interactions between number and space in parietal cortex. Nature Reviews Neuroscience 6: 435–48. [Google Scholar] [CrossRef] [PubMed]
- Jirout, Jamie, and Nora S. Newcombe. 2018. How much as compared to what: Relative magnitude as a key idea in mathematics cognition. In Visualizing Mathematics: The Role of Spatial Reasoning in Mathematical Thought. Edited by Kelly S. Mix and Michael T. Battista. Berlin: Springer, pp. 3–24. [Google Scholar]
- Jordan, Nancy C., Janellen Huttenlocher, and Susan Cohen Levine. 1994. Assessing early arithmetic abilities: Effects of verbal and nonverbal response types on the calculation performance of middle-and low-income children. Learning and Individual Differences 6: 413–32. [Google Scholar] [CrossRef]
- Kline, Rex B. 2016. Principles and Practice of Structural Equation Modeling, 4th ed. New York: The Guilford Press. [Google Scholar]
- Kozhevnikov, Maria, and Mary Hegarty. 2001. A dissociation between object manipulation spatial ability and spatial orientation ability. Memory & Cognition 29: 745–56. [Google Scholar] [CrossRef]
- Lauer, Jillian E., and Stella F. Lourenco. 2016. Spatial processing in infancy predicts both spatial and mathematical aptitude in childhood. Psychological Science 27: 1291–98. [Google Scholar] [CrossRef]
- Linn, Marcia C., and Anne C. Petersen. 1985. Emergence and characterization of sex differences in spatial ability: A meta-analysis. Child Development 56: 1479–98. [Google Scholar] [CrossRef] [PubMed]
- Logan, Tracy. 2015. The influence of test mode and visuospatial ability on mathematics assessment performance. Mathematics Education Research Journal 27: 423–41. [Google Scholar] [CrossRef]
- Logan, Tracy, and Tom Lowrie. 2017. Gender perspectives on spatial tasks in a national assessment: A secondary data analysis. Research in Mathematics Education 19: 199–216. [Google Scholar] [CrossRef]
- Lourenco, Stella F., Chi-Ngai Cheung, and Lauren S. Aulet. 2018. Is visuospatial reasoning related to early mathematical development? A critical review. In Heterogeneity of Function in Numerical Cognition. Edited by Avishai Henik and Wim Fias. London: Academic, pp. 177–210. [Google Scholar]
- Lowrie, Tom, and Carmel M. Diezmann. 2007. Solving graphics problems: Student performance in the junior grades. Journal of Educational Research 100: 369–77. [Google Scholar] [CrossRef]
- Lowrie, Tom, and Tracy Logan. 2018. The interaction between spatial reasoning constructs and mathematics understandings in elementary classrooms. In Visualizing Mathematics: The Role of Spatial Reasoning in Mathematical Thought. Edited by Kelly S. Mix and Michael T. Battista. Berlin: Springer, pp. 253–76. [Google Scholar]
- Lowrie, Tom, Danielle Harris, Tracy Logan, and Mary Hegarty. 2021. The impact of a spatial intervention program on students’ spatial reasoning and mathematics performance. The Journal of Experimental Education 89: 259–77. [Google Scholar] [CrossRef]
- Lowrie, Tom, Tracy Logan, and Ajay Ramful. 2016. Cross cultural comparison of grade 6 students’ performance and strategy use on graphic and non-graphic tasks. Learning and Individual Differences 52: 97–108. [Google Scholar] [CrossRef]
- Lowrie, Tom, Tracy Logan, and Ajay Ramful. 2017. Visuospatial training improves elementary students’ mathematics performance. British Journal of Educational Psychology 87: 170–86. [Google Scholar] [CrossRef]
- Lowrie, Tom, Tracy Logan, and Mary Hegarty. 2019. The influence of spatial visualization training on students’ spatial reasoning and mathematics performance. Journal of Cognition and Development 20: 729–51. [Google Scholar] [CrossRef]
- Lowrie, Tom, Tracy Logan, Danielle Harris, and Mary Hegarty. 2018. The impact of an intervention program on students’ spatial reasoning: Student engagement through mathematics-enhanced learning activities. Cognitive Research: Principles and Implications 3: 50. [Google Scholar] [CrossRef]
- Lubinski, David. 2010. Spatial ability and STEM: A sleeping giant for talent identification and development. Personality and Individual Differences 49: 344–51. [Google Scholar] [CrossRef]
- McGee, Mark G. 1979. Human spatial abilities: Psychometric studies and environmental, genetic, hormonal, and neurological influences. Psychological Bulletin 86: 889–918. [Google Scholar] [CrossRef] [PubMed]
- Mix, Kelly S. 2019. Why are spatial skill and mathematics related? Child Development Perspectives 13: 121–26. [Google Scholar] [CrossRef]
- Mix, Kelly S., and Yi-Ling Cheng. 2012. The relation between space and math: Developmental and educational implications. Advances in Child Development and Behavior 42: 197–243. [Google Scholar] [CrossRef]
- Mix, Kelly S., Susan C. Levine, Yi-Ling Cheng, Christopher J. Young, David Z. Hambrick, and Spyros Konstantopoulos. 2017. The latent structure of spatial skills and mathematics: A replication of the two-factor model. Journal of Cognition and Development 18: 465–92. [Google Scholar] [CrossRef]
- Mix, Kelly S., Susan C. Levine, Yi-Ling Cheng, Chris Young, D. Zachary Hambrick, Raedy Ping, and Spyros Konstantopoulos. 2016. Separate but correlated: The latent structure of space and mathematics across development. Journal of Experimental Psychology: General 145: 1206–27. [Google Scholar] [CrossRef]
- Mulligan, Joanne. 2015. Looking within and beyond the geometry curriculum: Connecting spatial reasoning to mathematics learning. ZDM Mathematics Education 47: 511–17. [Google Scholar] [CrossRef]
- Newcombe, Nora S., Wenke Möhring, and Andrea Frick. 2018. How big is many? Development of spatial and numerical magnitude understanding. In Heterogeneity of Function in Numerical Cognition. Edited by Avishai Henik and Wim Fias. London: Academic, pp. 157–76. [Google Scholar]
- OECD. 2014. PISA 2012 Results: What Students Know and Can Do (Volume I, Revised edition, February 2014): Student Performance in Mathematics, Reading and Science. Paris: PISA, OECD Publishing. [Google Scholar] [CrossRef]
- OECD. 2016. PISA 2015 Results (Volume I): Excellence and Equity in Education. Paris: PISA, OECD Publishing. [Google Scholar] [CrossRef]
- OECD. 2019. PISA 2018 Results (Volume I): What Students Know and Can Do. Paris: PISA, OECD Publishing. [Google Scholar] [CrossRef]
- Owens, Kay. 2001. Development of the Test: Thinking About 3D Shapes. Sydney: NSW Department of Education and Training. [Google Scholar]
- Patahuddin, Sitti, Tracy Logan, and Ajay Ramful. 2018. Characteristics of spatial visualisation: Perspectives from area of composite shapes. In Making Waves, Opening Spaces, Proceedings of the 41st Annual Conference of the Mathematics Education Research Group of Australasia. Edited by J. Hunter, P. Perger and L. Darragh. Auckland: MERGA, pp. 623–30. [Google Scholar]
- Ramful, Ajay, Thomas Lowrie, and Tracy Logan. 2017. Measurement of spatial ability: Construction and validation of the spatial reasoning instrument for middle school students. Journal of Psychoeducational Assessment 35: 709–27. [Google Scholar] [CrossRef]
- Shepard, Roger N., and Jacqueline Metzler. 1971. Mental Rotation of Three-Dimensional Objects. Science 171: 701–3. [Google Scholar] [CrossRef] [PubMed]
- Sinclair, Nathalie. 2004. The Roles of the Aesthetic in Mathematical Inquiry. Mathematical Thinking and Learning 6: 261–84. [Google Scholar] [CrossRef]
- Sorby, Sheryl A. 1999. Developing 3-D spatial visualization skills. Engineering Design Graphics Journal 63: 21–32. [Google Scholar]
- Stankov, Lazar. 2010. Unforgiving Confucian culture: A breeding ground for high academic achievement, test anxiety and self-doubt? Learning and Individual Differences 20: 555–63. [Google Scholar] [CrossRef]
- Tartre, Lindsay Anne. 1990. Spatial skills, gender & mathematics. In Mathematics and Gender: Influences on Teachers and Students. Edited by E. Fennema and G. Leder. New York: Teachers’ College Press, pp. 27–59. [Google Scholar]
- Uttal, David H., David I. Miller, and Nora S. Newcombe. 2013. Exploring and enhancing spatial thinking: Links to achievement in science, technology, engineering, and mathematics? Current Directions in Psychological Science: A Journal of the American Psychological Society 22: 367–73. [Google Scholar] [CrossRef]
- Uttal, David H., Nina Simms Kiley McKee, Mary Hegarty, and Nora S. Newcombe. 2024. How Can We Best Assess Spatial Skills? Practical and Conceptual Challenges. Journal of Intelligence 12: 8. [Google Scholar] [CrossRef]
- Verdine, Brian N., Roberta Michnick Golinkoff, Kathy Hirsh-Pasek, Nora S. Newcombe, and Drew H. Bailey. 2017. Links between spatial and mathematical skills across the preschool years. Monographs of the Society for Research in Child Development 82: 7–30. [Google Scholar] [CrossRef]
- Verdine, Brian N., Roberta M. Golinkoff, Kathryn Hirsh-Pasek, Nora S. Newcombe, Andrew T. Filipowicz, and Alicia Chang. 2014. Deconstructing building blocks: Preschoolers’ spatial assembly performance relates to early mathematical skills. Child Development 85: 1062–76. [Google Scholar] [CrossRef]
- Voyer, Daniel, Susan Voyer, and M. Philip Bryden. 1995. Magnitude of sex differences in spatial abilities: A meta-analysis and consideration of critical variables. Psychological Bulletin 117: 250–70. [Google Scholar] [CrossRef] [PubMed]
- Wai, Jonathan, David Lubinski, and Camilla P. Benbow. 2009. Spatial ability for STEM domains: Aligning over 50 years of cumulative psychological knowledge solidifies its importance. Journal of Educational Psychology 101: 817–35. [Google Scholar] [CrossRef]
- Walsh, Vincent. 2003. A theory of magnitude: Common cortical metrics of time, space and quantity. Trends in Cognitive Sciences 7: 483–88. [Google Scholar] [CrossRef] [PubMed]
- Xie, Fang, Li Zhang, Xu Chen, and Ziqiang Xin. 2020. Is spatial ability related to mathematical ability: A meta-analysis. Educational Psychology Review 32: 113–55. [Google Scholar] [CrossRef]
- Xu, Tianshu, Xiaopeng Wu, Siyu Sun, and Qiping Kong. 2023. Cognitive diagnostic analysis of students’ mathematical competency based on the DINA model. Psychology in the Schools 60: 3135–50. [Google Scholar] [CrossRef]
| Construct | Subject | Content | Item |
|---|---|---|---|
| Mental Rotation (MR) | 2D rotation | Determining the outcome of a rotation of a 2D object; differentiating between reflection and rotation, clockwise and anticlockwise turn | MR2, MR4, MR5, MR7, MR8, MR11 |
| 3D rotation | Determining the outcome of a rotation of a 3D object; differentiating between reflection and rotation | MR20, MR26, MR29 | |
| Spatial Orientation (SO) | Orientation and location | Determining the position of an object in the situation; determining the position of the object relative to that of another object or the observer | SO1, SO3, SO6, SO12, SO17 |
| Alternate views | Front, top, or side view; identifying the orthogonal views of an object | SO9, SO15 | |
| Navigating with maps | Moving and reorienting in a forward or inverted map according to the given route | SO13, SO24, SO30 | |
| Spatial Visualization (SV) | Part–whole relationships | Identifying parts from the whole and vice versa | SV14, SV18 |
| Reflection and symmetry | Finding the symmetry in an object; reflecting an object | SV3, SV4, SV5 | |
| Folding and cutting | Visualizing the outcome of folding/unfolding/cutting a particular configuration; identifying cross sections of 3D objects | SV25, SV28, SV32 | |
| Transformation between 2D and 3D | Constructing a 3D shape from a given 2D shape and vice versa | SV21, SV22, SV23, SV27, SV31 |
| Construct | Subject | Item |
|---|---|---|
| Mental Rotation (MR) | 3D mental rotation | 26. The diagram below represents a model made out of cubes. Which of the following is the same as the model?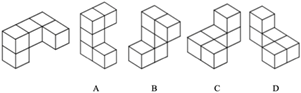 |
| Spatial Orientation (SO) | Navigating with maps | 24. A hamster was placed at the start of a maze, as shown below. The hamster ran through the maze. It turned to its right, then turned left, then turned right. Where did the hamster finish?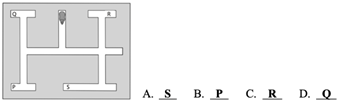 |
| Spatial Visualization (SV) | Transformation between 2D and 3D | 21. Diagram 1 represents a rectangular piece of paper. Which of the following hollow 3D shapes cannot be obtained by folding this rectangular paper? |
| Item ID | Content Domain | Item |
|---|---|---|
| M051091 | Number | Which fraction is not equal to the others? |
| M051123 | Geometric Shapes and Measures | How many lines of symmetry does this figure have?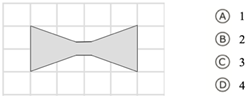 |
| M051109 | Data Display | How many children chose vanilla as their favorite flavor? |
| χ2 | df | χ2/df | CFI | TLI | RMSEA | |
|---|---|---|---|---|---|---|
| Second-order three-factor model | 653.664 | 461 | 1.418 | 0.918 | 0.912 | 0.030 |
| Mental Rotation | Spatial Orientation | Spatial Visualization | ||||||
|---|---|---|---|---|---|---|---|---|
| Item | p | D | Item | p | D | Item | p | D |
| 2 | 0.74 | 0.41 | 1 | 0.88 | 0.36 | 10 | 0.30 | 0.36 |
| 4 | 0.81 | 0.35 | 3 | 0.88 | 0.38 | 14 | 0.78 | 0.35 |
| 5 | 0.68 | 0.37 | 6 | 0.92 | 0.31 | 16 | 0.49 | 0.37 |
| 7 | 0.49 | 0.44 | 9 | 0.82 | 0.35 | 18 | 0.85 | 0.39 |
| 8 | 0.56 | 0.45 | 12 | 0.87 | 0.37 | 19 | 0.64 | 0.36 |
| 11 | 0.54 | 0.40 | 13 | 0.82 | 0.36 | 21 | 0.56 | 0.41 |
| 20 | 0.51 | 0.40 | 15 | 0.87 | 0.41 | 22 | 0.59 | 0.35 |
| 26 | 0.54 | 0.47 | 17 | 0.64 | 0.41 | 23 | 0.27 | 0.35 |
| 29 | 0.46 | 0.41 | 24 | 0.64 | 0.41 | 25 | 0.39 | 0.35 |
| 30 | 0.46 | 0.29 | 27 | 0.37 | 0.52 | |||
| 28 | 0.38 | 0.36 | ||||||
| 31 | 0.66 | 0.35 | ||||||
| 32 | 0.72 | 0.37 | ||||||
| Item | Difficulty Estimate | SE | Outfit MNSQ | Outfit t | Infit MNSQ | Infit t |
|---|---|---|---|---|---|---|
| 1 | −2.413 | 0.150 | 0.662 | −1.451 | 0.887 | −1.224 |
| 2 | −1.364 | 0.117 | 0.831 | −1.209 | 0.950 | −0.905 |
| 3 | −2.391 | 0.149 | 0.648 | −1.542 | 0.857 | −1.593 |
| 4 | −1.840 | 0.128 | 0.828 | −0.926 | 0.962 | −0.533 |
| 5 | −0.978 | 0.110 | 1.072 | 0.645 | 1.011 | 0.248 |
| 6 | −2.989 | 0.182 | 0.928 | −0.105 | 0.866 | −1.044 |
| 7 | 0.001 | 0.105 | 0.980 | −0.230 | 0.976 | −0.532 |
| 8 | −0.342 | 0.105 | 0.927 | −0.848 | 0.954 | −1.075 |
| 9 | −1.856 | 0.129 | 0.862 | −0.714 | 0.960 | −0.561 |
| 10 | 1.049 | 0.114 | 1.124 | 1.206 | 1.028 | 0.516 |
| 11 | −0.224 | 0.105 | 1.012 | 0.177 | 1.009 | 0.214 |
| 12 | −2.369 | 0.148 | 0.804 | −0.776 | 0.843 | −1.794 |
| 13 | −1.856 | 0.129 | 0.894 | −0.528 | 0.945 | −0.789 |
| 14 | −1.612 | 0.122 | 0.958 | −0.204 | 0.973 | −0.425 |
| 15 | −2.287 | 0.145 | 0.653 | −1.611 | 0.843 | −1.881 |
| 16 | 0.022 | 0.105 | 1.066 | 0.854 | 1.066 | 1.504 |
| 17 | −0.748 | 0.108 | 1.028 | 0.301 | 0.991 | −0.188 |
| 18 | −2.150 | 0.139 | 0.689 | −1.528 | 0.891 | −1.376 |
| 19 | −0.759 | 0.108 | 1.004 | 0.070 | 1.055 | 1.196 |
| 20 | −0.106 | 0.105 | 1.004 | 0.075 | 1.022 | 0.523 |
| 21 | −0.331 | 0.105 | 0.994 | −0.047 | 1.014 | 0.347 |
| 22 | −0.504 | 0.106 | 1.077 | 0.847 | 1.072 | 1.629 |
| 23 | 1.259 | 0.118 | 1.098 | 0.881 | 1.042 | 0.707 |
| 24 | −0.782 | 0.108 | 0.984 | −0.119 | 0.994 | −0.123 |
| 25 | 0.537 | 0.108 | 1.133 | 1.580 | 1.085 | 1.747 |
| 26 | −0.256 | 0.105 | 0.899 | −1.226 | 0.939 | −1.418 |
| 27 | 0.628 | 0.109 | 0.785 | −2.719 | 0.864 | −2.907 |
| 28 | 0.616 | 0.109 | 1.088 | 1.044 | 1.069 | 1.410 |
| 29 | 0.184 | 0.106 | 0.972 | −0.332 | 1.003 | 0.083 |
| 30 | 0.152 | 0.106 | 1.211 | 2.567 | 1.192 | 4.098 |
| 31 | −0.850 | 0.109 | 1.034 | 0.342 | 1.065 | 1.372 |
| 32 | −1.197 | 0.114 | 0.901 | −0.740 | 1.011 | 0.238 |
| Mean | SD | Min | Max | Skewness | Kurtosis | SE | |
|---|---|---|---|---|---|---|---|
| Spatial measures | |||||||
| Overall spatial score | 19.09 | 5.17 | 6 | 31 | −0.02 | −0.58 | 0.18 |
| Mental rotation | 5.11 | 2.28 | 0 | 9 | −0.1 | −0.8 | 0.08 |
| Spatial orientation | 7.38 | 1.8 | 1 | 10 | −0.59 | −0.08 | 0.06 |
| Spatial visualization | 6.6 | 2.3 | 1 | 13 | 0.01 | −0.4 | 0.08 |
| Mathematics measures | |||||||
| Overall mathematics score | 29.56 | 5.88 | 6 | 38 | −1.09 | 1.13 | 0.21 |
| Number | 15.7 | 3.48 | 1 | 20 | −1.06 | 1.11 | 0.12 |
| Geometric shapes and measures | 10 | 2.52 | 2 | 14 | −0.75 | 0.14 | 0.09 |
| Data display | 3.46 | 0.79 | 0 | 4 | −1.53 | 2.19 | 0.03 |
| Male (N = 432) | Female (N = 384) | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Mean | SD | Range | Skewness | Kurtosis | Mean | SD | Range | Skewness | Kurtosis | |
| Spatial measures | ||||||||||
| Overall spatial score | 19.53 | 5.35 | 6–31 | −0.08 | −0.66 | 18.59 | 4.93 | 6–31 | 0.01 | −0.5 |
| Mental rotation | 5.45 | 2.25 | 0–9 | −0.27 | −0.67 | 4.72 | 2.25 | 0–9 | 0.08 | −0.81 |
| Spatial orientation | 7.46 | 1.82 | 1–10 | −0.6 | −0.1 | 7.28 | 1.78 | 1–10 | −0.58 | −0.07 |
| Spatial visualization | 6.61 | 2.48 | 1–13 | 0 | −0.55 | 6.59 | 2.09 | 2–12 | 0.04 | −0.31 |
| Mathematics measures | ||||||||||
| Overall mathematics score | 29.81 | 5.95 | 7–38 | −1.09 | 1.03 | 29.29 | 5.81 | 6–38 | −1.1 | 1.25 |
| Number | 15.99 | 3.42 | 2–20 | −1.12 | 1.25 | 15.38 | 3.51 | 1–20 | −1 | 1 |
| Geometric shapes and measures | 10.43 | 2.51 | 2–14 | −0.75 | 0.16 | 10.37 | 2.52 | 2–14 | −0.75 | 0.1 |
| Data display | 3.39 | 0.84 | 0–4 | −1.47 | 2.07 | 3.54 | 0.73 | 1–4 | −1.54 | 1.82 |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | |
|---|---|---|---|---|---|---|---|---|
| Spatial measures | ||||||||
| 1. Overall spatial score | - | |||||||
| 2. Mental rotation | 0.82 *** | - | ||||||
| 3. Spatial orientation | 0.76 *** | 0.44 *** | - | |||||
| 4. Spatial visualization | 0.84 *** | 0.51 *** | 0.48 *** | - | ||||
| Mathematics measures | ||||||||
| 5. Overall mathematics score | 0.58 *** | 0.48 *** | 0.44 *** | 0.49 *** | - | |||
| 6. Number | 0.52 *** | 0.43 *** | 0.41 *** | 0.42 *** | 0.93 *** | - | ||
| 7. Geometric shapes and measures | 0.55 *** | 0.48 *** | 0.39 *** | 0.47 *** | 0.86 *** | 0.62 *** | - | |
| 8. Data display | 0.29 *** | 0.18 *** | 0.25 *** | 0.29 *** | 0.63 *** | 0.52 *** | 0.44 *** | - |
| Model | F | Adj. R2 | β | SE | t | |
|---|---|---|---|---|---|---|
| Overall Sample Regression (N = 816) | ||||||
| Step 1: Simple Linear Regression | ||||||
| Overall spatial score | 421.5 *** | 0.34 | 0.66 | 0.03 | 20.53 *** | |
| Step 2: Multiple Regression | ||||||
| 140.2 *** | 0.34 | |||||
| Mental rotation | 0.69 | 0.09 | 7.74 *** | |||
| Spatial orientation | 0.67 | 0.11 | 6.10 *** | |||
| Spatial visualization | 0.64 | 0.09 | 7.09 *** | |||
| Subsample Regression: Male (N = 432) Female (N = 384) | ||||||
| Step 3: Simple Linear Regression | ||||||
| Male | Overall spatial score | 240.8 *** | 0.36 | 0.67 | 0.04 | 15.52 *** |
| Female | Overall spatial score | 177.6 *** | 0.32 | 0.66 | 0.05 | 13.33 *** |
| Step 4: Multiple Regression | ||||||
| Male | 80.89 *** | 0.36 | ||||
| Mental rotation | 0.59 | 0.12 | 4.77 *** | |||
| Spatial orientation | 0.86 | 0.15 | 5.75 *** | |||
| Spatial visualization | 0.60 | 0.12 | 5.11 *** | |||
| Female | 60.69 *** | 0.32 | ||||
| Mental rotation | 0.81 | 0.13 | 6.17 *** | |||
| Spatial orientation | 0.45 | 0.16 | 2.78 ** | |||
| Spatial visualization | 0.68 | 0.14 | 4.77 *** | |||
| Model | F | Adj. R2 | β | SE | t | |
|---|---|---|---|---|---|---|
| Overall Sample Regression (N = 816) | ||||||
| Step 1: Simple Linear Regression | ||||||
| Overall spatial score | 301.7 *** | 0.27 | 0.35 | 0.02 | 17.37 *** | |
| Step 2: Multiple Regression | ||||||
| 101 *** | 0.27 | |||||
| Mental rotation | 0.37 | 0.06 | 6.68 *** | |||
| Spatial orientation | 0.41 | 0.07 | 5.98 *** | |||
| Spatial visualization | 0.29 | 0.06 | 5.19 *** | |||
| Subsample Regression: Male (N = 432) Female (N = 384) | ||||||
| Step 3: Simple Linear Regression | ||||||
| Male | Overall spatial score | 170.1 *** | 0.28 | 0.34 | 0.03 | 13.04 *** |
| Female | Overall spatial score | 126.3 *** | 0.25 | 0.36 | 0.03 | 11.24 *** |
| Step 4: Multiple Regression | ||||||
| Male | 57.6 *** | 0.28 | ||||
| Mental rotation | 0.27 | 0.07 | 3.55 *** | |||
| Spatial orientation | 0.47 | 0.09 | 5.55 *** | |||
| Spatial visualization | 0.32 | 0.07 | 4.46 *** | |||
| Female | 42.65 *** | 0.25 | ||||
| Mental rotation | 0.45 | 0.08 | 5.45 *** | |||
| Spatial orientation | 0.33 | 0.10 | 3.24 ** | |||
| Spatial visualization | 0.27 | 0.09 | 2.98 ** | |||
| Model | F | Adj. R2 | β | SE | t | |
|---|---|---|---|---|---|---|
| Overall Sample Regression (N = 816) | ||||||
| Step 1: Simple Linear Regression | ||||||
| Overall spatial score | 361.4 *** | 0.31 | 0.27 | 0.01 | 19.01 *** | |
| Step 2: Multiple Regression | ||||||
| 121.5 *** | 0.31 | |||||
| Mental rotation | 0.32 | 0.04 | 8.17 *** | |||
| Spatial orientation | 0.20 | 0.05 | 4.22 *** | |||
| Spatial visualization | 0.27 | 0.04 | 6.87 *** | |||
| Subsample Regression: Male (N = 432) Female (N = 384) | ||||||
| Step 3: Simple Linear Regression | ||||||
| Male | Overall spatial score | 291.8 *** | 0.34 | 0.273 | 0.02 | 14.83 *** |
| Female | Overall spatial score | 145.7 *** | 0.27 | 0.269 | 0.02 | 12.07 *** |
| Step 4: Multiple Regression | ||||||
| Male | 57.6 *** | 0.34 | ||||
| Mental rotation | 0.313 | 0.05 | 5.88 *** | |||
| Spatial orientation | 0.308 | 0.06 | 4.78 *** | |||
| Spatial visualization | 0.215 | 0.05 | 4.26 *** | |||
| Female | 42.65 *** | 0.28 | ||||
| Mental rotation | 0.346 | 0.06 | 5.94 *** | |||
| Spatial orientation | 0.084 | 0.07 | 1.18 | |||
| Spatial visualization | 0.331 | 0.06 | 5.24 *** | |||
| Model | F | Adj. R2 | β | SE | t | |
|---|---|---|---|---|---|---|
| Overall Sample Regression (N = 816) | ||||||
| Step 1: Simple Linear Regression | ||||||
| Overall spatial score | 77.15 *** | 0.10 | 0.05 | 0.01 | 8.78 *** | |
| Step 2: Multiple Regression | ||||||
| 29.82 *** | 0.10 | |||||
| Mental rotation | 0.00 | 0.01 | 0.12 | |||
| Spatial orientation | 0.06 | 0.02 | 3.43 *** | |||
| Spatial visualization | 0.08 | 0.01 | 5.43 *** | |||
| Subsample Regression: Male (N = 432) Female (N = 384) | ||||||
| Step 3: Simple Linear Regression | ||||||
| Male | Overall spatial score | 53.51 *** | 0.11 | 0.05 | 0.01 | 7.32 *** |
| Female | Overall spatial score | 29.31 *** | 0.07 | 0.04 | 0.01 | 5.41 *** |
| Step 4: Multiple Regression | ||||||
| Male | 19.61 *** | 0.34 | ||||
| Mental rotation | 0.01 | 0.02 | 0.54 | |||
| Spatial orientation | 0.09 | 0.02 | 3.42 *** | |||
| Spatial visualization | 0.07 | 0.02 | 3.40 *** | |||
| Female | 11.31 *** | 0.29 | ||||
| Mental rotation | 0.01 | 0.02 | 0.49 | |||
| Spatial orientation | 0.03 | 0.02 | 1.33 | |||
| Spatial visualization | 0.08 | 0.02 | 3.78 *** | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, T.; Sun, S.; Kong, Q. Spatial Reasoning and Its Contribution to Mathematical Performance Across Different Content Domains: Evidence from Chinese Students. J. Intell. 2025, 13, 41. https://doi.org/10.3390/jintelligence13040041
Xu T, Sun S, Kong Q. Spatial Reasoning and Its Contribution to Mathematical Performance Across Different Content Domains: Evidence from Chinese Students. Journal of Intelligence. 2025; 13(4):41. https://doi.org/10.3390/jintelligence13040041
Chicago/Turabian StyleXu, Tianshu, Siyu Sun, and Qiping Kong. 2025. "Spatial Reasoning and Its Contribution to Mathematical Performance Across Different Content Domains: Evidence from Chinese Students" Journal of Intelligence 13, no. 4: 41. https://doi.org/10.3390/jintelligence13040041
APA StyleXu, T., Sun, S., & Kong, Q. (2025). Spatial Reasoning and Its Contribution to Mathematical Performance Across Different Content Domains: Evidence from Chinese Students. Journal of Intelligence, 13(4), 41. https://doi.org/10.3390/jintelligence13040041





