Bone Abrasive Machining: Influence of Tool Geometry and Cortical Bone Anisotropic Structure on Crack Propagation
Abstract
:1. Introduction
2. Materials and Methods
2.1. Bone Characteristics
2.2. Bone Specimens
2.3. Cutting Tools Geometry
2.4. Experimental Setup
3. Results
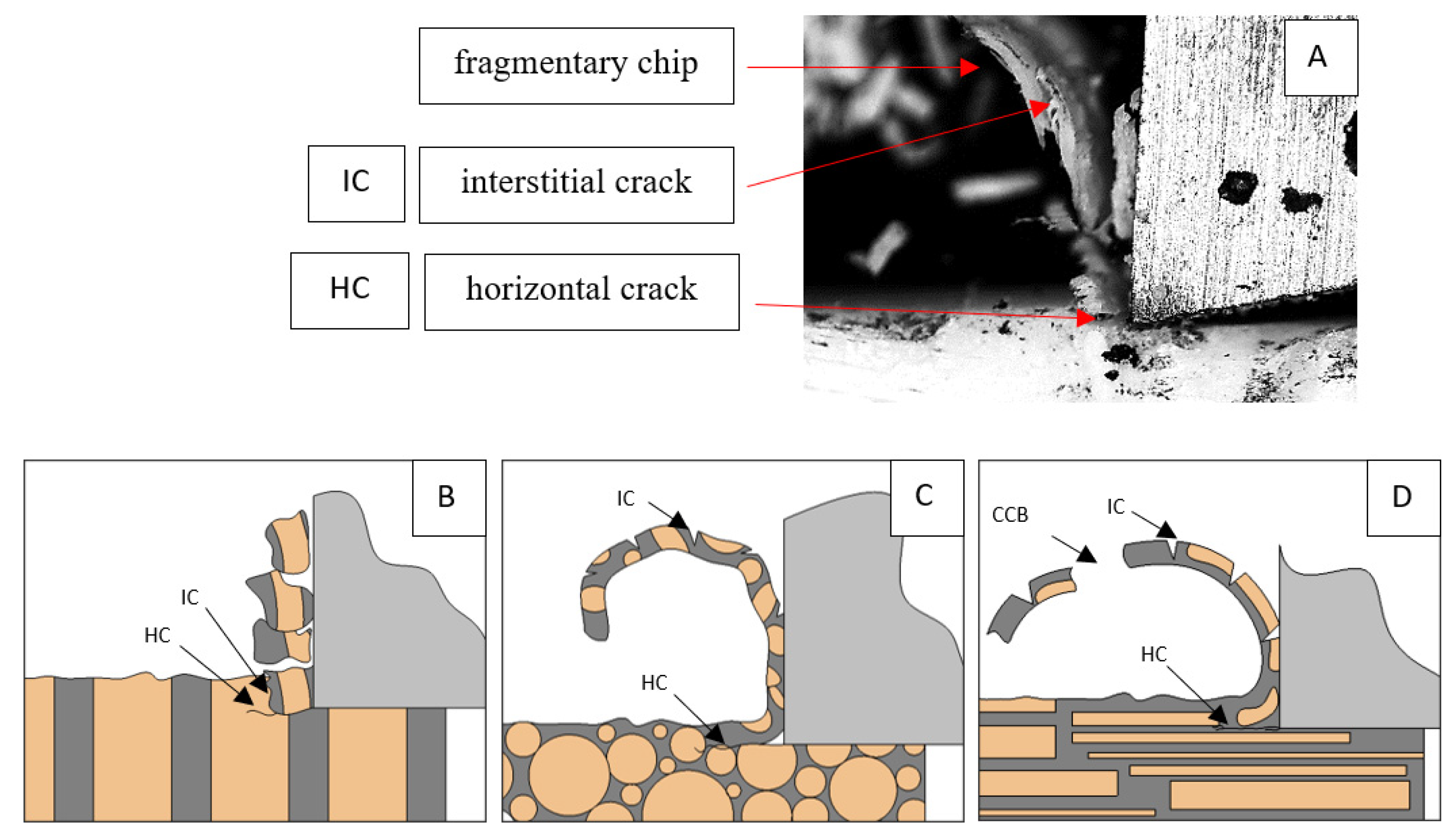
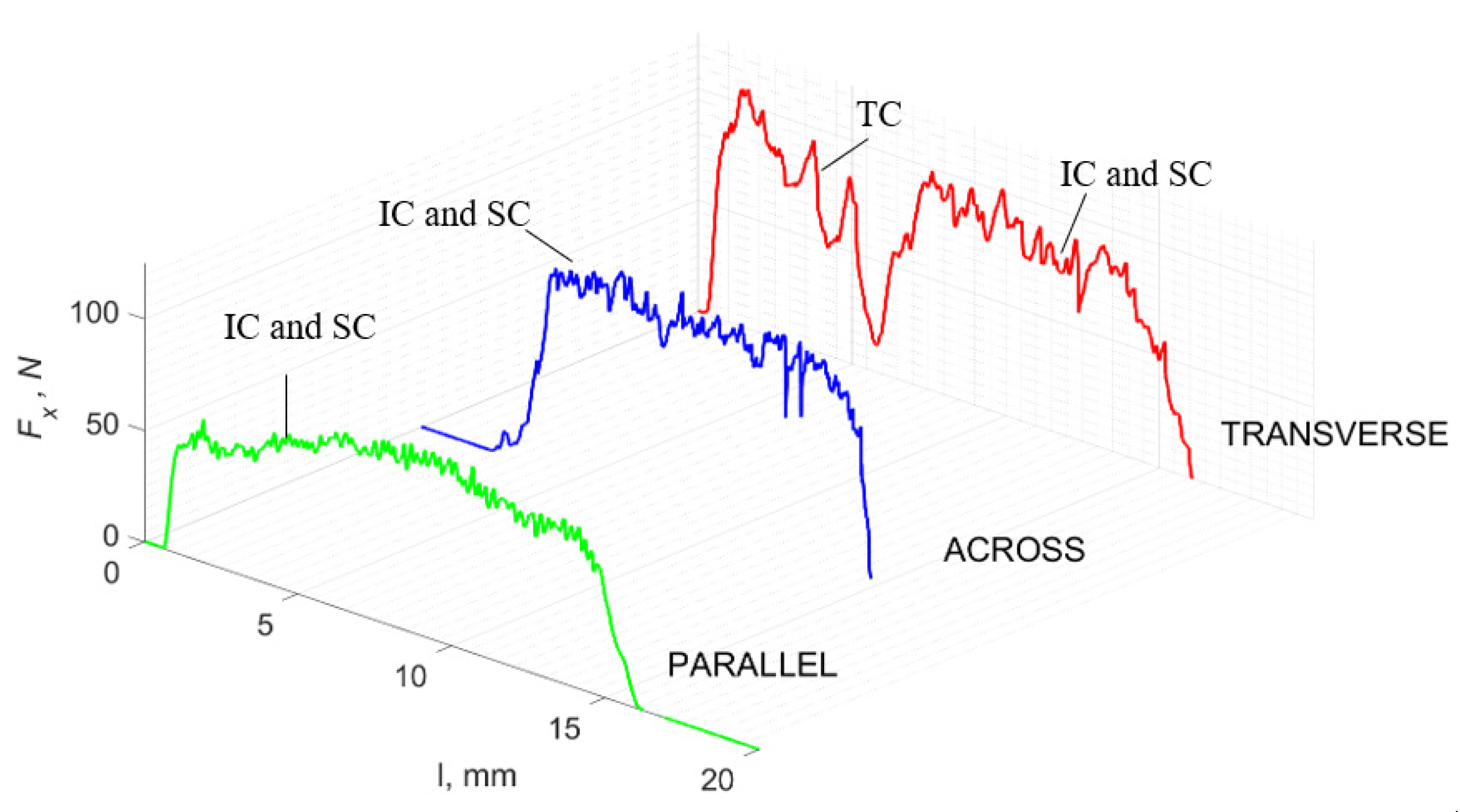
3.1. d = 175 μm
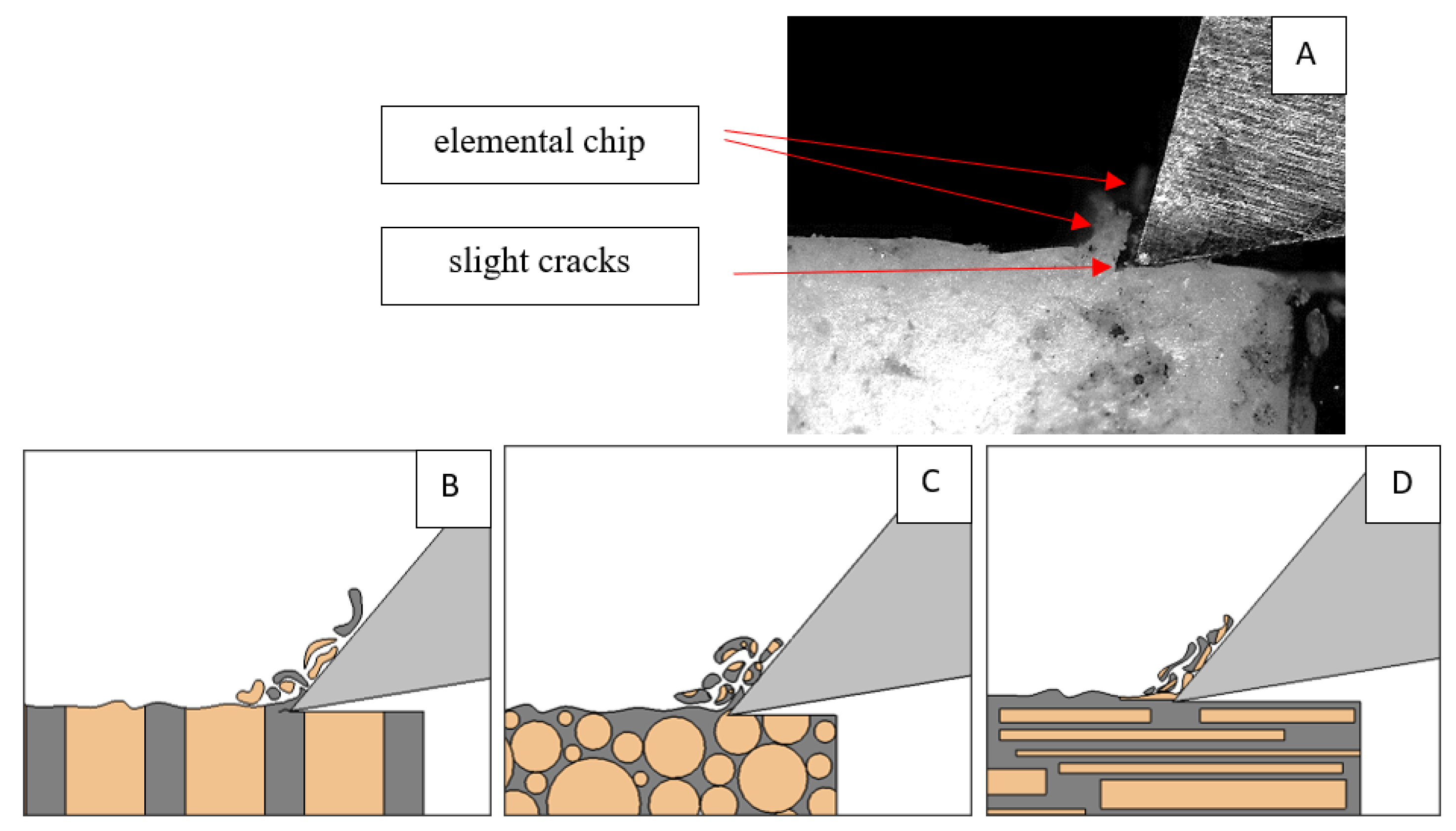
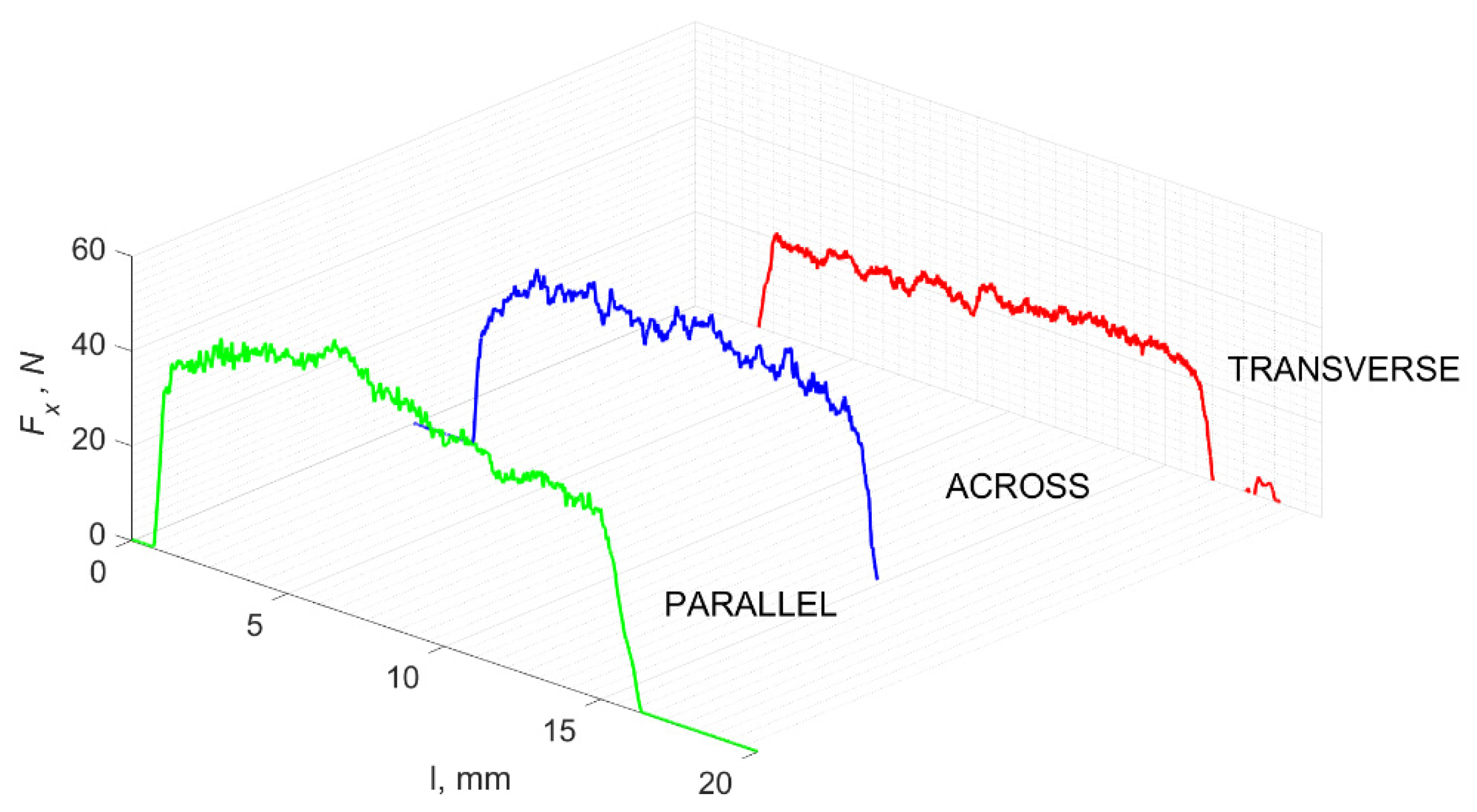
3.2. d = 50 μm
3.3. d = 5 μm
3.4. Surface Morphology
4. Discussion
5. Conclusions
- The most advantageous and predictable processes were obtained for positive rake angles and a depth cut below 100 µm.
- For negative rake angle values, depending on the depth of cut, the following were distinguished: no cutting (friction), brittle deformation and shear cracks. The following was obtained for positive rake angle values: slight penetrating, interstitial cracks with continuous chip formation and horizontal and transverse cracks. For the zero rake angle, shear cracks predominate.
- There are regular cracks along the cement line in the parallel cutting direction. In the across direction, the cracks also spread along the cement line, although they were shorter and less regular, and they strongly propagated deeper into the material. Shear fractures concerning the osteon predominate in the transverse cutting direction.
- Crack propagation occurs along the cement line for the across and parallel direction. In the case of the transverse direction, stresses accumulate in the osteons, followed by uncontrolled fracture.
- The morphological parameters of the surface maintain a clear correlation with the cutting direction. Cutting in the parallel direction provides a surface with the lowest volume damage and roughness.
- The clearance angles do not affect the crack propagation character.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Buis, N.; Esfandiari, H.; Hoch, A.; Fürnstahl, P. Overview of Methods to Quantify Invasiveness of Surgical Approaches in Orthopedic Surgery—A Scoping Review. Front. Surg. 2021, 8, 771275. [Google Scholar] [CrossRef] [PubMed]
- Rubin, L.E.; Murgo, K.T.; Ritterman, S.A.; McClure, P.K. Hip Resection Arthroplasty. JBJS Rev. 2014, 2, e3. [Google Scholar] [CrossRef] [PubMed]
- Lubowitz, J.H.; Appleby, D. Cost-Effectiveness Analysis of the Most Common Orthopaedic Surgery Procedures: Knee Arthroscopy and Knee Anterior Cruciate Ligament Reconstruction. Arthrosc. J. Arthrosc. Relat. Surg. 2011, 27, 1317–1322. [Google Scholar] [CrossRef] [PubMed]
- Dahotre, N.B.; Joshi, S.S. Machining of Bone and Hard Tissue; Springer: Denton, TX, USA, 2016. [Google Scholar]
- Gwenllian, T.; Rowe, P.J.; Riches, P.E. Thermal Damage Done to Bone by Burring and Sawing With and Without Irrigation in Knee Arthroplasty. J. Arthroplast. 2015, 31, 1102–1108. [Google Scholar]
- Robles-Linares, J.A.; Axinte, D.; Liao, Z.; Gameros, A. Machining-induced thermal damage in cortical bone: Necrosis and micro-mechanical integrity. Mater. Des. 2021, 197, 109215. [Google Scholar] [CrossRef]
- Bonfield, W. Advances in the fracture mechanics of cortical bone. J. Biomech. 1987, 20, 1071–1081. [Google Scholar] [CrossRef]
- An, B.; Liu, Y.; Arola, D.; Zhang, D. Fracture toughening mechanism of cortical bone: An experimental and numerical approach. J. Mech. Behav. Biomed. Mater. 2011, 4, 983–992. [Google Scholar] [CrossRef]
- Gustafsson, A.; Khayyeri, H.; Wallin, M.; Isaksson, H. An interface damage model that captures crack propagation at the microscale in cortical bone using XFEM. J. Mech. Behav. Biomed. Mater. 2019, 90, 556–565. [Google Scholar] [CrossRef]
- Gustafsson, A.; Wallin, M.; Khayyeri, H.; Isaksson, H. Crack propagation in cortical bone is affected by the characteristics of the cement line: A parameter study using an XFEM interface damage model. Biomech. Model. Mechanobiol. 2019, 18, 1247–1261. [Google Scholar] [CrossRef]
- Liao, Z.; Axinte, D.A. On chip formation mechanism in orthogonal cutting of bone. Int. J. Mach. Tools Manuf. 2019, 102, 41–55. [Google Scholar] [CrossRef]
- Bai, W.; Shu, L.; Sun, R.; Xu, J.; Silberschmidt, V.V.; Sugita, N. Mechanism of material removal in orthogonal cutting of cortical bone. J. Mech. Behav. Biomed. Mater. 2020, 104, 103618. [Google Scholar] [CrossRef] [PubMed]
- Jacobs, C.; Pope, M.; Berry, J.; Hoaglund, F. A study of the bone machining process—Orthogonal cutting. J. Biomech. 1974, 7, 131–132. [Google Scholar] [CrossRef]
- Wiggins, K.L.; Malkin, S. Orthogonal Machining of Bone. J. Biomech. Eng. 1978, 100, 122–130. [Google Scholar] [CrossRef]
- Wu, Y.; Xing, Y.; Liu, B. Analysis of isotropic and composite laminated plates and shells using a differential quad-rature hierarchical finite element method. Compos. Struct. 2018, 205, 11–25. [Google Scholar] [CrossRef]
- Yan, Y.; Liu, B.; Xing, Y.; Carrera, E.; Pagani, A. Free vibration analysis of variable stiffness composite laminated beams and plates by novel hierarchical differential quadrature finite elements. Compos. Struct. 2021, 274, 114364. [Google Scholar] [CrossRef]
- Kabir, H.; Aghdam, M. A robust Bézier based solution for nonlinear vibration and post-buckling of random checkerboard graphene nano-platelets reinforced composite beams. Compos. Struct. 2019, 212, 184–198. [Google Scholar] [CrossRef]
- Hashemi, S.; Esmaeili, S.; Ghadirinejad, M.; Saber-Samandari, S.; Sheikhbahai, E.; Kordjamshidi, A.; Khandan, A. Micro-Finite Element Model to Investigate the Mechanical Stimuli in Scaffolds Fabricated via Space Holder Technique for Cancellous Bone. ADMT J. 2020, 13, 51–58. [Google Scholar]
- McNamara, L. Bone as a Material. In Comprehensive Biomaterials; Elsevier: Amsterdam, The Netherlands, 2011; pp. 169–186. [Google Scholar] [CrossRef]
- MacNeil, J.A.; Boyd, S.K. Load distribution and the predictive power of morphological indices in the distal radius and tibia by high resolution peripheral quantitative computed tomography. Bone 2007, 41, 129–137. [Google Scholar] [CrossRef]
- Spadaro, J.A.; Werner, F.W.; Brenner, R.A.; Fortino, M.D.; Fay, L.A.; Edwards, W.T. Cortical and trabecular bone contribute strength to the osteopenic distal radius. J. Orthop. Res. 1994, 12, 211–218. [Google Scholar] [CrossRef]
- Augat, P.; Reeb, H.; Claes, L.E. Prediction of fracture load at different skeletal sites by geometric properties of the cortical shell. J. Bone Miner. Res. 1996, 11, 1356–1363. [Google Scholar] [CrossRef]
- Burghardt, A.J.; Kazakia, G.J.; Ramachandran, S.; Link, T.M.; Majumdar, S. Age and Gender Related Differences in the Geometric Properties and Biomechanical Significance of Intra-Cortical Porosity in the Distal Radius and Tibia. J. Bone Miner. Res. 2010, 25, 983–993. [Google Scholar] [CrossRef] [PubMed]
- Augat, P.; Schorlemmer, S. The role of cortical bone and its microstructure in bone strength. Age Ageing 2006, 35 (Suppl. 2), ii27–ii31. [Google Scholar] [CrossRef] [PubMed]
- Currey, J. Incompatible mechanical properties in compact bone. J. Theor. Biol. 2004, 231, 569–580. [Google Scholar] [CrossRef] [PubMed]
- Turnbull, T.L.; Baumann, A.P.; Roeder, R.K. Fatigue microcracks that initiate fracture are located near elevated intracortical po-rosity but not elevated mineralization. J. Biomech. 2014, 47, 3135–3142. [Google Scholar] [CrossRef]
- James, E.N.; Hanna, C.; Nair, L.S. Nanobiomaterials for Tissue Engineering Applications. In Stem Cell Biology and Tissue Engineering in Dental Sciences; Academic Press: Cambridge, MA, USA, 2015; pp. 221–234. [Google Scholar] [CrossRef]
- Wen, D.; Androjna, C.; Vasanji, A.; Belovich, J.; Midura, R.J. Lipids and Collagen Matrix Restrict the Hydraulic Permeability Within the Porous Compartment of Adult Cortical Bone. Ann. Biomed. Eng. 2010, 38, 558–569. [Google Scholar] [CrossRef]
- Granke, M.; Does, M.D.; Nyman, J.S. The Role of Water Compartments in the Material Properties of Cortical Bone. Calcif. Tissue Res. 2015, 97, 292–307. [Google Scholar] [CrossRef]
- Ferreiro, O.; Yubero, F.; Balestra, R.M.; Varella, M.; Monteiro, M. Bovine Bone Processing for Biofilter Application. Mater. Sci. Forum 2012, 727–728, 727–730. [Google Scholar] [CrossRef]
- Gibson, V.; Stover, S.; Gibeling, J.; Hazelwood, S.; Martin, R. Osteonal effects on elastic modulus and fatigue life in equine bone. J. Biomech. 2006, 39, 217–225. [Google Scholar] [CrossRef]
- Dahl, A.; Thompson, M. Mechanobiology of Bone. In Comprehensive Biotechnology, 2nd ed.; Academic Press: Cambridge, MA, USA, 2011; pp. 217–236. [Google Scholar] [CrossRef]
- Skedros, J.G.; Clark, G.C.; Sorenson, S.M.; Taylor, K.W.; Qiu, S. Analysis of the Effect of Osteon Diameter on the Potential Relationship of Osteocyte Lacuna Density and Osteon Wall Thickness. Anat. Rec. 2011, 294, 1472–1485. [Google Scholar] [CrossRef] [Green Version]
- Gong, J.K.; Arnold, J.S.; Cohn, S.H. Composition of trabecular and cortical bone. Anat. Rec. 1964, 149, 325. [Google Scholar] [CrossRef]
- Hodgskinson, R.; Currey, J.D.; Evans, G.P. Hardness, an indicator of the mechanical competence of cancellous bone. J. Orthop. Res. 1989, 7, 754–758. [Google Scholar] [CrossRef] [PubMed]
- Cowin, S.C. Bone Mechanics; CRC Press: Boca Raton, FL, USA, 1988. [Google Scholar]
- Cowin, S.; Sadegh, A. Non-interacting modes for stress, strain and energy in anisotropic hard tissue. J. Biomech. 1991, 24, 859–867. [Google Scholar] [CrossRef]
- Abdel-Wahab, A.A.; Maligno, A.R.; Silberschmidt, V.V. Micro-scale modelling of bovine cortical bone fracture: Analysis of crack propagation and microstructure using X-FEM. Comput. Mater. Sci. 2012, 52, 128–135. [Google Scholar] [CrossRef]
- Sugita, N.; Shu, L.; Shimada, T.; Oshima, M.; Kizaki, T.; Mitsuishi, M. Novel surgical machining via an impact cutting method based on fracture analysis with a discontinuum bone model. CIRP Ann. 2017, 66, 65–68. [Google Scholar] [CrossRef]
- Morgan, E.F.; Unnikrisnan, G.U.; Hussein, A.I. Bone Mechanical Properties in Healthy and Diseased States. Annu. Rev. Biomed. Eng. 2018, 20, 119–143. [Google Scholar] [CrossRef]
- Chen, P.Y.; Stokes, A.G.; McKittrick, J. Comparison of the structure and mechanical properties of bovine femur bone and ant-ler of the North American elk (Cervus elaphus canadensis). Acta Biomater. 2008, 5, 693–706. [Google Scholar] [CrossRef]
- Lee, S.; Nagata, F.; Kato, K.; Nakano, T. Bone apatite anisotropic structure control via designing fibrous scaffolds. RSC Adv. 2020, 10, 13500–13506. [Google Scholar] [CrossRef]
- Kaye, B. The Effects of Freezing on the Mechanical Properties of Bone. Open Bone J. 2012, 4, 14–19. [Google Scholar] [CrossRef]
- Van Haaren, E.H.; van der Zwaard, B.C.; van der Veen, A.J.; Heyligers, I.C.; Wuisman, P.I.J.M.; Smit, T.H. Effect of long-term preservation on the mechanical properties of cortical bone in goats. Acta Orthop. 2008, 79, 708–716. [Google Scholar] [CrossRef]
- Brazda, I.J.; Reeves, J.; Langohr, G.D.G.; Crookshank, M.C.; Schemitsch, E.H.; Zdero, R. Biomechanical properties and thermal characteristics of frozen versus thawed whole bone. Proc. Inst. Mech. Eng. Part H J. Eng. Med. 2020, 234, 874–883. [Google Scholar] [CrossRef]
- Tutunea-Fatan, O.R.; A Fakhri, M.; Bordatchev, E.V. Porosity and cutting forces: From macroscale to microscale machining correlations. Proc. Inst. Mech. Eng. Part B J. Eng. Manuf. 2011, 225, 619–630. [Google Scholar] [CrossRef]
- Akbari, M.; Buhl, S.; Leinenbach, C.; Wgener, K. A new value for Johnson Cook damage limit criterion in machining with large negative rake angle as basis for understanding of grinding. J. Mater. Process. Technol. 2016, 234, 58–71. [Google Scholar] [CrossRef]
- Aurich, J.; Dornfeld, D.; Arrazola, P.; Franke, V.; Leitz, L.; Min, S. Burrs—Analysis, control and removal. CIRP Ann. 2009, 58, 519–542. [Google Scholar] [CrossRef]
- Fang, N. Tool-chip friction in machining with a large negative rake angle tool. Wear 2005, 258, 890–897. [Google Scholar] [CrossRef]
- Zawadzki, P. Proposal for a Novel Abrasive Machining Method for Preparing the Surface of Periarticular Tissue during Orthopedic Surgery on Hip Joints. J. Funct. Biomater. 2021, 12, 50. [Google Scholar] [CrossRef]
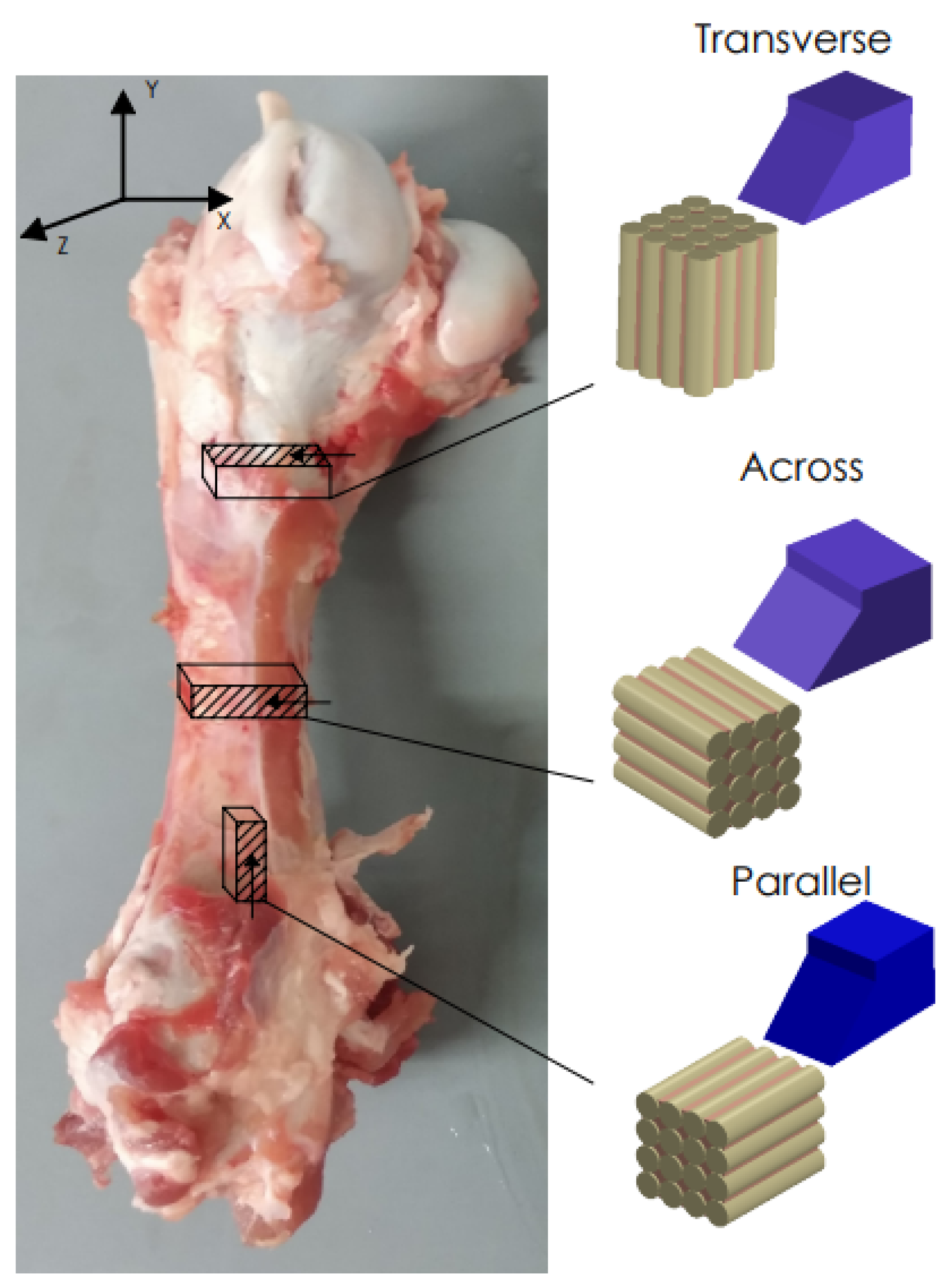




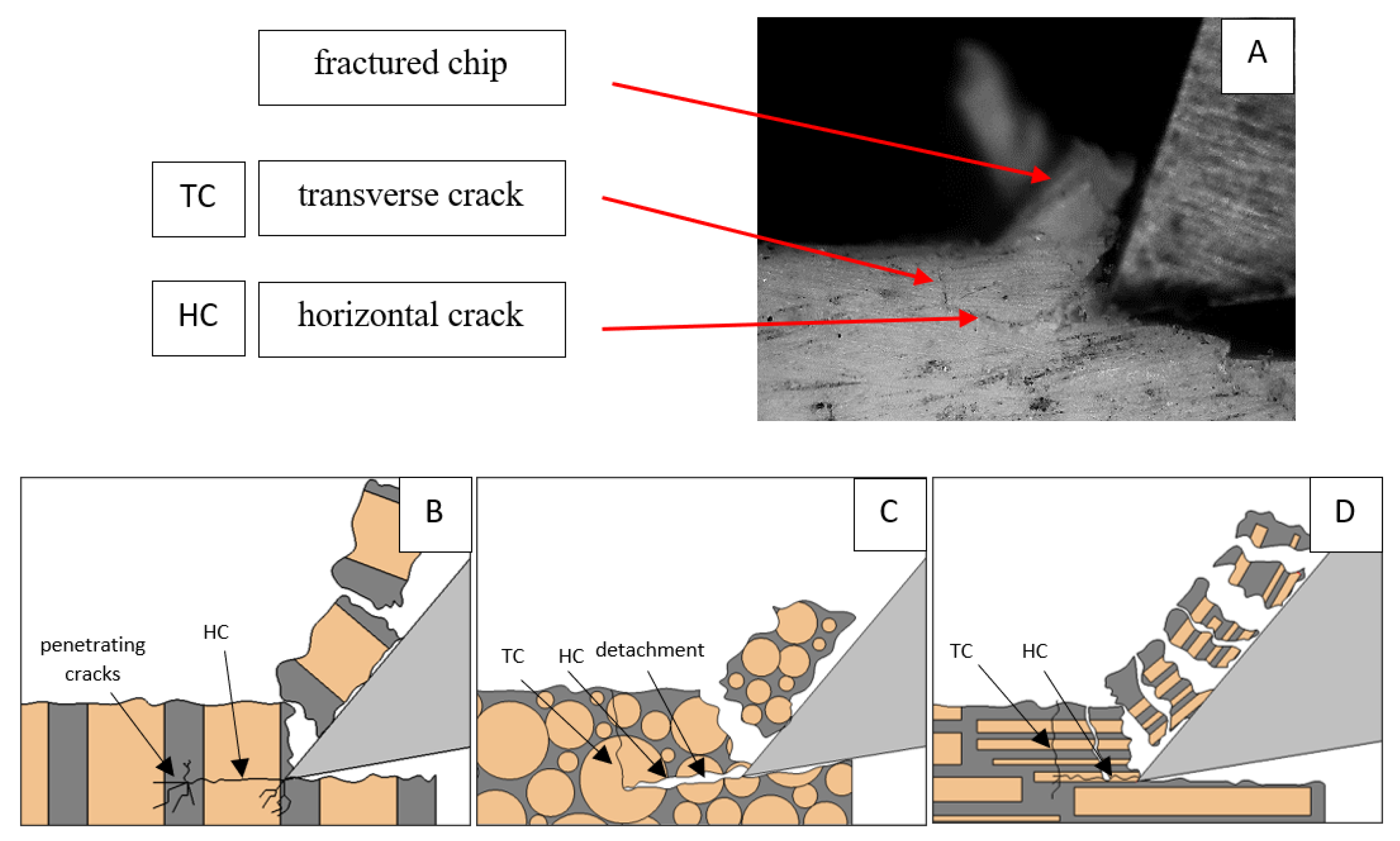
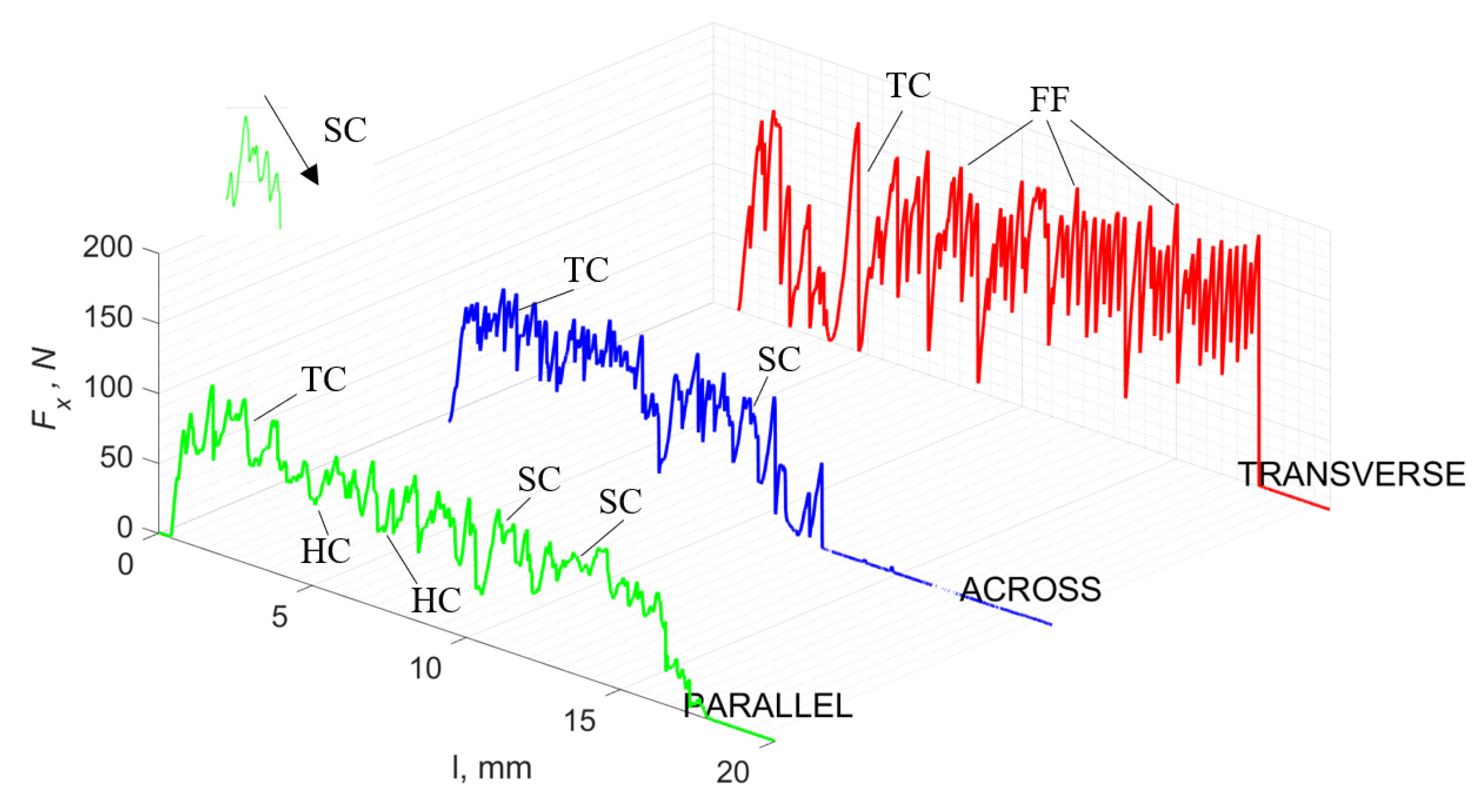





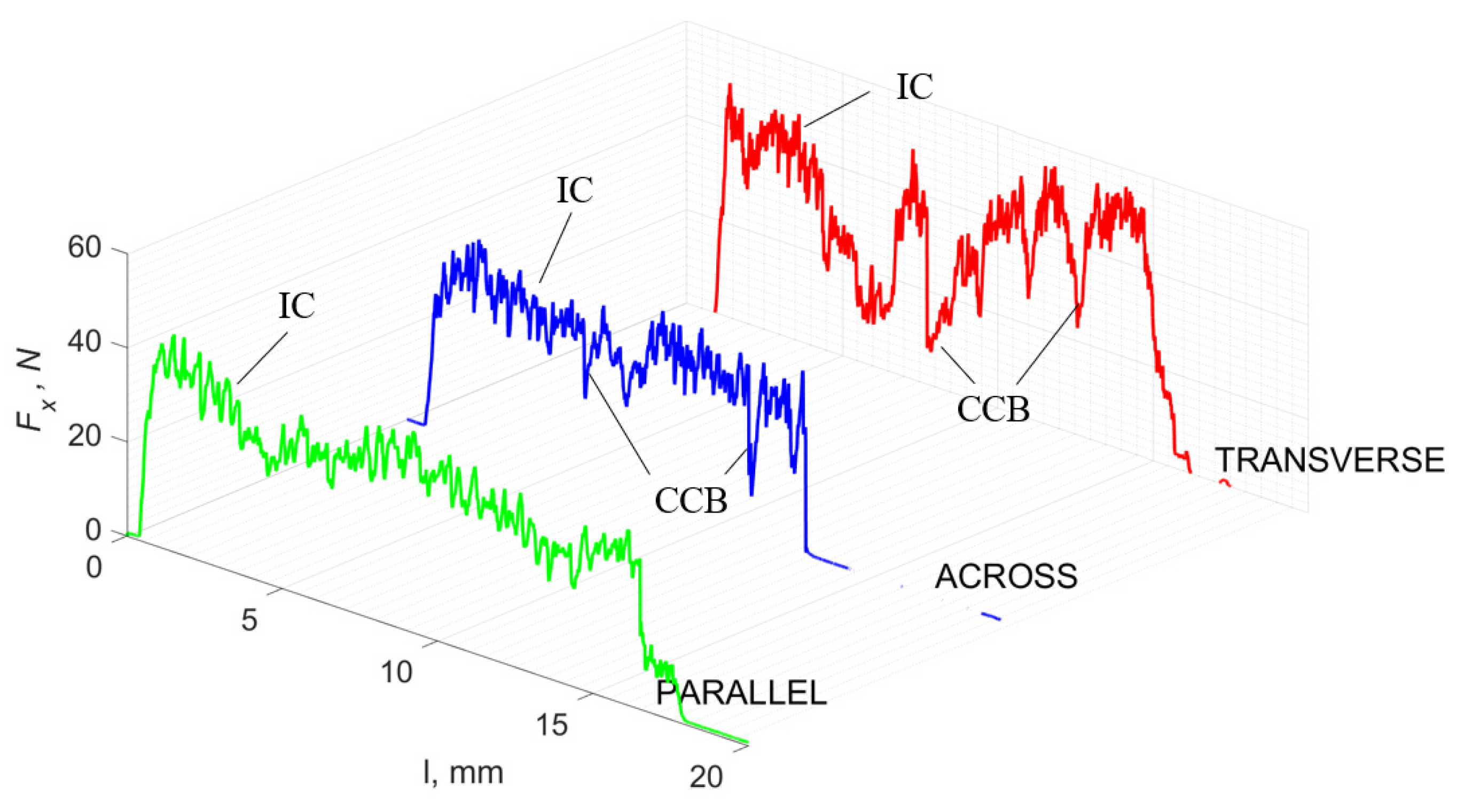




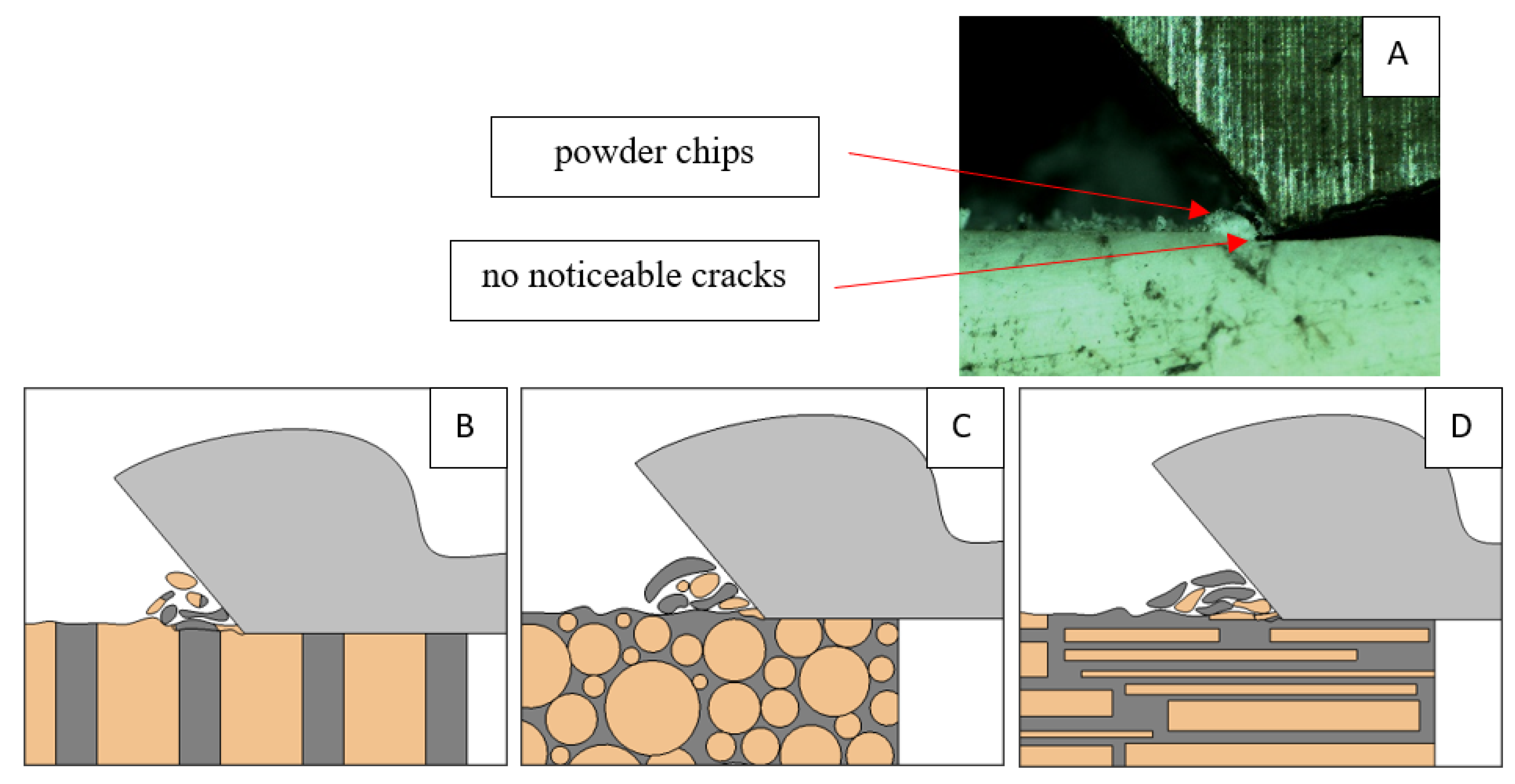
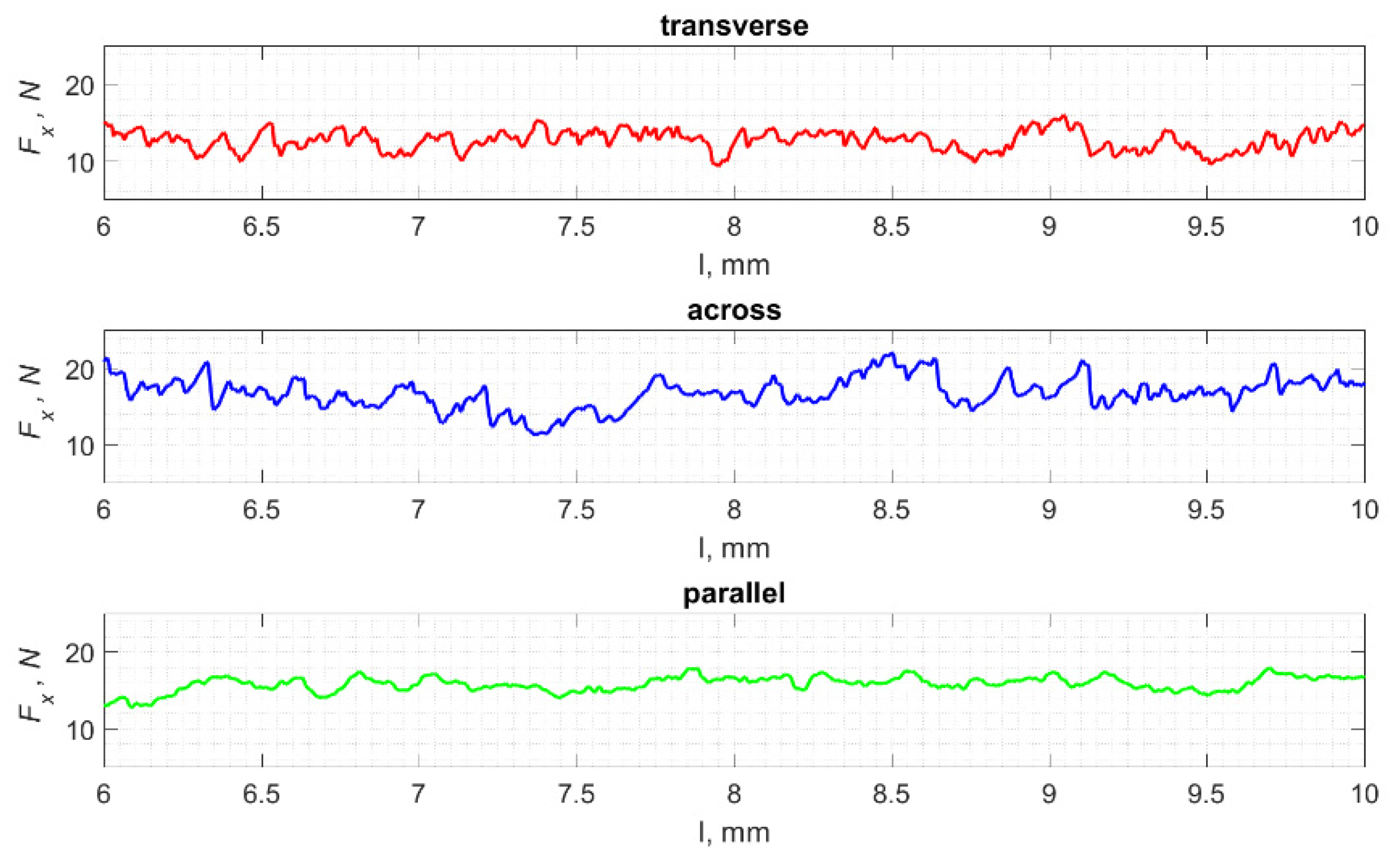

| Parameter | Nomenclature and Unit | Value |
|---|---|---|
| Rake angle | γ, ° | 40, 30, 20, 10, 0, −10, −20, −30, −40 |
| Clearance angle | α, ° | 5, 10, 15 |
| Cutting depth | d, μm | 0.5, 1, 2, 2.5, 5, 10, 25, 50, 100, 125, 150, 175 |
| Cutting velocity | vc, mm/min | 30 |
| Parameter | Direction | ||
|---|---|---|---|
| Transverse | Across | Parallel | |
| Vmp, mL/m2 | 0.1 | 0.118 | 0.0589 |
| Vmc, mL/m2 | 2.56 | 1.71 | 0.919 |
| Vvc, mL/m2 | 3.29 | 2.17 | 1.29 |
| Vvv, mL/m2 | 0.715 | 0.571 | 0.185 |
| Iz, % | 17.7 | 34.4 | 32.3 |
| df, μm | 5.22 | 3.74 | 1.88 |
| Rq, μm | 1.53 | 1.18 | 1.1 |
| γ, ° | d, μm | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.5 | 1 | 2.5 | 5 | 10 | 25 | 50 | 100 | 125 | 150 | 175 | |
| TRANSVERSE | |||||||||||
| −40 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −30 | N, F | N, F | N, F | F, B, D | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −20 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −10 | N, F | N, F | SP, F | SP, F | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| 0 | SP, F | SP, F | SP, F | S, C, I | S, C, I | S, C, I | S, C, I | S, C, I | S, B, D | S, B, D | S, B, D |
| 10 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H | T, H | T, H | T, H |
| 20 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| 30 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| 40 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| γ, ° | PARALLEL | ||||||||||
| −40 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −30 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −20 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −10 | N, F | SP, F | SP, F | SP, F | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| 0 | SP, F | SP, F | SP, F | I, C | I, C | I, C | S, I, C | S, I, C | S, B, D | S, B, D | S, B, D |
| 10 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H | T, H | T, H | T, H |
| 20 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| 30 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| 40 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| γ, ° | ACROSS | ||||||||||
| −40 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −30 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −20 | N, F | N, F | N, F | F, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| −10 | N, F | N, F | SP, F | SP, F | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D | S, B, D |
| 0 | SP, F | SP, F | SP, F | I, C | S, I, C | S, I, C | S, I, C | S, I, C | S, B, D | S, B, D | T, H |
| 10 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H | T, H | T, H | T, H |
| 20 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| 30 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
| 40 | SP | SP | SP | I, C | I, C | I, C | I, C | T, H, C | T, H | T, H | T, H |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zawadzki, P.; Talar, R. Bone Abrasive Machining: Influence of Tool Geometry and Cortical Bone Anisotropic Structure on Crack Propagation. J. Funct. Biomater. 2022, 13, 154. https://doi.org/10.3390/jfb13030154
Zawadzki P, Talar R. Bone Abrasive Machining: Influence of Tool Geometry and Cortical Bone Anisotropic Structure on Crack Propagation. Journal of Functional Biomaterials. 2022; 13(3):154. https://doi.org/10.3390/jfb13030154
Chicago/Turabian StyleZawadzki, Paweł, and Rafał Talar. 2022. "Bone Abrasive Machining: Influence of Tool Geometry and Cortical Bone Anisotropic Structure on Crack Propagation" Journal of Functional Biomaterials 13, no. 3: 154. https://doi.org/10.3390/jfb13030154






