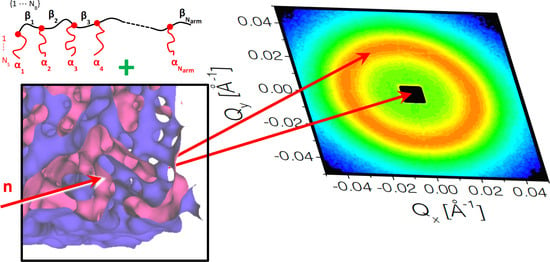
Amphiphilic Comb Polymers as New Additives in Bicontinuous Microemulsions
Abstract
1. Introduction
2. Materials and Methods
2.1. Polymer Synthesis and Characterization
2.2. Further Materials
2.3. Phase Diagram Measurements
2.4. Small-Angle Neutron Scattering (SANS)
3. Analysis Methods
3.1. Theory of Microemulsion Characterization
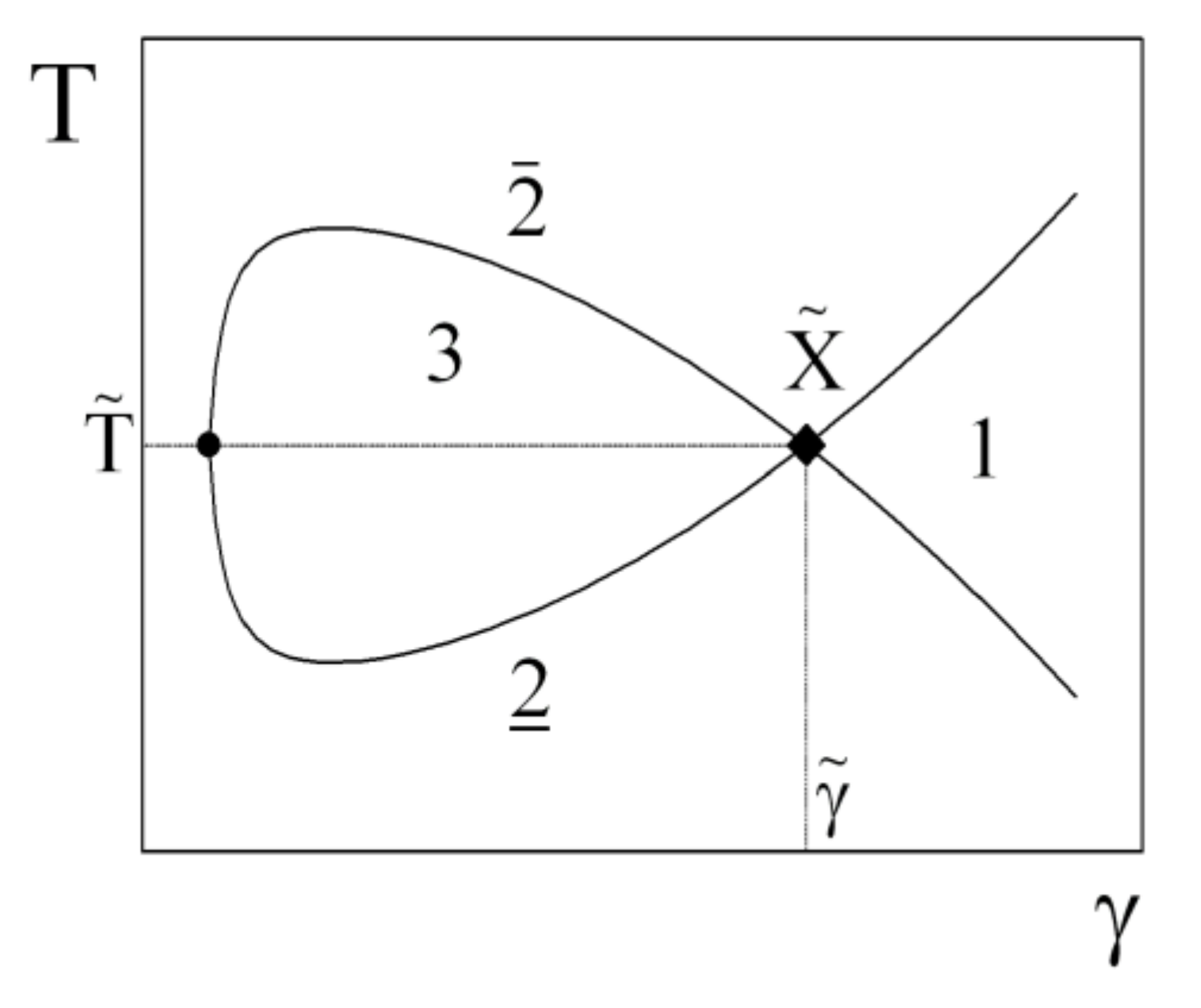
3.2. Phase Diagrams
3.3. Small-Angle Neutron Scattering on Domains
3.4. Contrast Variation SANS Measurements and Singular Value Decomposition
3.5. Polymer Scattering Model
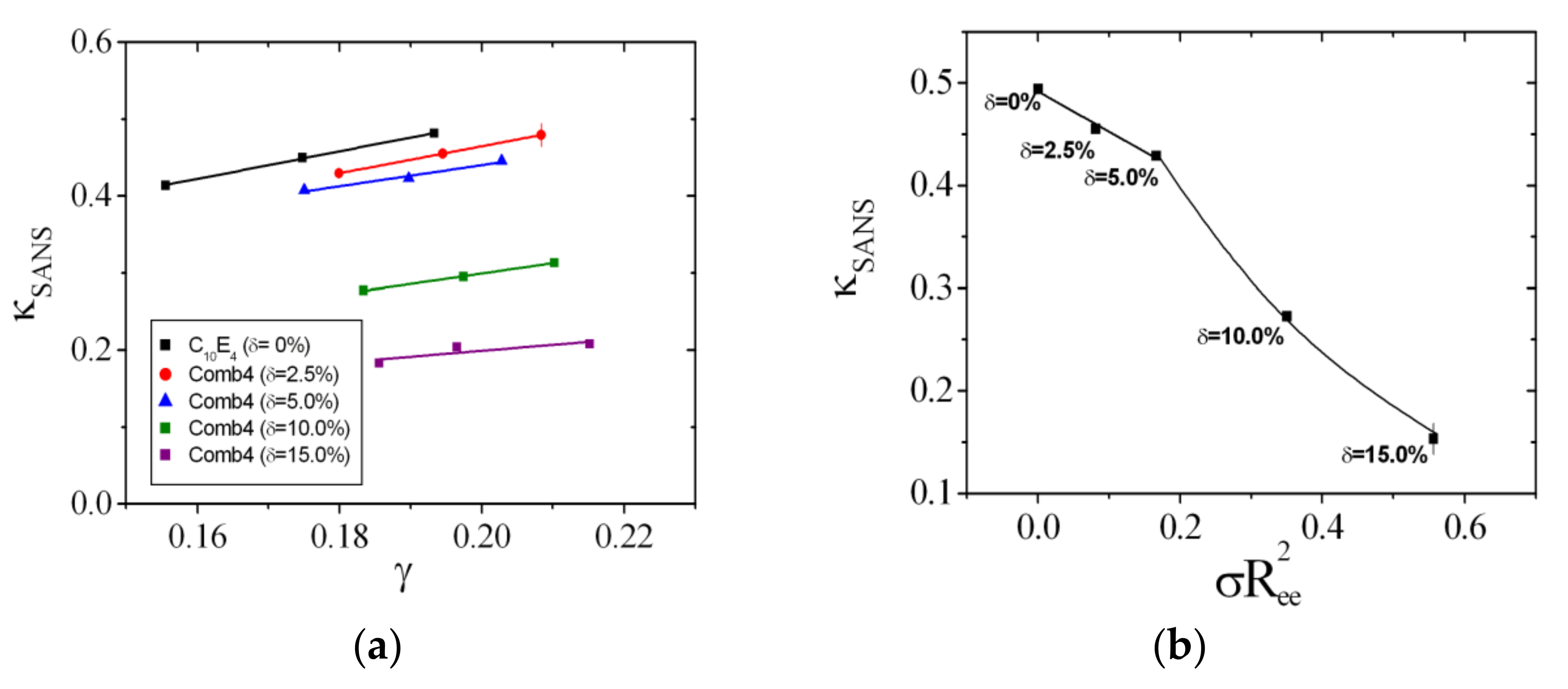
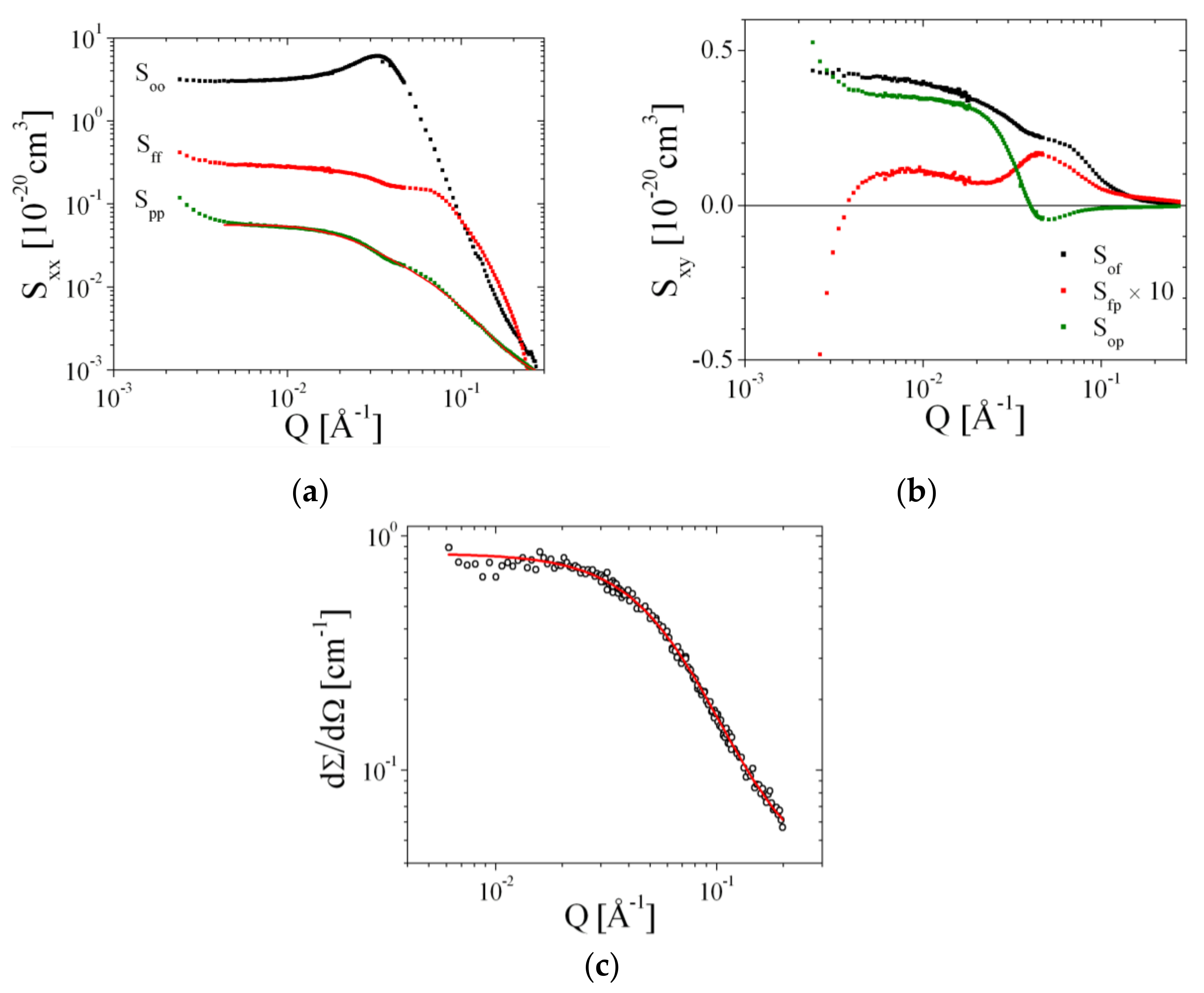
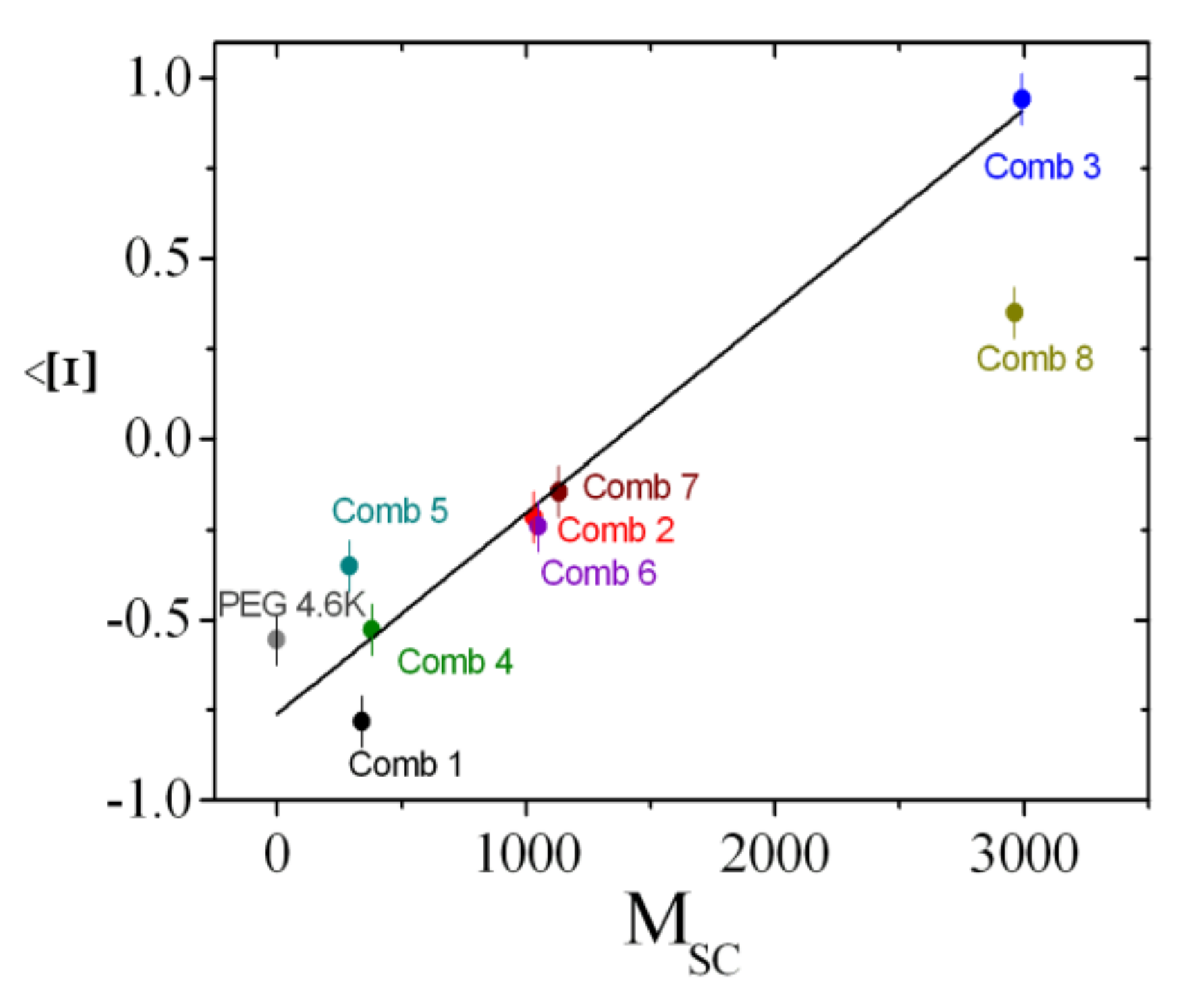
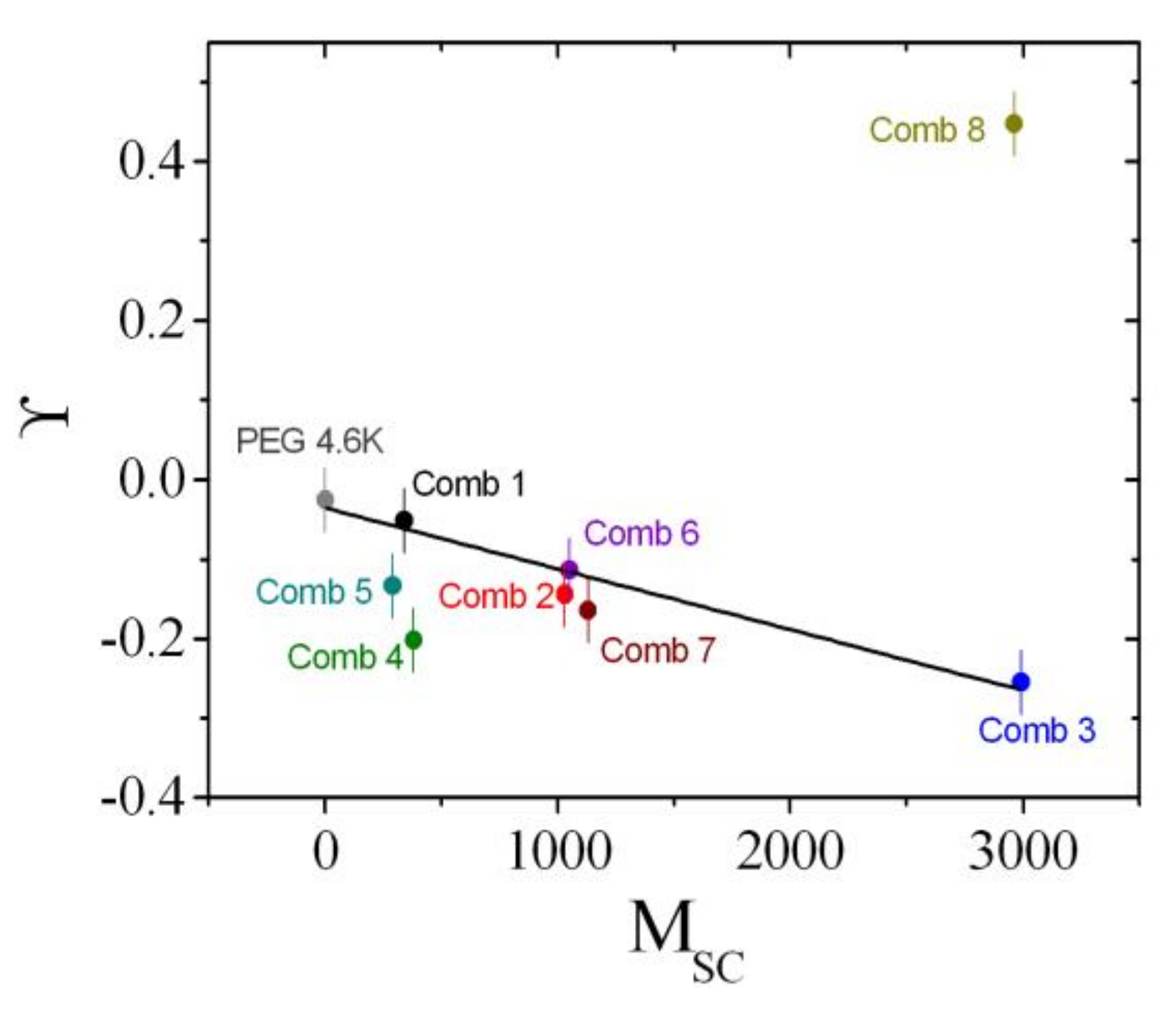
4. Results
4.1. Contrast Variation Experiments
4.2. Extensive Phase Diagram Measurements
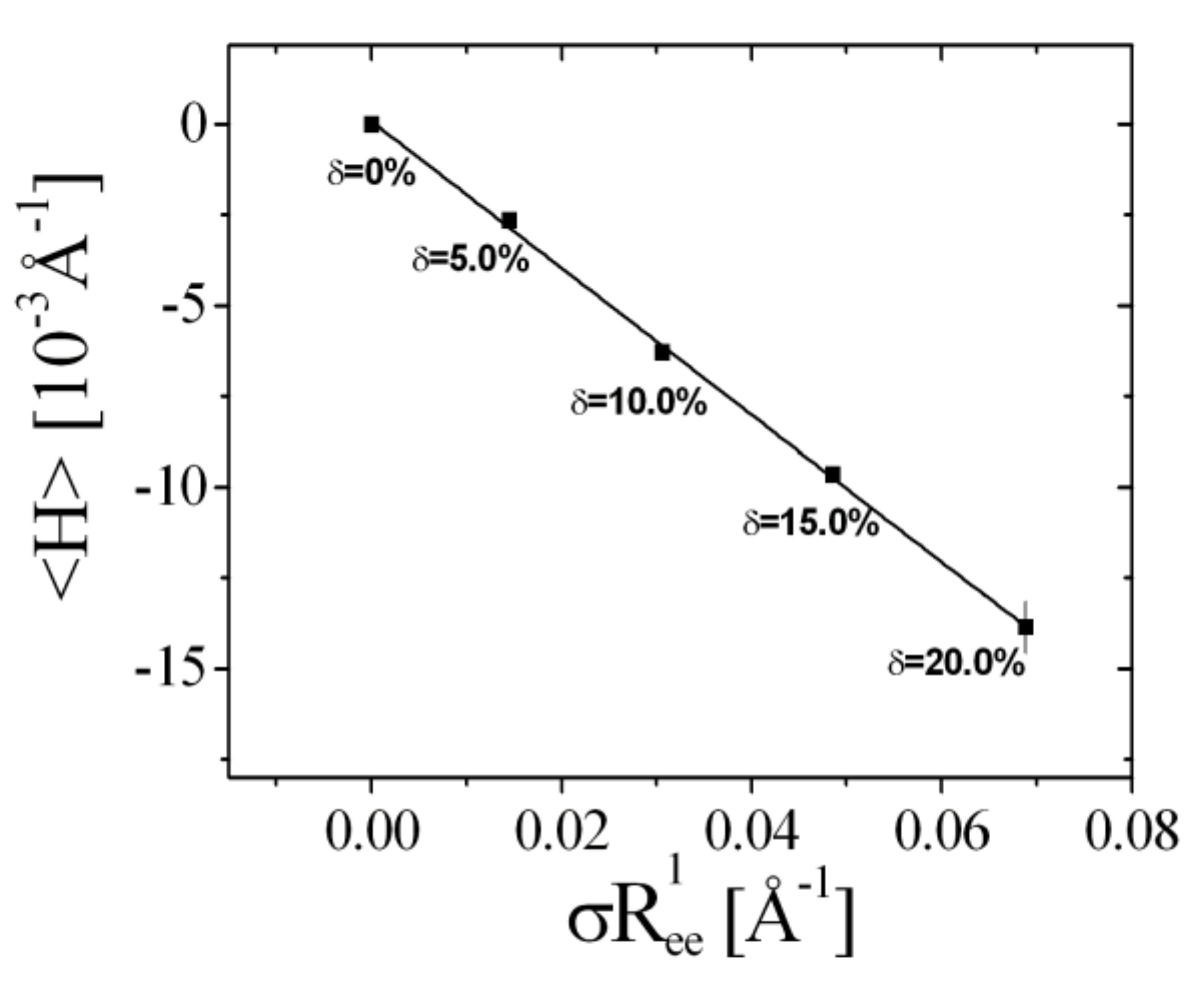
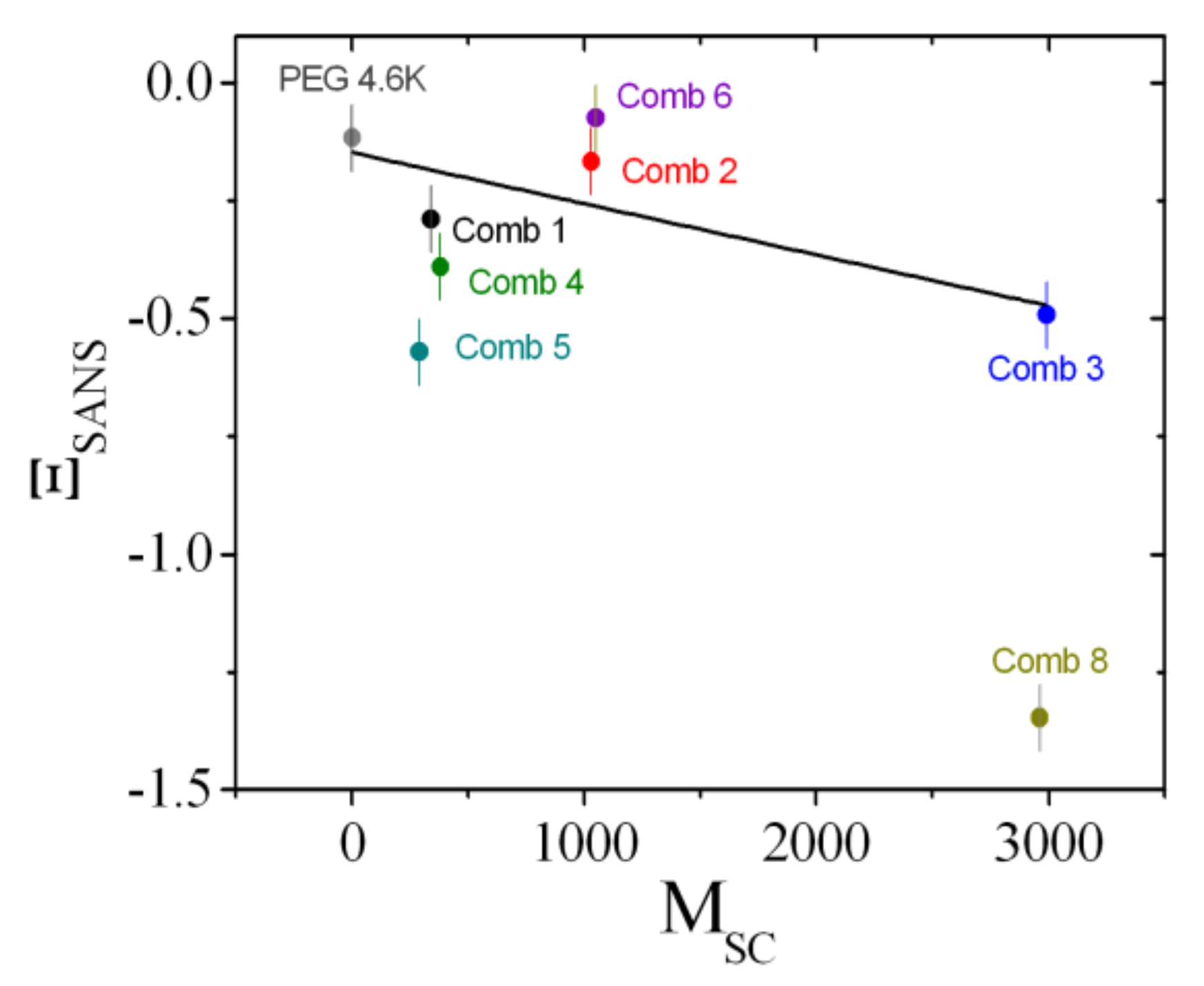
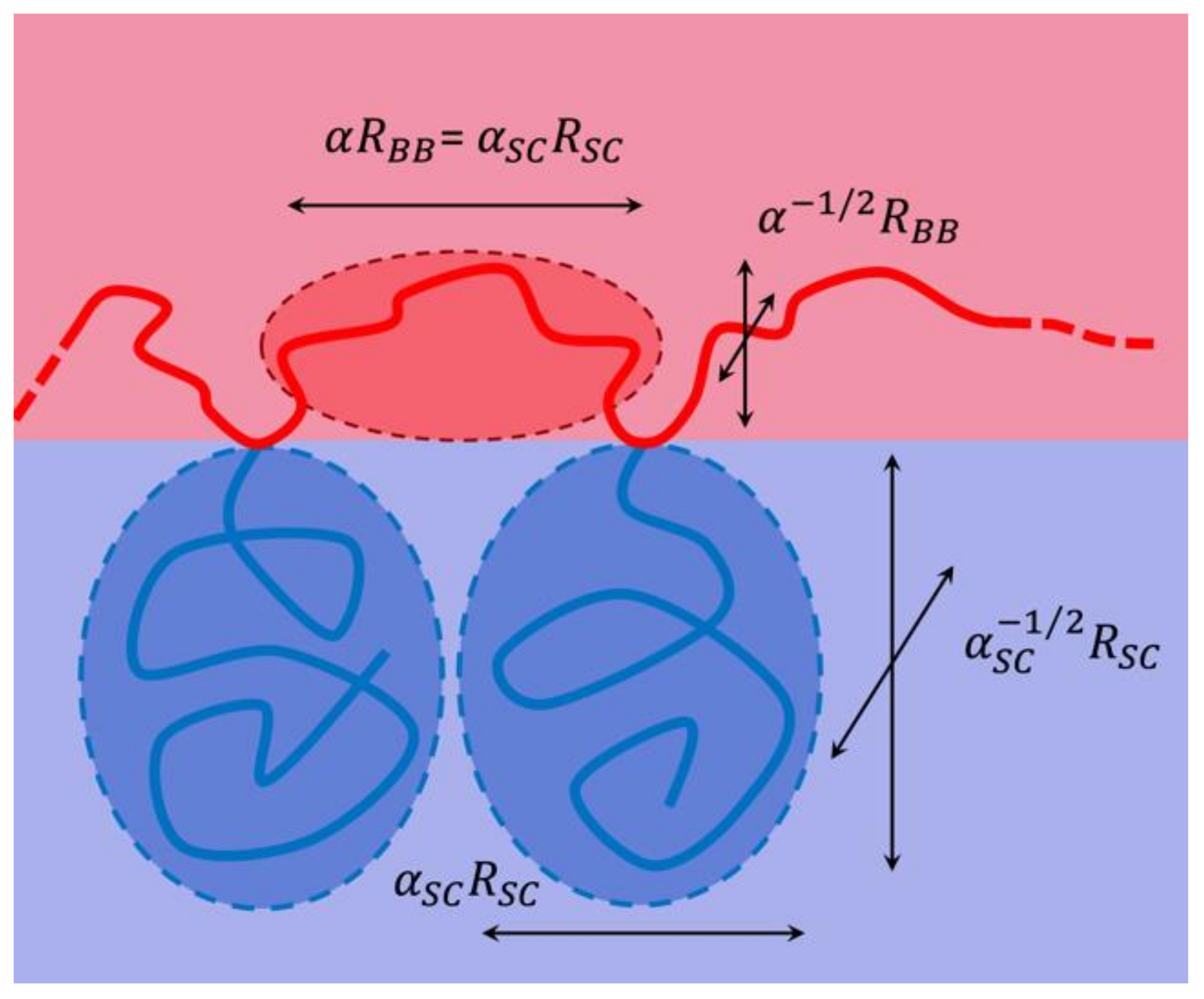
4.3. Rationalization
5. Discussion
6. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Endo, H.; Mihailescu, M.; Monkenbusch, M.; Allgaier, J.; Gompper, G.; Richter, D.; Jakobs, B.; Sottmann, T.; Strey, R.; Grillo, I. Effect of amphiphilic block copolymers on the structure and phase behavior of oil–water-surfactant mixtures. J. Chem. Phys. 2001, 115, 580–600. [Google Scholar] [CrossRef]
- Jakobs, B.; Sottmann, T.; Strey, R.; Allgaier, J.; Richter, D. Amphiphilic Block Copolymers as Efficiency Boosters for Microemulsions. Langmuir 1999, 15, 6707–6711. [Google Scholar] [CrossRef]
- Sottmann, T. Solubilization efficiency boosting by amphiphilic block co-polymers in microemulsions. Curr. Opin. Colloid Interface Sci. 2002, 7, 57–65. [Google Scholar] [CrossRef]
- Schneider, K.; Verkoyen, P.; Krappel, M.; Gardiner, C.; Schweins, R.; Frey, H.; Sottmann, T. Efficiency Boosting of Surfactants with Poly(ethylene oxide)-Poly(alkyl glycidyl ether)s: A New Class of Amphiphilic Polymers. Langmuir 2020, 36, 9849–9866. [Google Scholar] [CrossRef]
- Brodeck, M.; Maccarrone, S.; Saha, D.; Willner, L.; Allgaier, J.; Mangiapia, G.; Frielinghaus, H.; Holderer, O.; Faraone, A.; Richter, D. Erratum to: Asymmetric polymers in bicontinuous microemulsions and their accretion to the bending of the membrane. Colloid Polym. Sci. 2015, 293, 1267–1268. [Google Scholar] [CrossRef]
- Maccarrone, S.; Allgaier, J.; Frielinghaus, H.; Richter, D. Anchoring vs Bridging: New Findings on Polymer Additives in Bicontinuous Microemulsions. Langmuir 2014, 30, 1500–1505. [Google Scholar] [CrossRef]
- Klemmer, H.F.M.; Allgaier, J.; Frielinghaus, H.; Holderer, O.; Ohl, M. Influence of the amphiphilicity profile of copolymers on the formation of liquid crystalline mesophases in microemulsions. Colloid Polym. Sci. 2017, 295, 911–923. [Google Scholar] [CrossRef]
- Maccarrone, S.; Byelov, D.V.; Auth, T.; Allgaier, J.; Frielinghaus, H.; Gompper, G.; Richter, D. Confinement Effects in Block Copolymer Modified Bicontinuous Microemulsions. J. Phys. Chem. B 2013, 117, 5623–5632. [Google Scholar] [CrossRef]
- Byelov, D.; Frielinghaus, H.; Holderer, O.; Allgaier, J.; Richter, D. Microemulsion Efficiency Boosting and the Complementary Effect. 1. Structural Properties. Langmuir 2004, 20, 10433–10443. [Google Scholar] [CrossRef]
- Iwasaki, Y.; Akiyoshi, K. Design of Biodegradable Amphiphilic Polymers: Well-Defined Amphiphilic Polyphosphates with Hydrophilic Graft Chains via ATRP. Macromolecules 2004, 37, 7637–7642. [Google Scholar] [CrossRef]
- Iwasaki, Y.; Akiyoshi, K. Synthesis and Characterization of Amphiphilic Polyphosphates with Hydrophilic Graft Chains and Cholesteryl Groups as Nanocarriers. Biomacromolecules 2006, 7, 1433–1438. [Google Scholar] [CrossRef]
- Peng, T.; Cheng, S.-X.; Zhuo, R.-X. Synthesis and characterization of novel biodegradable amphiphilic graft polymers based on aliphatic polycarbonate. J. Polym. Sci. Part A Polym. Chem. 2004, 42, 1356–1361. [Google Scholar] [CrossRef]
- Strey, R. On the Stability Range of Microemulsions: From the Tricritical Point to the Lamellar Phase in Water/Formamide-Octane-CiEj Systems. Berichte der Bunsengesellschaft für physikalische Chemie 1993, 97, 742–750. [Google Scholar] [CrossRef]
- Larson, R.G. Self-assembly of surfactant liquid crystalline phases by Monte Carlo simulation. J. Chem. Phys. 1989, 91, 2479–2488. [Google Scholar] [CrossRef]
- Kahlweit, M.; Strey, R.; Haase, D.; Kunieda, H.; Schmeling, T.; Faulhaber, B.; Borkovec, M.; Eicke, H.-F.; Busse, G.; Eggers, F.; et al. How to study microemulsions. J. Colloid Interface Sci. 1987, 118, 436–453. [Google Scholar] [CrossRef]
- Tartaro, G.; Mateos, H.; Schirone, D.; Angelico, R.; Palazzo, G. Microemulsion Microstructure(s): A Tutorial Review. Nanomaterials 2020, 10, 1657. [Google Scholar] [CrossRef]
- Breidenich, M.; Netz, R.R.; Lipowsky, R. The shape of polymer-decorated membranes. EPL Europhys. Lett. 2000, 49, 431–437. [Google Scholar] [CrossRef]
- Hiergeist, C.; Lipowsky, R. Elastic Properties of Polymer-Decorated Membranes. J. Phys. II 1996, 6, 1465–1481. [Google Scholar] [CrossRef]
- Lipowsky, R. Bending of Membranes by Anchored Polymers. EPL Europhys. Lett. 1995, 30, 197–202. [Google Scholar] [CrossRef]
- Lipowsky, R. Flexible membranes with anchored polymers. Colloids Surf. A Physicochem. Eng. Asp. 1997, 128, 255–264. [Google Scholar] [CrossRef]
- Hiergeist, C.S. Elastische Eigenschaften Polymer-Dekorierter Membranen. Ph.D. Thesis, University Potsdam, Potsdam-Teltow, Germany, 1997. [Google Scholar]
- Koetz, J.; Beitz, T.; Tiersch, B. Self assembled polymer-surfactant systems. J. Dispers. Sci. Technol. 1999, 20, 139–163. [Google Scholar] [CrossRef]
- Bellocq, A.M. Effect of PEG on the stability of AOT microemulsions. In Trends in Colloid and Interface Science XI; Steinkopff: Dresden, Germany, 1997; pp. 290–293. [Google Scholar]
- Meier, W. Kerr Effect Measurements on a Poly(oxyethylene) Containing Water-in-Oil Microemulsion. J. Phys. Chem. B 1997, 101, 919–921. [Google Scholar] [CrossRef]
- Meier, W. Poly(oxyethylene) Adsorption in Water/Oil Microemulsions: A Conductivity Study. Langmuir 1996, 12, 1188–1192. [Google Scholar] [CrossRef]
- Allgaier, J.; Willbold, S.; Chang, T. Synthesis of Hydrophobic Poly(alkylene oxide)s and Amphiphilic Poly(alkylene oxide) Block Copolymers. Macromolecules 2007, 40, 518–525. [Google Scholar] [CrossRef]
- Allgaier, J.; Hövelmann, C.H.; Wei, Z.; Staropoli, M.; Pyckhout-Hintzen, W.; Lühmann, N.; Willbold, S. Synthesis and rheological behavior of poly(1,2-butylene oxide) based supramolecular architectures. RSC Adv. 2016, 6, 6093–6106. [Google Scholar] [CrossRef]
- Burauer, S.; Sachert, T.; Sottmann, T.; Strey, R. On microemulsion phase behavior and the monomeric solubility of surfactant. Phys. Chem. Chem. Phys. 1999, 1, 4299–4306. [Google Scholar] [CrossRef]
- Feoktystov, A.; Frielinghaus, H.; Di, Z.; Jaksch, S.; Pipich, V.; Appavou, M.-S.; Babcock, E.; Hanslik, R.; Engels, R.; Kemmerling, G.; et al. KWS-1 high-resolution small-angle neutron scattering instrument at JCNS: Current state. J. Appl. Crystallogr. 2015, 48, 61–70. [Google Scholar] [CrossRef]
- Frielinghaus, H.; Feoktystov, A.; Berts, I.; Mangiapia, G. KWS-1: Small-angle scattering diffractometer. J. Large-Scale Res. Facil. JLSRF 2015, 1, 28. [Google Scholar] [CrossRef]
- Endo, H.; Allgaier, J.; Gompper, G.; Jakobs, B.; Monkenbusch, M.; Richter, D.; Sottmann, T.; Strey, R. Membrane Decoration by Amphiphilic Block Copolymers in Bicontinuous Microemulsions. Phys. Rev. Lett. 2000, 85, 102–105. [Google Scholar] [CrossRef]
- Gompper, G.; Endo, H.; Mihailescu, M.; Allgaier, J.; Monkenbusch, M.; Richter, D.; Jakobs, B.; Sottmann, T.; Strey, R. Measuring bending rigidity and spatial renormalization in bicontinuous microemulsions. EPL Europhys. Lett. 2001, 56, 683–689. [Google Scholar] [CrossRef]
- Kawaguchi, S.; Imai, G.; Suzuki, J.; Miyahara, A.; Kitano, T.; Ito, K. Aqueous solution properties of oligo- and poly(ethylene oxide) by static light scattering and intrinsic viscosity. Polymer 1997, 38, 2885–2891. [Google Scholar] [CrossRef]
- Sottmann, T.; Strey, R.; Chen, S.-H. A small-angle neutron scattering study of nonionic surfactant molecules at the water–oil interface: Area per molecule, microemulsion domain size, and rigidity. J. Chem. Phys. 1997, 106, 6483–6491. [Google Scholar] [CrossRef]
- Sottmann, T.; Strey, R. Ultralow interfacial tensions in water–n-alkane–surfactant systems. J. Chem. Phys. 1997, 106, 8606–8615. [Google Scholar] [CrossRef]
- Lang, J.; Jada, A.; Malliaris, A. Structure and dynamics of water-in-oil droplets stabilized by sodium bis(2-ethylhexyl)sulfosuccinate. J. Phys. Chem. 1988, 92, 1946–1953. [Google Scholar] [CrossRef]
- Teubner, M.; Strey, R. Origin of the scattering peak in microemulsions. J. Chem. Phys. 1987, 87, 3195–3200. [Google Scholar] [CrossRef]
- Frank, C.; Frielinghaus, H.; Allgaier, J.; Prast, H. Nonionic Surfactants with Linear and Branched Hydrocarbon Tails: Compositional Analysis, Phase Behavior, and Film Properties in Bicontinuous Microemulsions. Langmuir 2007, 23, 6526–6535. [Google Scholar] [CrossRef]
- Beaucage, G. Small-Angle Scattering from Polymeric Mass Fractals of Arbitrary Mass-Fractal Dimension. J. Appl. Crystallogr. 1996, 29, 134–146. [Google Scholar] [CrossRef]
- Holderer, O.; Frielinghaus, H.; Monkenbusch, M.; Klostermann, M.; Sottmann, T.; Richter, D. Experimental determination of bending rigidity and saddle splay modulus in bicontinuous microemulsions. Soft Matter 2013, 9, 2308–2313. [Google Scholar] [CrossRef]
- Debye, P. Molecular-weight Determination by Light Scattering. J. Phys. Chem. 1947, 51, 18–32. [Google Scholar] [CrossRef]
- Svaneborg, C.; Pedersen, J.S. A formalism for scattering of complex composite structures. I. Applications to branched structures of asymmetric sub-units. J. Chem. Phys. 2012, 136, 104105. [Google Scholar] [CrossRef]
- Hammouda, B. Small-Angle Scattering From Branched Polymers. Macromol. Theory Simul. 2012, 21, 372–381. [Google Scholar] [CrossRef]
- Gerstl, C.; Schneider, G.J.; Pyckhout-Hintzen, W.; Allgaier, J.; Willbold, S.; Hofmann, D.; Disko, U.; Frielinghaus, H.; Richter, D. Chain Conformation of Poly(alkylene oxide)s Studied by Small-Angle Neutron Scattering. Macromolecules 2011, 44, 6077–6084. [Google Scholar] [CrossRef]
- Hanke, A.; Eisenriegler, E.; Dietrich, S. Polymer depletion effects near mesoscopic particles. Phys. Rev. E 1999, 59, 6853–6878. [Google Scholar] [CrossRef] [PubMed]
- James, H.M.; Guth, E. Statistical Thermodynamics of Rubber Elasticity. J. Chem. Phys. 1953, 21, 1039–1049. [Google Scholar] [CrossRef]
- Werner, M.; Sommer, J.-U. Translocation and Induced Permeability of Random Amphiphilic Copolymers Interacting with Lipid Bilayer Membranes. Biomacromolecules 2015, 16, 125–135. [Google Scholar] [CrossRef] [PubMed]
- Huang, S.W.; Wang, J.; Zhang, P.C.; Mao, H.Q.; Zhuo, R.X.; Leong, K.W. Water-Soluble and Nonionic Polyphosphoester: Synthesis, Degradation, Biocompatibility and Enhancement of Gene Expression in Mouse Muscle. Biomacromolecules 2004, 5, 306–311. [Google Scholar] [CrossRef]





| PBO Backbone | PBO-PEO Comb Polymer | |||||
|---|---|---|---|---|---|---|
| Polymer | MBBtot a (g/mol) | PDIBBtot b | PDIcomb b | mSC/mBB c | NSC d | MSC e (g/mol) |
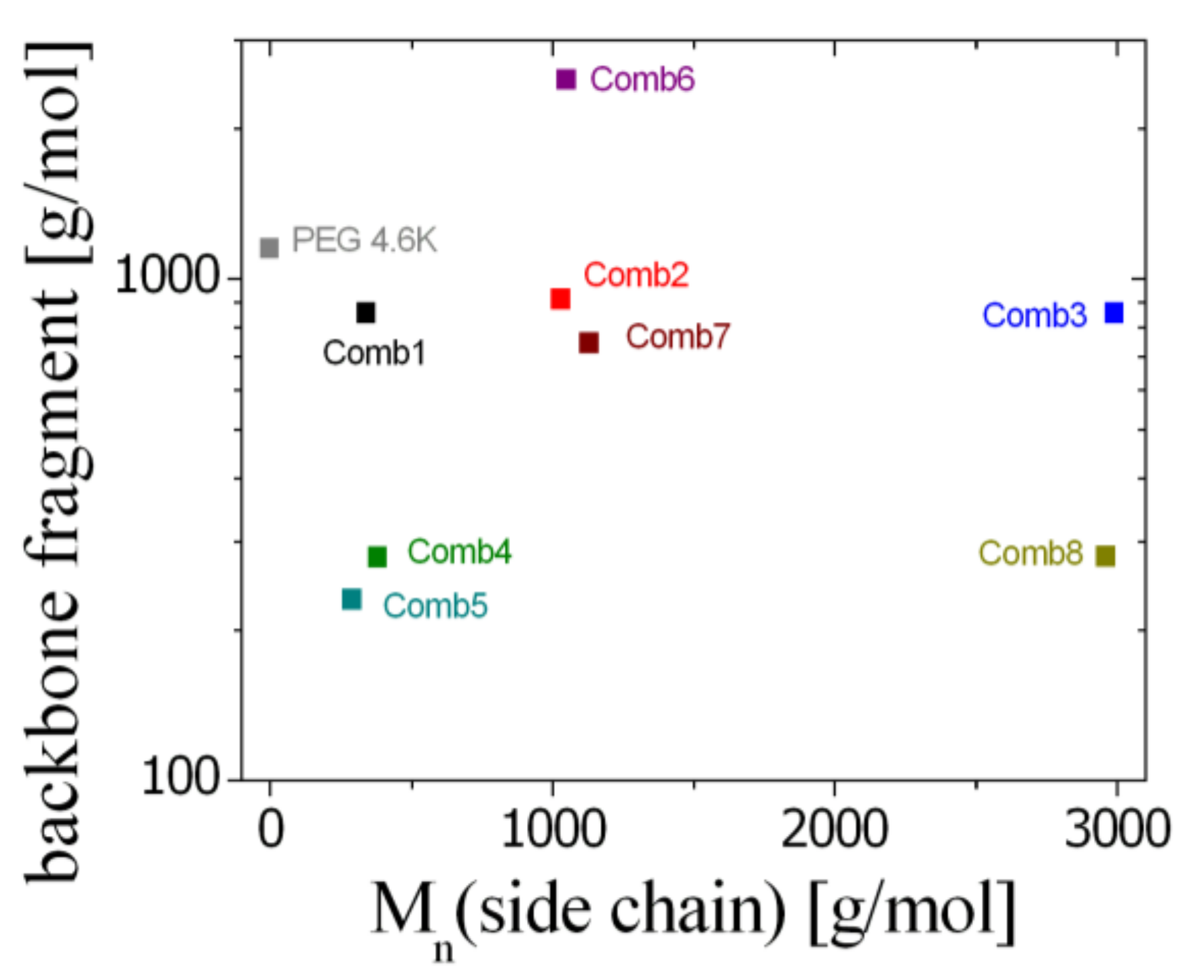
| Comb 1 | 11,200 | 1.03 | 1.04 | 0.40 | 13.1 | 340 |
| Comb 2 | 11,200 | 1.02 | 1.05 | 1.13 | 12.3 | 1030 |
| Comb 3 | 11,200 | 1.03 | 1.05 | 3.50 | 13.1 | 2990 |
| Comb 4 | 10,300 | 1.03 | 1.07 | 1.36 | 36.9 | 380 |
| Comb 5 | 11,400 | 1.03 | 1.07 | 1.14 | 44.8 | 290 |
| Comb 6 | 29,000 | 1.07 | 1.07 | 0.42 | 11.6 | 1050 |
| Comb 7 | 29,000 | 1.07 | 1.07 | 0.94 | 24.1 | 1130 |
| Comb 8 | 10,300 | 1.03 | 1.03 | 10.49 | 36.5 | 2960 |
| PEG 4.6 k | 4600 | 1.05 | (4.0) | |||
| Polymer | MBB a (g/mol) | ReeBB (Å) | ReeSC (Å) | ||
|---|---|---|---|---|---|
| Comb1 | 850 | 18.66 | 14.54 | 0.876 | 0.943 |
| Comb2 | 910 | 19.35 | 27.66 | 1.177 | 1.102 |
| Comb3 | 850 | 18.66 | 51.32 | 1.491 | 1.358 |
| Comb4 | 280 | 9.75 | 15.51 | 1.229 | 1.138 |
| Comb5 | 250 | 9.24 | 13.26 | 1.179 | 1.103 |
| Comb6 | 2500 | 34.77 | 27.97 | 0.892 | 0.950 |
| Comb7 | 1200 | 22.75 | 29.19 | 1.124 | 1.068 |
| Comb8 | 280 | 9.81 | 51.02 | 1.815 | 1.693 |
| PEG 4.6k | 850 | 29.49 | (0) | (1) | (1) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saha, D.; Peddireddy, K.R.; Allgaier, J.; Zhang, W.; Maccarrone, S.; Frielinghaus, H.; Richter, D. Amphiphilic Comb Polymers as New Additives in Bicontinuous Microemulsions. Nanomaterials 2020, 10, 2410. https://doi.org/10.3390/nano10122410
Saha D, Peddireddy KR, Allgaier J, Zhang W, Maccarrone S, Frielinghaus H, Richter D. Amphiphilic Comb Polymers as New Additives in Bicontinuous Microemulsions. Nanomaterials. 2020; 10(12):2410. https://doi.org/10.3390/nano10122410
Chicago/Turabian StyleSaha, Debasish, Karthik R. Peddireddy, Jürgen Allgaier, Wei Zhang, Simona Maccarrone, Henrich Frielinghaus, and Dieter Richter. 2020. "Amphiphilic Comb Polymers as New Additives in Bicontinuous Microemulsions" Nanomaterials 10, no. 12: 2410. https://doi.org/10.3390/nano10122410
APA StyleSaha, D., Peddireddy, K. R., Allgaier, J., Zhang, W., Maccarrone, S., Frielinghaus, H., & Richter, D. (2020). Amphiphilic Comb Polymers as New Additives in Bicontinuous Microemulsions. Nanomaterials, 10(12), 2410. https://doi.org/10.3390/nano10122410