Structure, Morphology and Electrical/Magnetic Properties of Ni-Mg Nano-Ferrites from a New Perspective
Abstract
:1. Introduction
2. Materials and Methods
2.1. Synthesis Procedure and Method
2.2. Characterization Methods
3. Results and Discussion
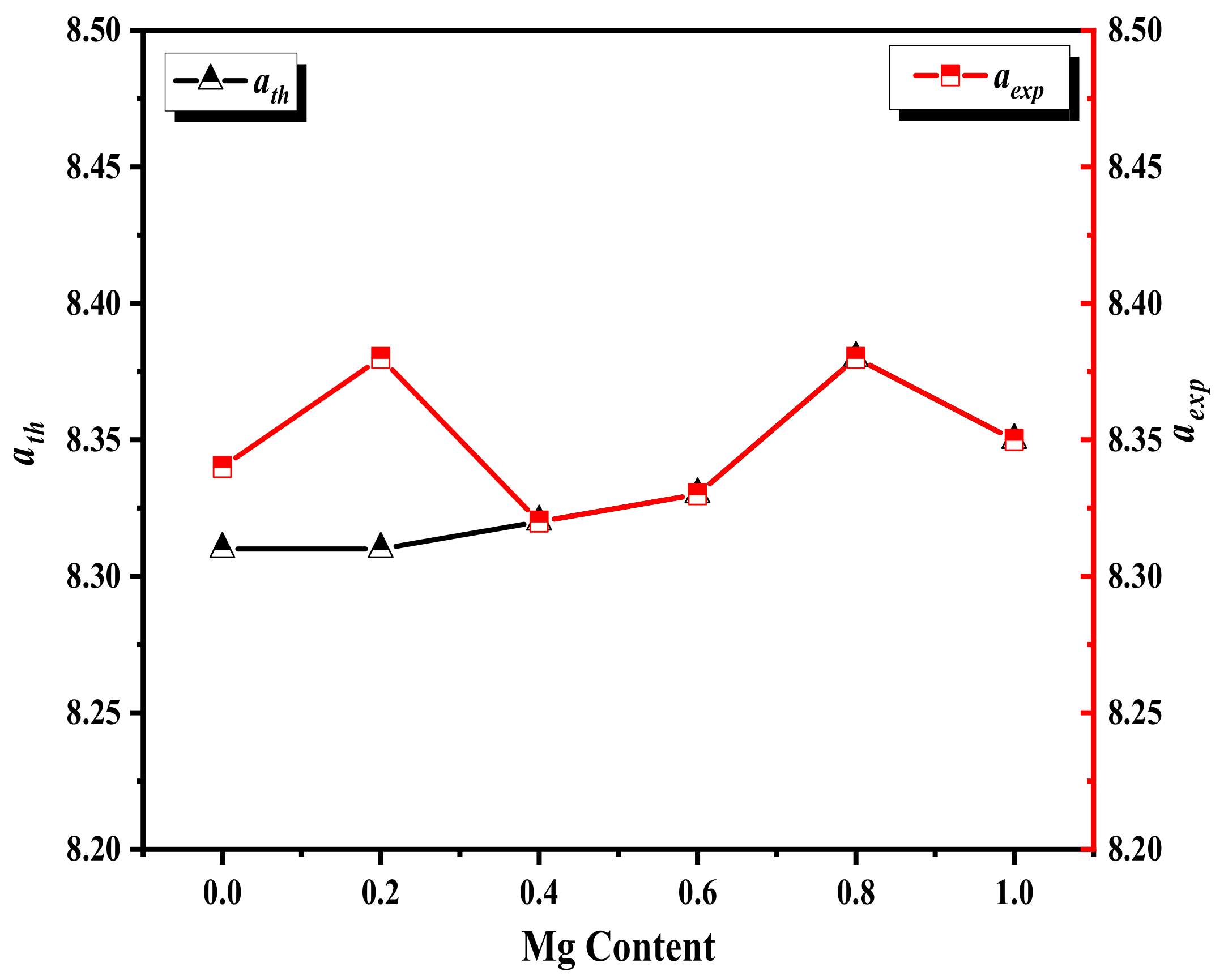
3.1. XRD (X-ray Diffraction) Discussion
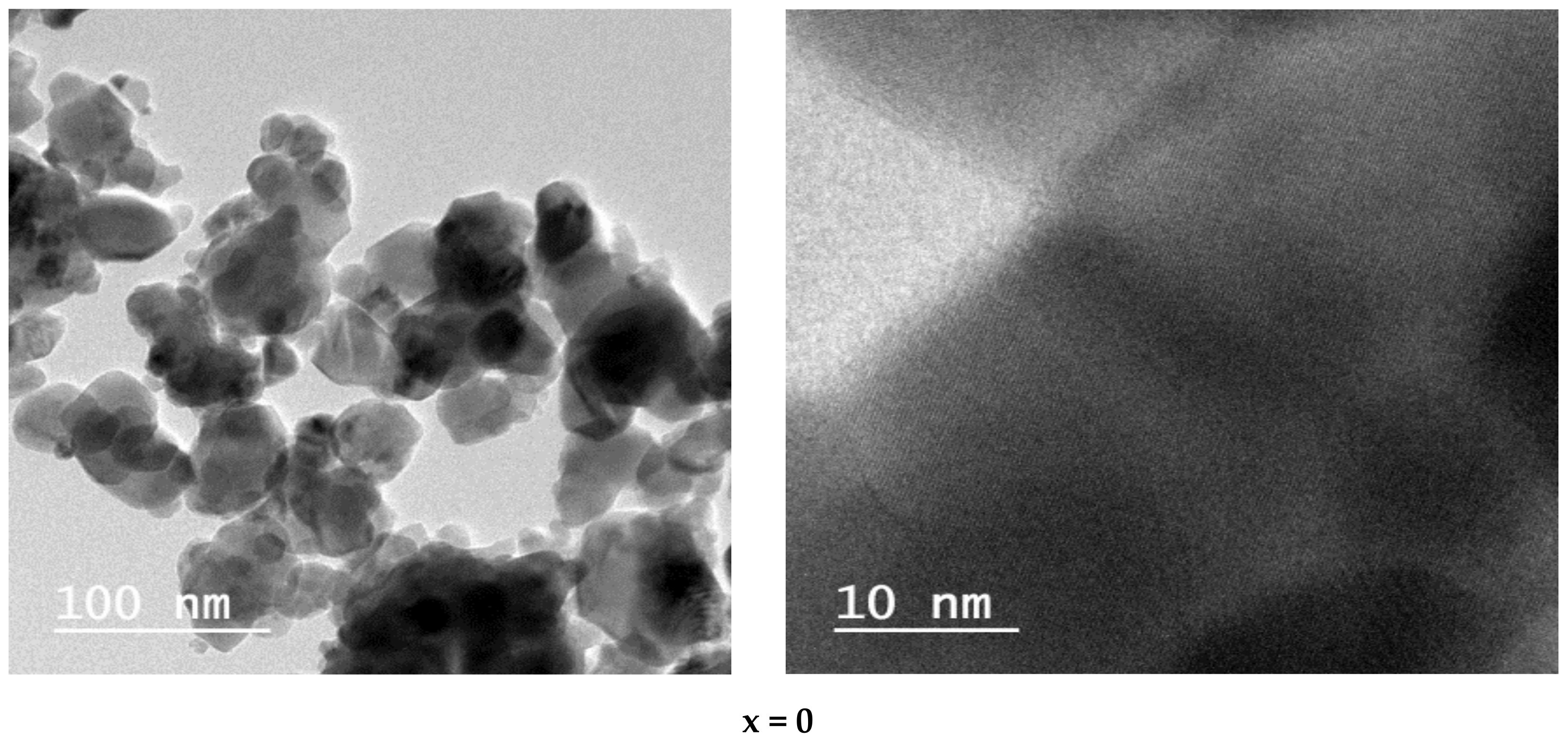
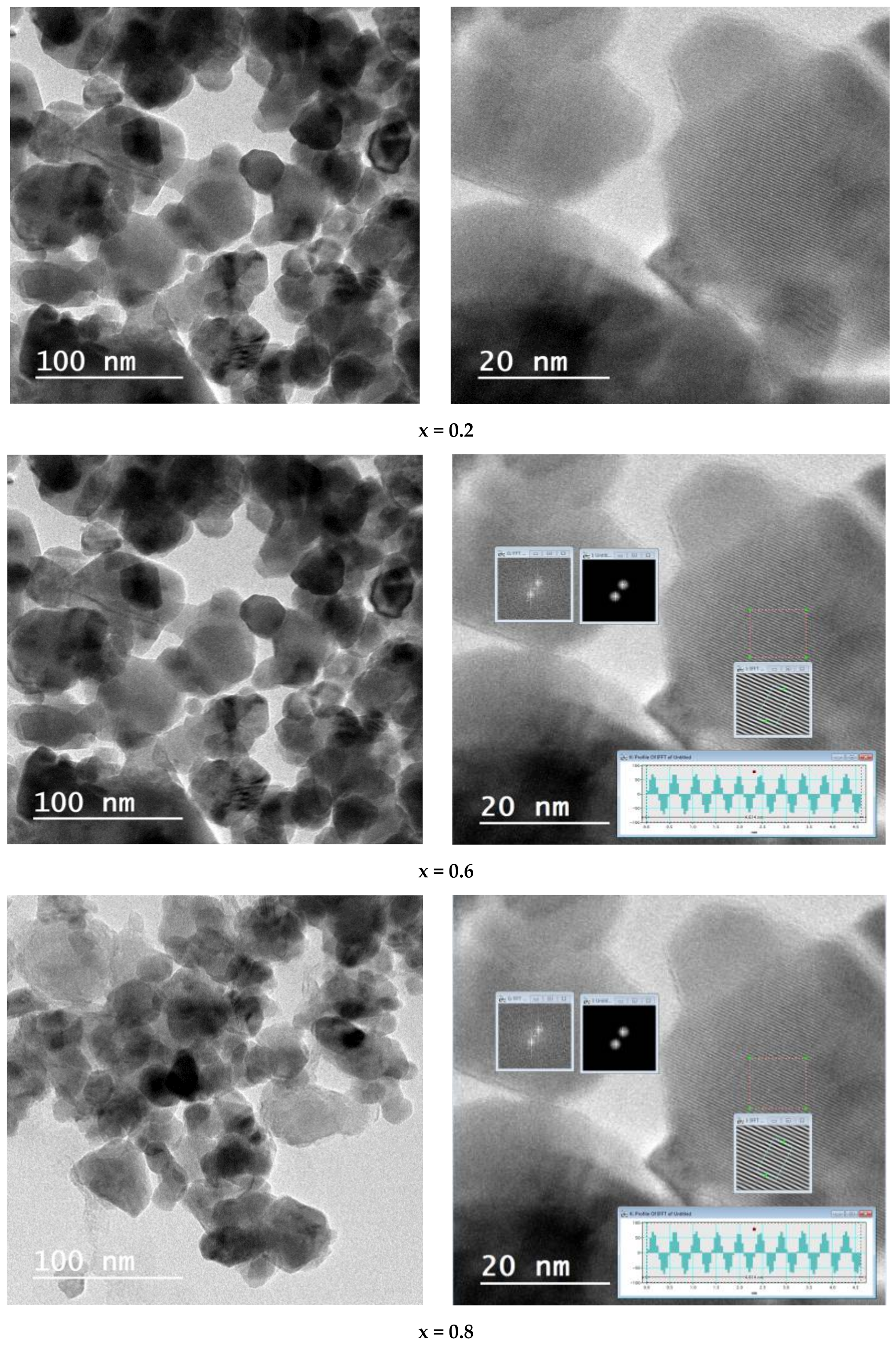
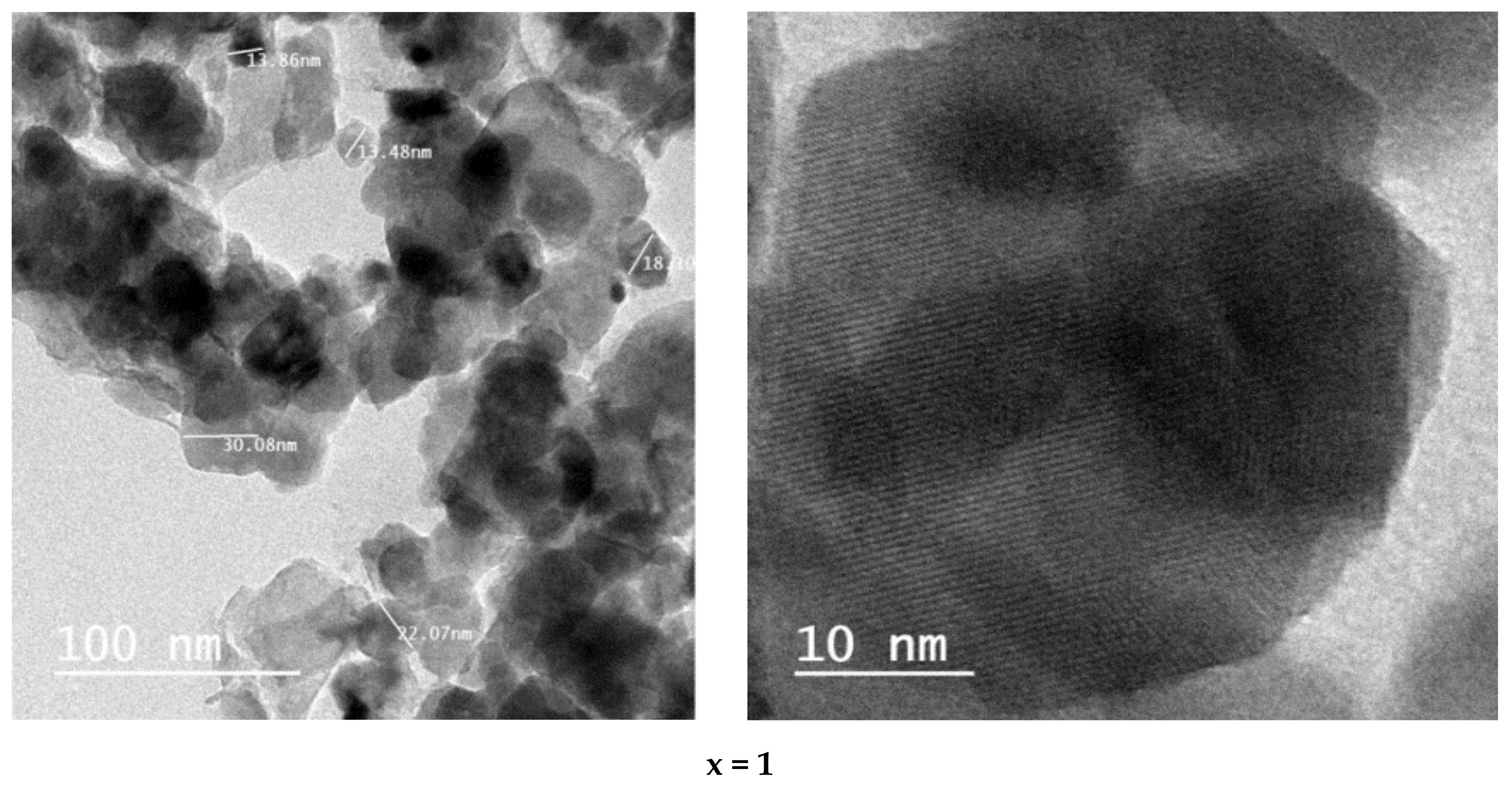
3.2. HRTEM (High-Resolution Transmission Electron Microscopy) Discussion
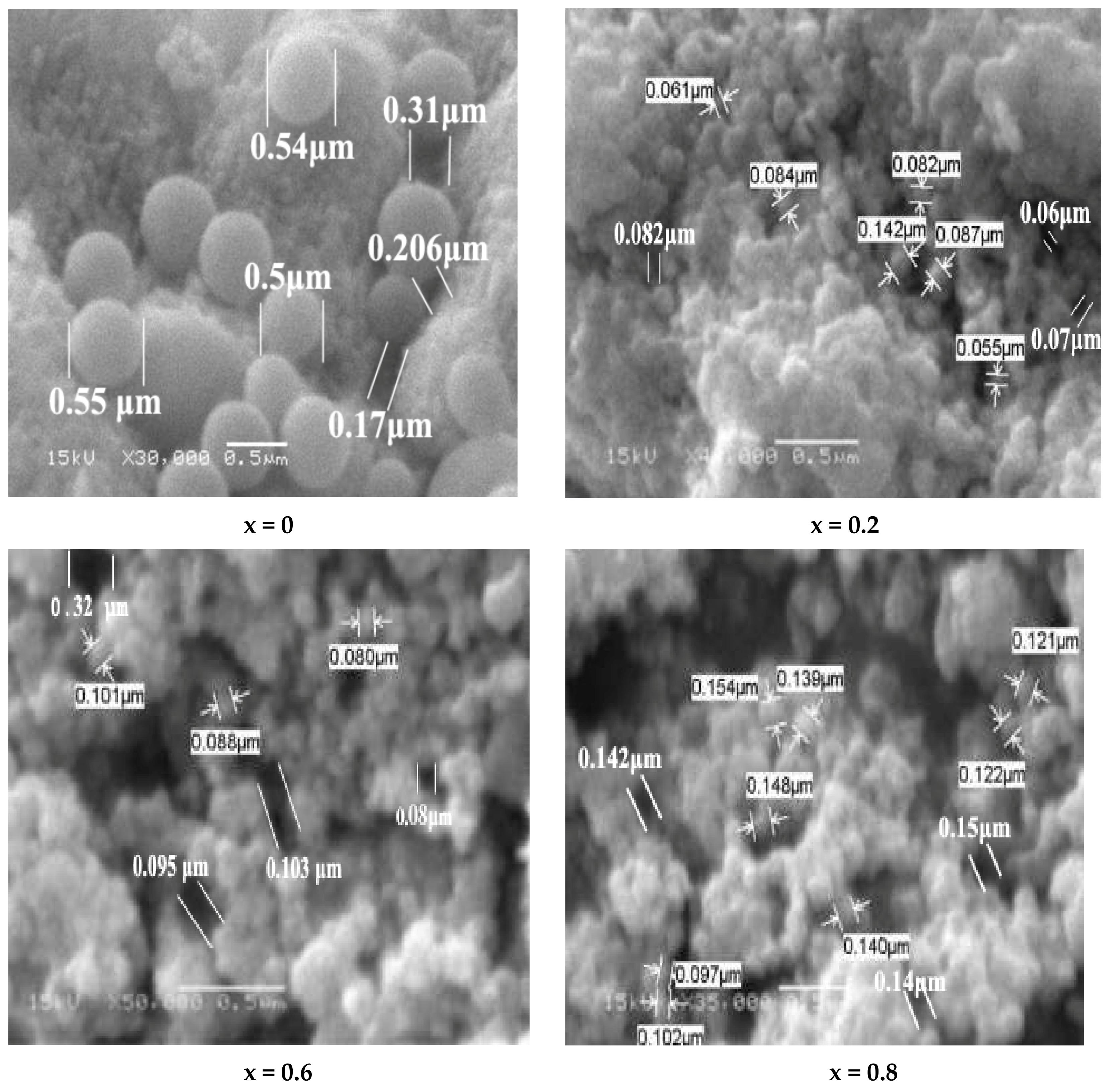
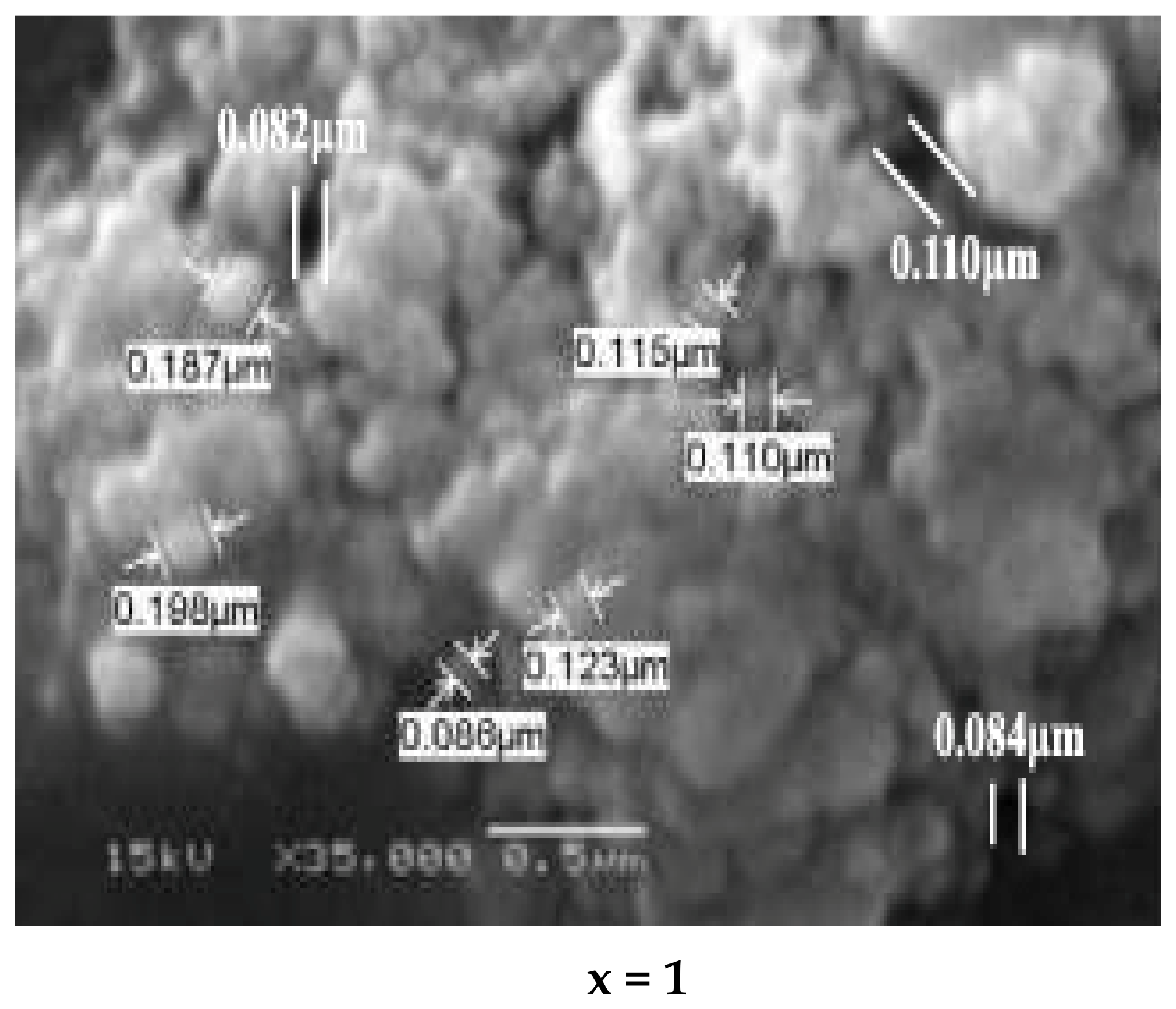
3.3. SEM (Scanning Electron Microscopy) Discussion
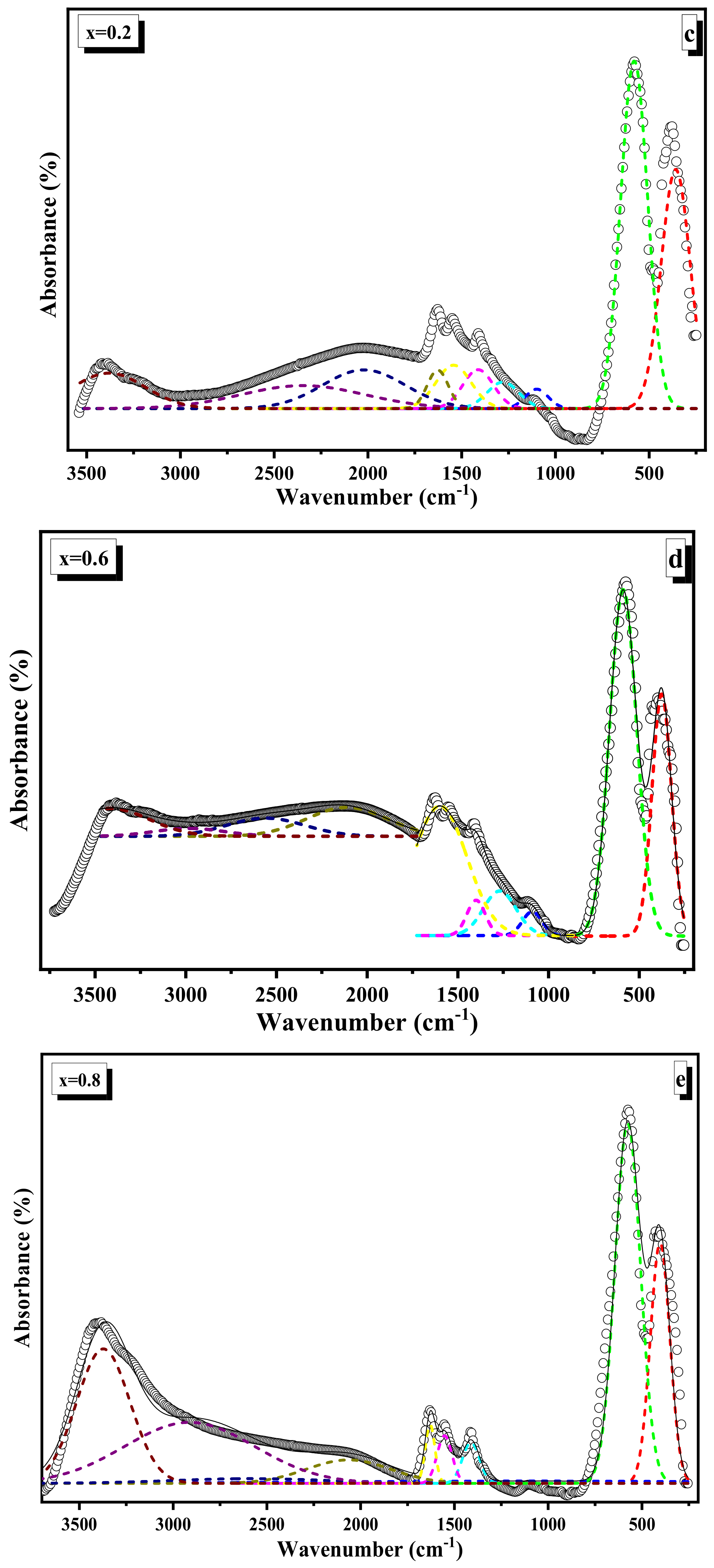
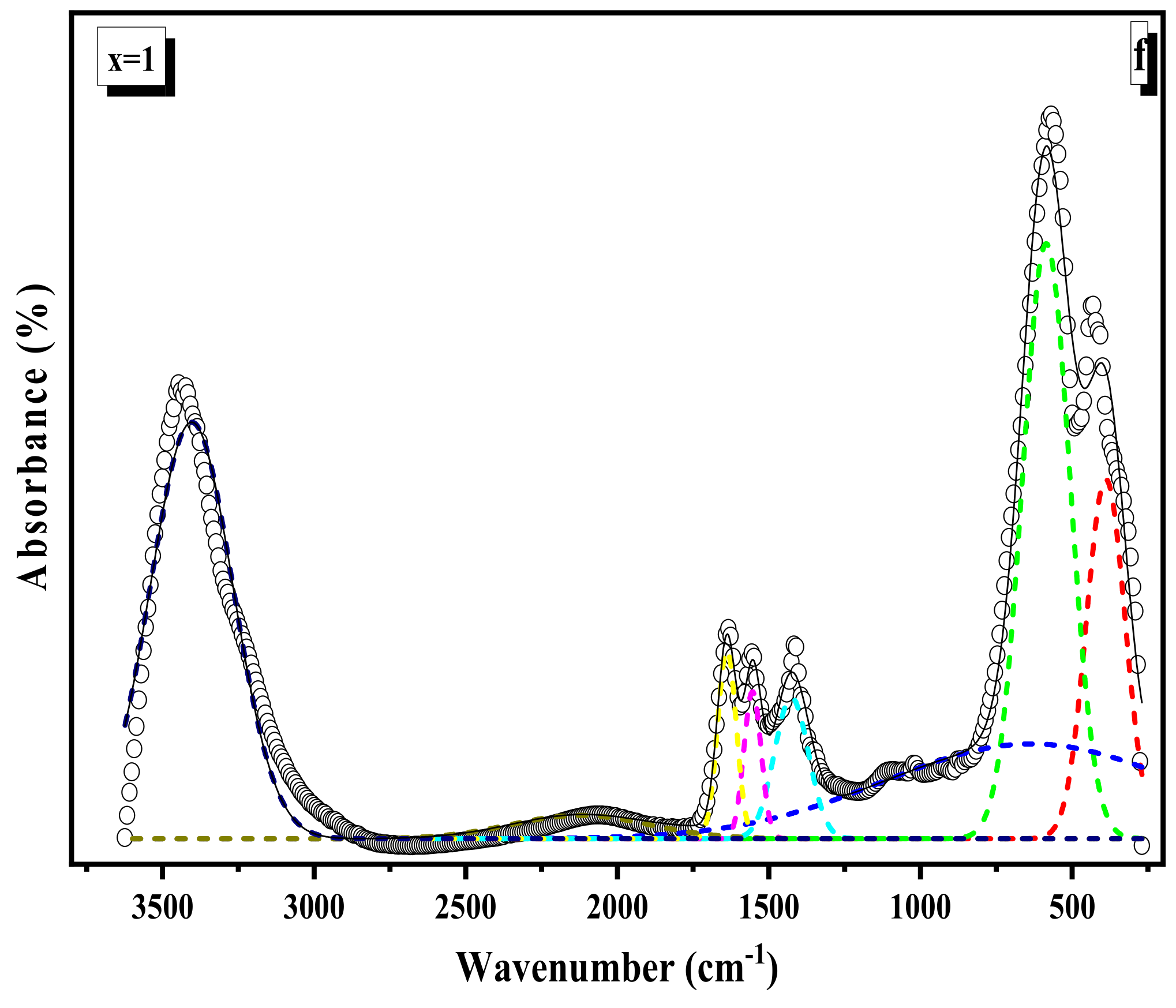
3.4. FTIR Spectroscopy Discussion
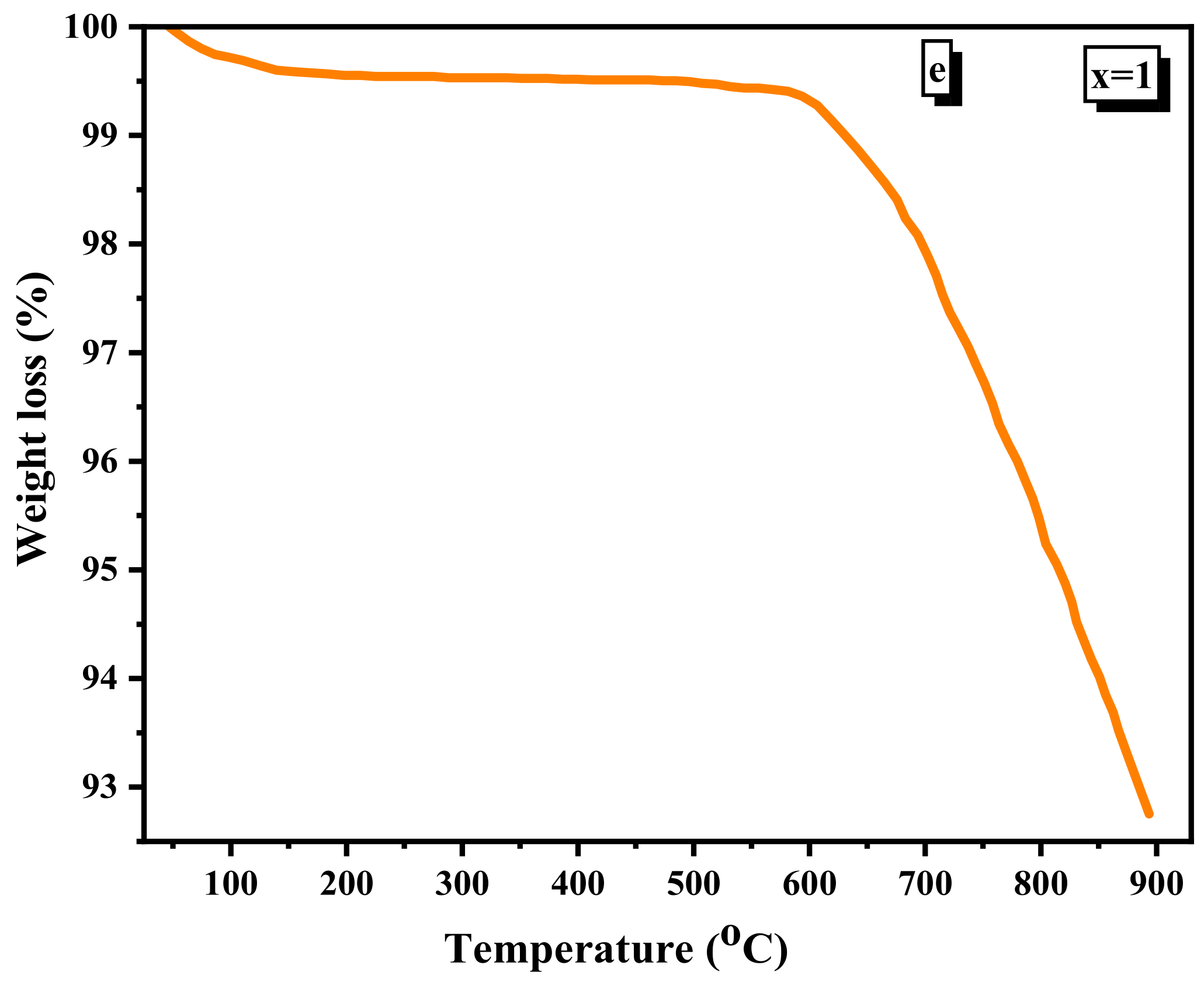
3.5. TGA (Thermogravimetric Analysis) Discussion
3.6. Magnetic Properties of the Prepared Samples
3.6.1. VSM (Vibrating Sample Magnetometer) Discussion
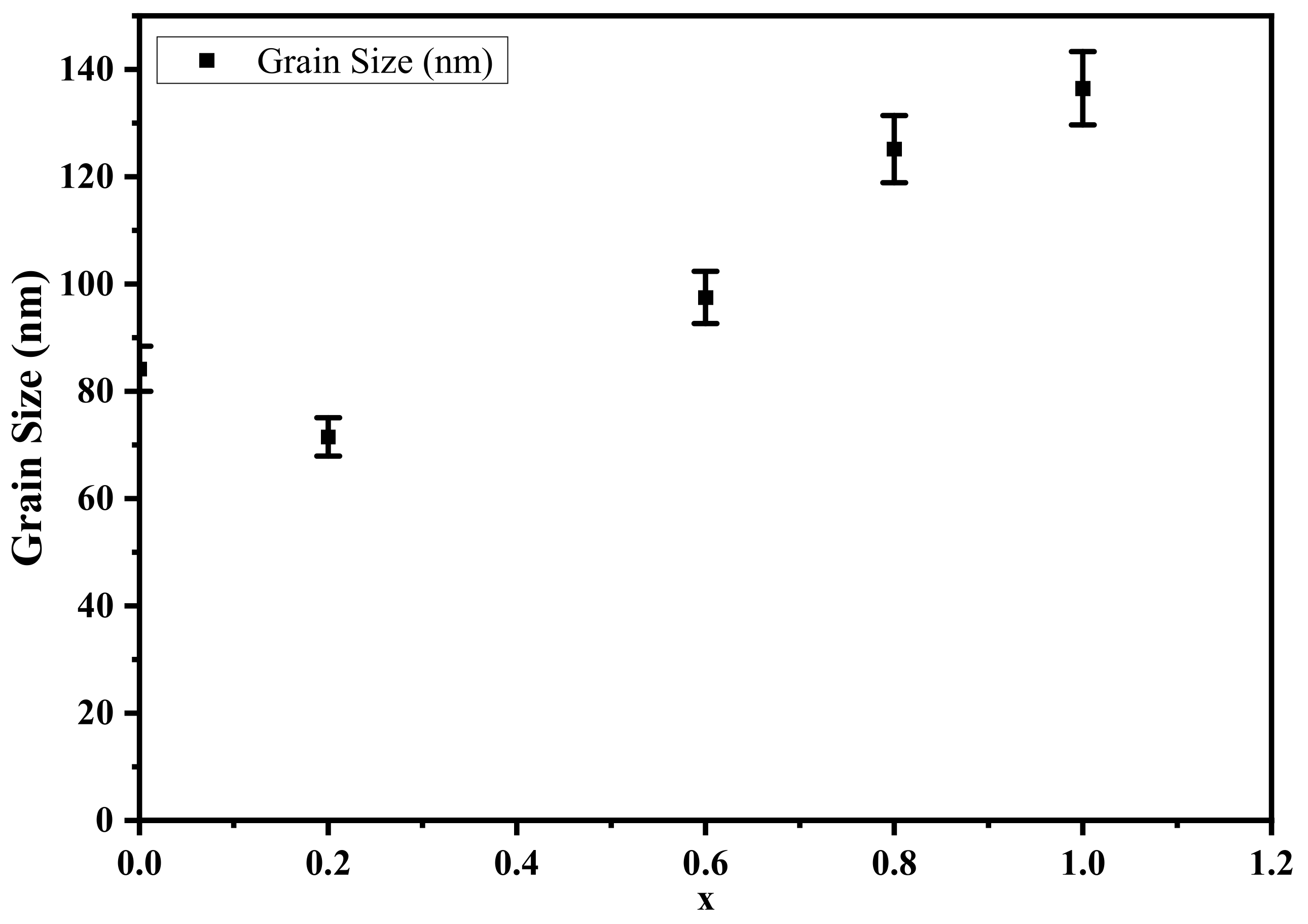
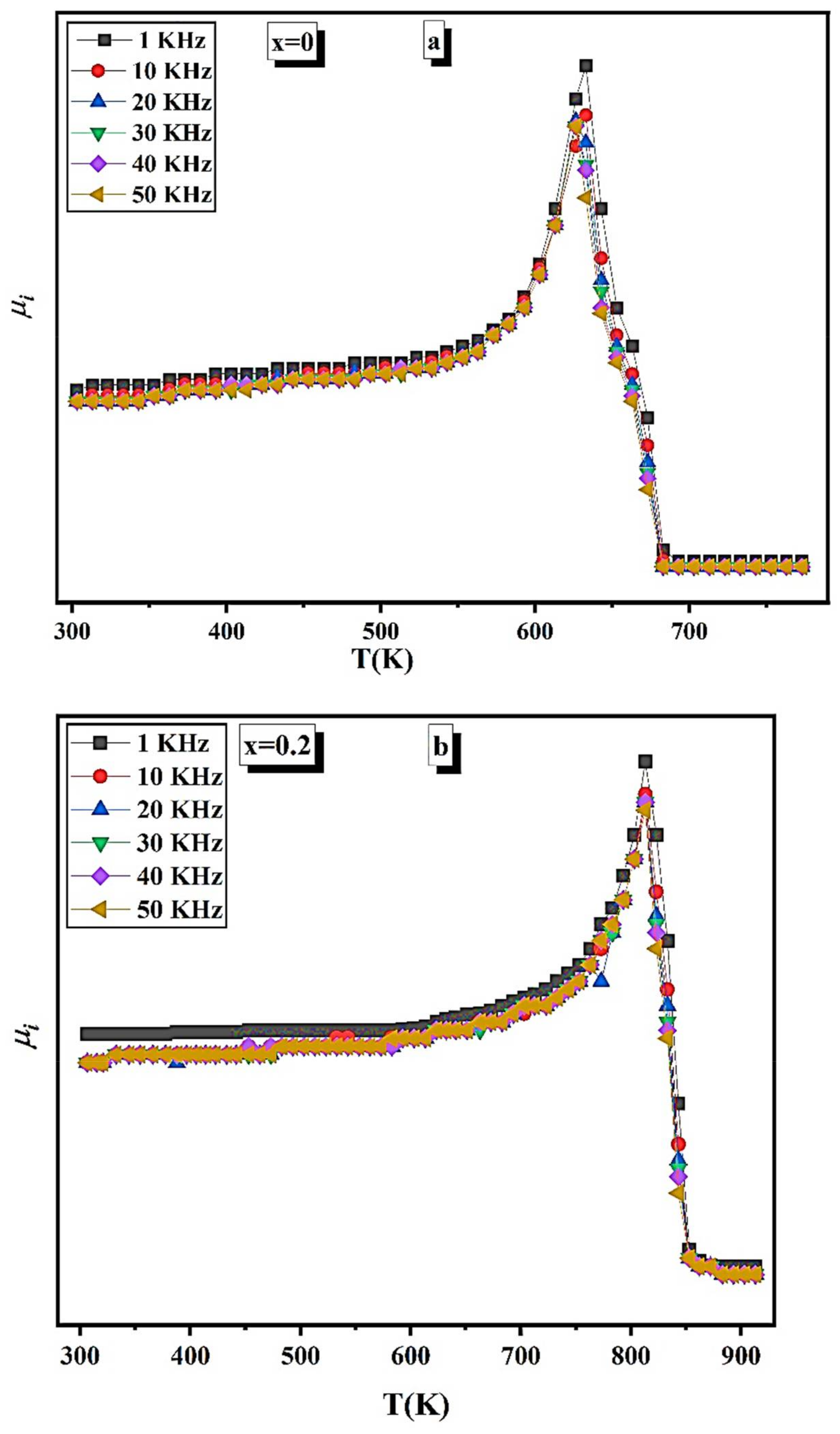
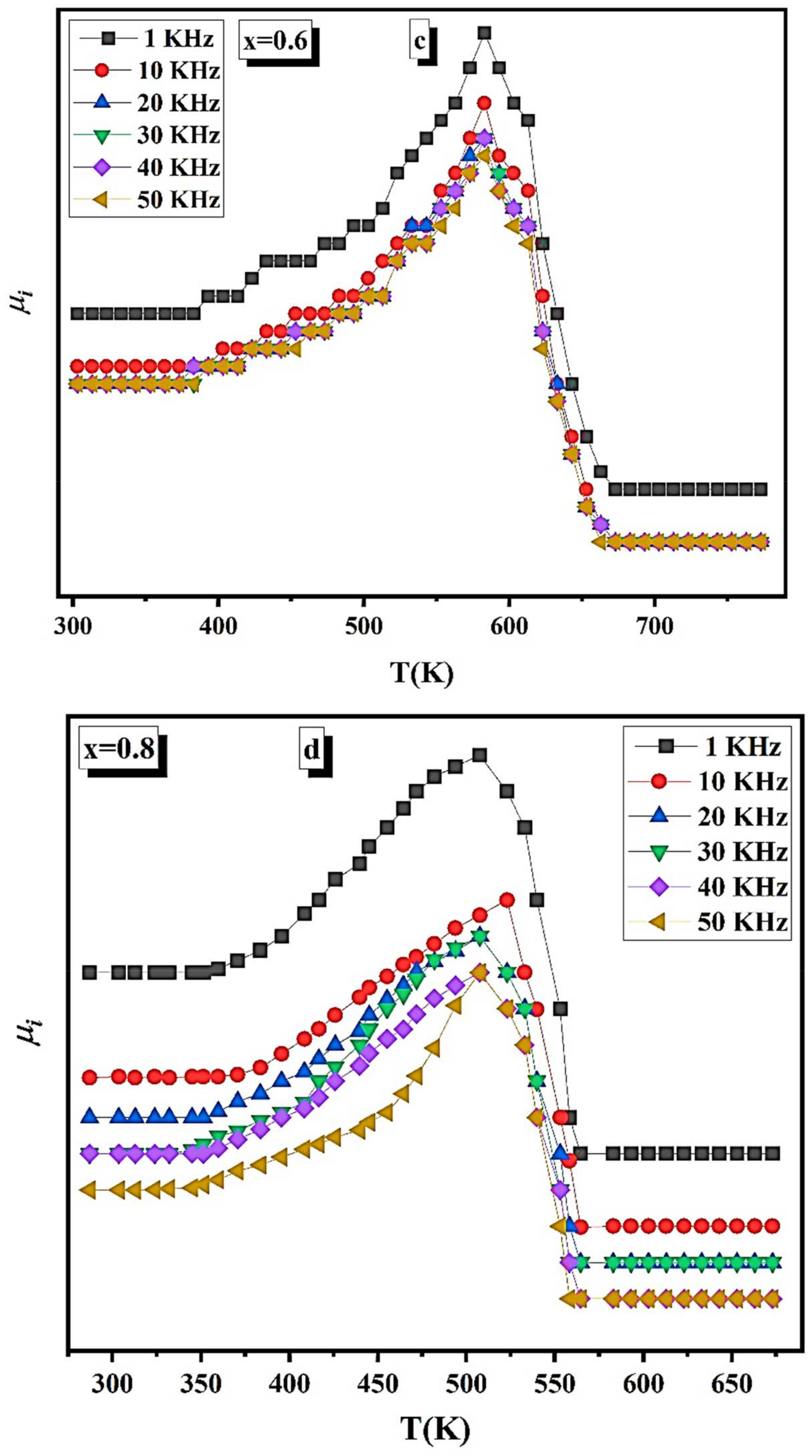
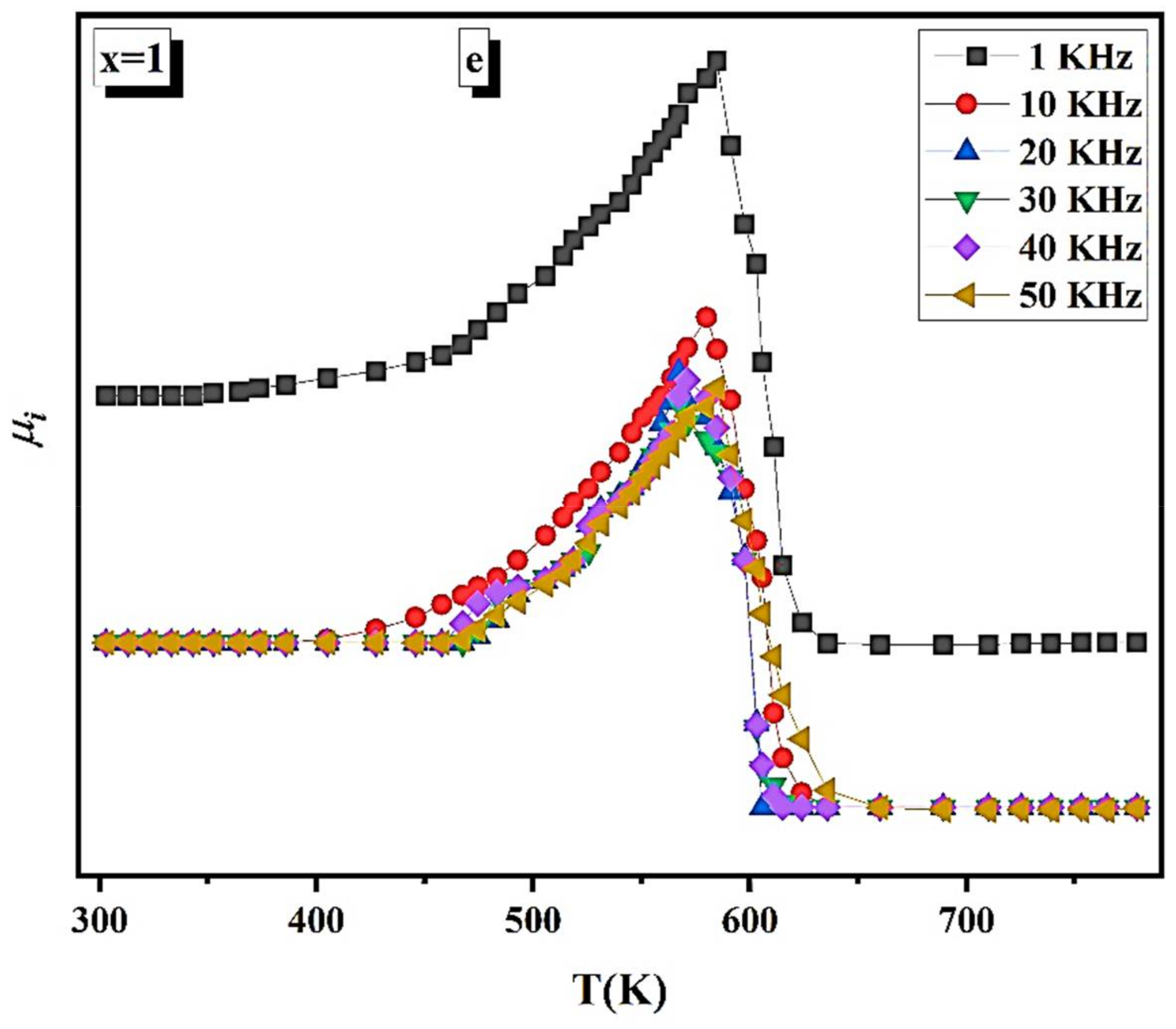
3.6.2. Initial Permeability Discussion
3.7. Electrical Properties of the Prepared Samples
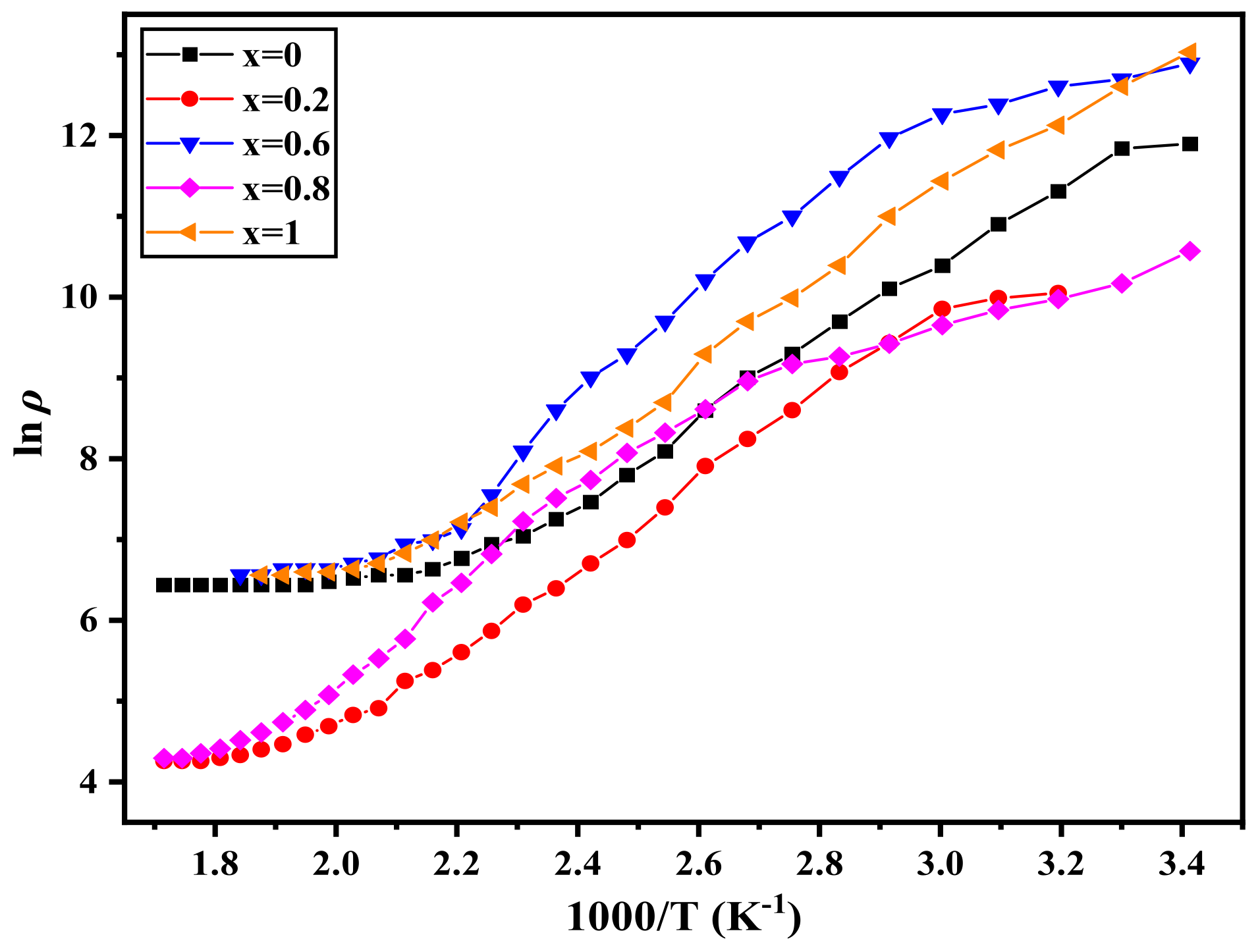
3.7.1. DC Resistivity Discussion
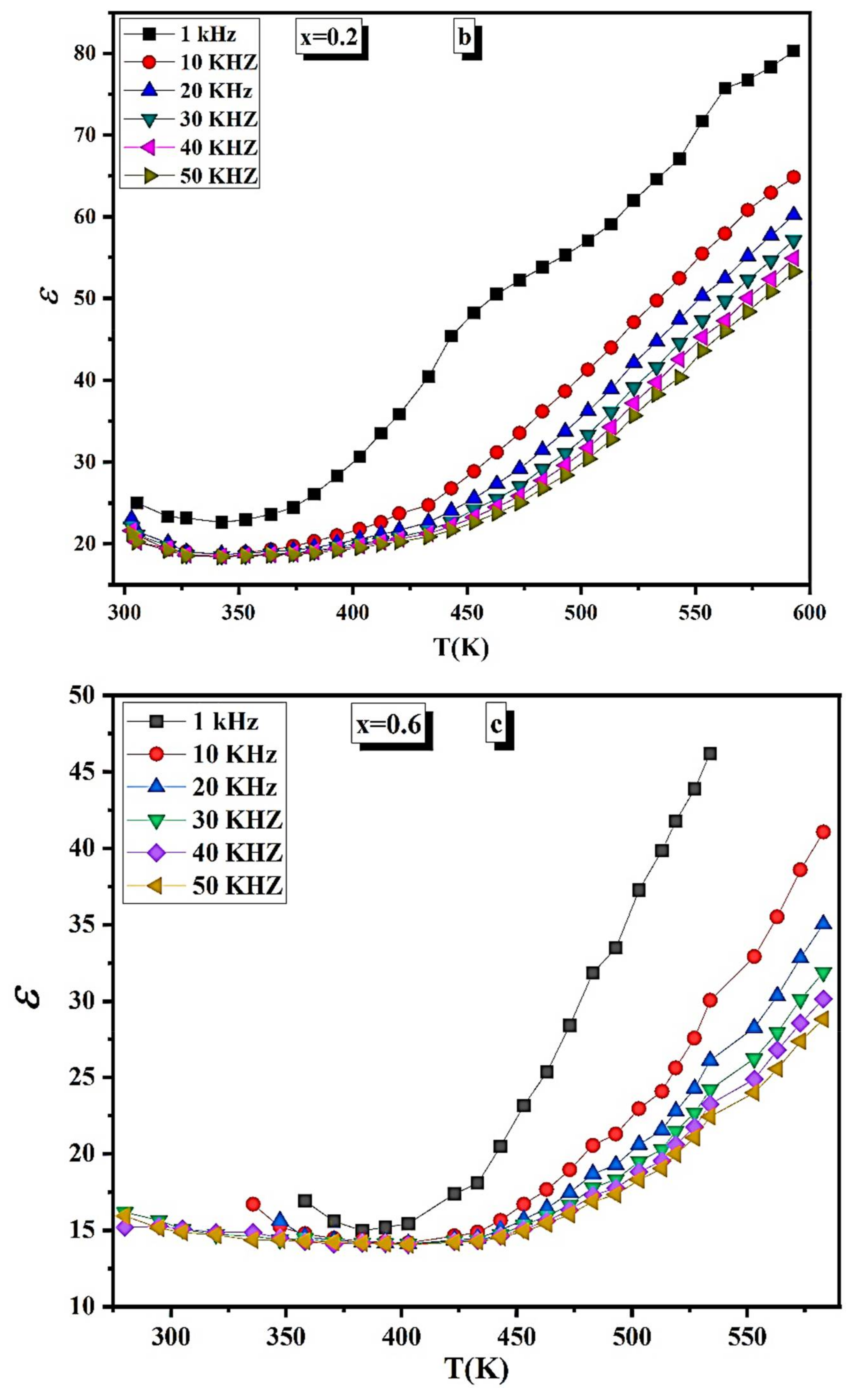
3.7.2. Dielectric Constant (The Real Part of Permittivity) Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Thomas, N.; Jithin, P.V.; Sudheesh, V.D.; Sebastian, V. Magnetic and dielectric properties of magnesium substituted cobalt ferrite samples synthesized via one step calcination free solution combustion method. Ceram. Int. 2017, 43, 7305–7310. [Google Scholar] [CrossRef]
- Sun, L.; Zhang, R.; Ni, Q.; Cao, E.; Hao, W.; Zhang, Y.; Ju, L. Magnetic and dielectric properties of MgxCo1−xFe2O4 ferrites prepared by the sol-gel method. Phys. B Condens. Matter 2018, 545, 4–11. [Google Scholar] [CrossRef]
- Mund, H.S.; Ahuja, B.L. Structural and magnetic properties of Mg doped cobalt ferrite nano particles prepared by sol-gel method. Mater. Res. Bull. 2017, 85, 228–233. [Google Scholar] [CrossRef]
- Deraz, N.M. Glycine-assisted fabrication of nanocrystalline cobalt ferrite system. J. Anal. Appl. Pyrolysis 2010, 88, 103–109. [Google Scholar] [CrossRef]
- Deraz, N.M. Size and crystallinity-dependent magnetic properties of copper ferrite nano-particles. J. Alloys Compd. 2010, 501, 317–325. [Google Scholar] [CrossRef]
- Costa, A.C.F.M.; Silva, V.J.; Cornejo, D.R.; Morelli, M.R.; Kiminami, R.H.G.A.; Gama, L. Magnetic and structural properties of NiFe2O4 ferrite nanopowder doped with Zn2+. J. Magn. Magn. Mater. 2008, 320, e370–e372. [Google Scholar] [CrossRef]
- Kapse, V.D.; Ghosh, S.A.; Raghuwanshi, F.C.; Kapse, S.D. Nanocrystalline spinel Ni0.6Zn0.4Fe2O4: A novel material for H2S sensing. Mater. Chem. Phys. 2009, 113, 638–644. [Google Scholar] [CrossRef]
- Kapse, V.D.; Ghosh, S.A.; Raghuwanshi, F.C.; Kapse, S.D.; Khandekar, U.S. Nanocrystalline Ni0.6Zn0.4Fe2O4: A novel semiconducting material for ethanol detection. Talanta 2009, 78, 19–25. [Google Scholar] [CrossRef] [PubMed]
- Albino, M.; Fantechi, E.; Innocenti, C.; López-Ortega, A.; Bonanni, V.; Campo, G.; Pineider, F.; Gurioli, M.; Arosio, P.; Orlando, T.; et al. Role of Zn2+ Substitution on the Magnetic, Hyperthermic, and Relaxometric Properties of Cobalt Ferrite Nanoparticles. J. Phys. Chem. C 2019, 123, 6148–6157. [Google Scholar] [CrossRef] [Green Version]
- Ukoba, K.O.; Eloka-Eboka, A.C.; Inambao, F.L. Review of nanostructured NiO thin film deposition using the spray pyrolysis technique. Renew. Sustain. Energy Rev. 2018, 82, 2900–2915. [Google Scholar] [CrossRef]
- Hankare, P.P.; Sankpal, U.B.; Patil, R.P.; Mulla, I.S.; Lokhande, P.D.; Gajbhiye, N.S. Synthesis and characterization of CoCrxFe2−xO4 nanoparticles. J. Alloys Compd. 2009, 485, 798–801. [Google Scholar] [CrossRef]
- Giri, S.; Samanta, S.; Maji, S.; Ganguli, S.; Bhaumik, A. Magnetic properties of α-Fe2O3 nanoparticle synthesized by a new hydrothermal method. J. Magn. Magn. Mater. 2005, 285, 296–302. [Google Scholar] [CrossRef]
- George, M.; Mary John, A.; Nair, S.S.; Joy, P.A.; Anantharaman, M.R. Finite size effects on the structural and magnetic properties of sol–gel synthesized NiFe2O4 powders. J. Magn. Magn. Mater. 2006, 302, 190–195. [Google Scholar] [CrossRef]
- Singjai, P.; Wongwigkarn, K.; Laosiritaworn, Y.; Yimnirun, R.; Maensiri, S. Carbon encapsulated nickel nanoparticles synthesized by a modified alcohol catalytic chemical vapor deposition method. Curr. Appl. Phys. 2007, 7, 662–666. [Google Scholar] [CrossRef]
- Krishnakanth, R.; Jayakumar, G.; Albert Irudayaraj, A.; Dhayal Raj, A. Structural and Magnetic Properties of NiO and Fe-doped NiO Nanoparticles Synthesized by Chemical Co-precipitation Method. Mater. Today Proc. 2016, 3, 1370–1377. [Google Scholar] [CrossRef]
- Hammad, T.M.; Salem, J.K.; Amsha, A.A.; Hejazy, N.K. Optical and magnetic characterizations of zinc substituted copper ferrite synthesized by a co-precipitation chemical method. J. Alloys Compd. 2018, 741, 123–130. [Google Scholar] [CrossRef]
- Hemeda, O.M.; Tawfik, A.; Mostafa, M.; Zaki, M.; Abd El Ati, M.I. Structural and Magnetic Properties of Nano Ferrite for Magnetoelectric Applications. J. Phys. Conf. Ser. 2019, 1253, 12026. [Google Scholar] [CrossRef]
- Jalaly, M.; Enayati, M.H.; Karimzadeh, F. Investigation of structural and magnetic properties of nanocrystalline Ni0.3Zn0.7Fe2O4 prepared by high energy ball milling. J. Alloys Compd. 2009, 480, 737–740. [Google Scholar] [CrossRef]
- Deraz, N.M.; Hessien, M.M. Structural and magnetic properties of pure and doped nanocrystalline cadmium ferrite. J. Alloys Compd. 2009, 475, 832–839. [Google Scholar] [CrossRef]
- Li, S.; Qin, J.; Fornara, A.; Toprak, M.; Muhammed, M.; Kim, D.K. Synthesis and magnetic properties of bulk transparent PMMA/Fe-oxide nanocomposites. Nanotechnology 2009, 20, 185607. [Google Scholar] [CrossRef]
- Angermann, A.; Töpfer, J. Synthesis of nanocrystalline Mn–Zn ferrite powders through thermolysis of mixed oxalates. Ceram. Int. 2011, 37, 995–1002. [Google Scholar] [CrossRef]
- Ozkaya, T.; Toprak, M.S.; Baykal, A.; Kavas, H.; Köseoğlu, Y.; Aktaş, B. Synthesis of Fe3O4 nanoparticles at 100 °C and its magnetic characterization. J. Alloys Compd. 2009, 472, 18–23. [Google Scholar] [CrossRef]
- Gözüak, F.; Köseoğlu, Y.; Baykal, A.; Kavas, H. Synthesis and characterization of CoxZn1−xFe2O4 magnetic nanoparticles via a PEG-assisted route. J. Magn. Magn. Mater. 2009, 321, 2170–2177. [Google Scholar] [CrossRef]
- Rezlescu, E.; Rezlescu, N.; Popa, P.D.; Rezlescu, L.; Pasnicu, C.; Craus, M.L. Effect of Copper Oxide Content on Intrinsic Properties of MgCuZn Ferrite. Mater. Res. Bull. 1998, 33, 915–925. [Google Scholar] [CrossRef]
- Sertkol, M.; Köseoğlu, Y.; Baykal, A.; Kavas, H.; Bozkurt, A.; Toprak, M.S. Microwave synthesis and characterization of Zn-doped nickel ferrite nanoparticles. J. Alloys Compd. 2009, 486, 325–329. [Google Scholar] [CrossRef]
- Kumar, S.; Singh, R.R.; Barman, P.B. Reitveld Refinement and Derivative Spectroscopy of Nanoparticles of Soft Ferrites (MgNiFe). J. Inorg. Organomet. Polym. Mater. 2021, 31, 528–541. [Google Scholar] [CrossRef]
- Shobana, M.K.; Kim, K.; Kim, J.-H. Impact of magnesium substitution in nickel ferrite: Optical and electrochemical studies. Phys. E Low-Dimens. Syst. Nanostructures 2019, 108, 100–104. [Google Scholar] [CrossRef]
- Ravinder, D.; Hashim, M.; Upadhyay, A.; Ismail, M.M.; Kumar, S.; Kumar, R.; Meena, S.S.; Khalilullah, A. Investigation of structural and magnetic properties of La doped Co–Mn ferrite nanoparticles in the presence of α-Fe2O3 phase. Solid State Commun. 2022, 342, 114629. [Google Scholar] [CrossRef]
- Anumol, C.N.; Chithra, M.; Rout, S.; Sahoo, S.C. Effect of Magnesium Substitution on Structural and Magnetic Properties of Nickel Ferrite Nanoparticles. J. Supercond. Nov. Magn. 2020, 33, 1611–1617. [Google Scholar] [CrossRef]
- Rostami, M.; Majles Ara, M.H. The dielectric, magnetic and microwave absorption properties of Cu-substituted Mg-Ni spinel ferrite-MWCNT nanocomposites. Ceram. Int. 2019, 45, 7606–7613. [Google Scholar] [CrossRef]
- Boon, M.; Rickard, W.D.A.; Rohl, A.L.; Jones, F. Stabilization of Aragonite: Role of Mg2+ and Other Impurity Ions. Cryst. Growth Des. 2020, 20, 5006–5017. [Google Scholar] [CrossRef]
- Gabal, M.A.; Al Angari, Y.M.; Zaki, H.M. Structural, magnetic and electrical characterization of Mg–Ni nano-crystalline ferrites prepared through egg-white precursor. J. Magn. Magn. Mater. 2014, 363, 6–12. [Google Scholar] [CrossRef]
- Raghuvanshi, S.; Tiwari, P.; Kane, S.N.; Avasthi, D.K.; Mazaleyrat, F.; Tatarchuk, T.; Mironyuk, I. Dual control on structure and magnetic properties of Mg ferrite: Role of swift heavy ion irradiation. J. Magn. Magn. Mater. 2019, 471, 521–528. [Google Scholar] [CrossRef]
- Chandra Babu Naidu, K.; RoopasKiran, S.; Madhuri, W. Investigations on transport, impedance and electromagnetic interference shielding properties of microwave processed NiMg ferrites. Mater. Res. Bull. 2017, 89, 125–138. [Google Scholar] [CrossRef]
- Naeem, M.; Shah, N.A.; Gul, I.H.; Maqsood, A. Structural, electrical and magnetic characterization of Ni–Mg spinel ferrites. J. Alloys Compd. 2009, 487, 739–743. [Google Scholar] [CrossRef]
- Mazen, S.; Abu-Elsaad, N.I.; Nawara, A.S. The Influence of Various Divalent Metal Ions (Mn2+, Co2+, and Cu2+) Substitution on the Structural and Magnetic Properties of Nickel–Zinc Spinel Ferrite. Phys. Solid State 2020, 62, 1183–1194. [Google Scholar] [CrossRef]
- Debnath, S.; Das, R. Study of the optical properties of Zn doped Mn spinel ferrite nanocrystals shows multiple emission peaks in the visible range—A promising soft ferrite nanomaterial for deep blue LED. J. Mol. Struct. 2020, 1199, 127044. [Google Scholar] [CrossRef]
- Sharma, R.; Thakur, P.; Sharma, P.; Sharma, V. Ferrimagnetic Ni2+ doped Mg-Zn spinel ferrite nanoparticles for high density information storage. J. Alloys Compd. 2017, 704, 7–17. [Google Scholar] [CrossRef]
- Akhtar, M.N.; Khan, M.A.; Ahmad, M.; Nazir, M.S.; Imran, M.; Ali, A.; Sattar, A.; Murtaza, G. Evaluation of structural, morphological and magnetic properties of CuZnNi (CuxZn0.5−xNi0.5Fe2O4) nanocrystalline ferrites for core, switching and MLCI’s applications. J. Magn. Magn. Mater. 2017, 421, 260–268. [Google Scholar] [CrossRef]
- Deepty, M.; Srinivas, C.; Kumar, E.R.; Mohan, N.K.; Prajapat, C.L.; Rao, T.V.C.; Meena, S.S.; Verma, A.K.; Sastry, D.L. XRD, EDX, FTIR and ESR spectroscopic studies of co-precipitated Mn–substituted Zn–ferrite nanoparticles. Ceram. Int. 2019, 45, 8037–8044. [Google Scholar] [CrossRef]
- Moradmard, H.; Farjami Shayesteh, S.; Tohidi, P.; Abbas, Z.; Khaleghi, M. Structural, magnetic and dielectric properties of magnesium doped nickel ferrite nanoparticles. J. Alloys Compd. 2015, 650, 116–122. [Google Scholar] [CrossRef]
- El Maalam, K.; Ben Ali, M.; El Moussaoui, H.; Mounkachi, O.; Hamedoun, M.; Masrour, R.; Hlil, E.K.; Benyoussef, A. Magnetic properties of tin ferrites nanostructures doped with transition metal. J. Alloys Compd. 2015, 622, 761–764. [Google Scholar] [CrossRef]
- Noor, A.; Akhtar, M.N.; Khan, S.N.; Nazir, M.S.; Yousaf, M. Synthesis, morphological and electromagnetic evaluations of Ca doped Mn spinel nanoferrites for GHz regime applications. Ceram. Int. 2020, 46, 13961–13968. [Google Scholar] [CrossRef]
- Henaish, A.M.A.; Mostafa, M.; Salem, B.I.; Hemeda, O.M. Improvement of magnetic and dielectric properties of magnetoelectric BST-NCZMF nano-composite. Phase Transit. 2020, 93, 470–490. [Google Scholar] [CrossRef]
- Chandramouli, K.; Rao, P.A.; Suryanarayana, B.; Raghavendra, V.; Mercy, S.J.; Parajuli, D.; Taddesse, P.; Mulushoa, S.Y.; Mammo, T.W.; Murali, N. Effect of Cu substitution on magnetic and DC electrical resistivity properties of Ni–Zn nanoferrites. J. Mater. Sci. Mater. Electron. 2021, 32, 15754–15762. [Google Scholar] [CrossRef]
- Chandramouli, K.; Suryanarayana, B.; Phanidhar Varma, P.V.S.K.; Raghavendra, V.; Emmanuel, K.A.; Taddesse, P.; Murali, N.; Wegayehu Mammo, T.; Parajuli, D. Effect of Cr3+ substitution on dc electrical resistivity and magnetic properties of Cu0.7Co0.3Fe2−xCrxO4 ferrite nanoparticles prepared by sol-gel auto combustion method. Results Phys. 2021, 24, 104117. [Google Scholar] [CrossRef]
- Zhivulin, V.E.; Trofimov, E.A.; Gudkova, S.A.; Pashkeev, I.Y.; Punda, A.Y.; Gavrilyak, M.; Zaitseva, O.V.; Taskaev, S.V.; Podgornov, F.V.; Darwish, M.A.; et al. Polysubstituted High-Entropy [LaNd](Cr0.2Mn0.2Fe0.2Co0.2Ni0.2)O3 Perovskites: Correlation of the Electrical and Magnetic Properties. Nanomaterials 2021, 11, 1014. [Google Scholar] [CrossRef]
- Verwey, E.J.W.; De Boer, J.H. Cation arrangement in a few oxides with crystal structures of the spinel type. Recl. Trav. Chim. Pays-Bas 1936, 55, 531–540. [Google Scholar] [CrossRef]
- Javed Iqbal, M.; Rukh Siddiquah, M. Structural, electrical and magnetic properties of Zr–Mg cobalt ferrite. J. Magn. Magn. Mater. 2008, 320, 845–850. [Google Scholar] [CrossRef]
- Fawzi, A.S.; Sheikh, A.D.; Mathe, V.L. Structural, dielectric properties and AC conductivity of Ni(1−x)ZnxFe2O4 spinel ferrites. J. Alloys Compd. 2010, 502, 231–237. [Google Scholar] [CrossRef]
- Darwish, M.A.; Trukhanov, A.V.; Senatov, O.S.; Morchenko, A.T.; Saafan, S.A.; Astapovich, K.A.; Trukhanov, S.V.; Trukhanova, E.L.; Pilyushkin, A.A.; Sombra, A.S.B.; et al. Investigation of AC-Measurements of Epoxy/Ferrite Composites. Nanomaterials 2020, 10, 492. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Boiko, O.; Koltunowicz, T.N.; Zukowski, P.; Fedotov, A.K.; Larkin, A.V. The effect of sputtering atmosphere parameters on dielectric properties of the ferromagnetic alloy–ferroelectric ceramics nanocomposite (FeCoZr)x(PbZrTiO3)(100−x). Ceram. Int. 2017, 43, 2511–2516. [Google Scholar] [CrossRef]
- Czarnacka, K.; Koltunowicz, T.N.; Zukowski, P.; Fedotov, A.K. Dielectric properties of multi-layer nanocomposites SiOx/ZrO2 after high temperature annealing. Ceram. Int. 2019, 45, 6499–6502. [Google Scholar] [CrossRef]
- Zukowski, P.; Koltunowicz, T.N.; Czarnacka, K.; Fedotov, A.K.; Tyschenko, I.E. Carrier transport and dielectric permittivity of SiO2 films containing ion-beam synthesized InSb nanocrystals. J. Alloys Compd. 2020, 846, 156482. [Google Scholar] [CrossRef]








| x | Cation Distribution | (Å) | (Å) | u (Å) | δ (Å) | Bond Length (Å) | |||
|---|---|---|---|---|---|---|---|---|---|
| A-Site | B-Site | ||||||||
| 0 | ( | 0.64 | 0.6650 | 0.3861 | 0.0111 | 1.9599 | 1.988 | 2.00 | |
| 0.2 | 0.6403 | 0.6670 | 0.3860 | 0.0110 | 1.9596 | 1.992 | 1.64 | ||
| 0.6 | 0.6409 | 0.6730 | 0.3858 | 0.0108 | 1.9609 | 1.998 | 0.92 | ||
| 0.8 | 0.6413 | 0.6760 | 0.3857 | 0.0107 | 1.9612 | 2.000 | 0.56 | ||
| 1 | 0.6416 | 0.6790 | 0.3856 | 0.0106 | 1.9615 | 2.011 | 0.2 | ||
| x | D (nm) | (g/cm3) | (g/cm3) | P (%) | D (nm) | (Å) | |
|---|---|---|---|---|---|---|---|
| XRD | HRTEM | ||||||
| 0 | 21 | 5.359 | 3.759 | 29.85 | 20.92 | 8.310 | 2.00 |
| 0.2 | 19 | 5.126 | 3.621 | 29.36 | 17.64 | 8.319 | 1.64 |
| 0.6 | 14 | 4.825 | 3.357 | 30.42 | 13.79 | 8.335 | 0.92 |
| 0.8 | 12 | 4.723 | 3.158 | 33.13 | 14.62 | 8.343 | 0.56 |
| 1 | 17 | 4.586 | 3.161 | 31.07 | 17.70 | 8.351 | 0.20 |
| x | Average of Pores Size (μm) |
|---|---|
| 0 | 0.23 |
| 0.2 | 0.07 |
| 0.6 | 0.15 |
| 0.8 | 0.144 |
| 1 | 0.09 |
| x | ν1 | ν2 | Ftetra (dyne/cm) × 105 | Focta (dyne/cm) × 105 | |
|---|---|---|---|---|---|
| 0 | 589 | 405 | 1.9599 | 2.54 | 1.20 |
| 0.2 | 580 | 382 | 1.9596 | 2.46 | 1.07 |
| 0.6 | 576 | 396 | 1.9609 | 2.43 | 1.15 |
| 0.8 | 574 | 403 | 1.9612 | 2.41 | 1.19 |
| 1 | 571 | 434 | 1.9615 | 2.39 | 1.38 |
| x = 0 | x = 0.2 | x = 0.6 | x = 0.8 | x = 1 | ||
|---|---|---|---|---|---|---|
| I | C | 393 | 358 | 376 | 403 | 389 |
| A | 19.00 | 22.00 | 17.00 | 12.00 | 19.00 | |
| II | C | 574 | 579 | 588 | 576 | 585 |
| A | 16.00 | 29.00 | 34.00 | 42.00 | 28.00 | |
| III | C | 1068 | 1099 | 1090 | 1112 | 974 |
| A | 0.77 | 1.30 | 1.60 | 2.30 | 1.50 | |
| IV | C | 1271 | 1279 | 1269 | - | - |
| A | 1.30 | 25.00 | 5.00 | - | - | |
| V | C | 1401 | 1412 | 1399 | 1411 | 1425 |
| A | 4.40 | 3.70 | 2.30 | 4.10 | 19.00 | |
| VI | C | 1554 | 1543 | 1599 | 1552 | 1554 |
| A | 4.20 | 13.00 | 25.00 | 2.10 | 22.00 | |
| VII | C | 1634 | 1627 | - | 1629 | 1638 |
| A | 1.20 | 2.50 | - | 3.00 | 4.00 | |
| VIII | C | 2105 | 2021 | 2111 | 2064 | 2123 |
| A | 24.60 | 10.00 | 8.30 | 3.00 | 2.70 | |
| IX | C | 2996 | 2346 | 2559 | 2911 | - |
| A | 18.90 | 9.00 | 5.10 | 2.60 | - | |
| X | C | 3433 | 3380 | 2981 | 3372 | 3403 |
| A | 9.60 | 8.00 | 1.90 | 29.00 | 12.00 | |
| x | Ms (emu/g) | Hc (Oe) | Mr (emu/g) | µexp (µB) | µth (µB) |
|---|---|---|---|---|---|
| 0 | 28.385 | 192.60 | 6.72 | 1.1912 | 2.00 |
| 0.2 | 29.256 | 178.96 | 6.39 | 1.1917 | 1.64 |
| 0.6 | 16.252 | 111.73 | 2.22 | 0.6219 | 0.92 |
| 0.8 | 10.527 | 95.462 | 0.96 | 0.3899 | 0.56 |
| 1 | 9.0194 | 88.344 | 0.58 | 0.3229 | 0.2 |
| x | TC (K) | Permeability Change Rate dµi/dT, (K−1) | Grain Size (D), (nm) |
|---|---|---|---|
| 0 | 633 | 2.243 | 84 |
| 0.2 | 813 | 1.740 | 71 |
| 0.6 | 584 | 0.977 | 97 |
| 0.8 | 513 | 0.514 | 125 |
| 1 | 614 | 0.447 | 136 |
| x | TC (K) | Ep (eV) | Ef (eV) |
|---|---|---|---|
| 0 | 656 | 0.27 | 0.39 |
| 0.2 | 666 | 0.40 | 0.43 |
| 0.6 | 686 | 0.53 | 0.40 |
| 0.8 | 705 | 0.51 | 0.19 |
| 1 | 666 | 0.41 | 0.34 |
| x | 0 | 0.2 | 0.6 | 0.8 | 1 |
|---|---|---|---|---|---|
| ε | 40.42 | 40.93 | 22.71 | 24.16 | 33.74 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mostafa, M.; Saleh, O.; Henaish, A.M.; Abd El-Kaream, S.A.; Ghazy, R.; Hemeda, O.M.; Dorgham, A.M.; Al-Ghamdi, H.; Almuqrin, A.H.; Sayyed, M.I.; et al. Structure, Morphology and Electrical/Magnetic Properties of Ni-Mg Nano-Ferrites from a New Perspective. Nanomaterials 2022, 12, 1045. https://doi.org/10.3390/nano12071045
Mostafa M, Saleh O, Henaish AM, Abd El-Kaream SA, Ghazy R, Hemeda OM, Dorgham AM, Al-Ghamdi H, Almuqrin AH, Sayyed MI, et al. Structure, Morphology and Electrical/Magnetic Properties of Ni-Mg Nano-Ferrites from a New Perspective. Nanomaterials. 2022; 12(7):1045. https://doi.org/10.3390/nano12071045
Chicago/Turabian StyleMostafa, Maha, Oday Saleh, Ahmed Maher Henaish, Samir Ali Abd El-Kaream, Ryad Ghazy, Osama M. Hemeda, Ali M. Dorgham, Hanan Al-Ghamdi, Aljawhara H. Almuqrin, M. I. Sayyed, and et al. 2022. "Structure, Morphology and Electrical/Magnetic Properties of Ni-Mg Nano-Ferrites from a New Perspective" Nanomaterials 12, no. 7: 1045. https://doi.org/10.3390/nano12071045
APA StyleMostafa, M., Saleh, O., Henaish, A. M., Abd El-Kaream, S. A., Ghazy, R., Hemeda, O. M., Dorgham, A. M., Al-Ghamdi, H., Almuqrin, A. H., Sayyed, M. I., Trukhanov, S. V., Trukhanova, E. L., Trukhanov, A. V., Zhou, D., & Darwish, M. A. (2022). Structure, Morphology and Electrical/Magnetic Properties of Ni-Mg Nano-Ferrites from a New Perspective. Nanomaterials, 12(7), 1045. https://doi.org/10.3390/nano12071045









