Topological Refraction in Kagome Split-Ring Photonic Insulators
Abstract
:1. Introduction
2. Model and Calculation Method
2.1. Model
2.2. Valley Chern Number
3. Results
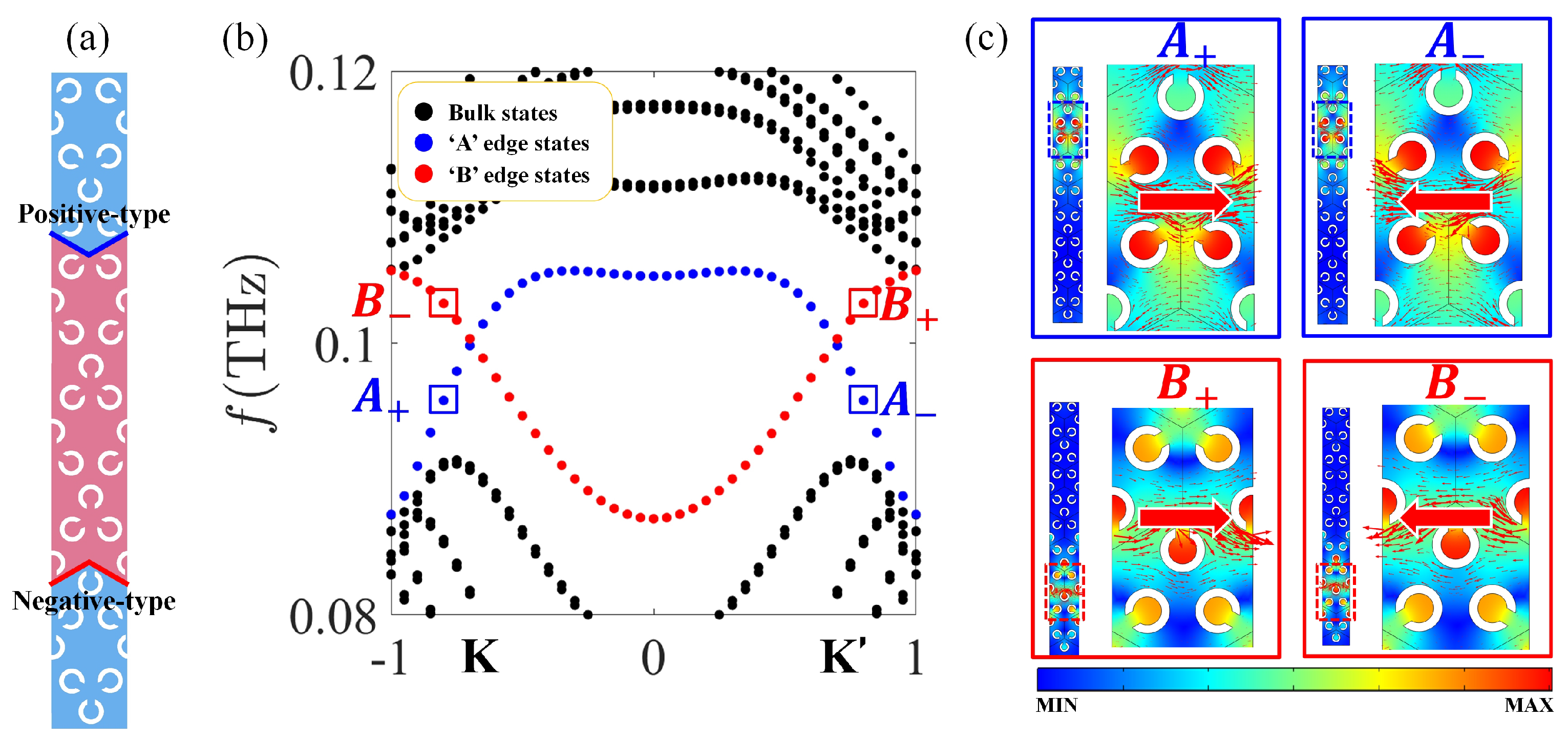
3.1. Band Inversion of Topological Valley-Hall-like States
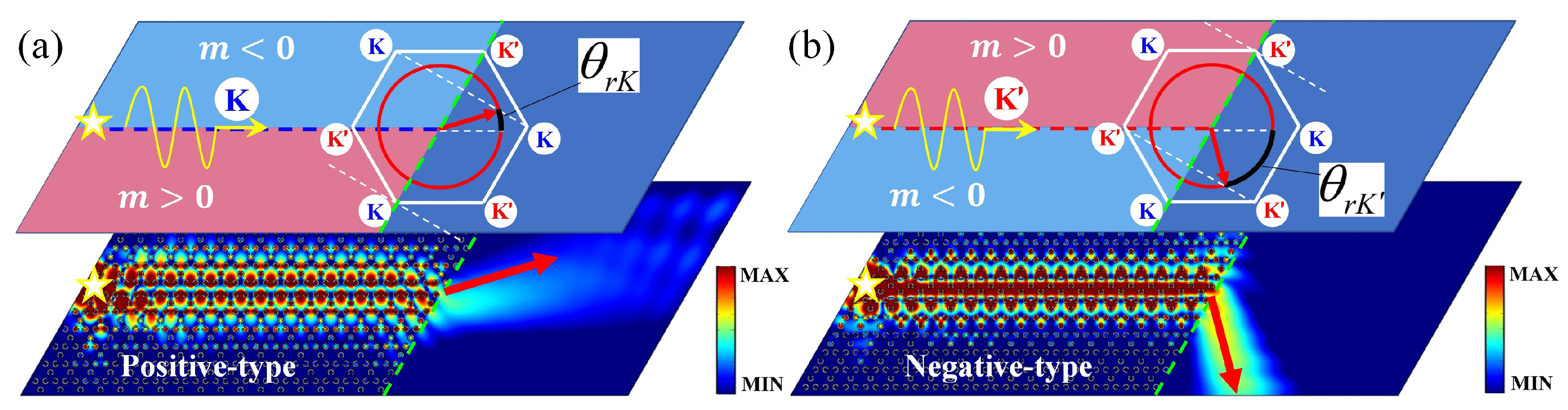
3.2. Valley Topological Refraction
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A

Appendix B

Appendix C

References
- Rycerz, A.; Tworzydło, J.; Beenakker, C.W.J. Valley filter and valley valve in graphene. Nat. Phys. 2007, 3, 172–175. [Google Scholar] [CrossRef] [Green Version]
- Xiao, D.; Yao, W.; Niu, Q. Valley-contrasting physics in graphene: Magnetic moment and topological transport. Phys. Rev. Lett. 2007, 99, 236809. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Garcia-Pomar, J.L.; Cortijo, A.; Nieto-Vesperinas, M. Fully valley-polarized electron beams in graphene. Phys. Rev. Lett. 2008, 100, 236801. [Google Scholar] [CrossRef] [PubMed]
- Hafezi, M.; Demler, E.A.; Lukin, M.D.; Taylor, J.M. Robust optical delay lines with topological protection. Nat. Phys. 2011, 7, 907–912. [Google Scholar] [CrossRef]
- Lai, K.; Ma, T.; Bo, X.; Anlage, S.; Shvets, G. Experimental realization of a reflections-free compact delay line based on a photonic topological insulator. Sci. Rep. 2016, 6, 28453. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.W.; Tian, Y.; Cheng, Y.; Wei, Q.; Liu, X.J.; Christensen, J. Topological acoustic delay line. Phys. Rev. Appl. 2018, 9, 034032. [Google Scholar] [CrossRef] [Green Version]
- Kang, Y.H.; Ni, X.; Cheng, X.J.; Khanikaev, A.B.; Genack, A.Z. Pseudo-spin–valley coupled edge states in a photonic topological insulator. Nat. Commun. 2018, 9, 3029. [Google Scholar] [CrossRef] [Green Version]
- Haldane, F.D.M.; Raghu, S. Possible realization of directional optical waveguides in photonic crystals with broken time-reversal symmetry. Phys. Rev. Lett. 2008, 100, 013904. [Google Scholar] [CrossRef] [Green Version]
- Lu, L.; Joannopoulos, J.D.; Soljačić, M. Topological photonics. Nat. Photonics 2014, 8, 821–829. [Google Scholar] [CrossRef] [Green Version]
- Khanikaev, A.B.; Fleury, R.; Mousavi, S.H.; Alu, A. Topologically robust sound propagation in an angular-momentum-biased graphene-like resonator lattice. Nat. Commun. 2015, 6, 8260. [Google Scholar] [CrossRef]
- Wang, P.; Lu, L.; Bertoldi, K. Topological phononic crystals with one-way elastic edge waves. Phys. Rev. Lett. 2015, 115, 104302. [Google Scholar] [CrossRef] [PubMed]
- Nash, L.M.; Kleckner, D.; Read, A.; Vitelli, V.; Turner, A.M.; Irvine, W.T.M. Topological mechanics of gyroscopic metamaterials. Proc. Natl. Acad. Sci. USA 2015, 112, 14495. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yang, Z.; Gao, F.; Shi, X.; Lin, X.; Gao, Z.; Chong, Y.; Zhang, B. Topological Acoustics. Phys. Rev. Lett. 2015, 114, 114301. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Peng, Y.; Zhu, Y.; Fan, X.; Yang, J.; Liang, B.; Zhu, X.; Wan, X.; Cheng, J. Experimental demonstration of acoustic chern insulators. Phys. Rev. Lett. 2019, 122, 014302. [Google Scholar] [CrossRef] [PubMed]
- Mousavi, S.H.; Khanikaev, A.B.; Wang, Z. Topologically protected elastic waves in phononic metamaterials. Nat. Commun. 2015, 6, 8682. [Google Scholar] [CrossRef]
- Mei, J.; Chen, Z.; Wu, Y. Pseudo-time-reversal symmetry and topological edge states in two-dimensional acoustic crystals. Sci. Rep. 2016, 6, 32752. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- He, C.; Ni, X.; Ge, H.; Sun, X.C.; Chen, Y.B.; Lu, M.H.; Liu, X.P.; Chen, Y.F. Acoustic topological insulator and robust one-way sound transport. Nat. Phys. 2016, 12, 1124–1129. [Google Scholar] [CrossRef]
- Xia, B.Z.; Liu, T.T.; Huang, G.L.; Dai, H.Q.; Jiao, J.R.; Zang, X.G.; Yu, D.J.; Zheng, S.J.; Liu, J. Topological phononic insulator with robust pseudospin-dependent transport. Phys. Rev. B 2017, 96, 094106. [Google Scholar] [CrossRef] [Green Version]
- Yves, S.; Fleury, R.; Lemoult, F.; Fink, M.; Lerosey, G. Topological acoustic polaritons: Robust sound manipulation at the subwavelength scale. New J. Phys. 2017, 19, 075003. [Google Scholar] [CrossRef]
- Miniaci, M.; Pal, R.K.; Morvan, B.; Ruzzene, M. Experimental Observation of Topologically Protected Helical Edge Modes in Patterned Elastic Plates. Phys. Rev. X 2018, 8, 031074. [Google Scholar] [CrossRef] [Green Version]
- Yang, L.; Yu, K.; Wu, Y.; Zhao, R.; Liu, S. Topological spin-hall edge states of flexural wave in perforated metamaterial plates. J. Phys. D 2018, 51, 325302. [Google Scholar] [CrossRef] [Green Version]
- Yu, S.Y.; He, C.; Wang, Z.; Liu, F.K.; Sun, X.C.; Li, Z.; Lu, H.Z.; Lu, M.H.; Liu, X.P.; Chen, Y.F. Elastic pseudospin transport for integratable topological phononic circuits. Nat. Commun. 2018, 9, 3072. [Google Scholar] [CrossRef] [PubMed]
- Chaunsali, R.; Chen, C.W.; Yang, J. Subwavelength and directional control of flexural waves in zone-folding induced topological plates. Phys. Rev. B 2018, 97, 054307. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.; Qiu, C.; Ye, L.; Fan, X.; Ke, M.; Zhang, F.; Liu, Z. Observation of topological valley transport of sound in sonic crystals. Nat. Phys. 2017, 13, 369–374. [Google Scholar] [CrossRef] [Green Version]
- Gao, F.; Xue, H.; Yang, Z.; Lai, K.; Yu, Y.; Lin, X.; Chong, Y.; Shvets, G.; Zhang, B. Topologically protected refraction of robust kink states in valley photonic crystals. Nat. Phys. 2018, 14, 140–144. [Google Scholar] [CrossRef]
- Makwana, M.P.; Craster, R.V. Geometrically navigating topological plate modes around gentle and sharp bends. Phys. Rev. B 2018, 98, 184105. [Google Scholar] [CrossRef] [Green Version]
- Wu, Y.; Chaunsali, R.; Yasuda, H.; Yu, K.; Yang, J. Dial-in topological metamaterials based on bistable stewart platform. Sci. Rep. 2018, 8, 112. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Bonello, B.; Djafari-Rouhani, B.; Pennec, Y. Topological valley, pseudospin, and pseudospin-valley protected edge states in symmetric pillared phononic crystals. Phys. Rev. B 2019, 100, 140101(R). [Google Scholar] [CrossRef] [Green Version]
- Zhu, Z.; Huang, X.; Lu, J.; Yan, M.; Li, F.; Deng, W.; Liu, Z. Negative refraction and partition in acoustic valley materials of a square lattice. Phys. Rev. Appl. 2019, 12, 024007. [Google Scholar] [CrossRef]
- Xie, B.; Liu, H.; Cheng, H.; Liu, Z.; Chen, S.; Tian, J. Acoustic topological transport and refraction in a kekulé lattice. Phys. Rev. Appl. 2019, 11, 044086. [Google Scholar] [CrossRef]
- Wang, W.; Bonello, B.; Djafari-Rouhani, B.; Pennec, Y. Polarization-dependent and valley-protected Lamb waves in asymmetric pillared phononic crystals. J. Phys. D 2019, 52, 505302. [Google Scholar] [CrossRef] [Green Version]
- Zheng, L.Y.; Achilleos, V.; Chen, Z.G.; Richoux, O.; Theocharis, G.; Wu, Y.; Mei, J.; Felix, S.; Tournat, V.; Pagneux, V. Acoustic graphene network loaded with helmholtz resonators: A first-principle modeling, dirac cones, edge and interface waves. New J. Phys. 2020, 22, 013029. [Google Scholar] [CrossRef]
- Zhang, Q.; Chen, Y.; Zhang, K.; Hu, G. Dirac degeneracy and elastic topological valley modes induced by local resonant states. Phys. Rev. B 2020, 101, 014101. [Google Scholar] [CrossRef]
- Lu, J.; Qiu, C.; Deng, W.; Huang, X.; Li, F.; Zhang, F.; Chen, S.; Liu, Z. Valley topological phases in bilayer sonic crystals. Phys. Rev. Lett. 2018, 120, 116802. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Xia, B.Z.; Zheng, S.J.; Liu, T.T.; Jiao, J.R.; Chen, N.; Dai, H.Q.; Yu, D.J.; Liu, J. Observation of valleylike edge states of sound at a momentum away from the high-symmetry points. Phys. Rev. B 2018, 97, 155124. [Google Scholar] [CrossRef] [Green Version]
- Lu, J.; Qiu, C.; Ke, M.; Liu, Z. Valley vortex states in sonic crystals. Phys. Rev. Lett. 2016, 116, 093901. [Google Scholar] [CrossRef] [Green Version]
- Wu, X.; Meng, Y.; Tian, J.; Huang, Y.; Xiang, H.; Han, D.; Wen, W. Direct observation of valley-polarized topological edge states in designer surface plasmon crystals. Nat. Commun. 2017, 8, 1304. [Google Scholar] [CrossRef]
- Raj, K.P.; Massimo, R. Edge waves in plates with resonators: An elastic analogue of the quantum valley Hall effect. New J. Phys. 2017, 19, 025001. [Google Scholar]
- Miniaci, M.; Pal, R.K.; Manna, R.; Ruzzene, M. Valley-based splitting of topologically protected helical waves in elastic plates. Phys. Rev. B 2019, 100, 024304. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Jin, Y.; Wang, W.; Bonello, B.; Djafari-Rouhani, B.; Fleury, R. Robust Fano resonance in a topological mechanical beam. Phys. Rev. B 2020, 101, 024101. [Google Scholar] [CrossRef]
- Zheng, L.Y.; Christensen, J. Topological radiation engineering in hyperbolic sonic semimetals. Phys. Rev. B 2021, 103, 064307. [Google Scholar] [CrossRef]
- He, H.; Qiu, C.; Ye, L.; Cai, X.; Fan, X.; Ke, M.; Zhang, F.; Liu, Z. Topological negative refraction of surface acoustic waves in a Weyl phononic crystal. Nat. (London) 2018, 560, 61–64. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zhang, Z.; Tian, Y.; Cheng, Y.; Liu, X.; Christensen, J. Experimental verification of acoustic pseudospin multipoles in a symmetry-broken snowflakelike topological insulator. Phys. Rev. B 2017, 96, 241306(R). [Google Scholar] [CrossRef] [Green Version]
- Huang, H.; Tan, Z.; Huo, S.; Feng, L.; Chen, J.; Han, X. Topologically protected zero refraction of elastic waves in pseudospin-hall phononic crystals. Commun. Phys. 2020, 3, 46. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Z.; Tian, Y.; Wang, Y.; Gao, S.; Cheng, Y.; Liu, X.; Christensen, J. Directional acoustic antennas based on valley-hall topological insulators. Adv. Mater. 2018, 30, 1803229. [Google Scholar] [CrossRef] [Green Version]
- Ju, L.; Shi, Z.; Nair, N.; Lv, Y.; Jin, C.; Velasco, J.; Ojeda-Aristizabal, J.C.; Bechtel, H.A.; Martin, M.C.; Zettl, A.; et al. Topological valley transport at bilayer graphene. Nature 2015, 520, 650–655. [Google Scholar] [CrossRef]
- Li, J.; Wang, K.; McFaul, K.J.; Zern, Z.; Ren, Y.; Watanabe, K.; Taniguchi, T.; Qiao, Z.; Zhu, J. Gate-controlled topological conducting channels in bilayer graphene. Nat. Nanotech. 2016, 11, 1060–1065. [Google Scholar] [CrossRef]
- Li, J.; Zhang, R.X.; Yin, Z.; Zhang, J.; Watanabe, K.; Taniguchi, T.; Liu, C.; Zhu, J. A valley valve and electron beam splitter. Science 2018, 362, 1149–1152. [Google Scholar] [CrossRef] [Green Version]
- Wang, Z.Y.; Yang, Y.Z.; Li, H.Y.; Jia, H.; Luo, J.L.; Huang, J.; Wang, Z.N.; Jiang, B.; Yang, N.J.; Jin, G.J.; et al. Multichannel Topological Transport in an Acoustic Valley Hall Insulator. Phys. Rev. Appl. 2021, 15, 024019. [Google Scholar] [CrossRef]
- Chen, Y.; He, X.T.; Cheng, Y.J.; Qiu, H.Y.; Feng, L.T.; Zhang, M.; Dai, D.X.; Guo, G.C.; Dong, J.W.; Ren, X.F. Topologically Protected Valley-Dependent Quantum Photonic Circuits. Phys. Rev. Lett. 2021, 126, 230503. [Google Scholar] [CrossRef]
- Jia, D.; Ge, Y.; Xue, H.R.; Yuan, S.Q.; Sun, H.X.; Yang, Y.H.; Liu, X.J.; Zhang, B.L. Topological refraction in dual-band valley sonic crystals. Phys. Rev. B 2021, 103, 144309. [Google Scholar] [CrossRef]
- Zhang, X.J.; Liu, L.; Lu, M.H.; Chen, Y.F. Valley-Selective Topological Corner States in Sonic Crystals. Phys. Rev. Lett. 2021, 126, 156401. [Google Scholar] [CrossRef] [PubMed]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, H.; Luo, C.; Zhang, T.; Xu, J.; Zhou, X.; Shen, Y.; Deng, X. Topological Refraction in Kagome Split-Ring Photonic Insulators. Nanomaterials 2022, 12, 1493. https://doi.org/10.3390/nano12091493
Li H, Luo C, Zhang T, Xu J, Zhou X, Shen Y, Deng X. Topological Refraction in Kagome Split-Ring Photonic Insulators. Nanomaterials. 2022; 12(9):1493. https://doi.org/10.3390/nano12091493
Chicago/Turabian StyleLi, Huichang, Chen Luo, Tailin Zhang, Jianwei Xu, Xiang Zhou, Yun Shen, and Xiaohua Deng. 2022. "Topological Refraction in Kagome Split-Ring Photonic Insulators" Nanomaterials 12, no. 9: 1493. https://doi.org/10.3390/nano12091493
APA StyleLi, H., Luo, C., Zhang, T., Xu, J., Zhou, X., Shen, Y., & Deng, X. (2022). Topological Refraction in Kagome Split-Ring Photonic Insulators. Nanomaterials, 12(9), 1493. https://doi.org/10.3390/nano12091493





