3D-Printed Piezoelectret Based on Foamed Polylactic Acid for Energy-Harvesting and Sensing Applications
Abstract
:1. Introduction
2. Material and Instrumental Setup
3. Results and Discussion
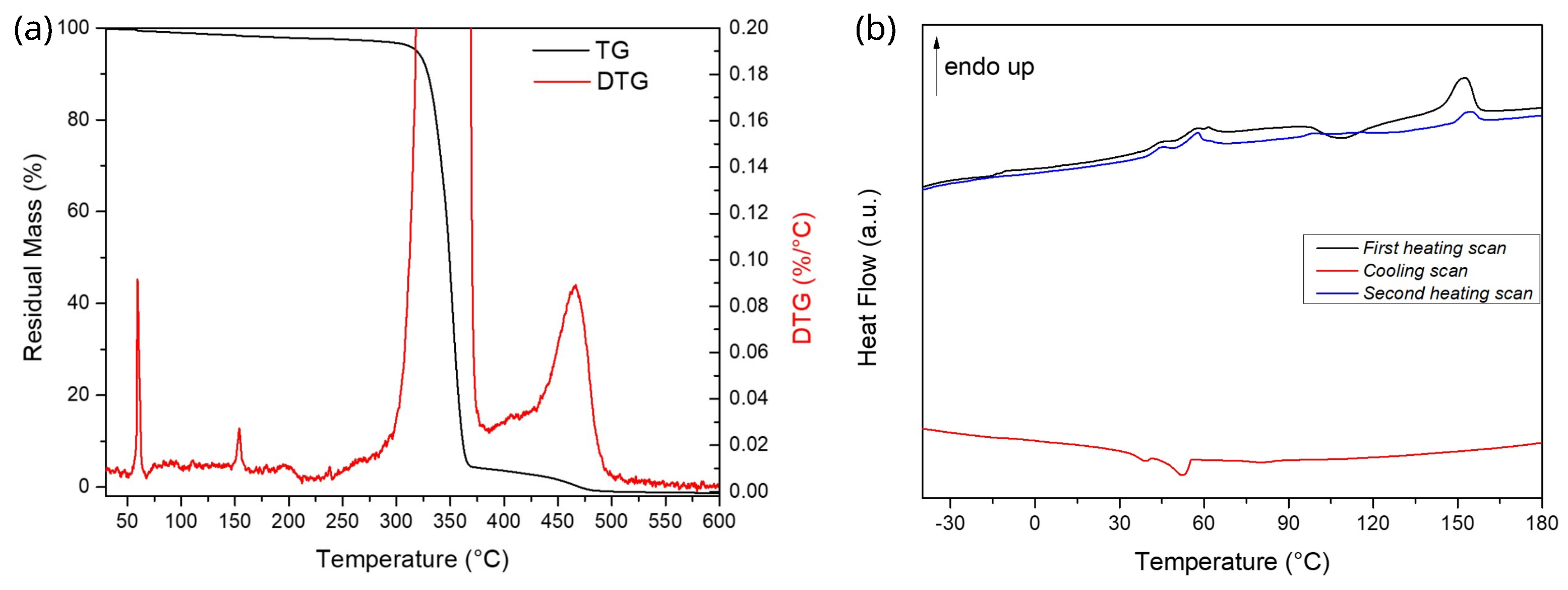
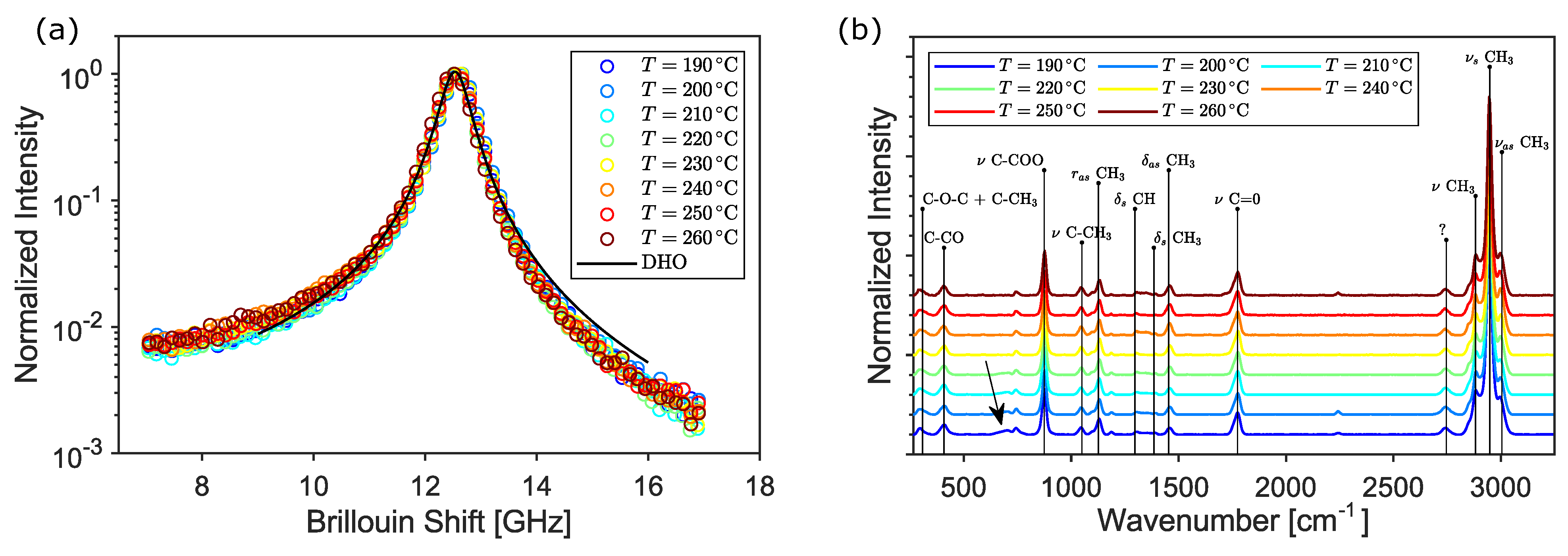
3.1. Material Characterization
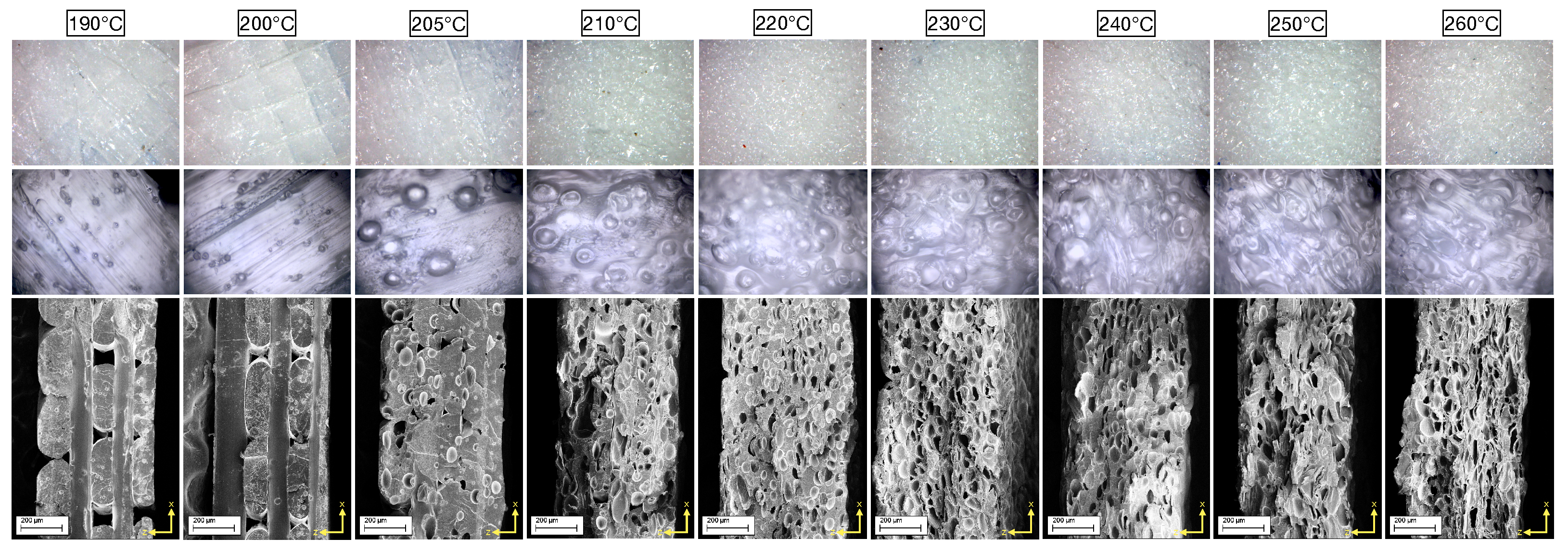
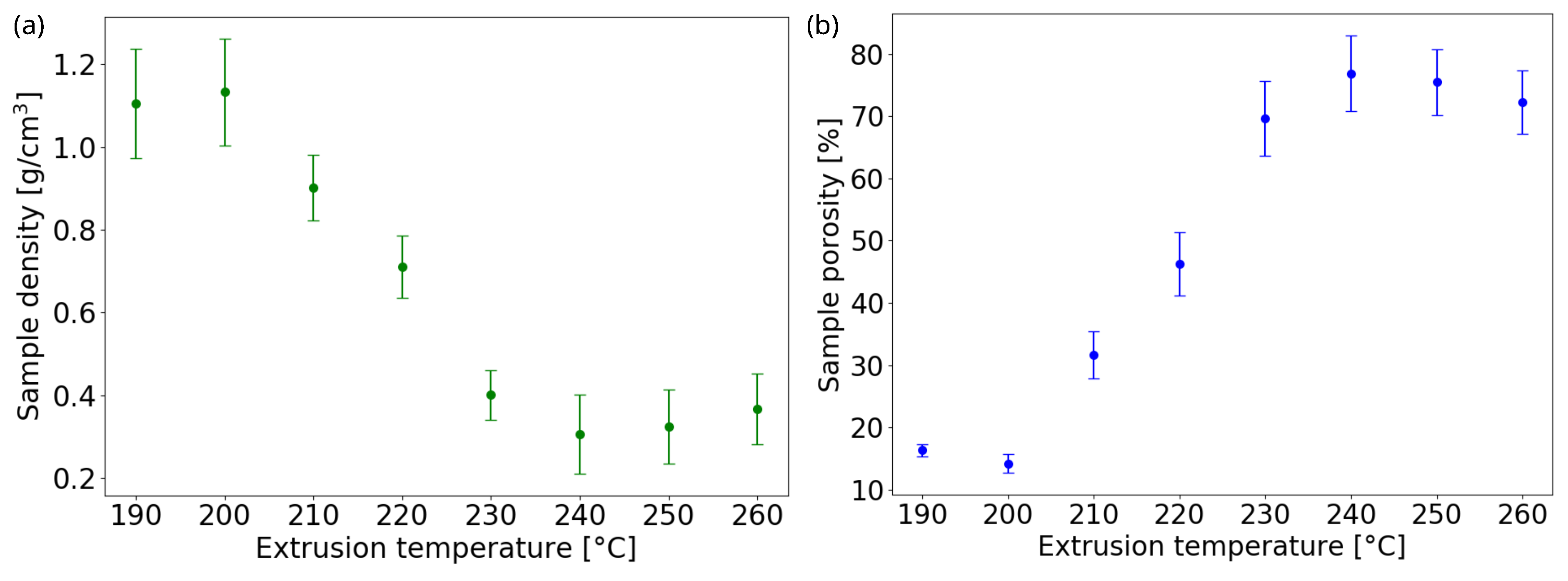
3.2. Morphological Characterization
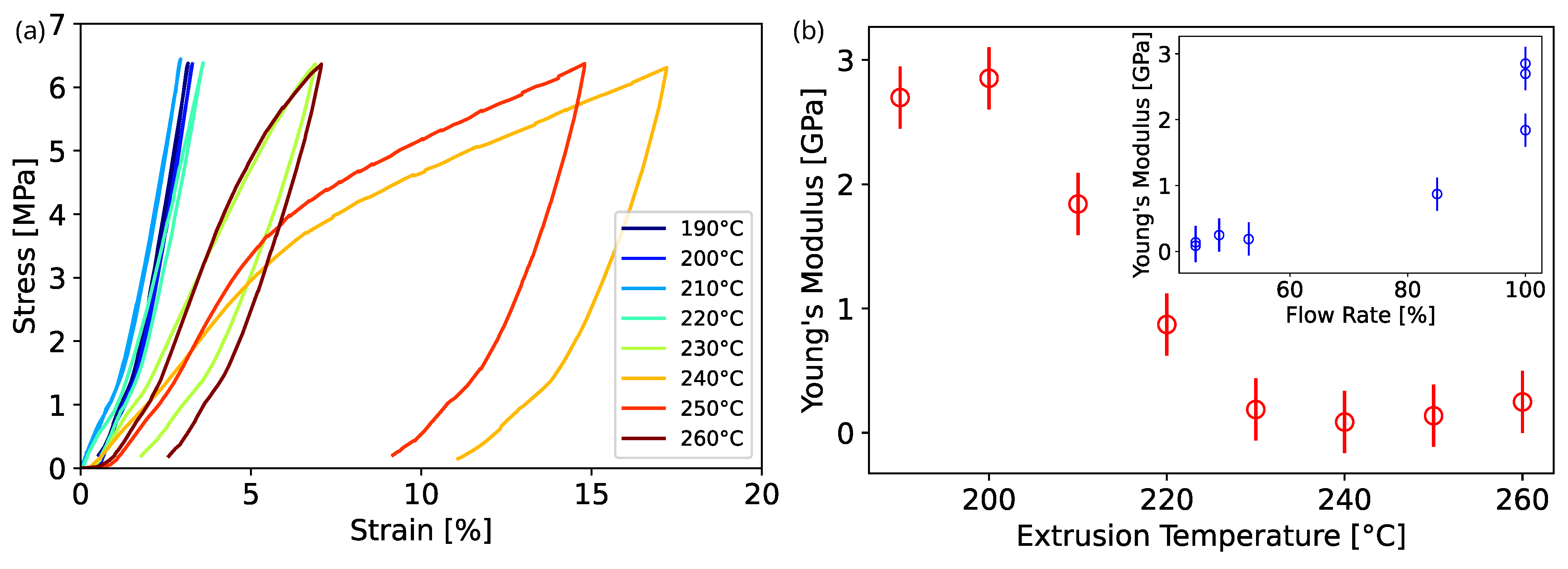
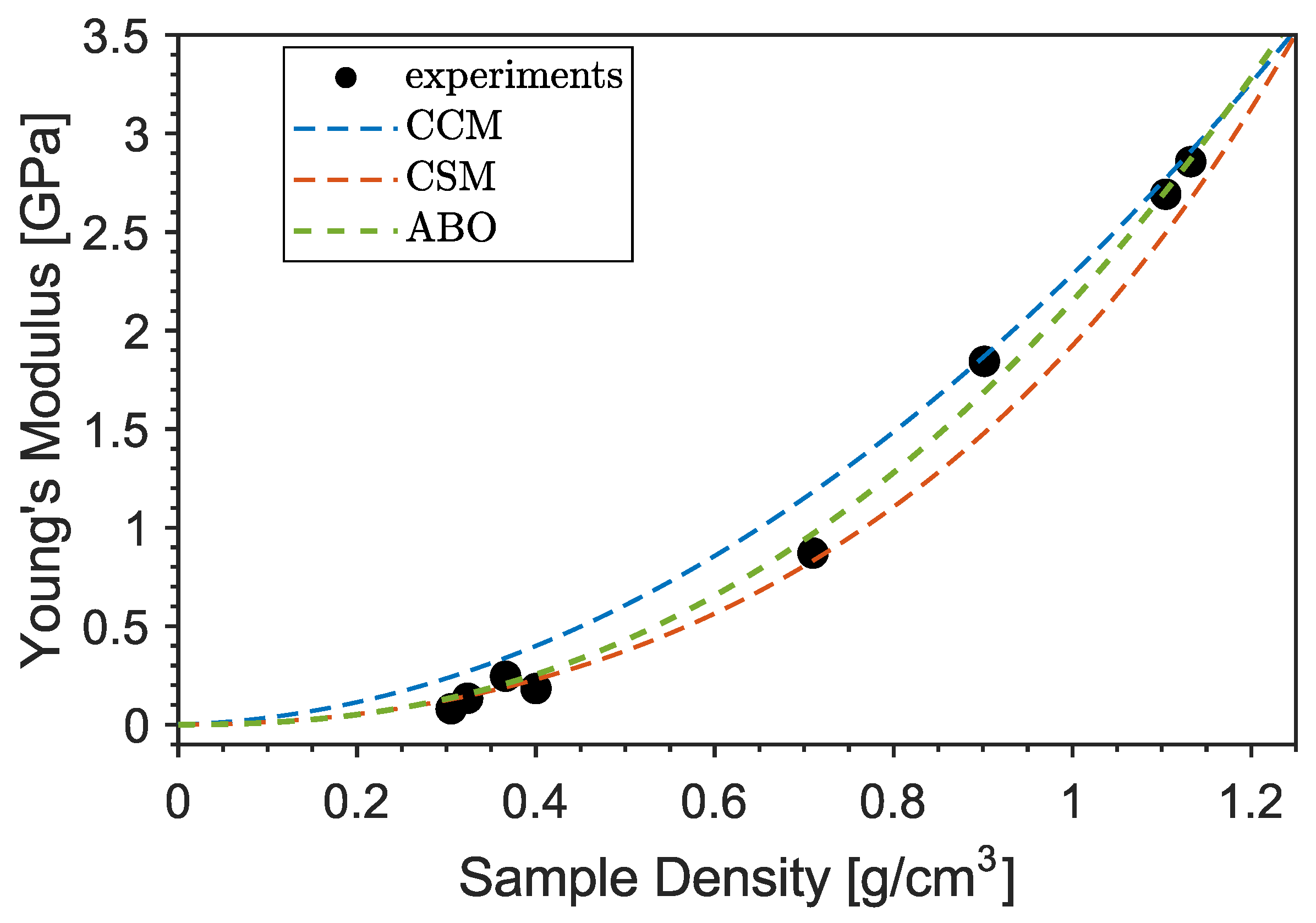
3.3. Mechanical Characterization
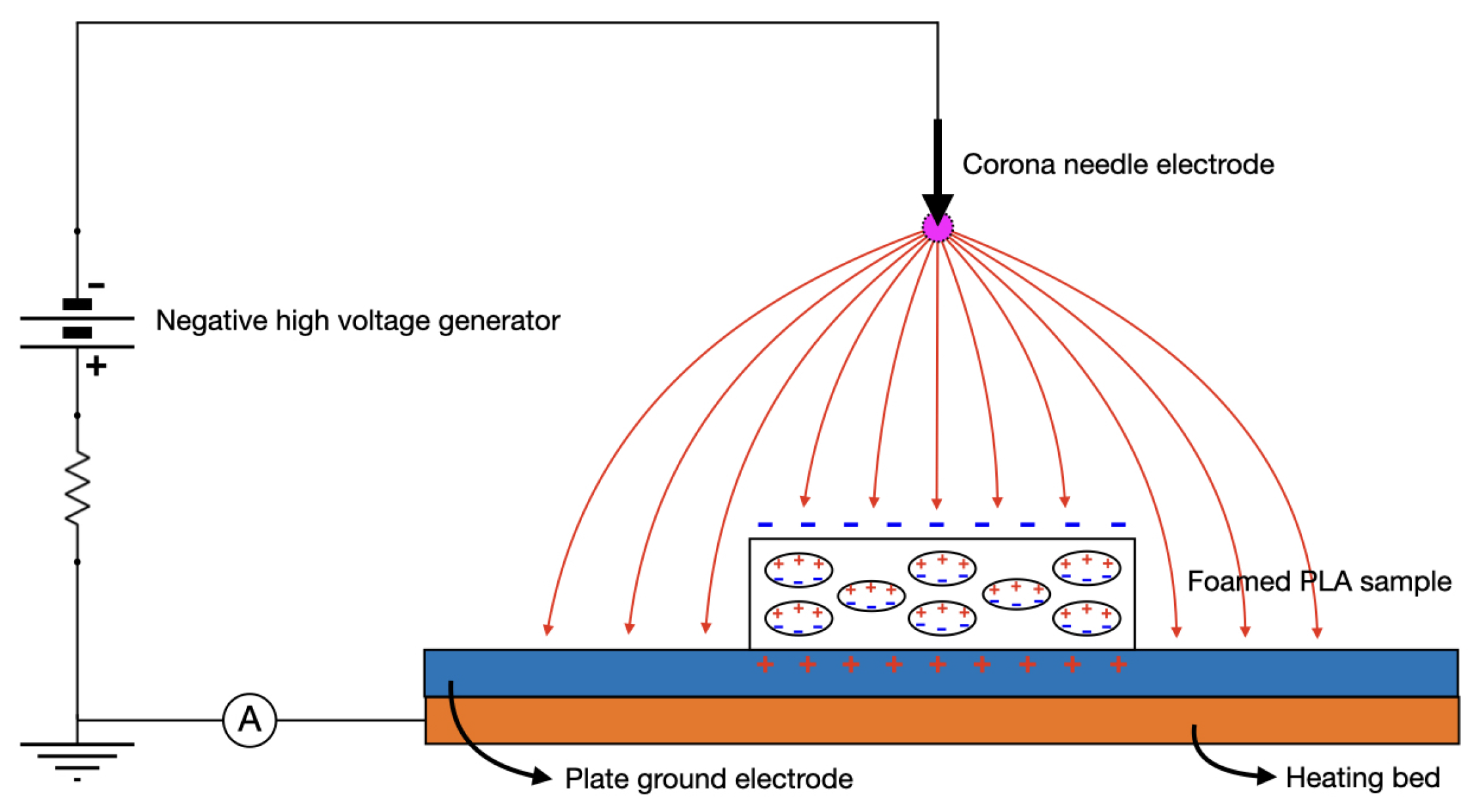
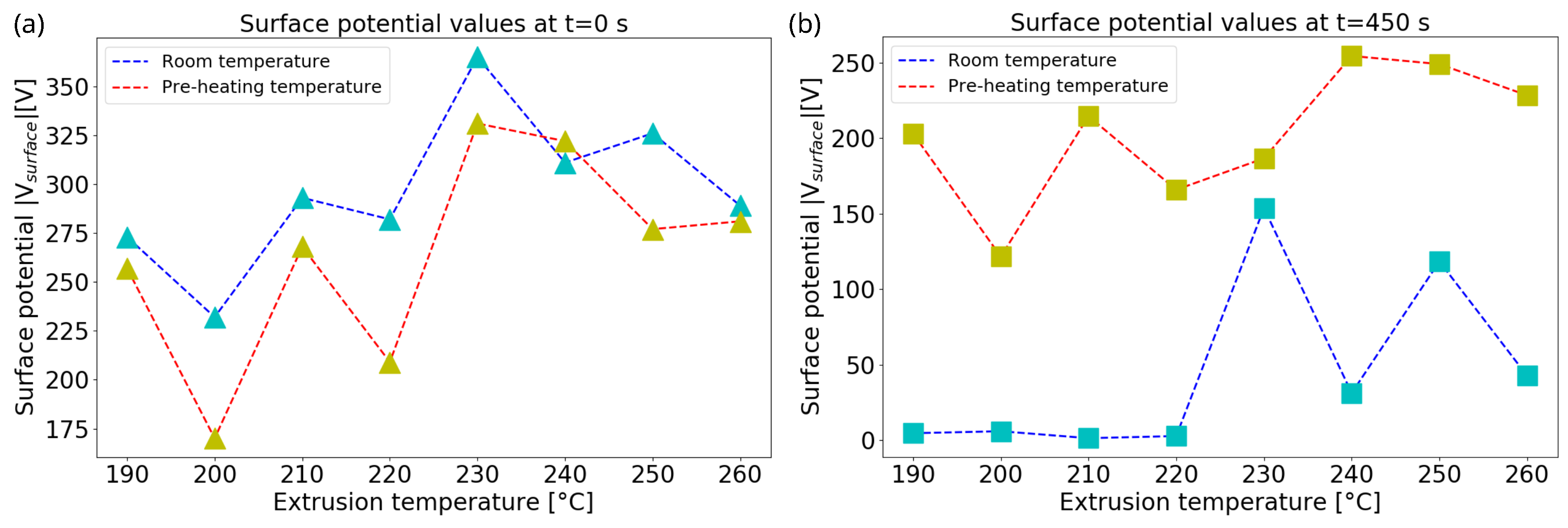
3.4. Charging Methods and Electrical Behaviour
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Clementi, G.; Cottone, F.; Di Michele, A.; Gammaitoni, L.; Mattarelli, M.; Perna, G.; López-Suárez, M.; Baglio, S.; Trigona, C.; Neri, I. Review on Innovative Piezoelectric Materials for Mechanical Energy Harvesting. Energies 2022, 15, 6227. [Google Scholar] [CrossRef]
- Li, X.; Wang, Y.; Xu, M.; Shi, Y.; Wang, H.; Yang, X.; Ying, H.; Zhang, Q. Polymer electrets and their applications. J. Appl. Polym. Sci. 2021, 138, 50406. [Google Scholar] [CrossRef]
- Eberle, G.; Schmidt, H.; Eisenmenger, W. Piezoelectric polymer electrets. IEEE Trans. Dielectr. Electr. Insul. 1996, 3, 624–646. [Google Scholar] [CrossRef]
- Goda, E.S.; Abu Elella, M.H.; Al Kiey, S.A.; Shehata, A.B.; Pandit, B. Polymeric Foam for Energy Applications. In Polymeric Foams: Applications of Polymeric Foams (Volume 2); ACS Publications: Washington, DC, USA, 2023; pp. 187–208. [Google Scholar]
- Hamdi, O.; Mighri, F.; Rodrigue, D. Piezoelectric cellular polymer films: Fabrication, properties and applications. AIMS Mater. Sci. 2018, 5, 845–869. [Google Scholar] [CrossRef]
- Lu, Y.; Cottone, F.; Boisseau, S.; Marty, F.; Galayko, D.; Basset, P. Low-frequency and ultra-wideband MEMS electrostatic vibration energy harvester powering an autonomous wireless temperature sensor node. In Proceedings of the 2016 IEEE 29th International Conference on Micro Electro Mechanical Systems (MEMS), Shanghai, China, 24–28 January 2016; pp. 33–36. [Google Scholar]
- Wu, N.; Cheng, X.; Zhong, Q.; Zhong, J.; Li, W.; Wang, B.; Hu, B.; Zhou, J. Cellular Polypropylene Piezoelectret for Human Body Energy Harvesting and Health Monitoring. Adv. Funct. Mater. 2015, 25, 4788–4794. [Google Scholar] [CrossRef]
- Mishra, S.; Unnikrishnan, L.; Nayak, S.K.; Mohanty, S. Advances in piezoelectric polymer composites for energy harvesting applications: A systematic review. Macromol. Mater. Eng. 2019, 304, 1800463. [Google Scholar] [CrossRef]
- Mohebbi, A.; Mighri, F.; Ajji, A.; Rodrigue, D. Cellular polymer ferroelectret: A review on their development and their piezoelectric properties. Adv. Polym. Technol. 2018, 37, 468–483. [Google Scholar] [CrossRef]
- Crichton, B.H. Gas discharge physics. In Proceedings of the IEE Colloquium on Advances in HV Technology, Glasgow, UK, 4 October 1996; Volume 3. [Google Scholar]
- Ansari, M.A.; Somdee, P. Piezoelectric Polymeric Foams as Flexible Energy Harvesters: A Review. Adv. Energy Sustain. Res. 2022, 3, 2200063. [Google Scholar] [CrossRef]
- Nofar, M.; Utz, J.; Geis, N.; Altstädt, V.; Ruckdäschel, H. Foam 3D printing of thermoplastics: A symbiosis of additive manufacturing and foaming technology. Adv. Sci. 2022, 9, 2105701. [Google Scholar] [CrossRef]
- Zhukov, S.; Ma, X.; Seggern, H.v.; Sessler, G.M.; Dali, O.B.; Kupnik, M.; Zhang, X. Biodegradable cellular polylactic acid ferroelectrets with strong longitudinal and transverse piezoelectricity. Appl. Phys. Lett. 2020, 117, 112901. [Google Scholar] [CrossRef]
- Ma, X.; Zhukov, S.; von Seggern, H.; Sessler, G.M.; Ben Dali, O.; Kupnik, M.; Dai, Y.; He, P.; Zhang, X. Biodegradable and Bioabsorbable Polylactic Acid Ferroelectrets with Prominent Piezoelectric Activity. Adv. Electron. Mater. 2023, 9, 2201070. [Google Scholar] [CrossRef]
- Vadas, D.; Bocz, K.; Igricz, T.; Volk, J.; Bordács, S.; Madarász, L.; Marosi, G. Novel manufacturing method for highly flexible poly(lactic acid) foams and ferroelectrets. Adv. Ind. Eng. Polym. Res. 2023. [Google Scholar] [CrossRef]
- Chang, J.S.; Lawless, P.; Yamamoto, T. Corona discharge processes. IEEE Trans. Plasma Sci. 1991, 19, 1152–1166. [Google Scholar] [CrossRef]
- Goldman, M.; Goldman, A.; Sigmond, R.S. The corona discharge: Its properties and specific uses. Pure Appl. Chem. 1985, 57, 1353–1362. [Google Scholar] [CrossRef]
- Stark, W. Electret formation by electrical discharge in air. J. Electrost. 1989, 22, 329–339. [Google Scholar] [CrossRef]
- Sessler, G.M. Electrets, 3rd ed.; Laplacian Press: Morgan Hill, CA, USA, 1999; Volume 1. [Google Scholar]
- ColorFabb Website. Available online: https://colorfabb.com/upgrading-from-lw-pla-to-lw-pla-ht-what-you-need-to-know (accessed on 18 September 2023).
- BS 2782-3 METHOD 345A:1979; Methods of Testing Plastics: Method 345A. Determination of Compressive Properties by Deformation at Constant Rate. British Standard: London, UK, 1979.
- ISO 604:2002; Plastics—Determination of Compressive Properties. British Standard: London, UK, 1999.
- Passeri, A.; Di Michele, A.; Neri, I.; Cottone, F.; Fioretto, D.; Mattarelli, M.; Caponi, S. Size and environment: The effect of phonon localization on micro-Brillouin imaging. Biomater. Adv. 2023, 147, 213341. [Google Scholar] [CrossRef]
- Mattarelli, M.; Capponi, G.; Passeri, A.A.; Fioretto, D.; Caponi, S. Disentanglement of Multiple Scattering Contribution in Brillouin Microscopy. ACS Photonics 2022, 9, 2087–2091. [Google Scholar] [CrossRef]
- Jin, F.L.; Zhao, M.; Park, M.; Park, S.J. Recent trends of foaming in polymer processing: A review. Polymers 2019, 11, 953. [Google Scholar] [CrossRef]
- Brischetto, S.; Torre, R. Tensile and compressive behavior in the experimental tests for PLA specimens produced via fused deposition modelling technique. J. Compos. Sci. 2020, 4, 140. [Google Scholar] [CrossRef]
- Zhao, Y.; Chen, Y.; Zhou, Y. Novel mechanical models of tensile strength and elastic property of FDM AM PLA materials: Experimental and theoretical analyses. Mater. Des. 2019, 181, 108089. [Google Scholar] [CrossRef]
- Hössinger-Kalteis, A.; Reiter, M.; Jerabek, M.; Major, Z. Overview and comparison of modelling methods for foams. J. Cell. Plast. 2021, 57, 951–1001. [Google Scholar] [CrossRef]
- Mattarelli, M.; Vassalli, M.; Caponi, S. Relevant Length Scales in Brillouin Imaging of Biomaterials: The Interplay between Phonons Propagation and Light Focalization. ACS Photonics 2020, 7, 2319–2328. [Google Scholar] [CrossRef]
- Farah, S.; Anderson, D.G.; Langer, R. Physical and mechanical properties of PLA, and their functions in widespread applications: A comprehensive review. Adv. Drug Deliv. Rev. 2016, 107, 367–392. [Google Scholar] [CrossRef]
- Bolskis, E.; Adomavičiūtė, E.; Griškonis, E. Formation and Investigation of Mechanical, Thermal, Optical and Wetting Properties of Melt-Spun Multifilament Poly(lactic acid) Yarns with Added Rosins. Polymers 2022, 14, 379. [Google Scholar] [CrossRef] [PubMed]
- Gibson, L.J.; Ashby, M.F. Cellular Solids: Structure and Properties, 2nd ed.; Cambridge Solid State Science Series; Cambridge University Press: Cambridge, UK, 1997. [Google Scholar]
- Ramakrishnan, N.; Arunachalam, V.S. Effective elastic moduli of porous solids. J. Mater. Sci. 1990, 25, 3930–3937. [Google Scholar] [CrossRef]
- Arnold, M.; Boccaccini, A.R.; Ondracek, G. Prediction of the Poisson’s ratio of porous materials. J. Mater. Sci. 1996, 31, 1643–1646. [Google Scholar] [CrossRef]
- Kim, O.V.; Dunn, P.F. Real-time direct charge measurements of microdroplets and comparison with indirect methods. Aerosol Sci. Technol. 2010, 44, 292–301. [Google Scholar] [CrossRef]
- Giacometti, J.A.; Oliveira, O.N. Corona charging of polymers. IEEE Trans. Electr. Insul. 1992, 27, 924–943. [Google Scholar] [CrossRef]
- Van Turnhout, J. Thermally stimulated discharge of polymer electrets. Polym. J. 1971, 2, 173–191. [Google Scholar] [CrossRef]
- Von Seggern, H.; West, J. Stabilization of positive charge in fluorinated ethylene propylene copolymer. J. Appl. Phys. 1984, 55, 2754–2757. [Google Scholar] [CrossRef]
- Llovera-Segovia, P.; Ortega-Braña, G.; Fuster-Roig, V.; Quijano-López, A. Charging of Piezoelectric Cellular Polypropylene Film by Means of a Series Dielectric Layer. Polymers 2021, 13, 333. [Google Scholar] [CrossRef] [PubMed]




| Temp | 190 °C | 200 °C | 210 °C | 220 °C | 230 °C | 240 °C | 250 °C | 260 °C |
|---|---|---|---|---|---|---|---|---|
| Flowrate | 100% | 100% | 100% | 85% | 53% | 44% | 44% | 48% |
| Temp | 190 °C | 200 °C | 205 °C | 210 °C | 220 °C | 230 °C | 240 °C | 250 °C | 260 °C |
|---|---|---|---|---|---|---|---|---|---|
| d (m) | 576.7 | 570.2 | 606.4 | 604.3 | 642.1 | 665.5 | 638.5 | 562.1 | 556.5 |
| / | / | 74.2 | 109.7 | 221.2 | 238.2 | 227.4 | 213.8 | 217.9 |
| Reference | Model | Adjustable Parameters |
|---|---|---|
| Gibson and Ashby [32] | ||
| Ramakrishnan and Arunachalam [33] | ||
| Arnold et al. [34] |
| L (cm) | 1 | 2 | 3 | 3.5 | 4 | 4.25 | 4.5 | 4.75 | 5 |
|---|---|---|---|---|---|---|---|---|---|
| V (kV) | −6 | −8 | −18 | −20 | −25 | −25 | −25 | −25 | −25 |
| E () | 1.45 | 0.84 | 1.16 | 1.07 | 1.15 | 1.07 | 1.00 | 0.94 | 0.88 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Perna, G.; Bonacci, F.; Caponi, S.; Clementi, G.; Di Michele, A.; Gammaitoni, L.; Mattarelli, M.; Neri, I.; Puglia, D.; Cottone, F. 3D-Printed Piezoelectret Based on Foamed Polylactic Acid for Energy-Harvesting and Sensing Applications. Nanomaterials 2023, 13, 2953. https://doi.org/10.3390/nano13222953
Perna G, Bonacci F, Caponi S, Clementi G, Di Michele A, Gammaitoni L, Mattarelli M, Neri I, Puglia D, Cottone F. 3D-Printed Piezoelectret Based on Foamed Polylactic Acid for Energy-Harvesting and Sensing Applications. Nanomaterials. 2023; 13(22):2953. https://doi.org/10.3390/nano13222953
Chicago/Turabian StylePerna, Gabriele, Francesco Bonacci, Silvia Caponi, Giacomo Clementi, Alessandro Di Michele, Luca Gammaitoni, Maurizio Mattarelli, Igor Neri, Debora Puglia, and Francesco Cottone. 2023. "3D-Printed Piezoelectret Based on Foamed Polylactic Acid for Energy-Harvesting and Sensing Applications" Nanomaterials 13, no. 22: 2953. https://doi.org/10.3390/nano13222953
APA StylePerna, G., Bonacci, F., Caponi, S., Clementi, G., Di Michele, A., Gammaitoni, L., Mattarelli, M., Neri, I., Puglia, D., & Cottone, F. (2023). 3D-Printed Piezoelectret Based on Foamed Polylactic Acid for Energy-Harvesting and Sensing Applications. Nanomaterials, 13(22), 2953. https://doi.org/10.3390/nano13222953












