Residual Stresses in Soft Magnetic FeTiB and FeZrN Films Obtained by Magnetron Deposition

Abstract

The coercive field of soft magnetic ferromagnets is a structure-sensitive property and, in particular, is substantially affected by residual stresses. In the present study, the phase and structural states and residual stresses of the FeTiB and FeZrN films of various compositions, which were prepared by magnetron deposition on glass substrates and subsequent 1-h annealing at temperatures of 200–600 °C, were investigated by X-ray diffraction. The formation of a nanocrystalline structure is observed. It comprises different phases having different lattice parameters and unit-cell volumes and is characterized by high level of microstrains of grains as well; the microstrains predetermine the formation of high compressive stresses in the deposited films. As the annealing temperature increases, the compressive stresses decrease and, at certain temperatures, gradually transform into thermal tensile stresses, which are induced by the difference in the thermal expansion coefficients of the film and substrate. Thus, the heat treatment is the efficient way to improve the soft magnetic properties of the studied class of film materials produced by magnetron deposition.

1. Introduction

The development of new magnetic materials that meet the ever-growing requirements of modern magnetic electronics is the important problem of materials science. In particular, information technology devices require soft magnetic film materials providing the combination of a maximum possible saturation induction (B_s > 2 T) and a low coercive field (H_c < 1 Oe).

Films of Fe-Ti-B and Fe-Zr-N system alloys with two-phases nanocrystalline structure (TiB₂ boride or ZrN nitride particles located in the main soft magnetic αFe phase), which are produced by magnetron deposition followed by annealing (300–500 °C), are able to ensure the combination of high saturation induction (B_s ~ 2 T) and low coercive field (H_c < 1 Oe) [1,2].

Such a class of film materials is prepared by magnetron sputtering. Energy parameters of the magnetron sputtering, unlike those of other preparation methods available for film materials [3], ensure the conditions for the preparation of films in amorphous and nanocrystalline states, which comprise high-temperature and thermodynamically stable phases, in particular, TiB₂ and ZrN.

The value of the coercive field is determined by the magnetic anisotropy energy, which includes contributions of the magnetocrystalline anisotropy (the anisotropy of crystal lattice of a material or its constituent phases) and magnetoelastic anisotropy (the magnetostriction, microstrains in the grain body of crystalline phases, and residual stresses).

Two main types of residual stresses can be induced in the films; these are the so-called intrinsic residual stresses formed in the course of magnetron deposition and thermal stresses, σ_T, formed during annealing of films. The intrinsic residual stresses are related to accumulated defects (crystal lattice distortions, stacking faults, impurity atoms, vacancies, etc.) and the evolution of the film structure during deposition [4]. The thermal stresses arise due to the difference in the thermal expansion coefficients of film and substrate materials [5].

The insight into quantitative relationships between residual stresses formed in ferromagnetic films and parameters of their phase and structural states allows the value of the coercive field of film material to be purposefully controlled. The present work is aimed at the study of quantitative relationships between residual stresses and parameters of the phase and structural states, which are realized in the FeTiB and FeZrN films prepared by magnetron deposition followed by annealing.

There are different methods for the determination of residual stresses; these are the nanoindentation [6], determination of substrate curvature [7], FIB-DIC method (focused ion beam-digital image correlation) [8], and X-ray diffraction sin²ψ method [8]. The following advantages of the X-ray diffraction can be considered, which determine its application in term of the present work: the method is non-destructive and allows the stresses to be determined at a depth less than 1 µm, and the value of stresses to be averaged over the diffracting volume.

2. Materials and Methods

The FeTiB films were prepared by a DC magnetron sputtering of a composite target, which consists of a Fe disk with uniformly arranged TiB₂ plated. The process is performed in an argon atmosphere at a pressure of P_Ar = 0.2–0.3 Pa, at the voltage U = 500–600 V and the current I = 1.5–1.7 A. To prepare the FeZrN films, the reactive magnetron sputtering is used. In this case, the Fe target with metallic Zr plates arranged on its surface along the erosion zone was sputtered in a gas mixture (Ar + N₂) atmosphere at P_Ar+N2 = 0.13–0.15 Pa, U = 450 V, and I = 1.5 A. The films were deposited on glass substrates 1.5 mm thick.

The substrate temperature during deposition did not exceed 200 °C. The chemical composition of the deposited films was determined by energy-dispersive X-ray spectroscopy and glow-discharge optical emission spectroscopy (GDOES). The film thickness was measured by scanning electron microscopy (SEM). The detailed descriptions of the preparation conditions and results of the chemical analysis of the films are reported in References [1,9]. The films were annealed in a vacuum (at a residual pressure of less than 3 × 10⁻⁴ Pa) for 1 h at temperatures of 200, 300, 400, 500, and 600 °C.

The phase analysis and the structural studies were performed by X-ray diffraction (XRD) using a Rigaku Ultima IV diffractometer equipped with a graphite monochromator, in the Bragg–Brentano geometry, using CuKα radiation; the scanning was carried out in a 2θ angular range of 20–120° at a step of 0.2°. The use of radiation from a tube equipped with a copper anode was due to the low penetration of the radiation (~2 µm) into the Fe-based material, which results from the large coefficient of linear attenuation. The software package [10] was applied for processing the experimental data using a full-profile Rietveld method. The phase composition, volume fractions of crystalline phases (ν_i), their lattice parameters (a/c), and structure of the films (size corresponding to grain size D and the Gaussian rms microstrains ε of the crystal lattice at the grain scale) were determined using XRD patterns. The lattice parameters of phases were determined from the position of the centers of gravity of high-angle peaks using the Voigt approximation.

The magnitude and sign of the residual stresses were determined using the sin²ψ method [11]. It is known that arising the residual stresses in a material results in uniform changing the interplanar spacings Δd_hkl and, therefore, to shifting X-ray diffraction reflections. The sin²ψ method is based on the measuring of the change in the position of reflections when patterns are taken at different angles ψ made by the sample surface normal and lattice plane normal. The obtained dependence of the angular position of reflection on sin²ψ is described by the expression:

$${\mathsf{\theta }}_{\mathsf{\psi }}={\mathsf{\theta }}_{\mathsf{\psi }=0}-\frac{1+\mathsf{\nu }}{\mathrm{E}}{\mathrm{tg}\mathsf{\theta }}_{\mathsf{\psi }=0}{\mathsf{\sigma }\sin }^2\mathsf{\psi }$$

(1)

where θ_ψ, θ_ψ=0 are the angular positions of diffraction reflections at different angles ψ; ν is the Poisson’s ratio; and E is the Young’s modulus. In this case, the change in position of the high-angle peak (211) of αFe was recorded while the angle ψ is changed in the sequence 0, 20.7, 30.0, 37.8, 45.0, 52.2, and 60.0°.

The measurement was performed by iso-inclination method [12]. The CrKα radiation characterized by the highest wavelength (2.2898 Å) was used because it provides a possibility to record the (211) peak at high angles 2θ~156° and, thereby, to reduce the error of determination of the stress value. The dependences of the change in the angular position 2θ of the (211) peak on sin²ψ were approximated by a linear function (Figure 1). The residual stresses were calculated using the Young’s modulus (E_f = 175 GPa) of the studied films and Poisson’s ratio of pure αFe (ν_f = 0.29).

3. Results and Discussion

3.1. Chemical Composition of the Films

The distribution of chemical elements across the film thickness was determined by GDOES (Figure 2a); the averaged chemical compositions of each film under study are given in

Table 1. Phase composition and structure of the studied films in as-deposited and annealed states.

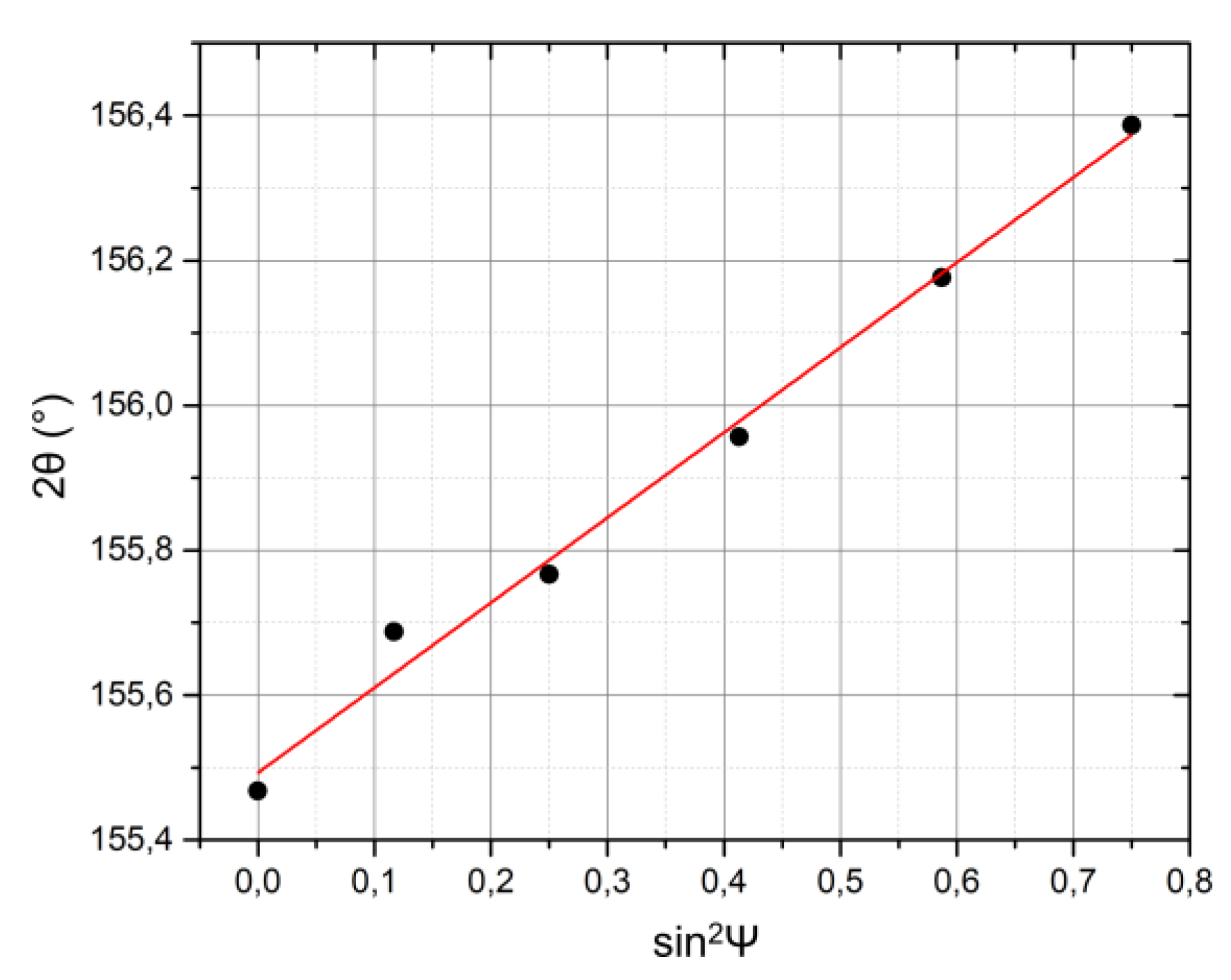
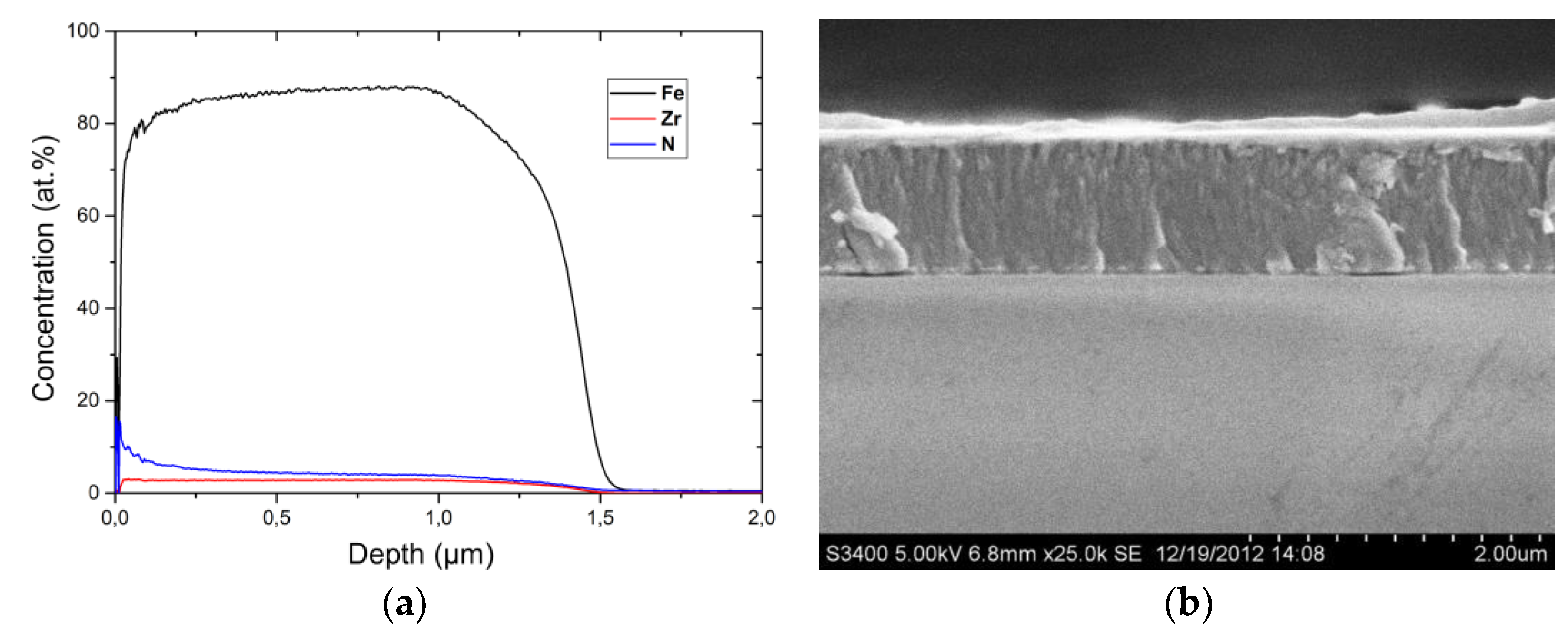
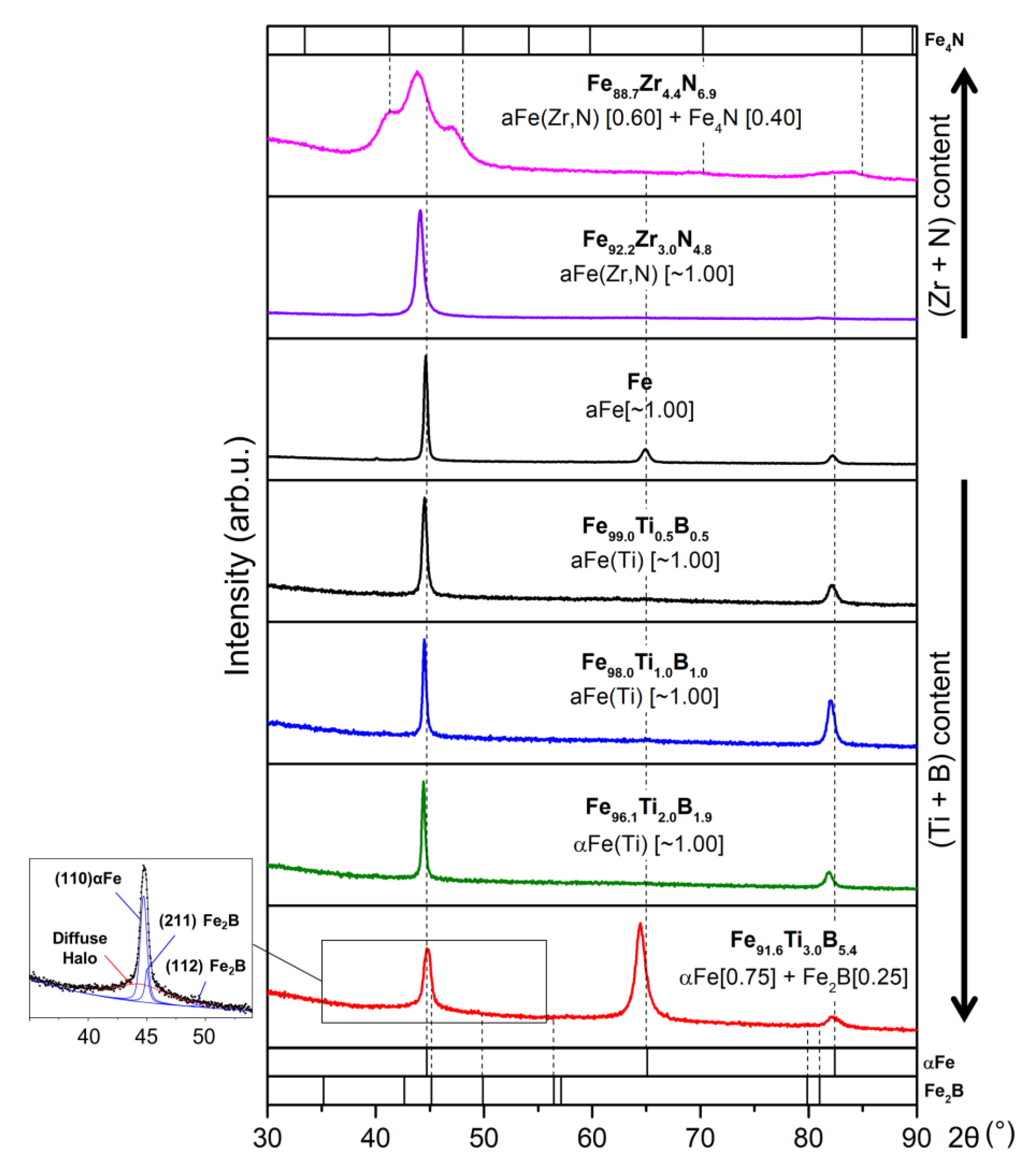
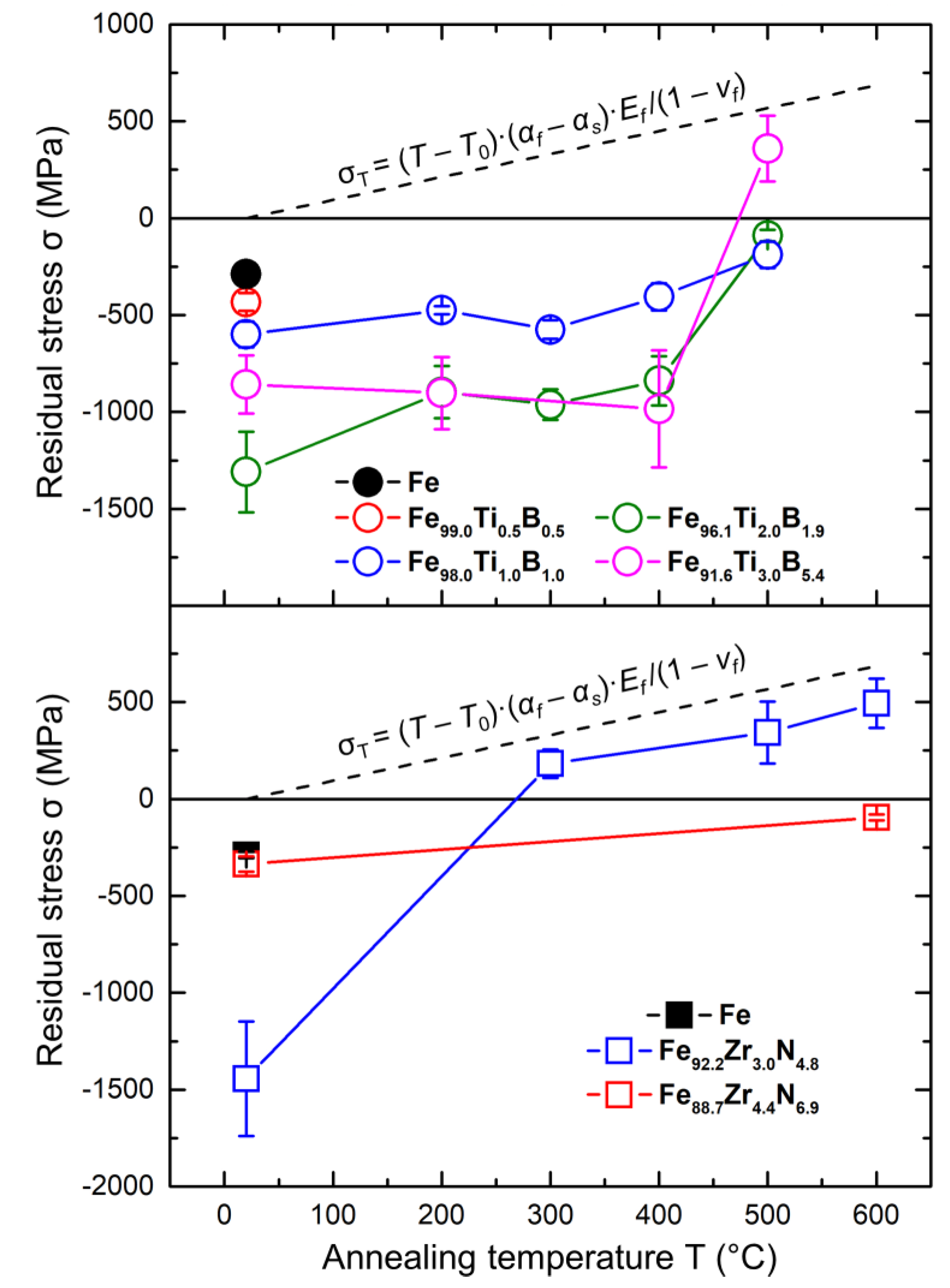
Film	Tann, °C	νi Phase	a/c, Å	D, nm	ε, %
Fe	As-dep.	~1.00 αFe	2.872	45.6	0.268
Fe99.0Ti0.5B0.5	As-sput.	~1.00 αFe(Ti)	2.879	24.4	0.247
-	200	~1.00 αFe(Ti)	2.877	24.7	0.248
-	300	~1.00 αFe(Ti)	2.874	26.1	0.256
-	400	~1.00 αFe	2.866	31.2	0.264
-	500	~1.00 αFe	2.866	32.4	0.250
Fe98.0Ti1.0B1.0	As-sput.	~1.00 αFe(Ti)	2.879	35.5	0.237
-	200	~1.00 αFe(Ti)	2.880	35.9	0.247
-	300	~1.00 αFe(Ti)	2.876	38.2	0.242
-	400	~1.00 αFe(Ti)	2.879	36.4	0.230
-	500	~1.00 αFe(Ti)	2.875	34.3	0.279
Fe96.1Ti2.0B1.9	As-sput.	~1.00 αFe(Ti)	2.885	34.1	0.205
-	200	~1.00 αFe(Ti)	2.883	38.5	0.229
-	300	~1.00 αFe(Ti)	2.879	30.7	0.187
-	400	~1.00 αFe(Ti)	2.878	27.4	0.216
-	500	~1.00 αFe(Ti)	2.871	34.5	0.239
Fe91.6Ti3.0B5.4	As-sput.	0.75 αFe 0.25 Fe2B	2.890 –	8.2 22.7	0.160 0.399
-	200	0.69 αFe 0.31 Fe2B	2.890 –	8.4 –	0.365 –
-	300	0.76 αFe 0.24 Fe2B	2.892 –	8.3 –	0.177 –
-	400	0.72 αFe 0.28 Fe2B	2.889 –	8.4 –	0.127 –
-	500	0.80 αFe 0.20 Fe2B	2.874 5.114/4.229	8.3 44.7	0.203 0.331
Fe92.2Zr3.0N4.8	As-sput.	~1.00 αFe(Zr,N)	2.904	13.6	0.247
-	300	~1.00 αFe(Zr,N)	2.900	14.0	0.301
-	400	~1.00 αFe(Zr,N)	2.898	14.8	0.270
-	500	~1.00 αFe(Zr,N)	2.883	18.4	0.300
-	600	~1.00 αFe(Zr,N)	2.877	21.4	0.302
Fe88.7Zr4.4N6.9	As-sput.	0.58 αFe(Zr,N) 0.42 Fe4N	2.887 3.818	2.0 5.5	0.066 0.745
-	400	0.61 αFe(Zr,N) 0.39 Fe4N	2.887 3.821	2.0 6.7	0.093 0.635
-	500	0.51 αFe(Zr,N) 0.49 Fe4N	2.872 3.793	2.9 8.2	0.131 0.482
-	600	0.28 αFe(Zr,N) 0.57 Fe3N 0.15 ZrO2	2.864 – 5.087	14.6 3.4 3.7	0.038 0.164 0.379

. The film thickness was measured using a SEM (Figure 2b). Thus, the films of the ternary FeTiB and FeZrN alloys and Fe (Table 1), the thickness of which is from 0.9 to 1.8 µm (Figure 2b), were prepared; the content of impurity oxygen in the films did not exceed 1.5–2 at.%.

3.2. Phase Composition of the Deposited Films

The full-profile XRD patterns taken for all deposited films (Figure 3) exhibit two intense peaks observed at 2θ angles of ~44.5° and ~82°, which are close to the angular positions of (110) and (211) reflections of body-centered cubic (bcc) αFe and are shifted to the lower angles. The shift of the peaks means the formation of a phase with the bcc crystal structure, the lattice parameter (2.890 Å for the Fe_91.6Ti_3.0B_5.4 film and 2.904 Å for the Fe_92.2Zr_3.0N_4.8 film) of which exceeds the tabulated value for αFe (2.866 Å), Table 1. The higher intensity of the (110) peak of the phase, as compared to that of the (110) peak for the αFe standard indicates the formation of the <011> texture in these films. The diffraction patterns taken for the Fe_91.6Ti_3.0B_5.4 films exhibit an additional intense (200) peak of αFe observed at 2θ ~ 64.4°, which indicates a pronounced <001> axial texture of these films. This indicates the formation of bcc αFe-based solid solutions, compositions of which, αFe(Ti) and αFe(Zr,N), in the corresponding films were studied in detail in our previous works [1,9]. Using the diffraction patterns of the Fe_91.6Ti_3.0B_5.4 and Fe_88.7Zr_4.4N_6.9 films, containing the largest amounts of alloying elements (Ti and B or Zr and N), the peaks of additional phases (Fe₂B boride with a tetragonal body-centered lattice and Fe₄N nitride with a face-centered cubic lattice, respectively (Figure 3, Table 1)) were identified. The volume fraction of additional phases does not exceed 0.42.

The XRD patterns of all films contain a wide (integral linewidth of more than 7°) diffuse peak, the angular position of which approaches that of the most intense reflection of αFe (~44.5°, inset in Figure 3). This indicates the formation of a mixed amorphous-crystalline structure in the films.

Upon deposition, a nanocrystalline structure is formed in all films. For single-phase films, the grain size of the bcc phase depends on content of alloying element in the bcc phase; so the grain size of the phase in the FeTiB films (~24–34 nm) is higher than that in the FeZrN films (~13 nm) (Table 1). The effect of the content of alloying element in the αFe-based solid solution on its grain size is confirmed by the biggest grains of the αFe phase in Fe films (~46 nm) (Table 1).

The grain size of the bcc phase in two-phase films is much smaller (8 nm and 2 nm in FeTiB and FeZrN films, respectively) as compared to those in the single-phase films (Table 1). It can be assumed that, upon deposition of two-phase films, the high-temperature phases Fe₂B and Fe₄N phases condense on the substrate before the bcc phase, and, thus, prevent the growth of the bcc phase grains.

It should be noted that the high microstrains are formed in the grains (~0.2–0.3% in the bcc phase grains and ~0.4–0.7% in grains of the boride and nitride phases, Table 1).

3.3. Phase Composition of the Annealed Films

The qualitative estimation of XRD patterns taken for the deposited and annealed films indicates that, after annealing, no significant changes in the reflections of the bcc phase and the boride–nitride phase are observed. This testifies the fact that the principal phase composition of the films was formed in the course of deposition process. The appearance of the third phase (αFe + Fe₃N + ZrO₂) in the Fe_88.7Zr_4.4N_6.9 films after annealing at 600 °C is associated with the occurrence of diffusion processes at the film–glass (SiO₂) interface. However, the quantitative estimations of the XRD patterns taken for all films indicate that, as annealing temperature increases, the lattice parameter of the bcc phase decreases; however, the lattice parameter retain higher than the tabulated lattice parameter of αFe, 2.866 Å, (Table 1). It means that, as annealing temperature increases, a slight depletion of the bcc solid solution in alloying elements occurs. The stability of the film structure formed during deposition is evidenced by both a slight increase in the phase grain sizes and a slight decrease in the microstrain in the grains after annealing.

Note, that TiB and TiB₂ phases in the FeTiB films were not identified although the chemical compositions of these films are close to the stoichiometry Ti:B~1:1 and ~1:2, respectively. The predominance of kinetic factors in the course of the film deposition probably explains this fact. It is also possible that the sensitivity of the XRD method is insufficient to identify a small volume of fine-grained phase.

3.4. Residual Stresses in the Films

The significant compressive stresses (up to ~1.5 GPa) are formed in all deposited films (Figure 4). According to data of [13], residual stresses in the deposited films arise either owing to the difference in coefficients of thermal expansion of the film and substrate (thermal stresses σ_T) or owing to the formation of crystallographic defects in a film material (so-called intrinsic stresses). According to data of [14], the dominant type of stresses is determined by the value of the ratio T_s/T_m, where T_s (K) is the substrate temperature and T_m (K) is the film material melting point. The thermal stresses, σ_T, prevail at T_s/T_m > 0.3; intrinsic stresses prevail at T_s/T_m < 0.3.

Since the melting temperature of the studied films is close to that of iron (1812 K) (the studied films contain at least 88 at.% Fe), and the substrate temperature is 473 K, the ratio T_s/T_m for the deposited films equals ~0.26, i.e., it is less than 0.3; therefore, the intrinsic stresses should prevail in the deposited films. This assumption agrees with experimental data obtained for the deposited films, namely, with the formation of αFe-based supersaturated solid solutions, formation of phases with different crystal lattice parameters (2.866 Å for αFe, 5.100/4.240 Å for Fe₂B, and 3.790 Å for Fe₄N), and unit-cell volumes (23.55 ų for αFe, 110.8 ų for Fe₂B, and 54.44 ų for Fe₄N) and with high level of microstrain in the grains as well (Table 1).

During annealing, as the temperature increases, the compressive stress values decrease and approach zero (Figure 4). The sign of stresses changes to the opposite at 500 °C for the Fe_91.6Ti_3.0B_5.4 films and at 300 °C for the Fe_92.2Zr_3.0N_4.8 films, and the further increase in the temperature results in the formation of tensile stresses of at least 500 MPa. The transition from compressive to tensile stresses after annealing in the temperature range of 300–500 °C was previously observed by us for similar films [15].

It is natural to assume that thermal stresses, σ_T, should prevail in the annealed films. The thermal stresses appear due to the difference in the thermal expansion coefficients of iron α_f and glass α_s (substrate material). The comparison of these values [16,17] gives the inequality:

α_f (11.8 × 10⁻⁶ K⁻¹) > α_s (7 × 10⁻⁶ K⁻¹)

(2)

therefore, according to [5], tensile thermal stresses, σ_T, should form in the studied films. The magnitude of σ_T is described by the dependence

σ_T = (T − T₀)·(α_f − α_s)·E_f/(1 − ν_f)

(3)

where T is the annealing temperature and T₀ is the room temperature. The dashed lines in Figure 4 graphically represent the dependence (3).

In accordance with (3), the thermal stresses σ_T can reach 686 MPa in the film cooled from a temperature of 600 °C. This is in good agreement with the experimentally obtained values of stresses in the studied films annealed at 500 and 600 °C. Thus, the linear dependence (3), plotted as a dashed line in Figure 4, indicates that, as the annealing temperature increases, the contribution of σ_T to the resulting stresses formed in the films becomes predominant.

It should be noted that the possibility to reduce or eliminate residual stresses in the films and thereby to reduce the magnitude of the coercive field of the film via the heat treatment is the efficient way to improve the soft magnetic properties of the film material.

4. Conclusions

• The phase and structural states and the residual stresses of the FeTiB and FeZrN films of various compositions, which were prepared by magnetron deposition on glass substrates and subsequent 1-h annealing at temperatures of 200–600 °C, were studied by X-ray diffraction.
• Depending on the content of alloying elements, Ti and B or Zr and N, in the Fe-based films, a single-phase (bcc αFe(Ti) and αFe(Zr,N) solid solutions) or two-phase (bcc solid solution + Fe₂B boride or Fe₄N nitride) nanocrystalline structure are formed during deposition. The grain size varies (2–46 nm) in accordance with the chemical and phase compositions of the films. All films exhibit the high level of microstrains in grains (~0.2–0.3% for the bcc phase; ~0.4–0.7% for Fe₂B and Fe₄N). The structure formed upon deposition is stable during heating.
• The significant compressive stresses (up to ~1.5 GPa) are formed in all deposited films, which are associated with the formation of αFe-based supersaturated solid solutions in the films, phases with markedly different lattice parameters and unit-cell volumes, and with the high microstrains in the nanograins of the phases as well. As the annealing temperature increases, the values of the compressive stresses decrease, gradually approaching zero, and after that, compressive stresses change into tensile ones, which are related to the different thermal expansion coefficients of iron and glass.
• The heat treatment is the efficient way in order to improve the soft magnetic properties of the studied class of film materials produced by magnetron deposition.