Tailoring Periodic Vertical Cracks in Thermal Barrier Coatings Enabling High Strain Tolerance
Abstract
1. Introduction
2. Model Development
2.1. TBC System Model
2.2. Boundary Conditions
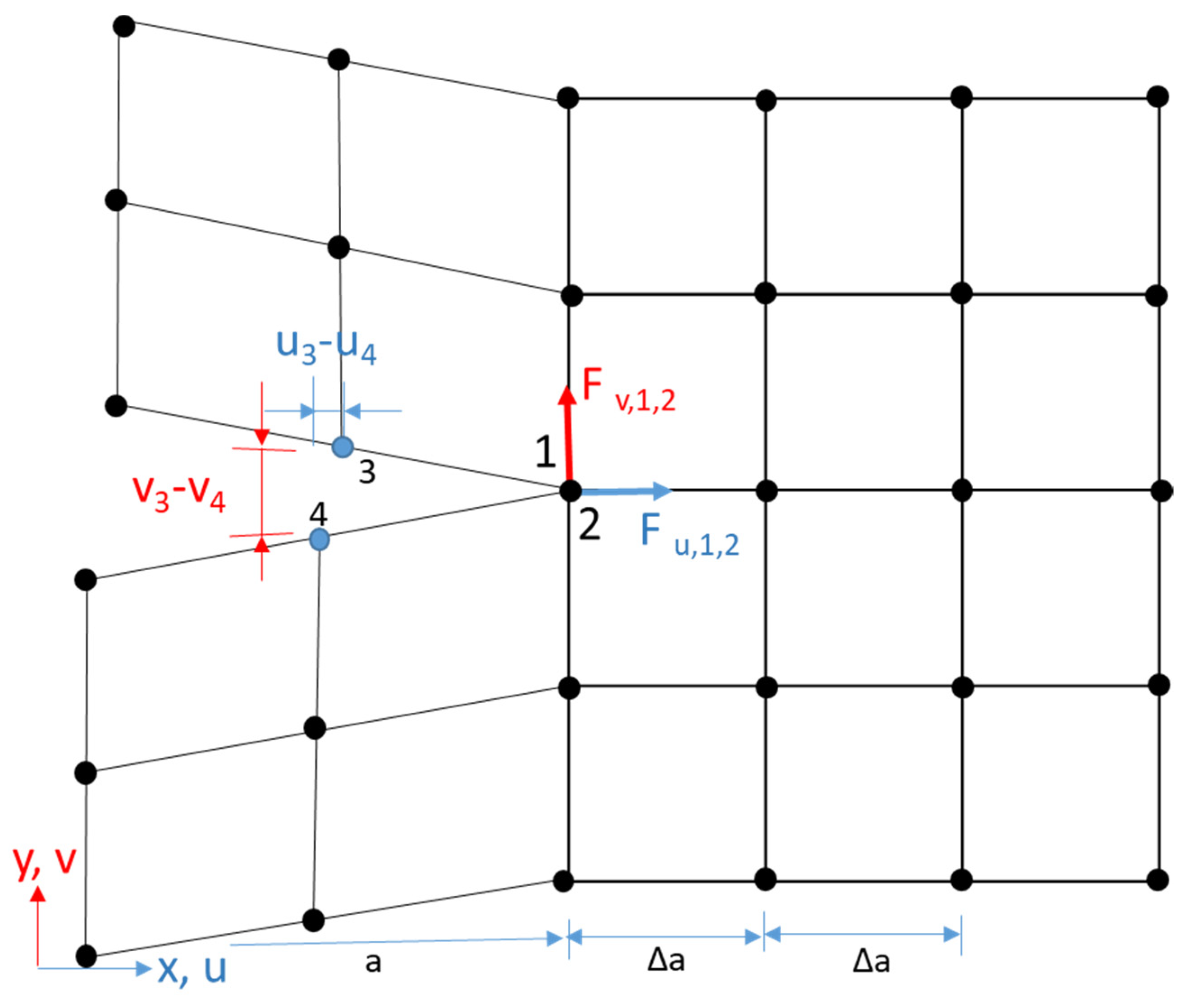
2.3. Cracking Description
3. Results and Discussion
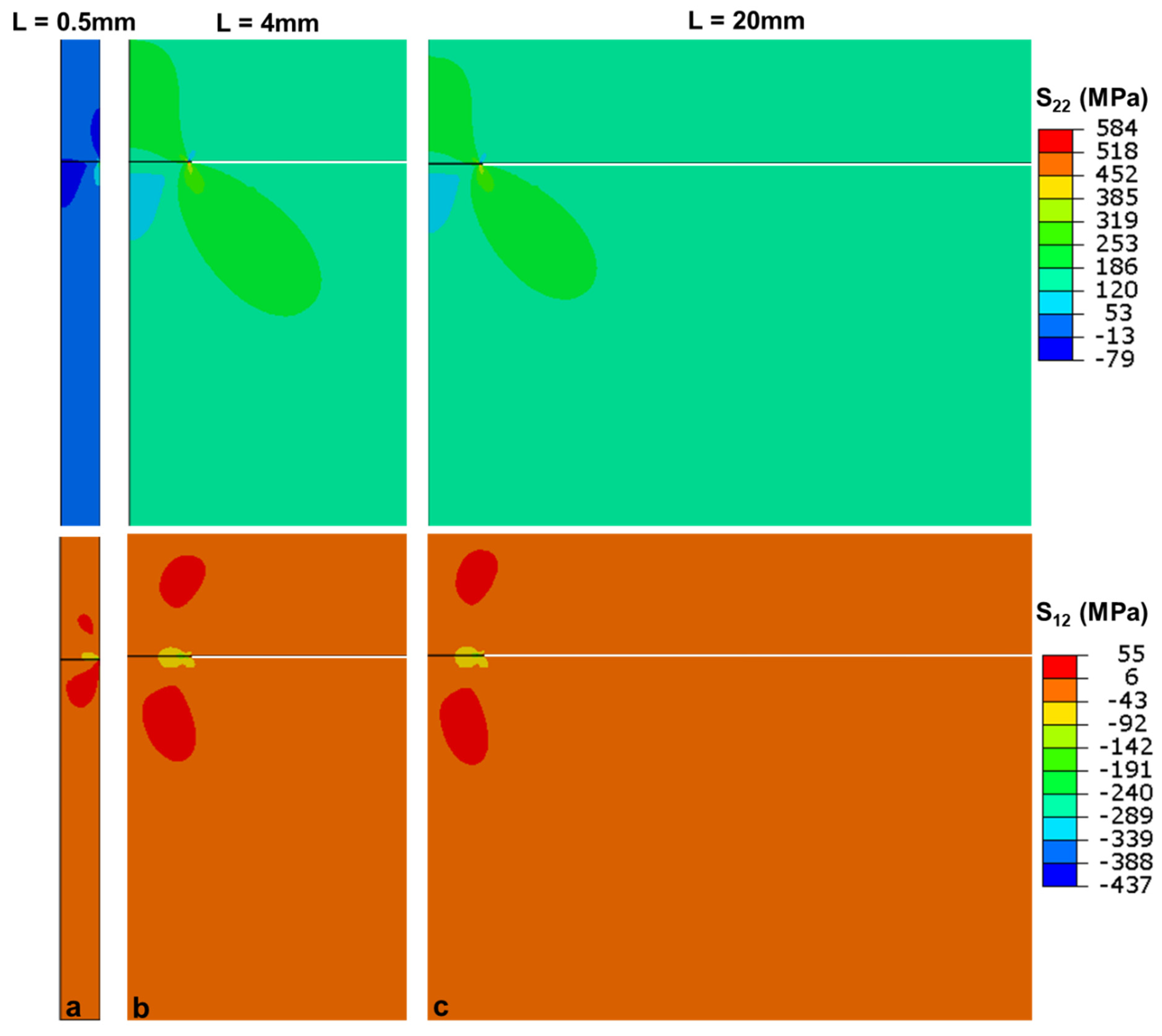
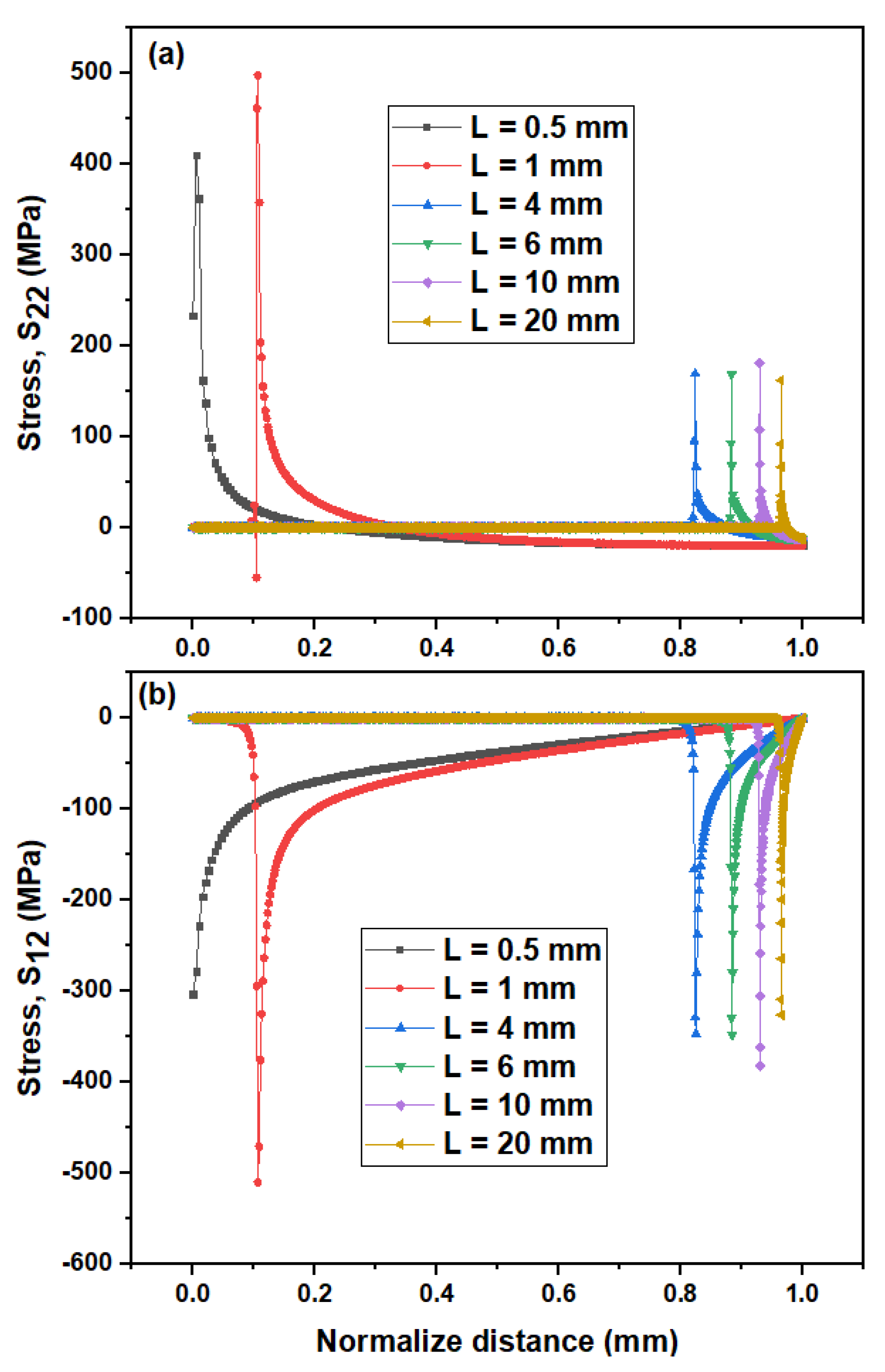
3.1. Influence of L on Stress Distribution in TC
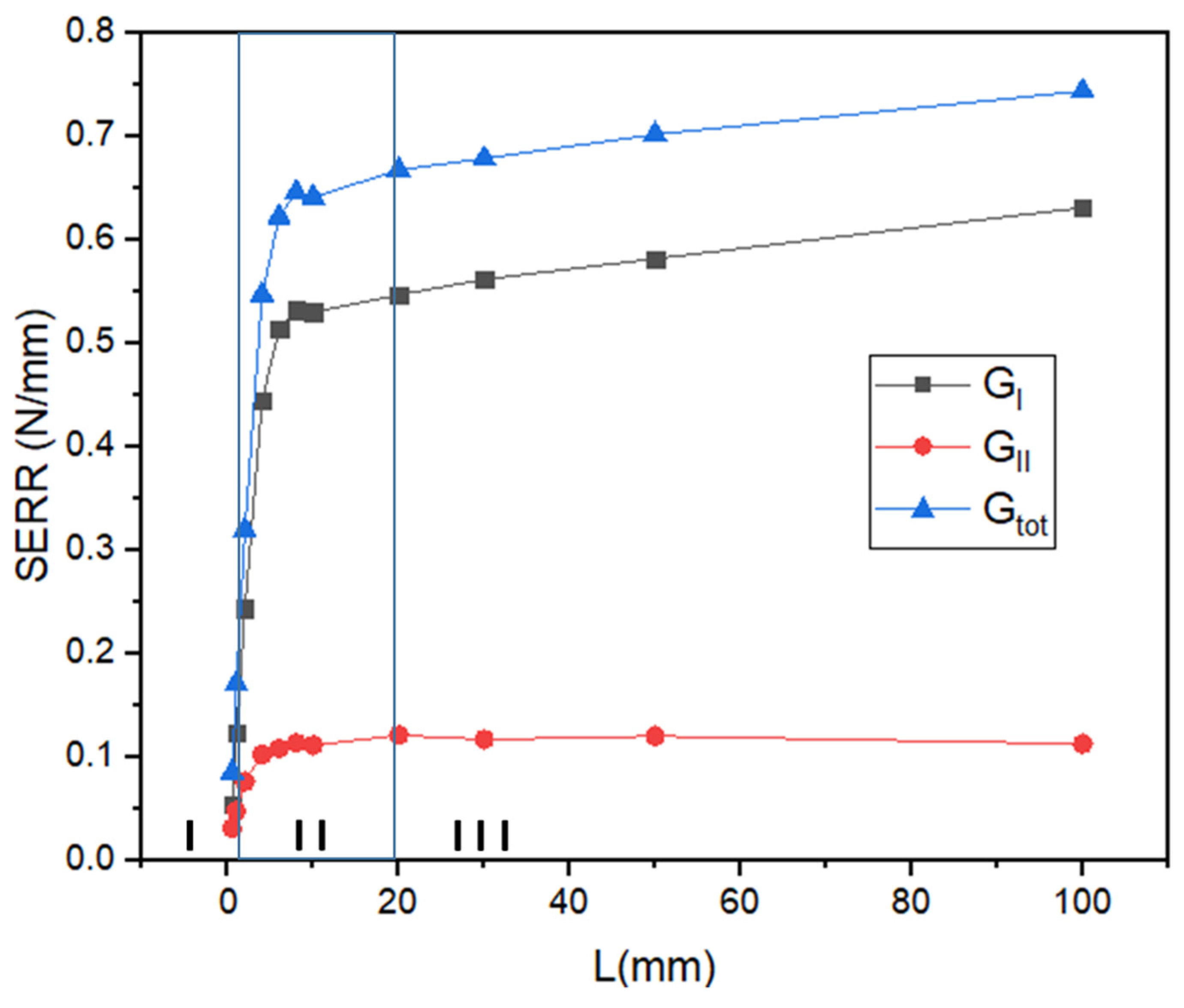
3.2. Effect of Driving Force on Crack Behavior
3.3. TC Crack Growth
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Padture, N.P. Advanced structural ceramics in aerospace propulsion. Nat. Mater. 2016, 15, 804–809. [Google Scholar] [CrossRef]
- s40145-020-0449-7.pdf. Available online: https://link.springer.com/content/pdf/10.1007/s40145-020-0449-7.pdf (accessed on 15 April 2021).
- Li, F.; Zhou, L.; Liu, J.-X.; Liang, Y.; Zhang, G.-J. High-entropy pyrochlores with low thermal conductivity for thermal barrier coating materials. J. Adv. Ceram. 2019, 8, 576–582. [Google Scholar] [CrossRef]
- Yang, L.; Chen, M.; Wang, J.; Qiao, Y.; Guo, P.; Zhu, S.; Wang, F. Microstructure and composition evolution of a single-crystal superalloy caused by elements interdiffusion with an overlay NiCrAlY coating on oxidation. J. Mater. Sci. Technol. 2020, 45, 49–58. [Google Scholar] [CrossRef]
- Guo, L.; Xin, H.; Li, Y.; Yu, Y.; Yan, Z.; Hu, C.; Ye, F. Self-crystallization characteristics of calcium-magnesium-alumina- silicate (CMAS) glass under simulated conditions for thermal barrier coating applications. J. Eur. Ceram. Soc. 2020, 40, 5683–5691. [Google Scholar] [CrossRef]
- Clarke, D.R.; Oechsner, M.; Padture, N.P. Thermal-barrier coatings for more efficient gas-turbine engines. MRS Bull. 2012, 37, 891–898. [Google Scholar] [CrossRef]
- Chen, H.; Zhao, Z.; Xiang, H.; Dai, F.-Z.; Xu, W.; Sun, K.; Liu, J.; Zhou, Y. High entropy (Y0.2Yb0.2Lu0.2Eu0.2Er0.2)3Al5O12: A novel high temperature stable thermal barrier material. J. Mater. Sci. Technol. 2020, 48, 57–62. [Google Scholar] [CrossRef]
- Cao, X.; Vassen, R.; Fischer, W.; Tietz, F.; Jungen, W.; Stöver, D. Lanthanum-cerium oxide as a thermal barrier-coating material for high-temperature applications. Adv. Mater. 2003, 15, 1438–1442. [Google Scholar] [CrossRef]
- Cao, X.Q.; Vassen, R.; Tietz, F.; Stoever, D. New double-ceramic-layer thermal barrier coatings based on zirconia–rare earth composite oxides. J. Eur. Ceram. Soc. 2006, 26, 247–251. [Google Scholar] [CrossRef]
- Cao, X.; Li, J.; Zhong, X.; Zhang, J.; Zhang, Y.; Vassen, R.; Stoever, D. La2(Zr0.7Ce0.3)2O7—A new oxide ceramic material with high sintering-resistance. Mater. Lett. 2008, 62, 2667–2669. [Google Scholar] [CrossRef]
- Wu, P.; Hu, M.Y.; Chong, X.Y.; Feng, J. The glass-like thermal conductivity in ZrO2–Dy3TaO7 ceramic for promising thermal barrier coating application. Appl. Phys. Lett. 2018, 112, 131903. [Google Scholar] [CrossRef]
- Liu, B.; Liu, Y.; Zhu, C.; Xiang, H.; Chen, H.; Sun, L.; Gao, Y.; Zhou, Y. Advances on strategies for searching for next generation thermal barrier coating materials. J. Mater. Sci. Technol. 2019, 35, 833–851. [Google Scholar] [CrossRef]
- Cao, X.Q.; Zhang, Y.F.; Zhang, J.F.; Zhong, X.H.; Wang, Y.; Ma, H.M.; Xu, Z.H.; He, L.M.; Lu, F. Failure of the plasma-sprayed coating of lanthanum hexaluminate. J. Eur. Ceram. Soc. 2008, 28, 1979–1986. [Google Scholar] [CrossRef]
- Li, D.; Jiang, P.; Gao, R.; Sun, F.; Jin, X.; Fan, X. Experimental and numerical investigation on the thermal and mechanical behaviours of thermal barrier coatings exposed to CMAS corrosion. J. Adv. Ceram. 2021. [Google Scholar] [CrossRef]
- Guo, L.; Xin, H.; Zhang, Z.; Zhang, X.; Ye, F. Microstructure modification of Y2O3 stabilized ZrO2 thermal barrier coatings by laser glazing and the effects on the hot corrosion resistance. J. Adv. Ceram. 2020, 9, 232–242. [Google Scholar] [CrossRef]
- Fan, W.; Bai, Y. Review of suspension and solution precursor plasma sprayed thermal barrier coatings. Ceram. Int. 2016, 42, 14299–14312. [Google Scholar] [CrossRef]
- Morelli, S.; Testa, V.; Bolelli, G.; Ligabue, O.; Molinari, E.; Antolotti, N.; Lusvarghi, L. CMAS corrosion of YSZ thermal barrier coatings obtained by different thermal spray processes. J. Eur. Ceram. Soc. 2020, 40, 4084–4100. [Google Scholar] [CrossRef]
- Shakhova, I.; Mironov, E.; Azarmi, F.; Safonov, A. Thermo-electrical properties of the alumina coatings deposited by different thermal spraying technologies. Ceram. Int. 2017, 43, 15392–15401. [Google Scholar] [CrossRef]
- Liu, M.-J.; Zhang, K.-J.; Zhang, Q.; Zhang, M.; Yang, G.-J.; Li, C.-X.; Li, C.-J. Thermodynamic conditions for cluster formation in supersaturated boundary layer during plasma spray-physical vapor deposition. Appl. Surf. Sci. 2019, 471, 950–959. [Google Scholar] [CrossRef]
- Vaßen, R.; Bakan, E.; Mack, D.; Schwartz-Lückge, S.; Sebold, D.; Sohn, Y.J.; Zhou, D.; Guillon, O. Performance of YSZ and Gd2Zr2O7/YSZ double layer thermal barrier coatings in burner rig tests. J. Eur. Ceram. Soc. 2020, 40, 480–490. [Google Scholar] [CrossRef]
- Zhang, X.; Zhou, K.; Xu, W.; Song, J.; Deng, C.; Liu, M. Reaction mechanism and thermal insulation property of al-deposited 7ysz thermal barrier coating. J. Mater. Sci. Technol. 2015, 31, 1006–1010. [Google Scholar] [CrossRef]
- Wang, L.; Liu, C.G.; Zhong, X.H.; Zhao, Y.X.; Zhao, H.Y.; Yang, J.S.; Tao, S.Y.; Wang, Y. Investigation of crack propagation behavior of atmospheric plasma-sprayed thermal barrier coatings under uniaxial tension using the acoustic emission technique. J. Therm. Spray Tech. 2015, 24, 296–308. [Google Scholar] [CrossRef]
- Gupta, M.; Li, X.-H.; Markocsan, N.; Kjellman, B. Design of high lifetime suspension plasma sprayed thermal barrier coatings. J. Eur. Ceram. Soc. 2020, 40, 768–779. [Google Scholar] [CrossRef]
- Lashmi, P.G.; Ananthapadmanabhan, P.V.; Unnikrishnan, G.; Aruna, S.T. Present status and future prospects of plasma sprayed multilayered thermal barrier coating systems. J. Eur. Ceram. Soc. 2020, 40, 2731–2745. [Google Scholar] [CrossRef]
- Wei, Z.-Y.; Cai, H.-N.; Li, C.-J. Comprehensive dynamic failure mechanism of thermal barrier coatings based on a novel crack propagation and TGO growth coupling model. Ceram. Int. 2018, 44, 22556–22566. [Google Scholar] [CrossRef]
- Cheng, B.; Yang, N.; Zhang, Q.; Zhang, M.; Zhang, Y.-M.; Chen, L.; Yang, G.-J.; Li, C.-X.; Li, C.-J. Sintering induced the failure behavior of dense vertically crack and lamellar structured TBCs with equivalent thermal insulation performance. Ceram. Int. 2017, 43, 15459–15465. [Google Scholar] [CrossRef]
- Wang, L.; Li, D.C.; Yang, J.S.; Shao, F.; Zhong, X.H.; Zhao, H.Y.; Yang, K.; Tao, S.Y.; Wang, Y. Modeling of thermal properties and failure of thermal barrier coatings with the use of finite element methods: A review. J. Eur. Ceram. Soc. 2016, 36, 1313–1331. [Google Scholar] [CrossRef]
- Mehboob, G.; Liu, M.-J.; Xu, T.; Hussain, S.; Mehboob, G.; Tahir, A. A review on failure mechanism of thermal barrier coatings and strategies to extend their lifetime. Ceram. Int. 2020, 46, 8497–8521. [Google Scholar] [CrossRef]
- Dong, T.-S.; Wang, R.; Di, Y.-L.; Wang, H.-D.; Li, G.-L.; Fu, B.-G. Mechanism of high temperature oxidation resistance improvement of double-layer thermal barrier coatings (TBCs) by La. Ceram. Int. 2019, 45, 9126–9135. [Google Scholar] [CrossRef]
- Abubakar, A.A.; Arif, A.F.M.; Al-Athel, K.S.; Akhtar, S.S.; Mostaghimi, J. Modeling residual stress development in thermal spray coatings: Current status and way forward. J. Therm. Spray Tech. 2017, 26, 1115–1145. [Google Scholar] [CrossRef]
- Chen, L.; Yang, G.-J. Epitaxial growth and cracking of highly tough 7YSZ splats by thermal spray technology. J. Adv. Ceram. 2018, 7, 17–29. [Google Scholar] [CrossRef]
- Li, G.; Yang, G. Understanding of degradation-resistant behavior of nanostructured thermal barrier coatings with bimodal structure. J. Mater. Sci. Technol. 2019, 35, 231–238. [Google Scholar] [CrossRef]
- Mao, W.; Wang, Y.; Shi, J.; Huang, H.; Wang, Y.; Lv, L.; Yang, H.; Zou, C.; Dai, C.; Zhu, X.; et al. Bending fracture behavior of freestanding (Gd0.9Yb0.1)2Zr2O7 coatings by using digital image correlation and FEM simulation with 3D geometrical reconstruction. J. Adv. Ceram. 2019, 8, 564–575. [Google Scholar] [CrossRef]
- Benini, E. Progress in Gas Turbine Performance; BoD-Books on Demand: Norderstedt, Germany, 2013. [Google Scholar]
- Chen, L.; Hu, M.; Guo, J.; Chong, X.; Feng, J. Mechanical and thermal properties of RETaO4 (RE = Yb, Lu, Sc) ceramics with monoclinic-prime phase. J. Mater. Sci. Technol. 2020, 52, 20–28. [Google Scholar] [CrossRef]
- Li, J.; Wei, L.; He, J.; Chen, H.; Guo, H. The role of Re in improving the oxidation-resistance of a Re modified PtAl coating on Mo-Rich single crystal superalloy. J. Mater. Sci. Technol. 2020, 58, 63–72. [Google Scholar] [CrossRef]
- Yang, Z.; Zhang, P.; Pan, W.; Han, Y.; Huang, M.; Chen, H.; Gong, Q.; Wan, C. Thermal and oxygen transport properties of complex pyrochlore RE2 In TaO7 for thermal barrier coating applications. J. Eur. Ceram. Soc. 2020, 40, 6229–6235. [Google Scholar] [CrossRef]
- Al Zoubi, W.; Kamil, M.P.; Fatimah, S.; Nashrah, N.; Ko, Y.G. Recent advances in hybrid organic-inorganic materials with spatial architecture for state-of-the-art applications. Prog. Mater. Sci. 2020, 112, 100663. [Google Scholar] [CrossRef]
- Al Zoubi, W.; Ko, Y.G. Chemical stability of synergistic inorganic materials for enhancing electrochemical performance. Compos. Sci. Technol. 2020, 199, 108383. [Google Scholar] [CrossRef]
- Xing, Q.; Shu-ai, Z.; Xiaofeng, G.; Renping, Y.; Yi, L.; Hui, T.; Shicheng, W.; Yongchao, F.; Hao, W.; Shengjian, X. Microstructure and thermal shock resistance of Nd2O3-doped YSZ-based thermal barrier coatings. Ceram. Int. 2020, 46, 26841–26853. [Google Scholar] [CrossRef]
- Izadinia, M.; Soltani, R.; Sohi, M.H. Effect of segmented cracks on TGO growth and life of thick thermal barrier coating under isothermal oxidation conditions. Ceram. Int. 2020, 46, 7475–7481. [Google Scholar] [CrossRef]
- Lv, B.; Jin, X.; Cao, J.; Xu, B.; Wang, Y.; Fang, D. Advances in numerical modeling of environmental barrier coating systems for gas turbines. J. Eur. Ceram. Soc. 2020, 40, 3363–3379. [Google Scholar] [CrossRef]
- Zhu, Y.; Yan, B.; Cai, D.; Wu, K.; Zhang, X. Structural parameter study on stress intensity factors of interfacial crack in thermal barrier coatings. Ceram. Int. 2021, 47, 14354–14365. [Google Scholar] [CrossRef]
- Abdul-Baqi, A.; van der Giessen, E. Indentation-induced interface delamination of a strong film on a ductile substrate. Thin Solid Film. 2001, 381, 143–154. [Google Scholar] [CrossRef]
- Rezaei, S.; Wulfinghoff, S.; Reese, S. Prediction of fracture and damage in micro/nano coating systems using cohesive zone elements. Int. J. Solids Struct. 2017, 121, 62–74. [Google Scholar] [CrossRef]
- Holmberg, K.; Laukkanen, A.; Ronkainen, H.; Wallin, K. Finite element analysis of coating adhesion failure in pre-existing crack field. Tribol. Mater. Surf. Interfaces 2013, 7, 42–51. [Google Scholar] [CrossRef]
- Wei, Z.-Y.; Cai, H.-N.; Meng, G.-H.; Tahir, A.; Zhang, W.-W. An innovative model coupling TGO growth and crack propagation for the failure assessment of lamellar structured thermal barrier coatings. Ceram. Int. 2020, 46, 1532–1544. [Google Scholar] [CrossRef]
- Rybicki, E.F.; Kanninen, M.F. A finite element calculation of stress intensity factors by a modified crack closure integral. Eng. Fract. Mech. 1977, 9, 931–938. [Google Scholar] [CrossRef]
- Ming-Che, L.; Erdogan, F. Stress intensity factors in two bonded elastic layers containing cracks perpendicular to and on the interface—I. Analysis. Eng. Fract. Mech. 1983, 18, 491–506. [Google Scholar] [CrossRef]
- Wu, C.-W.; Chen, G.-N.; Zhang, K.; Luo, G.-X.; Liang, N.-G. The effect of periodic segmentation cracks on the interfacial debonding: Study on interfacial stresses. Surf. Coat. Technol. 2006, 201, 287–291. [Google Scholar] [CrossRef][Green Version]
- Zhou, B.; Kokini, K. Effect of pre-existing surface crack morphology on the interfacial thermal fracture of thermal barrier coatings: A numerical study. Mater. Sci. Eng. A 2003, 348, 271–279. [Google Scholar] [CrossRef]
- Zhou, B.; Kokini, K. Effect of surface pre-crack morphology on the fracture of thermal barrier coatings under thermal shock. Acta Mater. 2004, 52, 4189–4197. [Google Scholar] [CrossRef]
- Mehboob, G.; Xu, T.; Li, G.-R.; Hussain, S.; Mehboob, G.; Tahir, A. Strain-Induced Cracking Behavior of Coating/Substrate Systems and Strain Tolerant Design for Thick Coatings. Coatings 2020, 10, 1066. [Google Scholar] [CrossRef]
- Lee, D.H.; Lee, K.S.; Kim, T.W.; Kim, C. Hertzian stress analysis and characterization of thermal barrier coatings containing unidirectional vertical cracks. Ceram. Int. 2019, 45, 21348–21358. [Google Scholar] [CrossRef]
- Wang, L.; Zhong, X.H.; Shao, F.; Ni, J.X.; Yang, J.S.; Tao, S.Y.; Wang, Y. What is the suitable segmentation crack density for atmospheric plasma sprayed thick thermal barrier coatings with the improved thermal shock resistance? Appl. Surf. Sci. 2018, 431, 101–111. [Google Scholar] [CrossRef]
- Fan, X.L.; Xu, R.; Zhang, W.X.; Wang, T.J. Effect of periodic surface cracks on the interfacial fracture of thermal barrier coating system. Appl. Surf. Sci. 2012, 258, 9816–9823. [Google Scholar] [CrossRef]
- Wei, Z.-Y.; Cai, H.-N.; Feng, R.-X.; Su, J.-Y. Dynamic crack growth mechanism and lifetime assessment in plasma sprayed thermal barrier system upon temperature cycling. Ceram. Int. 2019, 45, 14896–14907. [Google Scholar] [CrossRef]
- Rabiei, A.; Evans, A.G. Failure mechanisms associated with the thermally grown oxide in plasma-sprayed thermal barrier coatings. Acta Mater. 2000, 48, 3963–3976. [Google Scholar] [CrossRef]
- Wang, L.; Yang, J.S.; Ni, J.X.; Liu, C.G.; Zhong, X.H.; Shao, F.; Zhao, H.Y.; Tao, S.Y.; Wang, Y. Influence of cracks in APS-TBCs on stress around TGO during thermal cycling: A numerical simulation study. Surf. Coat. Technol. 2016, 285, 98–112. [Google Scholar] [CrossRef]
- AD0099305.pdf. Available online: https://apps.dtic.mil/sti/pdfs/AD0099305.pdf (accessed on 10 February 2021).
- Zhu, W.; Zhang, Z.B.; Yang, L.; Zhou, Y.C.; Wei, Y.G. Spallation of thermal barrier coatings with real thermally grown oxide morphology under thermal stress. Mater. Des. 2018, 146, 180–193. [Google Scholar] [CrossRef]
- Lu, S.; Huang, J.; Song, L.; Yi, M. A study on zoning coating method of absorbing materials for stealth aircraft. Optik 2020, 208, 163912. [Google Scholar] [CrossRef]
- Madhwal, M.; Jordan, E.H.; Gell, M. Failure mechanisms of dense vertically-cracked thermal barrier coatings. Mater. Sci. Eng. A 2004, 384, 151–161. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mehboob, G.; Xu, T.; Li, G.-R.; Yang, G.-J.; Tahir, A.; Ragab, M.; Hussain, S. Tailoring Periodic Vertical Cracks in Thermal Barrier Coatings Enabling High Strain Tolerance. Coatings 2021, 11, 720. https://doi.org/10.3390/coatings11060720
Mehboob G, Xu T, Li G-R, Yang G-J, Tahir A, Ragab M, Hussain S. Tailoring Periodic Vertical Cracks in Thermal Barrier Coatings Enabling High Strain Tolerance. Coatings. 2021; 11(6):720. https://doi.org/10.3390/coatings11060720
Chicago/Turabian StyleMehboob, Ghazanfar, Tong Xu, Guang-Rong Li, Guan-Jun Yang, Adnan Tahir, Mohamed Ragab, and Shahnwaz Hussain. 2021. "Tailoring Periodic Vertical Cracks in Thermal Barrier Coatings Enabling High Strain Tolerance" Coatings 11, no. 6: 720. https://doi.org/10.3390/coatings11060720
APA StyleMehboob, G., Xu, T., Li, G.-R., Yang, G.-J., Tahir, A., Ragab, M., & Hussain, S. (2021). Tailoring Periodic Vertical Cracks in Thermal Barrier Coatings Enabling High Strain Tolerance. Coatings, 11(6), 720. https://doi.org/10.3390/coatings11060720






