Modeling and Simulation of Vacuum Low Pressure Carburizing Process in Gear Steel
Abstract
:1. Introduction
2. Model Descriptions
- Temperature changes affect the diffusion rate of carbon atoms in austenite. Moreover, the diffusion process and subsequent phase transformation during the cooling process generate latent heat.
- With the increase in carbon concentration, the diffusion ability of carbon in austenite increases. In addition, the precipitation of alloy carbides can affect carbon diffusion during carburizing.
- The increase in the carbon concentration gradient in the carburized layer causes the appearance of diffusion stress. The non-uniform distribution of stress also affects the diffusion process of carbon atoms and the kinetic process of phase transformation, while the existence of an internal stress gradient affects the diffusion mechanism of carbon in steel.
2.1. Temperature Field Calculation
2.2. Diffusion Field Calculation
- Carburizing medium for chemical decomposition;
- Carbon atoms were transferred into the surface of the steel part;
- Due to the existence of a potential carbon concentration gradient, carbon atoms diffuse into the steel.
2.3. Phase Transformation Calculation
2.4. Hardness Regression Equation
2.5. Establishment of Multi-Field Multi-Scale Model for Low Pressure Vacuum Carburizing
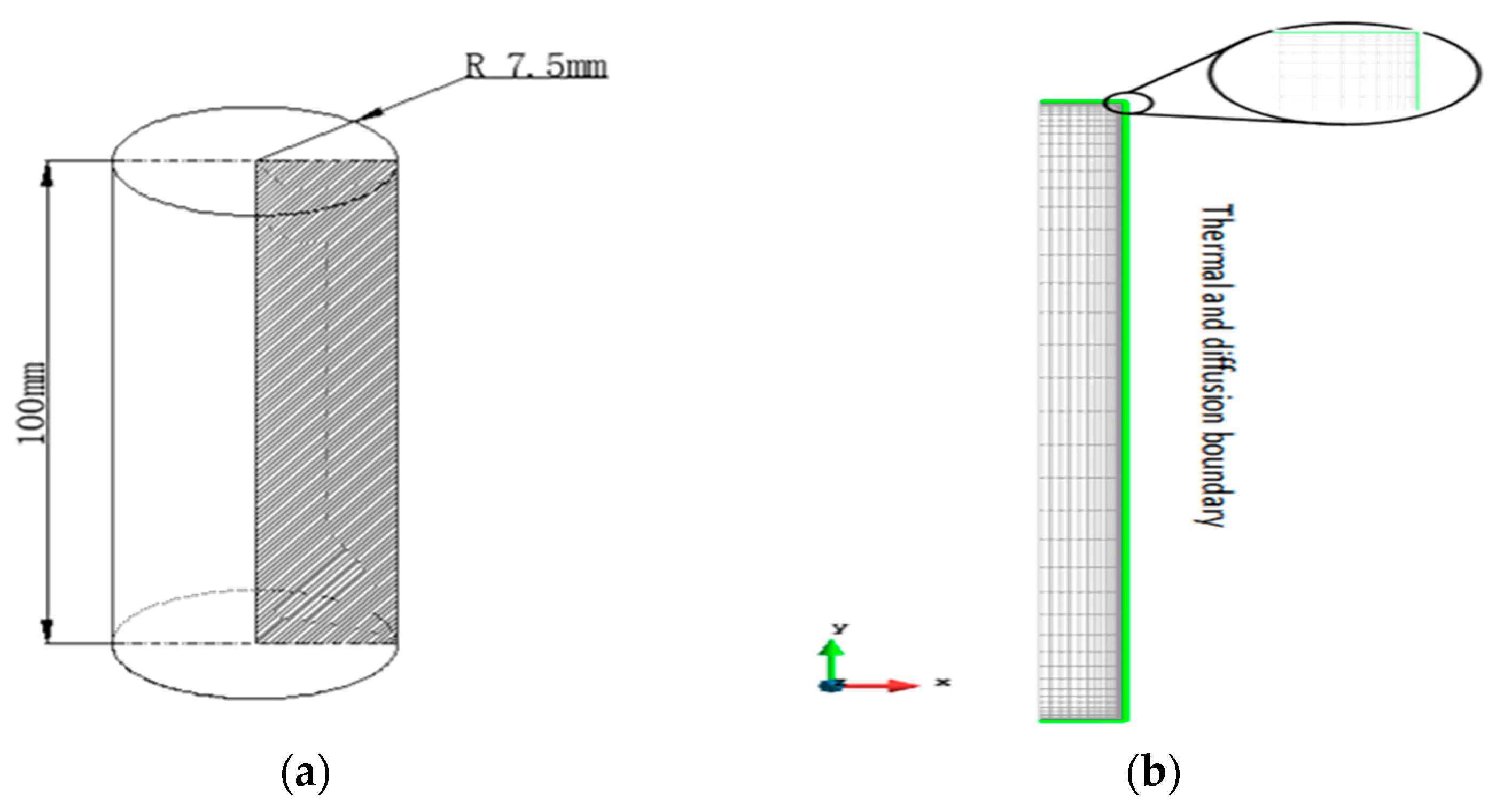
3. Material and Methods
3.1. Experimental Material
3.2. Experimental Methods
4. Results and Discussion
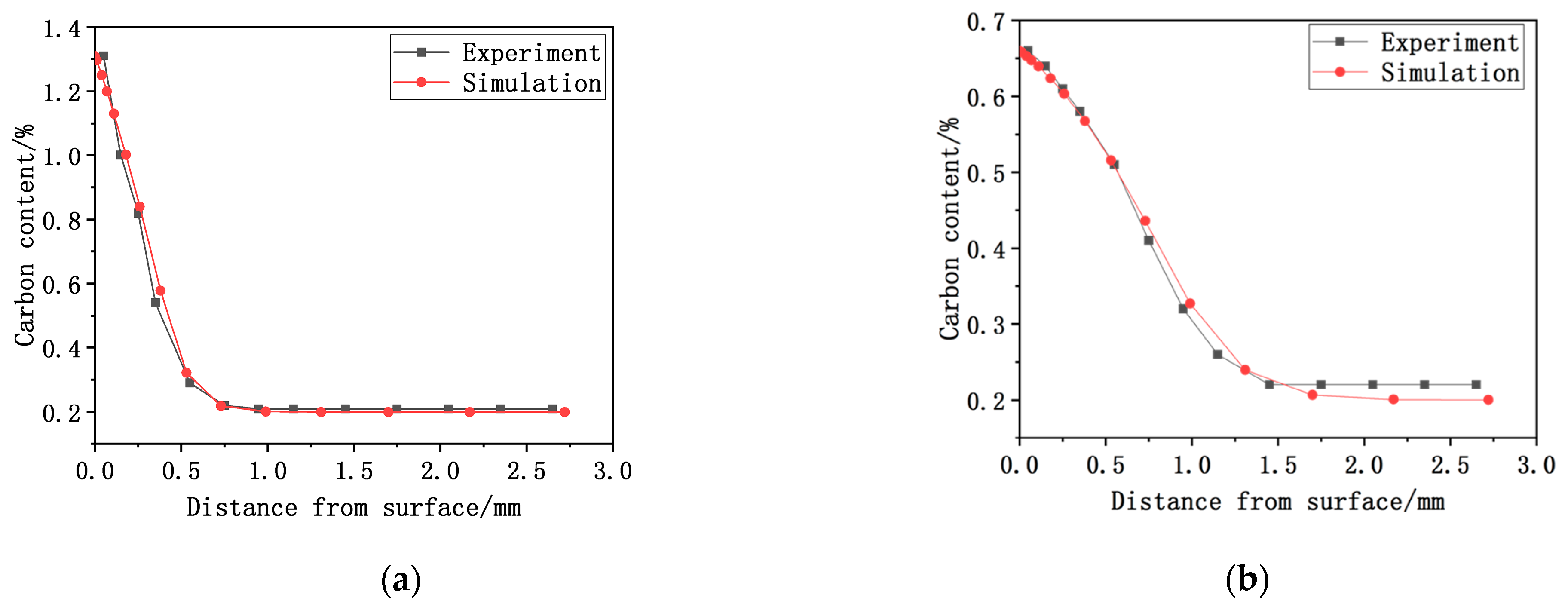
4.1. Carbon Concentration Analysis
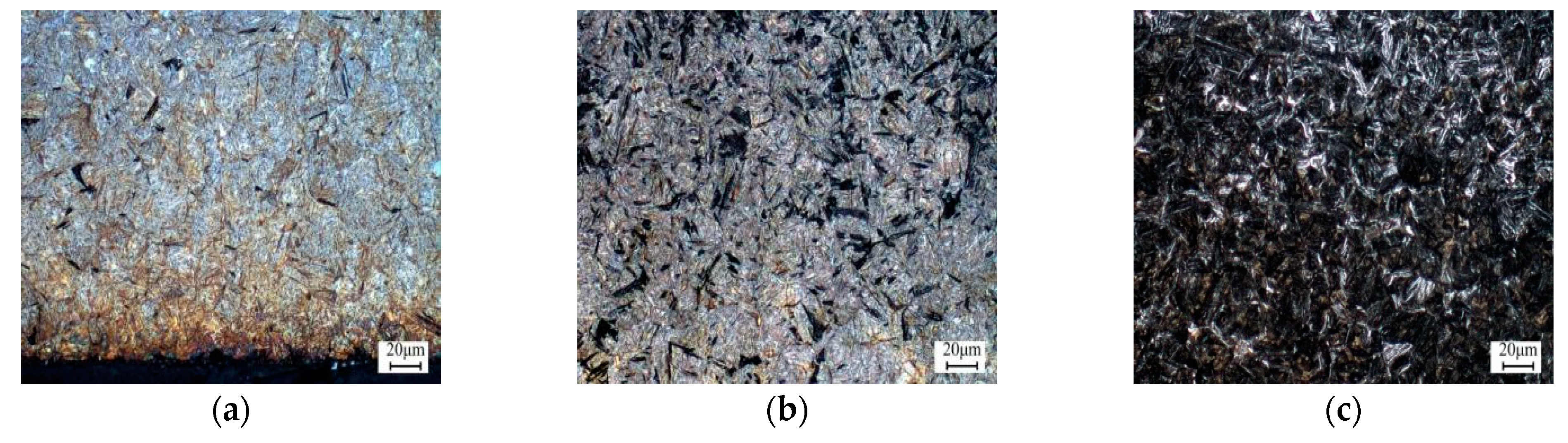
4.2. Microstructure and Hardness Analysis
4.3. Optimization Analysis
5. Conclusions
- The vacuum carburizing process under different process conditions was simulated and compared with the experimental results. The results show that the simulated surface carbon concentration results and experimental results are in good agreement. The maximum difference between the experimental and simulated carburized layer depth of process 2 is 0.052 mm. The error between simulation and experiment of the depth of the carburizing layer is less than 6%. The reason for the error in carbon concentration distribution may be related to the diffusion coefficient. The diffusion coefficient of the simulation calculation is considered such that the alloy composition and temperature of the sample are uniform. However, the alloy composition and temperature of the actual vacuum carburizing sample may be uneven.
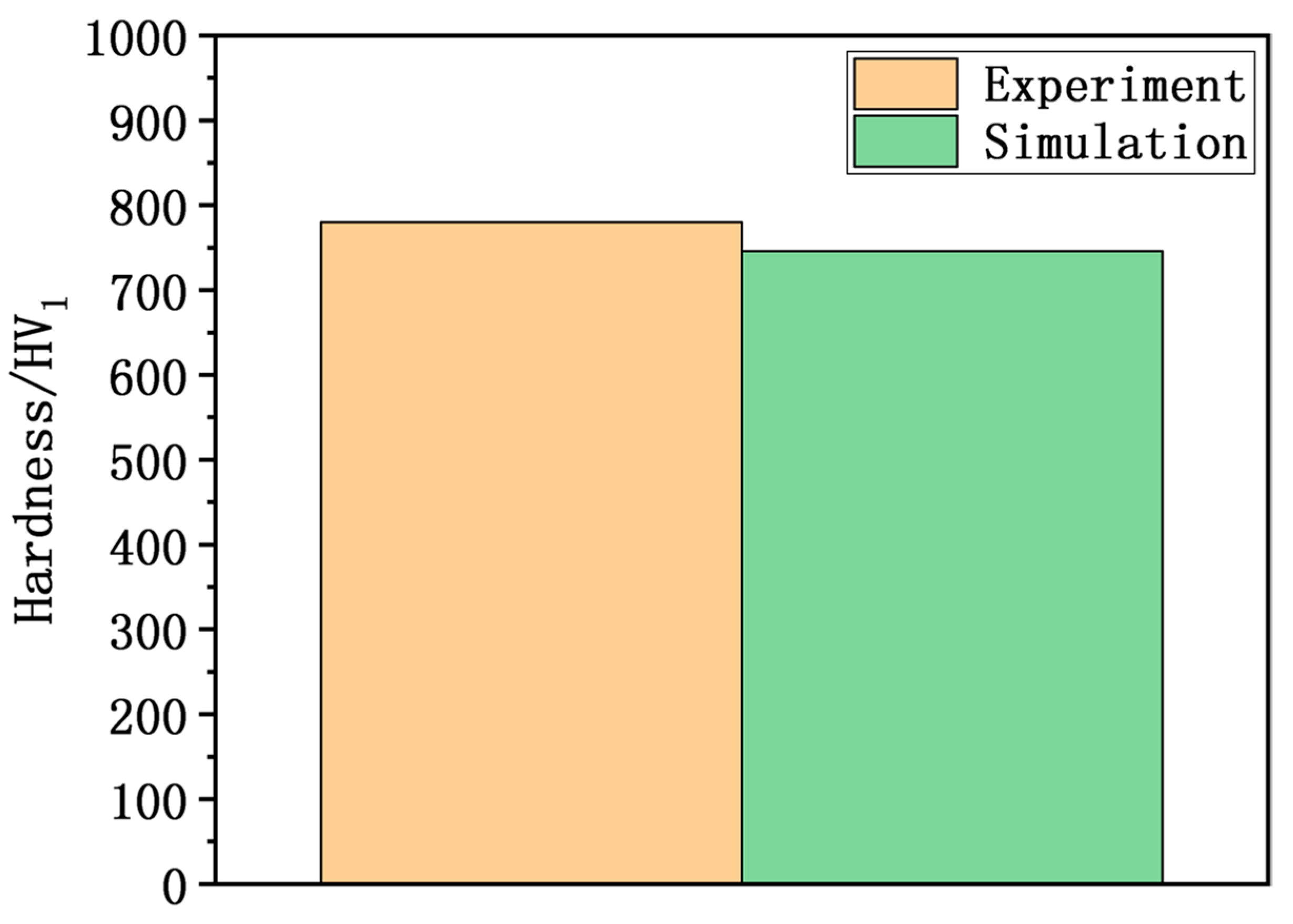
- The distribution and evolution of the martensite fraction and hardness are basically the same, but the hardness of the experimental surface is relatively high. The simulated surface hardness is 746 HV, and the difference between simulation and experiment is 34 HV. The error between the simulation and experiment of surface hardness is less than 5%. The main reason for the hardness error is that the measurement of the experimental hardness is in a small area, while the simulated hardness calculation is mainly carried out for the average value of the simulated domain.
- In order to achieve the corresponding carburizing target, vacuum carburizing processes with different diffusion times were developed for simulation. With an increase in diffusion time, the depth of the carburized layer increases. In contrast, surface carbon concentration decreases significantly. The results show that when the surface carbon concentration is 0.80% and the depth of carburizing layer is 0.90 mm, the optimal process parameters are a carburizing time of 42 min and a diffusion time of 105 min for L1.
- The simulation and optimization of vacuum low-pressure carburizing provide a reference for the formulation of the vacuum carburizing process for practical production and application. It can reduce the optimization cost of gear vacuum carburizing and improve production efficiency. Moreover, it can also improve the performance of vacuum carburizing furnaces and promote the development of vacuum carburizing technology.
- Future work will focus on the effects of carburizing temperature, carburizing pressure, and flow rate on the structure and property of vacuum carburizing. In addition, further research is necessary to simulate the residual stress and distortions in the vacuum carburizing of complex workpieces.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Qin, M. Vacuum carburizing and high-pressure gas quenching technology in automotive industry. Int. Heat. Treat. Surf. Eng. 2013, 2, 116–120. [Google Scholar] [CrossRef]
- Li, Q.; Cong, P.; Wang, H. Vacuum carburizing for 18CrNiMo7-6 steel gear. Metal. Heat. Treat. 2017, 42, 69–71. [Google Scholar]
- Ikehata, H.; Tanaka, K.; Takamiya, H. Modeling of Growth and Dissolution of Grain Boundary Cementite in Vacuum Carburizing Process. Solid State Phenom. 2011, 172, 1177–1182. [Google Scholar] [CrossRef]
- Wang, B.; He, Y.; Liu, Y. Mechanism of the Microstructural Evolution of 18Cr2Ni4WA Steel during Vacuum Low-Pressure Carburizing Heat Treatment and Its Effect on Case Hardness. Materials 2020, 13, 2352. [Google Scholar] [CrossRef]
- Sawicki, J.; Krupanek, K.; Stachurski, W. Algorithm Scheme to Simulate the Distortions during Gas Quenching in a Single-Piece Flow Technology. Coatings 2020, 10, 694. [Google Scholar] [CrossRef]
- Tian, Y.; Wang, H.; An, X. Experimental study on carbon flux in vacuum carburizing. Mater. Res. Express 2019, 6, 096516. [Google Scholar] [CrossRef]
- Woowiec-Korecka, E.; Korecki, M.; Sut, M. Calculation of the Mixture Flow in a Low-Pressure Carburizing Process. Metals 2019, 9, 439. [Google Scholar] [CrossRef] [Green Version]
- Wang, H.; Liu, J.; Tian, Y.; Wang, Z.; An, X. Mathematical Modeling of Carbon Flux Parameters for Low-Pressure Vacuum Carburizing with Medium-High Alloy Steel. Coatings 2020, 10, 1075. [Google Scholar] [CrossRef]
- Derevyanov, M.Y.; Livshits, M.Y.; Yakubovich, E.A. Vacuum Carburizing Process: Identification of Mathematical Model and Optimization. IOP Conf. Ser. Mater. Sci. Eng. 2018, 327, 022022. [Google Scholar] [CrossRef] [Green Version]
- Wołowiec-Korecka, E. Modeling methods for gas quenching, low-pressure carburizing and low-pressure nitriding. Eng. Struct. 2018, 177, 489–505. [Google Scholar] [CrossRef]
- Heintzberger, P.J. Influence of the Temperature of Vacuum Carburizing on the Thickness of the Carburized Layer and Properties of Steel Parts. Met. Sci. Heat. Treat. 2020, 62, 279–284. [Google Scholar] [CrossRef]
- Wołowiec, E.; Kula, P. The application of artificial neural networks in optimization of heat treatment processes of steel. J. Appl. Comp. Sci. 2011, 19, 161–169. [Google Scholar]
- Zajusz, M.; Tkacz-Śmiech, K.; Danielewski, M. Modeling of vacuum pulse carburizing of steel. Surf. Coat. Tech. 2014, 258, 646–651. [Google Scholar] [CrossRef]
- Kim, D.; Cho, Y.; Cho, H.; Kim, S.; Lee, W.; Lee, M.; Han, H.N. A numerical model for vacuum carburization of an automotive gear ring. Met. Mater. Int. 2011, 17, 885–890. [Google Scholar] [CrossRef]
- Wei, Y.; Zhang, L.R., Jr. Modeling of Carbon Concentration Profile Development During Both Atmosphere and Low-Pressure Carburizing Processes. J. Mater. Eng. Perform. 2013, 22, 1886–1891. [Google Scholar] [CrossRef]
- Kula, P.; Dybowski, K.; Wolowiec, E. “Boost-diffusion” vacuum carburizing-Process optimisation. Vacuum 2014, 99, 175–179. [Google Scholar] [CrossRef]
- Zajusz, M.; Tkacz-Śmiech, K.; Dychtoń, K.; Danielewski, M. Pulse Carburization of Steel-Model of the Process. Defect. Diff. Forum. 2014, 354, 145–152. [Google Scholar] [CrossRef]
- Tibbetts, G.G. Diffusivity of carbon in iron and steels at high temperatures. J. Appl. Phys. 1980, 51, 4813–4816. [Google Scholar] [CrossRef]
- Dong, Q.Z.; Zhu, Z.W. Problems and solutions for determination of carbon transfer coefficient β using steel foil carburising method. Int. Heat. Treat. Surf. Eng. 2014, 8, 162–167. [Google Scholar] [CrossRef]
- Karabelchtchikova, O.; Sisson, R.D. Calculation of Gas Carburizing Kinetics from Carbon Concentration Profiles based on Direct Flux Integration. Defect. Diff. Forum. 2007, 266, 171–180. [Google Scholar] [CrossRef]
- Goldstein, J.I.; Moren, A.E. Diffusion modeling of the carburization process. Metal. Mater. Trans. 1978, 9, 1515–1525. [Google Scholar] [CrossRef]
- Kula, P.; Pietrasik, R.; Dybowski, K. Vacuum carburizing-process optimization. J. Mater. Process. Tech. 2005, 164, 876–881. [Google Scholar] [CrossRef]
- Rokicki, K.P. Acetylene Flow Rate as a Crucial Parameter of Vacuum Carburizing Process of Modern Tool Steels. Arch. Metal. Mater. 2016, 61, 2009–2012. [Google Scholar] [CrossRef]
- Semenov, M.Y.; Smirnov, A.E.; Ryzhova, M.Y. Computation of carbon concentration curves in vacuum carburizing of steels. Met. Sci. Heat. Treat. 2013, 55, 38–42. [Google Scholar] [CrossRef]
- Jung, M.; Oh, S.; Lee, Y. Predictive model for the carbon concentration profile of vacuum carburized steels with acetylene. Met. Mater. Int. 2009, 15, 971–975. [Google Scholar] [CrossRef]
- Ryzhov, N.M.; Smirnov, A.E.; Fakhurtdinov, R.S. Control of Carbon Saturation of the Diffusion Layer in Vacuum Carburizing of Heat-Resistant Steels. Met. Sci. Heat. Treat. 2004, 46, 340–344. [Google Scholar] [CrossRef]
- Ochsner, A.; Gegner, J.; Mishuris, G. Effect of Diffusivity as a Function of the Method of Computation of Carbon Concentration Profiles in Steel. Met. Sci. Heat. Treat. 2004, 46, 148–151. [Google Scholar] [CrossRef]
- Buchholz, D.; Khan, R.U.; Bajohr, S.; Reimert, R. Computational Fluid Dynamics Modeling of Acetylene Pyrolysis for Vacuum Carburizing of Steel. Ind. Eng. Chem. Res. 2010, 49, 1130–1137. [Google Scholar] [CrossRef]
- Gegner, J.; Vasilyev, A.A.; Wilbrandt, P.J. Alloy Dependence of the Diffusion Coefficient of Carbon in Austenite and Analysis of Carburization Profiles in Case Hardening of Steels. In Proceedings of the MMT’2012, Ariel, Israel, 20 August 2012. [Google Scholar]
- Liu, C.; Ju, D.Y.; Inoue, T. A Numerical Modeling of Metallo-thermo-mechanical Behavior in Both Carburized and Carbonitrided Quenching Processes. ISIJ Int. 2002, 42, 1125–1134. [Google Scholar] [CrossRef] [Green Version]
- Deng, X.; Ju, D. Modelling and simulation of nitriding process in SCM420 steel. Int. J. Microstruct. Mater. Prop. 2017, 12, 5–6. [Google Scholar]
- Lee, S.J.; Matlock, D.K.; Tyne, C.J. An Empirical Model for Carbon Diffusion in Austenite Incorporating Alloying Element Effects. ISIJ Int. 2011, 51, 1903–1911. [Google Scholar] [CrossRef] [Green Version]
- Deng, X.; Ju, D. Modeling and Simulation of Quenching and Tempering Process in steels. Phys. Proc. 2013, 50, 368–374. [Google Scholar] [CrossRef] [Green Version]







| Compositions | C | Si | Mn | Cr | Mo | P | S | Fe |
|---|---|---|---|---|---|---|---|---|
| Content | 0.2 | 0.25 | 0.76 | 1.04 | 0.24 | 0.014 | 0.017 | Bal. |
| Mechanical Properties | Tensile Strength | Yield Strength | Elongation | Section Shrinkage |
|---|---|---|---|---|
| Value | ≥885 MPa | ≥685 MPa | ≥12% | ≥50% |
| Process No. | Austenitization Process | Vacuum Carburizing Process | Other Parameters | ||
|---|---|---|---|---|---|
| Carburizing Temperature/°C | Carburizing Time/min | Diffusion Time/min | |||
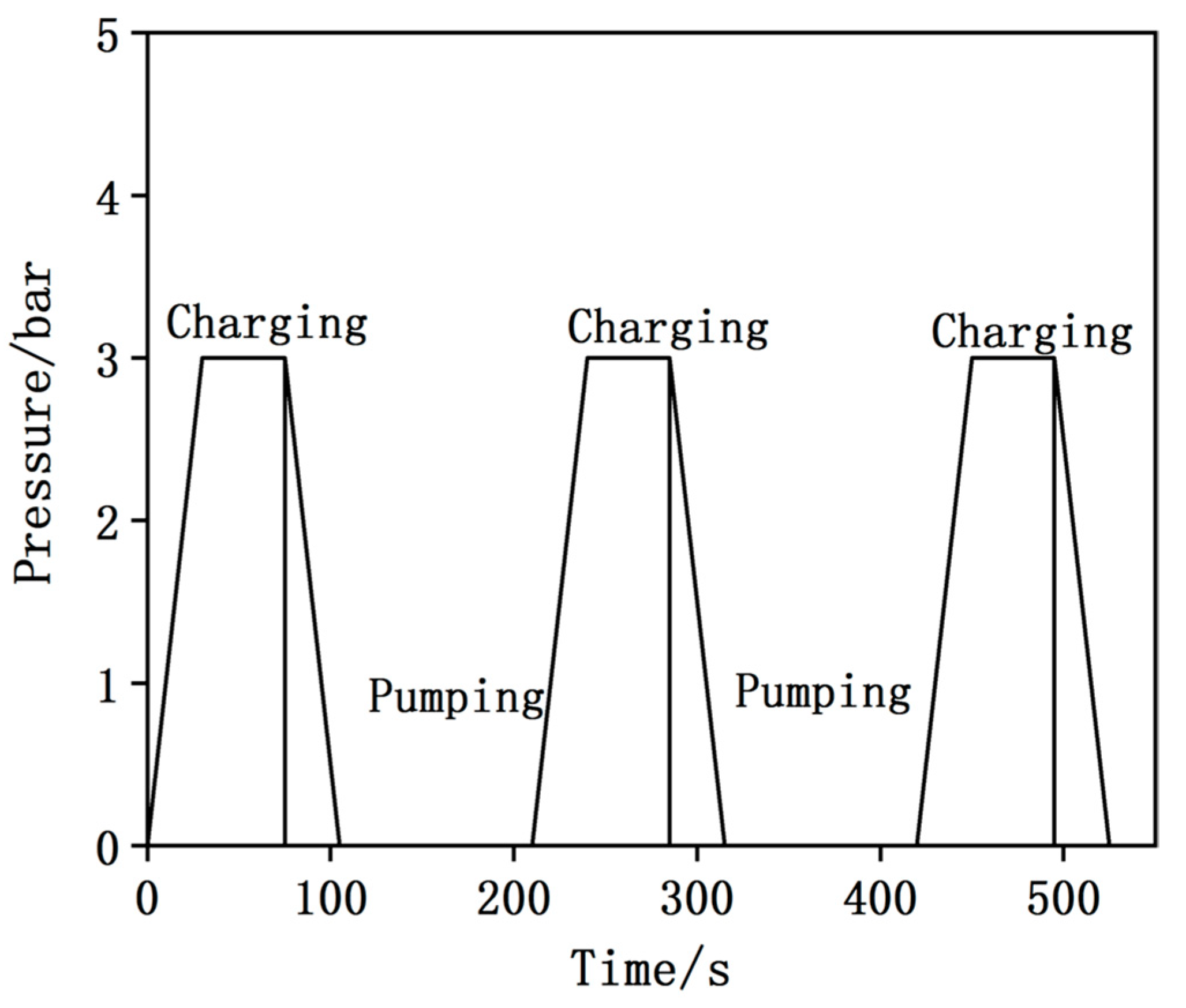
| 1 | Preheating: 650 °C × 30 min. After reaching carburizing temperature 930 °C × 30 min. | 930 | 42 | 0 | Carburizing pressure: 3 kPa. C2H2 and H2 flow rate: 8 L/min. Gas quenching pressure: 6 bar. |
| 2 | 930 | 42 | 140 | ||
| Number | Carburizing Time/min | Diffusion Time/min | Surface Carbon Concentration/% | Carburized Layer Depth/mm |
|---|---|---|---|---|
| L1 | 42 | 105 | 0.80 | 0.903 |
| L2 | 42 | 125 | 0.75 | 0.924 |
| L3 | 42 | 145 | 0.66 | 0.930 |
| L4 | 42 | 165 | 0.63 | 0.951 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, J.; Deng, X.; Wang, H.; Zhou, L.; Xu, Y.; Ju, D. Modeling and Simulation of Vacuum Low Pressure Carburizing Process in Gear Steel. Coatings 2021, 11, 1003. https://doi.org/10.3390/coatings11081003
Guo J, Deng X, Wang H, Zhou L, Xu Y, Ju D. Modeling and Simulation of Vacuum Low Pressure Carburizing Process in Gear Steel. Coatings. 2021; 11(8):1003. https://doi.org/10.3390/coatings11081003
Chicago/Turabian StyleGuo, Jingyu, Xiaohu Deng, Huizhen Wang, Leyu Zhou, Yueming Xu, and Dongying Ju. 2021. "Modeling and Simulation of Vacuum Low Pressure Carburizing Process in Gear Steel" Coatings 11, no. 8: 1003. https://doi.org/10.3390/coatings11081003
APA StyleGuo, J., Deng, X., Wang, H., Zhou, L., Xu, Y., & Ju, D. (2021). Modeling and Simulation of Vacuum Low Pressure Carburizing Process in Gear Steel. Coatings, 11(8), 1003. https://doi.org/10.3390/coatings11081003







