Steady Magnetohydrodynamic Micropolar Fluid Flow and Heat and Mass Transfer in Permeable Channel with Thermal Radiation
Abstract
:1. Introduction
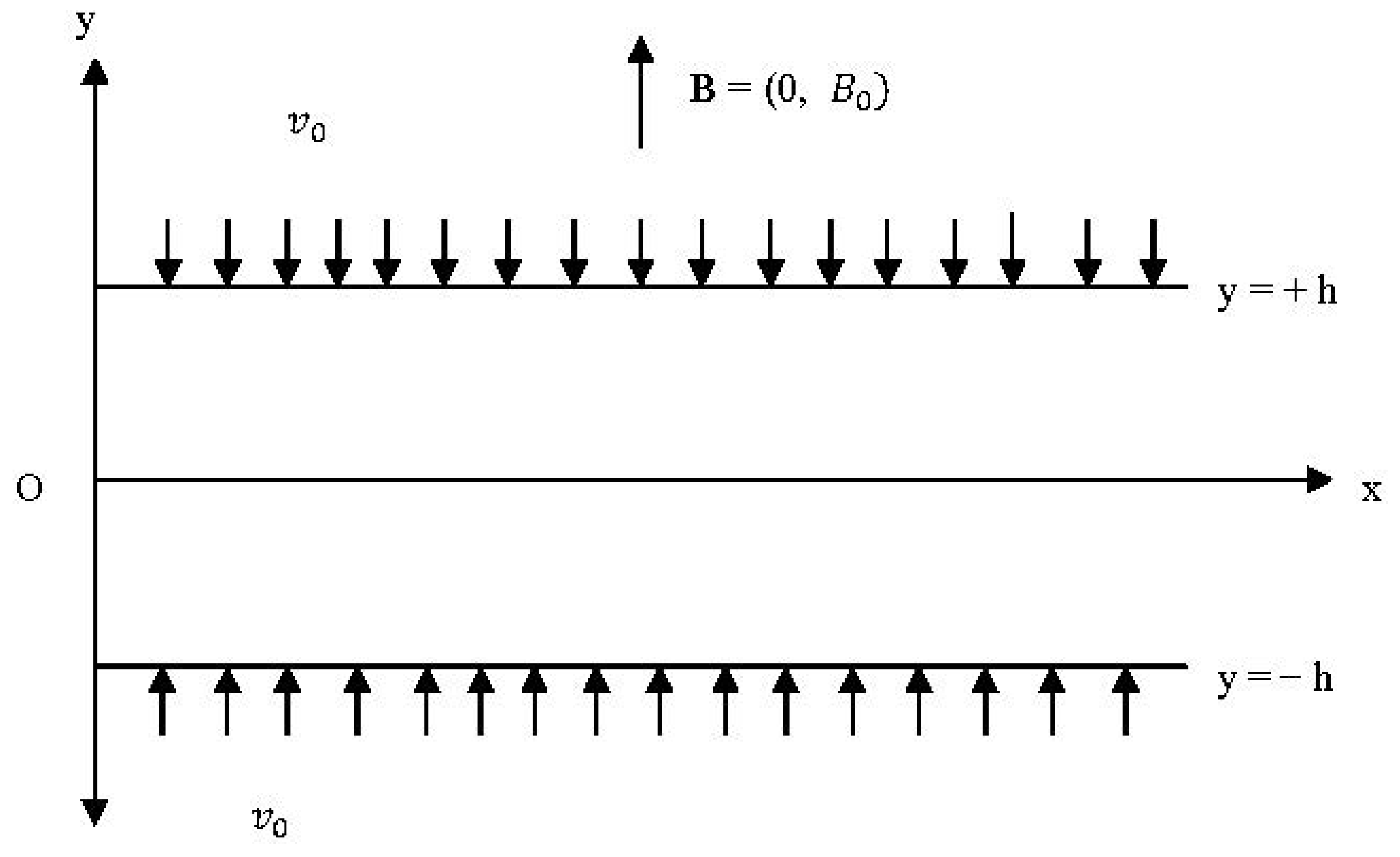
2. Formulation of the Problem
3. Analysis of the Homotopy Perturbation Method (HPM)
4. Solution of the Problem by HPM
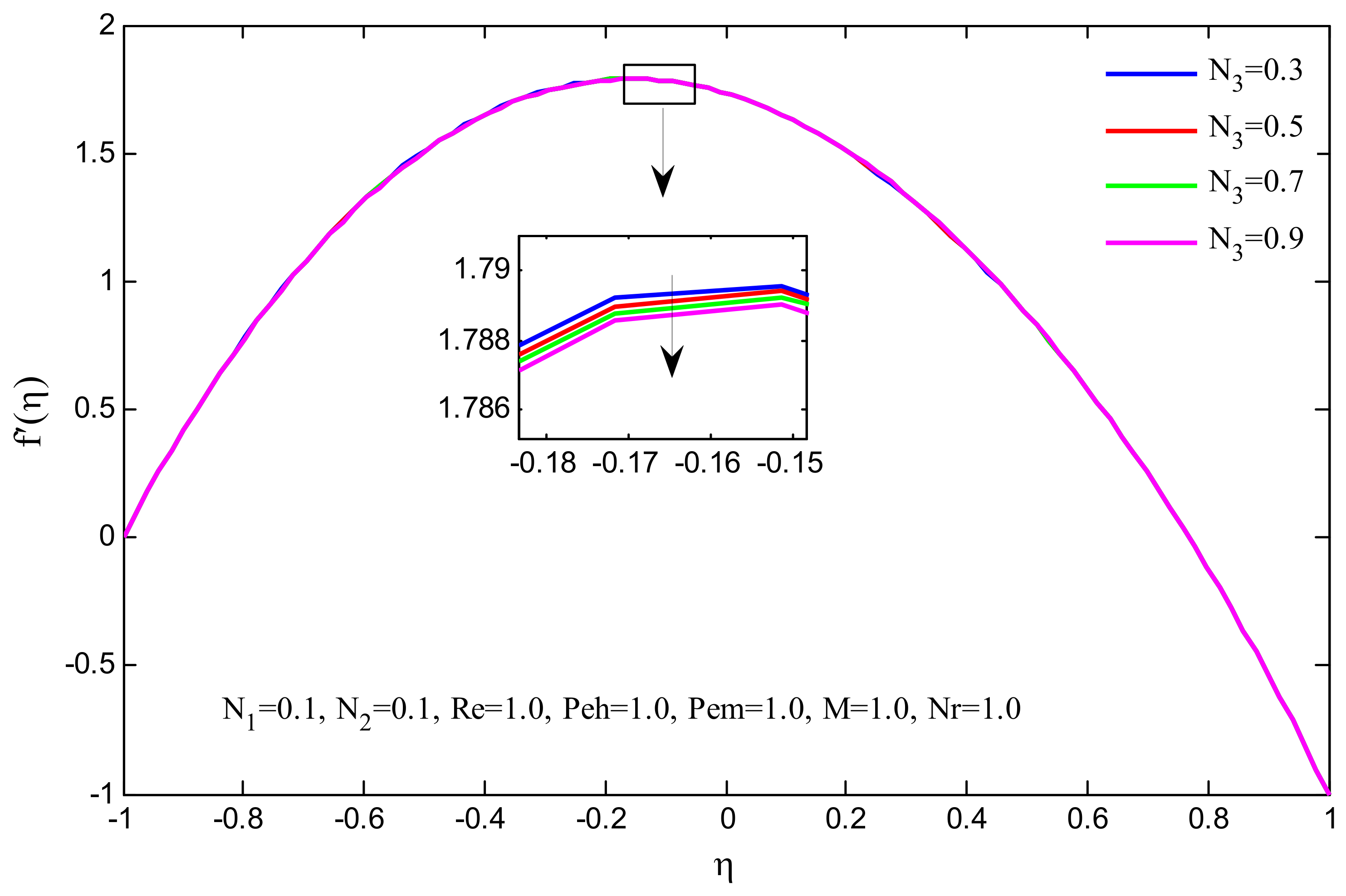
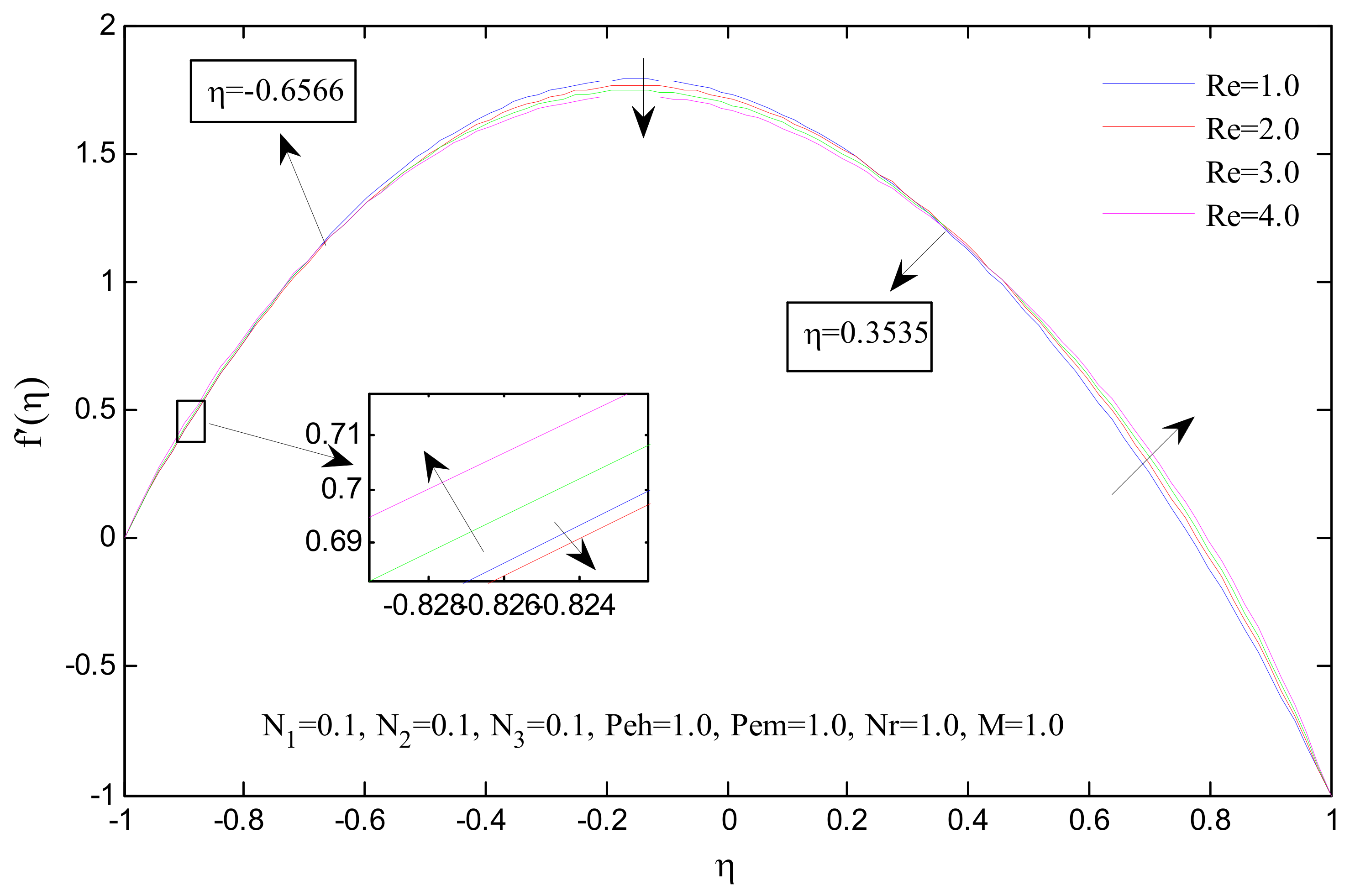
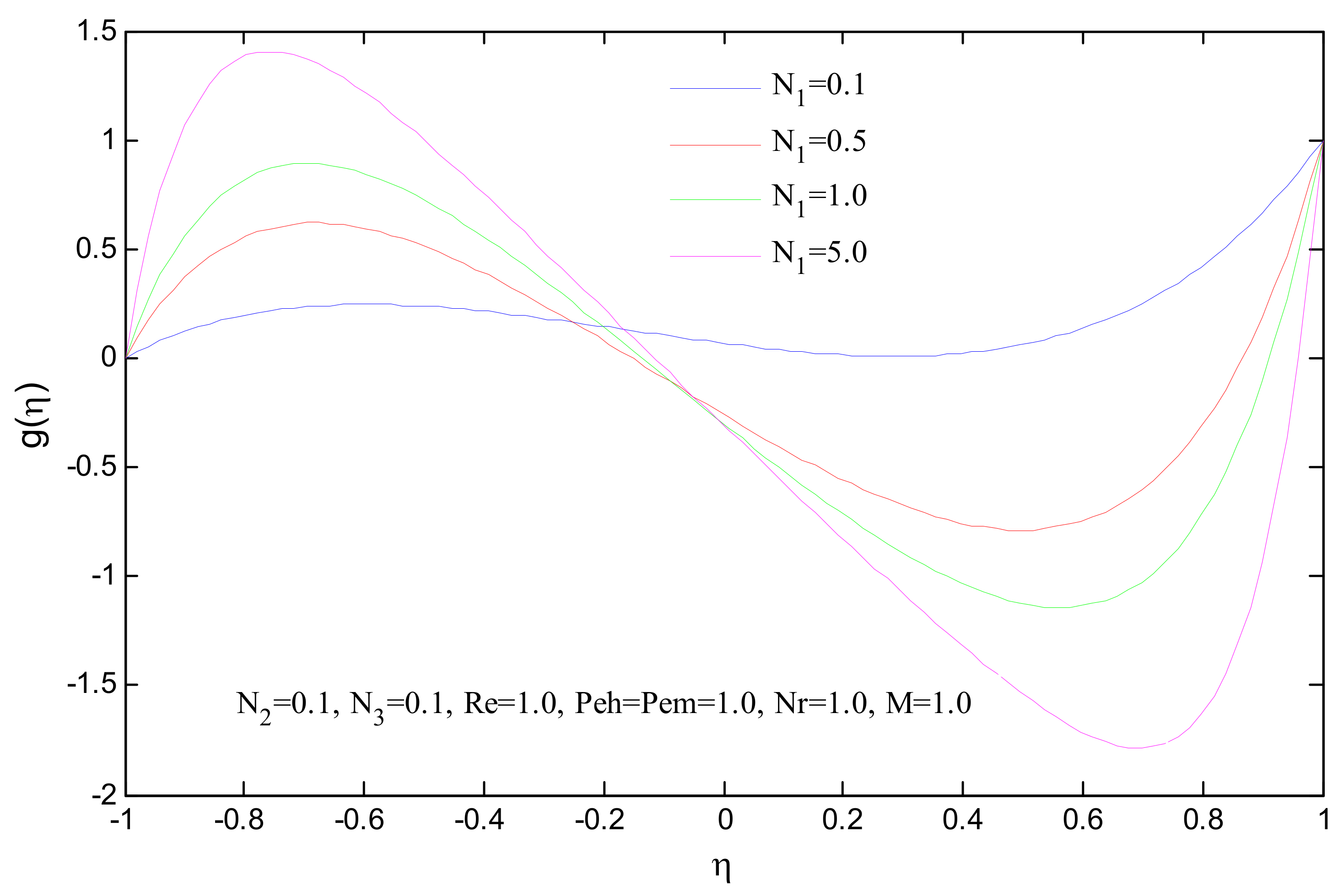
5. Results and Discussions
6. Conclusions
7. Comparison of This Work with Other Work
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| C | species concentration |
| D* | molecular diffusivity |
| thermal conductivity | |
| strength of constant applied magnetic field | |
| Ꞙ | dimensionless stream function |
| g | dimensionless microrotation |
| h | half width of channel |
| j | micro-inertia density |
| M | magnetic parameter |
| N | microrotation/angular velocity |
| N1, 2, 3 | dimensionless parameters |
| Local Nusselt number | |
| Local Sherwood number | |
| Sc | Schmidt number |
| P | pressure |
| Pr | Prandtl number |
| Peclet number for diffusion of heat | |
| Peclet number for diffusion of mass | |
| radiative heat flux | |
| Re | Reynolds number |
| T | fluid temperature |
| Nr | radiation parameter |
| s | microrotation boundary condition |
| (u, v) | Cartesian velocity components |
| (x, y) | Cartesian coordinate components parallel & normal to channel axis, respectively |
| HPM | homotopy perturbation method |
Greek Symbols
| η | similarity variable |
| μ | dynamic viscosity |
| ρ | Fluid density |
| stream function | |
| electric conductivity | |
| θ | dimensionless temperature |
| dimensionless mass transfer parameter | |
| κ | coupling coefficient |
| microrotation/spin-gradient viscosity |
References
- Abo-Eldahab, E.M.; Ghonaim, A.F. Radiation effect on heat transfer of a micropolar fluid through a porous medium. Appl. Math. Comput. 2005, 169, 500–510. [Google Scholar] [CrossRef]
- Perdikis, C.; Raptis, A. Heat transfer of a micropolar fluid by the presence of radiation. Heat Mass Transf. 1996, 31, 381–382. [Google Scholar] [CrossRef]
- Raptis, A. Flow of a micropolar fluid past a continuously moving plate by the presence of radiation. Int. J. Heat Mass Transf. 1998, 41, 2865–2866. [Google Scholar] [CrossRef]
- Seddeek, M.; Abdelmeguid, M. Effects of radiation and thermal diffusivity on heat transfer over a stretching surface with variable heat flux. Phys. Lett. A 2006, 348, 172–179. [Google Scholar] [CrossRef]
- El-Arabawy, H.A. Effect of suction/injection on the flow of a micropolar fluid past a continuously moving plate in the presence of radiation. Int. J. Heat Mass Transf. 2003, 46, 1471–1477. [Google Scholar] [CrossRef]
- Sharma, R.; Gupta, U. Thermal convection in micropolar fluids in porous medium. Int. J. Eng. Sci. 1995, 33, 1887–1892. [Google Scholar] [CrossRef]
- Ramachandran, P.; Mathur, M.; Ojha, S. Heat transfer in boundary layer flow of a micropolar fluid past a curved surface with suction and injection. Int. J. Eng. Sci. 1979, 17, 625–639. [Google Scholar] [CrossRef]
- Takhar, H.; Bhargava, R.; Agrawal, R.; Balaji, A. Finite element solution of micropolar fluid flow and heat transfer between two porous discs. Int. J. Eng. Sci. 2000, 38, 1907–1922. [Google Scholar] [CrossRef]
- Kelson, N.; Farrell, T. Micropolar flow over a porous stretching sheet with strong suction or injection. Int. Commun. Heat Mass Transf. 2001, 28, 479–488. [Google Scholar] [CrossRef]
- Ashraf, M.; Kamal, M.A.; Syed, K. Numerical study of asymmetric laminar flow of micropolar fluids in a porous channel. Comput. Fluids 2009, 38, 1895–1902. [Google Scholar] [CrossRef]
- Majdalani, J.; Zhou, C.; Dawson, C.A. Two-dimensional viscous flow between slowly expanding or contracting walls with weak permeability. J. Biomech. 2002, 35, 1399–1403. [Google Scholar] [CrossRef]
- Terrill, R.M.; Shrestha, G.M. Laminar flow through parallel and uniformly porous walls of different permeability. Z. Angew. Math. Phys. 1965, 16, 470–482. [Google Scholar] [CrossRef]
- Asghar, S.; Mushtaq, M.; Hayat, T. Flow in a slowly deforming channel with weak permeability: An analytical approach. Nonlinear Anal. Real World Appl. 2010, 11, 555–561. [Google Scholar] [CrossRef]
- He, J.-H. Homotopy perturbation method for solving boundary value problems. Phys. Lett. A 2006, 350, 87–88. [Google Scholar] [CrossRef]
- He, J.-H. Homotopy perturbation method for bifurcation of nonlinear problems. Int. J. Nonlinear Sci. Numer. Simul. 2005, 6, 207–208. [Google Scholar] [CrossRef]
- Ganji, D.D. The application of he’s homotopy perturbation method to nonlinear equations arising in heat transfer. Phys. Lett. A 2006, 355, 337–341. [Google Scholar] [CrossRef]
- Biazar, J.; Estami, M.; Ghazvini, H. Homotopy Perturbation Method for Systems of Partial Differential Equations. Int. J. Nonlinear Sci. Numer. Simul. 2007, 8, 411–416. [Google Scholar] [CrossRef]
- Fereidoon, A.; Ganji, D.D.; Rostamiyan, Y. Application of he’s homotopy perturbation and variational iteration methods to solving propagation of thermal stresses equation in an infinite elastic slab. Adv. Theor. Appl. Mech. 2008, 1, 199–215. [Google Scholar]
- Hemeda, A.A. Homotopy perturbation method for solving systems of nonlinear coupled equations. Appl. Math. Sci. 2012, 6, 4787–4800. [Google Scholar]
- Aminikhah, H.; Hemmatnezhad, M. A novel effective approach for solving nonlinear heat transfer equations. Heat Transf.-Asian Res. 2012, 41, 459–467. [Google Scholar] [CrossRef]
- Soltania, D.; Khorshidib, M.A. Application of homotopy perturbation and reconstruction of variational iteration methods for harry dym equation and compared with exact solution. Int. J. Multidiscip. Curr. Res. 2013, 1, 166–169. [Google Scholar]
- Yıldırım, A.; Kelleci, A. Homotopy perturbation method for numerical solutions of coupled Burger’s equations with time-and space-fractional derivatives. Int. J. Numer. Methods Heat Fluid Flow 2010, 20, 897–909. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Ganji, D.D.G.-D. Heat transfer of Cu-water nanofluid flow between parallel plates. Powder Technol. 2013, 235, 873–879. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Rokni, H.B.; Damiri-Ganji, D. Nanofluid flow in a semi-porous channel in the presence of uniform magnetic field. Int. J. Eng. 2013, 26, 653–662. [Google Scholar] [CrossRef]
- Sheikholeslami, M.; Hatami, M.; Ganji, D.D.G.-D. Micropolar fluid flow and heat transfer in a permeable channel using analytical method. J. Mol. Liq. 2014, 194, 30–36. [Google Scholar] [CrossRef]
- Mirzaaghaian, A.; Ganji, D. Application of differential transformation method in micropolar fluid flow and heat transfer through permeable walls. Alex. Eng. J. 2016, 55, 2183–2191. [Google Scholar] [CrossRef] [Green Version]
- He, J.H. Homotopy perturbation technique. Comput. Methods Appl. Mech. Eng. 1999, 178, 257–262. [Google Scholar] [CrossRef]
- Sibanda, P.; Awad, F. Flow of a micropolar fluid in channel with heat and mass transfer. In Proceedings of the 2010 International Conference on Theoretical and Applied Mechanics, International Conference on Fluid Mechanics and Heat & Mass Transfer, Corfu Island, Greece, 22–24 July 2010; pp. 112–120. [Google Scholar]
- Mirgolbabaee, H.; Ledari, S.; Ganji, D. Semi-analytical investigation on micropolar fluid flow and heat transfer in a permeable channel using AGM. J. Assoc. Arab. Univ. Basic Appl. Sci. 2017, 24, 213–222. [Google Scholar] [CrossRef] [Green Version]
- Singh, B. Thermal convection of MHD micropolar fluid layer heated from below saturating a porous medium. IOSR J. Eng. (IOSR-JEN) 2018, 8, 45–52. [Google Scholar]
- Singh, B. Hall effect on MHD flow of visco-elastic micro-polar fluid layer heated from below saturating a porous medium. Int. J. Eng. Sci. Technol. 2017, 9, 48–66. [Google Scholar] [CrossRef] [Green Version]
- Singh, B. Thermal instability of a rotating MHD micropolar fluid layer heated from below saturating a porous medium. Int. J. Pure Appl. Math. 2018, 118, 1393–1405. [Google Scholar]
- Mohammed, H.I.; Talebizadehsardari, P.; Mahdi, J.M.; Arshad, A.; Sciacovelli, A.; Giddings, D. Improved melting of latent heat storage via porous medium and uniform Joule heat generation. J. Energy Storage 2020, 31, 101747. [Google Scholar] [CrossRef]
- Ghaneifar, M.; Arasteh, H.; Mashayekhi, R.; Rahbari, A.; Mahani, R.B.; Talebizadehsardari, P. Thermohydraulic analysis of hybrid nanofluid in a multilayered copper foam heat sink employing local thermal non-equilibrium condition: Optimization of layers thickness. Appl. Therm. Eng. 2020, 181, 115961. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Zadeh, S.M.H.; Mehryan, S.; Pop, I.; Wen, D. Analysis of melting behavior of PCMs in a cavity subject to a non-uniform magnetic field using a moving grid technique. Appl. Math. Model. 2020, 77, 1936–1953. [Google Scholar] [CrossRef]
- Ali, F.H.; Hamzah, H.K.; Hussein, A.K.; Jabbar, M.Y.; Talebizadehsardari, P. MHD mixed convection due to a rotating circular cylinder in a trapezoidal enclosure filled with a nanofluid saturated with a porous media. Int. J. Mech. Sci. 2020, 181, 105688. [Google Scholar] [CrossRef]
- Ghalambaz, M.; Groşan, T.; Pop, I. Mixed convection boundary layer flow and heat transfer over a vertical plate embedded in a porous medium filled with a suspension of nano-encapsulated phase change materials. J. Mol. Liq. 2019, 293, 34. [Google Scholar] [CrossRef]



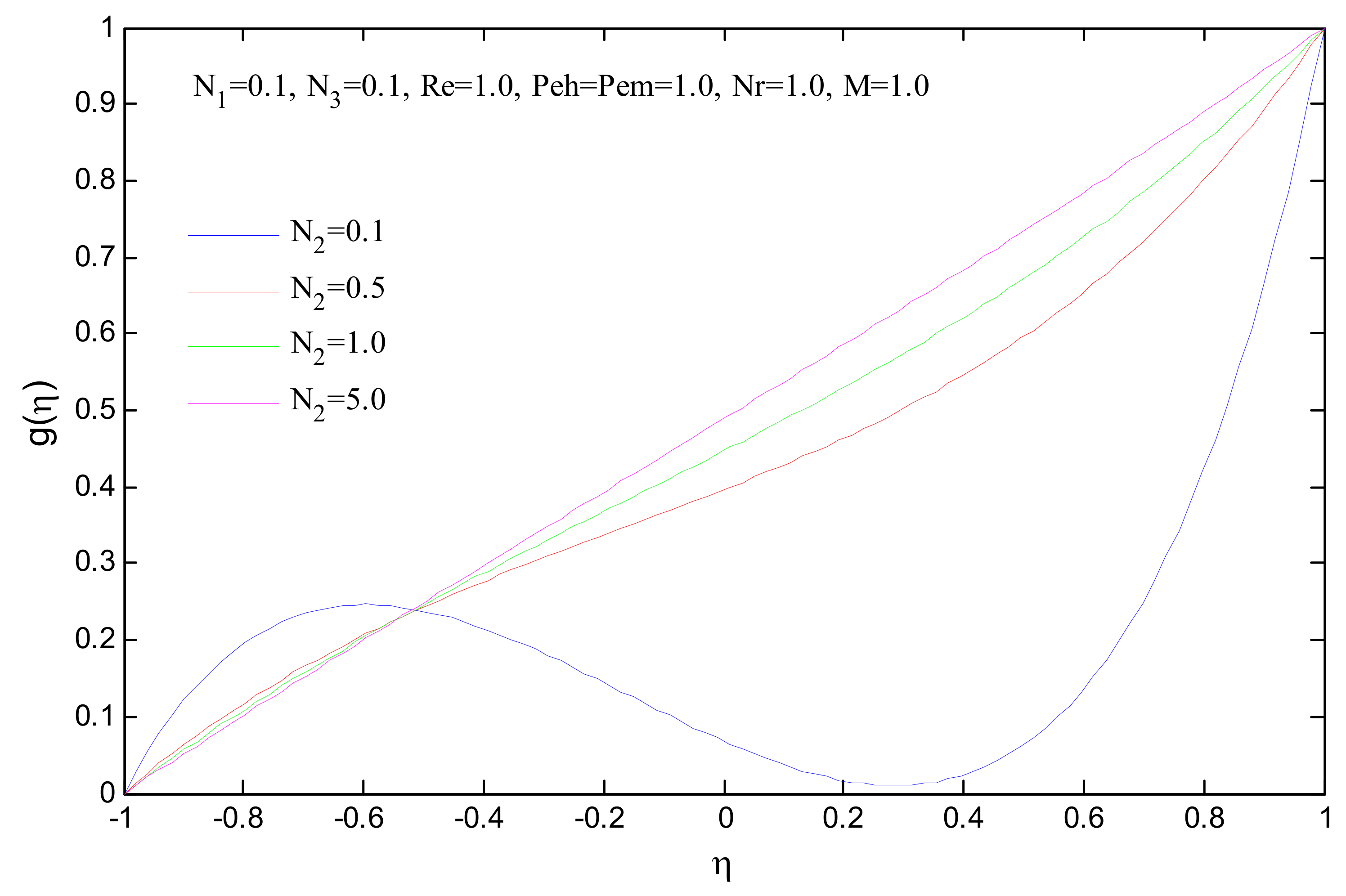



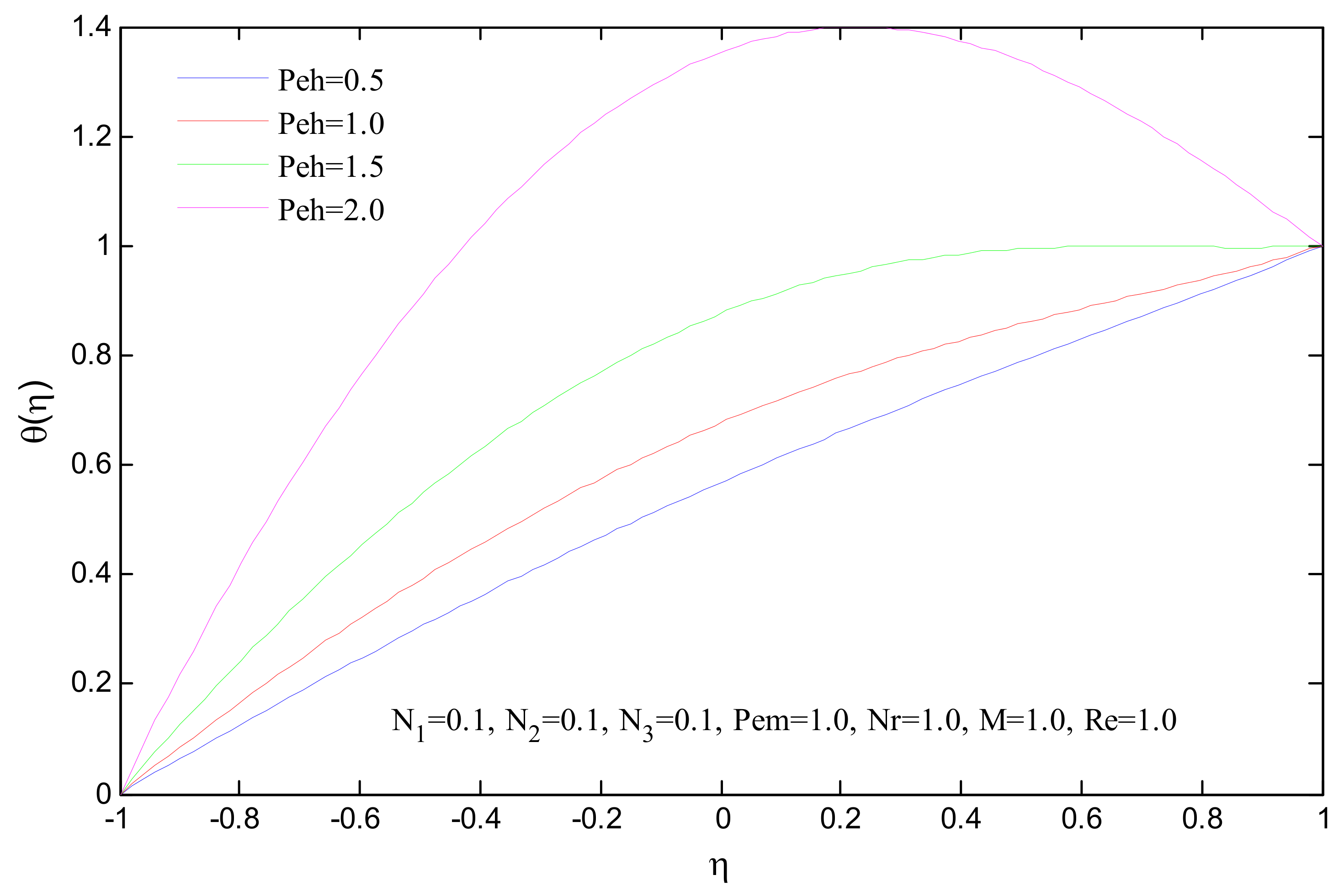
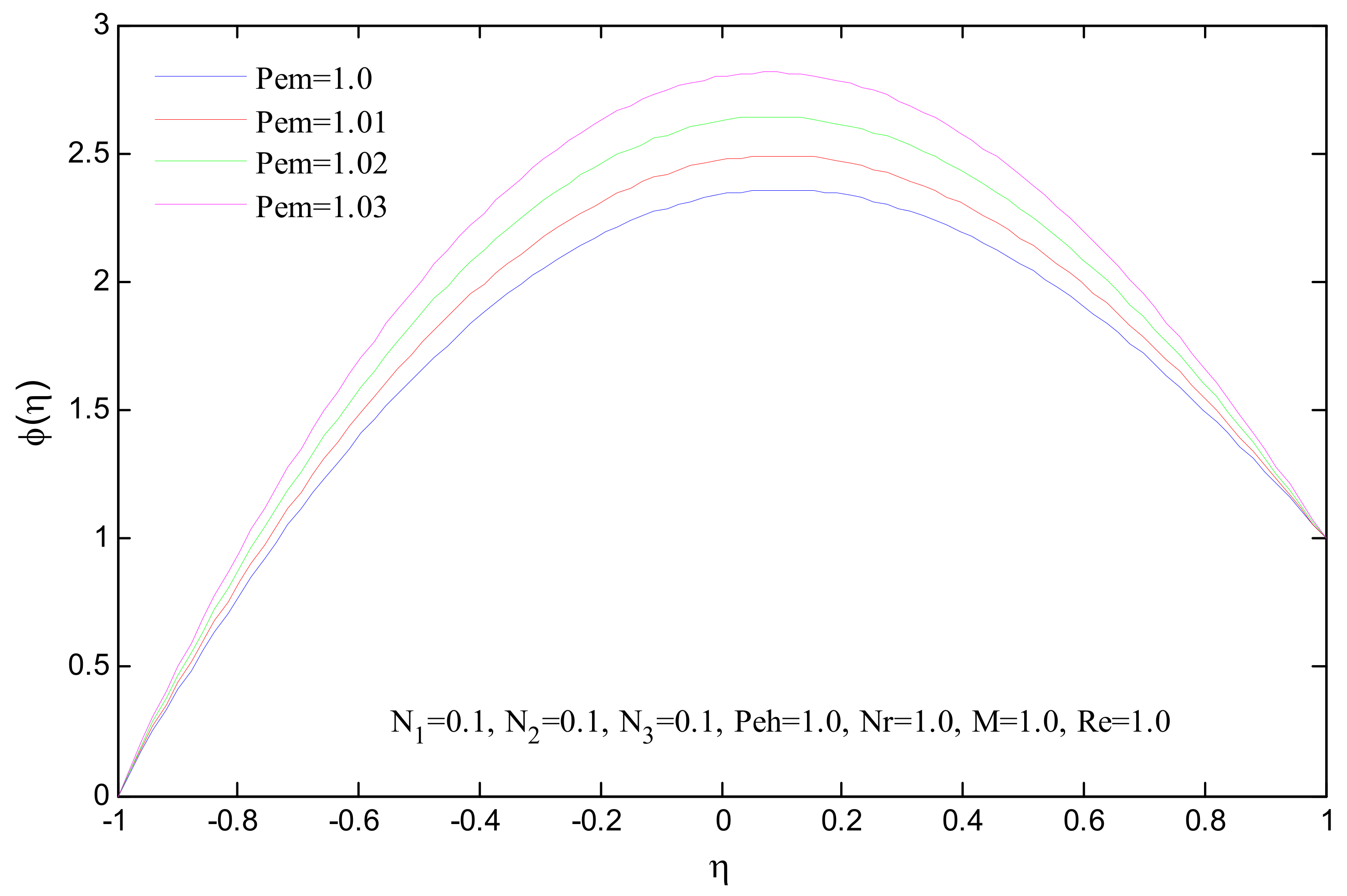
| N1 | N2 | N3 | Re | M | Nr | −θ′(−1) | ||
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5535 |
| 1.0 | 1.0 | 1.0 | 1.0 | 3.0 | 0.1 | 0.1 | 0.1 | 0.5536 |
| 1.0 | 1.0 | 1.0 | 1.0 | 5.0 | 0.1 | 0.1 | 0.1 | 0.5537 |
| 1.0 | 1.0 | 1.0 | 1.0 | 10.0 | 0.1 | 0.1 | 0.1 | 0.5537 |
| 1.0 | 1.0 | 1.0 | 1.0 | 15.0 | 0.1 | 0.1 | 0.1 | 0.5536 |
| 1.0 | 1.0 | 1.0 | 1.0 | 20.0 | 0.1 | 0.1 | 0.1 | 0.5535 |
| 1.0 | 1.0 | 1.0 | 3.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5552 |
| 1.0 | 1.0 | 1.0 | 5.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5538 |
| 1.0 | 1.0 | 1.0 | 10.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5532 |
| 1.0 | 1.0 | 1.5 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5532 |
| 1.0 | 1.0 | 1.6 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5530 |
| 1.0 | 1.0 | 2.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5520 |
| 1.0 | 1.5 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5533 |
| 1.0 | 1.6 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5532 |
| 1.0 | 2.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5530 |
| 1.5 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5537 |
| 1.6 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5538 |
| 2.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5539 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.2 | 0.1 | 0.1 | 0.6167 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.3 | 0.1 | 0.1 | 0.6922 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.4 | 0.1 | 0.1 | 0.7842 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.2 | 0.5475 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.3 | 0.5427 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.4 | 0.5387 |
| N1 | N2 | N3 | Re | M | Nr | −φ′(−1) | ||
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.1 | 0.1 | 0.5613 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.2 | 0.1 | 0.6355 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.3 | 0.1 | 0.7268 |
| 1.0 | 1.0 | 1.0 | 1.0 | 1.0 | 0.1 | 0.4 | 0.1 | 0.8420 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Agarwal, V.; Singh, B.; Kumari, A.; Jamshed, W.; Nisar, K.S.; Almaliki, A.H.; Zahran, H.Y. Steady Magnetohydrodynamic Micropolar Fluid Flow and Heat and Mass Transfer in Permeable Channel with Thermal Radiation. Coatings 2022, 12, 11. https://doi.org/10.3390/coatings12010011
Agarwal V, Singh B, Kumari A, Jamshed W, Nisar KS, Almaliki AH, Zahran HY. Steady Magnetohydrodynamic Micropolar Fluid Flow and Heat and Mass Transfer in Permeable Channel with Thermal Radiation. Coatings. 2022; 12(1):11. https://doi.org/10.3390/coatings12010011
Chicago/Turabian StyleAgarwal, Vandana, Bhupander Singh, Amrita Kumari, Wasim Jamshed, Kottakkaran Sooppy Nisar, Abdulrazak H. Almaliki, and H. Y. Zahran. 2022. "Steady Magnetohydrodynamic Micropolar Fluid Flow and Heat and Mass Transfer in Permeable Channel with Thermal Radiation" Coatings 12, no. 1: 11. https://doi.org/10.3390/coatings12010011
APA StyleAgarwal, V., Singh, B., Kumari, A., Jamshed, W., Nisar, K. S., Almaliki, A. H., & Zahran, H. Y. (2022). Steady Magnetohydrodynamic Micropolar Fluid Flow and Heat and Mass Transfer in Permeable Channel with Thermal Radiation. Coatings, 12(1), 11. https://doi.org/10.3390/coatings12010011








