3.1. Stabilization of Goethite Particles
As a small particle size is required to achieve transparency in particle-based coatings, a sufficiently high stability of the dispersions against agglomeration is a fundamental necessity. Accordingly, the colloidal fragments resulting from the milling process must be stabilized for appropriate processing. For goethite particles, a common type of electrostatic stabilization was applied, which is characteristic for oxide materials and caused by a specific adsorption or desorption of protons on the amphoteric hydroxylic groups present on the surfaces in an aqueous environment [
24,
25,
26]. Depending on the pH, repulsive electrostatic surface potentials can be induced, resulting in colloidal stability at a sufficient level [
27]. To estimate the magnitude of repulsive interactions between colloidal particles, the zeta potential provides a useful parameter that is comparatively easy to access and often used for a qualitative evaluation of the suspension’s stability [
28]. The measurements showed a positive potential for goethite in an acidic environment and a negative potential under basic conditions (
Figure 1a). The isoelectric point (IEP) was found to be at a pH* value of approximately 6.5. In general, the obtained zeta potential curve is in good agreement with data from the literature for purely aqueous suspensions [
18,
29,
30], indicating that ethanol content does not significantly affect the value of the measured potentials in dependence of the pH*. As observed in
Figure 1a, the highest absolute zeta potential is achieved under acidic conditions, assuming this range as favorable in terms of particle stability.
In addition, a more profound analysis was performed by calculating particle–particle pair interaction potentials (
Appendix A) at different pH* conditions for a uniform, characteristic particle size of 200 nm, which are shown in
Figure 1b. A potential barrier greater than 15 k
BT is solely present between particle surfaces at a low pH* value. The aforementioned value is generally considered to be the threshold for adequate suspension stability [
25,
31]. For higher pH* values of 6.6 and 10, attractive particle interaction dominates, presumably resulting in rapid agglomeration. In accordance with these results, a pH* value of 2.5 was set for processing goethite particles in the stirred media mill. However, especially for very small particle sizes and high solids contents, certain signs of suspension instability (e.g., gelling) were observed. This limitation could be diminished by lowering the temperature of the suspension. The fundamental connection between stability and a change of temperature is caused by particle–particle and particle–medium interactions. For electrostatic stabilization, in this respect, a shift of the charge density on the particle surfaces due to a displacement of the acid/base equilibrium between the particles and the surrounding medium, a change in dielectric properties of the medium and the temperature-dependent Brownian motion of the particles are considered to be decisive [
32]. As it is noticeable from
Figure 1a, comparable values for the zeta potential can be measured at a pH* value of 2.5 and a lower temperature of 10 °C. This suggests that the charge density at the particle’s interface appears to be mainly independent of temperature, which could also be confirmed from titration curves (
Figure S1 in Supplementary Materials). By contrast, the dielectric constant of the used water/ethanol mixture increases from 40.76 (25 °C) to 44.32 (10 °C) [
33], resulting in an overall higher potential barrier between the particles for the lower temperature (
Figure 1b). According to these results, grinding and coating were carried out at a suspension temperature of 10 °C.
3.2. Top-Down Formulation of Goethite
Even though they are often desired, fine particle sizes in the sub-100 nm range cannot be achieved by grinding for every solid material. The causes for this can be manifold, but the phenomenon is often explained by a physical grinding limit, by mechanical material properties changing with smaller particle sizes or by insufficient stabilization [
13,
19,
34]. Here, in order to provide a basic assessment of the grindability, typical relationships between particle size (
x50,3 and
x90,3) and the specific energy input whilst milling goethite particles are presented in
Figure 2.
With reference to the figure, it is evident that for an increasing specific energy input, a steady decrease in the measured particle’s size can be identified, resulting in a final
x50,3 value of 50 nm and an
x90,3 value of 85 nm. From a mechanical and stabilization-related point of view, this confirms that very fine particles of goethite material can be obtained by grinding with a stirred media mill. As expected, the drastic reduction in particle size is accompanied by a change in the optical properties of the suspensions (
Figure 3).
In fact, it can be clearly observed on the photographs of the sample suspensions (
wG = 0.5 × 10
−3) that optical appearance varies from completely opaque and turbid to clearly transparent for lower particle sizes. This effect can be essentially attributed to the fact that the smallest particle size of 50 nm, in particular, is well below the wavelength of the visible light, which significantly reduces light scattering by the particles; thus, a higher proportion of the light passes through the sample. The presence of particles of different sizes is further demonstrated by the respective SEM images in
Figure 3. Coarser particles (
x50,3 = 300 nm) exhibit a certain rod-like shape and still resemble the primary particles of the aggregated feed material (cf.
Figure S2 in Supplementary Materials). Therefore, it may be concluded that the first stage of grinding comprises a disaggregation, which is eventually transformed into a real grinding of the primary particles by further stressing, so that very small particle sizes (
x50,3 = 50 nm) can be achieved.
Particularly for the nanometer range, grinding processes with stirred media mills are usually energy-intensive, since, in addition to the mechanical stressing of the material, a high number of stressing events are required. Therefore, it is reasonable to consider not only the assurance of a certain product quality but also that its generation takes place under energetically optimized conditions. With regard to the specific energy
Em and a characteristic product property (e.g., the particle size), Kwade developed an expression that allows describing and optimizing a grinding process for a certain mill type, process unit and defined product formulation [
35]. In this context, the so-called stress energy of grinding media
SEGM can be used to link the effects of the major operating parameters grinding media size
dGM, grinding media density
ρGM and stirrer tip speed
vt with experimental data [
36,
37].
Typically, the relationship between
Em and
SEGM exhibits an optimum value (minimum of
Em) at which a certain product fineness is achieved under the most advantageous grinding conditions. As for a given stress energy, there is only one correlation between the achieved particle size and the specific energy; optimal operating parameters can be derived from the aforementioned minimum [
17].
For the performed grinding experiments,
Figure 4a shows the specific energy as function of the stress energy for two different target particle sizes. The complete grinding curves can be viewed in
Figures S3–S5 (Supplementary Materials). Taking the graphs into account, it is evident that within the range of investigated stress energies from 3 × 10
−6 to 1 × 10
−4 Nm, the required specific energy steadily decreases with lower stress energy. Consequently, smaller stress energies and in particular smaller grinding media sizes prove to be appropriate in terms of process optimization (cf. Equation (2)). However, typical minimum values of the specific energy cannot be identified from the curves and are presumably only reached at even lower stress energies. It should be noted that a further reduction in stress energy was not possible with the used mill, as the smallest permissible grinding media size (ranging from 200 to 300 µm) had already been applied and also lower tip speeds were not considered appropriate in terms of maintaining the characteristic bead movement in the grinding chamber.
For stress energies greater than their optimum value (
SEGM/
SEGM,opt > 1), according to Kwade, conclusions can be drawn regarding breakage characteristics and the comminution behavior of particle structures. To this end, the relationship between the specific energy and the stress energy is expressed by Equation (3), in which exponent α reflects the comminution behavior characteristically with respect to the material [
17,
38]. Thereby, α can be directly determined from the slope of the resulting straight line in a log–log scaling (
Figure S6 in Supplementary Materials).
The values of the exponent α can be found in
Table 1 for obtaining three different target particle sizes.
In general, an increasing grinding resistance of the material is accompanied by higher α, since the fineness of the fragments at each stress event becomes proportional to stress energy; thus, energy utilization remains constant (slope: 1 − α ≈ 0) [
38]. For deagglomeration, on the other hand, the result of a single stress event is independent of the stress energy as long as the stress energy is higher than its optimal value. Hence, a linear proportionality between specific energy and stress energy follows (slope: 1 − α ≈ 1) [
38]. Considering
Table 1, it can be essentially observed from the overall small α-values that goethite particles exhibit a comparatively low grinding resistance. Since the α-value represents a material constant, it can be further assumed from the increasing trend with decreasing target particle size that a change of particle structure occurs within the course of milling. As mentioned earlier, the deaggregation of the starting material is most likely dominant to reach a particle size of approximately 300 nm, which is reflected by the smaller value for α, as aggregates exhibit a lower resistance compared to primary particles of similar size. Accordingly, a certain increase in α seems plausible for achieving 200 or 100 nm, respectively, because single particles are primarily stressed. Within the investigated framework, this means that the impact of the stress energy on the required specific energy declines as the target particle size decreases [
38].
For the presumed optimum stress energy of 3 × 10
−6 Nm (
dGM = 243 µm,
vt = 6 m·s
−1), the influence of the solids content on the grinding efficiency was also investigated (
Figure 4b). This is shown by comparing the dependence between particle size and specific energy for a
wG of 0.05, 0.15 and 0.25.
Taking the figure into account, it quickly becomes evident that the specific energy requirement can be significantly reduced with higher solid content, which can be directly transferred to an increase in production capacity. This is due to the fact that individual stressing events are used more efficiently as a result of a higher stressing probability with respect to single and multiple particle stressing. Accordingly, the highest solids fraction of
wG = 0.25 proves to be an advantageous process adjustment, for which 30% or 70% of the required specific energy can be saved for achieving a size of 75 nm. It should be noted that a further increase in solid content can, in turn, result in a decrease in overall process efficiency, since higher viscosities of the product suspension may result in greater specific energy consumption [
13,
17]. However, the investigation of such an effect and, thus, an optimal solid content was not performed within this study.
With respect to the presented results, grinding at the energetic optimum does not automatically provide the highest production capacity. In order to maximize the output, the power draw of the mill must also be taken into account. As a general recommendation, power consumption should be as high as possible when utilizing the optimal grinding media size, which can be usually ensured by applying higher stirrer tip speeds and by the corresponding adjustment of the grinding media size to keep the stress energy constant [
17]. However, such an approach would be limited for the investigated material and used mill due to the requirement of smaller grinding media than the permissible size (see discussion regarding
Figure 4a). In this case, the only method to further increase production capacity would be to increase the stirrer tip speed, which can be followed by an increase in grinding media wear and may cause negative effects on the product’s quality. As an example, Breitung-Faes observed a decrease in transparency through the use of higher stirrer tip speeds when nanogrinding alumina suspensions, which was attributed to an increased degree of grinding media wear [
14]. Bearing this in mind, the following investigations into the production of transparent coatings were carried out with suspensions that were obtained at the energetic optimum (
SEGM = 3 × 10
−6 Nm), applying a grinding media size of 243 µm and a comparatively low tip speed of 6 m·s
−1.
3.3. Production of Transparent Glass Coatings
The coatings were generated via wet film deposition by dip coating. In such operations, the product’s quality is essentially controlled by both formulation properties of the suspension (particle size, viscosity
ηsusp, surface tension
σLV and density
ρsusp) and process properties (withdrawal speed
vw, wet film thickness
h0 and drying temperature
TDry). A widely used equation to describe a dip coating process is given by the approach of Laundau and Levich (Equtaion (4), [
39]), used particularly for low withdrawal speeds (≈1–10 mm·s
−1) and low viscosities, which is often the case for sol–gel film deposition [
9].
The equation presents a relationship between wet film thickness, h0, and the withdrawal speed, vw, as well as important formulation properties, thus providing a helpful tool in explaining factors influencing the film’s quality. In the following, some of these factors will be discussed in detail.
3.3.1. Solid Content and Withdrawal Speed
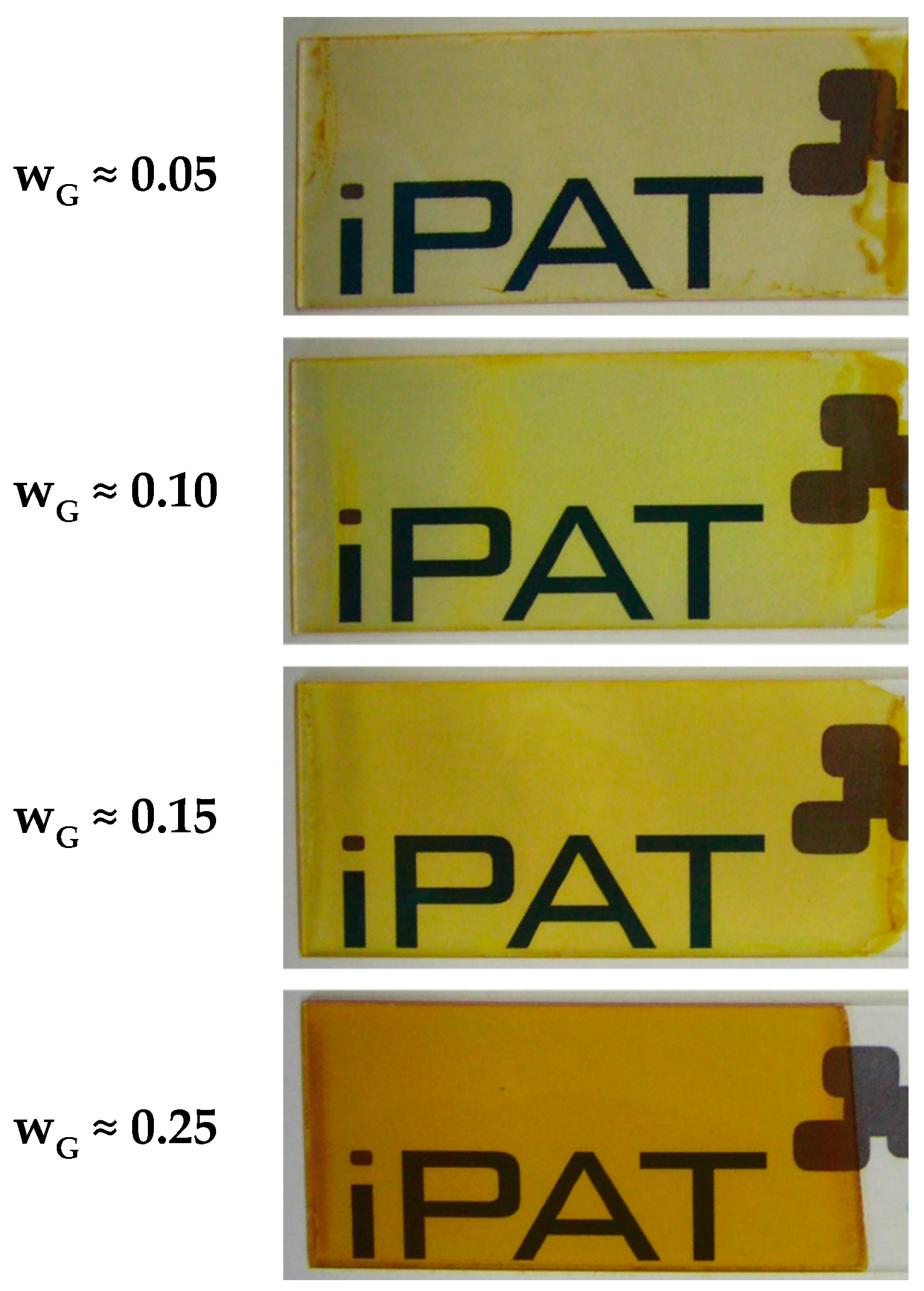
Figure 5 shows images of coatings obtained for a constant withdrawal speed of 50 mm·min
−1 using a suspension with average particle size of 50 nm at pH* of 2.5 and the addition of TEOS. Within this investigation, the solid’s content
wG of the coating suspensions was varied. Additional formulation parameters as well as the calculated wet film thickness
h0 can be viewed in
Table 2.
As expected from the observations in
Section 3.2, high transparency can be observed for all samples due to the small particle size. However, the color impression appears to differ with higher mass fraction from slightly yellowish to orange, presumably indicating increasing coating thickness. With respect to Equation (4) and
Table 2, this is plausible, since the viscosity of the suspension increases with increasing solid content, which tends to result in a thicker wet film
h0. In addition, as more goethite particles are applied onto the glass substrate, the thickness of the dried coating also increases. Furthermore, it can be observed that the coatings appear more homogeneous when using higher
wG, which also may be explained by viscosity effects. As a result of a high mobility of the fluid for low viscosities, clusters of several fluid layers are formed, especially at the edges of the glass substrate, before gelation takes place due to the onset of drying. It should be mentioned that the suspensions showed Newtonian viscosity behavior, which is the reason why no shear rate is provided for the viscosity values in
Table 2.
A more practical method to specifically adjust coating thickness is to vary the withdrawal speed
vw (cf. Equation (4)). Such an investigation was carried out for a coating suspension with a solid content of
wG = 0.25 and a particle size of 50 nm (
Figure 6). Regarding the optical appearance of coatings, the color impression changed with increasing withdrawal speeds from yellow to dark orange/brownish. As already mentioned in the discussion of
Figure 5, this again indicates an increase in coating thickness. Even though optical transparency is present in all samples, it is noticeable that film flaking occurred in certain areas on the substrate when applying higher withdrawal speeds (100 and 200 mm·min
−1). This represents a considerable reduction in product quality and may be attributed to excessive material deposition. Overall, it can be concluded from
Figure 5 and
Figure 6 that higher solids contents of the coating suspensions in combination with comparatively lower withdrawal speed prove to be advantageous for obtaining a homogenous and transparent coating.
The applied withdrawal speeds resulted in dry coating thicknesses
h between 0.5 and 2.5 µm (see
Table 3). In addition, wet film thicknesses,
h0, were calculated according to Equation (4). Taking all withdrawal speeds into account, the average, relative decrease from wet to dry film thickness yielded 73.2 ± 7.4% (
Table 3). The low deviation indicates that the coating deposition process can be adequately described by Equation (4).
3.3.2. Particle Size
As already demonstrated above, transparency can be significantly increased by reducing particle size in a suspension. In this section, this effect is examined on quality and transparency of the resulting coatings in more detail. In order to illustrate the influence,
Figure 7 shows images of coatings that were obtained by utilizing average particle sizes of approximately 300, 125 and 50 nm, respectively. Additionally, the corresponding high-resolution SEM images are presented. Suspensions with a solid content of
wG = 0.25 and a withdrawal speed of
vw = 10 mm·min
−1 were chosen according to previous analyses.
Clearly, the addressed effect can be observed, as the turbidity of the coatings is reduced significantly with decreasing particle size, resulting in a product with high optical transparency for the smallest size of 50 nm. Furthermore, the different particle sizes can be well identified in the SEM images, showing a more densely packed structure and higher homogeneity for the finer size class.
If a translucent coating that contains small particles is subjected to electromagnetic waves, an interaction takes place, causing reflection
R, transmission
T, absorption
A as well as scattering
S of these waves. In this regard, the decisive factor for the optical impression of transparency is that the intensity of the scattered light is small due to very low particle sizes [
5,
6]. This requires particles to be significantly smaller than the electromagnetic wavelength of the incident light. In order to demonstrate this phenomenon on the basis of the investigated films,
Figure 8 depicts the amount of light
S scattered by the coated glass substrate for wavelengths between 800 nm ≤
λ ≤ 1000 nm. This range was chosen deliberately since it lies outside the visible light region (380 nm ≤
λ ≤ 780 nm) and it is, therefore, assumed that an absorption of the pigment particles can be neglected. By measuring reflection and transmission, it is thereby possible to derive the amount of scattered light
S for these wavelengths according to Equation (5).
As expected, the lowest values for
S of about 3% are obtained for the smallest particle size of 50 nm (
Figure 8). Moreover, it can be observed that the amount of scattered light increases with decreasing wavelength, but only for coarser particle samples (e. g., 125 and 300 nm). To effectively reduce scattering effects, it is commonly proposed that a particle size should be at least 10-times smaller compared to the respective wavelength [
7,
8]. This is valid for the 50 nm particle size fraction, but not for median particle sizes of 125 and 300 nm. Therefore, if the wavelength-to-particle size ratio is lower for the two coarser particle fractions, scattering may occur and further increase when either smaller wavelengths or larger particles are encountered. Bearing this in mind, a higher scattering loss for lower wavelengths in
Figure 8 seems plausible, since coarser particles interact more with the incident light. In contrast, such an outcome cannot be observed for the particle size of 50 nm, as it can be assumed that these structures are sufficiently small even for the lowest
λ of 800 nm. However, this relationship is not quite as definite in a natural environment with wavelengths of the visible light (380 nm ≤
λ ≤ 780 nm). Especially for smaller wavelengths of around 400 nm, certain scattering effects cannot be completely excluded. Nevertheless, as shown in
Figure 7, very good results could already be achieved for a particle size of 50 nm in terms of coating transparency.
3.3.3. Drying Temperature
The influence of the drying temperature
TDry was investigated in order to induce a color change of the coatings. The yellow pigment goethite is known to be completely transformed into red hematite (α-Fe
2O
3) by a dehydration reaction in a temperature range of about 250–280 °C [
40,
41,
42,
43,
44]. This step was considered in more detail, since the control of the coating color by simple adjustment of the drying temperature provides an easily achievable and highly useful process modification.
The influence of the drying temperature for coatings obtained with a suspension of 50 nm in particle size is illustrated in
Figure 9. In this respect, a color change from yellow towards orange to red can be identified for the corresponding temperatures of 100, 200 and 300 °C that extends homogeneously over the entire coating (
Figure 9a). The visual impression of transparency remains unaffected, which confirms that it is generally possible to adjust the coating color via
TDry.
In order to support and further quantify these observations, the extinction spectra of the coated glass substrates are shown in
Figure 9b for the visible light range of 350 nm ≤
λ ≤ 780 nm. Referencing
Tdry = 100 °C, it can be observed from the curve that the highest extinction values are located at 380 nm ≤
λ ≤ 430 nm. According to this, the violet and blue light regions are mainly absorbed, resulting in the expected yellow color perception. If the film is dried at 200 °C, however, an additional absorption at slightly higher wavelengths within the green light range occurs that changes the color to orange. For this temperature, it can be assumed that a transformation of goethite into hematite has already begun but is incomplete. Consequently, considering an even higher drying temperature (
Tdry = 300 °C), both blue and green light ranges (380 nm ≤
λ ≤ 550 nm) are almost completely absorbed. This causes the coating to appear red, suggesting that the transformation to hematite has been accomplished. As a validation of the dehydration of goethite into hematite, XRD measurements of the feed material, previously exposed to the respective temperatures, were performed. These measurements can be found in
Supplementary Materials (Figure S7). Whilst thermal treatment of the goethite powder at 100 °C does not induce any change in phase composition and crystallinity of the material, after treatment at 300 °C, the goethite reflections vanished and the material has been transformed completely to hematite. Even though the conditions of dehydration are different within coatings, hence resulting in different kinetics, these results provide clear evidence of the formation of hematite leading to the observed color change. Therefore, using the drying temperature is an effective strategy to obtain transparent coatings of different colors when using goethite as the coating material.