Mechanical Characterization of Asphalt Mixtures Based on Polymeric Resin and Thixotropic Filler as a Substitute for Bitumen
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
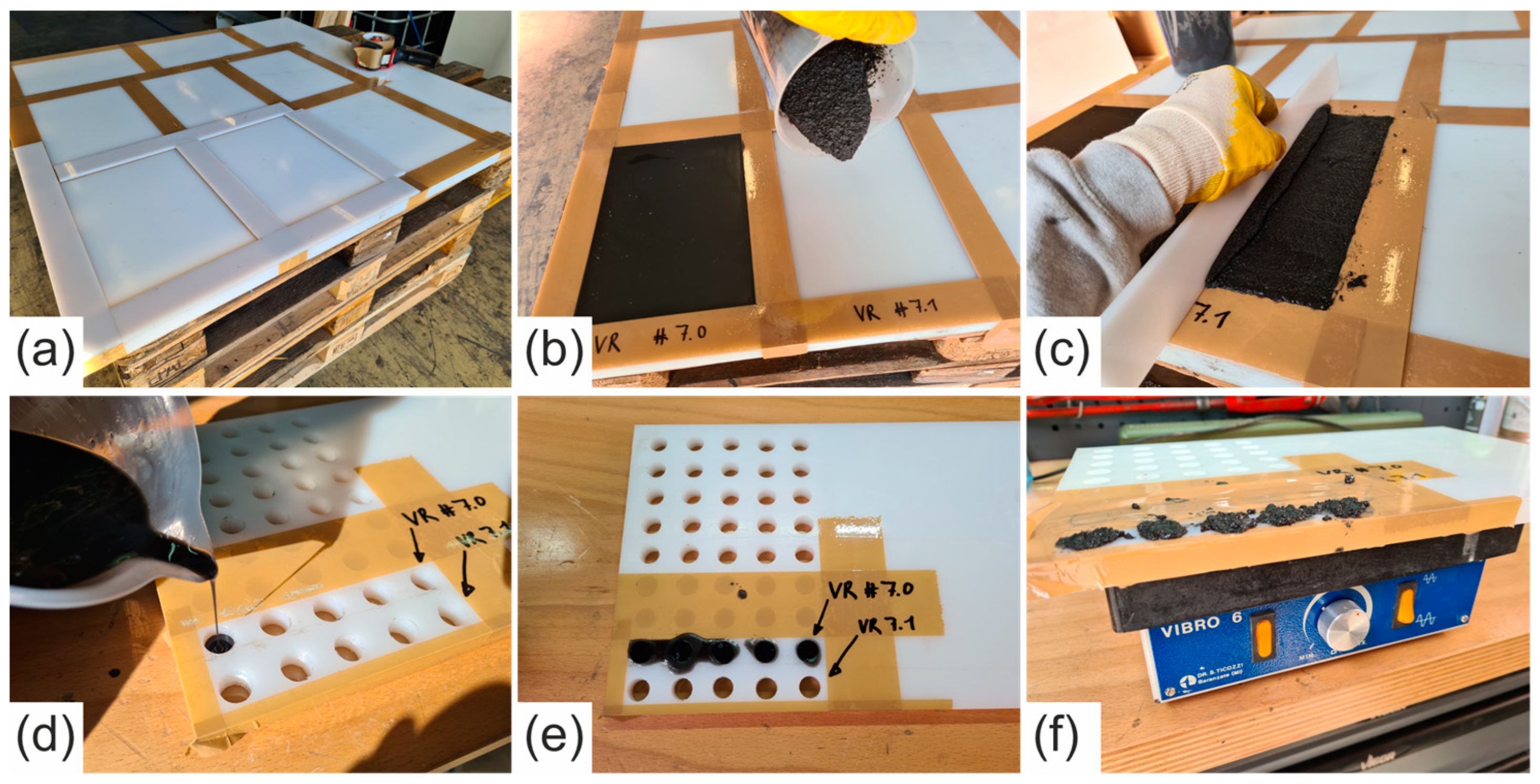
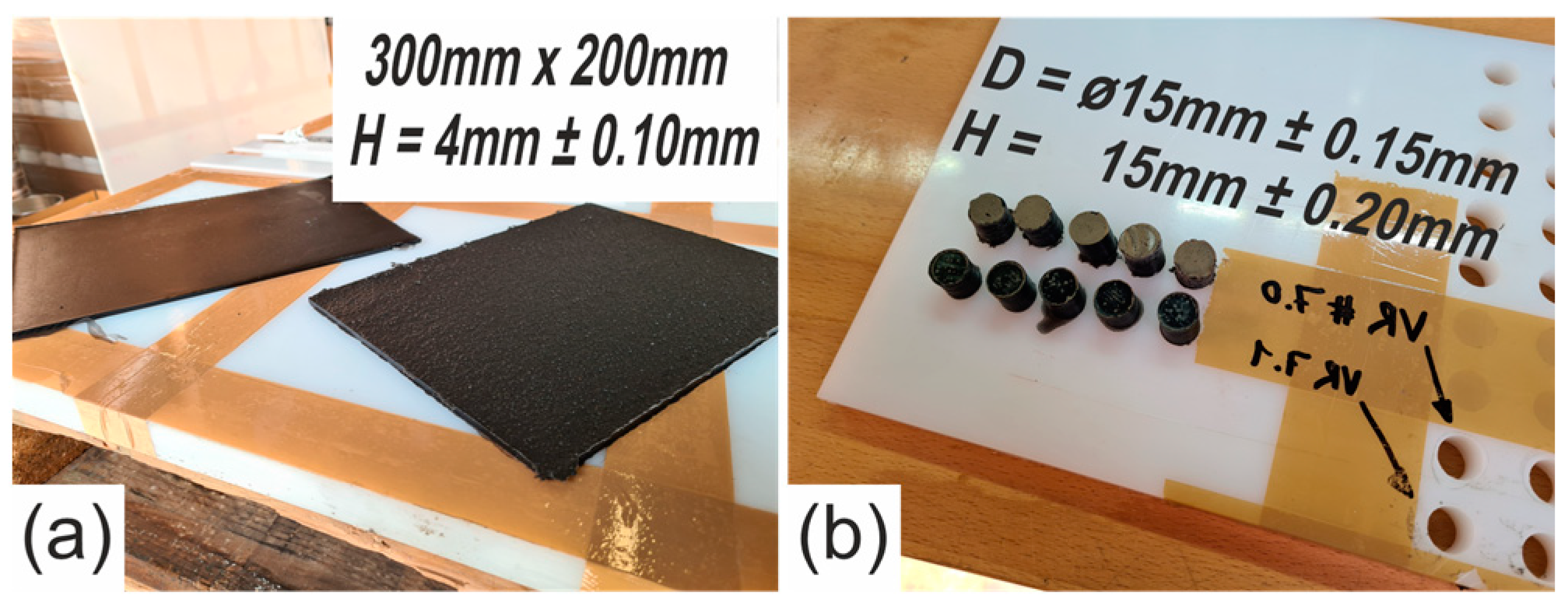
2.2. Specimen Production
2.3. Methods
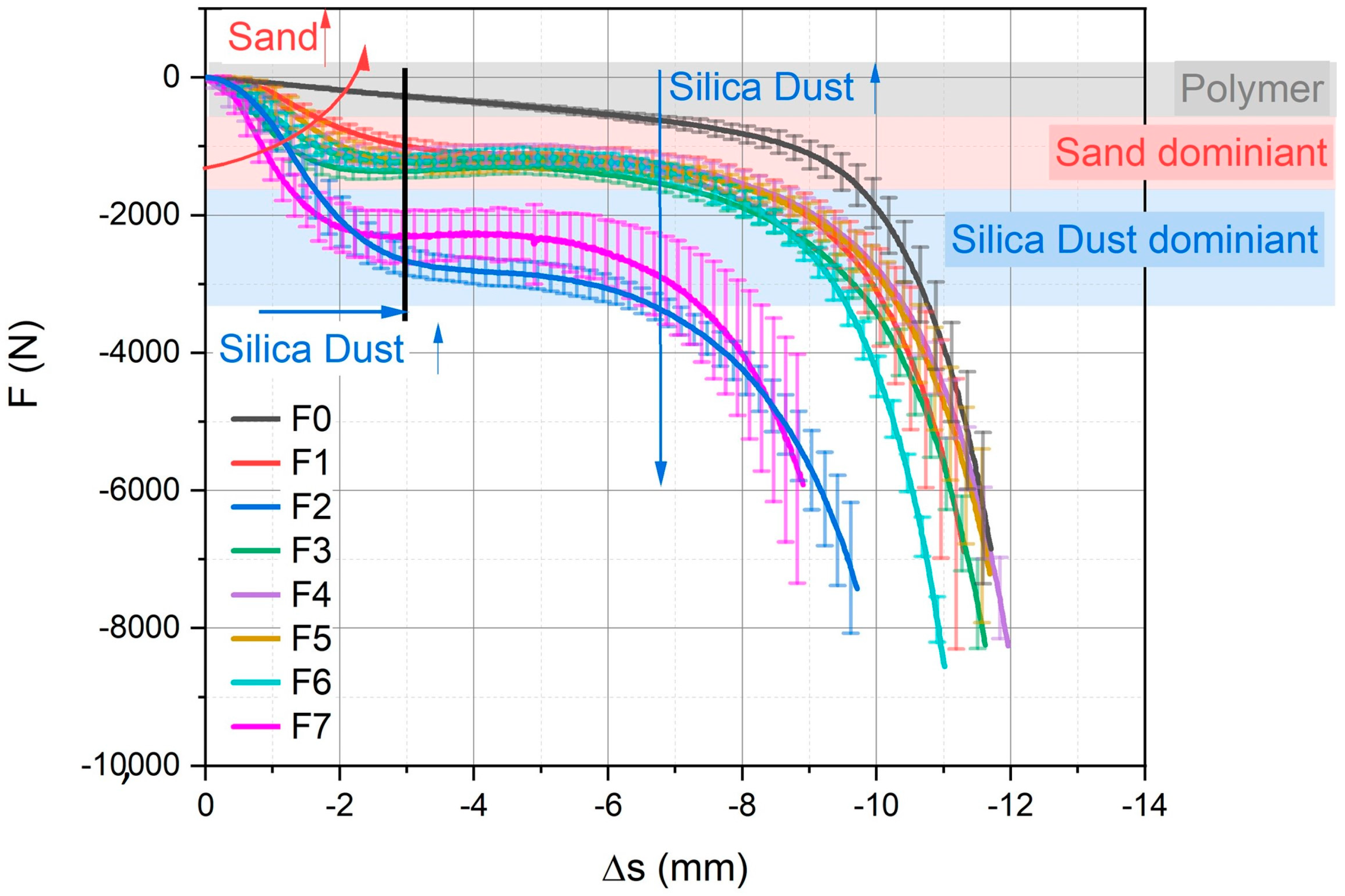
3. Results
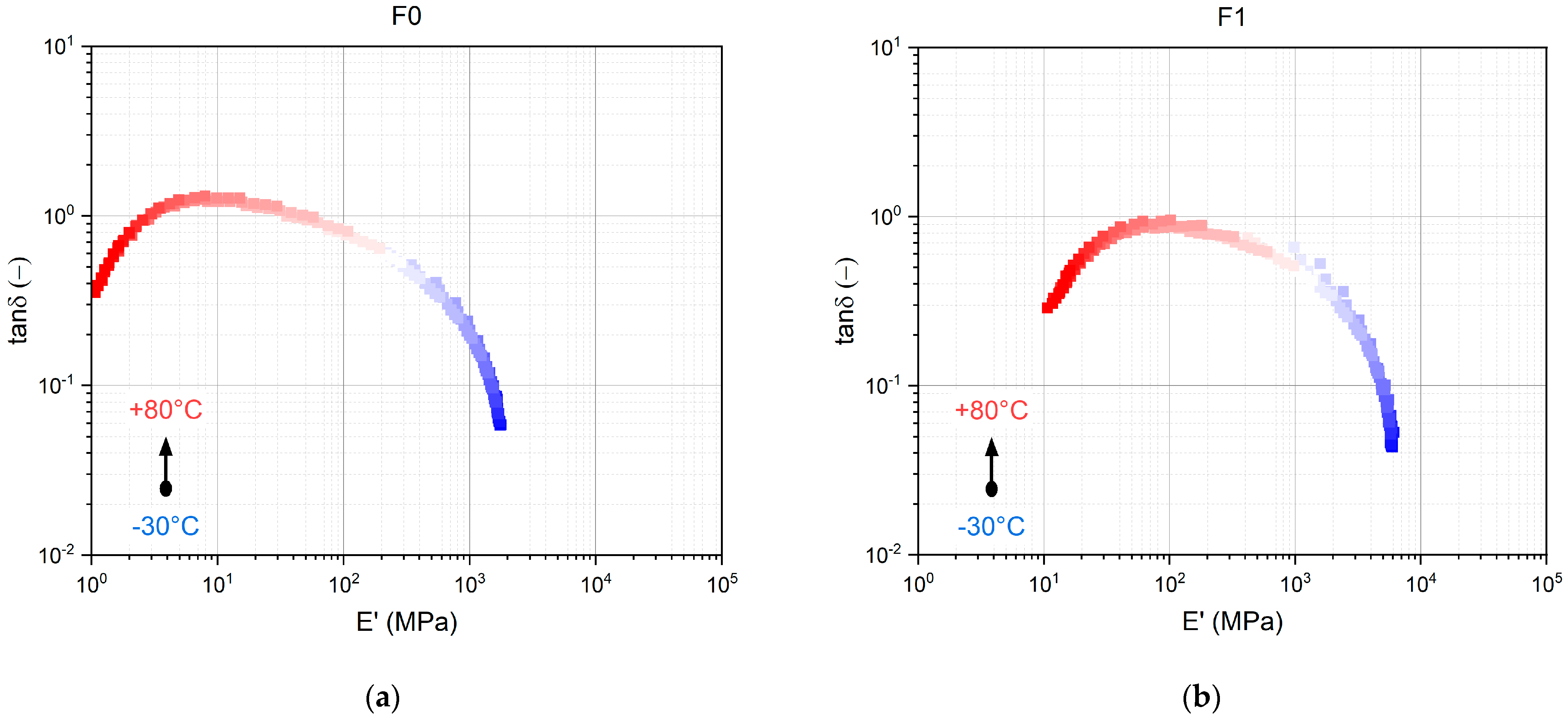
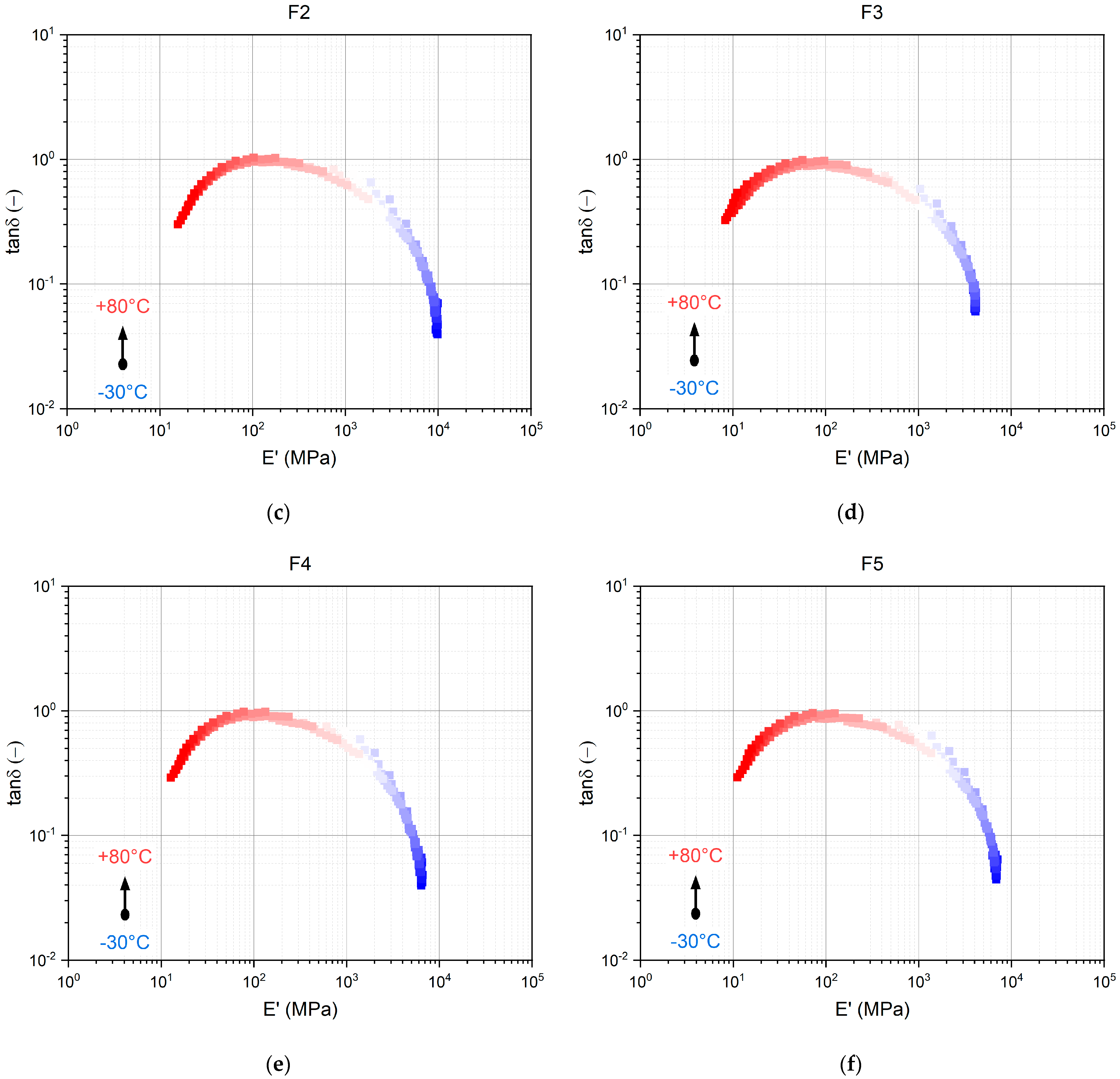
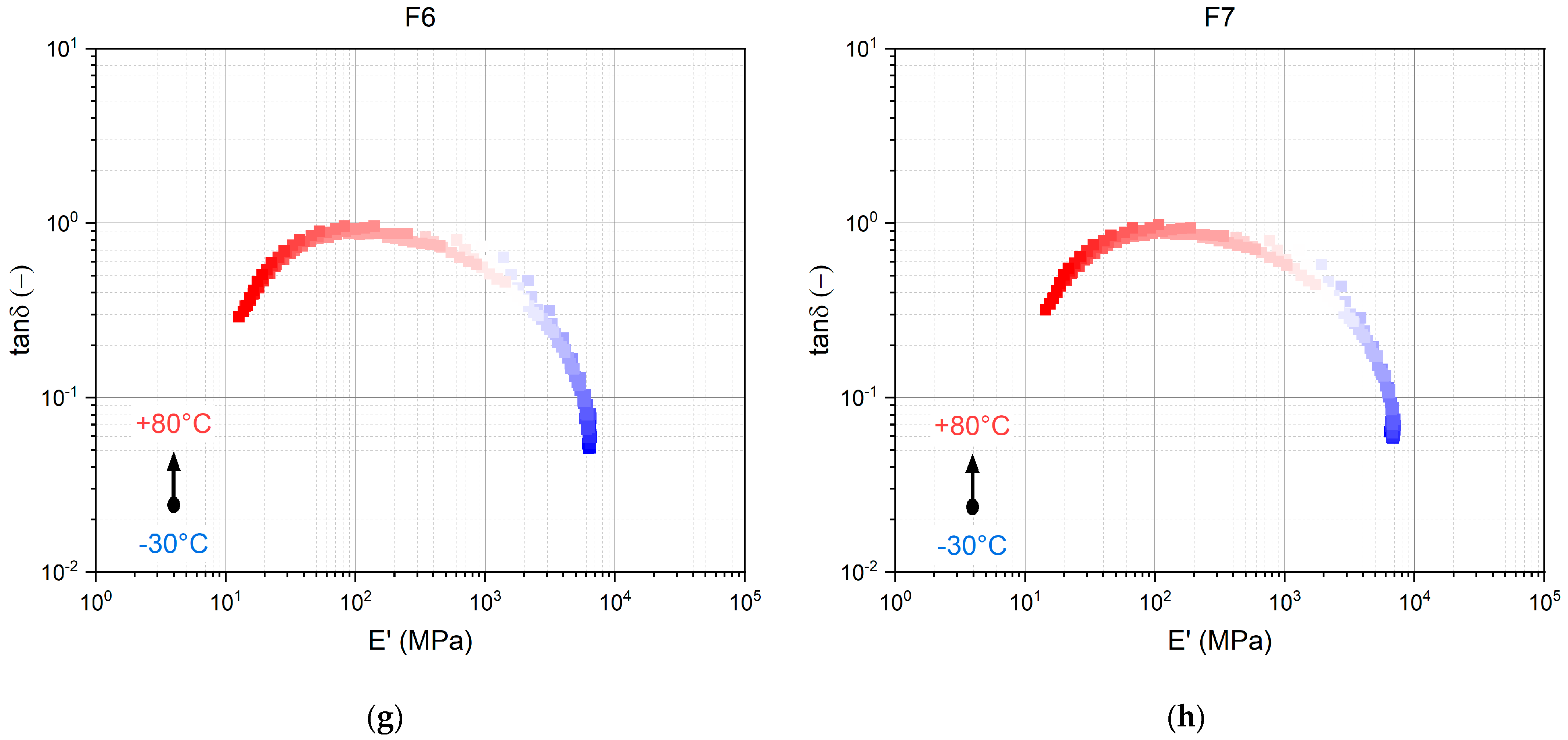
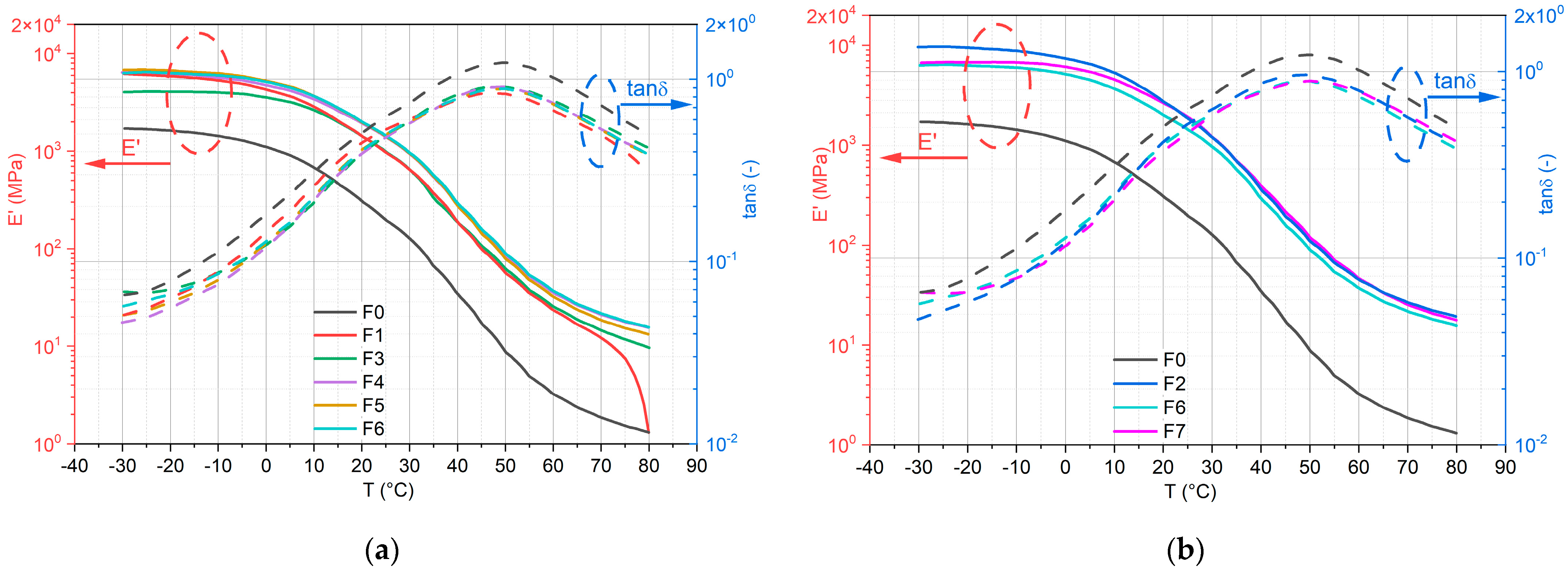
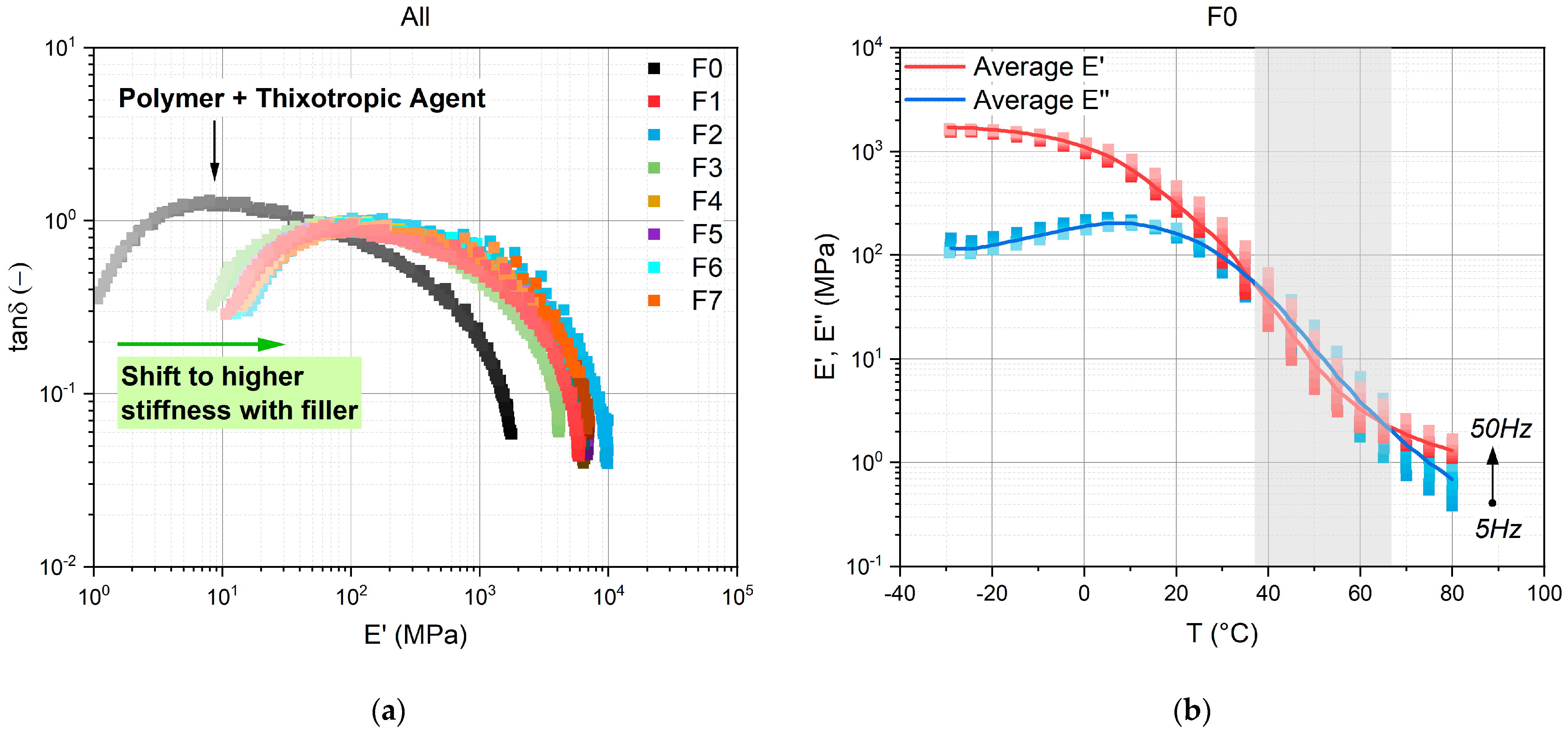
3.1. Results of the Dynamic Thermomechanical Analyses
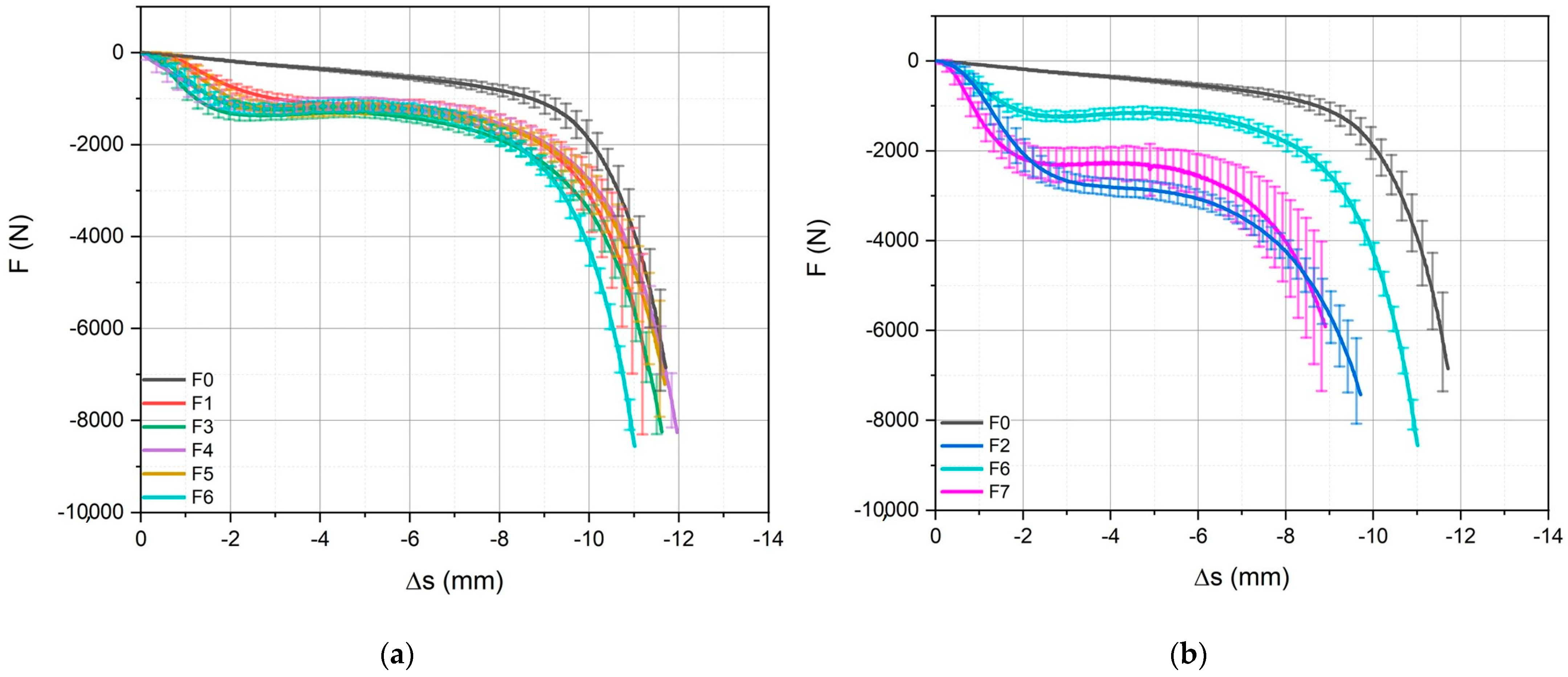
3.2. Results of the Compression Test
4. Discussion
5. Conclusions
- The silica dust influences the stiffness most and shows a distinct content-dependent behavior, with stiffness increasing as the silica dust content increases.
- The silica sand has a lower modulus compared to the basalt sand. This property leads to softer material behavior in the SS-dominant formulations compared to the BS-dominant formulations.
- The compression test shows that the stiffness of the blends is influenced by the amount of dust, while the force plateau is influenced by the amount of sand.
- The DTMA results show a superposition of the behavior of the thixotropy agent and the viscoelastic behavior of the polymer. This effect is negligible as soon as mineral fillers are added.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
Appendix A
References
- Mallick, R.B.; El-Korchi, T. (Eds.) Pavement Engineering: Principles and Practice; CRC Press: Boca Raton, FL, USA, 2022. [Google Scholar] [CrossRef]
- Omar, H.A.; Yusoff, N.I.M.; Mubaraki, M.; Ceylan, H. Effects of moisture damage on asphalt mixtures. J. Traffic Transp. Eng. 2020, 7, 600–628. [Google Scholar] [CrossRef]
- Saberi, F.; Fakhri, M.; Azami, A. Evaluation of warm mix asphalt mixtures containing reclaimed asphalt pavement and crumb rubber. J. Clean. Prod. 2017, 165, 1125–1132. [Google Scholar] [CrossRef]
- Mączka, E.; Mackiewicz, P. Asphalt Mixtures Fatigue Life Considering Various Environmental Impacts. Materials 2023, 16, 966. [Google Scholar] [CrossRef]
- Bressi, S.; Santos, J.; Orešković, M.; Losa, M. A comparative environmental impact analysis of asphalt mixtures containing crumb rubber and reclaimed asphalt pavement using life cycle assessment. Int. J. Pavement Eng. 2021, 22, 524–538. [Google Scholar] [CrossRef]
- Dehouche, N.; Kaci, M.; Mokhtar, K.A. Influence of thermo-oxidative aging on chemical composition and physical properties of polymer modified bitumens. Constr. Build. Mater. 2012, 26, 350–356. [Google Scholar] [CrossRef]
- Bao, B.; Liu, J.; Li, S.; Si, C.; Zhang, Q. Laboratory Evaluation of the Relationship of Asphalt Binder and Asphalt Mastic via a Modified MSCR Test. Coatings 2023, 13, 304. [Google Scholar] [CrossRef]
- Fan, S.; Zhu, H.; Lu, Z. Fatigue behavior and healing properties of aged asphalt binders. J. Mater. Civ. Eng. 2022, 34, 04022117. [Google Scholar] [CrossRef]
- Miglietta, F.; Tsantilis, L.; Baglieri, O.; Santagata, E. A new approach for the evaluation of time–temperature superposition effects on the self-healing of bituminous binders. Constr. Build. Mater. 2021, 287, 122987. [Google Scholar] [CrossRef]
- Lv, H.; Liu, H.; Zhang, C.; Wang, Y.; Han, M.; Tan, Y. Characterization of the healing potential of asphalt binders with separation of thixotropy after cyclic loading. Constr. Build. Mater. 2023, 370, 130686. [Google Scholar] [CrossRef]
- Partl, M.N. Towards improved testing of modern asphalt pavements. Mater. Struct. 2018, 51, 166. [Google Scholar] [CrossRef]
- Micaelo, R.; Jiménez, F.P.; Botella, R.; Miró, R.; Martínez, A.; da Costa, M.S. On the analysis of uniaxial cyclic strain sweep test results to bituminous binders: Stiffness-phase angle curve. Mater. Struct. 2022, 55, 90. [Google Scholar] [CrossRef]
- Çakmak, U.D.; Major, Z. Experimental Thermomechanical Analysis of Elastomers Under Uni- and Biaxial Tensile Stress State. Exp. Mech. 2014, 54, 653–663. [Google Scholar] [CrossRef]
- Çakmak, U.D.; Hiptmair, F.; Major, Z. Applicability of elastomer time-dependent behavior in dynamic mechanical damping systems. Mech. Time-Depend. Mater. 2014, 18, 139–151. [Google Scholar] [CrossRef]
- Çakmak, U.D.; Graz, I.; Moser, R.; Fischlschweiger, M.; Major, Z. Embedded NiTi Wires for Improved Dynamic Thermomechanical Performance of Silicone Elastomers. Materials 2020, 13, 5076. [Google Scholar] [CrossRef]
- Emminger, C.; Çakmak, U.D.; Preuer, R.; Graz, I.; Major, Z. Hyperelastic Material Parameter Determination and Numerical Study of TPU and PDMS Dampers. Materials 2021, 14, 7639. [Google Scholar] [CrossRef]
- Caro, S.; Sanches Diana, B.; Caicedo, B. Methodology to characterize non-standard asphalt materials using DMA testing: Application to natural asphalt mixtures. Int. J. Pavement Eng. 2015, 16, 1–10. [Google Scholar] [CrossRef]
- Gkyrtis, K.; Loizos, A.; Plati, C. A mechanistic framework for field response assessment of asphalt pavements. Int. J. Pavement Res. Technol. 2020, 14, 174–185. [Google Scholar] [CrossRef]
- Chabot, A.; Chupin, O.; Deloffre, L.; Duhamel, D. Viscoroute 2.0 A: Tool for the simulation of moving load effects on asphalt pavement. Road Mater. Pavement Des. 2010, 11, 227–250. [Google Scholar] [CrossRef][Green Version]
- Liu, H.; Zeiada, W.; Al-Khateeb, G.G.; Shanableh, A.; Samarai, M. A framework for linear viscoelastic characterization of asphalt mixtures. Mater. Struct. 2020, 53, 32. [Google Scholar] [CrossRef]
- Tan, G.; Wang, W.; Cheng, Y.; Wang, Y.; Zhu, Z. Master Curve Establishment and Complex Modulus Evaluation of SBS-Modified Asphalt Mixture Reinforced with Basalt Fiber Based on Generalized Sigmoidal Model. Polymers 2020, 12, 1586. [Google Scholar] [CrossRef]
- Zhao, R.; Jing, F.; Wang, R.; Cai, J.; Zhang, J.; Wang, Q.; Xie, H. Influence of oligomer content on viscosity and dynamic mechanical properties of epoxy asphalt binders. Constr. Build. Mater. 2022, 338, 127524. [Google Scholar] [CrossRef]
- Clara, E.; Barra, B.S.; Teixeira, L.H.; Mikowski, A.; Hughes, G.B.; Nguyen, M.L. Influence of polymeric molecular chain structure on the rheological-mechanical behavior of asphalt binders and porous asphalt mixes. Constr. Build. Mater. 2023, 369, 130575. [Google Scholar] [CrossRef]
- Jing, F.; Wang, R.; Zhao, R.; Li, C.; Cai, J.; Ding, G.; Wang, Q.; Xie, H. Enhancement of Bonding and Mechanical Performance of Epoxy Asphalt Bond Coats with Graphene Nanoplatelets. Polymers 2023, 15, 412. [Google Scholar] [CrossRef] [PubMed]
- Ma, X.; Wang, Y.; Hou, J.; Sheng, Y.; Zheng, W.; Wu, S. Study on Thixotropic Properties of Asphalt Mastics Based on Energy Viewpoint. Coatings 2023, 13, 650. [Google Scholar] [CrossRef]
- Mewis, J.; Wagner, N.J. Thixotropy. Adv. Colloid Interface Sci. 2009, 147, 214–227. [Google Scholar] [CrossRef]


| Material Formulation | Silica Dust (SD) in wt% | Silica Sand (SS) in wt% | Basalt Sand (BS) in wt% |
|---|---|---|---|
| F0 | 0 | 0 | 0 |
| F1 | 0 | 0 | 80 |
| F2 | 20 | 0 | 60 |
| F3 | 0 | 20 | 60 |
| F4 | 5 | 5 | 70 |
| F5 | 0 | 10 | 70 |
| F6 | 10 | 0 | 70 |
| F7 | 15 | 15 | 50 |
| Filler/Processing Agent | Bulk Density [g/cm3] |
|---|---|
| Thixotropy Agent | 2.2 |
| Pigment | 4.6 |
| Catalysator | 0.62 |
| Binder | 0.98 |
| Silica dust | 2.65 |
| Silica Sand | 2.65 |
| Basalt Sand | 2.71 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Emminger, C.; Cakmak, U.D.; Lackner, M.; Major, Z. Mechanical Characterization of Asphalt Mixtures Based on Polymeric Resin and Thixotropic Filler as a Substitute for Bitumen. Coatings 2023, 13, 932. https://doi.org/10.3390/coatings13050932
Emminger C, Cakmak UD, Lackner M, Major Z. Mechanical Characterization of Asphalt Mixtures Based on Polymeric Resin and Thixotropic Filler as a Substitute for Bitumen. Coatings. 2023; 13(5):932. https://doi.org/10.3390/coatings13050932
Chicago/Turabian StyleEmminger, Carina, Umut D. Cakmak, Michael Lackner, and Zoltan Major. 2023. "Mechanical Characterization of Asphalt Mixtures Based on Polymeric Resin and Thixotropic Filler as a Substitute for Bitumen" Coatings 13, no. 5: 932. https://doi.org/10.3390/coatings13050932
APA StyleEmminger, C., Cakmak, U. D., Lackner, M., & Major, Z. (2023). Mechanical Characterization of Asphalt Mixtures Based on Polymeric Resin and Thixotropic Filler as a Substitute for Bitumen. Coatings, 13(5), 932. https://doi.org/10.3390/coatings13050932







