A Study on the Adsorption Mechanism and Compactness of the TFS Coating Interfacial Layer
Abstract
:1. Introduction
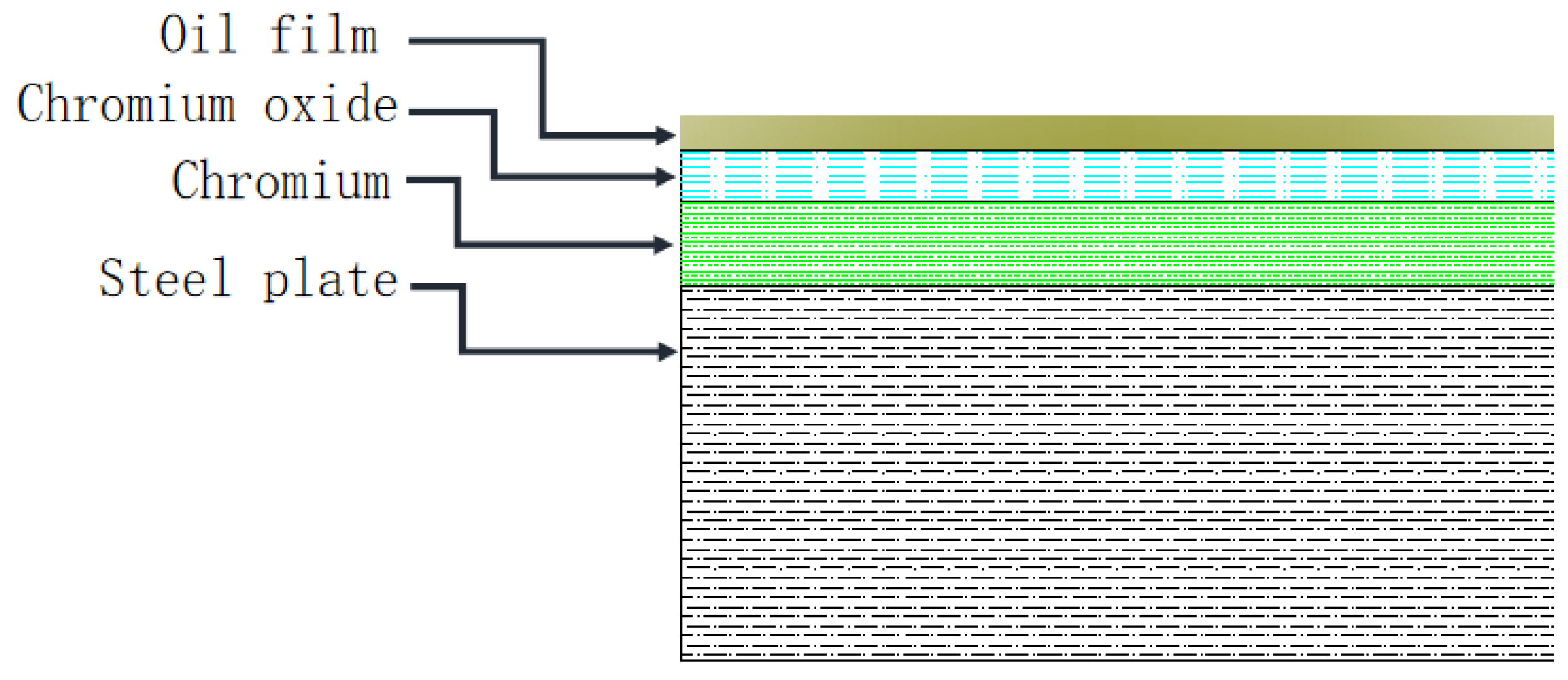
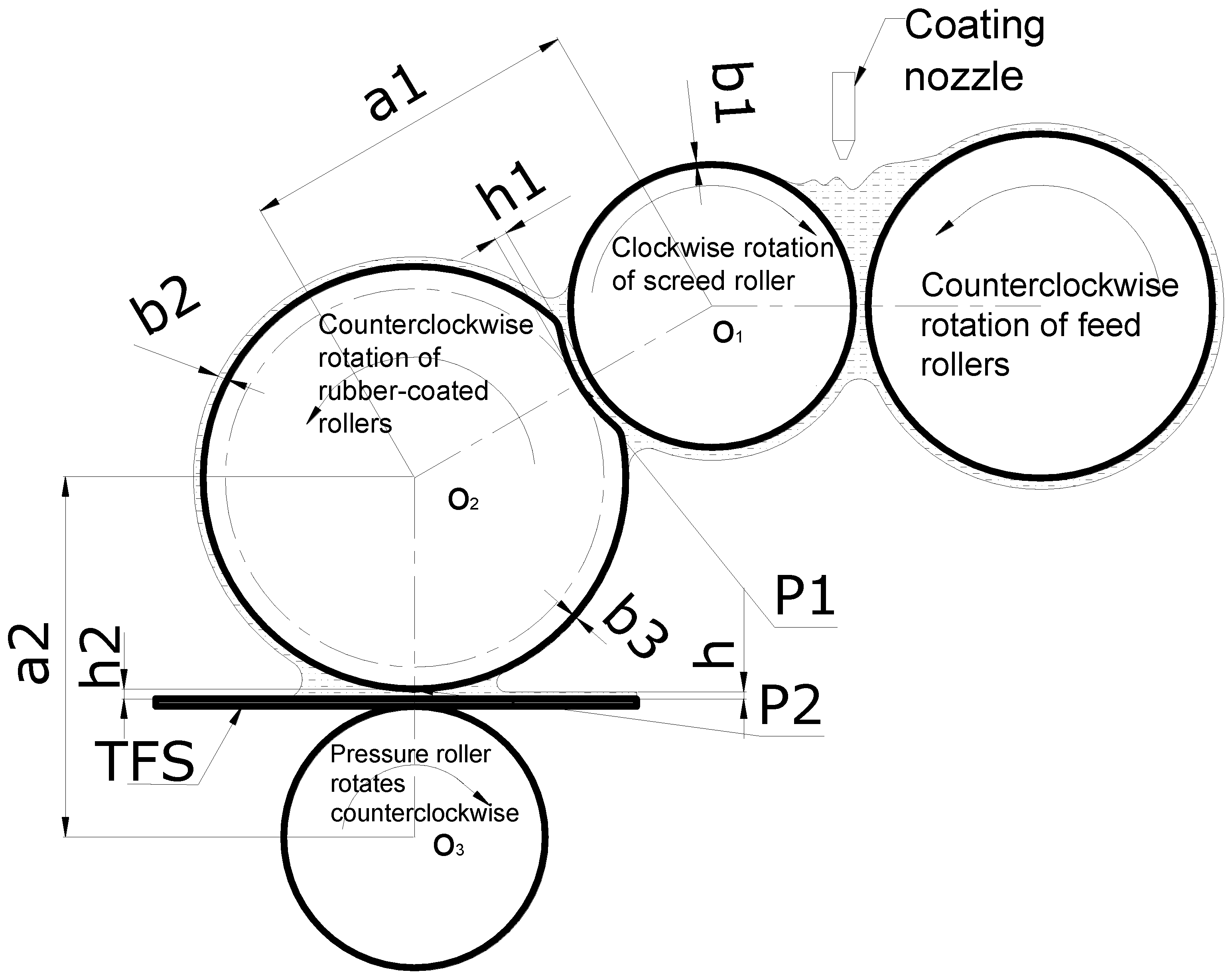
2. Materials and Methods
3. Discussion of Interfacial Layer Adsorption Force
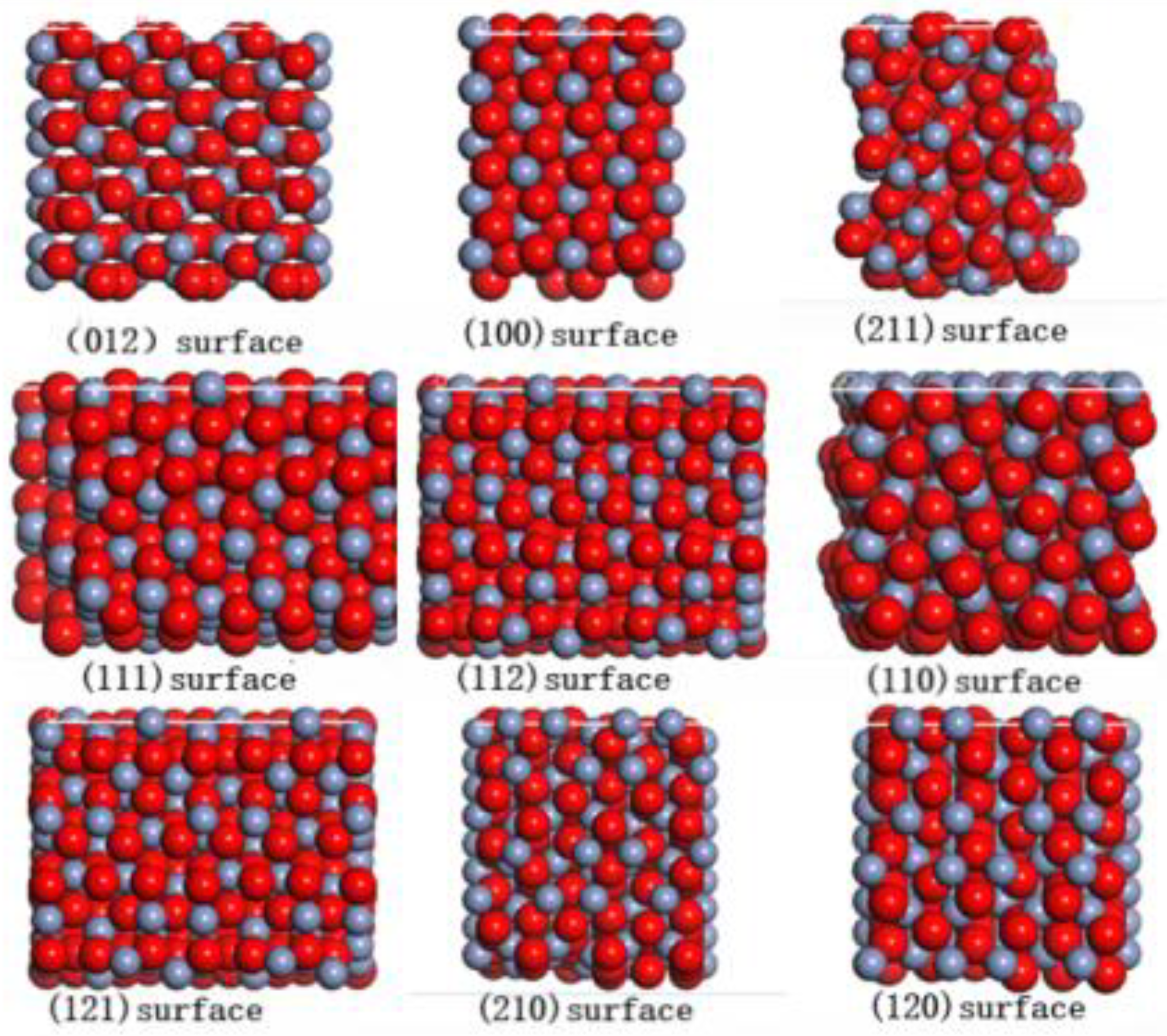
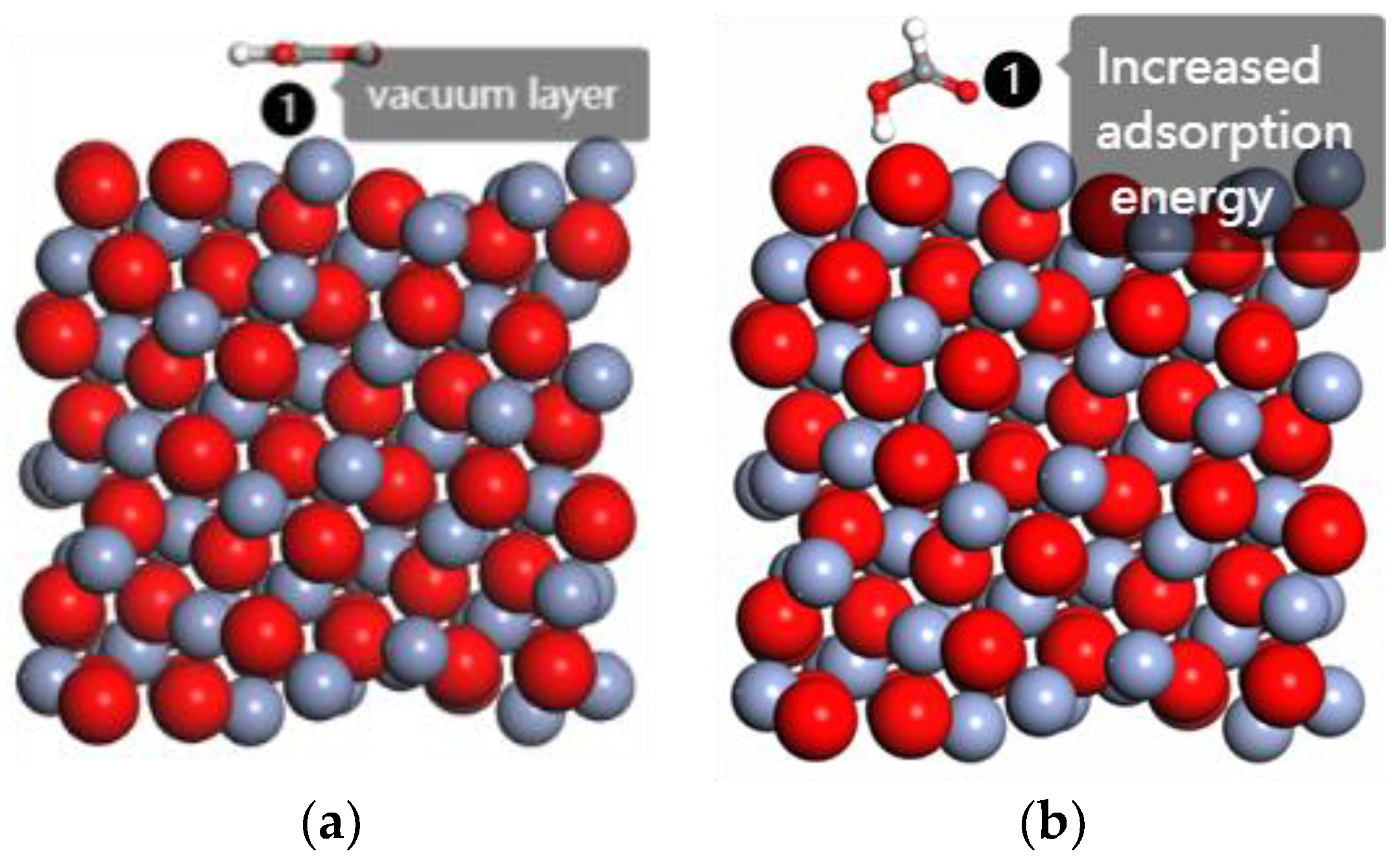
3.1. The Effect of Crystal Surface on the Adsorption Force
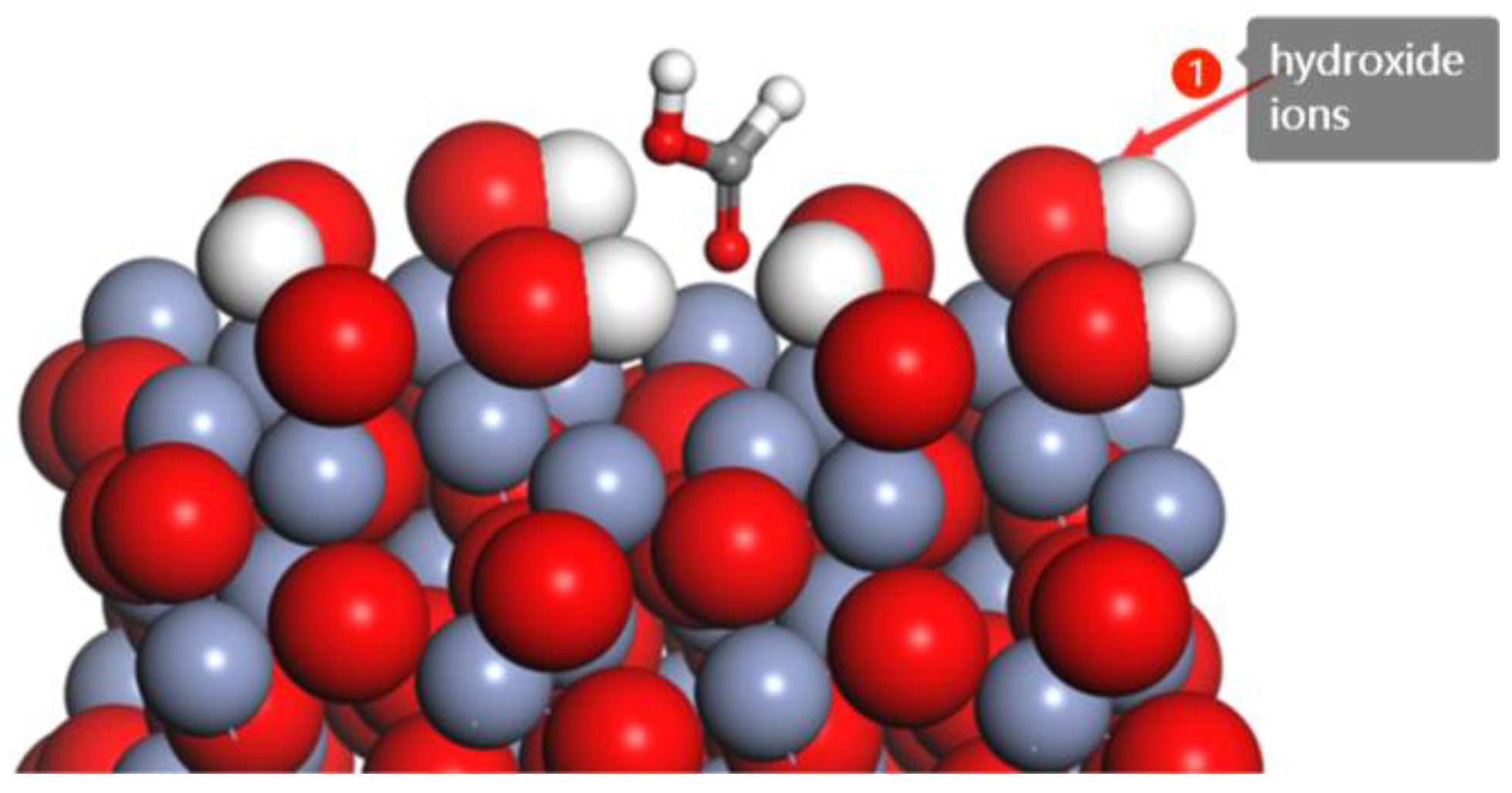
3.2. The Effect of Hydroxide Ions on Adsorption
3.3. Interfacial Layer Adsorption Type
3.4. Analysis of the Adsorption of Polymer Functional Groups in Coatings
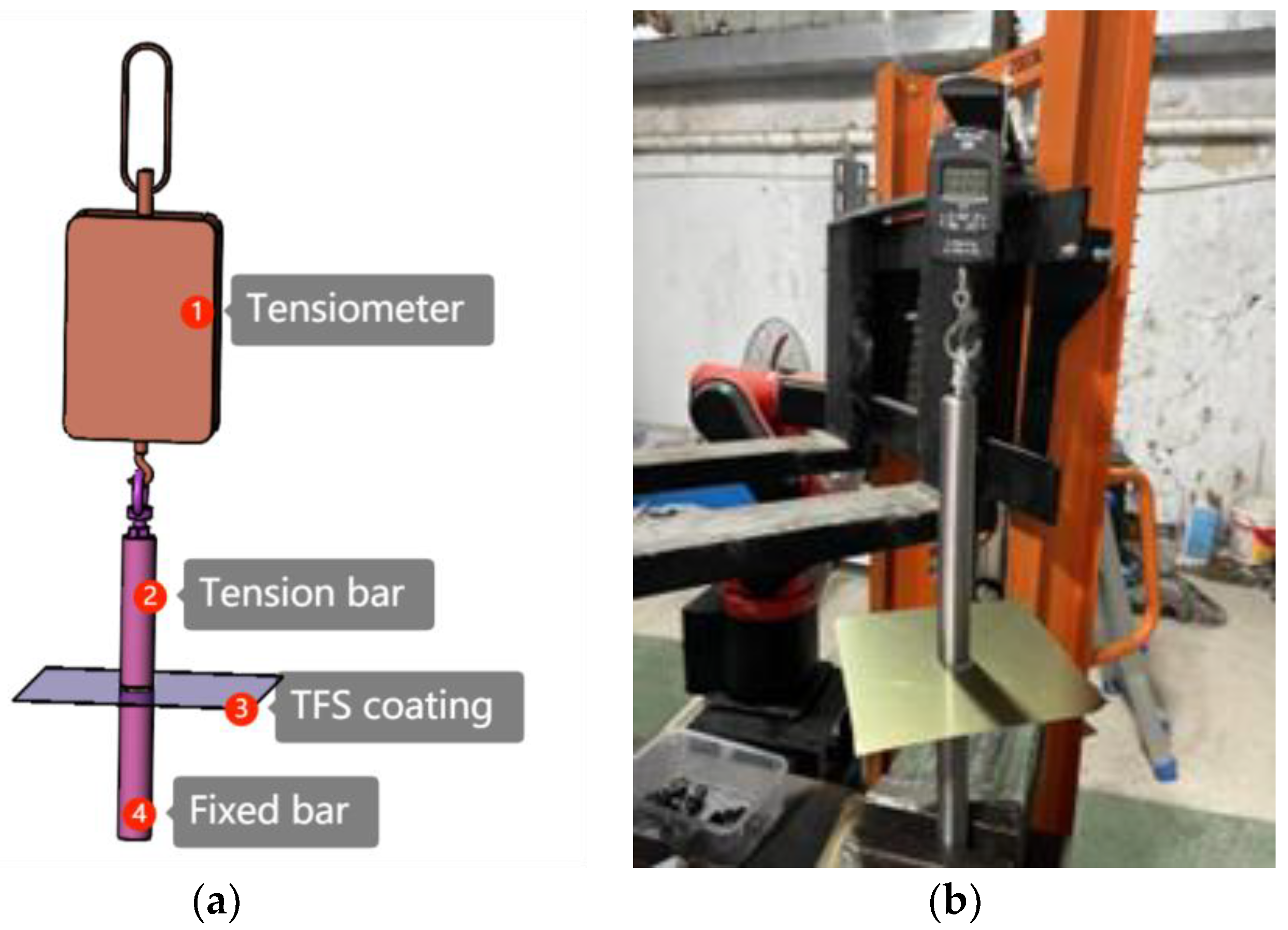
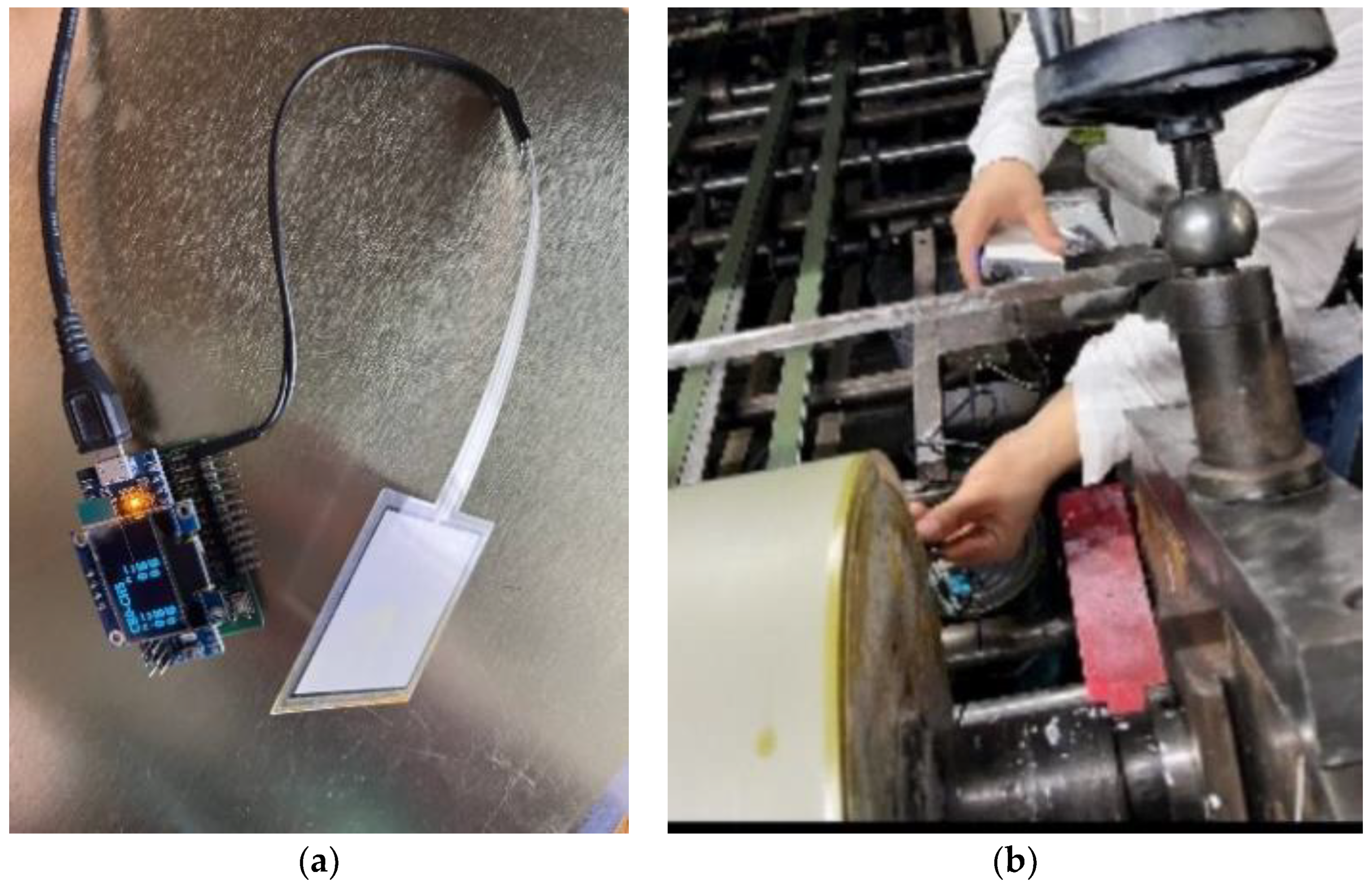
3.5. Experimental Verification
- The results of the experiments were:
- Experiment 1: polyether polyol side first off; w1 = 10.54 kg; w2 = 13.74 kg.
- Experiment 2: polyester-type polyurethane coating comes offside first; w3 = 14.48 kg; w4 = 17.79 kg.
4. Discussion of the Compactness of the Interface Layer
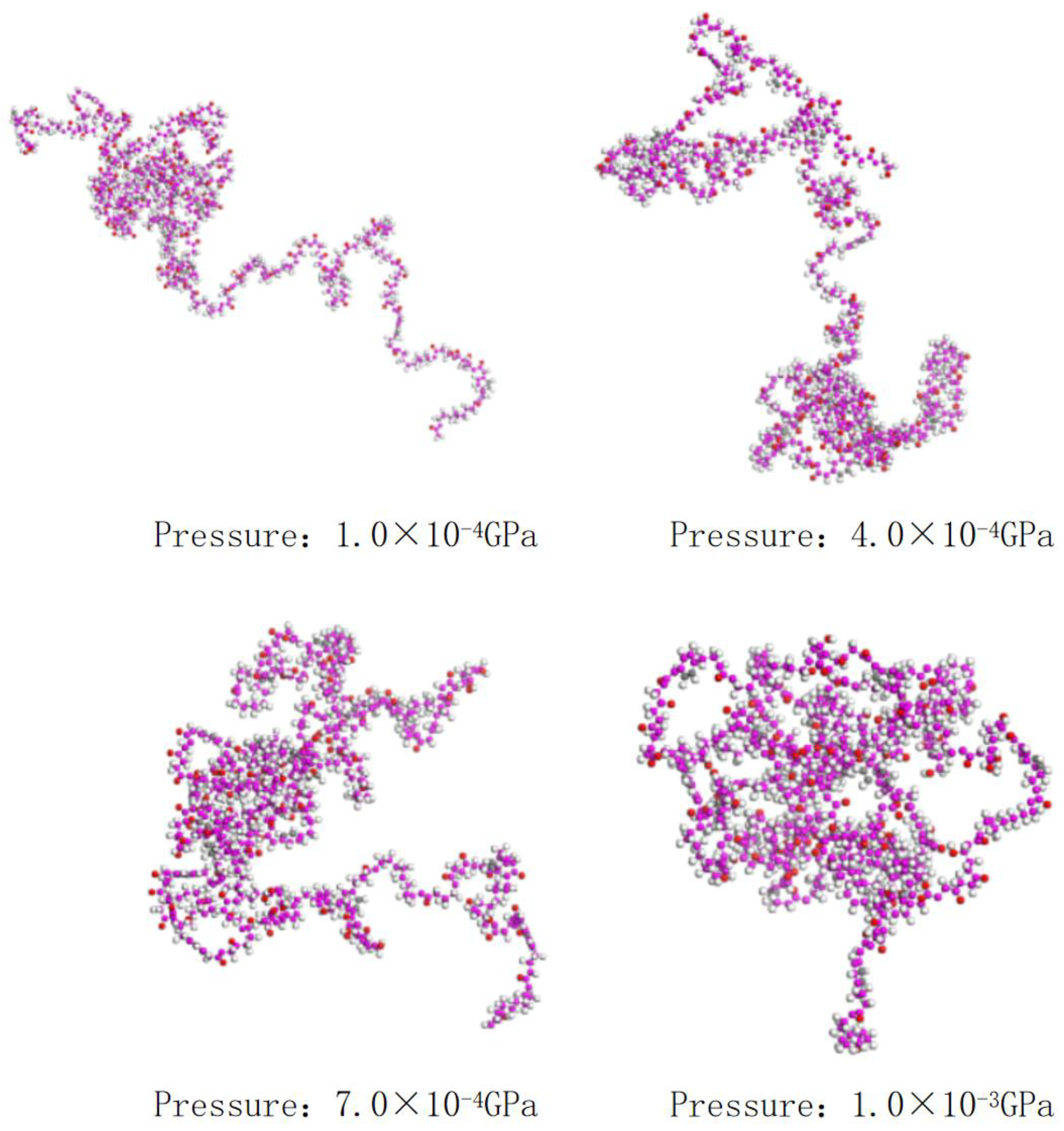
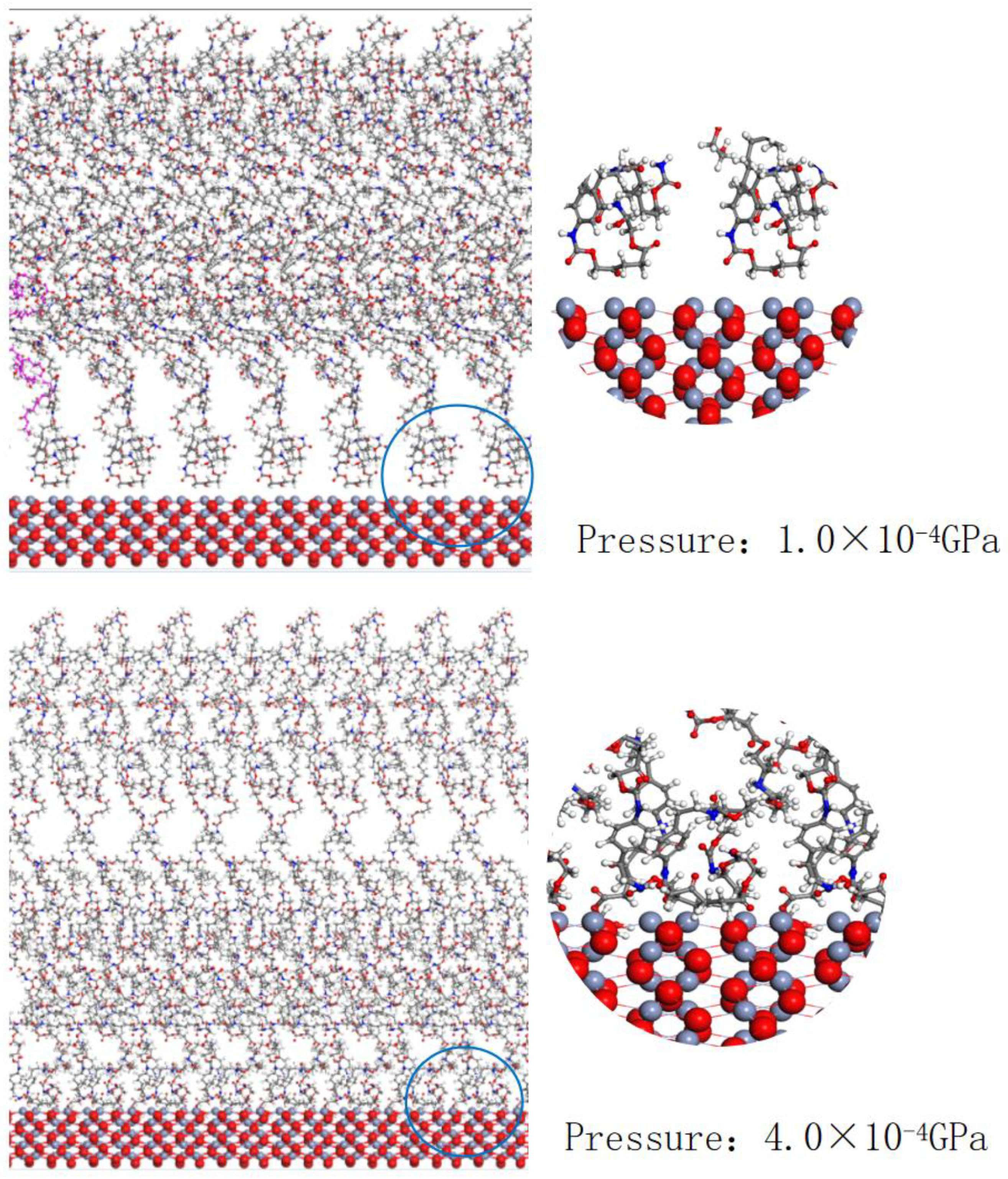
4.1. A Study of the Morphology and Location of Polymers under Different Pressures
4.2. Experimental Verification
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hong, Z. Development and Application of Electrolytic Chromium-Coatedsteel(TFS) New Products. South. Met. 2002, 127, 13–16. [Google Scholar]
- Pierce, P.E.; Donegan, V.A. The Rheology And Application Characteristics Of Thixotropic Coatings. J. Paint. Technol. 1966, 38, 1–8. [Google Scholar]
- Tanaka, A.; Hanafusa, T.; Kojyo, H.; Inui, T. Adhesion of Biaxially Oriented Polyethylene Terephthalate Film to Tin Free Steel. Trans. Iron Steel Inst. Jpn. 1986, 27, 638–644. [Google Scholar] [CrossRef] [Green Version]
- Ingo, G.M.; Giorgi, L.; Zacchetti, N.; Azzerri, N. Electrochemical and XPS studies on lacquer—Low tinplated steel adhesion. Corros. Sci. 1992, 33, 361–377. [Google Scholar] [CrossRef]
- Yong, Y. System Integration and Experimental Study of Laser Automatic Scratch Detection of Film-Based Interface Bond Strength. Master’s Thesis, Jiangsu University, Zhenjiang, China, 2009. [Google Scholar]
- Melvin, C.; Jewell, E.; de Vooys, A.C.A.; Lammers, K.R.; Murray, N.M. Surface and Adhesion Characteristics of Current and Next Generation Steel Packaging Materials. J. Packag. Technol. Res. 2018, 2, 93–103. [Google Scholar] [CrossRef] [Green Version]
- Liu, J.; Zhang, Q.; Zhang, B.; Yu, M. The Bonding Mechanism of the Micro-Interface of Polymer Coated Steel. Polymers 2020, 12, 3052. [Google Scholar] [CrossRef]
- Whiteside, J.; Vooys, A.C.A.d.; Sackett, E.E.; McMurray, H.N. Influence of uniaxial deformation on surface morphology and corrosion performance of chromium-based coatings for packaging steel. Corros. Sci. 2021, 190, 109662. [Google Scholar] [CrossRef]
- Manoj Prabhakar, J.; de Vooys, A.C.A.; Rohwerder, M. In situ microscopic investigation of ion migration on the surface of chromium coated steels. NPJ Mater. Degrad. 2022, 6, 76. [Google Scholar] [CrossRef]
- Yan, L.Z.S. Theory and Practice of Molecular Dynamics Simulation, 1st ed.; Science Press: Beijing, China, 2013; p. 222. [Google Scholar]
- Yuan, S.z.h. Molecular Simulation Theory and Experiment, 1st ed.; Chemical Industry Press: Beijing, China, 2016; pp. 2–3. [Google Scholar]
- Talman, J.D.; Mermin, N.D. Special Functions: A Group Theoretic Approach. Phys. Today 1970, 23, 71. [Google Scholar] [CrossRef]
- Goldstein. Classical Mechanics; Addison-Wesley: Boston, MA, USA, 1969. [Google Scholar]
- Pang, X. High-resolution study of the interface of chromium oxide thin films. J. Vac. Sci. Technol. 2008, 5, 420–423. [Google Scholar] [CrossRef]
- Liu, Z.S.; Yu, X.X.; Zhang, J.H.; Liu, X.H.; Ye, J.D.; Ren, F.F.; Wang, Y.W.; Xu, W.Z.; Zhou, D.; Zhang, R.; et al. Enhanced light output from deep ultraviolet light-emitting diodes enabled by high-order modes on a photonic crystal surface. Opt. Lett. 2023, 48, 247–250. [Google Scholar] [CrossRef] [PubMed]
- Zhang, H.; Gong, Y.D.; Liang, C.Y.; Sun, Y.; Zhao, J.B. Study on milling material removal mechanism and surface integrity of nickel-based single crystal superalloy DD5. Int. J. Adv. Manuf. Technol. 2023, 125, 2323–2338. [Google Scholar] [CrossRef]
- Tsai, T.H.; Wu, Y.C.; Yang, S.S.; Chen, C.H. Optimization of Amorphous Si/Crystalline Si Heterojunction Solar Cells by BF2 Ion Implantation. Jpn. J. Appl. Phys. 2012, 51, 04DP07. [Google Scholar] [CrossRef]
- Allen, M.P.; Tildesley, D.J. Computer Simulation of Liquids; Oxford University Press: Oxford, UK, 1988. [Google Scholar]
- Li, X.H.; Li, Y.L.; Qian, C.; Zhao, J.; Wang, S.J. Molecular dynamics study of the mechanical and tribological properties of graphene oxide-reinforced polyamide 66/nitrile butadiene rubber composites. Appl. Phys. A Mater. 2023, 129, 276. [Google Scholar] [CrossRef]
- Jia, Y.; Zhao, H.R.; Chen, Y.H.; Liang, X.Y.; Tao, H.G.; Xu, G.Z.; Chang, C. Experimental study combined with density functional theory and molecular dynamics simulation on the mechanism of glucose alcoholysis reaction. Asia-Pac. J. Chem. Eng. 2023, 18, e2901. [Google Scholar] [CrossRef]
- Rahnamoun, A.; Kaymak, M.C.; Manathunga, M.; Gotz, A.W.; van Duin, A.C.T.; Merz, K.M.; Aktulga, H.M. ReaxFF/AMBER-A Framework for Hybrid Reactive/Nonreactive Force Field Molecular Dynamics Simulations. J. Chem. Theory Comput. 2020, 16, 7645–7654. [Google Scholar] [CrossRef]
- Cascio, M.; Baroli, D.; Bordas, S.; Deretzis, I.; Falci, G.; Magliano, A.; La Magna, A. Coupled molecular-dynamics and finite-element-method simulations for the kinetics of particles subjected to field-mediated forces. Phys. Rev. E 2019, 99, 063307. [Google Scholar] [CrossRef]
- Deng, L.; Miyatani, K.; Amma, S.; Suehara, M.; Ono, M.; Yamamoto, Y.; Urata, S.; Du, J.C. Reaction Mechanisms and Interfacial Behaviors of Sodium Silicate Glass in an Aqueous Environment from Reactive Force Field-Based Molecular Dynamics Simulations. J. Phys. Chem. C 2019, 123, 21538–21547. [Google Scholar] [CrossRef]
- Kondratyuk, N.D.; Pisarev, V.V. Calculation of viscosities of branched alkanes from 0.1 to 1000 MPa by molecular dynamics methods using COMPASS force field. Fluid Phase Equilibria 2019, 498, 151–159. [Google Scholar] [CrossRef]
- Perez-Conesa, S.; Torrico, F.; Martinez, J.M.; Pappalardo, R.R.; Marcos, E.S. A general study of actinyl hydration by molecular dynamics simulations using ab initio force fields. J. Chem. Phys. 2019, 150, 104504. [Google Scholar] [CrossRef]
- Gupta, N.K.; Hernandez-Fontes, C.; Achary, S.N. Sodium/lithium 3d transition metalates for chemisorption of gaseous pollutants: A review. Mater. Today Chem. 2023, 27, 101329. [Google Scholar] [CrossRef]
- Kweon, S.; Lee, S.; Lee, J.H.; Park, M.B. Comparative study of interzeolite transformed metallosilicates for the chemisorption of benzene, toluene, and xylenes. Appl. Surf. Sci. 2023, 612, 155851. [Google Scholar] [CrossRef]
- Najjaran, A.; Meibodi, S.; Ma, Z.W.; Bao, H.S.; Roskilly, T. Experimentally Validated Modelling of an Oscillating Diaphragm Compressor for Chemisorption Energy Technology Applications. Energies 2023, 16, 489. [Google Scholar] [CrossRef]
- Yang, H.H.; Guan, P.F. Thickness-dependent oxygen chemisorption behaviors on (111) surfaces of two-dimensional FCC metals Al and Cu: First-principles study. Comput. Mater. Sci. 2023, 219, 112022. [Google Scholar] [CrossRef]
- Zheng, B.W.; Oliveira, F.L.; Ferreira, R.N.B.; Steiner, M.; Hamann, H.; Gu, G.X.; Luan, B.Q. Quantum Informed Machine-Learning Potentials for Molecular Dynamics Simulations of CO2′s Chemisorption and Diffusion in Mg-MOF-74. ACS Nano 2023, 17, 5579–5587. [Google Scholar] [CrossRef]
- Guohua, W. Factors affecting the adhesion of water-based polyurethane resins to metals and solutions. Coat. Ind. 2004, 7, 41–43+63. [Google Scholar]
- Shaoxiong, L.; Yingju, L. Polyurethane Adhesives, 1st ed.; Chemical Industry Press: Beijing, China, 1998; pp. 20–32. [Google Scholar]
- Khaled, K.F.; Amin, M.A. Electrochemical and molecular dynamics simulation studies on the corrosion inhibition of aluminum in molar hydrochloric acid using some imidazole derivatives. J. Appl. Electrochem. 2009, 39, 2553–2568. [Google Scholar] [CrossRef]





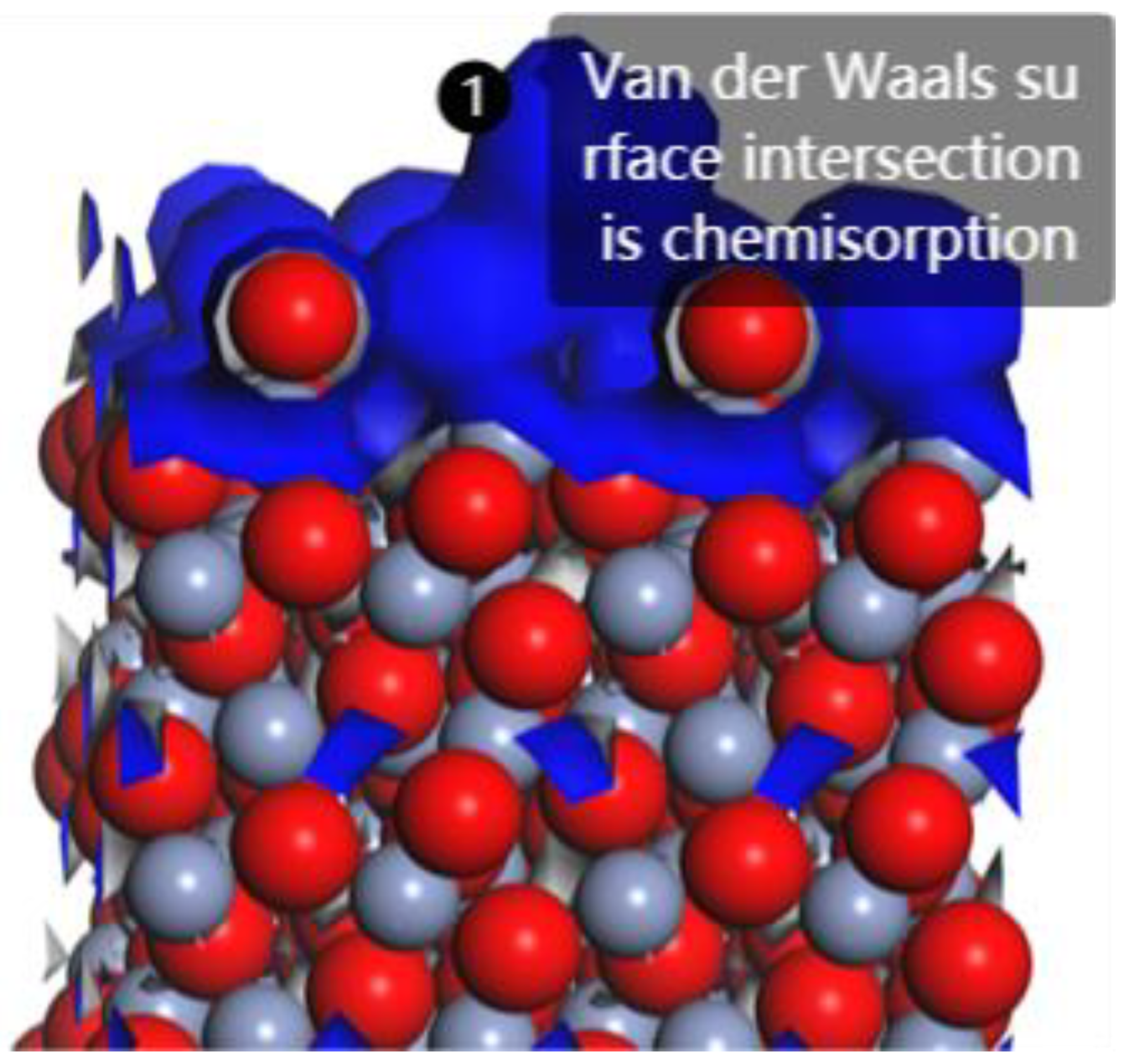
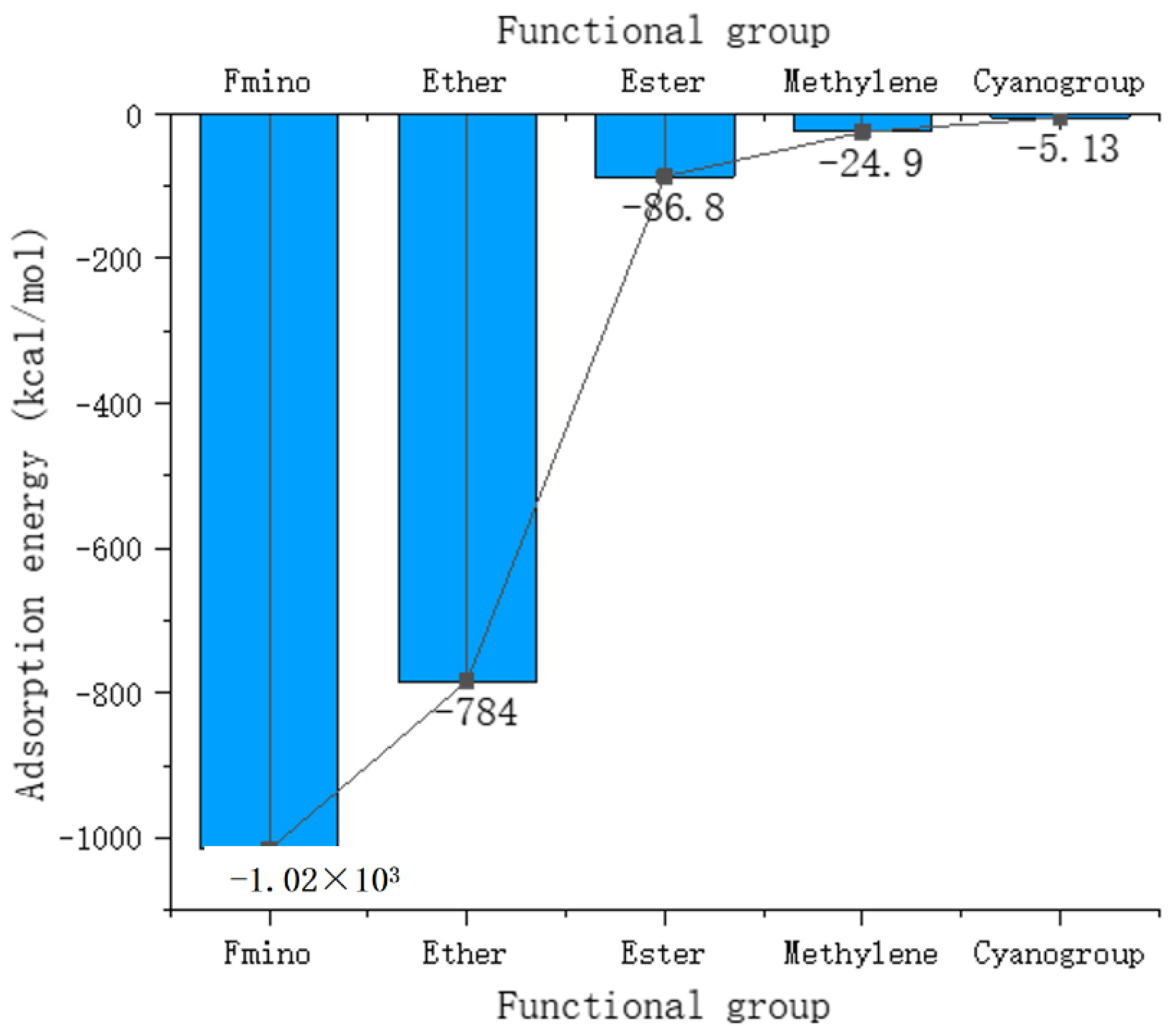





| Crystal Surface | Total Energy Ejmc | Chromium Oxide Energy Eyhg | Ester Group Molecular Energy Ezhiji | Adsorption Energy Exfn |
|---|---|---|---|---|
| Cr2O3 (100) | −59,232.10766 | −21.211918 | −59,192.03275 | −18.862986 |
| Cr2O3 (110) | −30,247.47664 | −12.539503 | −30,148.17373 | −86.763401 |
| Cr2O3 (111) | −98,680.97528 | −18.486182 | −98,611.51571 | −50.973385 |
| Cr2O3 (012) | −27,462.46695 | −23.911311 | −27,422.79271 | −15.762935 |
| Cr2O3 (120) | −158,228.6778 | −18.442777 | −158,170.6688 | −39.566181 |
| Cr2O3 (210) | −179,539.373 | −17.068746 | −179,476.3186 | −45.98569 |
| Cr2O3 (211) | −60,301.83219 | −17.26573 | −60,250.74186 | −33.824593 |
| Cr2O3 (121) | −119,487.8889 | −16.411669 | −119,417.4177 | −54.059536 |
| Cr2O3 (112) | −119,467.2344 | −19.72705 | −119,417.4177 | −30.089643 |
| Name | Condition | Toxic or Not | Color | Coating Method |
|---|---|---|---|---|
| Polyether polyol | Liquid | No | Transparent | Roll coating |
| Polyester polyol | Liquid | No | White | Roll coating |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, Y.; Zhao, T.; Liu, K. A Study on the Adsorption Mechanism and Compactness of the TFS Coating Interfacial Layer. Coatings 2023, 13, 1290. https://doi.org/10.3390/coatings13071290
Xie Y, Zhao T, Liu K. A Study on the Adsorption Mechanism and Compactness of the TFS Coating Interfacial Layer. Coatings. 2023; 13(7):1290. https://doi.org/10.3390/coatings13071290
Chicago/Turabian StyleXie, Yafei, Tong Zhao, and Kai Liu. 2023. "A Study on the Adsorption Mechanism and Compactness of the TFS Coating Interfacial Layer" Coatings 13, no. 7: 1290. https://doi.org/10.3390/coatings13071290
APA StyleXie, Y., Zhao, T., & Liu, K. (2023). A Study on the Adsorption Mechanism and Compactness of the TFS Coating Interfacial Layer. Coatings, 13(7), 1290. https://doi.org/10.3390/coatings13071290






