Polarization-Angle-Insensitive Dual-Band Perfect Metamaterial Absorbers in the Visible Region: A Theoretical Study
Abstract
:1. Introduction
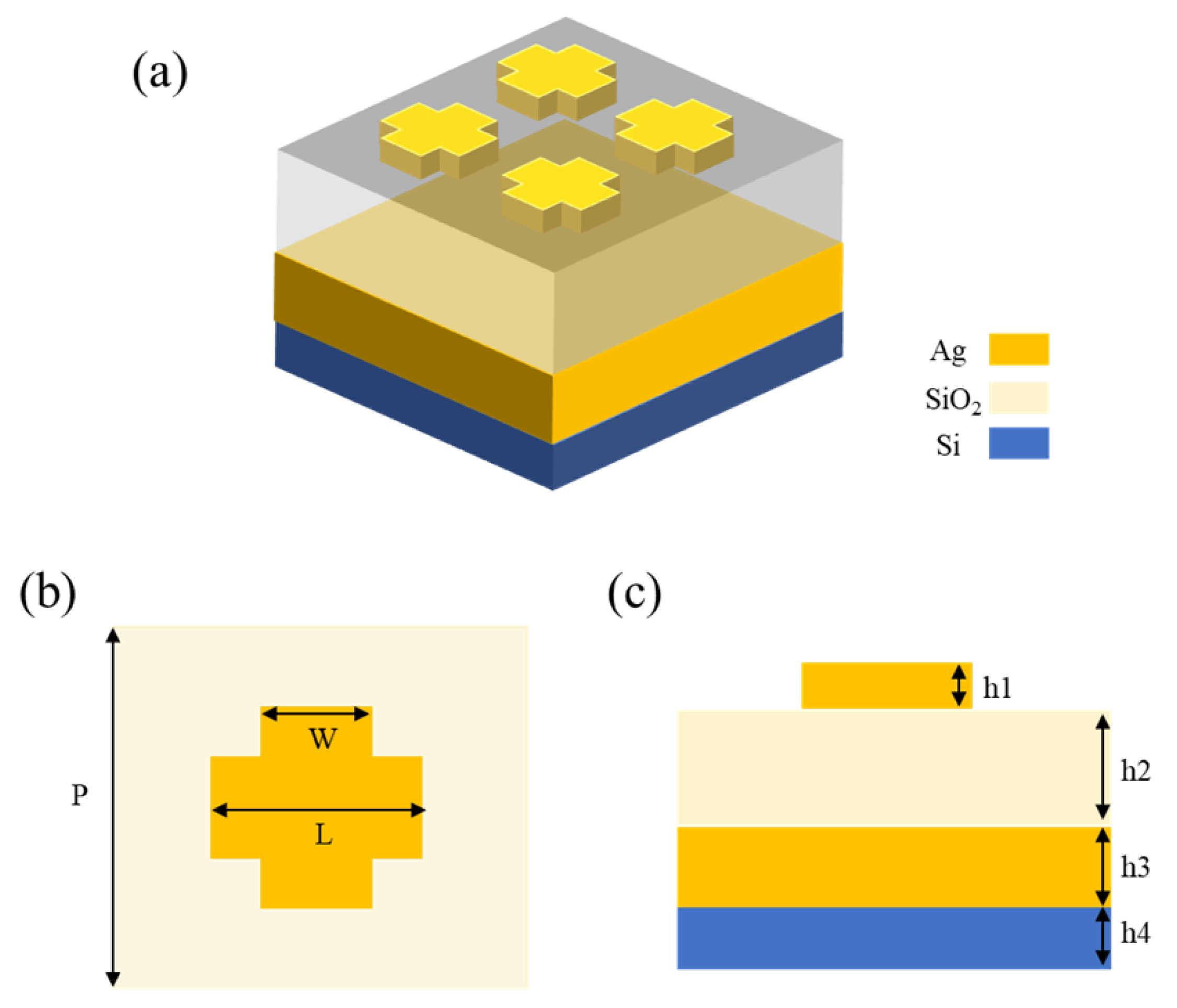
2. Structure Design and Methods
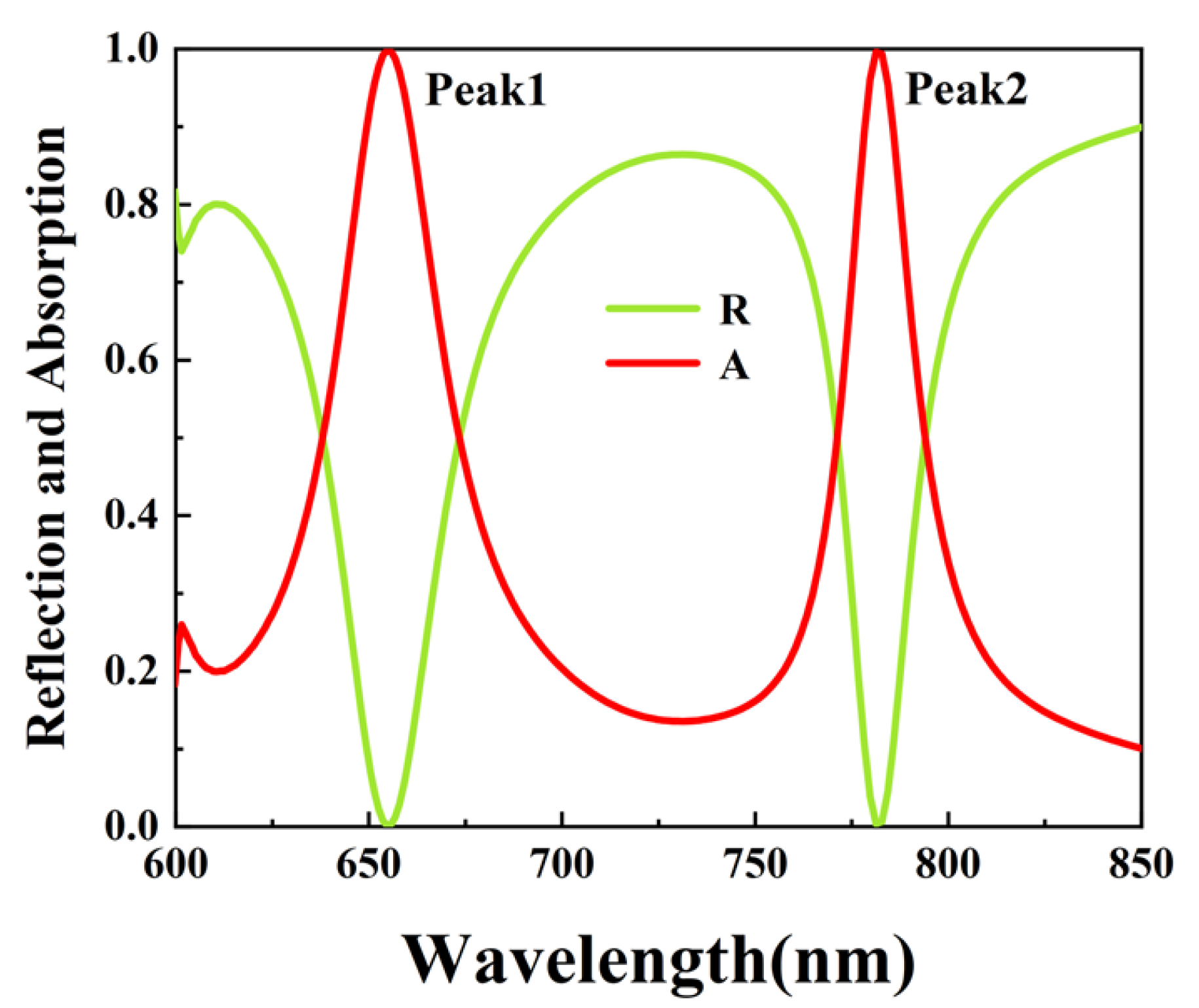
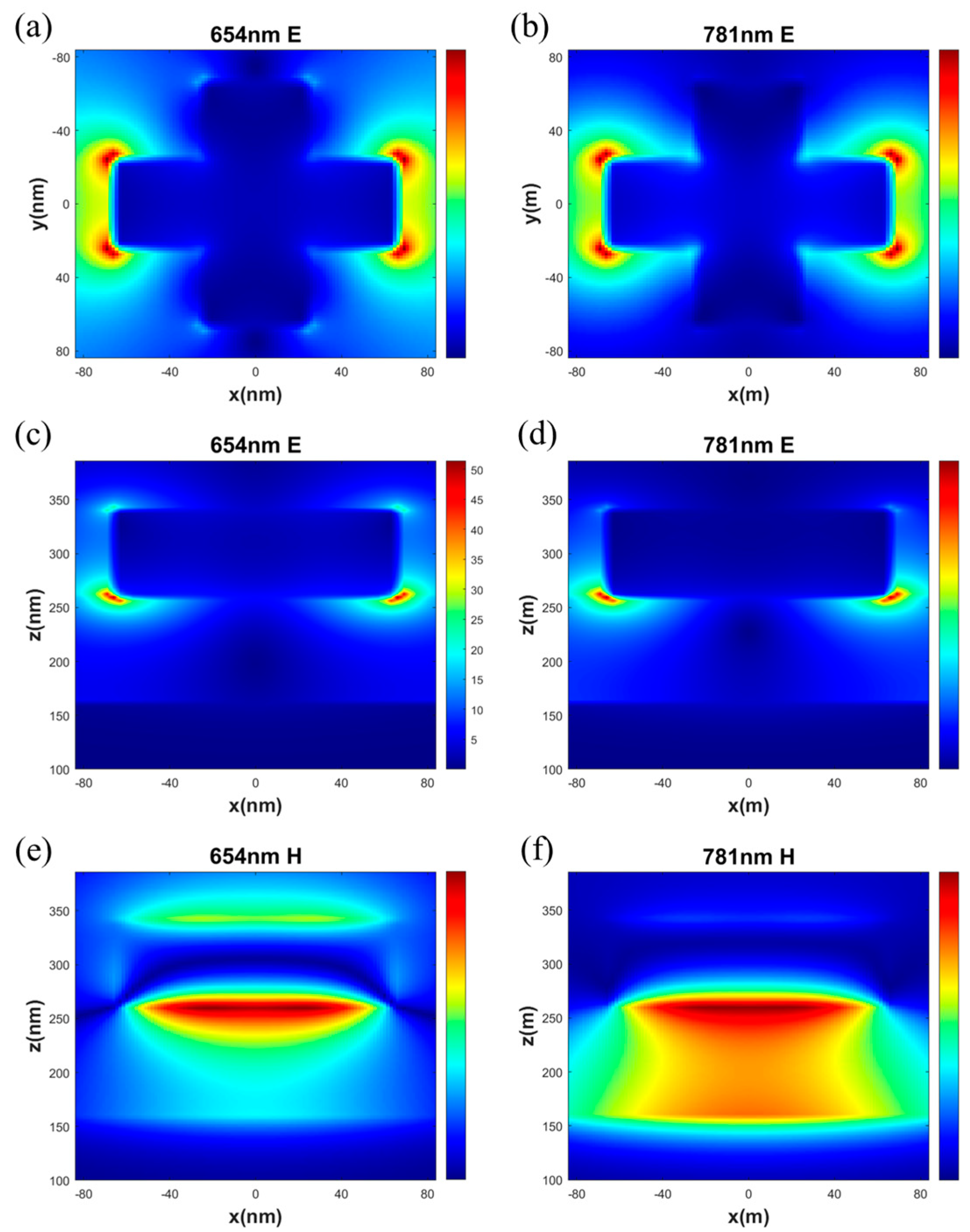
3. Results and Discussion
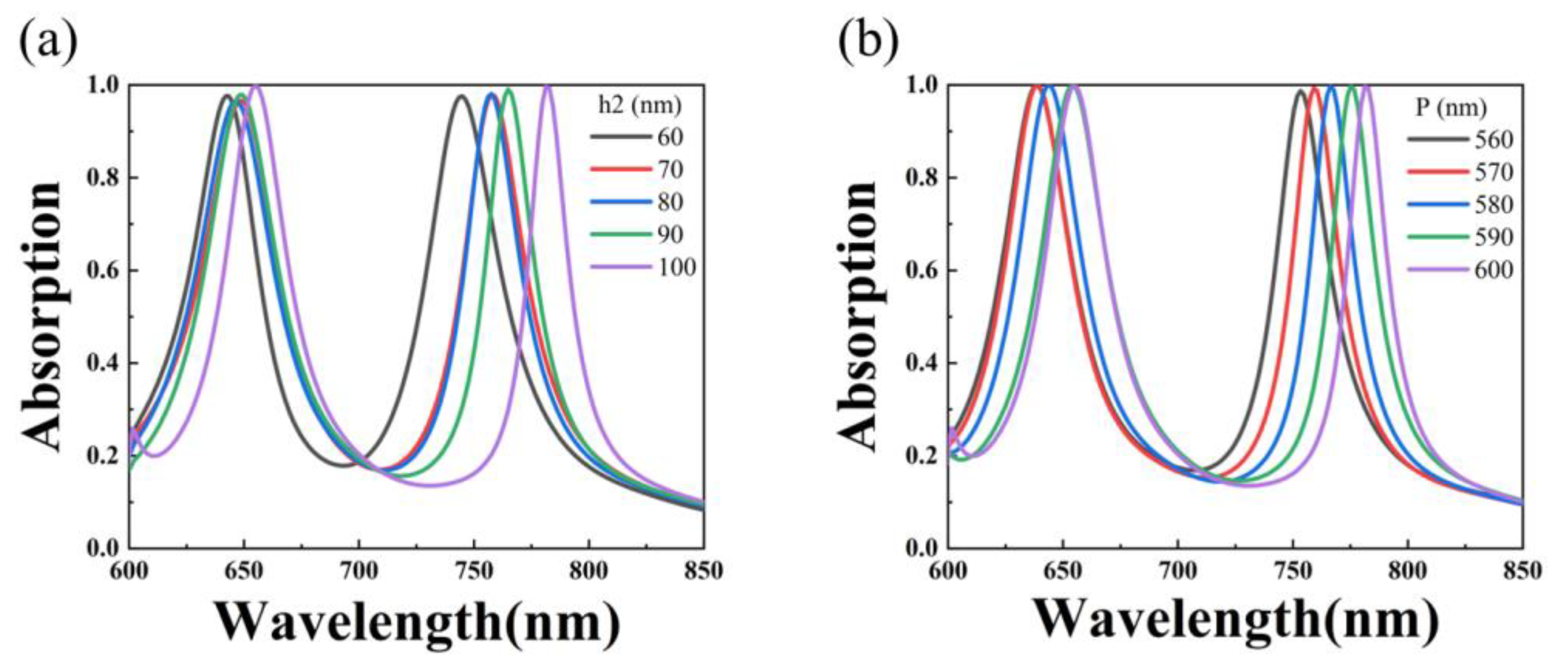
3.1. Modulation Analysis of the Period and Dielectric Thickness of the Absorber
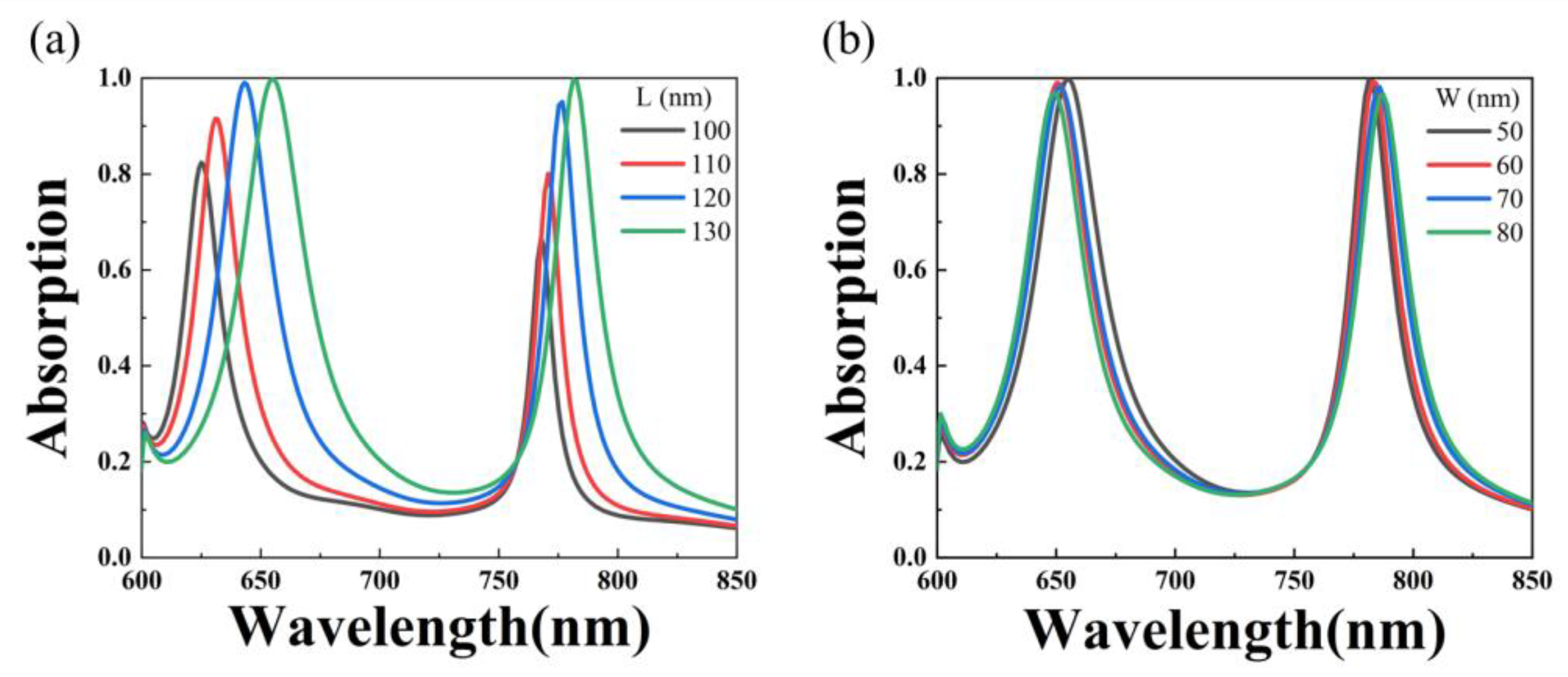
3.2. Size Modulation Analysis of the Cross-Structure
3.3. Modulation Analysis of Refractive Index and Polarization Angle of the Absorber
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dave, V.; Sorathiya, V.; Guo, T.; Patel, S.K. Graphene based tunable broadband far-infrared absorber. Superlattices Microstruct. 2018, 124, 113–120. [Google Scholar] [CrossRef]
- Chen, J.; Tang, P.; Liu, G.; Yi, Z.; Liu, X.; Pan, P.; Liu, Z.-q. Si nano-cavity enabled surface-enhanced Raman scattering signal amplification. Nanotechnology 2019, 30, 465204. [Google Scholar] [CrossRef] [PubMed]
- Liu, C.; Wang, L.; Yang, L.; Wang, F.; Xu, C.; Lv, J.; Fu, G.; Li, X.; Liu, Q.; Mu, H. The single-polarization filter composed of gold-coated photonic crystal fiber. Phys. Lett. A 2019, 383, 3200–3206. [Google Scholar] [CrossRef]
- Chen, C.; Wang, G.; Zhang, Z.; Zhang, K. Dual narrow-band absorber based on metal–insulator–metal configuration for refractive index sensing. Opt. Lett. 2018, 43, 3630–3633. [Google Scholar] [CrossRef] [PubMed]
- Hao, J.; Wang, J.; Liu, X.; Padilla, W.J.; Zhou, L.; Qiu, M. High performance optical absorber based on a plasmonic metamaterial. Appl. Phys. Lett. 2010, 96, 251104. [Google Scholar] [CrossRef]
- Liu, X.; Starr, T.; Starr, A.F.; Padilla, W.J. Infrared spatial and frequency selective metamaterial with near-unity absorbance. Phys. Rev. Lett. 2010, 104, 207403. [Google Scholar] [CrossRef] [PubMed]
- Wang, P.; Zeng, X.; Wang, J. AlN film-based Fabry–Perot cavity resonance enhanced absorption. J. Nanophotonics 2021, 15, 016002. [Google Scholar] [CrossRef]
- Liu, G.; Chen, J.; Pan, P.; Liu, Z. Hybrid metal-semiconductor meta-surface based photo-electronic perfect absorber. IEEE J. Sel. Top. Quantum Electron. 2018, 25, 1–7. [Google Scholar] [CrossRef]
- Wang, W.; Zhao, Z.; Guo, C.; Guo, K.; Guo, Z. Spin-selected dual-wavelength plasmonic metalenses. Nanomaterials 2019, 9, 761. [Google Scholar] [CrossRef]
- Xu, H.; Hu, L.; Lu, Y.; Xu, J.; Chen, Y. Dual-Band Metamaterial Absorbers in the Visible and Near-Infrared Regions. J. Phys. Chem. C 2019, 123, 10028–10033. [Google Scholar] [CrossRef]
- Chen, J.; Tang, F.; Wang, X.; Wu, J.; Wu, Y.; Ye, X.; Wang, Y.; Yang, L. High efficiency broadband near-infrared absorbers based on tunable SiO2-VO2-MoS2 multilayer metamaterials. Results Phys. 2021, 26, 104404. [Google Scholar] [CrossRef]
- Hou, E.; Qin, Z.; Liang, Z.; Meng, D.; Shi, X.; Yang, F.; Liu, W.; Liu, H.; Xu, H.; Smith, D.R.; et al. Dual-band metamaterial absorber with a low-coherence composite cross structure in mid-wave and long-wave infrared bands. Opt. Express 2021, 29, 36145–36154. [Google Scholar] [CrossRef]
- Luo, X.; Zhou, Y.; Cai, Y.; Cheng, Z.; Liu, Z.; Wan, W. A review of perfect absorbers based on the two dimensional materials in the visible and near-infrared regimes. J. Phys. D Appl. Phys. 2021, 55, 093002. [Google Scholar] [CrossRef]
- Qiu, Y.; Wang, J.; Xiao, M.; Lang, T. Broadband terahertz metamaterial absorber: Design and fabrication. Appl. Opt. 2021, 60, 10055–10061. [Google Scholar] [CrossRef]
- Zhang, Z.; Yu, Z.; Liang, Y.; Xu, T. Dual-band nearly perfect absorber at visible frequencies. Opt. Mater. Express 2018, 8, 463–468. [Google Scholar] [CrossRef]
- Li, Z.; Shang, S.; Wu, H.; Liao, W.; Tang, F.; Wu, J.; Zeng, T.; Kong, B.; Ye, X.; Jiang, X.; et al. Optical edge-enhanced imaging based on dielectric metasurfaces. Opt. Mater. 2023, 143, 114206. [Google Scholar] [CrossRef]
- Cen, C.; Zhang, Y.; Chen, X.; Yang, H.; Yi, Z.; Yao, W.; Tang, Y.; Yi, Y.; Wang, J.; Wu, P. A dual-band metamaterial absorber for graphene surface plasmon resonance at terahertz frequency. Phys. E Low-Dimens. Syst. Nanostruct. 2020, 117, 113840. [Google Scholar] [CrossRef]
- Qin, F.; Chen, Z.; Chen, X.; Yi, Z.; Yao, W.; Duan, T.; Wu, P.; Yang, H.; Li, G.; Yi, Y. A tunable triple-band near-infrared metamaterial absorber based on Au nano-cuboids array. Nanomaterials 2020, 10, 207. [Google Scholar] [CrossRef] [PubMed]
- Gu, L.; Guo, X.; Fu, Z.; Wan, W.; Zhang, R.; Tan, Z.; Cao, J. Optical-phonon-mediated photocurrent in terahertz quantum-well photodetectors. Appl. Phys. Lett. 2015, 106, 111107. [Google Scholar] [CrossRef]
- Chen, J.; Nie, H.; Peng, C.; Qi, S.; Tang, C.; Zhang, Y.; Wang, L.; Park, G.-S. Enhancing the magnetic plasmon resonance of three-dimensional optical metamaterials via strong coupling for high-sensitivity sensing. J. Light. Technol. 2018, 36, 3481–3485. [Google Scholar] [CrossRef]
- Xu, B.; Li, H.; Gao, S.; Hua, X.; Yang, C.; Chen, C.; Yan, F.; Zhu, S.; Li, T. Metalens-integrated compact imaging devices for wide-field microscopy. Adv. Photonics 2020, 2, 066004. [Google Scholar] [CrossRef]
- Li, B.; Piyawattanametha, W.; Qiu, Z. Metalens-Based Miniaturized Optical Systems. Micromachines 2019, 10, 310. [Google Scholar] [CrossRef]
- Li, Z.; Tang, F.; Shang, S.; Wu, J.; Shao, J.; Liao, W.; Kong, B.; Zeng, T.; Ye, X.; Jiang, X.; et al. Compact metalens-based integrated imaging devices for near-infrared microscopy. Opt. Express 2021, 29, 27041–27047. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Liu, X.; Huang, S.; Pan, P.; Chen, J.; Liu, G.; Gu, G. Automatically acquired broadband plasmonic-metamaterial black absorber during the metallic film-formation. ACS Appl. Mater. Interfaces 2015, 7, 4962–4968. [Google Scholar] [CrossRef]
- Li, Z.; Stan, L.; Czaplewski, D.A.; Yang, X.; Gao, J. Wavelength-selective mid-infrared metamaterial absorbers with multiple tungsten cross resonators. Opt. Express 2018, 26, 5616–5631. [Google Scholar] [CrossRef] [PubMed]
- Kenney, M.; Grant, J.; Cumming, D.R. Alignment-insensitive bilayer THz metasurface absorbers exceeding 100% bandwidth. Opt. Express 2019, 27, 20886–20900. [Google Scholar] [CrossRef]
- Huang, X.; Yang, F.; Gao, B.; Yang, Q.; Wu, J.; He, W. Metamaterial absorber with independently tunable amplitude and frequency in the terahertz regime. Opt. Express 2019, 27, 25902–25911. [Google Scholar] [CrossRef] [PubMed]
- Katrodiya, D.; Jani, C.; Sorathiya, V.; Patel, S.K. Metasurface based broadband solar absorber. Opt. Mater. 2019, 89, 34–41. [Google Scholar] [CrossRef]
- Fan, Y. Dual-band perfect light absorber in visible region based on cylinder silicon resonator. Optik 2019, 179, 1084–1090. [Google Scholar] [CrossRef]
- Luo, Y.; Liang, Z.; Meng, D.; Tao, J.; Liang, J.; Chen, C.; Lai, J.; Qin, Y.; Jinguang, L.; Zhang, Y. Ultra-broadband and high absorbance metamaterial absorber in long wavelength Infrared based on hybridization of embedded cavity modes. Opt. Commun. 2019, 448, 1–9. [Google Scholar] [CrossRef]
- Yan, Z.; Lu, X.; Chen, K.; Lv, Z.; Pu, X.; Tang, C.; Cai, P. Ultranarrow Dual-Band Perfect Absorption in Visible and Near-infrared Regimes Based on Three-Dimensional Metamaterials for Ultrahigh-Sensitivity Sensing. J. Light. Technol. 2021, 39, 7217–7222. [Google Scholar] [CrossRef]
- Zhou, Y.; Liang, Z.; Qin, Z.; Hou, E.; Shi, X.; Zhang, Y.; Xiong, Y.; Tang, Y.; Fan, Y.; Yang, F.; et al. Small-sized long wavelength infrared absorber with perfect ultra-broadband absorptivity. Opt. Express 2020, 28, 1279–1290. [Google Scholar] [CrossRef] [PubMed]
- Qi, L.; Liu, C. Broadband multilayer graphene metamaterial absorbers. Opt. Mater. Express 2019, 9, 1298–1309. [Google Scholar] [CrossRef]
- Cao, G.; Li, H.; Deng, Y.; Zhan, S.; He, Z.; Li, B. Systematic theoretical analysis of selective-mode plasmonic filter based on aperture-side-coupled slot cavity. Plasmonics 2014, 9, 1163–1169. [Google Scholar] [CrossRef]
- Shangguan, Q.; Chen, H.; Yang, H.; Liang, S.; Zhang, Y.; Cheng, S.; Yang, W.; Yi, Z.; Luo, Y.; Wu, P. A “belfry-typed” narrow-band tunable perfect absorber based on graphene and the application potential research. Diam. Relat. Mater. 2022, 125, 108973. [Google Scholar] [CrossRef]
- Meng, H.; Wang, L.; Liu, G.; Xue, X.; Lin, Q.; Zhai, X. Tunable graphene-based plasmonic multispectral and narrowband perfect metamaterial absorbers at the mid-infrared region. Appl. Opt. 2017, 56, 6022–6027. [Google Scholar] [CrossRef] [PubMed]
- Liu, F.; Zou, M.; Feng, Z.; Ni, B.; Ye, B.; Wang, Y. All-Dielectric Dual-Band Metamaterial Absorber Based on Ring Nanocavity in Visible Region for Sensing Applications. Photonics 2023, 10, 58. [Google Scholar] [CrossRef]
- Meng, Q.; Chen, F.; Cheng, S.; Xu, Y.; Yang, W.; Yi, Z. Near perfect and polarization insensitive broadband absorber based on Al2O3-Ti-Al2O3 triple layer structure. Phys. Scr. 2023, 98, 025511. [Google Scholar] [CrossRef]
- Houran, M.A.; Baqir, M.A.; Saqlain, M.; Dhasarathan, V. Polarization-insensitive and wide-angle absorber operating in the visible and near-infrared regimes. Optik 2023, 283, 170915. [Google Scholar] [CrossRef]
- Guan, Z.; Liu, Y.; Li, Y.; Zhao, P.; Zhang, Y.; Jiang, S. Dual-Band Terahertz Perfect Absorber Based on Metal Micro-Nano Structure. Coatings 2022, 12, 687. [Google Scholar] [CrossRef]
- Liu, Z.; Liu, G.; Huang, Z.; Liu, X.; Fu, G. Ultra-broadband perfect solar absorber by an ultra-thin refractory titanium nitride meta-surface. Sol. Energy Mater. Sol. Cells 2018, 179, 346–352. [Google Scholar] [CrossRef]
- Sayed, S.I.; Mahmoud, K.R.; Mubarak, R.I. Design and optimization of broadband metamaterial absorber based on manganese for visible applications. Sci. Rep. 2023, 13, 11937. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xiong, Z.; Li, Z.; He, G.; Su, K.; Huang, Y.; Deng, G. Polarization-Angle-Insensitive Dual-Band Perfect Metamaterial Absorbers in the Visible Region: A Theoretical Study. Coatings 2024, 14, 236. https://doi.org/10.3390/coatings14020236
Xiong Z, Li Z, He G, Su K, Huang Y, Deng G. Polarization-Angle-Insensitive Dual-Band Perfect Metamaterial Absorbers in the Visible Region: A Theoretical Study. Coatings. 2024; 14(2):236. https://doi.org/10.3390/coatings14020236
Chicago/Turabian StyleXiong, Zhihui, Zhixi Li, Guangqiang He, Kecheng Su, Yien Huang, and Guowei Deng. 2024. "Polarization-Angle-Insensitive Dual-Band Perfect Metamaterial Absorbers in the Visible Region: A Theoretical Study" Coatings 14, no. 2: 236. https://doi.org/10.3390/coatings14020236
APA StyleXiong, Z., Li, Z., He, G., Su, K., Huang, Y., & Deng, G. (2024). Polarization-Angle-Insensitive Dual-Band Perfect Metamaterial Absorbers in the Visible Region: A Theoretical Study. Coatings, 14(2), 236. https://doi.org/10.3390/coatings14020236





