Analytical Investigation on the Dynamic Behavior of Multi-Span Continuous Beams Supported on Soil with Finite Depth
Abstract
:1. Introduction
2. Basic Relationships
3. Analytical Solution of the Continuous Beam Vibration
4. Numerical Tests and Model Validation
4.1. Simply Supported Beam
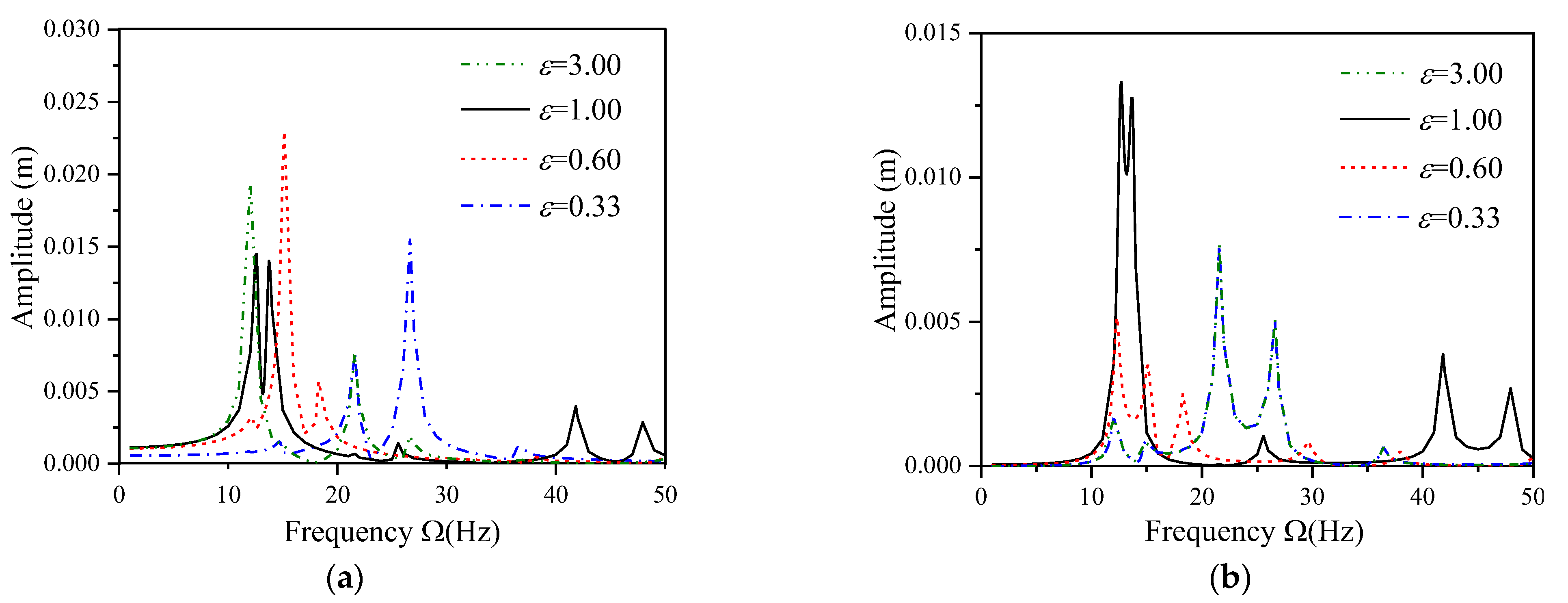
4.2. Two-Span Continuous Beam
5. Results and Discussion
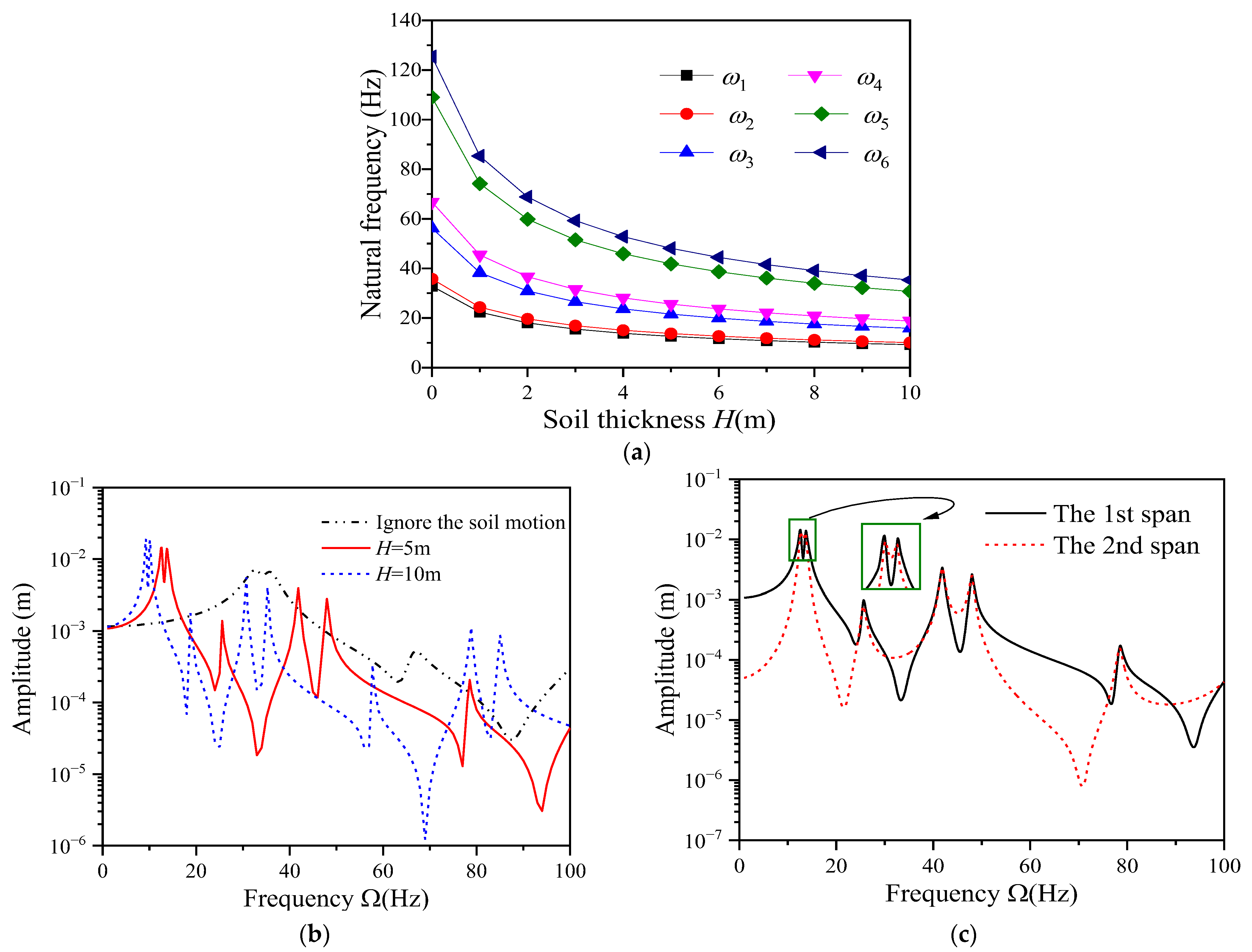
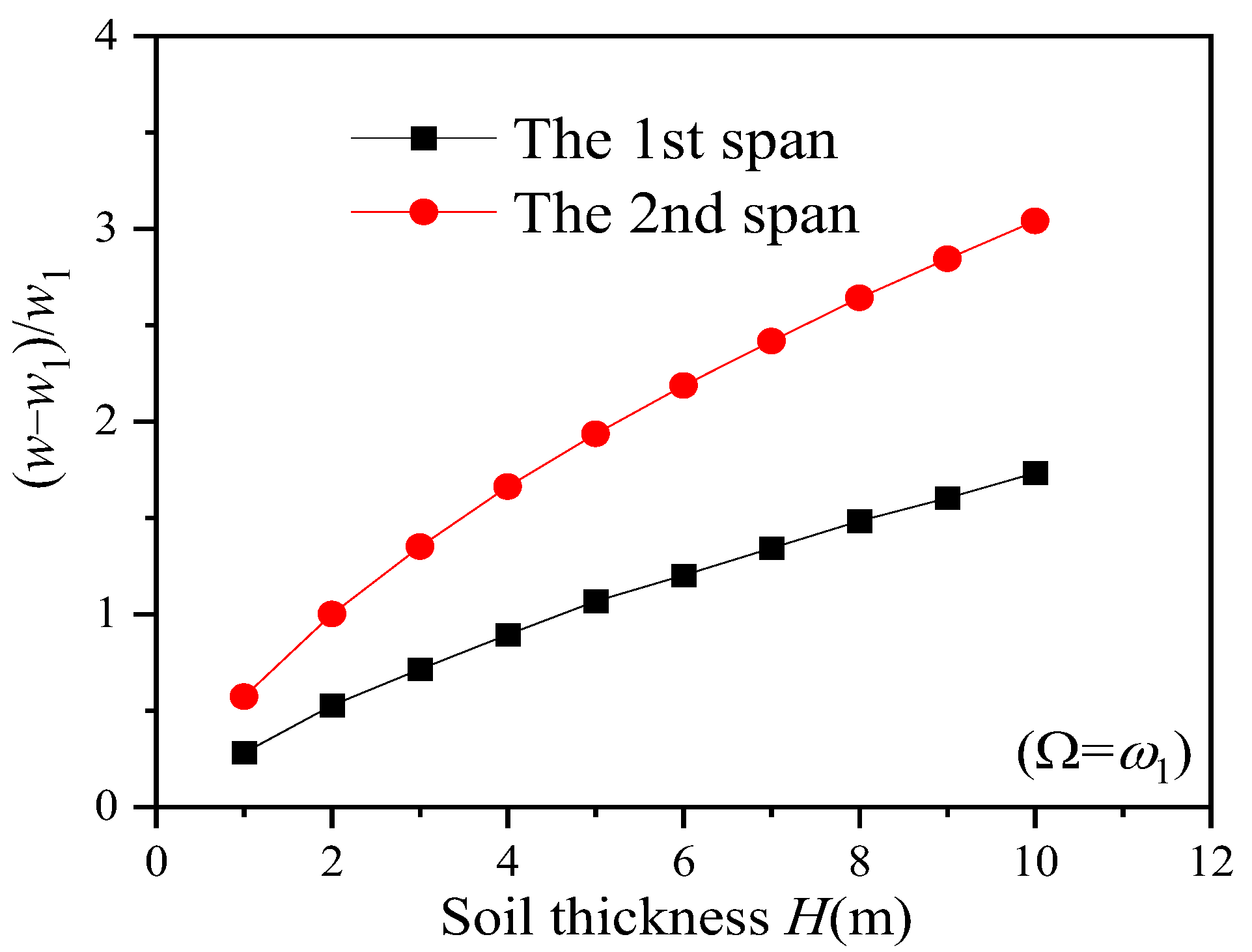
5.1. Effect of Soil Thickness
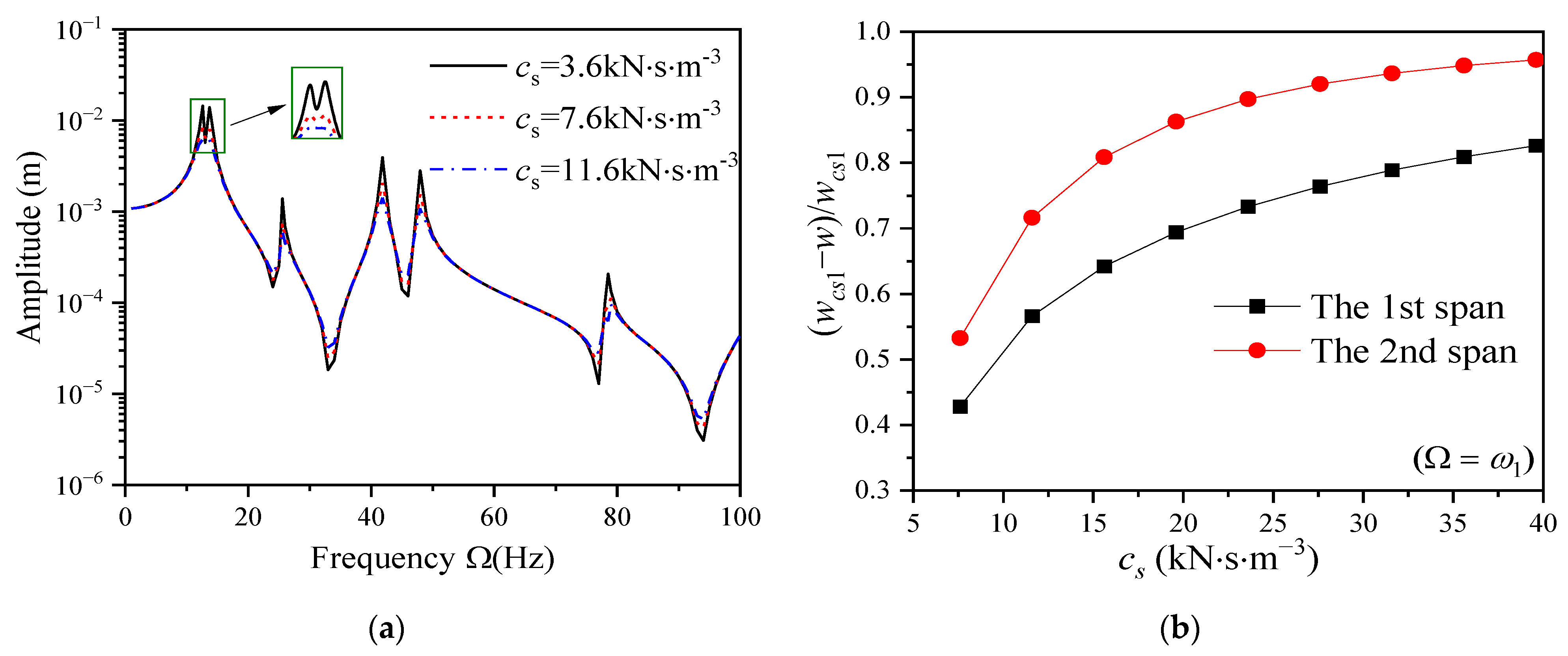
5.2. Effect of the Viscous Damping Coefficient of the Soil
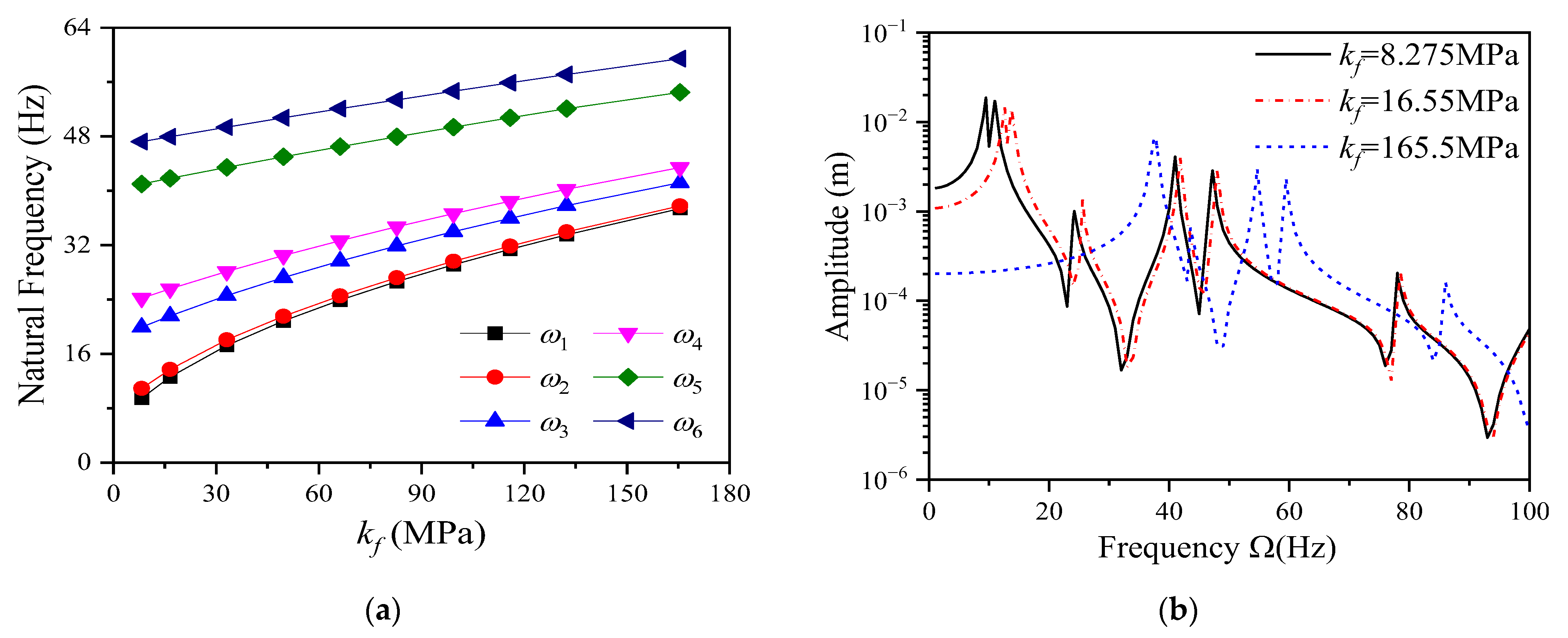
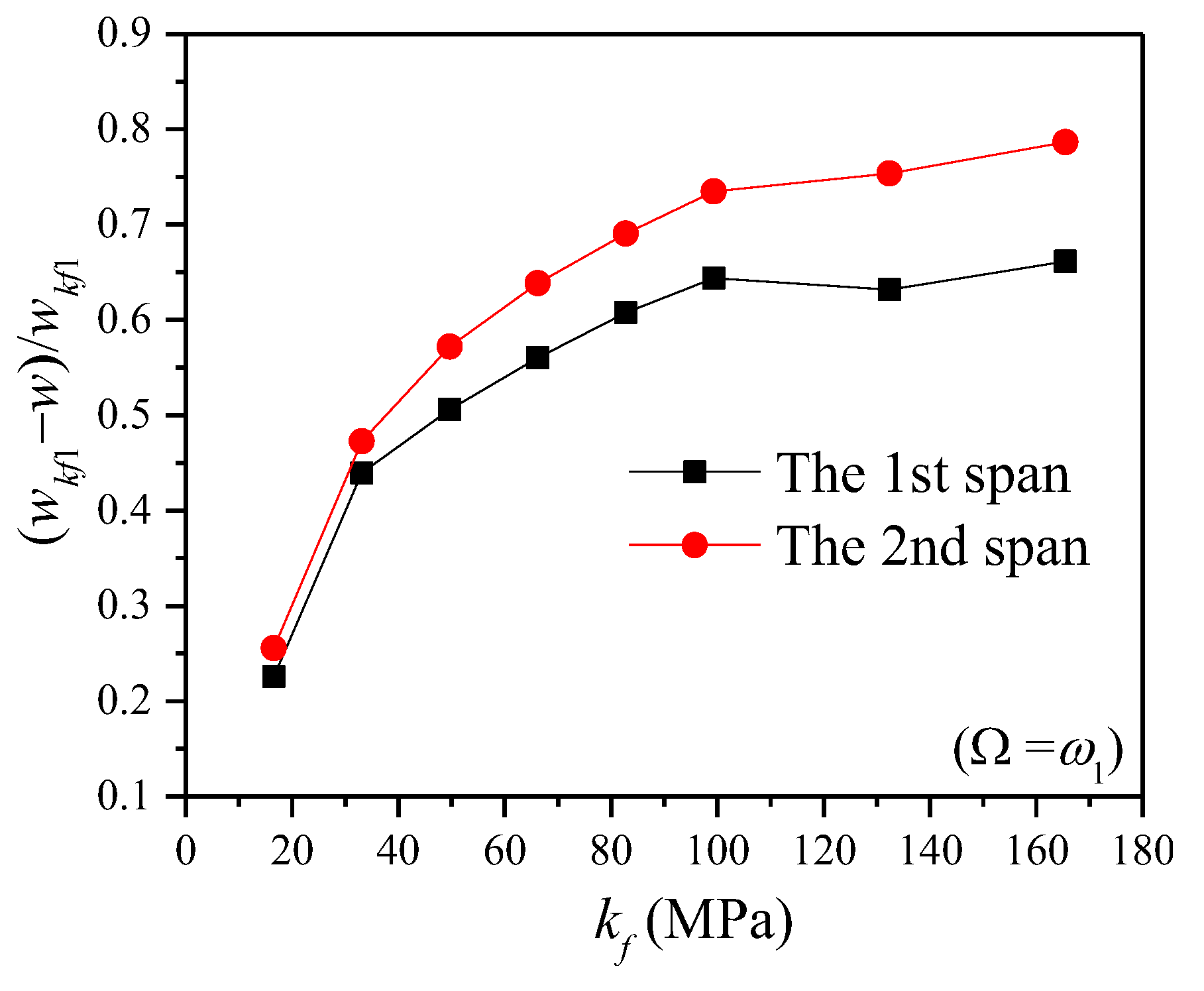
5.3. Effect of Subgrade Reaction Coefficient
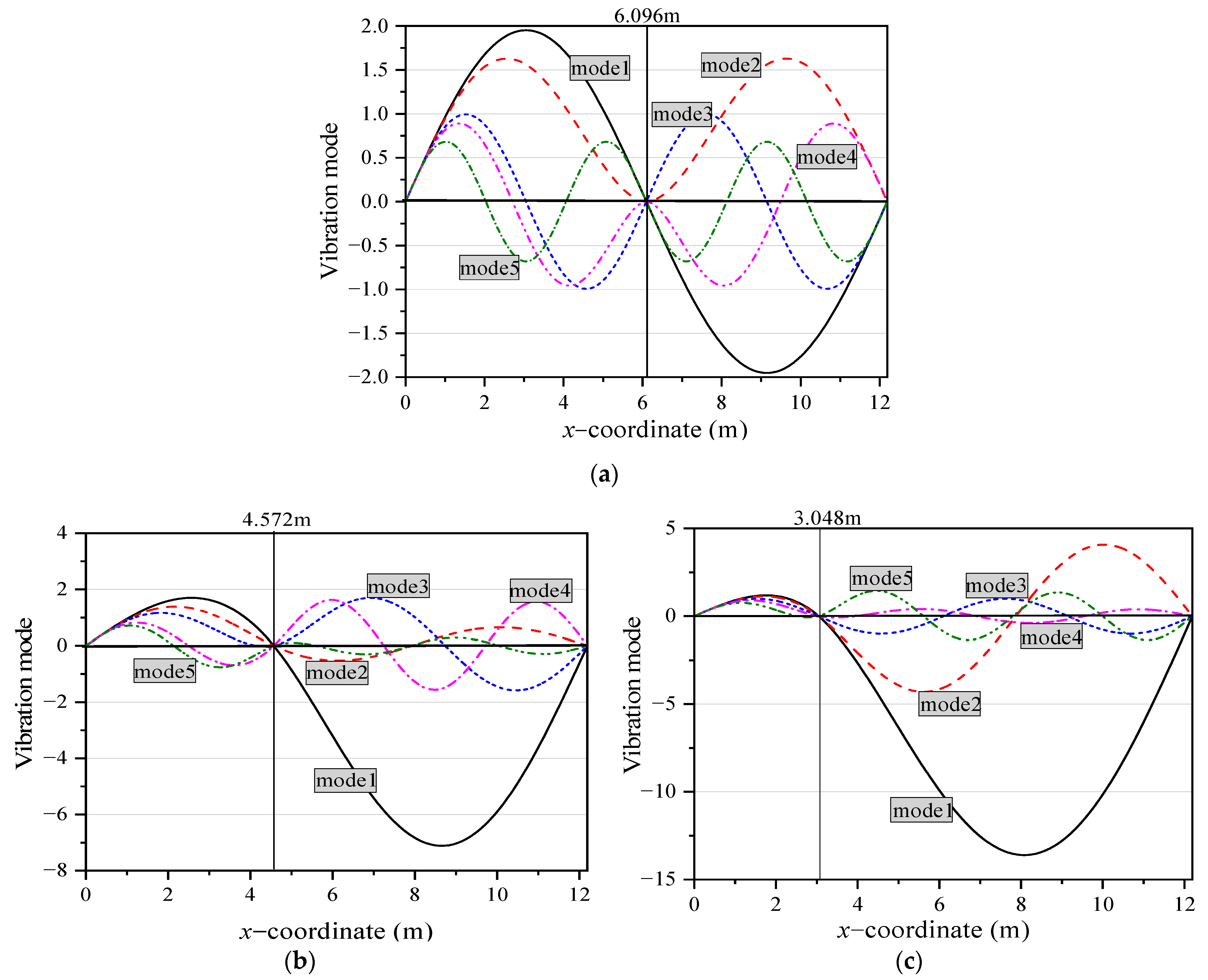
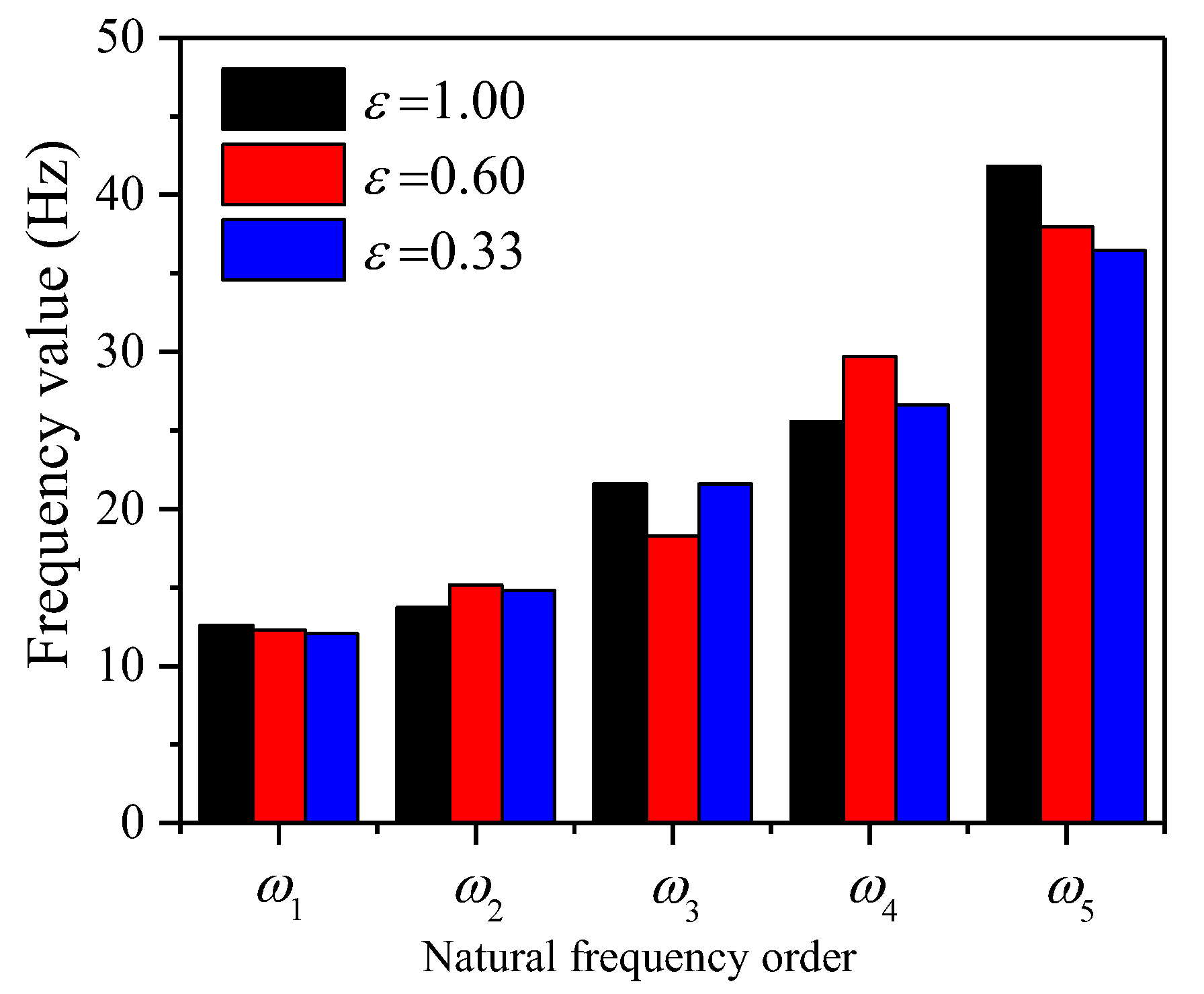
5.4. Effect of Span Ratio
6. Conclusions
- As the thickness of the soil involved in the movement increases, the continuous beam’s natural frequency will decrease, and the resonance amplitude increases significantly. When comparing the dynamic responses of different beams, the enhancement impact of finite depth soil motion on the response amplitude of the indirect excitation beam section is more significant than that of the direct excitation beam section.
- The viscous damping coefficient of the soil and the coefficient of subgrade reaction have a greater inhibition effect on the indirect influence span than on the direct excitation span. As the coefficient of the subgrade reaction increases, the system’s natural frequency increases, and the resonant response amplitude decreases.
- The adjustment of the span ratio has a significant influence on the dynamic response of the multi-span beam system. (1) Compared with other span ratios, the odd-order natural frequency is the largest and the even-order natural frequency is the smallest when . (2) The smaller the span ratio is, the more pronounced the increasing effect of the resonant frequency is. (3) When the span ratio is determined, no matter whether the load acts on the long span or the short span, the response amplitude of the beam span indirectly affected by the load is certain.
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Hayashikawa, T.; Watanabe, N. Free vibration analysis of continuous beams. J. Eng. Mech. 1985, 111, 639–652. [Google Scholar] [CrossRef]
- Busool, W.; Eisenberger, M. Vibrations of axially loaded continuous beams. Int. J. Struct. Stab. Dyn. 2002, 2, 117–133. [Google Scholar] [CrossRef]
- Lee, J. Eigenvalue analysis of double-span Timoshenko beams by pseudospectral method. J. Mech. Sci. Technol. 2005, 19, 1753–1760. [Google Scholar] [CrossRef]
- Tullini, N.; Tralli, A.; Baraldi, D. Stability of slender beams and frames resting on 2D elastic half-space. Arch. Appl. Mech. 2013, 83, 467–482. [Google Scholar] [CrossRef]
- Seetapan, P.; Chucheepsakul, S. Dynamic responses of a two-span beam subjected to high speed 2DOF sprung vehicles. Int. J. Struct. Stab. Dyn. 2020, 6, 413–430. [Google Scholar] [CrossRef]
- Wang, Y.J.; Wei, Q.C.; Yau, J.D. Interaction response of train loads moving over a two-span continuous beam. J. Struct. Stab. Dyn. 2013, 13, 1350002. [Google Scholar] [CrossRef]
- Wang, L.; Kang, X.; Jiang, P. Vibration analysis of a multi-span continuous bridge subject to complex traffic loading and vehicle dynamic interaction. KSCE J. Civ. Eng. 2016, 20, 323–332. [Google Scholar] [CrossRef]
- Tamaddon, S.; Hosseini, M.; Vasseghi, A. Effect of non-uniform vertical excitations on vertical pounding phenomenon in continuous-deck curved box girder RC bridges subjected to near-source earthquakes. J. Earthq. Eng. 2022, 26, 5360–5383. [Google Scholar] [CrossRef]
- Lee, S.M. Analysis of center frequency effect on damping parameters estimation using continuous wavelet transform. KSCE J. Civ. Eng. 2021, 25, 1399–1409. [Google Scholar] [CrossRef]
- Timoshenko, S. Vibration Problems in Engineering; Wiley: New York, NY, USA, 1974; pp. 309–312. [Google Scholar]
- Deng, H.Y.; Jin, X.Y.; Gu, M. A simplified method for evaluating the dynamic properties of structures considering soil–pile–structure interaction. Int. J. Struct. Stab. Dyn. 2018, 18, 1871005. [Google Scholar] [CrossRef]
- Bao, T.; Liu, Z. Evaluation of Winkler model and Pasternak model for dynamic soil-structure interaction analysis of structures partially embedded in soils. Int. J. Geomech. 2020, 20, 04019167. [Google Scholar] [CrossRef]
- Asadijafari, M.H.; Zarastvand, M.R.; Talebitooti, R. The effect of considering Pasternak elastic foundation on acoustic insulation of the finite doubly curved composite structures. Compos. Struct. 2021, 256, 113064. [Google Scholar] [CrossRef]
- Smith, J.; Brown, A. Analysis of Continuous Beams in Modern Bridge Construction. J. Struct. Eng. 2022, 148, 567–580. [Google Scholar] [CrossRef]
- Lee, C.; Kim, D. Vibration Analysis of Continuous Beams on Elastic Foundations. Int. J. Civ. Eng. 2021, 19, 345–356. [Google Scholar]
- Zhang, X.; Li, Y. Case Studies on the Performance of Continuous Beams Supported by Soil. Geotech. Eng. J. 2023, 54, 210–225. [Google Scholar]
- Miller, R.; Thompson, P. Structural Behavior of Continuous Beams Under Varying Soil Conditions. Civ. Eng. Res. 2020, 77, 92–108. [Google Scholar]
- Rades, M. Dynamic analysis of an inertial foundation model. Int. J. Solids Struct. 1972, 8, 1353–1372. [Google Scholar] [CrossRef]
- Jaiswal, O.R.; Iyengar, R.N. Dynamic response of a beam on elastic foundation of finite depth under a moving force. Acta Mech. 1993, 96, 67–83. [Google Scholar] [CrossRef]
- Metrikine, A.V.; Popp, K. Steady-state vibrations of an elastic beam on a visco-elastic layer under moving load. Arch. Appl. Mech. 2000, 70, 399–408. [Google Scholar] [CrossRef]
- Ma, J.J.; Liu, F.J.; Nie, M.Q.; Wang, J.B. Nonlinear free vibration of a beam on Winkler foundation with consideration of soil mass motion of finite depth. Nonlinear Dyn. 2018, 92, 429–441. [Google Scholar] [CrossRef]
- Chen, G.L.; Zeng, X.Y.; Liu, X.B.; Rui, X.T. Transfer matrix method for the free and forced vibration analyses of multi-step Timoshenko beams coupled with rigid bodies on springs. Appl. Math. Model. 2020, 87, 152–170. [Google Scholar] [CrossRef]
- Wang, Q.F.; Wang, L.Y.; Liu, Q.S. Seismic response of stepped frame-shear wall structures by using numerical method. Comput. Method. Appl. Mech. Eng. 1999, 173, 31–39. [Google Scholar] [CrossRef]
- Vallabhan, C.V.G.; Das, Y.C. Parametric study of beams on elastic foundation. J. Eng. Mech. 1988, 114, 2072–2082. [Google Scholar] [CrossRef]
- Rui, X.T.; Zhang, J.S.; Zhou, Q.B. Automatic deduction theorem of overall transfer equation of multibody system. Adv. Mech. Eng. 2014, 6, 378047. [Google Scholar] [CrossRef]
- Thambiratnam, D.; Zhuge, Y. Free vibration analysis of beams on elastic foundation. Comput. Struct. 1996, 60, 971–980. [Google Scholar] [CrossRef]
- Friswell, M.I.; Adhikari, S.; Lei, Y. Vibration analysis of beams with non-local foundations using the finite element method. Int. J. Numer. Meth. Eng. 2007, 71, 1365–1386. [Google Scholar] [CrossRef]
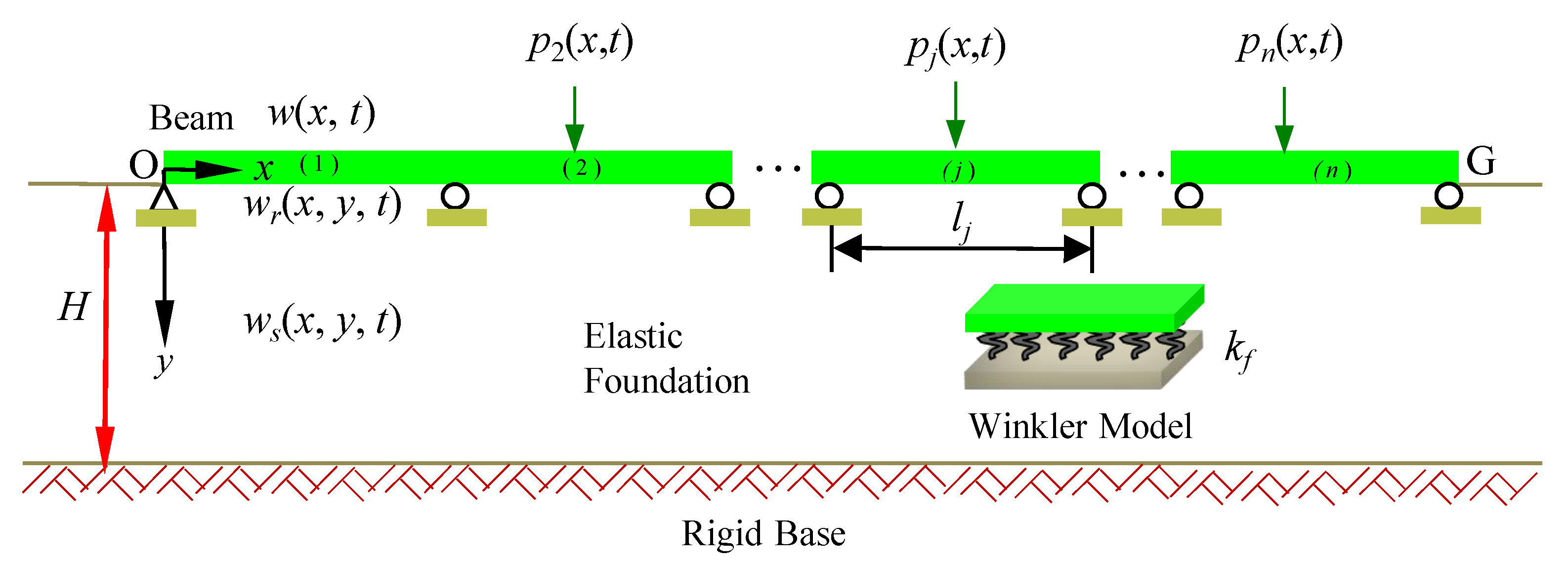


| Physical Meaning | Symbol | Unit | Numerical Value |
|---|---|---|---|
| The jth span length of a continuous beam | 6.096 | ||
| The beam’s width | 0.61 | ||
| The beam’s height | 0.305 | ||
| The per unit length beam’s mass | 447.08 | ||
| Damping factor per unit length | 1.0 | ||
| Moment of inertia of the per unit length beam | 3.466 | ||
| Young’s modulus of the beam | 2.482 × 104 | ||
| Poisson ratio of the beam | —— | 0.25 | |
| Soil mass per unit thickness of the per unit length beam | 1037 | ||
| Viscous damping coefficient of the soil per unit thickness of the per unit length beam | 3.6 | ||
| Modulus of subgrade reaction | 16.55 | ||
| Soil thickness | 5 |
| Natural Frequency (Hz) | (Timoshenko S., 1974) [10] | (Thambiratnam and Zhuge, 1996) [26] | (Friswell et al., 2007) [27] | The Present Study | |
|---|---|---|---|---|---|
| Euler-Bernoulli | Timoshenko | ||||
| 32.9063 | 32.9033 | 32.898 | 32.8749 | 32.8289 | |
| 56.8135 | 56.8193 | 56.808 | 56.8040 | 56.1037 | |
| 112.908 | 111.961 | 111.900 | 111.9186 | 108.303 | |
| 193.760 | 193.8085 | 182.7608 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, D.; Yang, H.; Ma, J.; Wang, J.; Wang, C.; Guo, Y. Analytical Investigation on the Dynamic Behavior of Multi-Span Continuous Beams Supported on Soil with Finite Depth. Coatings 2024, 14, 864. https://doi.org/10.3390/coatings14070864
Li D, Yang H, Ma J, Wang J, Wang C, Guo Y. Analytical Investigation on the Dynamic Behavior of Multi-Span Continuous Beams Supported on Soil with Finite Depth. Coatings. 2024; 14(7):864. https://doi.org/10.3390/coatings14070864
Chicago/Turabian StyleLi, Da, Hang Yang, Jianjun Ma, Ju Wang, Chaosheng Wang, and Ying Guo. 2024. "Analytical Investigation on the Dynamic Behavior of Multi-Span Continuous Beams Supported on Soil with Finite Depth" Coatings 14, no. 7: 864. https://doi.org/10.3390/coatings14070864
APA StyleLi, D., Yang, H., Ma, J., Wang, J., Wang, C., & Guo, Y. (2024). Analytical Investigation on the Dynamic Behavior of Multi-Span Continuous Beams Supported on Soil with Finite Depth. Coatings, 14(7), 864. https://doi.org/10.3390/coatings14070864






