Study on Friction and Lubrication Characteristics of Surface with Unidirectional Convergence Texture
Abstract
:1. Introduction
2. Experimental Methods
2.1. Design and Fabrication of Triangular Micro-Texture
2.2. Design and Fabrication of Triangular Groove Micro-Texture
2.3. Design and Fabrication of Semicircular Ring Micro-Texture
3. Fluid Simulation Analysis of Micro-Texture
3.1. Standard k-ε Model
3.2. Determination of Boundary Conditions
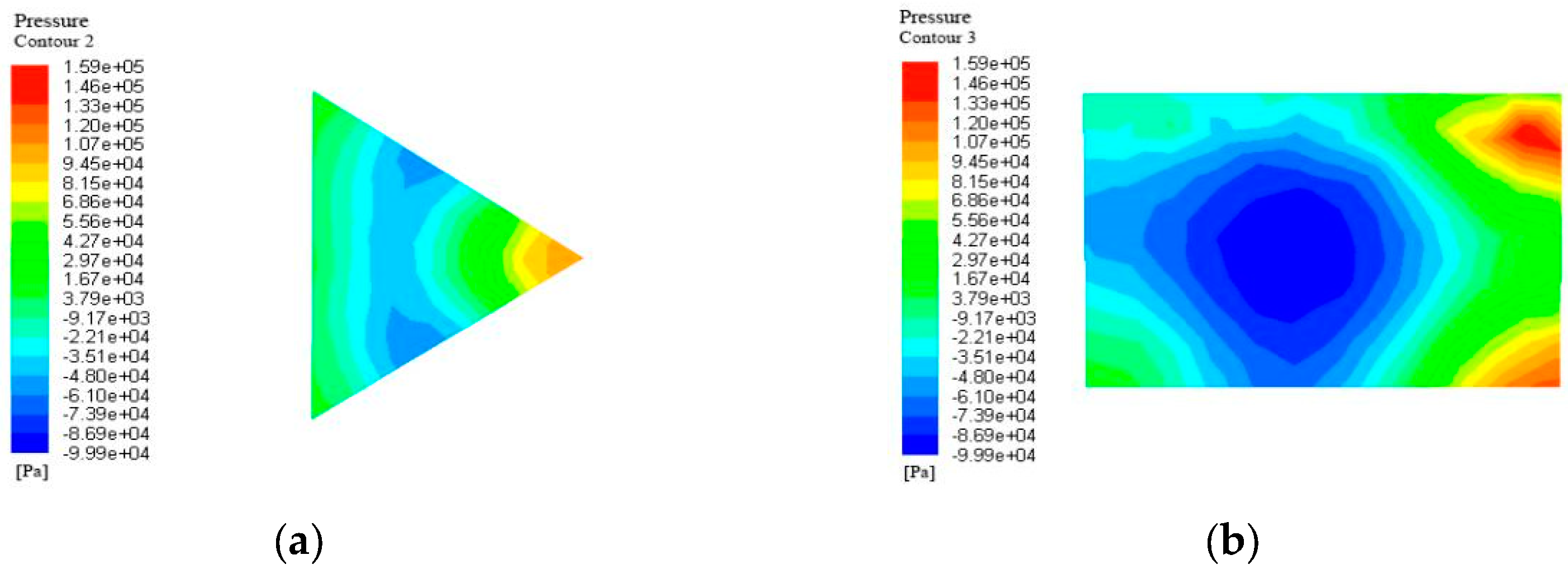
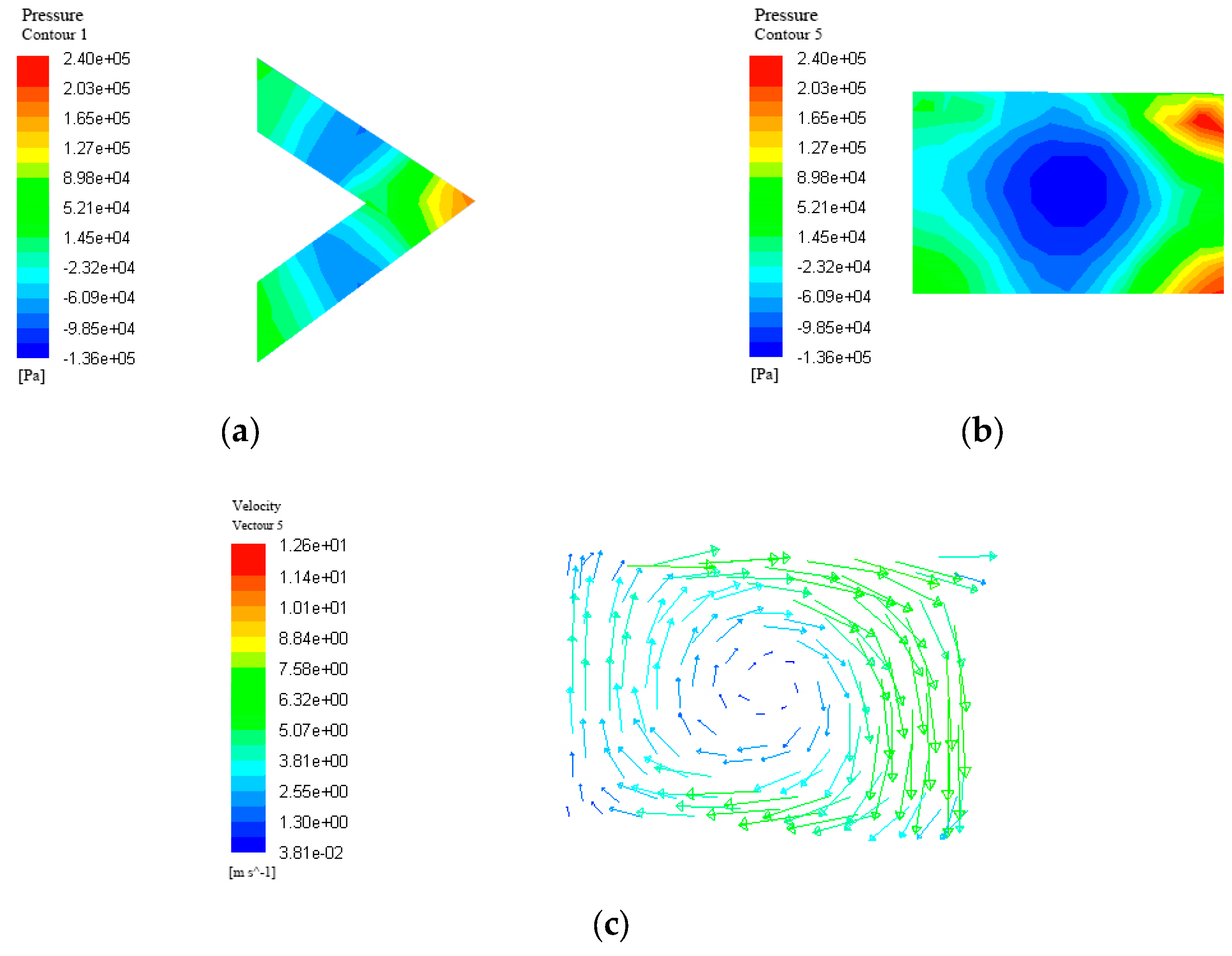
3.3. Pressure and Velocity Distribution of Fluid in Textures with Different Morphologies
4. Friction and Wear Test of Textured Surface
4.1. Friction and Wear Test Equipment
4.2. Friction Coefficients of Unpolished Sample and Polished Sample
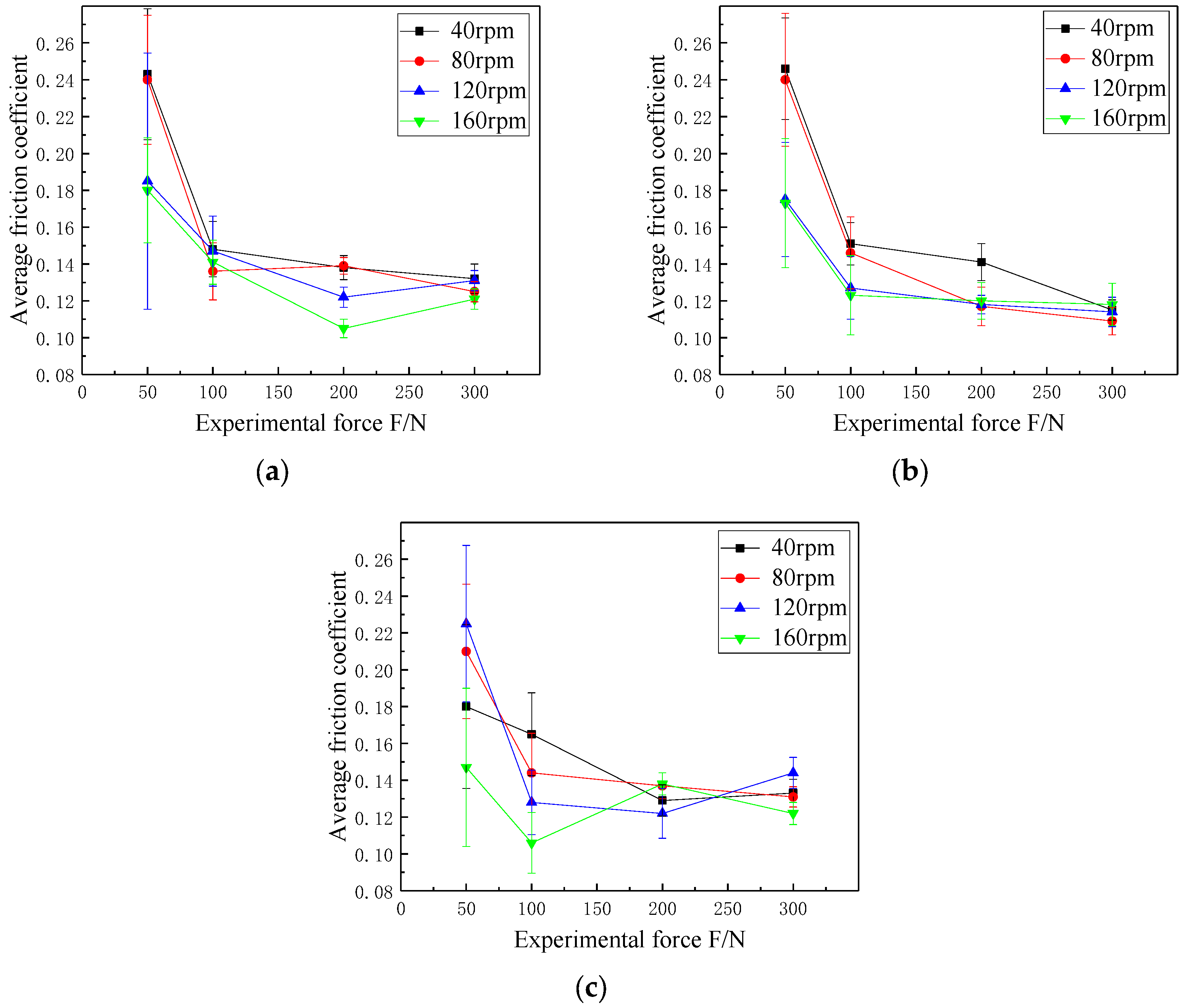
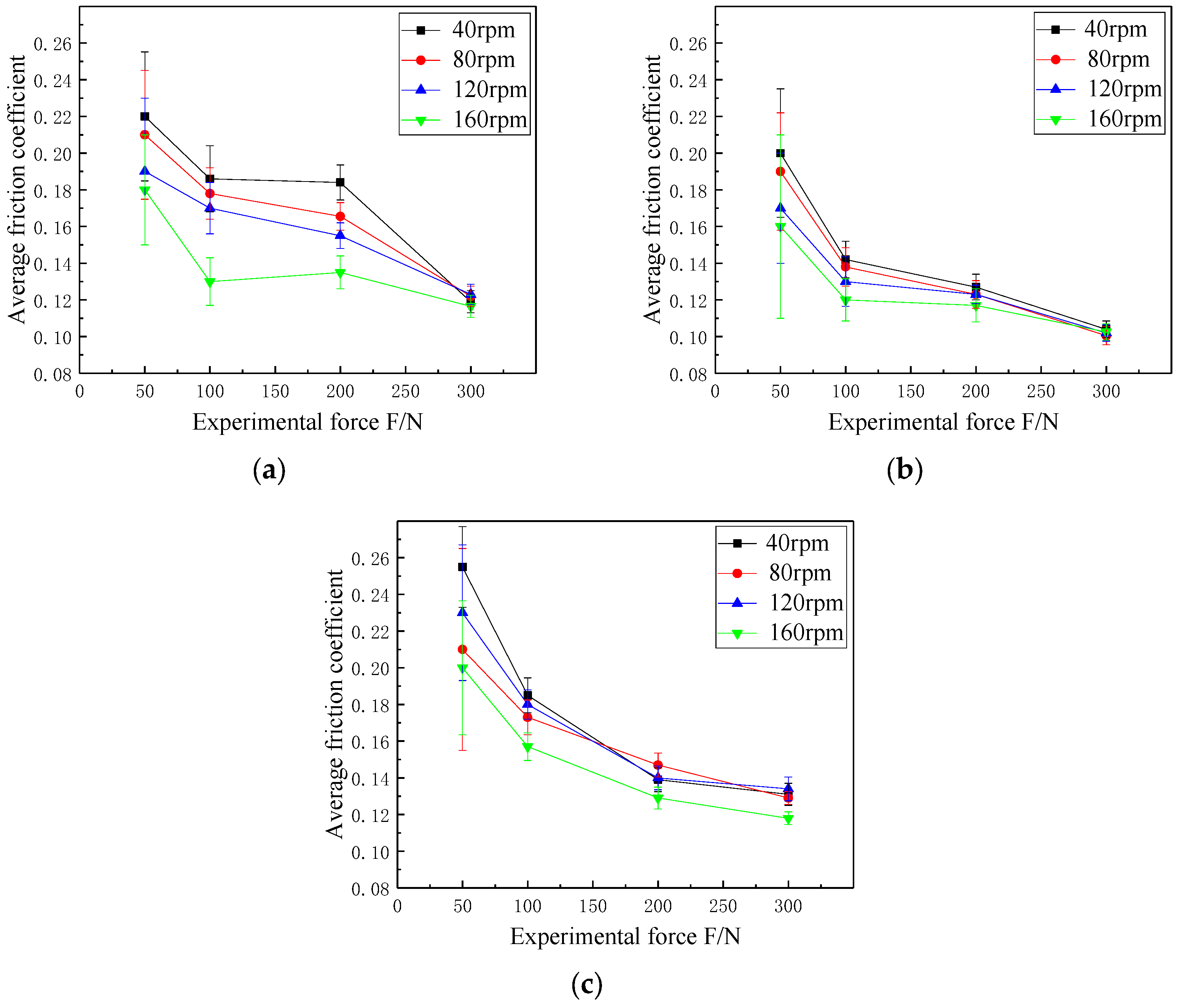
4.3. Friction Coefficients of Textured Surface
4.3.1. Friction Coefficients of Triangular Microtextures
4.3.2. Friction Coefficients of Triangular Groove Microtextures
4.3.3. Friction Coefficients of Semicircular Ring Microtextures
4.4. Effect of Area Occupancy Ratio on Friction Coefficient of Textured Surface
4.5. Effect of Texture Morphology on Friction Coefficient of Textured Surface
4.6. Wear Volume of Non-Textured Surface and Textured Surface
5. Analysis of Wear Mechanism of Non-textured Specimen and Textured Specimens
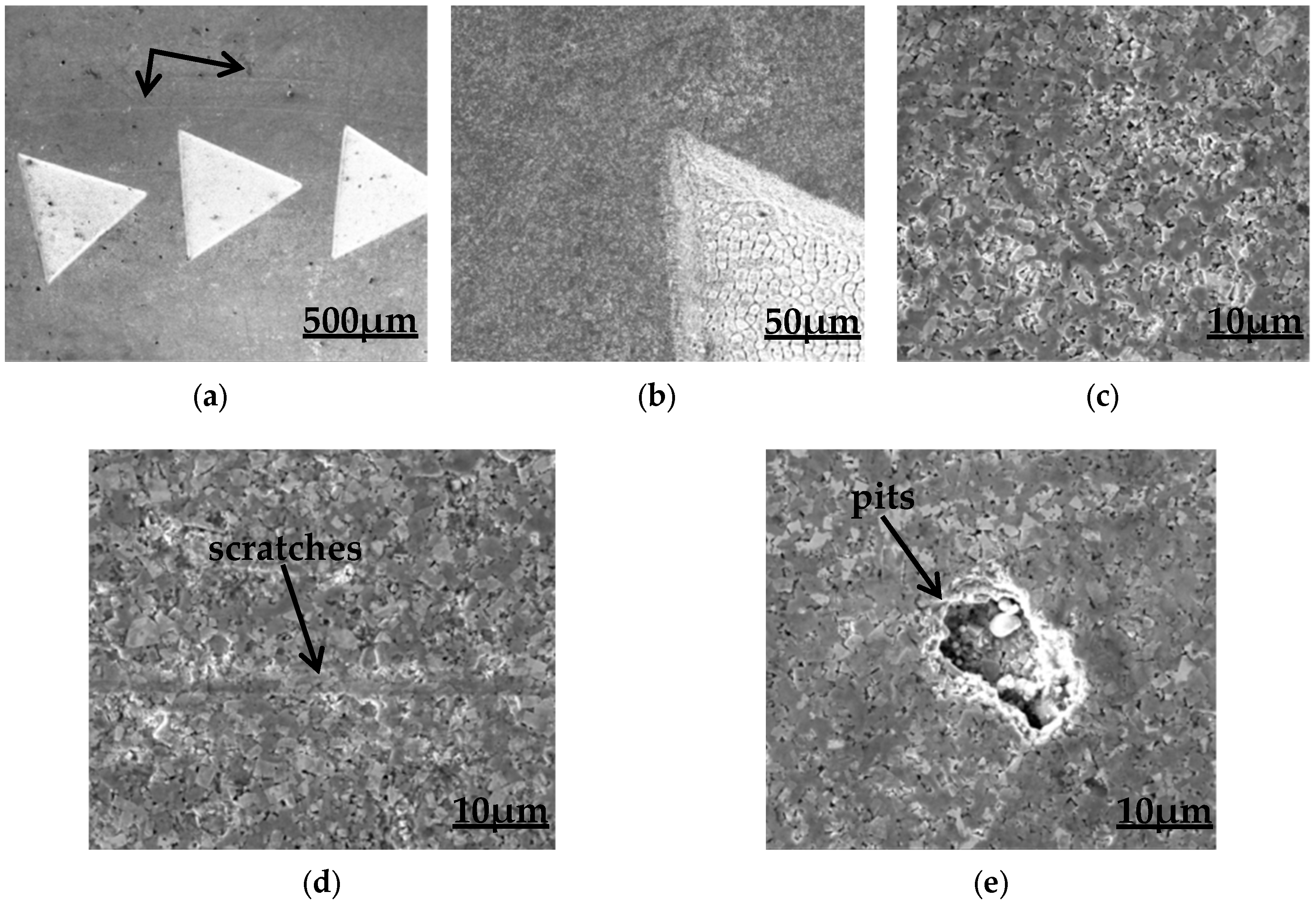
5.1. Analysis of Wear Mechanism of the Non-Textured Polished Specimen
5.2. Analysis of Wear Mechanism of the Textured Specimens
5.2.1. Surface Wear Morphology of Triangular Textured Specimens
5.2.2. Surface Wear Morphology of Triangular Groove Textured Specimens
5.2.3. Surface Wear Morphology of Semicircular Ring Textured Specimens
6. Conclusions
- The pressure and velocity distribution of fluid in different micro-textures were analyzed by Fluent fluid simulation. The result shows that the surface lubrication film of semicircular ring micro-textured specimens has the best bearing capacity, and the dynamic pressure lubrication effect is the most prominent.
- Comparing the friction coefficient of the surface of three textured specimens, the friction coefficient decreases with the increase of the experimental force at a certain rotational speed and becomes more and more stable. When the experimental force is 300 N, the friction coefficient is the lowest and most stable.
- When the area occupancy ratio of the three textures is about 10%, three types of textured specimens have the lowest surface friction coefficient, and the semicircular ring textured specimens have lower surface friction coefficients than the triangular and triangular groove.
- The wear forms of the non-textured polished specimen mainly include abrasive wear and contact fatigue wear generated under alternating contact stress. The wear forms of the surface of the triangular, triangular groove, and semicircular ring textured specimens are mainly abrasive wear and more or less adhesive wear, and cavitation pits are observed on the surface of the triangular groove and semicircular ring specimens.
Author Contributions
Funding
Conflicts of Interest
References
- Jianfei, S.; Shun, H.; Haitao, D.; Wuyi, C. Cutting performance and wear mechanism of Sialon ceramic tools in high speed face milling GH4099. Ceram. Int. 2019. [Google Scholar] [CrossRef]
- Datta, A.; Dutta, S.; Pal, S.K.; Sen, R. Progressive cutting tool wear detection from machined surfaceimages using Voronoi tessellation method. J. Mater. Proc. Technol. 2013, 213, 2339–2349. [Google Scholar] [CrossRef]
- Xuewei, Z.; Tianbiao, Y.; Ji, Z. Surface generation modeling of micro milling process with stochastic tool wear. Precis. Eng. 2019. [Google Scholar] [CrossRef]
- JingHui, Z.; LiZhi, Z.; Yuying, Y.; Jie, Z. Mechanical durability of superhydrophobic surfaces: The role of surface modification technologies. Appl. Surf. Sci. 2017, 392, 286–296. [Google Scholar] [CrossRef]
- Shota, I.; Kenta, T.; Shinya, S. Generation mechanism of friction anisotropy by surface texturing under boundary lubrication. Tribol. Int. 2019. [Google Scholar] [CrossRef]
- Boidi, G.; Tertuliano, I.S.; Profito, F.J.; de Rossi, W.; Machado, I.F. Effect of laser surface texturing on friction behaviour in elastohydrodynamically lubricated point contacts under different sliding-rolling conditions. Tribol. Int. 2019. [Google Scholar] [CrossRef]
- Hongzhi, Y.; Jianxin, D.; Dongliang, G.; Xuemu, L.; Yun, Z. Effect of surface texturing on tribological performance of sliding guideway under boundary lubrication. J. Manuf. Process. 2019, 47, 172–182. [Google Scholar] [CrossRef]
- Joaquin, A.; Jon-Emile, S.K.; Frederik, R.B.; Daniel, S.J.P. Dynamic prediction of fluid responsiveness during positive pressure ventilation: a review of the physiology underlying heart–lung interactions and a critical interpretation. Vet. Anaesth. Analg. 2019. [Google Scholar] [CrossRef]
- Weili, L.; Hongjian, N.; Hailong, C.; Peng, W. Numerical simulation and experimental investigation on tribological performance of micro-dimples textured surface under hydrodynamic lubrication. Int. J. Mech. Sci. 2019, 163. [Google Scholar] [CrossRef]
- Gachot, C.; Rosenkranz, A.; Hsu, S.M.; Costa, H.L. A critical assessment of surface texturing for friction and wear improvement. Wear. 2017, 372–373, 21–41. [Google Scholar] [CrossRef]
- Zeeshan, U.R.; Bon, H.K. Effect of Na2SiO.5H2O concentration on the secondary step coating produced on AZ91 magnesium alloy in the K2ZrF6-based el3ectrolyte solution. Surf. Coat. Technol. 2017, 317, 117–124. [Google Scholar]
- Faiz, M.; Mertcan, K.; Grzegorz, C.; Bora, D.; Adam, K.; Erdem, A.; Huseyin, C. Influence of alumina and zirconia incorporations on the structure and wear resistance of titania-based MAO coatings. Surf. Coat. Technol. 2019, 377. [Google Scholar] [CrossRef]
- Aliofkhazraei, M.; Sabour Rouhaghdam, A. Wear and coating removal mechanism of alumina/titania nanocomposite layer fabricated by plasma electrolysis. Surf. Coat. Technol. 2011, 205, S57–S62. [Google Scholar] [CrossRef]
- Shinkarenko, A.; Kligerman, Y.; Etsion, I. The effect of surface texturing in soft elasto-hydrodynamic lubrication. Tribol. Int. 2009, 42, 284–292. [Google Scholar] [CrossRef]
- Edeling, W.N.; Cinnella, P.; Dwight, R.P.; Bijl, H. Bayesian estimates of parameter variability in the k-ε turbulence model. J. Comput. Phys. 2014, 258, 73–94. [Google Scholar] [CrossRef] [Green Version]
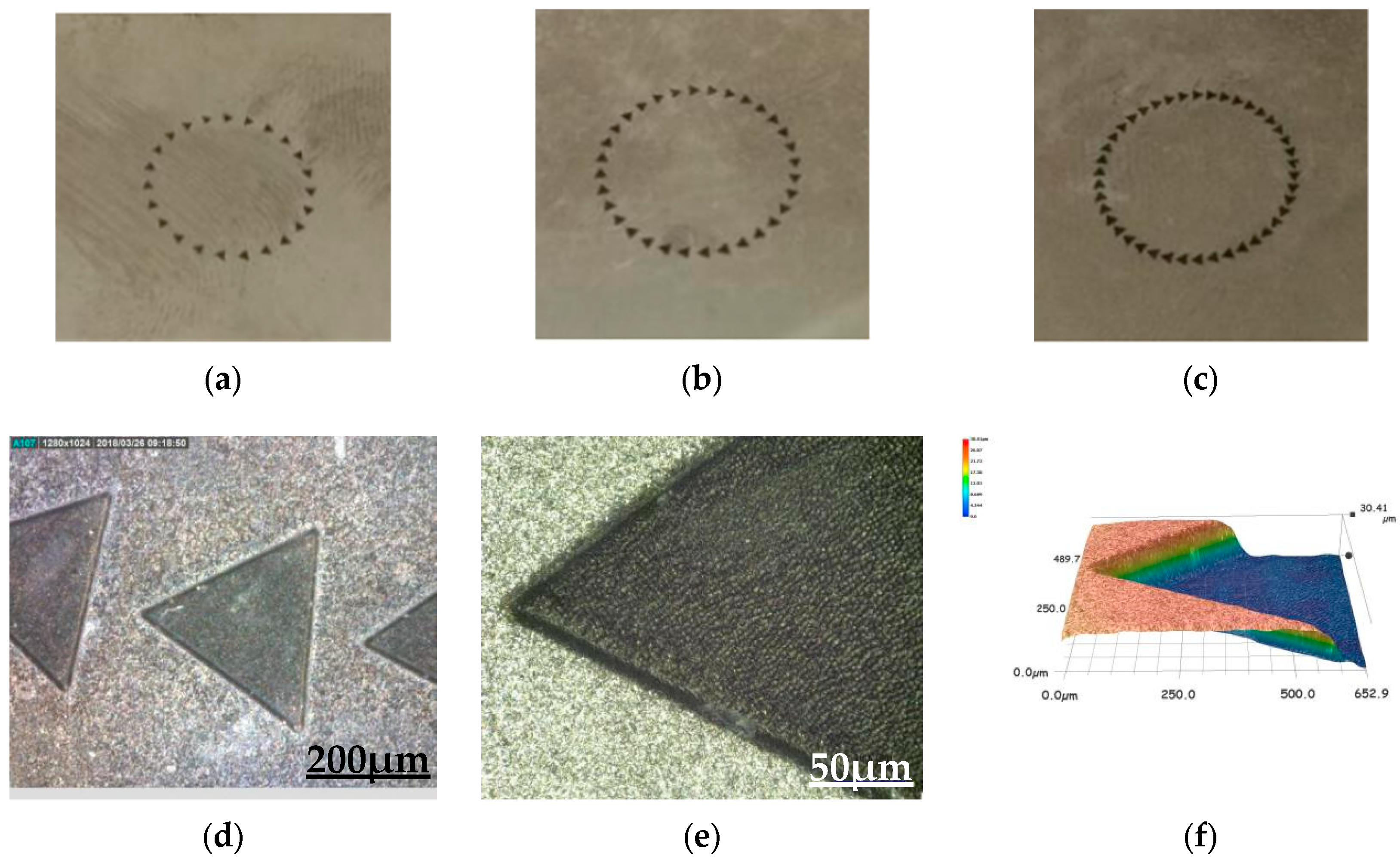

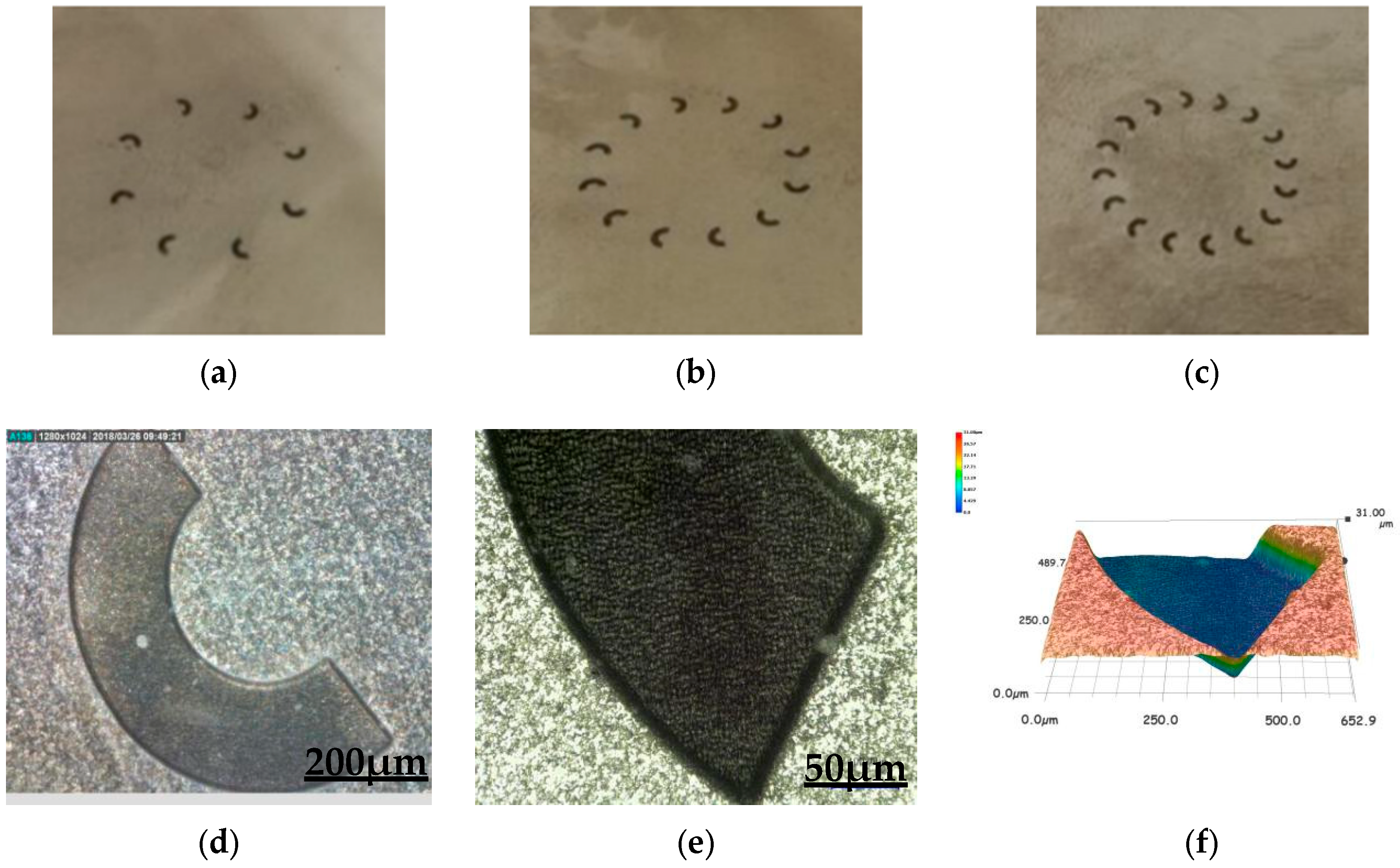






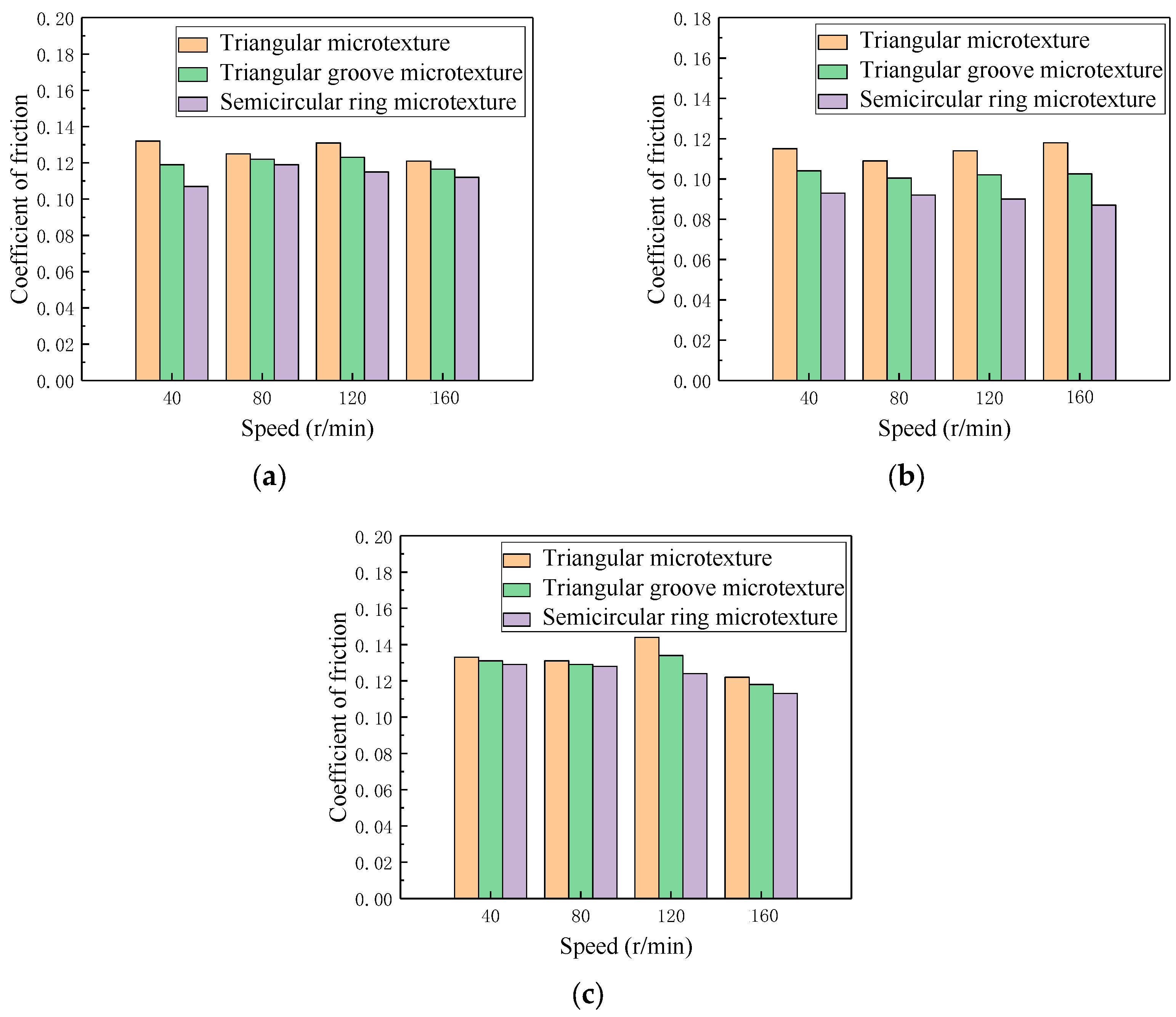



| Average Power | Pulse Frequency | Pulse Width | Laser Wavelength | Number of Scans |
|---|---|---|---|---|
| 250 mW | 10 kHz | 100 fs | 1000 nm | 10 times |
| Ingredient | Ingredient Content (%) | Hardness (HRA) | Density (g/cm3) | Bending Strength (MPa) |
|---|---|---|---|---|
| WC | 75 | 97 | 11.8 | 1180 |
| TiC | 16 | |||
| Co | 9 |
| w(C)% | w(Si)% | w(Mn)% | w(P)% | w(S)% | w(Cr)% | w(Ni)% | w(Cu)% |
|---|---|---|---|---|---|---|---|
| 0.4–0.5 | 0.18–0.36 | 0.6–0.9 | ≤ 0.035 | ≤ 0.035 | ≤ 0.25 | ≤ 0.25 | ≤ 0.25 |
| Number of Triangular Textures(n) | Area Occupancy Ratio/% |
|---|---|
| 23 | 7 |
| 32 | 10 |
| 42 | 13 |
| Number of Triangular Groove Textures (n) | Area Occupancy Ratio/% |
|---|---|
| 30 | 7 |
| 43 | 10 |
| 56 | 13 |
| Number of Semicircular Ring Textures(n) | Area Occupancy Ratio/% |
|---|---|
| 8 | 7 |
| 12 | 10 |
| 16 | 13 |
| Types of Micro-Textures | Area Occupancy Ratios | Experimental Force (N) | Rational Speed (rpm) | Form of Friction Pairs | Lubrication Condition |
|---|---|---|---|---|---|
| Triangle Triangular groove Semicircular ring | About 7% About 10% About 13% | 50 100 200 300 | 40 80 120 160 | Face contact (ring-disc friction pairs) | 25 °C Atmospheric pressure, Oil lubrication (Mobil 1 lubricant, viscosity 0–40 W) |
| Weight Loss of Non-Textured Specimens (mg) | Unpolished Sample | 28.4 |
|---|---|---|
| Polished Sample | 21.3 | |
| Weight loss of triangular textured specimens (mg) | Area occupancy ratio 7% | 16.9 |
| Area occupancy ratio 10% | 14.1 | |
| Area occupancy ratio 13% | 18.7 | |
| Weight loss of triangular groove textured specimens (mg) | Area occupancy ratio 7% | 15.4 |
| Area occupancy ratio 10% | 13.8 | |
| Area occupancy ratio 13% | 17.5 | |
| Weight loss of semicircular ring textured specimens (mg) | Area occupancy ratio 7% | 13.9 |
| Area occupancy ratio 10% | 11.7 | |
| Area occupancy ratio 13% | 14.2 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, C.; Yang, X.; Wang, S.; Wang, Y.; Lu, C.; Cao, J. Study on Friction and Lubrication Characteristics of Surface with Unidirectional Convergence Texture. Coatings 2019, 9, 780. https://doi.org/10.3390/coatings9120780
Li C, Yang X, Wang S, Wang Y, Lu C, Cao J. Study on Friction and Lubrication Characteristics of Surface with Unidirectional Convergence Texture. Coatings. 2019; 9(12):780. https://doi.org/10.3390/coatings9120780
Chicago/Turabian StyleLi, Chenchen, Xuefeng Yang, Shouren Wang, Yanjun Wang, Chongyang Lu, and Jinlong Cao. 2019. "Study on Friction and Lubrication Characteristics of Surface with Unidirectional Convergence Texture" Coatings 9, no. 12: 780. https://doi.org/10.3390/coatings9120780
APA StyleLi, C., Yang, X., Wang, S., Wang, Y., Lu, C., & Cao, J. (2019). Study on Friction and Lubrication Characteristics of Surface with Unidirectional Convergence Texture. Coatings, 9(12), 780. https://doi.org/10.3390/coatings9120780




