Innovative Flexural Repair Technique of Pre-Damaged T-Beams Using Eco-Friendly Steel-Fibre-Reinforced Geopolymer Concrete
Abstract
:1. Introduction
2. Experimental Program
2.1. Material and Mechanical Properties
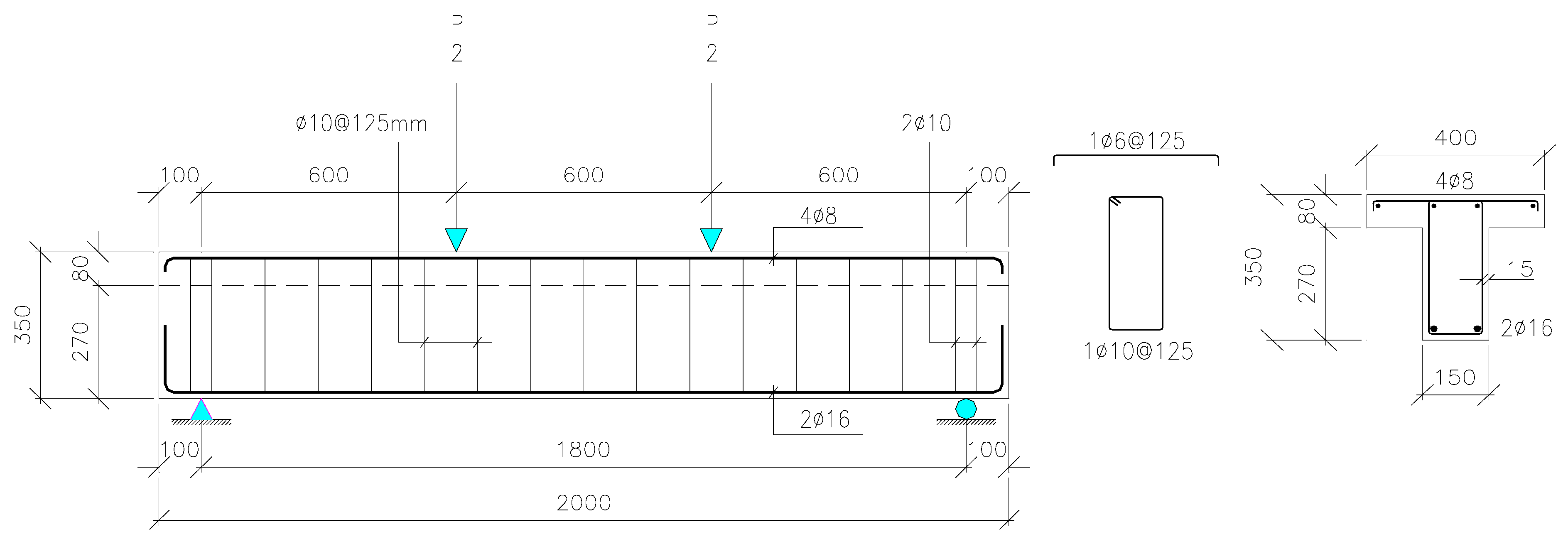
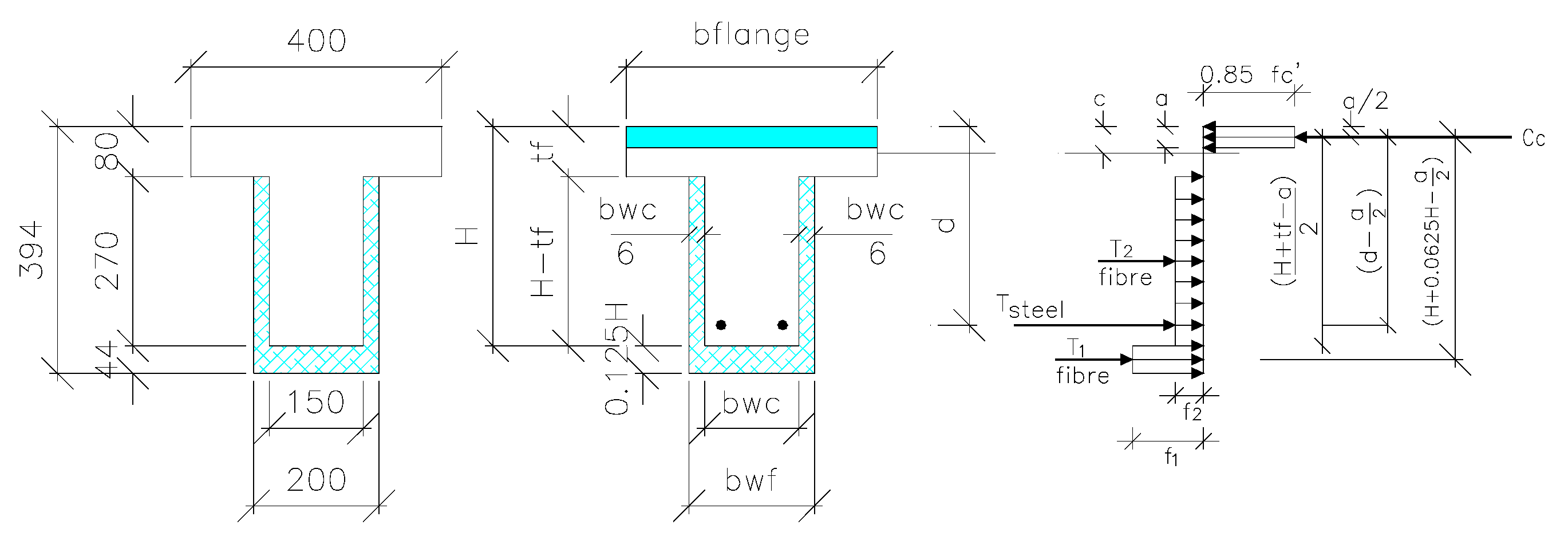
2.2. Concrete T-Beam Geometry and Reinforcement
2.3. Repaired T-Beams with SFRGPC
2.3.1. Preparation of Concrete T-Beams
2.3.2. Repair Techniques for Flexural
2.3.3. Preparation of the Pre-Damaged T-Beams
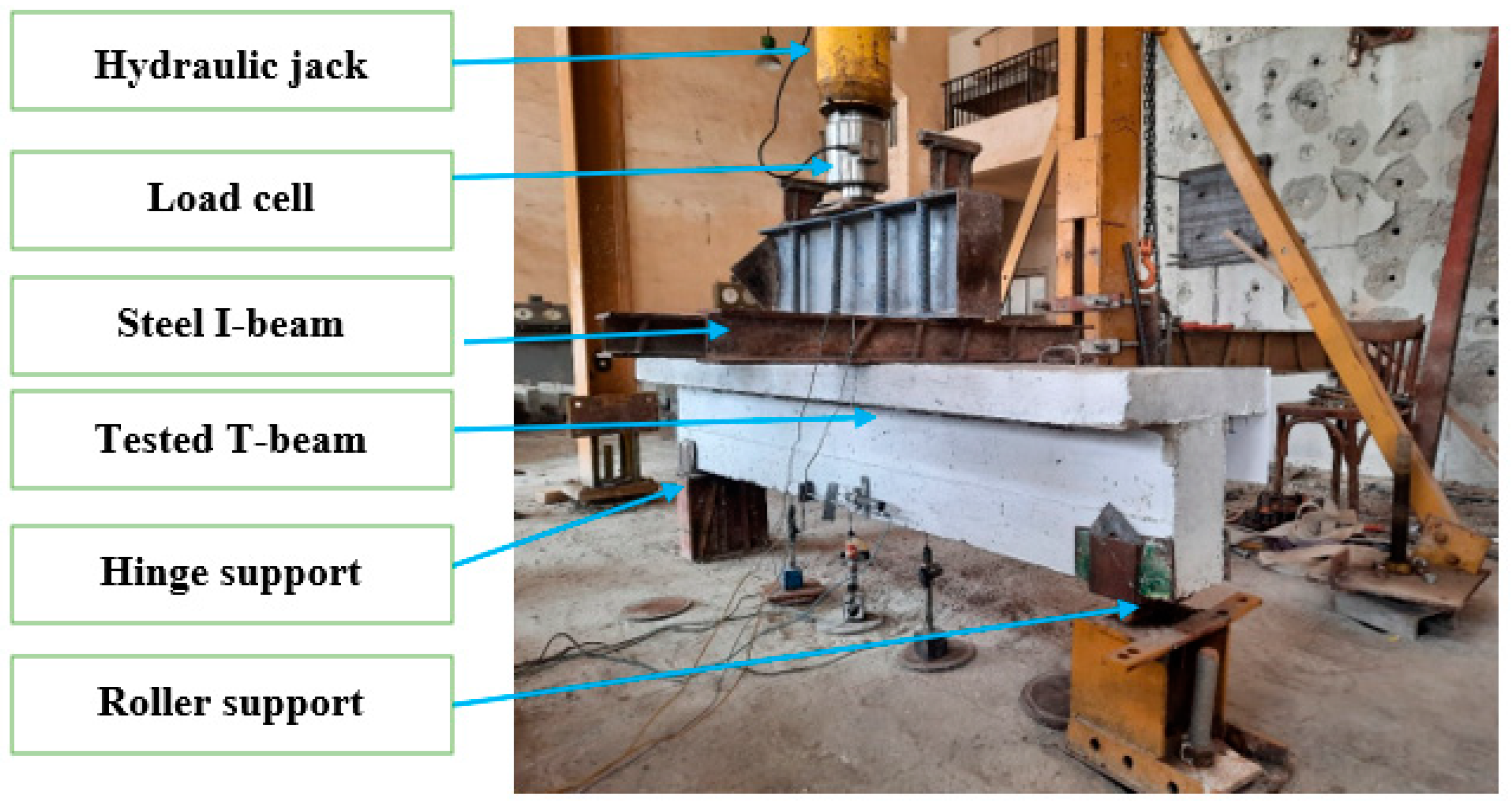
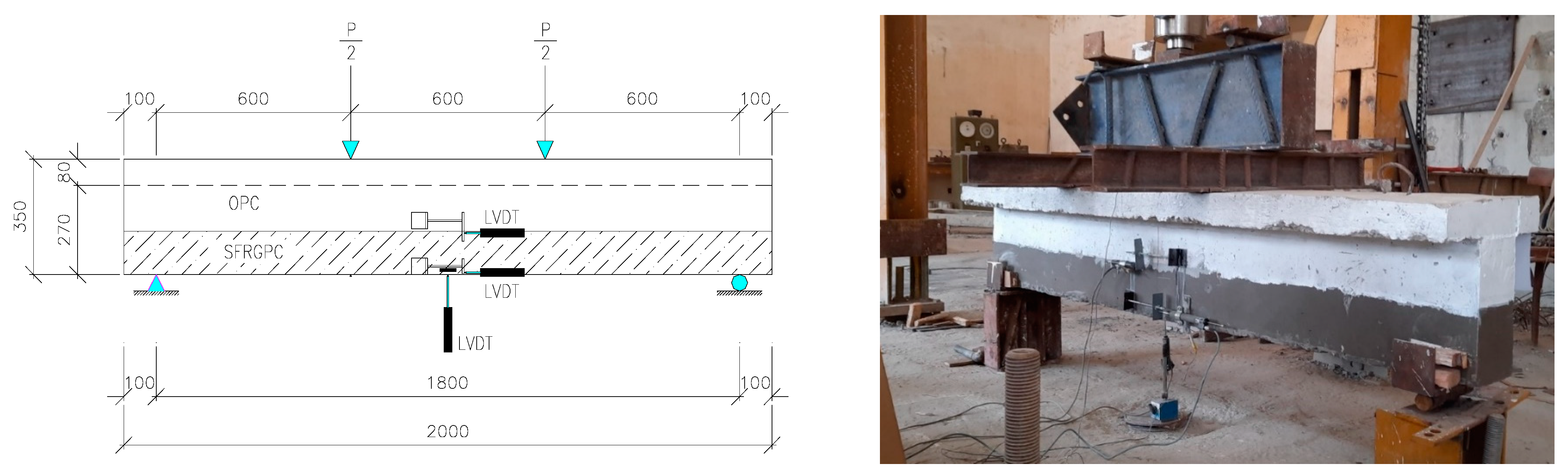
2.4. Mechanical Test Setup and Procedure
3. Analysis and Test Results
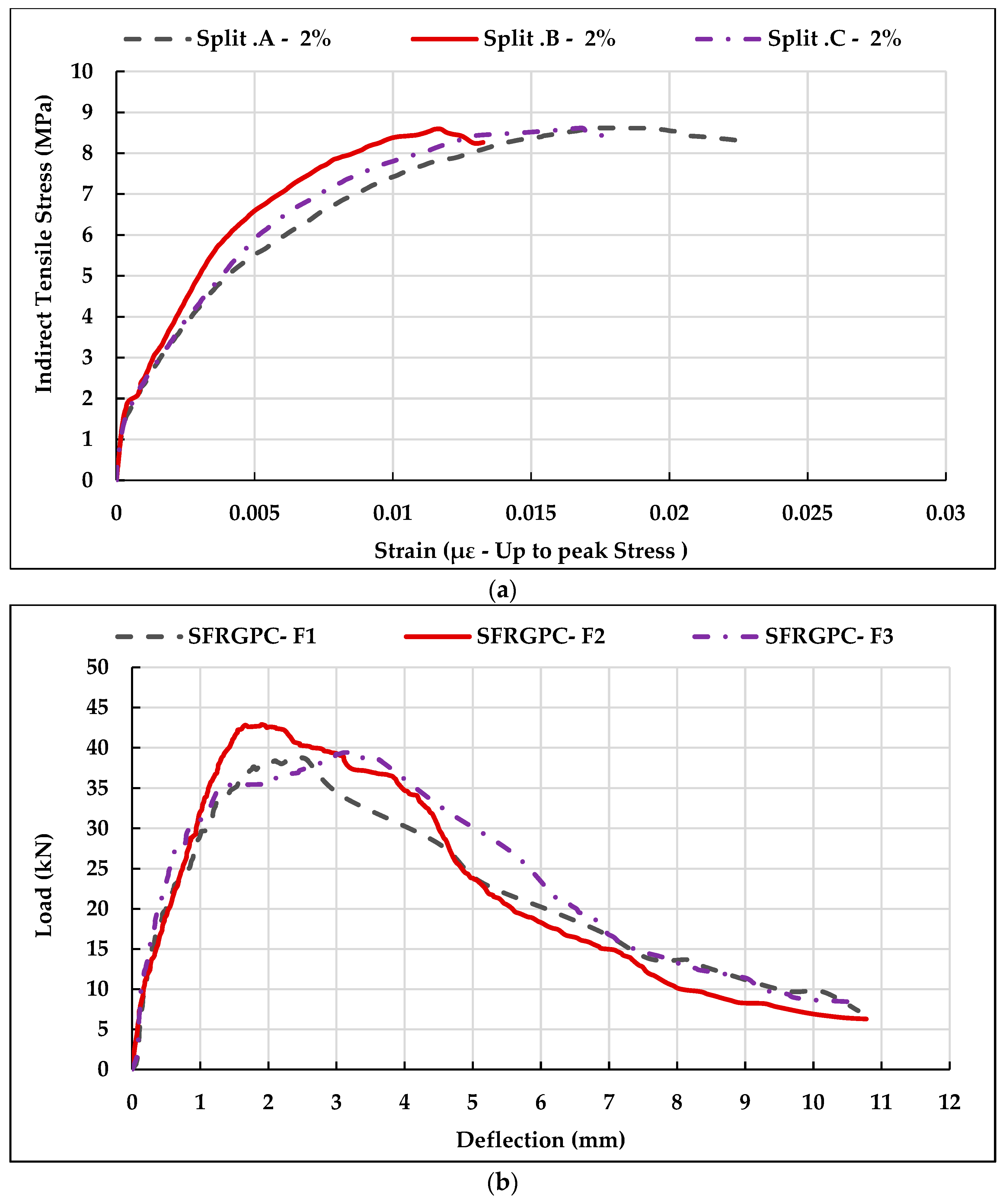
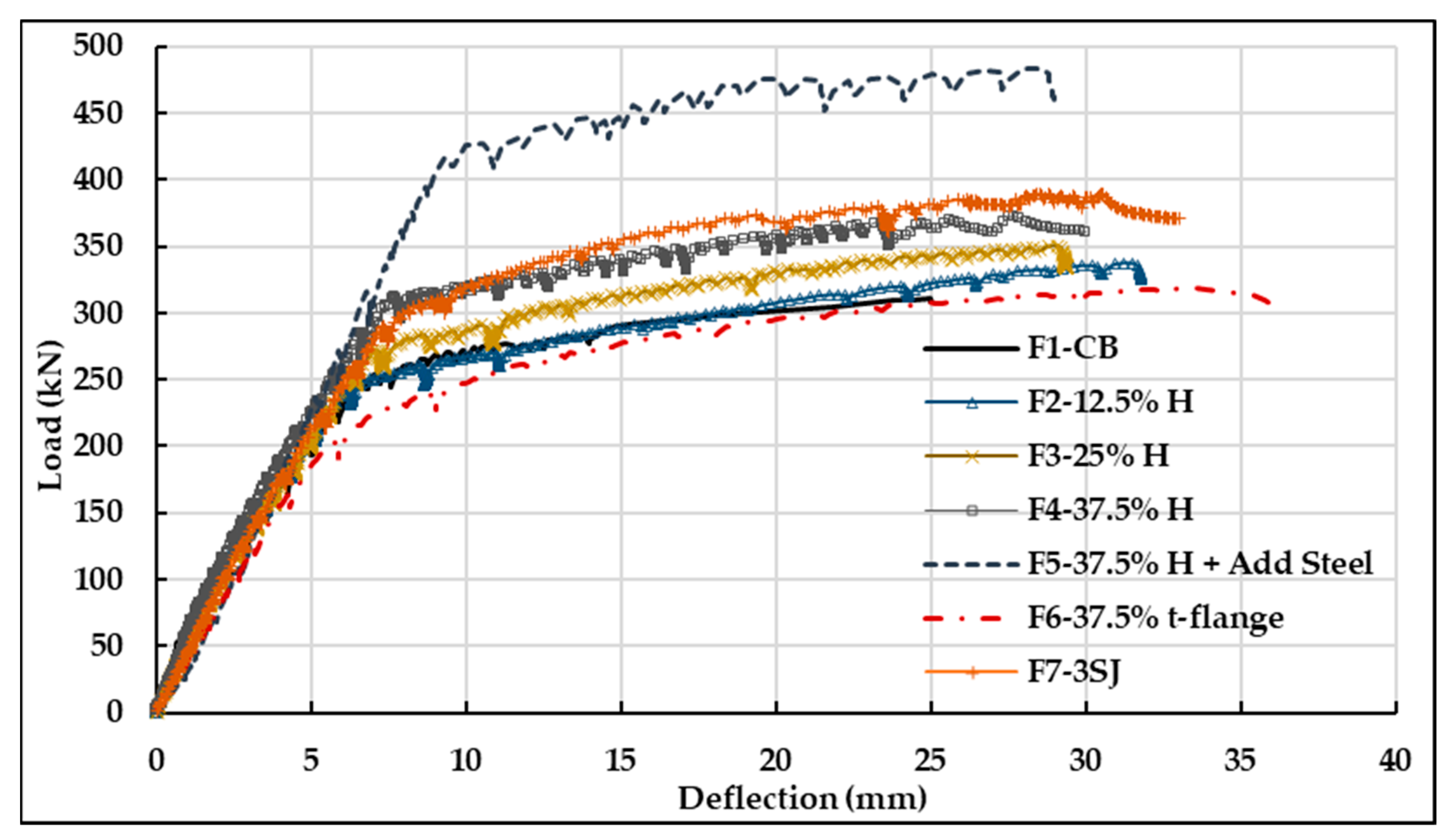
3.1. Load–Deflection Behaviour
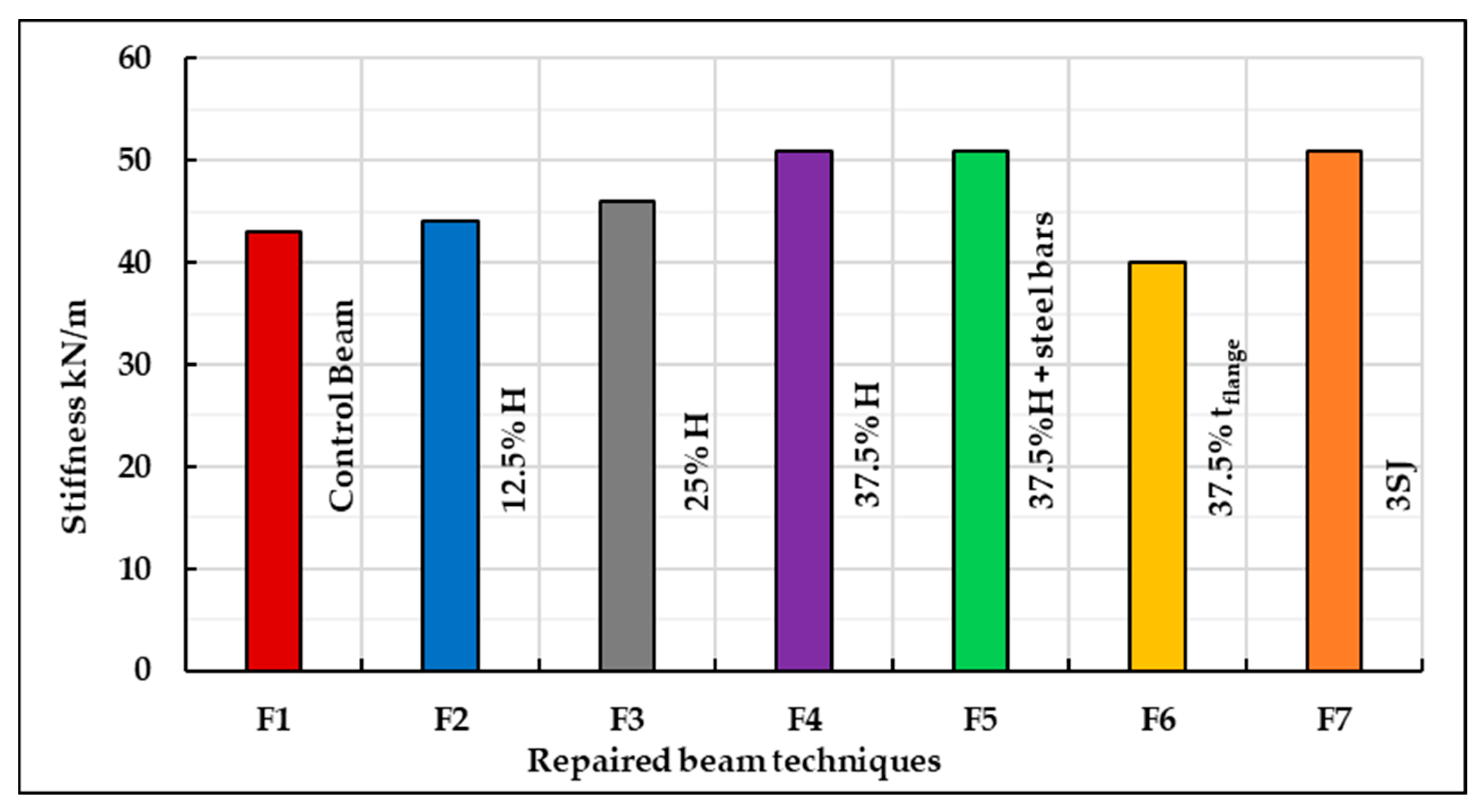
3.2. Repaired Beams Initial Stiffness
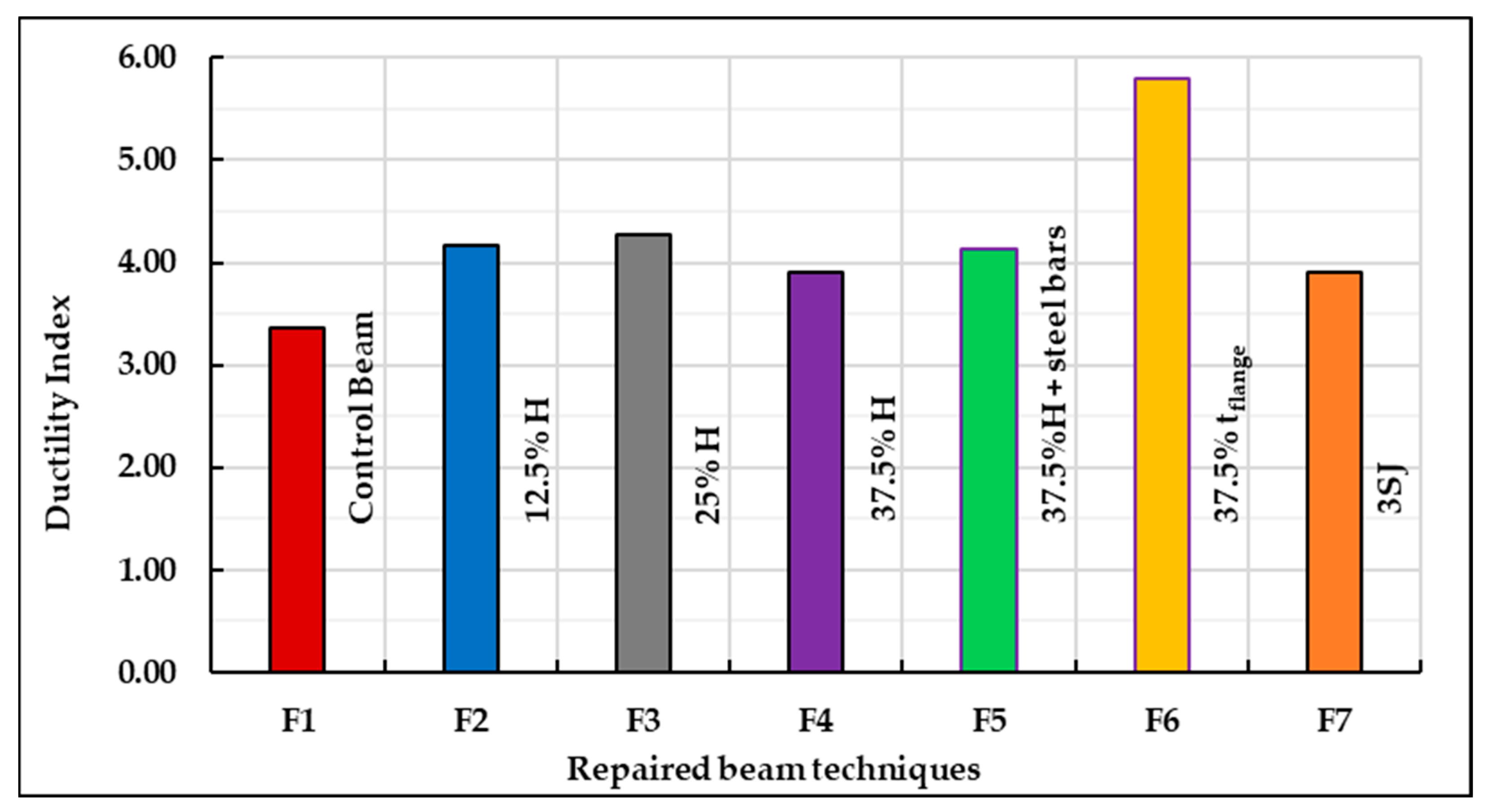
3.3. Repaired Beams Ductility Index
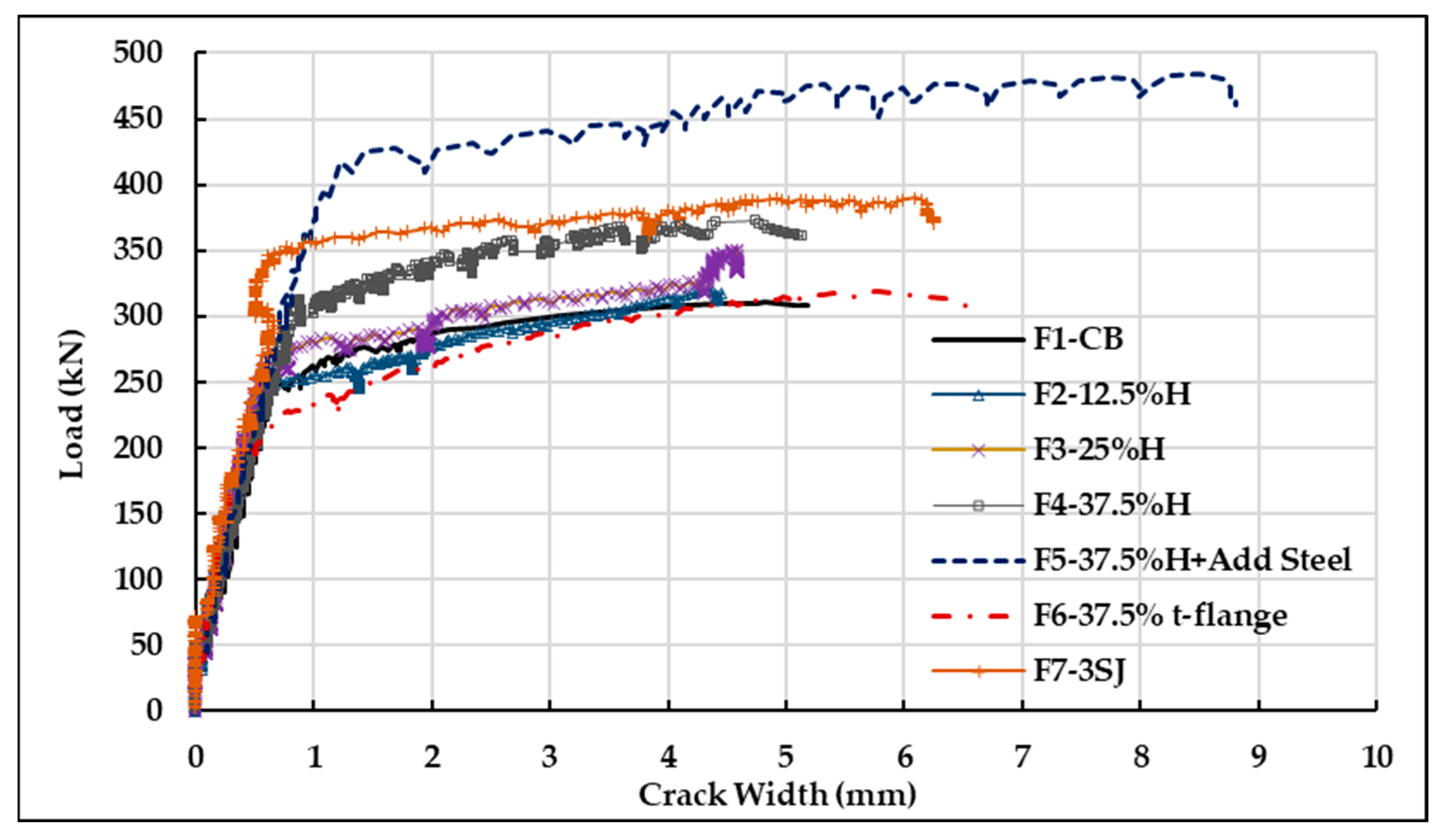
3.4. Repaired Beams Crack Width
3.5. Measured Strains
3.6. Interface Slippage Results of Repaired T-Beams
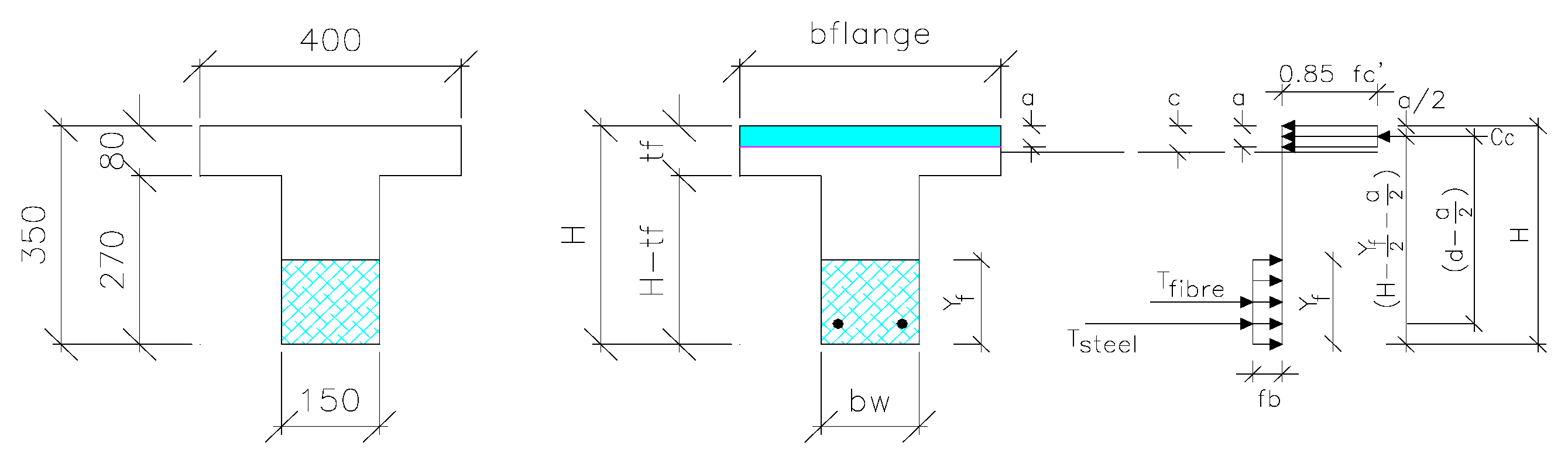
4. Analytical Modelling of Repaired T-Beams
4.1. Repair Beam at Tensile Side Only
4.2. Repair Beam at Compression Side
4.3. Repair Beam at Three Side Jacket
5. Conclusions
- The study’s findings suggest that SFRGPC has significant potential as a method for not only repairing damaged reinforced concrete beams but also as a strengthening material without changing beam dimensions.
- The repaired beams showed an increase in carrying capacity, stiffness, and ductility. Nonetheless, the failure mode identified in the repaired beams was identical to that of the control samples, which consisted of flexural fractures and mid-span crack propagation.
- The capacity of the repaired T-beams was significantly increased when steel bars were added to the SFRGPC layer, with a maximum load increase of 56% depending on the strength characteristics of the added steel bars.
- The best results (when using no additional steel) were obtained with three-sided jackets with fibrous geopolymer concrete, resulting in a load-carrying capacity increase of 25.8%, a 17% increase in ductility index, and an 18.6% increase in initial stiffness compared to the reference T-beams.
- SFRGPC helps the repaired T-beams to increase the first crack by 80% in the case of 3SJ compared to F1-CB.
- The bonding between SFRGPC and existing concrete was effective, as no slippage or disintegration at the interface of the two materials was observed during loading for all cases.
- The findings indicate the accuracy and effectiveness of the proposed model in predicting the flexural capacities of the repaired beams. However, the average prediction accuracy was 0.98, with a small standard deviation of 0.013.
- The suggested models and repair strategy have been shown to be very accurate and applicable due to the close relationship between expected and experimental findings under flexural loading conditions.
6. Recommendation for Future Studies
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Morsy, A.M.; El-Tony, M.; El-Naggar, M. Flexural repair/strengthening of pre-damaged R.C. beams using embedded CFRP rods. Alex. Eng. J. 2015, 54, 1175–1179. [Google Scholar] [CrossRef]
- Khan, A.R.; Fareed, S. Behaviour of Reinforced Concrete Beams Strengthened by CFRP Wraps with and without End Anchorages. Procedia Eng. 2014, 77, 123–130. [Google Scholar] [CrossRef]
- Chalioris, C.E.; Kosmidou, P.-M.K.; Papadopoulos, N.A. Investigation of a new strengthening technique for RC deep beams using carbon FRP ropes as transverse reinforcements. Fibers 2018, 6, 52. [Google Scholar] [CrossRef]
- Demir, A.; Ercan, E.; Demir, D. Strengthening of reinforced concrete beams using external steel members. Steel Compos. Struct. 2018, 27, 453–464. [Google Scholar]
- Christidis, K.I.; Vougioukas, E.; Trezos, K.G. Strengthening of non-conforming RC shear walls using different steel configurations. Eng. Struct. 2016, 124, 258–268. [Google Scholar] [CrossRef]
- Triantafillou, T.; Papanicolaou, C. Shear strengthening of reinforced concrete members with textile reinforced mortar (TRM) jackets. Mater. Struct. 2006, 39, 93–103. [Google Scholar] [CrossRef]
- Gopinath, S.; Murthy, A.; Iyer, N.; Dharinee, R. Investigations on textile-reinforced concrete as cover for RC beams. Mag. Concr. Res. 2016, 68, 1040–1050. [Google Scholar] [CrossRef]
- Behera, G.; Rao, T.; Rao, C. Torsional behaviour of reinforced concrete beams with ferrocement U-jacketing—Experimental study. Case Stud. Constr. Mater. 2016, 4, 15–31. [Google Scholar] [CrossRef]
- Bansal, P.; Kumar, M.; Kaushik, S. Effect of wire mesh orientation on strength of beams retrofitted using Ferrocement jackets. Int. J. Eng. 2008, 2, 8–19. [Google Scholar]
- El-Maaddawy, T.; El Refai, A. Innovative Repair of Severely Corroded T Beams Using Fabric-Reinforced Cementitious Matrix. J. Compos. Constr. 2015, 20, 1–10. [Google Scholar] [CrossRef]
- Jabr, A.; El-Ragaby, A.; Ghrib, F. Effect of the fiber type and axial stiffness of FRCM on the flexural strengthening of RC beams. Fibers 2017, 5, 2. [Google Scholar] [CrossRef]
- Al-Osta, M.; Isa, M.; Baluch, M.; Rahma, M. Flexural behavior of reinforced concrete beams strengthened with ultra-high performance fiber reinforced concrete. Constr. Build. Mater. 2017, 134, 279–296. [Google Scholar] [CrossRef]
- Mohammed, T.; Bakar, B.; Bunnori, N.; Mohammed, T.J.; Bakar, B.H.A.; Bunnori, N.M. Torsional improvement of reinforced concrete beams using ultra high-performance fiber reinforced concrete (UHPFC) jackets—Experimental study. Constr. Build. Mater. 2016, 106, 533–542. [Google Scholar] [CrossRef]
- Murthy, A.; Karihaloo, B.; Priya, D. Flexural behavior of RC beams retrofitted with ultra-high strength concrete. Constr. Build. Mater. 2018, 175, 815–824. [Google Scholar] [CrossRef]
- Al-Majidi, M.; Lampropoulos, A.; Cundy, A.; Tsioulou, O.; Al-Rekabi, S. A novel corrosion resistant repair technique for existing reinforced concrete (RC) elements using polyvinyl alcohol fibre reinforced geopolymer concrete (PVAFRGC). Constr. Build. Mater. 2018, 164, 603–619. [Google Scholar] [CrossRef]
- Ali, W.; Ibrahim, A.; Ebead, U. Flexural Behavior of RC Beams Strengthened with Steel Fibers. Int. J. Appl. Eng. Res. 2020, 15, 468–480. [Google Scholar]
- Thomas, J.; Ramaswamy, A. Mechanical properties of steel fiber-reinforced concrete. J. Mater. Civ. Eng. 2007, 19, 85–92. [Google Scholar] [CrossRef]
- Barnett, S.J.; Lataste, J.F.; Parry, T.; Millard, S.G.; Soutsos, M.N. Assessment of fiber orientation in ultra high performance fiber reinforced concrete and its effect on flexural strength. Mater. Struct. 2010, 43, 1009–1023. [Google Scholar] [CrossRef]
- Soutsos, M.; Lampropoulos, A. Flexural performance of fibre reinforced concrete made with steel and synthetic fibres. Constr. Build. Mater. 2012, 36, 704–710. [Google Scholar] [CrossRef]
- Davidovits, J.; Orlinski, J. Geoplymeric reactions in archaeological cements and in modern blended cements. In Proceedings of the 1st International Conference on Geopolymer, Compiegne, France, 1–3 June 1988; Volume 1, pp. 93–106. [Google Scholar]
- Davidovits, J. Synthetic Mineral Polymer Compound of the Silicoaluminates Family and Preparation Process. U.S. Patent 4,472,199, 18 September 1984. [Google Scholar]
- Davidovits, J. Geopolymers: Inorganic polymeric new materials. J. Mater. Educ. 1994, 16, 91–139. [Google Scholar] [CrossRef]
- Meng, L.; Ding, Y.; Wei, L.L.J.; Li, M.; Wang, J.; Cao, S.; Liu, J. Study on dynamic properties of lightweight ultra-high performance concrete (L-UHPC). Constr. Build. Mater. 2023, 399, 132526. [Google Scholar] [CrossRef]
- Kumar, V.S.; Ganesan, N.; Indira, P.; Murali, G.; Vatin, N. Behaviour of Hybrid Fibre-Reinforced Ternary Blend Geopolymer Concrete Beam-Column Joints under Reverse Cyclic Loading. Polymers 2022, 14, 2239. [Google Scholar] [CrossRef]
- Smith, J.; Comrie, D. Geoplymeric building materials in third world countries. In Proceedings of the 1st International Conference on Geopolymer, Compiegne, France, 1–3 June 1988; Volume 1, pp. 89–92. [Google Scholar]
- Duxson, P.; Fernández-Jiménez, A.; Provis, J.L.; Lukey, G.C.; Palomo, A.; Van Deventer, J.S.J. Geopolymer technology: The current state of the art. J. Mater. Sci. 2007, 42, 2917–2933. [Google Scholar] [CrossRef]
- Provis, J.L. Geopolymers and other alkali activated materials: Why, how, and what? Mater. Struct. 2014, 47, 11–25. [Google Scholar] [CrossRef]
- Aziz, I.; Abdullah, M.; Heah, C.; Liew, Y. Behaviour changes of Ground granulated blast furnace slag geopolymers at high temperature. Adv. Cem. Res. 2020, 32, 465–475. [Google Scholar] [CrossRef]
- Inazumiet, S.; Inazawa, T.; Soralump, S.; Saiki, O. Assessment of potassium silicate based surface penetration materials with low viscosity in the repair of concrete structures. Int. J. Geomate 2017, 12, 163–170. [Google Scholar]
- Yuan, Y.; Marosszeky, M. Major factor influence the performance of structural repair. Evaluation and rehabilitation of concrete structures and innovations in design. In Proceedings of the ACI International Conference, Hong Kong, China, 2–6 December 1991. [Google Scholar]
- Sayyad, A.; Patankar, S. Effect of steel fibres and low calcium fly ash on mechanical and elastic properties of geopolymer concrete composites. Indian J. Mater. Sci. 2013, 23, 1–8. [Google Scholar] [CrossRef]
- Ganesan, N.; Indira, P.; Santhakumar, A. Engineering properties of steel fibre reinforced geopolymer concrete. Adv. Concr. Constr. 2013, 1, 305–318. [Google Scholar] [CrossRef]
- Aydın, S.; Baradan, B. The effect of fibre properties on high performance alkali-activated slag/silica fume mortars. Compos. Part B 2013, 45, 63–69. [Google Scholar] [CrossRef]
- Cui, Y.; Kayali, O.; Zhao, T.; Zhang, C. Bond Strength of Steel Bar and Plain or Fibre Reinforced Geopolymer Concrete. In Proceedings of the World Congress on Advances in Structural Engineering and Mechanics, Ulsan, Republic of Korea, 28 August–1 September 2017. [Google Scholar]
- Khabaz, A. Monitoring of impact of hooked ends on mechanical behaviour of steel fibre in concrete. Constr. Build. Mater. 2016, 113, 857–863. [Google Scholar] [CrossRef]
- Çelik, A.İ.; Özkılıç, Y.O.; Bahrami, A.; Hakeem, I.Y. Mechanical performance of geopolymer concrete with micro silica fume and waste steel lathe scraps. Case Stud. Constr. Mater. 2023, 19, e02548. [Google Scholar] [CrossRef]
- Çelik, A.İ.; Özkılıç, Y.O.; Bahrami, A.; Hakeem, I.Y. Effects of Glass Fiber on Recycled Fly Ash and Basalt Powder Based Geopolymer Concrete. Case Stud. Constr. Mater. 2023, 19, e02659. [Google Scholar] [CrossRef]
- Çelik, A.L.I.; Özkılıç, Y. Geopolymer concrete with high strength, workability and setting time using recycled steel wires and basalt powder. Steel Compos. Struct. 2023, 46, 689–707. [Google Scholar]
- Rickard, W.D.; Borstel, C.D.; van Riesse, A. The effect of pre-treatment on the thermal performance of fly ash geopolymers. Thermochim. Acta 2013, 573, 130–137. [Google Scholar] [CrossRef]
- Shaikh, F.A.; Hosan, A. Mechanical properties of steel fibre reinforced geopolymer concretes at elevated temperatures. Constr. Build. Mater. 2016, 114, 15–28. [Google Scholar] [CrossRef]
- Momayez, A.; Ehsani, M.; Ramezanianpour, A.; Rajaie, H. Comparison of methods for evaluating bond strength between concrete substrate and repair materials. Cem. Concr. Res 2005, 35, 748–757. [Google Scholar] [CrossRef]
- Sprinkel, M.; Ozyildirim, C. Evaluation of High Performance Concrete Overlays Placed on Route 60 over Lynnhaven Inlet in Virginia; Virginia Transportation Research Council: Charlottesville, VA, USA, 2000.
- Jiang, B.; Qian, Z.; Gu, D.; Pan, J. Repair concrete structures with high-early-strength engineered cementitious composites (HES-ECC): Material design and interfacial behavior. J. Build. Eng. 2023, 68, 106060. [Google Scholar] [CrossRef]
- Al-Nsour, R.; Abdel-Jaber, M.; Ashteyat, A.; Shatarat, N. Flexural repairing of heat damaged reinforced concrete beams using NSM-BFRP bars and NSM-CFRP ropes. Compos. Part C Open Access 2023, 12, 100404. [Google Scholar] [CrossRef]
- Esmaeili, J.; Khoshkanabi, S.; Andalibi, K.; Kasaei, J. An innovative method for improving the cyclic performance of concrete beams retrofitted with prefabricated basalt-textile-reinforced ultra-high performance concrete. Structures 2023, 52, 813–823. [Google Scholar] [CrossRef]
- ASTM Standard C114-00; Standard Test Methods for chemical Analysis of Hydraulic Cement. ASTM International: West Conshohocken, PA, USA, 2000.
- ASTM Standard C114-18; Standard Test Methods for chemical Analysis of Hydraulic Cement. ASTM International: West Conshohocken, PA, USA, 2018.
- BS 882:1992; Specification for Aggregates from Natural Sources for Concrete. British Standards Institute: London, UK, 1992.
- Potassium, G. Abo-Ghanema Fertilizers and Chemical Industries Company; Giza, Egypt, 2020. [Online]. Available online: https://www.dalil140.com/company?lng=en&n=169371-abo-ghanema-trade-agencies (accessed on 9 October 2023).
- ASTM C191-08; Standard Test Methods for Time of Setting Hydraulic Cement by Vicat Needle. ASTM International: West Conshohocken, PA, USA, 2008.
- ASTM C 230-08; Standard Specification for Flow Table for Use in Tests of Hydraulic Cement. ASTM International: West Conshohocken, PA, USA, 2008.
- Özkılıç, Y.O.; Aksoylu, C.; Arslan, M.H. Numerical evaluation of effects of shear span, stirrup spacing and angle of stirrup on reinforced concrete beam behaviour. Struct. Eng. Mech. Int. J. 2021, 79, 309–326. [Google Scholar]
- Beushausen, H.; Alexander, M. Failure mechanisms and tensile relaxation of bonded concrete overlays subjected to differential shrinkage. Cem. Concr. Res. 2006, 36, 1908–1914. [Google Scholar] [CrossRef]
- Dittmer, T.; Beushausen, H. The effect of coarse aggregate content and size on the age at cracking of bonded concrete overlays subjected to restrained deformation. Constr. Build. Mater. 2014, 69, 73–82. [Google Scholar] [CrossRef]
- Thirugnanam, G.; Govindan, P.; Sethratnam, A. Ductile behaviour of Slurry Infiltrated Fibrous Concrete (SIFCON) structural members. J. Struct. Eng. 2001, 28, 27–32. [Google Scholar]
- fib Bulletin. No 43; Structural Connections for Precast Concrete Buildings. International Federation for Structural Concrete (fib): Lausanne, Switzerland, 2008.
- GRECO. Greek Retrofitting Code (Final Version). In Greek Organization for Seismic Planning and Protection; Greek Ministry for Environmental Planning and Public Works: Athens, Greek, 2012. [Google Scholar]
- MARÍ, A.; Cladera, A.; Bairán, J.; Oller, E.; Ribas, C. Shear–flexural strength mechanical model for the design and assessment of reinforced concrete beams subjected to point or distributed loads. Front. Struct. Civ. Eng. 2014, 8, 337–353. [Google Scholar] [CrossRef]
- Cladera, A.; Marí, A.; Ribas, C.; Bairán, J.; Oller, E. Predicting the shear–flexural strength of slender reinforced concrete T and I shaped beams. Eng. Struct. 2015, 101, 386–398. [Google Scholar] [CrossRef]
- Qiu, M.; Shao, X.; Wille, K.; Yan, B.; Wu, J. Experimental Investigation on Flexural Behavior of Reinforced Ultra High Performance Concrete Low-Profile T-Beams. Int. J. Concr. Struct. Mater. 2020, 14, 20. [Google Scholar] [CrossRef]
- ECCS Committee. Egyptian Code for Design and Construction of Concrete Structures (Code N 203–Ministerial Decision 712/2017); Dokki Housing and Building National Research Center (HBRC): Giza, Egypt, 2017. [Google Scholar]
- EN 1992–1–1:2008; Eurocode 2, Design of Concrete Structures—Part 1-1: General Rules and Rules for Buildings. European Committee for Standardization: Brussels, Belgium, 2008.
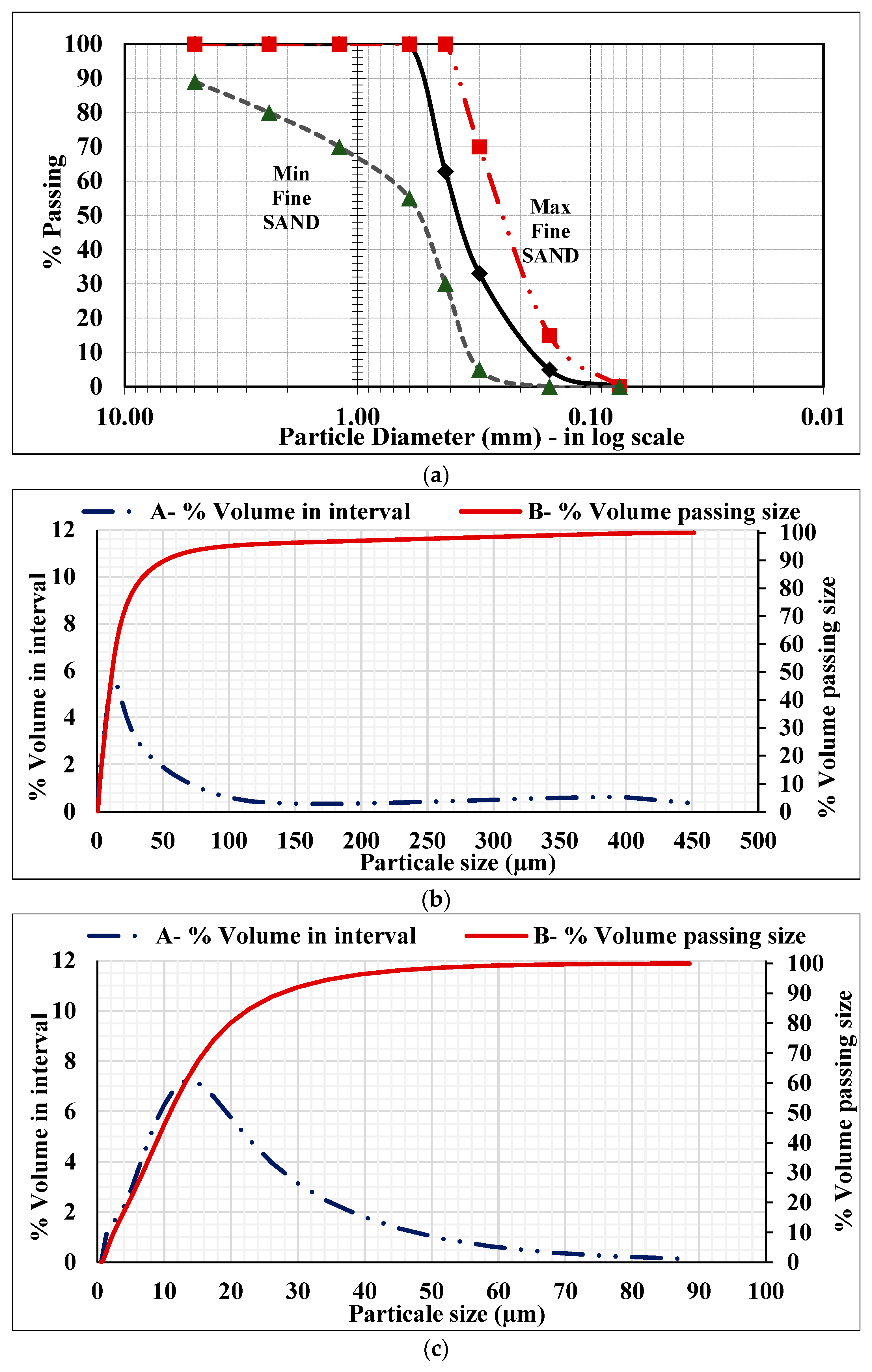
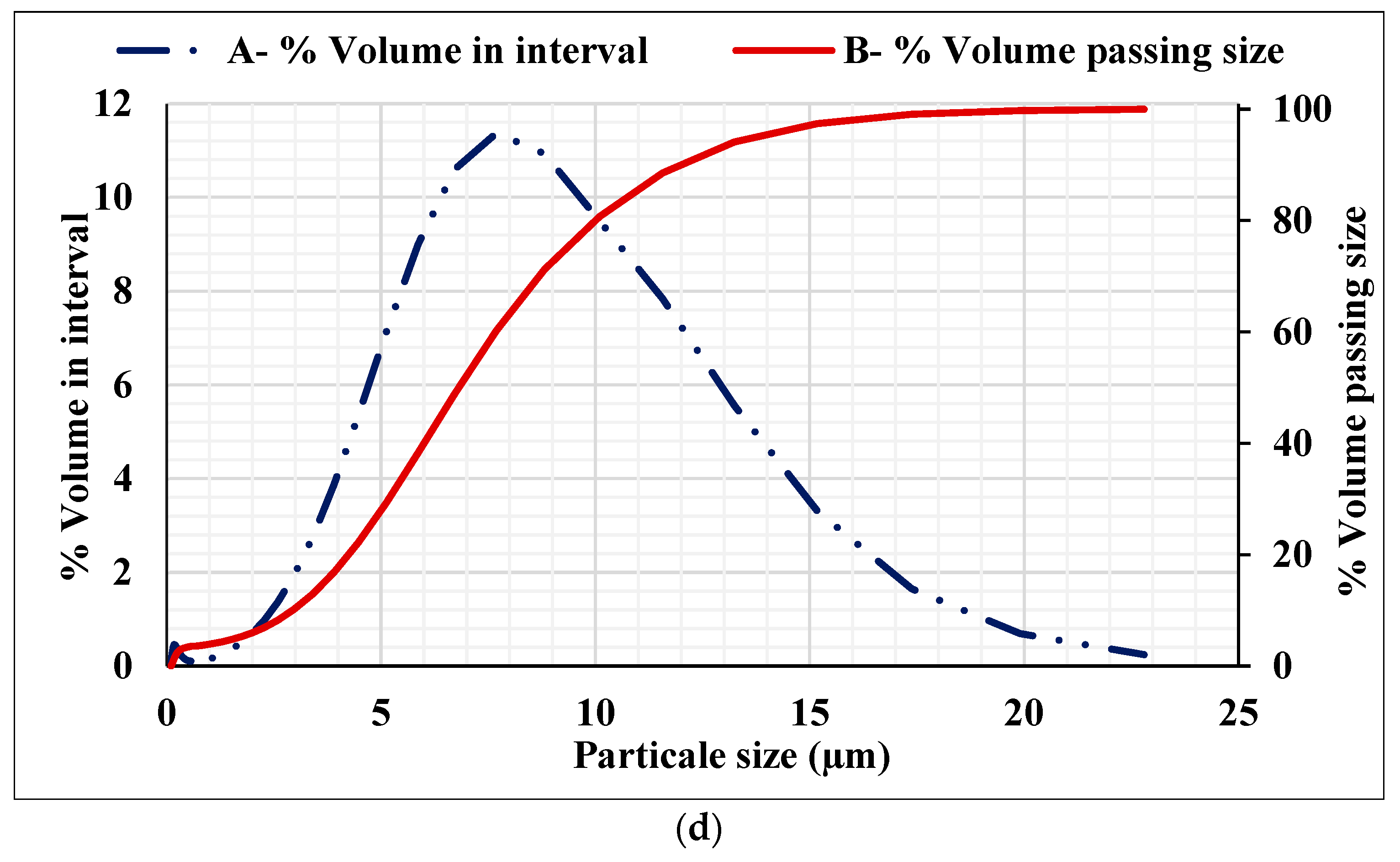










| Chemical Composition % | Symbol | FA | GGBS | USF | Cement |
|---|---|---|---|---|---|
| Silica (Silicon Dioxide) | SiO2 | 68.46 | 35.40 | 92.30 | 21.7 |
| Alumina (Aluminum Oxide) | Al2O3 | 11.20 | 17.40 | 0.37 | 6.3 |
| Calcium Oxide | CaO | 6.43 | 36.87 | 0.45 | 64.5 |
| Magnesium Oxide | MgO | 1.38 | 6.83 | 0.46 | 1.86 |
| Ferric Oxide | Fe2O3 | 8.52 | 1.40 | 2.57 | 3.4 |
| Manganese Oxide | MnO | 0.14 | 0.35 | 0.15 | 0.02 |
| Sodium Oxide | Na2O | 0.31 | 0.45 | 0.79 | 0.28 |
| Potassium Oxide | K2O | 0.54 | 0.97 | 0.56 | 0.54 |
| Loss on Ignition | LOI | 1.10 | <0.01 | 1.88 | 2.61 |
| Material | Mixture Composition kg/m3 |
|---|---|
| Fly ash (FA) | 387 |
| GGBS (Slag) | 310 |
| Undensified silica fume (USF) | 78 |
| Silica sand | 1052 |
| Alkaline activator/binder | 0.50 |
| Potassium silicate/potassium hydroxide | 2.50 |
| Molarity of potassium hydroxide | 16M |
| Alkaline activator solution | 277 K2SiO3 |
| 110 KOH | |
| Steel fibres volume (2%) | 157 |
| Beam No. | Beam Identification | Repair Pattern | Repair Technique Details |
|---|---|---|---|
| F1 | C.B | - | Control beam |
| F2 | 12.5%H | 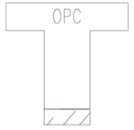 | Replacing at the tension side by 44 mm |
| F3 | 25%H | 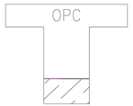 | Replacing at the tension side by 87.5 mm |
| F4 | 37.5%H | 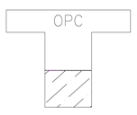 | Replacing at the tension side by 131.25 mm |
| F5 | 37.5%H+ 50%As | Replacing at the tension side by 131.25 mm with additional steel bars * | |
| F6 | 37.5% tflange |  | Replacing at the compression side by 30 mm |
| F7 | 3SJ |  | The web jacket thickness was 25 mm (1/6 width) for each side, and the bottom jacket thickness was 44 mm (12.5%H) |
| Beam No. | Preload Damage Level | Repair | First Crack | Peak Load | Prepair–Pcontrol | Failure Mode (S-F) * | |||
|---|---|---|---|---|---|---|---|---|---|
| Pcr kN | Δcr mm | Ppeak kN | Wc mm | Δp mm | |||||
| F1-CB | Ppeak | No | 40.17 | 0.6 | 310 | 5.1 | 25 | - | F |
| F2-12.5%H | 50% Ppeak | yes | 31.6 | 0.58 | 337 | 5.68 | 31.23 | 8.7% | F |
| F3-25%H | 50% Ppeak | yes | 44.38 | 0.87 | 350 | 4.6 | 29.08 | 12.9% | F |
| F4-37.5%H | 50% Ppeak | yes | 48.4 | 0.9 | 372.3 | 4.75 | 27.7 | 20.1% | F |
| F5-37.5%H+ 50%As | 50% Ppeak | yes | 44.83 | 1.25 | 483.7 | 8.5 | 28.5 | 56% | F |
| F6-37.5% tflange | 50% Ppeak | yes | 24.68 | 0.62 | 318.4 | 5.7 | 33.1 | 2.7% | F |
| F7-3SJ | 50% Ppeak | yes | 72.5 | 1.64 | 390 | 6.1 | 30.5 | 25.8% | F |
| Beam No. | Preload Damage Level | Repair | Experimental | Predicted | Experiment–Predicted | ||
|---|---|---|---|---|---|---|---|
| Pexp kN | Mexp kN.m | PPre kN | MPre kN.m | ||||
| F1-CB | Ppeak | No | 310 | 93 | 318 | 95.5 | 0.975 |
| F2-12.5%H | 50% Ppeak | yes | 337 | 101.1 | 346 | 103.7 | 0.974 |
| F3-25%H | 50% Ppeak | yes | 350 | 105 | 363 | 109 | 0.965 |
| F4-37.5%H | 50% Ppeak | yes | 372.3 | 111.69 | 378 | 113.4 | 0.985 |
| F5-37.5%H+ 50%As | 50% Ppeak | yes | 483.7 | 145.11 | 486.5 | 146 | 0.994 |
| F6-37.5% tflange | 50% Ppeak | yes | 318.4 | 95.52 | 331 | 99.5 | 0.962 |
| F7-3SJ | 50% Ppeak | yes | 389.7 | 116.91 | 392 | 117.7 | 0.994 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khalifa, A.; El-Thakeb, A.E.-W.; El-Sebai, A.; Elmannaey, A. Innovative Flexural Repair Technique of Pre-Damaged T-Beams Using Eco-Friendly Steel-Fibre-Reinforced Geopolymer Concrete. Fibers 2024, 12, 3. https://doi.org/10.3390/fib12010003
Khalifa A, El-Thakeb AE-W, El-Sebai A, Elmannaey A. Innovative Flexural Repair Technique of Pre-Damaged T-Beams Using Eco-Friendly Steel-Fibre-Reinforced Geopolymer Concrete. Fibers. 2024; 12(1):3. https://doi.org/10.3390/fib12010003
Chicago/Turabian StyleKhalifa, Ashraf, Abo El-Wafa El-Thakeb, Ahmed El-Sebai, and Ahmed Elmannaey. 2024. "Innovative Flexural Repair Technique of Pre-Damaged T-Beams Using Eco-Friendly Steel-Fibre-Reinforced Geopolymer Concrete" Fibers 12, no. 1: 3. https://doi.org/10.3390/fib12010003
APA StyleKhalifa, A., El-Thakeb, A. E.-W., El-Sebai, A., & Elmannaey, A. (2024). Innovative Flexural Repair Technique of Pre-Damaged T-Beams Using Eco-Friendly Steel-Fibre-Reinforced Geopolymer Concrete. Fibers, 12(1), 3. https://doi.org/10.3390/fib12010003







