Phaseless Characterization of Compact Antenna Test Range via Improved Alternating Projection Algorithm
Abstract
:1. Introduction
2. Phase Retrieval as a Feasibility Problem
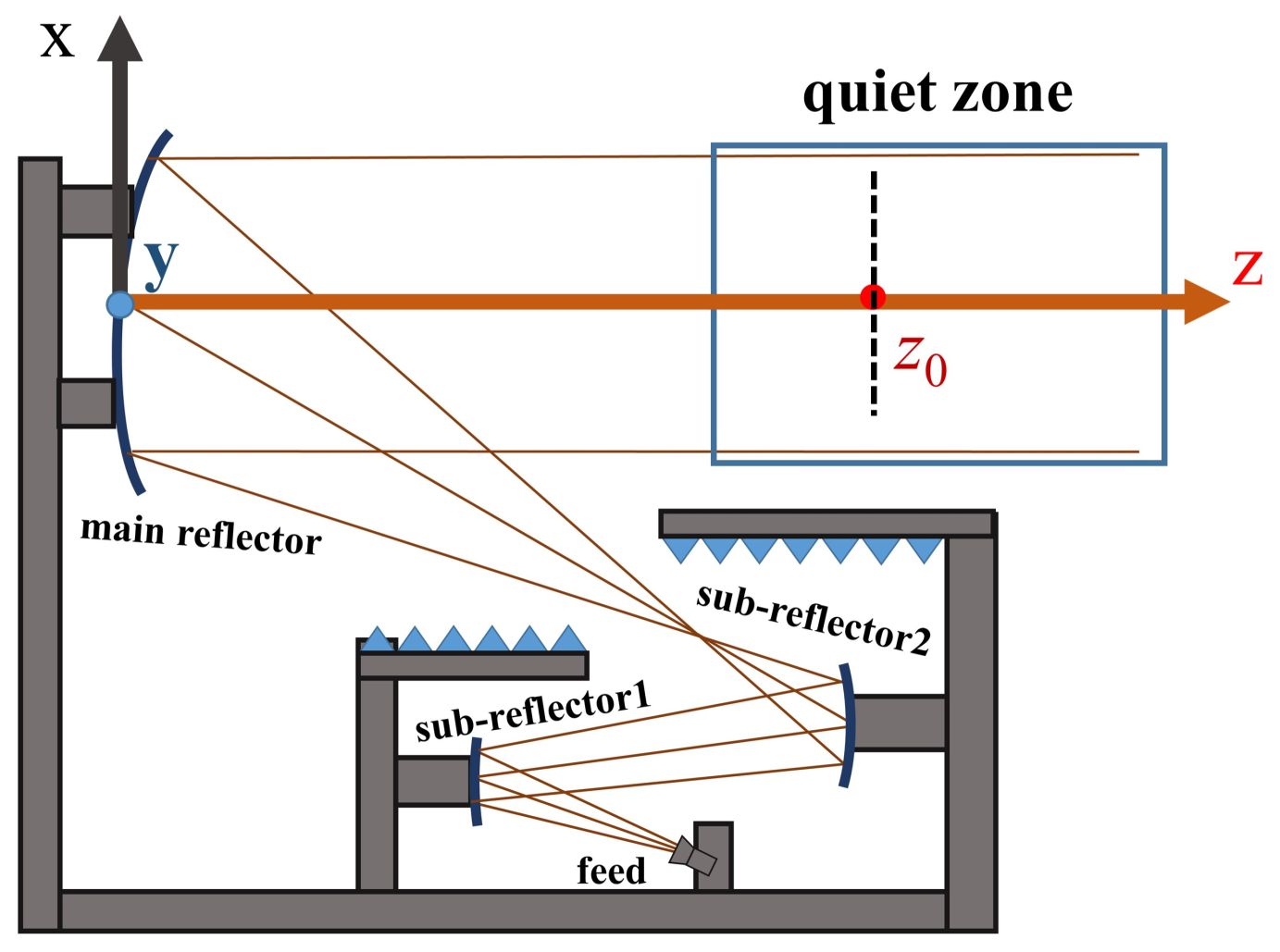
3. The Theory of Phase Retrieval of Quiet Zone
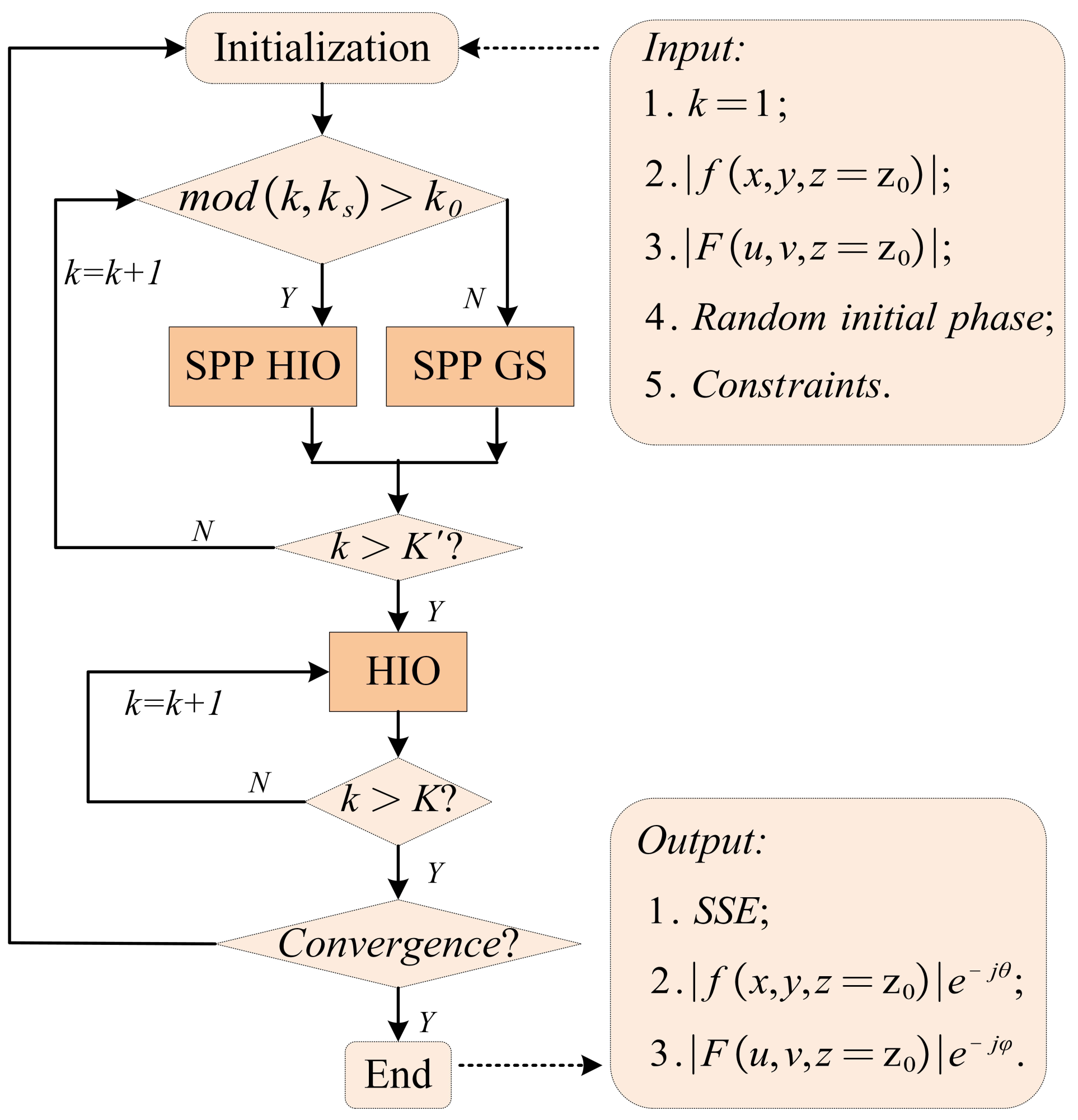
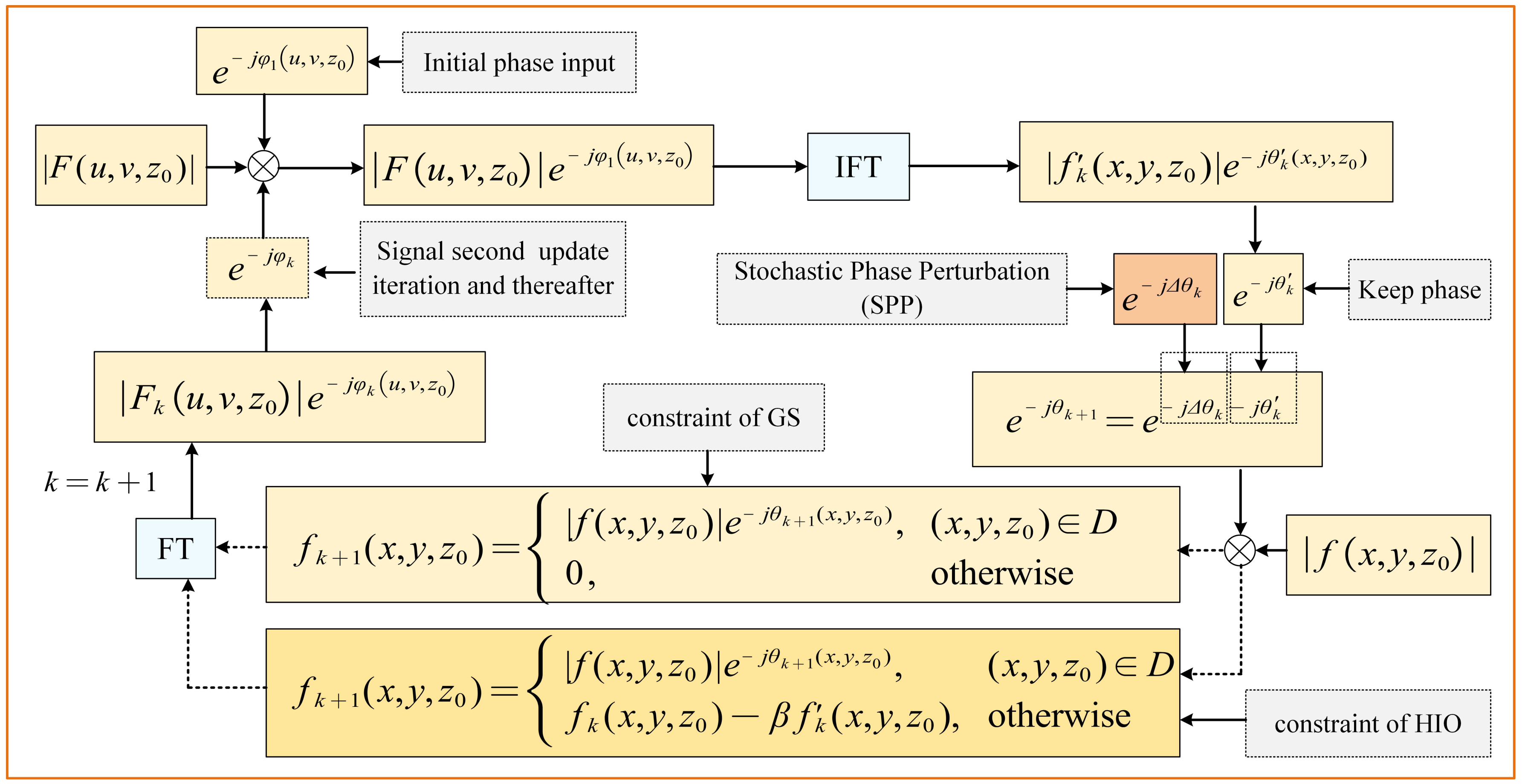
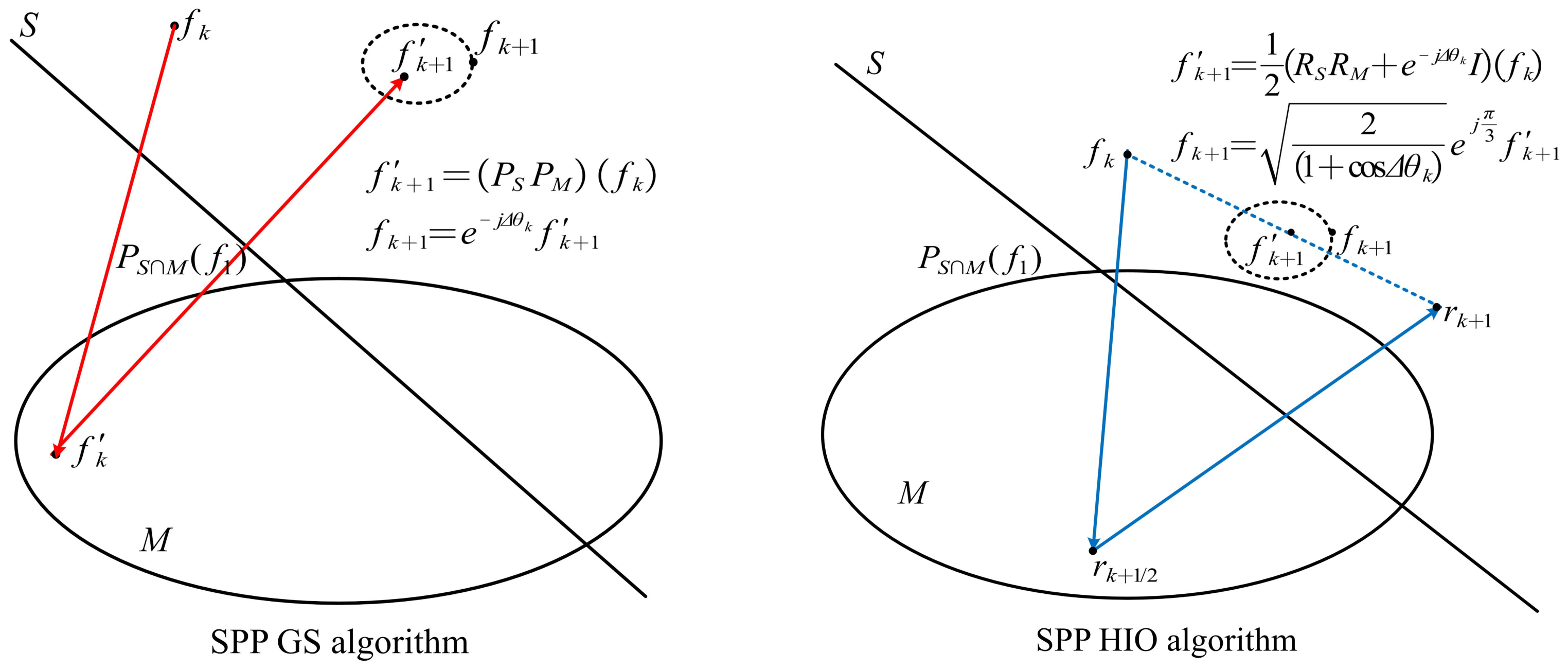
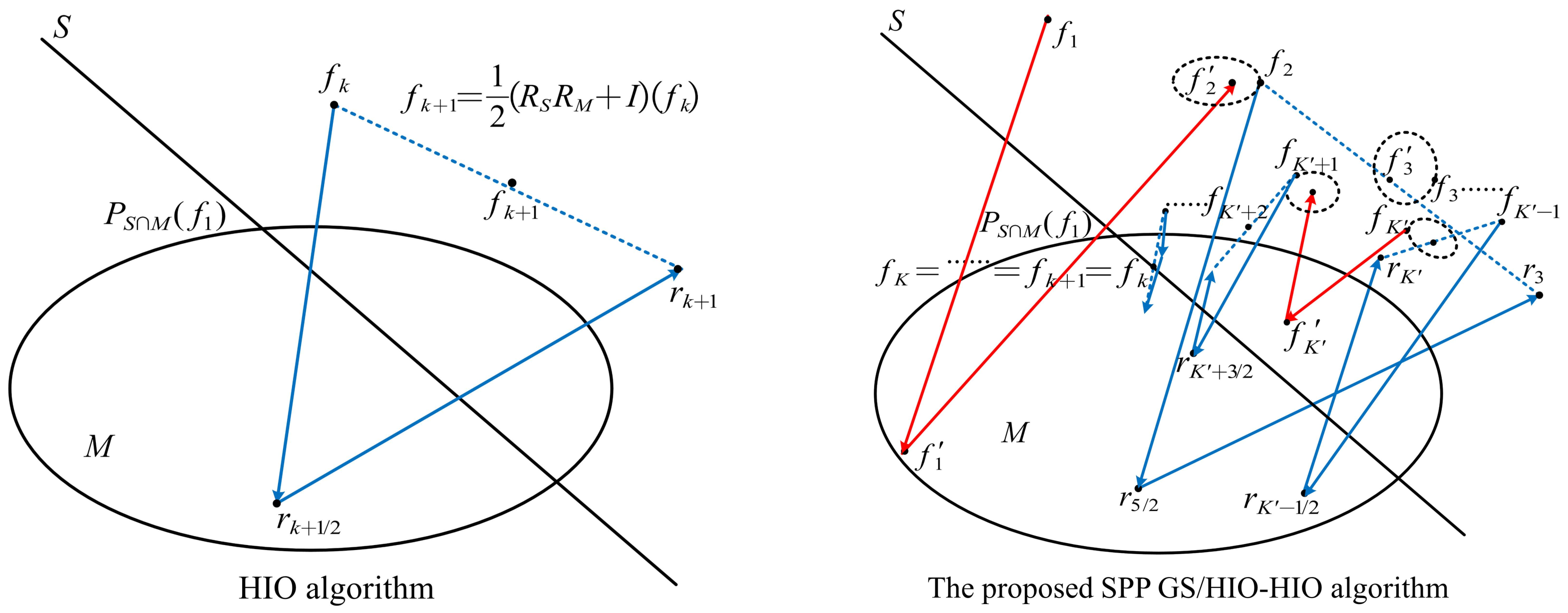
3.1. The Proposed SPP GS/HIO-HIO Algorithm
3.2. An Outline of the Solution Steps
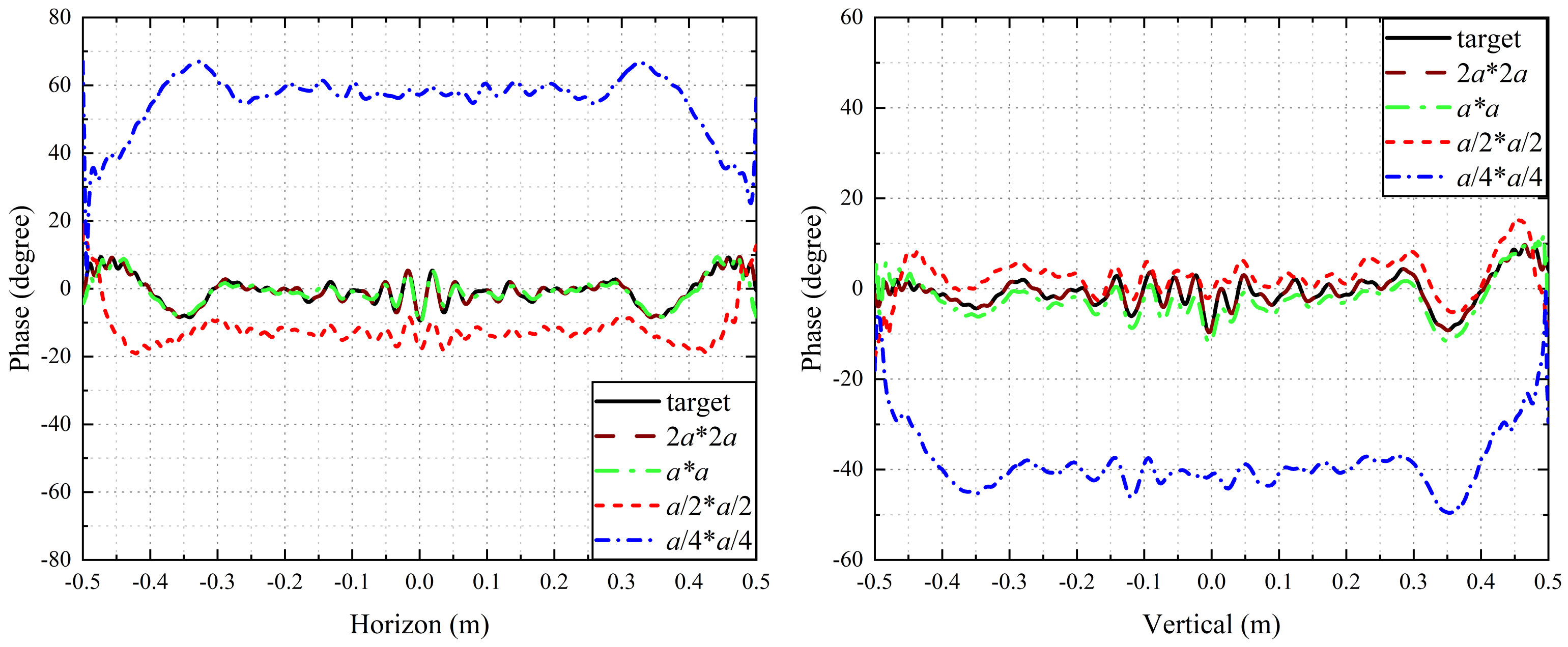
4. Numerical Verification of the Theoretical Results
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Liu, C.; Wang, X. Design and test of a 0.3 THz compact antenna test range. Prog. Electromagn. Res. 2017, 70, 81–87. [Google Scholar] [CrossRef] [Green Version]
- Gregson, S.F.; Parini, C.G. Compact Antenna Test Ranges: The Use of Simulation and Post-Processing Techniques in Support of 5G OTA Testing. In Proceedings of the 2019 13th European Conference on Antennas and Propagation (EuCAP), Krakow, Poland, 31 March–4 April 2019; IEEE: Piscataway, NJ, USA, 2019; p. 1. [Google Scholar]
- Gemmer, T.; Cornelius, R.; Pamp, J.; Heberling, D. Full-wave analysis of a compact antenna test range including probe effect. In Proceedings of the 2017 11th European Conference on Antennas and Propagation (EUCAP), Paris, France, 19–24 March 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 2571–2575. [Google Scholar]
- Rowell, C.; Derat, B.; Cardalda-Garcia, A. Multiple CATR Reflector System for Multiple Angles of Arrival Measurements of 5G Millimeter Wave Devices. IEEE Access 2020, 8, 211324–211334. [Google Scholar] [CrossRef]
- Yang, C.; Yu, J.; Yao, Y.; Liu, X.; Chen, X. Numerical synthesis of tri-reflector CATR with high cross-polarisation isolation. Electron. Lett. 2016, 52, 1286–1288. [Google Scholar] [CrossRef]
- PARINI, C.; Gregson, S. Examination of the Effectiveness of Far-field Mathematical Absorber Reflection Suppression in a CATR Through Electromagnetic Simulation. IET Microwaves Antennas Propag. 2017. [Google Scholar] [CrossRef]
- Wang, M.; Li, D.; Zhou, X.; He, G. Design, fabrication and on-site alignment of low-cost reflector used in large-scale compact antenna test range. In Proceedings of the 2017 11th European Conference on Antennas and Propagation (EUCAP), Paris, France, 19–24 March 2017; IEEE: Piscataway, NJ, USA, 2017; pp. 2590–2594. [Google Scholar]
- Descloux, A.; Grußmayer, K.; Bostan, E.; Lukes, T.; Bouwens, A.; Sharipov, A.; Geissbuehler, S.; Mahul-Mellier, A.L.; Lashuel, H.; Leutenegger, M.; et al. Combined multi-plane phase retrieval and super-resolution optical fluctuation imaging for 4D cell microscopy. Nat. Photonics 2018, 12, 165–172. [Google Scholar] [CrossRef] [Green Version]
- Fienup, C.; Dainty, J. Phase retrieval and image reconstruction for astronomy. Image Recovery Theory Appl. 1987, 231, 275. [Google Scholar]
- Miao, J.; Ishikawa, T.; Robinson, I.K.; Murnane, M.M. Beyond crystallography: Diffractive imaging using coherent X-ray light sources. Science 2015, 348, 530–535. [Google Scholar] [CrossRef] [Green Version]
- Jiang, H.; Yuan, X.C.; Zhou, Y.; Chan, Y.; Lam, Y. Single-step fabrication of diffraction gratings on hybrid sol–gel glass using holographic interference lithography. Opt. Commun. 2000, 185, 19–24. [Google Scholar] [CrossRef]
- Guo, C.; Liu, S.; Sheridan, J.T. Iterative phase retrieval algorithms. I: Optimization. Appl. Opt. 2015, 54, 4698–4708. [Google Scholar] [CrossRef] [Green Version]
- Knapp, J.; Paulus, A.; Kornprobst, J.; Siart, U.; Eibert, T.F. Multi-Frequency Phase Retrieval for Antenna Measurements. IEEE Trans. Antennas Propag. 2020, 69, 488–501. [Google Scholar] [CrossRef]
- Gerchberg, R.W. A practical algorithm for the determination of phase from image and diffraction plane pictures. Optik 1972, 35, 237–246. [Google Scholar]
- Fienup, J.R. Reconstruction of an object from the modulus of its Fourier transform. Opt. Lett. 1978, 3, 27–29. [Google Scholar] [CrossRef]
- Fienup, J. Space object imaging through the turbulent atmosphere. Opt. Eng. 1979, 18, 185529. [Google Scholar] [CrossRef]
- Fienup, J.R. Phase retrieval algorithms: A comparison. Appl. Opt. 1982, 21, 2758–2769. [Google Scholar] [CrossRef] [Green Version]
- Bucci, O.M.; D’Elia, G.; Leone, G.; Pierri, R. Far-field pattern determination from the near-field amplitude on two surfaces. IEEE Trans. Antennas Propag. 1990, 38, 1772–1779. [Google Scholar] [CrossRef]
- Isernia, T.; Leone, G.; Pierri, R. Radiation pattern evaluation from near-field intensities on planes. IEEE Trans. Antennas Propag. 1996, 44, 701. [Google Scholar] [CrossRef]
- Bucci, O.M.; D’Elia, G.; Migliore, M.D. An effective near-field far-field transformation technique from truncated and inaccurate amplitude-only data. IEEE Trans. Antennas Propag. 1999, 47, 1377–1385. [Google Scholar] [CrossRef]
- Yaccarino, R.G.; Rahmat-Samii, Y. Phaseless bi-polar planar near-field measurements and diagnostics of array antennas. IEEE Trans. Antennas Propag. 1999, 47, 574–583. [Google Scholar] [CrossRef]
- Las-Heras, F.; Sarkar, T.K. A direct optimization approach for source reconstruction and NF-FF transformation using amplitude-only data. IEEE Trans. Antennas Propag. 2002, 50, 500–510. [Google Scholar] [CrossRef] [Green Version]
- Razavi, S.F.; Rahmat-Samii, Y. A new look at phaseless planar near-field measurements: Limitations, simulations, measurements, and a hybrid solution. IEEE Antennas Propag. Mag. 2007, 49, 170–178. [Google Scholar] [CrossRef]
- Alvarez, Y.; Las-Heras, F.; Pino, M.R. The sources reconstruction method for amplitude-only field measurements. IEEE Trans. Antennas Propag. 2010, 58, 2776–2781. [Google Scholar] [CrossRef]
- Candes, E.J.; Strohmer, T.; Voroninski, V. Phaselift: Exact and stable signal recovery from magnitude measurements via convex programming. Commun. Pure Appl. Math. 2013, 66, 1241–1274. [Google Scholar] [CrossRef] [Green Version]
- Candes, E.J.; Eldar, Y.C.; Strohmer, T.; Voroninski, V. Phase retrieval via matrix completion. SIAM Rev. 2015, 57, 225–251. [Google Scholar] [CrossRef]
- Waldspurger, I.; d’Aspremont, A.; Mallat, S. Phase recovery, maxcut and complex semidefinite programming. Math. Program. 2015, 149, 47–81. [Google Scholar] [CrossRef] [Green Version]
- Xu, Y.; Ye, Q.; Hoorfar, A.; Meng, G. Extrapolative phase retrieval based on a hybrid of PhaseCut and alternating projection techniques. Opt. Lasers Eng. 2019, 121, 96–103. [Google Scholar] [CrossRef]
- Bahmani, S.; Romberg, J. Phase retrieval meets statistical learning theory: A flexible convex relaxation. In Proceedings of the 20th International Conference on Artificial Intelligence and Statistics, Fort Lauderdale, FL, USA, 20–22 April 2017; pp. 252–260. [Google Scholar]
- Goldstein, T.; Studer, C. Convex phase retrieval without lifting via PhaseMax. In Proceedings of the International Conference on Machine Learning, Sydney, Australia, 6–11 August 2017; pp. 1273–1281. [Google Scholar]
- Candes, E.J.; Li, X.; Soltanolkotabi, M. Phase retrieval via Wirtinger flow: Theory and algorithms. IEEE Trans. Inf. Theory 2015, 61, 1985–2007. [Google Scholar] [CrossRef] [Green Version]
- Wang, G.; Giannakis, G.B.; Eldar, Y.C. Solving systems of random quadratic equations via truncated amplitude flow. IEEE Trans. Inf. Theory 2017, 64, 773–794. [Google Scholar] [CrossRef]
- Goldstein, T.; Studer, C. Phasemax: Convex phase retrieval via basis pursuit. IEEE Trans. Inf. Theory 2018, 64, 2675–2689. [Google Scholar] [CrossRef]
- Wang, G.; Giannakis, G.B.; Saad, Y.; Chen, J. Phase retrieval via reweighted amplitude flow. IEEE Trans. Signal Process. 2018, 66, 2818–2833. [Google Scholar] [CrossRef]
- Brown, T.; Jeffrey, I.; Mojabi, P. Multiplicatively regularized source reconstruction method for phaseless planar near-field antenna measurements. IEEE Trans. Antennas Propag. 2017, 65, 2020–2031. [Google Scholar] [CrossRef]
- Morabito, A.F.; Palmeri, R.; Morabito, V.A.; Laganà, A.R.; Isernia, T. Single-surface phaseless characterization of antennas via hierarchically ordered optimizations. IEEE Trans. Antennas Propag. 2018, 67, 461–474. [Google Scholar] [CrossRef]
- Xie, Z.X.; Zhang, Y.H.; He, S.Y.; Zhu, G.Q. A novel method for source reconstruction based on spherical wave expansion and NF-FF transformation using amplitude-only data. IEEE Trans. Antennas Propag. 2019, 67, 4756–4767. [Google Scholar] [CrossRef]
- Capozzoli, A.; D’Elia, G.; Liseno, A. Phaseless characterisation of compact antenna test ranges. IET Microwaves Antennas Propag. 2007, 1, 860–866. [Google Scholar] [CrossRef]
- Chen, Y.; Yao, Y.; Yu, J.; Chen, X. Iteration-phase retrieval for quiet zone of compact antenna test ranges. In Proceedings of the 2019 Computing, Communications and IoT Applications (ComComAp), Shenzhen, China, 26–28 October 2019; IEEE: Piscataway, NJ, USA, 2019; pp. 36–39. [Google Scholar]
- Combettes, P. The convex feasibility problem in image recovery. In Advances in Imaging and Electron Physics; Elsevier: Amsterdam, The Netherlands, 1996; Volume 95, pp. 155–270. [Google Scholar]
- Stark, H. Image Recovery: Theory and Application; Elsevier: Amsterdam, The Netherlands, 2013. [Google Scholar]
- Bauschke, H.H.; Combettes, P.L.; Luke, D.R. Hybrid projection—Reflection method for phase retrieval. JOSA A 2003, 20, 1025–1034. [Google Scholar] [CrossRef]
- Yi, H.; Qu, S.W.; Ng, K.B.; Chan, C.H.; Bai, X. 3-D printed millimeter-wave and terahertz lenses with fixed and frequency scanned beam. IEEE Trans. Antennas Propag. 2015, 64, 442–449. [Google Scholar] [CrossRef]
- Fienup, J.; Wackerman, C. Phase-retrieval stagnation problems and solutions. JOSA A 1986, 3, 1897–1907. [Google Scholar] [CrossRef]
- Levi, A.; Stark, H. Image restoration by the method of generalized projections with application to restoration from magnitude. JOSA A 1984, 1, 932–943. [Google Scholar] [CrossRef]
- Lions, P.L.; Mercier, B. Splitting algorithms for the sum of two nonlinear operators. SIAM J. Numer. Anal. 1979, 16, 964–979. [Google Scholar] [CrossRef]
- Wang, H.; Yue, W.; Song, Q.; Liu, J.; Situ, G. A hybrid Gerchberg—Saxton-like algorithm for DOE and CGH calculation. Opt. Lasers Eng. 2017, 89, 109–115. [Google Scholar] [CrossRef] [Green Version]



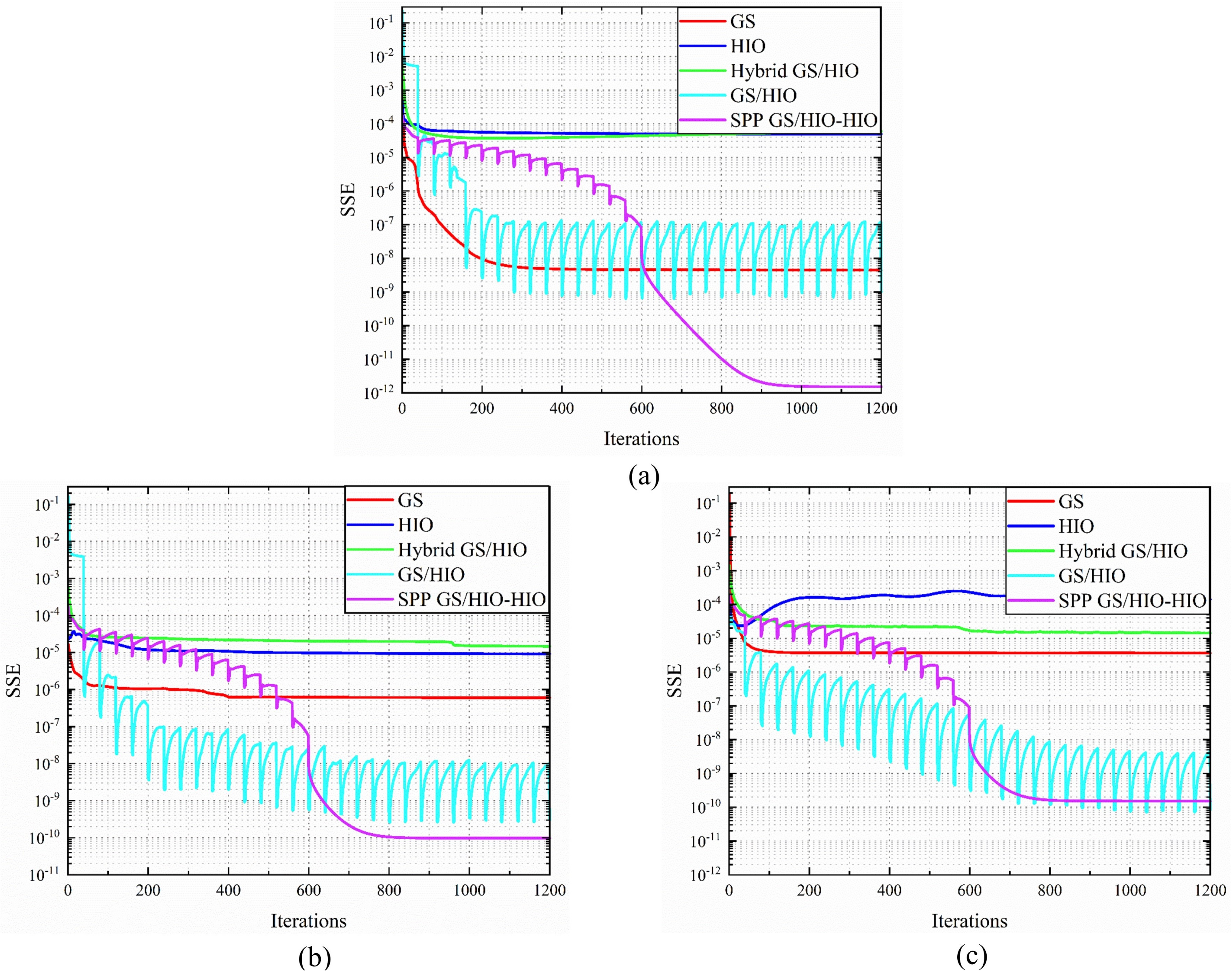
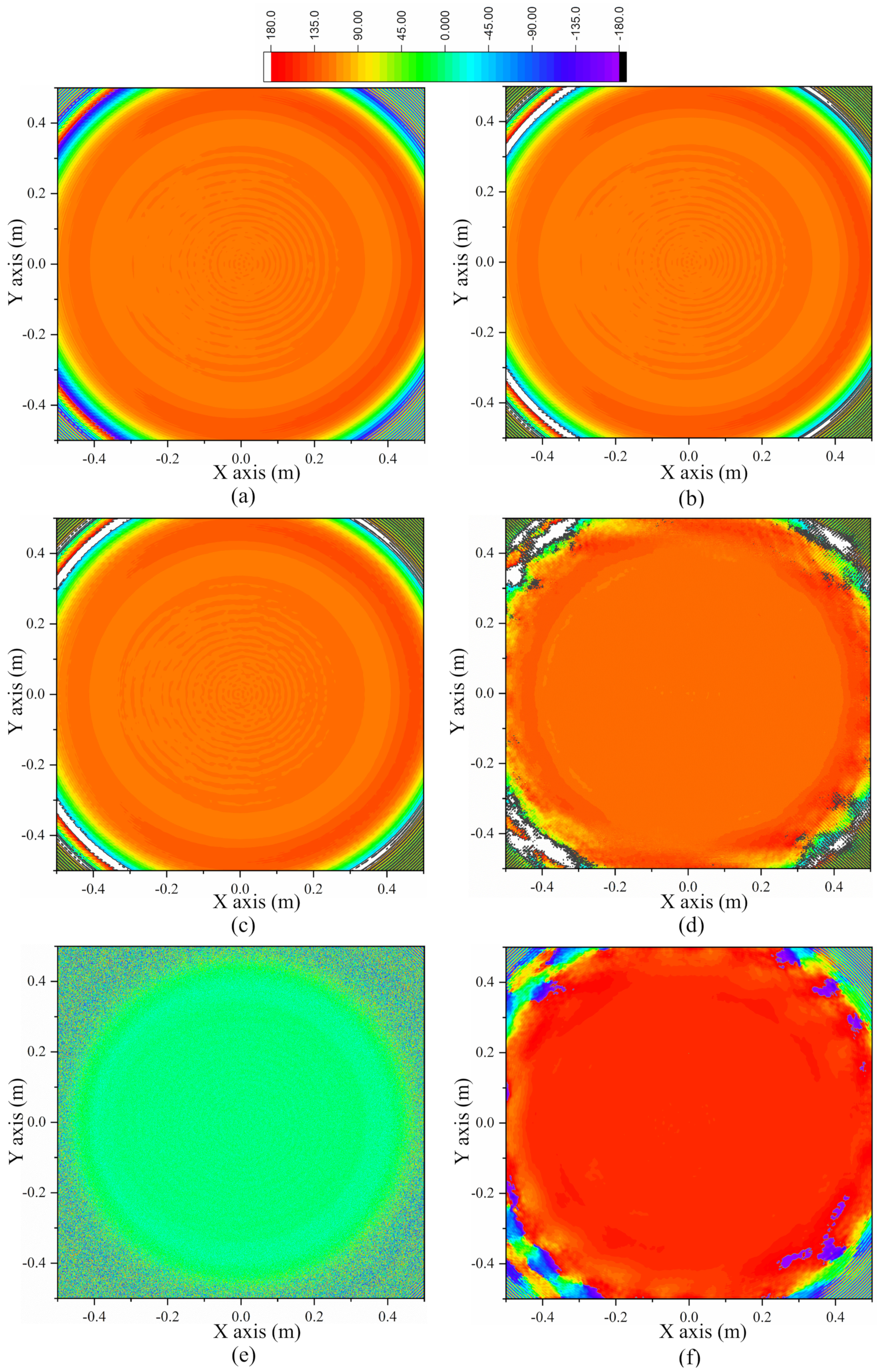

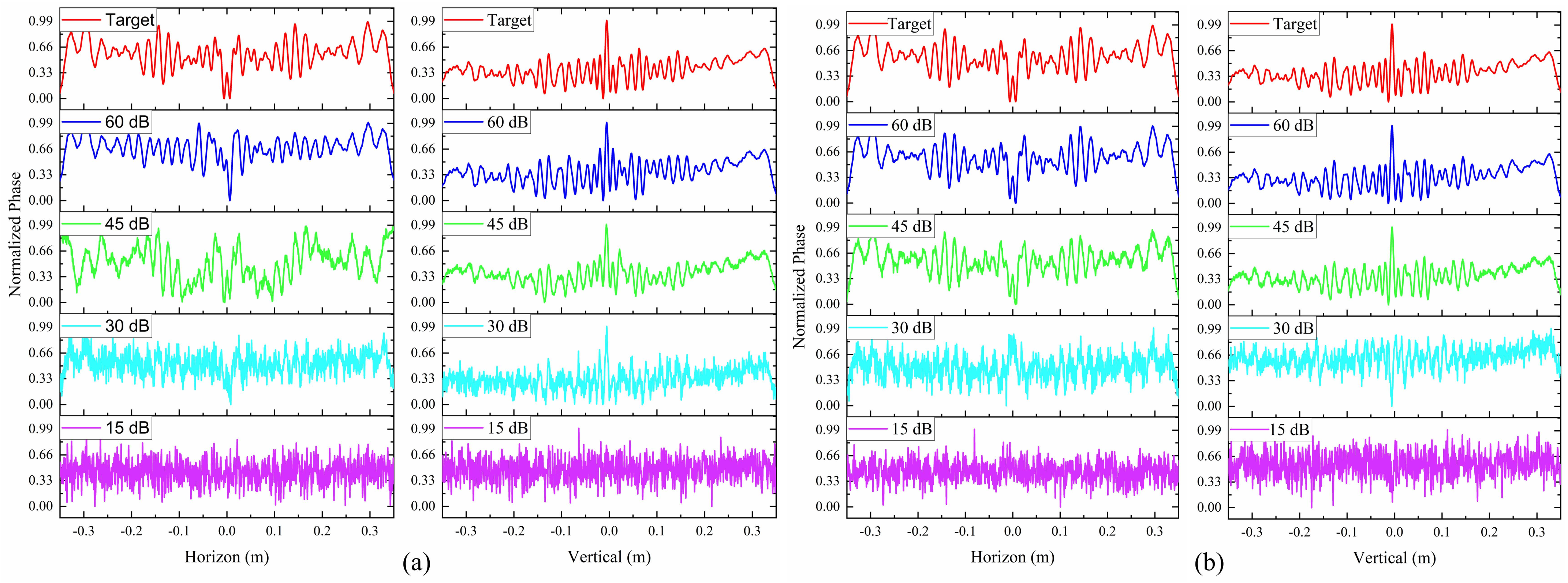


| Algorithm | 75 GHz | 135 GHz | 220 GHz |
|---|---|---|---|
| GS | 2.8 × 101 | 3.45 × 10−1 | 8.3 × 10−2 |
| HIO | 6.53 × 100 | 5 × 10−2 | 6.1 × 10−2 |
| Hybrid GS/HIO | 1.794 × 101 | 6.9 × 10−1 | 1.03 × 10−1 |
| GS/HIO | 1.7 × 10−3 | 3 × 10−3 | 1.1 × 10−2 |
| SPP GS/HIO-HIO | 1.32 × 10−5 | 6.54 × 10−4 | 9.66 × 10−4 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, Y.; Yao, Y.; Zhu, L.; Yu, H.; Cheng, X.; Yu, J.; Chen, X. Phaseless Characterization of Compact Antenna Test Range via Improved Alternating Projection Algorithm. Electronics 2021, 10, 1545. https://doi.org/10.3390/electronics10131545
Chen Y, Yao Y, Zhu L, Yu H, Cheng X, Yu J, Chen X. Phaseless Characterization of Compact Antenna Test Range via Improved Alternating Projection Algorithm. Electronics. 2021; 10(13):1545. https://doi.org/10.3390/electronics10131545
Chicago/Turabian StyleChen, Yuqing, Yuan Yao, Lei Zhu, Haiyang Yu, Xiaohe Cheng, Junsheng Yu, and Xiaodong Chen. 2021. "Phaseless Characterization of Compact Antenna Test Range via Improved Alternating Projection Algorithm" Electronics 10, no. 13: 1545. https://doi.org/10.3390/electronics10131545
APA StyleChen, Y., Yao, Y., Zhu, L., Yu, H., Cheng, X., Yu, J., & Chen, X. (2021). Phaseless Characterization of Compact Antenna Test Range via Improved Alternating Projection Algorithm. Electronics, 10(13), 1545. https://doi.org/10.3390/electronics10131545







