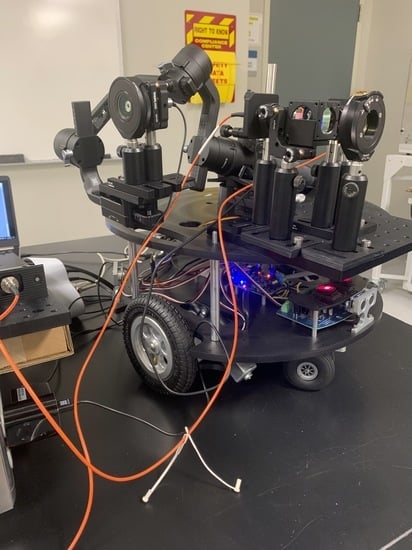
Quantum Mechatronics
Abstract
:1. Introduction
1.1. Mechatronics Systems
- Integrated.
- Unified.
- Unique.
- Systematic.
- Optimality and better component matching.
- Increased efficiency.
- Cost effectiveness.
- Ease of system integration and expansion/enhancement.
- Compatibility and ease of cooperation with other systems.
- Improved controllability.
- Increased reliability.
- Increased product life.
1.2. Quantum Technologies
1.3. Quantum Mechatronics
2. Quantum Technologies in Robotics Systems
3. Macroscopic Actuators via Quantum Effects
4. Education in Quantum Mechatronics
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- De Silva, C.W. Mechatronic Modeling and Domain Transformation of Multi-physics Systems. Inst. J. 2021, 8, 14. [Google Scholar]
- De Silva, C.W. Modeling of Dynamic Systems—With Engineering Applications; Taylor & Francis/CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- De Silva, C.W. A systematic approach for modeling multi-physics systems. Int. J. Mech. Eng. Educ. 2021, 49, 122–150. [Google Scholar] [CrossRef]
- De Silva, C.W. Minimal Realization of Linear Graph Models for Multi-physics Systems. Inst. J. 2019, 6, 72. [Google Scholar]
- Nielsen, M.A.; Chuang, I.L. Quantum Computation and Quantum Information; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- O’Brien, J.L.; Furusawa, A.; Vucković, J. Photonic quantum technologies. Nat. Phot. 2009, 3, 687. [Google Scholar] [CrossRef] [Green Version]
- Xu, F.; Ma, X.; Zhang, Q.; Lo, H.-K.; Pan, J.-W. Secure quantum key distribution with realistic devices. Rev. Mod. Phys. 2020, 92, 025002. [Google Scholar] [CrossRef]
- Liu, L.-Z.; Zhang, Y.-Z.; Li, Z.-D.; Zhang, R.; Yin, X.-F.; Fei, Y.-Y.; Li, L.; Liu, N.-L.; Xu, F.; Chen, Y.-A.; et al. Distributed quantum phase estimation with entangled photons. Nat. Photon 2021, 15, 137–142. [Google Scholar] [CrossRef]
- Khabiboulline, E.T.; Borregaard, J.; De Greve, K.; Lukin, M.D. Optical Interferometry with Quantum Networks. Phys. Rev. Lett. 2019, 123, 070504. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Luo, W. Distributed environmental modeling and adaptive sampling for multi-robot sensor coverage. In Proceedings of the 18th International Conference on Autonomous Agents and MultiAgent Systems, Montreal, QC, Canada, 13–17 May 2019. [Google Scholar]
- Meaney, C. Quantum Mechatronics. Ph.D. Thesis, The University of Queensland, Brisbane, Australia, 2011. [Google Scholar]
- Khoshnoud, F.; Esat, I.I.; De Silva, C.W.; Quadrelli, M.B. Quantum Network of Cooperative Unmanned Autonomous Systems. Unmanned Syst. 2019, 7, 137–145. [Google Scholar] [CrossRef]
- Khoshnoud, F.; Quadrelli, M.B.; Esat, I.I.; Robinson, D. Quantum Cooperative Robotics and Autonomy. Spec. Issue Instrum. J. 2019, 6, 93–111. [Google Scholar]
- Khoshnoud, F.; Esat, I.I.; Javaherian, S.; Bahr, B. Quantum Entanglement and Cryptography for Automation and Control of Dynamic Systems. Spec. Issue Instrum. J. 2019, 6, 109–127. [Google Scholar]
- Khoshnoud, F.; Lamata, L.; de Silva, C.W.; Quadrelli, M.B. Quantum teleportation for control of dynamical systems and autonomy. Mechatron. Syst. Control. 2021, 49, 124. [Google Scholar]
- Bennett, C.H.; Brassard, G.; Crépeau, C.; Jozsa, R.; Peres, A.; Wootters, W.K. Teleporting an unknown quantum state via dual classical and Einstein-Podolsky-Rosen channels. Phys. Rev. Lett. 1993, 70, 1895–1899. [Google Scholar] [CrossRef] [Green Version]
- Khoshnoud, F.; Ghazinejad, M. Automated quantum entanglement and cryptography for networks of robotic systems. In Proceedings of the IEEE/ASME International Conference on Mechatronic and Embedded Systems and Applications (MESA), IDETC-CIE 2021, Virtual Conference, 17–20 August 2021. [Google Scholar]
- Kim, J.-H.; Aghaeimeibodi, S.; Carolan, J.; Englund, D.; Waks, E. Hybrid integration methods for on-chip quantum photonics. Optica 2020, 7, 291–308. [Google Scholar] [CrossRef] [Green Version]
- Sun, C.; Wade, M.T.; Lee, Y.; Orcutt, J.S.; Alloatti, L.; Georgas, M.S.; Waterman, A.S.; Shainline, J.M.; Avizienis, R.R.; Lin, S.; et al. Single-chip microprocessor that communicates directly using light. Nature 2015, 528, 534. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Gentry, C.M.; Shainline, J.M.; Wade, M.T.; Stevens, M.J.; Dyer, S.D.; Zeng, X.; Pavanello, F.; Gerrits, T.; Nam, S.W.; Mirin, R.P.; et al. Quantum-correlated photon pairs, generated in a commercial 45 nm complementary metal-oxide semiconductor microelectronic chip. Optica 2015, 2, 1065. [Google Scholar] [CrossRef]
- Cabanillas, J.M.F.; Kramnik, D.; Ramesh, A.; Gentry, C.M.; Stojanović, V.; Kumar, P.; Popovic, M.A. Tunable Source of Quantum-Correlated Photons with Integrated Pump Rejection in a Silicon CMOS Platform. Presented at FiO+LS’2021, Frontiers in Optics & Laser Science Conference, Washington, DC, USA, 31 October–4 November 2021. [Google Scholar]
- Shapiro, J.H. Scintillation has minimal impact on far-field Bennett-Brassard 1984 protocol quantum key distribution. Phys. Rev. A 2011, 84, 032340. [Google Scholar] [CrossRef]
- Vetelino, F.S.; Young, C.; Andrews, L. Fade statistics and aperture averaging for Gaussian beam waves in moderate-to-strong turbulence. Appl. Opt. 2007, 46, 3780–3789. [Google Scholar] [CrossRef] [PubMed]
- Lee, K.F.; Reilly, D.R.; Moraw, P.; Kanter, G.S. Emulation of up-conversion based quantum key distribution scheme using active pump-controlled basis selection and adaptive thresholding. Opt. Commun. 2020, 475, 126258. [Google Scholar] [CrossRef]
- Erven, C.; Heim, B.; Meyer-Scott, E.; Bourgoin, J.P.; Laflamme, R.; Weihs, G.; Jennewein, T. Studying free-space transmission statistics and improving free-space quantum key distribution in the turbulent atmosphere. New J. Phys. 2012, 14, 123018. [Google Scholar] [CrossRef]
- Aspelmeyer, M.; Kippenberg, T.J.; Marquardt, F. Cavity optomechanics. Rev. Mod. Phys. 2014, 86, 1391. [Google Scholar] [CrossRef]
- Scully, M.O. Zubairy, M.S. Quantum Optics; Cambridge University Press: Cambridge, UK, 2001. [Google Scholar]
- Ashkin, A. Acceleration and Trapping of Particles by Radiation Pressure. Phys. Rev. Lett. 1970, 24, 156–159. [Google Scholar] [CrossRef] [Green Version]
- Shvedov, V.; Rode, A.; Izdebskaya, Y.V.; Desyatnikov, A.S.; Krolikowski, W.; Kivshar, Y.S. Giant Optical Manipulation. Phys. Rev. Lett. 2010, 105, 118103v. [Google Scholar] [CrossRef] [PubMed]
- Quadrelli, M.B.; Sidick, E.; Basinger, S.A. Unconventional imaging with contained granular media. In Proceedings of the Unconventional and Indirect Imaging, Image Reconstruction, and Wavefront Sensing, San Diego, CA, USA, 9–10 August 2017. [Google Scholar]
- Khoshnoud, F.; de Silva, C.W.; Quadrelli, M.B.; Lamata, L.; Bahr, B.; Aiello, C.D.; Padhi, S.; Esat, I.I.; Ghazinejad, M. Modernizing Mechatronics Course with Quantum Engineering. In Proceedings of the American Society for Engineering Education PSW 2021 Conference, Virtual Conference, 23–25 April 2021. [Google Scholar]
- Khoshnoud, F.; Robinson, D.; de Silva, C.W.; Esat, I.I.; Bonser, R.H.C.; Quadrelli, M.B. Research-informed service-learning in Mechatronics and Dynamic Systems. In Proceedings of the American Society for Engineering Education Conference, Los Angeles, CA, USA, 4–5 April 2019. [Google Scholar]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lamata, L.; Quadrelli, M.B.; de Silva, C.W.; Kumar, P.; Kanter, G.S.; Ghazinejad, M.; Khoshnoud, F. Quantum Mechatronics. Electronics 2021, 10, 2483. https://doi.org/10.3390/electronics10202483
Lamata L, Quadrelli MB, de Silva CW, Kumar P, Kanter GS, Ghazinejad M, Khoshnoud F. Quantum Mechatronics. Electronics. 2021; 10(20):2483. https://doi.org/10.3390/electronics10202483
Chicago/Turabian StyleLamata, Lucas, Marco B. Quadrelli, Clarence W. de Silva, Prem Kumar, Gregory S. Kanter, Maziar Ghazinejad, and Farbod Khoshnoud. 2021. "Quantum Mechatronics" Electronics 10, no. 20: 2483. https://doi.org/10.3390/electronics10202483
APA StyleLamata, L., Quadrelli, M. B., de Silva, C. W., Kumar, P., Kanter, G. S., Ghazinejad, M., & Khoshnoud, F. (2021). Quantum Mechatronics. Electronics, 10(20), 2483. https://doi.org/10.3390/electronics10202483