Collaborative Optimization Method of Power and Efficiency for LCC-S Wireless Power Transmission System
Abstract
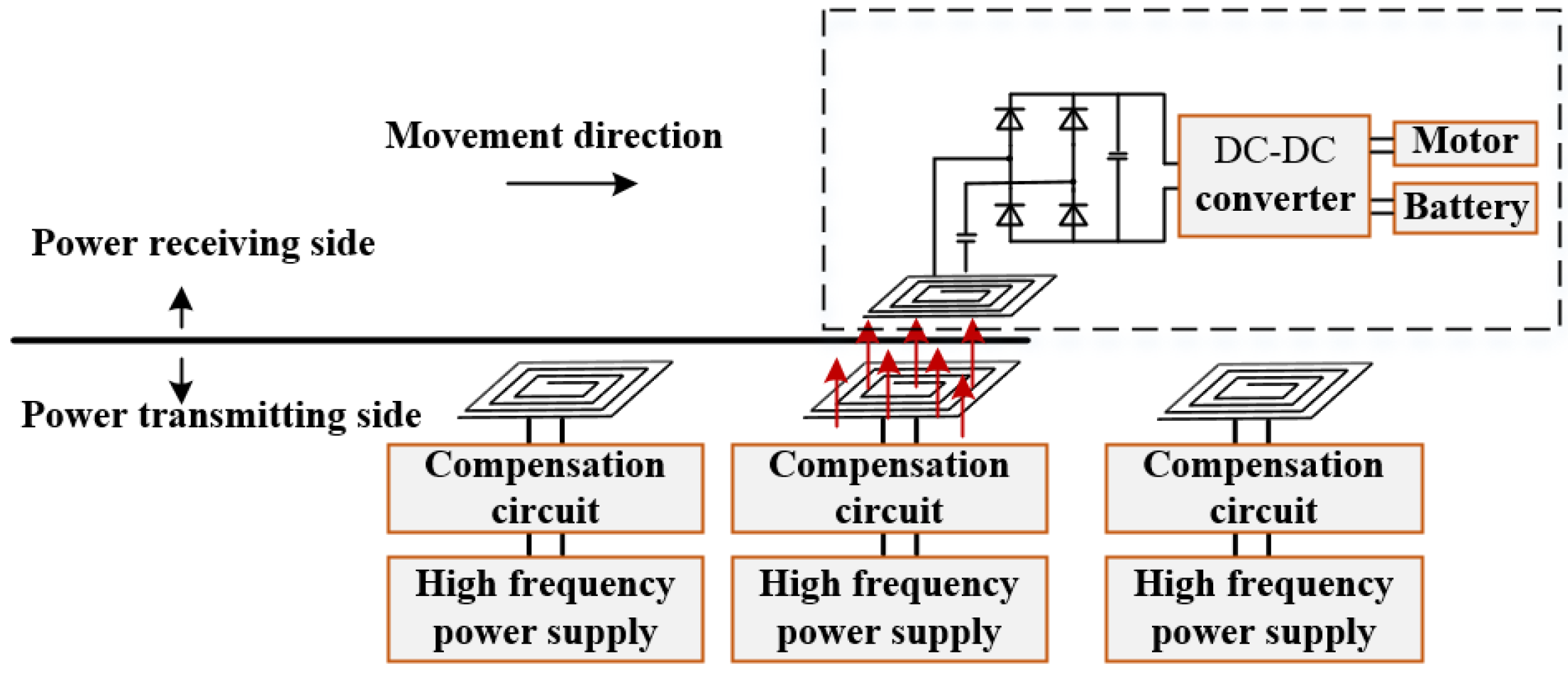
:1. Introduction
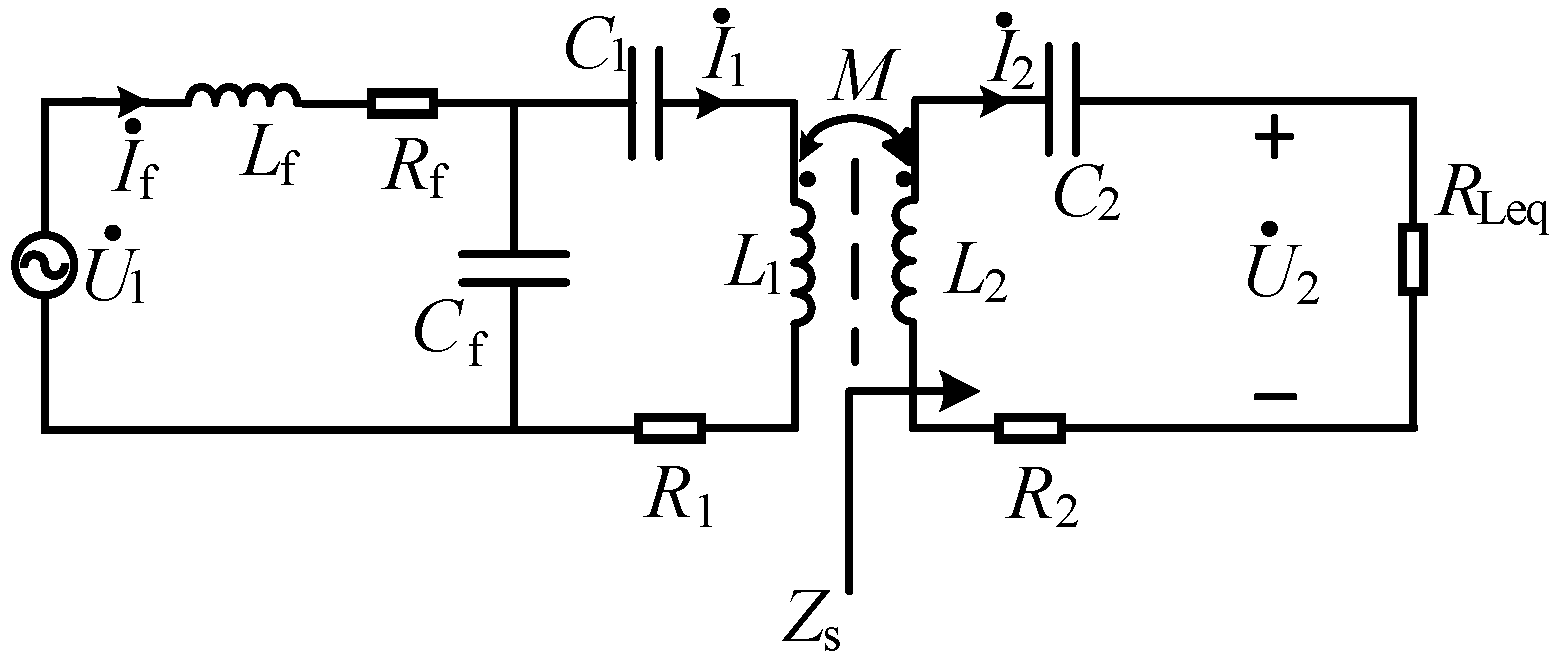
2. Analysis of Mathematical Model of LCC-S Compensation Topology
2.1. Theoretical Analysis
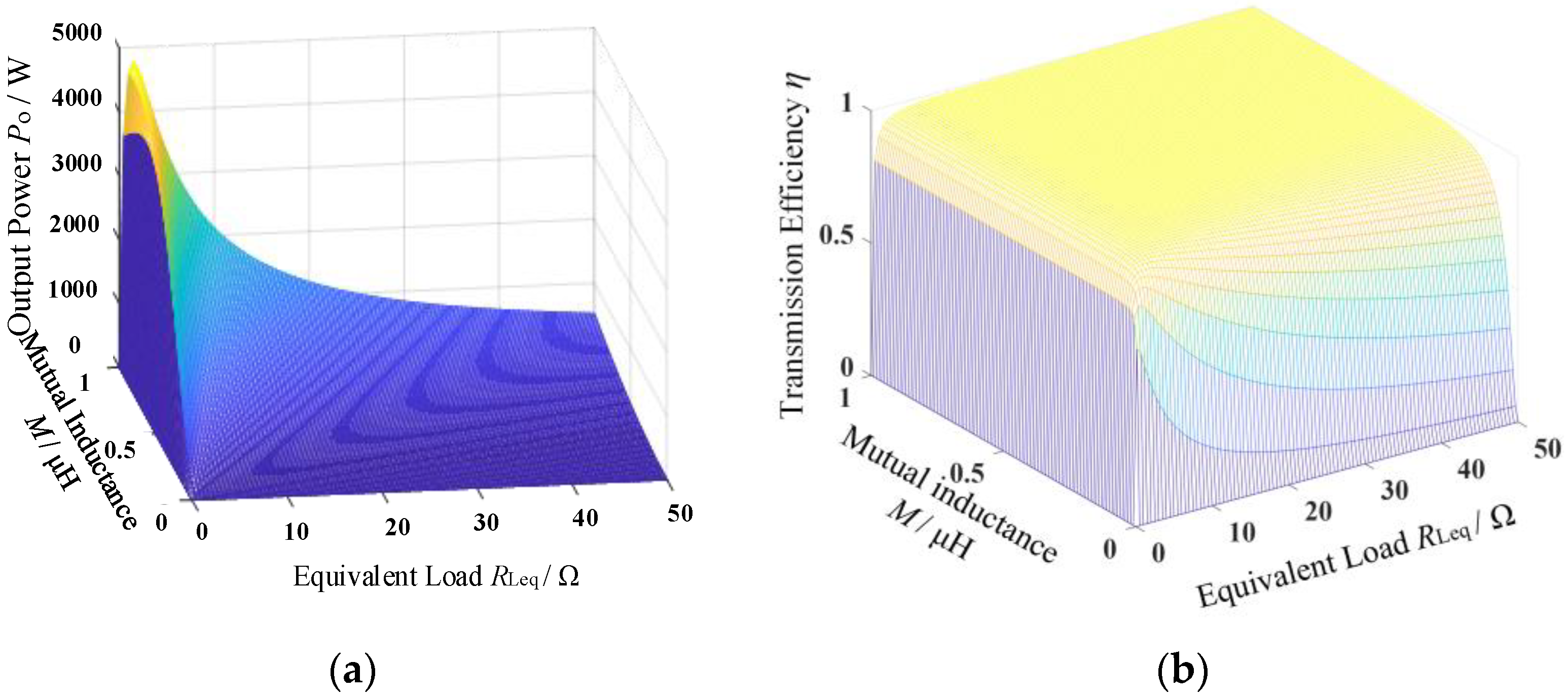
2.2. Analysis of Influencing Factors of System Power and Efficiency
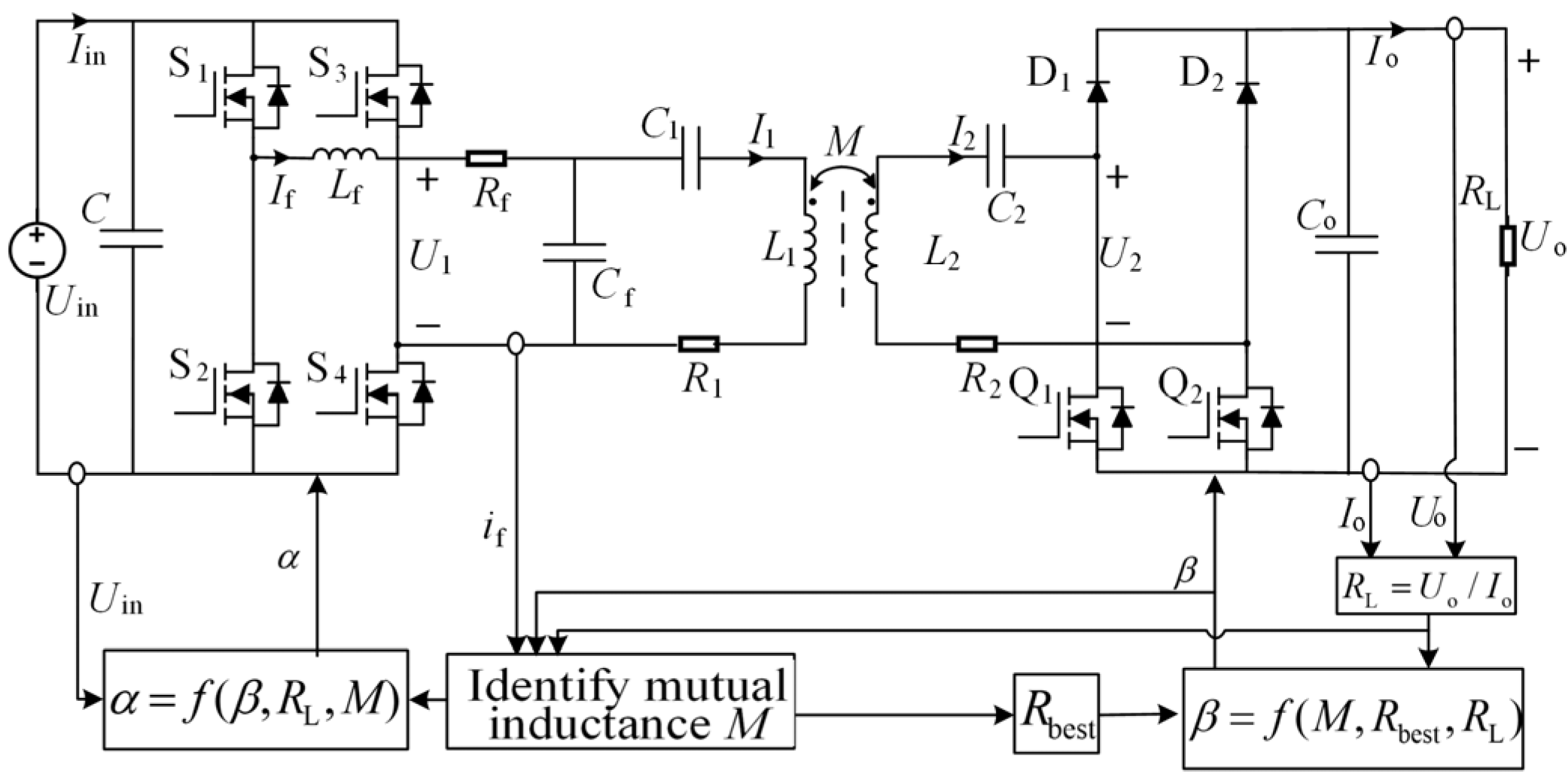
3. Research on Control Strategy of Efficacy System
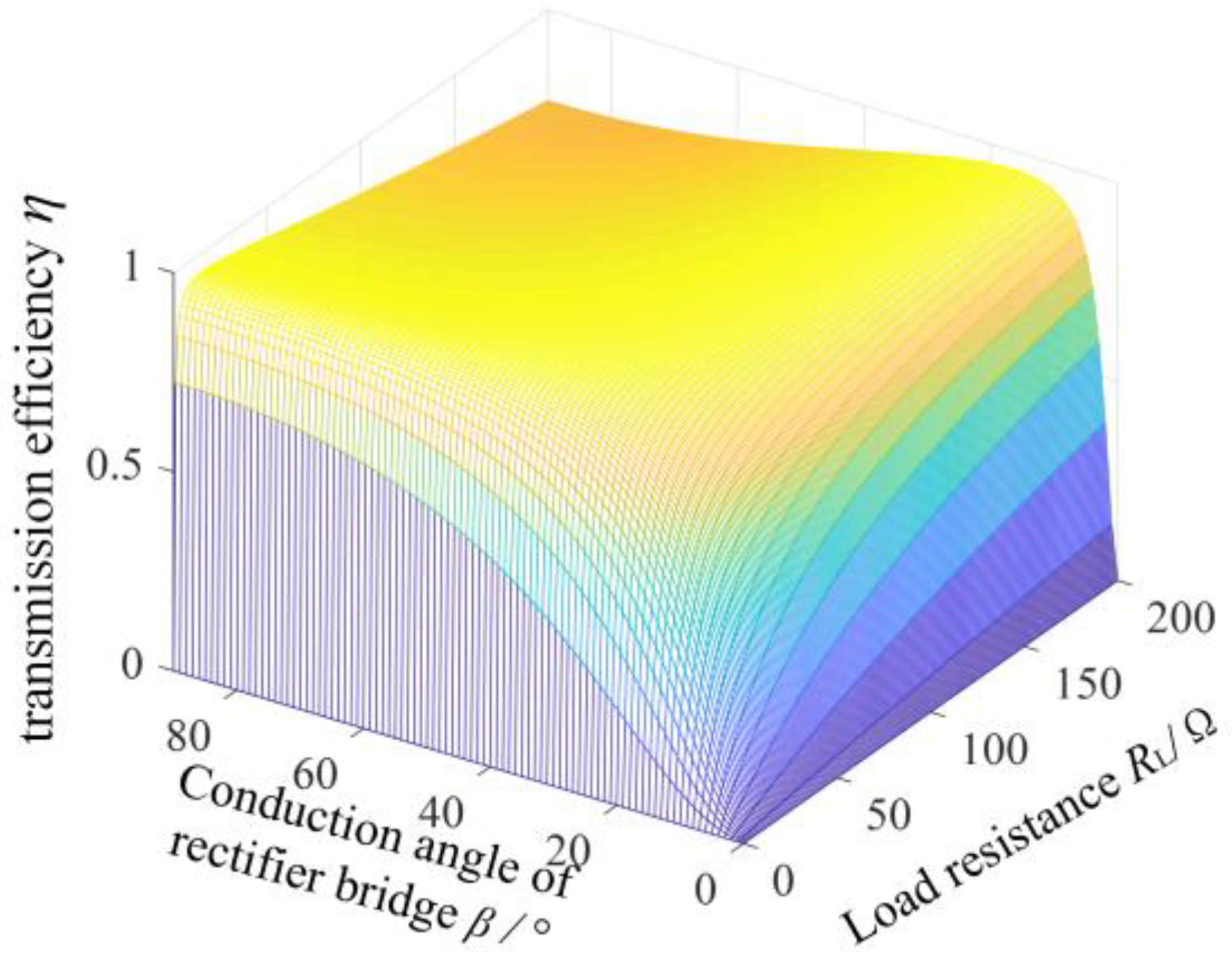
3.1. Optimal Equivalent Load Analysis under Maximum Efficiency
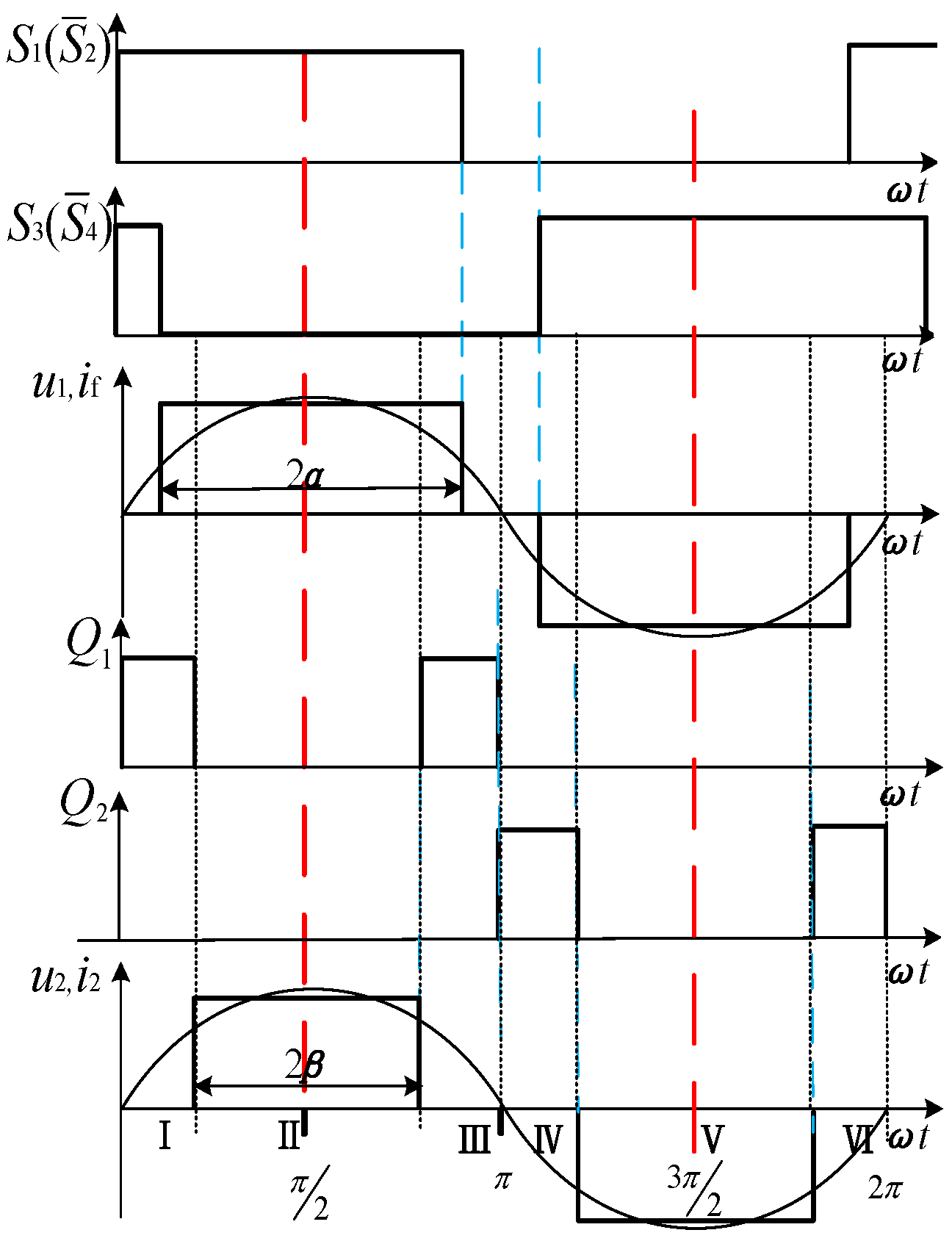
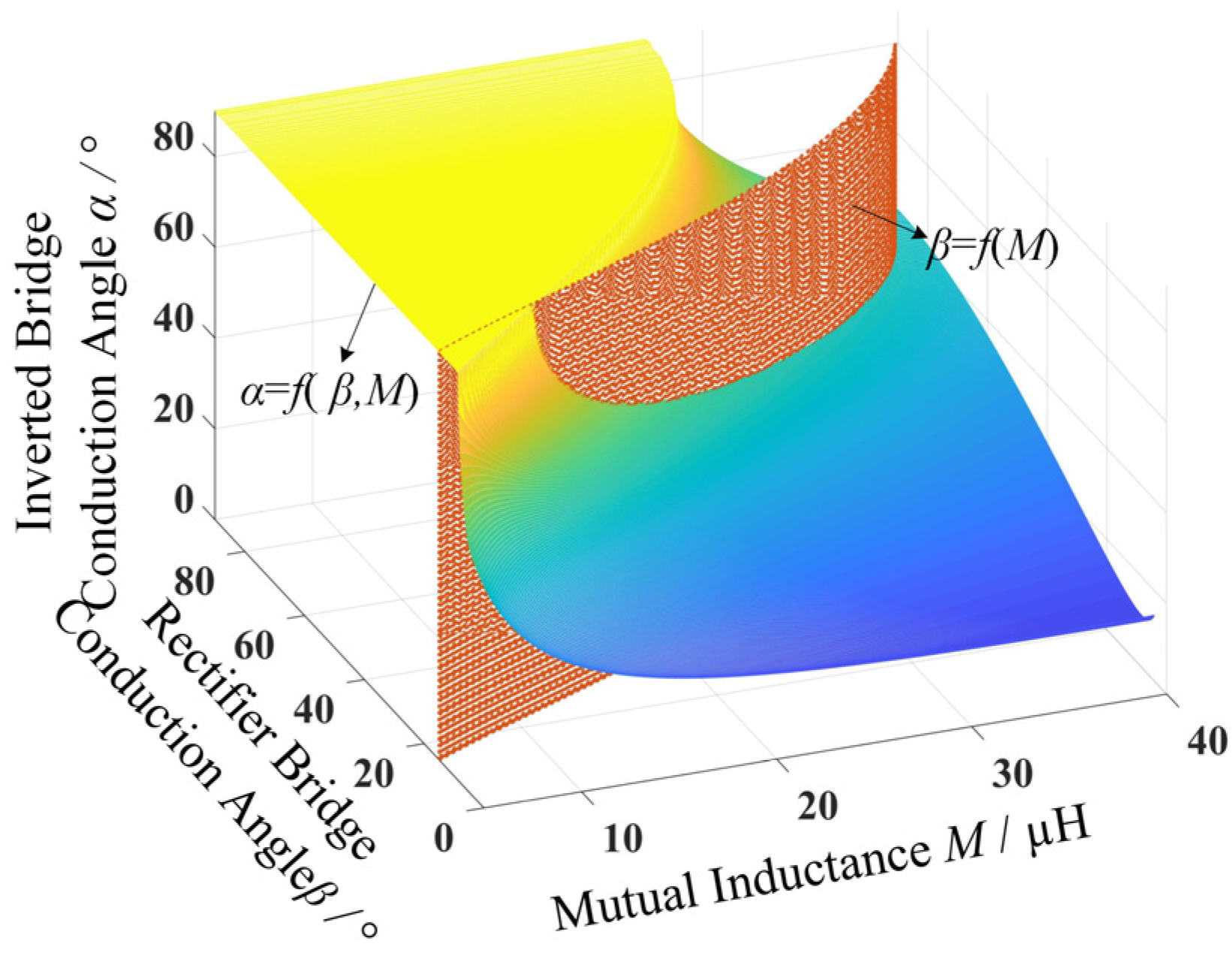
3.2. Transmission Efficiency and Receiving Power Control Strategy
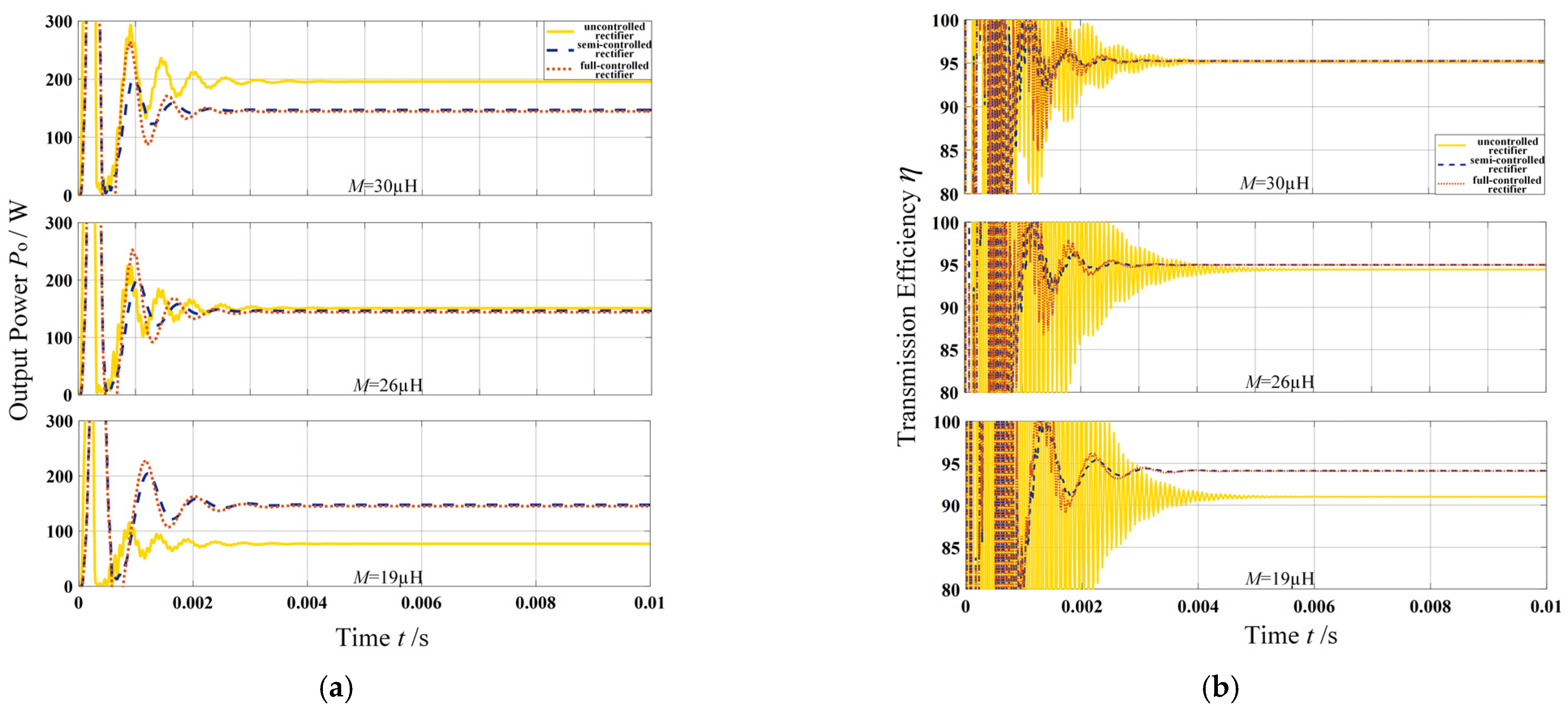
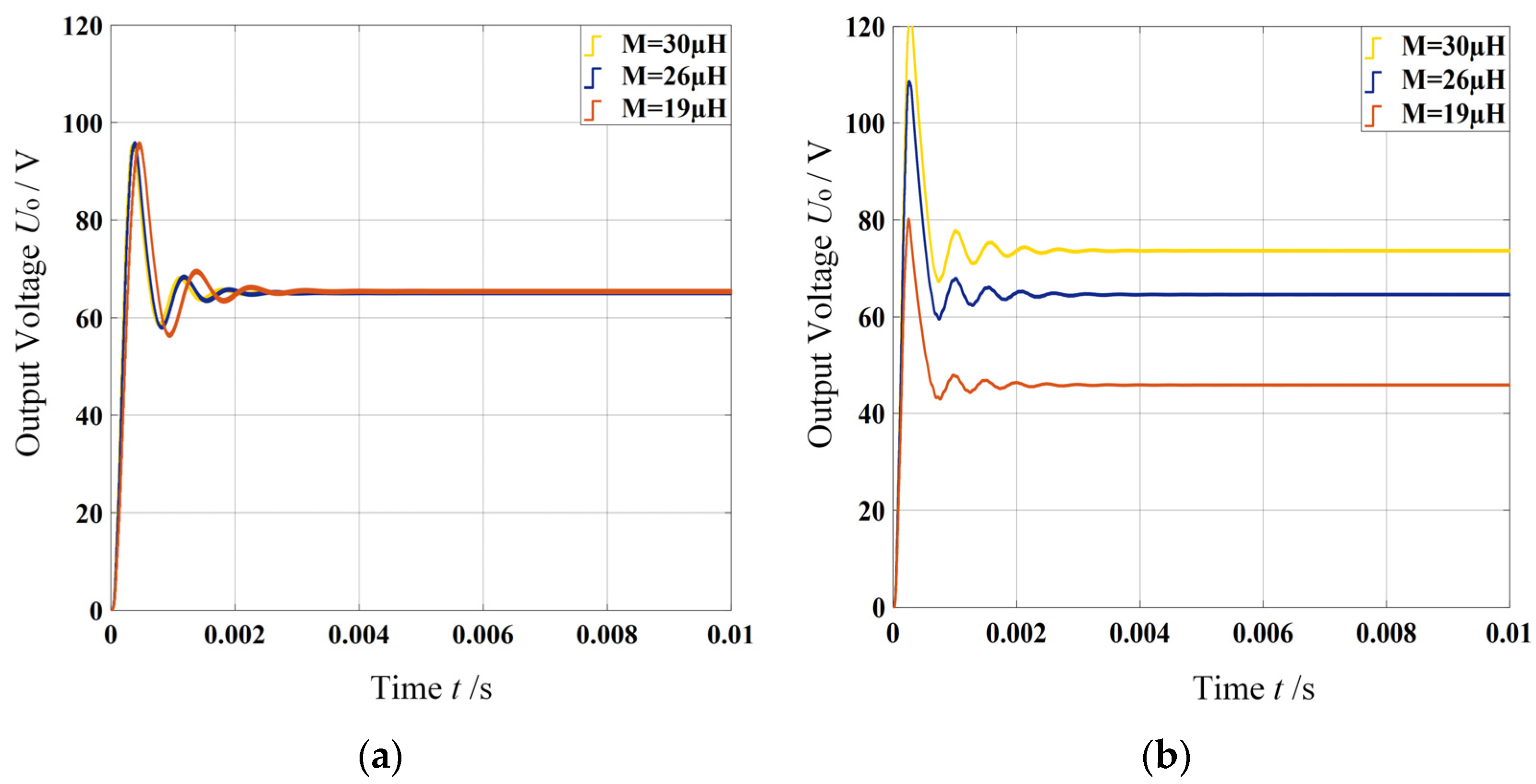
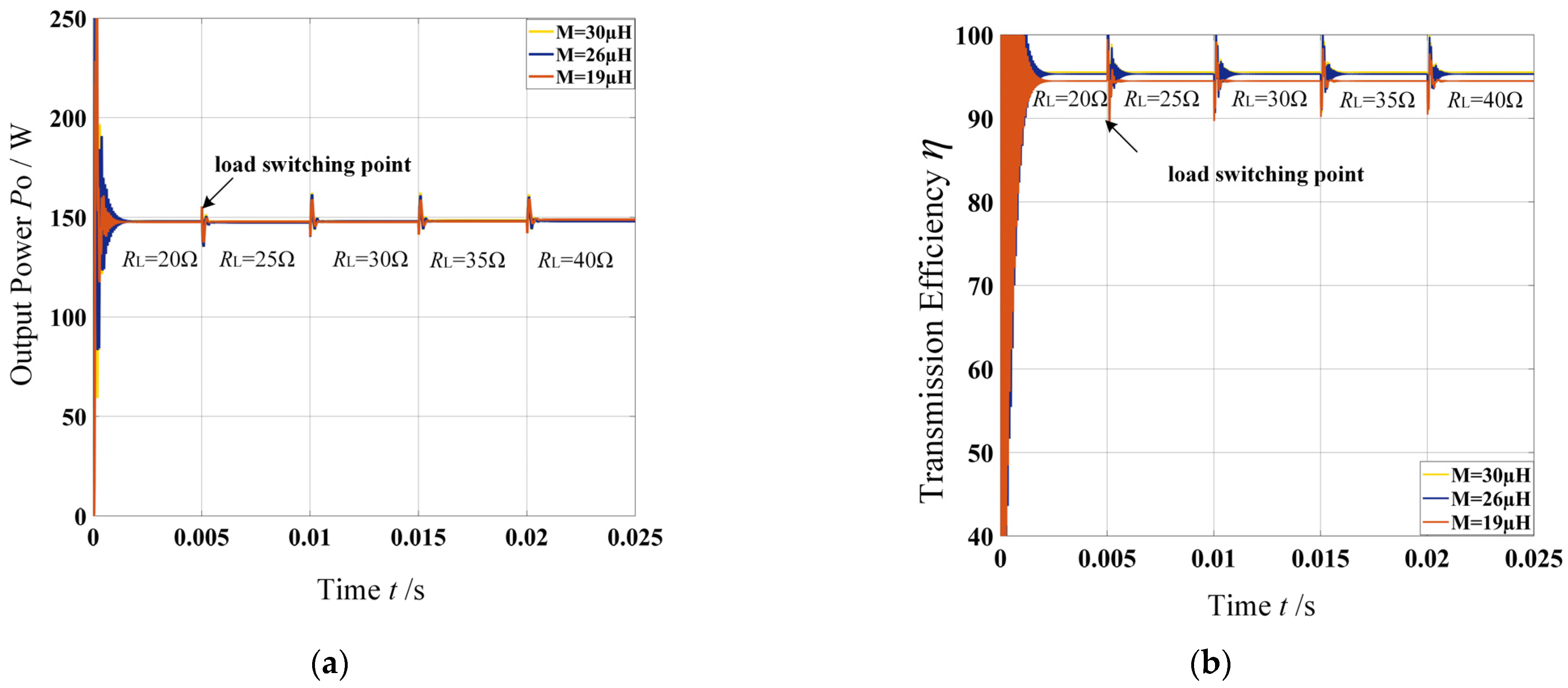
3.3. Simulation Analysis
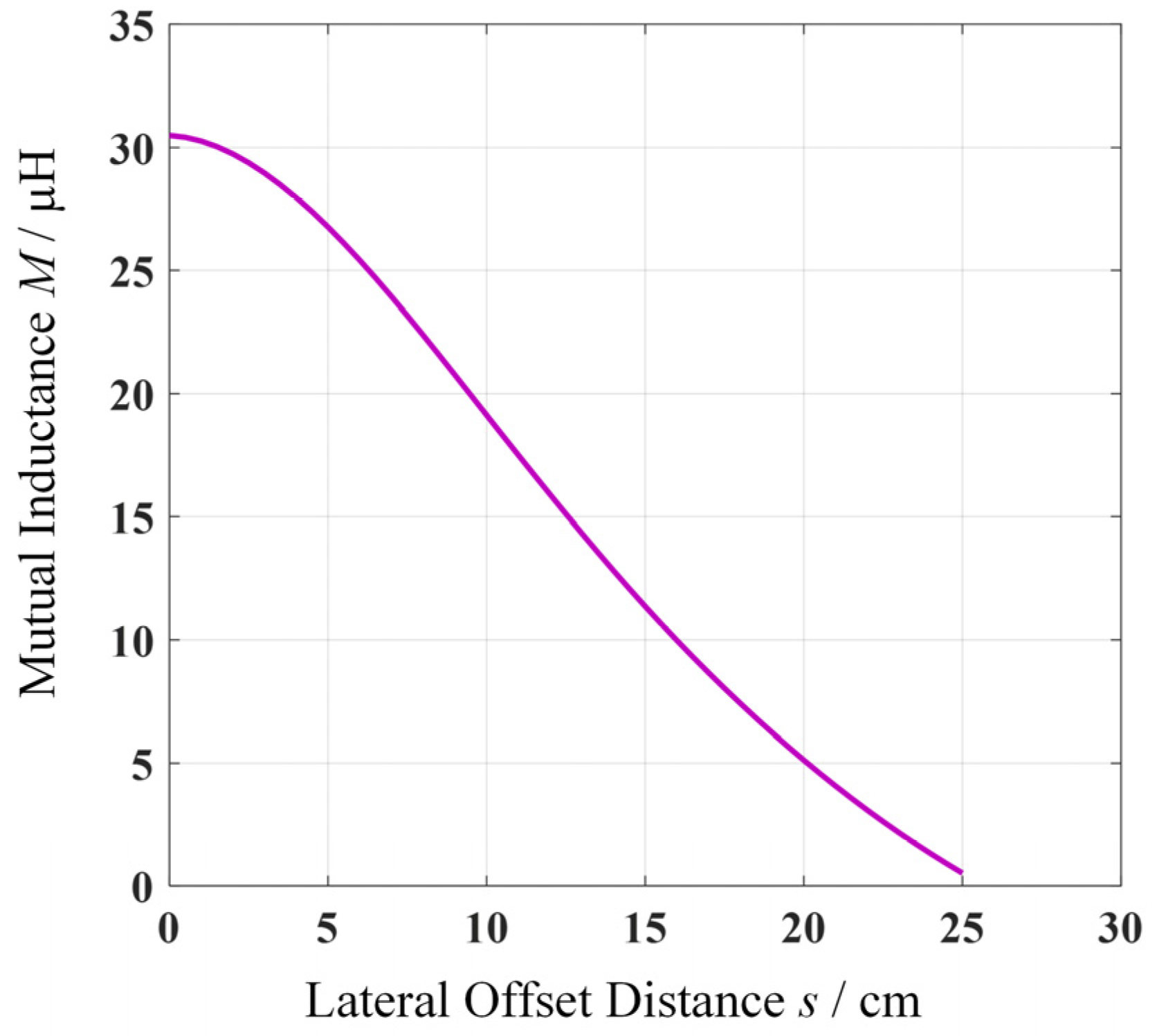
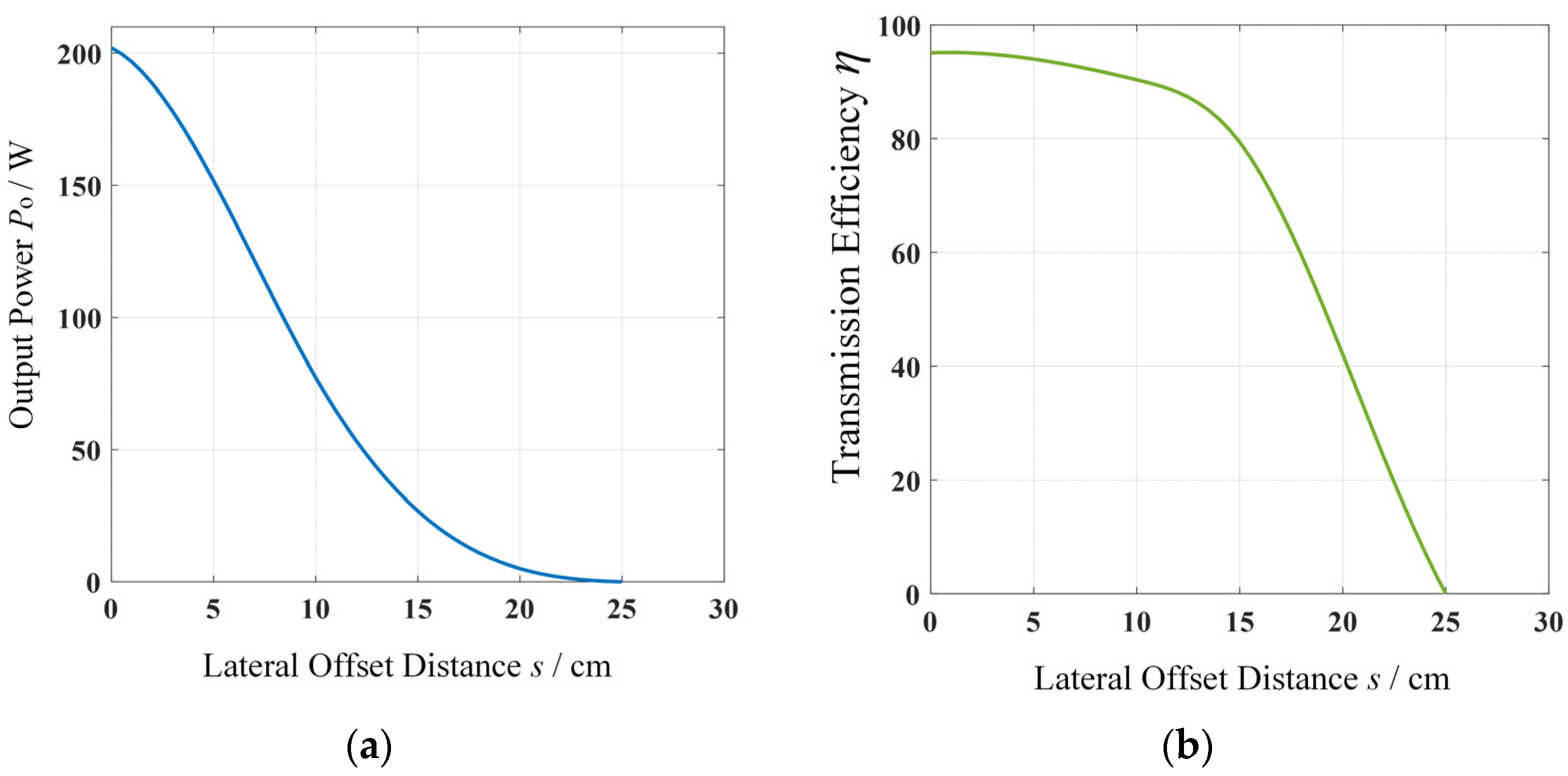
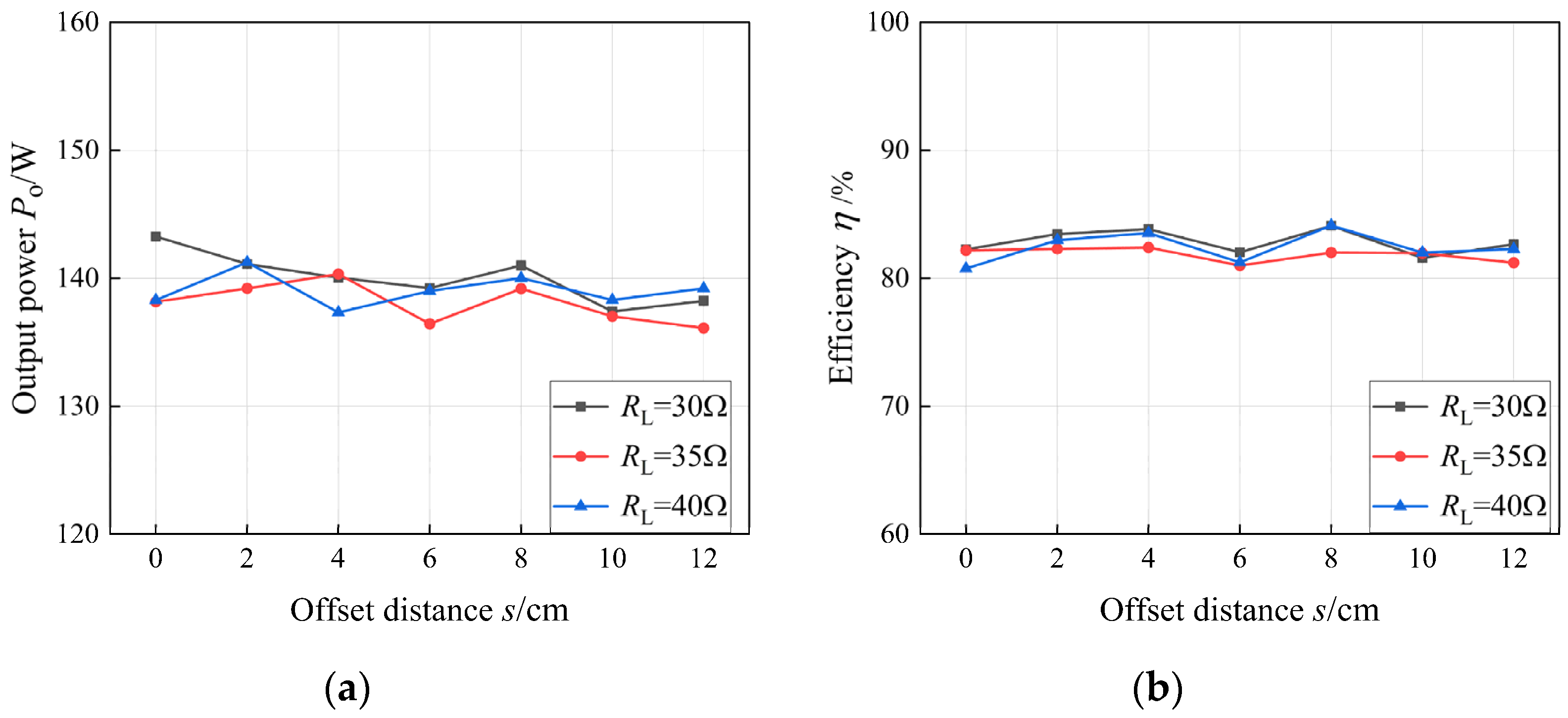
4. Experimental Verification
5. Conclusions
- Based on the LCC-S type topology, the model is analyzed. On this basis, the effects of mutual inductance, equivalent load and compensation inductance on the transmission characteristics of dynamic wireless power transmission system are studied, which provides guidance for the selection of system parameters.
- Considering the power and efficiency fluctuations in the operation of dynamic wireless power transmission system, and considering the energy transmission characteristics of the system, each voltage conduction angle is introduced into the inverter circuit and the semi-controlled rectifier circuit. In the adjustable range of mutual inductance, the efficiency optimization and power stable output control of the system are achieved by adjusting the degree-of-freedom of control on the transmitter side and receiver side. Both simulation and experimentation verify the validity and feasibility of the proposed bilateral control strategy.
Author Contributions
Funding
Conflicts of Interest
References
- Nikola, T. Apparatus for Transmitting Electrical Energy. U.S. Patent US1119732, 1 December 1914. [Google Scholar]
- Kurs, A.; Karalis, A.; Moffatt, R.; Joannopoulos, J.D.; Fisher, P.; Soljačić, M. Wireless Power Transfer via Strongly Coupled Magnetic Resonances. Science 2007, 317, 83–86. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Monti, G.; Tarricone, L.; Dionigi, M.; Mongiardo, M. Magnetically coupled resonant wireless power transmission: An artificial transmission line approach. In Proceedings of the 2012 42nd European Microwave Conference, Amsterdam, The Netherlands, 29 October–1 November 2012; pp. 233–236. [Google Scholar]
- Haider, M.F.; Hassan, M.M.; Ahmad, S.; You, F. Wireless Power Transmission Based on Magnetic Resonance Coupling. In Proceedings of the 2019 International Conference on Electrical, Communication, and Computer Engineering (ICECCE), Swat, Pakistan, 24–25 July 2019; pp. 1–3. [Google Scholar]
- Tan, L.; Guo, J.; Huang, X.; Liu, H.; Wang, W.; Yan, C.; Zhang, M. Coordinated Source Control for Output Power Stabilization and Efficiency Optimization in WPT Systems. IEEE Trans. Power Electron. 2018, 33, 3613–3621. [Google Scholar] [CrossRef]
- Ayisire, E.; El-Shahat, A.; Sharaf, A. Magnetic Resonance Coupling Modelling for Electric Vehicles Wireless Charging. In Proceedings of the 2018 IEEE Global Humanitarian Technology Conference (GHTC), San Jose, CA, USA, 18–21October 2018; pp. 1–2. [Google Scholar]
- Arvin, F.; Turgut, A.E.; Yue, S. COS: Artificial Pheromone System for Robotic Swarms Research. In Proceedings of the 2015 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Hamburg, Germany, 28 September–2 October 2015; pp. 407–412. [Google Scholar]
- Chow, J.P.W.; Chen, N.; Chung, H.S.H.; Chan, L.L.H. Misalignment tolerable coil structure for biomedical applications with wireless power transfer. In Proceedings of the Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Osaka, Japan, 3–7 July 2013; pp. 775–778. [Google Scholar]
- Feng, W.; Tianyu, F.; Chen, X. Design and optimisation of a wireless power transfer system for satellite application. IET Power Electron. 2019, 12, 2586–2598. [Google Scholar]
- Ruddell, S.; Madawala Udaya, K.; Thrimawithana Duleepa, J. Dynamic WPT system for EV charging with integrated energy storage. IET Power Electron. 2019, 12, 2660–2668. [Google Scholar] [CrossRef]
- Ruoqiong, L.; Junjie, W.; Xuan, Z.; Xin, L. Segmented Power Supply Preset Control Method of High-Speed Rail Contactless Traction Power Supply System considering Regenerative Braking Energy Recovery. Math. Probl. Eng. 2020, 2020, 6698688. [Google Scholar] [CrossRef]
- Nie, S.; Pathmanathan, M.; Yakop, N.; Luo, Z.; Lehn, P.W. Field orientation based three-coil decoupled wireless transmitter for electric vehicle charging with large lateral receiver misalignment tolerance. IET Power Electron. 2021, 14, 946–957. [Google Scholar] [CrossRef]
- Liu, Y.; Mai, R.; Liu, D.; Li, Y.; He, Z. Efficiency Optimization for Wireless Dynamic Charging System with Overlapped DD Coil Arrays. IEEE Trans. Power Electron. 2018, 33, 2832–2846. [Google Scholar] [CrossRef]
- Budhia, M.; Boys, J.T.; Covic, G.A.; Huang, C.Y. Development of a single-sided flux magnetic coupler for electric vehicle IPT charging systems. IEEE Trans. Ind. Electron. 2013, 60, 318–328. [Google Scholar] [CrossRef]
- Zaheer, A.; Covic, G.A.; Kacprzak, D. A bipolar pad in a 10-kHz 300-W distributed IPT system for AGV applications. IEEE Trans. Ind. Electron. 2014, 61, 3288–3301. [Google Scholar] [CrossRef]
- Mai, R.; Li, Q.; Chen, Y.; Yang, B.; He, Z. Variable parameter and variable frequency-based IPT charging system with configurable charge current and charge voltage. IET Power Electron. 2020, 13, 751–757. [Google Scholar] [CrossRef]
- Jianghao, H.; Jiankang, Z. Design of wireless power transfer system with input filter. IET Power Electron. 2020, 13, 1393–1402. [Google Scholar]
- Wang, S.; Chen, J.; Hu, Z.; Rong, C.; Liu, M. Optimisation design for series–series dynamic WPT system maintaining stable transfer power. IET Power Electron. 2017, 10, 987–995. [Google Scholar] [CrossRef]
- Zhang, W.; Wong, S.C.; Chi, K.T.; Chen, Q. Design for efficiency optimization and voltage controllability of series-series compensated inductive power transfer systems. IEEE Trans. Power Electron. 2014, 29, 191–200. [Google Scholar] [CrossRef]
- Fu, M.; Tang, Z.; Liu, M.; Ma, C.; Zhu, X. Full-bridge rectifier input reactance compensation in Megahertz wireless power transfer systems. In Proceedings of the 2015 IEEE PELS Workshop on Emerging Technologies: Wireless Power (WoW), Daejeon, Korea, 5–6 June 2015; pp. 1–5. [Google Scholar]
- Pellitteri, F.; Boscaino, V.; Miceli, R.; Udaya, K.M. Power tracking with maximum efficiency for wireless charging of E-bikes. In Proceedings of the 2014 IEEE International Electric Vehicle Conference (IEVC), Florence, Italy, 17–19 December 2014; pp. 1–7. [Google Scholar]
- Zhong, W.X.; Hui, S.Y.R. Maximum energy efficiency tracking for wireless power transfer systems. IEEE Trans. Power Electron. 2015, 30, 4025–4034. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Li, J.; Wang, K.; Chen, W.; Yang, X. A maximum efficiency point tracking control scheme for wireless power transfer systems using magnetic resonant coupling. IEEE Trans. Power Electron. 2015, 30, 3998–4008. [Google Scholar] [CrossRef]
- Fu, M.; Yin, H.; Zhu, X.; Ma, C. Analysis and tracking of optimal load in wireless power transfer systems. IEEE Trans. Power Electron. 2015, 30, 3952–3963. [Google Scholar] [CrossRef]
- Diekhans, T.; de Doncker, R.W. A dual-side controlled inductive power transfer system optimized for large coupling factor variations and partial load. IEEE Trans. Power Electron. 2015, 30, 6320–6328. [Google Scholar] [CrossRef]
- Berger, A.; Agostinelli, M.; Vesti, S.; Oliver, J.A.; Cobos, J.A.; Huemer, M. A wireless charging system applying phase-shift and amplitude control to maximize efficiency and extractable power. IEEE Trans. Power Electron. 2015, 30, 6338–6348. [Google Scholar] [CrossRef]
- Dai, X.; Li, X.; Li, Y.; Hu, A.P. Maximum Efficiency Tracking for Wireless Power Transfer Systems with Dynamic Coupling Coefficient Estimation. IEEE Trans. Power Electron. 2018, 6, 5005–5015. [Google Scholar] [CrossRef]
- Mai, R.; Liu, Y.; Li, Y.; Yue, P.; Cao, G.; He, Z. An Active-Rectifier-Based Maximum Efficiency Tracking Method Using an Additional Measurement Coil for Wireless Power Transfer. IEEE Trans. Power Electron. 2018, 1, 716–728. [Google Scholar] [CrossRef]




| Parameter | Value |
|---|---|
| Coil internal resistance on receiving side and transmitting side R1,2 | 0.13/0.13 Ω |
| Receiving side and transmitting side coil inductance L1,2 | 108.47/108 µH |
| Compensation capacitance on receiving side and transmitting side C1,2 | 114/93.8 nF |
| Frequency f | 50 kHz |
| Compensation resonant coil inductance Lf | 20 µH |
| Transmitting side parallel compensation capacitor Cf | 507 nF |
| Compensation of internal resistance of resonant coil Rf | 0.1 Ω |
| Parameter | M = 30 µH | M = 26 µH | M = 19 µH |
|---|---|---|---|
| Exert control if | 11.74% | 10.88% | 7.69% |
| Without control if | 21.80% | 28.63% | 51.77% |
| Exert control i1 | 0.88% | 1.08% | 0.80% |
| Without control i1 | 1.58% | 1.23% | 0.78% |
| Exert control i2 | 3.85% | 5.11% | 5.15% |
| Without control i2 | 10.34% | 10.10% | 9.84% |
| Number | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|---|
| Lateral offset distance s/cm | 0 | 6 | 12 | 0 | 6 | 12 | 0 | 6 | 12 |
| Load resistance RL/Ω | 30 | 30 | 30 | 35 | 35 | 35 | 40 | 40 | 40 |
| Simulation results of output power Po/W | 147.7 | 148.5 | 147.7 | 145.6 | 145.2 | 145.3 | 145.5 | 145.4 | 146.7 |
| Simulation results of transmission efficiency η | 0.869 | 0.873 | 0.869 | 0.856 | 0.854 | 0.855 | 0.856 | 0.855 | 0.863 |
| Experimental results of output power Po/W | 143.2 | 139.2 | 138.2 | 138.2 | 136.4 | 136.1 | 138.3 | 139.0 | 139.2 |
| Experimental results of transmission efficiency η | 0.823 | 0.821 | 0.827 | 0.822 | 0.810 | 0.812 | 0.808 | 0.812 | 0.823 |
| Error between the simulation and experimental results | 0.030 | 0.062 | 0.064 | 0.051 | 0.061 | 0.063 | 0.052 | 0.044 | 0.051 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xue, M.; Yang, Q.; Li, C.; Zhang, P.; Ma, S.; Zhang, X. Collaborative Optimization Method of Power and Efficiency for LCC-S Wireless Power Transmission System. Electronics 2021, 10, 3088. https://doi.org/10.3390/electronics10243088
Xue M, Yang Q, Li C, Zhang P, Ma S, Zhang X. Collaborative Optimization Method of Power and Efficiency for LCC-S Wireless Power Transmission System. Electronics. 2021; 10(24):3088. https://doi.org/10.3390/electronics10243088
Chicago/Turabian StyleXue, Ming, Qingxin Yang, Chunzhi Li, Pengcheng Zhang, Shuting Ma, and Xin Zhang. 2021. "Collaborative Optimization Method of Power and Efficiency for LCC-S Wireless Power Transmission System" Electronics 10, no. 24: 3088. https://doi.org/10.3390/electronics10243088
APA StyleXue, M., Yang, Q., Li, C., Zhang, P., Ma, S., & Zhang, X. (2021). Collaborative Optimization Method of Power and Efficiency for LCC-S Wireless Power Transmission System. Electronics, 10(24), 3088. https://doi.org/10.3390/electronics10243088






