Investigation on a 220 GHz Quasi-Optical Antenna for Wireless Power Transmission
Abstract
:1. Introduction
2. Structure and Design Principles
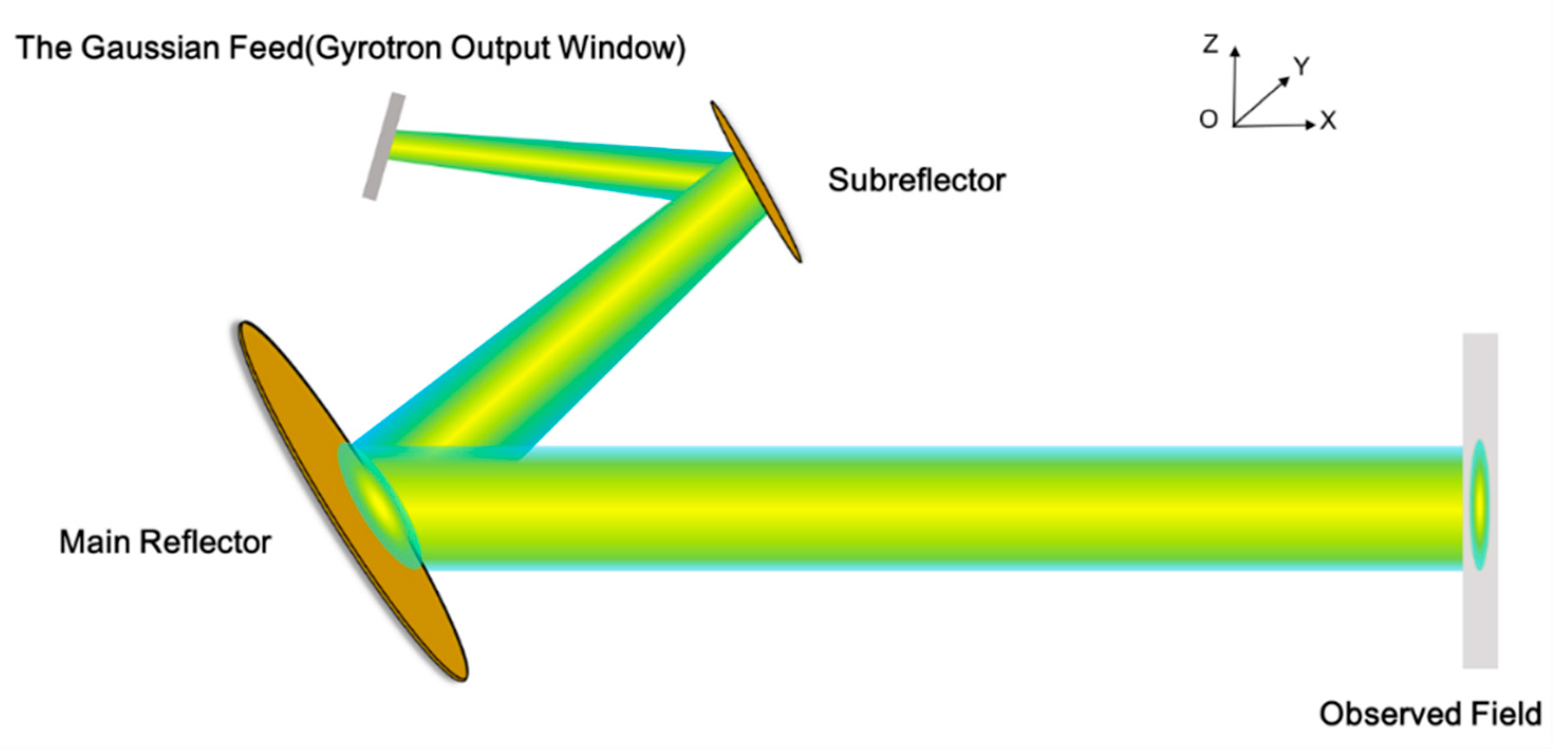
2.1. Antenna Structure
2.2. Gaussian Beam Propagation Theory
2.3. Design of the Reflectors
3. Simulation and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Glaser, P.E. Power from the Sun: Its Future. Science 1968, 162, 857–861. [Google Scholar] [CrossRef]
- Shinohara, N.; Kawasaki, S. Recent Wireless Power Transmission technologies in Japan for space solar power station/satellite. In Proceedings of the 2009 IEEE Radio and Wireless Symposium, San Diego, CA, USA, 18–22 June 2009; pp. 13–15. [Google Scholar]
- Schlesak, J.; Alden, A.; Ohno, T. SHARP rectenna and low altitude flight trials. In Proceedings of the GLOBECOM’85-Global Telecommunications Conference, New York, NY, USA, 2–5 December 1985; pp. 960–964. [Google Scholar]
- East, T.W. A self-steering array for the SHARP microwave-powered aircraft. IEEE Trans. Antennas Propag. 1992, 40, 1565–1567. [Google Scholar] [CrossRef]
- Button, J.K.; Wiltse, J.C. Infrared and Millimeter Waves V4: Millimeter Systems; Elsevier: Amsterdam, The Netherlands, 2014; pp. 1–2. [Google Scholar]
- Gilmour, A.S. Microwave and Millimeter-Wave Vacuum Electron Devices: Inductive Output Tubes, Klystrons, Traveling-Wave Tubes, Magnetrons, Crossed-Field Amplifiers, and Gyrotrons; Artech House: Boston, MA, USA, 2020. [Google Scholar]
- Barker, R.J.; Luhmann, N.C.; Booske, J.H.; Nusinovich, G.S. Modern Microwave and Millimeter-Wave Power Electronics; Institute of Electrical and Electronics Engineers (IEEE): Hoboken, NJ, USA, 2005. [Google Scholar]
- Chatterjee, R. Microwave and Millimeter-wave Vacuum Tube Electron Devices: Overview and State-of-the-art. IETE Tech. Rev. 1993, 10, 175–181. [Google Scholar] [CrossRef]
- Chu, K.R. The electron cycltron maser. Rev. Modern Phys. 2004, 76, 489–540. [Google Scholar] [CrossRef]
- Schneider, J. Stimulated Emission of Radiation by Relativistic Electrons in a Magnetic Field. Phys. Rev. Lett. 1959, 2, 504–505. [Google Scholar] [CrossRef]
- Hirshfield, J.L.; Wachtel, J.M. Electron Cyclotron Maser. Phys. Rev. Lett. 1964, 12, 533–536. [Google Scholar] [CrossRef]
- Flyagin, V.A.; Gaponov, A.V.; Petelin, I.; Yulpatov, V.K. The Gyrotron. IEEE Trans. Microw. Theory Tech. 1977, 25, 514–521. [Google Scholar] [CrossRef]
- Nusinovich, G.S.; Thumm, M.K.A.; Petelin, M.I. The Gyrotron at 50: Historical Overview. J. Infrared Millim. Terahertz Waves 2014, 35, 325–381. [Google Scholar] [CrossRef]
- Thumm, M. State-of-the-Art of High-Power Gyro-Devices and Free Electron Masers. J. Infrared Millim. Terahertz Waves 2020, 41, 1–140. [Google Scholar] [CrossRef] [Green Version]
- Prinz, O.; Arnold, A.; Gantenbein, G.; Liu, Y.-H.; Thumm, M.; Wagner, D. Highly Efficient Quasi-Optical Mode Converter for a Multifrequency High-Power Gyrotron. IEEE Trans. Electron Devices 2009, 56, 828–834. [Google Scholar] [CrossRef]
- Thumm, M.; Yang, X.; Arnold, A.; Dammertz, G.; Michel, G.; Pretterebner, J.; Wagner, D. A High-Efficiency Quasi-Optical Mode Converter for a 140-GHz 1-MW CW Gyrotron. IEEE Trans. Electron Devices 2005, 52, 818–824. [Google Scholar] [CrossRef]
- Zhao, G.; Xue, Q.; Wang, Y.; Wang, X.; Zhang, S.; Liu, G.; Feng, J.; Zhang, L. Design of Quasi-Optical Mode Converter for 170-GHz TE32,9-Mode High-Power Gyrotron. IEEE Trans. Plasma Sci. 2019, 47, 2582–2589. [Google Scholar] [CrossRef]
- Zhou, X.-Q.; Ann, B.N.K.; Seong, K.S. Single aspherical lens for deastigmatism, collimation, and circularization of a laser beam. Appl. Opt. 2000, 39, 1148–1151. [Google Scholar] [CrossRef] [PubMed]
- Shen, C.Y.; Chen, F.; Yu, X.D. Optical design of an optical system for free space optical communication. In Proceedings of the Advanced Optical Manufacturing Technologies—International Symposium on Advanced Optical Manufacturing & Testing Technologies, Xian, China, 23 February 2006; p. 614915. [Google Scholar]
- Rusch, W.; Prata, A.; Rahmat-Samii, Y.; Shore, R. Derivation and application of the equivalent paraboloid for classical offset Cassegrain and Gregorian antennas. IEEE Trans. Antennas Propag. 1990, 38, 1141–1149. [Google Scholar] [CrossRef]
- Granet, C. Designing axially symmetric Cassegrain or Gregorian dual-reflector antennas from combinations of prescribed geometric parameters. IEEE Antennas Propag. Mag. 1998, 40, 76–82. [Google Scholar] [CrossRef]
- Goldsmith, P.F. Quasioptical Systems: Gaussian Beam Quasioptical Propagation and Applications; IEEE Press: Piscataway, NJ, USA, 1998. [Google Scholar]
- Wang, L. Research on Quasi-Optical Technology in Millimeter Wave Space Beam Power Combining [Dissertation]; Southeast University: Nanjing, China, 2018. [Google Scholar]
- Jin, J.; Piosczyk, B.; Thumm, M.; Rzesnicki, T.; Zhang, S. Quasi-Optical Mode Converter/Mirror System for a High-Power Coaxial-Cavity Gyrotron. IEEE Trans. Plasma Sci. 2006, 34, 1508–1515. [Google Scholar] [CrossRef]
- Xie, Z.M.; Wang, L.X. A linear horn array feed dual reflector antenna for spatial power combining. In Proceedings of the 2012 International Conference on Microwave and Millimeter Wave Technology (ICMMT), Shengzhen, China, 5–8 May 2012; pp. 1–4. [Google Scholar]
- Kogelnik, H.; Li, T. Laser beams and resonator. Appl. Opt. 1966, 5, 1312–1325. [Google Scholar] [CrossRef]
- Marathay, A.S.; McCalmont, J.F. Vector diffraction theory for electromagnetic waves. J. Opt. Soc. Am. A 2001, 18, 2585–2593. [Google Scholar] [CrossRef] [PubMed]
- Kong, J.A. Electromagnetic Wave Theory; Wiley: New York, NY, USA, 1986; p. 381. [Google Scholar]
- Chen, C.; Huang, K.; Yang, Y. Microwave Transmitting System Based on Four-Way Master-Slave Injection-Locked Magnetrons and Horn Arrays With Suppressed Sidelobes. IEEE Trans. Microw. Theory Tech. 2018, 66, 2416–2424. [Google Scholar] [CrossRef]
- Zhang, C.X.; Fu, W.J.; Yan, Y. Study on a gyrotron quasi-optical mode converterfor terahertz imaging. J. Electromagn. Waves Appl. 2021, 35, 176–184. [Google Scholar] [CrossRef]
- Matsumoto, H. Research on solar power satellites and microwave power transmission in Japan. IEEE Microw. Mag. 2002, 3, 36–45. [Google Scholar] [CrossRef]
- Haus, H.A. Waves and Fields in Optoelectronics; Prentice-Hall: Englewood Cliffs, NJ, USA, 1984; pp. 113–115. [Google Scholar]











| Parameter | Value | Parameter | Value |
|---|---|---|---|
| (mm) | 9.5482 | (°) | 26 |
| (mm) | 78.4063 | (°) | 30 |
| (°) | 50 | (°) | 32.28 |
| (°) | 20 | (mm) | 190.9091 |
| (°) | 24 | (mm) | 132.4000 |
| Output Observation Position | Gain | 8.68-dB Beamwidth (°) | |
|---|---|---|---|
| (dBi) | E-Plane | H-Plane | |
| Gaussian feed with the dual reflector (ω0 = 5.6 mm) | 43.3442 | 1.0596 | 1.0639 |
| Gaussian feed (ω0 = 5.6 mm) | 31.0500 | 4.5031 | 4.5032 |
| Difference | 12.2942 | −3.4435 | −3.4393 |
| 3-dB Beamwidth (°) | SLL (dB) | BLL (dB) | ||||
|---|---|---|---|---|---|---|
| E-Plane | H-Plane | E-Plane | H-Plane | E-Plane | H-Plane | |
| Quasi-Optical Antenna | 0.8433 | 0.8444 | −57.2262 | −64.3269 | −67.84422 | −76.5442 |
| Output Observation Position(m) | 0.4 | 0.6 | 0.8 | 1.0 | 1.2 | 1.4 |
| Transmission Efficiency (%) | 99.574 | 99.569 | 99.566 | 99.562 | 99.558 | 99.554 |
| Output Observation Position(m) | 1.6 | 1.8 | 2.0 | 2.2 | 10 | 1000 |
| Transmission Efficiency (%) | 99.546 | 99.542 | 99.538 | 99.534 | 99.538 | 99.531 |
| Output Observation Position (m) | 0.4 | 0.6 | 0.8 | 1.0 | 1.2 | 1.4 |
| GBWR of the Gaussian feed (m) | 0.03151 | 0.04684 | 0.06226 | 0.07771 | 0.09318 | 0.10866 |
| GBWR of the Gaussian feed with the dual reflector (m) | 0.02273 | 0.02281 | 0.02331 | 0.02457 | 0.02598 | 0.02832 |
| Output Observation Position (m) | 1.6 | 1.8 | 2.0 | 2.2 | 10 | 1000 |
| GBWR of the Gaussian feed (m) | 0.12414 | 0.13963 | 0.15512 | 0.17062 | 0.77513 | 77.5125 |
| GBWR of the Gaussian feed with the dual reflector (m) | 0.03037 | 0.03349 | 0.03646 | 0.03923 | 0.18815 | 19.4156 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, M.; Guan, X.; Einat, M.; Fu, W.; Yan, Y. Investigation on a 220 GHz Quasi-Optical Antenna for Wireless Power Transmission. Electronics 2021, 10, 634. https://doi.org/10.3390/electronics10050634
Han M, Guan X, Einat M, Fu W, Yan Y. Investigation on a 220 GHz Quasi-Optical Antenna for Wireless Power Transmission. Electronics. 2021; 10(5):634. https://doi.org/10.3390/electronics10050634
Chicago/Turabian StyleHan, Meng, Xiaotong Guan, Moshe Einat, Wenjie Fu, and Yang Yan. 2021. "Investigation on a 220 GHz Quasi-Optical Antenna for Wireless Power Transmission" Electronics 10, no. 5: 634. https://doi.org/10.3390/electronics10050634
APA StyleHan, M., Guan, X., Einat, M., Fu, W., & Yan, Y. (2021). Investigation on a 220 GHz Quasi-Optical Antenna for Wireless Power Transmission. Electronics, 10(5), 634. https://doi.org/10.3390/electronics10050634






