Low Frequency Stability of AC Railway Traction Power Systems: Analysis of the Influence of Traction Unit Parameters
Abstract
:1. Introduction
1.1. Review of LFO Events
1.2. Content and Contribution
1.3. Organization
2. Railway Traction System Model for LFO Phenomenon Study
3. Impedance-Based Stability Analysis for the Study of the Low Frequency Oscillations Phenomenon
3.1. Impedance-Based Small-Signal Model
3.2. Network Impedance and Traction-Chain Admittance
3.3. Stability Criteria
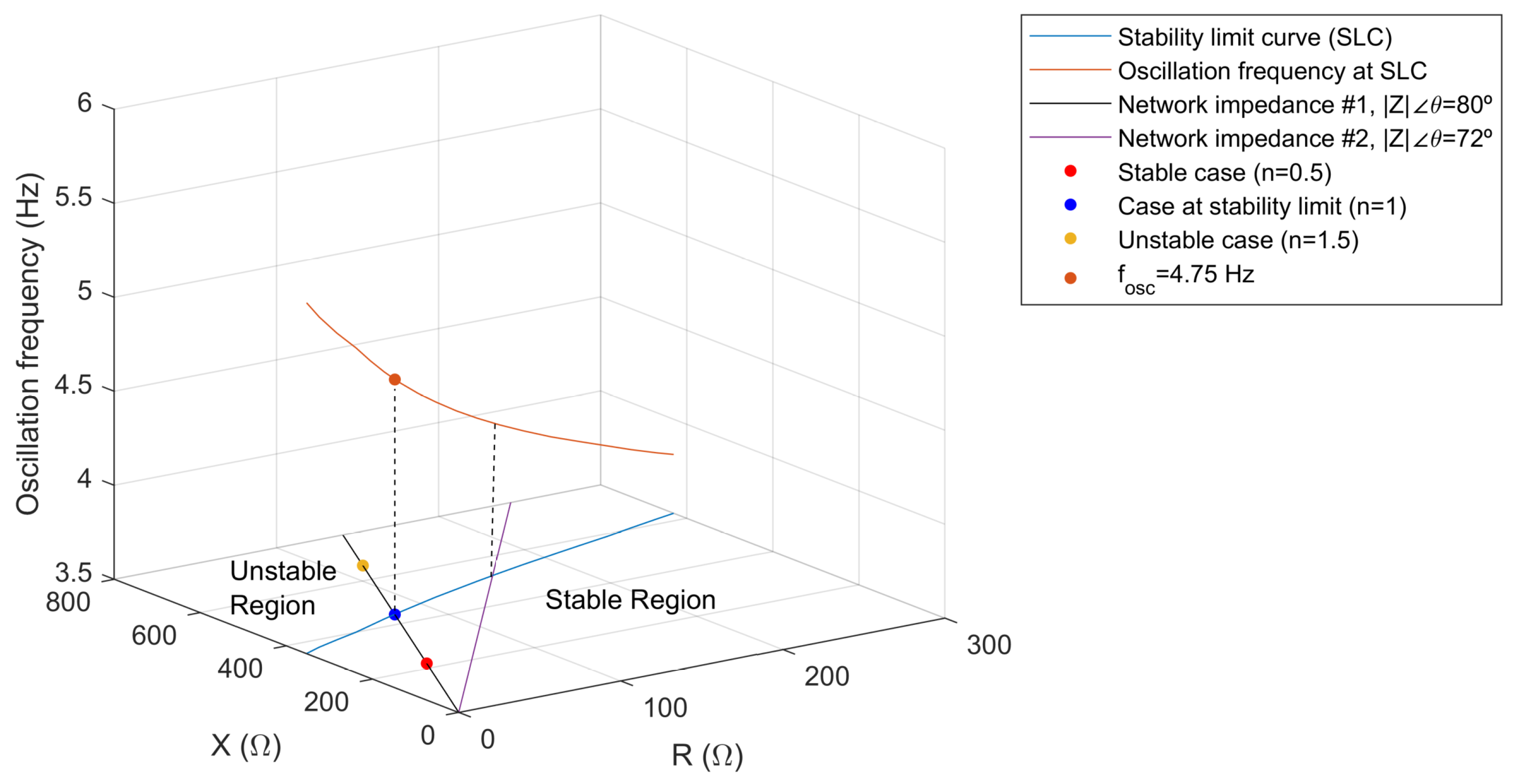
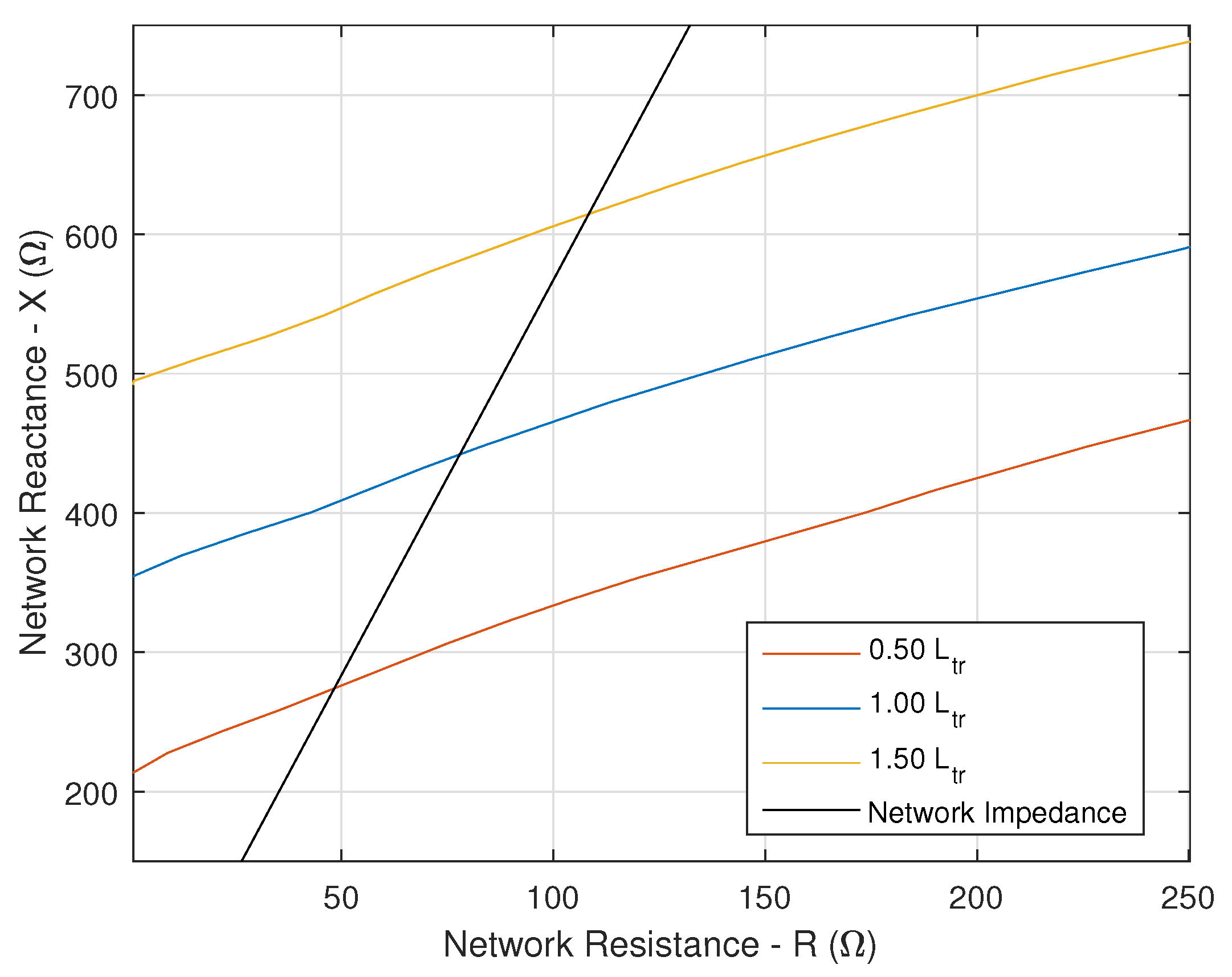
3.4. Stability Limit Curve in the Network Reactance (X) and Resistant (R) Complex Plane
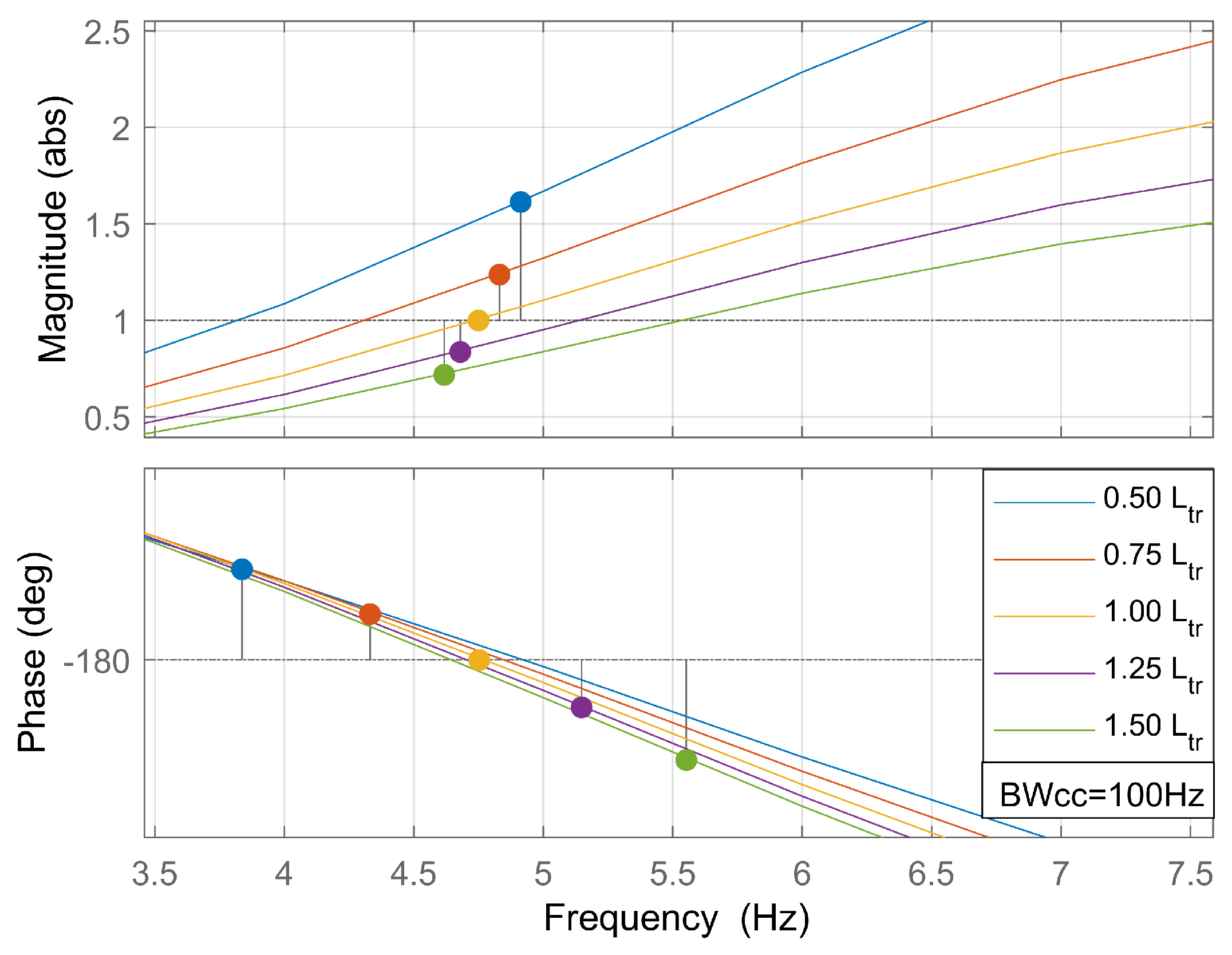
4. Influence of Contact-Line Length on the Railway Traction System Stability
4.1. Traction Chain Input Admittance
4.2. Network Impedance
- = (0.5 ; )
- = (5.5 ; )
- = (0.5 /km; )
4.3. Stability Analysis
4.4. Stability Limit Curve
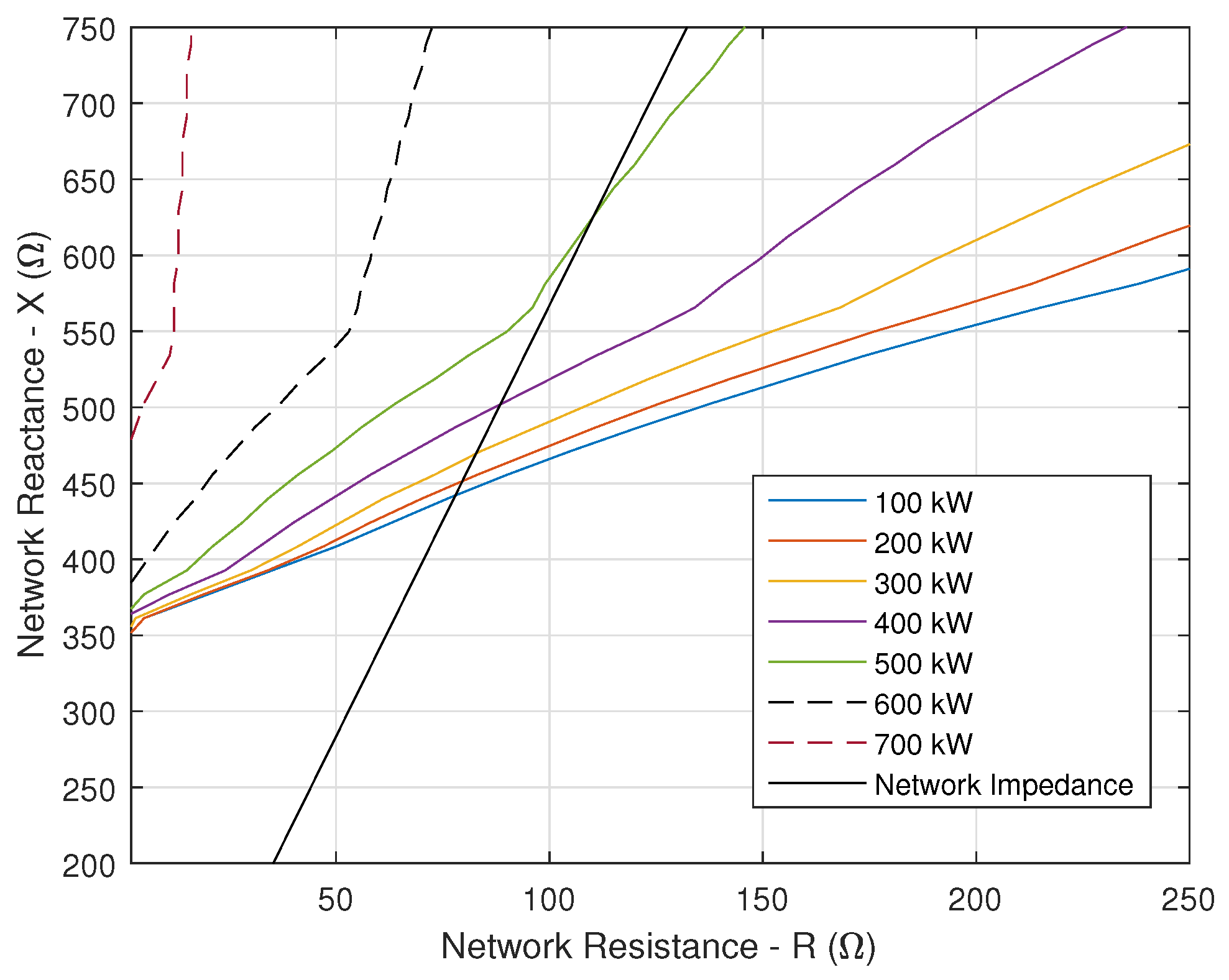
5. Influence of Power Absorbed at the DC-Link of the Traction Chain
6. Influence of Current Controller and 4QC Transformer’s Leakage Inductance
6.1. Influence of Current Controller
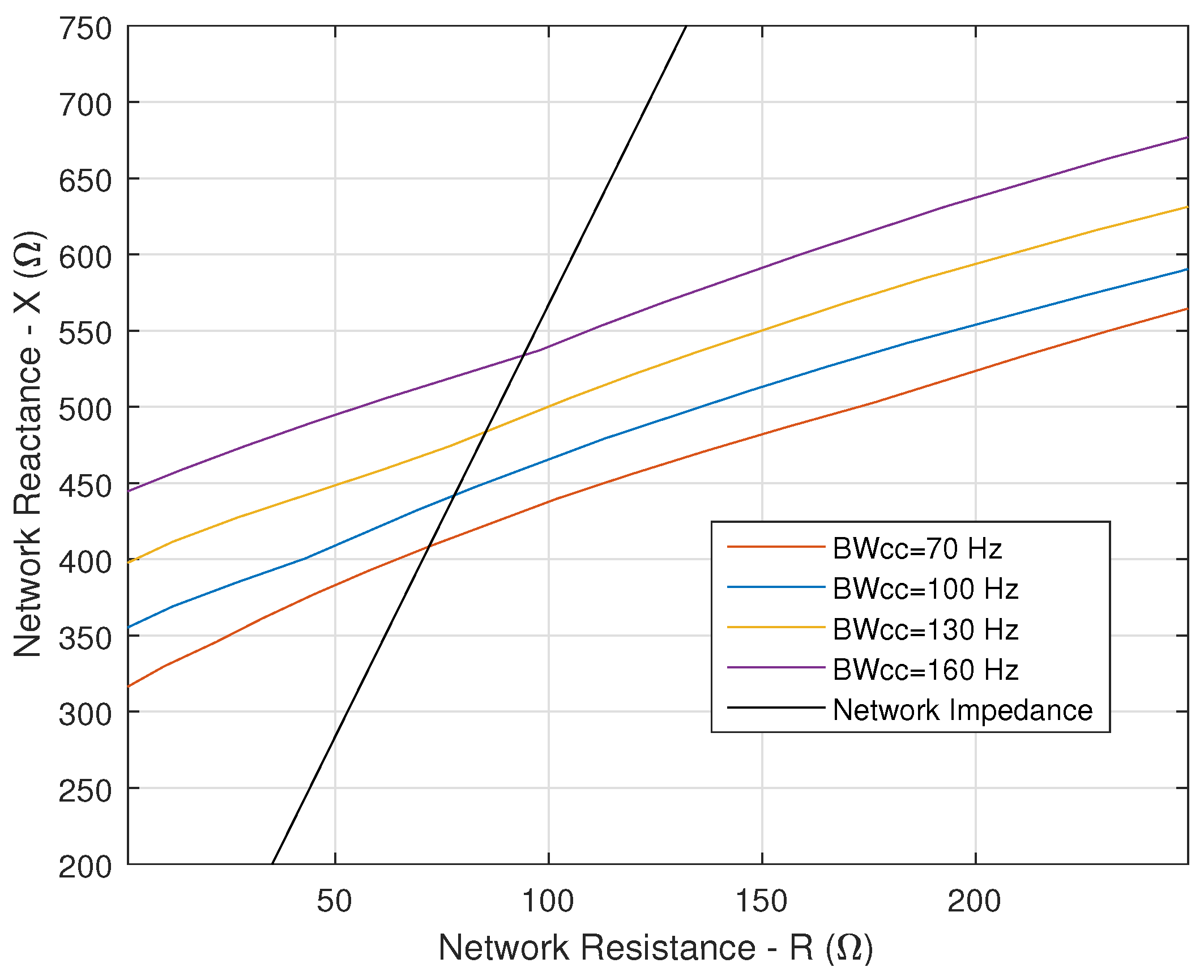
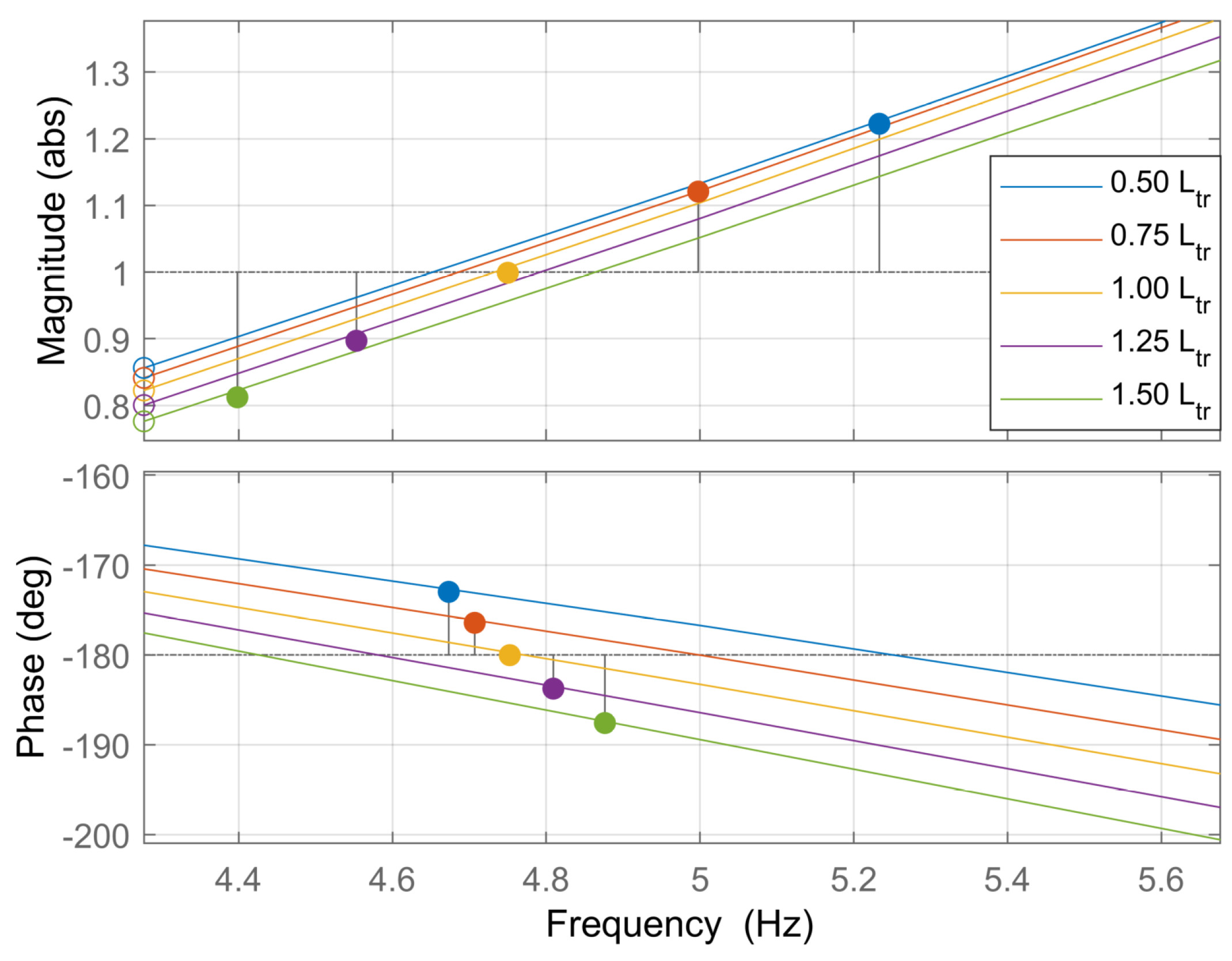
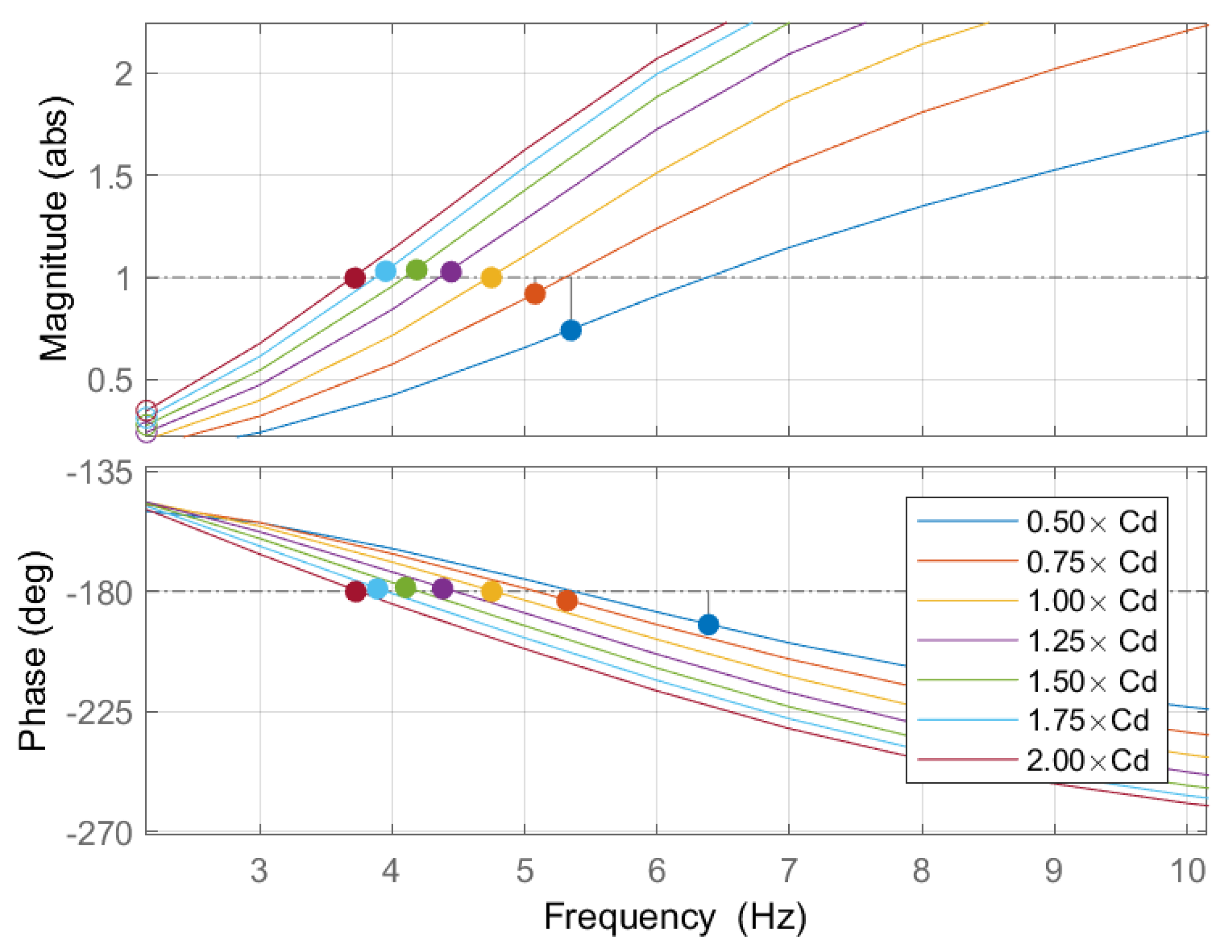
6.2. Influence of the Transformer Leakage Inductance
6.3. Transformer Leakage Inductance Variation with Constant Current Controller Bandwidth
6.4. Summary
7. Influence of Voltage Controller Bandwidth and DC-Link Capacitance
7.1. Influence of Voltage Controller Bandwidth
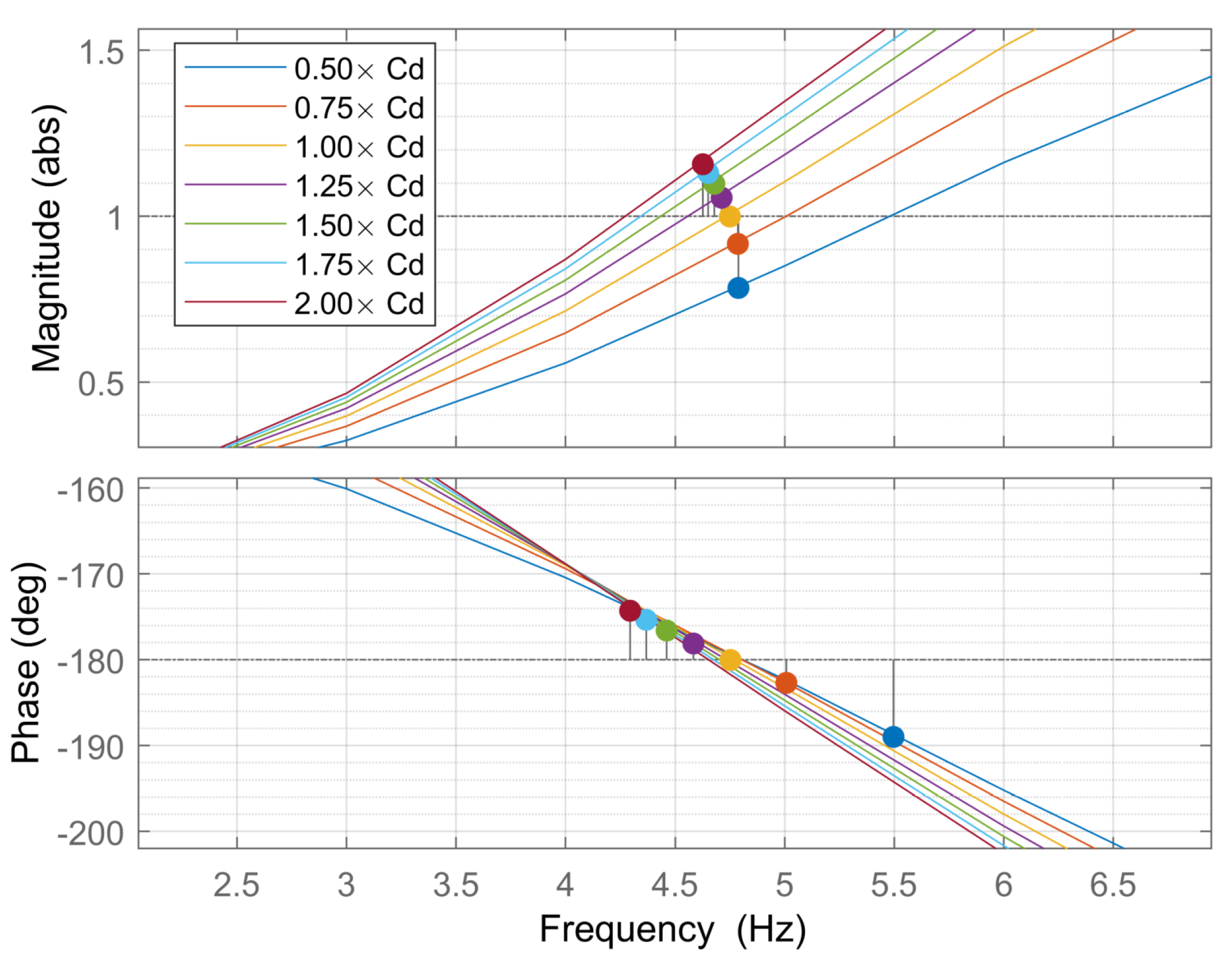
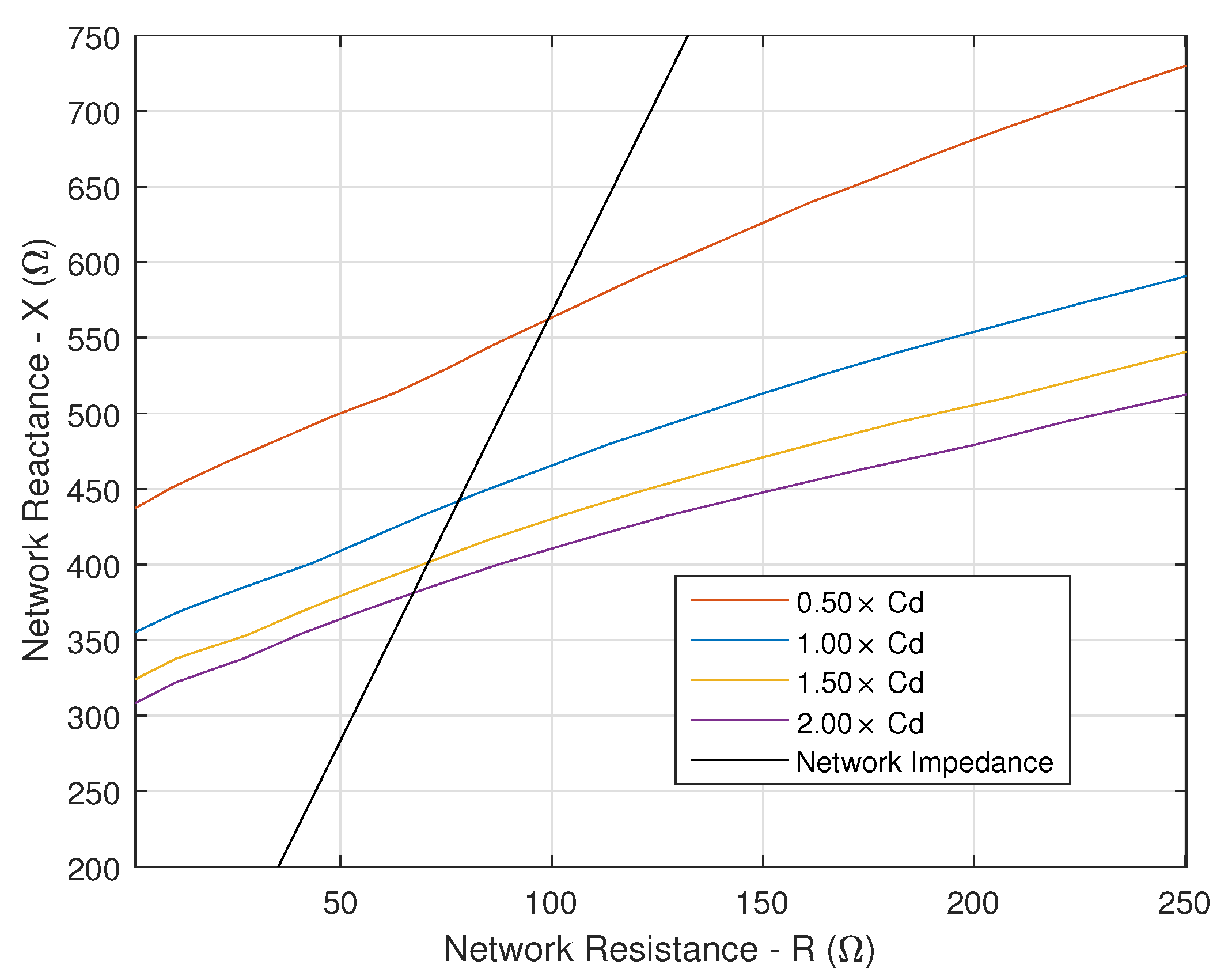
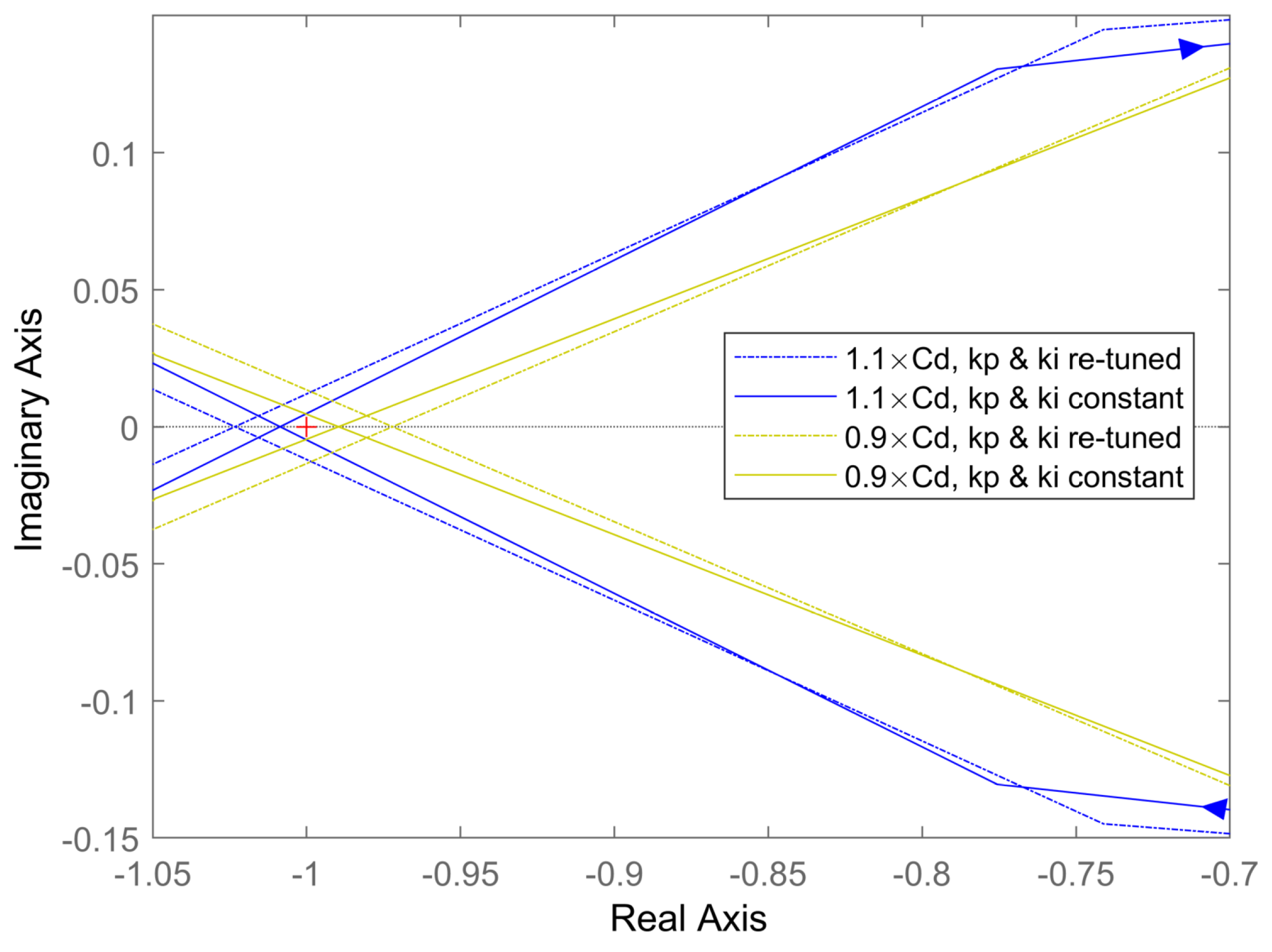
7.2. Influence of DC-Link Capacitance
7.3. DC-Link Capacitance Variation with Constant Voltage Controller Bandwidth
7.4. Discussion
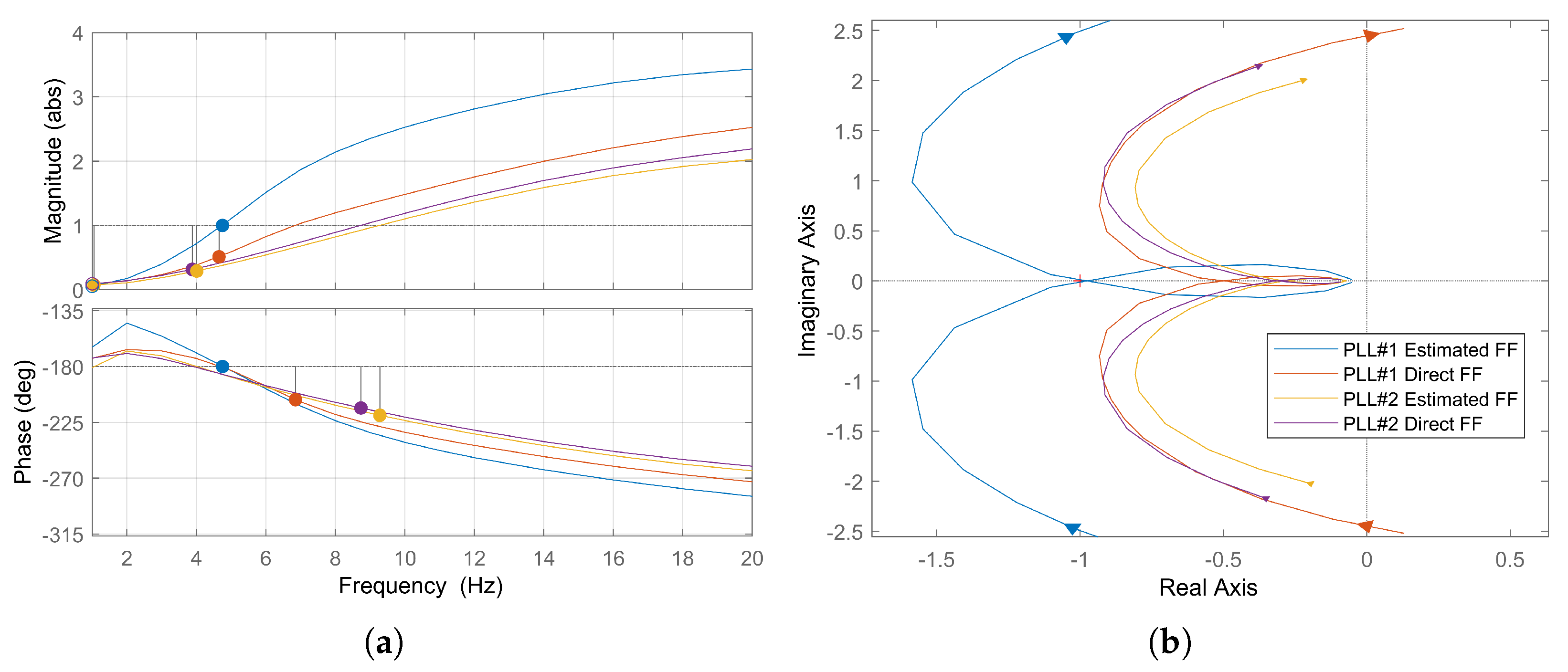
8. Impact of Phase-Locked Loop and Feedforward Signal
9. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Danielsen, S.; Molinas, M.; Toftevaag, T.; Fosso, O. Constant power load characteristic’s influence on the low-frequency interaction between advanced electrical rail vehicle and railway traction power supply with rotary converters. In Proceedings of the MET’ 2009, Gdanjsk, Poland, 24–26 September 2009. [Google Scholar]
- Buhrkall, L.; Danielsen, S.; Eisele, A.; Bergman, M.; Galic, J. Low-frequency oscillations in Scandinavian railway power supply—Part I: Basic considerations. eb-Elektr. Bahnen 2010, 108, 56–64. [Google Scholar]
- Buhrkall, L.; Danielsen, S.; Eisele, A.; Bergman, M.; Galic, J. Low-frequency oscillations in Scandinavian railway power supply—Part 2: Test of traction units. eb-Elektr. Bahnen 2010, 108, 103–111. [Google Scholar]
- Danielsen, S. Electric Traction Power System Stability: Low-Frequency Interaction between Advanced Rail Vehicles and a Rotary Frequency Converter. Ph.D. Thesis, Norwegian University of Science and Technology, Trondheim, Norway, April 2010. [Google Scholar]
- Suarez, J.; Ladoux, P.; Roux, N.; Caron, H.; Guillame, E. Measurement of locomotive input admittance to analyse low frequency instability on AC rail networks. In Proceedings of the 2014 International Symposium on Power Electronics, Electrical Drives, Automation and Motion, Ischia, Italy, 18–20 June 2014; pp. 790–795. [Google Scholar] [CrossRef]
- Menth, S.; Meyer, M. Low frequency power oscillations in electric railway systems. eb-Elektr. Bahnen 2006, 104, 216–221. [Google Scholar]
- Wang, H.; Mingli, W.; Sun, J. Analysis of Low-Frequency Oscillation in Electric Railways Based on Small-Signal Modeling of Vehicle-Grid System in dq Frame. IEEE Trans. Power Electron. 2015, 30, 5318–5330. [Google Scholar] [CrossRef]
- Hu, H.; Tao, H.; Blaabjerg, F.; Wang, X.; He, Z.; Gao, S. Train–Network Interactions and Stability Evaluation in High-Speed Railways–Part I: Phenomena and Modeling. IEEE Trans. Power Electron. 2018, 33, 4627–4642. [Google Scholar] [CrossRef]
- Hu, H.; Tao, H.; Wang, X.; Blaabjerg, F.; He, Z.; Gao, S. Train–Network Interactions and Stability Evaluation in High-Speed Railways—Part II: Influential Factors and Verifications. IEEE Trans. Power Electron. 2018, 33, 4643–4659. [Google Scholar] [CrossRef]
- Frutos, P.; Guerrero, J.M.; Muniategui, I.; Vicente, I.; Endemano, A.; Briz, F. Low-Frequency Oscillations Analysis in AC Railway Networks Using Eigenmode Identification. In Proceedings of the 2021 IEEE Energy Conversion Congress and Exposition (ECCE), Vancouver, BC, Canada, 10–14 October 2021; pp. 1573–1579. [Google Scholar] [CrossRef]
- Hachicha, Y.; Cypers, D.; Takuefou, M.; Belin, S.; Ladoux, P.; Roux, N. Use of a HIL railway traction simulator for low frequency network stability studies. In Proceedings of the 2018 IEEE International Conference on Electrical Systems for Aircraft, Railway, Ship Propulsion and Road Vehicles International Transportation Electrification Conference (ESARS-ITEC), Nottingham, UK, 7–9 November 2018; pp. 1–5. [Google Scholar] [CrossRef]
- Ladoux, P.; Hachicha, Y.; Cypers, D.; Meli, M.; Roux, N. New method for determining the low-frequency Stability Limit of a 50 Hz electric traction power system. Elektr. Bahnen 2020, 18, hal-03286350. [Google Scholar]
- Suarez, J. Étude et Modélisation des Interactions éLectriques entre les Engins et les Installations Fixes de Traction éLectrique 25 kV/50 Hz. Ph.D. Thesis, University of Toulouse, Toulouse, France, 2014. [Google Scholar]
- Liao, Y.; Liu, Z.; Zhang, H.; Wen, B. Low-Frequency Stability Analysis of Single-Phase System with dq-Frame Impedance Approach—Part I: Impedance Modeling and Verification. IEEE Trans. Ind. Appl. 2018, 54, 4999–5011. [Google Scholar] [CrossRef]
- Hachicha, Y.; Cypers, D.; Belin, S.; Meli, M.; Ladoux, P.; Roux, N. Towards a unified low frequency Stability criterion for 15 kV/16.7 Hz and 25 kV/50 Hz railway power system. In Proceedings of the PCIM Europe Digital Days 2020; International Exhibition and Conference for Power Electronics, Intelligent Motion, Renewable Energy and Energy Management, Virtual, 7–8 July 2020; pp. 1–8. [Google Scholar]
- Buhrkall, L. Traction system case study. In Proceedings of the 2008 IET Professional Development course on Electric Traction Systems, Manchester, UK, 3–7 November 2008; pp. 45–63. [Google Scholar] [CrossRef] [Green Version]
- EN 50388-2:2017. Railway Applications—Fixed Installations and Rolling Stock—Technical Criteria for the Coordination between Power Supply and Rolling Stock to Achieve Interoperability—Part 2: Stability and Harmonics. Available online: https://standards.iteh.ai/catalog/standards/clc/f5fb00d6-1f29-4dd4-a8fd-e3e478907499/pren-50388-2-2017 (accessed on 7 July 2017).
- Ogata, K. Modern Control Engineering (Instrumentation and Controls Series); Prentice-Hall, Inc.: Upper Saddle River, NJ, USA, 2010. [Google Scholar]
- Liao, Y.; Liu, Z.; Zhang, H.; Wen, B. Low-Frequency Stability Analysis of Single-Phase System With dq-Frame Impedance Approach—Part II: Stability and Frequency Analysis. IEEE Trans. Ind. Appl. 2018, 54, 5012–5024. [Google Scholar] [CrossRef]
- Pendharkar, I. A generalized Input Admittance Criterion for resonance stability in electrical railway networks. In Proceedings of the 2014 European Control Conference (ECC), Strasbourg, France, 24–27 June 2014; pp. 690–695. [Google Scholar] [CrossRef]
- Wang, X.; Blaabjerg, F. Harmonic Stability in Power Electronic-Based Power Systems: Concept, Modeling, and Analysis. IEEE Trans. Smart Grid 2019, 10, 2858–2870. [Google Scholar] [CrossRef] [Green Version]
- Teodorescu, R.; Liserre, M.; Rodriguez, P. Grid Synchronization in Single Phase Power Converters. In Grid Converters for Photovoltaic and Wind Power Systems; John Wiley & Sons: West Sussex, UK, 2011; pp. 43–91. [Google Scholar] [CrossRef]

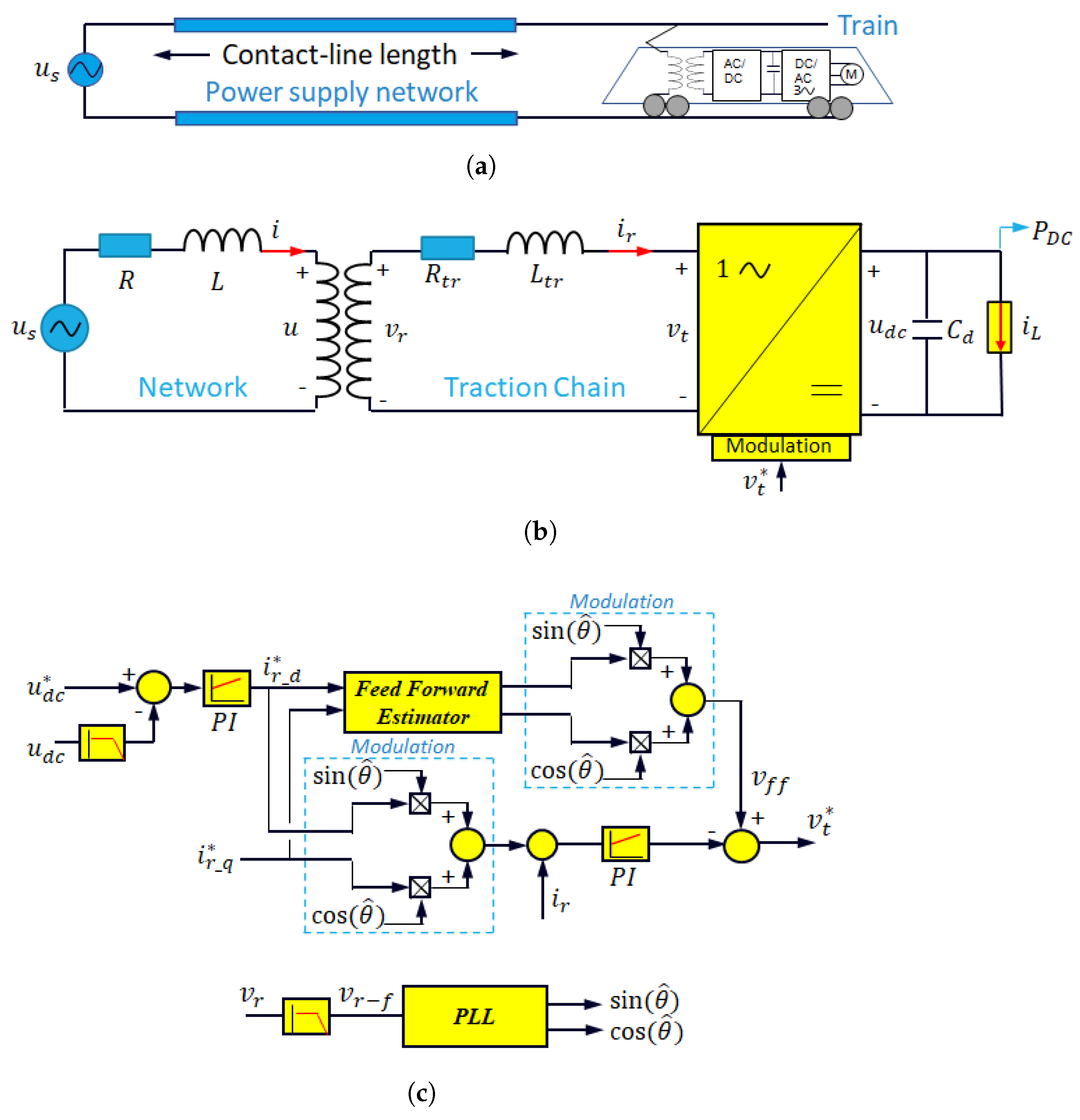
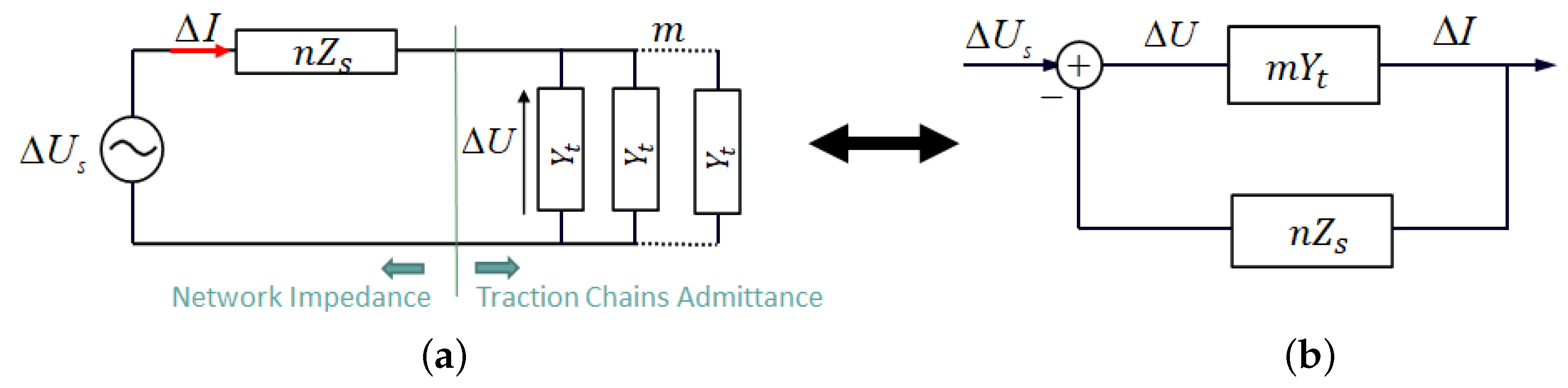

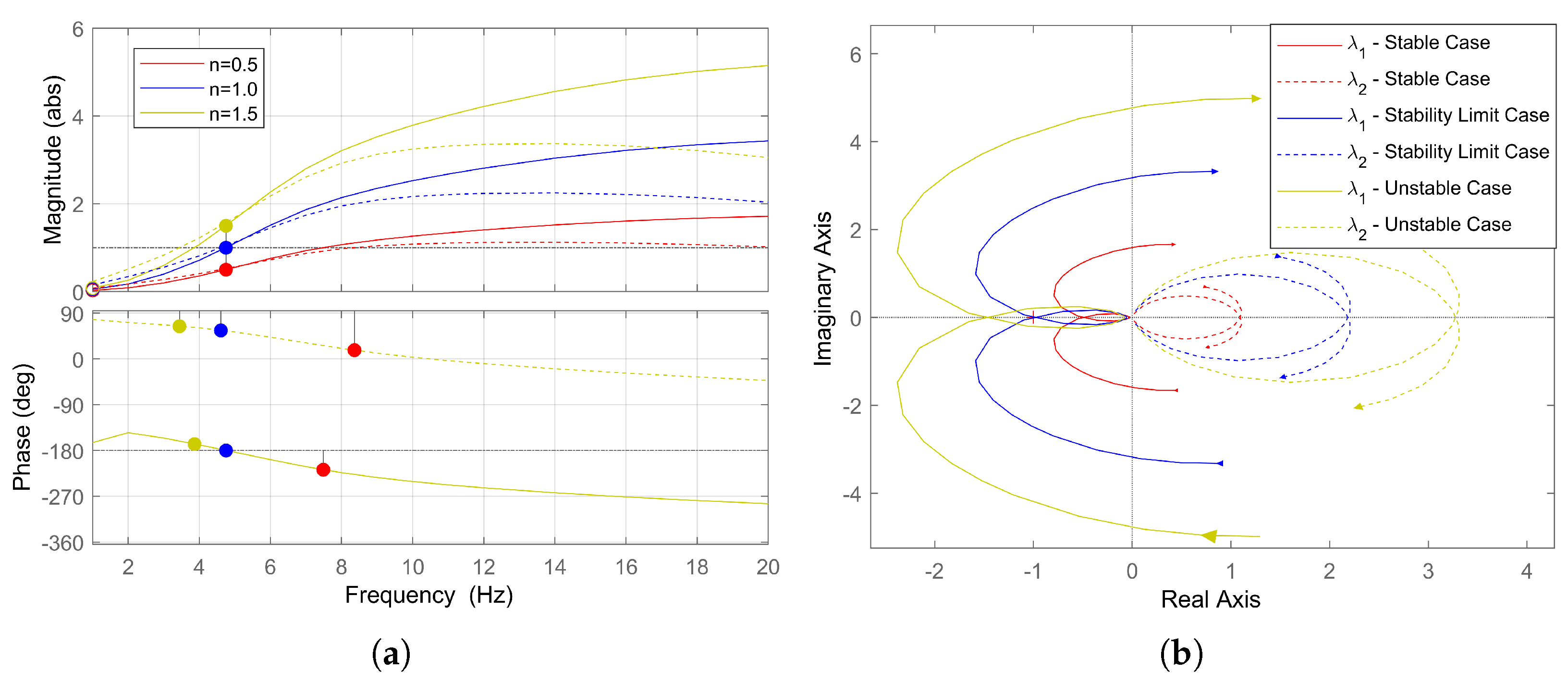
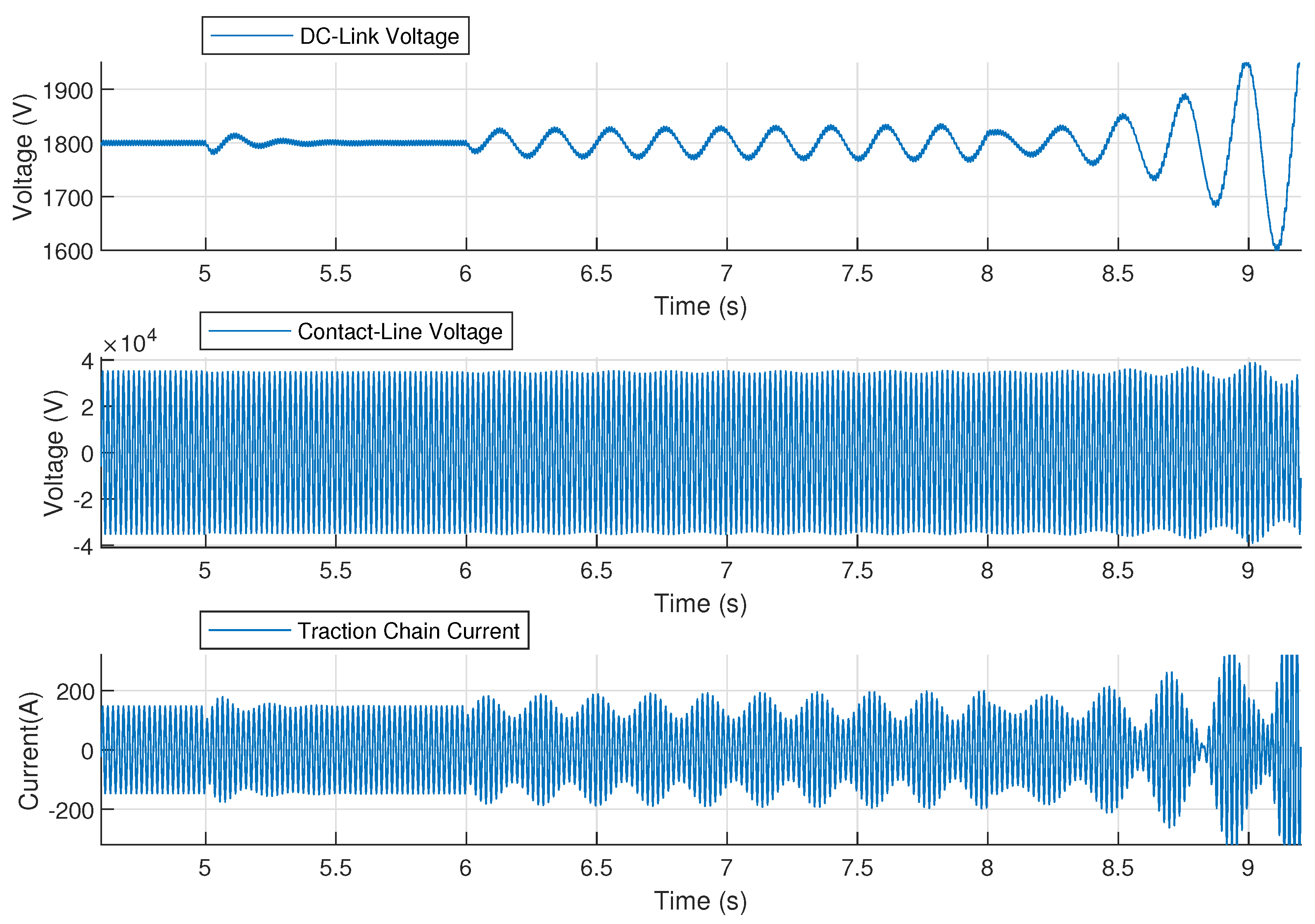










| N° | Case | (Hz) | (Hz) | Time (Year) |
|---|---|---|---|---|
| 1 | Zürich, Switzerland [1] | 16.7 | 5 | 1995 |
| 2 | Norway [2,3] | 16.7 | 1.6 | 2007 |
| 3 | Washington, USA [4] | 25 | 3 | 2006 |
| 4 | Thionville, France [5] | 50 | 5 | 2008 |
| 5 | Siemens test, Germany [6] | 50 | 7 | 2006 |
| 6 | Hudong Depot, China [7] | 50 | 2–4 | 2008 |
| 7 | Shanhaiguan Hub, China [8] | 50 | 6–7 | 2011 |
| Symbol | Description | Value |
|---|---|---|
| U | Contact-line voltage | 25 kV |
| Fundamental frequency | 50 Hz | |
| Leakage inductance | 1 mH | |
| DC-link capacitor | 16 mF | |
| Voltage control bandwidth | 8 Hz | |
| Current control bandwidth | 100 Hz | |
| Power at DC-link | 100 kW |
| & Adjusted | BWcc | Stability Margins | |
|---|---|---|---|
| - | YES | ↑ | ↑ ↑ |
| ↑ | YES | - | ↑ ↑ ↑ |
| ↑ | NO | ↓ | ↑ ↑ ↑ + ↓↓ = ↑ |
| & Adjusted | BWvc | Stability Margins | |
|---|---|---|---|
| - | YES | ↓ | ↑↑ |
| ↓ | YES | - | ↑↑↑ |
| ↓ | NO | ↑ | ↑↑↑ + ↓↓ = ↑ |
| PLL #1 | PLL #2 | Estimated FF | Direct FF | Stability Ranking | |
|---|---|---|---|---|---|
| Case 1 | X | X | BAD | ||
| Case 2 | X | X | GOOD | ||
| Case 3 | X | X | GOOD | ||
| Case 4 | X | X | GOOD |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Frutos, P.; Ladoux, P.; Roux, N.; Larrazabal, I.; Guerrero, J.M.; Briz, F. Low Frequency Stability of AC Railway Traction Power Systems: Analysis of the Influence of Traction Unit Parameters. Electronics 2022, 11, 1593. https://doi.org/10.3390/electronics11101593
Frutos P, Ladoux P, Roux N, Larrazabal I, Guerrero JM, Briz F. Low Frequency Stability of AC Railway Traction Power Systems: Analysis of the Influence of Traction Unit Parameters. Electronics. 2022; 11(10):1593. https://doi.org/10.3390/electronics11101593
Chicago/Turabian StyleFrutos, Paul, Philippe Ladoux, Nicolas Roux, Igor Larrazabal, Juan M. Guerrero, and Fernando Briz. 2022. "Low Frequency Stability of AC Railway Traction Power Systems: Analysis of the Influence of Traction Unit Parameters" Electronics 11, no. 10: 1593. https://doi.org/10.3390/electronics11101593
APA StyleFrutos, P., Ladoux, P., Roux, N., Larrazabal, I., Guerrero, J. M., & Briz, F. (2022). Low Frequency Stability of AC Railway Traction Power Systems: Analysis of the Influence of Traction Unit Parameters. Electronics, 11(10), 1593. https://doi.org/10.3390/electronics11101593







